Length–Weight Relationships, Growth Models of Two Croakers (Pennahia macrocephalus and Atrobucca nibe) off Taiwan and Growth Performance Indices of Related Species
Abstract
1. Introduction
2. Materials and Methods
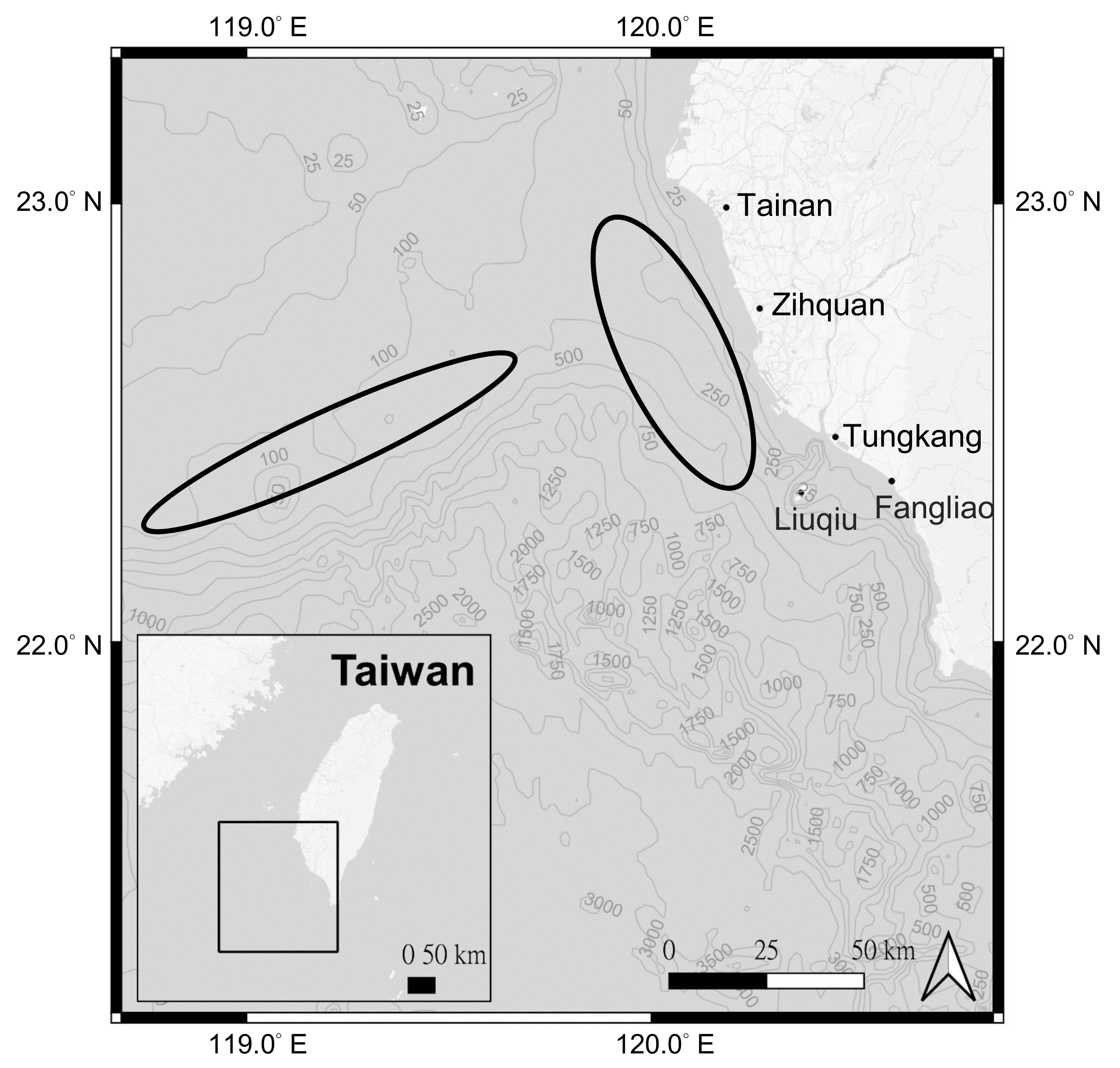
2.1. Data Sources and Estimation of Length-Weight Relationships (LWR)
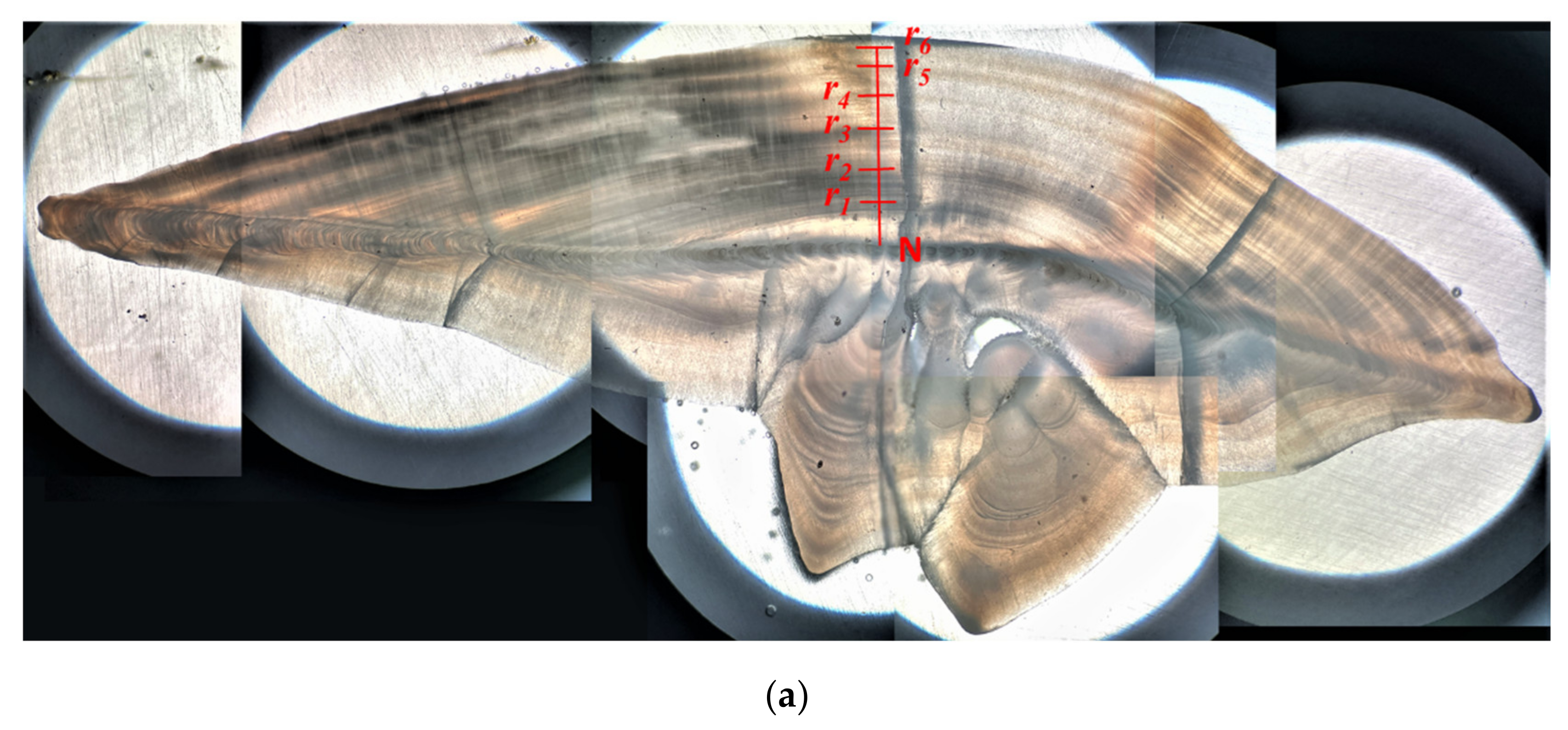
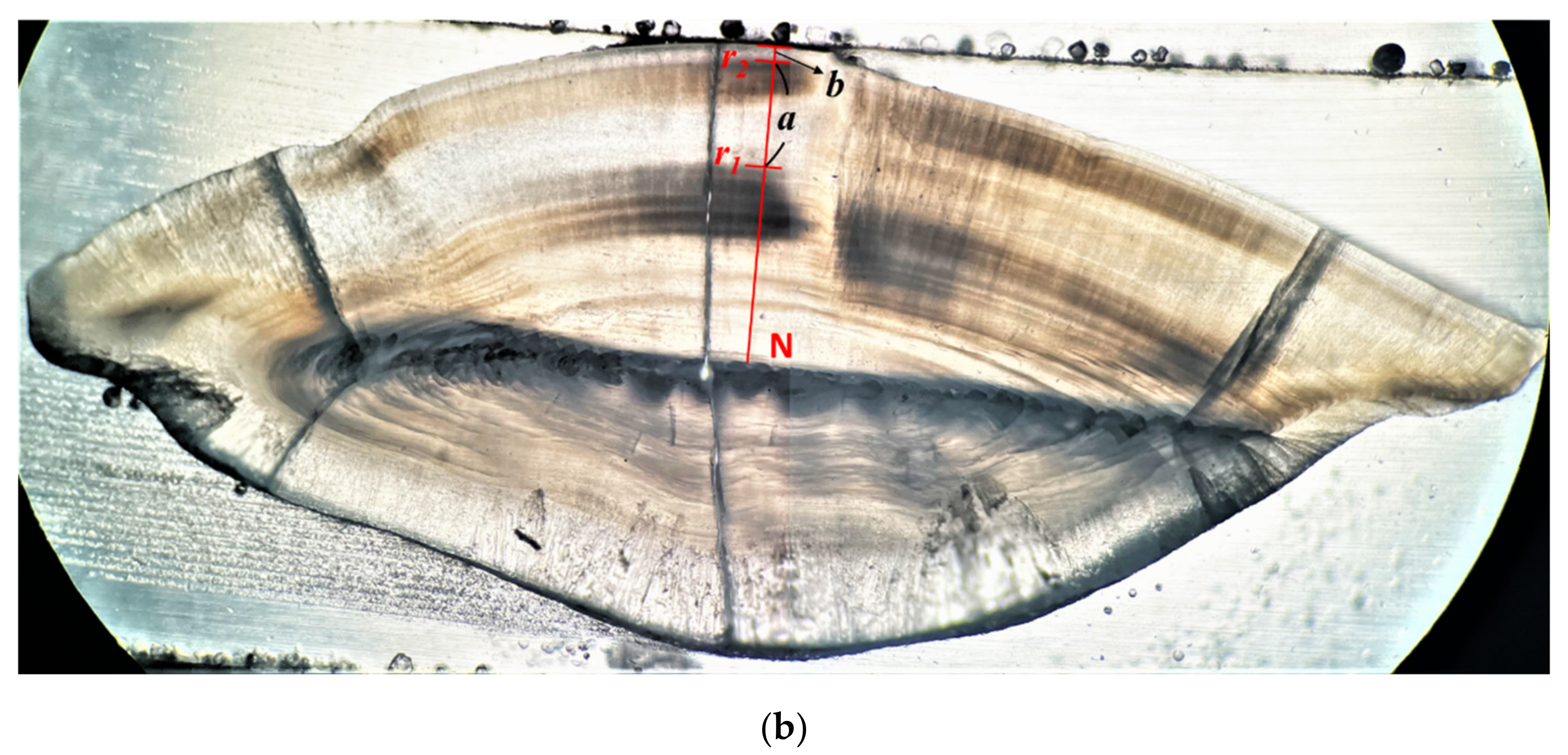
2.2. Otolith Processing, Periodicity of Increment and Age Determination
2.3. Growth Parameters Estimation
2.4. Global Estimate Compilation
TL = −0.37531 + 1.225 SL (R2 = 0.965, for P. argentata)
TL = FL (For P. anea)
3. Results
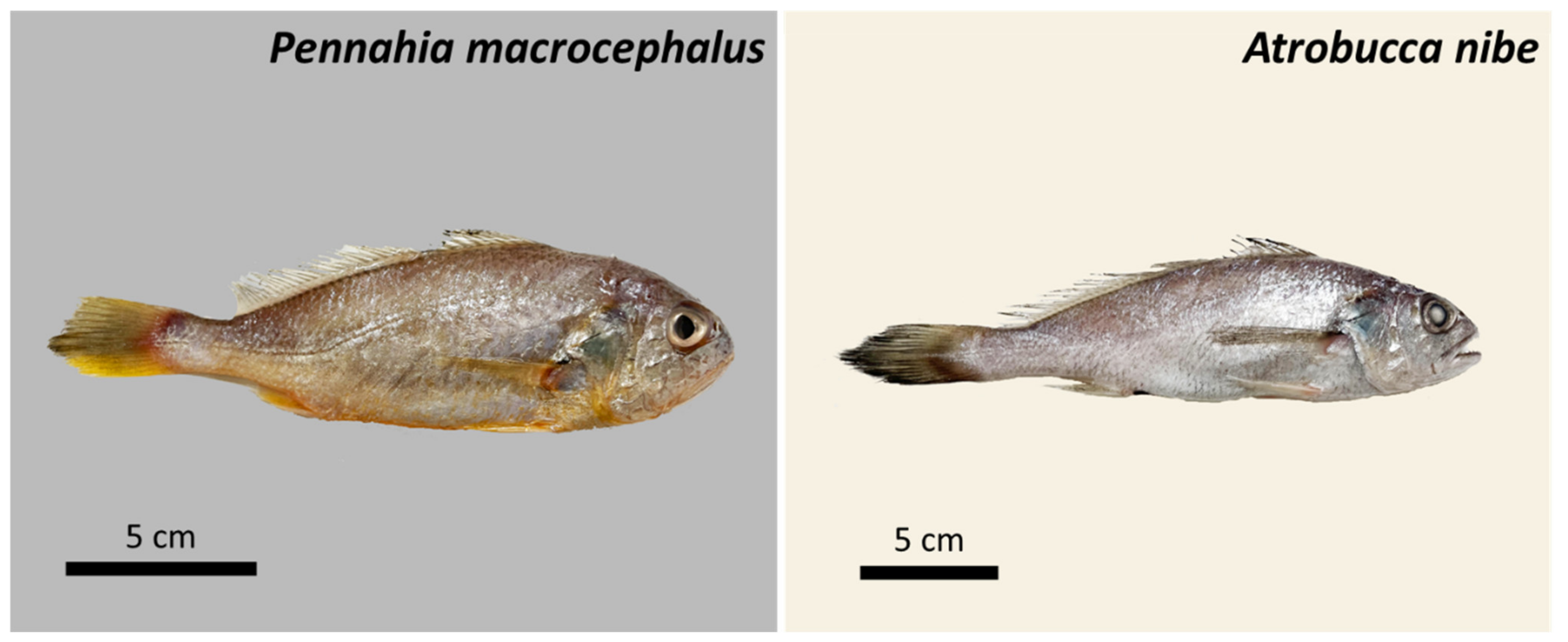
3.1. Samples and Length-Weight Relationships (LWR)
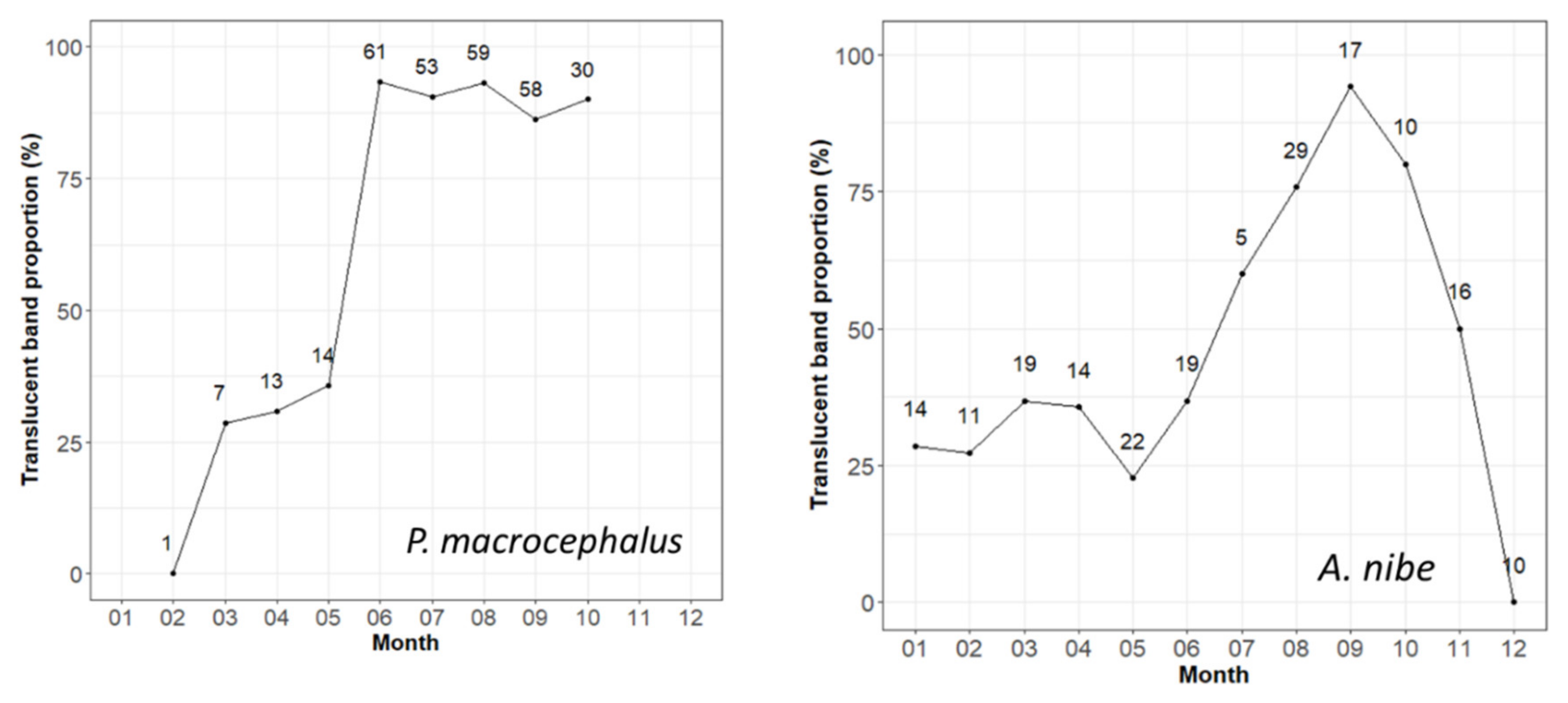
3.2. Periodicity of Increment Formation—Edge Analysis
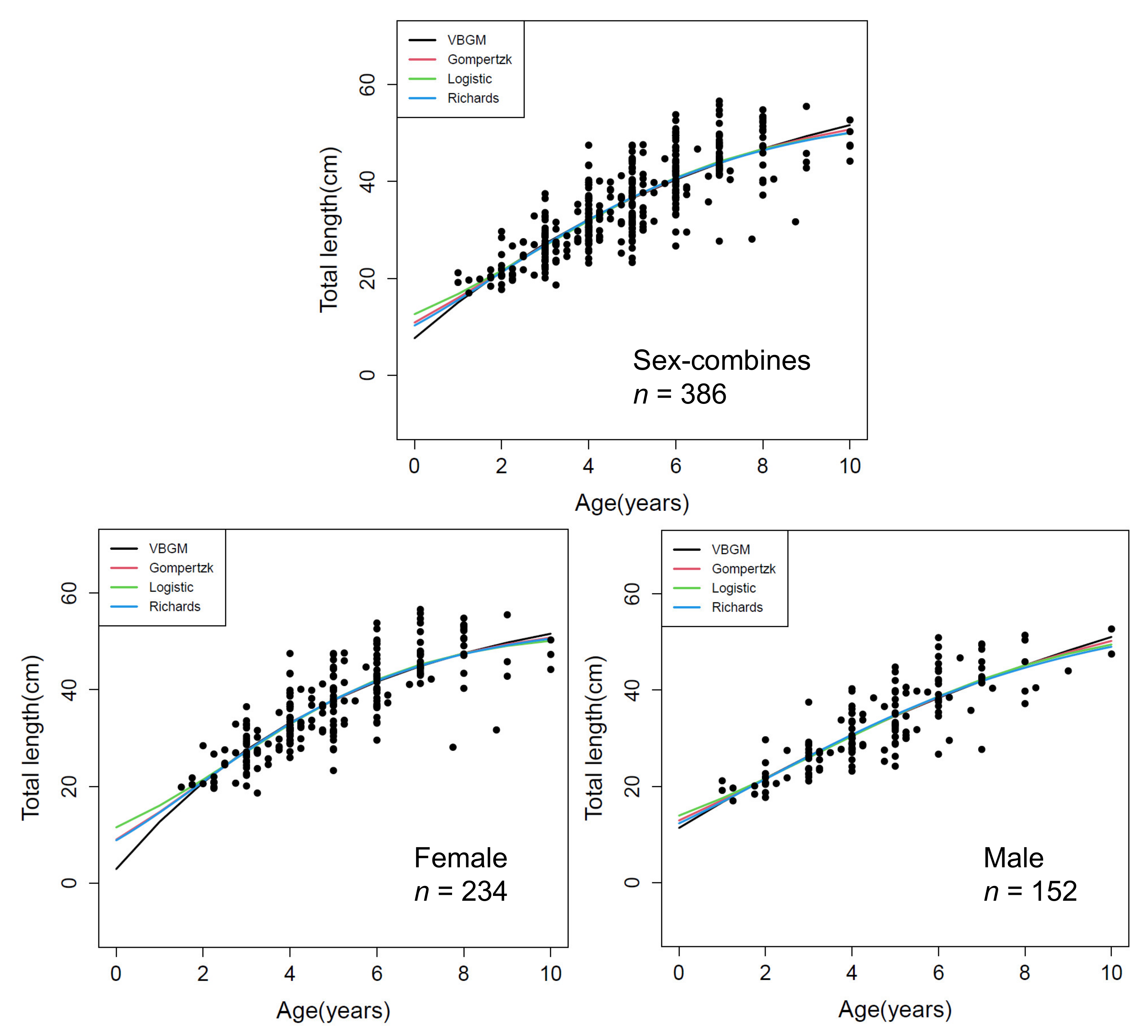
3.3. Age Determination Methods and Growth Parameters Estimation
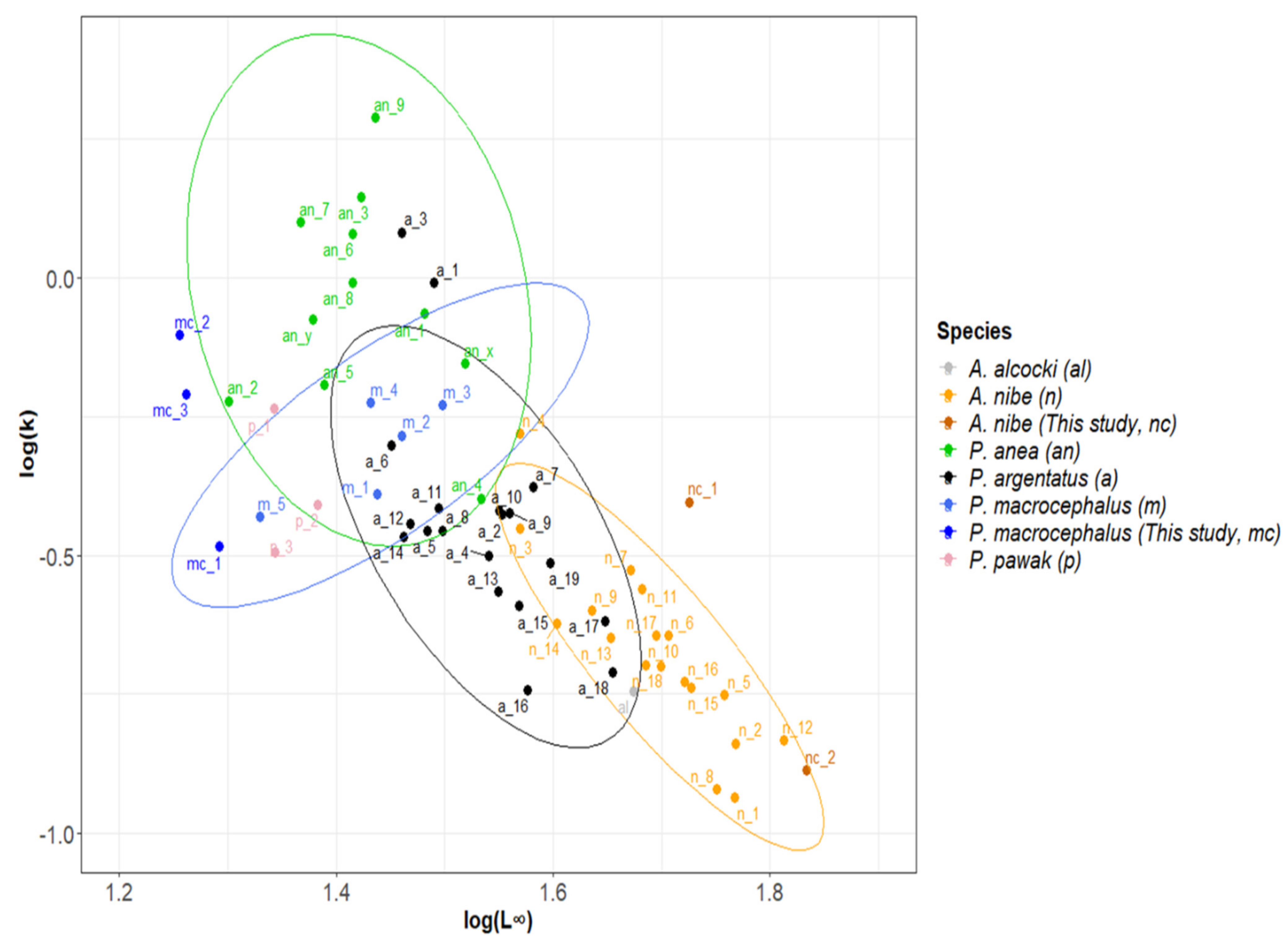
3.4. Global Estimates Compilation
4. Discussion
4.1. Length-Weight Relationships
4.2. Periodicity of Increment Formation—Edge Analysis
4.3. Age Determination Methods
4.4. Growth Parameter Estimates—Global and Local
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Maunder, M.N.; Piner, K.R. Contemporary Fisheries Stock Assessment: Many Issues Still Remain. ICES J. Mar. Sci. 2015, 72, 7–18. [Google Scholar] [CrossRef]
- Francis, R.I.C.C.; Aires-da-Silva, A.M.; Maunder, M.N.; Schaefer, K.M.; Fuller, D.W. Estimating Fish Growth for Stock Assessments Using Both Age–Length and Tagging-Increment Data. Fish. Res. 2016, 180, 113–118. [Google Scholar] [CrossRef]
- Then, A.Y.; Hoenig, J.M.; Hall, N.G.; Hewitt, D.A. Handling editor: Ernesto Jardim Evaluating the Predictive Performance of Empirical Estimators of Natural Mortality Rate Using Information on over 200 Fish Species. ICES J. Mar. Sci. 2015, 72, 82–92. [Google Scholar] [CrossRef]
- Farley, J.; Eveson, P.; Krusic-Golub, K.; Sanchez, C.; Roupsard, F.; Nicol, S.; Leroy, B.; Smith, N.; Chang, S.-K. Project 35: Age, Growth and Maturity of Bigeye Tuna in the Western and Central Pacific Ocean; CSIRO: Rarotonga, Cook Islands, 2017; p. 51. [Google Scholar]
- McKechnie, S.; Pilling, G.; Hampton, J. Stock Assessment of Bigeye Tuna in the Western and Central Pacific Ocean. In Proceedings of the Thirteenth Regular Session of the Scientific Committee, Rarotonga, Cook Islands, 9–17 August 2017; p. 149. [Google Scholar]
- Maunder, M.N.; Crone, P.R.; Punt, A.E.; Valero, J.L.; Semmens, B.X. Growth: Theory, Estimation, and Application in Fishery Stock Assessment Models. Fish. Res. 2016, 180, 1–3. [Google Scholar] [CrossRef]
- Jennings, S.; Greenstreet, S.P.R.; Reynolds, J.D. Structural Change in an Exploited Fish Community: A Consequence of Differential Fishing Effects on Species with Contrasting Life Histories. J. Anim. Ecol. 1999, 68, 617–627. [Google Scholar] [CrossRef]
- Polacheck, T.; Eveson, J.P.; Laslett, G.M. Increase in Growth Rates of Southern Bluefin Tuna (Thunnus maccoyii) over Four Decades: 1960 to 2000. Can. J. Fish. Aquat. Sci. 2004, 61, 307–322. [Google Scholar] [CrossRef]
- Reznick, D.N.; Ghalambor, C.K. Can Commercial Fishing Cause Evolution? Answers from Guppies (Poecilia reticulata). Can. J. Fish. Aquat. Sci. 2005, 62, 791–801. [Google Scholar] [CrossRef]
- Kimura, D.K. Extending the von Bertalanffy Growth Model Using Explanatory Variables. Can. J. Fish. Aquat. Sci. 2008, 65, 13. [Google Scholar] [CrossRef]
- Lavin, C.P.; Gordó-Vilaseca, C.; Stephenson, F.; Shi, Z.; Costello, M.J. Warmer Temperature Decreases the Maximum Length of Six Species of Marine Fishes, Crustacean, and Squid in New Zealand. Environ. Biol. Fishes 2022. [Google Scholar] [CrossRef]
- Campana, S.E.; Neilson, J.D. Microstructure of Fish Otoliths. Can. J. Fish. Aquat. Sci. 1985, 42, 1014–1032. [Google Scholar] [CrossRef]
- Campana, S.E. Accuracy, Precision and Quality Control in Age Determination, Including a Review of the Use and Abuse of Age Validation Methods. J. Fish Biol. 2001, 59, 197–242. [Google Scholar] [CrossRef]
- Morat, F.; Wicquart, J.; Schiettekatte, N.M.D. Individual Back-Calculated Size-at-Age Based on Otoliths from Pacific Coral Reef Fish Species. Sci. Data 2020, 7, 370. [Google Scholar] [CrossRef] [PubMed]
- Lai, H.L.; Gunderson, D.R. Effects of Ageing Errors on Estimates of Growth, Mortality, and Yield per Recruit for Walleye Pollock (Theragra chlacogramma). Fish. Res. 1987, 5, 287–302. [Google Scholar]
- Beamish, R.J.; McFarlane, G.A. A. A Discussion of the Importance of Ageing Errors, and an Application to Walleye Pollock: The World’s Largest Fishery. In Recent Developments in Fish Otolith Research; Secor, D.H., Dean, J.M., Campana, S.E., Eds.; University of South Carolina Press: Columbia, SC, USA, 1995; pp. 545–565. [Google Scholar]
- Porta, M.J.; Snow, R.A. Validation of Annulus Formation in White Perch Otoliths, Including Characteristics of an Invasive Population. J. Freshw. Ecol. 2017, 32, 489–498. [Google Scholar] [CrossRef]
- Froeschke, B.; Allen, L.G.; Pondella, D.J. Life History and Courtship Behavior of Black Perch, Embiotoca jacksoni (Teleostomi: Embiotocidae), from Southern California. Pac. Sci. 2007, 61, 521–531. [Google Scholar] [CrossRef]
- Okamura, H.; Punt, A.E.; Semba, Y.; Ichinokawa, M. Marginal Increment Analysis: A New Statistical Approach of Testing for Temporal Periodicity in Fish Age Verification. J. Fish Biol. 2013, 82, 1239–1249. [Google Scholar] [CrossRef]
- Smith, J. Age Validation of Lemon Sole (Microstomus kitt), Using Marginal Increment Analysis. Fish. Res. 2014, 157, 41–46. [Google Scholar] [CrossRef]
- Hidalgo-de-la-Toba, J.A.; Morales-Bojórquez, E.; González-Peláez, S.S.; Bautista-Romero, J.J.; Lluch-Cota, D.B. Modeling the Temporal Periodicity of Growth Increments Based on Harmonic Functions. PLoS ONE 2018, 13, 0196189. [Google Scholar] [CrossRef]
- Prince, E.D.; Lee, D.W.; Berkeley, S.A. Use of Marginal Increment Analysis to Validate the Anal Spine Method for Ageing Atlantic Swordfish and Other Alternatives for Age Determination. Col. Vol. Sci. Pap. ICCAT 1988, 27, 194–201. [Google Scholar]
- Pearson, D.E. Timing of Hyaline-Zone Formation as Related to Sex, Location, and Year of Capture in Otoliths of the Widow Rockfish, Sebastes entomelas. Fish. Bull. 1996, 94, 190–197. [Google Scholar]
- Laidig, T.E.; Pearson, D.E.; Sinclair, L.L. Age and Growth of Blue Rockfish (Sebastes mystinus) from Central and Northern California. Fish Bull 2003, 101, 800–808. [Google Scholar]
- Young, J.; Drake, A.; Groisson, A.-L. Age and Growth of Broadbill Swordfish (Xiphias glades) from Eastern Australian Waters—Preliminary Results; CSIRO, Division of Marine Research: Hobart, Australia, 2003. [Google Scholar]
- Chang, S.-K.; Chou, Y.-T.; Hoyle, S.D. Length-Weight Relationships and Otolith-Based Growth Curves for Brushtooth Lizardfish off Taiwan with Observations of Region and Aging-Material Effects on Global Growth Estimates. Front. Mar. Sci. 2022, 9, 921594. [Google Scholar] [CrossRef]
- Chang, S.-K.; Yuan, T.-L.; Hoyle, S.D.; Farley, J.H.; Shiao, J.-C. Growth Parameters and Spawning Season Estimation of Four Important Flyingfishes in the Kuroshio Current off Taiwan and Implications from Comparisons with Global Studies. Front. Mar. Sci. 2022, 8, 747382. [Google Scholar] [CrossRef]
- Yamaguchi, A.; Kume, G.; Takita, T. Geographic Variation in the Growth of White Croaker, Pennahia argentata, off the Coast of Northwest Kyushu, Japan. Environ. Biol. Fishes 2004, 71, 179–188. [Google Scholar] [CrossRef]
- Yan, Y.R.; Hou, G.; Lu, H.S.; Li, Z.L. Growth Characteristics and Population Composition of Big-Head Pennah Croaker, Pennahia macrocephalus in the Beibu Gulf. J. Fish. Sci. China 2010, 40, 61–68. [Google Scholar]
- Ju, P.L.; Yang, L.; Lu, Z.B.; Yang, S.Y.; Du, J.G.; Zhong, H.Q.; Chen, J.; Xiao, J.M.; Chen, M.R.; Zhang, C.Y. Age, Growth, Mortality and Population Structure of Silver Croaker Pennahia argentata (Houttuyn, 1782) and Red Bigeye Priacanthus macracanthus Cuvier, 1829 in the North-Central Taiwan Strait. J. Appl. Ichthyol. 2016, 32, 652–660. [Google Scholar] [CrossRef]
- Jeon, B.S.; Choi, J.H.; Kim, D.N.; Im, Y.J.; Lee, H.W. Age and Growth of White Croaker Pennahia argentata in the Southern Sea of Korea by Otolith Analysis. Korean J. Fish. Aquat. Sci. 2021, 54, 53–63. [Google Scholar]
- Attaqi, A.N. Age Structure and Growth of Big-Head Pannah Croaker, Pennahia macrocephalus in the Southwestern Waters off Taiwan: An Approach Using Both Thin-Section and Weight of Otoliths. Master’s Thesis, College of Ocean Science and Resource, National Taiwan Ocean University,, Keelung, Taiwan, 2018. [Google Scholar]
- Vanderkooy, S.; Carroll, J.; Elzey, S.; Gilmore, J.; Kipp, J. (Eds.) A Practical Handbook for Determining the Ages of Gulf of Mexico and Atlantic Coast Fishes, 3rd ed.; Gulf States Marine Fisheries Commission and Atlantic States Marine Fisheries Commission: Ocean Springs, MS, USA, 2020. [Google Scholar]
- Tsai, T.W. Age and Growth of Big Head Pennah Croaker (Pennahia macrocephalus) Sampled from the Southwestern Waters off Taiwan. Master’s Thesis, Department of Fisheries Production and Management of National Kaohsiung University of Science and Technology, Kaohsiung, Taiwan, 2009. [Google Scholar]
- Farley, J.; Krusic-Golub, K.; Eveson, P.; Clear, N.; Roupsard, F.; Sanchez, C.; Nicol, S.; Hampton, J. Age and Growth of Yellowfin and Bigeye Tuna in the Western and Central Pacific Ocean from Otoliths (No. WCPFC-SC16-2020/SA-WP-02). In Proceedings of the WCPFC Scientific Committee 16th Regular Session, Electronic Meeting, 11–20 August 2020. [Google Scholar]
- Roff, D.A. A Motion for the Retirement of the Von Bertalanffy Function. Can. J. Fish. Aquat. Sci. 1980, 37, 127–129. [Google Scholar] [CrossRef]
- Katsanevakis, S. Modelling Fish Growth: Model Selection, Multi-Model Inference and Model Selection Uncertainty. Fish. Res. 2006, 81, 229–235. [Google Scholar] [CrossRef]
- Katsanevakis, S.; Maravelias, C.D. Modelling Fish Growth Multi-model Inference as a Better Alternative to a Priori Using von Bertalanffy Equation. Fish Fish. 2008, 9, 178–187. [Google Scholar] [CrossRef]
- Williams, A.J.; Farley, J.H.; Hoyle, S.D.; Davies, C.R.; Nicol, S.J. Spatial and Sex-Specific Variation in Growth of Albacore Tuna (Thunnus alalunga) across the South Pacific Ocean. PLoS ONE 2012, 7, e39318. [Google Scholar] [CrossRef] [PubMed]
- Carbonara, P.; Intini, S.; Kolitari, J.; Joksimović, A.; Milone, N.; Lembo, G.; Casciaro, L.; Bitetto, I.; Zupa, W.; Spedicato, M.T.; et al. A Holistic Approach to the Age Validation of Mullus barbatus L., 1758 in the Southern Adriatic Sea (Central Mediterranean). Sci. Rep. 2018, 8, 13219. [Google Scholar] [CrossRef] [PubMed]
- Velasco, G.; Oddone, M.C. Growth Parameters and Growth Performance Indexes for Some Populations of Marine Catfishes (Actinopterygii, Siluriformes, Ariidae). Acta Biol. Leopoldensia 2004, 26, 307–313. [Google Scholar]
- Ba, K.; Thiaw, M.; Lazar, N.; Sarr, A.; Brochier, T.; Ndiaye, I.; Faye, A.; Sadio, O.; Panfili, J.; Thiaw, O.T.; et al. Resilience of Key Biological Parameters of the Senegalese Flat Sardinella to Overfishing and Climate Change. PLoS ONE 2016, 11, e0156143. [Google Scholar] [CrossRef]
- Murua, H.; Rodríguez-Marín, E.; Neilson, J.; Farley, J.; Juan-Jordá, M.J. Fast versus Slow Growing Tuna Species: Age, Growth, and Implications for Population Dynamics and Fisheries Management. Rev. Fish Biol. Fish. 2017, 27, 733–773. [Google Scholar] [CrossRef]
- Pauly, D.; Munro, J.L. Once More on the Comparison of Growth in Fish and Invertebrates. Fishbyte 1984, 2, 21. [Google Scholar]
- Juan-Jordá, M.J.; Mosqueira, I.; Dulvy, N. The Conservation and Management of Tunas and Their Relatives: Setting Life History Research Priorities. PLoS ONE 2013, 8, e70405. [Google Scholar] [CrossRef] [PubMed]
- Pauly, D.; Binohlan, C. FishBase and AUXIM as Tools for Comparing the Life-History Patterns, Growth and Natural Mortality of Fish: Applications to Snappers and Groupers. ICLARM Conf. Proc. 1996, 48, 223–247. [Google Scholar]
- Chang, S.-K.; DiNardo, G.; Farley, J.; Brodziak, J.; Yuan, Z.-L. Possible Stock Structure of Dolphinfish (Coryphaena hippurus) in Taiwan Coastal Waters and Globally Based on Reviews of Growth Parameters. Fish. Res. 2013, 147, 127–136. [Google Scholar] [CrossRef]
- Chang, S.-K.; Maunder, M. Aging Material Matters in the Estimation of von Bertalanffy Growth Parameters for Dolphinfish (Coryphaena hippurus). Fish. Res. 2012, 119–120, 147–153. [Google Scholar] [CrossRef]
- Nelson, J.S. Fishes of the World, 4th ed.; John Wiley & Sons Inc: Hoboken, NJ, USA, 2006. [Google Scholar]
- Shao, K.T. Taiwan Fish Database. WWW Web Electronic Publication. 2022. Available online: http://fishdb.sinica.edu.tw (accessed on 21 February 2022).
- Tang, D.-S. A study of sciaenoid fishes of China. Amoy Mar. Biol. Bull. 1937, 2, 47–88. [Google Scholar]
- Jordan, D.S.; Thompson, W.F. A Review of the Sciaenoid Fishes of Japan. Proc. United States Natl. Mus. 1911, 39, 241–261. [Google Scholar] [CrossRef]
- Chang, S.-K.; Yuan, T.L.; Wang, S.P.; Chang, Y.J.; DiNardo, G.; Chen, Y.; Mello, L. Deriving a Statistically Reliable Abundance Index from Landings Data: An Application to the Taiwanese Coastal Dolphinfish Fishery with a Multispecies Feature. Trans. Am. Fish. Soc. 2019, 148, 106–122. [Google Scholar] [CrossRef]
- Froese, R. Cube Law, Condition Factor and Weight–Length Relationships: History, Meta-Analysis and Recommendations. J. Appl. Ichthyol. 2006, 22, 241–253. [Google Scholar] [CrossRef]
- Zakeyudin, M.-S.; Mat, M.; Salmah, M.; Md Sah, A.S.R.; Hassan, A. Assessment of Suitability of Kerian River Tributaries Using Length-Weight Relationship and Relative Condition Factor of Six Freshwater Fish Species. J. Environ. Earth Sci. 2012, 2, 52–60. [Google Scholar]
- Jisr, N.; Younes, G.; Sukhn, C.; El-Dakdouki, M.H. Length-Weight Relationships and Relative Condition Factor of Fish Inhabiting the Marine Area of the Eastern Mediterranean City, Tripoli-Lebanon. Egypt. J. Aquat. Res. 2018, 44, 299–305. [Google Scholar] [CrossRef]
- James, G.; Witten, D.; Hastie, T.; Tibshirani, R. (Eds.) An Introduction to Statistical Learning: With Applications in R; Springer Texts in Statistics; Springer: New York, NY, USA, 2013; ISBN 978-1-4614-7137-0. [Google Scholar]
- Ashworth, E.C.; Hesp, S.A.; Hall, N.G. A New Proportionality-Based Back-Calculation Approach, Which Employs Traditional Forms of Growth Equations, Improves Estimates of Length at Age. Can. J. Fish. Aquat. Sci. 2017, 74, 1088–1099. [Google Scholar] [CrossRef]
- Ogle, D.H. Introductory Fisheries Analyses with R, 1st ed.; CRC Press: New York, NY, USA, 2016; ISBN 978-1-315-37198-6. [Google Scholar]
- Karlson, S.; Michalsen, K.; Folkvord, A.; Karlson, S.; Age, A. Age Determination of Atlantic Halibut (Hippoglossus hippoglossus L.) along the Coast of Norway: Status and Improvements. ICES J. Mar. Sci. 2012, 70, 50–55. [Google Scholar] [CrossRef]
- Gompertz, B. On the Nature of the Function Expressive of the Law of Human Mortality, and on a New Mode of Determining the Value of Life Contingencies. Philos. Trans. R. Soc. 1825, 115, 513–583. [Google Scholar] [CrossRef]
- Von Bertalanffy, L. A Quantitative Theory of Organic Growth. Hum. Biol. 1938, 10, 181–213. [Google Scholar]
- Richards, F.J. A Flexible Growth Function for Empirical Use. J. Exp. Bot. 1959, 10, 290–301. [Google Scholar] [CrossRef]
- Ricker, W.E. Computation and Interpretation of Biological Statistics of Fish Populations. Bull. Fish. Res. Board Can. 1975, 191, 1–382. [Google Scholar]
- Flinn, S.A.; Midway, S.R. Trends in Growth Modeling in Fisheries Science. Fishes 2021, 6, 1. [Google Scholar] [CrossRef]
- Quist, M.C.; Pegg, M.A.; Devries, D.R. Age and Growth. Fisheries Techniques. In Age and Growth. Fisheries Techniques; Zale, A.V., Parrish, D.L., Sutton, T.M., Eds.; American Fisheries Society: Bethesda, MD, USA, 2012; pp. 677–731. [Google Scholar]
- R-Development Core Team. R: A Language and Environment for Statistical Computing; R Foundation for Statistical Computing: Vienna, Austria, 2021. [Google Scholar]
- Akaike, H. Information Theory and an Extension of the Maximum Likelihood Principle. In Proceedings of the 2nd International Symposium on Information Theory, Tsahkadsor, Armenia, 2–8 September 1973; Volume I. pp. 267–281. [Google Scholar]
- Burnham, K.P.; Anderson, D.R. Model Selection and Multimodel Inference: A Practical Information-Theoretic Approach, 2nd ed.; Springer: New York, NY, USA, 2002; ISBN 978-0-387-95364-9. [Google Scholar]
- Wang, X.; Qiu, Y.; Du, F.; Lin, Z.; Sun, D.; Huang, S. Population Parameters and Dynamic Pool Models of Commercial Fishes in the Beibu Gulf, Northern South China Sea. Chin. J. Oceanol. Limnol. 2012, 30, 105–117. [Google Scholar] [CrossRef]
- Chen, Z.; Huang, Z.; Qiu, Y. 南海北部白姑鱼生长和死亡参数的估算 (Estimation of Growth and Mortality Parameters of Argyrosomus argentatus in Northern South China Sea). Chin. J. Appl. Ecol. 2005, 16, 712–716. (In Chinese). Available online: http://www.cqvip.com/qk/90626a/200504/15404417.html (accessed on 1 October 2021).
- FishBase. World Wide Web Electronic Publication. 2022. Available online: https://www.fishbase.de/summary/Pennahia-anea (accessed on 1 October 2021).
- Nhuận, M.C.; Khương, Đ.V. Stock Biological Characteristics of Big-Head Pennah Croacer (Pennahia macrocephalus, Tang 1937) in the Shared Fishing Zone in the Gulf of Tonkin, during 2006–2010. J. Fish. Sci. Technol. Vietnam. 2013, 2, 83–88. [Google Scholar]
- Liu, H.C.; Tzeng, W.N. Age and growth of the white croaker, Argyrosomus argentatus (HOUTTUYN), in the Southern part of the East China Sea and Taiwan Strait. J. Fish. Soc. Taiwan 1972, 1, 21–38. [Google Scholar]
- Lu, Z.; Dai, Q.; Yan, Y. Population dynamics of major demersal fish species offshore Fujian. J. Oceanogr. Taiwan Strait Chin. 1999, 18, 100–105. [Google Scholar]
- Hu, Y.; Qian, S. A study on the age and growth of the white croaker. Mar. Fish. 1989, 4, 158–162. [Google Scholar]
- Higuchi, T.; Yamaguchi, A.; Takita, T. Age and Growth of White Croaker, Pennahia argentata, in the Ariake Sound, Japan. Bull. Fac. Fish. Nagasaki Univ. Jpn. 2003, 47–51. [Google Scholar]
- Kakuda, S.; Matsumoto, K. On the Age and Growth of the White Croaker Argyrosomus argentatus. Hiroshima Univ. 1977, 16, 115–122. [Google Scholar]
- Yan, Y.; Hou, G.; Lu, H.; Yin, Q. Age and Growth of Pennahia pawak in the Beibu Gulf. Chin. Fish. Sci. 2011, 145–155. [Google Scholar]
- Yi, X.; Qiu, K.; Zhou, X.; Zhao, C.; Deng, Y.; He, X.; Yan, Y. Analysis of Fishery Biology of Pennahia pawak in Beibu Gulf. J. Shanghai Ocean Univ. 2021, 30, 515–524. [Google Scholar] [CrossRef]
- Bhuyan, S.K.; Kumar, P.; Jena, J.K.; Pillai, B.R.; Chakraborty, S.K. Studies on the Growth of Otolithes Ruber (Bloch & Schneider, 1801), Johnius Carutta Bloch, 1793 and Pennahia macrophthalmus (Bleeker, 1850) from Paradeep Coast, Orissa, India. Indian J. Fish 2012, 59, 89–93. [Google Scholar]
- Ingles, J.; Pauly, D. An Atlas of the Growth, Mortality and Recruitment of Philippine Fishes. Available online: https://www.worldfishcenter.org/publication/atlas-growth-mortality-and-recruitment-philippine-fishes (accessed on 2 May 2022).
- Ziegler, B. Growth and Mortality Rates of Some Fishes of Manila Bay, Philippines as Estimated from the Analysis of Length Frequencies. Master’s Thesis, Kiel University, Kiel, Germany, 1979. [Google Scholar]
- Abu Talib, A. Population Dynamics of Big-Eye Croaker (Pennahia macrophthalmus, Sciaenidae) off Kedah, Penang and Perak States, Malaysia; FAO Fisheries Report; FAO: Rome, Italy, 1988. [Google Scholar]
- Chakraborty, S.K.; Deshmukh, V.D.; Khan, M.Z.; Vidyasagar, K.; Raje, S.G. Estimates of Growth, Mortality, Recruitment Pattern and MSY of Important Resources from the Maharashtra Coast. J. Indian Fish. Assoc. 1994, 24, 1–39. [Google Scholar]
- Chakraborty, S.K. Stock assessment of big-eye croaker, Pennahia macropthalmus (Bleeker) (Pisces/Perciformes/Sciaenidae) from Bombay waters. Indian J. Mar. Sci. 1996, 25, 316–319. [Google Scholar]
- Jayasankar, P. Population Dynamics of Big-Eye Croaker Pennahia macrophthalmus and Blotched Croaker Nibea maculata (Pisces/Perciformes/Sciaenidae) in the Trawling Grounds off Rameswaram Island, East Coast of India. Indian J. Mar. Sci. 1995, 24, 153–157. [Google Scholar]
- Chakraborty, S.K.; Devadoss, P.; Manojkumar, P.P.; Feroz Khan, M.; Jayasankar, P.; Sivakami, S.; Gandi, V.; Appanna Sastry, Y.; Raju, A.; Livingston, P.; et al. Fishery, Biology and Stock Assessment of Jew Fish Resources of India. In Marine Fisheries Research and Management; Pillai, V.N., Menon, N.G., Eds.; Central Marine Fisheries Research Institute: Cochin, India, 2000; pp. 604–616. [Google Scholar]
- Chakraborty, S.K. Growth Studies of Sciaenids from Mumbai Waters Using Bhattarcharya’s Method. Naga ICLARM Q. 2001, 24, 40–41. [Google Scholar]
- Menon, M.; Maheswarudu, G.; Rohit, P.; Laxmilatha, P.; Madhumita, D.; Rao, K.N. Biology and Stock Assessment of the Bigeye Croaker Pennahia Anea (Bloch, 1793) Landed along Andhra Pradesh, North-East Coast of India. Indian J. Fish 2015, 62, 46–51. [Google Scholar]
- Wagiyo, K.; Tirtadanu, T.; Chodriyah, U.; Wang, X.; Qiu, Y.; Du, F.; Lin, Z.; Sun, D.; Huang, S. Biology Characteristic, Abundance Index and Fishing Aspect of Donkey Croaker Pennahia anea (Bloch, 1793) in the Tangerang Waters. E3S Web Conf. 2020, 30, 105–117. [Google Scholar] [CrossRef]
- Pauly, D. A Preliminary Compilation of Fish Length Growth Parameters. Ber. Inst. Meereskd. Univ. Kiel 1978, 55, 200. [Google Scholar]
- Kao, P. Stock Discrimination of Blackmouth Croaker Atrobucca Nibe in Taiwan. Master’s Thesis, The Institute of Fisheries Science, National Taiwan University, Taipei, Taiwan, 2019. (In Chinese). [Google Scholar]
- Sato, T. Fishery Biology of Black Croaker, Argyrosomus nibe (Jordan et Thompson). I. On the Age and Growth of the Black Croaker in the Central and Southern Parts of the East China Sea. Bull. Seikai Reg. Fish Res. Lab. 1963, 29, 75–96. [Google Scholar]
- Salarpouri, A.; Kaymaram, F.; Valinassab, T.; Behzadi, S.; Darvishi, M.; Kamali, E.; Rezwani, S.; Memarzadeh, M.; Karami, N. A Survey on Black Mouth Croaker (Atrobucca nibe) Resources in the North-West of Oman Sea; Iranian Fisheries Science Research Institute: Tehran, Iran, 2015. [Google Scholar]
- Chang, J.J. Effects of Fishing on Life History Parameters of Atrobucca Nibe from Surrounding Waters of Guei-Shan Island, Northeastern Taiwan. Master’s Thesis, College of Ocean Science and Resource, National Taiwan Ocean University, Keelung, Taiwan, 2008. [Google Scholar]
- Tsai, C.N. Age and growth of black croaker, Atrobucca nibe, in adjacent waters of Tungkang, Taiwan. Master’s Thesis, National Taiwan Ocean Univeristy, Keelung, Taiwan, 1993. [Google Scholar]
- Hwang, G.M.; Chen, C.T. Fishery Biology of Black Croaker, Atrobucca Nibe (Jordan et Thompson) from the Surrounding Waters of Guei Shan Island, Taiwan. J. Fish. Soc. Taiwan 1984, 11, 35–52. [Google Scholar]
- Memon, K.; Liu, Q.; Kalhoro, M.; Nazir, K.; Waryani, B.; Chang, M.S.; Mirjatt, A.N. Estimation of Growth and Mortality Parameters of Croaker Atrobucca alcocki in Pakistani Waters. J. Agric. Sci. Technol. 2016, 18, 669–679. [Google Scholar]
- Zhu, L.; Li, L.; Liang, Z. Comparison of Six Statistical Approaches in the Selection of Appropriate Fish Growth Models. Chin. J. Oceanol. Limnol. 2009, 27, 457–467. [Google Scholar] [CrossRef]
- Kimmerer, W.; Avent, S.R.; Bollens, S.M.; Feyrer, F.; Grimaldo, L.F.; Moyle, P.B.; Nobriga, M.; Visintainer, T. Variability in Length–Weight Relationships Used to Estimate Biomass of Estuarine Fish from Survey Data. Trans. Am. Fish. Soc. 2005, 134, 481–495. [Google Scholar] [CrossRef]
- Morey, G.; Moranta, J.; Massutí, E.; Grau, A.; Linde, M.; Riera, F.; Morales-Nin, B. Weight–Length Relationships of Littoral to Lower Slope Fishes from the Western Mediterranean. Fish. Res. 2003, 62, 89–96. [Google Scholar] [CrossRef]
- CMFRI/BOBP-IGO/GoI. Training Manual on Stock Assessment of Tropical Fishes; Central Marine Fisheries Research Institute (CMFRI): Kochi, India, 2016; Volume 156. [Google Scholar]
- Mredul, M.M.H.; Alam, M.R.; Akkas, A.B.; Sharmin, S.; Pattadar, S.N.; Ali, M.L. Some Reproductive and Biometric Features of the Endangered Gangetic Leaf Fish, Nandus nandus (Hamilton, 1822): Implication to the Baor Fisheries Management in Bangladesh. Aquac. Fish. 2021, 6, 634–641. [Google Scholar] [CrossRef]
- Tesch, F.W. Age and growth. In Methods for Assessment of Fish Production in Freshwaters; Ricker, W.E., Ed.; Blackwell Scientific Publications: Oxford, UK, 1968; pp. 101–113. [Google Scholar]
- Lee, J.J. Reproductive Biology of Big Head Pennah Croaker (Pennahia macrocephalus) Sampled from the Southwestern Waters off Taiwan. Master’s Thesis, Department of Fisheries Production and Management of National Kaohsiung University of Science and Technology, Kaohsiung, Taiwan, 2010. (In Chinese). [Google Scholar]
- Matsui, I. 東海黄海に於ける底曳網漁場と底棲生物群聚との関係に就て (Relation between the Trawling Grounds and the Benthic Association in the East China Sea and the Yellow Sea). Nippon Suisan Gakkaishi Jpn. 1951, 16, 159–167. [Google Scholar] [CrossRef]
- Kao, P.; Rong, J.; Zhang, W.; Li, Y. A Review of the Research on the Biology of Atrobucca nibe Fishery. Fish. Ext. Natl. Taiwan Univ. 2018, 29, 46–57. [Google Scholar]
- Liu, F.; Chen, J. A survey of Nibea nibe Jordan et Thompson in northern Taiwan. China Aquat. Prod. 1954, 24, 23–45. [Google Scholar]
- Hsiao, L.; Chen, C.; Wu, C.; Chen, Y.; He, J. Research on Reproductive Biology of Heliconia in the Southwest Waters of Taiwan. J. Taiwan Fish. Res. 2017, 25, 15–25. [Google Scholar]
- Schneider, J.C.; Laarman, P.W.; Gowing, H. Length-Weight Relationships. Chapter 17. In Manual of Fisheries Survey Methods II: With Periodic Updates; Michigan Department of Natural Resources, Fisheries Division: Lansing, MI, USA, 2000; pp. 1–18. [Google Scholar]
- De Giosa, M.; Czerniejewski, P.; Rybczyk, A. Seasonal Changes in Condition Factor and Weight-Length Relationship of Invasive Carassius gibelio (Bloch, 1782) from Leszczynskie Lakeland, Poland. Adv. Zool. 2014, 2014, e678763. [Google Scholar] [CrossRef]
- He, J.S.; Huang, X.H.; Wu, Y.S.; Lai, C.C.; Weng, J.S. Reproductive Biology of the Females of the Pennahia macrocephalus in the Southwestern Waters of Taiwan. J. Taiwan Fish. Res. 2020, 28, 13–24. [Google Scholar]
- Costa, M.D.P.; Muelbert, J.H.; Moraes, L.E.; Vieira, J.; Castello, J.P. Estuarine Early Life Stage Habitat Occupancy Patterns of Whitemouth Croaker Micropogonias furnieri (Desmarest, 1830) from the Patos Lagoon, Brazil. Fish. Res. 2014, 160, 77–84. [Google Scholar] [CrossRef]
- Putnis, I.; Korņilovs, G. Manual for Age Determination of Baltic Herring. In Proceedings of the ICES 2008 Report of the Workshop on Age Reading of Baltic Herring (WKARBH), Riga, Latvia, 9–13 June 2008; p. 37. [Google Scholar]
- Newman, S.J.; Dunk, I.J. Age Validation, Growth, Mortality, and Additional Population Parameters of the Goldband Snapper (Pristipomoides multidens) off the Kimberley Coast of Northwestern Australia. Fish. Bull. 2003, 101, 116–128. [Google Scholar]
- Hara, K.; Furumitsu, K.; Aoshima, T.; Kanehara, H.; Yamaguchi, A. Age, Growth, and Age at Sexual Maturity of the Commercially Landed Skate Species, Dipturus chinensis (Basilewsky, 1855), in the Northern East China Sea. J. Appl. Ichthyol. 2018, 34, 66–72. [Google Scholar] [CrossRef]
- Wang, S.B. Survey of Fisheries Economic Activities in the Nearby Area of Mai-Liao, Yunlin County, Taiwan; The Final Report for Capture Fisheries in 2012; Century Engineering Consultant Co., Ltd.: New Taipei, Taiwan, 2011. (In Chinese) [Google Scholar]
- Jones, C. Determining Age of Larval Fish with the Otolith Increment Technique. Fish. Bull. 1986, 84, 91–102. [Google Scholar]
- Cailliet, G.M.; Goldman, K.J.; Carrier, J.; Musick, J.A.; Heithaus, M. (Eds.) Age Determination and Validation in Chondrichthyan Fishes; CRC Press: Boca Raton, FL, USA, 2004. [Google Scholar]
- Chen, Y.; Mello, L.G.S. Growth and Maturation of Cod (Gadus morhua) of Different Year-Classes in the Northwest Atlantic, NAFO Subdivision 3Ps. Fish. Res. 1999, 42, 87–101. [Google Scholar] [CrossRef]
- Fisheries Agency. Fisheries Statistical Yearbook—Taiwan, Kinmen and Matsu Area; Fisheries Agency, Council of Agriculture, Executive Yuan: Taipei, Taiwan, 2021. (In Chinese) [Google Scholar]
- Haimovici, M.; Cavole, L.M.; Cope, J.M.; Cardoso, L.G. Long-Term Changes in Population Dynamics and Life History Contribute to Explain the Resilience of a Stock of Micropogonias furnieri (Sciaenidae, Teleostei) in the SW Atlantic. Fish. Res. 2021, 237, 105878. [Google Scholar] [CrossRef]
- Rice, J.; Gislason, H. Patterns of Change in the Size Spectra of Numbers and Diversity of the North Sea Fish Assemblage, as Reflected in Surveys and Models. ICES J. Mar. Sci. 1996, 53, 1214–1225. [Google Scholar] [CrossRef]


| Code | Species | Region | Material (Method) | L∞ (TL) | k (cm y−1) | φ | Ref |
|---|---|---|---|---|---|---|---|
| m_1 | P. macrocephalus | Beibu Gulf, SCS | Otolith (I) | 27.371 | 0.408 | 2.485 | [29] |
| m_2 | P. macrocephalus | Beibu Gulf, SCS | Len. freq. | 28.899 | 0.520 | 2.638 | [70] |
| m_3 | P. macrocephalus | Beibu Gulf, SCS | Scale | 31.502 | 0.590 | 2.768 | [73] |
| m_4 | P. macrocephalus | Southwest, TW | Otolith (D) | 27.028 | 0.596 | 2.639 | [34] |
| m_5 | P. macrocephalus | Yunlin, TW | Otolith (B) | 21.358 | 0.371 | 2.228 | [32] |
| mc_1 | P. macrocephalus | Southwest, TW | Otolith (I) | 19.592 | 0.328 | 2.099 | This study |
| mc_2 | P. macrocephalus | Southwest, TW | Otolith (Q) | 18.004 | 0.789 | 2.408 | This study |
| mc_3 | P. macrocephalus | Southwest, TW | Otolith (B) | 18.258 | 0.617 | 2.313 | This study |
| a_1 | P. argentata | TW strait | Scale | 30.887 | 0.978 | 2.970 | [74] |
| a_2 | P. argentata | TW strait | Scale | 35.713 | 0.375 | 2.680 | [74] |
| a_3 | P. argentata | Southern, ECS | Scale | 28.878 | 1.207 | 3.003 | [74] |
| a_4 | P. argentata | Southern, ECS | Scale | 34.733 | 0.315 | 2.580 | [74] |
| a_5 | P. argentata | Beibu Gulf, SCS | Scale | 30.500 | 0.350 | 2.513 | [75] |
| a_6 | P. argentata | Beibu Gulf, SCS | Scale | 28.230 | 0.500 | 2.600 | [76] |
| a_7 | P. argentata | Northern, SCS | Len. freq. | 38.200 | 0.420 | 2.787 | [71] |
| a_8 | P. argentata | Beibu Gulf, SCS | Len. freq. | 31.500 | 0.350 | 2.541 | [71] |
| a_9 | P. argentata | TW strait | Otolith | 36.301 | 0.377 | 2.696 | [30] |
| a_10 | P. argentata | Southern Sea, SK | Otolith | 35.529 | 0.380 | 2.681 | [31] |
| a_11 | P. argentata♀ | Ariake Sound, JP | Otolith | 31.200 | 0.384 | 2.573 | [77] |
| a_12 | P. argentata♂ | Ariake Sound, JP | Otolith | 29.400 | 0.360 | 2.493 | [77] |
| a_13 | P. argentata ♀ | Tachibana Bay, JP | Otolith | 35.400 | 0.272 | 2.533 | [28] |
| a_14 | P. argentata ♂ | Tachibana Bay, JP | Otolith | 29.000 | 0.342 | 2.459 | [28] |
| a_15 | P. argentata♀ | Omura Bay, JP | Otolith | 37.000 | 0.256 | 2.545 | [28] |
| a_16 | P. argentata♂ | Omura Bay, JP | Otolith | 37.700 | 0.181 | 2.410 | [28] |
| a_17 | P. argentata♀ | The Sea of Goto, JP | Otolith | 44.500 | 0.241 | 2.679 | [28] |
| a_18 | P. argentata♂ | The Sea of Goto, JP | Otolith | 45.200 | 0.195 | 2.600 | [28] |
| a_19 | P. argentata | Seto Inland Sea | Scale | 39.56 | 0.307 | 5.68 | [78] |
| p_1 | P. pawak | Beibu Gulf, SCS | Scale (Logistic) | 22.030 | 0.580 | 2.449 | [79] |
| p_2 | P. pawak | Beibu Gulf, SCS | Len. freq. | 24.150 | 0.390 | 2.357 | [80] |
| p_3 | P. pawak | Beibu Gulf, SCS | Len. freq. | 22.050 | 0.320 | 2.192 | [80] |
| an_1 | P. anea | Paradeep, IN | Len. freq. | 30.300 | 0.860 | 2.897 | [81] |
| an_2 | P. anea | San Miguel Bay, PHI | Len. freq. | 20.000 | 0.600 | 2.380 | [82] |
| an_3 | P. anea | Manila Bay, PHI | Len. freq. | 26.500 | 1.400 | 2.993 | [83] |
| an_4 | P. anea | Penang/Perak, MA | NA | 34.200 | 0.400 | 2.670 | [84] |
| an_5 | P. anea | Bombay, IN | Len. freq. | 24.500 | 0.640 | 2.585 | [85] |
| an_6 | P. anea | Bombay, IN | Len. freq. | 26.000 | 1.200 | 2.909 | [86] |
| an_7 | P. anea | Rameswaram, IN | Len. freq. | 23.300 | 1.260 | 2.835 | [87] |
| an_8 | P. anea | Mandapam, IN | Len. freq. | 26.000 | 0.980 | 2.821 | [88] |
| an_9 | P. anea | Bombay, IN | Len. freq. | 27.300 | 1.940 | 3.160 | [89] |
| an_x | P. anea | Andhra Pradesh, IN | Len. freq. | 33.000 | 0.700 | 2.882 | [90] |
| an_y | P. anea | Indonesia | Len. freq. | 23.890 | 0.840 | 2.681 | [91] |
| n_1 | A. nibe | Formosa Strait | NA | 58.500 | 0.116 | 2.599 | [92] |
| n_2 | A. nibe | North, TW | Len. freq. | 58.700 | 0.145 | 2.699 | [92] |
| n_3 | A. nibe | North, TW | Len. freq. | 37.100 | 0.354 | 2.688 | [92] |
| n_4 | A. nibe | North, TW | Len. freq. | 37.100 | 0.523 | 2.857 | [92] |
| n_5 | A. nibe | North, TW | Len. freq. | 57.300 | 0.177 | 2.764 | [92] |
| n_6 | A. nibe | South, SK | Len. freq. | 50.900 | 0.227 | 2.769 | [92] |
| n_7 | A. nibe | South, SK | Len. freq. | 46.900 | 0.297 | 2.815 | [92] |
| n_8 | A. nibe | TW | Scale | 56.290 | 0.120 | 2.580 | [93] |
| n_9 | A. nibe | ECS | Scale | 43.200 | 0.252 | 2.672 | [94] |
| n_10 | A. nibe | Oman Sea | Otolith | 50.000 | 0.200 | 2.699 | [95] |
| n_11 | A. nibe | Guei-Shan Island, TW | Otolith | 48.060 | 0.275 | 2.803 | [96] |
| n_12 | A. nibe | Guei-Shan Island, TW | Otolith | 64.910 | 0.147 | 2.792 | [96] |
| n_13 | A. nibe♀ | NA | Len. freq. | 45.000 | 0.225 | 2.659 | [92] |
| n_14 | A. nibe♂ | NA | Len. freq. | 40.100 | 0.238 | 2.583 | [92] |
| n_15 | A. nibe ♀ | Southwest, TW | Otolith | 53.384 | 0.183 | 2.717 | [97] |
| n_16 | A. nibe ♂ | Southwest, TW | Otolith | 52.577 | 0.187 | 2.713 | [97] |
| n_17 | A. nibe ♀ | Northeast, TW | Otolith | 49.527 | 0.227 | 2.746 | [98] |
| n_18 | A. nibe♂ | Northeast, TW | Otolith | 48.417 | 0.201 | 2.673 | [98] |
| nc_1 | A. nibe | Southwest, TW | Otolith (VBGM) | 68.149 | 0.130 | −0.919 | This study |
| nc_2 | A. nibe | Southwest, TW | Otolith (Logistic) | 53.111 | 0.394 | 2.958 | This study |
| al | A. alcocki | Pakistani waters | Len. freq. | 47.250 | 0.180 | 2.604 | [99] |
| Species | Model | Sex | L∞ (cm TL) | k (Year−1) | t0 (Year) | p | AICc | ∆AICc | w |
|---|---|---|---|---|---|---|---|---|---|
| P. macrocephalus | VBGM | C | 18.004 (0.315) | 0.789 (0.157) | −0.872 (0.368) | - | 1029.04 | 0 | 0.468 |
| P. macrocephalus | VBGM | F | 18.673 (0.982) | 0.495 (0.239) | −2.095 (1.280) | - | 445.08 | 0 | 0.386 |
| P. macrocephalus | VBGM | M | 17.864 (0.352) | 0.892 (0.202) | −0.571 (0.367) | - | 585.84 | 0 | 0.390 |
| P. macrocephalus | Gompertz | C | 17.921 (0.285) | 0.879 (0.162) | −0.564 (0.294) | - | 1029.81 | 0.774 | 0.318 |
| P. macrocephalus | Gompertz | F | 18.647 (0.955) | 0.532 (0.243) | −1.693 (1.015) | - | 445.39 | 0.311 | 0.330 |
| P. macrocephalus | Gompertz | M | 17.777 (0.316) | 1.002 (0.210) | −0.286 (0.290) | - | 586.17 | 0.328 | 0.331 |
| P. macrocephalus | Logistic | C | 17.850 (0.262) | 0.970 (0.168) | −0.311 (0.241) | - | 1030.59 | 1.556 | 0.215 |
| P. macrocephalus | Logistic | F | 18.643 (0.947) | 0.565 (0.246) | −1.354 (0.808) | - | 445.69 | 0.612 | 0.284 |
| P. macrocephalus | Logistic | M | 17.704 (0.288) | 1.112 (0.219) | −0.055 (0.236) | - | 586.52 | 0.678 | 0.278 |
| A. nibe | VBGM | C | 68.149 (8.182) | 0.130 (0.033) | −0.919 (0.413) | - | 2373.40 | 6.380 | 0.029 |
| A. nibe | VBGM | F | 60.548 (5.835) | 0.186 (0.047) | −0.266 (0.439) | - | 1443.83 | 4.090 | 0.075 |
| A. nibe | VBGM | M | 90.094 (37.291) | 0.070 (0.046) | −1.926 (0.870) | - | 911.61 | 1.736 | 0.180 |
| A. nibe | Gompertz | C | 57.162 (3.512) | 0.263 (0.036) | 1.919 (0.194) | - | 2369.53 | 2.514 | 0.202 |
| A. nibe | Gompertz | F | 54.732 (3.226) | 0.318 (0.052) | 1.853 (0.160) | - | 1441.39 | 1.648 | 0.255 |
| A. nibe | Gompertz | M | 62.594 (9.428) | 0.197 (0.050) | 2.313 (0.720) | - | 910.56 | 0.679 | 0.306 |
| A. nibe | Logistic | C | 53.111 (2.294) | 0.394 (0.040) | 2.958 (0.219) | - | 2367.02 | 0 | 0.709 |
| A. nibe | Logistic | F | 52.142 (2.306) | 0.448 (0.052) | 2.804 (0.160) | - | 1439.74 | 0 | 0. 582 |
| A. nibe | Logistic | M | 55.313 (5.285) | 0.322 (0.054) | 3.372 (0.615) | - | 909.88 | 0 | 0.429 |
| A. nibe | Richards | C | 55.345 (2.904) | 0.282 (0.035) | 1.843 (0.162) | −3.661 × 106 (3.477 × 106) | 2371.95 | 4.927 | 0.060 |
| A. nibe | Richards | F | 54.349 (3.260) | 0.322 (0.053) | 1.843 (0.183) | −1.067 × 105 (3.060 × 106) | 1443.53 | 3.788 | 0.088 |
| A. nibe | Richards | M | 57.794 (6.277) | 0.224 (0.049) | 1.944 (0.409) | −6.994 × 106 (6.283 × 106) | 913.12 | 3.248 | 0.085 |
| Species | Region | a | b | Reference |
|---|---|---|---|---|
| P. macrocephalus | Beibu Gulf, SCS | 2.37 × 10−5 | 3.248 | [29] |
| P. macrocephalus | Beibu Gulf, SCS | 2.16 × 10−2 | 3.032 | [70] |
| P. macrocephalus | Beibu Gulf, SCS | 0.01 × 10−6~0.01 × 10−7 | 2.95~3.57 | [73] |
| P. macrocephalus | Yunlin, TW | 4.30 × 10−2 | 2.747 | [32] |
| P. macrocephalus | Southwest, TW ♀ | 4.70 × 10−2 | 2.730 | [106] |
| P. macrocephalus | Southwest, TW ♂ | 4.08 × 10−2 | 2.786 | [106] |
| P. macrocephalus | Southwest, TW | 9.90 × 10−3 | 3.337 | [34] |
| P. macrocephalus | Southwest, TW | 2.77 × 10−2 | 2.937 | This study |
| A. nibe | Yellow Sea and ECS | 2.03 × 10−3 | 3.463 | [107]; cited in [108] |
| A. nibe | ECS | 2.84 × 10−3 | 3.220 | [94]; cited in [108] |
| A. nibe | North, TW | 6.39 × 10−4 | 3.207 | [109]; cited in [108] |
| A. nibe | Northeast, TW | 1.41 × 10−3 | 2.934 | [98]; cited in [108] |
| A. nibe | Northeast, TW | 6.92 × 10−3 | 3.100 | [96] |
| A. nibe | Southwest, TW | 1.21 × 10−3 | 2.968 | [97] |
| A. nibe | Southwest, TW | 2.00 × 10−3 | 2.907 | [110] |
| A. nibe | Oman Sea ♀ | 1.21 × 10−2 | 2.939 | [95] |
| A. nibe | Oman Sea ♂ | 7.5 × 10−3 | 3.074 | [95] |
| A. nibe | Southwest, TW | 1.45 × 10−2 | 2.886 | This study |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Huang, S.-C.; Chang, S.-K.; Lai, C.-C.; Yuan, T.-L.; Weng, J.-S.; He, J.-S. Length–Weight Relationships, Growth Models of Two Croakers (Pennahia macrocephalus and Atrobucca nibe) off Taiwan and Growth Performance Indices of Related Species. Fishes 2022, 7, 281. https://doi.org/10.3390/fishes7050281
Huang S-C, Chang S-K, Lai C-C, Yuan T-L, Weng J-S, He J-S. Length–Weight Relationships, Growth Models of Two Croakers (Pennahia macrocephalus and Atrobucca nibe) off Taiwan and Growth Performance Indices of Related Species. Fishes. 2022; 7(5):281. https://doi.org/10.3390/fishes7050281
Chicago/Turabian StyleHuang, Shu-Chiang, Shui-Kai Chang, Chi-Chang Lai, Tzu-Lun Yuan, Jinn-Shing Weng, and Jia-Sin He. 2022. "Length–Weight Relationships, Growth Models of Two Croakers (Pennahia macrocephalus and Atrobucca nibe) off Taiwan and Growth Performance Indices of Related Species" Fishes 7, no. 5: 281. https://doi.org/10.3390/fishes7050281
APA StyleHuang, S.-C., Chang, S.-K., Lai, C.-C., Yuan, T.-L., Weng, J.-S., & He, J.-S. (2022). Length–Weight Relationships, Growth Models of Two Croakers (Pennahia macrocephalus and Atrobucca nibe) off Taiwan and Growth Performance Indices of Related Species. Fishes, 7(5), 281. https://doi.org/10.3390/fishes7050281






