Structural and Experimental Study of a Multi-Finger Synergistic Adaptive Humanoid Dexterous Hand
Abstract
1. Introduction
2. Dexterous Hand Structural Design
2.1. Overall Design of the Dexterous Hand
2.1.1. Selection of Parameter
2.1.2. Selection of Materials
2.2. Gesture Analysis of Human Hand Grasping
2.3. Underactuation Structure Design
2.3.1. Overall Drive Design
2.3.2. Finger Structure Design
2.3.3. Adaptive Differential Structure Design
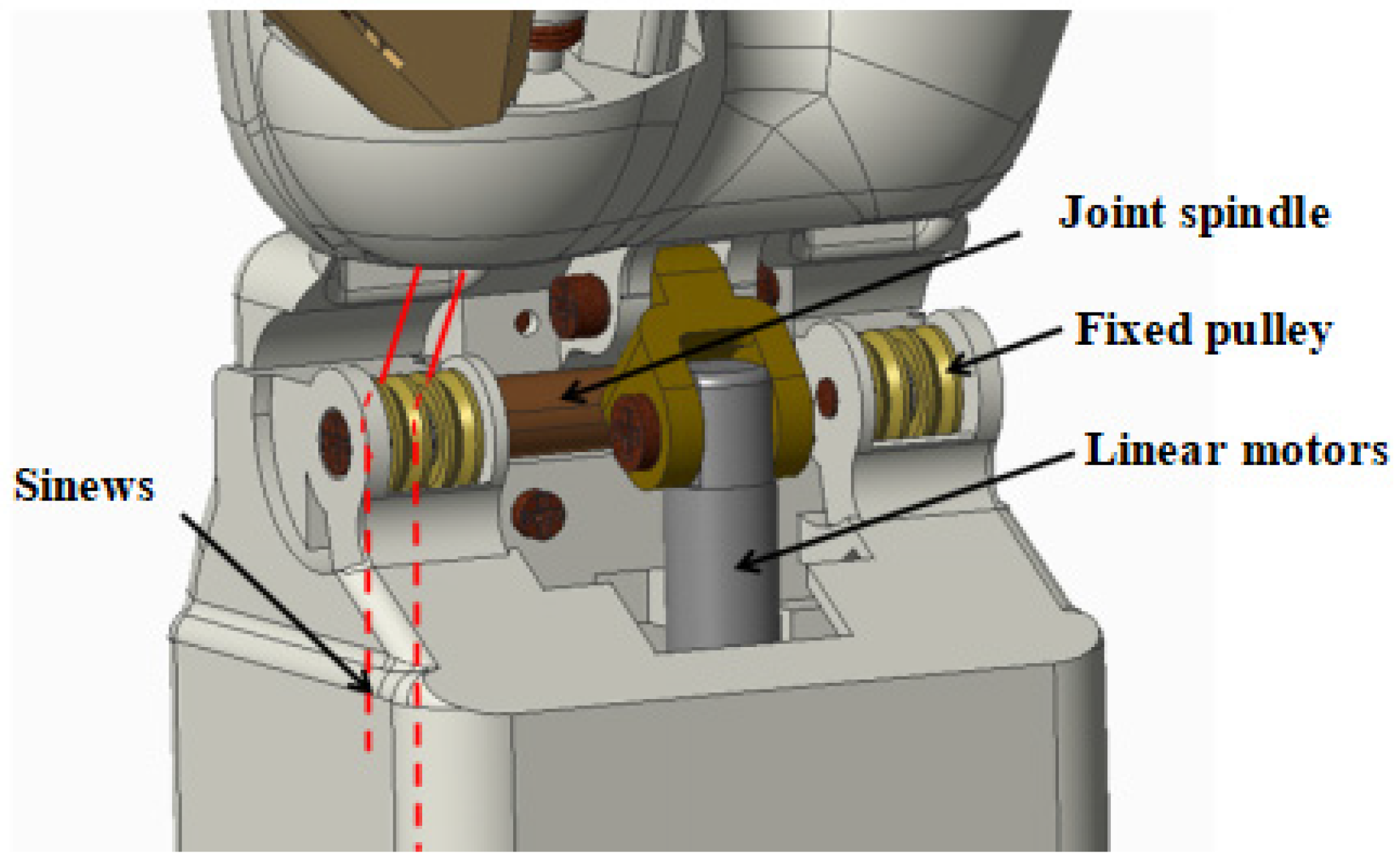
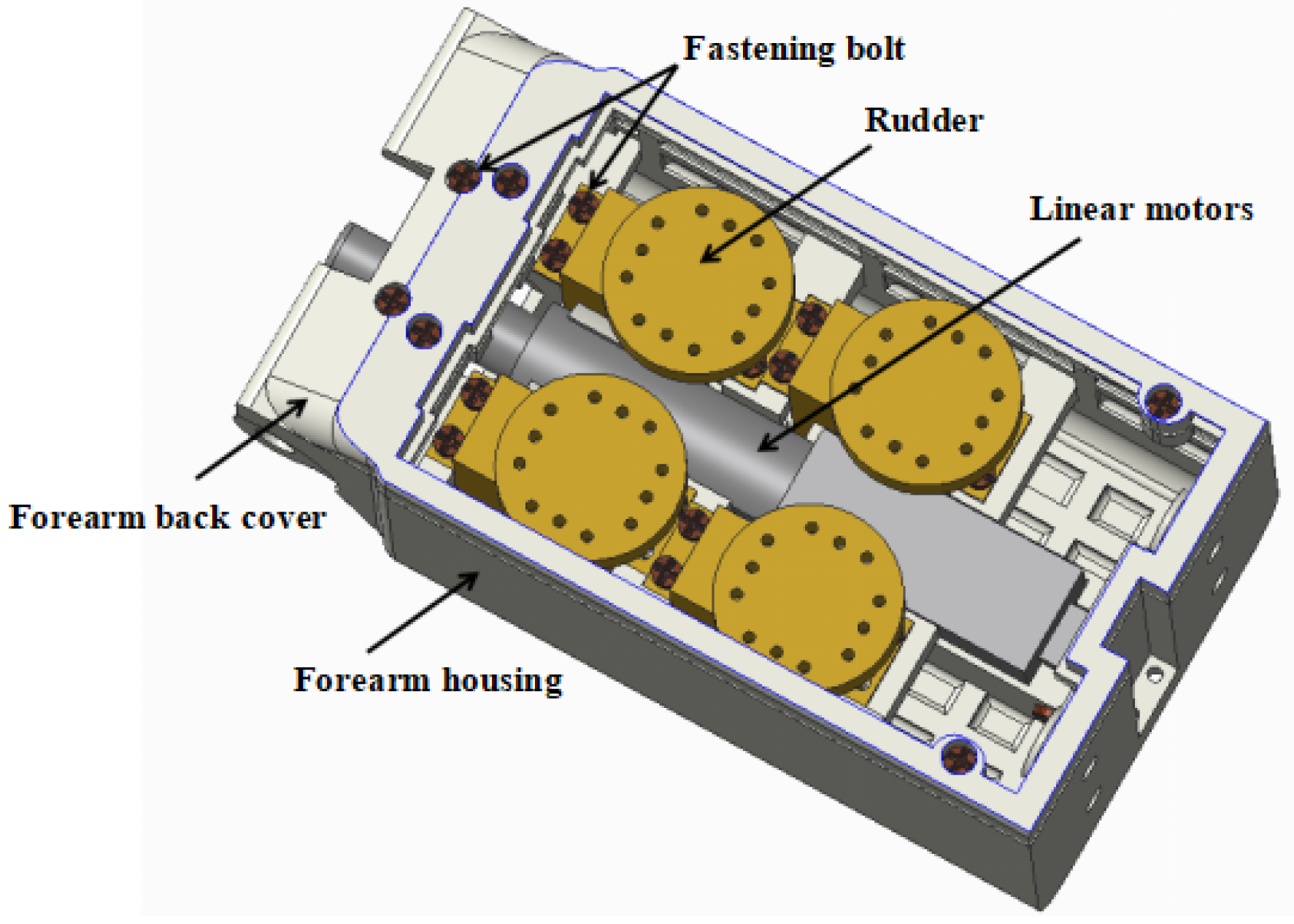
2.3.4. Wrist and Forearm Design
2.4. Control System Design
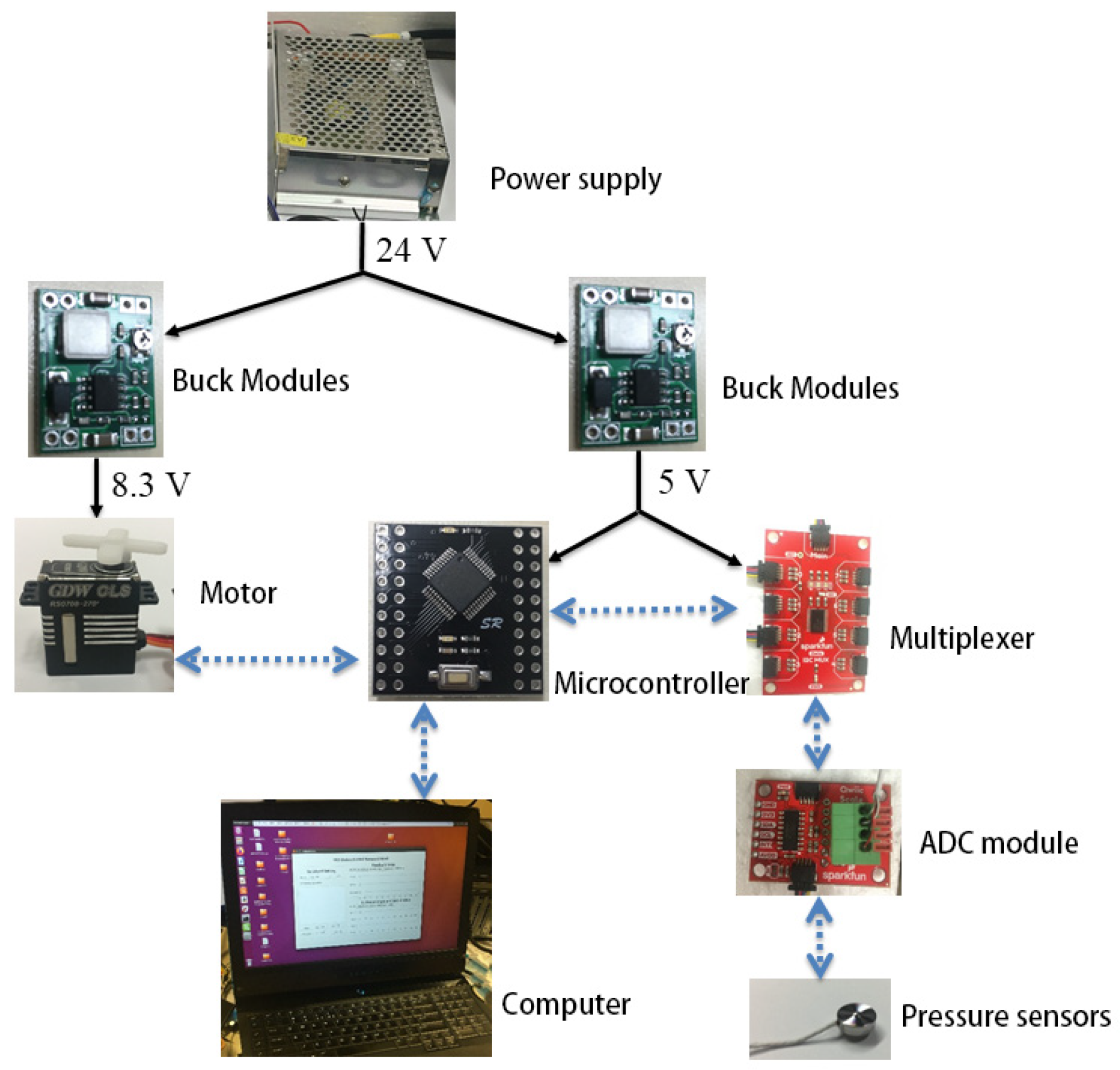
2.4.1. Construction of the Control System
2.4.2. The PID Control Based on Pressure Sensor
3. Motion Analysis of Underactuation Dexterous Hand
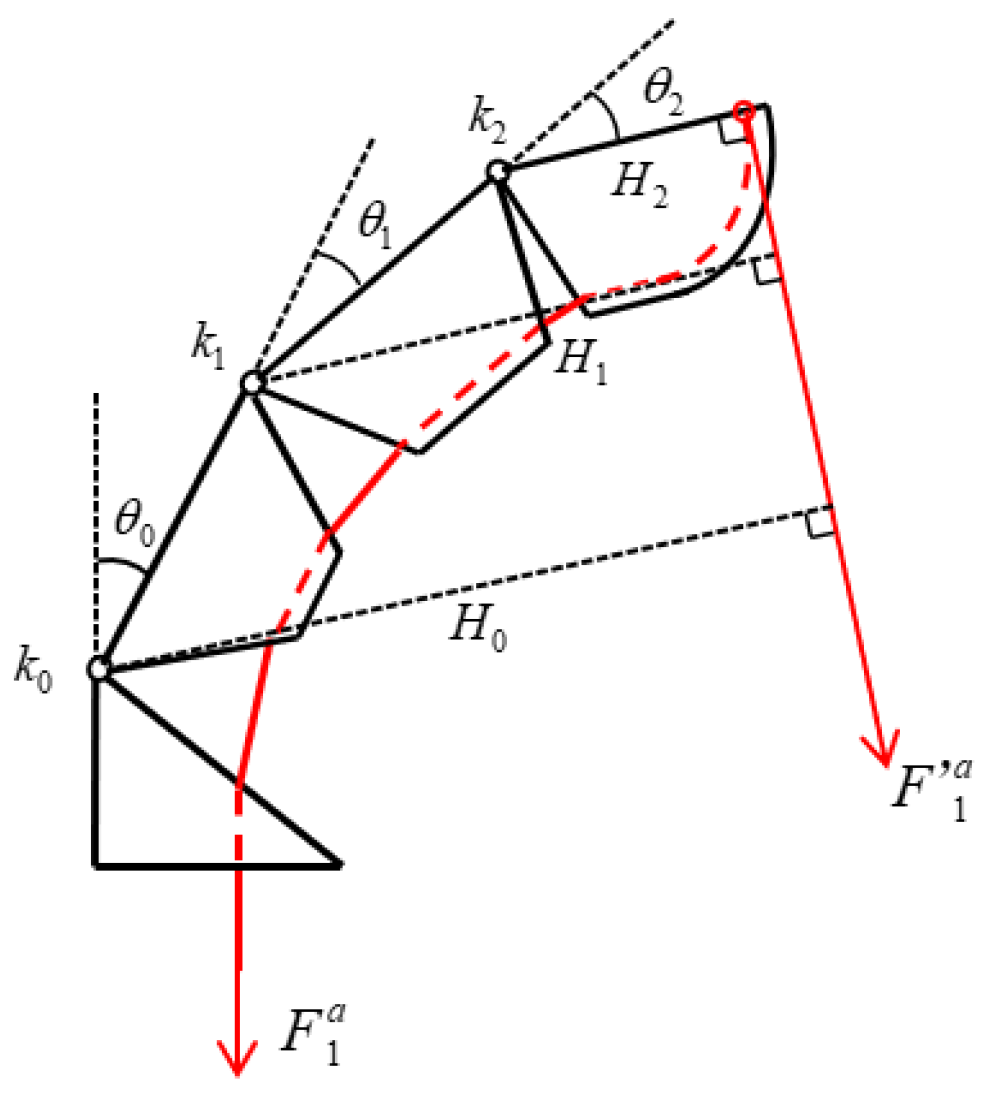
3.1. Driver Key Rope Mapping Relationships
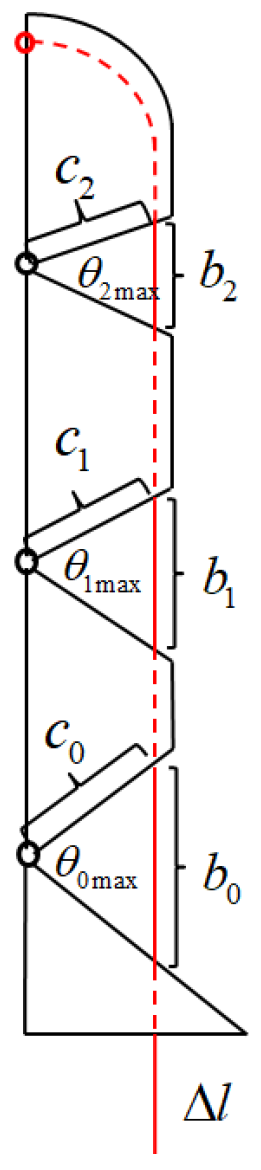
3.2. Dexterous Fingertip Trajectory Analysis
4. Grasping Ability Test
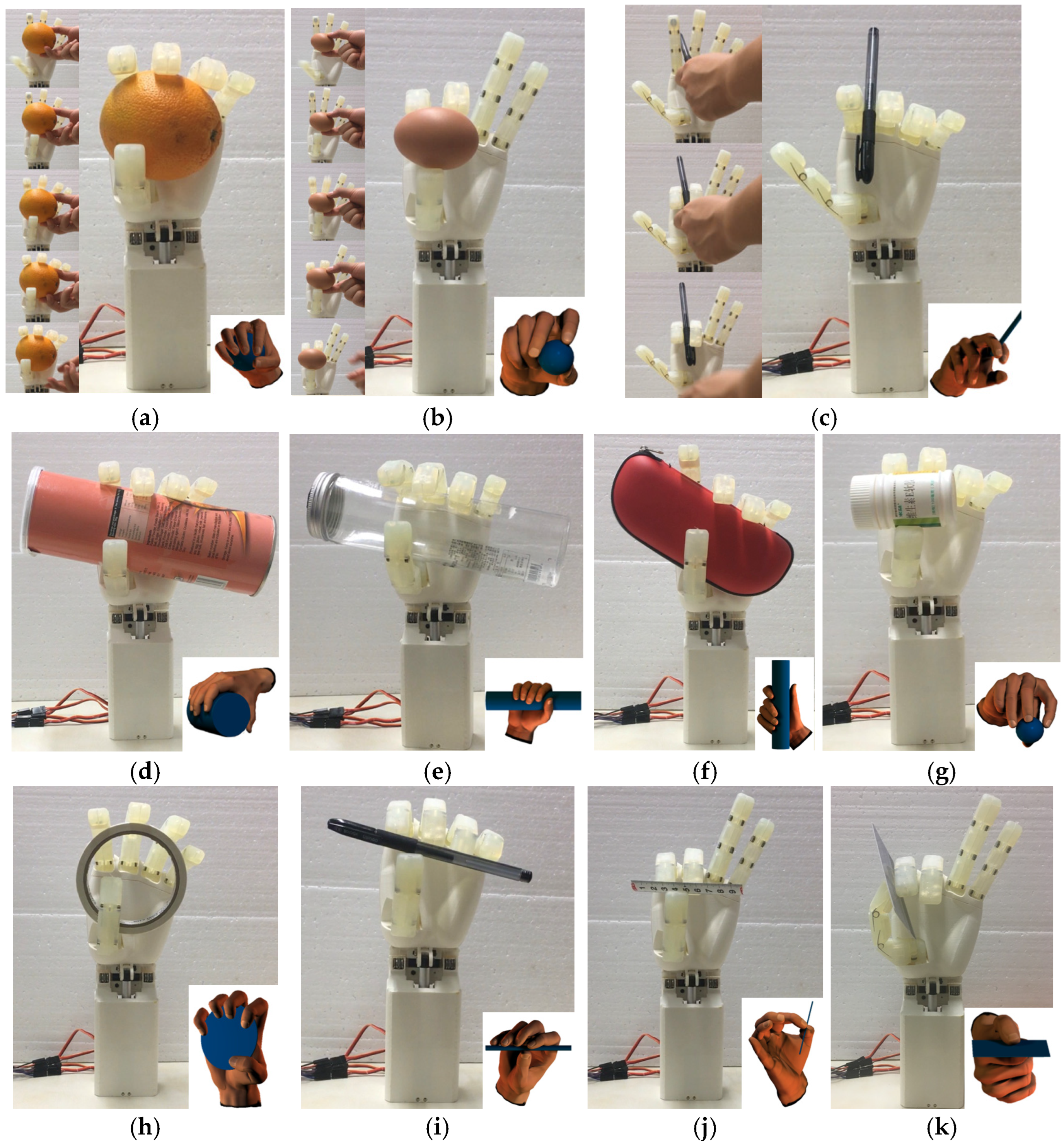
4.1. Grasping Posture Test
4.2. Grip Strength Test
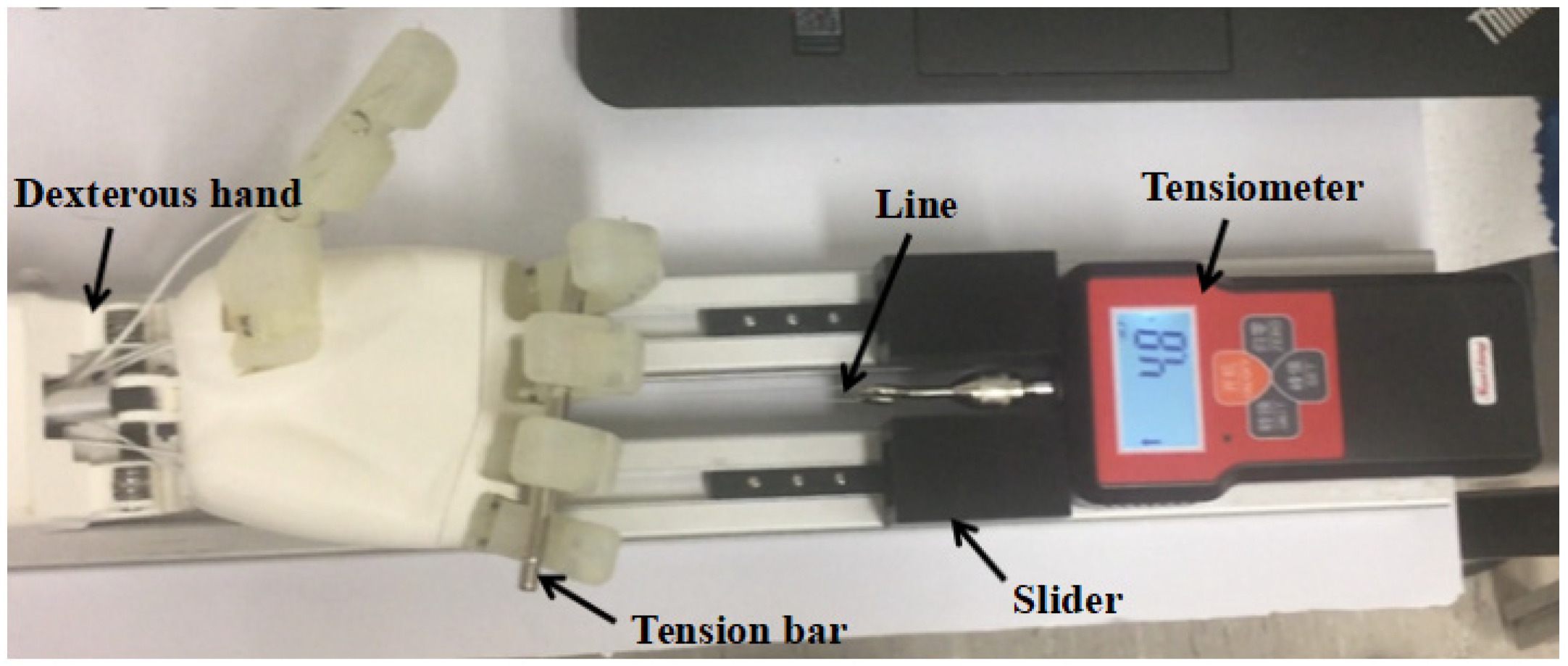
4.2.1. Passive Grip Strength Test
4.2.2. Active Grip Test
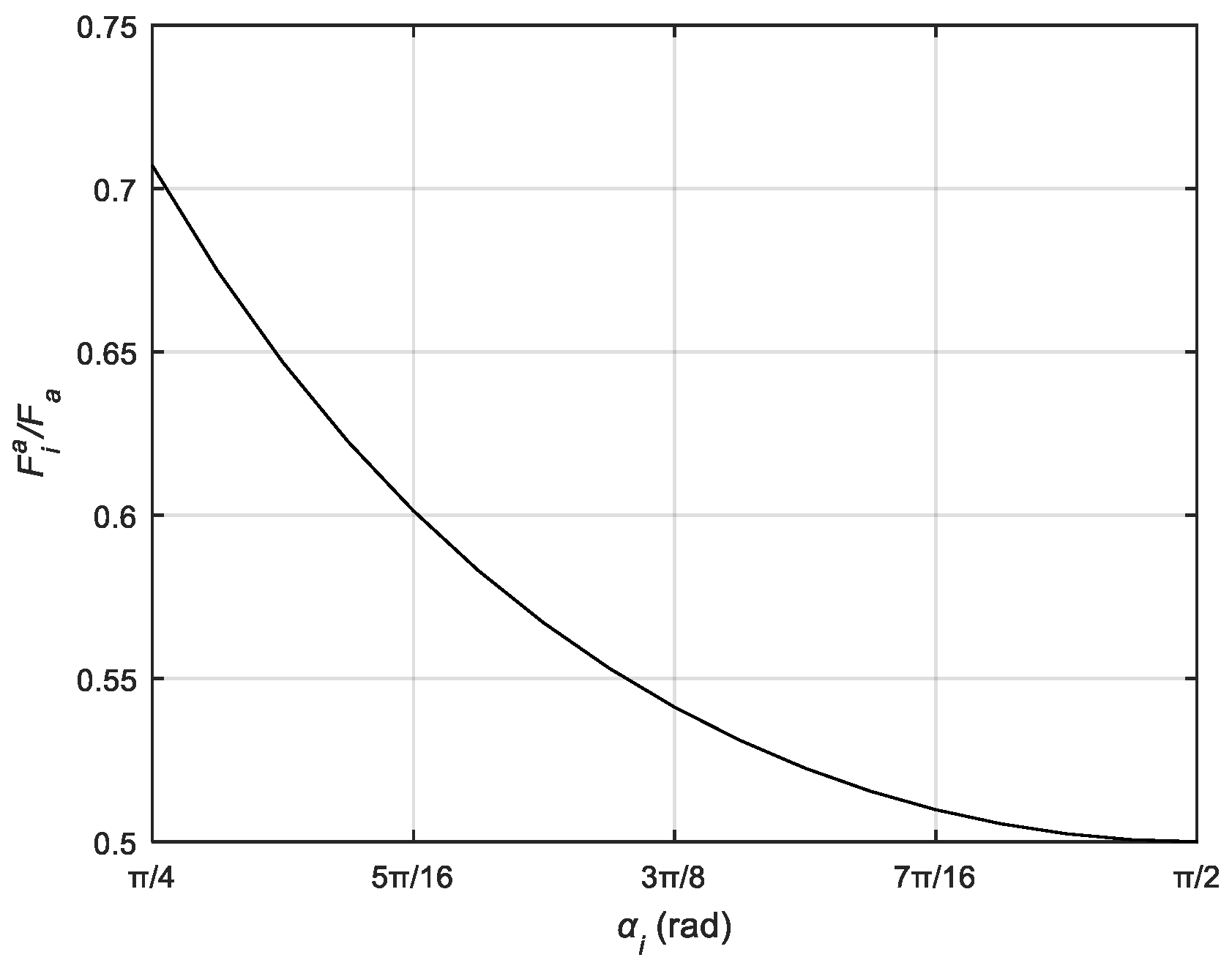
4.2.3. Grip Ratio Test
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Piazza, C.; Grioli, G.; Catalano, M.G.; Bicchi, A. A Century of Robotic Hands. Annu. Rev. Control Robot. Auton. Syst. 2019, 2, 1–32. [Google Scholar] [CrossRef]
- Su, H.; Ovur, S.E.; Xu, Z.; Alfayad, S. Exploring the Potential of Fuzzy Sets in Cyborg Enhancement: A Comprehensive Review. IEEE Trans. Fuzzy Syst. 2025, 33, 810–827. [Google Scholar] [CrossRef]
- Su, H.; Sheiban, F.J.; Qi, W.; Ovur, S.E.; Alfayad, S. A bioinspired virtual reality toolkit for robot-assisted medical application: BioVRbot. IEEE Trans. Hum.-Mach. Syst. 2024, 54, 688–697. [Google Scholar] [CrossRef]
- Qi, W.; Fan, H.; Karimi, H.R.; Su, H. An adaptive reinforcement learning-based multimodal data fusion framework for human–robot confrontation gaming. Neural Netw. 2023, 164, 489–496. [Google Scholar] [CrossRef] [PubMed]
- Qi, W.; Xu, X.; Qian, K.; Schuller, B.W.; Fortino, G.; Aliverti, A. A Review of AIoT-based Human Activity Recognition: From Application to Technique. IEEE J. Biomed. Health Inform. 2024, 1–14. [Google Scholar] [CrossRef] [PubMed]
- Kemp, C.C.; Edsinger, A.; Torres-Jara, E. Challenges for robot manipulation in human environments [Grand Challenges of Robotics]. IEEE Robot. Autom. Mag. 2007, 14, 20–29. [Google Scholar] [CrossRef]
- Zappatore, G.A.; Reina, G.; Messina, A. Analysis of a highly underactuated robotic hand. Int. J. Mech. Control 2017, 18, 17–24. [Google Scholar]
- Massa, B.; Roccella, S.; Carrozza, M.C.; Dario, P. Design and development of an underactuated prosthetic hand. In Proceedings of the 2002 IEEE International Conference on Robotics and Automation (Cat. No.02CH37292), Washington, DC, USA, 11–15 May 2002; Volume 4, pp. 3374–3379. [Google Scholar] [CrossRef]
- Birglen, L.; Laliberté, T.; Gosselin, C.M. Underactuated Robotic Hands; Springer: Berlin/Heidelberg, Germany, 2007; Volume 40, pp. 7–13. [Google Scholar]
- Odhner, L.U.; Jentoft, L.P.; Claffee, M.R.; Corson, N.; Tenzer, Y.; Ma, R.R.; Buehler, M.; Kohout, R.; Howe, R.D.; Dollar, A.M. A compliant, underactuated hand for robust manipulation. Int. J. Robot. Res. 2014, 33, 736–752. [Google Scholar] [CrossRef]
- Catalano, M.G.; Grioli, G.; Farnioli, E.; Serio, A.; Piazza, C.; Bicchi, A. Adaptive synergies for the design and control of the Pisa/IIT SoftHand. Int. J. Robot. Res. 2014, 33, 768–782. [Google Scholar] [CrossRef]
- Della Santina, C.; Piazza, C.; Grioli, G.; Catalano, M.G.; Bicchi, A. Toward dexterous manipulation with augmented adaptive synergies: The pisa/iit softhand 2. IEEE Trans. Robot. 2018, 34, 1141–1156. [Google Scholar] [CrossRef]
- Mitsui, K.; Ozawa, R.; Kou, T. An under-actuated robotic hand for multiple grasps. In Proceedings of the IEEE/RSJ International Conference on Intelligent Robots and Systems, Tokyo, Japan, 3–7 November 2013; IEEE: Piscataway, NJ, USA, 2013; pp. 5475–5480. [Google Scholar] [CrossRef]
- Zhou, J.; Yi, J.; Chen, X.; Liu, Z.; Wang, Z. BCL-13: A 13-DOF soft robotic hand for dexterous grasping and in-hand manipulation. IEEE Robot. Autom. Lett. 2018, 3, 3379–3386. [Google Scholar] [CrossRef]
- Luo, C.; Zhang, W. A flexible self-adaptive underactuated hand with series passive joints. Ind. Robot Int. J. 2019, 45, 516–525. [Google Scholar] [CrossRef]
- Tavakoli, M.; Enes, B.; Santos, J.; Marques, L.; de Almeida, A.T. Underactuated anthropomorphic hands: Actuation strategies for a better functionality. Robot. Auton. Syst. 2015, 74, 267–282. [Google Scholar] [CrossRef]
- Dalley, A.F. Grant’s Atlas of Anatomy; Lippincott Williams & Wilkins: Philadelphia, PA, USA, 2009; p. 163. [Google Scholar]
- Miller, K.H. Man/System Integration Standards for Space Systems. Proc. Hum. Factors Soc. Annu. Meet. 1986, 30, 358–362. [Google Scholar] [CrossRef]
- Minami, A.; An, K.-N.; Cooney, W.P., III; Linscheid, R.L.; Chao, E.Y.S. Ligament stability of the metacarpophalangeal joint: A biomechanical study. J. Hand Surg. 1985, 10, 255–260. [Google Scholar] [CrossRef]
- Birglen, L.; Gosselin, C.M. Automation, Kinetostatic analysis of underactuated fingers. IEEE Trans. Robot. Autom. 2004, 20, 211–221. [Google Scholar] [CrossRef]
- Ma, R.R.; Odhner, L.U.; Dollar, A.M. A modular, open-source 3D printed underactuated hand. In Proceedings of the 2013 IEEE International Conference on Robotics and Automation, Karlsruhe, Germany, 6–10 May 2013; pp. 2737–2743. [Google Scholar] [CrossRef]
- Shahrubudin, N.; Lee, T.C.; Ramlan, R. An overview on 3D printing technology: Technological, materials, and applications. Procedia Manuf. 2019, 35, 1286–1296. [Google Scholar] [CrossRef]
- Lee, J.-Y.; An, J.; Chua, C.K. Fundamentals and applications of 3D printing for novel materials. Appl. Mater. Today 2017, 7, 120–133. [Google Scholar] [CrossRef]
- Dunai, L.; Novak, M.; García Espert, C. Human Hand Anatomy-Based Prosthetic Hand. Sensors 2021, 21, 137. [Google Scholar] [CrossRef]
- Zhou, X.; Fu, H.; Shentu, B.; Wang, W.; Cai, S.; Bao, G. Design and Control of a Tendon-Driven Robotic Finger Based on Grasping Task Analysis. Biomimetics 2024, 9, 370. [Google Scholar] [CrossRef]
- Feix, T.; Romero, J.; Schmiedmayer, H.-B.; Dollar, A.M.; Kragic, D. The grasp taxonomy of human grasp types. IEEE Trans. Hum.-Mach. Syst. 2015, 46, 66–77. [Google Scholar] [CrossRef]
- Jara, C.A.; Pomares, J.; Candelas, F.A.; Torres, F. Control framework for dexterous manipulation using dynamic visual servoing and tactile sensors’ feedback. Sensors 2014, 14, 1787–1804. [Google Scholar] [CrossRef] [PubMed]
- Fukaya, N.; Ogasawara, Y. Development of humanoid hand with cover integrated link mechanism for daily life work. In Proceedings of the 2017 IEEE 6th Global Conference on Consumer Electronics (GCCE), Nagoya, Japan, 24–27 October 2017; IEEE: Piscataway, NJ, USA, 2017; pp. 1–4. [Google Scholar] [CrossRef]
- Rossi, C.; Savino, S. Gripping Tests on an Underactuated Self-adapting Hand Prototype. In Proceedings of the ROMANSY 21-Robot Design, Dynamics and Control: Proceedings of the 21st CISM-IFToMM Symposium, Udine, Italy, 20–23 June 2016; Springer: Cham, Switzerland, 2016; pp. 199–206. [Google Scholar] [CrossRef]
- Laliberté, T.; Baril, M.; Guay, F.; Gosselin, C. Towards the design of a prosthetic underactuated hand. Mech. Sci. 2010, 1, 19–26. [Google Scholar] [CrossRef]
- Laliberté, T.; Birglen, L.; Gosselin, C.M. Underactuation in robotic grasping hands. Mach. Intell. Robot. Control 2002, 4, 1–11. [Google Scholar]

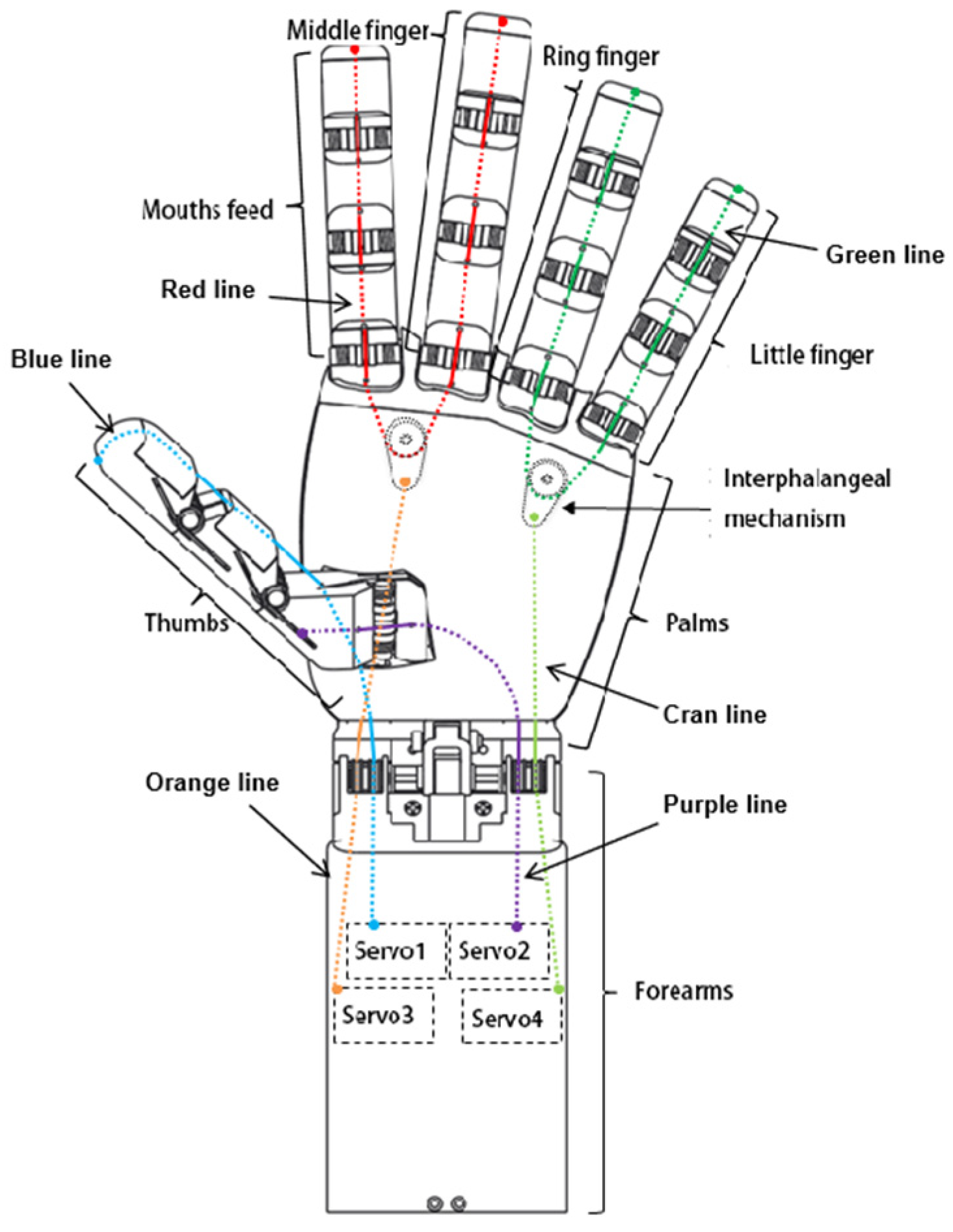

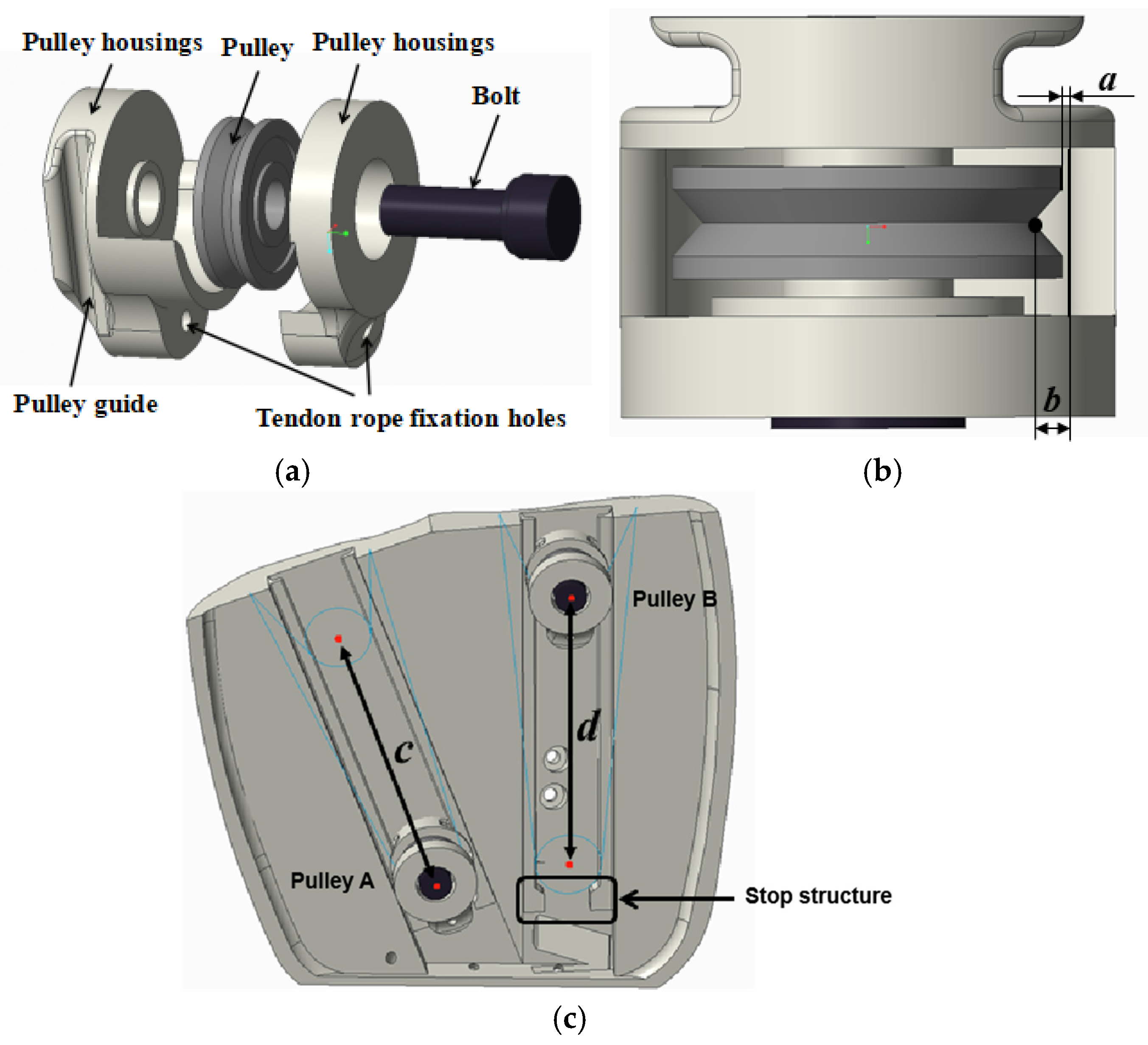
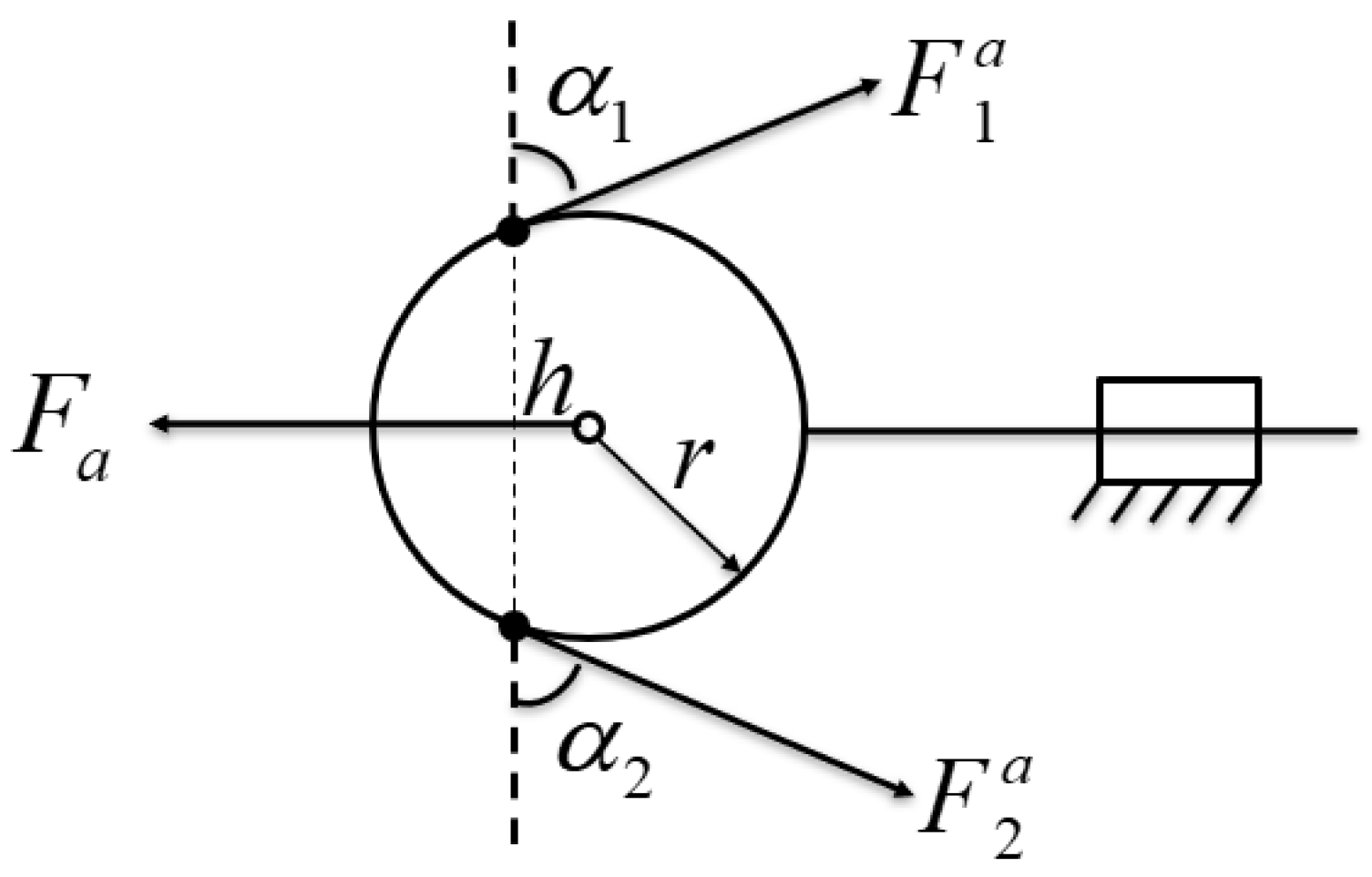
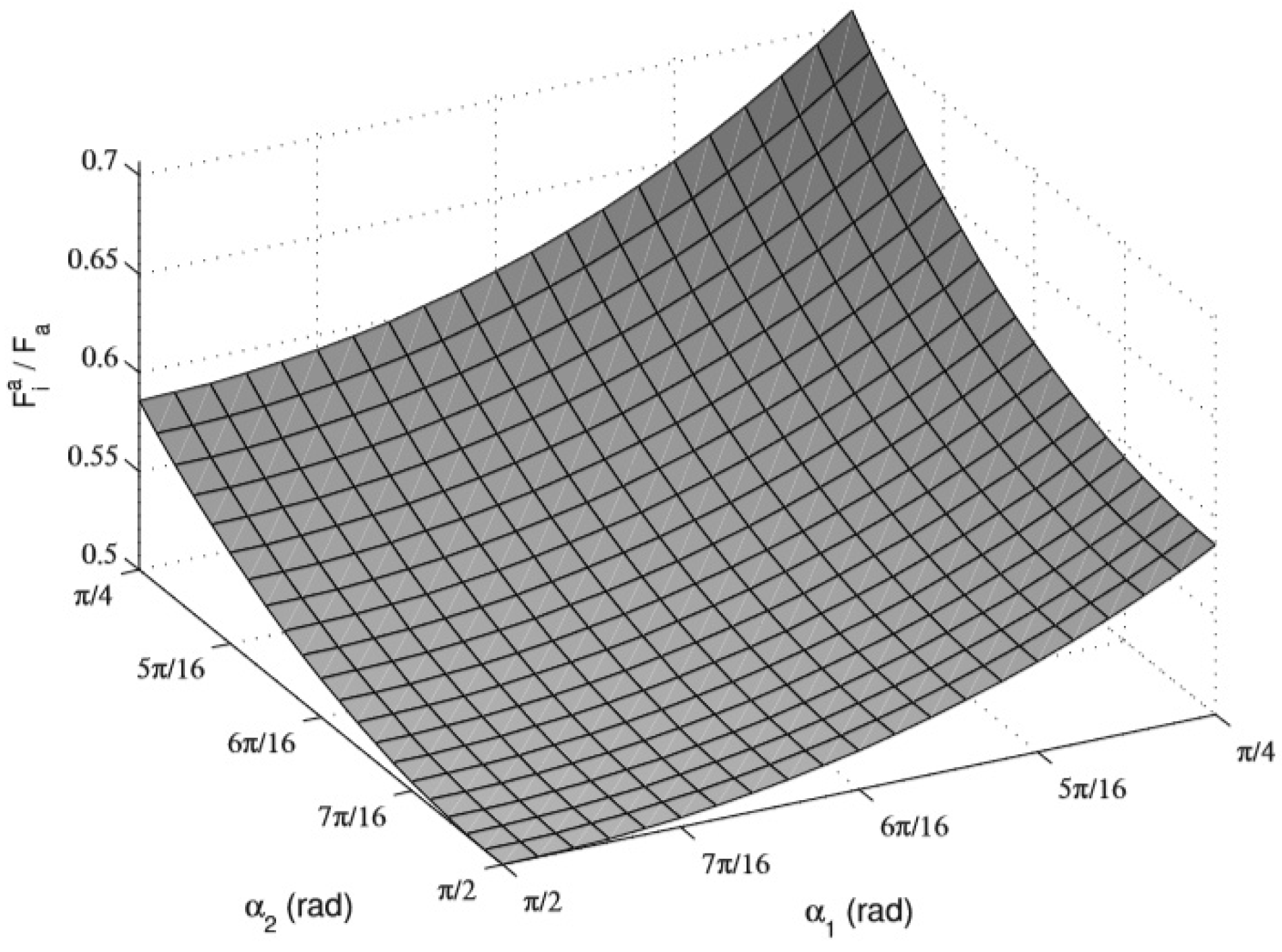





| Dimensions (mm) | Average Length | Average Width | Average Hand Circumference |
|---|---|---|---|
| Adult male hand | 193 | 89 | 218 |
| Adult female hand | 173 | 79 | 178 |
| Dexterous hand | 180 | 82 | 202 |
| Dimensions (mm) | Thumb | Mouths Feed | Middle Finger | Ring Finger | Little Finger |
|---|---|---|---|---|---|
| Distal joint | 28 | 24 | 27 | 24 | 20 |
| Middle joint | - | 26 | 29 | 26 | 22 |
| Proximal joint | 31 | 29 | 32 | 29 | 25 |
| Total length | 59 | 79 | 88 | 79 | 67 |
| Finger width | 19 | 17 | 17 | 17 | 15 |
| Finger thickness | 16 | 16 | 16 | 16 | 14 |
| Thumb | Mouths Feed | Middle Finger | Ring Finger | Little Finger | |
|---|---|---|---|---|---|
| DIP () | 0–70 | ||||
| PIP () | - | 0–85 | |||
| MCP () | 0–90 | ||||
| CMC () | 0–100 | - | - | - | - |
| Material | Molding Method | Tensile Modulus (GPa) | Yield Elongation (%) | Heat Deflection Temperature at 0.45 MPa (°C) |
|---|---|---|---|---|
| PLA | FDM | 2.34 | 3.3 | 56.0 |
| General Resin | SLA | 2.80 | 6.2 | 58.4 |
| Wear-resistant resin | SLA | 1.26 | 49.0 | 43.3 |
| Contact Area | Thumbs Up for Participation | Adductor Hallucis Longus | Thumbs Participation | Five-Finger Participation | Four-Finger Participation | Three-Finger Participation | Two-Finger Participation | Power-Based | Precision | Intermediate |
|---|---|---|---|---|---|---|---|---|---|---|
| intermediate | 31.4% | 10.2% | 21.6% | 31.4% | 0.4% | 0% | 0% | 31.8% | 0% | 0% |
| facet | 44.7% | 2.0% | 42.7% | 17.1% | 4.5% | 16.3% | 6.8% | 7.3% | 37.4% | 0% |
| digit side | 21.6% | 20.9% | 2.9% | 1.6% | 0% | 0.7% | 21.5% | 0% | 0.7% | 23.1% |
| total frequency | 97.7% | 33.1% | 67.2% | 50.1% | 4.9% | 17% | 28.3% | 39.1% | 38.1% | 23.1% |
| Tom Thumb | Mouths Feed | Middle Finger | Ring Finger | Little Finger | Finesse | Total | |
|---|---|---|---|---|---|---|---|
| Number of bending degrees of freedom | 2 | 3 | 3 | 3 | 3 | 1 | 15 |
| Number of degrees of freedom for side pendulum | 1 | 0 | 1 | ||||
| Number of drives | 2 | 1 | 1 | 1 | 5 | ||
| Range | Output Sensitivity | Zero Output | Nolinear | Repeatability | Hysteresis | Creep |
|---|---|---|---|---|---|---|
| 10 kg | % F.S | 0.5–1% F.S | 0.3% F.S | 0.3% F.S | 0.25% F.S |
| DIP | PIP | MCP | CMC | |
|---|---|---|---|---|
| Material | SUS304WPB | |||
| () | 186,000 | 186,000 | 186,000 | 186,000 |
| (mm) | 0.7 | 0.65 | 0.6 | 0.6 |
| Number of laps | 3 | 3 | 3 | 3 |
| () | 5.3 | 5.4/5.3 | 5.4 | 5.4 |
| Preload angle () | 20 | 20 | 20 | 20 |
| Number of torsion spring | 2 | 1/1 | 2 | 2 |
| Coefficient of elasticity | 0.76 | 0.56 | 0.41 | 0.41 |
| Initial torque () | 0.531 | 0.391 | 0.286 | 0.286 |
| Input Voltage (V) | 6.0 | 7.4 | 8.4 |
|---|---|---|---|
| Speed (s/60) | 0.12 | 0.1 | 0.08 |
| Torsion () | 6.0 | 6.8 | 7.5 |
| Operating current (mA) | 400 | 500 | 600 |
| Plugging current (mA) | 1200 | 1350 | 1500 |
| Weights (g) | 20 | ||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cao, S.; Bao, G.; Pan, L.; Yang, B.; Zhou, X. Structural and Experimental Study of a Multi-Finger Synergistic Adaptive Humanoid Dexterous Hand. Biomimetics 2025, 10, 155. https://doi.org/10.3390/biomimetics10030155
Cao S, Bao G, Pan L, Yang B, Zhou X. Structural and Experimental Study of a Multi-Finger Synergistic Adaptive Humanoid Dexterous Hand. Biomimetics. 2025; 10(3):155. https://doi.org/10.3390/biomimetics10030155
Chicago/Turabian StyleCao, Shengke, Guanjun Bao, Lufeng Pan, Bangchu Yang, and Xuanyi Zhou. 2025. "Structural and Experimental Study of a Multi-Finger Synergistic Adaptive Humanoid Dexterous Hand" Biomimetics 10, no. 3: 155. https://doi.org/10.3390/biomimetics10030155
APA StyleCao, S., Bao, G., Pan, L., Yang, B., & Zhou, X. (2025). Structural and Experimental Study of a Multi-Finger Synergistic Adaptive Humanoid Dexterous Hand. Biomimetics, 10(3), 155. https://doi.org/10.3390/biomimetics10030155






