Numerical Study on Cross-Linked Cold Plate Design for Thermal Management of High-Power Lithium-Ion Battery
Abstract
1. Introduction
2. Physical Model
3. Mathematical Model
3.1. Governing Equations
3.2. Battery Model
3.3. Initial and Boundary Conditions
3.4. Numerical Method and Validations
4. Results and Discussion
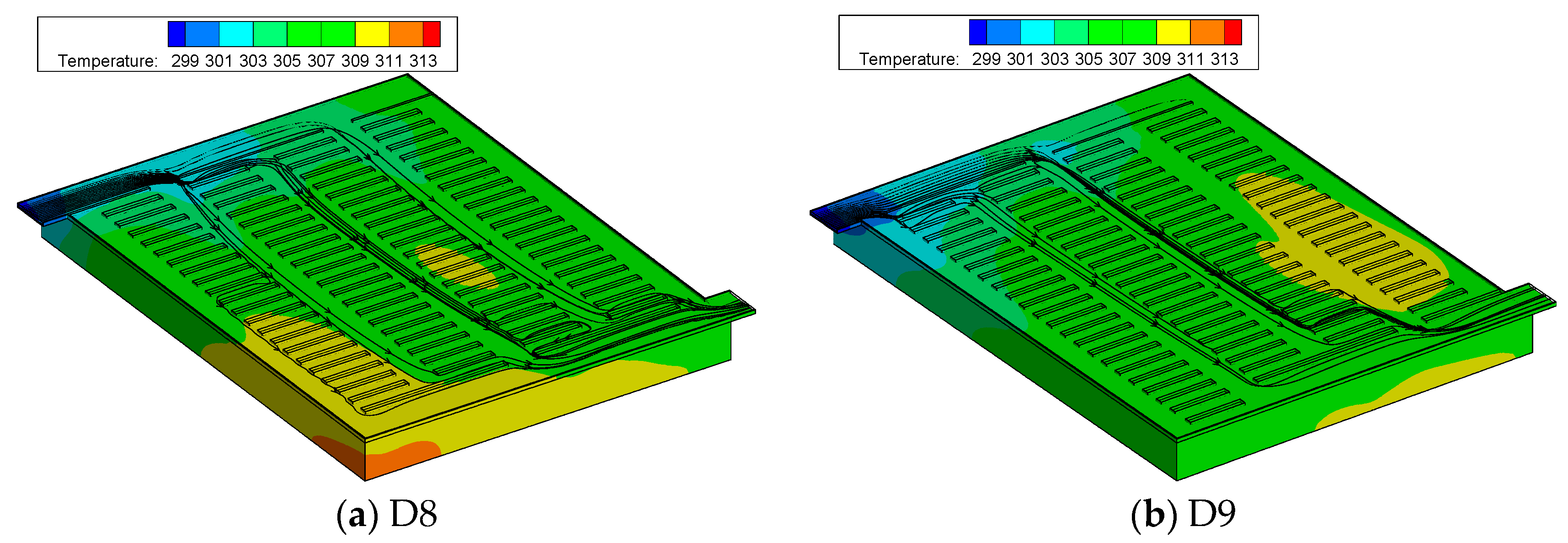
4.1. Temperature Distribution in Different Designs
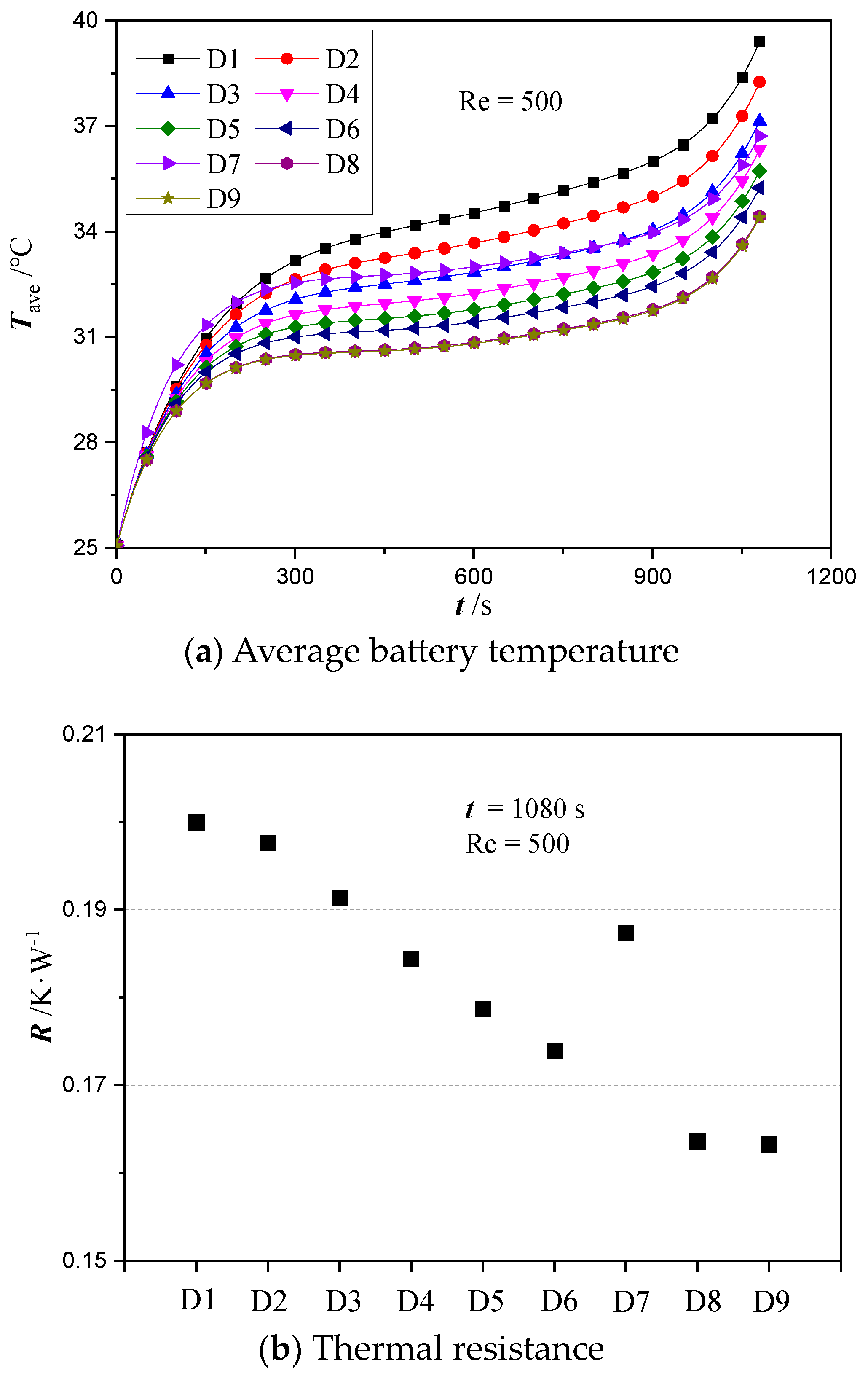
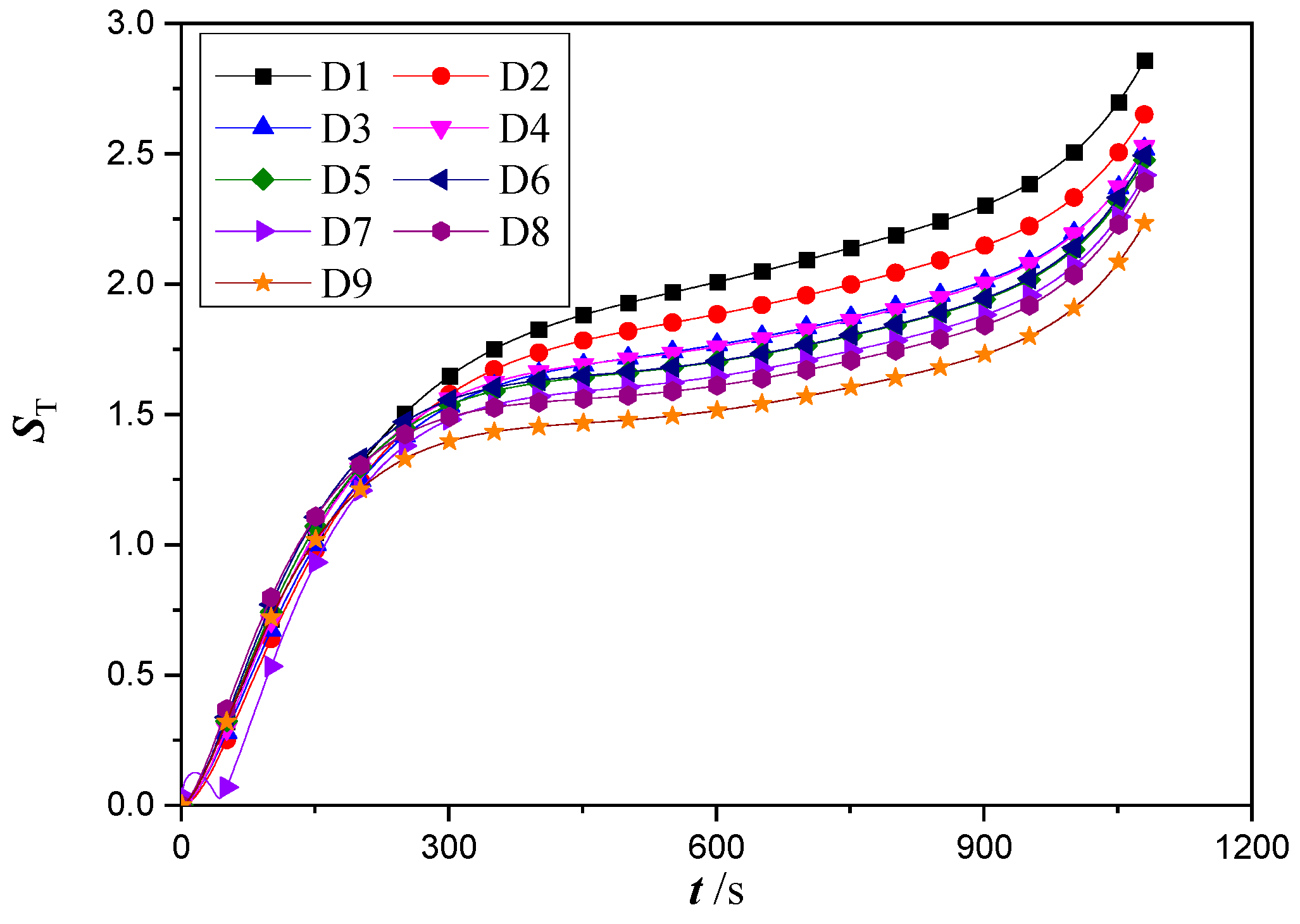
4.2. Thermal-Hydraulic Performance in Different Designs
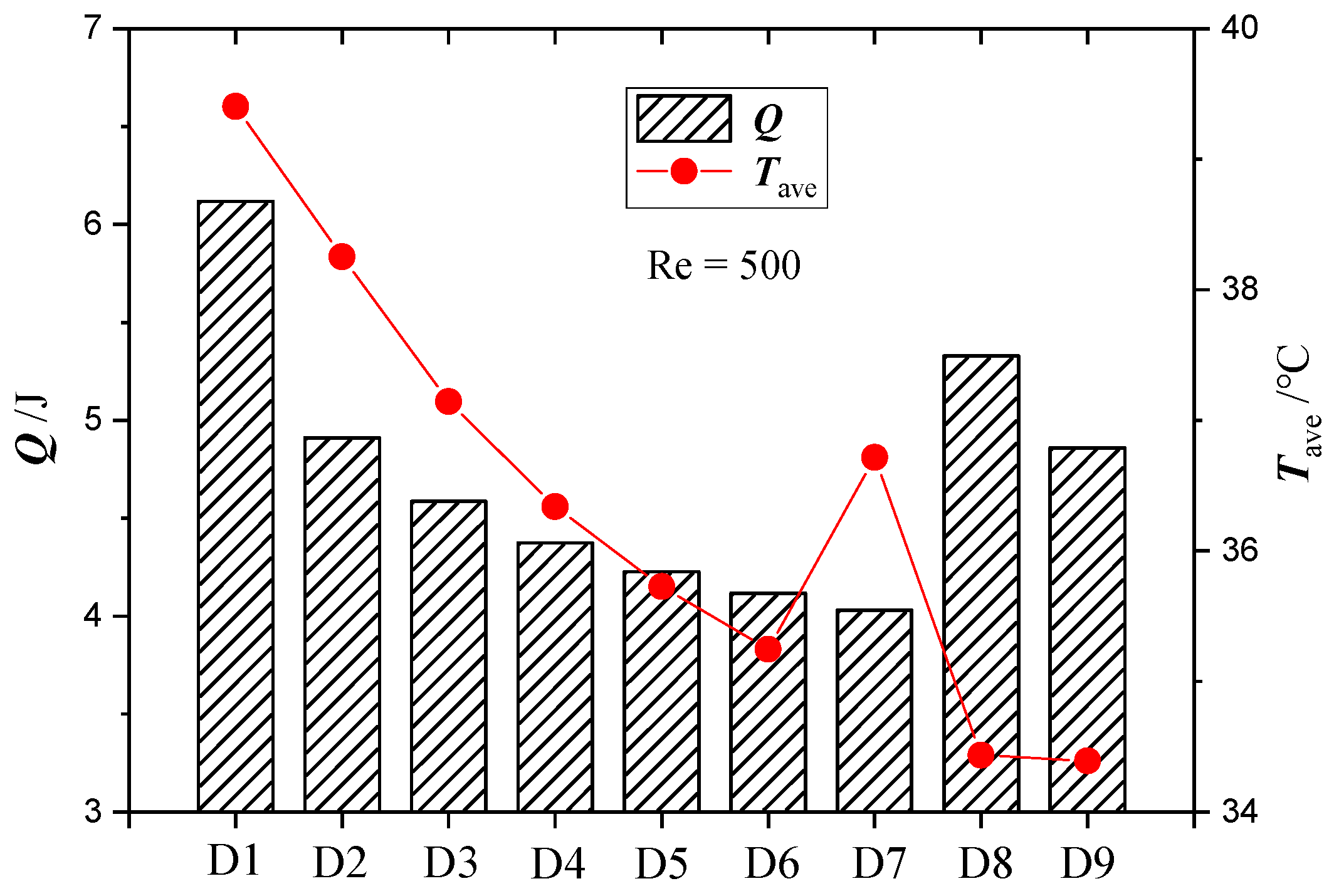
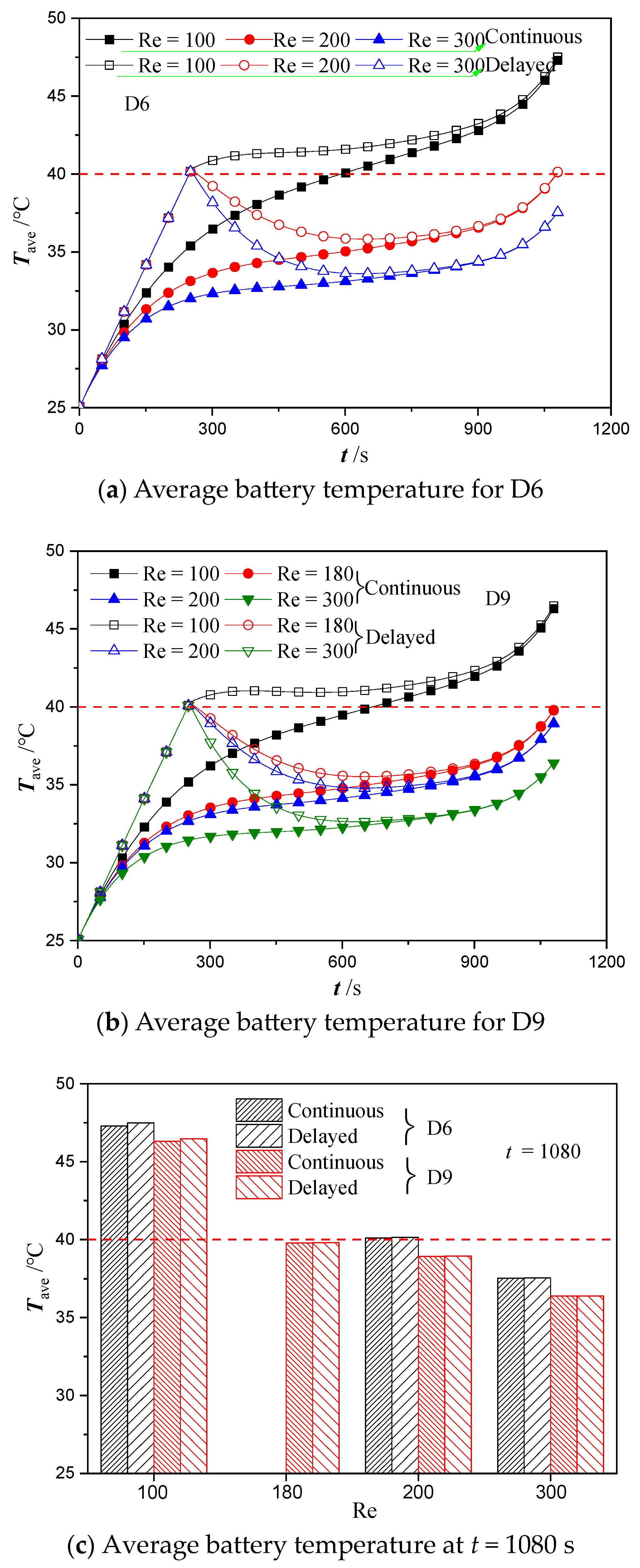
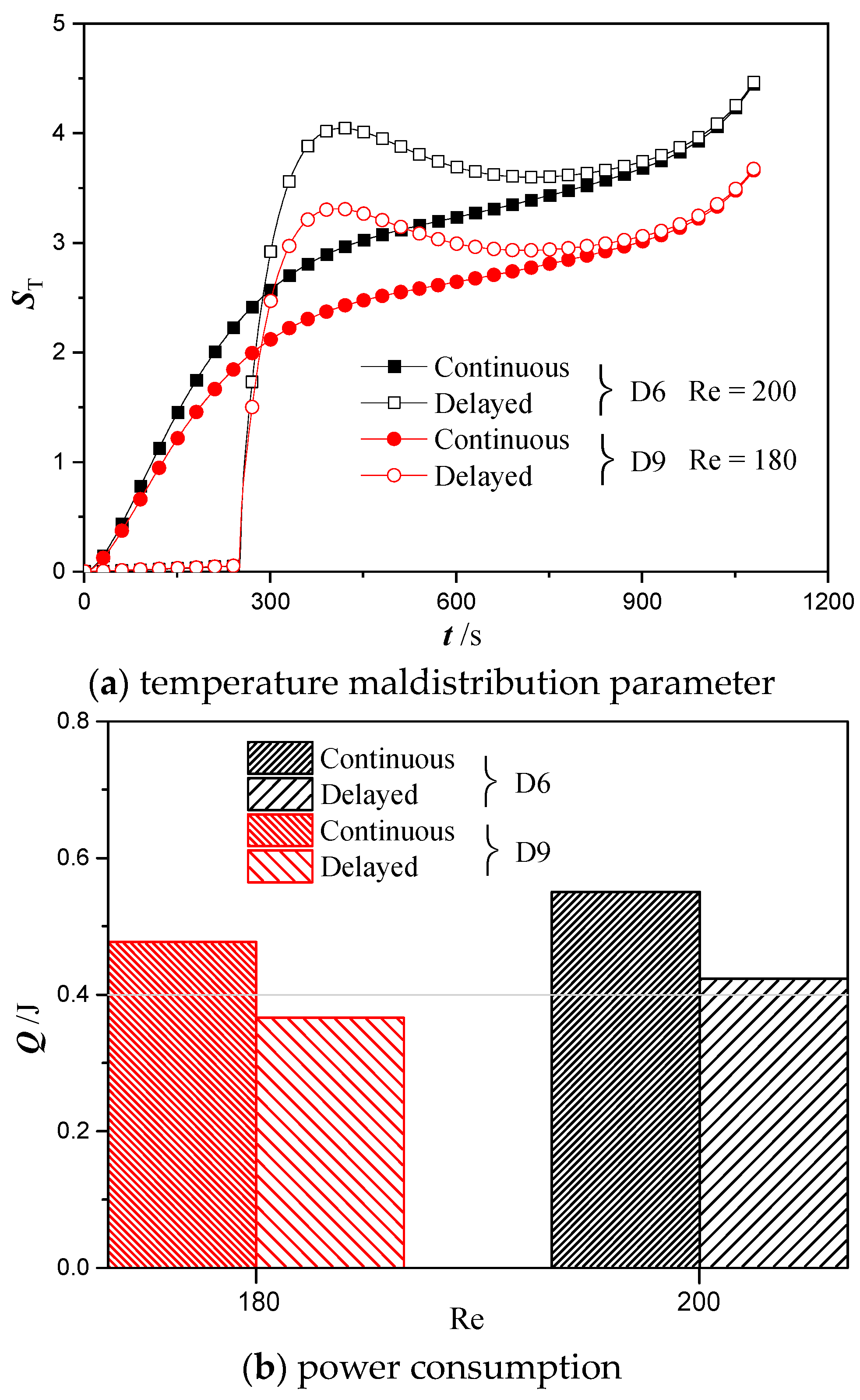
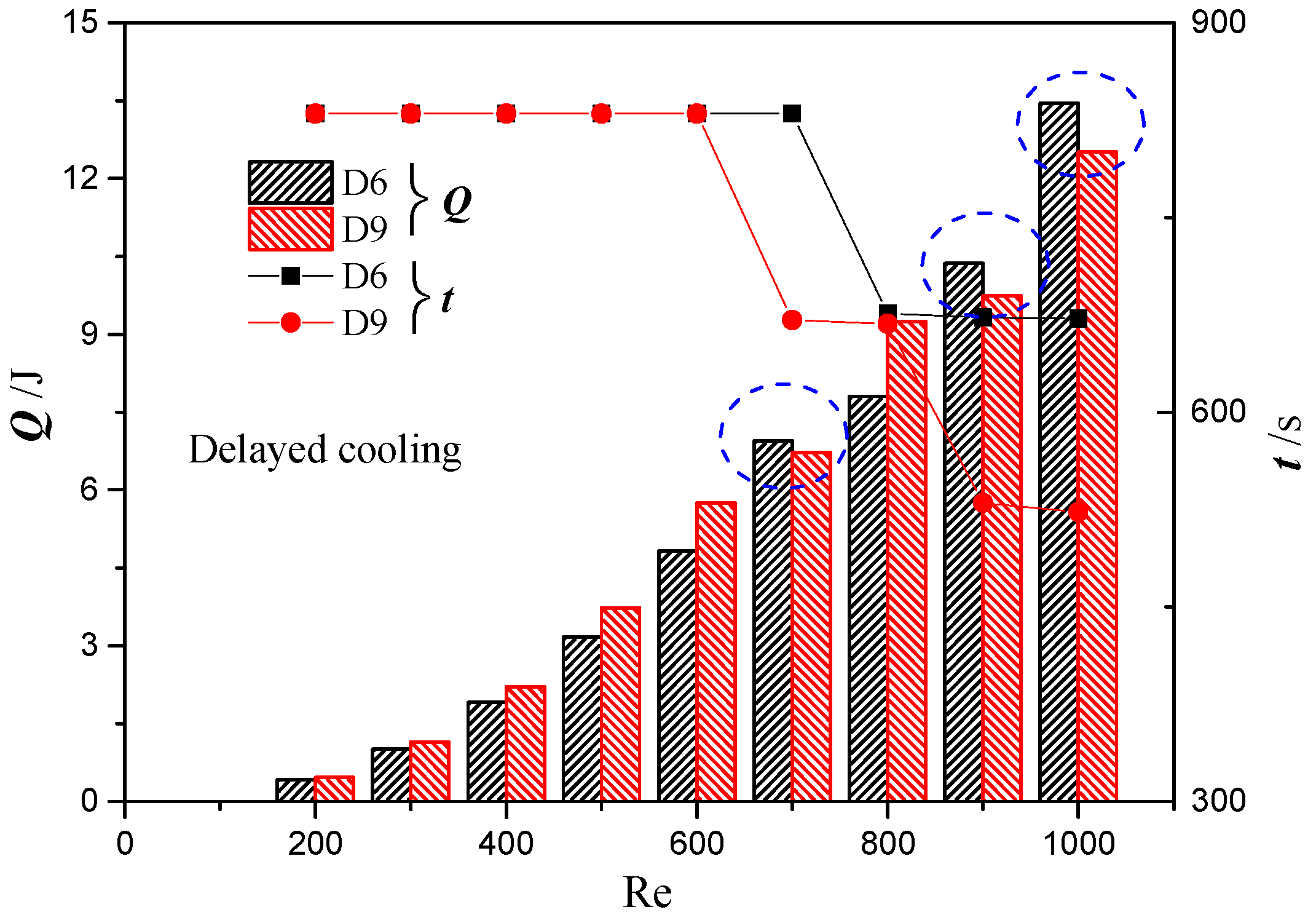
4.3. Performance Comparison under Different Cooling Strategies
5. Conclusions
- (1)
- The best thermal performance was obtained at a channel number of eight in the Z-type parallel channel cold plates. Cross-linked channel cold plates show a significantly better cooling performance than Z-type parallel channel cold plates at the price of a higher power consumption.
- (2)
- The operating temperature of a battery can be maintained within a safe range at Re = 200 and 180 for designs D6 and D9, respectively, under both continuous and delayed cooling schemes. At these operating conditions, design D9 requires 13% less pumping power and provides a better temperature uniformity than design D6. Therefore, design D9 has the best thermo-hydraulic performance than the rest of the designs.
- (3)
- Under the premise that the battery temperature is kept within the safe range, the delayed cooling strategy can greatly reduce the power consumption by shortening the duration of liquid cooling. In particular, the power consumption of design D9 can be decreased by 23% after switching from the continuous cooling scheme to the delayed cooling scheme.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| Latin symbols | |
| b | inlet and outlet channel width, mm |
| c, c1 | fin space of longitudinal channel, mm |
| cp | heat capacity, J·K−1·kg−1 |
| d | fin thickness of longitudinal channel, mm |
| e | fin thickness of transverse channel, mm |
| f | fin space of transverse channel, W·m−2·K−1 |
| p | pressure, Pa |
| Q | power consumption, J |
| Qgen | heat generation rate, W·m−3 |
| R | convection thermal resistance, °C·W−1 |
| ST | temperature maldistribution parameter, |
| t | time, s |
| T | temperature, °C |
| v | velocity, m·s−1 |
| Greek symbols | |
| ρ | density, kg·m−3 |
| k | thermal conductivity, W·m−1·K−1 |
| μ | dynamic viscosity, kg·m−1·s−1 |
| Subscripts | |
| ave | average |
| b | battery |
| j | serial number |
| l | liquid |
| w | wall |
References
- Feng, X.; Zheng, S.; Ren, D.; He, X.; Wang, L.; Cui, H.; Liu, X.; Jin, C.; Zhang, F.; Xu, C.; et al. Investigating the thermal runaway mechanisms of lithium-ion batteries based on thermal analysis database. Appl. Energy 2019, 246, 53–64. [Google Scholar] [CrossRef]
- Chen, J.; Kang, S.; E, J.; Huang, Z.; Wei, K.; Zhang, B.; Zhu, H.; Deng, Y.; Zhang, F.; Liao, G. Effects of different phase change material thermal management strategies on the cooling performance of the power lithium ion batteries: A review. J. Power Sources 2019, 442, 227228. [Google Scholar] [CrossRef]
- Zheng, C. Examining the Benefits of Using Boron Compounds in Lithium Batteries: A Comprehensive Review of Literature. Batteries 2022, 8, 187. [Google Scholar] [CrossRef]
- Kim, J.; Oh, J.; Lee, H. Review on battery thermal management system for electric vehicles. J. Appl. Therm. Eng. 2019, 149, 192–212. [Google Scholar] [CrossRef]
- Pesaran, A.A. Battery thermal models for hybrid vehicle simulations. J. Power Sources 2002, 110, 377–382. [Google Scholar] [CrossRef]
- Zhang, L.; Zhao, P.; Xu, M.; Wang, X. Computational identification of the safety regime of Li-ion battery thermal runaway. Appl. Energy 2020, 261, 114440. [Google Scholar] [CrossRef]
- Chen, K.; Song, M.; Wei, W.; Wang, S. Design of the structure of battery pack in parallel air-cooled battery thermal management system for cooling efficiency improvement. Int. J. Heat Mass Transf. 2019, 132, 309–321. [Google Scholar] [CrossRef]
- Chen, W.; Hou, S.; Shi, J.; Han, P.; Liu, B.; Wu, B.; Lin, X. Numerical Analysis of Novel Air-Based Li-Ion Battery Thermal Management. Batteries 2022, 8, 128. [Google Scholar] [CrossRef]
- Liu, Y.; Zhang, J. Self-adapting J-type air-based battery thermal management system via model predictive control. Appl. Energy 2020, 263, 114640. [Google Scholar] [CrossRef]
- Lloyd, R.; Akrami, M. A Critical Analysis of Helical and Linear Channel Liquid Cooling Designs for Lithium-Ion Battery Packs. Batteries 2022, 8, 236. [Google Scholar] [CrossRef]
- Park, S.; Jang, D.S.; Lee, D.; Hong, S.H.; Kim, Y. Simulation on cooling performance characteristics of a refrigerant-cooled active thermal management system for lithium ion batteries. Int. J. Heat Mass Transf. 2019, 135, 131–141. [Google Scholar] [CrossRef]
- Lan, C.; Xu, J.; Qiao, Y.; Ma, Y. Thermal management for high power lithium-ion battery by minichannel aluminum tubes. Appl. Therm. Eng. 2016, 101, 284–292. [Google Scholar] [CrossRef]
- Xiong, M.; Wang, N.; Li, W.; Garg, A.; Gao, L. Study on the Heat Dissipation Performance of a Liquid Cooling Battery Pack with Different Pin-Fins. Batteries 2023, 9, 44. [Google Scholar] [CrossRef]
- Ping, P.; Zhang, Y.; Kong, D.; Du, J. Investigation on battery thermal management system combining phase changed material and liquid cooling considering non-uniform heat generation of battery. J. Energy Storage 2021, 36, 102448. [Google Scholar] [CrossRef]
- Yang, H.; Li, Y.; Zhang, L.; Zhu, Y. Thermal performance enhancement of phase change material heat sinks for thermal management of electronic devices under constant and intermittent power loads. Int. J. Heat Mass Transf. 2021, 181, 121899. [Google Scholar] [CrossRef]
- Sun, Z.; Fan, R.; Yan, F.; Zhou, T.; Zheng, N. Thermal management of the lithium-ion battery by the composite PCM-Fin structures. Int. J. Heat Mass Transf. 2019, 145, 118739. [Google Scholar] [CrossRef]
- Yang, H.; Li, Y.; Yang, Y.; Chen, D.; Zhu, Y. Effective thermal conductivity of high porosity open-cell metal foams. Int. J. Heat Mass Transf. 2020, 147, 118974. [Google Scholar] [CrossRef]
- He, L.; Tang, X.; Luo, Q.; Liao, Y.; Luo, X.; Liu, J.; Ma, L.; Dong, D.; Gan, Y.; Li, Y. Structure optimization of a heat pipe-cooling battery thermal management system based on fuzzy grey relational analysis. Int. J. Heat Mass Transf. 2022, 182, 121924. [Google Scholar] [CrossRef]
- Wu, W.; Yang, X.; Zhang, G.; Chen, K.; Wang, S. Experimental investigation on the thermal performance of heat pipe-assisted phase change material based battery thermal management system. Energy Convers. Manag. 2017, 138, 486–492. [Google Scholar] [CrossRef]
- Yang, H.; Li, M.; Wang, Z.; Ma, B. A compact and lightweight hybrid liquid cooling system coupling with Z-type cold plates and PCM composite for battery thermal management. Energy 2023, 263. [Google Scholar] [CrossRef]
- Widyantara, R.D.; Zulaikah, S.; Juangsa, F.B.; Budiman, B.A.; Aziz, M. Review on Battery Packing Design Strategies for Superior Thermal Management in Electric Vehicles. Batteries 2022, 8, 287. [Google Scholar] [CrossRef]
- Akbarzadeh, M.; Jaguemont, J.; Kalogiannis, T.; Karimi, D.; He, J.; Jin, L.; Xie, P.; Van Mierlo, J.; Berecibar, M. A novel liquid cooling plate concept for thermal management of lithium-ion batteries in electric vehicles. Energy Convers. Manag. 2021, 231, 113862. [Google Scholar] [CrossRef]
- Yue, Q.; He, C.; Wu, M.; Zhao, T. Advances in thermal management systems for next-generation power batteries. Int. J. Heat Mass Transf. 2021, 181, 121853. [Google Scholar] [CrossRef]
- Lin, J.; Liu, X.; Li, S.; Zhang, C.; Yang, S. A review on recent progress, challenges and perspective of battery thermal management system. Int. J. Heat Mass Transf. 2021, 167, 120834. [Google Scholar] [CrossRef]
- Mousavi, S.; Siavashi, M.; Zadehkabir, A. A new design for hybrid cooling of Li-ion battery pack utilizing PCM and mini channel cold plates. Appl. Therm. Eng. 2021, 197, 117398. [Google Scholar] [CrossRef]
- E, J.; Han, D.; Qiu, A.; Zhu, H.; Deng, Y.; Chen, J.; Zhao, X.; Zuo, W.; Wang, H.; Chen, J.; et al. Orthogonal experimental design of liquid-cooling structure on the cooling effect of a liquid-cooled battery thermal management system. Appl. Therm. Eng. 2018, 132, 508–520. [Google Scholar] [CrossRef]
- Huo, Y.; Rao, Z.; Liu, X.; Zhao, J. Investigation of power battery thermal management by using mini-channel cold plate. Energy Convers. Manag. 2015, 89, 387–395. [Google Scholar] [CrossRef]
- Qian, Z.; Li, Y.; Rao, Z. Thermal performance of lithium-ion battery thermal management system by using mini-channel cooling. Energy Convers. Manag. 2016, 126, 622–631. [Google Scholar] [CrossRef]
- Li, X.; Zhou, D.; Zhang, G.; Wang, C.; Lin, R.; Zhong, Z. Experimental investigation of the thermal performance of silicon cold plate for battery thermal management system. Appl. Therm. Eng. 2019, 155, 331–340. [Google Scholar] [CrossRef]
- Deng, T.; Zhang, G.; Ran, Y. Study on thermal management of rectangular Li-ion battery with serpentine-channel cold plate. Int. J. Heat Mass Transf. 2018, 125, 143–152. [Google Scholar] [CrossRef]
- Kong, W.; Zhu, K.; Lu, X.; Jin, J.; Ni, M. Enhancement of lithium-ion battery thermal management with the divergent-shaped channel cold plate. J. Energy Storage 2021, 42, 103027. [Google Scholar] [CrossRef]
- Huang, Y.; Mei, P.; Lu, Y.; Huang, R.; Yu, X.; Chen, Z.; Roskilly, A.P. A novel approach for Lithium-ion battery thermal management with streamline shape mini channel cooling plates. Appl. Therm. Eng. 2019, 157, 113623. [Google Scholar] [CrossRef]
- Mo, X.; Zhi, H.; Xiao, Y.; Hua, H.; He, L. Topology optimization of cooling plates for battery thermal management. Int. J. Heat Mass Transf. 2021, 178, 121612. [Google Scholar] [CrossRef]
- Kalkan, O.; Celen, A.; Bakirci, K.; Dalkilic, A.S. Experimental investigation of thermal performance of novel cold plate design used in a Li-ion pouch-type battery. Appl. Therm. Eng. 2021, 191, 116885. [Google Scholar] [CrossRef]
- Amalesh, T.; Narasimhan, N.L. Introducing new designs of minichannel cold plates for the cooling of Lithium-ion batteries. J. Power Sources 2020, 479, 228775. [Google Scholar] [CrossRef]
- Chen, Y.; Chen, K.; Dong, Y.; Wu, X. Bidirectional symmetrical parallel mini-channel cold plate for energy efficient cooling of large battery packs. Energy 2021, 242, 122553. [Google Scholar] [CrossRef]
- Gungor, S.; Cetkin, E.; Lorente, S. Canopy-to-canopy liquid cooling for the thermal management of lithium-ion batteries, a constructal approach. Int. J. Heat Mass Transf. 2022, 182, 121918. [Google Scholar] [CrossRef]
- Sun, H.; Dixon, R. Development of cooling strategy for an air cooled lithium-ion battery pack. J. Power Sources 2014, 272, 404–414. [Google Scholar] [CrossRef]
- Guo, R.; Li, L. Heat dissipation analysis and optimization of lithium-ion batteries with a novel parallel-spiral serpentine channel liquid cooling plate. Int. J. Heat Mass Transf. 2022, 189, 122706. [Google Scholar] [CrossRef]
- Shen, X.; Cai, T.; He, C.; Yang, Y.; Chen, M. Thermal analysis of modified Z-shaped air-cooled battery thermal management system for electric vehicles. J. Energy Storage 2023, 58, 106356. [Google Scholar] [CrossRef]
- Faizan; Pati, S.; Randive, P. Effect of channel configurations on the thermal management of fast discharging Li-ion battery module with hybrid cooling. Energy 2023, 267, 126358. [Google Scholar] [CrossRef]
- Guo, Z.; Xu, Q.; Ni, M. A numerical study on the battery thermal management system with mini-channel cold plate considering battery aging effect. Appl. Therm. Eng. 2023, 219, 119564. [Google Scholar] [CrossRef]
- Chen, K.; Song, M.; Wei, W.; Wang, S. Structure optimization of parallel air-cooled battery thermal management system with U-type flow for cooling efficiency improvement. Energy 2018, 145, 603–613. [Google Scholar] [CrossRef]
- Lu, Z.; Yu, X.; Wei, L.; Qiu, Y.; Zhang, L.; Meng, X.; Jin, L. Parametric study of forced air cooling strategy for lithium-ion battery pack with staggered arrangement. Appl. Therm. Eng. 2018, 136, 28–40. [Google Scholar] [CrossRef]
- Liu, Y.; Zhang, J. Design a J-type air-based battery thermal management system through surrogate-based optimization. Appl. Energy 2019, 252, 113426. [Google Scholar] [CrossRef]
- Cao, J.; Feng, J.; Fang, X.; Ling, Z.; Zhang, Z. A delayed cooling system coupling composite phase change material and nano phase change material emulsion. Appl. Therm. Eng. 2021, 191, 116888. [Google Scholar] [CrossRef]
- Cao, J.; Ling, Z.; Fang, X.; Zhang, Z. Delayed liquid cooling strategy with phase change material to achieve high temperature uniformity of Li-ion battery under high-rate discharge. J. Power Sources 2020, 450, 227673. [Google Scholar] [CrossRef]
- Cao, W.; Zhao, C.; Wang, Y.; Dong, T.; Jiang, F. Thermal modeling of full-size-scale cylindrical battery pack cooled by channeled liquid flow. Int. J. Heat Mass Transf. 2019, 138, 1178–1187. [Google Scholar] [CrossRef]
- Pan, Y.-W.; Hua, Y.; Zhou, S.; He, R.; Zhang, Y.; Yang, S.; Liu, X.; Lian, Y.; Yan, X.; Wu, B. A computational multi-node electro-thermal model for large prismatic lithium-ion batteries. J. Power Sources 2020, 459, 228070. [Google Scholar] [CrossRef]
- Li, T. Study on thermal effects of lithium-ion battery in electric vehicle and battery package dissipation structural optimization. Master’s Thesis, Chongqing University, Chongqing, China, 2013. [Google Scholar]

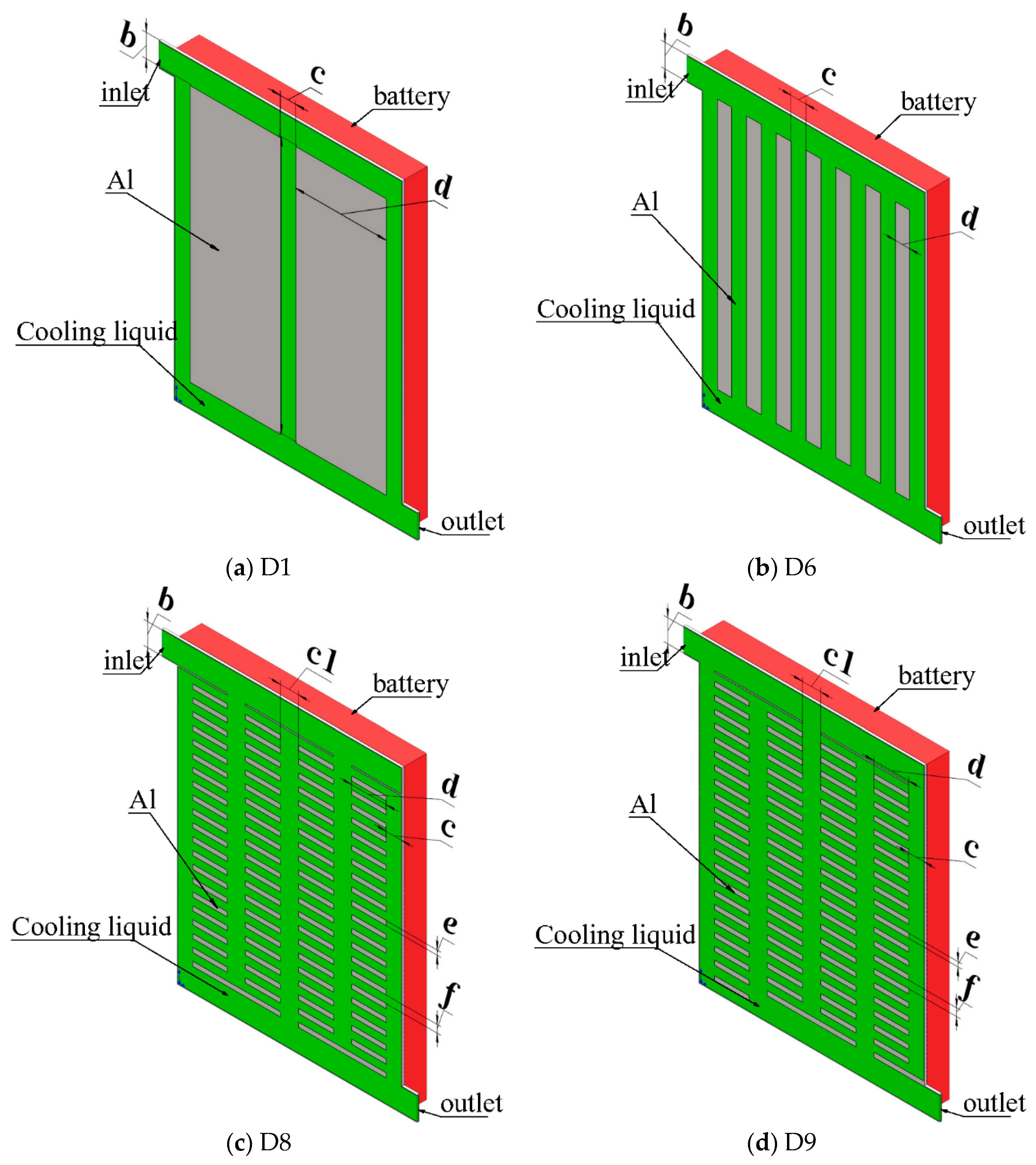
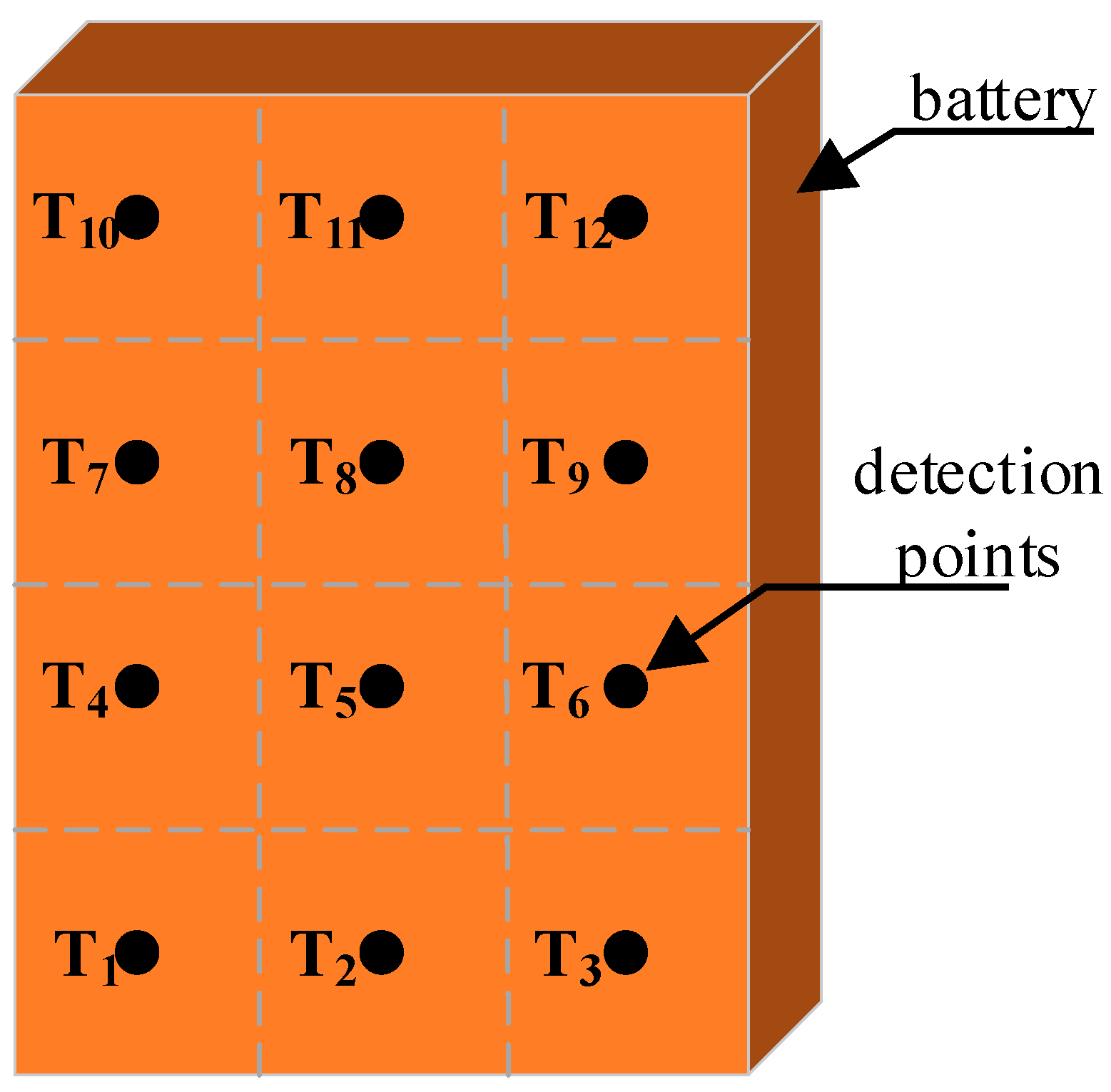
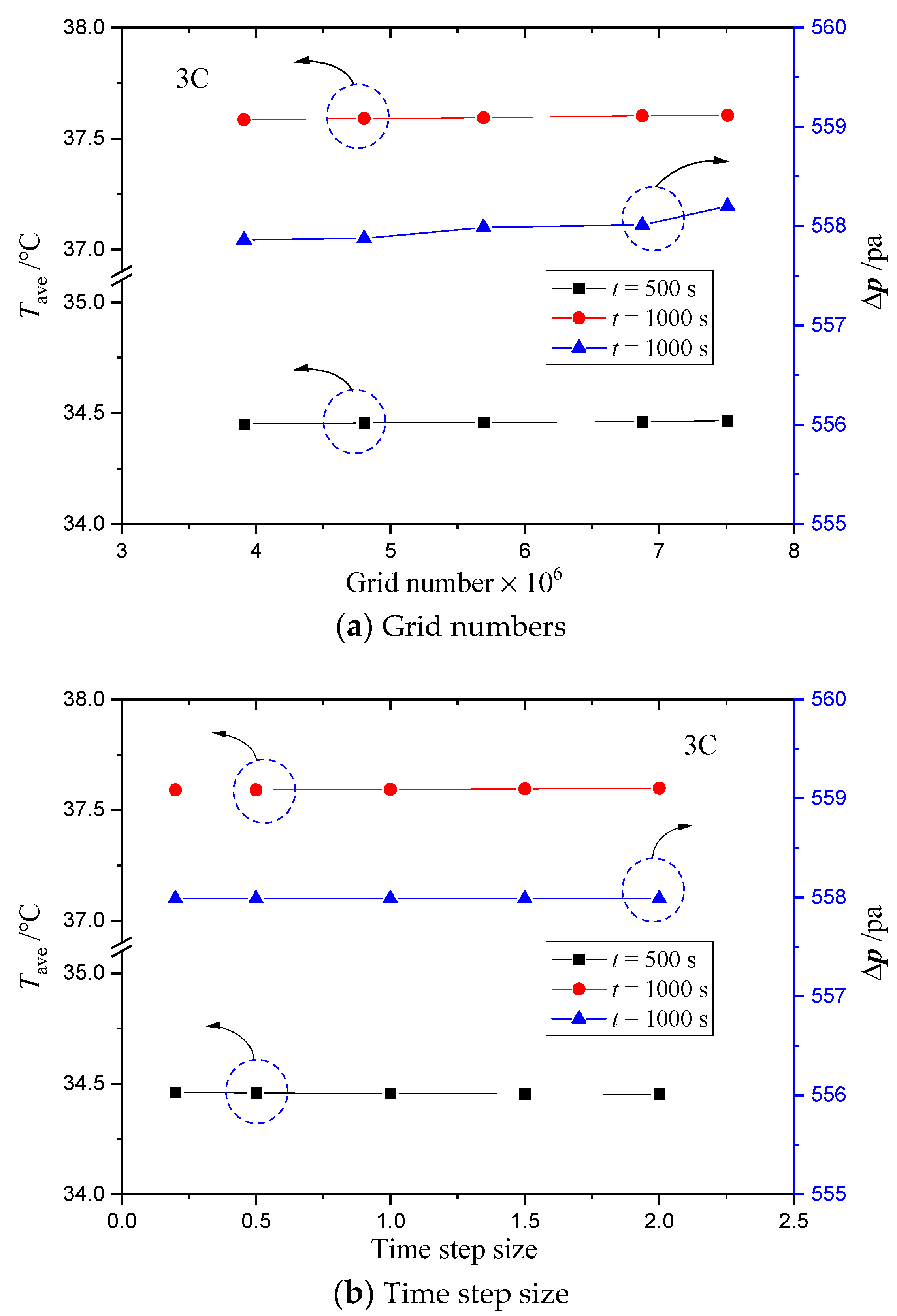


| Parameters | Battery | Aluminum | Coolant (40% Ethanol Solution) |
|---|---|---|---|
| Nominal Capacity (Ah) | 45 | ||
| Nominal Voltage (V) | 3.2 | ||
| Charge Cut-off Voltage (V) | 3.6 | ||
| Discharge Cut-off Voltage (V) | 2.5 | ||
| Maximum charging current (A) | ≤3 | ||
| Maximum discharge current (A) | ≤4 | ||
| Internal resistance (mΩ) | ≤10 | ||
| Size (mm) | 150 × 200 × 30 | ||
| Density ρ (kg·m−3) | 2090 | 2719 | 1055.39 |
| Heat capacity cp (J·K−1·kg−1) | 1014.4 | 871 | 3502 |
| Thermal conductivity k (W·m−1·K−1) | kx = 1.696 ky = kz= 29.94 | 202.4 | 0.412 |
| Dynamic viscosity μ (kg·m−1·s−1) | 0.00226 |
| Discharge Rates | A1 | A2 | A3 | A4 | A5 | A6 | A7 |
|---|---|---|---|---|---|---|---|
| 1C | 4.9132 × 10−16 | −3.7742 × 10−12 | 1.0679 × 10−8 | −1.3417 × 10−5 | 0.0076 | −2.2208 | 17,151.7482 |
| 2C | 1.2578 × 10−13 | −4.8310 × 10−10 | 6.8347 × 10−7 | −4.2934 × 10−4 | 0.1216 | −17.7630 | 66,623.3365 |
| 3C | 3.2235 × 10−12 | −8.2542 × 10−9 | 7.7851 × 10−6 | −3.2303 × 10−3 | 0.6157 | −59.9607 | 148,414.7651 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, H.; Wang, Z.; Li, M.; Ren, F.; Ma, B. Numerical Study on Cross-Linked Cold Plate Design for Thermal Management of High-Power Lithium-Ion Battery. Batteries 2023, 9, 220. https://doi.org/10.3390/batteries9040220
Yang H, Wang Z, Li M, Ren F, Ma B. Numerical Study on Cross-Linked Cold Plate Design for Thermal Management of High-Power Lithium-Ion Battery. Batteries. 2023; 9(4):220. https://doi.org/10.3390/batteries9040220
Chicago/Turabian StyleYang, Huizhu, Zehui Wang, Mingxuan Li, Fengsheng Ren, and Binjian Ma. 2023. "Numerical Study on Cross-Linked Cold Plate Design for Thermal Management of High-Power Lithium-Ion Battery" Batteries 9, no. 4: 220. https://doi.org/10.3390/batteries9040220
APA StyleYang, H., Wang, Z., Li, M., Ren, F., & Ma, B. (2023). Numerical Study on Cross-Linked Cold Plate Design for Thermal Management of High-Power Lithium-Ion Battery. Batteries, 9(4), 220. https://doi.org/10.3390/batteries9040220








