An Overview of the Design and Optimized Operation of Vanadium Redox Flow Batteries for Durations in the Range of 4–24 Hours
Abstract
1. Introduction
2. Modeling
3. Materials
3.1. Membranes
3.2. Electrode Development
3.3. Bipolar Plate Development
3.4. Electrolyte Development
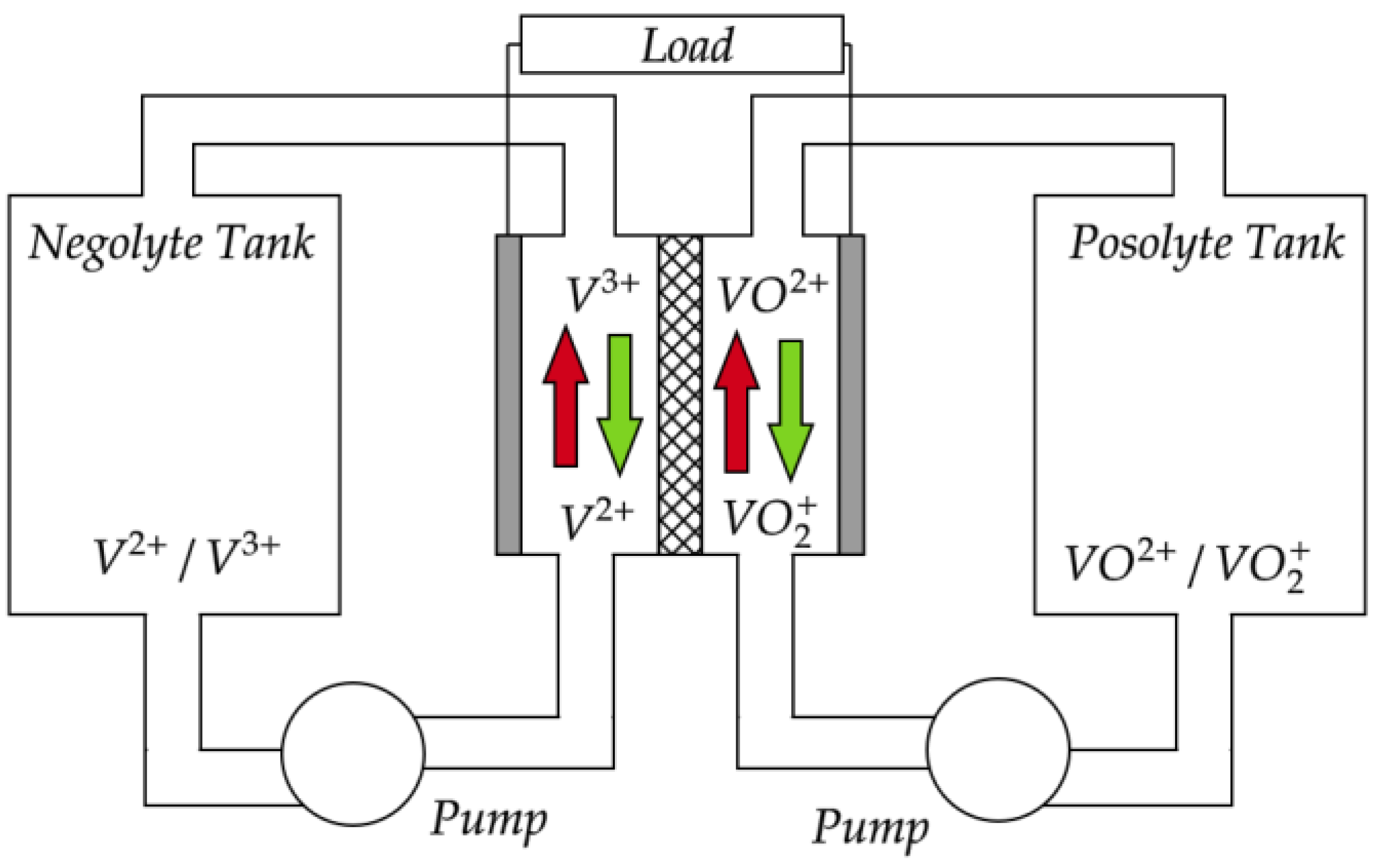
4. State of Charge, Crossover and State of Health Estimation
4.1. SOC Estimation
4.2. Crossover
4.3. SOH Estimation
5. Battery Management System
5.1. Flow Optimization
5.2. Operation Strategy
6. Stack Design
6.1. Stack Sizing and Architecture
6.2. Flow Field Design
6.3. Electrode Design for Various Flow Fields
7. Environmental Impact
8. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- U.S. Department of Energy Office of Clean Energy Demonstrations. Biden-Harris Administration Announces Nearly $350 Million for Long-Duration Energy Storage Demonstration Projects. 2022. Available online: https://www.energy.gov/articles/biden-harris-administration-announces-nearly-350-million-long-duration-energy-storage (accessed on 29 January 2023).
- Sun, C.; Chen, J.; Zhang, H.; Han, C.; Luo, Q. Hybrid inorganic-organic proton-conducting membranes based on SPEEK doped with WO3 nanoparticles for application in vanadium redox flow batteries. Electrochim. Acta 2019, 309, 311–325. [Google Scholar] [CrossRef]
- Sangwon, K. Vanadium Redox Flow Batteries: Electrochemical Engineering. In Energy Storage Devices; Demirkan, M., Adel, A., Eds.; IntechOpen: Rijeka, Croatia, 2019; Chapter 7. [Google Scholar]
- Huang, Z.; Mu, A.; Wu, L.; Yang, B.; Qian, Y.; Wang, J. Comprehensive Analysis of Critical Issues in All-Vanadium Redox Flow Battery. ACS Sustain. Chem. Eng. 2022, 10, 7786–7810. [Google Scholar] [CrossRef]
- Reed, D.; Thomsen, E.; Li, B.; Wang, W.; Nie, Z.; Koeppel, B.; Kizewski, J.; Sprenkle, V. Stack Developments in a kW Class All Vanadium Mixed Acid Redox Flow Battery at the Pacific Northwest National Laboratory. J. Electrochem. Soc. 2016, 163, A5211. [Google Scholar] [CrossRef]
- Viswanathan, V.; Crawford, A.; Stephenson, D.; Kim, S.; Wei, W.; Bin, L.; Coffey, G.; Thomsen, E.; Gordon, G.; Balducci, P.; et al. Cost and performance model for redox flow batteries. J. Power Sources 2014, 247, 1040–1051. [Google Scholar] [CrossRef]
- Crawford, A.; Viswanathan, V.; Stephenson, D.; Wang, W.; Thomsen, E.; Reed, D.; Li, B.; Balducci, P.; Kintner-Meyer, M.; Sprenkle, V. Comparative analysis for various redox flow batteries chemistries using a cost performance model. J. Power Sources 2015, 293, 388–399. [Google Scholar] [CrossRef]
- Ma, R.X.; Seltzer, B.P.; Gong, K.; Shuang, G.; Yushan, Y. A General, Analytical Model for Flow Battery Costing and Design. J. Electrochem. Soc. 2018, 165, A2209. [Google Scholar] [CrossRef]
- Viswanathan, V.; Mongird, K.; Franks, R.; Li, X.; Sprenkle, V. Grid Energy Storage Technology Cost and Performance Assessment; Pacific Northwest National Laboratory: Richland, WA, USA, 2022.
- Zhang, H.; Sun, C.; Ge, M. Review of the Research Status of Cost-Effective Zinc–ndash;Iron Redox Flow Batteries. Batteries 2022, 8, 202. [Google Scholar] [CrossRef]
- Iwakiri, I.; Antunes, T.; Almeida, H.; Sousa, J.P.; Figueira, R.B.; Mendes, A. Redox Flow Batteries: Materials, Design and Prospects. Energies 2021, 14, 5643. [Google Scholar] [CrossRef]
- Petek, T.J.; Hoyt, N.C.; Savinell, R.F.; Wainright, J.S. Slurry electrodes for iron plating in an all-iron flow battery. J. Power Sources 2015, 294, 620–626. [Google Scholar] [CrossRef]
- Duduta, M.; Ho, B.; Wood, V.C.; Limthongkul, P.; Brunini, V.E.; Carter, W.C.; Chiang, Y.-M. Semi-Solid Lithium Rechargeable Flow Battery. Adv. Energy Mater. 2011, 1, 511–516. [Google Scholar] [CrossRef]
- Qi, Z.; Liu, A.; Koenig, G. Carbon-free Solid Dispersion LiCoO2 Redox Couple Characterization and Electrochemical Evaluation for All Solid Dispersion Redox Flow Batteries. Electrochim. Acta 2017, 228, 91–99. [Google Scholar] [CrossRef]
- Cheng, Y.; Wang, X.; Huang, S.; Samarakoon, W.; Xi, S.; Ji, Y.; Zhang, F.; Du, Y.; Feng, Z.; Adams, S.; et al. Redox Targeting-Based Vanadium Redox-Flow Battery. ACS Energy Lett. 2019, 4, 3028–3035. [Google Scholar] [CrossRef]
- Yan, R.; Wang, Q. Redox-Targeting-Based Flow Batteries for Large-Scale Energy Storage. Adv. Mater. 2018, 30, e1802406. [Google Scholar] [CrossRef] [PubMed]
- Li, Y.; Van Nguyen, T.A. Solid/Liquid High-Energy-Density Storage Concept for Redox Flow Batteries and Its Demonstration in an H2-V System. J. Electrochem. Soc. 2022, 169, 110509. [Google Scholar] [CrossRef]
- Puleston, T.; Clemente, A.; Costa-Castelló, R.; Serra, M. Modelling and Estimation of Vanadium Redox Flow Batteries: A Review. Batteries 2022, 8, 121. [Google Scholar] [CrossRef]
- Clemente, A.; Costa-Castelló, R. Redox Flow Batteries: A Literature Review Oriented to Automatic Control. Energies 2020, 13, 4514. [Google Scholar] [CrossRef]
- Vynnycky, M. Analysis of a model for the operation of a vanadium redox battery. Energy 2011, 36, 2242–2256. [Google Scholar] [CrossRef]
- Chen, Y.; Xu, Z.; Wang, C.; Bao, J.; Koeppel, B.; Yan, L.; Gao, P.; Wang, W. Analytical modeling for redox flow battery design. J. Power Sources 2021, 482, 228817. [Google Scholar] [CrossRef]
- Li, Y.; Skyllas-Kazacos, M.; Bao, J. A dynamic plug flow reactor model for a vanadium redox flow battery cell. J. Power Sources 2016, 311, 57–67. [Google Scholar] [CrossRef]
- Xu, Q.; Zhao, T. Fundamental models for flow batteries. Prog. Energy Combust. Sci. 2015, 49, 40–58. [Google Scholar] [CrossRef]
- Poly Processing Solutions Simplified. Vertical Tanks. Available online: https://www.polyprocessing.com/tank-specifications/our-tank-offerings/vertical-tanks/ (accessed on 13 January 2023).
- Shi, Y.; Eze, C.; Xiong, B.; He, W.; Zhang, H.; Lim, T.M.; Ukil, A.; Zhao, J. Recent development of membrane for vanadium redox flow battery applications: A review. Appl. Energy 2019, 238, 202–224. [Google Scholar] [CrossRef]
- Thiam, B.G.; Vaudreuil, S. Review—Recent Membranes for Vanadium Redox Flow Batteries. J. Electrochem. Soc. 2021, 168, 070553. [Google Scholar] [CrossRef]
- Thiam, B.G.; El Magri, A.; Vaudreuil, S. An overview on the progress and development of modified sulfonated polyether ether ketone membranes for vanadium redox flow battery applications. High Perform. Polym. 2021, 34, 131–148. [Google Scholar] [CrossRef]
- Mazur, P.; Mrlik, J.; Pocedic, J.; Vrana, J.; Dundalek, J.; Kosek, J.; Bystron, T. Effect of graphite felt properties on the long-term durability of negative electrode in vanadium redox flow battery. J. Power Sources 2019, 414, 354–365. [Google Scholar] [CrossRef]
- He, Z.; Lv, Y.; Zhang, T.; Zhu, Y.; Dai, L.; Yao, S.; Zhu, W.; Wang, L. Electrode materials for vanadium redox flow batteries: Intrinsic treatment and introducing catalyst. Chem. Eng. J. 2022, 427, 131680. [Google Scholar] [CrossRef]
- Gencten, M.; Sahin, Y. A critical review on progress of the electrode materials of vanadium redox flow battery. Int. J. Energy Res. 2020, 44, 7903–7923. [Google Scholar] [CrossRef]
- Maleki, M.; El-Nagar, G.A.; Bernsmeier, D.; Schneider, J.; Roth, C. Fabrication of an efficient vanadium redox flow battery electrode using a free-standing carbon-loaded electropunk nanofibrous composite. Sci. Rep. 2020, 10, 11153. [Google Scholar] [CrossRef] [PubMed]
- Taylor, S.M.; Pătru, A.; Perego, D.; Fabbri, E.; Schmidt, T.J. Influence of Carbon Material Properties on Activity and Stability of the Negative Electrode in Vanadium Redox Flow Batteries: A Model Electrode Study. ACS Appl. Energy Mater. 2018, 1, 1166–1174. [Google Scholar] [CrossRef]
- Gautam, R.K.; Kumar, A. A review of bipolar plate materials and flow field designs in the all-vanadium redox flow battery. J. Energy Storage 2022, 48, 104003. [Google Scholar] [CrossRef]
- Satola, B. Review—Bipolar Plates for the Vanadium Redox Flow Battery. J. Electrochem. Soc. 2021, 168, 060503. [Google Scholar] [CrossRef]
- Kim, K.H.; Kim, B.; Lee, D. Development of carbon composite bipolar plate (BP) for vanadium redox flow battery (VRFB). Compos. Struct. 2014, 109, 253–259. [Google Scholar] [CrossRef]
- Murugesan, V.; Li, L.; Gordon, G.; Liu, J.; Zhang, H.; Yang, Z.; Hu, J.Z. Towards understanding the poor thermal stability of V5+ electrolyte solution in Vanadium Redox Flow Batteries. J. Power Sources 2011, 196, 3669–3672. [Google Scholar]
- Murugesan, V.; Zimin, N.; Zhang, X.; Gao, P.; Zhu, Z.; Huang, Q.; Yan, L.; Reed, D.; Wang, W. Accelerated design of vanadium redox flow battery electrolytes through tunable solvation chemistry. Cell Rep. Phys. Sci. 2021, 2, 100323. [Google Scholar] [CrossRef]
- Choi, C.; Kim, S.; Kim, R.; Choi, Y.; Kim, S.; Jung, H.-Y.; Yang, J.H.; Kim, H.-T. A review of vanadium electrolytes for vanadium redox flow batteries. Renew. Sustain. Energy Rev. 2017, 69, 263–274. [Google Scholar] [CrossRef]
- Roznyatovskaya, N.; Noack, J.; Mild, H.; Fühl, M.; Fischer, P.; Pinkwart, K.; Tübke, J.; Skyllas-Kazacos, M. Vanadium Electrolyte for All-Vanadium Redox-Flow Batteries: The Effect of the Counter Ion. Batteries 2019, 5, 13. [Google Scholar] [CrossRef]
- Truckmen, T.; Kuhn, P.; Ressel, S. A combined in situ monitoring approach for half-cell state of charge and state of health of vanadium redox flow batteries. Electrochim. Acta 2020, 362, 137174. [Google Scholar]
- Khaki, B.; Das, P. Fast and Simplified Algorithms for SoC and SoH Estimation of Vanadium Redox Flow Batteries. In Proceedings of the 2021 IEEE Green Technologies Conference (GreenTech), Denver, CO, USA, 7–9 April 2021. [Google Scholar]
- Song, Y.; Li, X.; Xiong, J.; Yang, L.; Pan, G.; Yan, C.; Tang, A. Electrolyte transfer mechanism and optimization strategy for vanadium flow batteries adopting a Nafion membrane. J. Power Sources 2020, 449, 227503. [Google Scholar] [CrossRef]
- Jienkulsawad, P.; Jirabovornwisut, T.; Chen, Y.-S.; Arpornwichanop, A. Improving the Performance of an All-Vanadium Redox Flow Battery under Imbalance Conditions: Online Dynamic Optimization Approach. ACS Sustain. Chem. Eng. 2020, 8, 13610–13622. [Google Scholar] [CrossRef]
- Vardner, J.T.; Ye, A.A.; Valdes, D.A.; West, A.C. Current-Driven Vanadium Crossover as a Function of SOC and SOD in the Vanadium Redox Flow Battery. J. Electrochem. Soc. 2020, 167, 080512. [Google Scholar] [CrossRef]
- Knehr, K.W.; Agar, E.; Dennison, C.R.; Kalidindi, A.R.; Kumbur, E.C. A Transient Vanadium Flow Battery Model Incorporating Vanadium Crossover and Water Transport through the Membrane. J. Electrochem. Soc. 2012, 159, A1446. [Google Scholar] [CrossRef]
- Agar, E.; Knehr, K.W.; Chen, D.; Hickner, M.A.; Kumbur, E.C. Species transport mechanisms governing capacity loss in vanadium flow batteries: Comparing Nafion and sulfonated Radel membranes. Electrochim. Acta 2013, 98, 66–74. [Google Scholar] [CrossRef]
- Haisch, T.; Ji, H.; Holtz, L.; Struckmann, T.; Weidlich, C. Half-Cell State of Charge Monitoring for Determination of Crossover in VRFB—Considerations and Results Concerning Crossover Direction and Amount. Membranes 2021, 11, 232. [Google Scholar] [CrossRef] [PubMed]
- Luo, Q.; Li, L.; Wang, W.; Nie, Z.; Wei, X.; Li, B.; Chen, B.; Yang, Z.; Sprenkle, V. Capacity Decay and Remediation of Nafion-based All-Vanadium Redox Flow Batteries. ChemSusChem 2013, 6, 268–274. [Google Scholar] [CrossRef]
- Oh, K.; Won, S.; Ju, H. A comparative study of species migration and diffusion mechanisms in all-vanadium redox flow batteries. Electrochim. Acta 2015, 181, 238–247. [Google Scholar] [CrossRef]
- Won, S.; Oh, K.; Ju, H. Numerical analysis of vanadium crossover effects in all-vanadium redox flow batteries. Electrochim. Acta 2015, 177, 310–320. [Google Scholar] [CrossRef]
- Park, J.H.; Park, J.J.; Park, O.O.; Yang, J.H. Capacity Decay Mitigation by Asymmetric Positive/Negative Electrolyte Volumes in Vanadium Redox Flow Batteries. ChemSusChem 2016, 9, 3181–3187. [Google Scholar] [CrossRef] [PubMed]
- Xie, W.; Darling, R.; Perry, M. Processing and Pretreatment Effects on Vanadium Transport in Nafion Membranes. J. Electrochem. Soc. 2016, 163, A5084. [Google Scholar] [CrossRef]
- Zhang, Y.; Ma, K.; Kuang, X.; Liu, L.; Sun, Y.; Xi, J. Real-Time Study of the Disequilibrium Transfer in Vanadium Flow Batteries at Different States of Charge via Refractive Index Detection. J. Phys. Chem. C 2018, 122, 28550–28555. [Google Scholar] [CrossRef]
- Boettcher, P.A.; Agar, E.; Dennison, C.R.; Kumbur, E.C. Modeling of Ion Crossover in Vanadium Redox Flow Batteries: A Computationally-Efficient Lumped Parameter Approach for Extended Cycling. J. Electrochem. Soc. 2016, 163, A5244. [Google Scholar] [CrossRef]
- Liu, Y.; Yu, L.; Loi, L.; Xi, J. Tailoring the vanadium/proton ratio of electrolytes to boost efficiency and stability of vanadium flow batteries over a wide temperature range. Appl. Energy 2021, 301, 117454. [Google Scholar] [CrossRef]
- Shin, J.; Jeong, B.; Chinannai, M.F.; Ju, H. Mitigation of water and electrolyte imbalance in all-vanadium redox flow batteries. Electrochim. Acta 2021, 390, 138858. [Google Scholar] [CrossRef]
- Shin, J.; Kim, C.; Jeong, B.; Vaz, N.; Ju, H. New operating strategy for all-vanadium redox flow batteries to mitigate electrolyte imbalance. J. Power Sources 2022, 526, 231144. [Google Scholar] [CrossRef]
- Darling, R.M.; Weber, A.Z.; Tucker, M.C.; Perry, M.L. The Influence of Electric Field on Crossover in Redox-Flow Batteries. J. Electrochem. Soc. 2016, 163, A5014. [Google Scholar] [CrossRef]
- Hao, L.; Wang, Y.; He, Y. Modeling of Ion Crossover in an All-Vanadium Redox Flow Battery with the Interfacial Effect at Membrane/Electrode Interfaces. J. Electrochem. Soc. 2019, 166, A1310. [Google Scholar] [CrossRef]
- Schafner, K.; Becker, M.; Turek, T. Capacity balancing for vanadium redox flow batteries through continuous and dynamic electrolyte overflow. J. Appl. Electrochem. 2021, 51, 1217–1228. [Google Scholar] [CrossRef]
- Liu, H.; Xu, W.; Yan, C.; Qiao, Y. Corrosion behavior of a positive graphite electrode in vanadium redox flow battery. Electrochim. Acta 2011, 56, 8783–8790. [Google Scholar] [CrossRef]
- Darling, R.M.; Shiau, H.-S.; Weber, A.Z.; Perry, M.L. The Relationship between Shunt Currents and Edge Corrosion in Flow Batteries. J. Electrochem. Soc. 2017, 164, E3081. [Google Scholar] [CrossRef]
- Liu, L.; Li, Z.; Xi, J.; Zhou, H.; Wu, Z.; Qiu, X. Rapid detection of the positive side reactions in vanadium flow batteries. Appl. Energy 2017, 185, 452–462. [Google Scholar] [CrossRef]
- Nourani, M.; Dennison, C.R.; Jin, X.; Liu, F.; Agar, E. Elucidating Effects of Faradaic Imbalance on Vanadium Redox Flow Battery Performance: Experimental Characterization. J. Electrochem. Soc. 2019, 166, A3844. [Google Scholar] [CrossRef]
- Yu, L.; Lin, F.; Xu, L.; Xi, J. A recast Nafion/graphene oxide composite membrane for advanced vanadium redox flow batteries. RSC Adv. 2016, 6, 3756–3763. [Google Scholar] [CrossRef]
- Zhang, H.; Zhang, H.; Li, X.; Mai, Z.; Wei, W. Silica modified nanofiltration membranes with improved selectivity for redox flow battery application. Energy Environ. Sci. 2012, 5, 6299–6303. [Google Scholar] [CrossRef]
- Kim, J.; Jeon, J.-D.; Kwak, S.-Y. Nafion-based composite membrane with a permselective layered silicate layer for vanadium redox flow battery. Electrochem. Commun. 2014, 38, 68–70. [Google Scholar] [CrossRef]
- Cecchetti, M.; Ebaugh, T.A.; Yu, H.; Bonville, L.; Gambaro, C.; Meda, L.; Maric, R.; Casalegno, A.; Zago, M. Design and Development of an Innovative Barrier Layer to Mitigate Crossover in Vanadium Redox Flow Batteries. J. Electrochem. Soc. 2020, 167, 130535. [Google Scholar] [CrossRef]
- Beyer, K.; Gross Austing, J.; Satola, B.; Di Nardo, T.; Zobel, M.; Agert, C. Electrolyte Imbalance Determination of a Vanadium Redox Flow Battery by Potential-Step Analysis of the Initial Charging. ChemSusChem 2020, 13, 2066–2071. [Google Scholar] [CrossRef] [PubMed]
- Di Noto, V.; Vezzu, K.; Crivellaro, G.; Pagot, G.; Sun, C.; Meda, L.; Rutkowska, I.A.; Kulesza, P.J.; Zawodzinski, T.A. A general electrochemical formalism for vanadium redox flow batteries. Electrochim. Acta 2022, 408, 139937. [Google Scholar] [CrossRef]
- Blanc, C.; Rufer, A. Optimization of the operating point of a vanadium redox flow battery. In Proceedings of the 2009 IEEE Energy Conversion Congress and Exposition, San Jose, CA, USA, 20–24 September 2009. [Google Scholar]
- Tang, A.; Bao, J.; Skyllas-Kazacos, M. Studies on pressure losses and flow rate optimization in vanadium redox flow battery. J. Power Sources 2014, 248, 154–162. [Google Scholar] [CrossRef]
- König, S.; Suriyah, M.; Leibfried, T. Innovative model-based flow rate optimization for vanadium redox flow batteries. J. Power Sources 2016, 333, 134–144. [Google Scholar] [CrossRef]
- Akter, M.P.; Li, Y.; Bao, J.; Skyllas-Kazacos, M.; Rahman, M.F. Optimal Charging of Vanadium Redox Flow Battery with Time-Varying Input Power. Batteries 2019, 5, 20. [Google Scholar] [CrossRef]
- Fialho, L.; Fartaria, T.; Narvarte, L.; Collares Pereira, M. Implementation and Validation of a Self-Consumption Maximization Energy Management Strategy in a Vanadium Redox Flow BIPV Demonstrator. Energies 2016, 9, 496. [Google Scholar] [CrossRef]
- Foles, A.; Fialho, L.; Collares Pereira, M.; Horta, P. An approach to implement photovoltaic self-consumption and ramp-rate control algorithm with a vanadium redox flow battery day-to-day forecast charging. Sustain. Energy Grids Netw. 2022, 30, 100626. [Google Scholar] [CrossRef]
- Parmeshwarappa, P.; Gundlapalli, R.; Jayanti, S. Power and Energy Rating Considerations in Integration of Flow Battery with Solar PV and Residential Load. Batteries 2021, 7, 62. [Google Scholar] [CrossRef]
- Khaki, B.; Das, P. Voltage loss and capacity fade reduction in vanadium redox battery by electrolyte flow control. Electrochim. Acta 2022, 405, 139842. [Google Scholar] [CrossRef]
- Moore, M.; Watson, J.; Zawodzinski, T.A.; Counce, R.; Kamath, H. A Step by Step Design Methodology for an All-Vanadium Redox-Flow Battery. Chem. Eng. Educ. 2012, 46, 239. [Google Scholar]
- Gibson, C.; Morrissey, K.G. A New Design Method for Vanadium Redox Batteries in Renewable Energy Systems. Inq. Univ. Ark. Undergrad. Res. J. 2015, 18, 6. [Google Scholar]
- Zhang, X.; Li, Y.; Skyllas-Kazacos, M.; Bao, J. Optimal Sizing of Vanadium Redox Flow Battery Systems for Residential Applications Based on Battery Electrochemical Characteristics. Energies 2016, 9, 857. [Google Scholar] [CrossRef]
- Ressel, S.; Laube, A.; Fischer, S.; Chica, A.; Flower, T.; Struckmann, T. Performance of a vanadium redox flow battery with tubular cell design. J. Power Sources 2017, 355, 199–205. [Google Scholar] [CrossRef]
- Houser, J.; Clement, J.; Pezeshki, A.; Mench, M.M. Influence of architecture and material properties on vanadium redox flow battery performance. J. Power Sources 2016, 302, 369–377. [Google Scholar] [CrossRef]
- Kumar, S.; Jayanti, S. Effect of flow field on the performance of an all-vanadium redox flow battery. J. Power Sources 2016, 307, 782–787. [Google Scholar] [CrossRef]
- Messaggi, M.; Canzi, P.; Mereu, R.; Baricci, A.; Inzoli, F.; Casalegno, A.; Zago, M. Analysis of flow field design on vanadium redox flow battery performance: Development of 3D computational fluid dynamic model and experimental validation. Appl. Energy 2018, 228, 1057–1070. [Google Scholar] [CrossRef]
- Aramendia, I.; Fernandez-Gamiz, U.; Martinez-San-Vicente, A.; Zulueta, E.; Lopez-Guede, J.M. Vanadium Redox Flow Batteries: A Review Oriented to Fluid-Dynamic Optimization. Energies 2021, 14, 176. [Google Scholar] [CrossRef]
- Huang, Z.; Mu, A.; Wu, L.; Wang, H. Vanadium redox flow batteries: Flow field design and flow rate optimization. J. Energy Storage 2022, 45, 103526. [Google Scholar] [CrossRef]
- Prumbohm, E.; Becker, M.; Flaischlen, S.; Wehlinger, G.D.; Turek, T. Flow field designs developed by comprehensive CFD model decrease system costs of vanadium redox-flow batteries. J. Flow Chem. 2021, 11, 461–481. [Google Scholar] [CrossRef]
- Jyothi Latha, T.; Jayanti, S. Ex-situ experimental studies on serpentine flow field design for redox flow battery systems. J. Power Sources 2014, 248, 140–146. [Google Scholar] [CrossRef]
- He, Q.; Yu, J.; Guo, Z.; Sun, J.; Zhao, S.; Zhao, T.; Ni, M. Modeling of vanadium redox flow battery and electrode optimization with different flow fields. e-Prime Adv. Electr. Eng. Electron. Energy 2021, 1, 100001. [Google Scholar] [CrossRef]
- Gundlapalli, R.; Kumar, S.; Jayanti, S. Stack Design Considerations for Vanadium Redox Flow Battery. INAE Lett. 2018, 3, 149–157. [Google Scholar] [CrossRef]
- Sujali, S.; Mohamed, M.R.; Oumer, A.N.; Ahmad, A.; Leung, P. Study on architecture design of electroactive sites on Vanadium Redox Flow Battery (V-RFB). E3S Web Conf. 2019, 80, 02004. [Google Scholar] [CrossRef]
- Ghimire, P.C.; Bhattarai, A.; Schweiss, R.; Scherer, G.G.; Wai, N.; Yan, Q. A comprehensive study of electrode compression effects in all vanadium redox flow batteries including locally resolved measurements. Appl. Energy 2018, 230, 974–982. [Google Scholar] [CrossRef]
- He, H.; Tian, S.; Tarroja, B.; Ogunseitan, O.A.; Samuelsen, S.; Schoenung, J.M. Flow battery production: Materials selection and environmental impact. J. Clean. Prod. 2020, 269, 121740. [Google Scholar] [CrossRef]

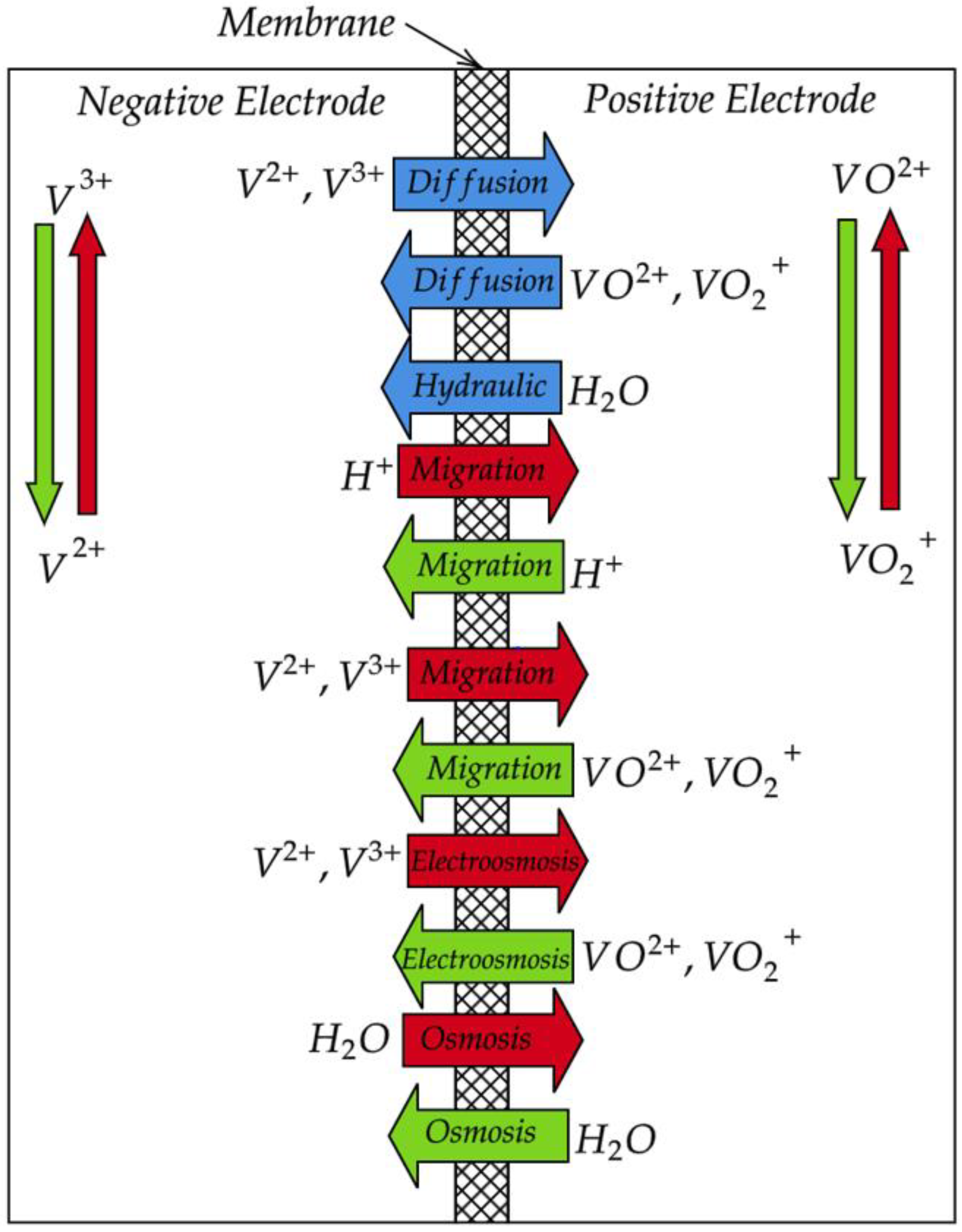
| Mode | Charge | Discharge | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| Species | V+2 | V+3 | V+4 | V+5 | V+2 | V+3 | V+4 | V+5 | Net V Transport after Each Cycle | |
| Mechanism | Reference | |||||||||
| Diffusion | [45] | + | - | + | - | |||||
| [46] | + | + | - | - | + | + | - | - | ||
| [46] S-PEEK | +(negligible) | +(negligible) | −(negligible) | ~0 | +(negligible) | +(negligible) | −(negligible) | ~0 | ||
| [49] | + | + | - | - | ||||||
| Hydraulic convection | [45] | - | - | + | +(negligible) | |||||
| [46] | +(negligible) | +(negligible) | +(negligible) | +(negligible) | + | + | + | + | ||
| [46] S-PEEK | - | - | - | - | + | + | + | |||
| [49] | ||||||||||
| Migration | [45] | - | - | + | + | |||||
| [46] | −(negligible) | −(negligible) | −(negligible) | ~0 | +(negligible) | +(negligible) | +(negligible) | ~0 | ||
| [46] S-PEEK | ~0 | ~0 | ~0 | ~0 | ~0 | ~0 | ~0 | |||
| [49] | - | - | - | - | ||||||
| Total transport of each species for half cycle | [45] | + | - | + | - | |||||
| [46] | + | + | - | - | + | + | - | + | ||
| [46] S-PEEK | - | - | - | - | + | + | + | + | ||
| [49] | +(negligible) | +(negligible) | - | - | ||||||
| Net V transport after each cycle | [45] | - | ||||||||
| [46] | + | |||||||||
| [46] S-PEEK | + | |||||||||
| [49] | + | |||||||||
| Reference | [45,46] | [47] | [4] | [50] | [54] | [56] | [58] | [59] | [60] |
| Model type | 2-D | 1-D | 3-D | 3-D | 0-D | 3-D | 1-D | 3-D | 0-D |
| Thermal coupling? | No | No | Yes | Yes | No | No | No | No | No |
| V species transport mechanisms | |||||||||
| Diffusion? | Yes | Yes | Yes | Yes | Yes | Yes | Yes | Yes | Yes |
| Migration? | Yes | Yes | Yes | Yes | Yes | No | Yes | Yes | Yes |
| Hydraulic convection of electrolyte due to delta P across membrane? | Yes | No | No | No | Yes | No | No | No | No |
| Electro-osmotic? | Yes | Yes | No | No | Yes | No | Yes | Yes | Yes |
| Water transport mechanisms | |||||||||
| Osmotic transport of water? | No | No | No | No | No | No | No | No | No |
| Transfer of water via electro-osmosis | No | No | No | No | No | Yes | No | No | Yes |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Viswanathan, V.V.; Crawford, A.J.; Thomsen, E.C.; Shamim, N.; Li, G.; Huang, Q.; Reed, D.M. An Overview of the Design and Optimized Operation of Vanadium Redox Flow Batteries for Durations in the Range of 4–24 Hours. Batteries 2023, 9, 221. https://doi.org/10.3390/batteries9040221
Viswanathan VV, Crawford AJ, Thomsen EC, Shamim N, Li G, Huang Q, Reed DM. An Overview of the Design and Optimized Operation of Vanadium Redox Flow Batteries for Durations in the Range of 4–24 Hours. Batteries. 2023; 9(4):221. https://doi.org/10.3390/batteries9040221
Chicago/Turabian StyleViswanathan, Vilayanur V., Alasdair J. Crawford, Edwin C. Thomsen, Nimat Shamim, Guosheng Li, Qian Huang, and David M. Reed. 2023. "An Overview of the Design and Optimized Operation of Vanadium Redox Flow Batteries for Durations in the Range of 4–24 Hours" Batteries 9, no. 4: 221. https://doi.org/10.3390/batteries9040221
APA StyleViswanathan, V. V., Crawford, A. J., Thomsen, E. C., Shamim, N., Li, G., Huang, Q., & Reed, D. M. (2023). An Overview of the Design and Optimized Operation of Vanadium Redox Flow Batteries for Durations in the Range of 4–24 Hours. Batteries, 9(4), 221. https://doi.org/10.3390/batteries9040221








