Investigation of the Effects of Charging Processes on Lithium-Ion Cells with SiC Anodes at Low Temperatures
Abstract
1. Introduction
1.1. Motivation
1.2. Lithium Plating as a Potential Aging Mechanism while Charging
1.3. Content of This Study
2. Experimental
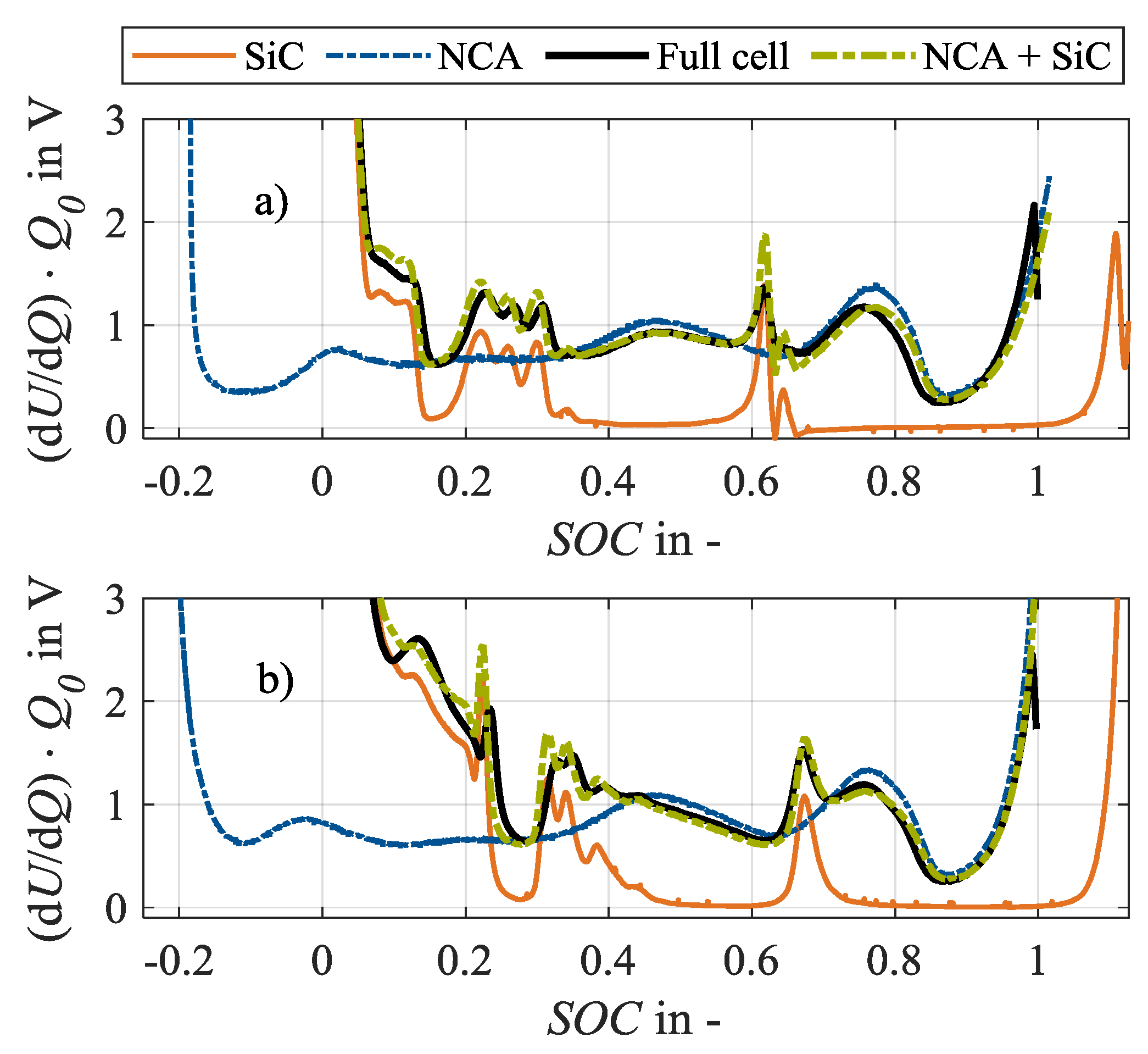
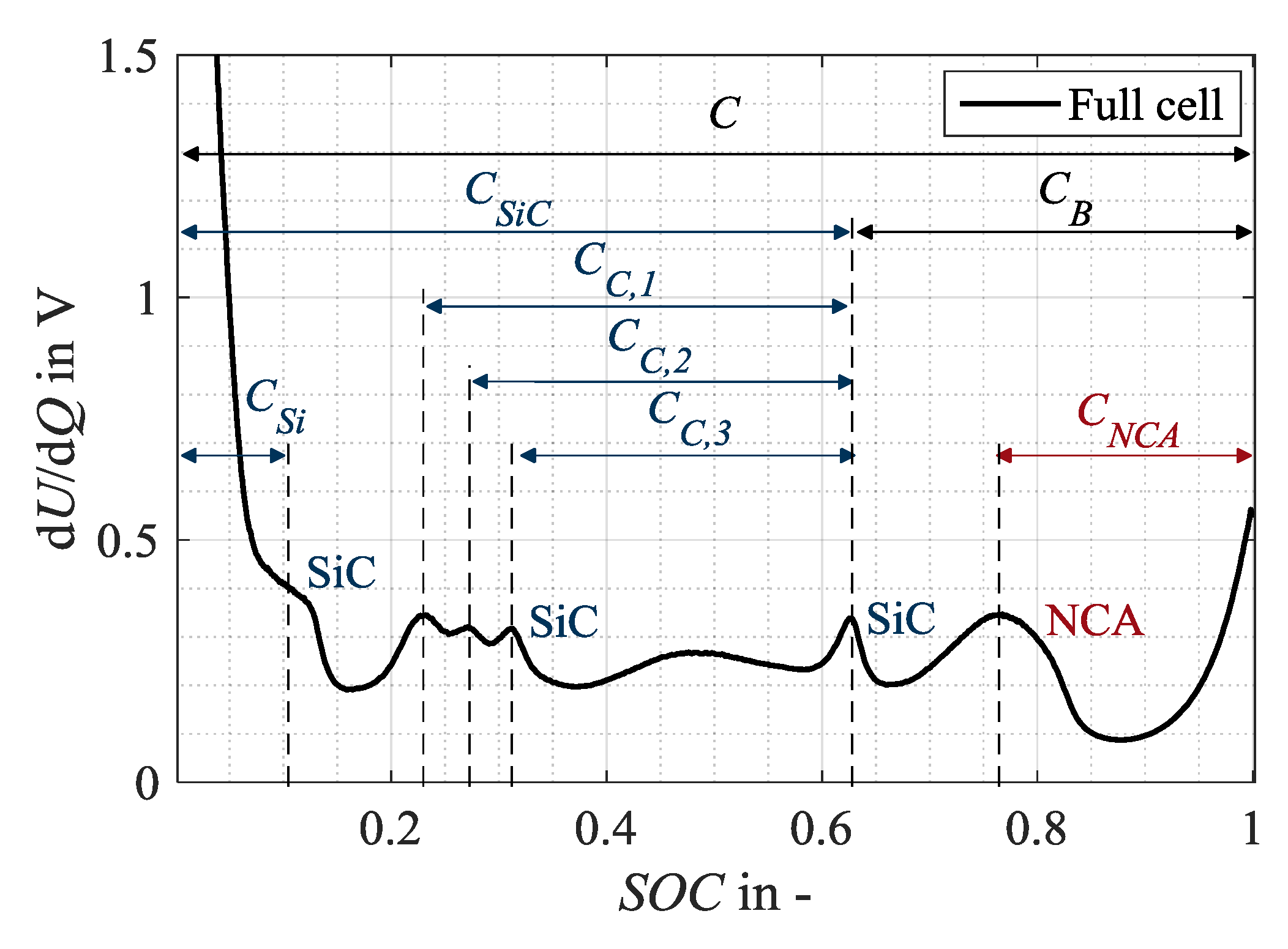
2.1. Initial Cell Characterization
2.2. Regular Check-Up Test
2.3. Definition and Implementation of the Test Series
3. Results and Discussion
3.1. Evolution of Cell Temperature during Charging
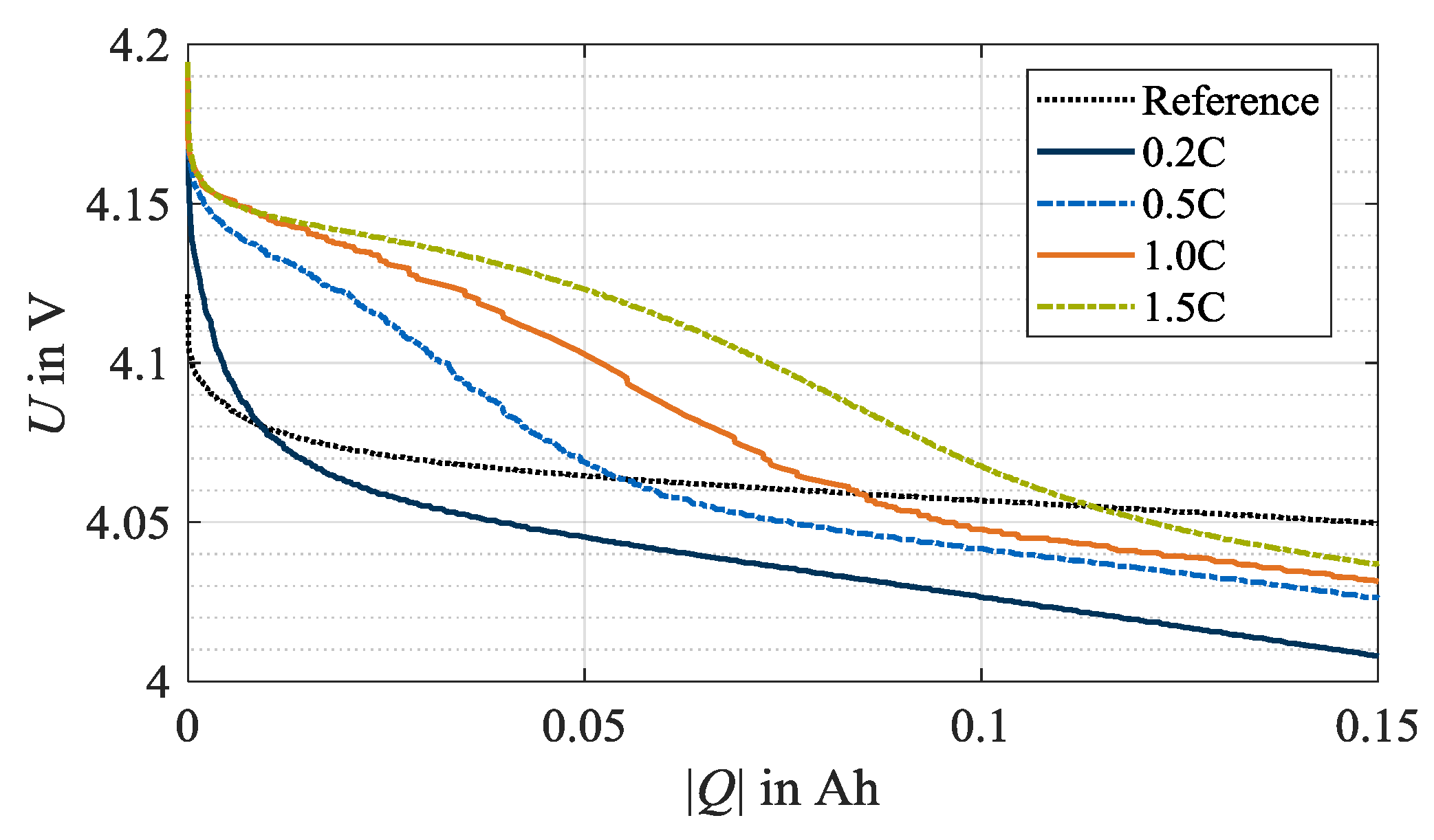
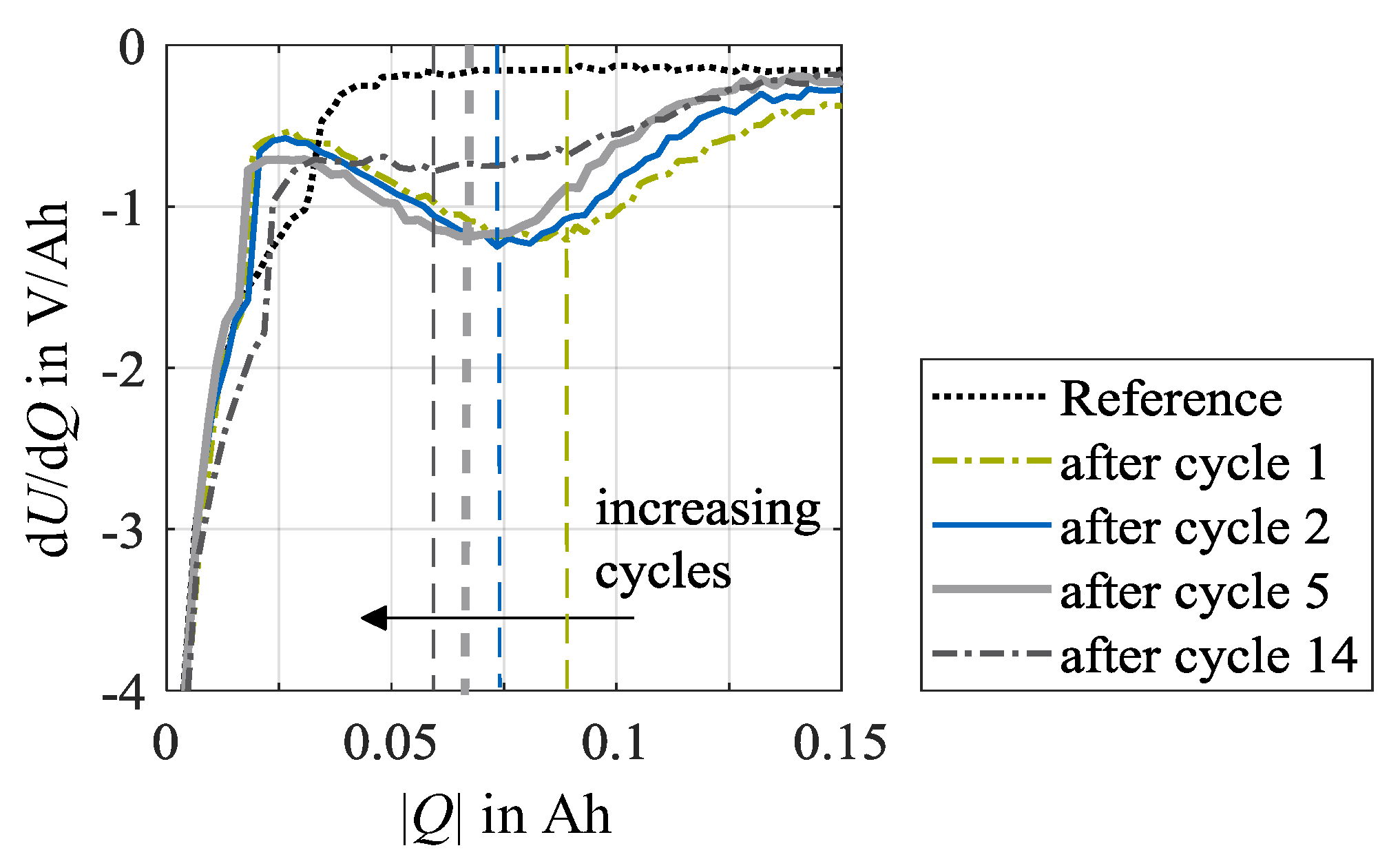
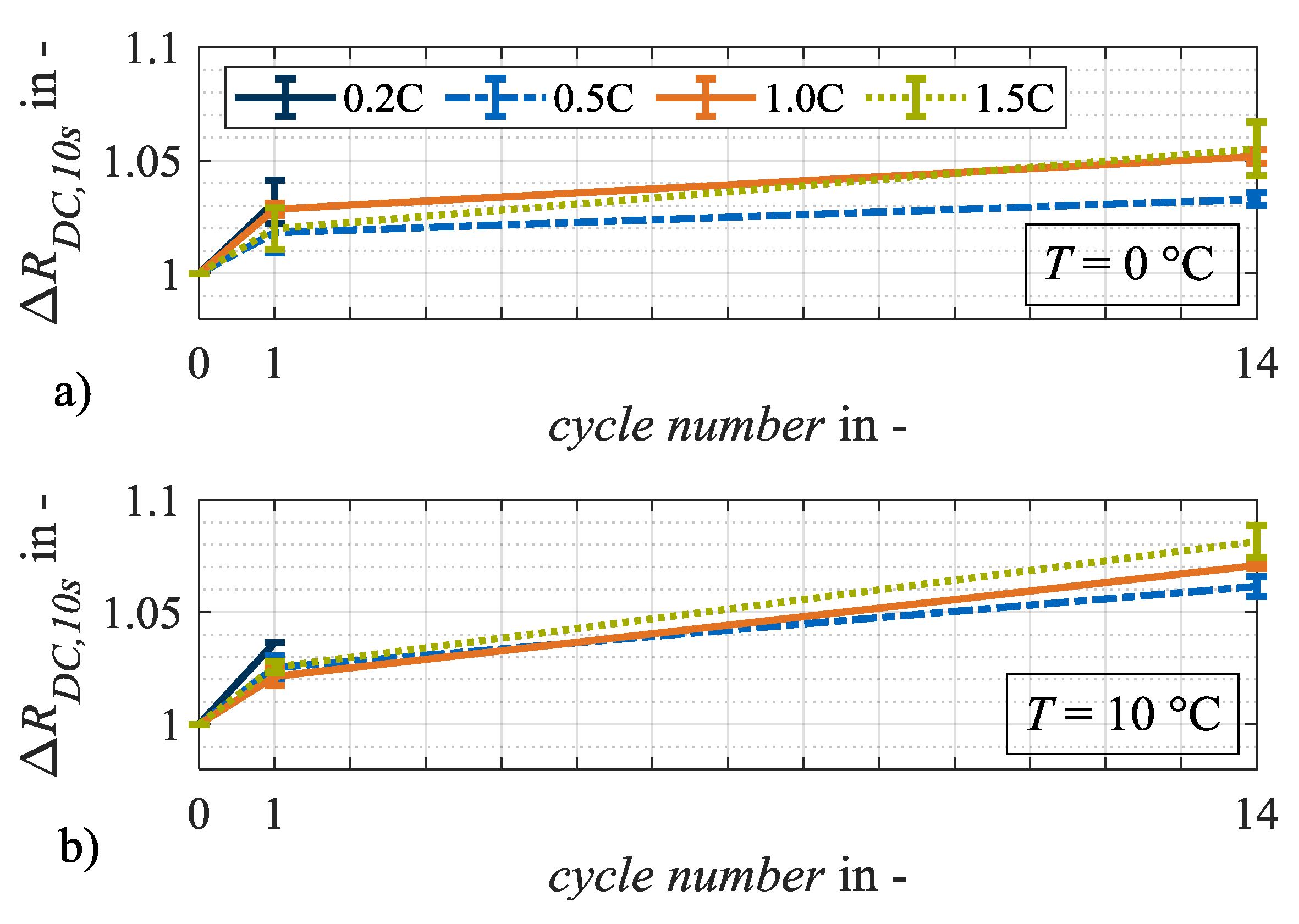
3.2. Investigation of Reversible Lithium Plating
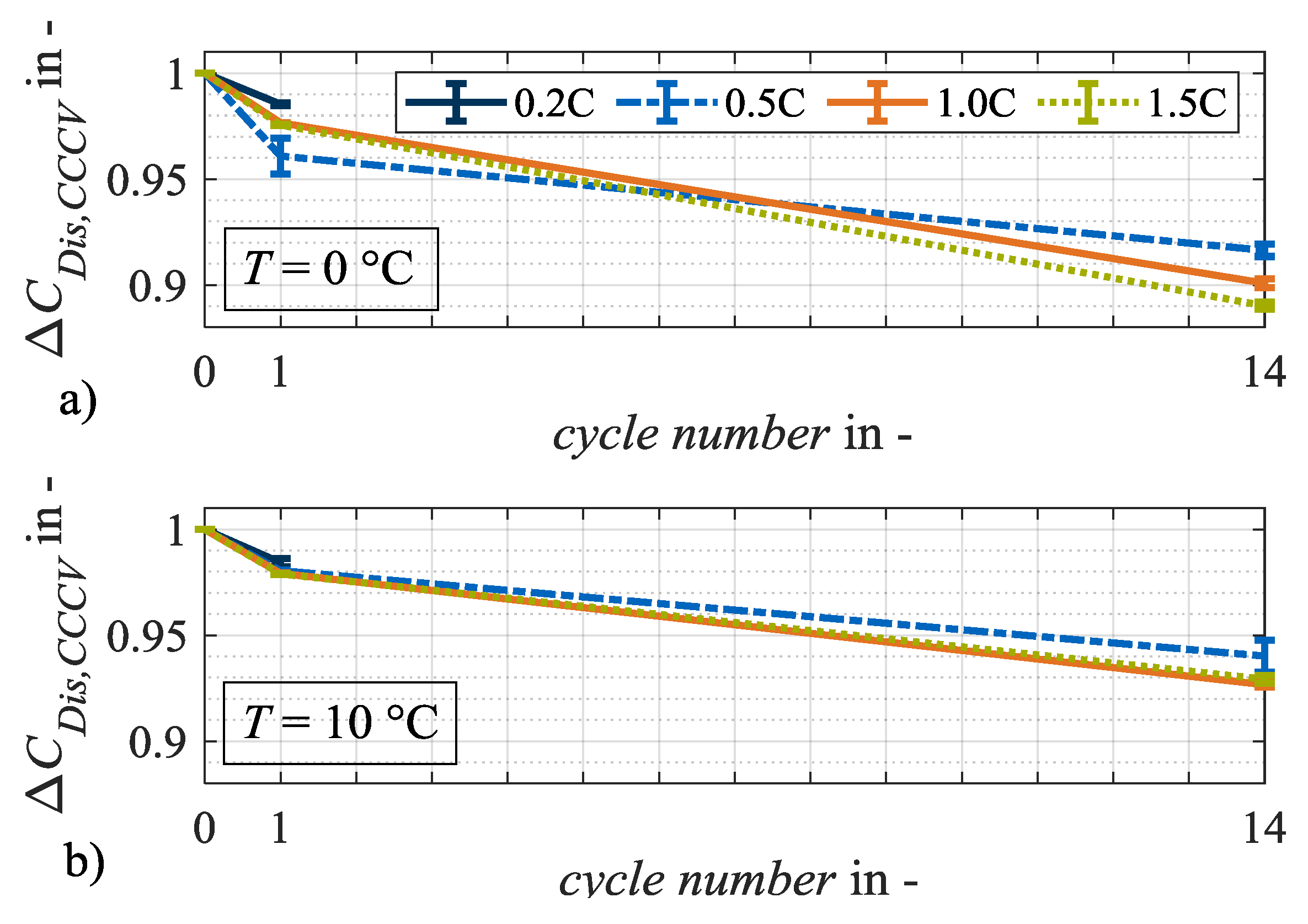
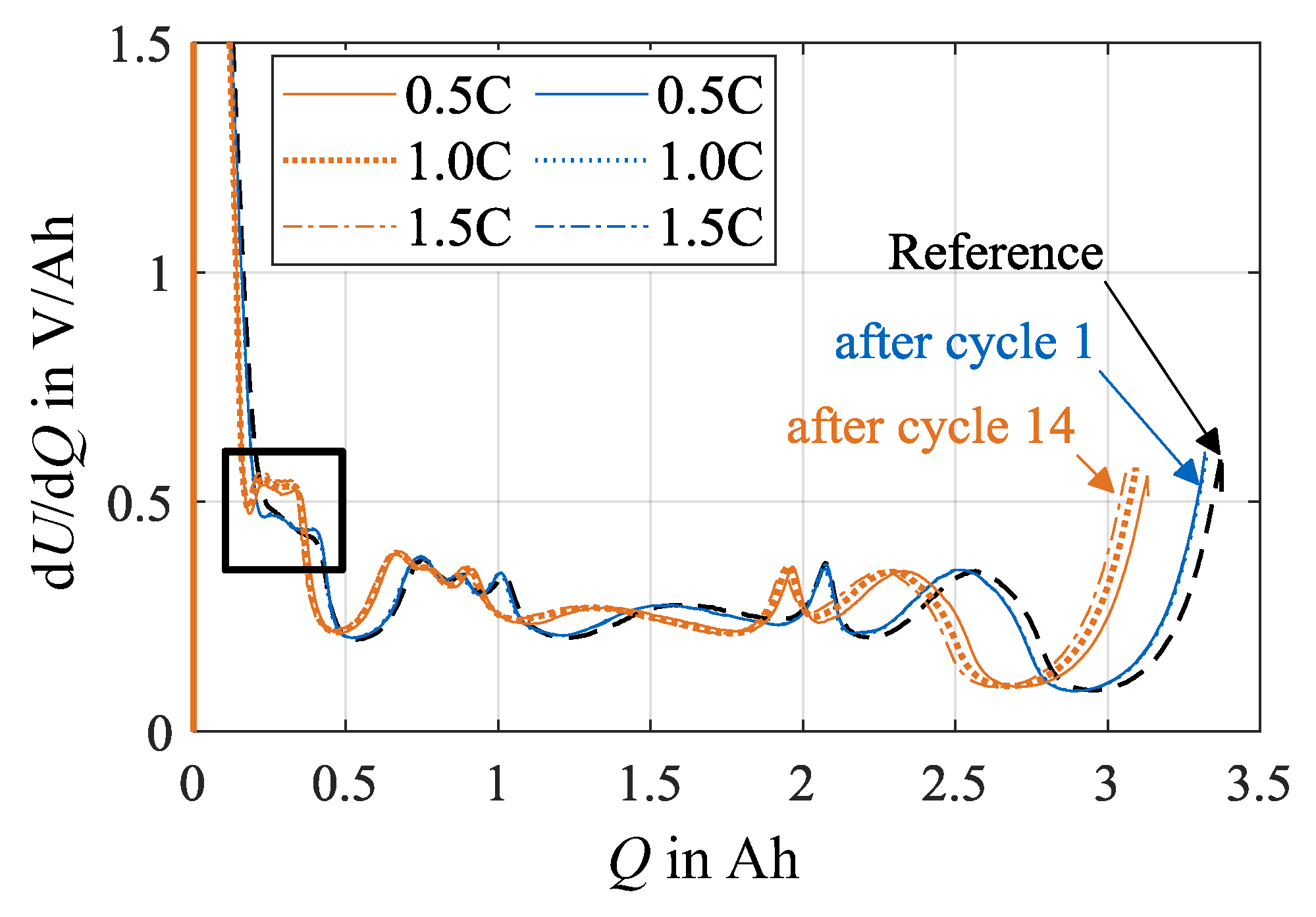
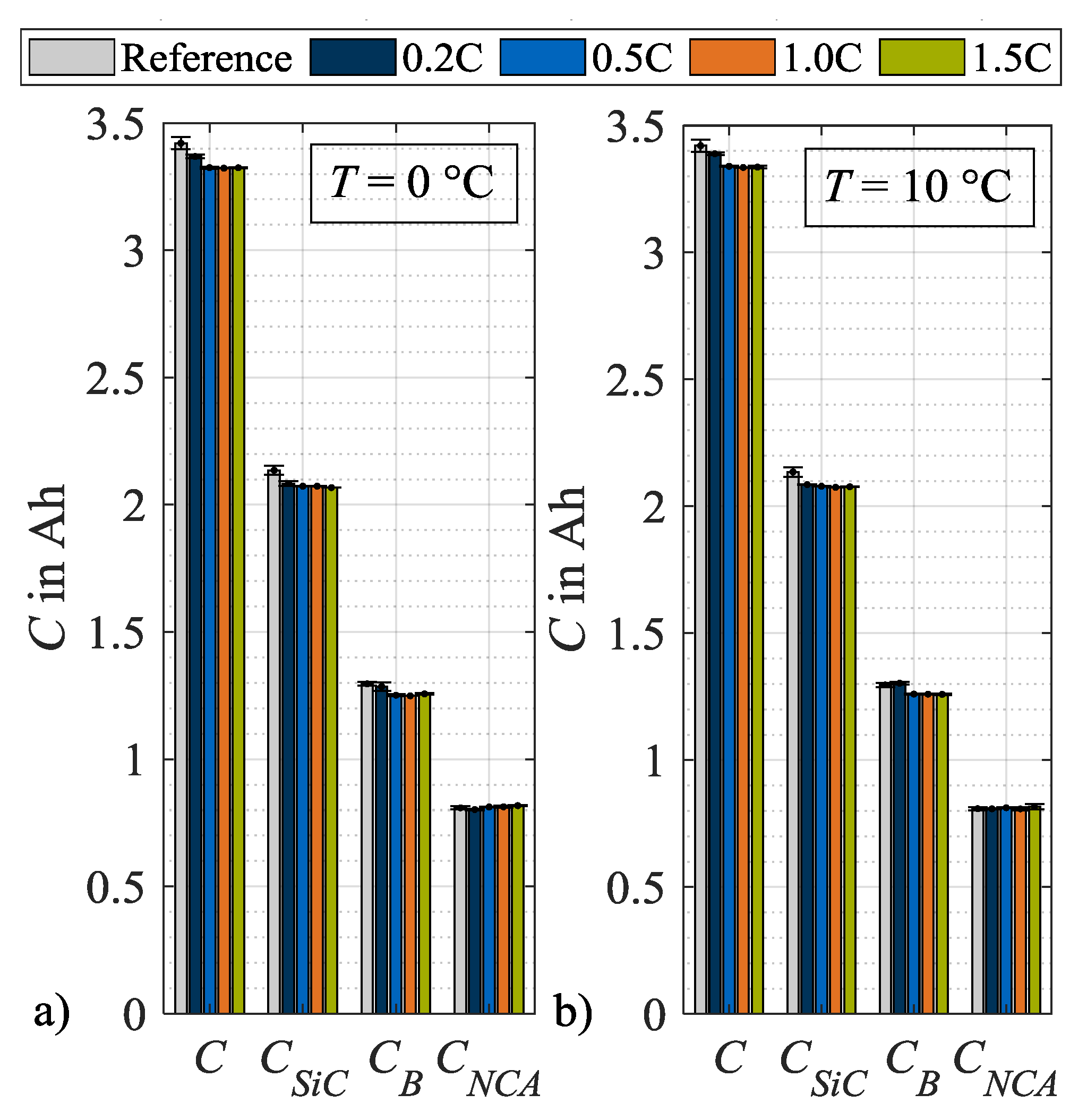
3.3. Investigation of Irreversible Lithium Plating and Aging Effects
4. Summary and Conclusions
4.1. Findings on Reversible and Irreversible Lithium Plating
4.2. Degradation Sensitivity of Silicon Share
4.3. Recommendations for the Implementation of Charging Protocols
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A
| Charge Rate | Characteristic Capacity | ||||
|---|---|---|---|---|---|
| ΔC (mAh) | ΔC (mAh) | ΔCB (mAh) | ΔCNCA (mAh) | ΔCC,1–3 (mAh) | |
| 0.2C | −52 | −53 | −10 | −7 | −17 |
| 0.5C | −96 | −63 | −44 | +3 | −17 |
| 1.0C | −99 | −63 | −47 | +4 | −13 |
| 1.5C | −97 | −64 | −39 | +8 | −15 |
| Charge Rate | Characteristic Capacity | ||||
|---|---|---|---|---|---|
| ΔC (mAh) | ΔC (mAh) | ΔCB (mAh) | ΔCNCA (mAh) | ΔCC,1–3 (mAh) | |
| 0.2C | −32 | −50 | + 7 | 0 | −16 |
| 0.5C | −81 | −57 | −36 | + 4 | −15 |
| 1.0C | −86 | −61 | −36 | −1 | −14 |
| 1.5C | −84 | −59 | −37 | + 8 | −15 |
| Charge Rate | Characteristic Capacity | ||||
|---|---|---|---|---|---|
| ΔC (mAh) | ΔC (mAh) | ΔCB (mAh) | ΔCNCA (mAh) | ΔCC,1–3 (mAh) | |
| 0.2C | - | - | - | - | - |
| 0.5C | −282 | −169 | −124 | −8 | −37 |
| 1.0C | −334 | −187 | −158 | −7 | −38 |
| 1.5C | −354 | −200 | −165 | −4 | −44 |
| Charge Rate | Characteristic Capacity | ||||
|---|---|---|---|---|---|
| ΔC (mAh) | ΔC (mAh) | ΔCB (mAh) | ΔCNCA (mAh) | ΔCC,1–3 (mAh) | |
| 0.2C | - | - | - | - | - |
| 0.5C | −297 | −180 | −128 | −14 | −43 |
| 1.0C | −319 | −195 | −136 | −12 | −47 |
| 1.5C | −315 | −194 | −132 | −17 | −47 |
References
- National Platform for Electric Mobility. Roadmap for an Integrated Cell and Battery Production in Germany. WG 2 Battery Technology. 2016. Available online: http://nationale-plattform-elektromobilitaet.de/fileadmin/user_upload/Redaktion/Publikationen/AG2_Roadmap_Zellfertigung_eng_bf.pdf (accessed on 31 May 2019).
- Myung, S.-T.; Maglia, F.; Park, K.-J.; Yoon, C.S.; Lamp, P.; Kim, S.-J.; Sun, Y.-K. Nickel-Rich Layered Cathode Materials for Automotive Lithium-Ion Batteries: Achievements and Perspectives. ACS Energy Lett. 2017, 2, 196–223. [Google Scholar] [CrossRef]
- Khomenko, V.G.; Barsukov, V.Z.; Doninger, J.E.; Barsukov, I. V600. Lithium-ion batteries based on carbon–silicon–graphite composite anodes. J. Power Sources 2007, 165, 598–608. [Google Scholar] [CrossRef]
- Wu, J.J.; Bennett, W.R. Fundamental investigation of Si anode in Li-Ion cells. In 2012 IEEE Energytech; IEEE: Cleveland, OH, USA, 2012; pp. 1–5. [Google Scholar]
- Li, X.; Colclasure, A.M.; Finegan, D.P.; Ren, D.; Shi, Y.; Feng, X.; Cao, L.; Yang, Y.; Smith, K. Degradation mechanisms of high capacity 18650 cells containing Si-graphite anode and nickel-rich NMC cathode. Electrochim. Acta 2019, 297, 1109–1120. [Google Scholar] [CrossRef]
- Zilberman, I.; Sturm, J.; Jossen, A. Reversible self-discharge and calendar aging of 18650 nickel-rich, silicon-graphite lithium-ion cells. J. Power Sources 2019, 425, 217–226. [Google Scholar] [CrossRef]
- Sturm, J.; Rheinfeld, A.; Zilberman, I.; Spingler, F.B.; Kosch, S.; Frie, F.; Jossen, A. Modeling and simulation of inhomogeneities in a 18650 nickel-rich, silicon-graphite lithium-ion cell during fast charging. J. Power Sources 2019, 412, 204–223. [Google Scholar] [CrossRef]
- Dose, W.M.; Piernas-Muñoz, M.J.; Maroni, V.A.; Trask, S.E.; Bloom, I.; Johnson, C.S. Capacity fade in high energy silicon-graphite electrodes for lithium-ion batteries. Chem. Commun. 2018, 54, 3586–3589. [Google Scholar] [CrossRef] [PubMed]
- Kalaga, K.; Rodrigues, M.-T.F.; Trask, S.E.; Shkrob, I.A.; Abraham, D.P. Calendar-life versus cycle-life aging of lithium-ion cells with silicon-graphite composite electrodes. Electrochim. Acta 2018, 280, 221–228. [Google Scholar] [CrossRef]
- Michan, A.L.; Divitini, G.; Pell, A.J.; Leskes, M.; Ducati, C.; Grey, C.P. Solid Electrolyte Interphase Growth and Capacity Loss in Silicon Electrodes. J. Am. Chem. Soc. 2016, 138, 7918–7931. [Google Scholar] [CrossRef]
- Petzl, M.; Kasper, M.; Danzer, M.A. Lithium plating in a commercial lithium-ion battery—A low-temperature aging study. J. Power Sources 2015, 275, 799–807. [Google Scholar] [CrossRef]
- Waldmann, T.; Kasper, M.; Wohlfahrt-Mehrens, M. Optimization of Charging Strategy by Prevention of Lithium Deposition on Anodes in high-energy Lithium-ion Batteries—Electrochemical Experiments. Electrochim. Acta 2015, 178, 525–532. [Google Scholar] [CrossRef]
- Yang, X.-G.; Liu, T.; Gao, Y.; Ge, S.; Leng, Y.; Wang, D.; Wang, C.-Y. Asymmetric Temperature Modulation for Extreme Fast Charging of Lithium-Ion Batteries. Joule 2019. [Google Scholar] [CrossRef]
- Waldmann, T.; Hogg, B.-I.; Wohlfahrt-Mehrens, M. Li plating as unwanted side reaction in commercial Li-ion cells–A review. J. Power Sources 2018, 384, 107–124. [Google Scholar] [CrossRef]
- Li, Z.; Huang, J.; Yann Liaw, B.; Metzler, V.; Zhang, J. A review of lithium deposition in lithium-ion and lithium metal secondary batteries. J. Power Sources 2014, 254, 168–182. [Google Scholar] [CrossRef]
- Smart, M.C.; Ratnakumar, B.V. Effects of Electrolyte Composition on Lithium Plating in Lithium-Ion Cells. J. Electrochem. Soc. 2011, 158, A379. [Google Scholar] [CrossRef]
- Schindler, S.; Bauer, M.; Petzl, M.; Danzer, M.A. Voltage relaxation and impedance spectroscopy as in-operando methods for the detection of lithium plating on graphitic anodes in commercial lithium-ion cells. J. Power Sources 2016, 304, 170–180. [Google Scholar] [CrossRef]
- Gallagher, K.G.; Trask, S.E.; Bauer, C.; Woehrle, T.; Lux, S.F.; Tschech, M.; Lamp, P.; Polzin, B.J.; Ha, S.; Long, B.; et al. Optimizing Areal Capacities through Understanding the Limitations of Lithium-Ion Electrodes. J. Electrochem. Soc. 2016, 163, A138–A149. [Google Scholar] [CrossRef]
- Petzl, M.; Danzer, M.A. Nondestructive detection, characterization, and quantification of lithium plating in commercial lithium-ion batteries. J. Power Sources 2014, 254, 80–87. [Google Scholar] [CrossRef]
- Vetter, J.; Novák, P.; Wagner, M.R.; Veit, C.; Möller, K.-C.; Besenhard, J.O.; Winter, M.; Wohlfahrt-Mehrens, M.; Vogler, C.; Hammouche, A. Ageing mechanisms in lithium-ion batteries. J. Power Sources 2005, 147, 269–281. [Google Scholar] [CrossRef]
- Legrand, N.; Knosp, B.; Desprez, P.; Lapicque, F.; Raël, S. Physical characterization of the charging process of a Li-ion battery and prediction of Li plating by electrochemical modelling. J. Power Sources 2014, 245, 208–216. [Google Scholar] [CrossRef]
- von Lüders, C.; Keil, J.; Webersberger, M.; Jossen, A. Modeling of lithium plating and lithium stripping in lithium-ion batteries. J. Power Sources 2019, 414, 41–47. [Google Scholar] [CrossRef]
- Zinth, V.; von Lüders, C.; Hofmann, M.; Hattendorff, J.; Buchberger, I.; Erhard, S.; Rebelo-Kornmeier, J.; Jossen, A.; Gilles, R. Lithium plating in lithium-ion batteries at sub-ambient temperatures investigated by in situ neutron diffraction. J. Power Sources 2014, 271, 152–159. [Google Scholar] [CrossRef]
- von Lüders, C.; Zinth, V.; Erhard, S.V.; Osswald, P.J.; Hofmann, M.; Gilles, R.; Jossen, A. Lithium plating in lithium-ion batteries investigated by voltage relaxation and in situ neutron diffraction. J. Power Sources 2017, 342, 17–23. [Google Scholar] [CrossRef]
- Bloom, I.; Jansen, A.N.; Abraham, D.P.; Knuth, J.; Jones, S.A.; Battaglia, V.S.; Henriksen, G.L. Differential voltage analyses of high-power, lithium-ion cells: 1. Technique and application. J. Power Sources 2005, 139, 295–303. [Google Scholar] [CrossRef]
- Keil, P.; Jossen, A. Calendar Aging of NCA Lithium-Ion Batteries Investigated by Differential Voltage Analysis and Coulomb Tracking. J. Electrochem. Soc. 2017, 164, A6066–A6074. [Google Scholar] [CrossRef]
- Birkl, C.R.; Roberts, M.R.; McTurk, E.; Bruce, P.G.; Howey, D.A. Degradation diagnostics for lithium ion cells. J. Power Sources 2017, 341, 373–386. [Google Scholar] [CrossRef]
- Lewerenz, M.; Marongiu, A.; Warnecke, A.; Sauer, D.U. Differential voltage analysis as a tool for analyzing inhomogeneous aging: A case study for LiFePO4|Graphite cylindrical cells. J. Power Sources 2017, 368, 57–67. [Google Scholar] [CrossRef]
- Burns, J.C.; Stevens, D.A.; Dahn, J.R. In-Situ Detection of Lithium Plating Using High Precision Coulometry. J. Electrochem. Soc. 2015, 162, A959–A964. [Google Scholar] [CrossRef]
- Smith, A.J.; Burns, J.C.; Trussler, S.; Dahn, J.R. Precision Measurements of the Coulombic Efficiency of Lithium-Ion Batteries and of Electrode Materials for Lithium-Ion Batteries. J. Electrochem. Soc. 2010, 157, A196. [Google Scholar] [CrossRef]
- Xu, J.; Deshpande, R.D.; Pan, J.; Cheng, Y.-T.; Battaglia, V.S. Electrode Side Reactions, Capacity Loss and Mechanical Degradation in Lithium-Ion Batteries. J. Electrochem. Soc. 2015, 162, A2026–A2035. [Google Scholar] [CrossRef]
- Same, A.; Battaglia, V.; Tang, H.-Y.; Park, J.W. In situ neutron radiography analysis of graphite/NCA lithium-ion battery during overcharge. J. Appl. Electrochem. 2012, 42, 1–9. [Google Scholar] [CrossRef]
- Wilhelm, J.; Seidlmayer, S.; Keil, P.; Schuster, J.; Kriele, A.; Gilles, R.; Jossen, A. Cycling capacity recovery effect: A coulombic efficiency and post-mortem study. J. Power Sources 2017, 365, 327–338. [Google Scholar] [CrossRef]
- Gewald, T.; Lienkamp, M.; Lehmkuhl, D.; Hahn, A. Accelerated Aging Characterization of Lithium-Ion Cells: Limitation of Arrhenius Dependency. In Proceedings of the 2019 Fourteenth International Conference on Ecological Vehicles and Renewable Energies (EVER), Monte-Carlo, Monaco, 8–10 May 2019; pp. 1–10. [Google Scholar]
- Yang, X.-G.; Ge, S.; Liu, T.; Leng, Y.; Wang, C.-Y. A look into the voltage plateau signal for detection and quantification of lithium plating in lithium-ion cells. J. Power Sources 2018, 395, 251–261. [Google Scholar] [CrossRef]
- Naumann, M.; Schimpe, M.; Keil, P.; Hesse, H.C.; Jossen, A. Analysis and modeling of calendar aging of a commercial LiFePO4/graphite cell. J. Energy Storage 2018, 17, 153–169. [Google Scholar] [CrossRef]
- Rumpf, K.; Naumann, M.; Jossen, A. Experimental investigation of parametric cell-to-cell variation and correlation based on 1100 commercial lithium-ion cells. J. Energy Storage 2017, 14, 224–243. [Google Scholar] [CrossRef]
- Schmalstieg, J.; Käbitz, S.; Ecker, M.; Sauer, D.U. A holistic aging model for Li(NiMnCo)O2 based 18650 lithium-ion batteries. J. Power Sources 2014, 257, 325–334. [Google Scholar] [CrossRef]
- German Environment Agency. Trends der Lufttemperatur [Trends in Air Temperature]. Available online: https://www.umweltbundesamt.de/daten/klima/trends-der-lufttemperatur#textpart-1 (accessed on 29 May 2019).
- Drake, S.J.; Wetz, D.A.; Ostanek, J.K.; Miller, S.P.; Heinzel, J.M.; Jain, A. Measurement of anisotropic thermophysical properties of cylindrical Li-ion cells. J. Power Sources 2014, 252, 298–304. [Google Scholar] [CrossRef]
- Waldmann, T.; Wohlfahrt-Mehrens, M. In-Operando Measurement of Temperature Gradients in Cylindrical Lithium-Ion Cells during High-Current Discharge. ECS Electrochem. Lett. 2015, 4, A1–A3. [Google Scholar] [CrossRef]

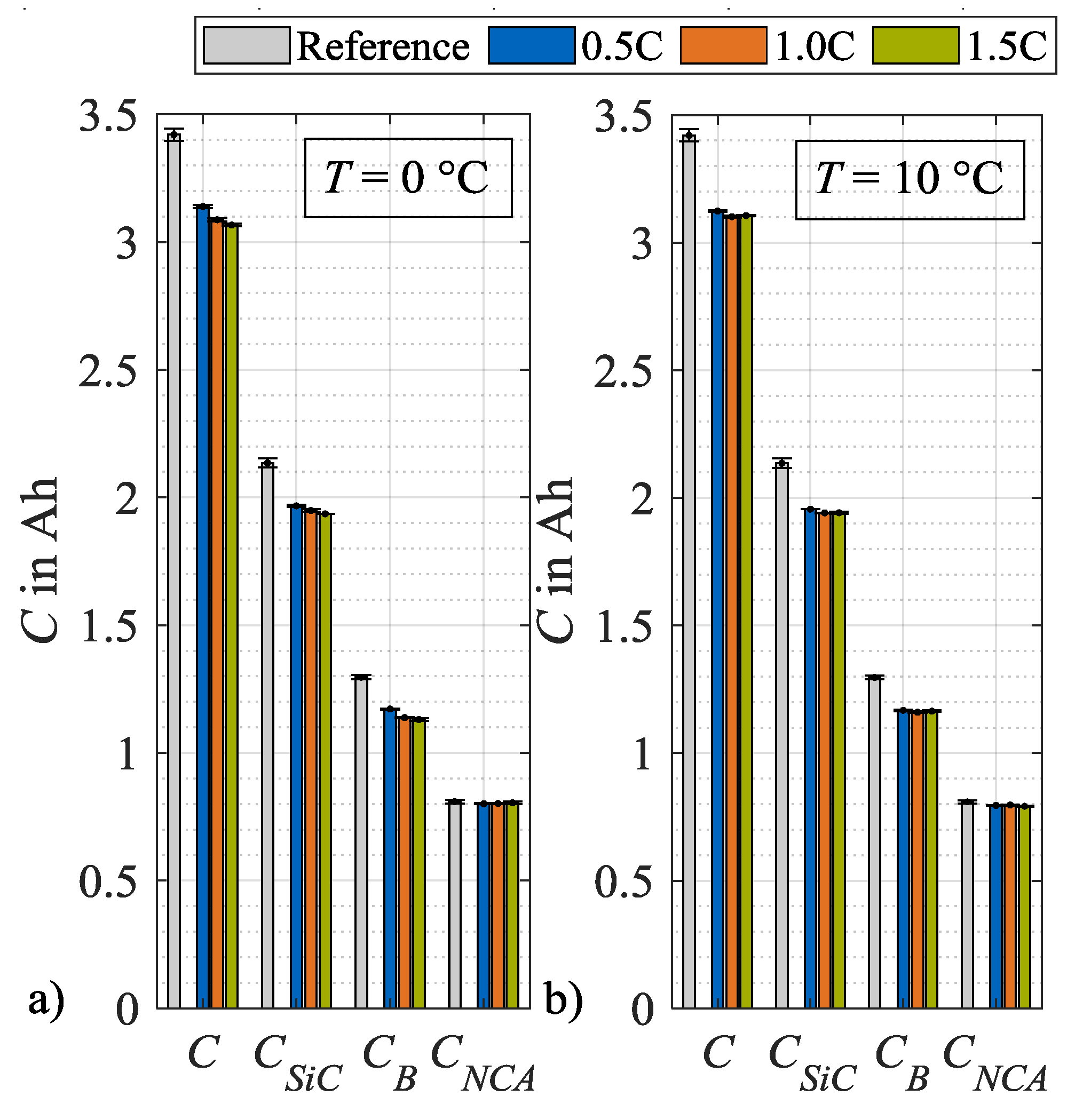
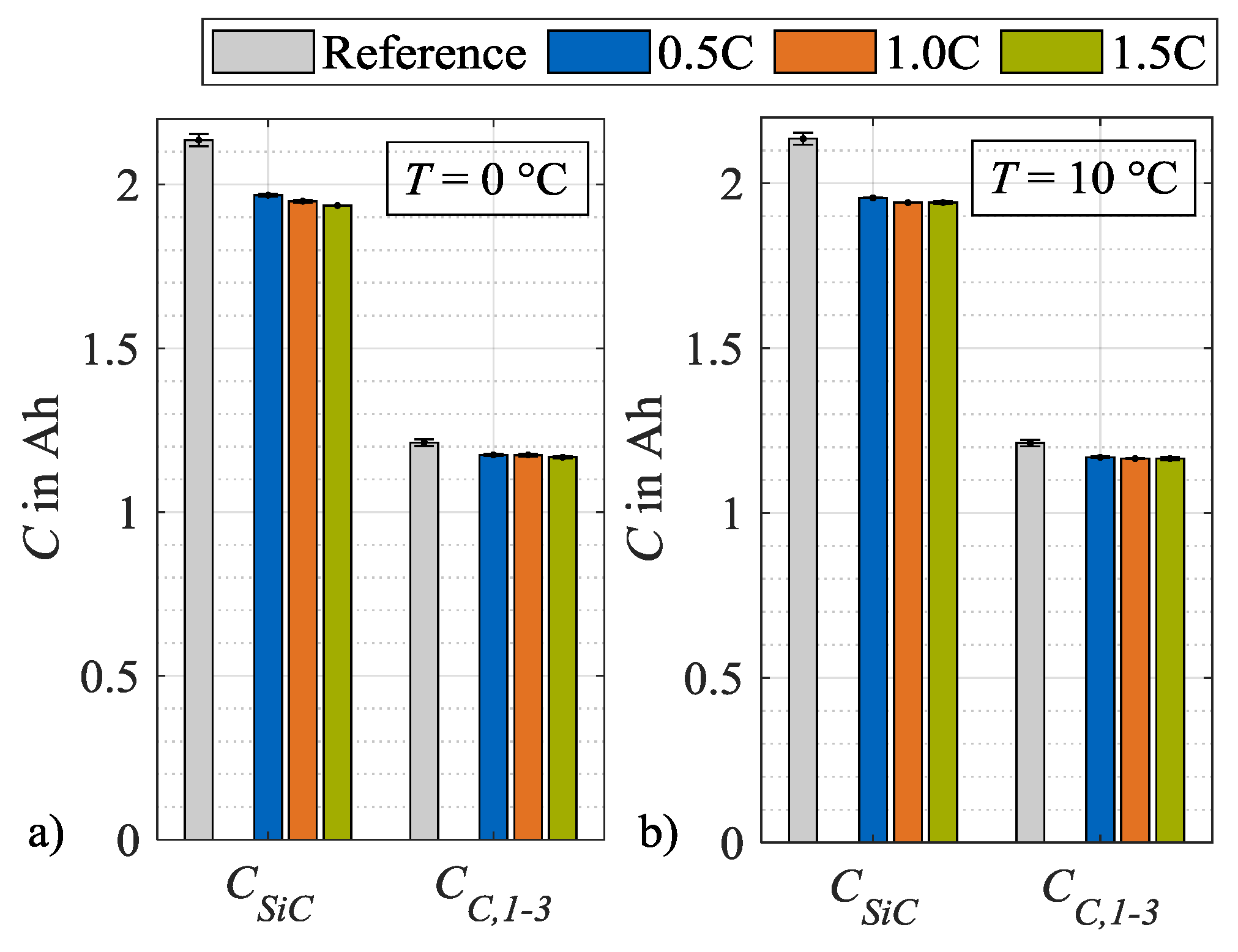
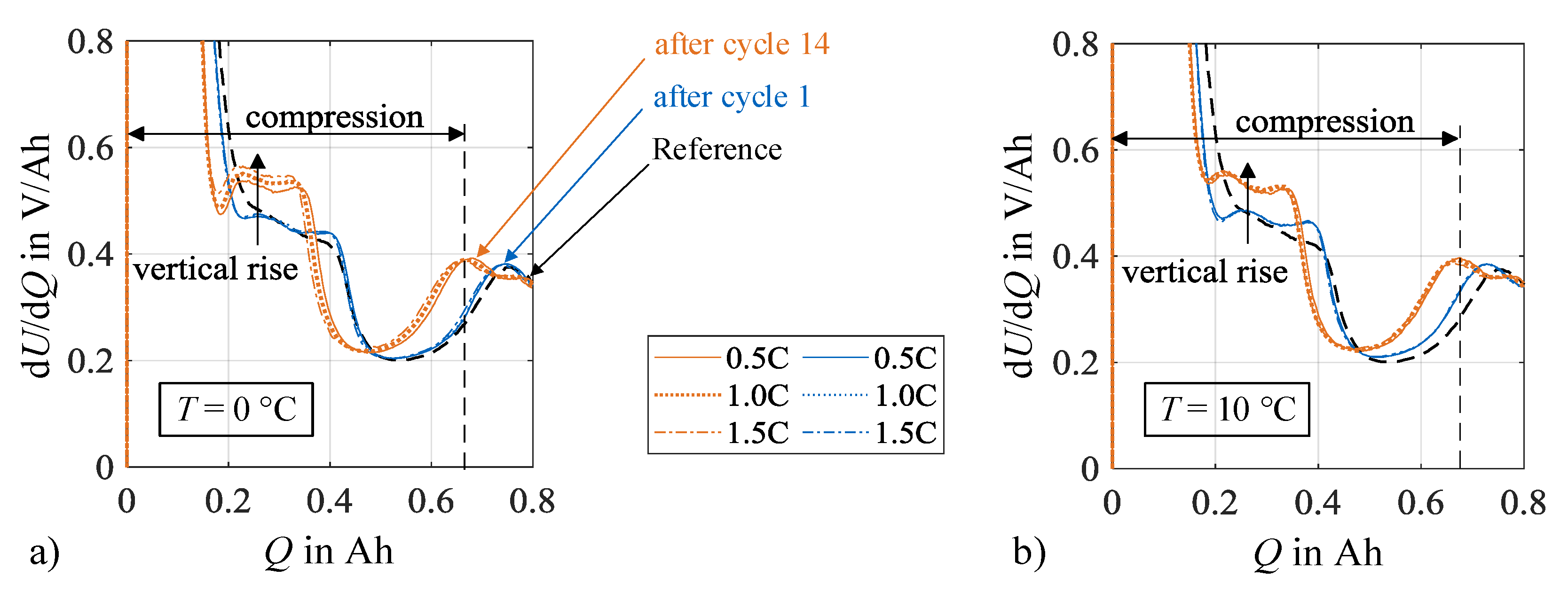
| Step | Temperature | Method | Parameter |
|---|---|---|---|
| 1 | 0 °C/10 °C | Stripping discharge | Evidence lithium plating |
| 2 | 25 °C | DVA | Characteristic capacities |
| 3 | 25 °C | Two CCCV cycles | CDis,CCCV |
| 4 | 25 °C | Two-stage pulse test | RDC,10s |
| T/Ich | 0.2C | 0.5C | 1.0C | 1.5C |
|---|---|---|---|---|
| 0 °C | • | • | • | • |
| 10 °C | • | • | • | • |
| Step | Action | Step | Action |
|---|---|---|---|
| 1 | Activation cycles | 6 | Stripping discharge |
| 2 | Check-up test 0 | 7 | Load cycles 3–5 |
| 3 | Load cycle 1 | 8 | Stripping discharge |
| 4 | Check-up test 1 | 9 | Load cycles 6–14 |
| 5 | Load cycle 2 | 10 | Check-up test 2 |
| Charge Rate | Cycle Number | |||
|---|---|---|---|---|
| 1 | 2 | 5 | 14 | |
| 0.2C | x | x | - | - |
| 0.5C | • | • | • | x |
| 1.0C | • | • | • | • |
| 1.5C | • | • | • | • |
| Charge Rate | Cycle Number | |||
|---|---|---|---|---|
| 1 | 2 | 5 | 14 | |
| 0.2C | x | x | - | - |
| 0.5C | x | x | x | x |
| 1.0C | • | • | • | • |
| 1.5C | • | • | • | • |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bednorz, R.; Gewald, T. Investigation of the Effects of Charging Processes on Lithium-Ion Cells with SiC Anodes at Low Temperatures. Batteries 2020, 6, 34. https://doi.org/10.3390/batteries6020034
Bednorz R, Gewald T. Investigation of the Effects of Charging Processes on Lithium-Ion Cells with SiC Anodes at Low Temperatures. Batteries. 2020; 6(2):34. https://doi.org/10.3390/batteries6020034
Chicago/Turabian StyleBednorz, Ralph, and Tanja Gewald. 2020. "Investigation of the Effects of Charging Processes on Lithium-Ion Cells with SiC Anodes at Low Temperatures" Batteries 6, no. 2: 34. https://doi.org/10.3390/batteries6020034
APA StyleBednorz, R., & Gewald, T. (2020). Investigation of the Effects of Charging Processes on Lithium-Ion Cells with SiC Anodes at Low Temperatures. Batteries, 6(2), 34. https://doi.org/10.3390/batteries6020034





