New Manufacturing Process for Granular Texture Management in Polycrystalline BaM Hexaferrites through the Goethite Crystallite Laths Aspect Ratio, and a Specialized Law of Approach to the Magnetic Saturation for Partly Polarized Uniaxial Materials
Abstract
1. Introduction
2. Experimental Details
3. Results and Discussion
3.1. Manufacture of a Self-Polarized BaM Sample
3.2. The Aspect Ratio of the Goethite Particles: Its Major Role on the Quality of the BaM Particles Stacking
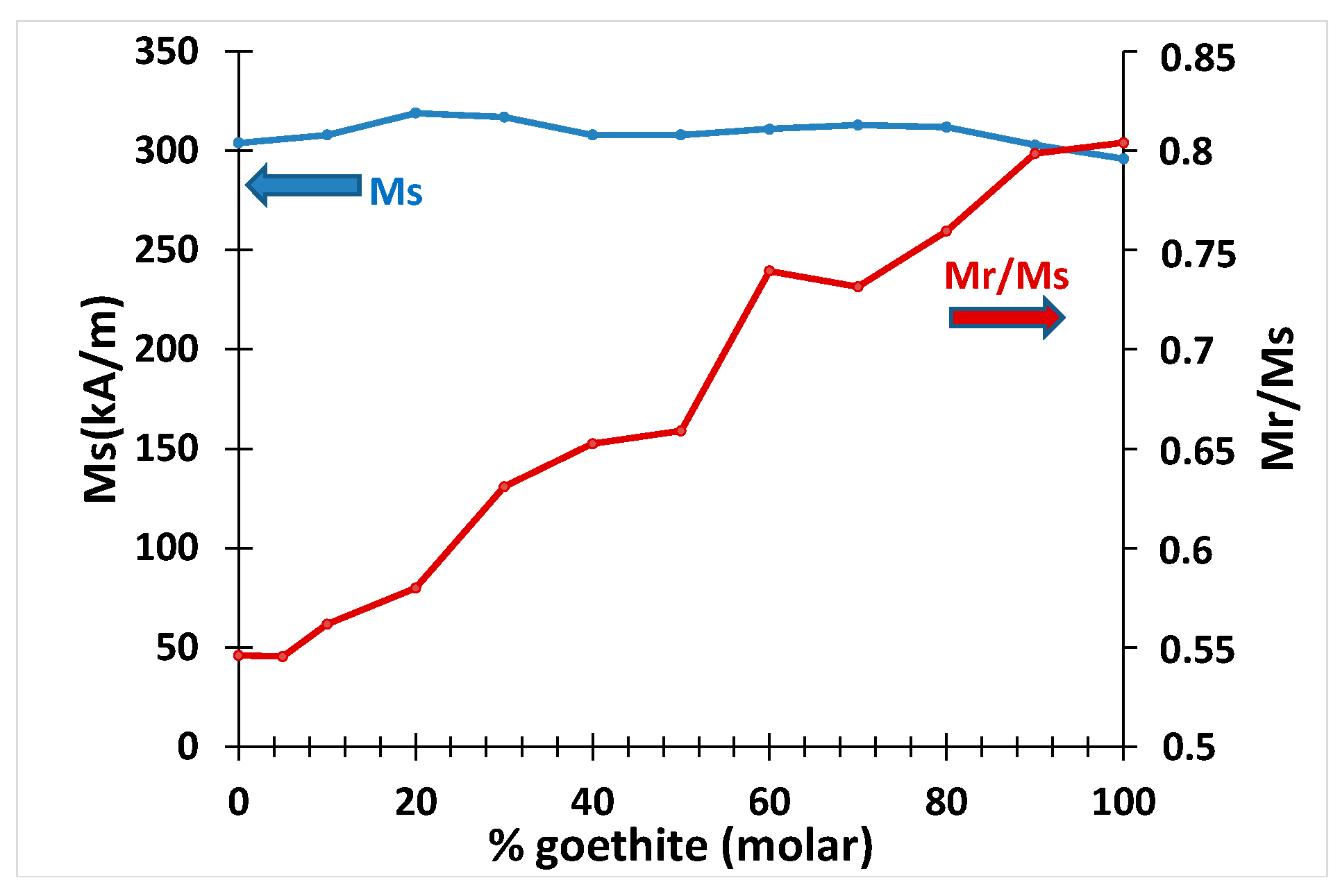
3.3. Manufacturing of BaM Samples with Differents Grades of Orientation
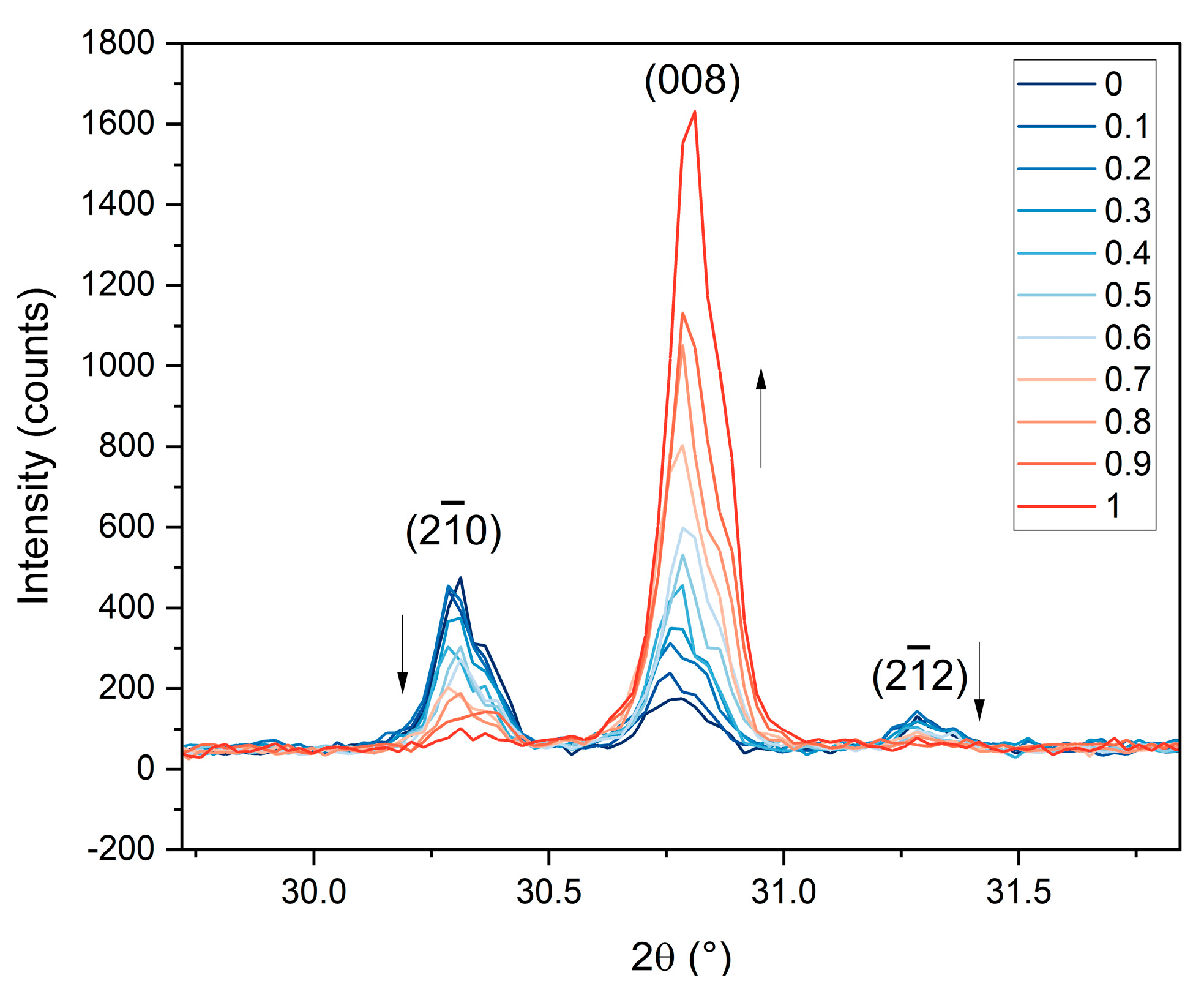
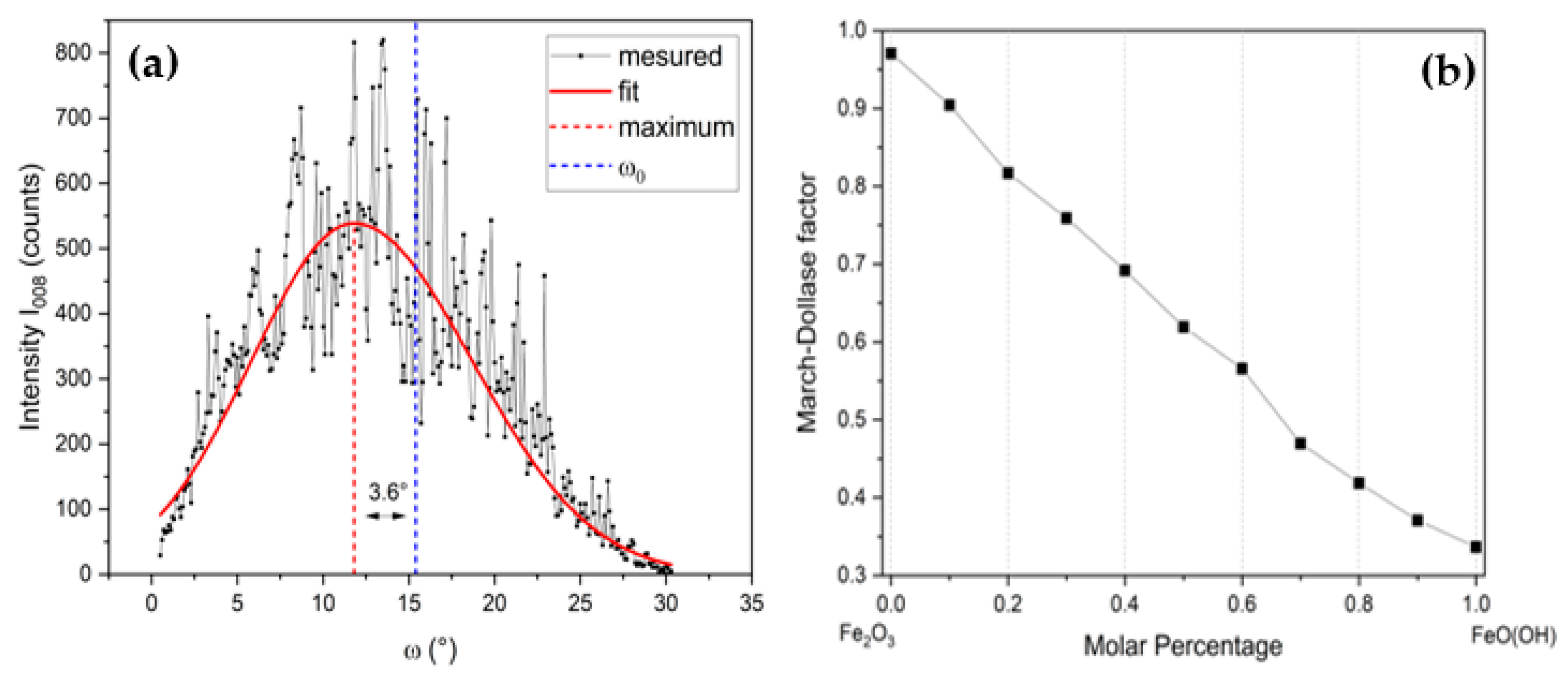
3.4. Crystallographic Study of BaM Samples Manufactured with Different Texturations
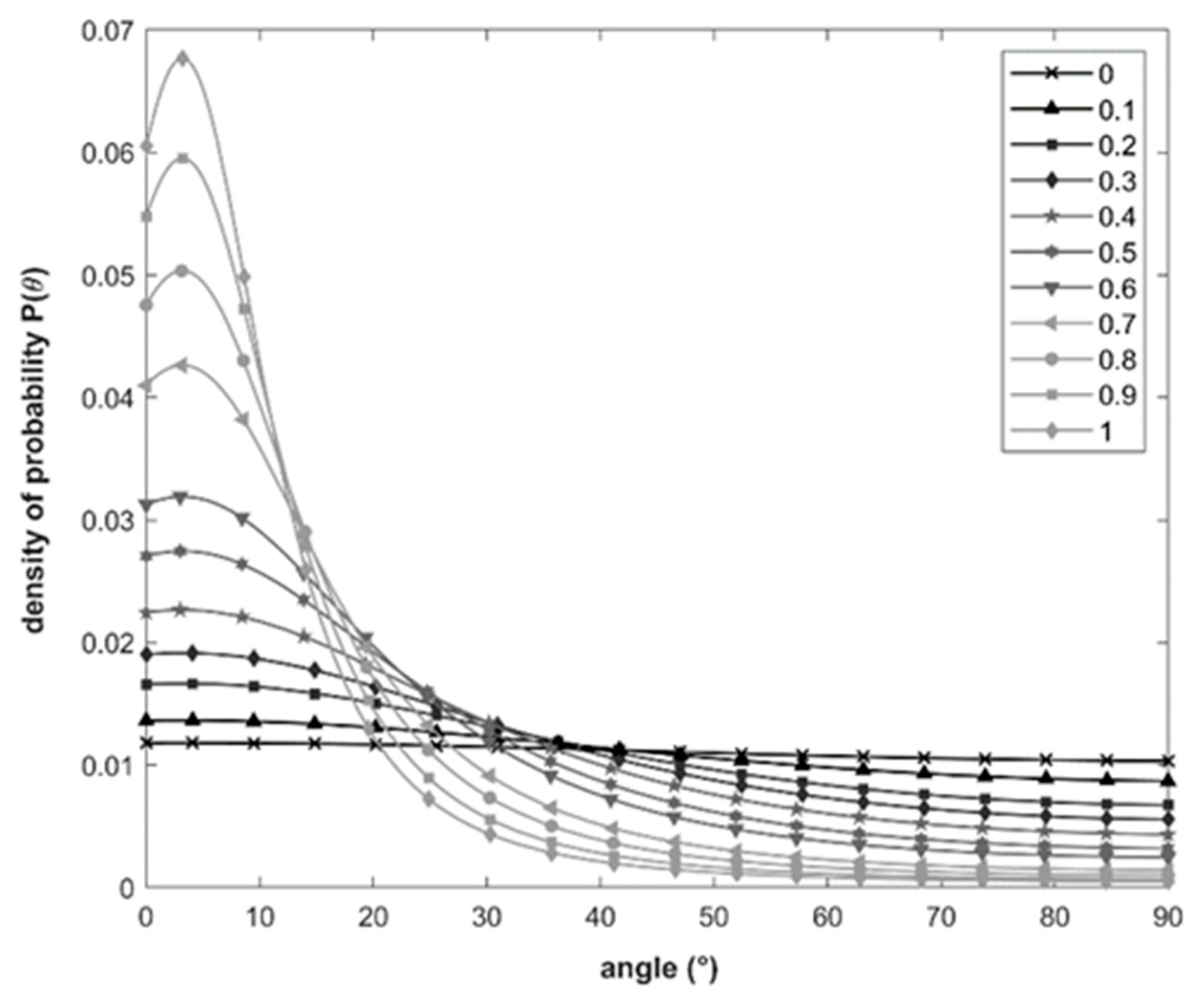
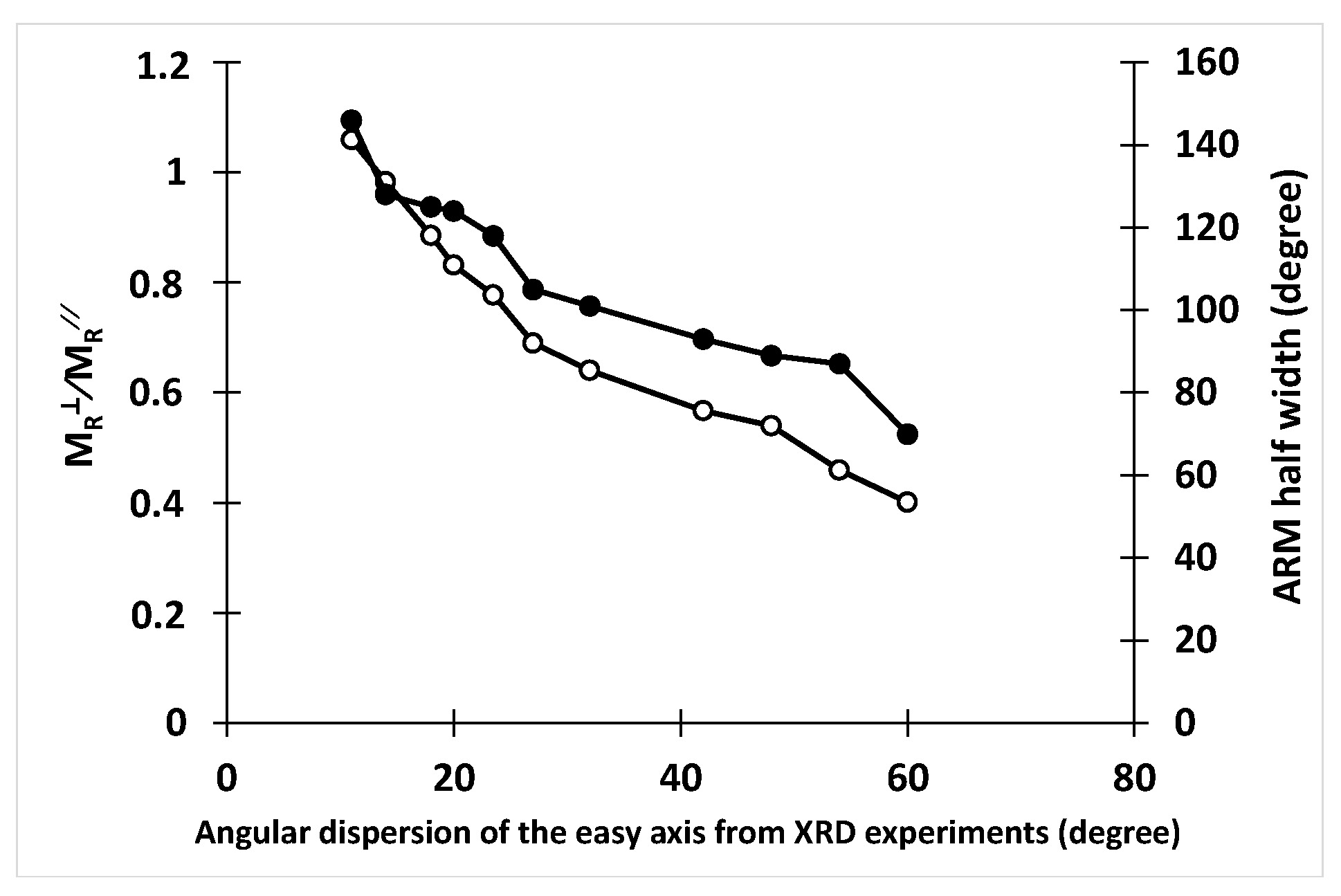
3.5. Experimental Investigations of the Magnetocrystalline Anisotropy
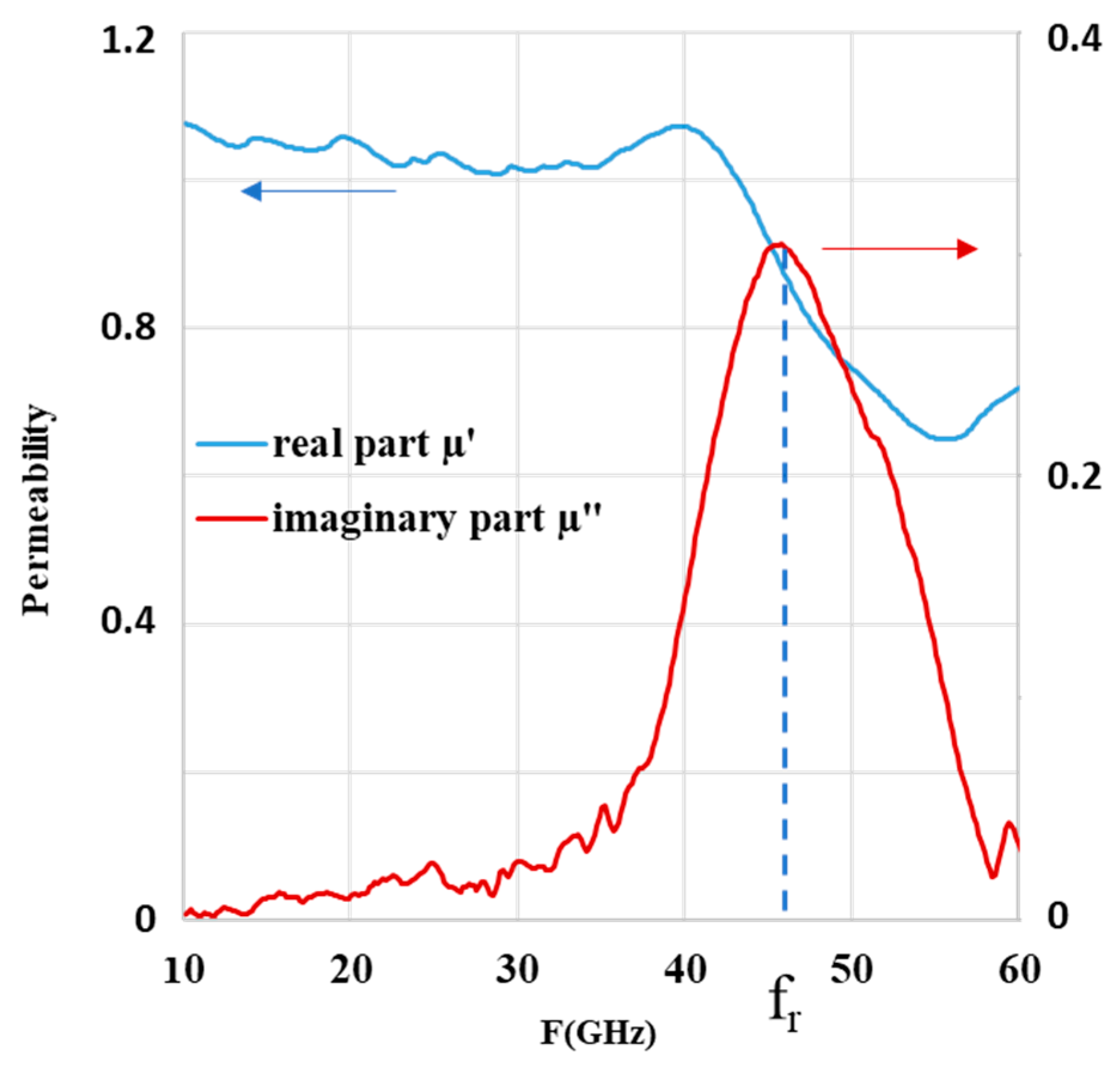
3.6. Microwave Measurement Method for Determining Anisotropy
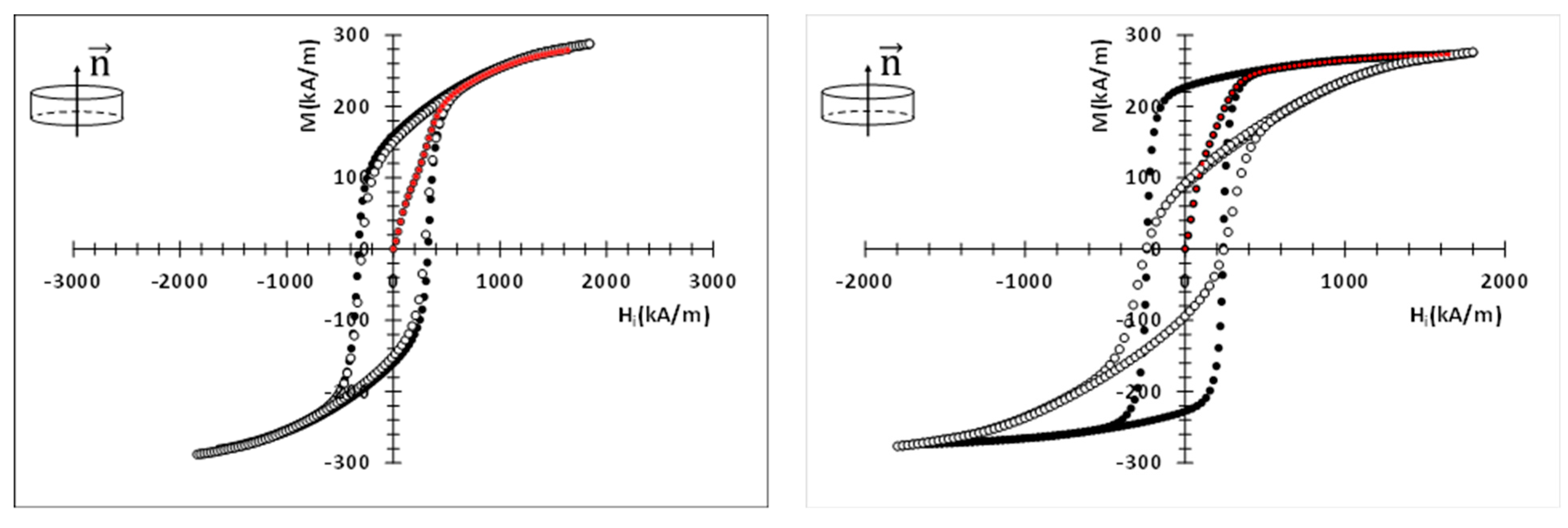
3.7. Isothermal Remanence Measurements
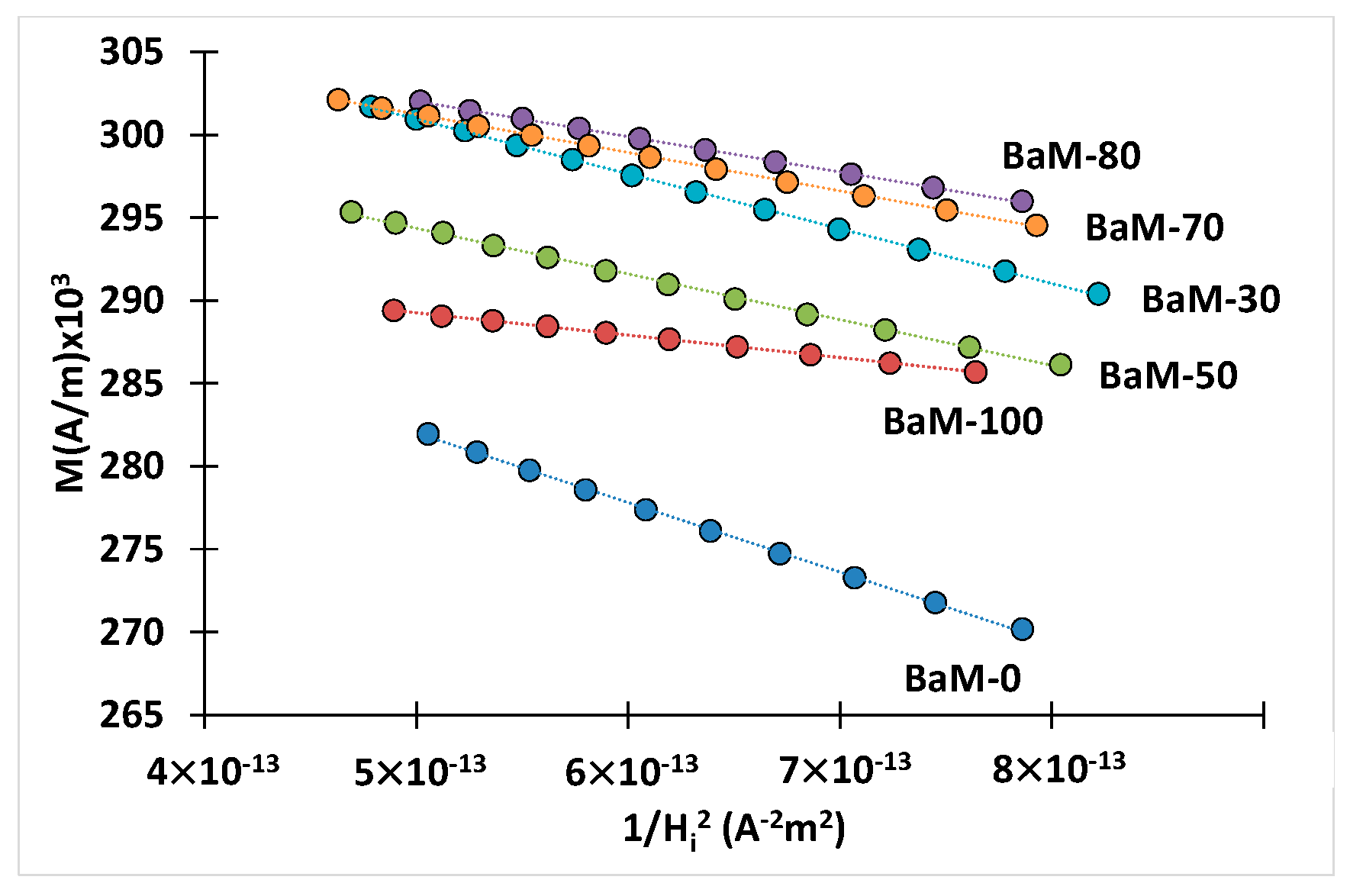
3.8. The First Magnetization Curve and the Law of Approach to Saturation
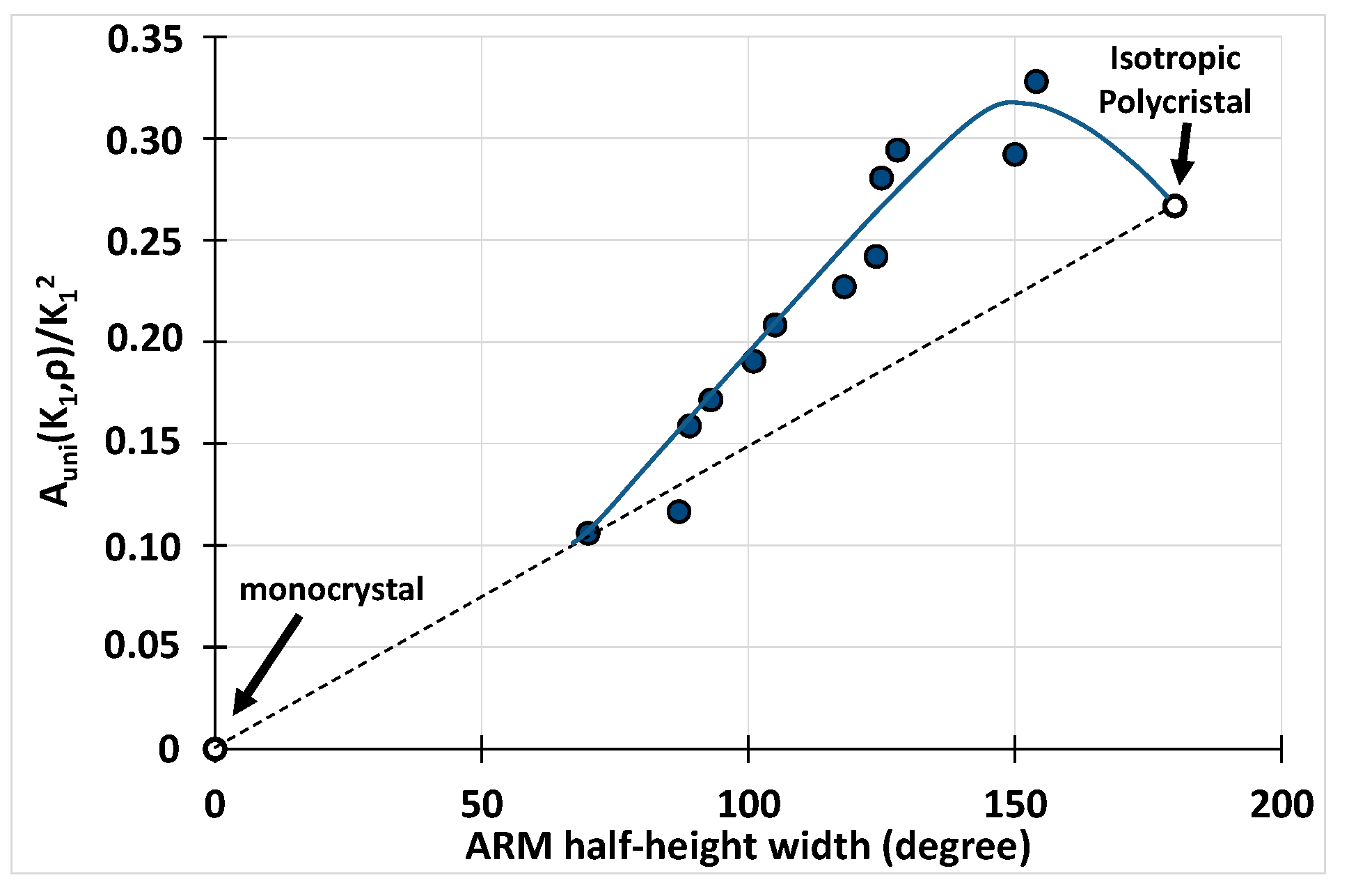
3.9. Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Mazaleyrat, F.; Zehani, K.; Pasko, A.; Loyau, V.; LoBue, M. XXIst century ferrites. J. Phys. Conf. Ser. 2012, 365, 12001. [Google Scholar] [CrossRef]
- Coey, J.M.D. Magnetism and Magnetic Materials; Cambridge University Press: Cambridge, UK, 2010. [Google Scholar]
- Coey, J.M.D. Permanent magnets: Plugging the gap. Scr. Mater. 2012, 67, 524–529. [Google Scholar] [CrossRef]
- Harris, V.G.; Geiler, A.; Chen, Y.; Yoon, S.D.; Wu, M.; Yang, A.; Chen, Z.; He, P.; Parimi, P.V.; Zuo, X.; et al. Recent advances in processing and applications of microwave ferrites. J. Magn. Magn. Mater. 2009, 321, 2035–2047. [Google Scholar] [CrossRef]
- Geiler, A.; Daigle, A.; Wang, J.; Chen, Y.; Vittoria, C.; Harris, V. Consequences of magnetic anisotropy in realizing practical microwave hexaferrite devices. J. Magn. Magn. Mater. 2012, 324, 3393–3397. [Google Scholar] [CrossRef]
- Mattei, J.L.; Le, C.N.; Chevalier, A.; Maalouf, A.; Noutehou, N.; Queffelec, P.; Laur, V. A simple process to obtain anisotropic self-biased magnets constituted of stacked barium ferrite single domain particles. J. Magn. Magn. Mater. 2018, 451, 208–213. [Google Scholar] [CrossRef]
- Turki, H.; Huitema, L.; Monediere, T.; Lenoir, B.; Breuil, C. Complete Methodology of Low-loss Ultra-wideband Junction Circulator. In Proceedings of the IEEE MTT-S International Microwave Symposium Digest, Philadelphia, PA, USA, 10–15 June 2018; Volume 2018, pp. 746–749. [Google Scholar]
- Olivier, V.; Huitema, L.; Lenoir, B.; Turki, H.; Breuil, C.; Pouliguen, P.; Monediere, T. Dual-Band Ferrite Circulators Operating on Weak Field Conditions: Design Methodology and Bandwidths’ Improvement. IEEE Trans. Microw. Theory Tech. 2020, 68, 2521–2530. [Google Scholar] [CrossRef]
- Turki, H.; Huitema, L.; Monediere, T.; Lenoir, B.; Breuil, C. New concept validation of low-loss dual-band stripline circulator. IEEE Trans. Microw. Theory Tech. 2019, 67, 845–850. [Google Scholar] [CrossRef]
- Pullar, R.C. Hexagonal ferrites: A review of the synthesis, properties and applications of hexaferrite ceramics. Prog. Mater. Sci. 2012, 57, 1191–1334. [Google Scholar] [CrossRef]
- El Shater, R.; El-Ghazzawy, E.; El-Nimr, M.K. Study of the sintering temperature and the sintering time period effects on the structural and magnetic properties of M-type hexaferrite BaFe12O19. J. Alloys Compd. 2018, 739, 327–334. [Google Scholar] [CrossRef]
- Barrera, V.; Betancourt, I. Hard magnetic properties of nanosized Sr(Fe,Al)12O19 hexaferrites obtained by Pechini method. J. Phys. Chem. Solids 2016, 93, 1–6. [Google Scholar] [CrossRef]
- Lotgering, F.K. Topotactical reactions with ferrimagnetic oxides having hexagonal crystal structures—I. J. Inorg. Nucl. Chem. 1959, 9, 113–123. [Google Scholar] [CrossRef]
- Vijayan, H.; Povlsen, A.; Thomas-Hunt, J.; Mørch, M.I.; Christensen, M. Exploiting different morphologies of non-ferromagnetic interacting precursor’s for preparation of hexaferrite magnets. J. Alloys Compd. 2022, 915, 165333. [Google Scholar] [CrossRef]
- Vijiayan, H. Ultrathin nanoplatelets of six-line ferrihydrite enhances the magnetic properties of hexaferrite. Mater. Chem. Front. 2021, 5, 3699–3709. [Google Scholar] [CrossRef]
- Sakai, T.; Chen, Y.; Chinnasamy, C.N.; Vittoria, C.; Harris, V.G. Textured Sc-Doped Barium—Ferrite Compacts for Microwave Applications Below 20 GHz. IEEE Trans. Magn. 2006, 42, 3353–3355. [Google Scholar] [CrossRef]
- Chen, Y.; Fitchorov, T.; Gao, J.; Koblischka-Veneva, A.; Koblischka, M.R.; Vittoria, C.; Harris, V.G. Topochemical growth of textured polycrystalline barium hexaferrite from oriented antiferromagnetic α-FeOOH nanorods. Nanotechnology 2009, 20, 445606. [Google Scholar] [CrossRef]
- Wang, J.; Yang, A.; Chen, Y.; Chen, Z.; Geiler, A.; Gillette, S.M.; Harris, V.G.; Vittoria, C. Self biased Y-junction circulator at Ku band. IEEE Microw. Wirel. Compon. Lett. 2011, 21, 292–294. [Google Scholar] [CrossRef]
- Harris, V.G.; Sokolov, A.S. The Self-Biased Circulator: Ferrite Materials Design and Process Considerations. J. Supercond. Nov. Magn. 2019, 32, 97–108. [Google Scholar] [CrossRef]
- Chen, Y.; Geiler, A.L.; Sakai, T.; Yoon, S.D.; Vittoria, C.; Harris, V.G. Microwave and magnetic properties of self-biesed barium ferrite sreen printed thick films. J. Appl. Pysics 2006, 99, 08M904. [Google Scholar] [CrossRef]
- Andreev, S.; Bartashevich, M.; Pushkarskya, V.; Maltsev, V.; Pamyatnykh, L.; Tarasov, E.; Kudrevatykh, N.; Goto, T. Law of approach to saturation in highly anisotropic ferromagnets Application to Nd-Fe-B melt-spun ribbons. J. Alloys Compd. 1997, 260, 196–200. [Google Scholar] [CrossRef]
- Chen, D.X.; Brug, J.A.; Goldfarb, R.B. Demagnetizing factors for cylinders. IEEE Trans. Magn. 1991, 27, 3601–3619. [Google Scholar] [CrossRef]
- Petrícek, V.; Dušek, M.; Palatinus, L. Crystallographic computing system JANA2006: General features. Z. Krist. 2014, 229, 345–352. [Google Scholar] [CrossRef]
- Cudennec, Y.; Lecerf, A. Topotactic Transformations of Goethite and Lepidocrocite into Hematite and Maghemite. Solid State Sci. 2005, 7, 520–529. [Google Scholar] [CrossRef]
- Pankov, V.; Bartholdson, Å.; Stukalov, O.; Smolenchuk, S.; Babushkin, O.; Gremenok, V. Growth of BaFe12O19 Thin Films Formed by Reactive Diffusion. J. Cryst. Growth 2003, 252, 382–390. [Google Scholar] [CrossRef]
- Timofeev, A.V.; Kostishin, V.G.; Chitanov, D.N. The influence of power ferritization technology on the degree of magnetic structure in plates of BaFe12O19 and SrFe12O19 hexaferrites. Technical Physics Letters 2019, 45, 401–403. [Google Scholar] [CrossRef]
- Degen, T.; Sadki, M.; Bron, E.; König, U.; Nénert, G. The HighScore Suite. Powder Diffr. 2014, 29, S13–S18. [Google Scholar] [CrossRef]
- Cullity, B.D.; Stock, S.R. Elements of X-ray Diffraction; Prentice-Hall: Hoboken, NJ, USA, 2001; ISBN 0201610914. [Google Scholar]
- Uda, M. The Structure of synthetic Fe3S4 and the Nature of Transition to FeS Z. Anorg. Allg. Chem. 1967, 350, 105. [Google Scholar] [CrossRef]
- Capkova, P.; Valvoda, V. Preferred orientation in powder samples of magnesium and magnesium-cadmium alloys. Czech. J. Phys. 1974, 24, 891–900. [Google Scholar]
- Dollase, W.A. Correction of intensities for preferred orientation in powder diffractometry: Application of the March model. J. Appl. Crystallogr. 1986, 19, 267. [Google Scholar] [CrossRef]
- Zevin, L.; Kimmel, G. Quantitative X-ray Diffractometry; Springer: New York, NY, USA, 1995. [Google Scholar]
- Durst, K.D.; Kronmüller, H. Determination of intrinsic magnetic material parameters of Nd2Fe14B from magnetic measurements of sintered Nd15Fe77B8 magnets. J. Magn. Magn. Mater. 1986, 59, 86–94. [Google Scholar] [CrossRef]
- Kreisel, J.; Vincent, H.; Tasset, F.; Paté, M.; Ganne, J.P. An investigation of the magnetic anisotropy change in BaFe12-2xTixCoxO19 single crystals. J. Magn. Magn. Mater. 2001, 24, 17–29. [Google Scholar] [CrossRef]
- Smit, J.W. Ferrites: Physical Properties of Ferrimagnetic Oxydes in Relation to Their Technical Applications; Wiley: New York, NY, USA, 1959. [Google Scholar]
- Baker-Jarvis, J.; Janezic, M.D.; Riddle, B.F.; Johnk, R.T.; Kabos, P.; Holloway, C.L.; Geyer, R.G.; Grosvenor, C.A. Measuring the Permittivity and permeability of Lossy Materials: Solid, Metals, Building Materials, and Negative-Index Materials; U.S. Government Printing Office: Washington, DC, USA, 2004.
- Pucel, R.; Masse, D. Microstrip propagation on magnetic substrates—Part I: Design theory. IEEE Microw. Theory Tech. 1972, 20, 304–308. [Google Scholar] [CrossRef]
- Wan, C.; Hoorfar, A. Improved Design Equations for Multilayer Microstrip Lines. IEEE Microw. Guided Wave Lett. 2000, 10, 223–224. [Google Scholar]
- Pfeiffer, H. Determination of Anisotropy Field Distribution. Phys. Satus Soliidi A 1990, 118, 295–306. [Google Scholar] [CrossRef]
- Stoner, E.C.; Wolhfarth, E.P. A mecanism of hysteresis in heterogeneous alloys. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 1948, 840, 599–642. [Google Scholar]
- Grossinger, R. A Critical Examination of the Law of Approach to Saturation. Phys. Stat. Sol. 1981, 66, 665–674. [Google Scholar] [CrossRef]
- Néel, L.; Pautenet, R.; Rimet, G.; Giron, V. On the laws of magnetization of ferromagnetic single crystals and polycrystals. Application to uniaxial compounds. J. Appl. Phys. 1960, 31, S27–S29. [Google Scholar] [CrossRef]
- Herbst, J.; Pinkerton, F. Law of approach to saturation for polycrystalline ferromagnets: Remanent initial state. Phys. Rev. B Condens. Matter Mater. Phys. 1998, 57, 10733–10739. [Google Scholar] [CrossRef]
- Celasco, M.; Mazzeti, P. Saturation Approach Law for Grain-Oriented Polycrystalline Magnetic Materials. IEEE Trans. Magn. 1969, 5, 372–378. [Google Scholar] [CrossRef]
- Grössinger, R.; Sassik, H.; Holzer, D.; Pillmayr, N. Magnetic characterization of soft magnetic materials—Experiments and analysis. J. Magn. Magn. Mater. 2003, 254–255, 7–13. [Google Scholar] [CrossRef]
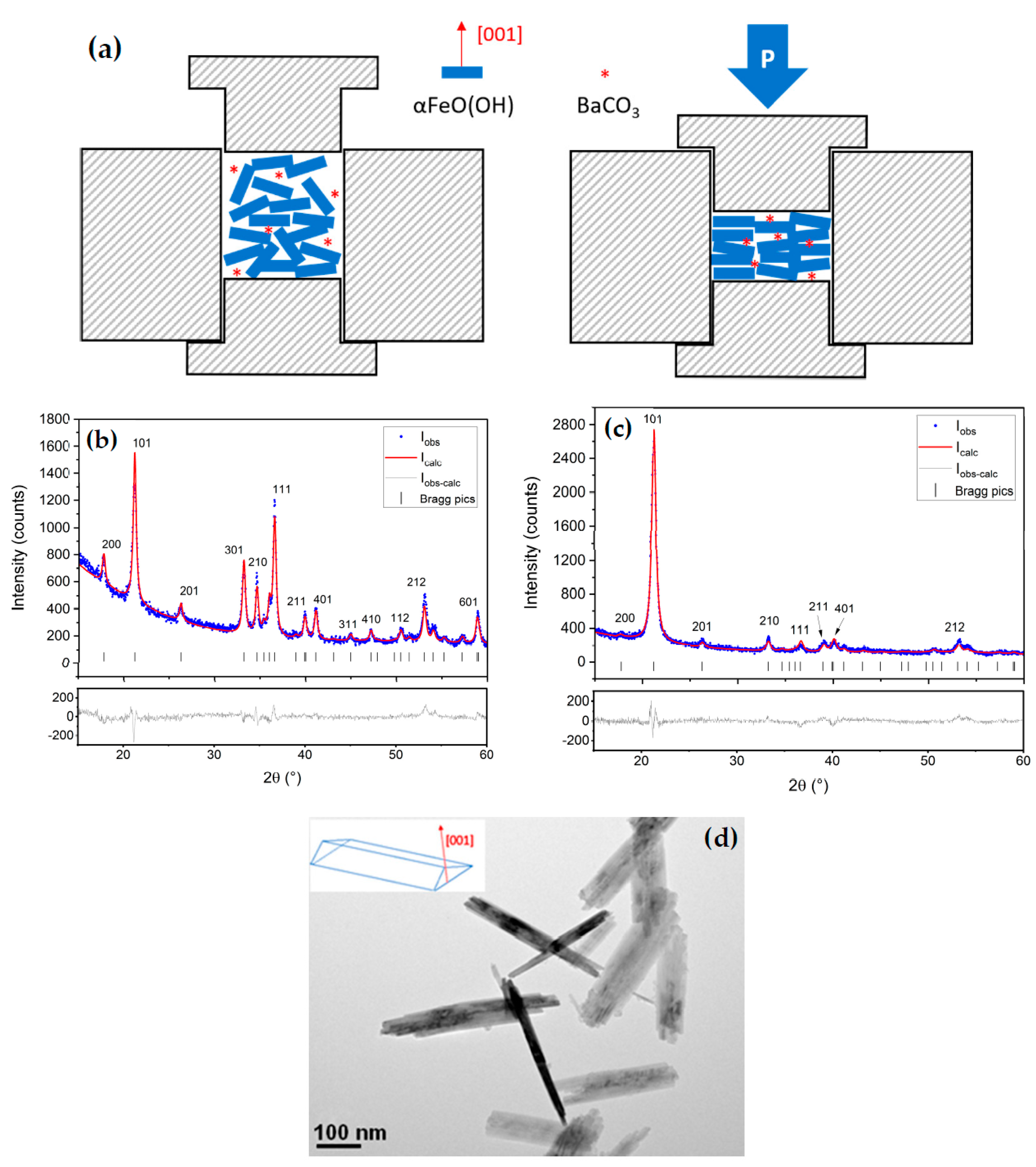
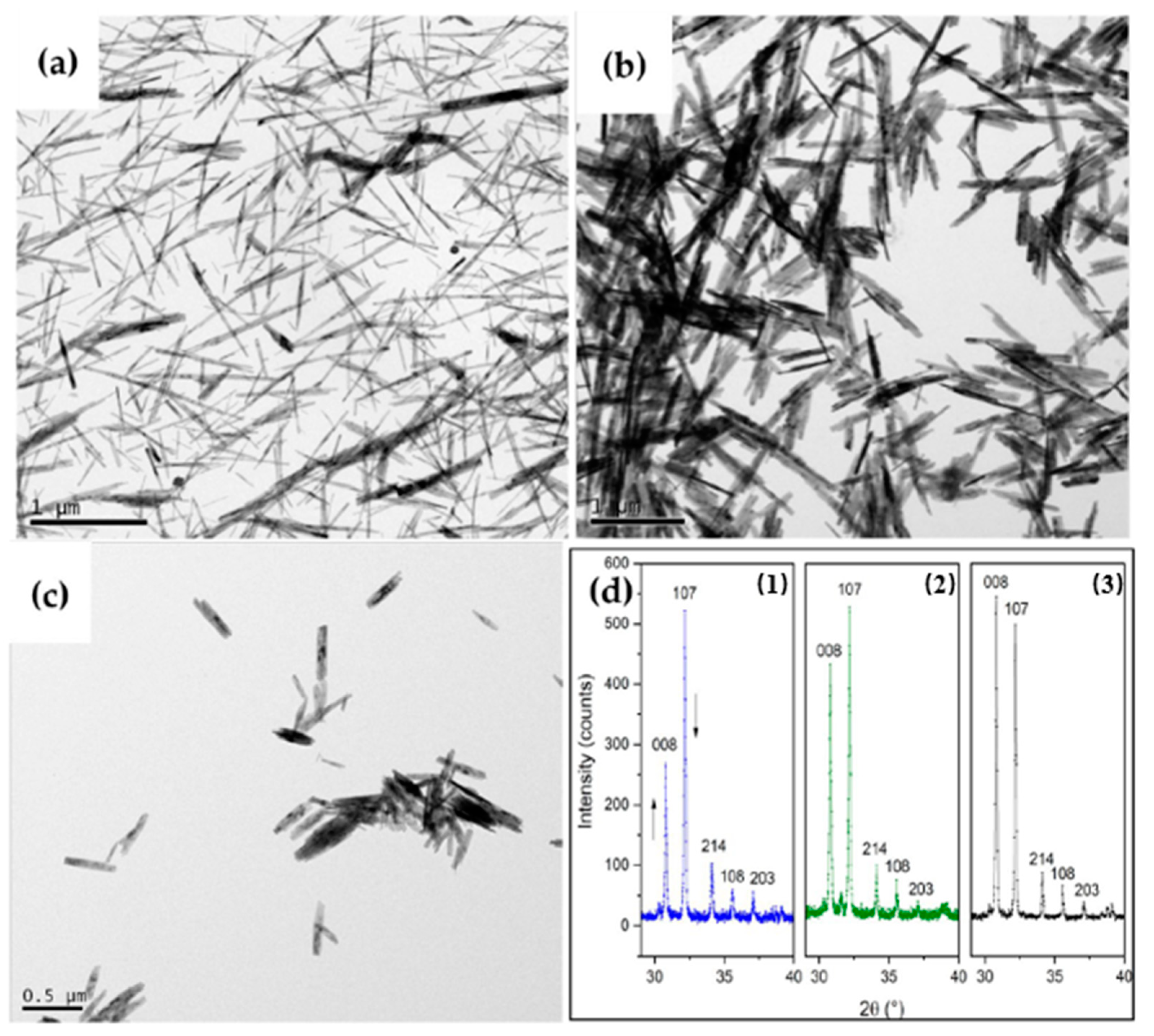
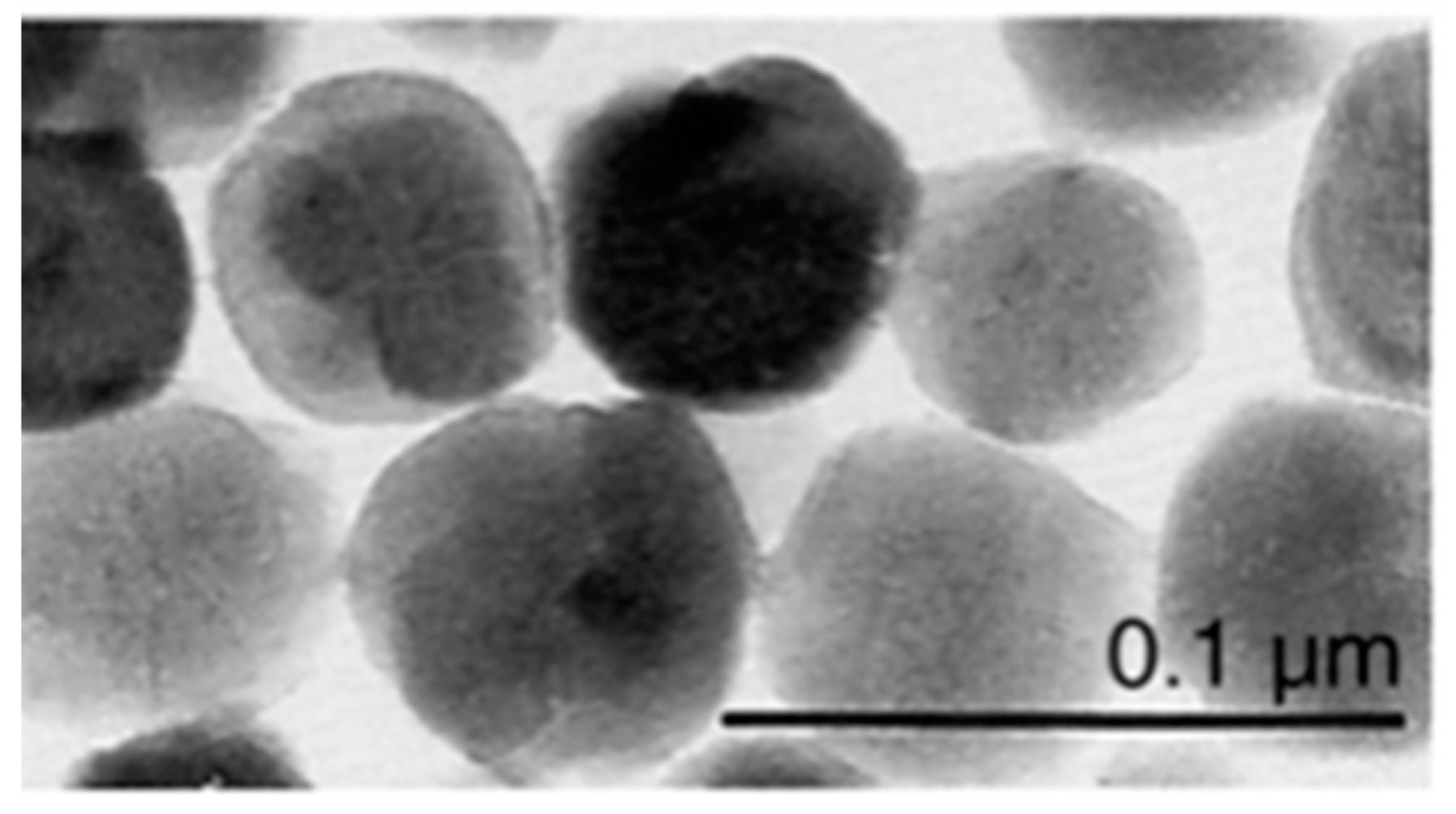

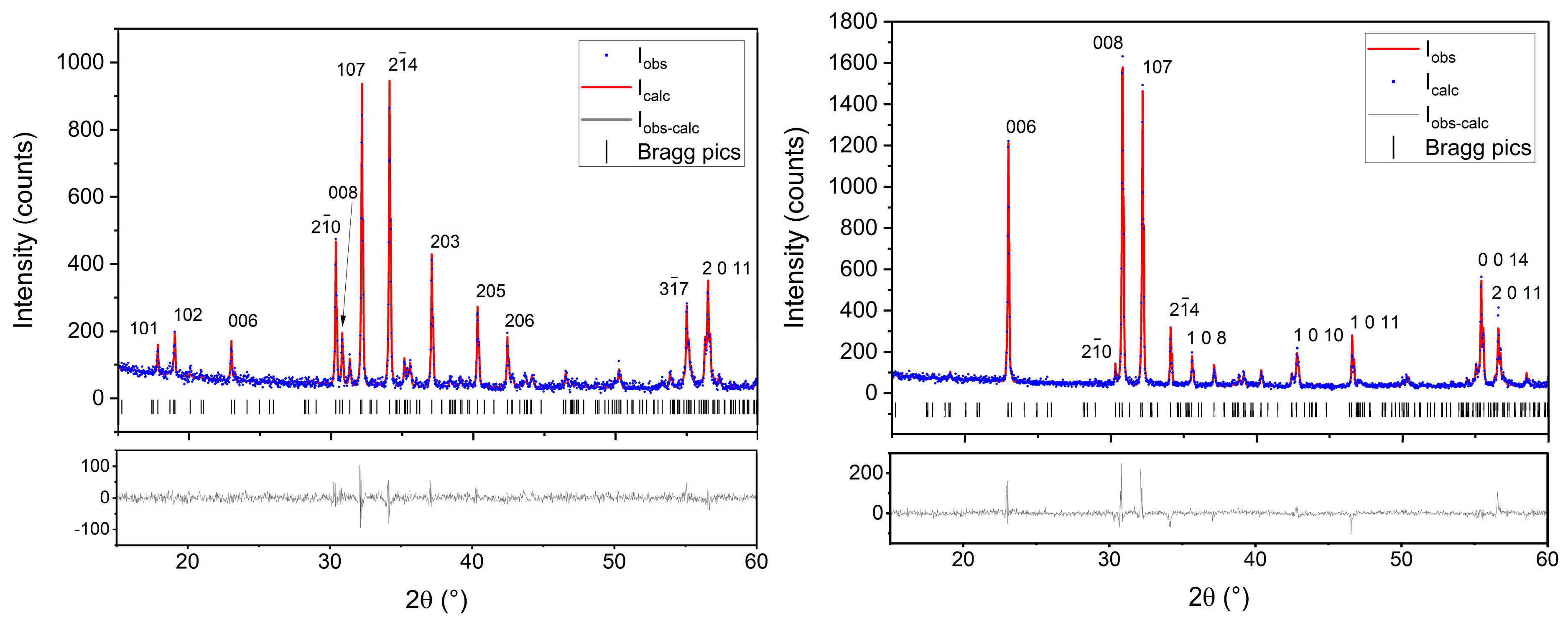


| Goethite before Compaction | Goethite after Compaction | |
|---|---|---|
| a, b, c (Å) | 9.9734(12), 3.0288(4), 4.6257(7) | 9.9740(6), 3.0255(15), 4.6151(8) |
| Space Group | Pnma | Pnma |
| March–Dollase r | 1 | 0.3997 |
| Robs, wRobs, Rall, wRall, GOF | 6.15, 6.53, 6.57, 6.54, 1.39 | 13.98, 13.15, 14.36, 13.16, 1.39 |
| Goethite | G1 | G2 | G3 |
|---|---|---|---|
| η | 0.06 | 0.15 | 0.2 |
| I(008)/I(107) | 0.5 | 0.8 | 1.2 |
| Goethite (% molar) | 0 | 5 | 10 | 20 |
| Hematite (% molar) | 100 | 95 | 90 | 80 |
| Notation in this paper | BaM-0 | BaM-5 | BaM-10 | BaM-20 |
| Goethite (% molar) | 30 | 40 | 50 | 60 |
| Hematite (% molar) | 70 | 60 | 50 | 40 |
| Notation in this paper | BaM-30 | BaM-40 | BaM-50 | BaM-60 |
| Goethite (% molar) | 70 | 80 | 90 | 100 |
| Hematite (% molar) | 30 | 20 | 10 | 0 |
| Notation in this paper | BaM-70 | BaM-80 | BaM-90 | BaM-100 |
| MS (kA/m) | MR// (kA/m) | MR///MS (kA/m) | MR///MR⊥ (kA/m) | α50 (°) | Auni(K1,ρ)/K12 | |
|---|---|---|---|---|---|---|
| BaM-0 | 304 | 166 | 0.55 | 1.06 | 154 | 0.33 |
| BaM-5 | 319 | 174 | 0.55 | 1 | 150 | 0.29 |
| BaM-10 | 308 | 173 | 0.56 | 0.98 | 128 | 0.29 |
| BaM-20 | 319 | 185 | 0.58 | 0.88 | 125 | 0.28 |
| BaM-30 | 317 | 200 | 0.63 | 0.83 | 123 | 0.24 |
| BaM-40 | 308 | 201 | 0.65 | 0.78 | 118 | 0.23 |
| BaM-50 | 308 | 203 | 0.66 | 0.69 | 105 | 0.21 |
| BaM-60 | 311 | 229 | 0.74 | 0.64 | 101 | 0.19 |
| BaM-70 | 313 | 230 | 0.73 | 0.57 | 93 | 0.17 |
| BaM-80 | 312 | 237 | 0.76 | 0.54 | 89 | 0.16 |
| BaM-90 | 303 | 242 | 0.8 | 0.46 | 87 | 0.12 |
| BaM-100 | 299 | 238 | 0.81 | 0.40 | 70 | 0.1 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hoëz, A.; Mattei, J.-L.; Chevalier, A. New Manufacturing Process for Granular Texture Management in Polycrystalline BaM Hexaferrites through the Goethite Crystallite Laths Aspect Ratio, and a Specialized Law of Approach to the Magnetic Saturation for Partly Polarized Uniaxial Materials. Magnetochemistry 2023, 9, 30. https://doi.org/10.3390/magnetochemistry9010030
Hoëz A, Mattei J-L, Chevalier A. New Manufacturing Process for Granular Texture Management in Polycrystalline BaM Hexaferrites through the Goethite Crystallite Laths Aspect Ratio, and a Specialized Law of Approach to the Magnetic Saturation for Partly Polarized Uniaxial Materials. Magnetochemistry. 2023; 9(1):30. https://doi.org/10.3390/magnetochemistry9010030
Chicago/Turabian StyleHoëz, Antoine, Jean-Luc Mattei, and Alexis Chevalier. 2023. "New Manufacturing Process for Granular Texture Management in Polycrystalline BaM Hexaferrites through the Goethite Crystallite Laths Aspect Ratio, and a Specialized Law of Approach to the Magnetic Saturation for Partly Polarized Uniaxial Materials" Magnetochemistry 9, no. 1: 30. https://doi.org/10.3390/magnetochemistry9010030
APA StyleHoëz, A., Mattei, J.-L., & Chevalier, A. (2023). New Manufacturing Process for Granular Texture Management in Polycrystalline BaM Hexaferrites through the Goethite Crystallite Laths Aspect Ratio, and a Specialized Law of Approach to the Magnetic Saturation for Partly Polarized Uniaxial Materials. Magnetochemistry, 9(1), 30. https://doi.org/10.3390/magnetochemistry9010030






