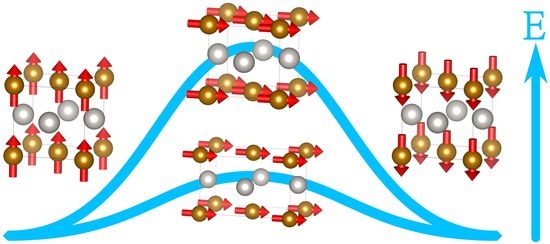
Magnetic Transition State Searching: Beyond the Static Ion Approximation
Abstract
1. Introduction
2. Theory
2.1. Magnetic Transition State Searching
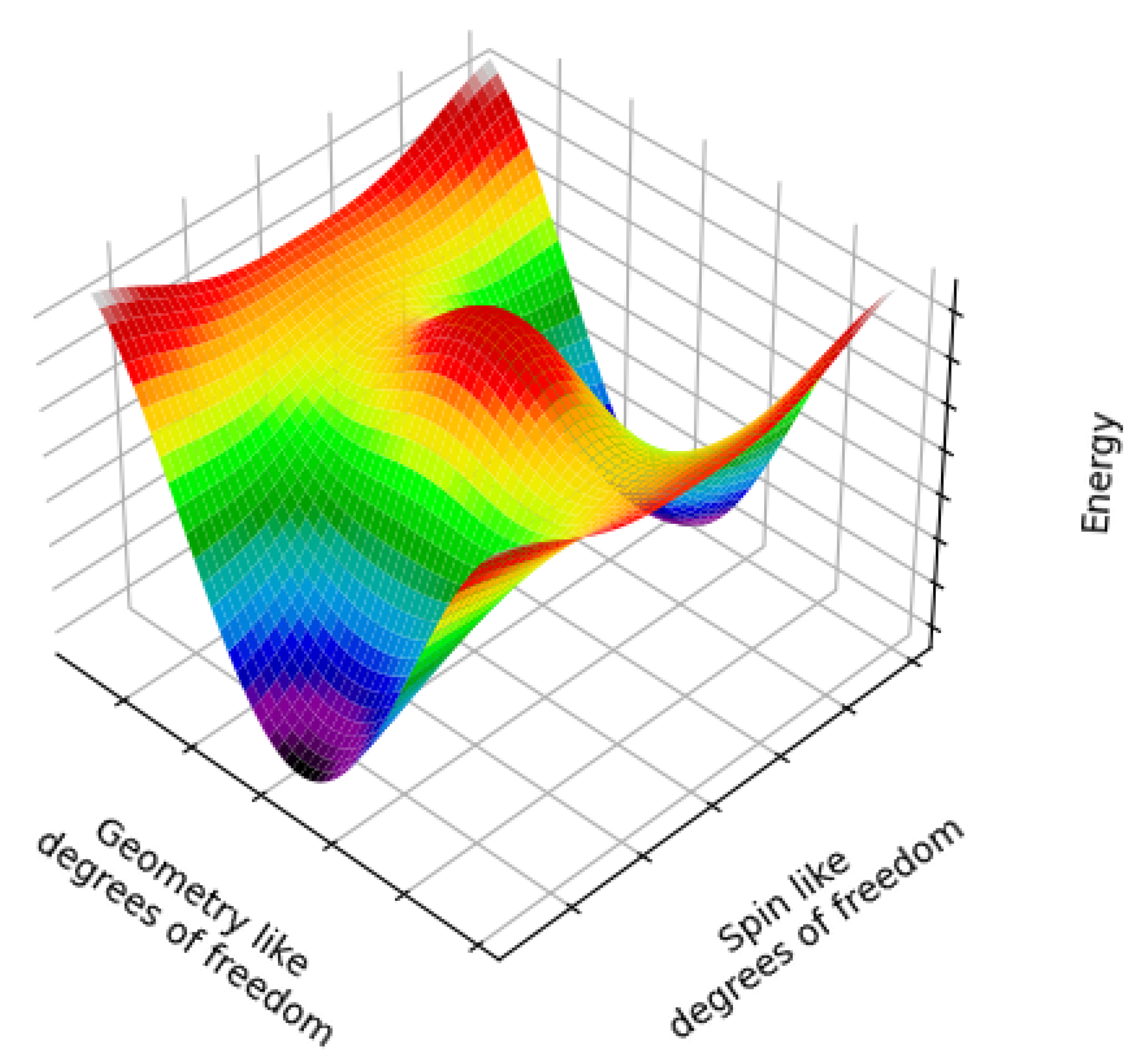
2.2. Quasiparticle Picture of Magnetic Anisotropy
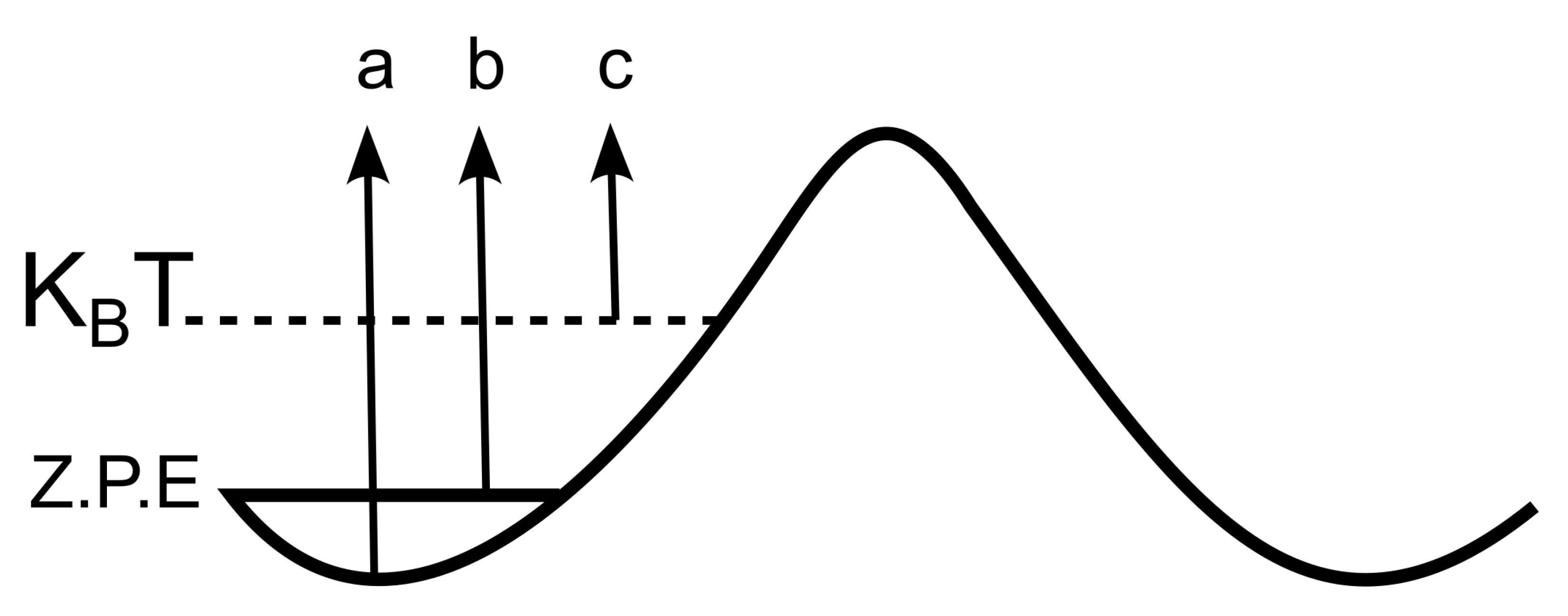
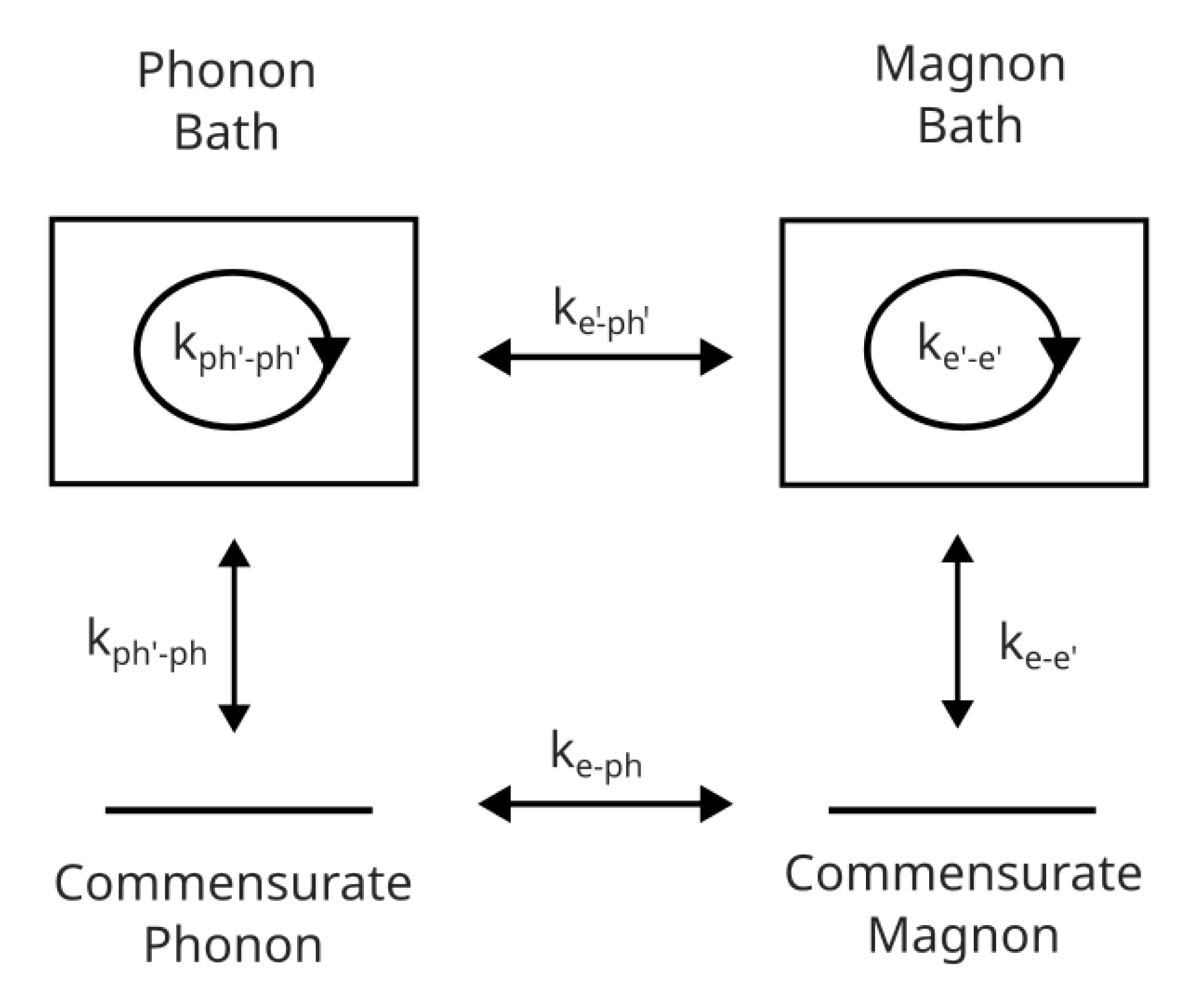
2.3. Attempt Frequency
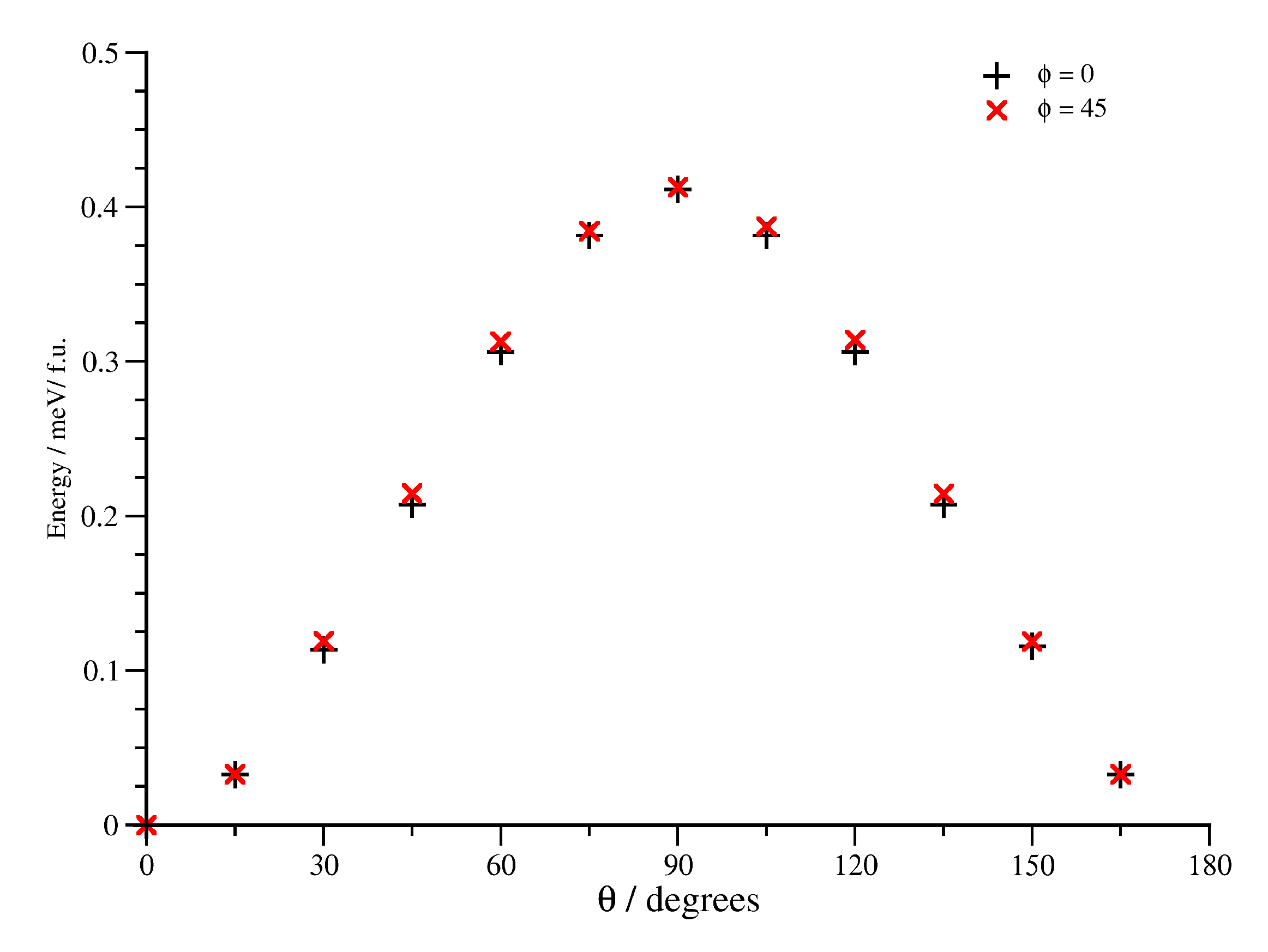
2.3.1. Symmetry Considerations
2.3.2. Conservation of Energy
2.4. Recap
- The MAE is the minimum energy barrier to transition between two magnetic states which correspond to easy axis alignment.
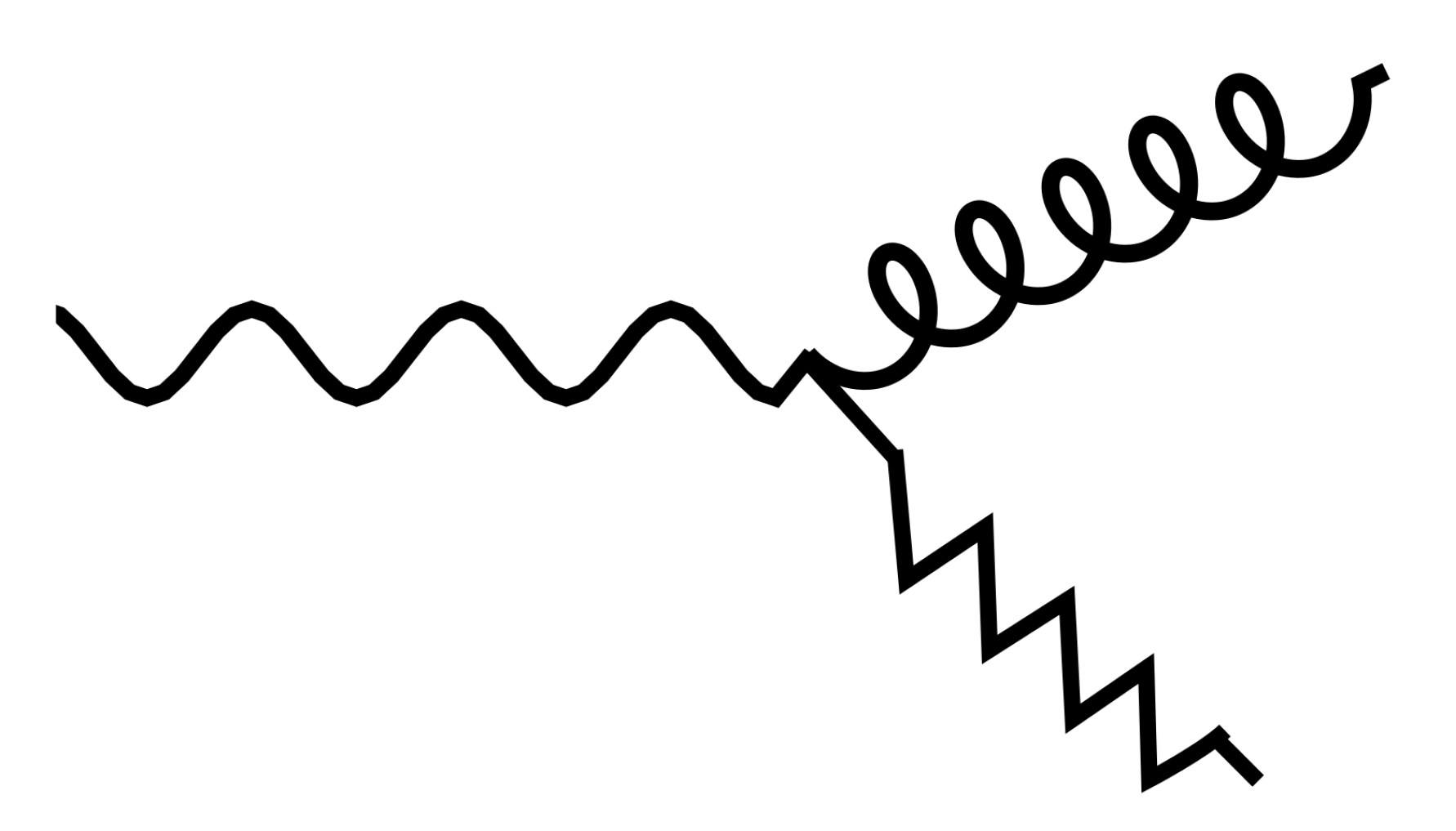
- This corresponds to the creation of a magnon mode, which may or may not be simultaneously coupled to a phonon mode (a magnetophonon).
- For FM materials, this phonon mode is a long-wavelength acoustic mode and consequently should only give a small correction to the MAE, but will generate magnetostriction.
- For AFM materials, this phonon mode is a long-wavelength optical mode, and consequently will provide a more significant change to the MAE due to the higher energy of the optical mode, and no magnetostriction.
- When the magnon–phonon coupling between these modes is strong, the MAE is represented by a magnetophonon that has a different energy to either the magnon or phonon when they are considered separately.
- Coupling to an optical rather than an acoustic mode has a three-orders of magnitude effect on the attempt frequency due to the difference in phonon lifetimes, leading to faster switching speeds for AFM-based devices.
3. Methodology
3.1. Density Functional Theory Parameters
Applied Constraints
3.2. Geometry Optimisation Parameters
4. Results and Discussion
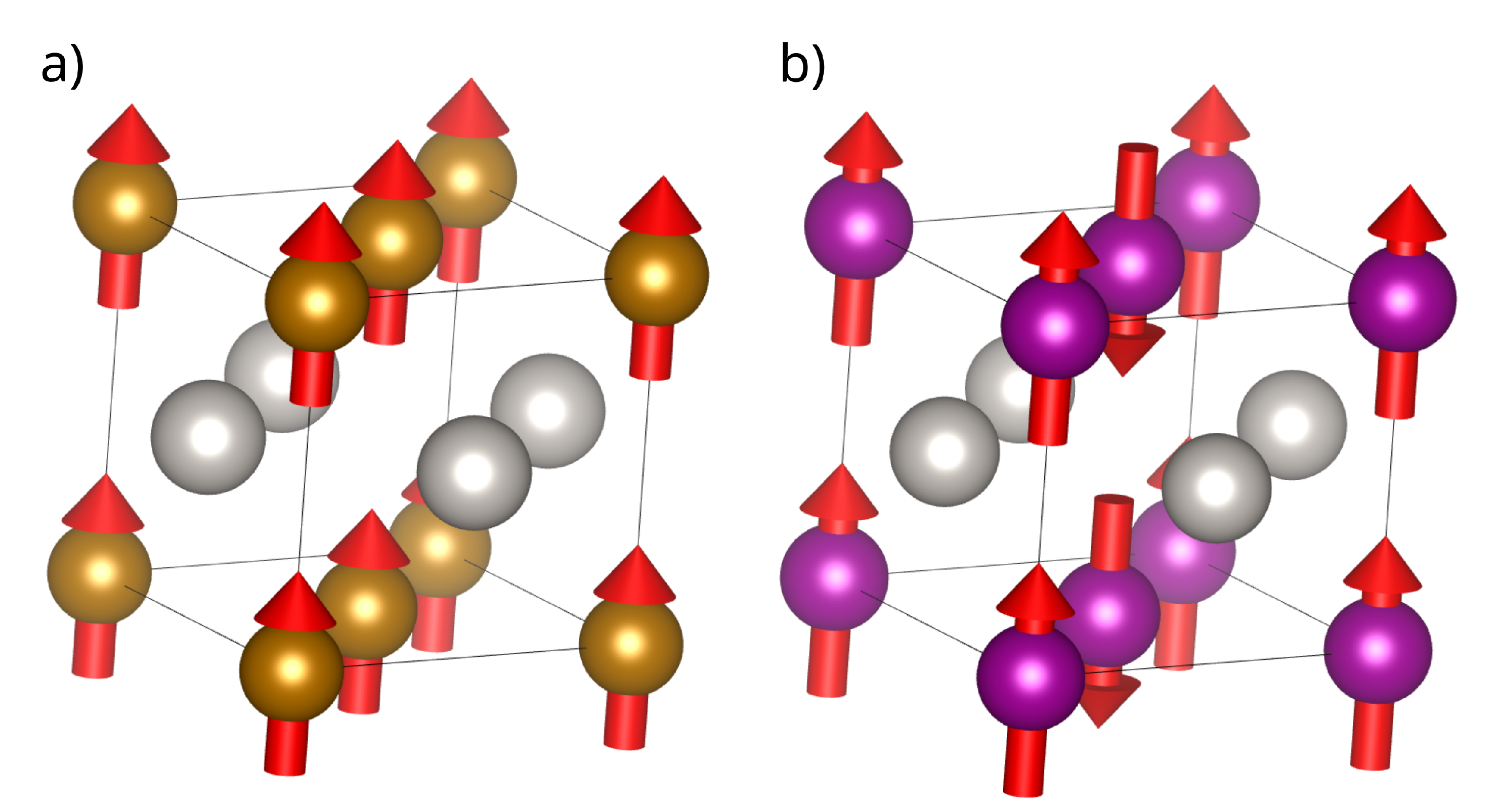
4.1. Materials
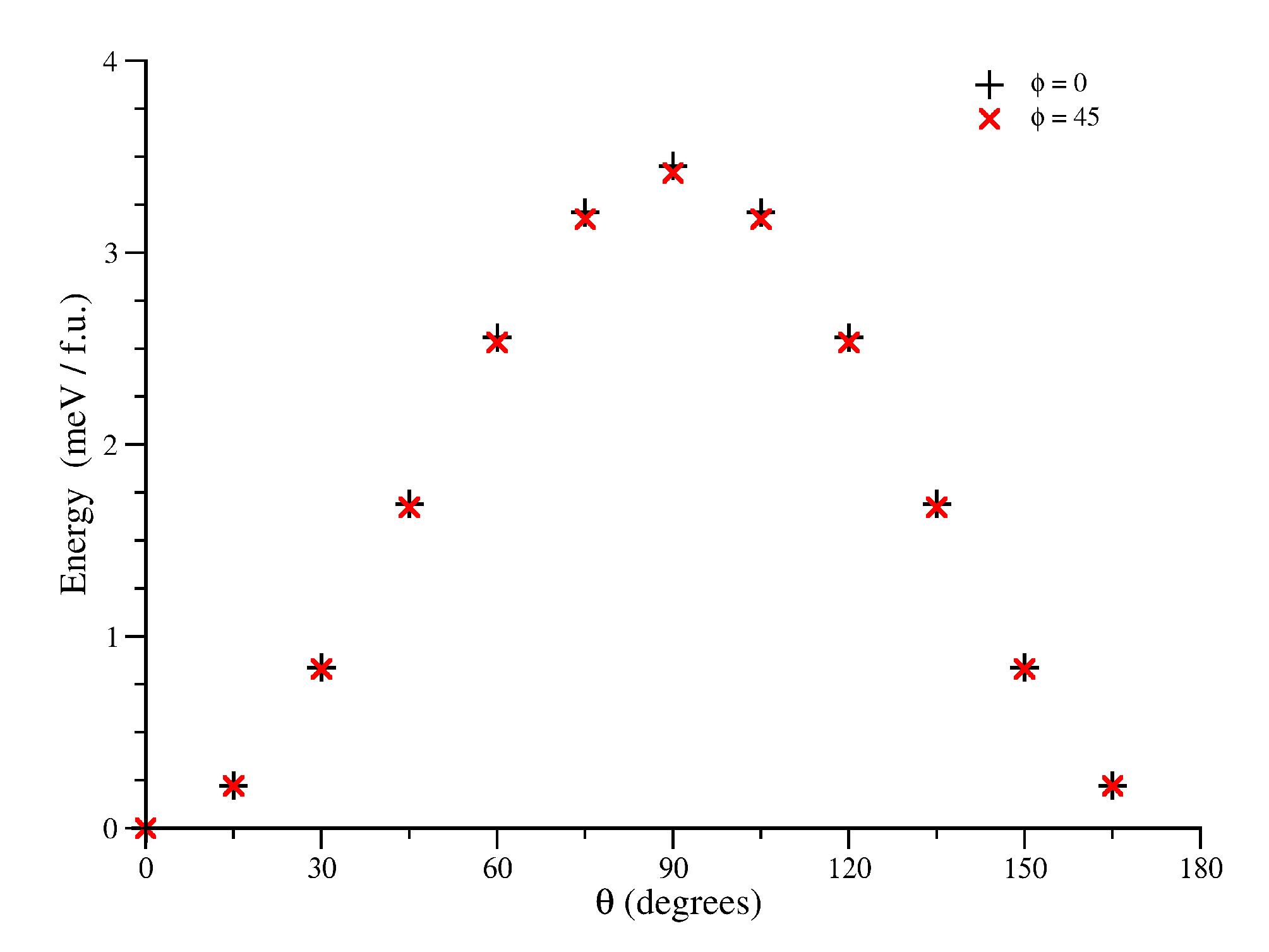
4.2. Magnetic-Only Transition State
4.2.1. FePt
4.2.2. PtMn
4.3. Magnetostructural Transition State
4.3.1. FePt
4.3.2. PtMn
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Mattioli, M. The Apollo Guidance Computer. IEEE Micro 2021, 41, 179–182. [Google Scholar] [CrossRef]
- Nordrum, A. The fight for the future of the disk drive. IEEE Spectr. 2019, 56, 44–47. [Google Scholar] [CrossRef]
- Wang, K.L.; Alzate, J.G.; Amiri, P.K. Low-power non-volatile spintronic memory: STT-RAM and beyond. J. Phys. D: Appl. Phys. 2013, 46, 074003. [Google Scholar] [CrossRef]
- Kosub, T.; Kopte, M.; Hühne, R.; Appel, P.; Shields, B.; Maletinsky, P.; Hübner, R.; Liedke, M.O.; Fassbender, J.; Schmidt, O.G.; et al. Purely antiferromagnetic magnetoelectric random access memory. Nat. Commun. 2017, 8, 13985. [Google Scholar] [CrossRef]
- Charap, S.; Lu, P.L.; He, Y. Thermal stability of recorded information at high densities. IEEE Trans. Magn. 1997, 33, 978–983. [Google Scholar] [CrossRef]
- Martins, A.; Trippe, S.; Santos, A.; Pelegrini, F. Spin-wave resonance and magnetic anisotropy in FePt thin films. J. Magn. Magn. Mater. 2007, 308, 120–125. [Google Scholar] [CrossRef]
- O’Grady, K.; Fernandez-Outon, L.; Vallejo-Fernandez, G. A new paradigm for exchange bias in polycrystalline thin films. J. Magn. Magn. Mater. 2010, 322, 883–899. [Google Scholar] [CrossRef]
- Shick, A.B.; Mryasov, O.N. Coulomb correlations and magnetic anisotropy in ordered L10 CoPt and FePt alloys. Phys. Rev. B 2003, 67, 172407. [Google Scholar] [CrossRef]
- Khan, S.A.; Blaha, P.; Ebert, H.; Minár, J.; Šipr, O. Magnetocrystalline anisotropy of FePt: A detailed view. Phys. Rev. B 2016, 94, 144436. [Google Scholar] [CrossRef]
- Nauman, M.; Kiem, D.H.; Lee, S.; Son, S.; Park, J.G.; Kang, W.; Han, M.J.; Jo, Y. Complete mapping of magnetic anisotropy for prototype Ising van der Waals FePS3. 2D Mater. 2021, 8, 035011. [Google Scholar] [CrossRef]
- Higgins, E.J.; Hasnip, P.J.; Probert, M.I. Simultaneous Prediction of the Magnetic and Crystal Structure of Materials Using a Genetic Algorithm. Crystals 2019, 9, 439. [Google Scholar] [CrossRef]
- Zhu, B.; Lu, Z.; Pickard, C.J.; Scanlon, D.O. Accelerating cathode material discovery through ab initio random structure searching. APL Mater. 2021, 9, 121111. [Google Scholar] [CrossRef]
- Burkert, T.; Eriksson, O.; Simak, S.I.; Ruban, A.V.; Sanyal, B.; Nordström, L.; Wills, J.M. Magnetic anisotropy of L10 FePt and Fe1-xMnxPt. Phys. Rev. B 2005, 71, 134411. [Google Scholar] [CrossRef]
- Shick, A.B.; Máca, F.; Lichtenstein, A.I. Magnetic anisotropy of single 3d spins on a CuN surface. Phys. Rev. B 2009, 79, 172409. [Google Scholar] [CrossRef]
- Jónsson, H.; Mills, G.; Jacobsen, K.W. Nudged elastic band method for finding minimum energy paths of transitions. In Classical and Quantum Dynamics in Condensed Phase Simulations: Proceedings of the International School of Physics, Lerici, Villa Marigola, 7–18 July 1997; Berne, B., Ciccoti, G., Coker, D.F., Eds.; World Scientific: Singapore, 1998. [Google Scholar] [CrossRef]
- Henkelman, G.; Uberuaga, B.P.; Jónsson, H. A climbing image nudged elastic band method for finding saddle points and minimum energy paths. J. Chem. Phys. 2000, 113, 9901–9904. [Google Scholar] [CrossRef]
- Halgren, T.A.; Lipscomb, W.N. The synchronous-transit method for determining reaction pathways and locating molecular transition states. Chem. Phys. Lett. 1977, 49, 225–232. [Google Scholar] [CrossRef]
- Peterson, A.A. Acceleration of saddle-point searches with machine learning. J. Chem. Phys. 2016, 145, 074106. [Google Scholar] [CrossRef] [PubMed]
- Hammond, G.S. A Correlation of Reaction Rates. J. Am. Chem. Soc. 1955, 77, 334–338. [Google Scholar] [CrossRef]
- Zhu, Y.A.; Dai, Y.C.; Chen, D.; Yuan, W.K. First-principles calculations of CH4 dissociation on Ni(100) surface along different reaction pathways. J. Mol. Catal. A: Chem. 2007, 264, 299–308. [Google Scholar] [CrossRef]
- Lechner, B.A.J.; Hedgeland, H.; Ellis, J.; Allison, W.; Sacchi, M.; Jenkins, S.J.; Hinch, B.J. Quantum Influences in the Diffusive Motion of Pyrrole on Cu(111). Angew. Chem. Int. Ed. 2013, 52, 5085–5088. [Google Scholar] [CrossRef]
- Rudin, S.P. Generalization of soft phonon modes. Phys. Rev. B 2018, 97, 134114. [Google Scholar] [CrossRef]
- Wilczek, F. Majorana returns. Nat. Phys. 2009, 5, 614–618. [Google Scholar] [CrossRef]
- Vallejo-Fernandez, G.; Aley, N.P.; Chapman, J.N.; O’Grady, K. Measurement of the attempt frequency in antiferromagnets. Appl. Phys. Lett. 2010, 97, 222505. [Google Scholar] [CrossRef]
- Roca, A.G.; Vallejo-Fernández, G.; O’Grady, K. An Analysis of Minor Hysteresis Loops of Nanoparticles for Hyperthermia. IEEE Trans. Magn. 2011, 47, 2878–2881. [Google Scholar] [CrossRef]
- Sharma, S.; Shallcross, S.; Elliott, P.; Dewhurst, J.K. Making a case for femto-phono-magnetism with FePt. Sci. Adv. 2022, 8. [Google Scholar] [CrossRef] [PubMed]
- Maldonado, P.; Carva, K.; Flammer, M.; Oppeneer, P.M. Theory of out-of-equilibrium ultrafast relaxation dynamics in metals. Phys. Rev. B 2017, 96, 174439. [Google Scholar] [CrossRef]
- Liu, S.; del Águila, A.G.; Bhowmick, D.; Gan, C.K.; Do, T.T.H.; Prosnikov, M.; Sedmidubský, D.; Sofer, Z.; Christianen, P.C.; Sengupta, P.; et al. Direct Observation of Magnon-Phonon Strong Coupling in Two-Dimensional Antiferromagnet at High Magnetic Fields. Phys. Rev. Lett. 2021, 127, 097401. [Google Scholar] [CrossRef]
- fan Qi, S.; Jing, J. Magnon-assisted photon-phonon conversion in the presence of structured environments. Phys. Rev. A 2021, 103, 043704. [Google Scholar] [CrossRef]
- Aguilar, R.V.; Sushkov, A.B.; Zhang, C.L.; Choi, Y.J.; Cheong, S.W.; Drew, H.D. Colossal magnon-phonon coupling in multiferroic Eu0.75Y0.25MnO3. Phys. Rev. B 2007, 76, 060404. [Google Scholar] [CrossRef]
- Man, H.; Shi, Z.; Xu, G.; Xu, Y.; Chen, X.; Sullivan, S.; Zhou, J.; Xia, K.; Shi, J.; Dai, P. Direct observation of magnon-phonon coupling in yttrium iron garnet. Phys. Rev. B 2017, 96, 100406. [Google Scholar] [CrossRef]
- Shen, P.; Kim, S.K. Magnetic field control of topological magnon-polaron bands in two-dimensional ferromagnets. Phys. Rev. B 2020, 101, 125111. [Google Scholar] [CrossRef]
- Hohenberg, P.; Kohn, W. Inhomogeneous electron gas. Phys. Rev. 1964, 136, B864–B871. [Google Scholar] [CrossRef]
- Kohn, W.; Sham, L.J. Self-consistent equations including exchange and correlation effects. Phys. Rev. 1965, 140, A1133–A1138. [Google Scholar] [CrossRef]
- Clark, S.J.; Segall, M.D.; Pickard, C.J.; Hasnip, P.J.; Probert, M.J.; Refson, K.; Payne, M. First principles methods using CASTEP. Z. Kristall. 2005, 220, 567–570. [Google Scholar] [CrossRef]
- Perdew, J.P.; Zunger, A. Self-interaction correction to density-functional approximations for many-electron systems. Phys. Rev. B 1981, 23, 5048–5079. [Google Scholar] [CrossRef]
- Scalmani, G.; Frisch, M.J. A New Approach to Noncollinear Spin Density Functional Theory beyond the Local Density Approximation. J. Chem. Theory Comput. 2012, 8, 2193–2196. [Google Scholar] [CrossRef]
- Monkhorst, H.J.; Pack, J.D. Special points for Brillouin-zone integrations. Phys. Rev. B 1976, 13, 5188–5192. [Google Scholar] [CrossRef]
- Cuadrado, R.; Pruneda, M.; García, A.; Ordejón, P. Implementation of non-collinear spin-constrained DFT calculations in SIESTA with a fully relativistic Hamiltonian. J. Phys. Mater. 2018, 1, 015010. [Google Scholar] [CrossRef]
- Laio, A.; Parrinello, M. Escaping free-energy minima. Proc. Natl. Acad. Sci. USA 2002, 99, 12562–12566. [Google Scholar] [CrossRef]
- Barreteau, C.; Spanjaard, D. Magnetic and electronic properties of bulk and clusters of FePt L10. J. Phys. Condens. Matter 2012, 24, 406004. [Google Scholar] [CrossRef]
- Aissat, D.; Baadji, N.; Mazouz, H.; Boussendel, A. Connection between lattice parameters and magnetocrystalline anisotropy in the case of L10 ordered antiferromagnetic MnPt. J. Magn. Magn. Mater. 2022, 563, 170013. [Google Scholar] [CrossRef]
- Klemmer, T.J.; Shukla, N.; Liu, C.; Wu, X.W.; Svedberg, E.B.; Mryasov, O.; Chantrell, R.W.; Weller, D.; Tanase, M.; Laughlin, D.E. Structural studies of L10 FePt nanoparticles. Appl. Phys. Lett. 2002, 81, 2220–2222. [Google Scholar] [CrossRef]
- Hervé, M.; Dupé, B.; Lopes, R.; Böttcher, M.; Martins, M.D.; Balashov, T.; Gerhard, L.; Sinova, J.; Wulfhekel, W. Stabilizing spin spirals and isolated skyrmions at low magnetic field exploiting vanishing magnetic anisotropy. Nat. Commun. 2018, 9, 1015. [Google Scholar] [CrossRef]
- Mao, S.; Gao, Z. Characterization of magnetic and thermal stability of PtMn spin valves. IEEE Trans. Magn. 2000, 36, 2860–2862. [Google Scholar] [CrossRef]
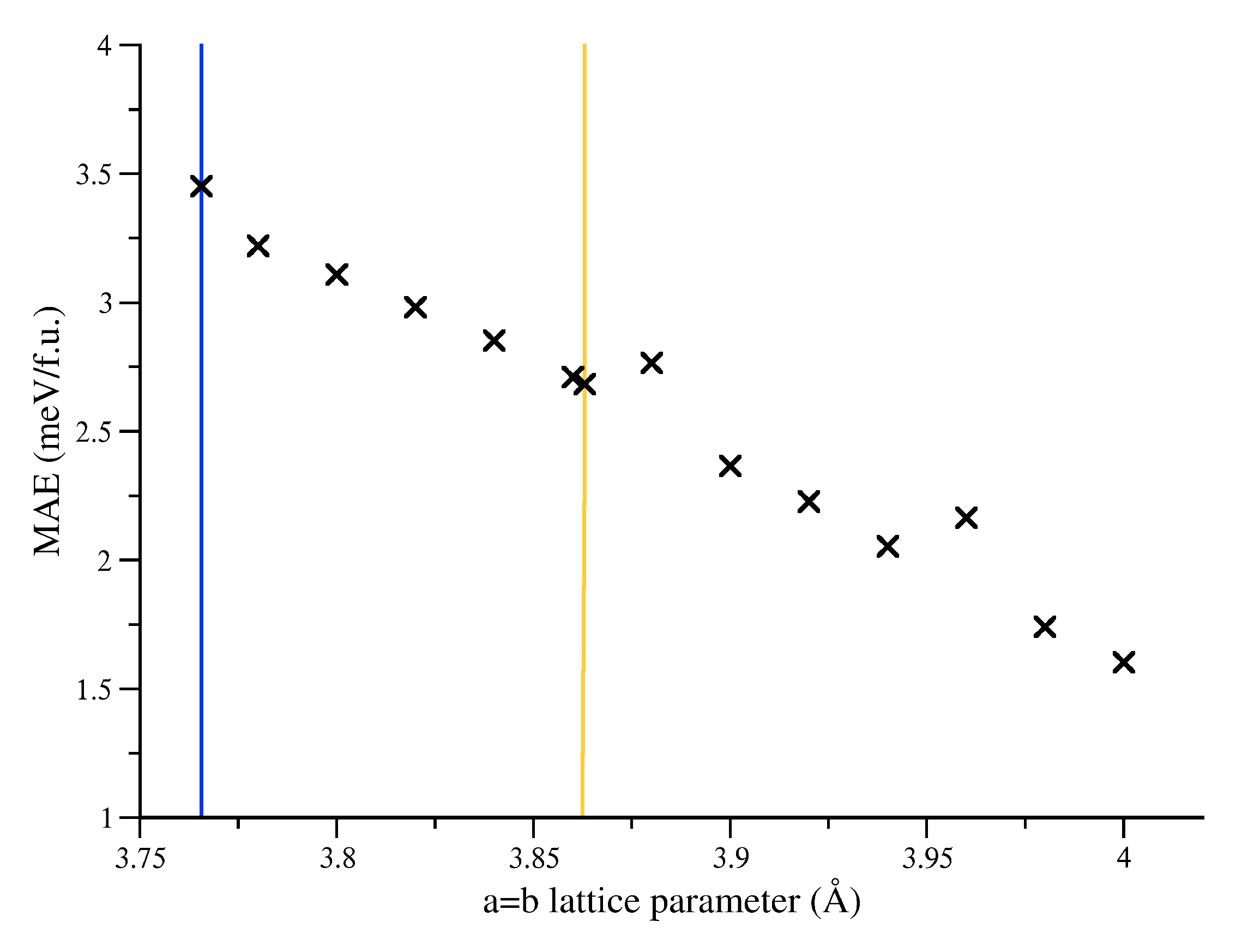

| Easy Geometry | 3.7656 | 3.7656 | 3.6841 |
| Hard Geometry | 3.7688 | 3.7644 | 3.6835 |
| LDA + U, Easy | 3.863 | 3.863 | 3.783 |
| Experimental [43] | 3.87 | 3.87 | 3.77 |
| Easy Geometry | Hard Geometry | |
|---|---|---|
| Easy Axis | 0 | 0.014 |
| Hard Axis | 3.453 | 3.439 |
| Easy Geometry | 3.8464 | 3.8464 | 3.6593 |
| Hard Geometry | 3.8464 | 3.8464 | 3.6593 |
| LDA + U, Easy | 4.025 | 4.025 | 3.665 |
| Experimental [45] | 3.96 | 3.96 | 3.73 |
| Easy | Fractional co-ordinates | ||
| Geometry | |||
| Pt 1 | 0.5 | 0.0 | 0.5 |
| Pt 2 | 0.0 | 0.5 | 0.5 |
| Mn 1 | 0.0 | 0.0 | 0.0 |
| Mn 2 | 0.5 | 0.5 | 0.0 |
| Hard | Fractional co-ordinates | ||
| Geometry | |||
| Pt 1 | 0.5 | 0.0 | 0.5008 |
| Pt 2 | 0.0 | 0.5 | 0.4992 |
| Mn 1 | 0.0 | 0.0 | 0.0 |
| Mn 2 | 0.5 | 0.5 | 0.0 |
| Easy Geometry | Hard Geometry | |
|---|---|---|
| Easy Axis | 0 | 0.0945 |
| Hard Axis | 0.4108 | 0.4108 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lawrence, R.A.; Donaldson, S.J.; Probert, M.I.J. Magnetic Transition State Searching: Beyond the Static Ion Approximation. Magnetochemistry 2023, 9, 42. https://doi.org/10.3390/magnetochemistry9020042
Lawrence RA, Donaldson SJ, Probert MIJ. Magnetic Transition State Searching: Beyond the Static Ion Approximation. Magnetochemistry. 2023; 9(2):42. https://doi.org/10.3390/magnetochemistry9020042
Chicago/Turabian StyleLawrence, Robert A., Scott J. Donaldson, and Matt I. J. Probert. 2023. "Magnetic Transition State Searching: Beyond the Static Ion Approximation" Magnetochemistry 9, no. 2: 42. https://doi.org/10.3390/magnetochemistry9020042
APA StyleLawrence, R. A., Donaldson, S. J., & Probert, M. I. J. (2023). Magnetic Transition State Searching: Beyond the Static Ion Approximation. Magnetochemistry, 9(2), 42. https://doi.org/10.3390/magnetochemistry9020042