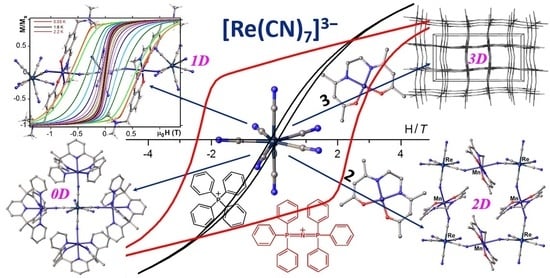
Application of the Heptacyanidorhenate(IV) as a Metalloligand in the Design of Molecular Magnets

Abstract

This review is devoted to an analysis of currently known heterometallic molecular magnets based on an orbitally degenerate 5d metalloligand, [Re^IV(CN)₇]³⁻. Heptacyanidometallates with a pentagonal bipyramidal structure of the coordination site and degenerate ground spin state are the source of anisotropic magnetic exchange interactions upon the formation of cyanide-bonded assemblies involving the paramagnetic complexes of the first transition series. Therefore, the development of methods for chemical design using such molecular magnetic modules is extremely important. If for the 4d congener, isoelectronic [Mo^III(CN)₇]³⁻, a family of approximately 40 heterometallic compounds, was obtained, whereas for heptacyanorhenate(IV), no more than 20 are known. However, as a result of recent studies, heterobimetallic magnetic assemblies of all dimensionalities have been synthesized, from 0D to 1D, demonstrating slow magnetization relaxation, to 2D networks and 3D frameworks possessing large magnetic hysteresis. The most anisotropic is a 2D network, PPN[{Mn^III(acacen)}₂Re^IV(CN)₇]·Solv, with a critical temperature of 20 K and magnetic hysteresis with a record coercivity for cyanide-bridged molecular materials.

1. Introduction

Orbitally degenerate coordination compounds of heavier d-metal ions with unquenched orbit angular momentum and a strong spin orbital interaction are promising in the design of highly anisotropic magnetic materials. It was first theoretically predicted that 4d/5d heptacyanidometallates are especially efficient in introducing strong anisotropy in heterometallic molecular systems [1,2], which was confirmed experimentally by preparation of a row of single-molecule magnets (SMMs) involved in these metalloligands [3,4,5,6,7,8]. Its main property is the absence of single-ion magnetic anisotropy owing to their low-spin ground state (S = 1/2). In this case, magnetic anisotropy is created cooperatively, together with bounded high-spin 3d complexes through pair-ion anisotropic exchange interactions.

In contrast to the relatively numerous cyano-bridged unidemensional magnetic assemblies comprising 3d centers [9,10,11,12,13], single-chain magnets (SCMs) including polycyanides of heavier d metals are less known [14,15,16,17,18,19]. Among them, chains involving low-spin (S = 1/2), orbitally degenerate cyanidometallates are extremely rare, with only a few SCMs comprising Fe^III [20,21,22,23] and Os^III cyanides [14] and five 1D coordination polymers involving pentagonal bipyramidal (PBP) [Mo(CN)₇]⁴⁻ (4d³) [8,24,25], only three of which are SCMs. The only known 2D anionic network is a compound, K₂Mn₃(H₂O)₆[Mo(CN)₇]₂·6H₂O [26]. In contrast, 3D heterobimetallic assemblies based on orbitally degenerate heavy d cyanidometallates are more common. Eleven bimetallic frameworks based on [Mo^III(CN)₇]⁴⁻ have been obtained [8], including five 3D polymers: one neutral material, i.e., Mn₂(H₂O)₅Mo(CN)₇·4H₂O [27] and four anionic complexes—[Mn(dpop)]₃[Mn(dpop)H₂O][Mo(CN)₇]₂·13.5H₂O or [Mn(dpop)]₂[Mo(CN)₇]·2H₂O [7], [Mn₂(pyrim)₂Mo(CN)₇]·2H₂O, and one involving V(II) [V₂(pyrim)₂Mo(CN)₇]·xH₂O [28].

Although heptacyanidorhenate (IV) (5d³) is much more resistant to light and atmospheric oxygen than its 4d congener, its heterobimetallic compounds are less studied. Furthermore, it should be especially noted that an absence of a large amount of [Re^IV(CN)₇]³⁻ assemblies with Mn^II ions possessing a maximum number of unpaired electrons for a 3d ion is associated with a reduction of Re^IV ions.

The aim of this report is to review all currently known heterometallic compounds involving an [Re^IV(CN)₇]³⁻ anion as a source of anisotropic coupling, which is extremely important in the design of high-performance, low-dimensional nanomagnets, as the use of heavier cyanidometallates has its benefits. First and foremost, versus their 3d congeners, they have higher anisotropy and more diffuse orbitals, offering stronger magnetic exchange interactions [5].

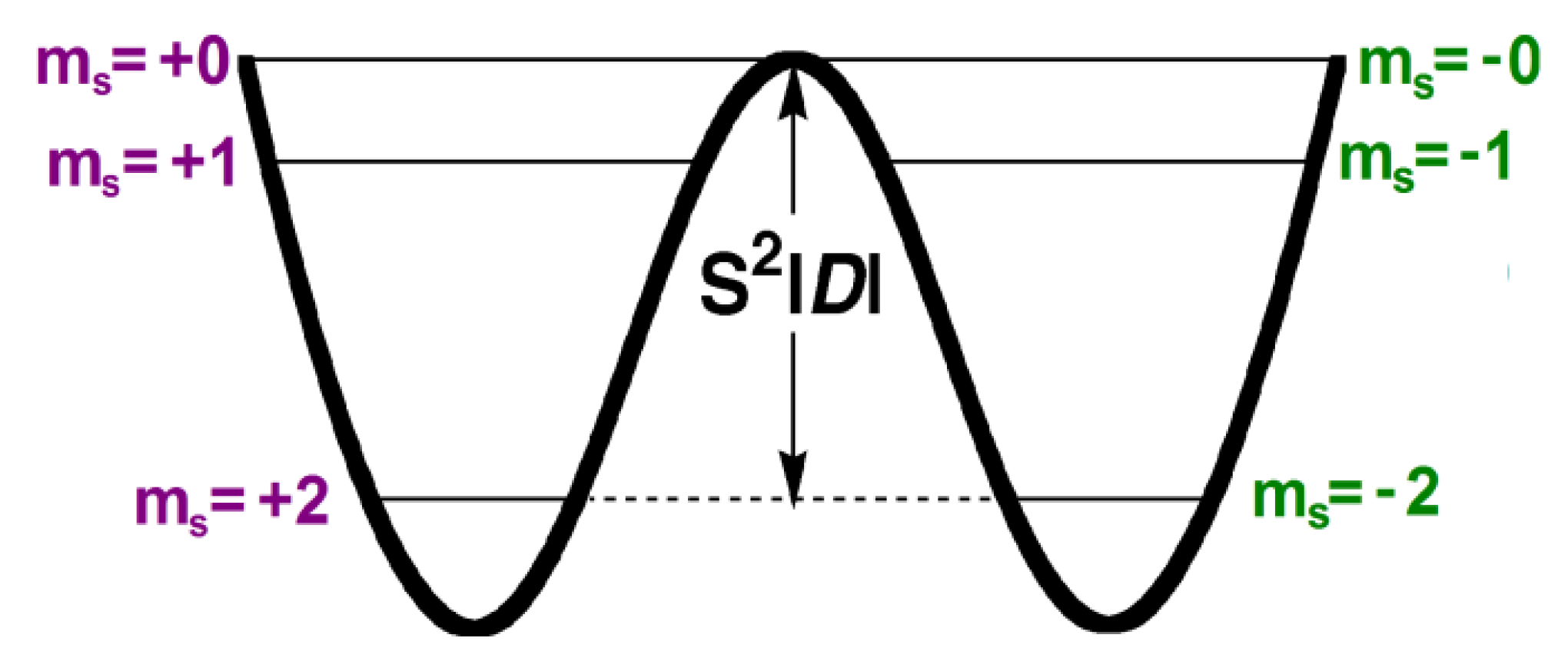
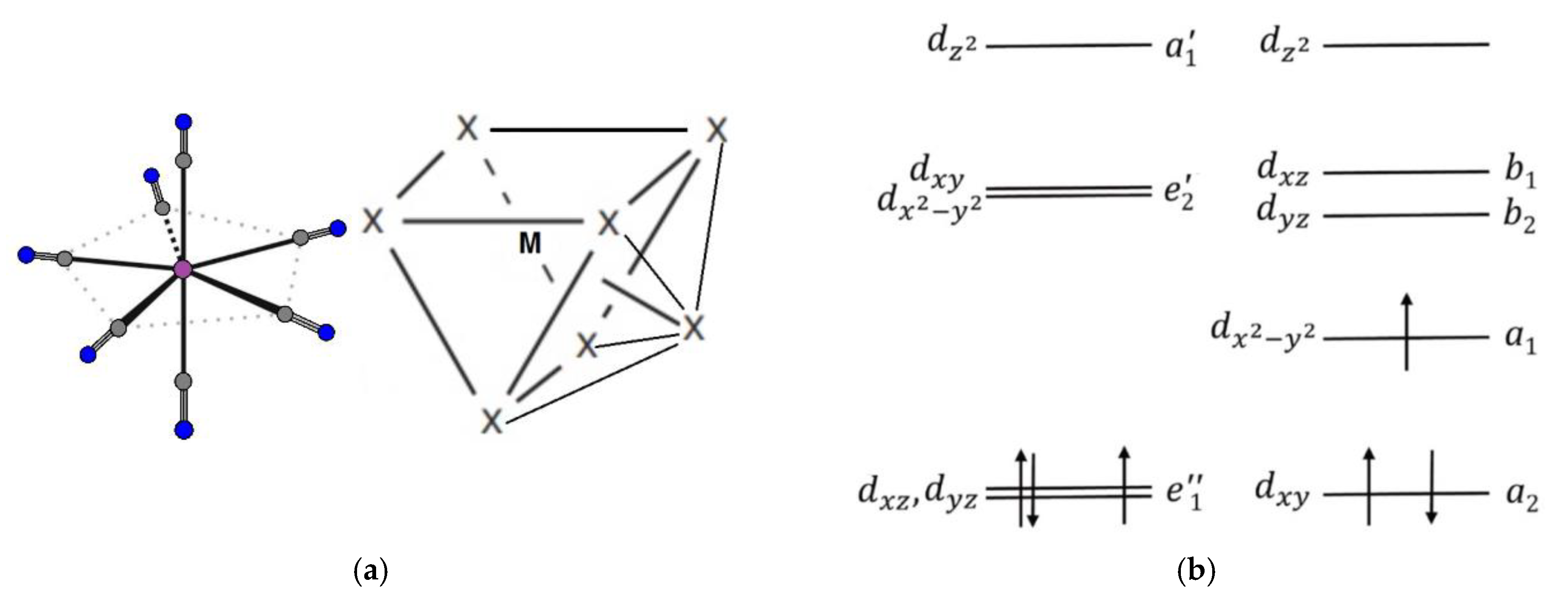
2. Theoretical Background

LD magnetic systems (0D and 1D) are molecular polynuclear complexes, which have a ground state with a high spin displaying slow relaxation of magnetization, in combination with a hysteresis loop under the blocking temperature (T_B). In SMMs, this takes place because of a bistability. The interaction between the spins is defined by a Hamiltonian, H = D[S_z²− S(S + 1)/3] + E(S_x²–S_y²), where D and E are the zero field-splitting (ZFS) constants [29,30]. When the axial parameter D is negative, the system potential is described by a double-well function (Scheme 1). For this reason, the two lowest quantum states, + M_s and −M_s, are separated by an anisotropy barrier (U_eff) to be overcome for magnetization reversing. The constant E denotes an axial distortion. Therefore, the barrier energy dependence includes a high-spin ground state (S) and magnetic anisotropy (D): U_eff = |D|S² and |D|(S² − 1/4) for integer and half-integer spins, respectively [29,30]. Note that in first-row transition metal complexes, ZFS is the most common source of magnetic anisotropy. The anisotropy constants are decisive in the processes of slow magnetic relaxation [31]. This situation relates to systems with uniaxial anisotropy (called Ising anisotropy), for which D should be large, while E should be as small as possible to prevent binding among m_s components. Such a coupling is responsible for tunneling between two energy equal states m_s = ±1.

The 1D coordination polymers are also able to display slow magnetic relaxation [32]. The SCMs take precedence over SMMs to obtain higher anisotropy barriers. This profit originates from an addendum in U_eff, Δ_ξ named correlation energy, the source of the latter being an exchange coupling (J) between the spins. Considering that a spin–spin coupling is −2JS₁·S₂, for an isotropic model (Ising limit), Δ_ξ = 4|J|S² when |D/J| > 4/3. In the case of |D| << |J| (Heisenberg limit)—Δ_ξ = 4S²|JD|^1/2 [10]. Then, the total spin–reversal barrier for an infinite magnetic wire is U_eff = Δ_A + 2Δ_ξ [33,34]. However, in a finite-length 1D polymer, U_eff = Δ_A + Δ_ξ owing to a nucleation effect of the chain ends [35].

If there are no long-range magnetic ordering at a certain temperature, the LD molecular compounds are capable to lock their magnetization after switching the field. This is due to slow magnetic relaxation going down T_B. In such a relaxation process, anisotropy plays a key role. For the majority octahedral complexes, there is no anisotropy for a perfect O_h symmetry. For the 3d complexes, a symmetry lowering through octahedron distortion is a source of magnetic anisotropy. However, it is difficult to rationally set such a deformation in complexes of Co²⁺, Mn³⁺, and Ni²⁺ coordination compounds, which exhibit anisotropy with |D|< 10 cm⁻¹ [30].

Heptacyanidometallates [M(CN)₇]ⁿ⁻ (M = Mo^III n = 4 [1,8,36] or Re^IV n = 3 [3,37,38]) represent the metalloligand with almost ideal PBP polyhedrons; such a geometry predetermines the exchange interactions with a greater degree of anisotropy with high-spin 3d metal complexes. This is due to the strong spin-orbit coupling (SOC) resulting from an unquenched orbital momentum (L) of PBP heptacyanides [1,2,39].

The heptacoordinated environment of the central atom in a homoleptic complex can be realized in two ways: a pentagonal bipyramid (D_5h) or a less symmetrical monocapped trigonal prism (C_2v). Various coordination holyhedral of the central ion lead to various ligand field-splitting diagrams and ground states with diverse electronic configurations (Scheme 2). For a metal at the center of a pentagonal bipyramid, the D_5h symmetry provides a doubly degenerated ²E₁-(e₁′′)³ electronic configuration to the ground state, whereas in the case of a monocapped triangular prism with C_2v symmetry, the ground configuration state is denoted as ²A₁-(a₂)²(a₁)¹. From the available crystallographic data for [Mo^III(CN)₇]⁴⁻based assemblies [8,40], it can be concluded that for LD compounds, the PBP geometry of the coordination polyhedron is realized; for 2D and 3D compounds, on the contrary, MCP polyhedrons are quite common. For Re^IV, the only case of MCP geometry of was observed in the 2D assembly of {(Mn(^5MeSalen))₆(H₂O)₂Re(CN)₇} clusters, and will be discussed in the final part of the current review.

3. Heterobimetallic Assemblies Based on Pentagonal Bipyramidal Tecton [Re^IV(CN)₇]³⁻

3.1. [Re^IV(CN)₇]³⁻ Metalloligand

Despite the fact that n-tetrabutylammonium heptacyanidorhenate(IV) (Scheme 2a) was first obtained 20 years ago [38], relatively few heterometallic compounds have been obtained with this metalloligand. The latter was synthesized by a ligand exchange reaction: (n-Bu₄N)₂[ReCl₆] + 7(n-Bu₄N)CN = (n-Bu₄N)₃[Re(CN)₇], 1. The physical properties of [Re(CN)₇]³⁻ are associated with the ²E₁′′ ground state of a PBP environment and a low-spin d³ electron configuration (µ_eff = 2.02 µ_B, g_av = 2.33). EPR study confirms the anisotropy of the g factor (g_‖ = 3.66 and g_⊥ = 1.59) displaying A_‖ = 129 G, a hyperfine splitting in the parallel component. The lowest in energy spin allowed a component of the e₁′′ →e₂′ transition of [Re^IV(CN)₇]³⁻, which is responsible for a peak at 25,300 cm⁻¹ (395 nm) in the electronic absorption spectrum of 1, whereas for [Mo(CN)₇]⁴⁻, a similar transition arises at 24,900 cm⁻¹ [40].

3.2. Reduction to [Re^III(CN)₇]³⁻ by M^II

Prolonged heating of an aqueous solution of 1 and MnCl₂ at 85 °C yielded the 3D polymer compound [fac-Mn(H₂O)₃][cis-Mn(H₂O)₂][Re(CN)₇]·3H₂O (2) [38], exhibiting a complicated polymorphic transform like to that found for [mer-Mn(H₂O)₃][cis-Mn(H₂O)₂][Mo(CN)₇]·4H₂O [27]. The use of noncoordinating oxidants to prevent rhenium reduction resulted in [Re^V(CN)₈]³⁻ formation.

The interaction of 1 with [(Py₅Me₂)Mn^II(MeCN)](PF₆)₂ (3) (S = ⁵/₂) in acetonitrile solution leads to a blue compound [(Py₅Me₂)₄Mn₄Re(CN)₇](PF₆)₅·6H₂O (4) [3]. Under ambient conditions, the reaction mixture color changes from blue to green, then to yellow, forming the once-reduced complex [(Py₅Me₂)₄Mn₄Re(CN)₇](PF₆)₄·10H₂O (5). Crystal structures show that the both molecules have a cross-like form, wherein four [(Py₅Me₂)Mn]²⁺ elements are attached to a central PBP [Re(CN)₇]^3−/4− anion (Figure 1).

Treatment of the dc (Figure 2a) and ac (Figure 3) data of 4 revealed ferromagnetic spin coupling between the metal ions, an S = ²¹/₂ ground state, with axial ZFS of D = −0.44 cm⁻¹, as the SMM parameters of 4: U_eff = 33 cm⁻¹and τ₀ = 2.4 × 10⁻⁸ s. Under ambient conditions, a one-electron reduction of Re ions occurred in 4, affording the paramagnetic complex 5 with four noninteracting Mn^II centers [3].

The reaction between the heptacyanidorhenate(IV) and [(Py₅Me₂)M(MeCN)]²⁺ (M = Co, Ni, Cu) yields a number of pentanuclear complex cations of the general formula [(PyY₅Me₂)₄M₄Re(CN)₇]⁵⁺ (M = Co (6), Ni (7), Cu (8)) and [(Py₅Me₂)₄Cu₄Re(CN)₇]⁴⁺ (9) [4]. The molecular structure of these heterometallic cations is similar to the cross-like organization of 4 and 5 (Figure 2a). Unlike complex 4, an intramolecular reduction process (Co^II → Re^IV) takes place during a formation of the Co^II₄Re^IV core resulting in a Co^II₃Co^IIIRe^III species. Nevertheless, for the Cu^II₄Re^IV moiety, a thermally induced reduction similar to 4 is also observed to afford a Cu^II₄Re^III moiety. However, such a reduction may be precluded at low temperatures. It should be noted that the one-electron reduction was registered during a slow diffusion of the reagents in the case of the reaction of (n-Bu₄N)₃[Re(CN)₇] with [Cu(cyclam)Cl₂] [41]. However, no reductive process was observed when using the nickel(II) complex [Ni(cyclam)Cl₂] as a high-spin partner [41].

A simulation of the χT·plots for the pentanuclear compounds (M^II = Mn, Ni, Cu) (Figure 2) was conducted using an exchange Hamiltonian of Ĥ = –2JŜ_Re (Ŝ_M(1) + Ŝ_M(2) + Ŝ_M(3) + Ŝ_M(4)) [3,4]. The magnetic measurement data demonstrate intramolecular ferromagnetic exchange interactions (Figure 2), high mono-axial anisotropy, and slow magnetization relaxation in the Ni^II₄Re^IV (Figure 3, inset) and Cu^II₄Re^IV molecular cations. Next, the SMM characteristics (U_eff = 17 cm⁻¹ and τ₀ = 1.4 × 10⁻⁷ s⁻¹) were extracted from an Arrhenius plot made for 9. Temperature dependencies of ac susceptibility measured on 8 also show a frequency-dependent signal at low temperatures, which prevented determination of the relaxation parameters [4].

3.3. Heterobimetallic Assemblies of [Re^IV(CN)₇]³⁻ and M^III Shiff Base Complexes

According to the above studies on the preparation of heterobimetallic assemblies involving divalent 3d metal ions, the latter can reduce Re(IV) to form a diamagnetic metalloligand [Re^III(CN)₇]⁴⁻. To avoid such a reduction process, it is necessary to use high-spin complexes containing 3d metal in a higher oxidation state than + 2. The most reasonable choice is to use Mn^III compounds with a spin ground state of S = 2. This choice is also suitable because to date, no whole family of Mn^III complexes with various N₂O₂ Schiff bases (SB), condensation products of diamines, and derivatives of either salicylaldehyde or acetylacetone has been synthesized and characterized [42,43]. Furthermore, the monocationic complexes [Mn(SB)]⁺ have been widely used in the design of cyanidometallate-based molecular magnetic materials [9].

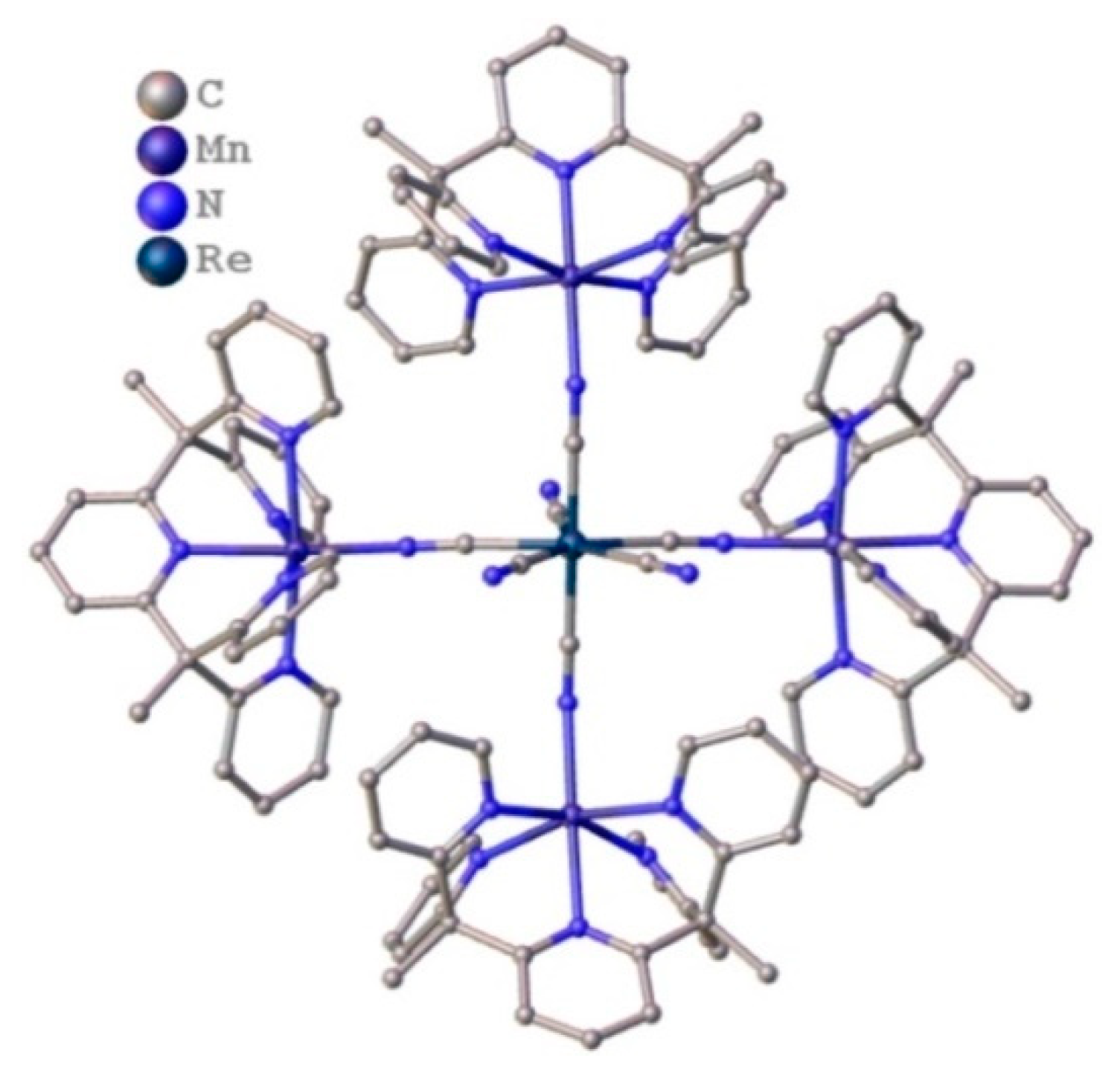
3.3.1. 3D Framework of [Mn(acacen)]₃[Re^III(CN)₇] (11)

Employment of the least utilized molecular magnet complex in the engineering of LD, Mn(acacen)Cl (acacen = N,N′-ethylenebis (acetylacetonylideneaminato)) (10), previously led to the preparation of anionic SCMs based on orbitally degenerate hexacyanidometallates (Cation)₂[Mn^III(acacen)M^III(CN)₆], M^III = Fe and Os, Cation = Et₄N⁺ for iron, and Ph₄P⁺ for iron and osmium [14,21]. Note that the mixing of [Mn(acacen)]⁺ (Scheme 3) with iron(III) cyanidometallate resulted in a family of heterobimetallic compounds of varying dimensionality (0D, 1D, and 2D), just changing the solvents and heating temperature and time [43]. However, the interaction of 1 with 10 in a ratio of 1:1 gave a 3D coordination polymer, i.e., [Mn(acacen)]₃[Re^III(CN)₇] (11), regardless of the solvent (MeOH or CH₂Cl₂) [44].

Crystal Structure of [Mn(acacen)]₃[Re^III(CN)₇]

To understand the nature of magnetism of 11, it is extremely important to analyze its crystal structure in more detail. The analysis of the crystal structure of 11 includes [Mn(acacen)]⁺ and [Re(CN)₇]³⁻ ions form a neutral 3D coordination polymer (Figure 1 and Figure 2) with an independent unit comprising six Mn^III and two Re^III centers. All Mn³⁺ centers have a distorted octahedral environment comprised of two O and two N atoms in the equatorial plane of the acacen²⁻ ligand and two N atoms of CN linkers in axial positions of [Re(CN)₇]³⁻ complexes. The Mn–N_CN distances in the range of 2.297(5)–2.388(5) Å, which is usual for Jahn–Teller distortion in Mn^III compounds, with each Re ion in a slightly distorted PBP environment. In fact, [Re(CN)₇]³⁻ is much less distorted than its 4d equivalent, [Mo(CN)₇]⁴⁻, in Mn₂(H₂O)₅Mo(CN)₇·4H₂O [27] and Mn₄L₄(H₂O)₂(Mo(CN)₇)₂ [45], but the distortion of the Re polyhedron of 11 is comparable with that of molybdenum in the Mn^II₂Mo^III framework [28]. The Re–C average distance is 2.106(19) Å, which is comparable to that which was established for (n-Bu₄N)₃[Re(CN)₇] (2.09(2) Å) [38]. Six cyanides of the [Re(CN)₇]³⁻ unit bond to six [Mn(acacen)]⁺ cations, making a framework with a distorted cubic topology (Figure 4 and Figure 5). The framework structure of 11 is a singular example of 3D heterobimetallic polymer constructed from a [Mn^IIISB]⁺ unit and a polycyanidometallate, which can be explained by the relatively small size of [Mn(acacen)]⁺ compared to the Mn^III complexes of salen-based SB and the larger size of the 5d metal cyanide complex. It is also probable that the PBP arrangement of the metalloligand is favorable for the formation of a 3D polymer. We found no published examples of the related bimetallic compounds based on octahedral cyanidometallates.

In order to interpret the magnetic behavior of a heterometallic system, it is useful to analyze the mutual arrangement of structural elements in the crystal of a compound under study. Thus, based on the structure of 11, it can be assumed that the direction along the b axis is an easy magnetization axis, as the apical axes, CN–Re–CN, are located in this direction, despite their small slope relative to each other (Figure 4). Owing to the pseudocubic structure of 11, all the Jahn–Teller axes of the Mn^III complexes in parallel Re–Mn–Re–Mn chains are also mutually inclined [44].

Because one of the cell angles is slightly greater than 90° and the space group is different from PῙ, the directions of the hard and easy magnetization axes do not coincide with other crystallographic axes, as illustrated in Figure 6 [44].

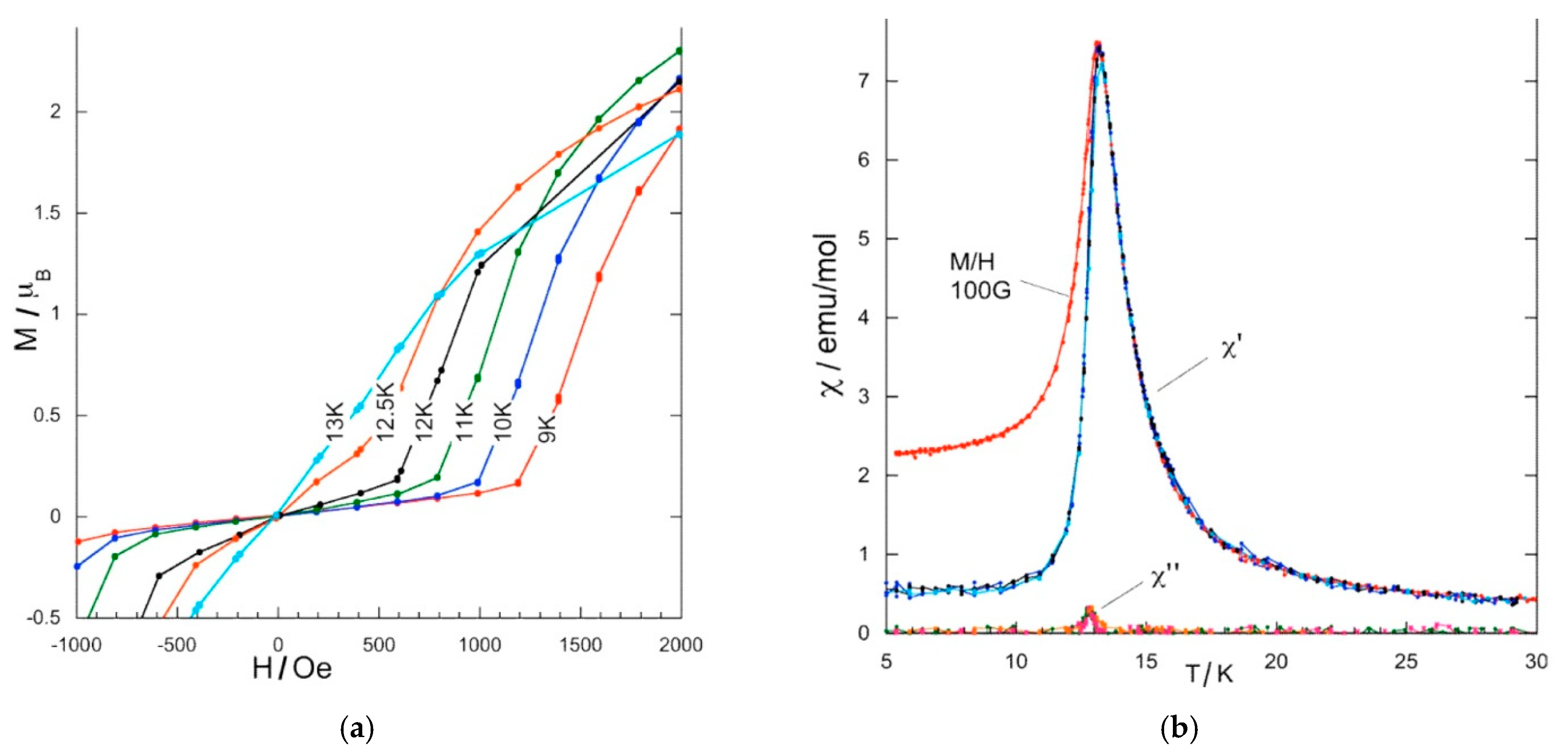
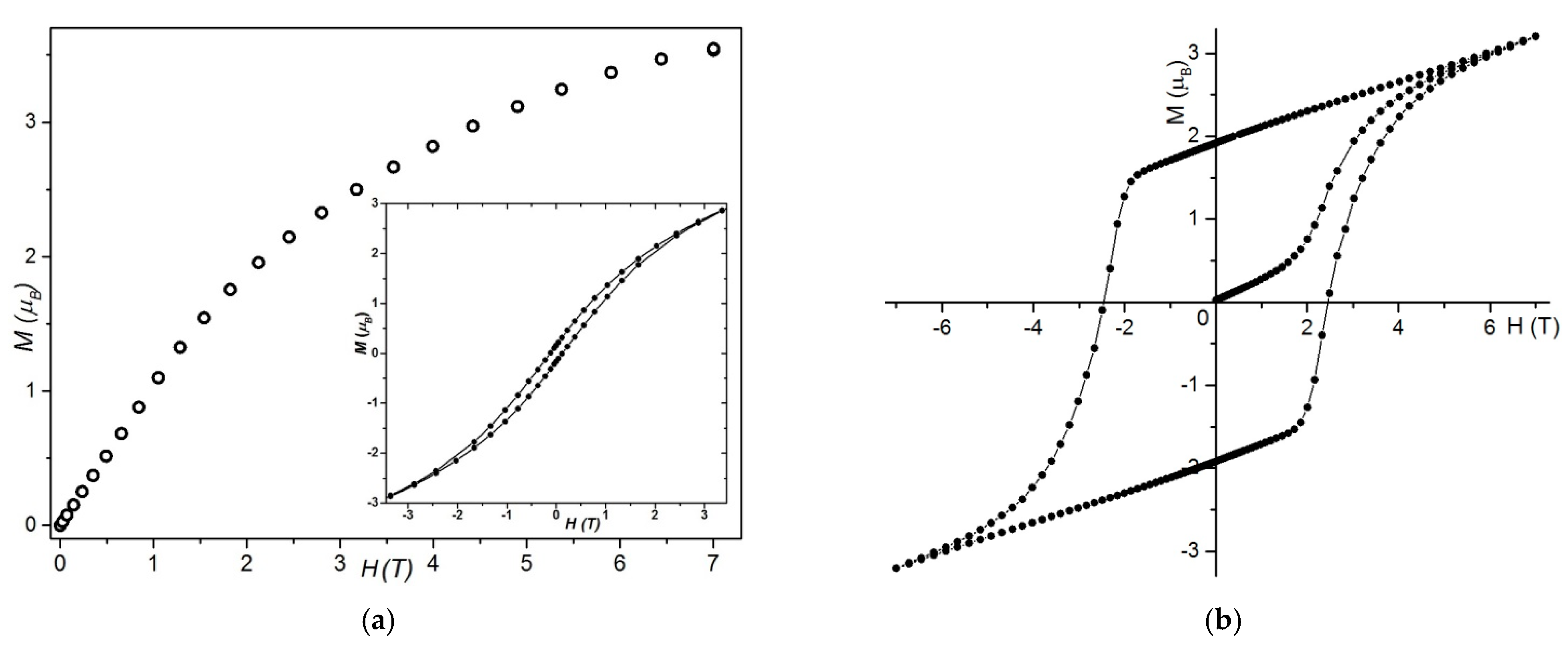
Magnetic Studies of Polycrystalline Samples

The high-temperature χT vs. T graphic of a polycrystalline 11 is presented in Figure 7. At 300 K, the observed value of 9.55 matches the theoretical value of 9.51 cm³ K mol⁻¹ for one Re^IV (S = 1/2, g = 2.33) [38] and three Mn^III (S = 2) uncoupled spins. Beginning from 300 K, the plot falls progressively and comes to a shallow minimum of 9 cm³·K·mol⁻¹ at 80 K (Figure 7, inset 1) [44]. Such a conduct of χT indicates ferrimagnetic interactions between the Mn^III and Re^IV ions. The data for the temperature region of 100–300 K shows that χ follows the Curie–Weiss law C = 9.86 cm³ K mol⁻¹ and with Θ = –9.8 K, confirming an entire antiferromagnetic character of exchange couplings in 11. Below 50 K, χT increases and achieves a sharp maximum at 13 K, attesting to a magnetic phase transition in 11. Analogous behavior was previously reported in a Mn₂(H₂O)₅Mo(CN)₇·4H₂O framework containing an isoelectronic PBP [Mo^III(CN)₇]⁴⁻ unit [27].

A metamagnetic transition in 11 occurs at 2 K in a field of approximately 5 kOe (see insert 2 of Figure 7). This comportment emerges at temperatures below 13 K, as evidenced by M vs. H plots collected at different temperatures (Figure 8a). The ac susceptibility data obtained for several frequencies do not confirm a frequency dependence (Figure 8b), suggesting a phase transition at the Néel temperature, i.e., T_N = 13 K, below which the framework becomes a metamagnet.

The ac data of 11 obtained in a field of 1 Oe for different frequencies do not demonstrate a frequency dependence (Figure 8b), with a small imaginary part (χ″) and showing only a pimple at the location of the χ′ peak. Such a phenomenon at 13 K is additional proof of a phase transition taking place. The dc magnetic data registered in a field of 100 Oe indicate that the maximum susceptibility occurs at a Néel temperature of T_N = 13 K_., below which the system exhibits metamagnetic behavior [44].

In spite of the absence of ferromagnetic order, hysteresis was observed for a powder of 11 at 200 mK (Figure 9a). The blue plot shows the initial magnetization, the latter with magnetization jumps or avalanches when the field attains a critical value of approximately 1.3 T and ~2 T; the hysteresis is closed only when the field is greater than 4 T [44]. The full hysteresis of 11 displays a very high coercive field of ~1 T. Furthermore, M does not saturate at 200 mK in fields up to 8 T and exhibits a linear dependence with high fields, which is typical for strongly anisotropic systems [44]. The maximum obtained value of M is ~8.8 µ_B. This is far from the theoretical expectation (13µ_B or 11µ_B for ferro- or antiferromagnetic interaction between the paramagnetic centers). Furthermore, at H = 0, M reaches ~3.6 µ_B, which is significantly less than half of the saturation value, attesting to uniaxial anisotropy. This suggests a spin canting arising from the noncollinearity of both the [Re(CN)₇]³⁻ apical axes and the Jahn–Teller [Mn(acacen)]⁺ axes caused by the pseudo-cubic organization of the 3D framework [44].

Magnetic Studies on a Single Crystal

To probe the anisotropic comportment of compound 11, a magnetic investigation was carried out on a single crystal. The hysteresis loops measured at 160 mK along the b (⊥010) and c (⊥100) directions, as well the initial magnetization curve for the c direction, are presented in Figure 9b [44]. The initial magnetization curve demonstrates two different fields with changes in magnetization at 4 and 6 T [44]. Alternatively, for the direction, b (⊥010), at 1 T a step of magnetization takes place, with M not reaching a saturation value at 8 T and 160 mK. This indicates that the spins are not reciprocal along this direction. The easy magnetization axis (b) coincides with a preferred direction of the apical axes of the [Re(CN)₇]³⁻ pentagonal bipyramids (Figure 4, Figure 5 and Figure 6), whereas the second orientation is in their equatorial plane (a or c) [44].

In summary, the magnetic investigation shows that the [Mn(acacen)]₃[Re^III(CN)₇] undergoes a magnetic order of a complex character below 13 K, exhibiting metamagnetism and strong anisotropy relative to those observed in Mn^II₂[Mo(CN)₇] frameworks, in which Mn²⁺ is magnetically isotropic. Further, we show that these unusual magnetic properties are due to an extremely sophisticated interplay of anisotropic spin coupling of Mn^III-CN-Re^IV linkages and single-ion ZFS anisotropy of [Mn(acacen)]⁺ complexes in the 3D network of 11 [44].

Theoretical Analysis of Anisotropic Spin Coupling in Re–CN–Mn Binding

The previously elaborated the superexchange theory to describe the anisotropic spin coupling between high-spin Mn^II ions (S = 5/2) cyanide bonded to [Mo^III(CN)₇]⁴⁻ with an ideal PBP polyhedron was used to find the exchange parameters in 11, as heptacyanidomolibdate(III) is isoelectronic to [Re(CN)₇]³⁻ (nd³-configuration) [1]. For the regular D_5h symmetry or slightly distorted PBP coordination polyhedron of the [Re(CN)₇]³⁻, the spin coupling in the bent and distorted Re–CN–Mn fragments is described by the same uniaxial anisotropic spin Hamiltonian, $-J_{xy}(S_{\mathrm{M}\mathrm{n}}^x{S}_{\mathrm{R}\mathrm{e}}^x+S_{\mathrm{M}\mathrm{n}}^y{S}_{\mathrm{R}\mathrm{e}}^y)-J_z{S}_{\mathrm{M}\mathrm{n}}^z{S}_{\mathrm{R}\mathrm{e}}^z$, independent of the local symmetry of [Mn^IIISB]⁺ complexes [44]. This means that the lowering of symmetry in Re–CN–Mn groups does not break up the total uniaxial symmetry of the spin Hamiltonian if the Re PBP remains ideal or only slightly distorted [44]. The numerical microscopic calculations of anisotropic exchange parameters J_z and J_xy for all Re–CN–Mn fragments in structure of 11 were performed in terms of a many-electron superexchange theory [44].

Consequently, it was demonstrated that in the case of a small distortion in the Re^IV bipyramid, L of [Re(CN)₇]³⁻ is not quenched, and the ground state wave functions are almost the same as those in an ideal D_5h polyhedron, as the splitting energy of ²Φ_± is much less than the spin-orbit splitting energy ≈ 2400 cm⁻¹ [44]. Hence, the application of the regular D_5h geometry of [Re(CN)₇]³⁻ is a satisfying approach in theoretical modeling of anisotropic spin coupling in compound 11. The dependence of J_z and J_xy on the C–N–Mn bond angle for all 12 nonequivalent Re–CN–Mn exchange-coupled pairs found in the crystal structure of 11 was calculated within the framework of the theory of many-electron superexchange [44]. The calculation results reveal a very complex interplay of anisotropic spin coupling between apical and equatorial Re–CN–Mn fragments in 11. Although in all pair, the anisotropic spin Hamiltonian (Equation (1)) maintains an axial symmetry with the anisotropy z axis parallel to a polar axis of the Re^IV PBP, J_z and J_xy parameters diverge significantly from pair to pair [44]. The exchange anisotropy for the apical pairs possesses a pronounced Ising-like character (|J_z|>|J_xy|), whereas the spin coupling in equatorial pairs is close to isotropic, with a small xy contribution [44].

$${\stackrel{⌢}{H}}_{\mathrm{e}\mathrm{f}\mathrm{f}}=-J_{xy}(S_{\mathrm{M}\mathrm{n}}^x{S}_{\mathrm{R}\mathrm{e}}^x+S_{\mathrm{M}\mathrm{n}}^y{S}_{\mathrm{R}\mathrm{e}}^y)-J_z{S}_{\mathrm{M}\mathrm{n}}^z{S}_{\mathrm{R}\mathrm{e}}^z$$

(1)

Using the largest parameters determined by microscopic calculations—J_z = −18 and J_xy = −8 cm⁻¹ for the apical Re–CN–Mn fragment and J_z = −19 and J_xy = −20 cm⁻¹ for the equatorial groups—sufficiently accurate agreement > 30 K was achieved between the experimental and simulated χT plots (D = −4.0 cm⁻¹, E = 0, and g_Mn = 2.03 for Mn^III were fixed), (Figure 10a), with the minimum in the χT graph around 75 K well-reproduced. The minimum is a result of a peculiar configuration of the theoretical spin-energy spectrum of 11 [44]. Uniaxial anisotropic antiferromagnetic spin coupling of alternating S_Re = 1/2 and S_Mn = 2 spins tends to stabilize collective spin states with large M_S projection on the polar z axis of the Re site (Figure 10b). In the middle energy range ~60 cm⁻¹, there is a low density of energy levels, which are represented by spin states with reduced M_S spin projection, in contrast to the upper spin states corresponding the high M_S projection (Figure 10b). Therefore, at low temperatures, the thermal population of low-lying spin states with large M_S results in a robust increase in susceptibility below 25 K, whereas the subsequent thermal population of the next excited spin states first results in a flat minimum at moderate temperatures and then a steady increase in χT at higher temperatures (Figure 10a) [44].

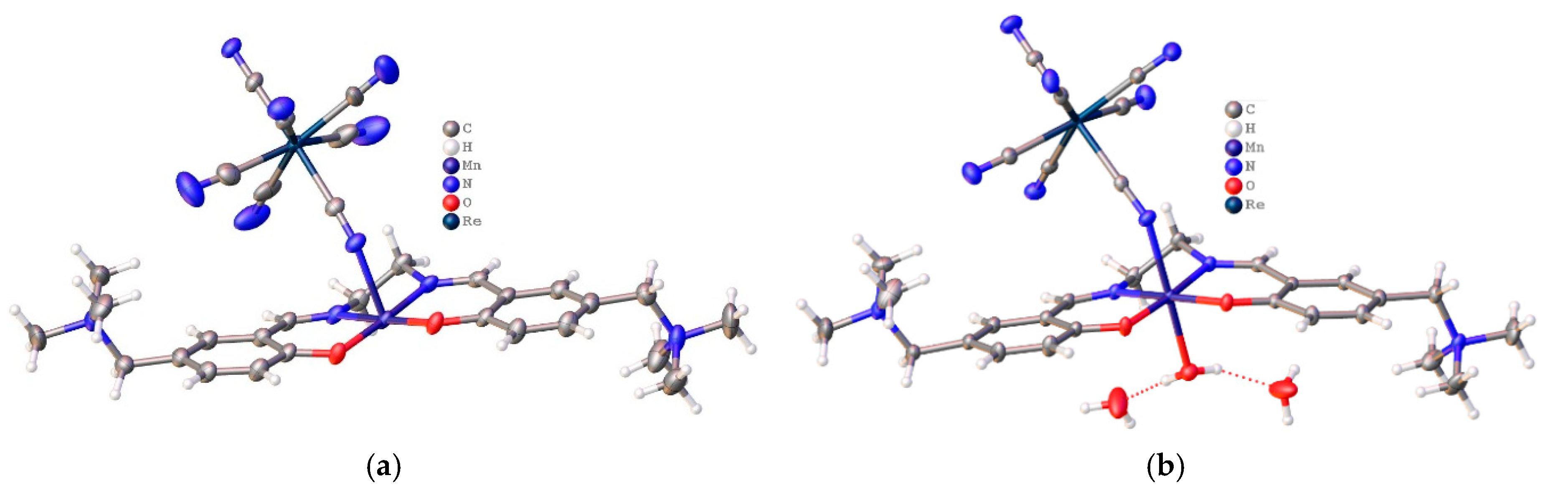
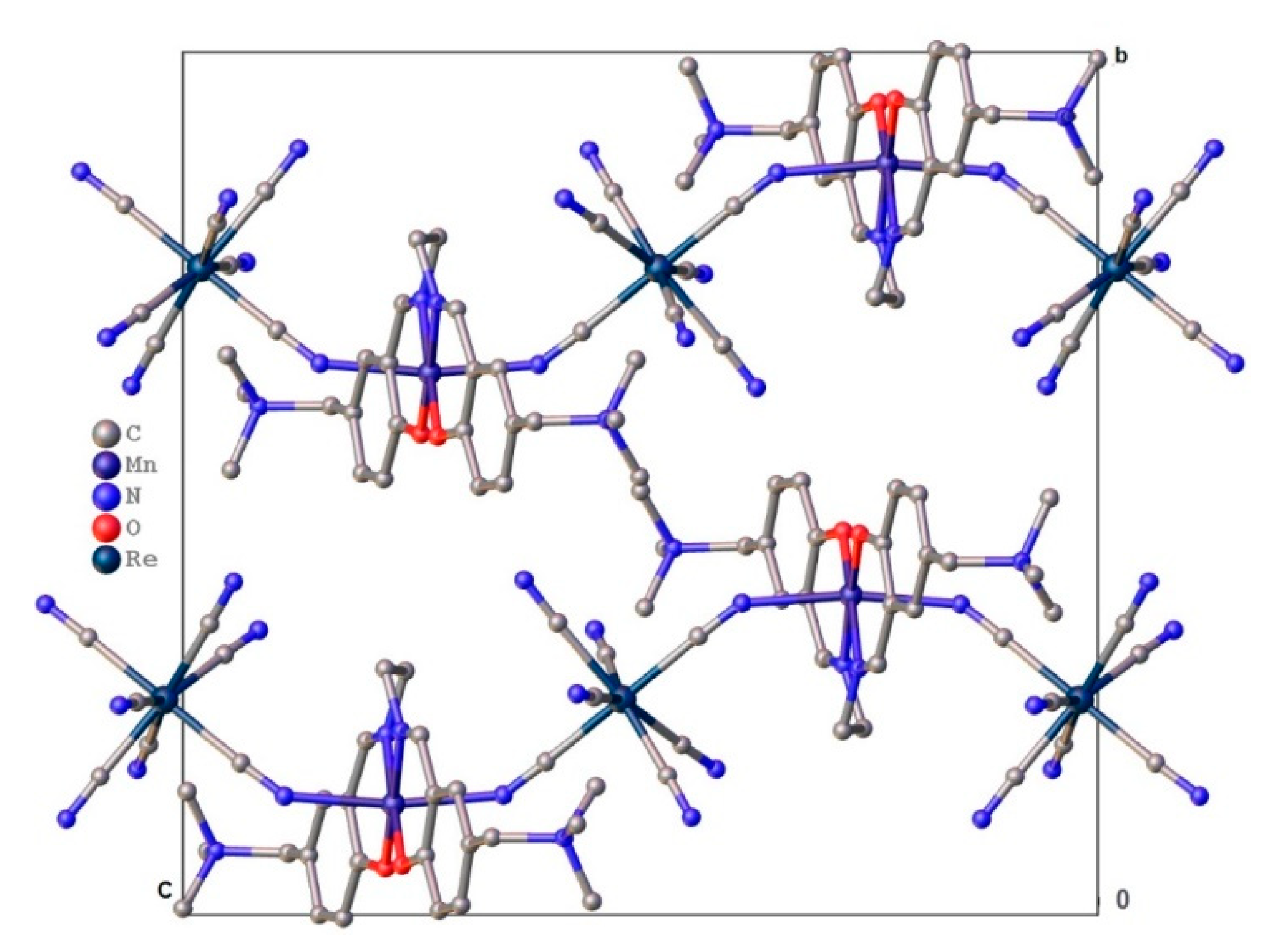
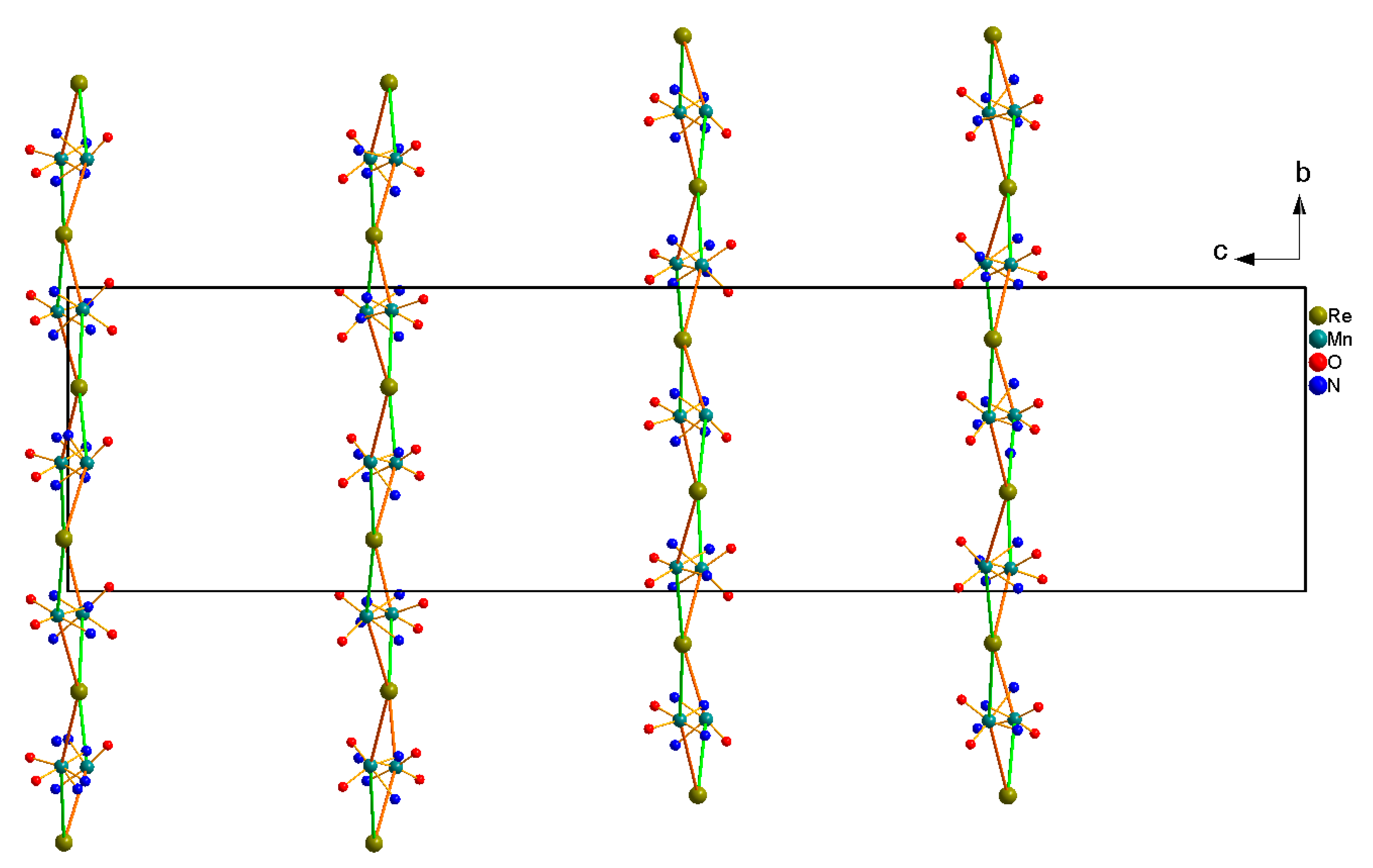
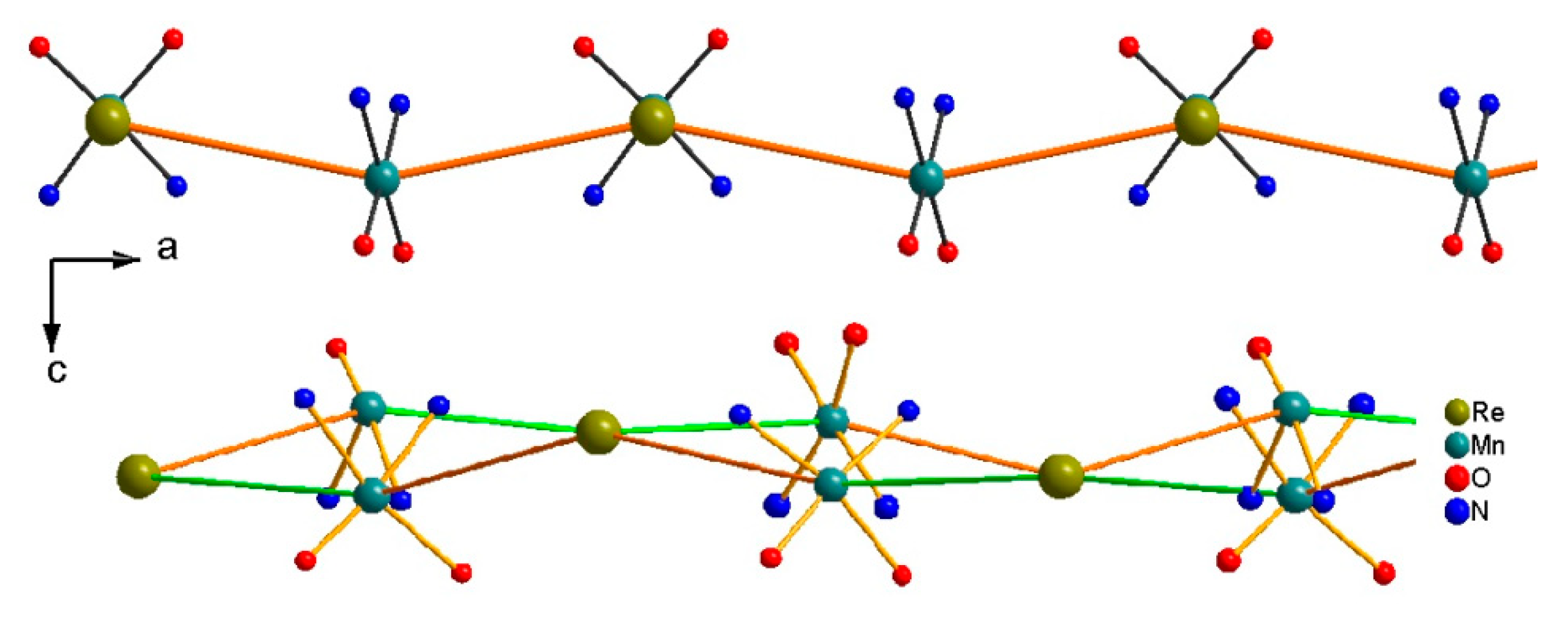
3.3.2. LD Neutral Assemblies [Mn(SB²⁺)Re(CN)₇]·xH₂O

Electroneutrality is an important contribution to the self-assembly of molecular building blocks in heterometallic coordination compounds. Mn^III complexes with Schiff bases of both the acacen and salen type generally have a charge of +1, whereas heptacyanidorhenate is a trianion. Hence, to obtain a 1D alternating –Re–CN–Mn–NC–Re–CN–polymer (1:1 ratio) two additional noncoordinating cations are required. This approach was fruitful for hexacyanide complexes of Fe^III and Os^III [14,21]. However, when using PPN⁺ and Ph₄P⁺, layered compounds were obtained, which will be described in the last part of this review.

Previously, a family of neutral magnetic wires with a 1:1 Mn^III/cyanidometallate ratio were obtained and 0D species: [Mn^III(SB²⁺)M^III(CN)₆]·4H₂O (M^III = Fe, Cr, Mn) [20] and [Mn^III(SB²⁺)(H₂O)W^V(CN)₈]·8H₂O, as well as [Mn(SB²⁺)(H₂O)W^V(CN)₈]·5H₂O [15], where [Mn^III(SB²⁺)]³⁺ is a trication (see Scheme 4). Therefore, in order to prepare the neutral LD species containing [Re^IV(CN)₇]³⁻, the same methodology was employed using the same starting complex, [Mn(SB²⁺)(H₂O)₂](ClO₄)₃·H₂O, as a source of high-spin molecular units. When an acetonitrile solution of (Bu₄N)₃[Re(CN)₇] is layered on an aqueous solution of a manganese(III) complex, large, dark crystals of a 1D polymer (12) are formed [46]. Compound 12 can also be synthesized as a crystalline powder by the gentle addition of a solution of a Mn^III complex to a solution of cyanidometallate. The slow recrystallization of compound 12 powder in aqueous medium resulted in the formation in a small amount of crystals of the binuclear complex [Mn(SB²⁺)(H₂O)Re(CN)₇]·2H₂O (13).

Crystal Structure Description

SCXRD showed that the assembly 13 has a 0D structure, whereas 12 is a 1D chain polymer. Molecular views of the repeating unit in the complex {[Mn(SB²⁺)Re(CN)₇]}_n (12) and an asymmetric unit of [Mn(SB²⁺)(H₂O)Re(CN)₇]·2H₂O (13) are presented in Figure 11. Both compounds are neutral bimetallic assemblies consisting of one [Re(CN)₇]³⁻ anion and one cation ([Mn(SB²⁺)]³⁺ for 12 or [Mn(SB²⁺)(H₂O)]³⁺ for 13 [46].

In both compounds, a slightly distorted PBP coordination environment of the rhenium ion involves seven cyanides. In fact, [Re(CN)₇]³⁻ is considerably less deformed than its iso-electronic equivalent, [Mo(CN)₇]⁴⁻ [26,27,28]. The Re–C distances are close to those for (Bu₄N)₃[Re(CN)₇] [38]. The geometry of the manganese atom is a tetragonal bipyramid typical for the Jahn–Teller elongation.

The Mn–(N≡C)_axial angle departs noticeably from 180° and equates to 144.2° and 145.1° for 12 and 13, correspondingly, close to the value of 144.4° for [Mn(SB²⁺)Fe(CN)₆] [20]. Such a trend is typical for cyanide-based Mn–M(CN)_n complexes [9,43,47,48,49,50,51,52]. Nevertheless, for the coordination compounds of SB²⁺, which is a sterically demanding ligand, this angle is particularly deviated from 180° [47]. For example, for a much less bulky SB complex, such as [Mn(acacen)]⁺, the value of this angle for the Re^IV-Mn^III system fluctuates within the bounds of 152.9–163.7° [9,43,44]. Furthermore, the Mn–(N≡C) angle is 162.6 and 160.1° in 0D complexes [Mn(^MeSB²⁺)(H₂O)Fe(CN)₆] [47] and [Mn(SB²⁺)(H₂O)W(CN)₈] [15], respectively. Analysis of the geometric parameters in heterometallic compounds is extremely important. For example, the greater the deviance from 180° in the Mn–(N≡C)– fragment, the greater the contribution of sigma binding to the Mn–Re exchange interaction.

Contrary to the {Mn^III(SB)M(CN)_m} compounds—where SB is a salen-type ligand, for which a dimerization of the Mn^III(SB) fragments is fairly common [9]—the trications [Mn(SB²⁺)H₂O]³⁺ in the 0D complexes involving hexa- or heptacyanidometallates are not dimerized in a crystal due to the trans location of the [Me₃N⁺CH₂] groups relative to the SB²⁺-ligand plane [46,47], whereas the molecules of [Mn(SB²⁺)(H₂O)W(CN)₈] [15] are dimerized due to hydrogen bonding, π–π stacking, and nonvalent CN…H interactions of the [Me₃N⁺CH₂] groups, which are cis located relative to each other.

In a crystal, the chains of 12 (Figure 12) are connected to the layers through a system of hydrogen bonds. Compound 13 possesses a pseudo-3D structure, as it also has the developed system of hydrogen bonds. Coordinated to the Mn center, a water molecule participates in the formation of a 1D system of hydrogen bonds that connects the discrete binuclear species in the chains (Figure 13), which are then bounded into the layers interconnected by the sufficiently short contacts C≡N…H-C and C-O…HC of ~2.5 Å [46].

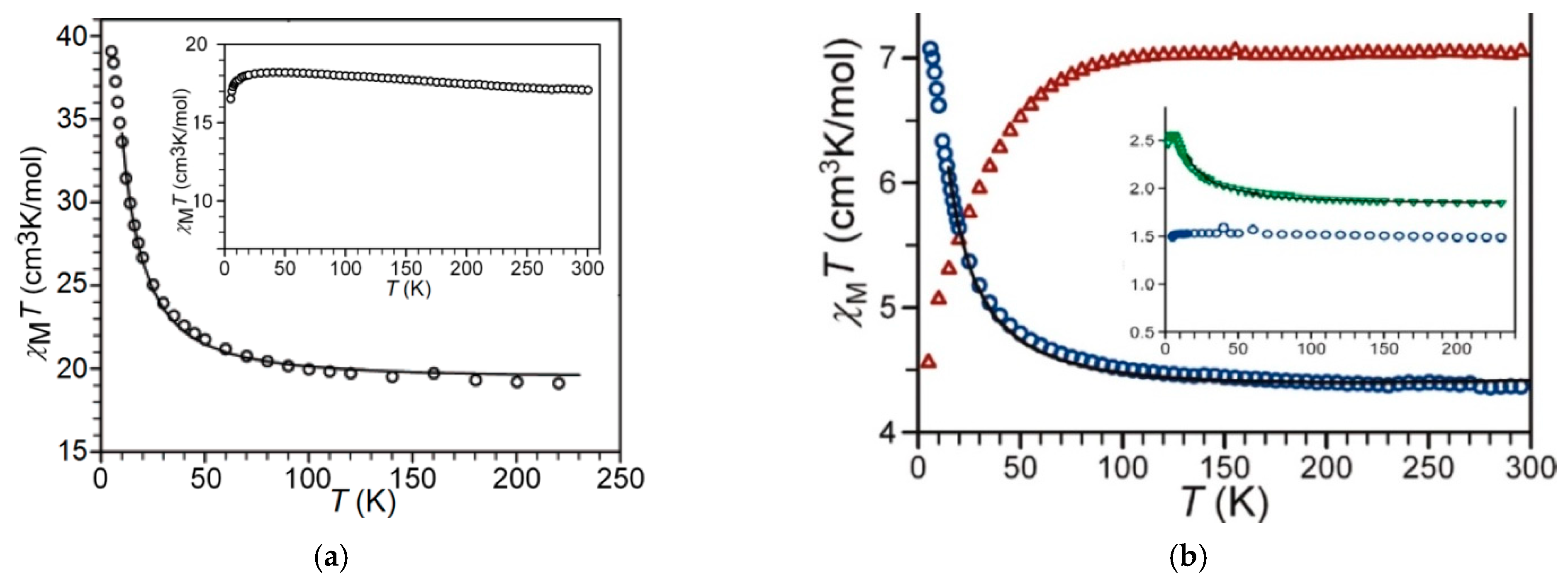
Static Magnetic Behaviors

The temperature dependence of the dc molar χT for the polycrystalline sample of 12 is presented in Figure 14. At 300 K, the χT value of 3.68 is very close to the 3.60 emu K mol⁻¹ expected for one Mn^III (S = 2, g = 2.03) [44] and one Re^IV (S = 1/2, g = 2.33) [38] as nonintersected spins. The χT decreases slightly, reaching a shallow minimum of 3.28 emu K mol⁻¹ at ~75 K. This comportment of χT is typical of antiferromagnetic coupling of the Mn^II/III and Re^IV ions within the chains [44,52]. Below 50 K, χT increases, reaching a maximum of 9.30 emu·K·mol⁻¹ at ~5 K before falling to 6.48 emu K mol⁻¹ at 2 K.

The M(H) plot of 12 registered at 2 K demonstrates a full magnetization reversibility (Figure 14, inset). The M value reached at 50 kOe is 2.86 μ_B per Mn^III–Re^IV units, which is far from 5 μ_B (theoretical value consistent with five unpaired electrons) [46]. The calculated magnetic field saturation (H_A) for 12 is approximately 151 kOe, being much greater than the values of 100, 108, and 120 kOe established for [(SB²⁺)(Cr/Fe)(CN)₆] [20] and [Mn^III(acacen)Fe^III(CN)₆]²⁻ [21], respectively.

The magnetic behavior of 13 was studied on a sample consisting of the crystals manually sorted and scrupulously separated from the possible powder impurities of the 1D precursor [46]. The magnetic properties of 13 (Figure 15) are somewhat similar to those of 12; the χT temperature plot also shows a sharp maximum, and the shape of the graphics for M(H) is comparable to that for 12. Moreover, the χT value of 3.68 emu·K·mol⁻¹ at 300 K practically coincides with that registered for 12. Furthermore, unlike 13, χT increases slowly, indicating a ferromagnetic interaction between the spins, with approximately 3.85 emu·K·mol⁻¹ at 70 K, after which the χT values begin to increase, reaching a peak of 8.21 emu·K·mol⁻¹ at 5 K and then dropping abruptly [46]. The existence of the peak on the χT curve at 5 K is unexpected for a 0D species, as no peak was found in χT (T) of the related compounds: [Et₄N]₂[Mn(saldmen)(H₂O)Fe(CN)₆] (saldmen is N,N’-(1,1-dimethylethylene)bis(salicylideneiminate)) [53] and [Mn^III(^MeSB²⁺)(H₂O)Fe(CN)₆]) [47].

The M(H) plot for 13 is shown in Figure 15 (inset). Note the value of M of 3.58 μ_B for [Mn^III(^MeSB²⁺)(H₂O)Fe(CN)₆] [47] at 50 kOe is close to the 3.45 μ_B of the binuclear complex [Et₄N]₂[Mn(saldmen)(H₂O)Fe(CN)₆] [53]. For 13, the M value at the maximum available field of 70 kOe is 3.99 μ_B, which is less than 5 μ_B, the value estimated for ferromagnetically paired Mn^III (S = 2) and Re^IV (S = ½), with a g_av = 2.0, resulting in a magnetic field (H_A) of approximately 120 kOe. This is less than the estimated lower limit of the saturation magnetic field (H_A) for 12. Unlike the chain, the dimer displays magnetic hysteresis at T = 1.8 K, with a very small coercive field of 156 Oe [46].

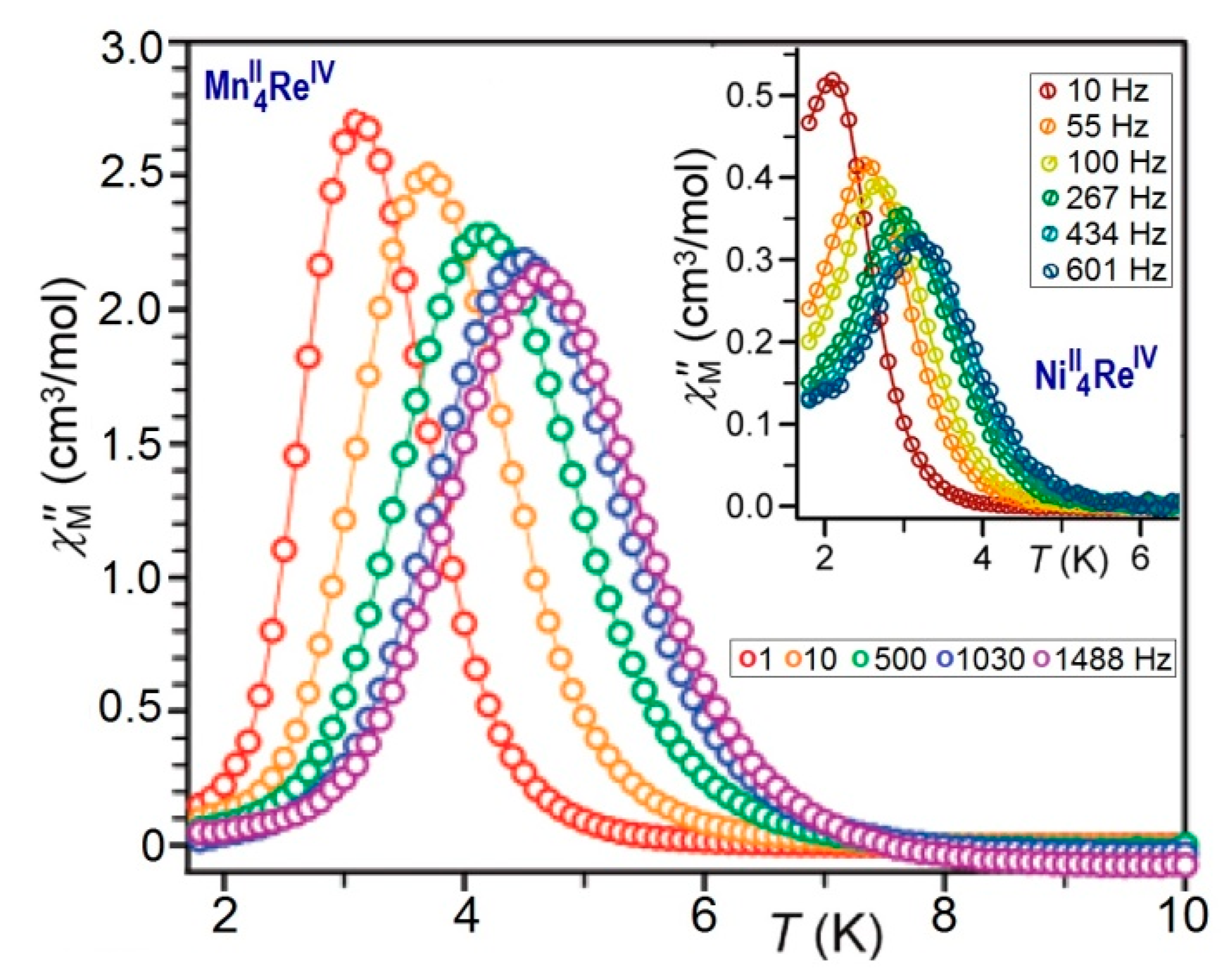
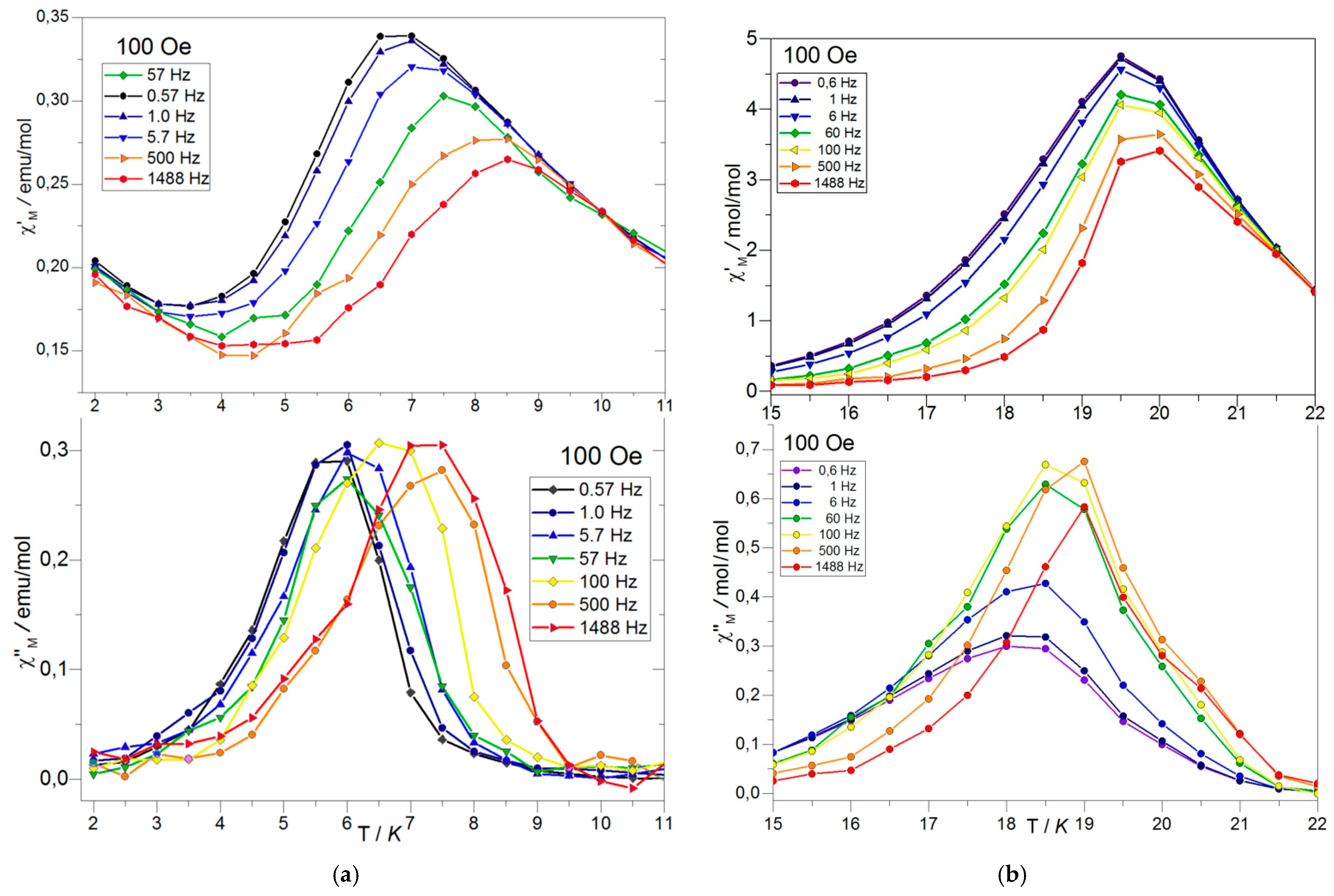
AC Magnetic Measurements

The temperature-dependent comportment of the ac susceptibilities of 12 was investigated in a zero-dc field. The results undoubtedly show that both the real and imaginary parts of the ac susceptibility have pronounced frequency dependence below 4 K [46]. However, not a single peak was recorded for χ″(T) plots at the temperatures 2–4 K, as the magnetization-blocking temperature (T_b) for 12 appears to be below 2 K [46].

For a anisotropic magnetic molecular wire, the χ’T is proportional to the correlation length (ξ) and obeys the formula χ’T = C_effexp(Δ_ξ/k_BT), Δ_ξ being an energy necessary to produce a domain wall, and C_eff being the effective Curie constant per magnetic entity [10,11,54,55]. Thus, the ac susceptibility data for 12 from 2 to 11 K obtained using an H_ac = 2.7 Oe and H_dc = 0 Oe field and a frequency of 1 Hz were employed to analyze the SCM behavior of the compound. As expected, the ln(χ’T) vs. 1/T plot) exhibits a linear interval from 10 to 6 K, yielding Δξ/k_B = 6.66 K and C_eff = 1.005 emuK mol⁻¹ [46]. At 4.5 K, ln(χ′T) achieves a peak ((χ′T)_max = 10.3 emu K mol⁻¹) and then drops linearly with temperature, reducing until χ′ is blocked. An intersection of this linear region occurring at ~4.5 K [46] is a crossover temperature (T*), where the magnetic correlation is physically limited by crystal defects, and temperatures below T* comprise the finite-sized regime [46,54].

The dynamic magnetic properties of complex 13 were studied in a frequency interval of 1–1500 Hz under an oscillating ac magnetic field of 3.5 Oe using H_dc = 100 Oe (Figure 16) [46]. Both sets of plots indicate the presence of two relaxation processes, which may be due either to an expression of two diverse relaxation mechanisms or to the existence of a second phase, the latter option being the most likely explanation, as a stratum of a desolvated solid could be formed on the surface of crystals of 13 [46].

Figure 13 demonstrates that the dimers of 13 are linked in a chain by a set of hydrogen bonds. The removal of at least one H₂O molecule inevitably leads to a rearrangement of the entire packaging of binuclear entities. This results in a change in the intermolecular distances and geometric features in a dimer after the removal of all H₂O in 13. Moreover, a reorganization in a 1D polymer is possible; the [Mn(SB²⁺)]³⁺ cations are linked to Re^IV ions by apical and equatorial cyanides. Such an organization of the chain in the crystal should promote a mutual alignment of both the apical axes of the Re(CN)₇ anions and the Jahn–Teller axes of the [Mn(SB²⁺)]³⁺ cations, which is important to increase the coercivity of LD magnets. It should be noted that the mutual slope of the (N≡C–Re–C≡N)_apical and N–Mn–N axes (predetermined by the Mn–(N≡C) angle) is responsible for the nature and magnitude of the intrachain coupling affecting the spin–reversal barrier height and the value of T_b [46].

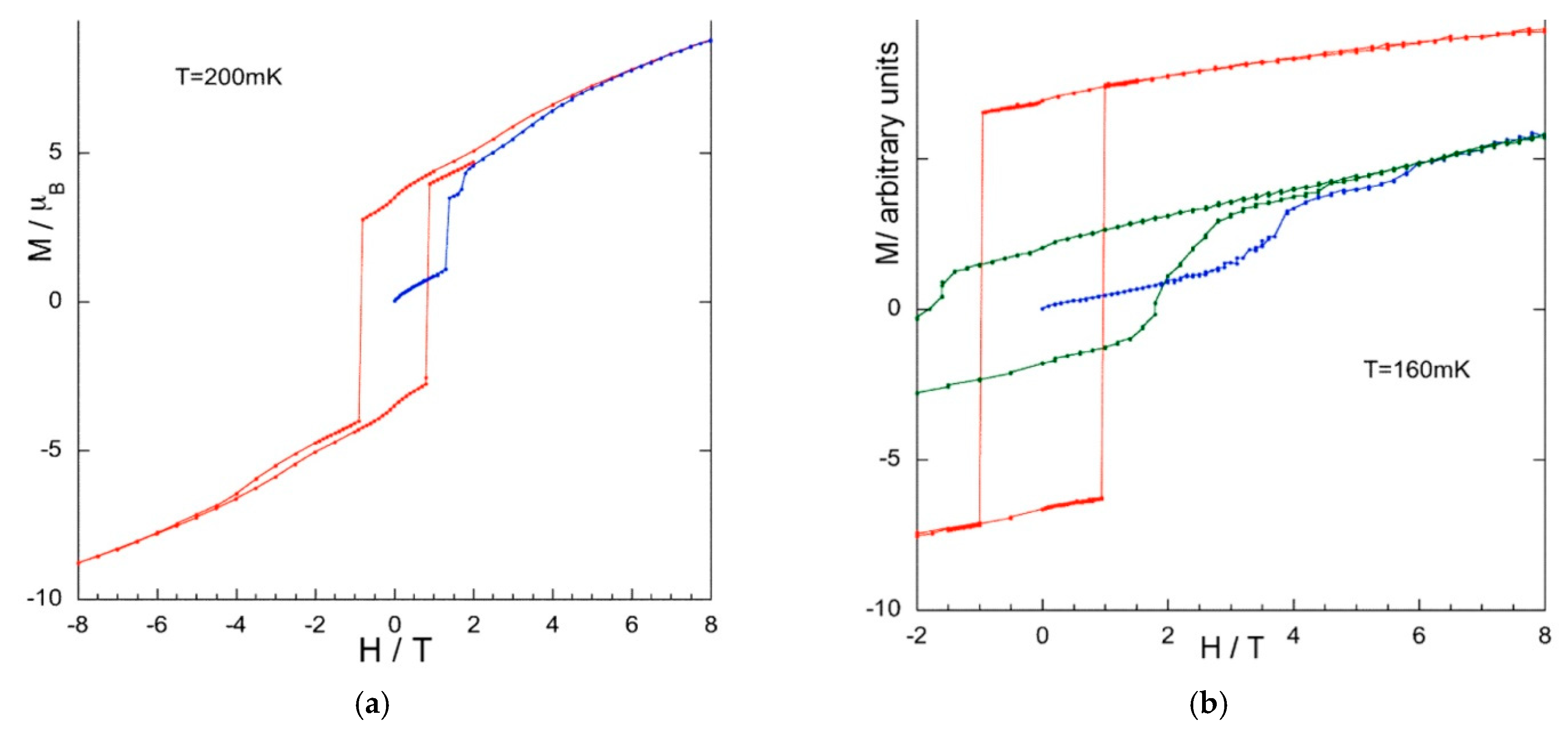
Magnetic Measurements at Very Low Temperatures

Additional magnetization data (Figure 14 and Figure 15) were collected from single crystals down to 30 mK. As shown in Figure 17a for 12, the M/M_s(H) hysteresis opens only below 1.6 K. For this reason, hysteresis was not registered at 2 K for the polycrystalline sample of 12 (Figure 14), whereas for the polycrystalline sample of 13, a tiny hysteresis loop was detected at 1.8 K [46]. The coercivity of 1.3 T is observed for a crystal of 12 at 0.4 K, which is less than the value of 1.68 T obtained for its congener [Mn(SB²⁺)Fe(CN)₇]·4H₂O [20]. Considering the 1D nature of 12, based on the ln(χ′T)—1/T plot, the magnetization slow relaxation is also evident from the hysteresis loops and strongly supports the concept that 12 is a new example of a single-chain magnet with T_b ≈ 1.6 K [46].

Despite the presence of hysteresis, the pronounced frequency dependence of molar susceptibility, and the 0D structure, a definition of the relaxation type (SMM or SCM) of complex 13 is not straightforward. In contrast to 12, a hysteresis loop opens at 1.8 K for a powder of 13. Moreover, as shown by low-temperature studies (Figure 17b), the shape of the hysteresis and the coercivity of 13 are preserved for a single crystal up to 0.8 K [46]. At 0.5 K, the coercivity at H = 0 collapses and, starting from ~0.4 K, the shape of the hysteresis changes, which may also be related to the existence of the aforementioned dehydrated layer, which leads to the coexistence of two different magnetic phases with two different magnetization-blocking temperatures of ~1.8 and ~0.4 K—presumably for 1D and 0D phases, respectively [46]. Such an assumption is also maintained by the finding of straight sectors on the ln(χ’T)—1/T plot [46].

Thus, two neutral, LD heterometallic assemblies consisting of the dimer [Mn^III(SB²⁺)Re(CN)₇] unit were obtained. As preliminary magnetic studies showed, the compound {[Mn(SB²⁺)Re(CN)₇](H₂O)₇}_n (12) is the only SCM based on orbitally degenerate PBP heptacyanidorhenate. The χT behavior of 1D and 0D compounds in a high-temperature region diverges considerably, approving the ferrimagnetic and ferromagnetic character of magnetic exchange interactions (EIs) for 12 and 13, respectively [46]. However, at temperatures below 60 K, ferromagnetic EIs prevail in both compounds up to a temperature of 4.5 K, at which a maximum is observed in the χT—T plot; then, χT rapidly decreases due to saturation in the field effect [46]. For both compounds, even under a field of 70 kOe, the magnetization does not reach the maximum value of 5 μ_B, which is expected for five unpaired electrons, which is due to the high anisotropy of these systems, as evidenced by the estimated values of the field H_A of 151 and 120 kOe for 12 and 13, respectively [46].

The unusual magnetic properties of both compounds originate from the interplay of Re−Mn anisotropic spin coupling and the ZFS effect of Mn^III ions with a noncollinear orientation of the local magnetic axes in crystals of the compounds, which was initially found for a Mn₃Re 3D framework [44]. The obtained experimental data in this study could be used to theoretically model this intriguing magnetic behavior in order to determine the magnetic characteristics for anisotropic exchange, as was performed by employing microscopic theory for 3D [(Mn^IIIacacen)₃Re^IV(CN)₇]_n [44] and [{MnL^N5(H₂O)}₂Mo(CN)₇] [39].

3.3.3. 2D {Cat[{Mn(acacen)}₂Re^III(CN)₇]}_n, Cat = Ph₄P⁺ (14) and PPN⁺(15)

Employing tetraphenylphosphonium (Ph₄P⁺) as an additional cation led to a preparation of 1D heterometallic negatively charged polymers (Ph₄P)₂[Mn^III(acacen)M^III(CN)₆] (M^III = Fe or Os) possessing SCM properties [14,21,48]. This prompted us to explore the large organic cations for the design of anionic LD molecular magnets comprising [Re^IV(CN)₇]³⁻ and [Mn^III(acacen)]⁺ tectons. However, when an assembling process was implemented in an alcohol mixture (MeOH, EtOH, and li-PrOH (1:1:1)), a 2D heterometallic complex Ph₄P[{Mn(acacen)}₂Re(CN)₇]·Solv (14) was obtained for the first time. However, the replacement of Ph₄P⁺ by bulkier PPN⁺ did not result in the formation of an anionic chain. However, in this case, a layered material, PPN[{Mn(acacen)}₂Re(CN)₇]·Solv (15), was also formed, with a 2D coordination network possessing unique magnetic properties [56].

Crystal Structure Descriptions

In spite of the fact that single crystals were grown for layered compounds, a structure with a satisfactory R factor was solved only for the PPN derivative 15. The independent part of a layer in 15 and a cation PPN⁺ are shown in Figure 18. Figure 19 demonstrates a two-dimensional network structure consisting of two sets of crossed chains; in one, the [Re(CN)₇]³⁻ metalloligand binds the neighboring [Mn(acacen)₂]⁺ moieties (see Scheme 2) by its apical axis, and the other does so with two equatorial CN ligands [56]. The hybrid layered packing of 15 consists of the anionic coordination networks separated by a space containing individual organic cations. It can be plainly observed by projecting the structure onto the ac or bc plane. The distances between the Re centers located in the nodes of the 2D network correspond to the values of the crystal cell parameters a and b (a = b = 10.9343(2) Å), which are close to those of the neutral material, [Mn(acacen)]₂[Ru(CN)₅NO] [41]. The distance between the planes of adjacent layers matches parameter c of the 2D network [56]. The 2D polymer 15 is the least symmetrical coordination network among the known earlier layered assemblies involving cyanide metalloligands [[K(18-cr)(2-PrOH)₂][Mn(acacen)]₂[Fe(CN)₆] [57] and K[Mn(acacen)]₂[W(CN)₈]·2H₂O [58].

Each [Re^IV(CN)₇]³⁻ metalloligand is linked to four [Mn(acacen)]⁺ cations by means of four cyanides with corresponding distances: Mn1–N31_apical of 2.384(3) Å and Mn2–N32_equat of 2.299(5) Å. The Mn–N≡C bond angles are 154.5(5) and 155.2(3)° for apical and equatorial cyanide respectively, indicating that the bridging CN ligands coordinate to Mn^III ions in a bent mode [56], although this bending is substantially less than the 144.2° and 145.1° observed in 12 and 13, respectively.

The Re polyhedron is sufficiently close to an ideal PBP because the Re-C bond length changes in the range of 2.090(5)—2.115(3) Å, with the C—Re—C angles only slightly differing from the ideal geometry. The Mn environment is an elongated octahedron characteristic of the high-spin Mn^III d⁴ electronic configuration. This is due to the Jahn–Teller effect of the combination with a strong in-plane donation of the acacen²⁻ ligand [56]. The in-plane Mn—ligand bond distances fall in the 1.898(4)–1.988(6) Å range, whereas the axial Mn–N bond distances are elongated: Mn1—N31 = 2.384(3), Mn1—N34 = 2.321(3) Å, Mn2—N32 = 2.299(5), and Mn2—N35 = 2.279(5) Å. Such a situation is typical for heterobimetallic materials, including Mn^III(SB) complexes and polycyanide metalloligands [9,57].

The space between the anionic layers is occupied by PPN⁺ cations and highly disordered solvent molecules. When deposited in CCDC, the latter were refined as water molecules; however, in accordance with the result of elemental analysis, they were approximately defined as a mixture of water and ethanol in order to establish a system of hydrogen bonds. In particular, it should be noted that the crystals of 15 are able to lose part of the solvent, retaining their integrity [56].

Although several batches of the crystals of 14 were verified, their structure was resolved only partially. An SCXRD investigation for 14 was conducted at 298 K, yielding the following cell parameters for 14: a = b = 15.1259(15), c = 61.625(7) Å, β = 90°, V = 14,105(3) ų), Z = 8—much higher than those of 15. Notwithstanding that, a layered organization was also defined for 14 with four layers per cell located perpendicular to the c axis (Figure 20) [56].

The space group was chosen based on the distribution of heavy atoms. In addition, it was able to localize most of the atoms from the nearest environment of Re and Mn. This was sufficient to establish inner-layer organization. In the 2D network of 15, there are two types of perpendicular chains, in one of which the Mn ions are connected by two apical cyanides of the metalloligand, and in the other, by equatorial CN-groups, whereas in the 2D material 14, the layers are more “corrugated” (see Figure 21) because their mutually perpendicular chains are organized equally; the Mn centers are connected to the Re ions both by apical and equatorial cyanides. This is manifested in the distances between metal centers. If in a layer of 15, there are only two types of distances between Mn atoms, i.e., 10.8268(5) and 1.7016(5) Å (for apical and equatorial cyanides, respectively), then in the layers of 14, there are as many as four possible distances of Mn—Mn for each layer (for the middle layer, the “apical” and “equatorial” distances are 10.7825(8) and 10.7612(8) Å, 10.6374(5) and 10.6173(5) Å respectively. These structural features are extremely important for understanding the difference in magnetic behavior between 14 and 15 [56].

Static Magnetic Studies

The temperature dependencies of the dc molar susceptibility for the powder samples of 14 and 15 are presented in Figure 22 as χT vs. T plots. At 300 K, the χT values of 6.55 and 6.50 emu K mol⁻¹ (for 14 and 15, respectively) are close to the 6.51 emu K mol⁻¹ expected for two Mn^III (S = 2, g = 2.03) [44] and one Re^IV (S = 1/2, g = 2.33) [38] nonintersected spins [56]. As can be seen in the inset of Figure 22, showing the zoomed-in plots in the χT range from 4 to 11 emu K mol⁻¹, the magnetic behavior of the two networks is similar [56]. This is particularly true at high temperatures. Starting from 300 K, the χT curves decrease slightly and then reach a light minimum of 5.94 emu K mol⁻¹ at ~85 K for 15 and 5.57 emu K mol⁻¹ at ~35 K for 14 [56]. With a further decrease in temperature, the χT plots increase and reach maximums of 10.51 emu·K·mol⁻¹ at ~11.5 K for 14 and 181.45 emu·K·mol⁻¹ at 18 K for 15 before dropping to 7.75 and 27.54 emu K mol⁻¹ at 2 K for 14 and 15, respectively [56]. Such comportment of χT is characteristic of the ferrimagnetic interaction between the metal ions inside the chains [44,52].

In Figure 23, the magnetization data for both the layered materials are presented. The high-field magnitude of 3.62 μ_B M for 14 is close to the 3.58 μ_B registered for the chain {[Mn^III(SB²⁺)Re^IV(CN)₇]}_n (12) [46]. The initial magnetization plot for 15 displays an S shape typical for metamagnetic materials. In the field of 23205, a metamagnetic transition takes place at 2 K [56]. The M value of 3.2 μ_B at the maximum available field of 7 T is very far even from the 7 μ_B calculated for antiferromagnetically coupled Mn^III (S = 2) and Re^IV (S = ½) with g_av = 2.0. The limit of the anisotropic magnetic field (H_A) for 15 estimated by linear approximation is 26 T [56], which is considerably larger than an estimation of 15 T obtained for 12. Studies of initial M(H) plots for 15 at different temperatures (Figure 24) show that the S shape of the curve disappears at temperatures above 5 K [56]. Such behavior of M below 5 K is reminiscent of a metamagnetic transition from an antiferromagnetic state to a polarized state, as found for the related 3D framework material 11 [44].

As can be seen in Figure 23, both 2D polymers exhibit magnetic hysteresis at ~2K, with compound 14 showing a hysteresis with a small coercive field of 1150 Oe, whereas for 15, this parameter reaches a value of 2.486 T, which is much larger than that of 1.5 T observed for an oriented single crystal of SCM, [Mn^II(bida)(H₂O)]₂[Mo^III(CN)₇]·6H₂O [17]. The M(H) of 15 does not saturate in the field of 7 T (70 kOe) and reveals a linear dependence at high fields, which is typical for strongly anisotropic systems [56]. The hysteresis plots of 15 measured at higher temperatures are presented in Figure 24a, with the inset of this figure demonstrating that a very tiny hysteresis loop is still visible at 16 K, which indicates that the critical temperature for this 2D material is only slightly higher than 16 K [56].

Dynamic Magnetic Studies

The ac data of the [Mn^III(acacen)Re^IV(CN)₇]²⁻ networks were also collected as the temperature- and frequency-dependent susceptibilities. The study results are shown in Figure 25. The temperature dependencies for different frequencies of χ′ and χ″ susceptibility data for 14 and 15 were determined in H_dc = 100 Oe and H_ac = 3 Oe. Both ac components of 14 are frequency-dependent, and the χ′ susceptibility of 14 displays two apparent relaxation processes, whereas no second relaxation process was found for χ” [56].

The lack of points for the ac data for 15 (Figure 25b) prevents determination of the positions of the peaks for both, as well as parts of the ac susceptibility. It seems that for χ′, the effect of the frequency results only in a reduction in the peak intensity, without affecting the position of the peaks at ~19.75 K. However, for χ’’, in addition to increasing the intensity of the peak, the peaks shift to slightly higher temperatures.

An SCM behavior test was also carried out for 14 and 15 by means of the ln(χ′T)—1/T plot. Figure 26 shows the ln(χ′T)—1/T plots for 14 and 15. Linear regions are presented for both compounds. For 14, the temperature range of 11–8.5 K results in C_eff = 0.48 emuK mol⁻¹ and Δ_ξ/k_B = 3.62 K 4, with ln(χ′T) reaching a maximum at 8 K and then experiencing a straight decrease with reduced temperature until χ′ blocking. An intersection of the two linear intervals at ~7.1 K corresponds to a crossover temperature (T*) [54,55]. Hence, it can be assumed that the dependence of χ′ on frequency is due to slow magnetic relaxation of the chains, of which the 2D network of 14 is composed [56].

An analogous procedure performed for 15 as presented in Figure 26b showed that in a linear region in the range of 24–21.5 K with Δ_ξ/k_B = 326.74 and C_eff = 11.42 emuK mol⁻¹, the crossover temperature, T* = 20.1 K, was somewhat higher than the T_B of ~16 K based on magnetization measurements for 15 but very close to the value of 19.75 K based on χ′ data (Figure 25b). Unlike the 2D networks of [Mn^III(acacen)Re^IV(CN)₇]²⁻ studied in ref. [56], the frequency dependence of the ac data was not found for 3D framework 11 [44], the nearest relative. For the latter, nevertheless, a phase transition was also found at 13 K, which is ~7 K lower than for 15.

The presented combined dc and ac magnetic data, as well as the positive testing for SCM behavior of both networks, may testify to the existence of magnetic relaxation in the chains of which the anionic layers of coordination polymers 14 and 15 are composed. As follows from the SCRD data, the layers are well-insulated from each other by organic cations, which preclude the realization of a pseudo-3D structure, resulting in long-range 3D order characteristic of conventional magnets [56]. Thus, the magnetic hysteresis recorded for these two materials is scarcely a result of a phase transition, owing to the presence of mutually perpendicular 1D polymers composed of anisotropically coupled spin carriers [56]. This is confirmed by a noteworthy dissimilarity in magnetic behavior of 14 and 15, as there is an essential difference in the structure of the -Re-Mn-Re-Mn- chains for these materials [56]. The lower T_B for 14 is due to the alternating binding of Mn ions in the parallel chains by the metalloligand [Re(CN)₇]³⁻, which combines two kinds connections: through apically located cyanides and equatorial cyanides in the same chain. Moreover, such an alternation is observed for both types of perpendicularly located chains in a layer. Whereas in 15, these chains differ significantly in the manner of binding—apical in one type of parallel chains and equatorial in the chains perpendicular to the first. This combination considerably increases the magnetic anisotropy of the Re^IV—Mn^III heterobimetallic system, emphasizing a direction along the crystal axis (b) as the direction of easy magnetization [56]. In contrast, the orientation along the a axis results in less magnetization, and the orientation along the c axis corresponds to the hard magnetization axis [56].

Of particular note is the coercive field of 2.486 T for 15, which is a record for heterobimetallic molecular magnets based on homoleptic polycyanide metalloligands. The unusual magnetic properties of both compounds originate from the interplay of Re−Mn anisotropic spin coupling and the ZFS effect of Mn^III ions with a noncollinear orientation of the local magnetic axes in crystals of the compounds [56].

3.3.4. Heterobimetallic Assemblies of [Re^IV(CN)₇]³⁻ with Some Monocationic Complexes [Mn^III(SB)]⁺ of Salen-Type Schiff Bases

Here, we briefly describe the compounds obtained on the basis of two salen-based Mn^III complexes, as their magnetic properties have not been studied. However, they deserve mentioned in this review, as there are not many heterometallic assemblies containing such salen complexes of Mn^III in their composition. We would like to draw the reader’s attention on the four heterometallic complexes, which were relatively recently synthesized, studied, and structurally characterized [59].

Two compounds were used as precursors—one was a previously known mononuclear complex, [(Mn(^3MeOSalen))₂(H₂O)₂](ClO₄)₂·2H₂O (16) [60], and the other was a binuclear complex, [Mn₂(^5MeSalen)OAc]PF₆ (17), which was obtained by us. In the case of 16, a slow diffusion of the component solutions led to the ionic compound Ph₄P[Mn(^3MeOSalen)(H₂O)₂]₂ [Re(CN)₇]·6H₂O (18) [59]. The direct mixing of the same solutions resulted in a microcrystalline and nearly insoluble solid, [Mn(^3MeOSalen)(H₂O))₄Re(CN)₇]ClO₄·1.5MeCN·6.5H₂O, which is likely to comprise the pentanuclear clusters [(Mn^III(SB)(H₂O))₄Re(CN)₇]⁺ [59]. The employment of 16 resulted in a 2D network assembly of octanuclear clusters, [{(Mn(^5MeSalen))₆(H₂O)₂Re(CN)₇}₂ Re(CN)₇]Cl₂(PF₆)·H₂O (19), incorporating two Re centers in a PBP coordination environment, whereas another had a strongly distorted capped trigonal prism as a coordination polyhedron [59]. The latter was observed for the first time for Re(IV) complexes [59]. A synthetic challenge to obtain the 0D assemblies with a ratio of Re: Mn ≥ 3 yielded a hexanuclear compound, [Mn(^5MeSalen)H₂O(i-PrOH)] [(Mn(^5MeSalen))₅H₂O(i-PrOH)₂Re(CN)₇](PF₆)₂(OAc)·2i-PrOH (19), which is a 1D polymer formed by a bridging phenoxyl group [59].

4. Conclusions

The recently studied 1D and 2D heterometallic Mn^III–Re^IV compounds have made the [Re^IV(CN)₇]³⁻ family more representative in terms of the dimensionality of the known assemblies. All magnetically characterized family members have a strong anisotropy, which is determined by complexly organized anisotropic exchange interactions caused by the unquenched orbital momentum of Re^IV and local ZFC of Mn^III ions, as well as by the mutual arrangement of the apical axes, i.e., the Jahn–Teller axes for Mn and the D_5h axes of the Re PBP.

Two 2D heterobimetallic networks based on orbitally degenerate heptacyanidorhenate(IV) were synthesized for the first time and magnetically studied. An analysis of the magnetic data sets for the polycrystalline samples of Ph₄P[{Mn(acacen)}₂Re(CN)₇]·Solv (14) and PPN[{Mn(acacen)}₂Re(CN)₇]·Solv (15) showed that both materials display slow relaxation of magnetization at temperatures: below 10 and 21 K for 14 and 15, respectively [56]. Regardless of the same molecular units that make up the networks, the studied anionic layers are quite different in terms of magnetic anisotropy, as they possess a magnetic hysteresis at 2 K: small (0.23 T) for 14 and large (~5 T) for 15. Furthermore, for these polymers, the M(H) value does not saturate at the maximum available field of 7 T, and the network if 15 is a metamagnet. This intriguing difference originates from the interplay of Re−Mn anisotropic spin coupling and the ZFS effect of Mn^III ions with a noncollinear orientation of the local magnetic axes in crystals of the compounds [56]. The most well-known anisotropic is the PPN[{Mn^III(acacen)}₂Re^IV(CN)₇]·Solv 2D network, which possesses a critical temperature of 20 K and magnetic hysteresis with a record for cyanide-bound molecular material coercivity. The factors presented above makes it very difficult to theoretically model the magnetic behavior of such systems. In fact, only the group of Prof. V. Mironov has proposed an adequate approach to this problem, using the ligand field microscopic theory.

In our opinion, there are several possible directions for the development of research on the Re^IV—CN—Mn^III system, including an improvement of synthetic approaches for the reliable preparation of both neutral and negatively charged LD assemblies. Despite the advantages of [Re^IV(CN)₇]³⁻ as a magnetic unit, the main obstacle to the rational design of LD nanomagnets is the polydentate nature of this metalloligand. As shown in this review, this nature leads to the formation of 2D and 3D polymers due to the participation of equatorially located cyano groups in the coordination. Although this can be avoided by using sterically hindered tricationic complexes based on [Mn(SB²⁺)]³⁺, for the latter, the range of metal ions can be expanded by using Fe³⁺ and Cr³⁺ ions. The latter, acting as high-spin blocks and being magnetically isotropic, will not only provide more predictable magnetic behavior of target magnetic materials but also simplify its theoretical modeling. In the longer term, we are planning to develop a synthetic approach to the synthesis of doubly connected SCMs, such as {[M(bida)(H₂O)]₂[Mo(CN)₇]·6H₂O}_n [17].

The most promising direction in the chemical engineering of new highly anisotropic LD assemblies with slow magnetic relaxation at high temperatures is the synthesis of orbitally degenerate PBP Re^IV complexes with pentadentate planar organic macrocycles, which simultaneously set the D_5h symmetry of the target complex and block the equatorial positions of PBP. Recently, this was achieved for the (Et₄N)[Mo^III(DAPBH)Cl₂] [61] electronic analogue [Re^IV(CN)₇]³⁻, but no heterometallic compounds based on it have been published to date.

Equally important is the development of theoretical modeling of the magnetic behavior for molecular magnets based on heterometallic assemblies of orbitally degenerate PBP complexes of d elements, which are the source of anisotropic exchange interactions. In order to develop theoretical methods for modeling magnetic properties of such complicated objects, it is necessary to obtain direct experimental data for their magnetic parameters, i.e., D and tensor quantities J and g. The accomplishment of this task is possible only with the use of high frequencies and high-magnetic-field spectroscopic methods. The set of theoretical parameters thus obtained will be used in the future for practical recommendations for the rational design of high-performance SMMs.