A Ti/Pt/Co Multilayer Stack for Transfer Function Based Magnetic Force Microscopy Calibrations
Abstract
1. Introduction
2. Results
2.1. Fabrication of the Multilayer Stack
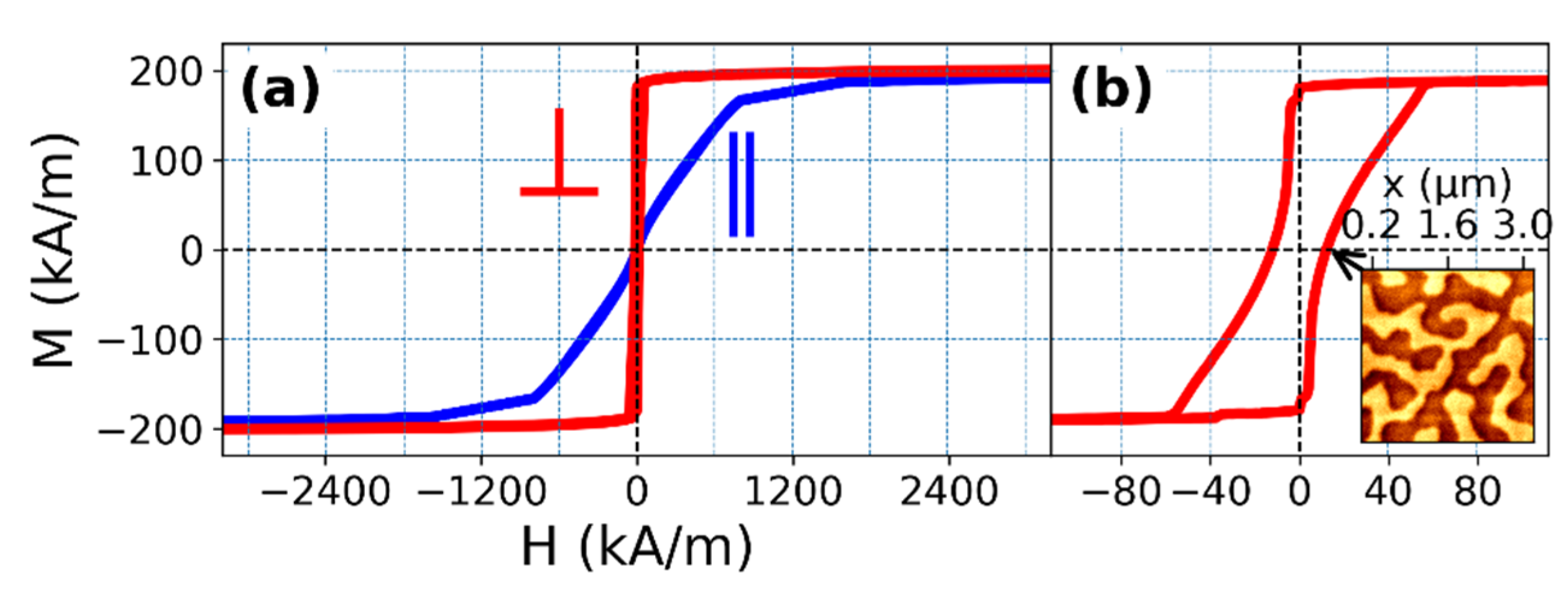
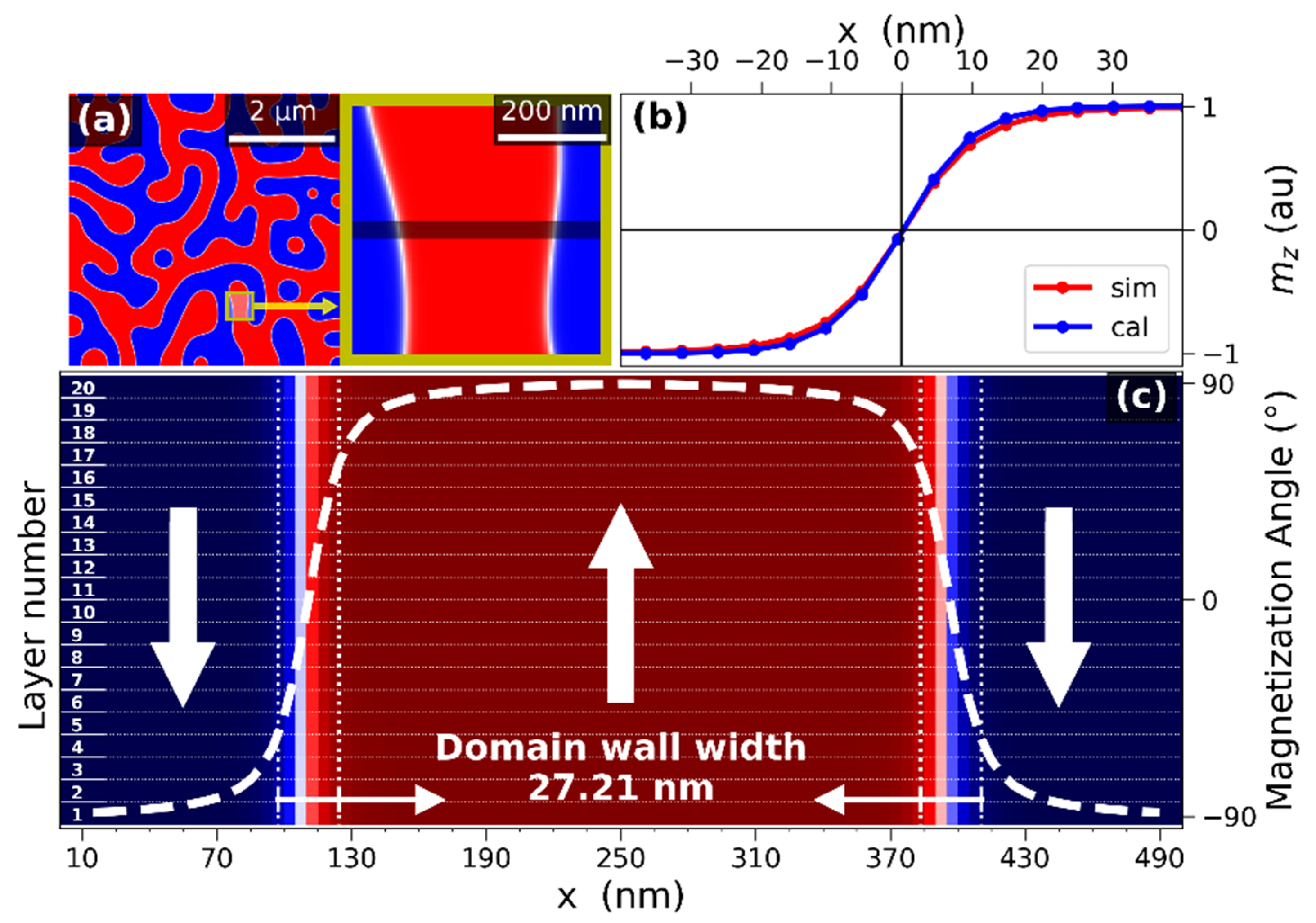
2.2. Magnetic and Geometric Characterization of the Ti/Pt/Co Sample
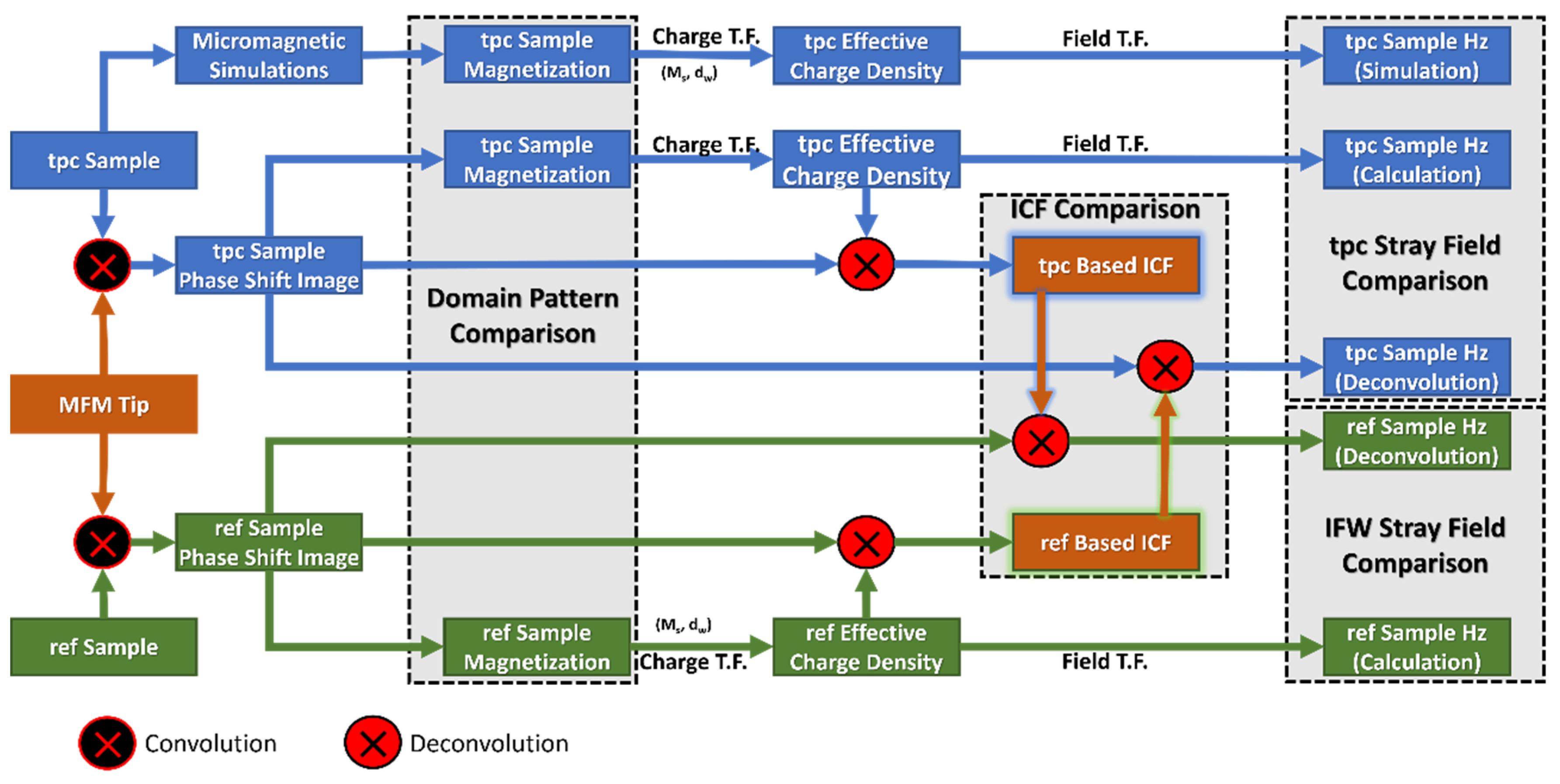
- The domain pattern comparison is used to prove a good understanding of the micromagnetics of the Ti/PtCo material.
- The tpc stray field comparison serves the purpose of demonstrating that the reference sample is well understood and thus calculable and that different approaches (micromagnetic simulations, discrimination + forward calculation, qMFM) give the same magnetic stray field.
- The IFW stray field comparison will show that the Ti/Pt/Co sample, when actually used as a reference sample, gives correct quantitative stray field data in calibrated measurements, as validated by a comparison of Ti/Pt/Co-calibrated qMFM data on the Co/Pt sample with the results from discrimination and forward calculation.
- Finally, the ICF comparison will show that, not merely a proper quantitative analysis of “unknown” samples is achieved, but also a very good agreement of the ICF and the thereof derived tip magnetic properties, compared to calibrations with another reference sample.
2.3. Micromagnetic Simulations of the Ti/Pt/Co Sample’s Magnetization Structure
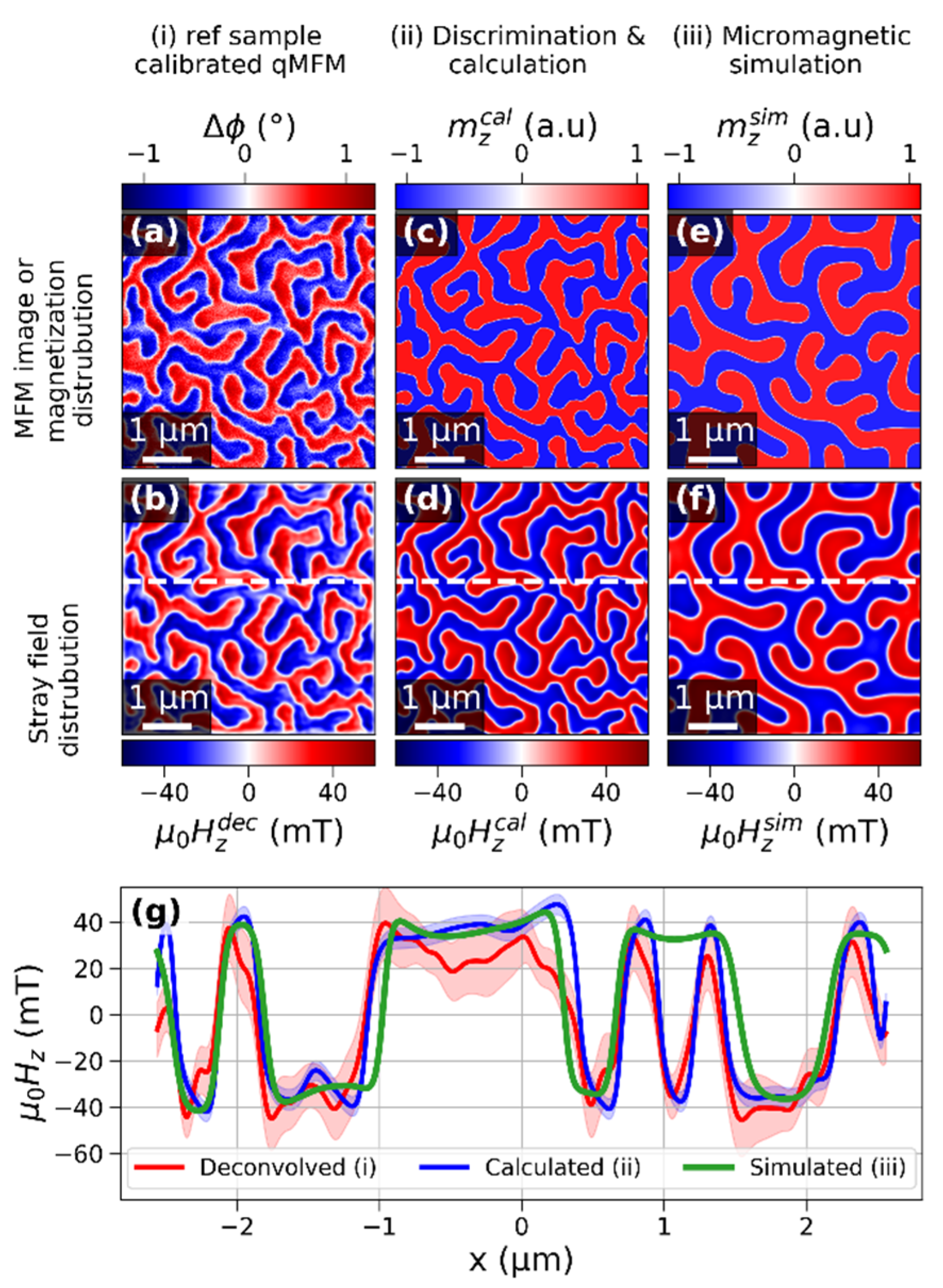
2.4. Validation with qMFM and Stray Field Simulations
- (i)
- qMFM characterization of the Ti/Pt/Co sample
- (ii)
- MFM domain pattern-based simulations
- (iii)
- Micromagnetic simulations
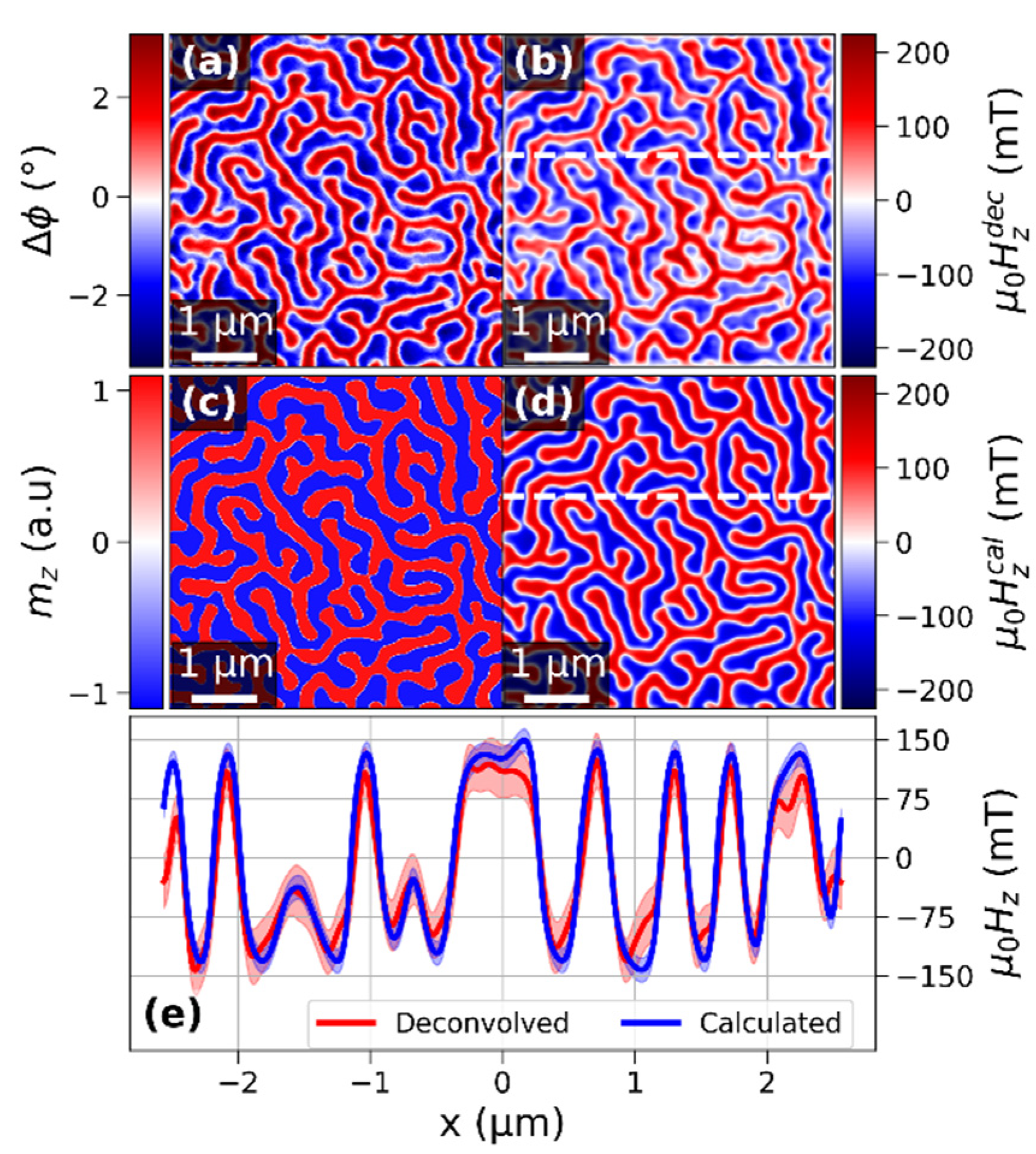
2.5. Cross Validation of the Co/Pt Reference Sample by Ti/Pt/Co Calibrated qMFM
2.6. Feature Size Spectra
3. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. XPS Study and Calibration of Deposition
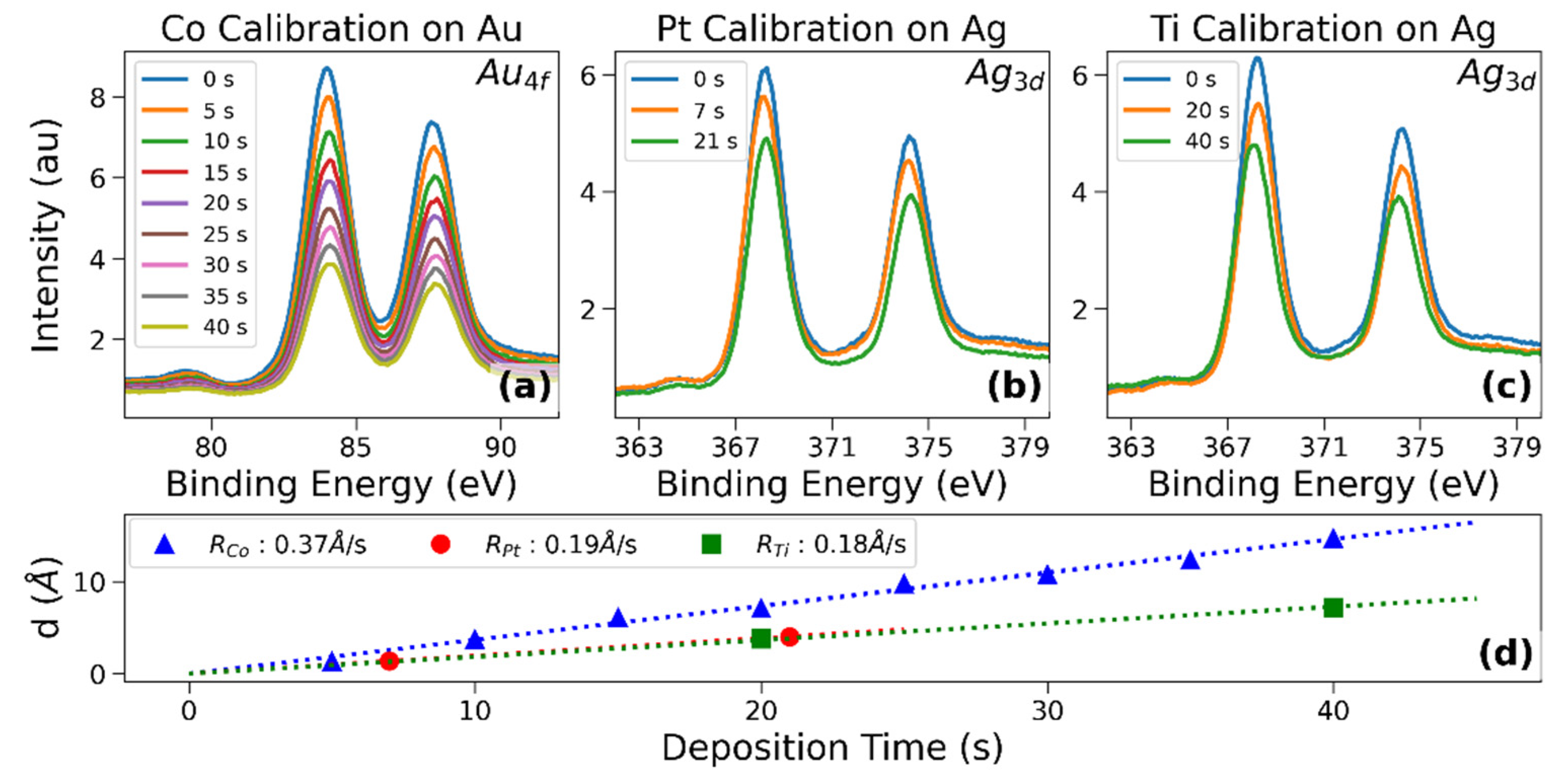

Appendix B. A Self-Correlation-Based Analysis of Domain Wall Widths

Appendix C. Determination of the Ti/Pt/Co Sample’s Uniaxial Anisotropy Constant, Ku, from the VSM Data
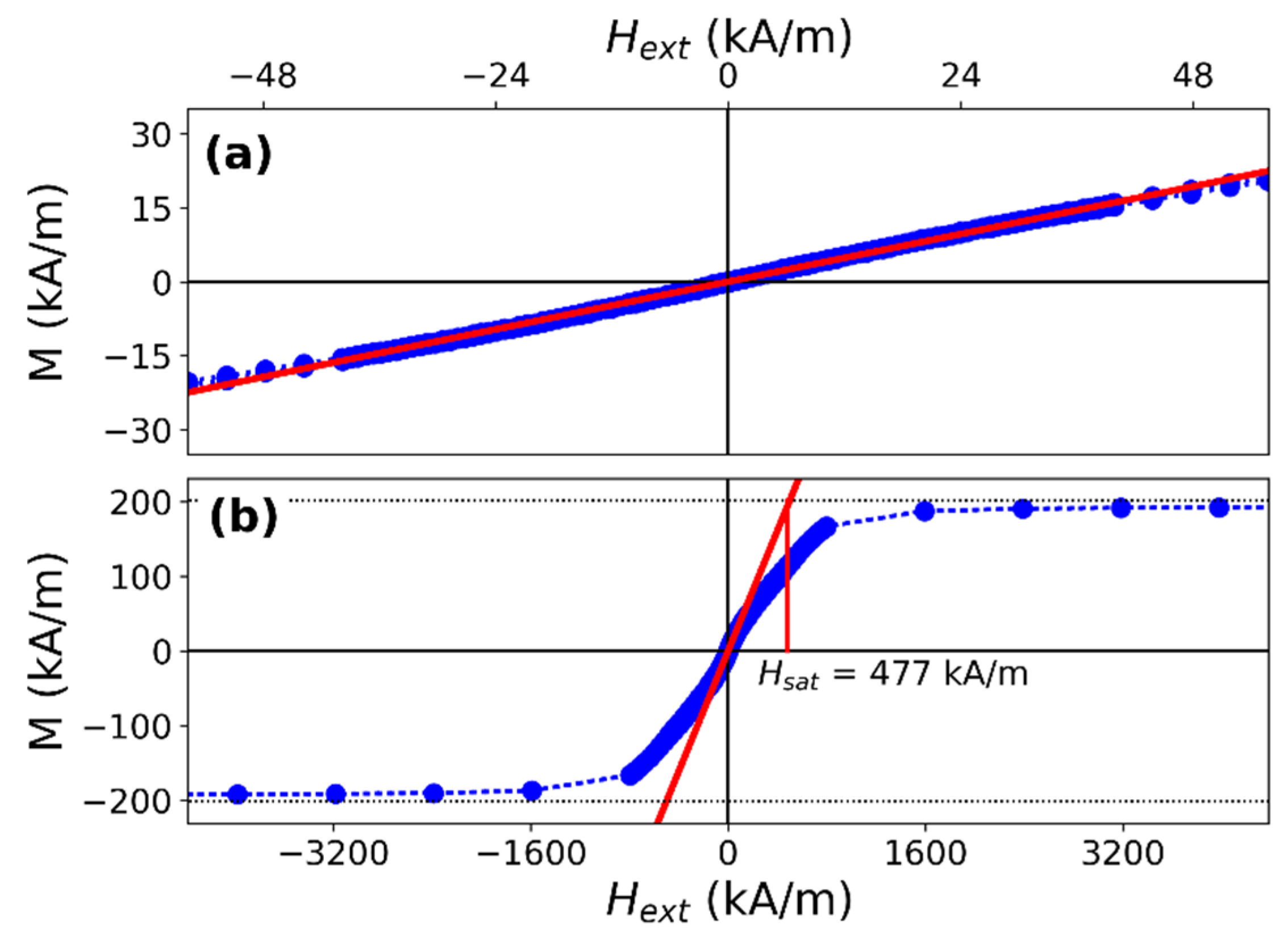
Appendix D. Domain Wall Kernel
Appendix E. Uncertainties Used in Uncertainty Calculations
| Parameter | Uncertainty |
|---|---|
| MFM phase shift Δ | |
| regularization parameter, | : 1% |
| stack thickness tpc sample, | = 2 nm |
| stack thickness ref sample, | = 4 nm |
| saturation magnetization tpc sample, | : 6% |
| saturation magnetization Co/Pt sample, | : 6% |
| measurement height, |
Appendix F. Estimation of Accessible Spatial Frequency Range
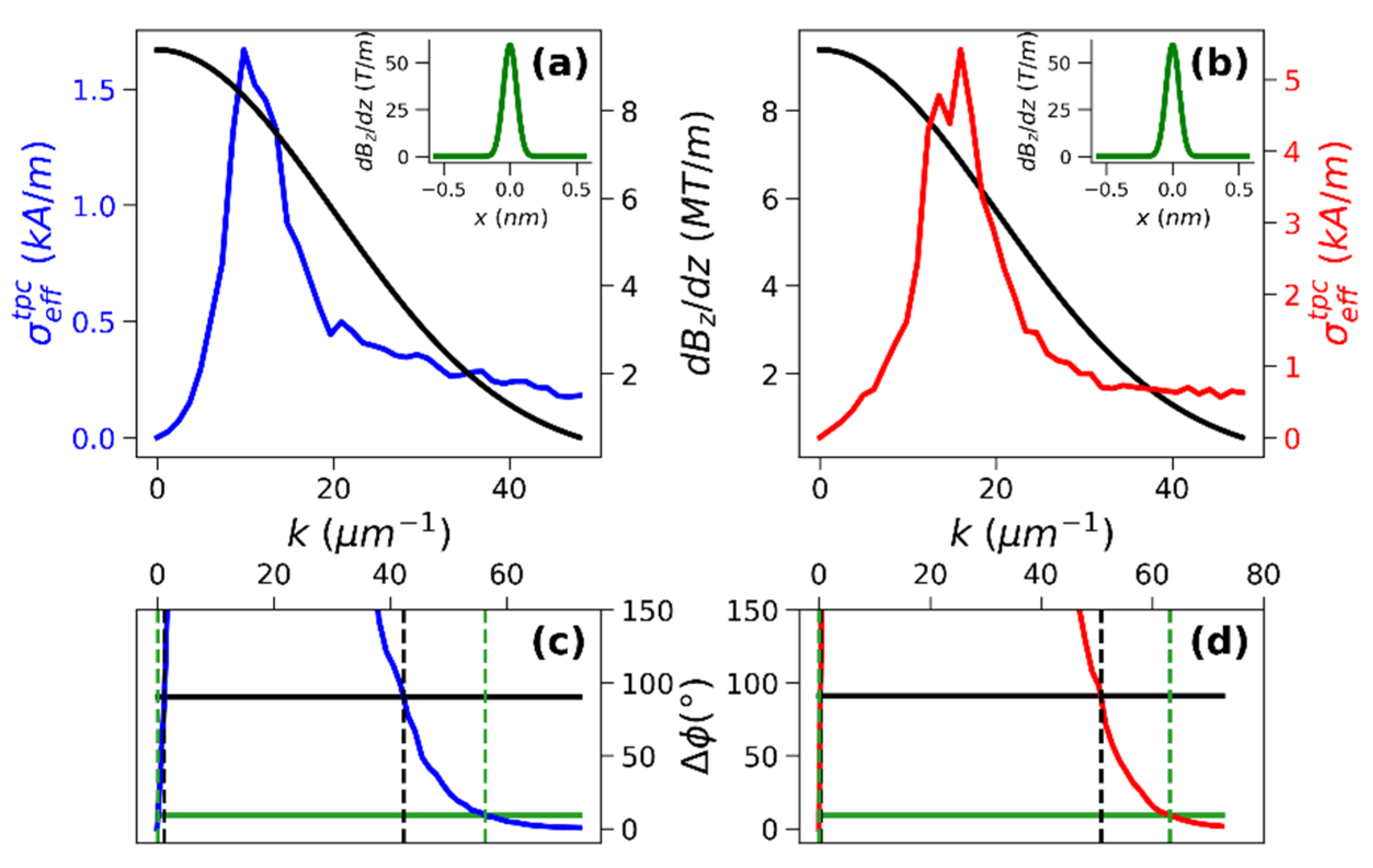
| Δϕ | Low Cut-Off | High Cut-Off | ||
|---|---|---|---|---|
| Frequency | Wavelength | Frequency | Wavelength | |
| Ti/Pt/Co multilayer Stack (tpc) | ||||
| 0.02° | <1.22 µm−1 | >5.12 µm | 42.256 µm−1 | 149 nm |
| 0.2° | <1.22 µm−1 | >5.12 µm | 56.295 µm−1 | 112 nm |
| Co/Pt Stack (ref) | ||||
| 0.02° | <1.22 µm−1 | >5.12 µm | 50.726 µm−1 | 124 nm |
| 0.2° | <1.22 µm−1 | >5.12 µm | 63.231 µm−1 | 99 nm |
Appendix G. Estimation of the Ti/Pt/Co Sample Surface Roughness

References
- Kazakova, O.; Puttock, R.; Barton, C.; Corte-León, H.; Jaafar, M.; Neu, V.; Asenjo, A. Frontiers of magnetic force microscopy. J. Appl. Phys. 2019, 125, 60901. [Google Scholar] [CrossRef]
- Amos, N.; Lavrenov, A.; Fernandez, R.; Ikkawi, R.; Litvinov, D.; Khizroev, S. High-resolution and high-coercivity FePtL10 magnetic force microscopy nanoprobes to study next-generation magnetic recording media. J. Appl. Phys. 2009, 105, 07D526. [Google Scholar] [CrossRef]
- Zhao, X.; Schwenk, J.; Mandru, A.O.; Penedo, M.; Baćani, M.; Marioni, M.A.; Hug, H.J. Magnetic force microscopy with frequency-modulated capacitive tip–sample distance control. New J. Phys. 2018, 20, 13018. [Google Scholar] [CrossRef]
- Yamaoka, T.; Watanabe, K.; Shirakawabe, Y.; Chinone, K.; Saitoh, E.; Tanaka, M.; Miyajima, H. Applications of high-resolution MFM system with low-moment probe in a vacuum. IEEE Trans. Magn. 2005, 41, 3733–3735. [Google Scholar] [CrossRef]
- Babcock, K.L.; Elings, V.B.; Shi, J.; Awschalom, D.D.; Dugas, M. Field-dependence of microscopic probes in magnetic force microscopy. Appl. Phys. Lett. 1996, 69, 705–707. [Google Scholar] [CrossRef]
- Lohau, J.; Kirsch, S.; Carl, A.; Dumpich, G.; Wassermann, E.F. Quantitative determination of effective dipole and monopole moments of magnetic force microscopy tips. J. Appl. Phys. 1999, 86, 3410–3417. [Google Scholar] [CrossRef]
- McVitie, S.; Ferrier, R.P.; Scott, J.; White, G.S.; Gallagher, A. Quantitative field measurements from magnetic force microscope tips and comparison with point and extended charge models. J. Appl. Phys. 2001, 89, 3656–3661. [Google Scholar] [CrossRef]
- Kong, L.; Chou, S.Y. Quantification of magnetic force microscopy using a micronscale current ring. Appl. Phys. Lett. 1997, 70, 2043–2045. [Google Scholar] [CrossRef]
- Kebe, T.; Carl, A. Calibration of magnetic force microscopy tips by using nanoscale current-carrying parallel wires. J. Appl. Phys. 2004, 95, 775–792. [Google Scholar] [CrossRef]
- Rice, P.; Russek, S.E.; Haines, B. Magnetic Imaging Reference Sample. IEEE Trans. Magn. 1996, 32, 4133. [Google Scholar] [CrossRef]
- Rice, P.; Russek, S.; Hoinville, J.; Kelley, M. The NIST Magnetic Imaging Reference Sample. In Proceedings of the Fourth Workshop on Industrial Applications of Scanned Probe Microscopy, Gaithersburg, MD, USA, 1997. Available online: https://tsapps.nist.gov/publication/get_pdf.cfm?pub_id=620490 (accessed on 28 May 2021).
- Corte-León, H.; Neu, V.; Manzin, A.; Barton, C.; Tang, Y.; Gerken, M.; Klapetek, P.; Schumacher, H.W.; Kazakova, O. Comparison and Validation of Different Magnetic Force Microscopy Calibration Schemes. Small 2020, 16, e1906144. [Google Scholar] [CrossRef]
- Panchal, V.; Corte-León, H.; Gribkov, B.; Rodriguez, L.A.; Snoeck, E.; Manzin, A.; Simonetto, E.; Vock, S.; Neu, V.; Kazakova, O. Calibration of multi-layered probes with low/high magnetic moments. Sci. Rep. 2017, 7, 7224. [Google Scholar] [CrossRef] [PubMed]
- Di Giorgio, C.; Scarfato, A.; Longobardi, M.; Bobba, F.; Iavarone, M.; Novosad, V.; Karapetrov, G.; Cucolo, A.M. Quantitative magnetic force microscopy using calibration on superconducting flux quanta. Nanotechnology 2019, 30. [Google Scholar] [CrossRef]
- Hug, H.J.; Stiefel, B.; Van Schendel, P.J.A.; Moser, A.; Hofer, R.; Martin, S.; Güntherodt, H.J.; Porthun, S.; Abelmann, L.; Lodder, J.C.; et al. Quantitative magnetic force microscopy on perpendicularly magnetized samples. J. Appl. Phys. 1998, 83, 5609–5620. [Google Scholar] [CrossRef]
- Van Schendel, P.J.A.; Hug, H.J.; Stiefel, B.; Martin, S.; Güntherodt, H.J. A method for the calibration of magnetic force microscopy tips. J. Appl. Phys. 2000, 88, 435–445. [Google Scholar] [CrossRef]
- Baćani, M.; Marioni, M.A.; Schwenk, J.; Hug, H.J. How to measure the local Dzyaloshinskii-Moriya Interaction in Skyrmion Thin-Film Multilayers. Sci. Rep. 2019, 9, 3114. [Google Scholar] [CrossRef]
- Zingsem, N.; Ahrend, F.; Vock, S.; Gottlob, D.; Krug, I.; Doganay, H.; Holzinger, D.; Neu, V.; Ehresmann, A. Magnetic charge distribution and stray field landscape of asymmetric néel walls in a magnetically patterned exchange bias layer system. J. Phys. D Appl. Phys. 2017, 50, 495006. [Google Scholar] [CrossRef]
- Mandru, A.-O.; Yıldırım, O.; Tomasello, R.; Heistracher, P.; Penedo, M.; Giordano, A.; Suess, D.; Finocchio, G.; Hug, H.J. Coexistence of distinct skyrmion phases observed in hybrid ferromagnetic/ferrimagnetic multilayers. Nat. Commun. 2020, 11, 6365. [Google Scholar] [CrossRef]
- Dai, G.; Hu, X.; Sievers, S.; Fernández Scarioni, A.; Neu, V.; Fluegge, J.; Schumacher, H.W. Metrological large range magnetic force microscopy. Rev. Sci. Instrum. 2018, 89, 93703. [Google Scholar] [CrossRef]
- Abelmann, L.; Porthun, S.; Haast, M.; Lodder, C.; Moser, A.; Best, M.E.; van Schendel, P.J.A.; Stiefel, B.; Hug, H.J.; Heydon, G.P.; et al. Comparing the resolution of magnetic force microscopes using the CAMST reference samples. J. Magn. Magn. Mater. 1998, 190, 135–147. [Google Scholar] [CrossRef]
- Vock, S.; Hengst, C.; Wolf, M.; Tschulik, K.; Uhlemann, M.; Sasvári, Z.; Makarov, D.; Schmidt, O.G.; Schultz, L.; Neu, V. Magnetic vortex observation in FeCo nanowires by quantitative magnetic force microscopy. Appl. Phys. Lett. 2014, 105, 172409. [Google Scholar] [CrossRef]
- Neu, V.; Vock, S.; Sturm, T.; Schultz, L. Epitaxial hard magnetic SmCo5 MFM tips-a new approach to advanced magnetic force microscopy imaging. Nanoscale 2018, 10, 16881–16886. [Google Scholar] [CrossRef]
- Gao, L.; Yue, L.P.; Yokota, T.; Skomski, R.; Liou, S.H.; Takahoshi, H.; Saito, H.; Ishio, S. Focused ion beam milled CoPt magnetic force microscopy tips for high resolution domain images. IEEE Trans. Magn. 2004, 40, 2194–2196. [Google Scholar] [CrossRef]
- Schwenk, J. Multi-modal and quantitative Magnetic Force Microscopy–Application to Thin Film Systems with interfacial Dzyaloshinskii-Moriya Interaction. Univ. Basel Basel 2016. [Google Scholar] [CrossRef]
- Hansen, P.C. The L-Curve and its Use in the Numerical Treatment of Inverse Problems. Comput. Inverse Probl. Electrocardiol. Ed. P. Johnston Adv. Comput. Bioeng. 2000, 4, 119–142. [Google Scholar]
- Engl, W.; Sulzbach, T. Force Constant Determination of AFM Cantilevers with Calibrated Thermal Tune Method. In Proceedings of the 12th Euspen International Conference, Stockholm, Sweden, 2012; pp. 292–296. Available online: https://www.euspen.eu/knowledge-base/ICE12173.pdf (accessed on 28 May 2021).
- Brand, U.; Gao, S.; Engl, W.; Sulzbach, T.; Stahl, S.W.; Milles, L.F.; Nesterov, V.; Li, Z. Comparing AFM cantilever stiffness measured using the thermal vibration and the improved thermal vibration methods with that of an SI traceable method based on MEMS. Meas. Sci. Technol. 2017, 28, 34010. [Google Scholar] [CrossRef]
- Kim, M.S.; Pratt, J.R.; Brand, U.; Jones, C.W. Report on the first international comparison of small force facilities: A pilot study at the micronewton level. Metrologia 2012, 49, 70–81. [Google Scholar] [CrossRef]
- Marti, K.; Wuethrich, C.; Aeschbacher, M.; Russi, S.; Brand, U.; Li, Z. Micro-force measurements: A new instrument at METAS. Meas. Sci. Technol. 2020, 31. [Google Scholar] [CrossRef]
- Dejong, M.D.; Livesey, K.L. Analytic theory for the switch from Bloch to Néel domain wall in nanowires with perpendicular anisotropy. Phys. Rev. B Condens. Matter Mater. Phys. 2015, 92, 214420. [Google Scholar] [CrossRef]
- Hu, X.; Dai, G.; Sievers, S.; Fernández Scarioni, A.; Corte-León, H.; Puttock, R.; Barton, C.; Kazakova, O.; Ulvr, M.; Klapetek, P.; et al. Round robin comparison on quantitative nanometer scale magnetic field measurements by magnetic force microscopy. J. Magn. Magn. Mater. 2020, 511, 166947. [Google Scholar] [CrossRef]
- Sbiaa, R.; Bilin, Z.; Ranjbar, M.; Tan, H.K.; Wong, S.J.; Piramanayagam, S.N.; Chong, T.C. Effect of magnetostatic energy on domain structure and magnetization reversal in (Co/Pd) multilayers. J. Appl. Phys. 2010, 107, 103901. [Google Scholar] [CrossRef]
- Quantum Design Accuracy of the Reported Moment: Sample Shape Effects. SQUID VSM Application Note 1500-015. Rev. A0.1 October 4-2010. Available online: https://www.qdusa.com/siteDocs/appNotes/1500-015.pdf (accessed on 28 May 2021).
- Stoner, E.C.; Wohlfarth, E.P. A mechanism of magnetic hysteresis in heterogeneous alloys. Philos. Trans. R. Soc. Lond. Ser. A Math. Phys. Sci. 1948, 240, 599–642. [Google Scholar] [CrossRef]
- Fukami, S.; Suzuki, T.; Nakatani, Y.; Ishiwata, N.; Yamanouchi, M.; Ikeda, S.; Kasai, N.; Ohno, H. Current-induced domain wall motion in perpendicularly magnetized CoFeB nanowire. Appl. Phys. Lett. 2011, 98, 82504. [Google Scholar] [CrossRef]
- Lim, S.T.; Tran, M.; Chenchen, J.W.; Ying, J.F.; Han, G. Effect of different seed layers with varying Co and Pt thicknesses on the magnetic properties of Co/Pt multilayers. J. Appl. Phys. 2015, 117, 17A731. [Google Scholar] [CrossRef]
- Öcal, M.T.; Sakar, B.; Öztoprak, İ.; Balogh-Michels, Z.; Neels, A.; Öztürk, O. Structural and Morphological Effect of Ti Underlayer on Pt/Co/Pt Magnetic Ultra-Thin Film. arXiv 2021, arXiv:2105.09355. [Google Scholar]
- Demirci, E.; Öztürk, M.; Sınır, E.; Ulucan, U.; Akdoğan, N.; Öztürk, O.; Erkovan, M. Temperature-dependent exchange bias properties of polycrystalline PtxCo1−x/CoO bilayers. Thin Solid Films 2014, 550, 595–601. [Google Scholar] [CrossRef]
- Öztürk, M.; Sınır, E.; Demirci, E.; Erkovan, M.; Öztürk, O.; Akdoğan, N. Exchange bias properties of [Co/CoO]n multilayers. J. Appl. Phys. 2012, 112, 93911. [Google Scholar] [CrossRef]
- Vansteenkiste, A.; Leliaert, J.; Dvornik, M.; Helsen, M.; Garcia-Sanchez, F.; Van Waeyenberge, B. The design and verification of MuMax3. AIP Adv. 2014, 4, 107133. [Google Scholar] [CrossRef]
- Moreau-Luchaire, C.; Moutafis, C.; Reyren, N.; Sampaio, J.; Vaz, C.A.F.; Van Horne, N.; Bouzehouane, K.; Garcia, K.; Deranlot, C.; Warnicke, P.; et al. Additive interfacial chiral interaction in multilayers for stabilization of small individual skyrmions at room temperature. Nat. Nanotechnol. 2016, 11, 444–448. [Google Scholar] [CrossRef]
- Schott, M.; Ranno, L.; Béa, H.; Baraduc, C.; Auffret, S.; Bernand-Mantel, A. Electric field control of interfacial Dzyaloshinskii-Moriya interaction in Pt/Co/AlOx thin films. J. Magn. Magn. Mater. 2021, 520, 167122. [Google Scholar] [CrossRef]
- Deger, C.; Yavuz, I.; Yildiz, F. Impact of interlayer coupling on magnetic skyrmion size. J. Magn. Magn. Mater. 2019, 489, 165399. [Google Scholar] [CrossRef]
- Lilley, B.A. LXXI. Energies and widths of domain boundaries in ferromagnetics. Lond. Edinb. Dublin Philos. Mag. J. Sci. 1950, 41, 792–813. [Google Scholar] [CrossRef]
- Thiaville, A.; Nakatani, Y. Chapter 6—Micromagnetics of Domain-Wall Dynamics in Soft Nanostrips. In Nanomagnetism and Spintronics; Shinjo, T., Ed.; Elsevier: Amsterdam, The Netherlands, 2009; pp. 231–276. ISBN 978-0-444-53114-8. [Google Scholar]
- Joint Committee for Guides in Metrology. Evaluation of Measurement Data—Guide to the Expression of Uncertainty in Measurement. International Bureau of Weights and Measures (BIPM), Sèvres, France, September 2008. BIPM, IEC, IFCC, ILAC, ISO, IUPAC, IUPAP and OIML, JCGM 100:2008, GUM 1995 with Minor Corrections. Available online: https://www.bipm.org/documents/20126/2071204/JCGM_100_2008_E.pdf/cb0ef43f-baa5-11cf-3f85-4dcd86f77bd6 (accessed on 28 May 2021).
- Hu, X.; Dai, G.; Sievers, S.; Fernández Scarioni, A.; Neu, V.; Bieler, M.; Schumacher, H.W. Uncertainty Analysis of Stray Field Measurements by Quantitative Magnetic Force Microscopy. IEEE Trans. Instrum. Meas. 2020, 69, 8187–8195. [Google Scholar] [CrossRef]
- Paynter, R.W. Modification of the Beer–Lambert equation for application to concentration gradients. Surf. Interface Anal. 1981, 3, 186–187. [Google Scholar] [CrossRef]
- Powell, C.J.; Jablonski, A. National Institute of Standards and Technology, Gaithersburg, MD. 2010. Available online: https://www.nist.gov/system/files/documents/srd/SRD71UsersGuideV1-2.pdf (accessed on 28 May 2021).
- Navas, D.; Nam, C.; Velazquez, D.; Ross, C.A. Shape and strain-induced magnetization reorientation and magnetic anisotropy in thin film Ti/CoCrPt/Ti lines and rings. Phys. Rev. B 2010, 81, 224439. [Google Scholar] [CrossRef]

| Ti/Pt/Co Multilayer Stack | Co/Pt Stack | |
|---|---|---|
| Saturation Magnetization Ms | 201 kA/m | 500 kA/m |
| Stack Thickness t | 20 × 3.8 nm | 100 × 1.3 nm |
| Domain Wall Width | 27 nm | 16 nm |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sakar, B.; Sievers, S.; Fernández Scarioni, A.; Garcia-Sanchez, F.; Öztoprak, İ.; Schumacher, H.W.; Öztürk, O. A Ti/Pt/Co Multilayer Stack for Transfer Function Based Magnetic Force Microscopy Calibrations. Magnetochemistry 2021, 7, 78. https://doi.org/10.3390/magnetochemistry7060078
Sakar B, Sievers S, Fernández Scarioni A, Garcia-Sanchez F, Öztoprak İ, Schumacher HW, Öztürk O. A Ti/Pt/Co Multilayer Stack for Transfer Function Based Magnetic Force Microscopy Calibrations. Magnetochemistry. 2021; 7(6):78. https://doi.org/10.3390/magnetochemistry7060078
Chicago/Turabian StyleSakar, Baha, Sibylle Sievers, Alexander Fernández Scarioni, Felipe Garcia-Sanchez, İlker Öztoprak, Hans Werner Schumacher, and Osman Öztürk. 2021. "A Ti/Pt/Co Multilayer Stack for Transfer Function Based Magnetic Force Microscopy Calibrations" Magnetochemistry 7, no. 6: 78. https://doi.org/10.3390/magnetochemistry7060078
APA StyleSakar, B., Sievers, S., Fernández Scarioni, A., Garcia-Sanchez, F., Öztoprak, İ., Schumacher, H. W., & Öztürk, O. (2021). A Ti/Pt/Co Multilayer Stack for Transfer Function Based Magnetic Force Microscopy Calibrations. Magnetochemistry, 7(6), 78. https://doi.org/10.3390/magnetochemistry7060078






