Magnon Excitation Modes in Ferromagnetic and Antiferromagnetic Systems
Abstract
1. Introduction
2. Overview Magnetic Resonance Modes
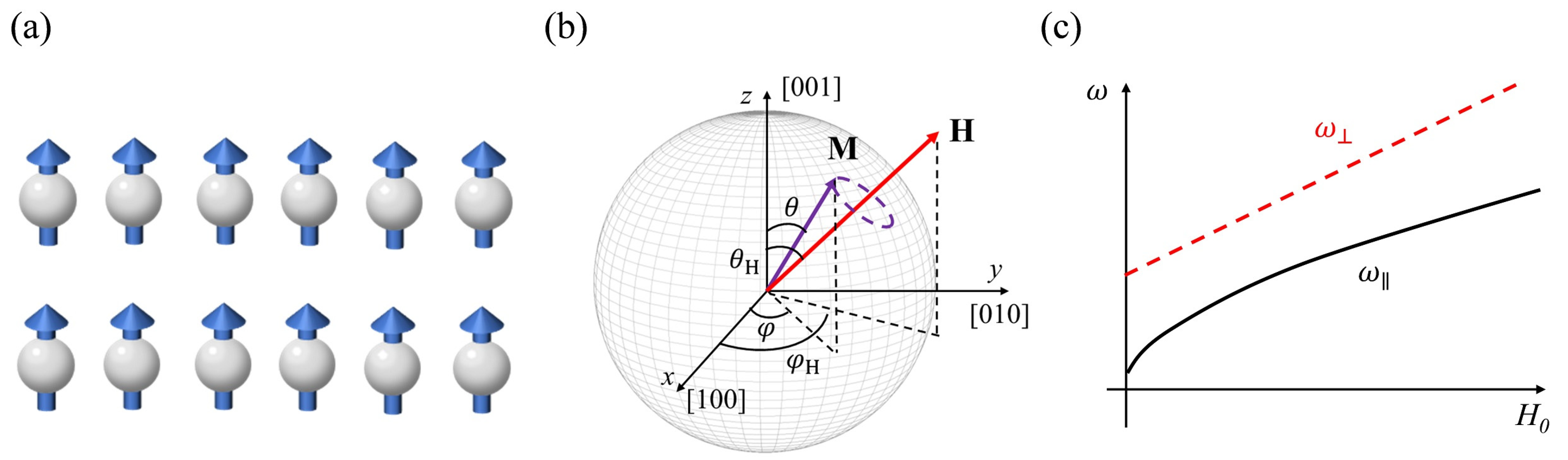
2.1. Resonance Modes in Ferromagnets
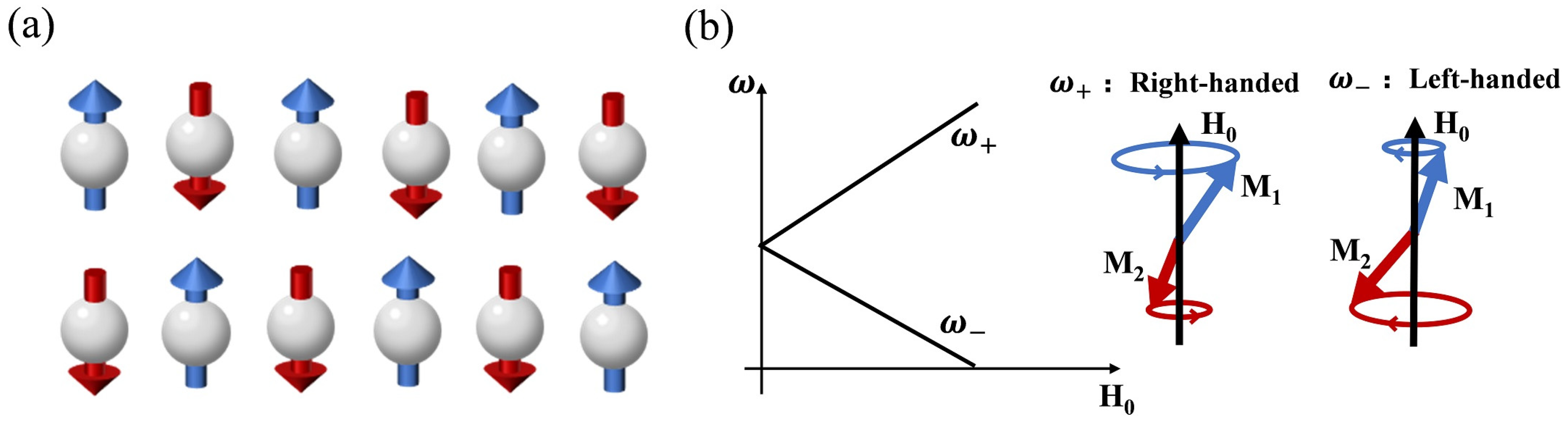
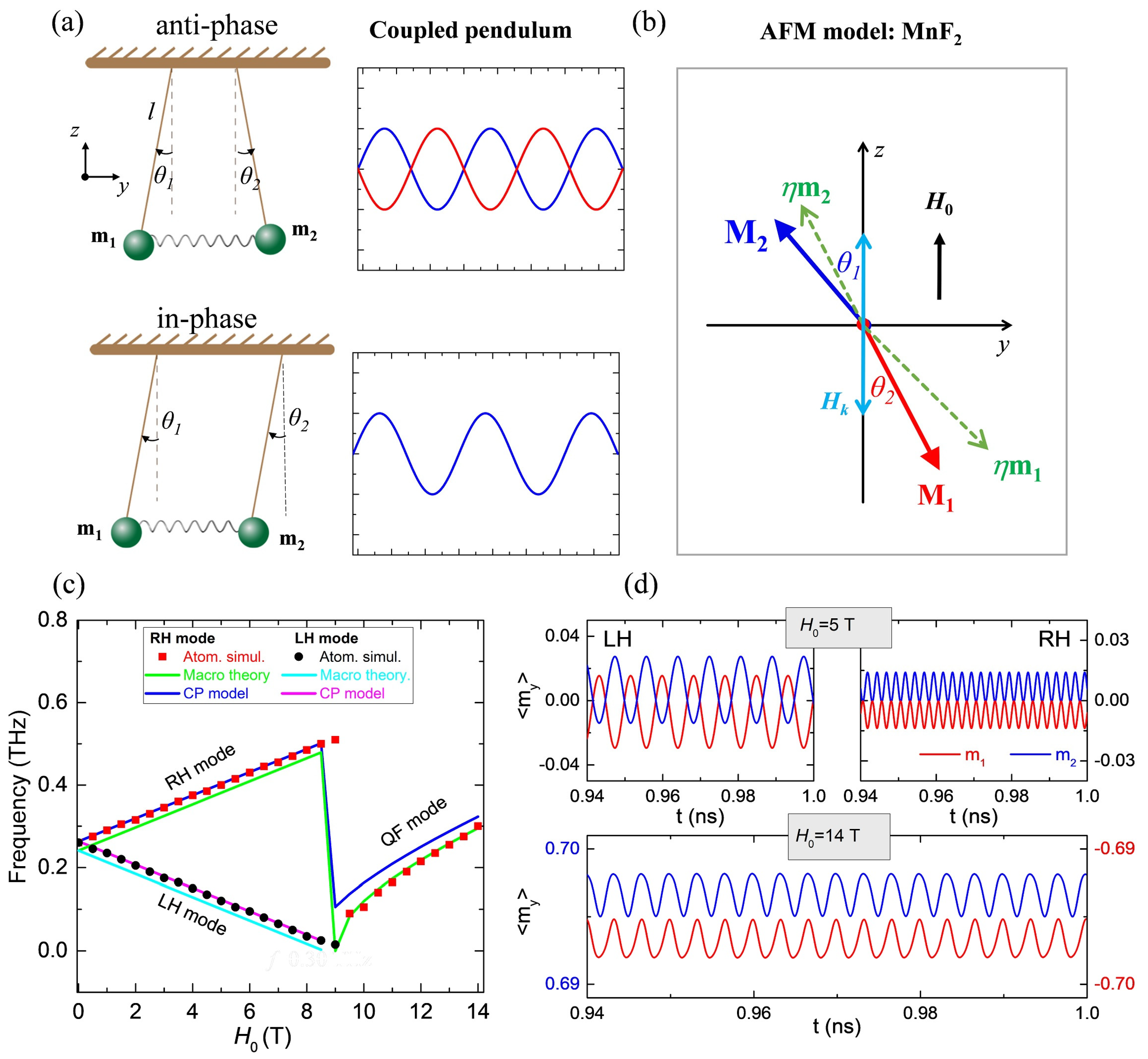
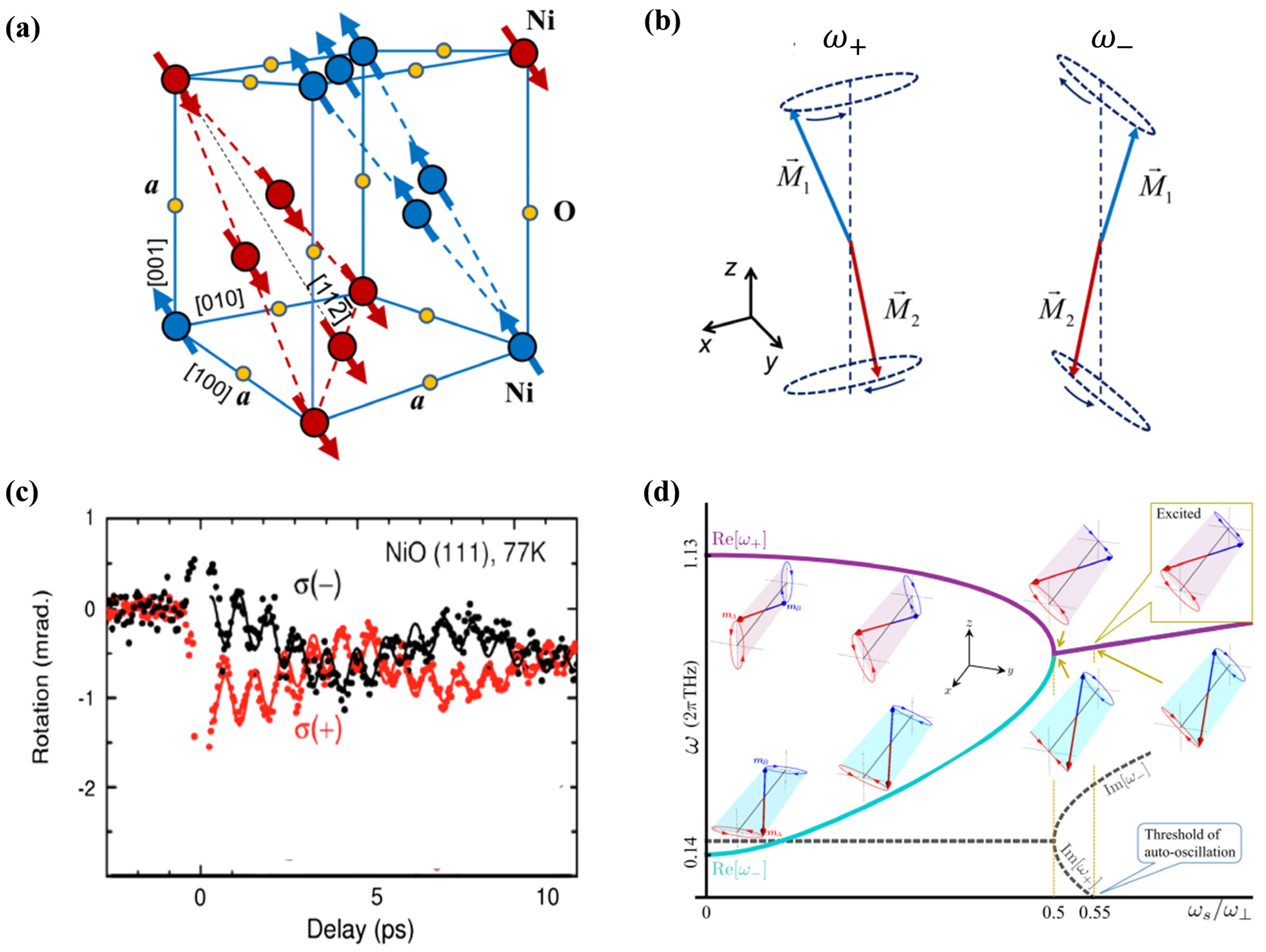
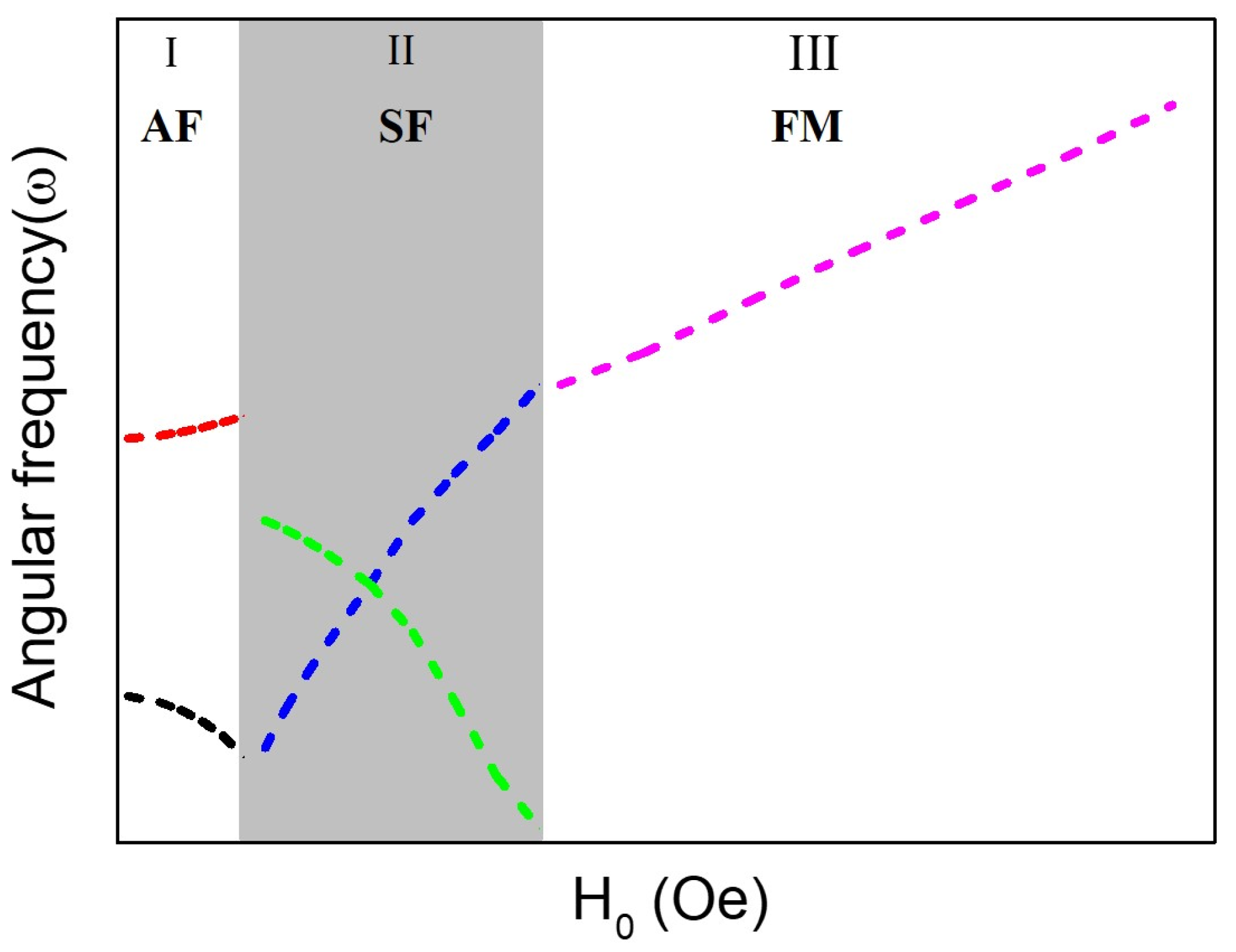
2.2. Resonance Modes in Antiferromagnets
2.3. Resonance Modes in Synthetic Antiferromagnets
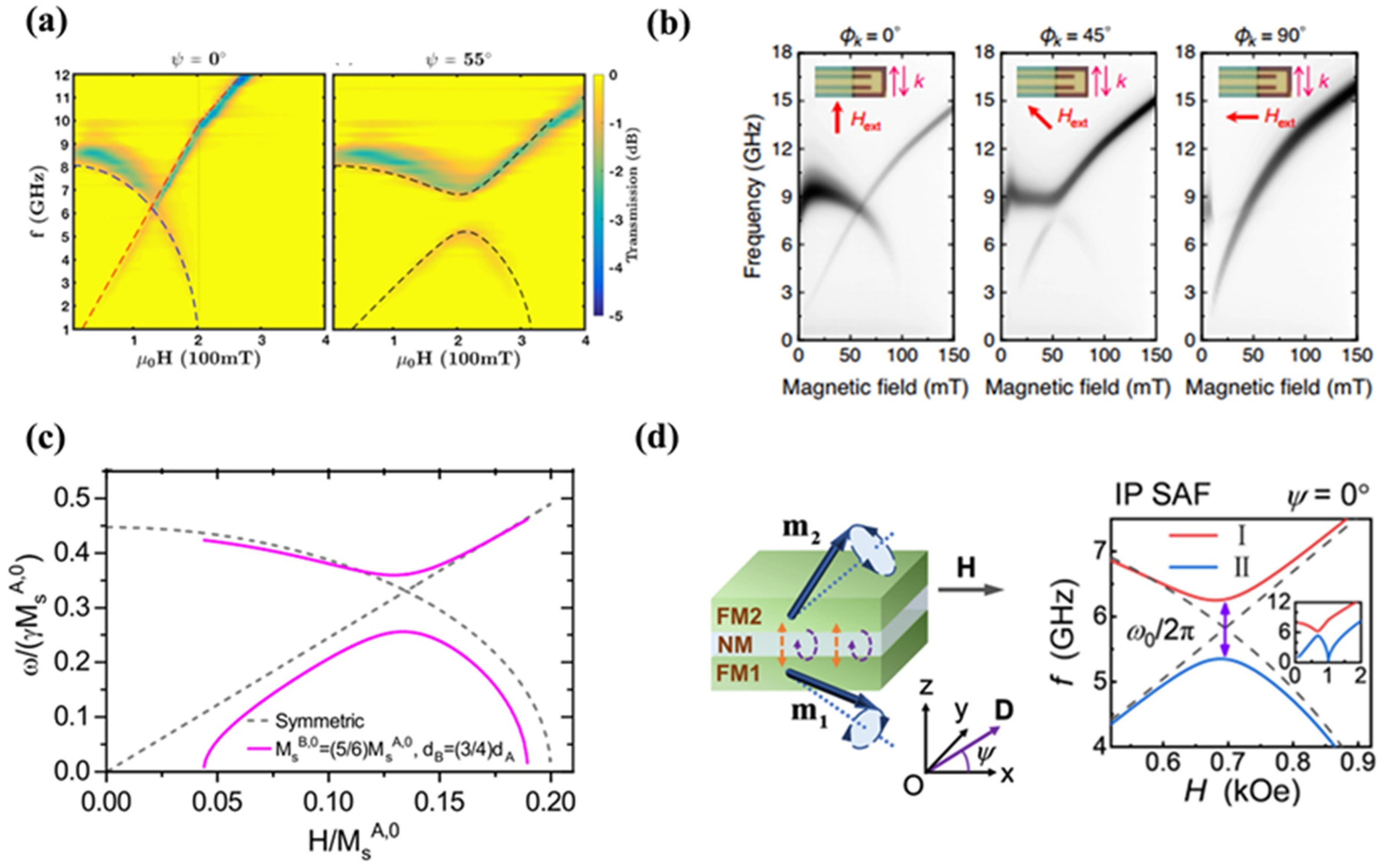
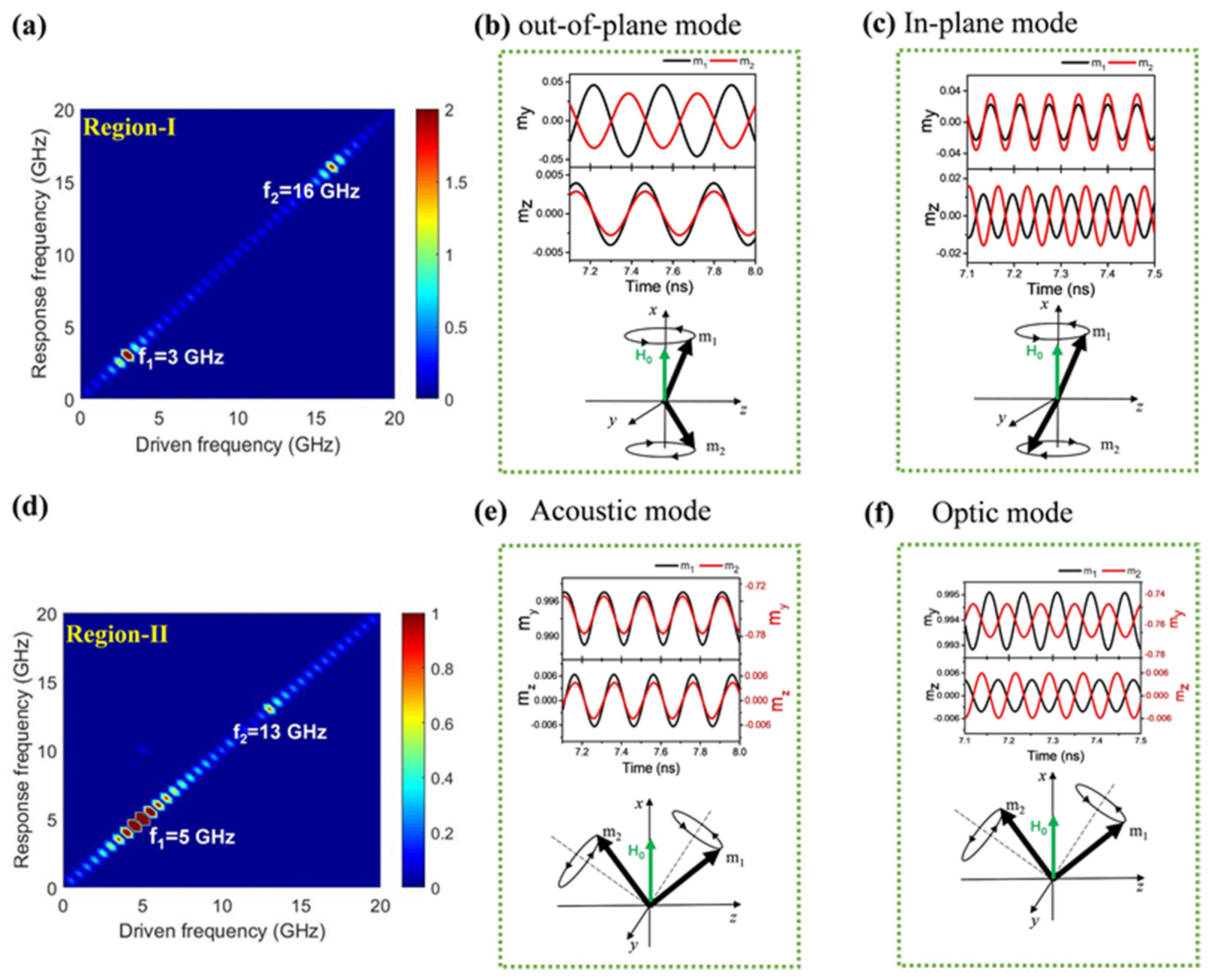
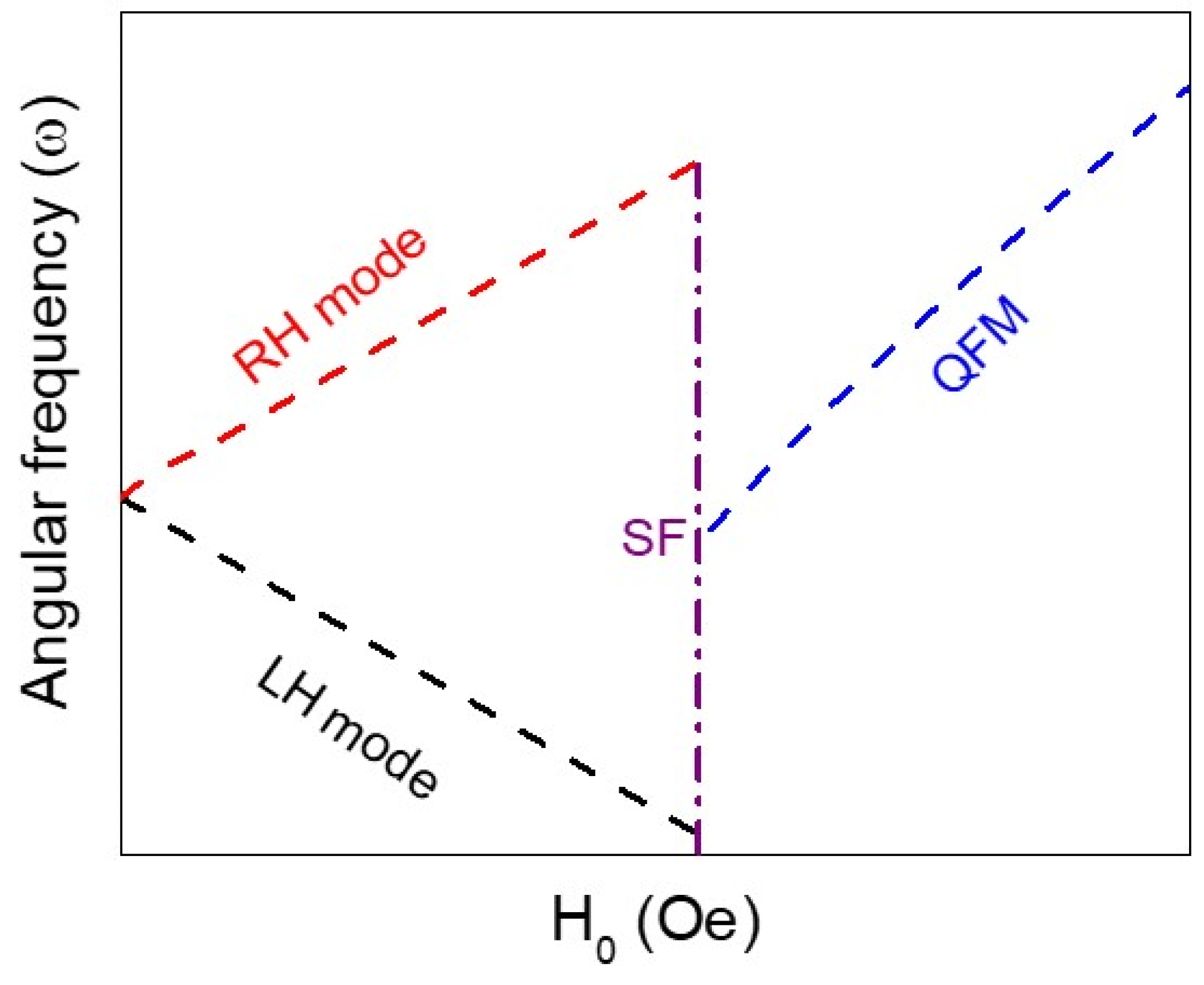
2.3.1. FMR Modes in SAFs with In-Plane Uniaxial Anisotropy
2.3.2. FMR Modes in SAFs with Perpendicular Magnetic Anisotropy
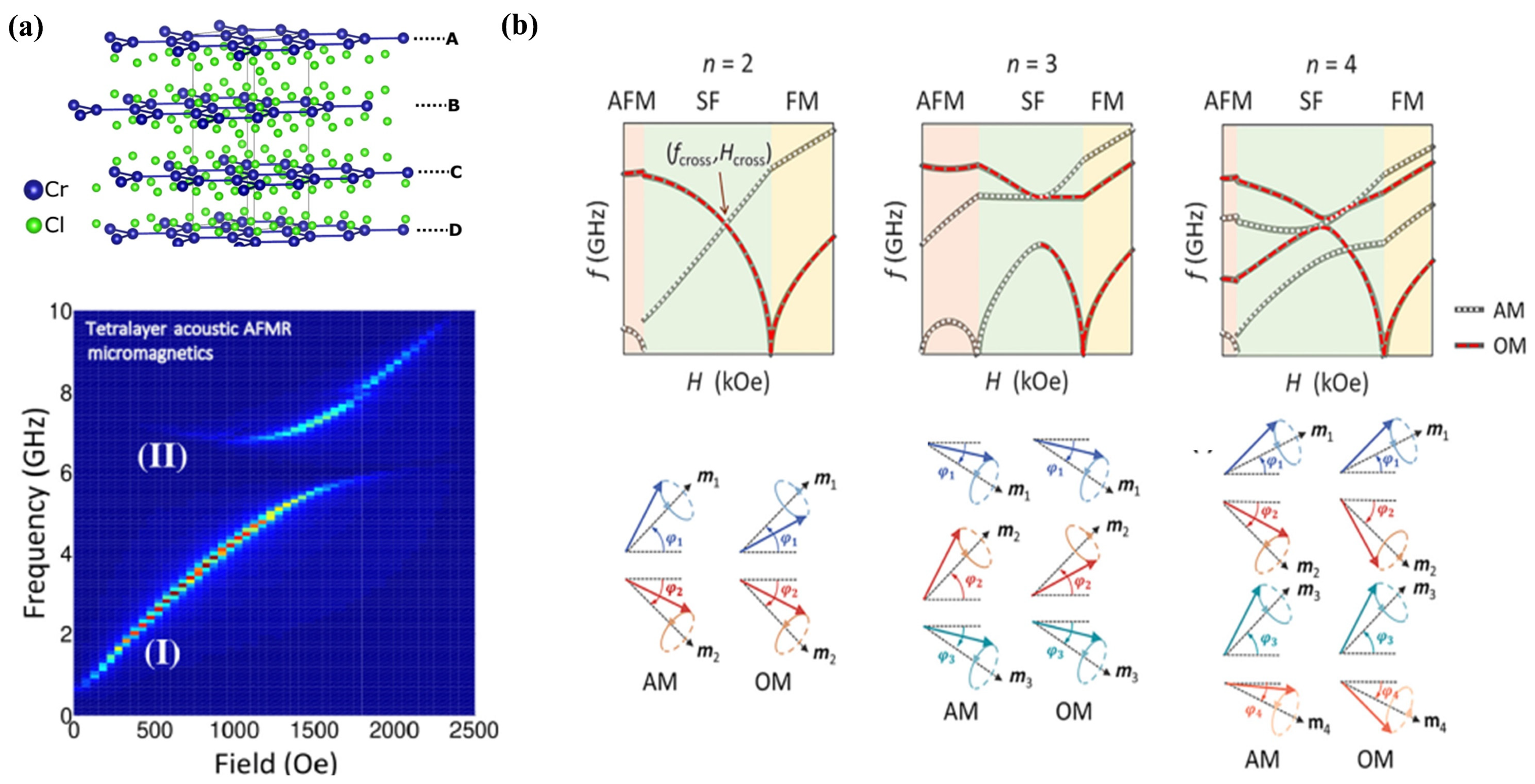
2.3.3. Magnon-Magnon Coupling
3. Conclusions and Perspectives
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Chumak, A.V.; Vasyuchka, V.I.; Serga, A.A.; Hillebrands, B. Magnon spintronics. Nat. Phys. 2015, 11, 453–461. [Google Scholar] [CrossRef]
- Barman, A.; Gubbiotti, G.; Ladak, S.; Adeyeye, A.O.; Krawczyk, M.; Gräfe, J.; Adelmann, C.; Cotofana, S.; Naeemi, A.; Vasyuchka, V.I.; et al. The 2021 Magnonics Roadmap. J. Phys. Condens. Matt. 2021, 33, 413001. [Google Scholar] [CrossRef] [PubMed]
- Yuan, H.Y.; Cao, Y.; Kamra, A.; Duine, R.A.; Yan, P. Quantum magnonics: When magnon spintronics meets quantum information science. Phys. Rep. 2022, 965, 1–74. [Google Scholar] [CrossRef]
- Li, Z.; Ma, M.; Chen, Z.; Xie, K.; Ma, F. Interaction between magnon and skyrmion: Toward quantum magnonics. J. Appl. Phys. 2022, 132, 210702. [Google Scholar] [CrossRef]
- Khitun, A.; Bao, M.; Wang, K.L. Magnonic logic circuits. J. Phys. D Appl. Phys. 2010, 43, 264005. [Google Scholar] [CrossRef]
- Lenk, B.; Ulrichs, H.; Garbs, F.; Münzenberg, M. The building blocks of magnonics. Phys. Rep. 2011, 507, 107–136. [Google Scholar] [CrossRef]
- Rezende, S.M. Fundamentals of Magnonics; Lecture notes in Physics; Springer: Berlin/Heidelberg, Germany, 2020; Volume 969. [Google Scholar]
- Rameshti, B.Z.; Kusminskiy, S.V.; Haigh, J.A.; Usami, K.; Lachance-Quirion, D.; Nakamura, Y.; Hu, C.M.; Tang, H.X.; Bauer, G.E.W.; Blanter, Y.M.; et al. Cavity magnonics. Phys. Rep. 2022, 979, 1–61. [Google Scholar] [CrossRef]
- Yu, H.; Xiao, J.; Schultheiss, H. Magnetic texture based magnonics. Phys. Rep. 2021, 905, 1–59. [Google Scholar] [CrossRef]
- Pirro, P.; Vasyuchka, V.I.; Serga, A.A.; Hillebrands, B. Advances in coherent magnonics. Nat. Rev. Mater. 2021, 6, 1114–1135. [Google Scholar] [CrossRef]
- Bloch, F. Zur Theorie des Austauschproblems und der Remanenzerscheinung der Ferromagnetika. Z. Phys. 1932, 74, 295–335. [Google Scholar] [CrossRef]
- Chumak, A.V.; Serga, A.A.; Hillebrands, B. Magnonic crystals for data processing. J. Phys. D Appl. Phys. 2017, 50, 244001. [Google Scholar] [CrossRef]
- Seki, S.; Okamura, Y.; Kondou, K.; Shibata, K.; Kubota, M.; Takagi, R.; Kagawa, F.; Kawasaki, M.; Tatara, G.; Otani, Y.; et al. Magnetochiral nonreciprocity of volume spin wave propagation in chiral-lattice ferromagnets. Phys. Rev. B 2016, 93, 235131. [Google Scholar] [CrossRef]
- Gallardo, R.A.; Alvarado-Seguel, P.; Schneider, T.; Gonzalez-Fuentes, C.; Roldán-Molina, A.; Lenz, K.; Lindner, J.; Landeros, P. Spin-wave non-reciprocity in magnetization-graded ferromagnetic films. New J. Phys. 2019, 21, 033026. [Google Scholar] [CrossRef]
- Wang, X.S.; Zhang, H.W.; Wang, X.R. Topological Magnonics: A Paradigm for Spin-Wave Manipulation and Device Design. Phys. Rev. Appl. 2018, 9, 024029. [Google Scholar] [CrossRef]
- Yao, W.; Li, C.; Wang, L.; Xue, S.; Dan, Y.; Iida, K.; Kamazawa, K.; Li, K.K.; Fang, C.; Li, Y. Topological spin excitations in a three-dimensional antiferromagnet. Nat. Phys. 2018, 14, 1011–1015. [Google Scholar] [CrossRef]
- Popov, P.A.; Sharaevskaya, A.Y.; Beginin, E.N.; Sadovnikov, A.V.; Stognij, A.I.; Kalyabin, D.V.; Nikitov, S.A. Spin wave propagation in three-dimensional magnonic crystals and coupled structures. J. Magn. Magn. Mater. 2019, 476, 423–427. [Google Scholar] [CrossRef]
- Demokritov, S.; Demidov, V.; Dzyapko, O.; Melkov, G.; Serga, A.A.; Hillebrands, B.; Slavin, A.N. Bose-Einstein condensation of quasi-equilibrium magnons at room temperature under pumping. Nature 2006, 443, 430–433. [Google Scholar] [CrossRef]
- Serga, A.A.; Tiberkevich, V.S.; Sandweg, C.W.; Vasyuchka, V.I.; Bozhko, D.A.; Chumak, A.V.; Neumann, T.; Obry, B.; Melkov, G.A.; Slavin, A.N.; et al. Bose–Einstein condensation in an ultra-hot gas of pumped magnons. Nat. Commun. 2014, 5, 3452. [Google Scholar] [CrossRef]
- Bozhko, D.A.; Serga, A.A.; Clausen, P.; Vasyuchka, V.I.; Heussner, F.; Melkov, G.A.; Pomyalov, A.; L’vov, V.S.; Hillebrands, B. Supercurrent in a room-temperature Bose–Einstein magnon condensate. Nat. Phys. 2016, 12, 1057–1062. [Google Scholar] [CrossRef]
- Safranski, C.; Barsukov, I.; Lee, H.K.; Schneider, T.; Jara, A.A.; Smith, A.; Chang, H.; Lenz, K.; Lindner, J.; Tserkovnyak, Y.; et al. Spin caloritronic nano-oscillator. Nat. Commun. 2017, 8, 117. [Google Scholar] [CrossRef]
- Walowski, J.; Münzenberg, M. Perspective: Ultrafast magnetism and THz spintronics. J. Appl. Phys. 2016, 120, 140901. [Google Scholar] [CrossRef]
- Baltz, V.; Manchon, A.; Tsoi, M.; Moriyama, T.; Ono, T.; Tserkovnyak, Y. Antiferromagnetic spintronics. Rev. Mod. Phys. 2018, 90, 015005. [Google Scholar] [CrossRef]
- Jungwirth, T.; Sinova, J.; Manchon, A.; Marti, X.; Wunderlich, J.; Felser, C. The multiple directions of antiferromagnetic spintronics. Nat. Phys. 2018, 14, 200–203. [Google Scholar] [CrossRef]
- Gomonay, O.; Baltz, V.; Brataas, A.; Tserkovnyak, Y. Antiferromagnetic spin textures and dynamics. Nat. Phys. 2018, 14, 213–216. [Google Scholar] [CrossRef]
- Razdolski, I.; Alekhin, A.; Ilin, N.; Meyburg, J.P.; Roddatis, V.; Diesing, D.; Bovensiepen, U.; Melnikov, A. Nanoscale interface confinement of ultrafast spin transfer torque driving non-uniform spin dynamics. Nat. Commun. 2017, 8, 15007. [Google Scholar] [CrossRef] [PubMed]
- Kampfrath, T.; Sell, A.; Klatt, G.; Pashkin, A.; Mährlein, S.; Dekorsy, T.; Wolf, M.; Fiebig, M.; Leitenstorfer, A.; Huber, R. Coherent terahertz control of antiferromagnetic spin waves. Nat. Photo. 2011, 5, 31–34. [Google Scholar] [CrossRef]
- Patil, R.A.; Su, C.-W.; Chuang, C.-J.; Lai, C.-C.; Liou, Y.; Ma, Y.R. Terahertz spin-wave waveguides and optical magnonics in one-dimensional NiO nanorods. Nanoscale 2016, 8, 12970–12976. [Google Scholar] [CrossRef] [PubMed]
- Cheng, R.; Xiao, D.; Brataas, A. Terahertz Antiferromagnetic Spin Hall Nano-Oscillator. Phys. Rev. Lett. 2016, 116, 207603. [Google Scholar] [CrossRef] [PubMed]
- Vaidya, P.; Morley, S.A.; van Tol, J.; Liu, Y.; Cheng, R.; Brataas, A.; Lederman, D.; del Barco, E. Subterahertz spin pumping from an insulating antiferromagnet. Science 2020, 368, 160. [Google Scholar] [CrossRef]
- Olejník, K.; Seifert, T.; Kašpar, Z.; Novák, V.; Wadley, P.; Campion, R.P.; Baumgartner, M.; Gambardella, P.; Nemec, P.; Wunderlich, J. Terahertz electrical writing speed in an antiferromagnetic memory. Sci. Adv. 2017, 4, eaar3566. [Google Scholar] [CrossRef]
- Yang, Y.; Wilson, R.B.; Gorchon, J.; Lambert, C.-H.; Salahuddin, S.; Bokor, J. Ultrafast magnetization reversal by picosecond electrical pulses. Sci. Adv. 2017, 3, 1603117. [Google Scholar] [CrossRef] [PubMed]
- Griffiths, J.H.E. Anomalous High-frequency Resistance of Ferromagnetic Metals. Nature 1946, 158, 670. [Google Scholar] [CrossRef]
- Landau, L.D.; Lifshitz, E.M. On the theory of the dispersion of magnetic permeability in ferromagnetic bodies. Phys. Z. Sowjet 1935, 8, 153–169. [Google Scholar]
- Zhang, S.; Lin, J.; Miao, G.-X.; Li, S.; Zhao, G.; Wang, X.; Li, Q.; Cao, D.; Xu, J.; Yan, S. Ultrahigh Frequency and Anti-Interference Optical-Mode Resonance with Biquadratic Coupled FeCoB/Ru/FeCoB Trilayers. ACS Appl. Mater. Interfaces 2019, 11, 48230–48238. [Google Scholar] [CrossRef] [PubMed]
- Chen, X.; Zheng, C.; Zhou, S.; Liu, Y.; Zhang, Z. Ferromagnetic resonance modes of a synthetic antiferromagnet at low magnetic fields. J. Phys. Condens. Matt. 2021, 34, 015802. [Google Scholar] [CrossRef] [PubMed]
- Chen, X.; Zheng, C.; Zhang, Y.; Zhou, S.; Liu, Y.; Zhang, Z. Identification and manipulation of spin wave polarizations in perpendicularly magnetized synthetic antiferromagnets. New J. Phys. 2021, 23, 113029. [Google Scholar] [CrossRef]
- Slonczewski, J.C. Current-driven excitation of magnetic multilayers. J. Magn. Magn. Mater. 1996, 159, L1–L7. [Google Scholar] [CrossRef]
- Berger, L. Emission of spin waves by a magnetic multilayer traversed by a current. Phys. Rev. B 1996, 54, 9353–9358. [Google Scholar] [CrossRef]
- Tulapurkar, A.A.; Suzuki, Y.; Fukushima, A.; Kubota, H.; Maehara, H.; Tsunekawa, K.; Djayaprawira, D.D.; Watanabe, N.; Yuasa, S. Spin-torque diode effect in magnetic tunnel junctions. Nature 2005, 438, 339–342. [Google Scholar] [CrossRef]
- Yu, T.; Naganuma, H.; Oogane, M.; Ando, Y. DC Bias Reversal Behavior of Spin-Torque Ferromagnetic Resonance Spectra in CoFeB/MgO/CoFeB Perpendicular Magnetic Tunnel Junction. IEEE Trans. Magn. 2017, 53, 1–5. [Google Scholar] [CrossRef]
- Sankey, J.C.; Braganca, P.M.; Garcia, A.G.F.; Krivorotov, I.N.; Buhrman, R.A.; Ralph, D.C. Spin-Transfer-Driven Ferromagnetic Resonance of Individual Nanomagnets. Phys. Rev. Lett. 2006, 96, 227601. [Google Scholar] [CrossRef] [PubMed]
- Chen, W.; Beaujour, J.M.L.; de Loubens, G.; Kent, A.D.; Sun, J.Z. Spin-torque driven ferromagnetic resonance of Co/Ni synthetic layers in spin valves. Appl. Phys. Lett. 2008, 92, 012507. [Google Scholar] [CrossRef]
- Tsoi, M.; Jansen, A.G.M.; Bass, J.; Chiang, W.C.; Seck, M.; Tsoi, V.; Wyder, P. Excitation of a Magnetic Multilayer by an Electric Current. Phys. Rev. Lett. 1998, 80, 4281–4284. [Google Scholar] [CrossRef]
- Kiselev, S.I.; Sankey, J.C.; Krivorotov, I.N.; Emley, N.C.; Schoelkopf, R.J.; Buhrman, R.A.; Ralph, D.C. Microwave oscillations of a nanomagnet driven by a spin-polarized current. Nature 2003, 425, 380–383. [Google Scholar] [CrossRef] [PubMed]
- Fuchs, G.D.; Emley, N.C.; Krivorotov, I.N.; Braganca, P.M.; Ryan, E.M.; Kiselev, S.I.; Sankey, J.C.; Ralph, D.C.; Buhrman, R.A.; Katine, J.A. Spin-transfer effects in nanoscale magnetic tunnel junctions. Appl. Phys. Lett. 2004, 85, 1205–1207. [Google Scholar] [CrossRef]
- Rippard, W.H.; Pufall, M.R.; Kaka, S.; Russek, S.E.; Silva, T.J. Direct-Current Induced Dynamics in Co90Fe10/Ni80Fe20 Point Contacts. Phys. Rev. Lett. 2004, 92, 027201. [Google Scholar] [CrossRef] [PubMed]
- Houssameddine, D.; Ebels, U.; Delaet, B.; Rodmacq, B.; Firastrau, I.; Ponthenier, F.; Brunet, M.; Thirion, C.; Michel, J.-P.; Prejbeanu-Buda, L.; et al. Spin-torque oscillator using a perpendicular polarizer and a planar free layer. Nat. Mater. 2007, 6, 447–453. [Google Scholar] [CrossRef] [PubMed]
- Kubota, H.; Fukushima, A.; Yakushiji, K.; Nagahama, T.; Yuasa, S.; Ando, K.; Maehara, H.; Nagamine, Y.; Tsunekawa, K.; Djayaprawira, D.D.; et al. Quantitative measurement of voltage dependence of spin-transfer torque in MgO-based magnetic tunnel junctions. Nat. Phys. 2008, 4, 37–41. [Google Scholar] [CrossRef]
- Zeng, Z.; Finocchio, G.; Zhang, B.; Amiri, P.K.; Katine, J.A.; Krivorotov, I.N.; Huai, Y.M.; Langer, J.; Azzerboni, B.; Wang, K.L.; et al. Ultralow-current-density and bias-field-free spin-transfer nano-oscillator. Sci. Rep. 2013, 3, 1426. [Google Scholar] [CrossRef]
- Fuchs, G.D.; Sankey, J.C.; Pribiag, V.S.; Qian, L.; Braganca, P.M.; Garcia, A.G.F.; Ryan, E.M.; Li, Z.P.; Ozatay, O.; Ralph, D.C.; et al. Spin-Torque Ferromagnetic Resonance Measurements of Damping in Nanomagnets. Appl. Phys. Lett. 2007, 91, 062507. [Google Scholar] [CrossRef]
- Kupferschmidt, J.N.; Adam, S.; Brouwer, P.W. Theory of the spin-torque-driven ferromagnetic resonance in a ferromagnet/normal-metal/ferromagnet structure. Phys. Rev. B 2006, 74, 134416. [Google Scholar] [CrossRef]
- Kovalev, A.A.; Bauer, G.E.W.; Brataas, A. Current-driven ferromagnetic resonance, mechanical torques, and rotary motion in magnetic nanostructures. Phys. Rev. B 2007, 75, 014430. [Google Scholar] [CrossRef]
- Tserkovnyak, Y.; Brataas, A.; Bauer, G.E.W. Spin pumping and magnetization dynamics in metallic multilayers. Phys. Rev. B 2002, 66, 224403. [Google Scholar] [CrossRef]
- Torres, L.; Finocchio, G.; Lopez-Diaz, L.; Martinez, E.; Carpentieri, M.; Consolo, G.; Azzerboni, B. Micromagnetic modal analysis of spin-transfer-driven ferromagnetic resonance of individual nanomagnets. J. Appl. Phys. 2007, 101, A502–A503. [Google Scholar] [CrossRef]
- Sankey, J.C.; Cui, Y.-T.; Sun, J.Z.; Slonczewski, J.C.; Buhrman, R.A.; Ralph, D.C. Measurement of the spin-transfer-torque vector in magnetic tunnel junctions. Nat. Phys. 2008, 4, 67–71. [Google Scholar] [CrossRef]
- Frankowski, M.; Chȩciński, J.; Skowroński, W.; Stobiecki, T. Perpendicular magnetic anisotropy influence on voltage-driven spin-diode effect in magnetic tunnel junctions: A micromagnetic study. J. Magn. Magn. Mater. 2017, 429, 11–15. [Google Scholar] [CrossRef]
- Borlenghi, S.; Mahani, M.R.; Fangohr, H.; Franchin, M.; Delin, A.; Fransson, J. Micromagnetic simulations of spin-torque driven magnetization dynamics with spatially resolved spin transport and magnetization texture. Phys. Rev. B 2017, 96, 094428. [Google Scholar] [CrossRef]
- Chernyshov, A.; Overby, M.; Liu, X.; Furdyna, J.K.; Lyanda-Geller, Y.; Rokhinson, L.P. Evidence for reversible control of magnetization in a ferromagnetic material by means of spin–orbit magnetic field. Nat. Phys. 2009, 5, 656. [Google Scholar] [CrossRef]
- Fang, D.; Kurebayashi, H.; Wunderlich, J.; Vyborny, K.; Zarbo, L.P.; Campion, R.P.; Casiraghi, A.; Gallagher, B.L.; Jungwirth, T.; Ferguson, A.J. Spin-orbit-driven ferromagnetic resonance. Nat. Nanotech. 2011, 6, 413–417. [Google Scholar] [CrossRef]
- Miron, I.M.; Garello, K.; Gaudin, G.; Zermatten, P.-J.; Costache, M.V.; Auffret, S.; Bandiera, S.; Rodmacq, B.; Schuhl, A.; Gambardella, P. Perpendicular switching of a single ferromagnetic layer induced by in-plane current injection. Nature 2011, 476, 189–193. [Google Scholar] [CrossRef]
- Hirsch, J.E. Spin Hall Effect. Phys. Rev. Lett. 1999, 83, 1834–1837. [Google Scholar] [CrossRef]
- Zhang, S. Spin Hall Effect in the Presence of Spin Diffusion. Phys. Rev. Lett. 2000, 85, 393–396. [Google Scholar] [CrossRef] [PubMed]
- Valenzuela, S.O.; Tinkham, M. Direct electronic measurement of the spin Hall effect. Nature 2006, 442, 176. [Google Scholar] [CrossRef] [PubMed]
- Liu, L.; Moriyama, T.; Ralph, D.C.; Buhrman, R.A. Spin-Torque Ferromagnetic Resonance Induced by the Spin Hall Effect. Phys. Rev. Lett. 2011, 106, 036601. [Google Scholar] [CrossRef] [PubMed]
- Nan, T.; Emori, S.; Boone, C.T.; Wang, X.; Oxholm, T.M.; Jones, J.G.; Howe, B.M.; Brown, G.J.; Sun, N.X. Comparison of spin-orbit torques and spin pumping across NiFe/Pt and NiFe/Cu/Pt interfaces. Phys. Rev. B 2015, 91, 214416. [Google Scholar] [CrossRef]
- Sinova, J.; Valenzuela, S.O.; Wunderlich, J.; Back, C.H.; Jungwirth, T. Spin Hall effects. Rev. Mod. Phys. 2015, 87, 1213–1260. [Google Scholar] [CrossRef]
- Fan, Y.; Upadhyaya, P.; Kou, X.; Lang, M.; Takei, S.; Wang, Z.X.; Tang, J.S.; He, L.; Chang, L.T.; Montazeri, M.; et al. Magnetization switching through giant spin–orbit torque in a magnetically doped topological insulator heterostructure. Nat. Mater. 2014, 13, 699–704. [Google Scholar] [CrossRef]
- Shiomi, Y.; Nomura, K.; Kajiwara, Y.; Eto, K.; Novak, M.; Segawa, K.; Ando, Y.; Saitoh, E. Spin-electricity conversion induced by spin injection into topological insulators. Phys. Rev. Lett. 2014, 113, 196601. [Google Scholar] [CrossRef]
- Lee, J.S.; Richardella, A.; Hickey, D.R.; Mkhoyan, K.A.; Samarth, N. Mapping the chemical potential dependence of current-induced spin polarization in a topological insulator. Phys. Rev. B 2015, 92, 155312. [Google Scholar] [CrossRef]
- Kondou, K.; Yoshimi, R.; Tsukazaki, A.; Fukuma, Y.; Matsuno, J.; Takahashi, K.S.; Kawasaki, M.; Tokura, Y.; Otani, Y. Fermi-level-dependent charge-to-spin current conversion by Dirac surface states of topological insulators. Nat. Phys. 2016, 12, 1027–1031. [Google Scholar] [CrossRef]
- Zhang, W.; Jungfleisch, M.B.; Jiang, W.; Pearson, J.E.; Hoffmann, A.; Freimuth, F.; Mokrousov, Y. Spin Hall Effects in Metallic Antiferromagnets. Phys. Rev. Lett. 2014, 113, 196602. [Google Scholar] [CrossRef] [PubMed]
- Kimata, M.; Chen, H.; Kondou, K.; Sugimoto, S.; Muduli, P.K.; Ikhlas, M.; Omori, Y.; Tomita, T.; MacDonald, A.H.; Nakatsuji, S.; et al. Magnetic and magnetic inverse spin Hall effects in a non-collinear antiferromagnet. Nature 2019, 565, 627–630. [Google Scholar] [CrossRef] [PubMed]
- Chen, X.; Shi, S.; Shi, G.; Fan, X.; Song, C.; Zhou, X.; Bai, H.; Liao, L.; Zhou, Y.; Zhang, H.; et al. Observation of the antiferromagnetic spin Hall effect. Nat. Mater. 2021, 20, 800–804. [Google Scholar] [CrossRef] [PubMed]
- Hu, S.; Shao, D.F.; Yang, H.; Pan, C.; Fu, Z.; Tang, M.; Yang, Y.; Fan, W.; Zhou, S.; Tsymbal, E.Y.; et al. Efficient perpendicular magnetization switching by a magnetic spin Hall effect in a noncollinear antiferromagnet. Nat. Commun. 2022, 13, 4447. [Google Scholar] [CrossRef] [PubMed]
- Kalarickal, S.S.; Krivosik, P.; Wu, M.; Patton, C.E.; Schneider, M.L.; Kabos, P.; Silva, T.J.; Nibarger, J.P. Ferromagnetic resonance linewidth in metallic thin films: Comparison of measurement methods. J. Appl. Phys. 2006, 99, 3060–3645. [Google Scholar] [CrossRef]
- Banholzer, A.; Narkowicz, R.; Hassel, C.; Meckenstock, R.; Stienen, S.; Posth, O.; Suter, D.; Farle, M.; Lindner, J. Visualization of spin dynamics in single nanosized magnetic elements. Nanotechnology 2011, 22, 295713. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Azevedo, A.; Santos, O.A.; Guerra, G.A.F.; Cunha, R.O.; Rodriguez-Suarez, R.; Rezende, S.M. Competing spin pumping effects in magnetic hybrid structures. Appl. Phys. Lett. 2014, 104, 117601. [Google Scholar] [CrossRef]
- Freeman, M.R.; Brady, M.J.; Smyth, J. Extremely high frequency pulse magnetic resonance by picosecond magneto-optic sampling. Appl. Phys. Lett. 1992, 60, 2555–2557. [Google Scholar] [CrossRef]
- Neudecker, I.; Perzlmaier, K.; Hoffmann, F.; Woltersdorf, G.; Buess, M.; Weiss, D.; Back, C.H. Modal spectrum of permalloy disks excited by in-plane magnetic fields. Phys. Rev. B 2006, 73, 134426. [Google Scholar] [CrossRef]
- Eschenlohr, A.; Battiato, M.; Maldonado, P.; Pontius, N.; Kachel, T.; Holldack, K.; Mitzner, R.; Foehlisch, A.; Oppeneer, P.M.; Stamm, C. Ultrafast spin transport as key to femtosecond demagnetization. Nat. Mater. 2013, 12, 332–336. [Google Scholar] [CrossRef]
- Boone, C.T.; Nembach, H.T.; Shaw, J.M.; Silva, T.J. Spin transport parameters in metallic multilayers determined by ferromagnetic resonance measurements of spin-pumping. J. Appl. Phys. 2013, 113, 1217–1226. [Google Scholar] [CrossRef]
- Capua, A.; Yang, S.-H.; Phung, T.; Parkin, S.S.P. Determination of intrinsic damping of perpendicularly magnetized ultrathin films from time-resolved precessional magnetization measurements. Phys. Rev. B 2015, 92, 224402. [Google Scholar] [CrossRef]
- Schreiber, F.; Hoffmann, M.; Geisau, O.; Pelzl, J. Investigation of the photothermally modulated ferromagnetic resonance signal from magnetostatic modes in yttrium iron garnet films. Appl. Phys. A 1993, 57, 545–551. [Google Scholar] [CrossRef]
- Meckenstock, R. Invited Review Article: Microwave spectroscopy based on scanning thermal microscopy: Resolution in the nanometer range. Rev. Sci. Instrum. 2008, 79, 041101. [Google Scholar] [CrossRef] [PubMed]
- Bilzer, C.; Devolder, T.; Crozat, P.; Chappert, C.; Cardoso, S.; Freitas, P.P. Vector network analyzer ferromagnetic resonance of thin films on coplanar waveguides: Comparison of different evaluation methods. J. Appl. Phys. 2007, 101, 074505. [Google Scholar] [CrossRef]
- Michael, F. Ferromagnetic resonance of ultrathin metallic layers. Rep. Prog. Phys. 1998, 61, 755. [Google Scholar]
- Kittel, C. On the Theory of Ferromagnetic Resonance Absorption. Phys. Rev. 1948, 73, 155–161. [Google Scholar] [CrossRef]
- Smit, J.; Beljers, H.G. Ferromagnetic resonance absorption in BaFe12O19, a highly anisotropic crystal. Philips Res. Rep. 1955, 10, 113. [Google Scholar]
- Phuoc, N.N.; Hung, L.T.; Ong, C.K. Ultra-high ferromagnetic resonance frequency in exchange-biased system. J. Alloys Compd. 2010, 506, 504–507. [Google Scholar] [CrossRef]
- Layadi, A. Ferromagnetic resonance modes in single and coupled layers with oblique anisotropy axis. Phys. Rev. B 2001, 63, 174410. [Google Scholar] [CrossRef]
- Wang, C.; Zhang, S.; Qiao, S.; Du, H.; Liu, X.; Sun, R.; Chu, X.; Miao, G.; Dai, Y.; Kang, S.; et al. Dual-mode ferromagnetic resonance in an FeCoB/Ru/FeCoB synthetic antiferromagnet with uniaxial anisotropy. Appl. Phys. Lett. 2018, 112, 192401. [Google Scholar] [CrossRef]
- Castel, V.; Youssef, J.B.; Boust, F.; Weil, R.; Pigeau, B.; De Loubens, G.; Naletov, V.V.; Klein, O.; Vukadinovic, N. Perpendicular ferromagnetic resonance in soft cylindrical elements: Vortex and saturated states. Phys. Rev. B 2012, 85, 184419. [Google Scholar] [CrossRef]
- Nozaki, T.; Shiota, Y.; Miwa, S.; Murakami, S.; Bonell, F.; Ishibashi, S.; Kubota, H.; Yakushiji, K.; Saruya, T.; Fukushima, A. Electric-field-induced ferromagnetic resonance excitation in an ultrathin ferromagnetic metal layer. Nat. Phys. 2012, 8, 491–496. [Google Scholar] [CrossRef]
- Chen, X.; Qin, J.; Yu, T.; Han, X.-F.; Liu, Y. Micromagnetic simulation of spin torque ferromagnetic resonance in nano-ring-shape confined magnetic tunnel junctions. Appl. Phys. Lett. 2018, 113, 142406. [Google Scholar] [CrossRef]
- Qin, J.; Chen, X.; Yu, T.; Wang, X.; Guo, C.; Wan, C.; Feng, J.; Wei, H.; Liu, Y.; Han, X. Microwave Spin-Torque-Induced Magnetic Resonance in a Nanoring-Shape-Confined Magnetic Tunnel Junction. Phys. Rev. Appl. 2018, 10, 044067. [Google Scholar] [CrossRef]
- Oates, C.J.; Ogrin, F.Y.; Lee, S.L.; Riedi, P.C.; Smith, G.M.; Thomson, T. High field ferromagnetic resonance measurements of the anisotropy field of longitudinal recording thin-film media. J. Appl. Phys. 2002, 91, 1417–1422. [Google Scholar] [CrossRef]
- Stiles, M.D.; Miltat, J. Spin Transfer Torque and Dynamics. In Spin Dynamics in Confined Magnetic Structures III; Hillebrands, B., Thiaville, A., Eds.; Springer: Berlin/Heidelberg, Germany, 2006. [Google Scholar]
- Ralph, D.C.; Stiles, M.D. Spin transfer torques. J. Magn. Magn. Mater. 2008, 320, 1190–1216. [Google Scholar] [CrossRef]
- Ogrodnik, P.; Wilczyński, M.; Świrkowicz, R.; Barnaś, J. Spin transfer torque and magnetic dynamics in tunnel junctions. Phys. Rev. B 2010, 82, 134412. [Google Scholar] [CrossRef]
- Demidov, V.E.; Urazhdin, S.; Demokritov, S.O. Direct observation and mapping of spin waves emitted by spin-torque nano-oscillators. Nat. Mater. 2010, 9, 984–988. [Google Scholar] [CrossRef]
- Madami, M.; Bonetti, S.; Consolo, G.; Tacchi, S.; Carlotti, G.; Gubbiotti, G.; Mancoff, F.B.; Yar, M.A.; Akerman, J. Direct observation of a propagating spin wave induced by spin-transfer torque. Nat. Nanotech. 2011, 6, 635–638. [Google Scholar] [CrossRef]
- Bazaliy, Y.B.; Jones, B.A.; Zhang, S.-C. Modification of the Landau-Lifshitz equation in the presence of a spin-polarized current in colossal- and giant-magnetoresistive materials. Phys. Rev. B 1998, 57, R3213–R3216. [Google Scholar] [CrossRef]
- Hoefer, M.A.; Silva, T.J.; Keller, M.W. Theory for a dissipative droplet soliton excited by a spin torque nanocontact. Phys. Rev. B 2010, 82, 054432. [Google Scholar] [CrossRef]
- Mohseni, S.M.; Sani, S.R.; Persson, J.; Nguyen, T.N.A.; Chung, S.; Pogoryelov, Y.; Muduli, P.K.; Iacocca, E.; Eklund, A.; Dumas, R.K.; et al. Spin Torque–Generated Magnetic Droplet Solitons. Science 2013, 339, 1295–1298. [Google Scholar] [CrossRef] [PubMed]
- Macià, F.; Backes, D.; Kent, A.D. Stable magnetic droplet solitons in spin-transfer nanocontacts. Nat. Nanotech. 2014, 9, 992–996. [Google Scholar] [CrossRef]
- Xiao, D.; Liu, Y.; Zhou, Y.; Mohseni, S.M.; Chung, S.; Akerman, J. Merging droplets in double nanocontact spin torque oscillators. Phys. Rev. B 2016, 93, 094431. [Google Scholar] [CrossRef]
- Xiao, D.; Tiberkevich, V.; Liu, Y.H.; Liu, Y.W.; Mohseni, S.M.; Chung, S.; Ahlberg, M.; Slavin, A.N.; Akerman, J.; Zhou, Y. Parametric autoexcitation of magnetic droplet soliton perimeter modes. Phys. Rev. B 2017, 95, 024106. [Google Scholar] [CrossRef]
- Slavin, A.; Tiberkevich, V. Spin Wave Mode Excited by Spin-Polarized Current in a Magnetic Nanocontact is a Standing Self-Localized Wave Bullet. Phys. Rev. Lett. 2005, 95, 237201. [Google Scholar] [CrossRef]
- Bonetti, S.; Tiberkevich, V.; Consolo, G.; Finocchio, G.; Muduli, P.; Mancoff, F.; Slavin, A.; Akerman, J. Experimental Evidence of Self-Localized and Propagating Spin Wave Modes in Obliquely Magnetized Current-Driven Nanocontacts. Phys. Rev. Lett. 2010, 105, 217204. [Google Scholar] [CrossRef]
- Zhou, Z.W.; Wang, X.G.; Nie, Y.Z.; Xia, Q.L.; Zeng, Z.M.; Guo, G.H. Left-handed polarized spin waves in ferromagnets induced by spin-transfer torque. Phys. Rev. B 2019, 99, 014420. [Google Scholar] [CrossRef]
- Nishimura, N.; Hirai, T.; Koganei, A.; Ikeda, T.; Okano, K.; Sekiguchi, Y.; Osada, Y. Magnetic tunnel junction device with perpendicular magnetization films for high-density magnetic random access memory. J. Appl. Phys. 2002, 91, 5246–5249. [Google Scholar] [CrossRef]
- Falloon, P.E.; Jalabert, R.A.; Weinmann, D.; Stamps, R.L. Circuit model for domain walls in ferromagnetic nanowires: Application to conductance and spin transfer torques. Phys. Rev. B 2004, 70, 174424. [Google Scholar] [CrossRef]
- Xiao, J.; Bauer, G.E.W. Spin-Wave Excitation in Magnetic Insulators by Spin-Transfer Torque. Phys. Rev. Lett. 2012, 108, 217204. [Google Scholar] [CrossRef] [PubMed]
- Ulrichs, H.; Demidov, V.E.; Demokritov, S.O.; Urazhdin, S. Spin-torque nano-emitters for magnonic applications. Appl. Phys. Lett. 2012, 100, 162406. [Google Scholar] [CrossRef]
- Demidov, V.E.; Urazhdin, S.; Liu, R.; Divinskiy, B.; Telegin, A.; Demokritov, S.O. Excitation of coherent propagating spin waves by pure spin currents. Nat. Commun. 2016, 7, 10446. [Google Scholar] [CrossRef] [PubMed]
- Divinskiy, B.; Demidov, V.E.; Urazhdin, S.; Freeman, R.; Rinkevich, A.B.; Demokritov, S.O. Excitation and Amplification of Spin Waves by Spin–Orbit Torque. Adv. Mater. 2018, 30, 1802837. [Google Scholar] [CrossRef] [PubMed]
- Gurevich, A.G.; Melkov, G.A. Magnetization Oscillations and Waves; CRC Press: Boca Raton, FL, USA, 1996. [Google Scholar]
- Houshang, A.; Khymyn, R.; Fulara, H.; Gangwar, A.; Haidar, M.; Etesami, S.R.; Ferreira, R.; Freitas, P.P.; Dvornik, M.; Dumas, R.K.; et al. Spin transfer torque driven higher-order propagating spin waves in nano-contact magnetic tunnel junctions. Nat. Commun. 2018, 9, 4374. [Google Scholar] [CrossRef]
- Wang, Y.; Zhu, D.; Yang, Y.; Lee, K.; Mishra, R.; Go, G.; Oh, S.H.; Kim, D.H.; Cai, K.M.; Liu, E.L. Magnetization switching by magnon-mediated spin torque through an antiferromagnetic insulator. Science 2019, 366, 1125–1128. [Google Scholar] [CrossRef]
- Demidov, V.E.; Urazhdin, S.; Anane, A.; Cros, V.; Demokritov, S.O. Spin–orbit-torque magnonics. J. Appl. Phys. 2020, 127, 170901. [Google Scholar] [CrossRef]
- Wienholdt, S.; Hinzke, D.; Nowak, U. THz Switching of Antiferromagnets and Ferrimagnets. Phys. Rev. Lett. 2012, 108, 247207. [Google Scholar] [CrossRef]
- Wadley, P.; Howells, B.; Zelezny, J.; Andrews, C.; Hills, V.; Campion, R.P.; Novak, V.; Olejnik, K.; Maccherozzi, F.; Dhesi, S.S.; et al. Electrical switching of an antiferromagnet. Science 2016, 351, 587–590. [Google Scholar] [CrossRef]
- Khymyn, R.; Lisenkov, I.; Tiberkevich, V.; Ivanov, B.A.; Slavin, A. Antiferromagnetic THz-frequency Josephson-like Oscillator Driven by Spin Current. Sci. Rep. 2017, 7, 43705. [Google Scholar] [CrossRef]
- Li, J.; Wilson, C.B.; Cheng, R.; Lohmann, M.; Kavand, M.; Yuan, W.; Aldosary, M.; Agladze, N.; Wei, P.; Sherwin, M.S.; et al. Spin current from sub-terahertz-generated antiferromagnetic magnons. Nature 2020, 578, 70–74. [Google Scholar] [CrossRef]
- Gomonay, E.V.; Loktev, V.M. Spintronics of antiferromagnetic systems (Review Article). Low Temp. Phys. 2014, 40, 17–35. [Google Scholar] [CrossRef]
- Zhang, X.; Zhou, Y.; Ezawa, M. Antiferromagnetic Skyrmion: Stability, Creation and Manipulation. Sci. Rep. 2016, 6, 24795. [Google Scholar] [CrossRef]
- Gomonay, O.; Jungwirth, T.; Sinova, J. Concepts of antiferromagnetic spintronics. Phys. Status. Solidi-R 2017, 11, 1700022. [Google Scholar] [CrossRef]
- Šmejkal, L.; Mokrousov, Y.; Yan, B.; MacDonald, A.H. Topological antiferromagnetic spintronics. Nat. Phys. 2018, 14, 242–251. [Google Scholar] [CrossRef]
- Železný, J.; Wadley, P.; Olejník, K.; Hoffmann, A.; Ohno, H. Spin transport and spin torque in antiferromagnetic devices. Nat. Phys. 2018, 14, 220–228. [Google Scholar] [CrossRef]
- Duine, R.A.; Lee, K.-J.; Parkin, S.S.P.; Stiles, M.D. Synthetic antiferromagnetic spintronics. Nat. Phys. 2018, 14, 217–219. [Google Scholar] [CrossRef] [PubMed]
- Song, C.; You, Y.; Chen, X.; Zhou, X.; Wang, Y.; Pan, F. How to manipulate magnetic states of antiferromagnets. Nanotechnology 2018, 29, 112001. [Google Scholar] [CrossRef]
- Rezende, S.M.; Azevedo, A.; Rodríguez-Suárez, R.L. Introduction to antiferromagnetic magnons. J. Appl. Phys. 2019, 126, 151101. [Google Scholar] [CrossRef]
- Bai, H.; Zhang, Y.C.; Han, L.; Zhou, Y.J.; Pan, F.; Song, C. Antiferromagnetism: An efficient and controllable spin source. Appl. Phys. Rev. 2022, 9, 041316. [Google Scholar] [CrossRef]
- Šmejkal, L.; MacDonald, A.H.; Sinova, J.; Nakatsuji, S.; Jungwirth, T. Anomalous Hall antiferromagnets. Nat. Rev. Mater. 2022, 7, 482–496. [Google Scholar] [CrossRef]
- Keffer, F.; Kittel, C. Theory of Antiferromagnetic Resonance. Phys. Rev. 1952, 85, 329–337. [Google Scholar] [CrossRef]
- Cheng, R.; Xiao, J.; Niu, Q.; Brataas, A. Spin Pumping and Spin-Transfer Torques in Antiferromagnets. Phys. Rev. Lett. 2014, 113, 057601. [Google Scholar] [CrossRef] [PubMed]
- Cheng, R.; Niu, Q. Dynamics of antiferromagnets driven by spin current. Phys. Rev. B 2014, 89, 081105. [Google Scholar] [CrossRef]
- Cheng, R.; Daniels, M.W.; Zhu, J.G.; Xiao, D. Antiferromagnetic Spin Wave Field-Effect Transistor. Sci. Rep. 2016, 6, 24233. [Google Scholar] [CrossRef] [PubMed]
- Cheng, R.; Xiao, D.; Zhu, J.-G. Antiferromagnet-based magnonic spin-transfer torque. Phys. Rev. B 2018, 98, 020408. [Google Scholar] [CrossRef]
- Zheng, C.; Chen, X.; Zhou, S.; Liu, Y. Terahertz magnetic excitation in antiferromagnets: Atomistic spin simulations versus a coupled pendulum model. J. Phys. Condens. Matt. 2022, 35, 085801. [Google Scholar] [CrossRef] [PubMed]
- Satoh, T.; Cho, S.J.; Iida, R.; Shimura, T.; Kuroda, K.; Ueda, H.; Ueda, Y.; Ivanov, B.A.; Nori, F.; Fiebig, M. Spin Oscillations in Antiferromagnetic NiO Triggered by Circularly Polarized Light. Phys. Rev. Lett. 2010, 105, 077402. [Google Scholar] [CrossRef]
- Parthasarathy, A.; Cogulu, E.; Kent, A.D.; Rakheja, S. Precessional spin-torque dynamics in biaxial antiferromagnets. Phys. Rev. B 2021, 103, 024450. [Google Scholar] [CrossRef]
- Yosida, K. Magnetic Properties of Cu-Mn Alloys. Phys. Rev. 1957, 106, 893–898. [Google Scholar] [CrossRef]
- Grunberg, P.; Schreiber, R.; Pang, Y.; Brodsky, M.B.; Sowers, H. Layered magnetic structures: Evidence for antiferromagnetic coupling of Fe layers across Cr interlayers. Phys. Rev. Lett. 1986, 57, 2442–2445. [Google Scholar] [CrossRef] [PubMed]
- Binasch, G.; Grünberg, P.; Saurenbach, F.; Zinn, W. Enhanced magnetoresistance in layered magnetic structures with antiferromagnetic interlayer exchange. Phys. Rev. B 1989, 39, 4828–4830. [Google Scholar] [CrossRef] [PubMed]
- Parkin, S.S.P.; More, N.; Roche, K.P. Oscillations in exchange coupling and magnetoresistance in metallic superlattice structures: Co/Ru, Co/Cr, and Fe/Cr. Phys. Rev. Lett. 1990, 64, 2304–2307. [Google Scholar] [CrossRef] [PubMed]
- Lau, Y.C.; Betto, D.; Rode, K.; Coey, J.M.D.; Stamenov, P. Spin-orbit torque switching without an external field using interlayer exchange coupling. Nat. Nanotech. 2016, 11, 758–762. [Google Scholar] [CrossRef] [PubMed]
- Bruno, P.; Chappert, C. Oscillatory coupling between ferromagnetic layers separated by a nonmagnetic metal spacer. Phys. Rev. Lett. 1991, 67, 1602–1605. [Google Scholar] [CrossRef] [PubMed]
- Parkin, S.S.P.; Mauri, D. Spin engineering: Direct determination of the Ruderman-Kittel-Kasuya-Yosida far-field range function in ruthenium. Phys. Rev. B 1991, 44, 7131–7134. [Google Scholar] [CrossRef] [PubMed]
- Yang, S.-H.; Ryu, K.-S.; Parkin, S. Domain-wall velocities of up to 750 m s−1 driven by exchange-coupling torque in synthetic antiferromagnets. Nat. Nanotech. 2015, 10, 221. [Google Scholar] [CrossRef] [PubMed]
- Devolder, T. Ferromagnetic resonance of exchange-coupled perpendicularly magnetized bilayers. J. Appl. Phys. 2016, 119, 153905. [Google Scholar] [CrossRef]
- Li, S.; Wang, C.; Chu, X.M.; Miao, G.X.; Xue, Q.; Zou, W.; Liu, M.; Xu, J.; Li, Q.; Dai, Y.; et al. Engineering optical mode ferromagnetic resonance in FeCoB films with ultrathin Ru insertion. Sci. Rep. 2016, 6, 33349. [Google Scholar] [CrossRef]
- Wu, G.; Chen, S.; Lou, S.; Liu, Y.; Jin, Q.Y.; Zhang, Z. Annealing effect on laser-induced magnetization dynamics in Co/Ni-based synthetic antiferromagnets with perpendicular magnetic anisotropy. Appl. Phys. Lett. 2019, 115, 142402. [Google Scholar] [CrossRef]
- Waring, H.J.; Johansson, N.A.B.; Vera-Marun, I.J.; Thomson, T. Zero-field Optic Mode Beyond 20 GHz in a Synthetic Antiferromagnet. Phys. Rev. Appl. 2020, 13, 034035. [Google Scholar] [CrossRef]
- Gusakova, D.; Houssameddine, D.; Ebels, U.; Dieny, B.; Buda-Prejbeanu, L.; Cyrille, M.C.; Delaet, B. Spin-polarized current-induced excitations in a coupled magnetic layer system. Phys. Rev. B 2009, 79, 104406. [Google Scholar] [CrossRef]
- Houssameddine, D.; Sierra, J.F.; Gusakova, D.; Delaet, B.; Ebels, U.; Buda-Prejbeanu, L.D.; Cyrille, M.C.; Dieny, B.; Ocker, B.; Langer, J.; et al. Spin torque driven excitations in a synthetic antiferromagnet. Appl. Phys. Lett. 2010, 96, 3149. [Google Scholar] [CrossRef]
- Baláž, P.; Barnaś, J. Current-induced dynamics of composite free layer with antiferromagnetic interlayer exchange coupling. Phys. Rev. B 2011, 83, 104422. [Google Scholar] [CrossRef]
- Zhang, Z.; Zhou, L.; Wigen, P.E.; Ounadjela, K. Angular dependence of ferromagnetic resonance in exchange-coupled Co/Ru/Co trilayer structures. Phys. Rev. B 1994, 50, 6094–6112. [Google Scholar] [CrossRef] [PubMed]
- Rezende, S.M.; Chesman, C.; Lucena, M.A.; Azevedo, A.; de Aguiar, F.M.; Parkin, S.S.P. Studies of coupled metallic magnetic thin-film trilayers. J. Appl. Phys. 1998, 84, 958–972. [Google Scholar] [CrossRef]
- Zivieri, R.; Giovannini, L.; Nizzoli, F. Acoustical and optical spin modes of multilayers with ferromagnetic and antiferromagnetic coupling. Phys. Rev. B 2000, 62, 14950–14955. [Google Scholar] [CrossRef]
- Belmeguenai, M.; Martin, T.; Woltersdorf, G.; Maier, M.; Bayreuther, G. Frequency- and time-domain investigation of the dynamic properties of interlayer-exchange-coupled Ni81Fe19/Ru/Ni81Fe19 thin films. Phys. Rev. B 2007, 76, 104414. [Google Scholar] [CrossRef]
- Shiota, Y.; Taniguchi, T.; Ishibashi, M.; Moriyama, T.; Ono, T. Tunable Magnon-Magnon Coupling Mediated by Dynamic Dipolar Interaction in Synthetic Antiferromagnets. Phys. Rev. Lett. 2020, 125, 017203. [Google Scholar] [CrossRef]
- Sud, A.; Zollitsch, C.W.; Kamimaki, A.; Dion, T.; Khan, S.; Iihama, S.; Mizukami, S.; Kurebayashi, H. Tunable magnon-magnon coupling in synthetic antiferromagnets. Phys. Rev. B 2020, 102, 100403. [Google Scholar] [CrossRef]
- Kamimaki, A.; Iihama, S.; Suzuki, K.Z.; Yoshinaga, N.; Mizukami, S. Parametric Amplification of Magnons in Synthetic Antiferromagnets. Phys. Rev. Appl. 2020, 13, 044036. [Google Scholar] [CrossRef]
- Li, Y.; Zhao, C.; Amin, V.P.; Zhang, Z.; Vogel, M.; Xiong, Y.; Sklenar, J.; Divan, R.; Pearson, J.; Stiles, M.D.; et al. Phase-resolved electrical detection of coherently coupled magnonic devices. Appl. Phys. Lett. 2021, 118, 202401. [Google Scholar] [CrossRef] [PubMed]
- Wigen, P.E.; Zhang, Z.; Zhou, L.; Ye, M.; Cowen, J.A. The dispersion relation in antiparallel coupled ferromagnetic films. J. Appl. Phys. 1993, 73, 6338–6340. [Google Scholar] [CrossRef]
- MacNeill, D.; Hou, J.T.; Klein, D.R.; Zhang, P.; Jarillo-Herrero, P.; Liu, L. Gigahertz Frequency Antiferromagnetic Resonance and Strong Magnon-Magnon Coupling in the Layered Crystal CrCl3. Phys. Rev. Lett. 2019, 123, 047204. [Google Scholar] [CrossRef] [PubMed]
- Zhu, J.-G. Spin valve and dual spin valve heads with synthetic antiferromagnets. IEEE Trans. Magn. 1999, 35, 655–660. [Google Scholar]
- Chen, X.; Zheng, C.; Zhou, S.; Liu, Y.; Zhang, Z. Manipulation of Time- and Frequency-Domain Dynamics by Magnon-Magnon Coupling in Synthetic Antiferromagnets. Magnetochemistry 2021, 8, 7. [Google Scholar] [CrossRef]
- Yakata, S.; Kubota, H.; Suzuki, Y.; Yakushiji, K.; Fukushima, A.; Yuasa, S.; Ando, K. Influence of perpendicular magnetic anisotropy on spin-transfer switching current in CoFeB/MgO/CoFeB magnetic tunnel junctions. J. Appl. Phys. 2009, 105, 103. [Google Scholar] [CrossRef]
- Schellekens, A.J.; Kuiper, K.C.; De Wit, R.R.J.C.; Koopmans, B. Ultrafast spin-transfer torque driven by femtosecond pulsed-laser excitation. Nat. Commun. 2014, 5, 4333. [Google Scholar] [CrossRef]
- Wu, D.; Zhang, Z.; Li, L.; Zhang, Z.; Zhao, H.B.; Wang, J.; Ma, B.; Jin, Q.Y. Perpendicular magnetic anisotropy and magnetization dynamics in oxidized CoFeAl films. Sci. Rep. 2015, 5, 12352. [Google Scholar] [CrossRef]
- Vemulkar, T.; Mansell, R.; Fernández-Pacheco, A.; Cowburn, R.P. Toward Flexible Spintronics: Perpendicularly Magnetized Synthetic Antiferromagnetic Thin Films and Nanowires on Polyimide Substrates. Adv. Funct. Mater. 2016, 26, 4704–4711. [Google Scholar] [CrossRef]
- Dieny, B.; Chshiev, M. Perpendicular magnetic anisotropy at transition metal/oxide interfaces and applications. Rev. Mod. Phys. 2017, 89, 025008. [Google Scholar] [CrossRef]
- Yi, L.; Yu, J.; Zhong, H.J.J.O.M.; Materials, M. Strong antiferromagnetic interlayer exchange coupling in [Co/Pt]6/Ru/[Co/Pt]4 structures with perpendicular magnetic anisotropy. J. Magn. Magn. Mater. 2018, 473, 381–386. [Google Scholar]
- Shiota, Y.; Arakawa, T.; Hisatomi, R.; Moriyama, T.; Ono, T. Polarization-Selective Excitation of Antiferromagnetic Resonance in Perpendicularly Magnetized Synthetic Antiferromagnets. Phys. Rev. Appl. 2022, 18, 014032. [Google Scholar] [CrossRef]
- Tabuchi, Y.; Ishino, S.; Ishikawa, T.; Yamazaki, R.; Usami, K.; Nakamura, Y. Hybridizing ferromagnetic magnons and microwave photons in the quantum limit. Phys. Rev. Lett. 2014, 113, 083603. [Google Scholar] [CrossRef]
- Lachance-Quirion, D.; Tabuchi, Y.; Gloppe, A.; Usami, K.; Nakamura, Y. Hybrid quantum systems based on magnonics. Appl. Phys. Express 2019, 12, 070101. [Google Scholar] [CrossRef]
- Li, Y.; Zhang, W.; Tyberkevych, V.; Kwok, W.-K.; Hoffmann, A.; Novosad, V. Hybrid magnonics: Physics, circuits, and applications for coherent information processing. J. Appl. Phys. 2020, 128, 130902. [Google Scholar] [CrossRef]
- Hu, B.; Xie, Z.-K.; Lu, J.; He, W. Mapping the magnon–magnon hybrid state onto the Bloch sphere. Appl. Phys. Lett. 2024, 124, 232402. [Google Scholar] [CrossRef]
- Kruglyak, V.V.; Demokritov, S.O.; Grundler, D. Magnonics. J. Phys. D Appl. Phys. 2010, 43, 264001. [Google Scholar] [CrossRef]
- Zhang, X.; Zou, C.L.; Jiang, L.; Tang, H.X. Strongly Coupled Magnons and Cavity Microwave Photons. Phys. Rev. Lett. 2014, 113, 156401. [Google Scholar] [CrossRef]
- Wang, Y.; Zhang, Y.; Li, C.; Wei, J.; He, B.; Xu, H.; Xia, J.; Luo, X.; Li, J.; Dong, J.; et al. Ultrastrong to nearly deep-strong magnon-magnon coupling with a high degree of freedom in synthetic antiferromagnets. Nat. Commun. 2024, 15, 2077. [Google Scholar] [CrossRef] [PubMed]
- Chumak, A.V.; Serga, A.A.; Hillebrands, B. Magnon transistor for all-magnon data processing. Nat. Commun. 2014, 5, 4700. [Google Scholar] [CrossRef] [PubMed]
- Chen, J.; Liu, C.; Liu, T.; Xiao, Y.; Xia, K.; Bauer, G.E.W.; Wu, M.; Yu, H. Strong Interlayer Magnon-Magnon Coupling in Magnetic Metal-Insulator Hybrid Nanostructures. Phys. Rev. Lett. 2018, 120, 217202. [Google Scholar] [CrossRef] [PubMed]
- Dieny, B.; Prejbeanu, I.L.; Garello, K.; Gambardella, P.; Freitas, P.; Lehndorff, R.; Raberg, W.; Ebels, U.; Demokritov, S.O.; Akerman, J.; et al. Opportunities and challenges for spintronics in the microelectronics industry. Nat. Electron. 2020, 3, 446–459. [Google Scholar] [CrossRef]
- Mahmoud, A.; Ciubotaru, F.; Vanderveken, F.; Chumak, A.V.; Hamdioui, S.; Adelmann, C.; Cotofana, S. Introduction to spin wave computing. J. Appl. Phys. 2020, 128, 161101. [Google Scholar] [CrossRef]
- Xiao, X.; Chen, Z.; Dai, C.; Ma, F. Magnon mode transition in synthetic antiferromagnets induced by perpendicular magnetic anisotropy. J. Appl. Phys. 2022, 131, 093905. [Google Scholar] [CrossRef]
- Li, Z.; Sun, J.; Ma, F. Floquet engineering of selective magnon–magnon coupling in synthetic antiferromagnets. Appl. Phys. Lett. 2023, 123, 232406. [Google Scholar] [CrossRef]
- Comstock, A.H.; Chou, C.T.; Wang, Z.; Wang, T.; Song, R.; Sklenar, J.; Amassian, A.; Zhang, W.; Lu, H.; Liu, L.; et al. Hybrid magnonics in hybrid perovskite antiferromagnets. Nat. Commun. 2023, 14, 1834. [Google Scholar] [CrossRef]
- Hayashi, D.; Shiota, Y.; Ishibashi, M.; Hisatomi, R.; Moriyama, T.; Ono, T. Observation of mode splitting by magnon–magnon coupling in synthetic antiferromagnets. Appl. Phys. Express 2023, 16, 053004. [Google Scholar] [CrossRef]
- Rong, Y.; Jiang, C.; Wang, H.; Sun, L.; Liu, F.; Lu, J.; Wu, T.; Zhang, Y.; Zhao, Y.; Ma, F.; et al. Layer-dependent magnon-magnon coupling in a synthetic antiferromagnet. Phys. Rev. Appl. 2024, 21, 054050. [Google Scholar] [CrossRef]
- Liensberger, L.; Kamra, A.; Maier-Flaig, H.; Geprags, S.; Erb, A.; Goennenwein, S.T.B.; Gross, R.; Belzig, W.; Huebl, H.; Weiler, M. Exchange-Enhanced Ultrastrong Magnon-Magnon Coupling in a Compensated Ferrimagnet. Phys. Rev. Lett. 2019, 123, 117204. [Google Scholar] [CrossRef] [PubMed]
- Dai, C.; Ma, F. Strong magnon–magnon coupling in synthetic antiferromagnets. Appl. Phys. Lett. 2021, 118, 112405. [Google Scholar] [CrossRef]
- Li, M.; Lu, J.; He, W. Symmetry breaking induced magnon-magnon coupling in synthetic antiferromagnets. Phys. Rev. B 2021, 103, 064429. [Google Scholar] [CrossRef]
- He, W.; Xie, Z.K.; Sun, R.; Yang, M.; Li, Y.; Zhao, X.T.; Liu, W.; Zhang, Z.D.; Cai, J.W.; Cheng, Z.H.; et al. Anisotropic Magnon–Magnon Coupling in Synthetic Antiferromagnets. Chin. Phys. Lett. 2021, 38, 057502. [Google Scholar] [CrossRef]
- Hu, B.; He, W. Tunable magnon-magnon coupling mediated by in-plane magnetic anisotropy in synthetic antiferromagnets. J. Magn. Magn. Mater. 2023, 565, 170283. [Google Scholar] [CrossRef]
- Wang, Y.; Xia, J.; Wan, C.; Han, X.; Yu, G. Ultrastrong magnon-magnon coupling in synthetic antiferromagnets induced by interlayer Dzyaloshinskii-Moriya interaction. Phys. Rev. B 2024, 109, 054416. [Google Scholar] [CrossRef]
- Sklenar, J.; Zhang, W. Self-Hybridization and Tunable Magnon-Magnon Coupling in van der Waals Synthetic Magnets. Phys. Rev. Appl. 2021, 15, 044008. [Google Scholar] [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, X.; Zheng, C.; Liu, Y. Magnon Excitation Modes in Ferromagnetic and Antiferromagnetic Systems. Magnetochemistry 2024, 10, 50. https://doi.org/10.3390/magnetochemistry10070050
Chen X, Zheng C, Liu Y. Magnon Excitation Modes in Ferromagnetic and Antiferromagnetic Systems. Magnetochemistry. 2024; 10(7):50. https://doi.org/10.3390/magnetochemistry10070050
Chicago/Turabian StyleChen, Xing, Cuixiu Zheng, and Yaowen Liu. 2024. "Magnon Excitation Modes in Ferromagnetic and Antiferromagnetic Systems" Magnetochemistry 10, no. 7: 50. https://doi.org/10.3390/magnetochemistry10070050
APA StyleChen, X., Zheng, C., & Liu, Y. (2024). Magnon Excitation Modes in Ferromagnetic and Antiferromagnetic Systems. Magnetochemistry, 10(7), 50. https://doi.org/10.3390/magnetochemistry10070050





