Theoretical Analysis and Experimental Research on the Apple Auto-Orientation Based on Flexible Roller
Abstract
:1. Introduction
2. Materials and Methods
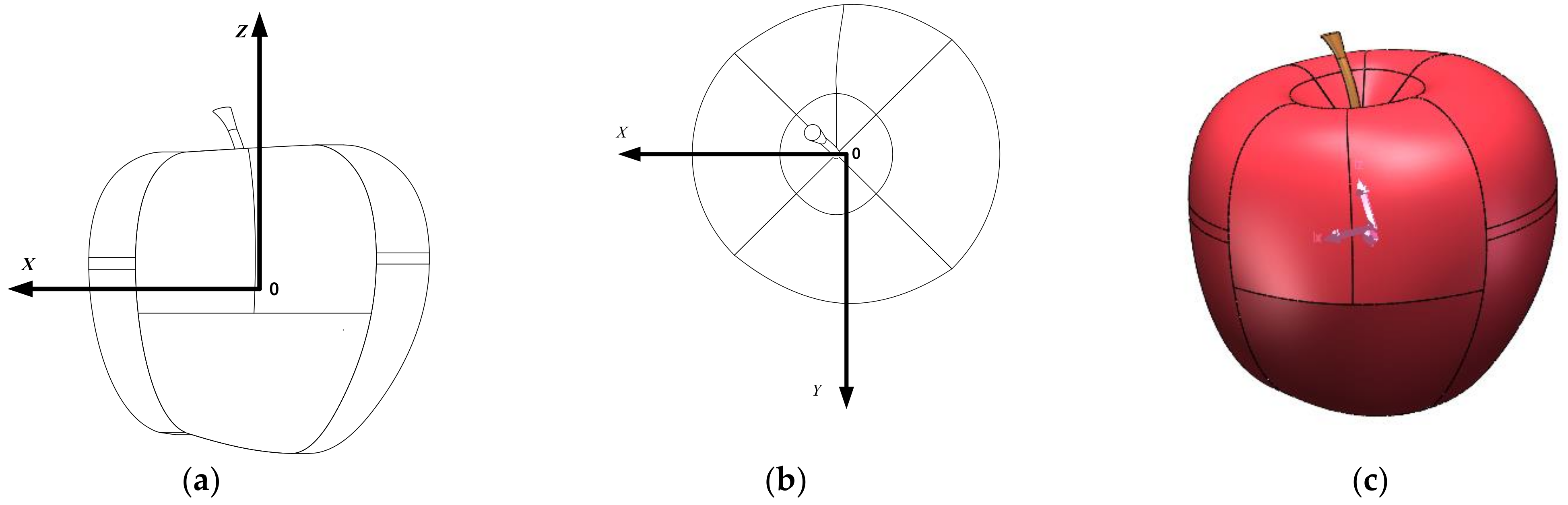
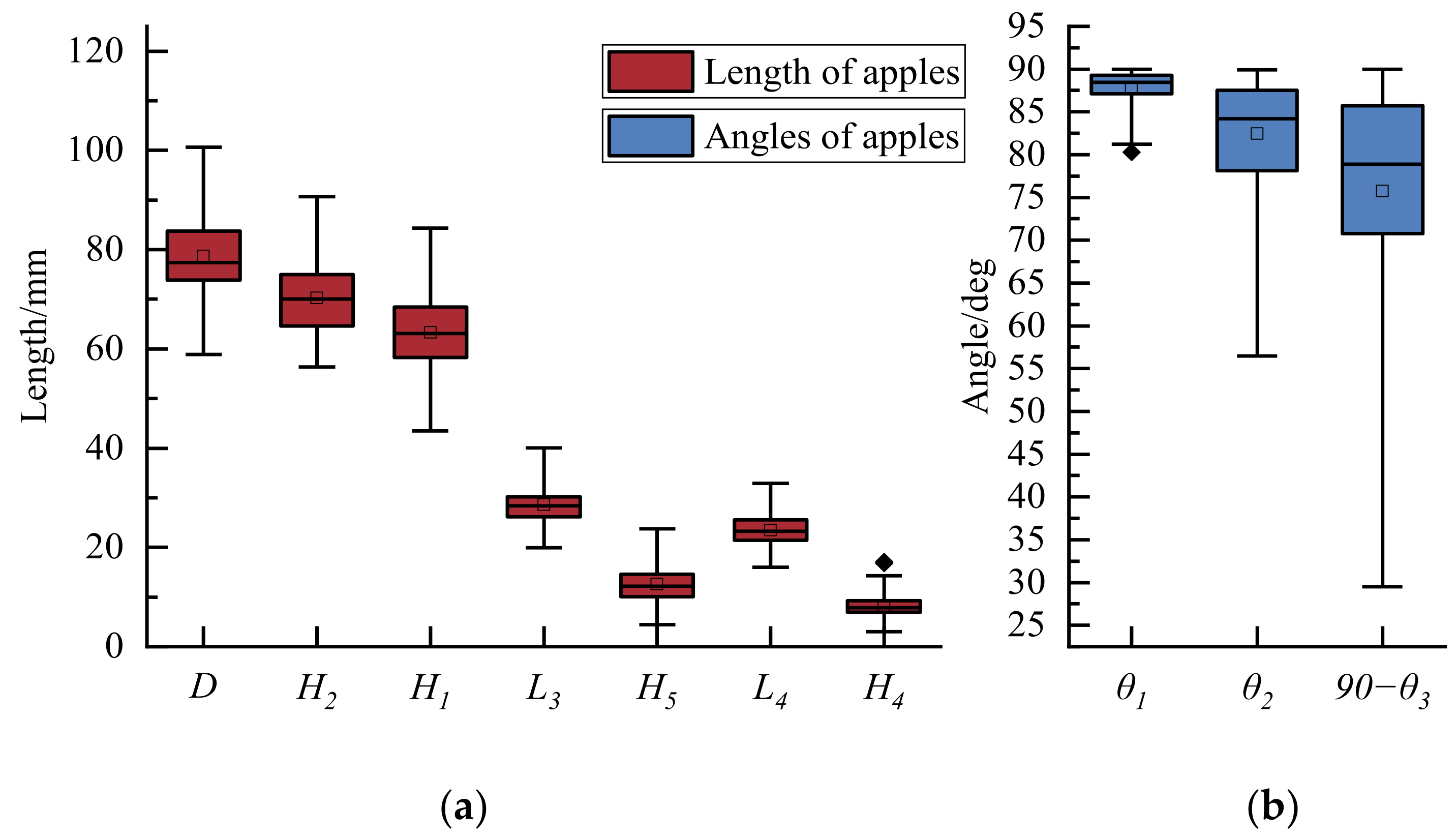
2.1. Apple Shape Statistics and Modeling
2.2. Analysis of the Apple Rotational Orientation Theory
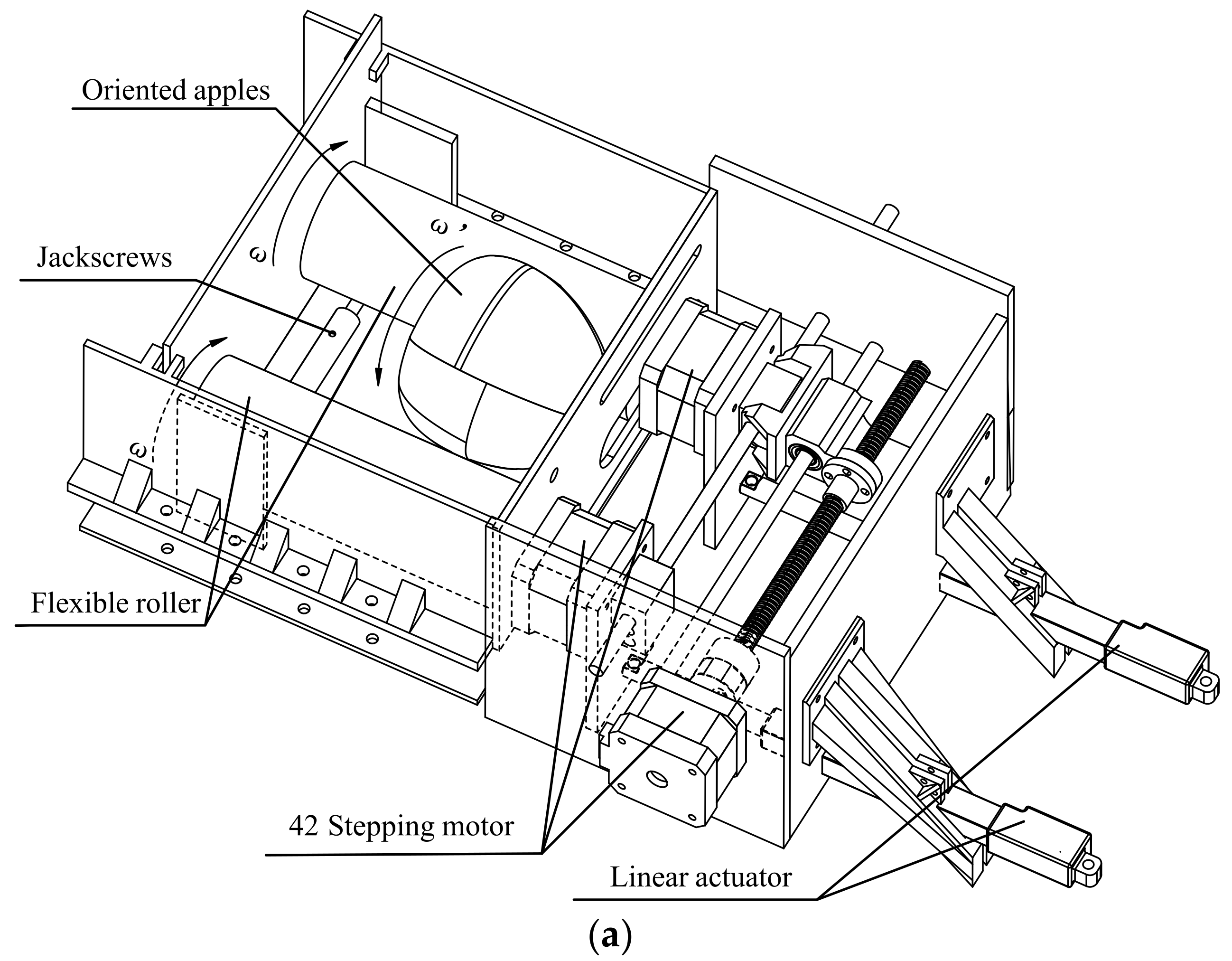
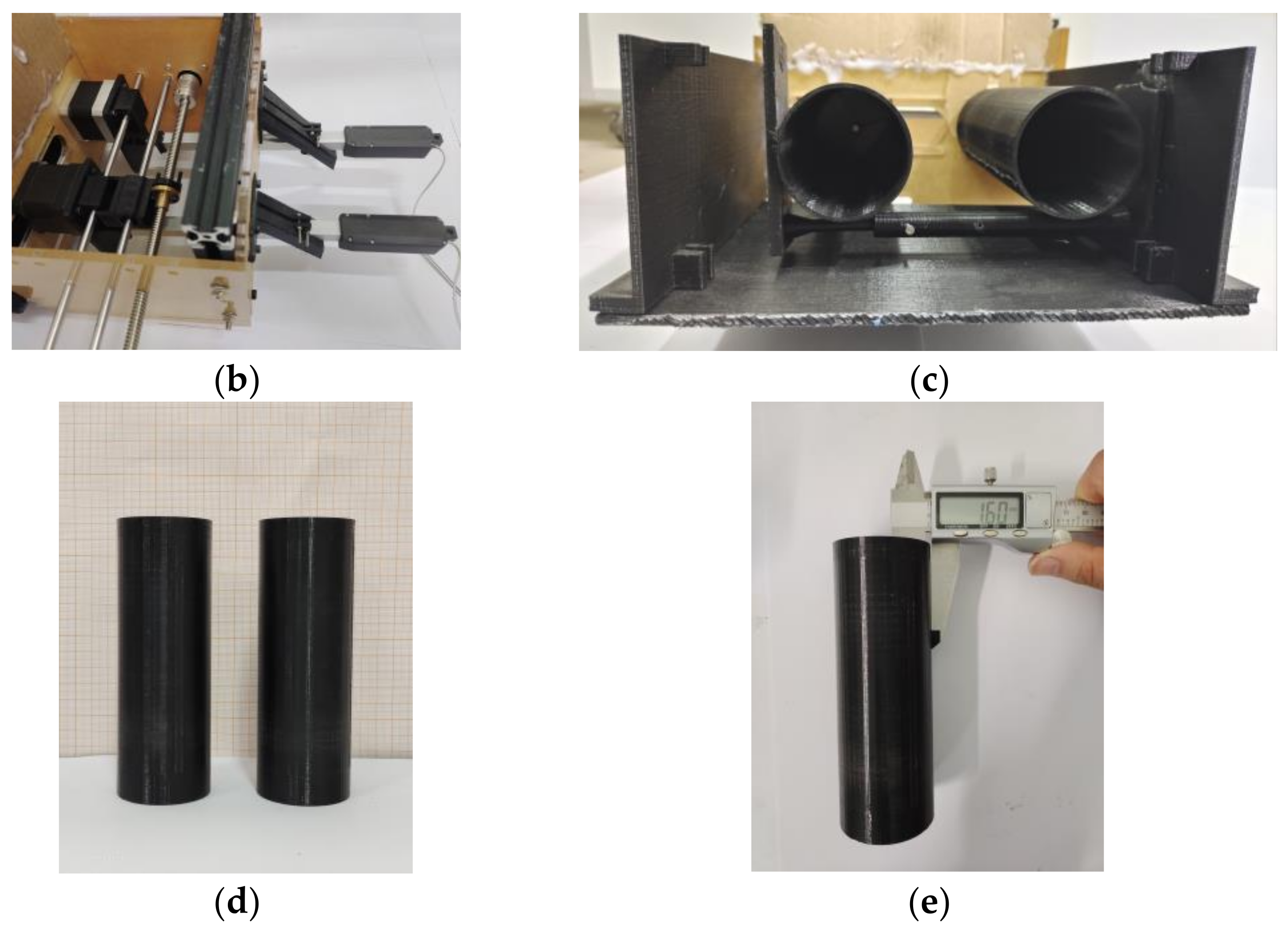
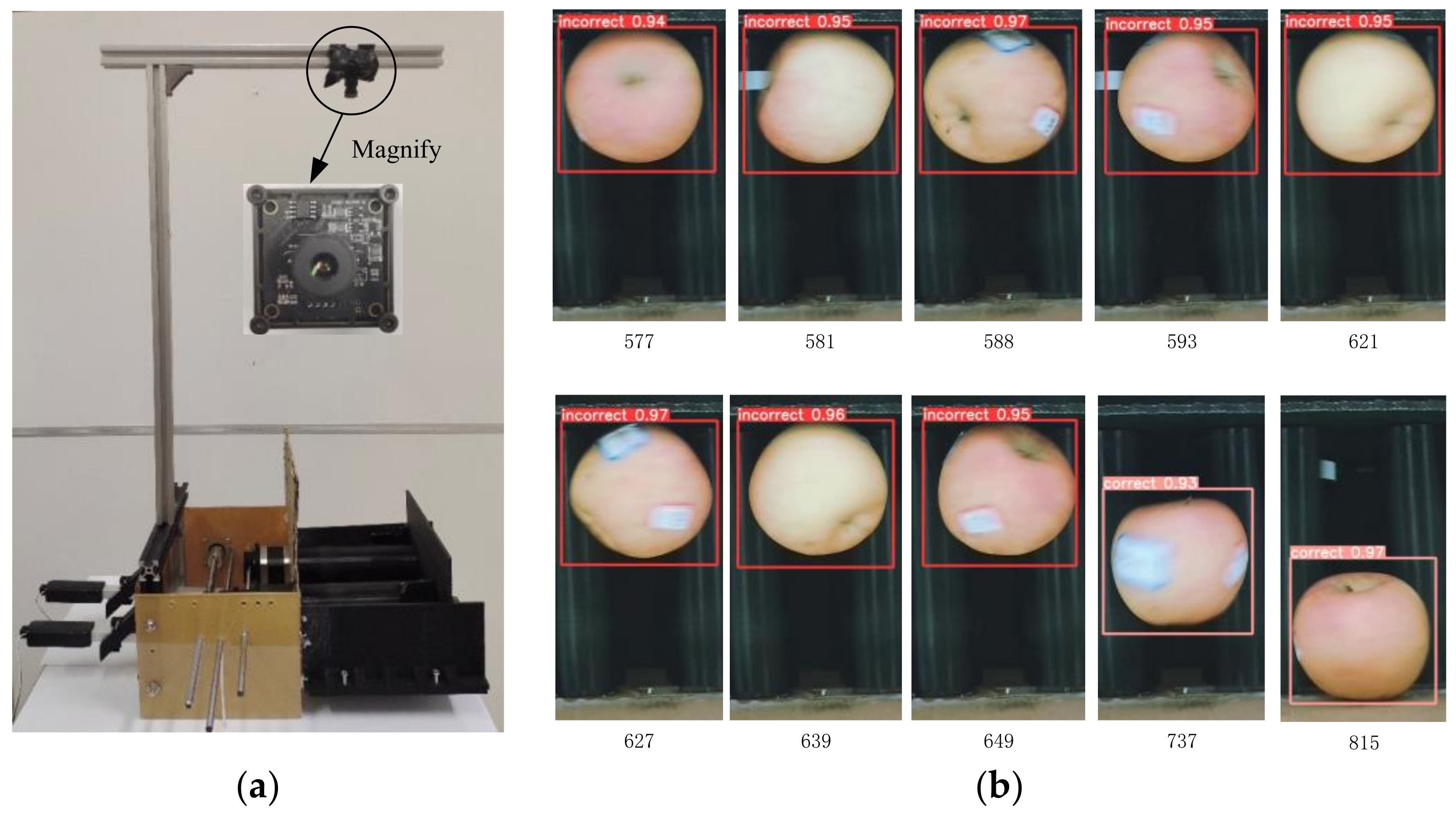
2.3. Construction of the Orientation Test Platform
2.3.1. Double Roller Installation and Restraint
2.3.2. Camera and Posture Determination Equipment
2.4. Apple Orientation Experiment
3. Results and Discussion
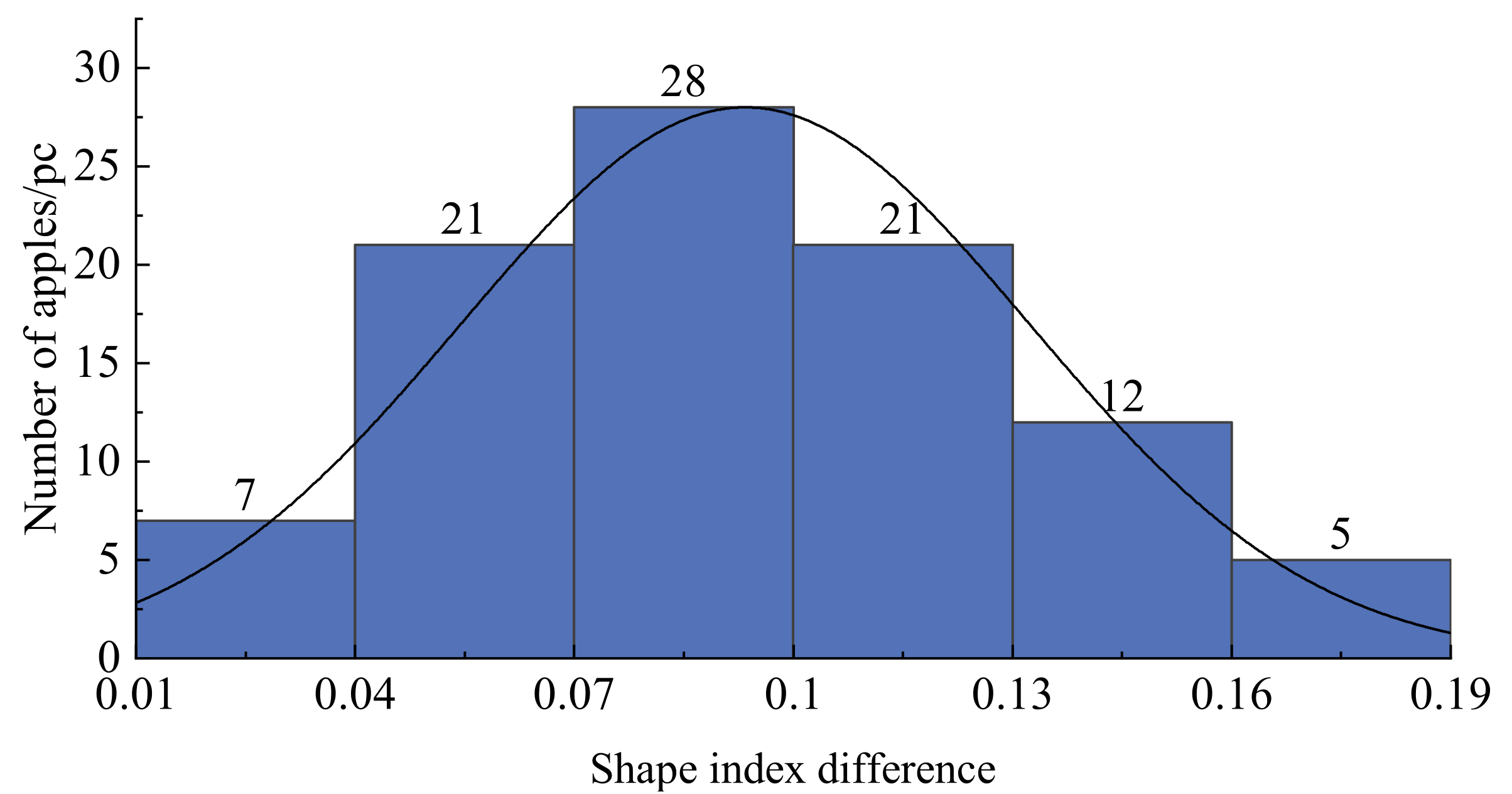
3.1. Apple Shape Characterization Results
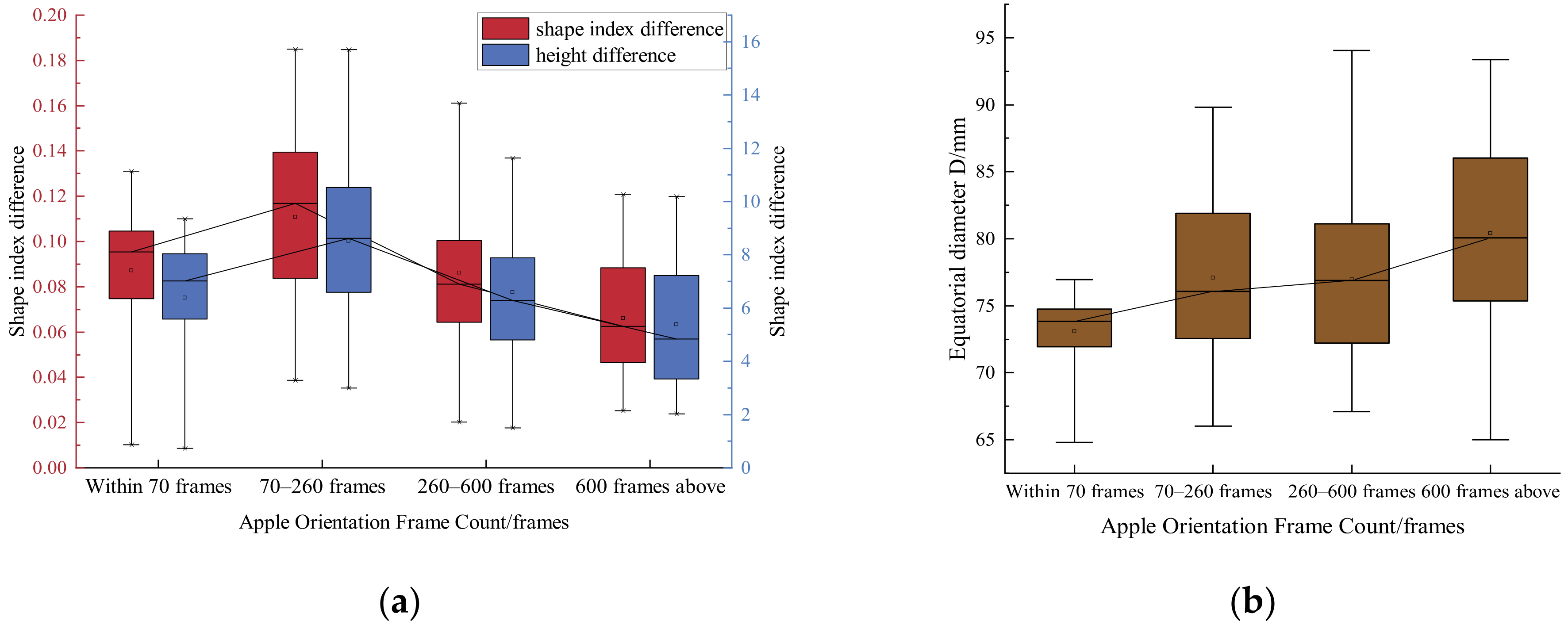
3.2. Association Results between Apple Orientation Time and Apple Shape
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Ren, C.; Zhou, X.; Wang, C.; Guo, Y.; Diao, Y.; Shen, S.; Reis, S.; Li, W.; Xu, J.; Gu, B. Ageing threatens sustainability of smallholder farming in China. Nature 2023, 616, 96–103. [Google Scholar] [CrossRef] [PubMed]
- Yang, P.P.; Liang, H.L.; Wang, T.; Fan Wu, X.; Wu, H.Q. An apple picking tool that can cut short fruit stalks. Chinese patent CN110383988B, 14 May 2021. [Google Scholar]
- GB/T 10651-2008 Fresh Apples; General Administration of Quality Supervision, Inspection and Quarantine of the People’s Republic of China. China Standards Press: Beijing, China, 2008.
- NY/T 1086-2006; Technical Specification for Apple Harvestings. Sun, X. (Ed.) Ministry of Agriculture of the People’s Republic of China, China Agricultural Press: Beijing, China, 2006.
- United States Department of Agriculture. Apples Shipping Point and Market Inspection Instructions. Available online: https://www.ams.usda.gov/grades-standards/apple-grades-standards (accessed on 23 May 2023).
- Hanrahan, I. WA 38 Stem Punctures & Semi-Commercial Packout in the 2017–2018 Storage Season. 2018. Available online: https://treefruit.wsu.edu/wa-38-stem-punctures-packout/ (accessed on 15 April 2023).
- Wang, Z.; Jin, L.; Wang, S.; Xu, H. Apple stem/calyx real-time recognition using YOLO-v5 algorithm for fruit automatic loading system. Postharvest Biol. Technol. 2022, 185, 111808. [Google Scholar] [CrossRef]
- Throop, J.A.; Aneshansley, D.J.; Anger, W.C.; Peterson, D.L. Quality evaluation of apples based on surface defects: Development of an automated inspection system. Postharvest Biol. Technol. 2005, 36, 281–290. [Google Scholar] [CrossRef]
- Narayanan, P.; Lefcourt, A.A.; Tasch, U.; Rostamian, R.; Kim, M.S. Orientation of apples using their inertial properties. Trans. ASABE 2008, 51, 2073–2081. [Google Scholar] [CrossRef]
- Lefcourt, A.M.; Narayanan, P.; Tasch, U.; Kim, M.S.; Reese, D.; Rostamian, R.; Lo, Y.M. Orienting apples for imaging using their inertial properties and random apple loading. Biosyst. Eng. 2009, 104, 64–71. [Google Scholar] [CrossRef]
- Flemmer, C.; Bakker, H.; Flemmer, R. Analysis of the stochastic excursions of tumbling apples. Computers and Electronics in Agriculture 2021, 188, 106362. [Google Scholar] [CrossRef]
- Luo, T.; Zhao, J.; Gu, Y.; Zhang, S.; Qiao, X.; Tian, W.; Han, Y. Classification of Weed Seeds Based on Visual Images and Deep Learning. Inf. Process. Agric. 2021, 10, 40–51. [Google Scholar] [CrossRef]
- Kang, N.B.; Qiang, F.; Wu, L.G.; Wang, S.L.; He, J.G. Design and experiment of synchronization actuator system based on electromagnetism for fresh jujube classifier. Trans. Chin. Soc. Agric. Eng. (Trans. CSAE) 2017, 33, 254–260. [Google Scholar] [CrossRef]
- Pothula, A.K.; Zhang, Z.; Lu, R. Design features and bruise evaluation of an apple harvest and in-field presorting machine. Trans. ASABE 2018, 61, 1135–1144. [Google Scholar] [CrossRef]
- Lu, Y.Z.; Lu, R.F.; Zhang, Z. Development and preliminary evaluation of a new apple harvest assist and in-field sorting machine. Appl. Eng. Agric. 2022, 38, 23–35. [Google Scholar] [CrossRef]
- Zhang, Z.; Lu, Y.; Lu, R. Development and evaluation of an apple infield grading and sorting system. Postharvest Biol. Technol. 2021, 180, 111588. [Google Scholar] [CrossRef]
- Zhang, Z.; Pothula, A.K.; Lu, R. Economic analysis of a self-propelled apple harvest and infield sorting machine for the apple industry. In Proceedings of the 2016 ASABE Annual International Meeting, Orlando, FL, USA, 17–20 July 2016. [Google Scholar] [CrossRef]
- Bhargava, A.; Bansal, A. Classification and Grading of Multiple Varieties of Apple Fruit. Food Anal. Methods 2021, 14, 1359–1368. [Google Scholar] [CrossRef]
- Wei, X.; Song, W.; Fan, Y.; Sun, Y.; Li, Z.; Chen, S.; Shi, J.; Zhang, D.; Zou, X.; Xu, X. A SERS aptasensor based on a flexible substrate for interference-free detection of carbendazim in apple. Food Chem. 2024, 431, 137120. [Google Scholar] [CrossRef] [PubMed]
- Gonzalez-Garcia, S.; Argiz, L.; Miguez, P.; Gullon, B. Exploring the production of bio-succinic acid from apple pomace using an environmental approach. Chem. Eng. J. 2018, 350, 982–991. [Google Scholar] [CrossRef]
- Zhang, Z.; Pothula, A.; Lu, R. Economic Evaluation of Apple Harvest and In-Field Sorting Technology. Trans. ASABE (Am. Soc. Agric. Biol. Eng.) 2017, 60, 1537–1550. [Google Scholar] [CrossRef]
- Tian, Y.N.; Yang, G.D.; Wang, Z.; Wang, H.; Li, E.; Liang, Z.Z. Apple detection during different growth stages in orchards using the improved YOLO-V3 model. Comput. Electron. Agric. 2019, 157, 417–426. [Google Scholar] [CrossRef]
- Ayaz, H.; Rodriguez-Esparza, E.; Ahmad, M.; Oliva, D.; Perez-Cisneros, M.; Sarkar, R. Classification of Apple Disease Based on Non-Linear Deep Features. Appl. Sci. 2021, 11, 6422. [Google Scholar] [CrossRef]
- Mizushima, A.; Lu, R. Development of a cost-effective machine vision system for infield sorting and grading of apples: Fruit orientation and size estimation. In Proceedings of the American Society of Agricultural and Biological Engineers Annual International Meeting 2011, Louisville, KY, USA, 7–10 August 2011; pp. 3193–3211. [Google Scholar]
- Sun, F.G.; Wang, Y.L.; Lan, P.; Zhang, X.D.; Chen, X.D.; Wang, Z.J. Identification of apple fruit diseases using improved YOLOv5s and transfer learning. Trans. Chin. Soc. Agric. Eng. (Trans. CSAE) 2022, 38, 171–179. [Google Scholar] [CrossRef]
- Thapa, R.; Zhang, K.; Snavely, N.; Belongie, S.; Khan, A. The Plant Pathology Challenge 2020 data set to classify foliar disease of apples. Appl. Plant Sci. 2020, 8, e11390. [Google Scholar] [CrossRef]
- Sabzi, S.; Nadimi, M.; Abbaspour-Gilandeh, Y.; Paliwal, J. Non-Destructive Estimation of Physicochemical Properties and Detection of Ripeness Level of Apples Using Machine Vision. Int. J. Fruit Sci. 2022, 22, 628–645. [Google Scholar] [CrossRef]
- Yuan, Y.; Yang, Z.; Liu, H.; Wang, H.; Li, J.; Zhao, L. Detection of early bruise in apple using near-infrared camera imaging technology combined with deep learning. Infrared Phys. Technol. 2022, 127, 104442. [Google Scholar] [CrossRef]
- Mamat, N.; Othman, M.F.; Abdulghafor, R.; Alwan, A.A.; Gulzar, Y. Enhancing Image Annotation Technique of Fruit Classification Using a Deep Learning Approach. Sustainability 2023, 15, 901. [Google Scholar] [CrossRef]
- Reese, D.; Lefcourt, A.M.; Kim, M.S.; Lo, Y.M. Using parabolic mirrors for complete imaging of apple surfaces. Bioresour. Technol. 2009, 100, 4499–4506. [Google Scholar] [CrossRef] [PubMed]
- Keshavarzpour, F.; Rashidi, M. Classification of Apple Size and Shape Based on Mass and Outer Dimensions. Am. Eurasian J. Agric. Environ. Sci. 2022, 9, 618–621. [Google Scholar]
- Liu, X.P.; Cao, Z.T.; Yang, L.; Chen, H.; Zhang, Y.L. Research on Damage Properties of Apples Based on Static Compression Combined with the Finite Element Method. Foods 2022, 11, 1851. [Google Scholar] [CrossRef]
- Christ, J.F.; Aliheidari, N.; Ameli, A.; Potschke, P. 3D printed highly elastic strain sensors of multiwalled carbon nanotube/thermoplastic polyurethane nanocomposites. Mater. Des. 2017, 131, 394–401. [Google Scholar] [CrossRef]
- Dhiman, P.; Kaur, A.; Balasaraswathi, V.R.; Gulzar, Y.; Alwan, A.A.; Hamid, Y. Image Acquisition, Preprocessing and Classification of Citrus Fruit Diseases: A Systematic Literature Review. Sustainability 2023, 15, 9643. [Google Scholar] [CrossRef]
- van Meekeren, A.; Gavari, M.A.; Dijkstra, K. Exploring the Effectiveness of Dataset Synthesis: An application of Apple Detection in Orchards. arXiv preprint 2023, arXiv:2306.11763v1. [Google Scholar]
- Cui, G.P.; Zheng, X.M.; Cui, Y.J.; Wang, J.Z.; Shi, Y.G.; Fu, L.S. Automatic Orientation Method for Postharvest Cabbage Based on Moment of Inertia. Trans. Chin. Soc. Agric. Mach. 2020, 51, 183–195. [Google Scholar] [CrossRef]
- Zhao, H.; Su, L.; Zhang, Y.; Cui, H.; Wang, J.; Ren, K. Analysis and Optimized Design of Rigid-Flexible Coupling Characteristics of Crab Apple Picking Machines and Crab Apple Trees. Appl. Sci. 2023, 13, 10502. [Google Scholar] [CrossRef]
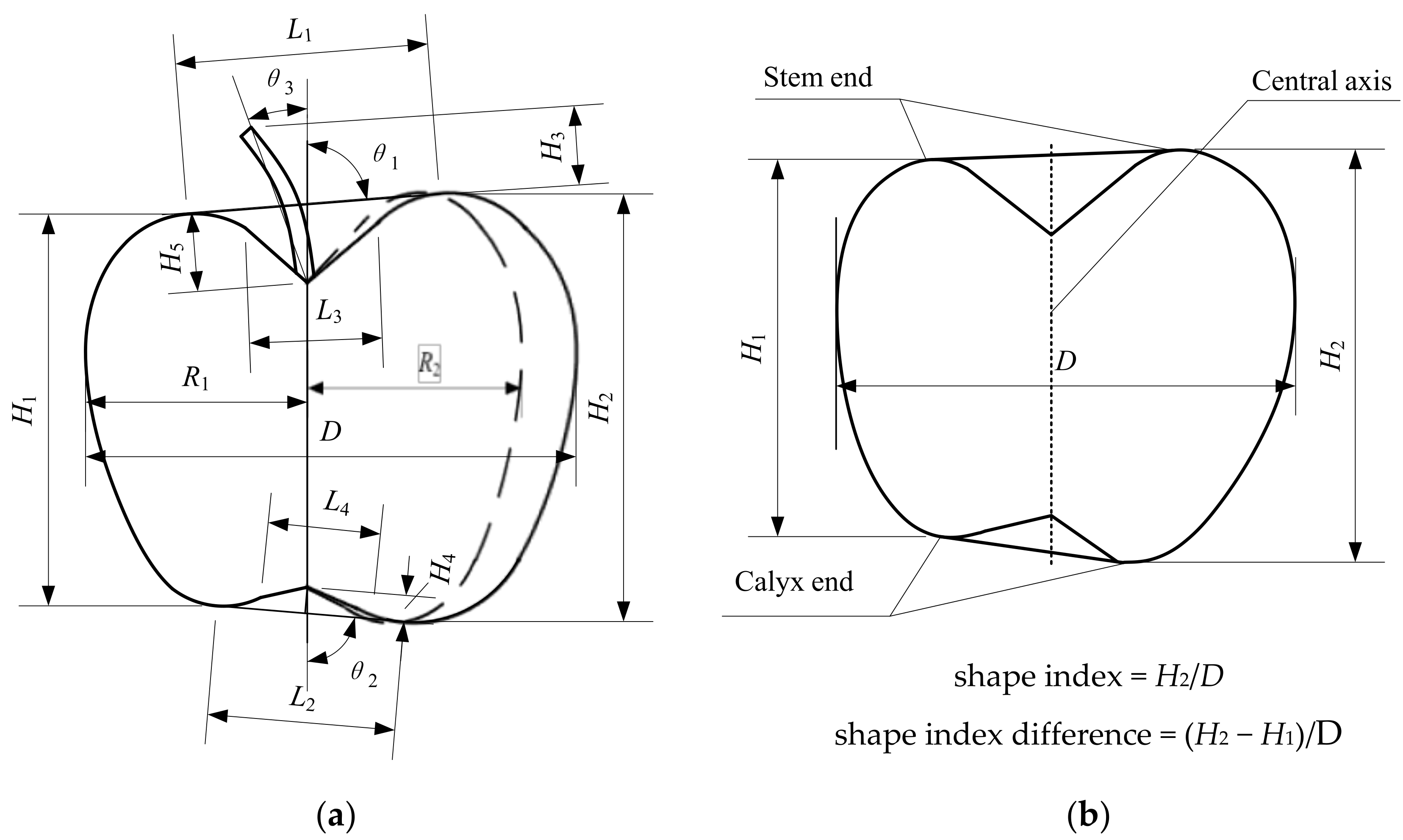


| Apple’s Dimensions | Maximum Values | Minimum Value | Average Value | Standard Deviation |
|---|---|---|---|---|
| R1 (mm) | 59.70 | 26.18 | 40.41 | 5.41 |
| R2 (mm) | 55.30 | 21.80 | 39.60 | 5.16 |
| L1 (mm) | 58.50 | 25.30 | 37.62 | 5.52 |
| L2 (mm) | 50.10 | 18.90 | 29.29 | 4.66 |
| H3 (mm) | 26.10 | 0.00 | 9.29 | 4.60 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Luo, T.; Zhou, J.; Zhang, S.; Chen, J.; Hu, G.; Sugirbay, A. Theoretical Analysis and Experimental Research on the Apple Auto-Orientation Based on Flexible Roller. Horticulturae 2023, 9, 1235. https://doi.org/10.3390/horticulturae9111235
Luo T, Zhou J, Zhang S, Chen J, Hu G, Sugirbay A. Theoretical Analysis and Experimental Research on the Apple Auto-Orientation Based on Flexible Roller. Horticulturae. 2023; 9(11):1235. https://doi.org/10.3390/horticulturae9111235
Chicago/Turabian StyleLuo, Tongyun, Jianguo Zhou, Shuo Zhang, Jun Chen, Guangrui Hu, and Adilet Sugirbay. 2023. "Theoretical Analysis and Experimental Research on the Apple Auto-Orientation Based on Flexible Roller" Horticulturae 9, no. 11: 1235. https://doi.org/10.3390/horticulturae9111235
APA StyleLuo, T., Zhou, J., Zhang, S., Chen, J., Hu, G., & Sugirbay, A. (2023). Theoretical Analysis and Experimental Research on the Apple Auto-Orientation Based on Flexible Roller. Horticulturae, 9(11), 1235. https://doi.org/10.3390/horticulturae9111235






