Research on Mixing Law of Liquid Fertilizer Injected into Irrigation Pipe
Abstract
1. Introduction
2. Materials and Methods
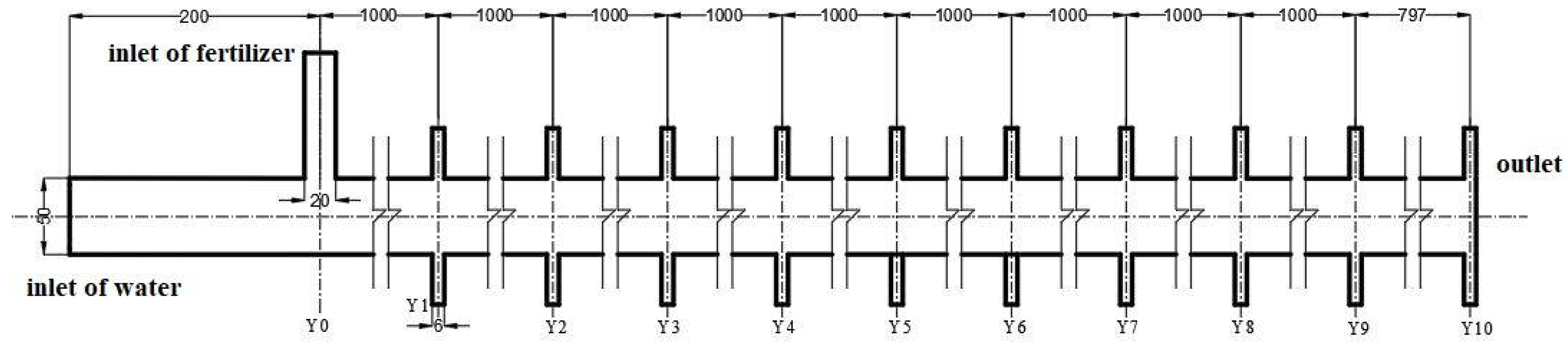
2.1. Experimental Establishment
2.2. Computational Fluid Dynamics Simulation
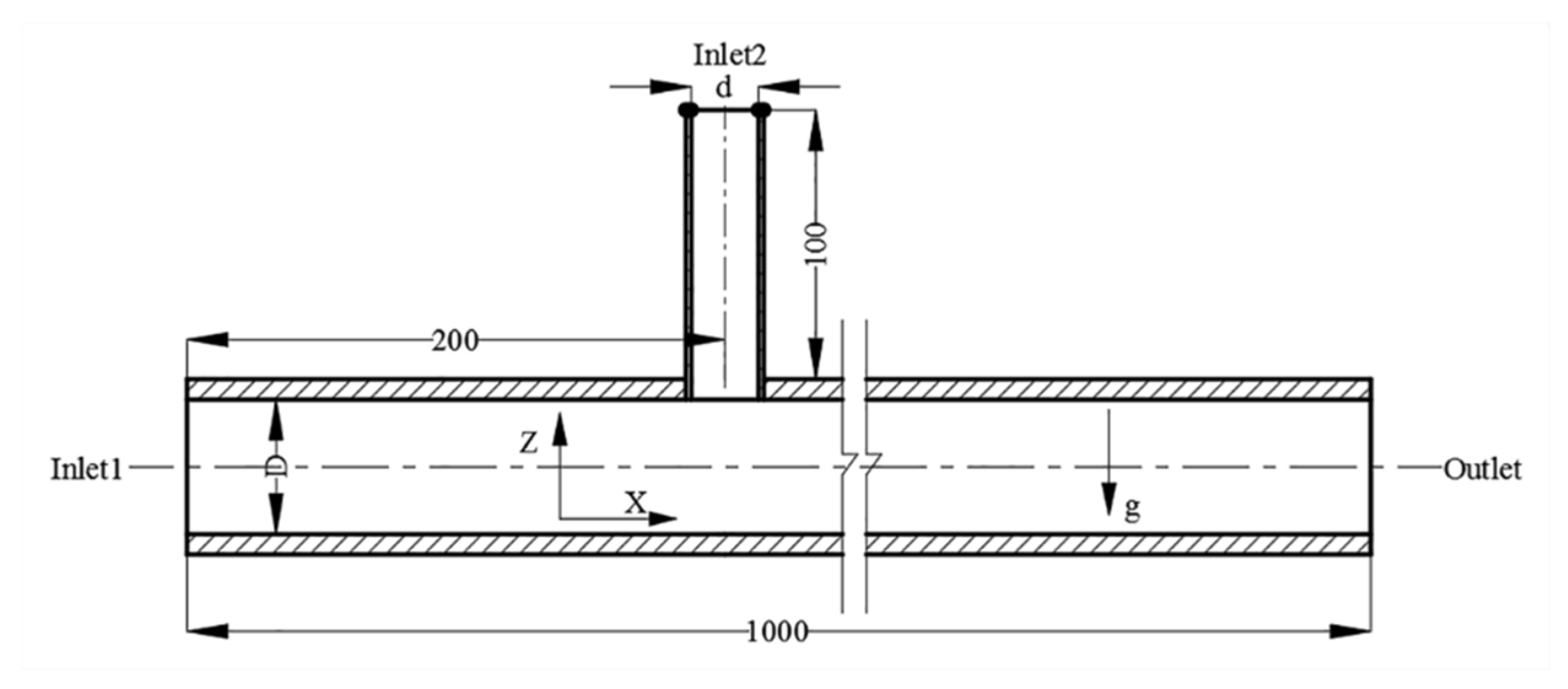
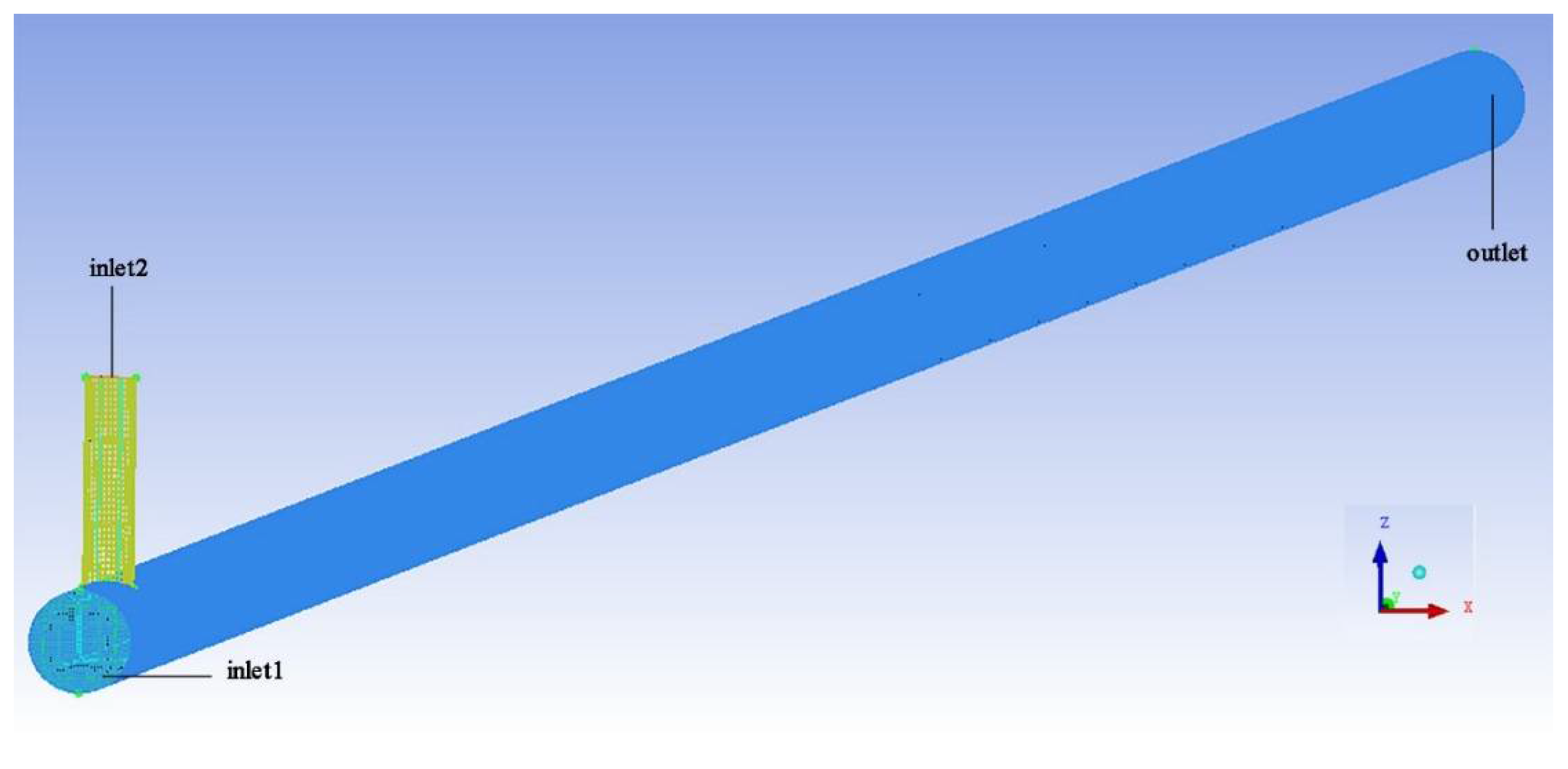
2.2.1. The Geometric Model
2.2.2. Governing Equation
2.2.3. Boundary Conditions
2.3. Mesh Independence Check
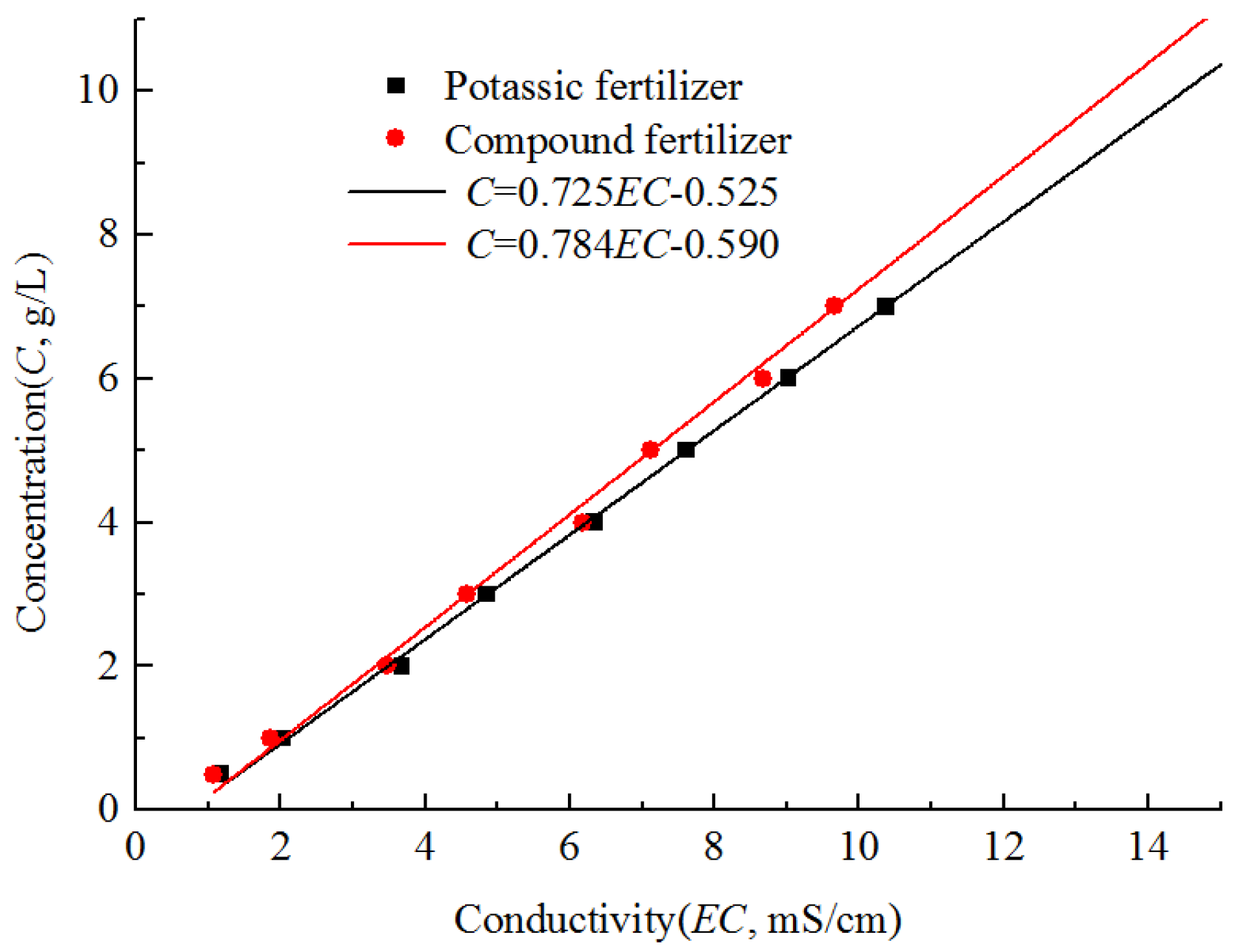
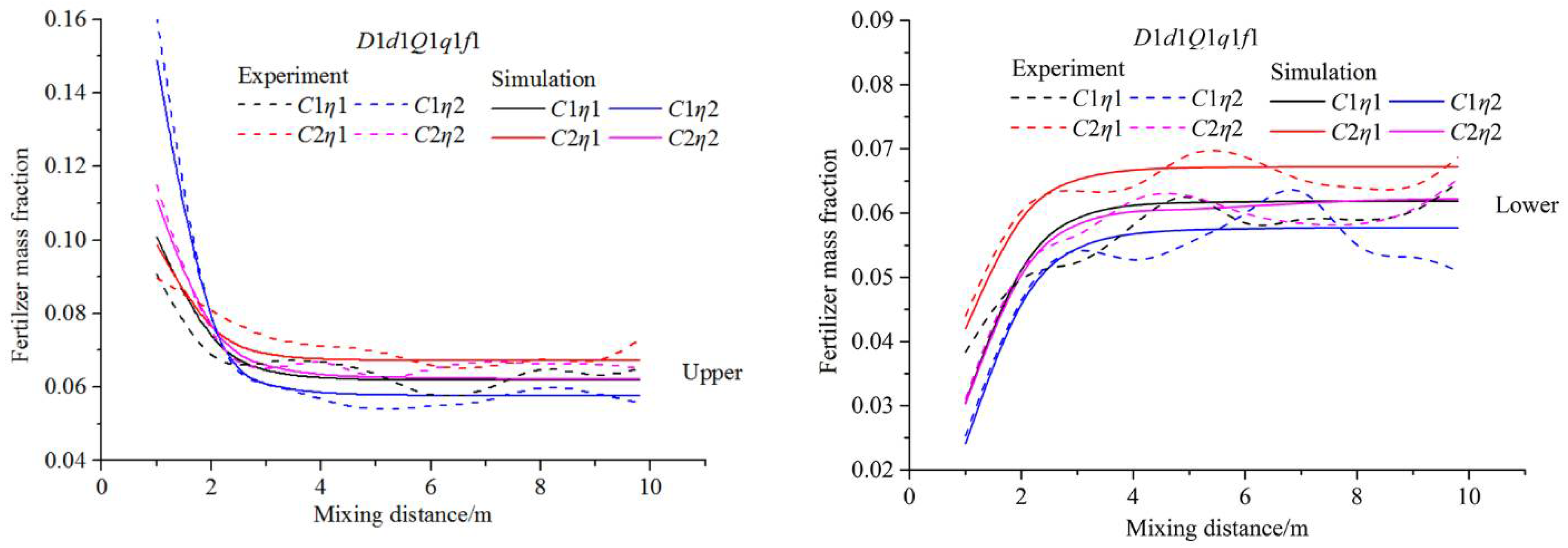
2.4. Comparison of Numerical and Experimental Results
3. Results and Discussion
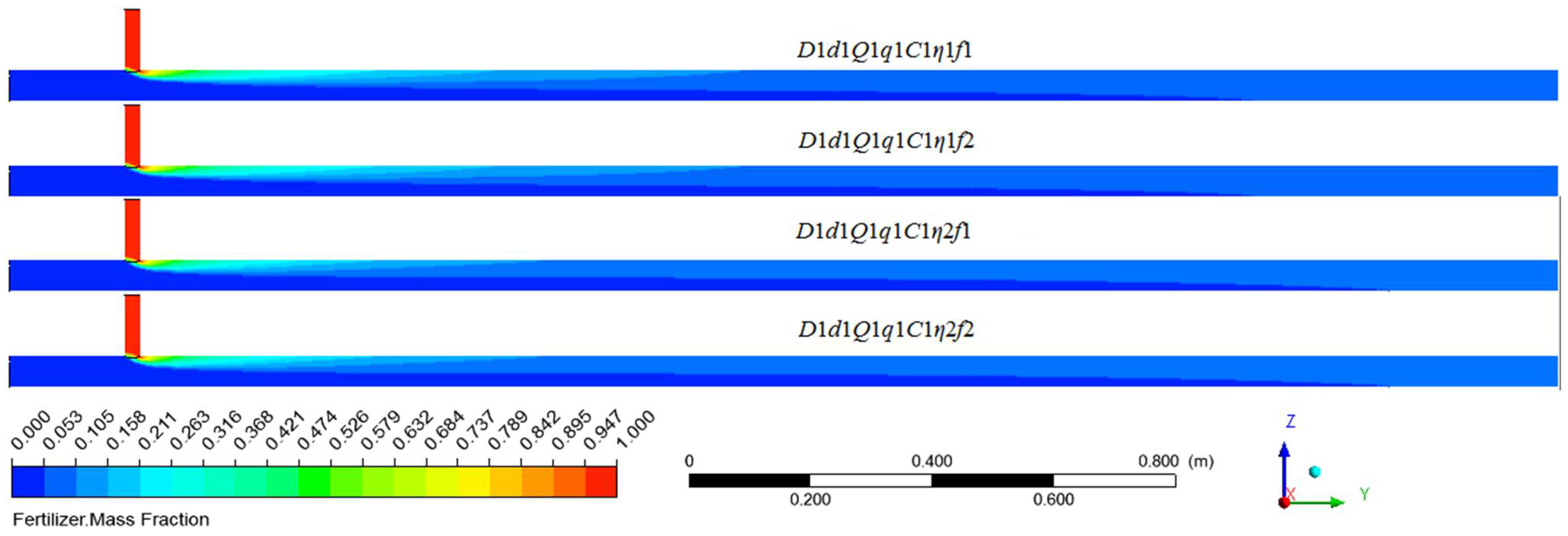
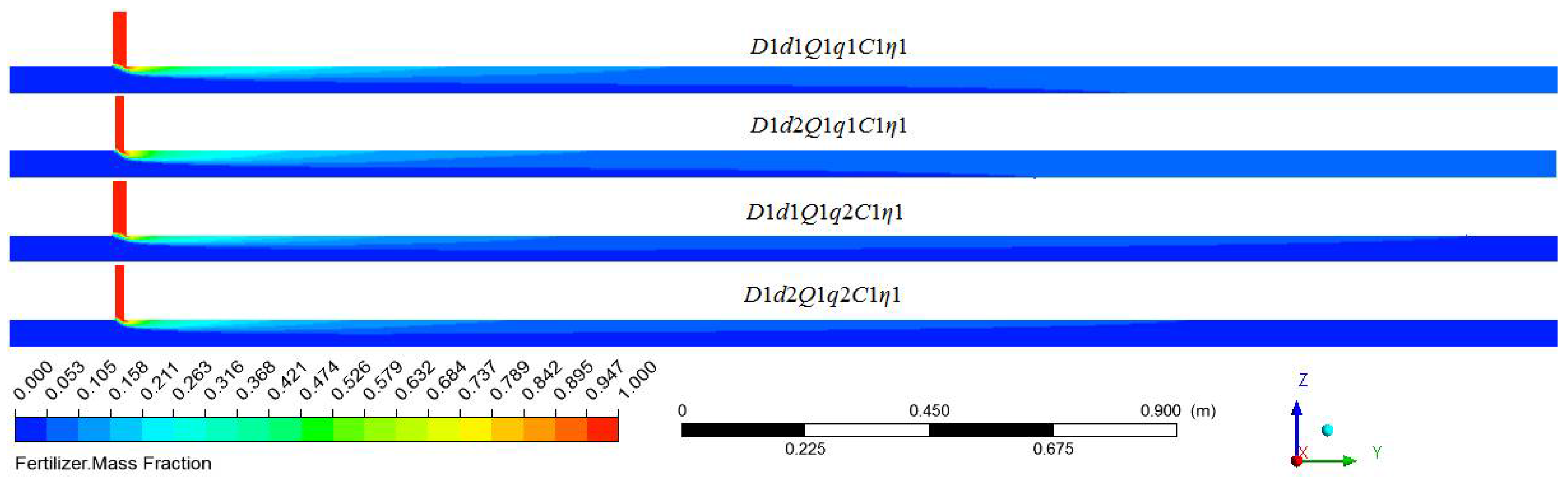
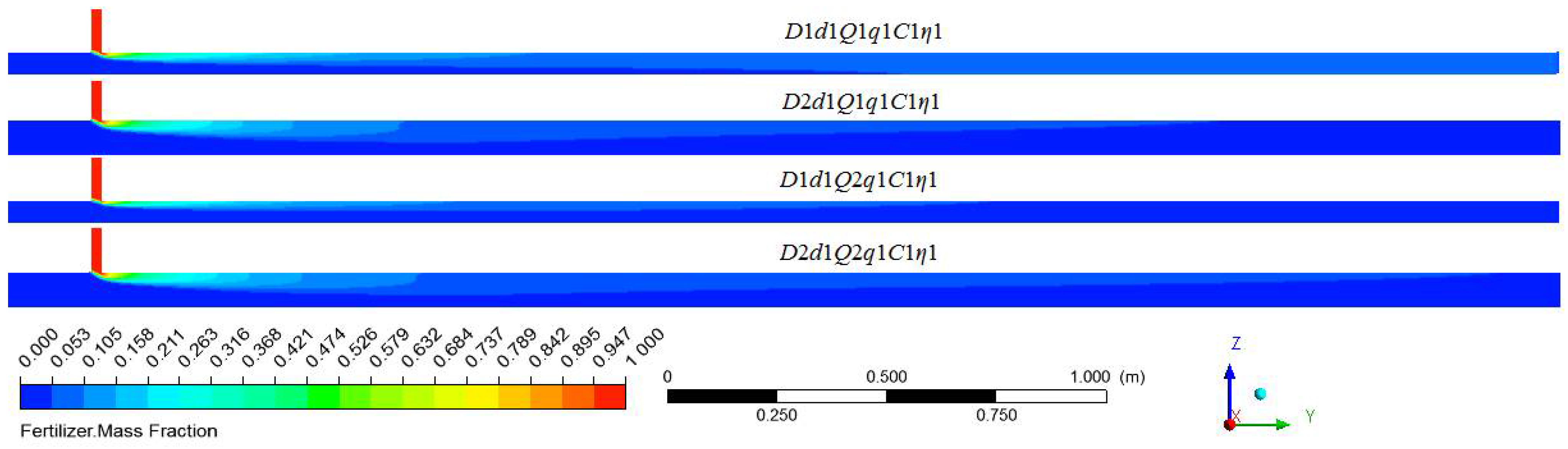
3.1. Fertilizer–Water Mixing State in the Pipes at Different Positions and Time
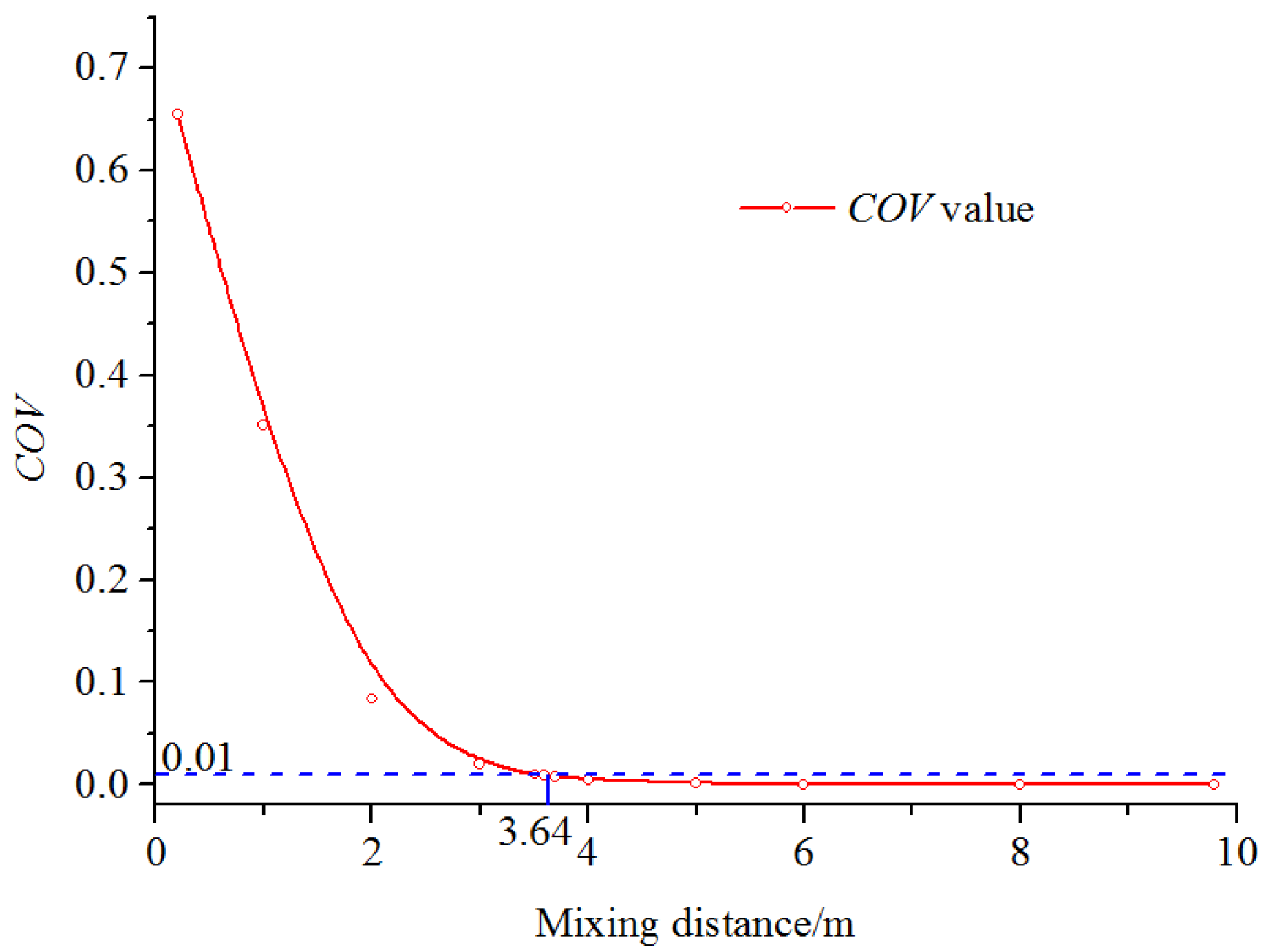
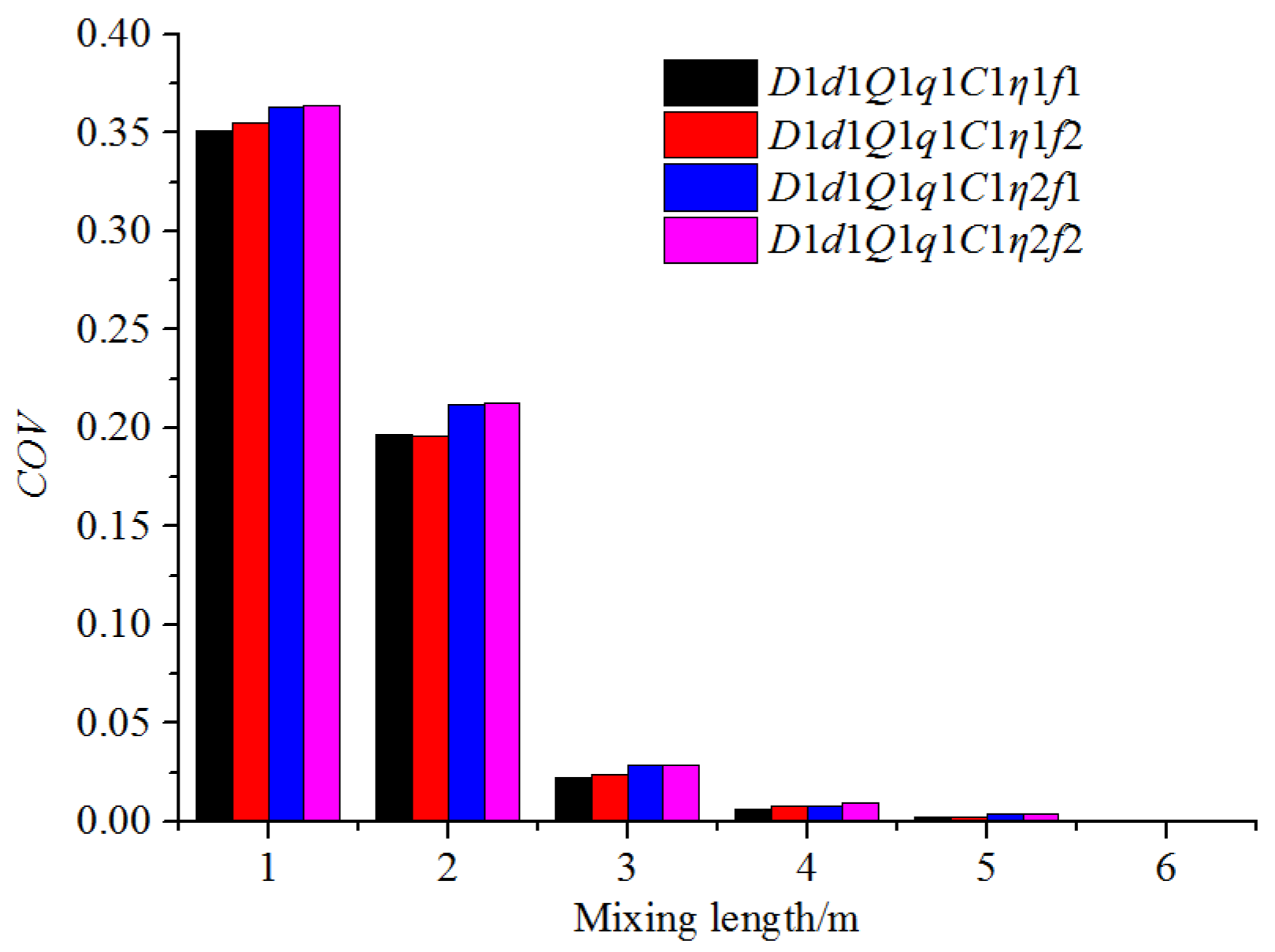
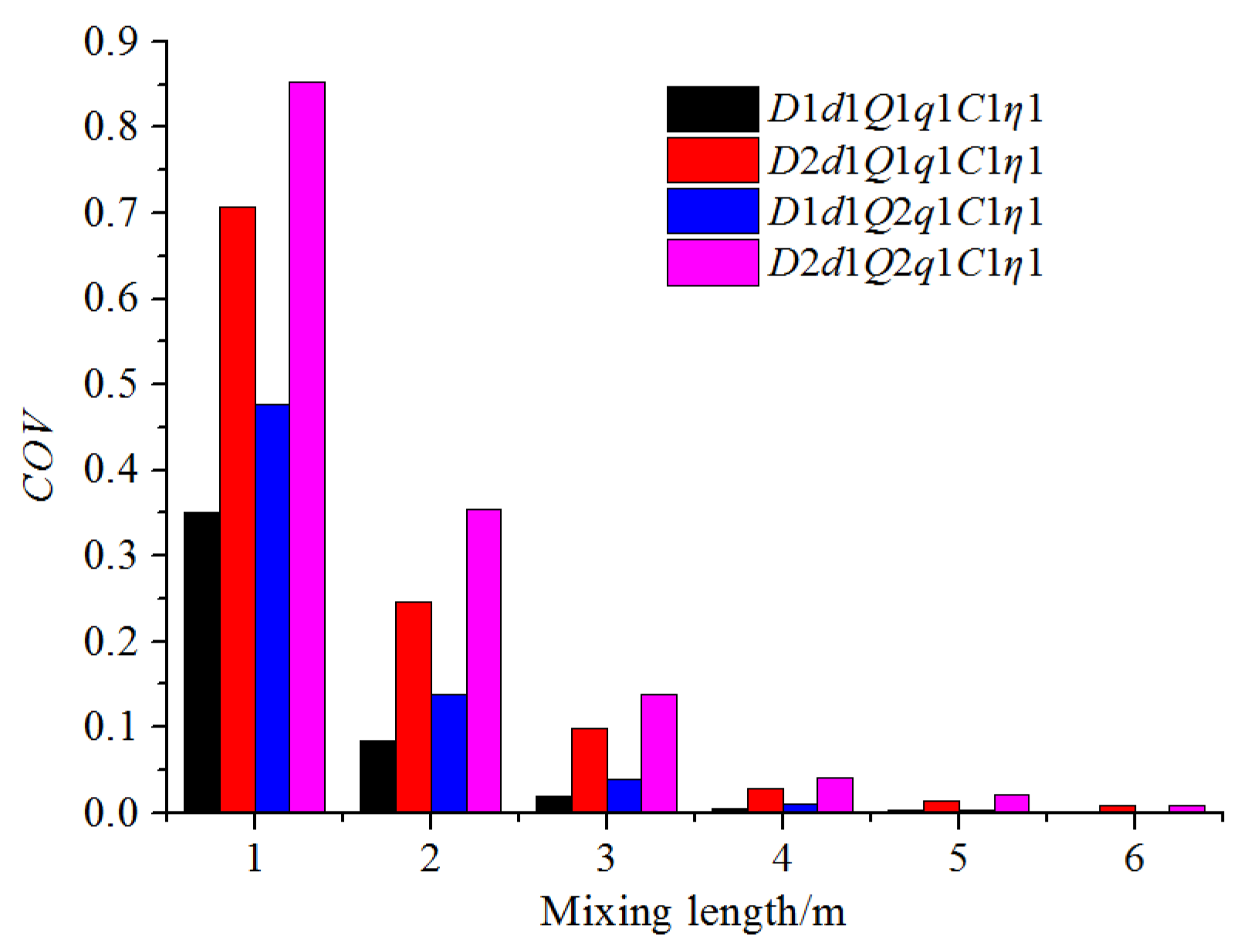
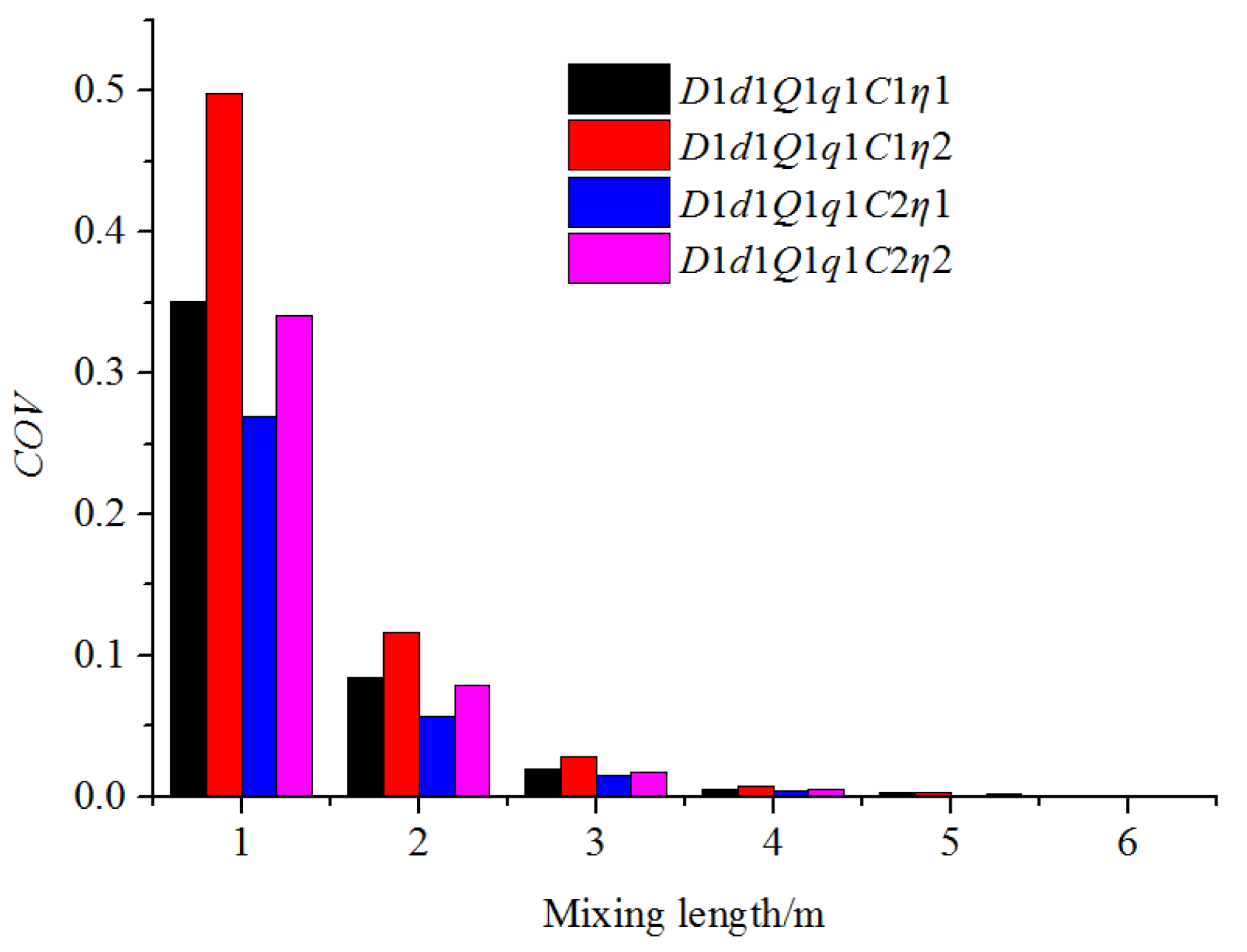
3.2. Factors Affecting the Mixing Speed and Uniform Mixing Length
3.3. Dimensional Analysis of the Uniformity Mixing Length
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Zhang, X.; Bol, R.; Rahn, C.; Xiao, G.; Meng, F.; Wu, W. Agricultural sustainable intensification improved nitrogen use efficiency and maintained high crop yield during 1980–2014 in Northern China. Sci. Total Environ. 2017, 596–597, 61–68. [Google Scholar] [CrossRef]
- Kaplan, M.; Kara, K.; Unlukara, A.; Kale, H.; Beyzi, S.B.; Varol, I.S.; Kizilsimsek, M.; Kamalak, A. Water deficit and nitrogen affects yield and feed value of sorghum sudangrass silage. Agric. Water Manage. 2019, 218, 30–36. [Google Scholar] [CrossRef]
- Stefani, I.E.A.; Roberto, K.H.G.; Mario, C.U.A.; Sillas, H. Video-based fractional order identification of diffusion dynamics for the analysis of migration rates of polar and nonpolar liquids: Water and oil studies. Rev. Sci. Instrum. 2021, 92, 035106. [Google Scholar]
- Han, H.; Furst, E.M.; Kim, C. Lagrangian analysis of consecutive images: Quantification of mixing processes in drops moving in a microchannel. Rheol. Acta 2014, 53, 489–499. [Google Scholar] [CrossRef]
- Hansen, L.; Guilkey, J.E.; Mcmurtry, P.A.; Klewicki, J.C. The use of photoactivatable fluorophores in the study of turbulent pipe mixing: Effects of inlet geometry. Meas. Sci. Technol. 2000, 11, 1235. [Google Scholar] [CrossRef]
- Ger, A.M.; Holley, E.R. Comparison of single-point injections in pipe flow. J. Hydraul. Div. 1976, 102, 731–746. [Google Scholar] [CrossRef]
- Nakayama, H.; Hirota, M.; Shinoda, K.; Koide, S. Flow and mixing characteristics in counter-type t-junction (influence of flow ratio on mixing characteristics). Trans. Jpn. Soc. Mech. Eng. Part B 2007, 73, 1813–1820. [Google Scholar] [CrossRef]
- Mi, Z.; Kulenovic, R.; Laurien, E. T-junction experiments to investigate thermal-mixing pipe flow with combined measurement techniques. Appl. Therm. Eng. 2018, 150, 237–249. [Google Scholar]
- Enrique, L.; Aguila, E. Application of sprinkler system for nitrogen fertilization in corn. Rev. Fac. Agron. 2019, 36, 490–496. [Google Scholar]
- Finzi, A.; Guido, V.; Riva, E.; Ferrari, O.; Provolo, G. Performance and sizing of filtration equipment to replace mineral fertilizer with digestate in drip and sprinkler fertigation. J. Clean. Prod. 2021, 317, 128431. [Google Scholar] [CrossRef]
- Zughbi, H.D. Effects of jet protrusion on mixing in pipelines with side-tees. Chem. Eng. Res. Des. 2006, 84, 993–1000. [Google Scholar] [CrossRef]
- Lateef, M.O.; Olusola, K.; John, A. On the numerical simulation of turbulent pipe flow pattern using comsol multiphysics. Int. J. Sci. World 2013, 1, 13–18. [Google Scholar]
- Lin, G.H.; Chen, M.S.; Ferng, Y.M. Investigating thermal mixing and reverse flow characteristics in a t-junction using cfd methodology. Appl. Therm. Eng. 2016, 102, 733–741. [Google Scholar] [CrossRef]
- Sun, B.; Lu, Y.; Liu, Q.; Fang, H.; Zhang, C.; Zhang, J. Experimental and numerical analyses on mixing uniformity of water and saline in pipe flow. Water 2020, 12, 2281. [Google Scholar] [CrossRef]
- Tang, P.; Li, H.; Issaka, Z.; Chen, C. Effect of manifold layout and fertilizer solution concentration on fertilization and flushing times and uniformity of drip irrigation systems. Agri. Water Manag. 2018, 200, 71–79. [Google Scholar] [CrossRef]
- Saoudi, O.; Ghaouar, N.; Othman, T. Conductivity measurements of laccase for various concentrations, pH and ionic liquid solutions. Fluid Phase Equilib. 2017, 443, 184–192. [Google Scholar] [CrossRef]
- Somkhuean, R.; Niwitpong, S.; Niwitpong, S.A. Upper Bounds of Generalized p-values for Testing the Coefficients of Variation of Lognormal Distributions. Chiang Mai J. Sci. 2016, 43, 672–682. [Google Scholar]
- Mohammed, H.I.; Giddings, D.; Walker, G.S. CFD simulation of a concentrated salt nanofluid flow boiling in a rectangular tube. Int. J. Heat Mass Transf. 2018, 125, 218–228. [Google Scholar] [CrossRef]
- Tang, P.; Juárez, J.M.; Li, H. Investigation on the effect of structural parameters on cavitation characteristics for the venturi tube using the cfd method. Water 2019, 11, 2194. [Google Scholar] [CrossRef]
- Spiessl, S.M.; Prommer, H.; Licha, T.; Sauter, M.; Zheng, C. A process-based reactive hybrid transport model for coupled discrete conduit–continuum systems. J. Hydrol. 2007, 347, 23–34. [Google Scholar] [CrossRef]
- Sugiharto; Stegowski, Z.; Furman, L.; Su’Ud, Z.; Abidin, Z. Dispersion determination in a turbulent pipe flow using radiotracer data and cfd analysis. Comput. Fluids. 2013, 79, 77–81. [Google Scholar] [CrossRef]
- Widiatmojo, A.; Sasaki, K.; Widodo, N.P.; Sugai, Y. Discrete tracer point method to evaluate turbulent diffusion in circular pipe flow. J. Flow Control. Meas. Vis. 2013, 1, 57–68. [Google Scholar] [CrossRef][Green Version]
- Duda, J.L.; Vrentas, J.S.; Ju, S.T.; Liu, H.T. Prediction of diffusion coefficients for polymer-solvent systems. AIChE J. 2010, 28, 279–285. [Google Scholar] [CrossRef]
- Olaye, O.; Ojo, O. Time variation of concentration-dependent interdiffusion coefficient obtained by numerical simulation analysis. Materialia 2021, 16, 101056. [Google Scholar] [CrossRef]
- Amsden, B. Solute diffusion within hydrogels. mechanisms and models. Macromolecules 1998, 31, 8382–8395. [Google Scholar] [CrossRef]
- Mazza, M.G.; Giovambattista, N.; Stanley, H.E.; Starr, F.W. Connection of translational and rotational dynamical heterogeneities with the breakdown of the stokes-einstein and stokes-einstein-debye relations in water. Phys. Rev. E 2007, 76, 031203. [Google Scholar] [CrossRef] [PubMed]
- Asce, K.S.L.M.; Reeuwijk, M.V.; Maksimovic, C. Simplified numerical and analytical approach for solutes in turbulent flow reacting with smooth pipe walls. J. Hydraul. Eng. 2010, 136, 626–632. [Google Scholar]
- Lari, K.S.; van Reeuwijk, M.; Maksimovic, C.; Sharifan, S. Combined bulk and wall reactions in turbulent pipe flow: Decay coefficients and concentration profiles. J. Hydroinform. 2011, 13, 324–333. [Google Scholar] [CrossRef][Green Version]






| Concentration (gL−1) | 1 | 2 | 5 | 10 | 20 | 50 | 100 | 200 | |
|---|---|---|---|---|---|---|---|---|---|
| Viscosity (kgm−1s−1) | Compound fertilizer | 0.0021 | 0.0022 | 0.0022 | 0.0022 | 0.0023 | 0.0022 | 0.0022 | 0.0022 |
| Potassium chloride | 0.0055 | 0.0055 | 0.0055 | 0.0055 | 0.0054 | 0.0053 | 0.0055 | 0.0055 | |
| Levels | Factors | ||||||
|---|---|---|---|---|---|---|---|
| D (m) | d (m) | Q (m3h−1) | q (m3h−1) | C (gL−1) | η (kgm−1s−1) | f (HZ) | |
| 1 | 0.05 | 0.025 | 5 | 0.3 | 100 | 0.0022 | 2 |
| 2 | 0.08 | 0.015 | 8 | 0.2 | 200 | 0.0055 | 4 |
| Number of Cells | Fertilizer Mass Fraction | |||||
|---|---|---|---|---|---|---|
| Upper of Y1 | Lower of Y1 | Upper of Y2 | Lower of Y2 | Upper of Y3 | Lower of Y3 | |
| 2,432,007 | 0.101 | 0.305 | 0.699 | 0.541 | 0.639 | 0.599 |
| 2,625,480 | 0.100 | 0.302 | 0.702 | 0.539 | 0.631 | 0.606 |
| 2,932,580 | 0.100 | 0.319 | 0.709 | 0.523 | 0.625 | 0.607 |
| Group | Lu | Group | Lu | Group | Lu | Group | Lu |
|---|---|---|---|---|---|---|---|
| D1d1Q1q1C1η1 | 3.64 | D1d2Q1q1C1η1 | 3.46 | D2d1Q1q1C1η1 | 5.55 | D2d2Q1q1C1η1 | 5.15 |
| D1d1Q1q1C1η2 | 3.75 | D1d2Q1q1C1η2 | 3.51 | D2d1Q1q1C1η2 | 5.71 | D2d2Q1q1C1η2 | 5.38 |
| D1d1Q1q1C2η1 | 3.32 | D1d2Q1q1C2η1 | 3.18 | D2d1Q1q1C2η1 | 5.31 | D2d2Q1q1C2η1 | 4.94 |
| D1d1Q1q1C2η2 | 3.55 | D1d2Q1q1C2η2 | 3.33 | D2d1Q1q1C2η2 | 5.42 | D2d2Q1q1C2η2 | 5.29 |
| D1d1Q1q2C1η1 | 3.88 | D1d2Q1q2C1η1 | 3.59 | D2d1Q1q2C1η1 | 6.05 | D2d2Q1q2C1η1 | 5.56 |
| D1d1Q1q2C1η2 | 4.05 | D1d2Q1q2C1η2 | 3.89 | D2d1Q1q2C1η2 | 6.13 | D2d2Q1q2C1η2 | 6.01 |
| D1d1Q1q2C2η1 | 3.73 | D1d2Q1q2C2η1 | 3.48 | D2d1Q1q2C2η1 | 5.75 | D2d2Q1q2C2η1 | 5.43 |
| D1d1Q1q2C2η2 | 3.93 | D1d2Q1q2C2η2 | 3.63 | D2d1Q1q2C2η2 | 5.98 | D2d2Q1q2C2η2 | 5.79 |
| D1d1Q2q1C1η1 | 4.08 | D1d2Q2q1C1η1 | 3.83 | D2d1Q2q1C1η1 | 5.93 | D2d2Q2q1C1η1 | 5.74 |
| D1d1Q2q1C1η2 | 4.34 | D1d2Q2q1C1η2 | 3.95 | D2d1Q2q1C1η2 | 6.21 | D2d2Q2q1C1η2 | 6.05 |
| D1d1Q2q1C2η1 | 3.92 | D1d2Q2q1C2η1 | 3.63 | D2d1Q2q1C2η1 | 5.83 | D2d2Q2q1C2η1 | 5.32 |
| D1d1Q2q1C2η2 | 4.15 | D1d2Q2q1C2η2 | 3.81 | D2d1Q2q1C2η2 | 6.11 | D2d2Q2q1C2η2 | 5.76 |
| D1d1Q2q2C1η1 | 4.51 | D1d2Q2q2C1η1 | 4.26 | D2d1Q2q2C1η1 | 6.52 | D2d2Q2q2C1η1 | 6.22 |
| D1d1Q2q2C1η2 | 4.71 | D1d2Q2q2C1η2 | 4.43 | D2d1Q2q2C1η2 | 6.96 | D2d2Q2q2C1η2 | 6.55 |
| D1d1Q2q2C2η1 | 4.32 | D1d2Q2q2C2η1 | 3.92 | D2d1Q2q2C2η1 | 6.39 | D2d2Q2q2C2η1 | 6.03 |
| D1d1Q2q2C2η2 | 4.47 | D1d2Q2q2C2η2 | 4.11 | D2d1Q2q2C2η2 | 6.68 | D2d2Q2q2C2η2 | 6.32 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, Z.; Chen, C.; Li, H.; Tang, P. Research on Mixing Law of Liquid Fertilizer Injected into Irrigation Pipe. Horticulturae 2022, 8, 200. https://doi.org/10.3390/horticulturae8030200
Zhang Z, Chen C, Li H, Tang P. Research on Mixing Law of Liquid Fertilizer Injected into Irrigation Pipe. Horticulturae. 2022; 8(3):200. https://doi.org/10.3390/horticulturae8030200
Chicago/Turabian StyleZhang, Zhiyang, Chao Chen, Hong Li, and Pan Tang. 2022. "Research on Mixing Law of Liquid Fertilizer Injected into Irrigation Pipe" Horticulturae 8, no. 3: 200. https://doi.org/10.3390/horticulturae8030200
APA StyleZhang, Z., Chen, C., Li, H., & Tang, P. (2022). Research on Mixing Law of Liquid Fertilizer Injected into Irrigation Pipe. Horticulturae, 8(3), 200. https://doi.org/10.3390/horticulturae8030200





