Reduction of Device Operating Temperatures with Graphene-Filled Thermal Interface Materials
Abstract
:1. Introduction
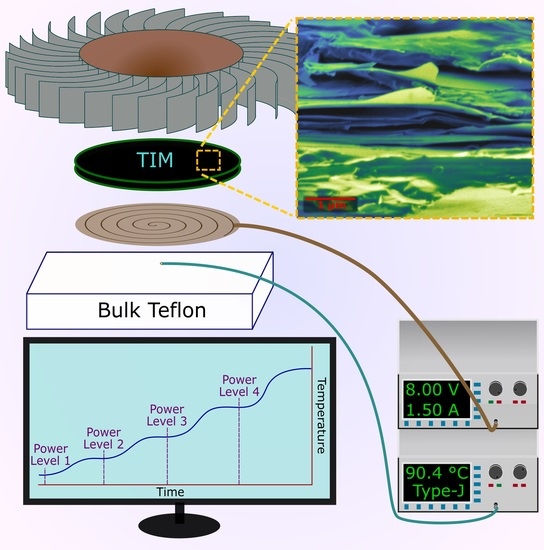
2. Materials and Methods
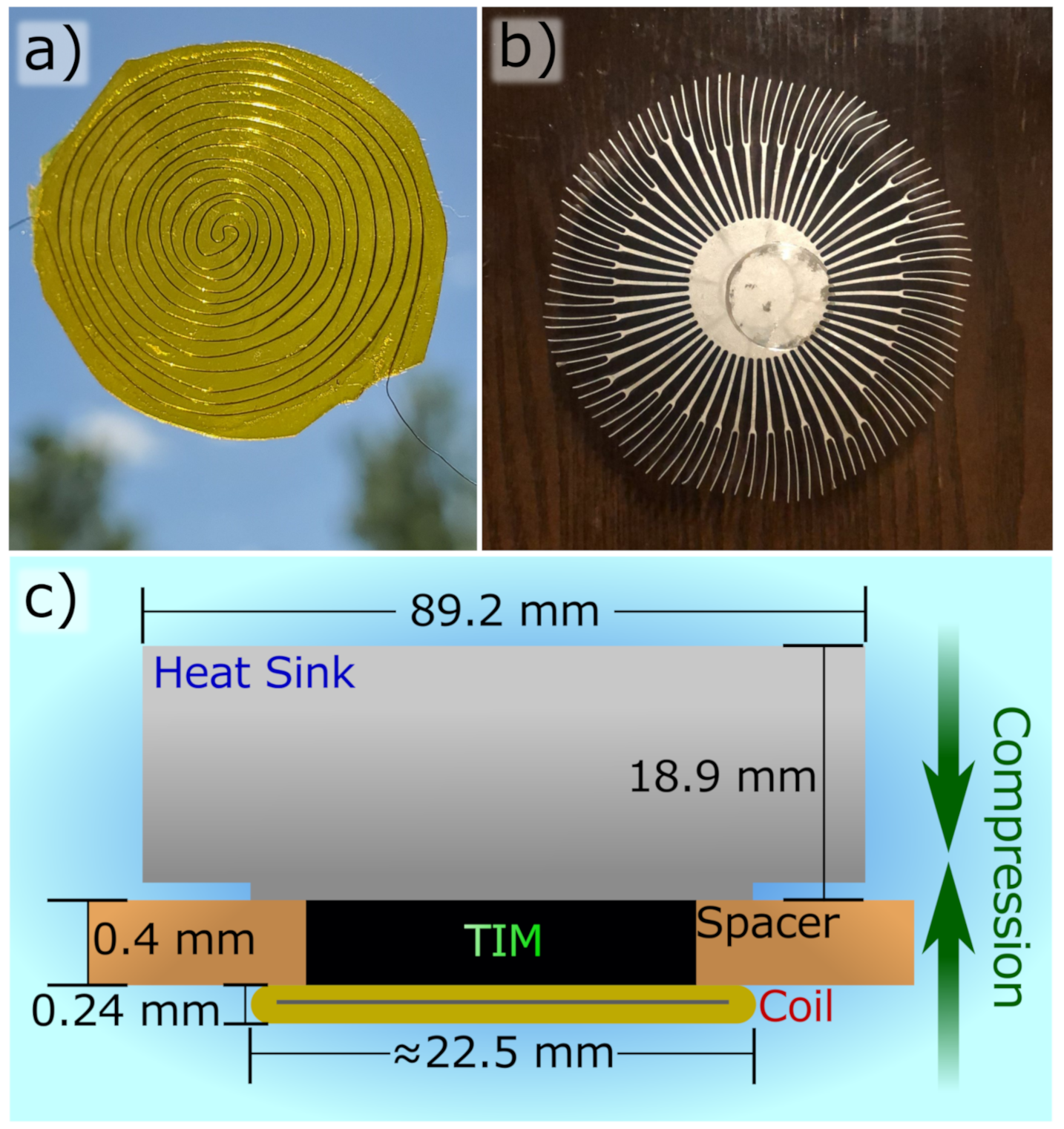
2.1. Sample Preparation
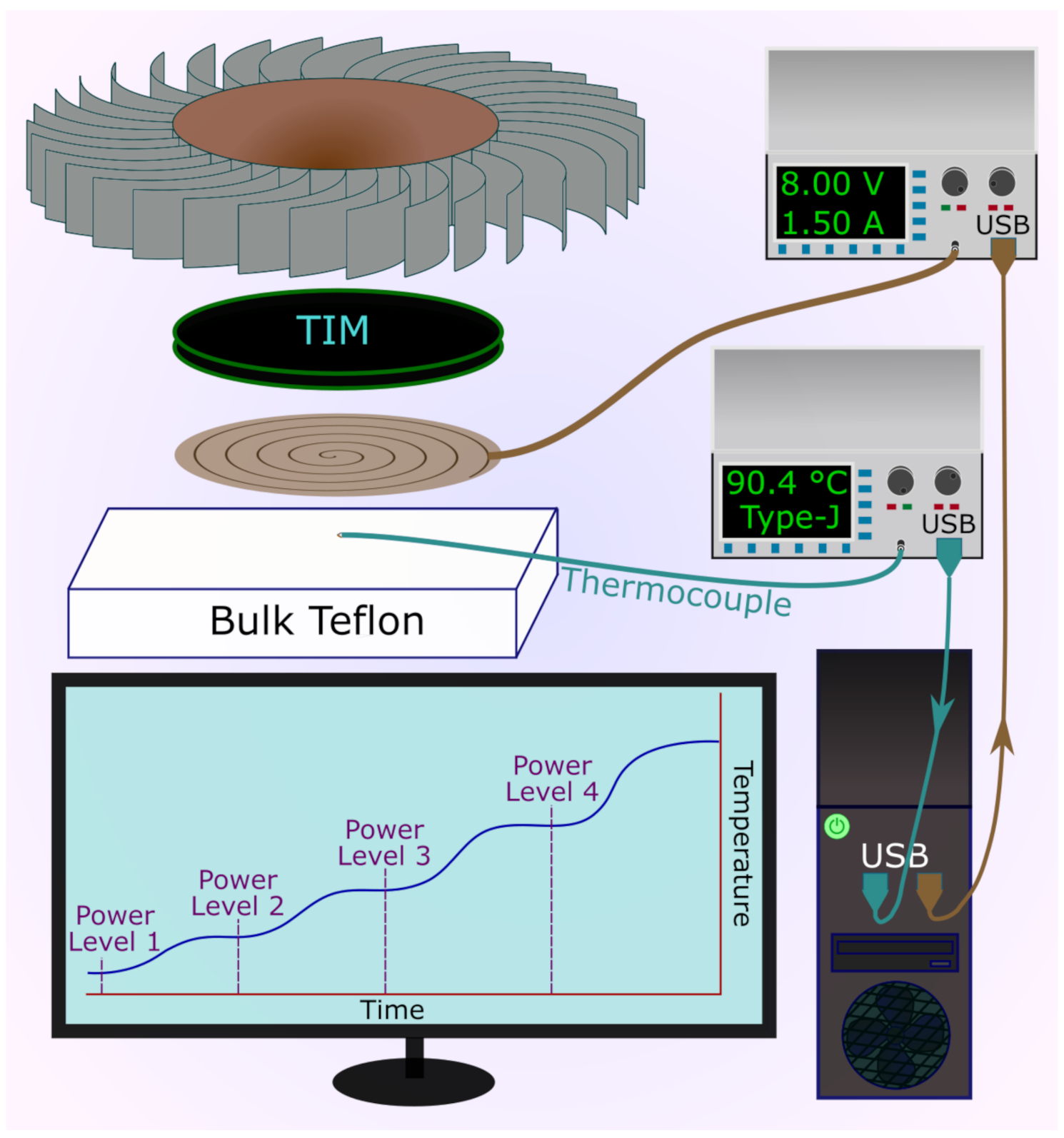
2.2. Experimental Procedure
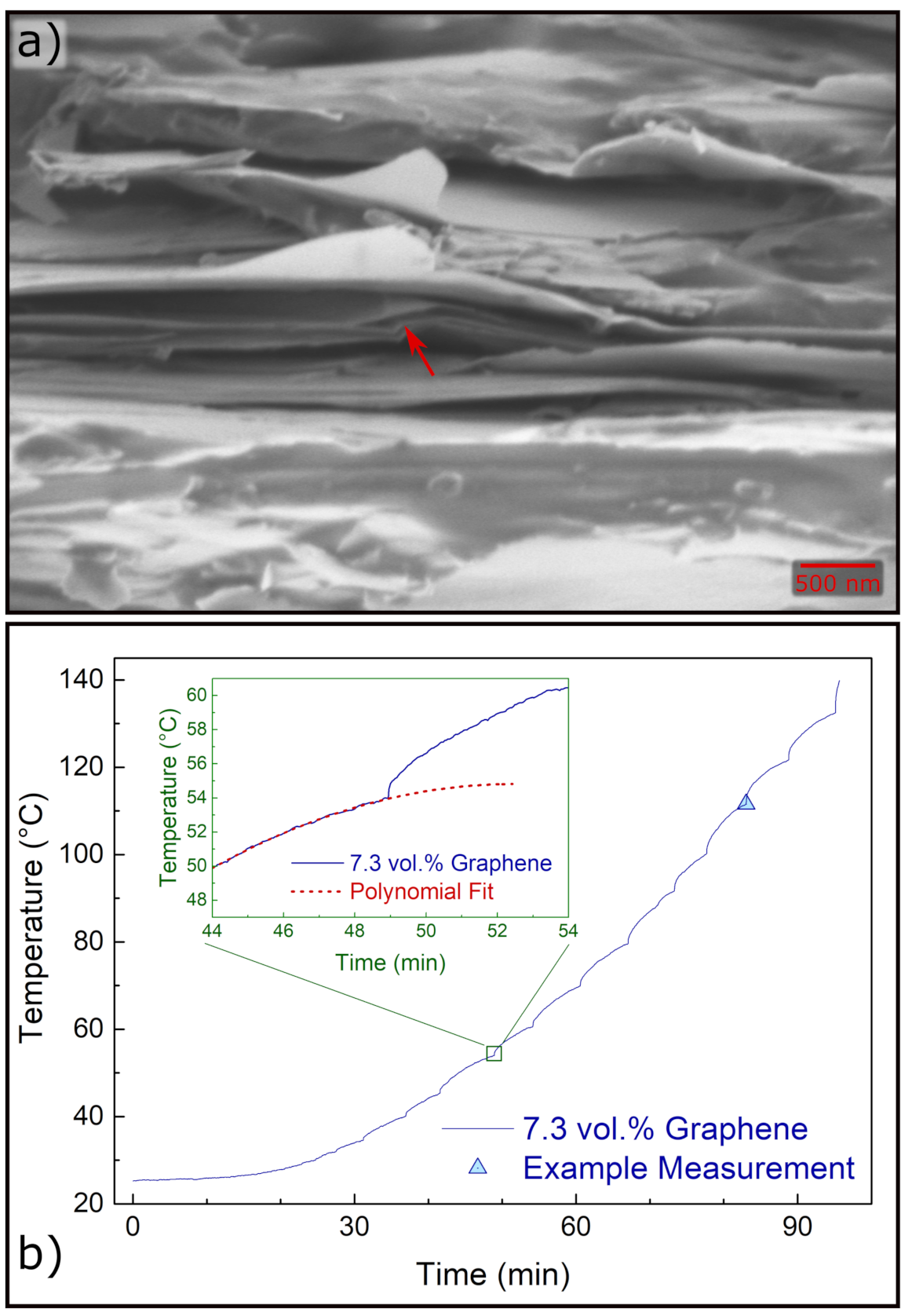
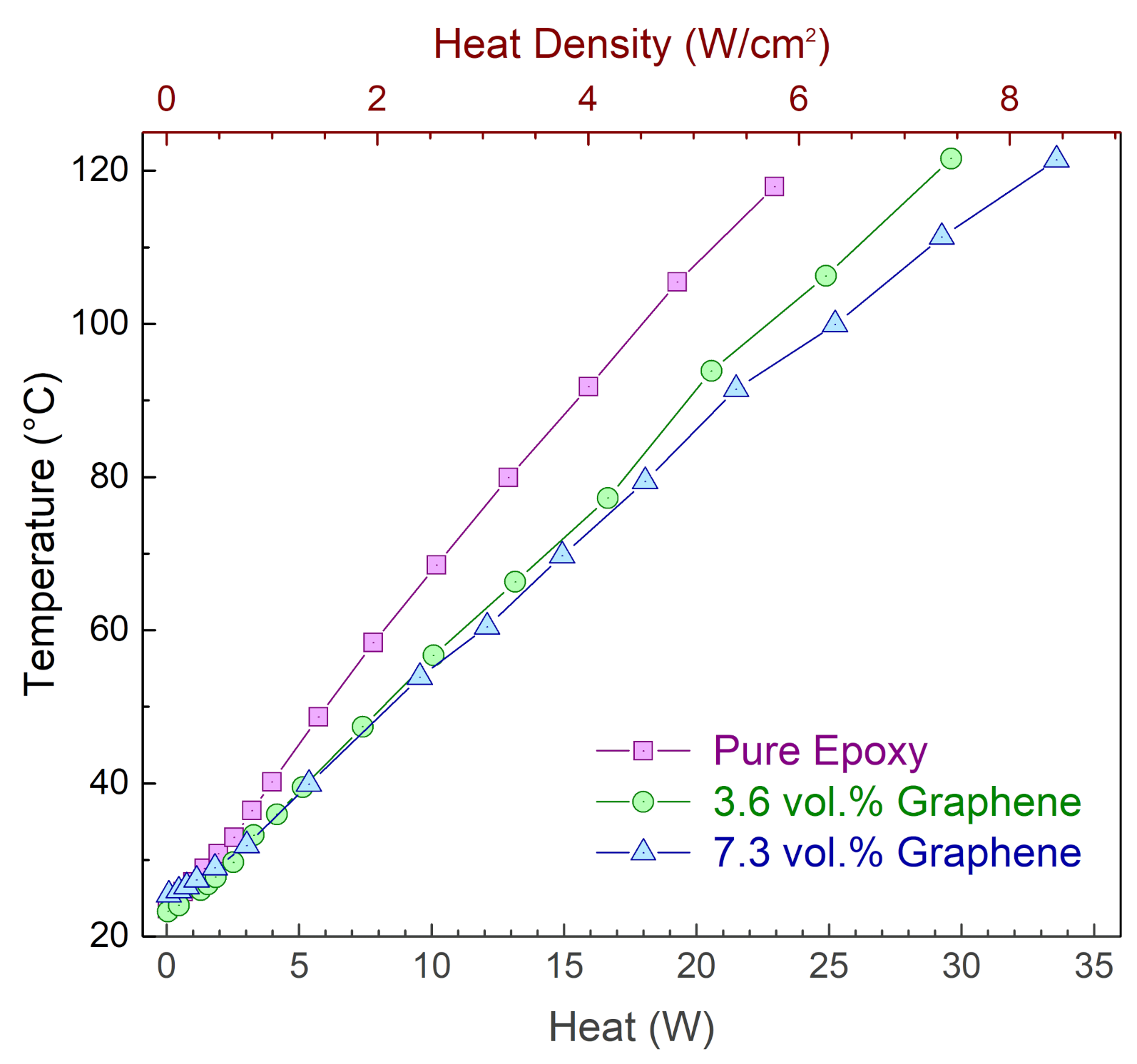
3. Results
4. Discussion
5. Conclusions
Supplementary Materials
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| TIM | Thermal interface material |
| FLG | few layer graphene |
| DGEBA | Diglycidyl ether of Bisphenol A |
| SCPI | standard commands for programmable instruments |
| Sensor time constant | |
| The experimental temperature recording x iterations previous to the most recent | |
| Least squares fit of equilibrium temperature as a function of applied heat | |
| q | Applied heat |
References
- Lewis, J.S. Thermal and Electrical Performance Control and Lifespan Progression of Graphene-Based Polymer Composites. Ph.D. Thesis, University of California-Riverside, Riverside, CA, USA, 2020. [Google Scholar]
- McCune, E. Fundamentals for Energy-Efficient Massive MIMO. In Proceedings of the 2017 IEEE Wireless Communications and Networking Conference Workshops (WCNCW), San Francisco, CA, USA, 19–22 March 2017; pp. 1–6. [Google Scholar] [CrossRef]
- Guo, A.; Del Alamo, J.A. Unified Mechanism for Positive-A nd Negative-Bias Temperature Instability in GaN MOSFETs. IEEE Trans. Electron Devices 2017, 64, 2142–2147. [Google Scholar] [CrossRef]
- Yu, Z.; Wang, R.; Hao, P.; Guo, S.; Ren, P.; Huang, R. Non-Universal Temperature Dependence of Hot Carrier Degradation (HCD) in FinFET: New Observations and Physical Understandings. In Proceedings of the 2018 IEEE Electron Devices Technology and Manufacturing Conference, EDTM 2018-Proceedings, Kobe, Japan, 13–16 March 2018; Institute of Electrical and Electronics Engineers Inc.: Manhattan, NY, USA, 2018; pp. 34–36. [Google Scholar] [CrossRef]
- Bury, E.; Chasin, A.; Kaczer, B.; Chuang, K.H.; Franco, J.; Simicic, M.; Weckx, P.; Linten, D. Self-heating-aware CMOS reliability characterization using degradation maps. In Proceedings of the IEEE International Reliability Physics Symposium Proceedings, Burlingame, CA, USA, 1–15 March 2018; Institute of Electrical and Electronics Engineers Inc.: Manhattan, NY, USA, 2018; Volume 2018, pp. 2A.31–2A.36. [Google Scholar] [CrossRef]
- Aslan, Y.; Kiper, C.E.; Johannes van den Biggelaar, A.; Johannsen, U.; Yarovoy, A. Passive Cooling of mm-Wave Active Integrated 5G Base Station Antennas Using CPU Heatsinks. In Proceedings of the 2019 16th European Radar Conference (EuRAD), Paris, France, 2–4 October 2019; pp. 121–124. [Google Scholar]
- Smoyer, J.L.; Norris, P.M. Brief Historical Perspective in Thermal Management and the Shift Toward Management at the Nanoscale. Heat Transf. Eng. 2019, 40, 269–282. [Google Scholar] [CrossRef]
- Saadah, M.; Hernandez, E.; Balandin, A. Thermal Management of Concentrated Multi-Junction Solar Cells with Graphene-Enhanced Thermal Interface Materials. Appl. Sci. 2017, 7, 589. [Google Scholar] [CrossRef] [Green Version]
- Mahadevan, B.K.; Naghibi, S.; Kargar, F.; Balandin, A.A. Non-Curing Thermal Interface Materials with Graphene Fillers for Thermal Management of Concentrated Photovoltaic Solar Cells. C J. Carbon Res. 2019, 6, 2. [Google Scholar] [CrossRef] [Green Version]
- Lewis, J.S.; Perrier, T.; Barani, Z.; Kargar, F.; Balandin, A.A. Thermal interface materials with graphene fillers: Review of the state of the art and outlook for future applications. Nanotechnology 2021, 32, 142003. [Google Scholar] [CrossRef] [PubMed]
- Wong, C.P.; Bollampally, R.S. Thermal conductivity, elastic modulus, and coefficient of thermal expansion of polymer composites filled with ceramic particles for electronic packaging. J. Appl. Polym. Sci. 1999, 74, 3396–3403. [Google Scholar] [CrossRef]
- Murshed, S.; Leong, K.; Yang, C. Enhanced thermal conductivity of TiO2—Water based nanofluids. Int. J. Therm. Sci. 2005, 44, 367–373. [Google Scholar] [CrossRef]
- Sim, L.; Ramanan, S.; Ismail, H.; Seetharamu, K.; Goh, T. Thermal characterization of Al2O3 and ZnO reinforced silicone rubber as thermal pads for heat dissipation purposes. Thermochim. Acta 2005, 430, 155–165. [Google Scholar] [CrossRef]
- Cola, B.A.; Xu, X.; Fisher, T.S. Increased real contact in thermal interfaces: A carbon nanotube/foil material. Appl. Phys. Lett. 2007, 90, 093513. [Google Scholar] [CrossRef]
- Zeng, J.; Cao, Z.; Yang, D.; Sun, L.; Zhang, L. Thermal conductivity enhancement of Ag nanowires on an organic phase change material. J. Therm. Anal. Calorim. 2009, 101, 385–389. [Google Scholar] [CrossRef]
- Yu, W.; Xie, H.; Li, Y.; Chen, L. Experimental investigation on thermal conductivity and viscosity of aluminum nitride nanofluid. Particuology 2011, 9, 187–191. [Google Scholar] [CrossRef]
- Gao, Z.; Zhao, L. Effect of nano-fillers on the thermal conductivity of epoxy composites with micro-Al2O3 particles. Mater. Des. 2015, 66, 176–182. [Google Scholar] [CrossRef]
- Burger, N.; Laachachi, A.; Mortazavi, B.; Ferriol, M.; Lutz, M.; Toniazzo, V.; Ruch, D. Alignments and network of graphite fillers to improve thermal conductivity of epoxy-based composites. Int. J. Heat Mass Transf. 2015, 89, 505–513. [Google Scholar] [CrossRef]
- Yu, W.; Zhao, J.; Wang, M.; Hu, Y.; Chen, L.; Xie, H. Thermal conductivity enhancement in thermal grease containing different CuO structures. Nanoscale Res. Lett. 2015, 10, 1–8. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Du, H.; Qi, Y.; Yu, W.; Yin, J.; Xie, H. T-shape ZnO whisker: A more effective thermal conductive filler than spherical particles for the thermal grease. Int. J. Heat Mass Transf. 2017, 112, 1052–1056. [Google Scholar] [CrossRef]
- Quinton, B.; Elston, L.; Scofield, J.; Mukhopadhyay, S. Aligned Carbon Nanotube Arrays Bonded to Solid Graphite Substrates: Thermal Analysis for Future Device Cooling Applications. C J. Carbon Res. 2018, 4, 28. [Google Scholar] [CrossRef] [Green Version]
- NarayanPrabhu, K. The effect of load and addition of MWCNTs on silicone based TIMs on thermal contact heat transfer across Cu/Cu interface. Mater. Res. Express 2019, 6, 1165h9. [Google Scholar] [CrossRef]
- Theerthagiri, J.; Salla, S.; Senthil, R.A.; Nithyadharseni, P.; Madankumar, A.; Arunachalam, P.; Maiyalagan, T.; Kim, H.S. A review on ZnO nanostructured materials: Energy, environmental and biological applications. Nanotechnology 2019, 30, 392001. [Google Scholar] [CrossRef] [PubMed]
- Sharma, V.; Kagdada, H.L.; Jha, P.K.; Śpiewak, P.; Kurzydłowski, K.J. Thermal transport properties of boron nitride based materials: A review. Renew. Sustain. Energy Rev. 2020, 120, 109622. [Google Scholar] [CrossRef]
- Balandin, A.A.; Ghosh, S.; Bao, W.; Calizo, I.; Teweldebrhan, D.; Miao, F.; Lau, C.N. Superior Thermal Conductivity of Single-Layer Graphene. Nano Lett. 2008, 8, 902–907. [Google Scholar] [CrossRef] [PubMed]
- Balandin, A.A. Thermal properties of graphene and nanostructured carbon materials. Nat. Mater. 2011, 10, 569–581. [Google Scholar] [CrossRef] [Green Version]
- Ghosh, S.; Calizo, I.; Teweldebrhan, D.; Pokatilov, E.P.; Nika, D.L.; Balandin, A.A.; Bao, W.; Miao, F.; Lau, C.N. Extremely high thermal conductivity of graphene: Prospects for thermal management applications in nanoelectronic circuits. Appl. Phys. Lett. 2008, 92, 151911. [Google Scholar] [CrossRef]
- Seol, J.H.; Jo, I.; Moore, A.L.; Lindsay, L.; Aitken, Z.H.; Pettes, M.T.; Li, X.; Yao, Z.; Huang, R.; Broido, D.; et al. Two-Dimensional Phonon Transport in Supported Graphene. Science 2010, 328, 213–216. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Cai, W.; Moore, A.L.; Zhu, Y.; Li, X.; Chen, S.; Shi, L.; Ruoff, R.S. Thermal transport in suspended and supported monolayer graphene grown by chemical vapor deposition. Nano Lett. 2010, 10, 1645–1651. [Google Scholar] [CrossRef] [PubMed]
- Wang, H.; Kurata, K.; Fukunaga, T.; Ago, H.; Takamatsu, H.; Zhang, X.; Ikuta, T.; Takahashi, K.; Nishiyama, T.; Takata, Y. Simultaneous measurement of electrical and thermal conductivities of suspended monolayer graphene. J. Appl. Phys. 2016, 119, 244306. [Google Scholar] [CrossRef]
- Zhang, P.; Zeng, J.; Zhai, S.; Xian, Y.; Yang, D.; Li, Q. Thermal Properties of Graphene Filled Polymer Composite Thermal Interface Materials. Macromol. Mater. Eng. 2017, 302, 1700068. [Google Scholar] [CrossRef]
- Li, A.; Zhang, C.; Zhang, Y.F. Thermal Conductivity of Graphene-Polymer Composites: Mechanisms, Properties, and Applications. Polymers 2017, 9, 437. [Google Scholar] [CrossRef] [Green Version]
- Balandin, A.A. Phononics of graphene and related materials. ACS Nano 2020, 14, 5170–5178. [Google Scholar] [CrossRef] [PubMed]
- Shahil, K.M.; Balandin, A.A. Graphene-multilayer graphene nanocomposites as highly efficient thermal interface materials. Nano Lett. 2012, 12, 861–867. [Google Scholar] [CrossRef] [Green Version]
- Fu, Y.X.; He, Z.X.; Mo, D.C.; Lu, S.S. Thermal conductivity enhancement of epoxy adhesive using graphene sheets as additives. Int. J. Therm. Sci. 2014, 86, 276–283. [Google Scholar] [CrossRef]
- Shtein, M.; Nadiv, R.; Buzaglo, M.; Regev, O. Graphene-Based Hybrid Composites for Efficient Thermal Management of Electronic Devices. Acs Appl. Mater. Interfaces 2015, 7, 23725–23730. [Google Scholar] [CrossRef] [PubMed]
- Kargar, F.; Barani, Z.; Salgado, R.; Debnath, B.; Lewis, J.S.; Aytan, E.; Lake, R.K.; Balandin, A.A. Thermal Percolation Threshold and Thermal Properties of Composites with High Loading of Graphene and Boron Nitride Fillers. ACS Appl. Mater. Interfaces 2018, 10, 37555–37565. [Google Scholar] [CrossRef] [PubMed]
- Kargar, F.; Barani, Z.; Balinskiy, M.; Magana, A.S.; Lewis, J.S.; Balandin, A.A. Dual-Functional Graphene Composites for Electromagnetic Shielding and Thermal Management. Adv. Electron. Mater. 2019, 5, 1800558. [Google Scholar] [CrossRef] [Green Version]
- Naghibi, S.; Kargar, F.; Wright, D.; Huang, C.Y.T.; Mohammadzadeh, A.; Barani, Z.; Salgado, R.; Balandin, A.A. Noncuring Graphene Thermal Interface Materials for Advanced Electronics. Adv. Electron. Mater. 2020, 1901303. [Google Scholar] [CrossRef]
- Biercuk, M.J.; Llaguno, M.C.; Radosavljevic, M.; Hyun, J.K.; Johnson, A.T.; Fischer, J.E. Carbon nanotube composites for thermal management. Appl. Phys. Lett. 2002, 80, 2767–2769. [Google Scholar] [CrossRef]
- Martin, C.; Sandler, J.; Shaffer, M.; Schwarz, M.K.; Bauhofer, W.; Schulte, K.; Windle, A. Formation of percolating networks in multi-wall carbon-nanotube—Epoxy composites. Compos. Sci. Technol. 2004, 64, 2309–2316. [Google Scholar] [CrossRef]
- Stankovich, S.; Dikin, D.A.; Dommett, G.H.; Kohlhaas, K.M.; Zimney, E.J.; Stach, E.A.; Piner, R.D.; Nguyen, S.B.T.; Ruoff, R.S. Graphene-based composite materials. Nature 2006, 442, 282–286. [Google Scholar] [CrossRef]
- Pang, H.; Chen, T.; Zhang, G.; Zeng, B.; Li, Z.M. An electrically conducting polymer/graphene composite with a very low percolation threshold. Mater. Lett. 2010, 64, 2226–2229. [Google Scholar] [CrossRef]
- Zhang, H.B.; Zheng, W.G.; Yan, Q.; Yang, Y.; Wang, J.W.; Lu, Z.H.; Ji, G.Y.; Yu, Z.Z. Electrically conductive polyethylene terephthalate/graphene nanocomposites prepared by melt compounding. Polymer 2010, 51, 1191–1196. [Google Scholar] [CrossRef]
- Potts, J.R.; Dreyer, D.R.; Bielawski, C.W.; Ruoff, R.S. Graphene-based polymer nanocomposites. Polymer 2011, 52, 5–25. [Google Scholar] [CrossRef] [Green Version]
- Wang, Q.; Gao, W.; Xie, Z. Highly thermally conductive room-temperature-vulcanized silicone rubber and silicone grease. J. Appl. Polym. Sci. 2003, 89, 2397–2399. [Google Scholar] [CrossRef]
- Yu, A.; Ramesh, P.; Sun, X.; Bekyarova, E.; Itkis, M.E.; Haddon, R.C. Enhanced Thermal Conductivity in a Hybrid Graphite Nanoplatelet—Carbon Nanotube Filler for Epoxy Composites. Adv. Mater. 2008, 20, 4740–4744. [Google Scholar] [CrossRef]
- Zhou, T.; Wang, X.; Liu, X.; Xiong, D. Improved thermal conductivity of epoxy composites using a hybrid multi-walled carbon nanotube/micro-SiC filler. Carbon 2010, 48, 1171–1176. [Google Scholar] [CrossRef]
- Li, T.L.; Hsu, S.L.C. Enhanced Thermal Conductivity of Polyimide Films via a Hybrid of Micro- and Nano-Sized Boron Nitride. J. Phys. Chem. B 2010, 114, 6825–6829. [Google Scholar] [CrossRef]
- Kemaloglu, S.; Ozkoc, G.; Aytac, A. Properties of thermally conductive micro and nano size boron nitride reinforced silicon rubber composites. Thermochim. Acta 2010, 499, 40–47. [Google Scholar] [CrossRef]
- Yang, K.; Gu, M. Enhanced thermal conductivity of epoxy nanocomposites filled with hybrid filler system of triethylenetetramine-functionalized multi-walled carbon nanotube/silane-modified nano-sized silicon carbide. Compos. Part A Appl. Sci. Manuf. 2010, 41, 215–221. [Google Scholar] [CrossRef]
- Pak, S.Y.; Kim, H.M.; Kim, S.Y.; Youn, J.R. Synergistic improvement of thermal conductivity of thermoplastic composites with mixed boron nitride and multi-walled carbon nanotube fillers. Carbon 2012, 50, 4830–4838. [Google Scholar] [CrossRef]
- Teng, C.C.; Ma, C.C.M.; Chiou, K.C.; Lee, T.M. Synergetic effect of thermal conductive properties of epoxy composites containing functionalized multi-walled carbon nanotubes and aluminum nitride. Compos. Part B Eng. 2012, 43, 265–271. [Google Scholar] [CrossRef]
- Lewis, J.S.; Barani, Z.; Magana, A.S.; Kargar, F.; Balandin, A.A. Thermal and electrical conductivity control in hybrid composites with graphene and boron nitride fillers. Mater. Res. Express 2019, 6, 085325. [Google Scholar] [CrossRef] [Green Version]
- Yang, S.Y.; Lin, W.N.; Huang, Y.L.; Tien, H.W.; Wang, J.Y.; Ma, C.C.M.; Li, S.M.; Wang, Y.S. Synergetic effects of graphene platelets and carbon nanotubes on the mechanical and thermal properties of epoxy composites. Carbon 2011, 49, 793–803. [Google Scholar] [CrossRef]
- Ma, A.-j.; Chen, W.; Hou, Y. Enhanced Thermal Conductivity of Epoxy Composites with MWCNTs/AlN Hybrid Filler. Polym. Plast. Technol. Eng. 2012, 51, 1578–1582. [Google Scholar] [CrossRef]
- Yu, L.; Park, J.S.; Lim, Y.S.; Lee, C.S.; Shin, K.; Moon, H.J.; Yang, C.M.; Lee, Y.S.; Han, J.H. Carbon hybrid fillers composed of carbon nanotubes directly grown on graphene nanoplatelets for effective thermal conductivity in epoxy composites. Nanotechnology 2013, 24, 155604. [Google Scholar] [CrossRef] [PubMed]
- Fang, X.; Fan, L.W.; Ding, Q.; Wang, X.; Yao, X.L.; Hou, J.F.; Yu, Z.T.; Cheng, G.H.; Hu, Y.C.; Cen, K.F. Increased thermal conductivity of eicosane-based composite phase change materials in the presence of graphene nanoplatelets. Energy Fuels 2013, 27, 4041–4047. [Google Scholar] [CrossRef]
- Goli, P.; Legedza, S.; Dhar, A.; Salgado, R.; Renteria, J.; Balandin, A.A. Graphene-enhanced hybrid phase change materials for thermal management of Li-ion batteries. J. Power Sources 2014, 248, 37–43. [Google Scholar] [CrossRef] [Green Version]
- Prolongo, S.G.; Moriche, R.; Jiménez-Suárez, A.; Sánchez, M.; Ureña, A. Epoxy Adhesives Modified with Graphene for Thermal Interface Materials. J. Adhes. 2014, 90, 835–847. [Google Scholar] [CrossRef]
- Zhang, P.; Li, Q.; Xuan, Y. Thermal contact resistance of epoxy composites incorporated with nano-copper particles and the multi-walled carbon nanotubes. Compos. Part A Appl. Sci. Manuf. 2014, 57, 1–7. [Google Scholar] [CrossRef]
- Olowojoba, G.B.; Eslava, S.; Gutierrez, E.S.; Kinloch, A.J.; Mattevi, C.; Rocha, V.G.; Taylor, A.C. In situ thermally reduced graphene oxide/epoxy composites: Thermal and mechanical properties. Appl. Nanosci. 2016, 6, 1015–1022. [Google Scholar] [CrossRef]
- Lian, G.; Tuan, C.C.; Li, L.; Jiao, S.; Wang, Q.; Moon, K.S.; Cui, D.; Wong, C.P. Vertically Aligned and Interconnected Graphene Networks for High Thermal Conductivity of Epoxy Composites with Ultralow Loading. Chem. Mater. 2016, 28, 6096–6104. [Google Scholar] [CrossRef]
- Moriche, R.; Prolongo, S.; Sánchez, M.; Jiménez-Suárez, A.; Chamizo, F.; Ureña, A. Thermal conductivity and lap shear strength of GNP/epoxy nanocomposites adhesives. Int. J. Adhes. Adhes. 2016, 68, 407–410. [Google Scholar] [CrossRef]
- Huang, T.; Zeng, X.; Yao, Y.; Sun, R.; Meng, F.; Xu, J.; Wong, C. Boron nitride@graphene oxide hybrids for epoxy composites with enhanced thermal conductivity. RSC Adv. 2016, 6, 35847–35854. [Google Scholar] [CrossRef]
- Yuan, C.; Xie, B.; Huang, M.; Wu, R.; Luo, X. Thermal conductivity enhancement of platelets aligned composites with volume fraction from 10% to 20%. Int. J. Heat Mass Transf. 2016, 94, 20–28. [Google Scholar] [CrossRef]
- Zhao, Y.H.; Zhang, Y.F.; Wu, Z.K.; Bai, S.L. Synergic enhancement of thermal properties of polymer composites by graphene foam and carbon black. Compos. Part B Eng. 2016, 84, 52–58. [Google Scholar] [CrossRef]
- Dmitriev, A.A.; Dmitriev, A.S.; Makarov, P.; Mikhailova, I. New nanocomposite surfaces and thermal interface materials based on mesoscopic microspheres, polymers and graphene flakes. AIP Conf. Proc. 2018, 1957, 020003. [Google Scholar] [CrossRef]
- Renteria, J.; Legedza, S.; Salgado, R.; Balandin, M.; Ramirez, S.; Saadah, M.; Kargar, F.; Balandin, A. Magnetically-functionalized self-aligning graphene fillers for high-efficiency thermal management applications. Mater. Des. 2015, 88, 214–221. [Google Scholar] [CrossRef] [Green Version]
- Suh, D.; Moon, C.M.; Kim, D.; Baik, S. Ultrahigh Thermal Conductivity of Interface Materials by Silver-Functionalized Carbon Nanotube Phonon Conduits. Adv. Mater. 2016, 28, 7220–7227. [Google Scholar] [CrossRef]
- Dmitriev, A.S.; Valeev, A.R. Graphene nanocomposites as thermal interface materials for cooling energy devices. J. Phys. Conf. Ser. 2017, 891, 012359. [Google Scholar] [CrossRef] [Green Version]
- Mai, P.T.; Bui, T.A.; Van Tran, H.; Van Pham, T.; Nguyen, D.N.; Phan, M.N.; Bui, T.H. Application of Graphene Silicone Grease in heat dissipation for the Intel Core i5 Processor. JOIV Int. J. Informatics Vis. 2019, 3, 222–226. [Google Scholar] [CrossRef] [Green Version]
- Plazek, D.J.; Choy, I.C. The physical properties of bisphenol-a-based epoxy resins during and after curing. II. Creep behavior above and below the glass transition temperature. J. Polym. Sci. Part B Polym. Phys. 1989, 27, 307–324. [Google Scholar] [CrossRef]
- Prasher, R.; Matayabas, J. Thermal contact resistance of cured gel polymeric thermal interface material. IEEE Trans. Compon. Packag. Technol. 2004, 27, 702–709. [Google Scholar] [CrossRef]
- Due, J.; Robinson, A.J. Reliability of thermal interface materials: A review. Appl. Therm. Eng. 2013, 50, 455–463. [Google Scholar] [CrossRef]
- Naghibi, S. Noncuring Graphene Thermal Interface Materials for Advanced Electronics. Ph.D. Thesis, University of California-Riverside, Riverside, CA, USA, 2020. [Google Scholar]
- Nouri-Borujerdi, A.; Kazemi-Ranjbar, S. Thermal and electrical conductivity of a graphene-based hybrid filler epoxy composite. J. Mater. Sci. 2021, 56, 15151–15161. [Google Scholar] [CrossRef]
- Arnold, A. Nickel-chromium-aluminium-copper resistance wire. Proc. IEE Part B Radio Electron. Eng. 1956, 103, 439–447. [Google Scholar] [CrossRef]
- Yao, Y.; Arajs, S.; Anderson, E. Electrical resistivity of nickel-rich nickel-chromium alloys between 4 and 300 K. J. Low Temp. Phys. 1975, 21, 369–376. [Google Scholar] [CrossRef]
- Shahil, K.M.F.; Hossain, M.Z.; Goyal, V.; Balandin, A.A. Micro-Raman spectroscopy of mechanically exfoliated few-quintuple layers of Bi2Te3, Bi2Se3, and Sb2Te3 materials. J. Appl. Phys. 2012, 111, 054305. [Google Scholar] [CrossRef] [Green Version]
- Paton, K.R.; Varrla, E.; Backes, C.; Smith, R.J.; Khan, U.; O’Neill, A.; Boland, C.; Lotya, M.; Istrate, O.M.; King, P.; et al. Scalable production of large quantities of defect-free few-layer graphene by shear exfoliation in liquids. Nat. Mater. 2014, 13, 624–630. [Google Scholar] [CrossRef] [PubMed]
- Henning, C.; Parker, R. Transient response of an intrinsic thermocouple. J. Heat Transf. 1967, 89, 146–152. [Google Scholar] [CrossRef]
- Farahmand, K.; Kaufman, J.W. Experimental measurement of fine thermocouple response time in air. Exp. Heat Transf. 2001, 14, 107–118. [Google Scholar] [CrossRef]
- Yang, L.; Dick, R.P.; Memik, G.; Dinda, P. HAPPE: Human and Application-Driven Frequency Scaling for Processor Power Efficiency. IEEE Trans. Mob. Comput. 2013, 12, 1546–1557. [Google Scholar] [CrossRef] [Green Version]
- Podzimek, A.; Bulej, L.; Chen, L.Y.; Binder, W.; Tuma, P. Analyzing the Impact of CPU Pinning and Partial CPU Loads on Performance and Energy Efficiency. In Proceedings of the 2015 15th IEEE/ACM International Symposium on Cluster, Cloud and Grid Computing, Shenzhen, China, 4–7 May 2015; pp. 1–10. [Google Scholar] [CrossRef]
- Travers, M. Cpu Power Consumption Experiments and Results Analysis of Intel i7-4820k; Technical Report; Newcastle University: Newcastle upon Tyne, UK, 2015. [Google Scholar]
- Rumi, M.A.; Hasibul Hasan, D.M. CPU power consumption reduction in android smartphone. In Proceedings of the 2015 3rd International Conference on Green Energy and Technology (ICGET), Dhaka, Bangladesh, 11 September 2015; pp. 1–6. [Google Scholar] [CrossRef]
- Zajac, P.; Janicki, M.; Szermer, M.; Napieralski, A. Evaluating the impact of scaling on temperature in FinFET-technology multicore processors. Microelectron. J. 2014, 45, 1806–1813. [Google Scholar] [CrossRef]
- Tang, B.; Hu, G.; Gao, H.; Hai, L. Application of graphene as filler to improve thermal transport property of epoxy resin for thermal interface materials. Int. J. Heat Mass Transf. 2015, 85, 420–429. [Google Scholar] [CrossRef]
- Zhang, Y.; Zhang, M. Behavior of a graphene/epoxy composite used as thermal interface material for LED heat dissipation. Mater. Test. 2017, 59, 1037–1042. [Google Scholar] [CrossRef]
- Shenogina, N.; Shenogin, S.; Xue, L.; Keblinski, P. On the lack of thermal percolation in carbon nanotube composites. Appl. Phys. Lett. 2005, 87, 133106. [Google Scholar] [CrossRef] [Green Version]
- Bonnet, P.; Sireude, D.; Garnier, B.; Chauvet, O. Thermal properties and percolation in carbon nanotube-polymer composites. Appl. Phys. Lett. 2007, 91, 201910. [Google Scholar] [CrossRef]
- Zheng, R.; Gao, J.; Wang, J.; Feng, S.P.; Ohtani, H.; Wang, J.; Chen, G. Thermal percolation in stable graphite suspensions. Nano Lett. 2012, 12, 188–192. [Google Scholar] [CrossRef] [PubMed]
- Gu, J.; Xie, C.; Li, H.; Dang, J.; Geng, W.; Zhang, Q. Thermal percolation behavior of graphene nanoplatelets/polyphenylene sulfide thermal conductivity composites. Polym. Compos. 2014, 35, 1087–1092. [Google Scholar] [CrossRef]
- Shtein, M.; Nadiv, R.; Buzaglo, M.; Kahil, K.; Regev, O. Thermally conductive graphene-polymer composites: Size, percolation, and synergy effects. Chem. Mater. 2015, 27, 2100–2106. [Google Scholar] [CrossRef]
- Lewis, J.S.; Perrier, T.; Mohammadzadeh, A.; Kargar, F.; Balandin, A.A. Power Cycling and Reliability Testing of Epoxy-Based Graphene Thermal Interface Materials. C J. Carbon Res. 2020, 6, 26. [Google Scholar] [CrossRef]
- Sudhindra, S.; Kargar, F.; Balandin, A.A. Noncured Graphene Thermal Interface Materials for High-Power Electronics: Minimizing the Thermal Contact Resistance. Nanomaterials 2021, 11, 1699. [Google Scholar] [CrossRef] [PubMed]
| Measurement Position | Condition |
|---|---|
| 1–4 previous | , or , or , or |
| 5 previous | |
| 10 previous | |
| 20 previous | |
| 30 previous |
| TIM Load Level | Least Squares Fit | |
|---|---|---|
| 0 vol.% | 0.9996 | |
| 3.6 vol.% | 0.9998 | |
| 7.3 vol.% | 0.9995 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lewis, J.S. Reduction of Device Operating Temperatures with Graphene-Filled Thermal Interface Materials. C 2021, 7, 53. https://doi.org/10.3390/c7030053
Lewis JS. Reduction of Device Operating Temperatures with Graphene-Filled Thermal Interface Materials. C. 2021; 7(3):53. https://doi.org/10.3390/c7030053
Chicago/Turabian StyleLewis, Jacob S. 2021. "Reduction of Device Operating Temperatures with Graphene-Filled Thermal Interface Materials" C 7, no. 3: 53. https://doi.org/10.3390/c7030053
APA StyleLewis, J. S. (2021). Reduction of Device Operating Temperatures with Graphene-Filled Thermal Interface Materials. C, 7(3), 53. https://doi.org/10.3390/c7030053