1. Introduction
The behavior of the wake flow trailing a slender vehicle at a steep angle of attack bears a strong resemblance to the flow behind a circular cylinder oriented transversely, which undergoes Karman vortex shedding [
1]. A study of the bluff-body wake is important in flow control especially with a body at a high angle of attack. Besides, most objects facing the flow are bluff in the front so the wind or stream is severely disturbed to produce unsteady forces and moments acting on them. Common examples of a flow passing over a fore-body include the tapered wing of an aircraft, a high-rise building, or even chimneys. Typically, these entities are limited in length and open-ended in the free stream. The associated flow field is of a three-dimensional nature. In practical scenarios, the cross-sectional area of these entities may exhibit variations in the spanwise direction.
Gaster [
2] initially conducted a comprehensive study on the vortex-shedding patterns in the wakes behind slender cones and tapered cylinders. The wake’s velocity fluctuations are amplitude-modulated, with the modulation frequency maintaining near constancy across the spanwise direction. Gaster further demonstrated that the vortex-shedding frequency’s spanwise distribution exhibits a cellular pattern, with velocity fluctuations undergoing modulation at the juncture of adjacent cells [
3]. The unique circumstance where vortices are not aligned between neighboring cells is referred to as “vortex splitting” by Eisenlohr and Eckelmann [
4]. Piccirillo and Van Atta [
5] found that vortex splitting began with a kink in vortex lines and suggested that the mechanism leading to vortex splitting might involve a spanwise pressure gradient. Besides, a two-dimensional (2-D) circular cylinder can generate a cellular frequency distribution resulting from end effects or the non-uniformity of oncoming flow [
4,
6,
7]. Hsiao and Chiang [
8] experimentally found two to three constant-frequency vortex cells in the shedding vortices generated along the spanwise direction of tapered circular cylinders at Reynolds numbers over 10,000 with hot-wire anemometry. In the experiment on a trapezoidal cylinder with an aspect ratio of 4.7, Miau et al. [
9] concluded that the low-frequency fluctuations observed in the near-wake region exhibit a global characteristic, which could be linked to the spanwise motions in the separated shear layer. Peng et al. [
10] conducted an experimental study concerning vortex shedding from a circular cylinder with a slit normal to the stream. They found that the slit in the circular cylinder does weaken the coherence of the shedding vortex. Visscher et al. [
11] applied particle image velocimetry to provide more spatial information than point-based measurements and offer insight into Reynolds numbers of 1250, 2500, and 5000. According to their observations, the shape and position of the vortex-formation region were found to be in accordance with findings from uniform cylinders, while the local Strouhal numbers (St) show some fundamental variation due to the locally varying diameter. Seyed-Aghazadeh et al. [
12] conducted a series of experiments in a re-circulating water tunnel with an open surface test section for a Reynolds number range of 370–2300. Hybrid shedding modes were observed for cases with velocities up to the value corresponding to the peak amplitude of oscillations.
One of the questions that arises with the cellular distribution of vortex-shedding frequencies is how the vortices link between cells with different frequencies. The vortex lines are aligned through the whole span if the shedding frequency is uniformly distributed, e.g., the vortices in the wake behind a 2-D circular cylinder. However, the distribution of shedding frequencies is cellular in the wake behind a tapered cylinder, and the numbers of shedding vortices generated in different cells are not the same at a certain period of time. Thus, it is impossible to always align the vortex lines throughout the entire span. Clearly, the flow structures in the intersection region of the neighboring cells require more exploration of vortex splitting.
Numerical simulations are becoming increasingly popular in engineering across various industries. Computational fluid dynamic (CFD) analyses are widely employed to predict flow fields for engineering design and to overcome experimental limitations. In this study, the Semi-Implicit Method for Pressure Linked Equations (SIMPLE) algorithm, developed by Patankar for solving incompressible flow, was utilized [
13,
14]. Besides, to generate a turbulent wake for vortex-shedding simulations, turbulent flow was modeled numerically with a high Reynolds number. Computations for this transient problem can be traced back to Jespersen and Levit [
15]. They computed low Reynolds number flow (local Re < 137.23) past a tapered cylinder in a wind tunnel. VallÈS et al. solved full unsteady three-dimensional Navier–Stokes equations numerically to explore the vortex-shedding phenomena along the span of the cylinder [
16]. In their research, the Reynolds number based on the velocity of the incoming flow was in the range from 130 to 180. However, the entire flow fields prevailing in such low Reynolds numbers are laminar flows. A turbulent wake in the high-Reynolds-number regions is common in real engineering problems. It corresponds to a Reynolds number greater than 300 or so. Parnaudeau et al. [
17] performed three-dimensional direct numerical simulations of vortex shedding behind cylinders when the body diameter and the incoming flow involved spanwise linear non-uniformity. Their computations highlighted distinct vortical features between the shear case and the tapered case. Both cases yielded similarities in flow topology. In our previous work [
18], flow fields of a vortex flowmeter with various inflow conditions were simulated numerically via large eddy simulation (LES). The results show that the predominant frequency patterns are altered due to various inlet velocity profiles with the constant-shape vortex generator in a spanwise direction; however, no multiple cells were observed.
The objective of this paper is to explore the characteristics of cellular vortical structures behind a tapered cylinder with a Reynolds number of 4000. This paper studied vortex line structure unalignment or “vortex splitting” via numerical flow visualization techniques. The CFD code of ANSYS FLUENT was used to solve the turbulent flow modeled via LES with a tapered cylinder installed in a wind tunnel. The Strouhal numbers obtained based on the numerical results were validated using experimental data, and then, the numerical methods were further used to analyze the transient phenomena of the vortex splitting mechanism. This study validates the numerical results via experimental data and analyzes the transient phenomena of the flow field. The governing equations and boundary and initial conditions are described in
Section 2.
Section 2 outlines the governing equations along with the boundary and initial conditions.
Section 3 details the numerical methods used for the simulation, including grid-independent tests for verification and experimental validation. The results and discussions are presented in
Section 4, while
Section 5 provides a summary of the conclusions.
2. Physical Model and Governing Equations
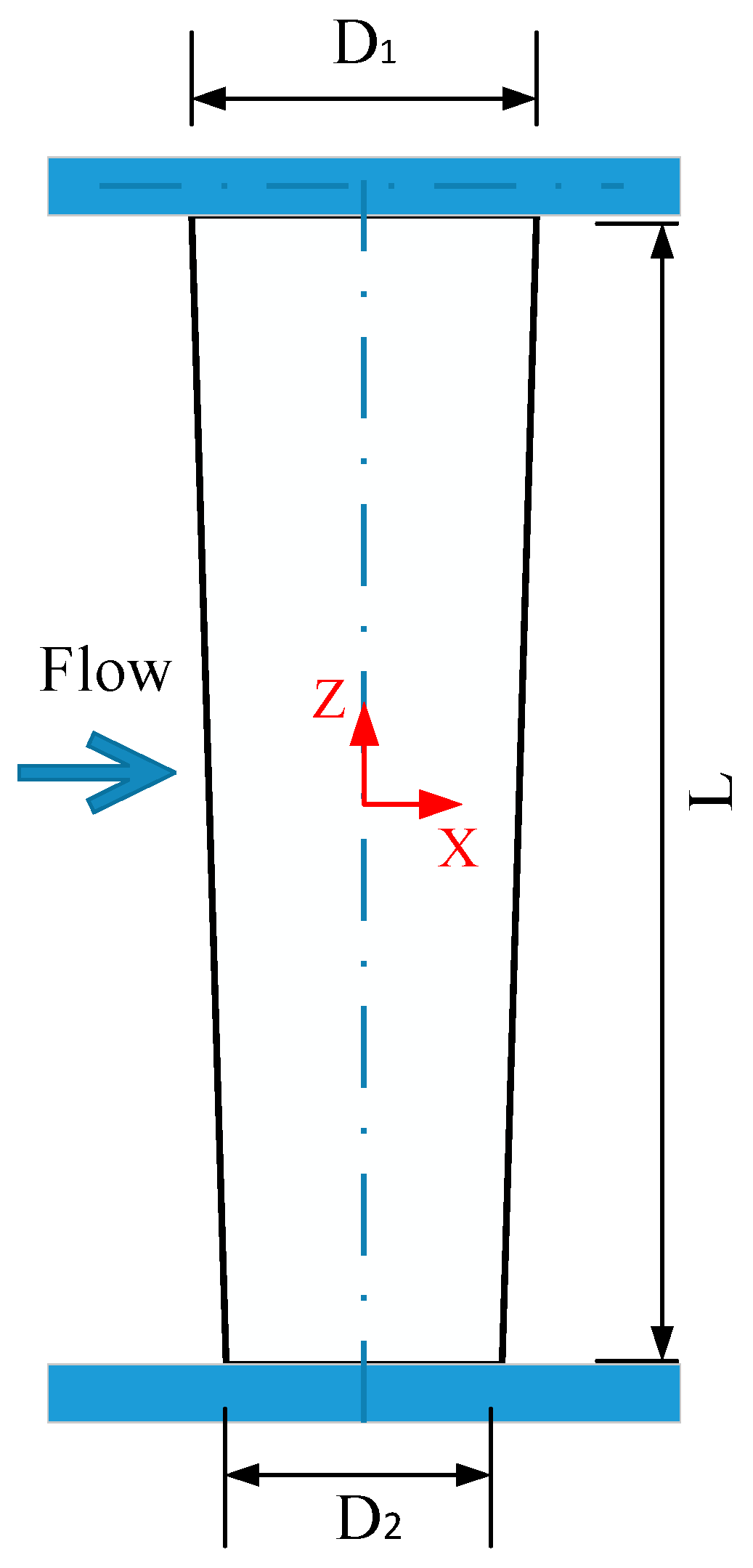
The primary distinction between the wakes behind tapered and 2-D circular cylinders lies in the spanwise alterations in vortex-shedding frequencies. In this context, the distribution of the vortex-shedding frequency across the span was examined to accurately characterize the cellular vortex-shedding phenomenon. The experiments for comparisons were conducted in a low-speed suction-type wind tunnel as shown in Figure 3 of Ref. [
8]. Additionally, a block diagram of the measuring and data acquisition system is illustrated in Figure 5 of Ref. [
8]. The cross-section of the test section is 0.2 m wide and 0.4 m high with air as the working fluid. The taper ratio based on Gaster [
2] and Noack et al. [
19] is defined by
and
, where L is the span length of the tapered cylinder and
is the mean diameter with D
1 and D
2 as the maximum and minimum diameters, respectively. The inlet Reynolds number is 4000 based on
with a turbulence intensity less than 0.5%. The Cartesian coordinate system shown in
Figure 1 was used throughout the numerical simulations and experimental validation.
The CFD simulation was simplified to model a transient, incompressible, turbulent flow with no heat generation. Non-slip boundary conditions were applied to the walls of both the duct and the tapered cylinder. The transient conservation equation for transport of a scalar quantity ϕ is written in integral form for an arbitrary control volume V as follows [
20]:
Here, ρ indicates density; is the velocity vector; is the surface area vector; V is volume; Γ is the diffusion coefficient for ϕ, and Sϕ is the source term of ϕ per unit volume. The symbol ϕ can be replaced by 1 for the continuity equation, and u, v and w are the momentum equations in the X, Y and Z directions, respectively.
The Reynolds number is 4000 in the wind tunnel in which the boundary layer on the fore surface of the cylinder is laminar, but the wake is turbulent [
8]. Since the fluid used in the present simulations was air, the density was assumed to be constant in the flow field for air speeds far less than a 0.3 Mach number. We also calculated turbulent flows via LES, which is one of the trustworthy computational tools for studying turbulent flow fields [
21,
22,
23]. Equation (1) can be reformulated in terms of generalized coordinates by employing the finite volume method for numerical simulation.
The discretized formulation for each cell (control volume) based on Equation (1) yields
where f denotes the face of the control volume and
is the face area; N
f is the number of faces enclosing cell;
is the velocity of
flux; and V is the volume of the control volume. After combining boundary conditions, Equation (2) can be linearized and expressed in the computational domain as follows:
where the subscript nb refers to neighboring cells; a
p and a
nb are the linearized coefficients for ϕ and ϕ
nb; and b is the source term. The number of neighbors for each cell depends on the grid topology but will typically equal the number of faces enclosing the cell. Similar equations can be written for each cell in the grid, and this results in a set of algebraic equations to be solved using the SIMPLE algorithm [
13].
In experimental measurements [
8], the major device needed for velocity fluctuations was the constant-temperature hot-wire anemometer. This method can identify the dominant vortex-shedding frequencies by detecting velocity fluctuations in the flow field. However, our numerical approach focused on capturing the waveform of the total lift coefficient (C
l = F/(0.5ρv
2A); F is lift) by directly integrating the surface of the tapered cylinder over a time period. The power spectral density of the lift-coefficient fluctuation can be calculated using a fast Fourier transform (FFT) method [
24], which allows for the identification of the dominant frequency. The Strouhal number is defined as
, where
f is the predominant vortex-shedding frequency.
3. Numerical Methodology
To investigate the structure of vortex splitting, the signal generated by the tapered cylinder was numerically analyzed to obtain frequency characteristics using pre- and post-CFD techniques. Calculations were performed with ANSYS FLUENT ver. 18 with a desktop computer and a workstation. A standard case tested in the computer with a CPU 7–3960X@3.30 GHz and 4 × 8 GB DDR3-1333 took three weeks; a workstation with 2 × Intel Xeon E5-2620v4 8cores 2.1 GHz and 4 × 32 GB DDR4-2133 ECC RDIMM (Intel, Santa Clara, CA, USA) took three days using the parallel computations by sharing memory allocations on the local machine. Nevertheless, the workstation hardware still requires approximately 3 days for one case, even in single-precision mode, because simulating transient turbulent flow is very time-consuming.
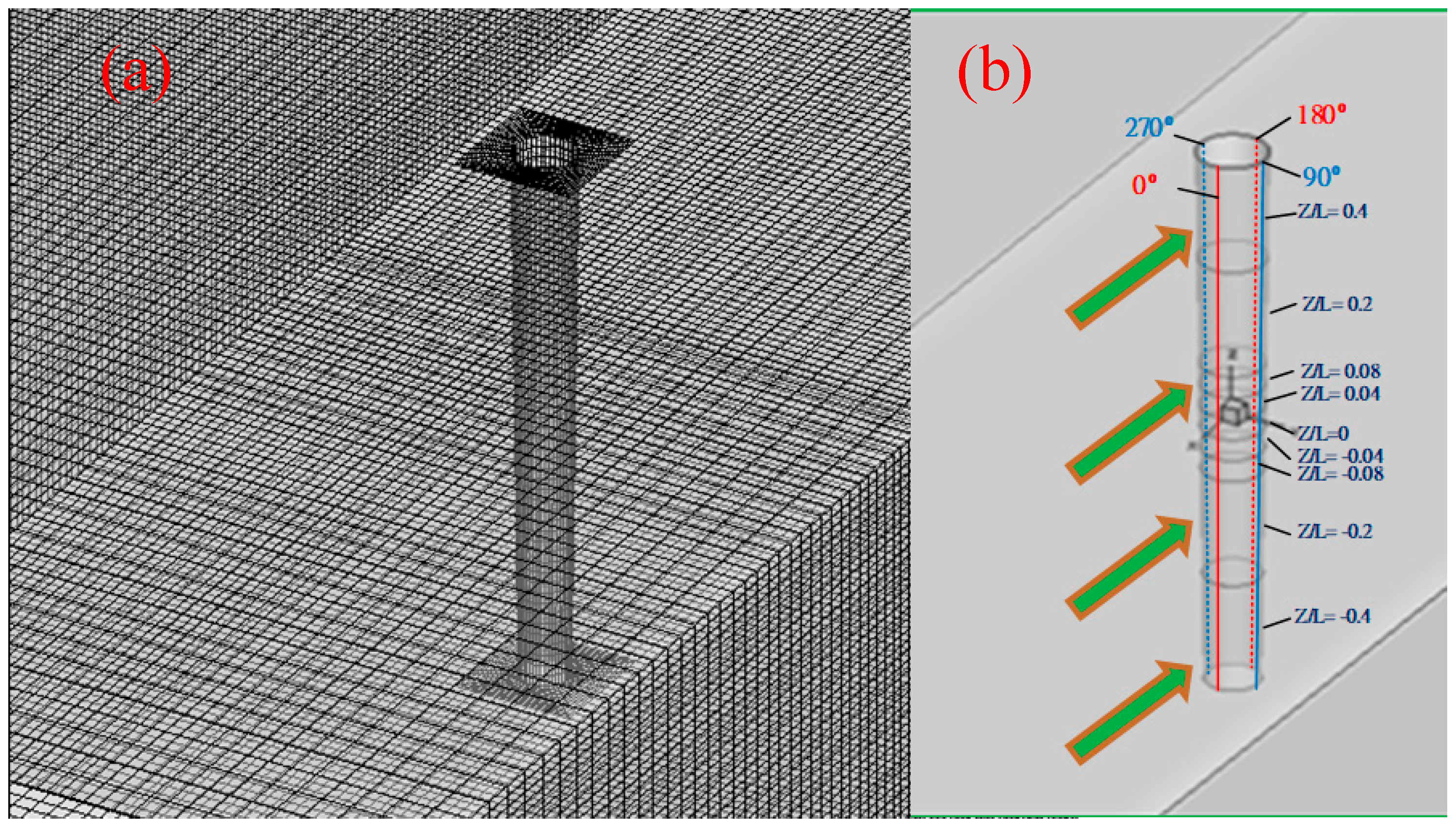
A mesh system corresponding to the experimental setup in
Figure 1 was constructed as shown in
Figure 2. The tapered cylinder is 0.2 m long with an upper-end diameter D
1 = 22.575 mm, lower-end diameter D
2 = 17.6 mm, average diameter
= 20.0875 mm, and R
T = 40.2, according to a previous definition.
To establish appropriate boundary conditions, both upstream and downstream in the simulations, the computational domain was extended 0.45 m in front of and behind the cylinder.
Figure 2b presents a Cartesian coordinate system used for the analysis, with the origin positioned at the center of the tapered cylinder. The
X-axis is the direction of the axial flow along the wind tunnel, the
Z-axis is the spanwise direction of the cylinder, and the
Y-axis is the transverse direction with respect to the cylinder. We used 9 specific zones at Z/L = ±0.4, ±0.2, ±0.08, ±0.04 and 0, as shown in
Figure 2b, to numerically obtain the dynamic response, i.e., transient pressure, or lift coefficients, caused by vortex shedding of the tapered cylinder.
The inlet flow was assumed to be a plug flow with a uniform velocity of U = 3.10066 m/s, which is equivalent to a Reynolds number of 4000 in the wind tunnel. The Reynolds number is defined as , where U is the inlet velocity and μ is the dynamic viscosity of the fluid. Dry air at a temperature of 25 °C and pressure were adopted in the simulations. A zero-order extrapolation was applied at the outflow boundary condition for the relevant flow properties. A non-slip condition was imposed on all surfaces, including the inner wall of the wind tunnel and the tapered cylinder. The following numerical techniques were employed to ensure stable and accurate solutions.
(i) When ANSYS FLUENT employs an implicit formulation to solve equations that are time-dependent, it may require multiple iterations at each time step. Users are required to define a maximum limit for the number of iterations per time step. If the solution meets the convergence criteria before reaching this iteration limit, it will proceed to the next time step. It is crucial to carefully determine the maximum iterations per time step to prevent the propagation of errors. A globally-scaled residual of was adopted to check the absolute convergence criteria at each time step.
(ii) The standard k-epsilon turbulence model was initially employed to approximate the Reynolds stresses without considering time evolution, as vortices generated from a 2-D circular cylinder become turbulent when the Reynolds number exceeds 300 [
25,
26]. The data were then imported to the solver serving as the initial conditions in transient calculations using LES—this has great potential for calculating these complex flows [
23,
27,
28,
29]. According to the literature, the Smagorinsky–Lilly model in LES was used for the subgrid model, and the Smagorinsky constant was set to be 0.1 (default value by ANSYS FLUENT). At the inlet boundary, the turbulence intensity was set to be 0.4% based on the experimental data (<0.5%). This calculation procedure was found to yield rather stable calculations for this time-marching problem.
(iii) The FFT algorithm was used to acquire the predominant frequency of vortex shedding based on the time history of the induced lift. Our understanding is that the use of the standard FFT to analyze the spectra of a data string containing a non-sinusoidal component results in spectra with an envelope that decays exponentially. Data for the low frequency part are highly contaminated. On the other hand, the non-periodic condition often yields an adverse effect of frequency contamination. Therefore, an iterative filter proposed by Jeng et al. [
24] was adopted to remove the non-sinusoidal trend and reduce the error induced by the non-periodic conditions.
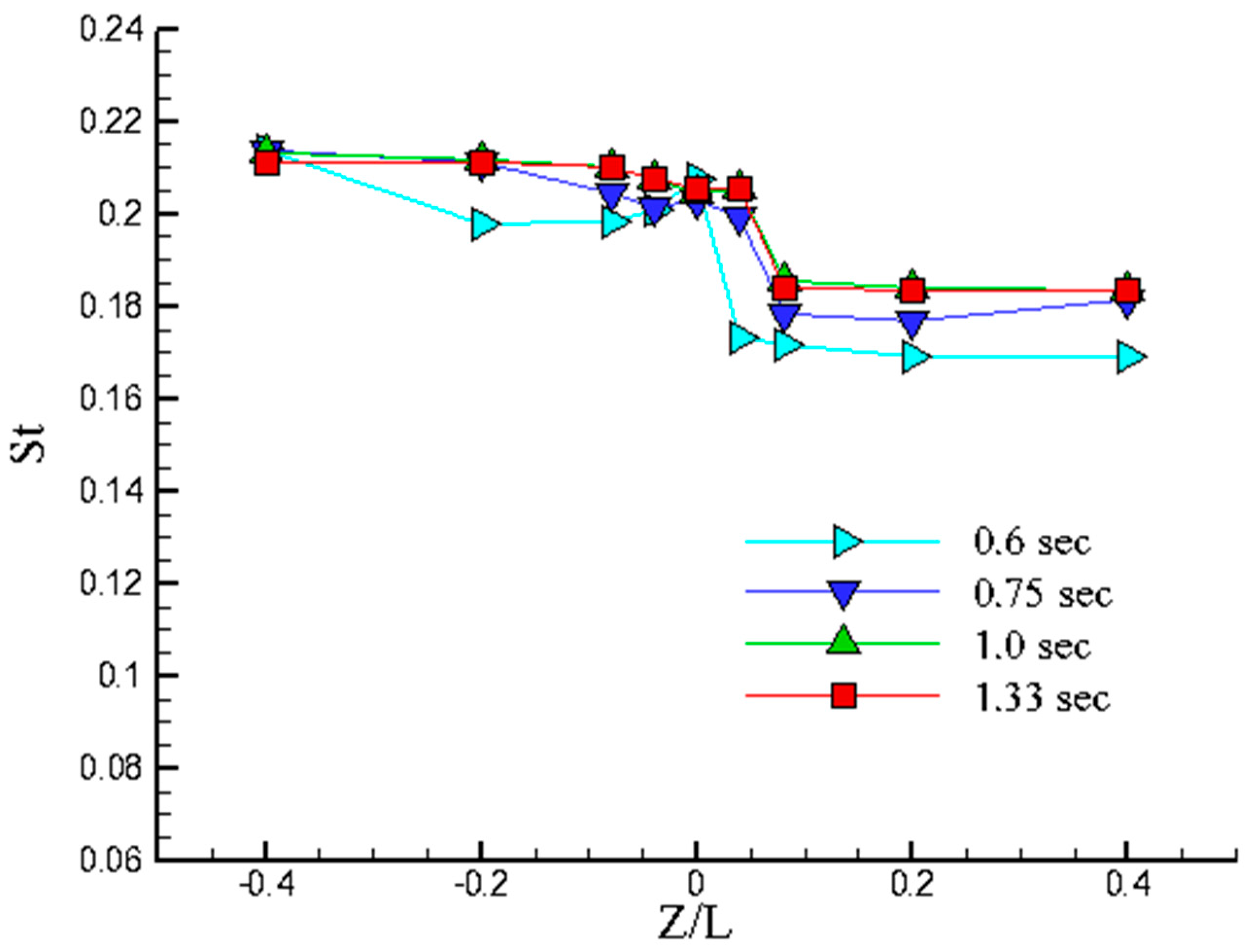
(iv) For the computation, the time steps need to be adequately minuscule to ensure a satisfactory resolution in the time domain and precise identification of the dominant frequency using the FFT algorithm. In this case, the time step is established at 0.0001 s, and the scale of the time step in relation to the small-eddy time scale is estimated to be roughly two orders of magnitude. In
Figure 3, the numerical experiments show that distributions of St along the tapered cylinder proceed to converge after one second. Therefore, the final data—in which calculations ceased at an elapsed time of 1.33 s—are used in the following analysis. The Cl plot of 13,300 points was analyzed using FFT to obtain frequency spectra for the case studies.
Figure 4 presents the implicit time integration method and compares it with experimental results, using a time step of 0.0001 s. The typical vortex shedding frequency in these cases is approximately 30 Hz, corresponding to a time period of about 0.0333 s. As a result, 333 calculation steps were used to resolve a single complete cycle of vortex shedding.
(v) Dominant frequencies of convergent solutions are sensitive to sources of potential disturbance, such as minor variations from the grid number, grid distribution, initial conditions, boundary conditions, convergent criteria, etc. [
30]. In practice, not only the quantity of the mesh is related to the accuracy of the solution in this research, but also factors that are unavoidable in the experiment, such as the inlet turbulence level, cylinder surface, acoustic noise, and the boundary of the cylinder end, are all sensitive to the phenomenon of vortex shedding [
28,
29]. Though an exact agreement between the numerical and experimental data most likely cannot be expected in this study, it does not seem to be in conflict with interpreting the vortex-splitting mechanism from a qualitative viewpoint. To effectively compute the present flow fields, the flow domain was divided into smaller subdomains. Mixed grid types were used to fit the subdomain around the tapered cylinder. Subsequently, structured hexahedral grids were utilized to create the mesh encircling the vortex generator, which is represented by the tapered cylinder. Furthermore, finer grids were implemented along the boundary wall to accurately capture the turbulent boundary layer, and the grid scale in relation to the viscous scale was assessed prior to the calculations.
Figure 4 shows calculations to check for grid independence and comparisons between the numerical and experimental data. The dominant frequency was obtained by performing FFT for the evolution of C
l over the elapsed time, and St was obtained based on the definition of the Strouhal number. Though the St distribution of simulated results for the case with 1.7 million grids appears favorable versus experiments, that computation took too long to achieve the required convergence. In general, the calculations in
Figure 4 show that 0.63 million grids are suitable for this study. Besides, the mesh with 10 uniform zones along the cylinder does not capture the Strouhal-number-jump at the right location, but the mesh with 9 non-uniform zones in
Figure 2b does. Thereafter, the 0.63 million grids with 9 non-uniform zones along the tapered cylinder were adopted in the simulations and analysis.
Generally, the numerical and the experimental values are consistent for the case of Re = 4000 in
Figure 4. Strouhal numbers at the narrow end of the cylinder (Z/L < 0) by CFD are a little higher than experiments, and the splitting locations show only little discrepancy for both the CFD and experiments around 0 < Z/L < 0.05. These minor deviations in the lower portion of the tapered cylinder might result from the combined effects of the specified numerical boundary and initial conditions of the tunnel wall [
31], as well as numerical errors, including the mesh quality and accumulated errors in transient calculations [
30].
4. Results and Discussion
Time-marching calculations were adopted to study this transient problem. After reaching a stable oscillation of the lift coefficient (C
l), 20 more time-step calculations were executed for further analysis. This took 20 × 0.0001 = 0.002 s for every output. A total of 20 outputs (T1–T20) were recorded. Therefore, the total elapsed time is 0.04 s. The nine zones of the tapered cylinder in
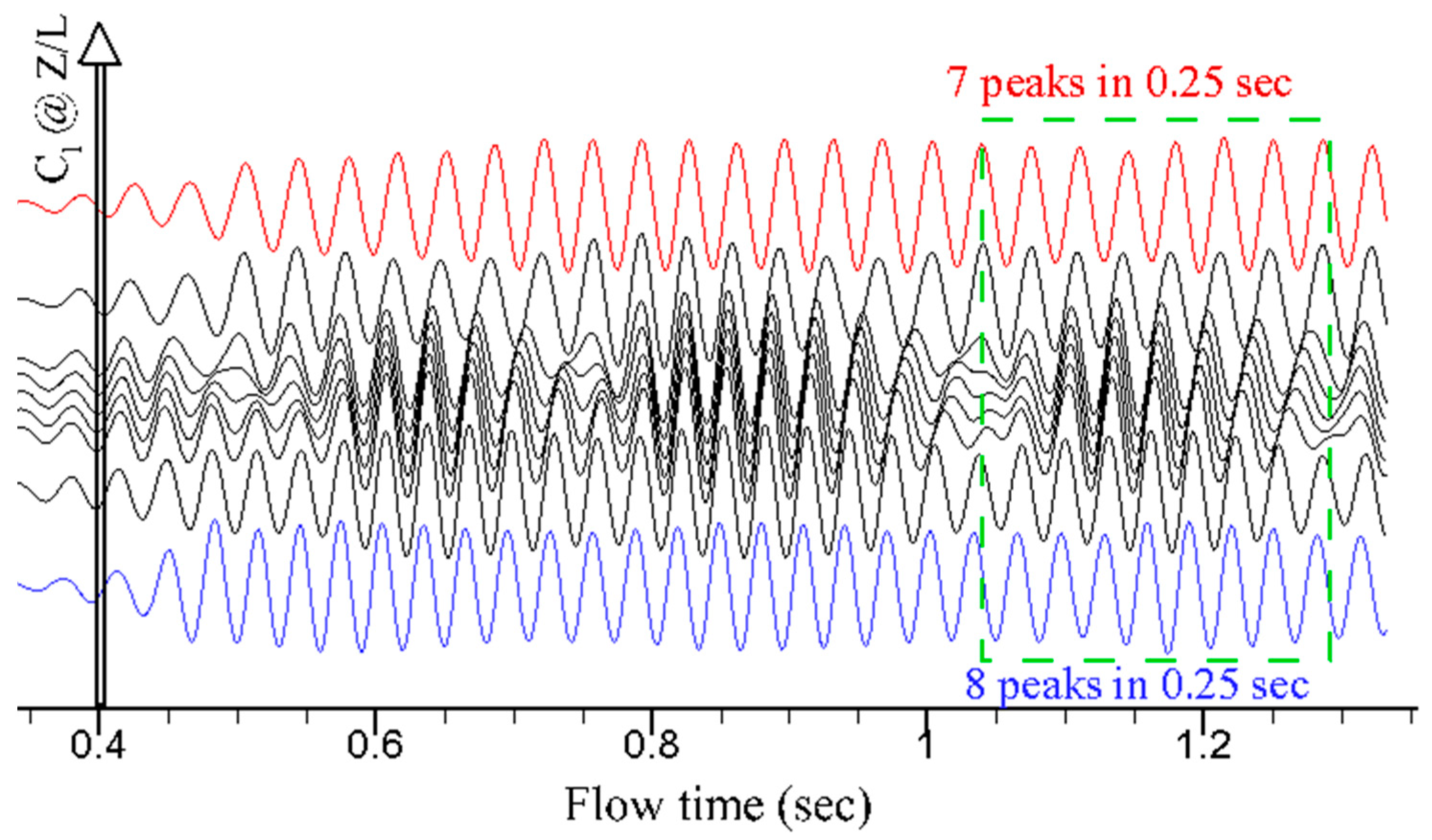
Figure 2b were selected to acquire the time traces for the lift coefficients in
Figure 5, in which the amplitudes of the C
l fluctuations are all normalized to be one. This enhances the images of the transition of vortex splitting. The C
l oscillations at all zones are similar and stabilize after 0.6 s, while phase lags exist around the middle zones. The higher peaks in the trace, at each location of Z/L = −0.08, −0.04, 0, 0.04 and 0.08, determine the period of primary oscillation. The relationship between vortex numbers of the higher-frequency cell and the lower-frequency cell at the vortex splitting can be spotlighted in the dashed rectangle as designated in
Figure 5. There are approximately 7 peaks in the 0.25 s period in the lower-frequency cell (near the wide end) and 8 peaks in the higher-frequency cell (near the narrow end) of the tapered cylinder. Therefore, there are 28 peaks for one second in the lower-frequency cell and 32 peaks in the higher-frequency cell of the tapered cylinder. In other words, the mismatching of numbers of vortices between cells in different shedding frequencies is compensated for by vortex splitting during vortex shedding (greater than 0.6 s in
Figure 5).
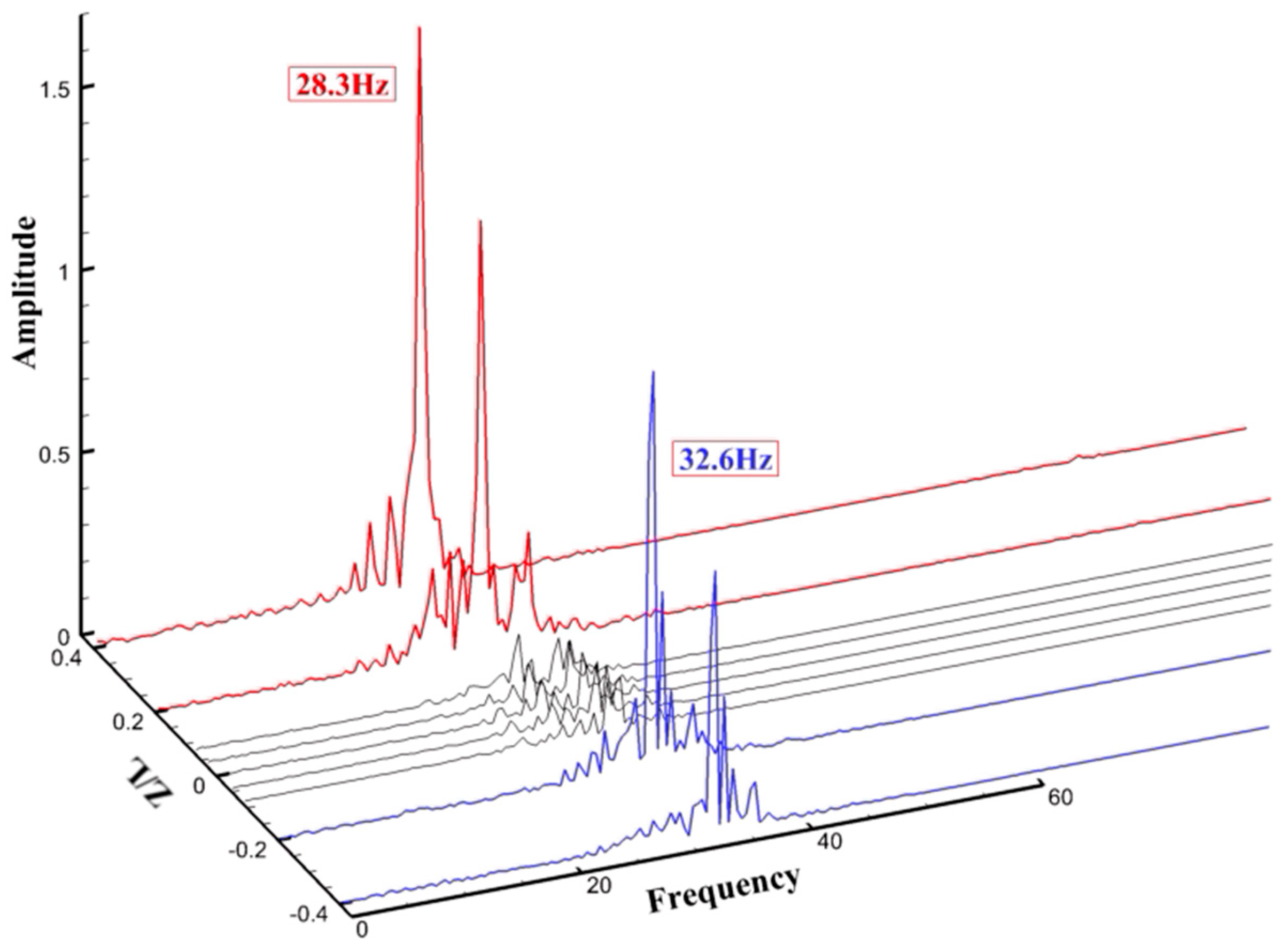
The time traces in
Figure 5 can be expressed as the frequency power spectra via the FFT technique. The power spectra of
Figure 6 have two global maxima, one of which is located at Z/L > 0 with a frequency of 28.3 (28 peaks per second in
Figure 5), and the other is located at Z/L < 0 with a frequency of 32.6 (32 peaks per second in
Figure 5). Between these maxima, the spectra are modulated and have phase jumps. The phenomena—including discrete spectra, nonlinear beat and phase jumps in the spectra—seem to be universal for vortex shedding behind bluff bodies with a spanwise geometric inhomogeneity in the cylinder.
A simplified model proposed by Hsiao and Chiang [
8] can explain the synchronous appearance of the vortex splitting and the decreasing amplitude of the C
l fluctuations. The frequency of vortex splitting is equal to the difference between the dominant frequencies of the adjacent cells. The present simulated results support this model. The occurrence of vortex splitting can be formulated as follows:
Here, we have f1 = 28.3, f2 = 32.6, and Tint is calculated to be 0.233 s.
In this simulation, the period is 0.0353 s in the lower-frequency cell (
f1 = 28.3), and the period is 0.0307 s in the higher-frequency cell (
f2 = 32.6). If we count the period of occurrence of vortex splitting, then, there are seven peaks for T = 0.0353 s per cycle or 0.247 s total. There are eight peaks for T = 0.0307 s per cycle or 0.246 s total. As stated previously, 0.25 s was expected to be the T
int in
Figure 5, and it is close to the 0.233 s predicted by the proposed model [
8].
One of the questions that comes with the cellular distribution of vortex-shedding frequencies is how the vortices link between cells with different frequencies. If the shedding frequency is evenly distributed, encompassing the vortices in the wake trailing a 2-D cylinder, the vortex lines maintain alignment across the full span. However, the distribution of shedding frequencies is cellular in the wake behind tapered cylinders, and the number of shedding vortices generated in different cells is not equal over the same duration of time. In addition, the three-dimensional (3-D) effect of cellular vortical structures might accelerate the mixture of momentum and the transition of the wakes making the situation fully turbulent. The vortex line is then impossible to be always aligned throughout the entire span. Numerical flow visualization in
Figure 7 displays oblique vortex shedding in terms of the normalized vorticity magnitude at a certain time step. Just behind the tapered cylinder, the red lines of the vortex core appear distinct except for the middle zone where vortex splitting occurs. Thus, splitting is accompanied by decreases in amplitude, and this is reasonable because the vortices in different cells are out of phase at vortex splitting.
According to
Figure 7, the axial vortices dominate the wake flow leading to fluctuations in pressure. Furthermore, the vortices might be more inclined before and after vortex splitting; the spacing between vortex lines extends in the lower-frequency side and reduces in the higher-frequency side.
Figure 8 presents the vortex lines of vortex shedding in terms of pressure contours at a certain time step. The gradual transition from two peaks to three occupies the middle zone where no obvious vortex lines can be observed. Besides, the flow visualization showed that the formation of the cellular vortical structure and the roll-up of the shedding vortices occur right behind the tapered cylinder. That means the formation of the vortical structure is closely related to boundary-layer separation on the surface of the tapered cylinder.
The separation of the boundary layer and instability of the wake flow is a major reason for the formation of the vortex street [
19]; thus, it is inherently important in the formation of cellular vortical structures. In
Figure 8, the inclination angle is measured to be 21.2 degrees after 6 mean diameters of the tapered cylinder. The invariance of the inclination angle of vortex lines in the downstream indicates the formation of vortical structures right behind the cylinder. Inside the vortex-splitting region, the vortex line inclination, phase difference, instantaneous frequency and vortex spacing change suddenly when vortex splitting occurs.
The shedding frequency determined by 2-D instability in the wake behind a tapered cylinder is not the same at different spanwise locations. The variation in diameter along the span is the reason for this. Consequently, the fluctuations in velocity and pressure at a single spanwise location introduce further disturbances at other locations. The shedding frequency that emerges is a result of both the local 2-D instability and the 3-D impact caused by disturbances spreading from other spanwise positions. If the supplementary disturbances grow and surpass the initially most unstable waves, it will diverge from its inherent value [
18]. The resulting vortex-shedding frequency depends on the intensity, frequency and emitting position of the additional excitation. Similarly, the interaction or the spanwise coupling between the local stability waves and the perturbations from the tapered cylinder results in the cellular distribution of shedding frequencies.
The fluctuations of pressure dominate the wake flow and cause axial vortices. The vortex formation, inclination and downstream movement are affected by the disturbance transported by the momentum changes, e.g., the pressure gradient [
5]. To study possible causes of oblique vortex-shedding formation behind the tapered cylinder, spanwise lines at the perimeter of the tapered cylinder were specified as @0° (cylinder’s front), @90° (cylinder’s right-hand side), @180° (cylinder’s back) and @270° (cylinder’s left-hand side) in
Figure 2b (counter-clockwise and downstream-facing view).
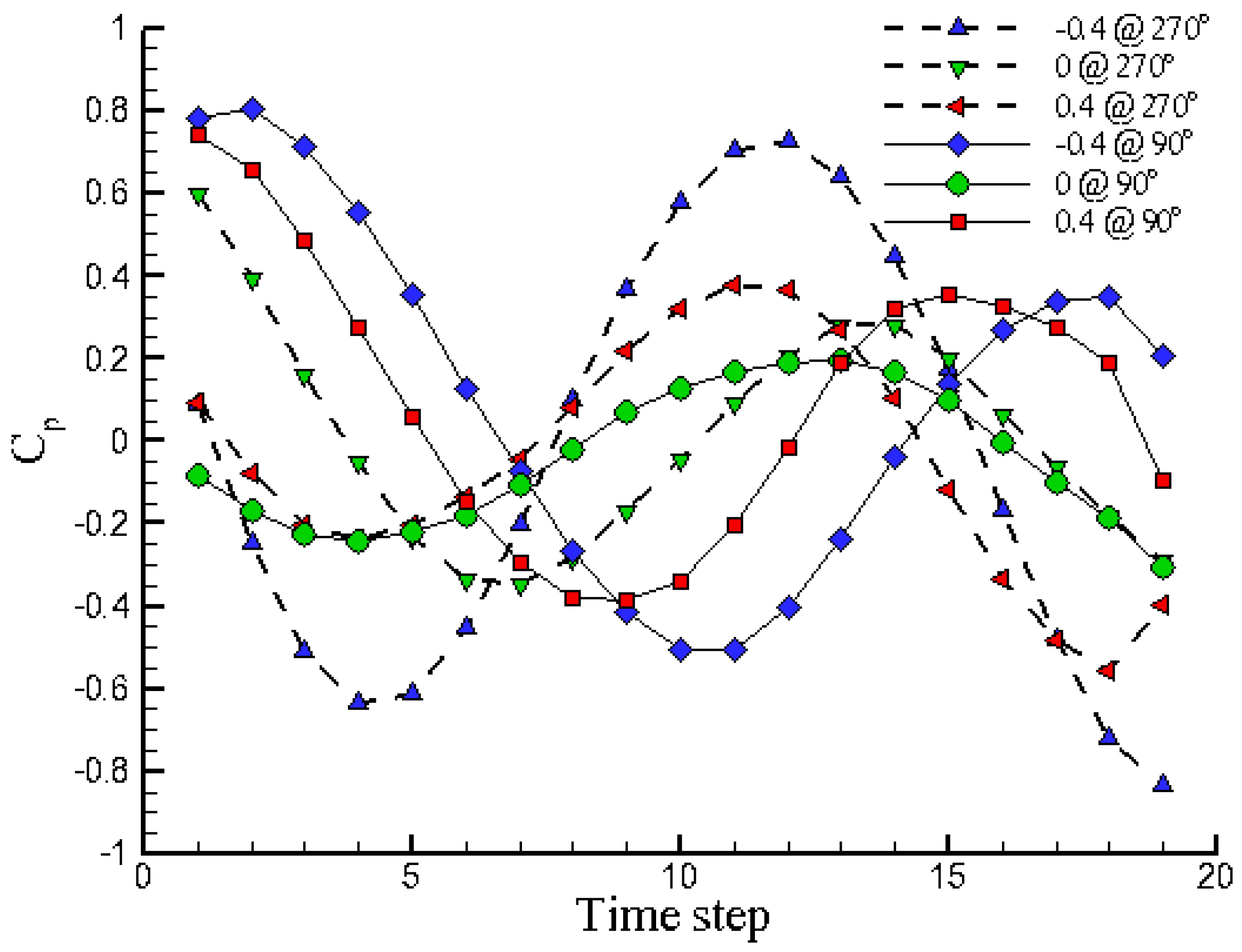
Figure 9 shows the time traces of pressure coefficients (Cp) on Z/L = 0, ±0.4 zones at positions of 90° and 270°. On the middle zone (Z/L = 0), the variations of Cp curves seem milder compared to those on both end zones. In addition, the Cp variations are out of phase on the same zone for positions of 90° and 270° because of vortex paring behind the tapered cylinder. At the same sides for both end zones, e.g., Z/L = ±0.4 at positions of 90° or 270°, the Cp variations are also out of phase but have smaller amplitudes.
Figure 9 suggests that the Cp variations are not in phase and caused by the tapered geometry, as well as the instability of the resulting vortex shedding at positions of 90° and 270° of the cylinder.
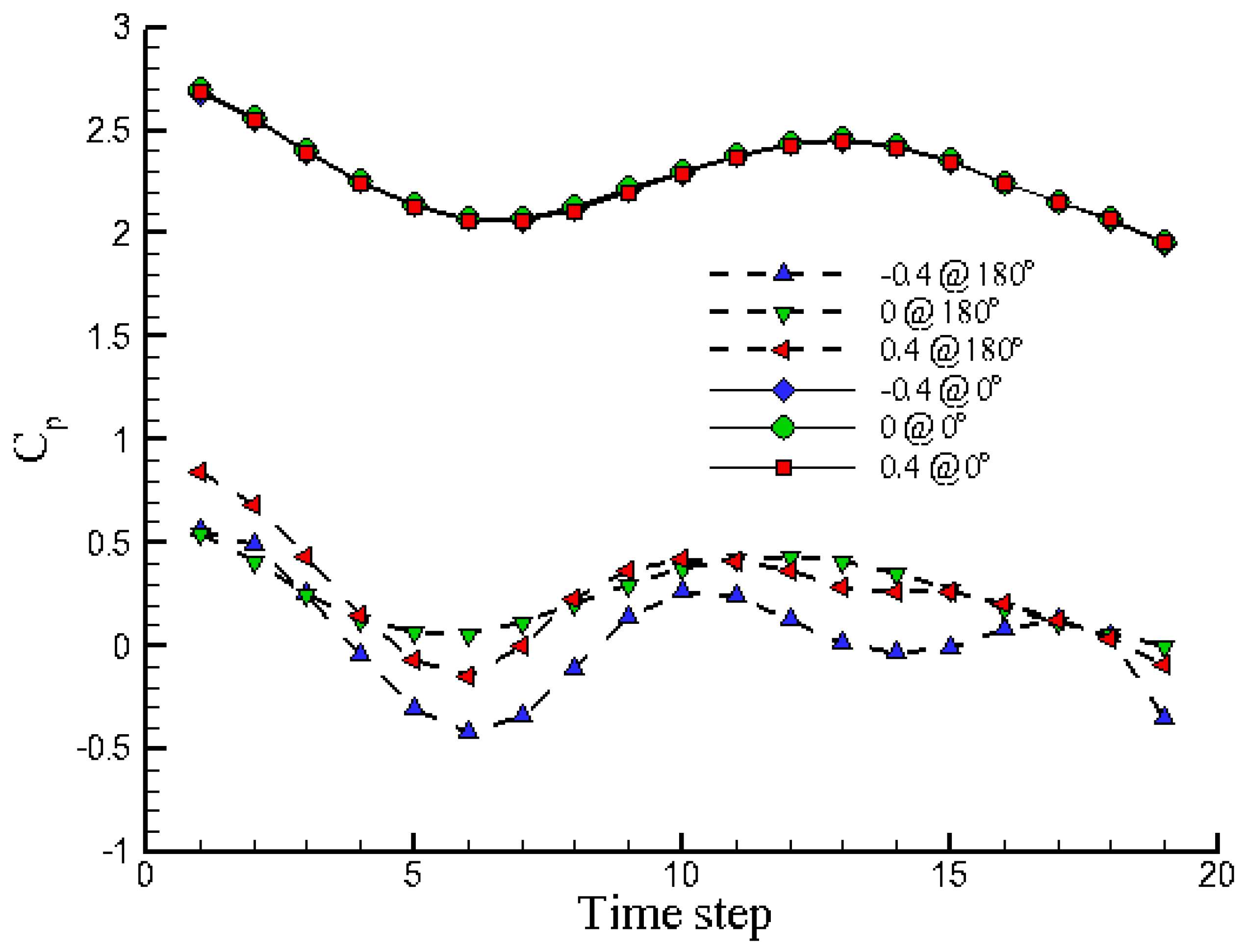
Figure 10 shows time traces of Cp curves on Z/L = 0, ±0.4 zones at positions of 0° and 180°, for about one cycle (20 time steps). Interestingly, at the front position, the Cp variations become wave-like even for those located at the forehead of the cylinder. This reveals that the disturbances from vortex shedding propagate not only in a spanwise direction but also in the upstream direction to the front side of the cylinder. However, one cycle is not long enough to show frequency modulation, and the three curves on Z/L = 0, ±0.4 zones, collapse to nearly become one. It also implies that the disturbances in the spanwise direction based on the tapered geometry of the cylinder hardly affect the upstream flow. Besides, at the position of 180° (behind the tapered cylinder), the Cp variations are almost in-phase and with more moderate amplitudes.
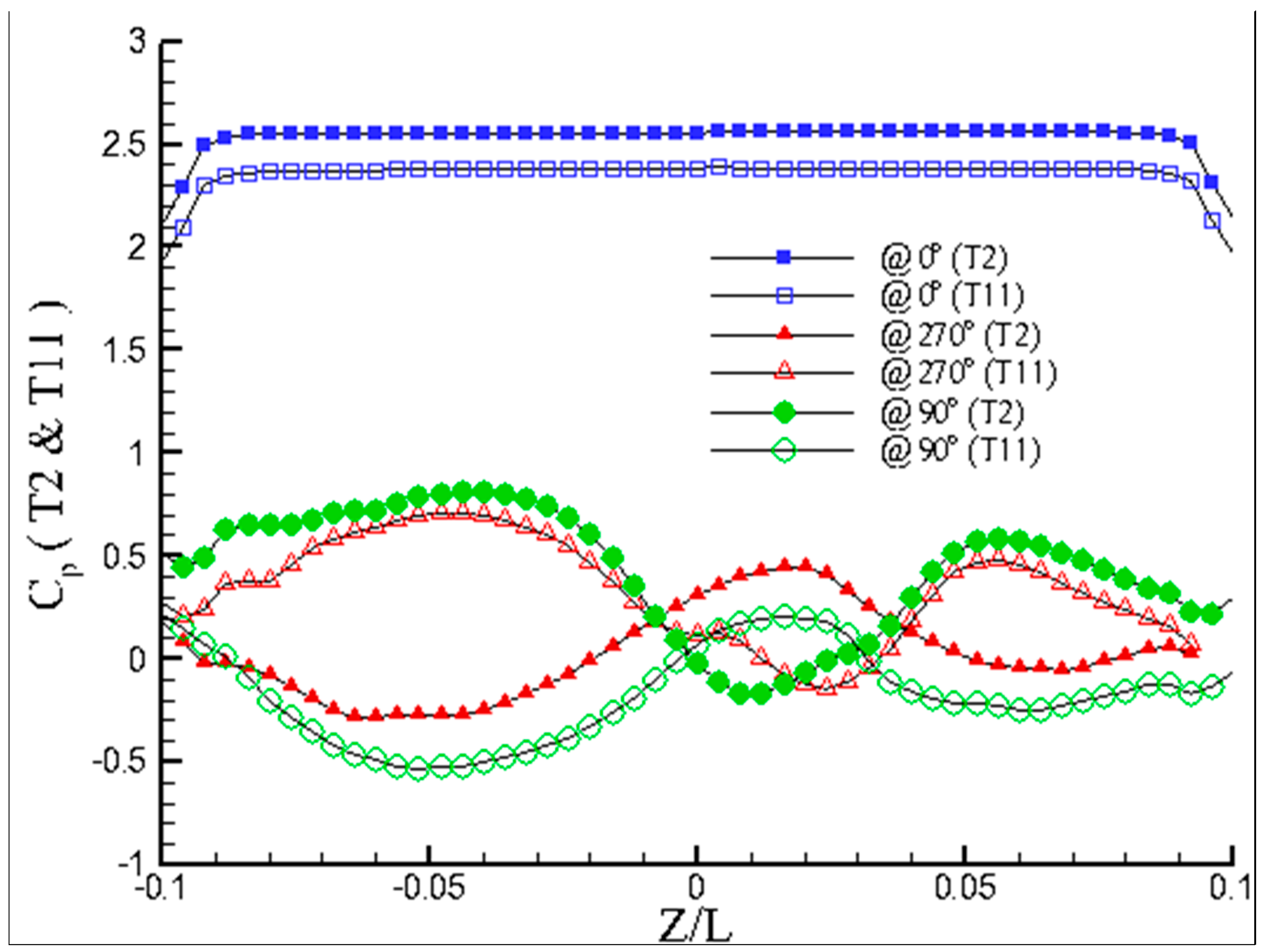
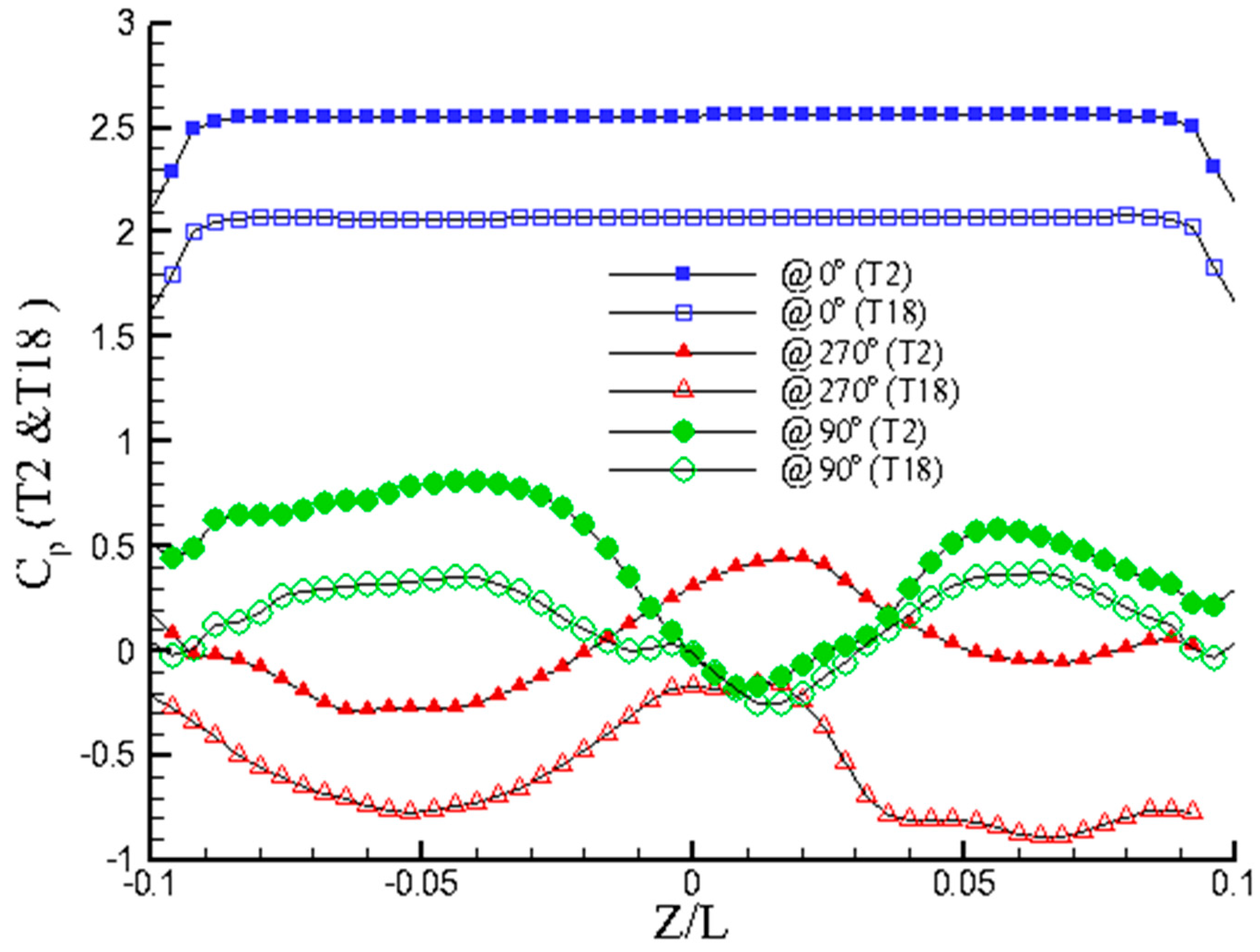
Figure 11 and
Figure 12 show spanwise C
p curves on lines of 0°, 90° and 270° at time step T2 and T11 (half period interval) and at time step T2 and time step T18 (one period interval), respectively. In
Figure 11 and
Figure 12, spanwise C
p distributions on the 0° line of the cylinder act without obvious variations except around both ends of the cylinder. The flat C
p distributions along the front tapered cylinder indicate that the pressure at the cylinder’s front has no direct influence on the formation of vortex splitting. This also indicates that the spanwise disturbances from vortex shedding propagate in the upstream direction but do not significantly affect the pressure on the front side of the cylinder. Indeed, if the cylinder is tapered and there are no 3-D influences, the shedding frequencies are solely dictated by the local 2-D instability, resulting in nearly identical spanwise Strouhal numbers. Hence, the 3-D effect causes the Strouhal number to be different from the 2-D value in these simulations. The C
p curves on lines of 90° and 270° at T2 or T11 in
Figure 11 are out of phase due to pairing shedding vortices. This is similar to T2 and T18 in
Figure 10. In addition, the C
p curves on lines of 90° and 270° at time step T2 are out of phase with regards to both respective lines at T11 (due to half period), but they are in phase with both respective lines at T18 (due to one period).
Indeed, a more accurate simulation requires a better grid system (including the number, type and distribution of grids) and powerful computing power. Because the presentation of the data results of this transient study requires much computing power, in the near future, we are going to use the supercomputer (NVIDIA A100 GPU*8) in I-Shou University, hoping to use the numerical technology developed in this research to simulate and explore more detailed flow fields and understand the physical mechanism of vortex-splitting formation.