Characterization of the Wing Tone around the Antennae of a Mosquito-like Model
Abstract
1. Introduction
2. Methods
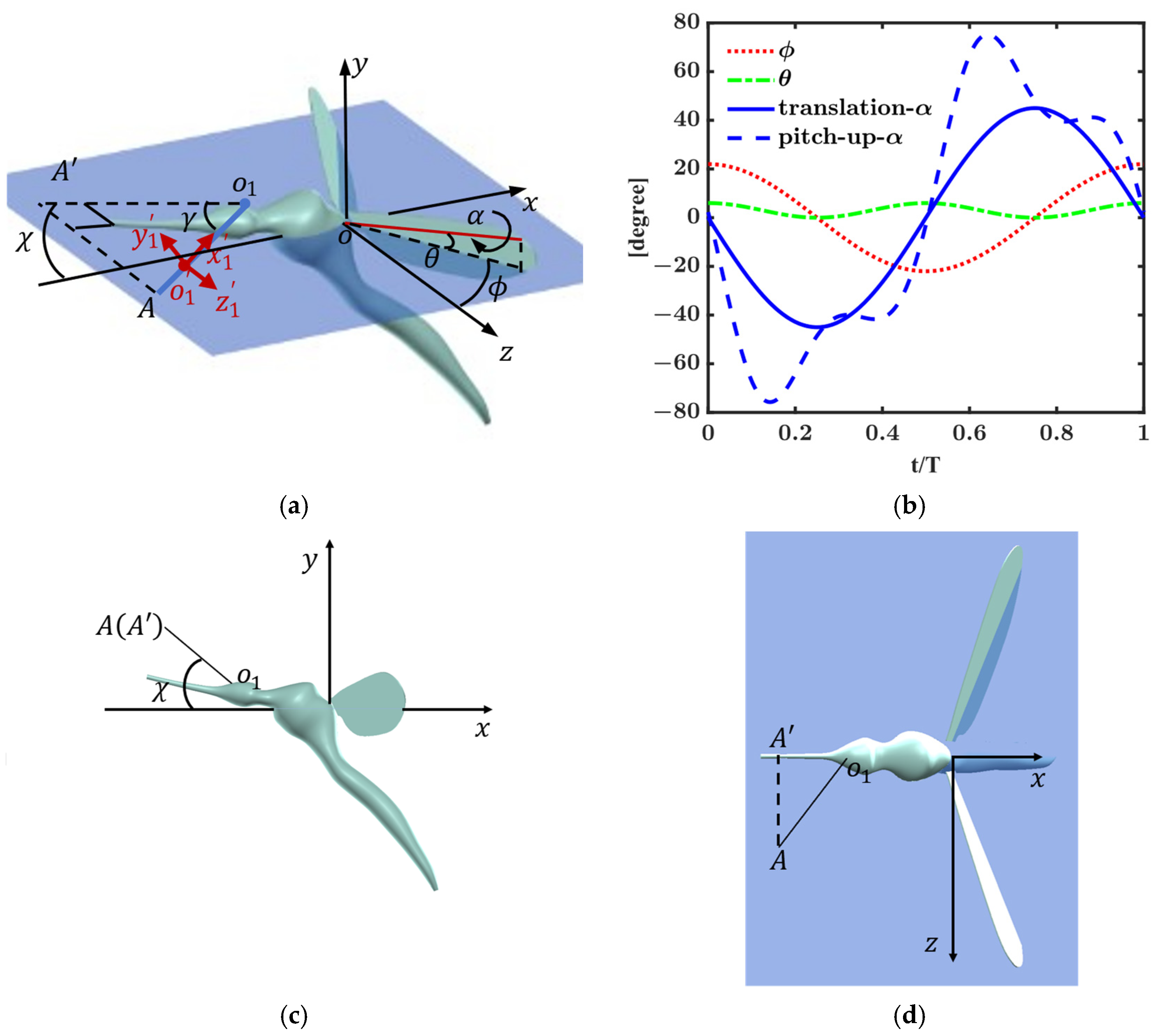
2.1. Mosquito Wing Geometry and Flapping Kinematics
2.2. Aerodynamics Simulation
2.3. Prediction of Sound Particle Velocity Induced by Wing Tone
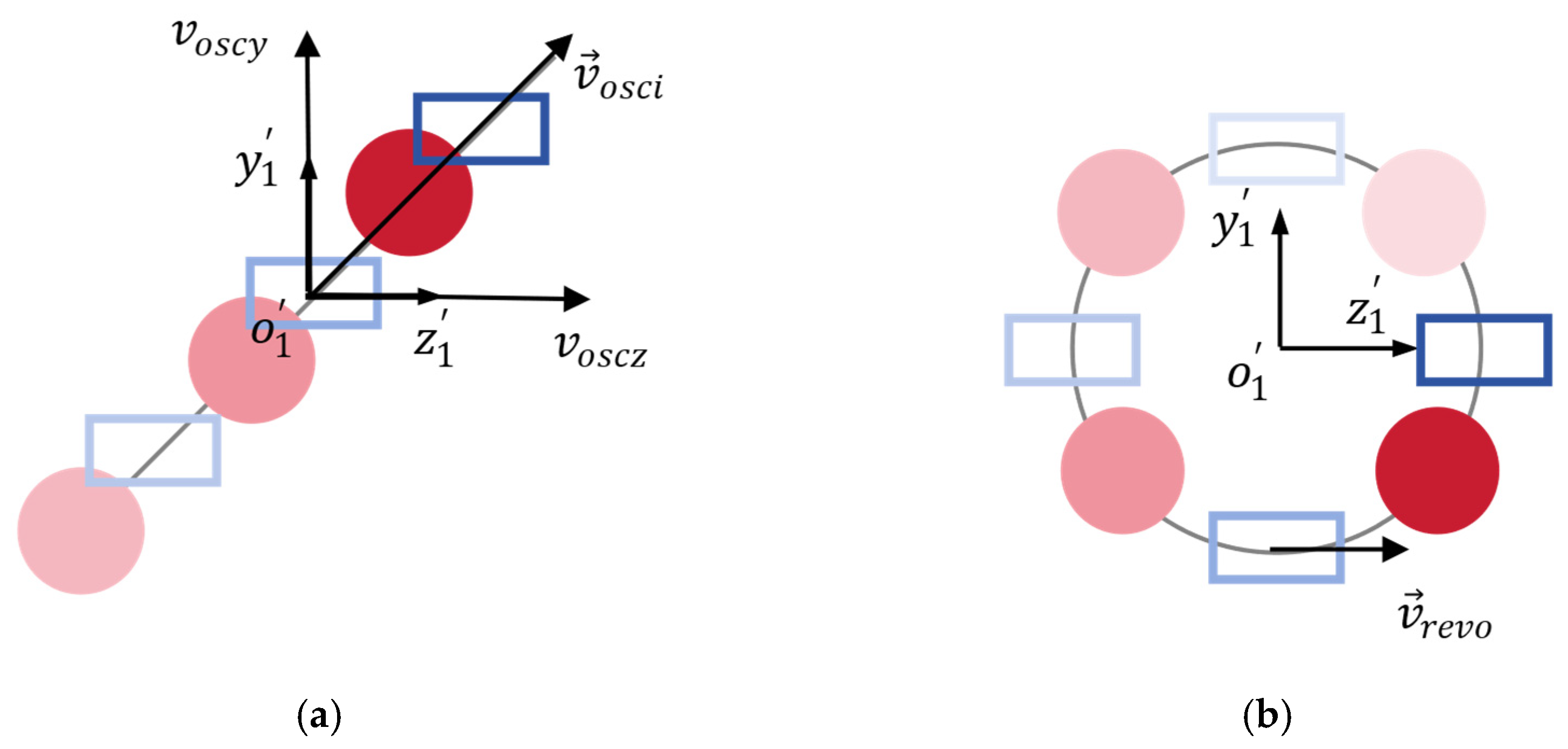
2.4. Characterization of Air Movements around the Antennae
3. Results and Discussion
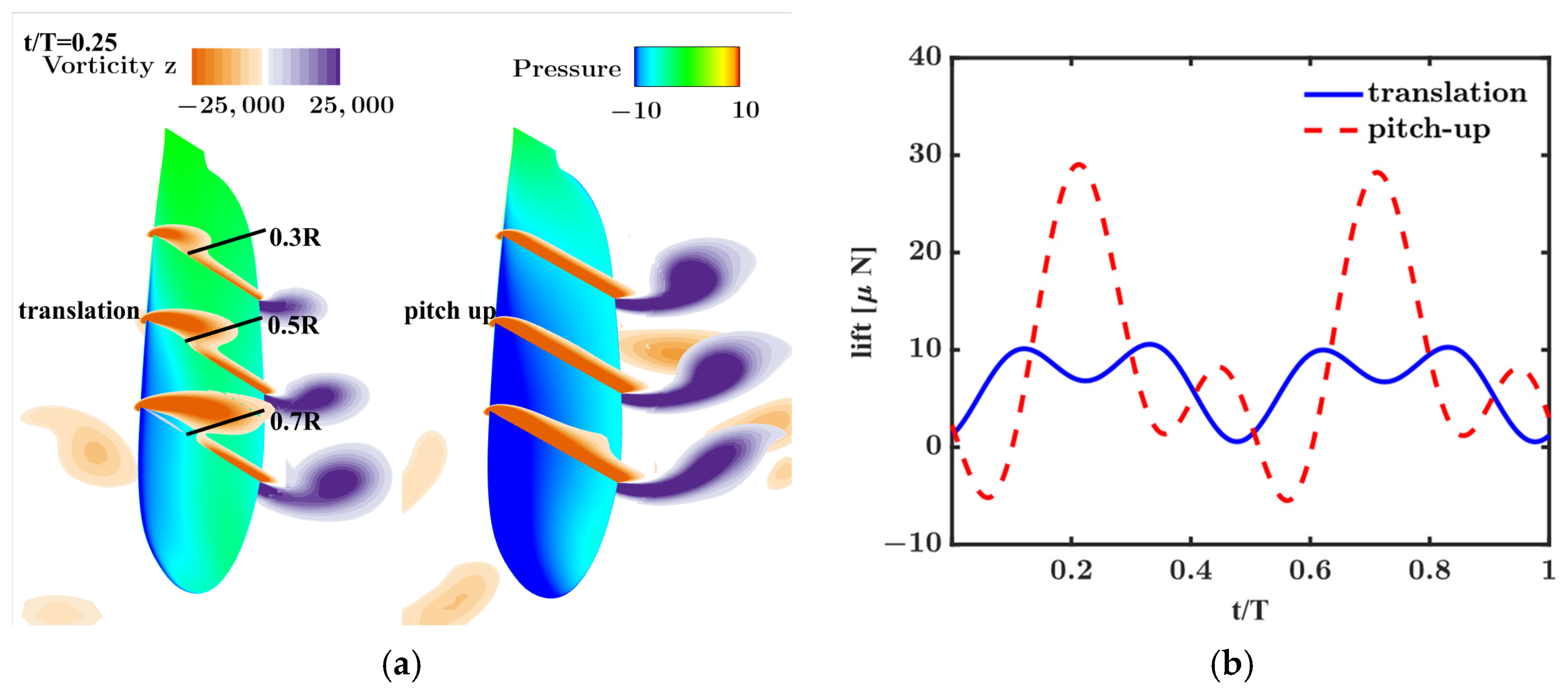
3.1. Aerodynamics
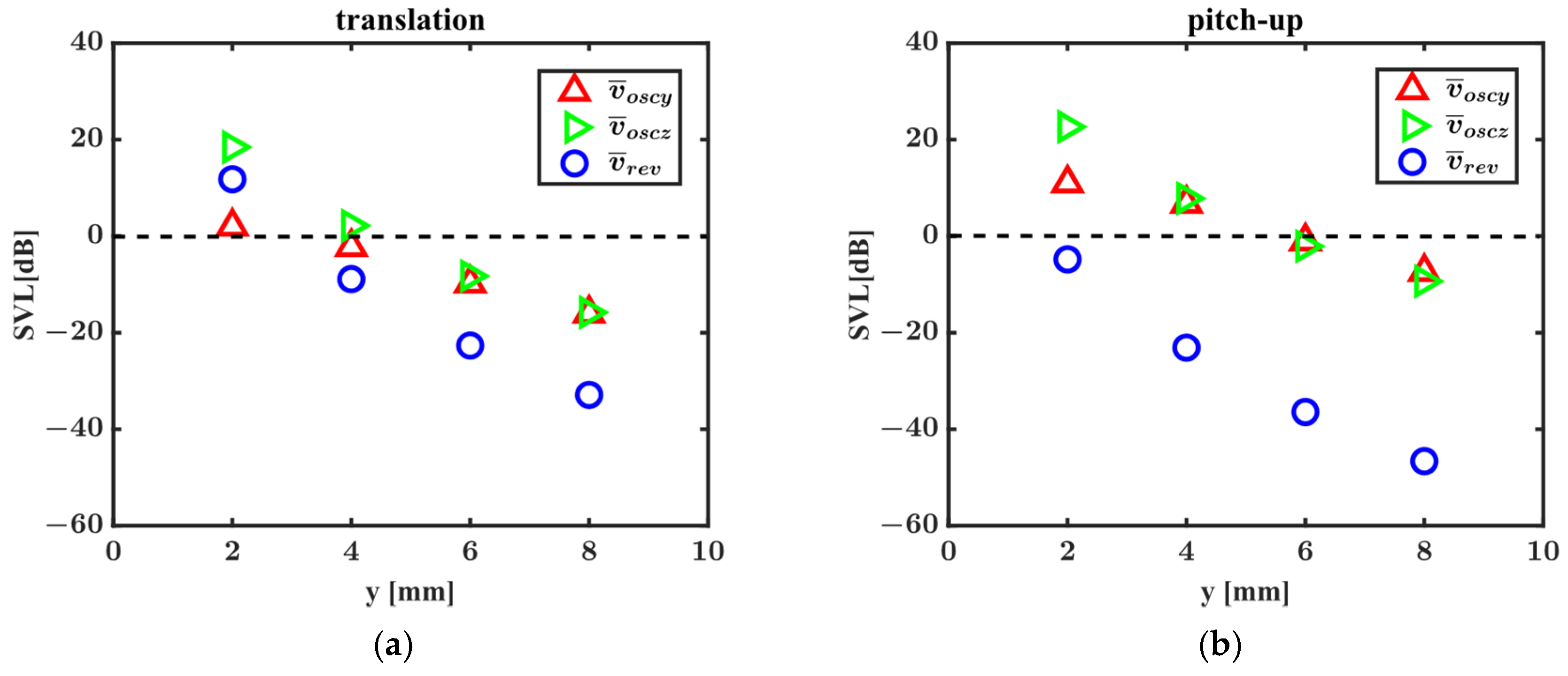
3.2. Sound Particle Velocity Induced by the Self-Generated Wing Tone
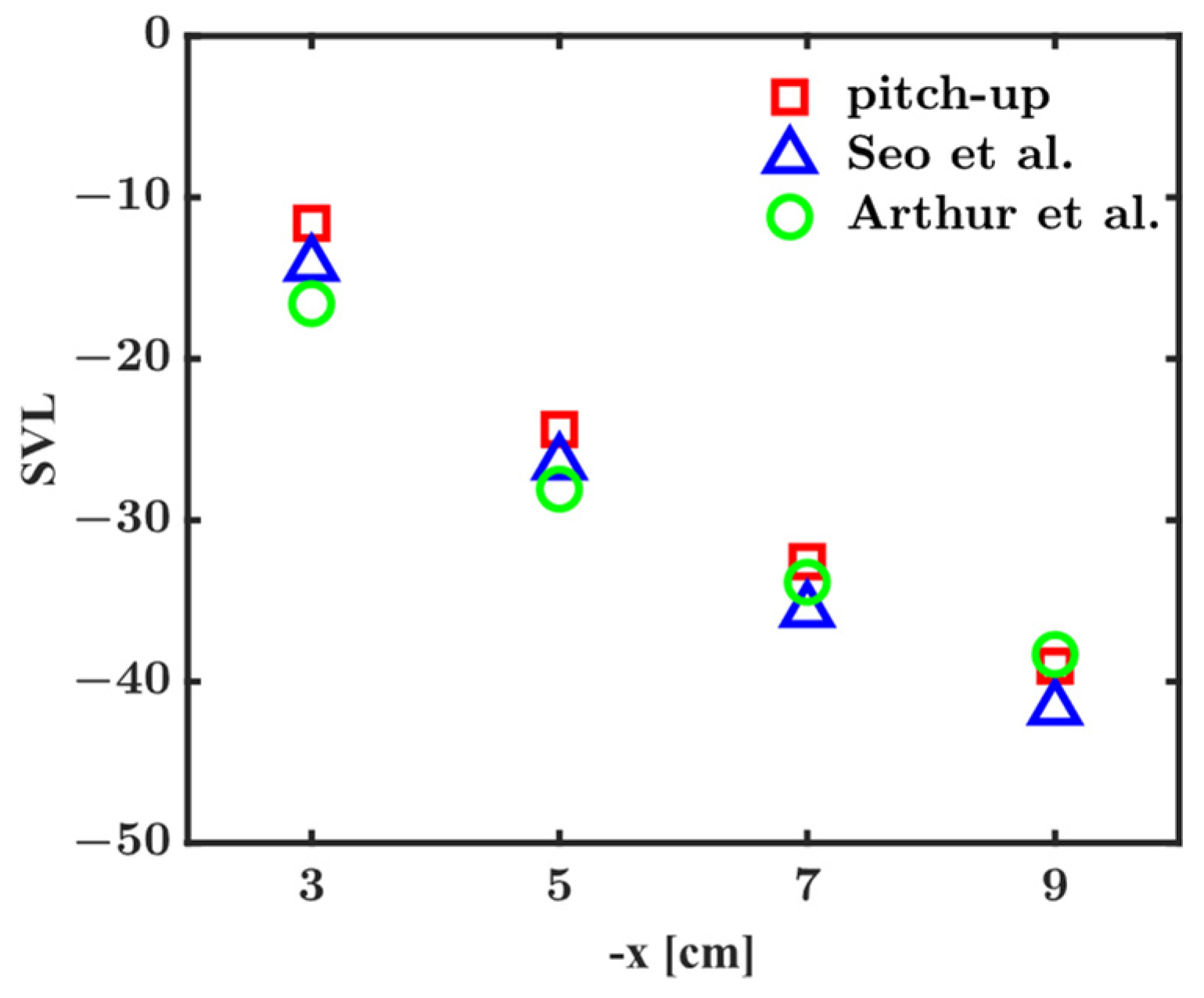
3.3. Sound Particle Velocity Induced by the Reflected Wing Tone
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
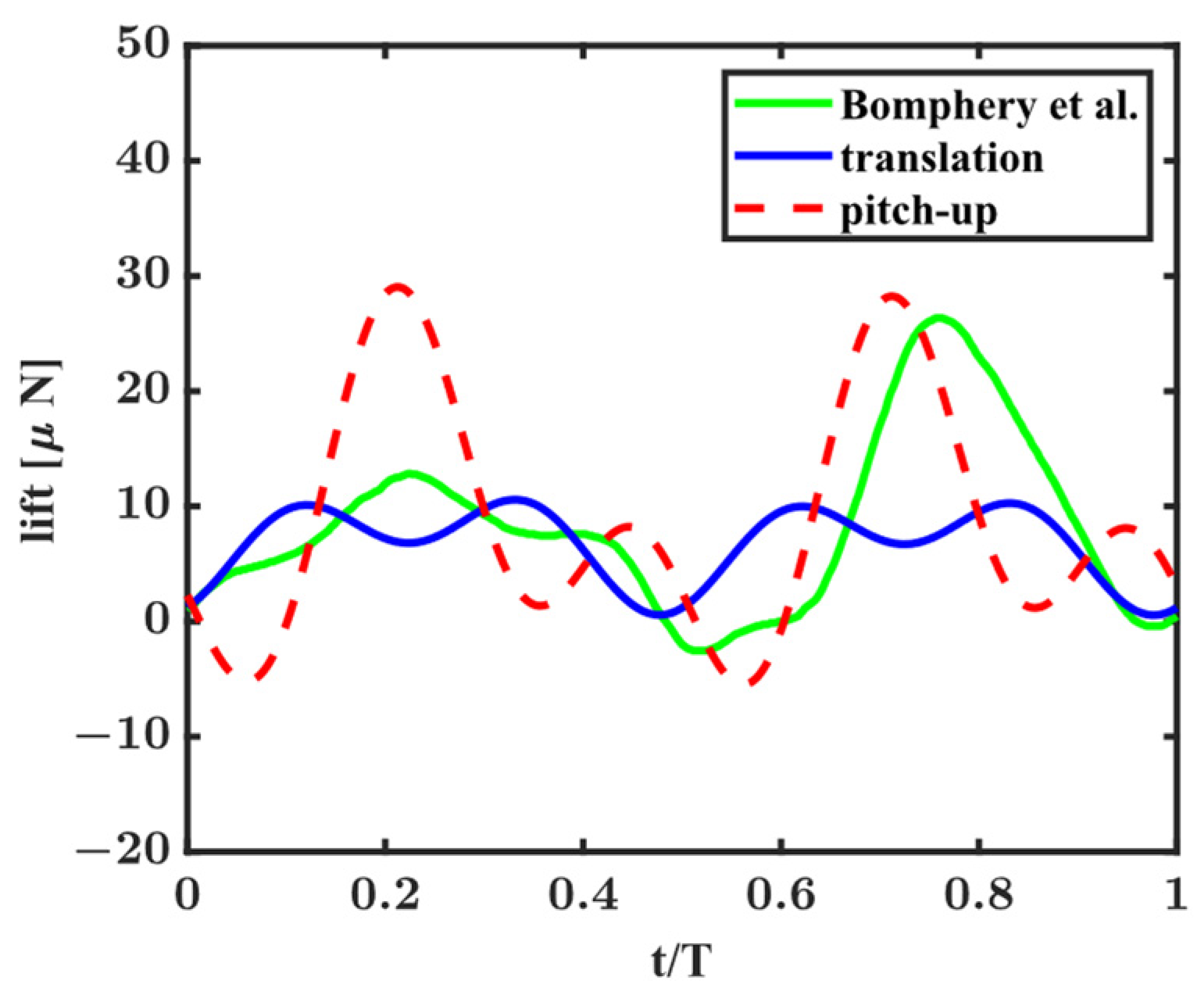
Appendix A. Grid Convergence
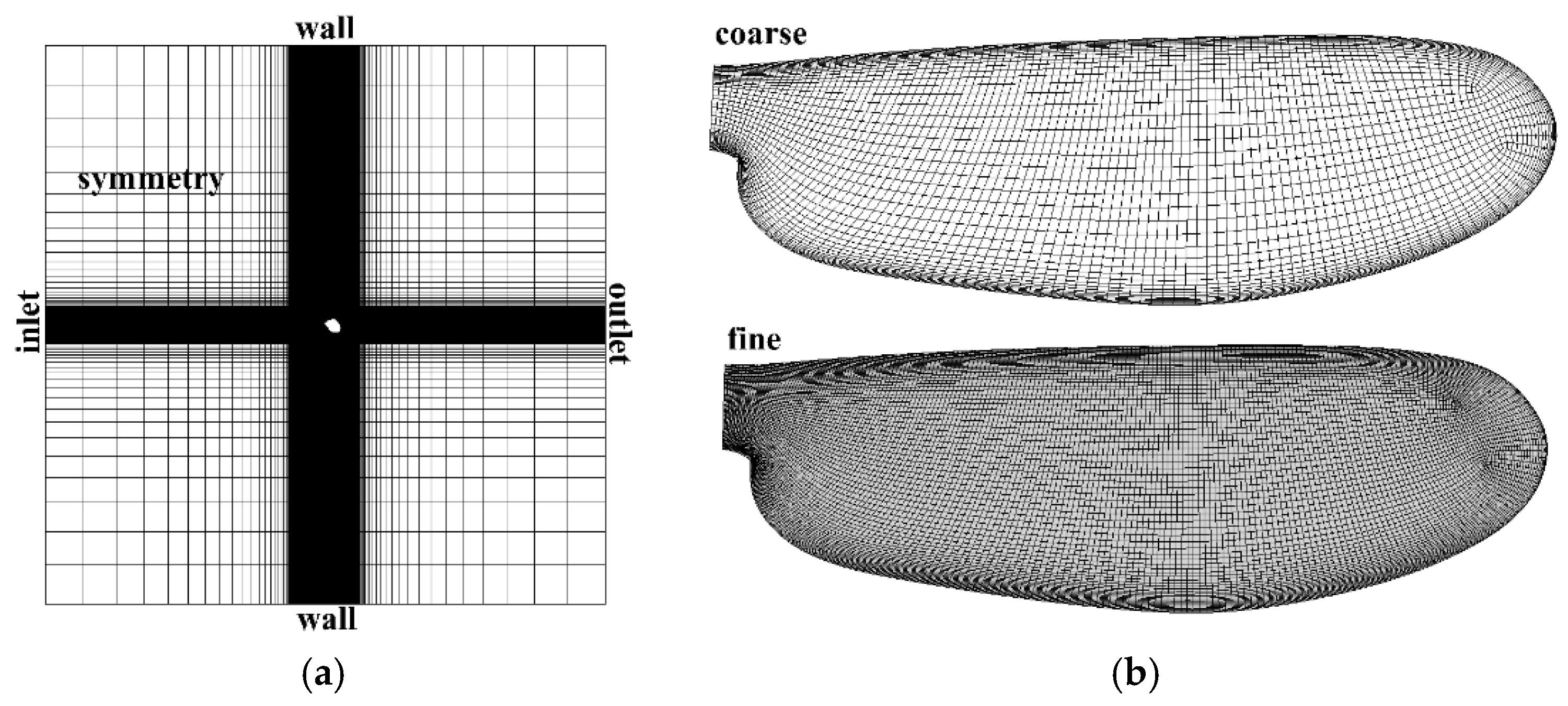
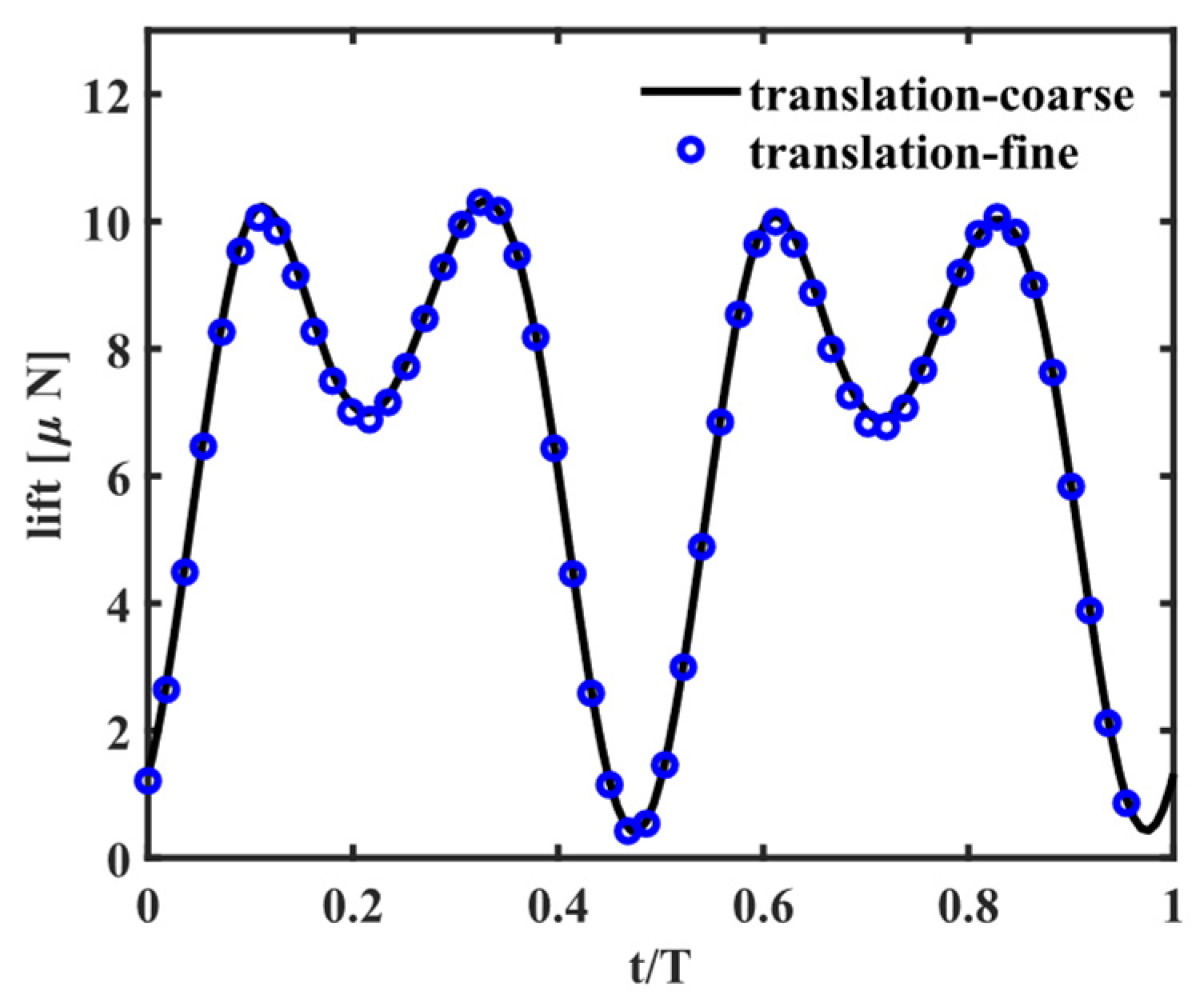
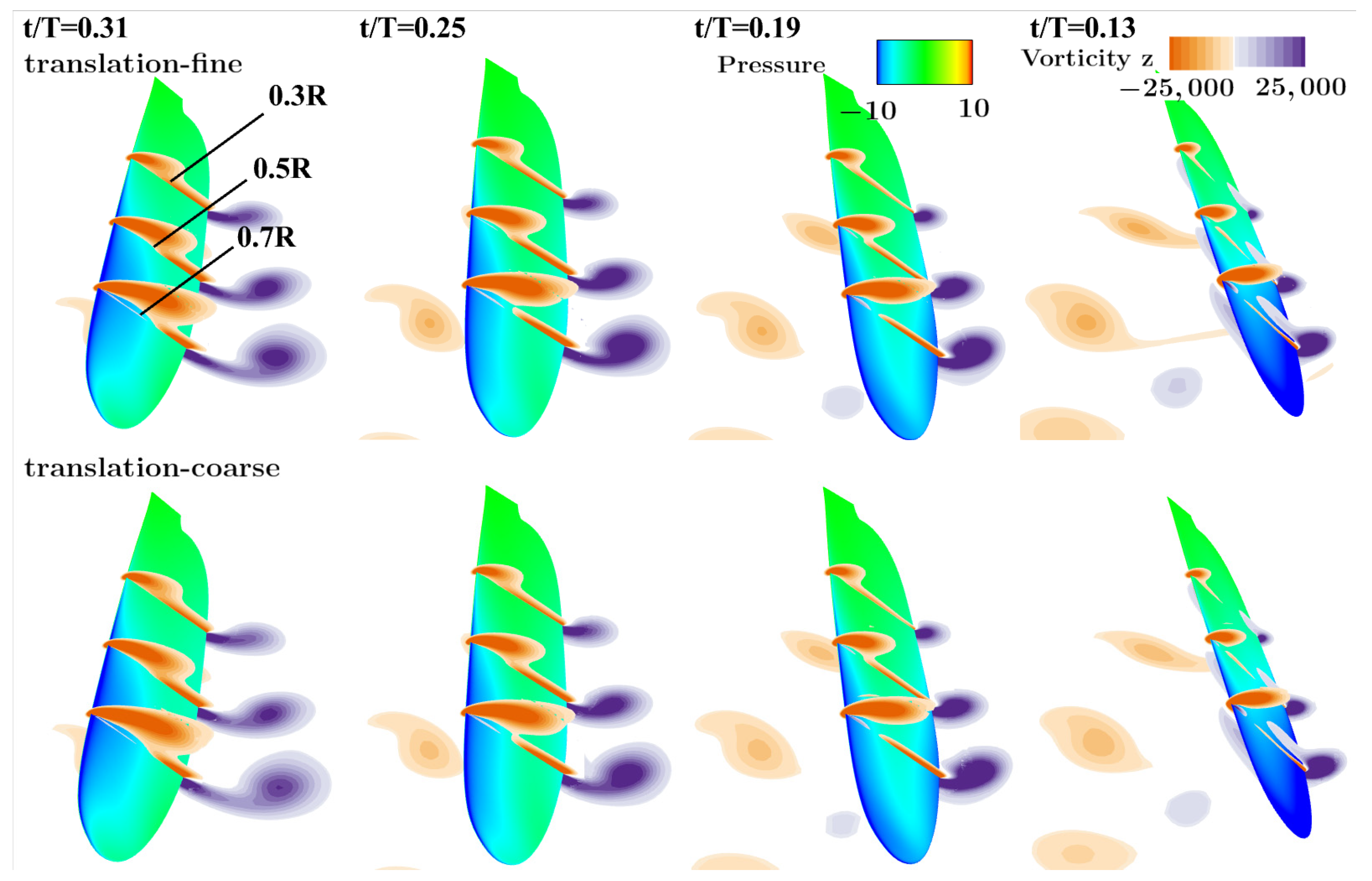
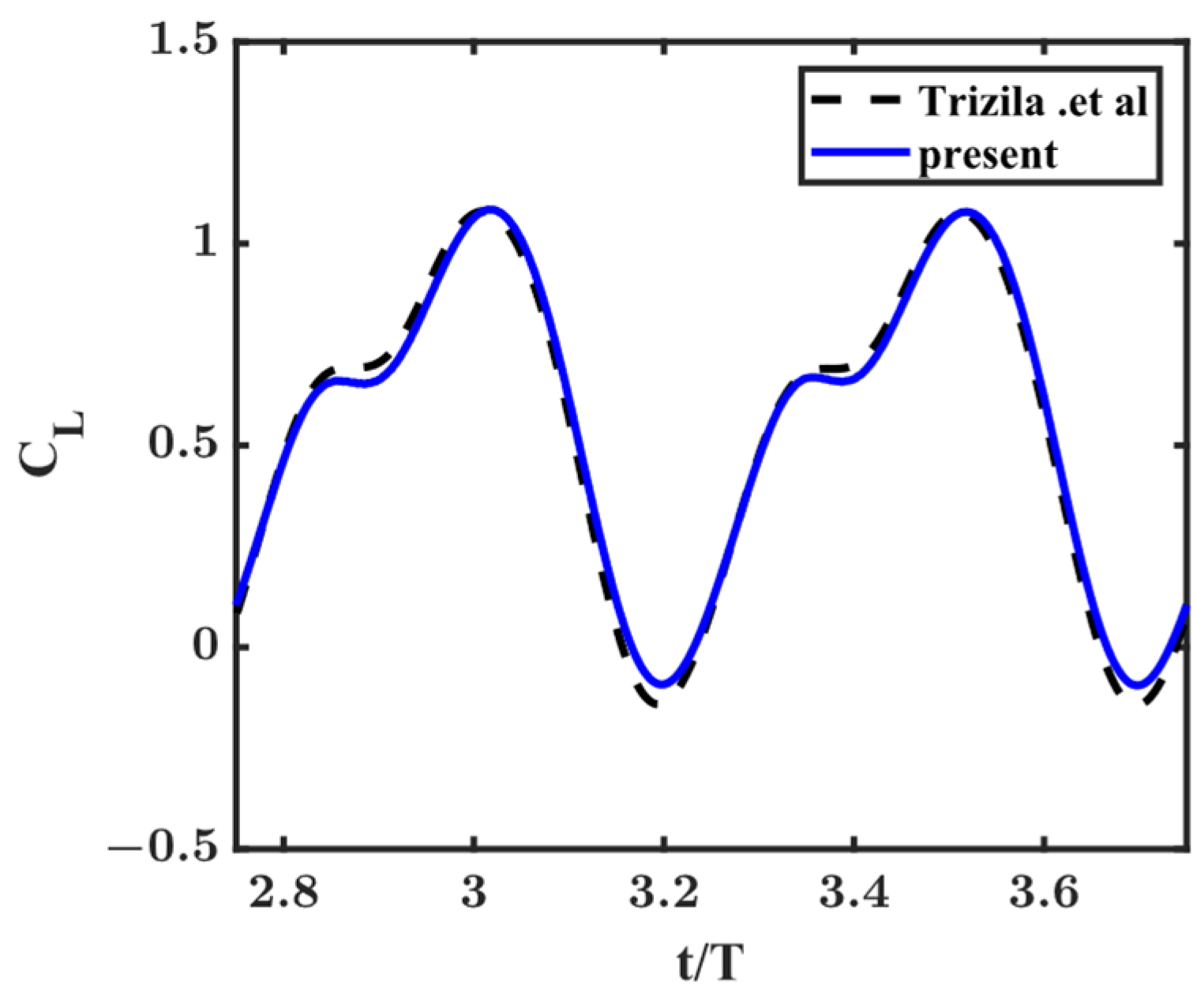
Appendix B. Validation of the CFD Solver
References
- Hawkes, F.; Gibson, G. Seeing is believing: The nocturnal malarial mosquito Anopheles coluzzii responds to visual host-cues when odour indicates a host is nearby. Parasit. Vectors 2016, 9, 1–13. [Google Scholar] [CrossRef]
- Nakata, T.; Phillips, N.; Simões, P.; Russell, I.J.; Cheney, J.A.; Walker, S.M.; Bomphrey, R.J. Aerodynamic imaging by mosquitoes inspires a surface detector for autonomous flying vehicles. Science 2020, 368, 634–637. [Google Scholar] [CrossRef]
- Feugère, L.; Simões, P.; Russell, I.J.; Gibson, G. The role of hearing in mosquito behaviour. In Sensory Ecology of Disease Vectors; Ignell, R., Lazzari, C.R., Lorenzo, M.G., Hill, S.R., Eds.; Wageningen Academic Publishers: Wageningen, The Netherlands, 2022; pp. 683–708. [Google Scholar]
- Meng, X.; Han, Y.; Chen, Z.; Ghaffar, A.; Chen, G. Aerodynamic effects of ceiling and ground vicinity on flapping wings. Appl. Sci. 2022, 12, 4012. [Google Scholar] [CrossRef]
- Su, M.P.; Andrés, M.; Boyd-Gibbins, N.; Somers, J.; Albert, J.T. Sex and species specific hearing mechanisms in mosquito flagellar ears. Nat. Commun. 2018, 9, 3911. [Google Scholar] [CrossRef]
- Simões, P.M.; Ingham, R.A.; Gibson, G.; Russell, I.J. A role for acoustic distortion in novel rapid frequency modulation behaviour in free-flying male mosquitoes. J. Exp. Biol. 2016, 219, 2039–2047. [Google Scholar] [CrossRef]
- Nakata, T.; Simões, P.; Walker, S.M.; Russell, I.J.; Bomphrey, R.J. Auditory sensory range of male mosquitoes for the detection of female flight sound. J. R. Soc. Interface 2022, 19, 20220285. [Google Scholar] [CrossRef]
- Warren, B.; Gibson, G.; Russell, I.J. Sex Recognition through midflight mating duets in Culex mosquitoes is mediated by acoustic distortion. Curr. Biol. 2009, 19, 485–491. [Google Scholar] [CrossRef]
- Bae, Y.; Moon, Y.J. Aerodynamic sound generation of flapping wing. J. Acoust. Soc. Am. 2008, 124, 72–81. [Google Scholar] [CrossRef]
- Geng, B.; Xue, Q.; Zheng, X.; Liu, G.; Ren, Y.; Dong, H. The effect of wing flexibility on sound generation of flapping wings. Bioinspir. Biomim. 2017, 13, 016010. [Google Scholar] [CrossRef]
- Wang, L.; Tian, F.B. Numerical study of sound generation by three-dimensional flexible flapping wings during hovering flight. J. Fluids. Struct. 2020, 99, 103–165. [Google Scholar] [CrossRef]
- Debiasi, M.; Lu, Z.; Nguyen, Q.V.; Chan, W.L. Low-noise flapping wings with tensed membrane. AIAA J. 2020, 58, 2388–2397. [Google Scholar] [CrossRef]
- Nedunchezian, K.; Kang, C.K.; Aono, H. Effects of flapping wing kinematics on the aeroacoustics of hovering flight. J. Sound Vib. 2019, 442, 366–383. [Google Scholar] [CrossRef]
- Inada, Y.; Aono, H.; Liu, H.; Aoyama, T. Numerical analysis of sound generation of insect flapping wings. Theor. Appl. Mech. Jpn. 2009, 57, 437–447. [Google Scholar]
- Glegg, S.; Devenport, W. Aeroacoustics of Low Mach Number Flows: Fundamentals, Analysis, and Measurement; Academic Press: London, UK, 2017; p. 50. [Google Scholar]
- Lapshin, D.N.; Vorontsov, D.D. Directional and frequency characteristics of auditory neurons in Culex male mosquitoes. J. Exp. Biol. 2019, 222, jeb208785. [Google Scholar] [CrossRef]
- Bomphrey, R.J.; Nakata, T.; Phillips, N.; Walker, S.M.J.N. Smart wing rotation and trailing-edge vortices enable high frequency mosquito flight. Nature 2017, 544, 92–95. [Google Scholar] [CrossRef]
- Liu, L.; Sun, M. Dynamic flight stability of hovering mosquitoes. J. Theor. Biol. 2019, 464, 149–158. [Google Scholar] [CrossRef]
- Zhang, J.-D.; Huang, W.-X. On the role of vortical structures in aerodynamic performance of a hovering mosquito. Phys. Fluids 2019, 31, 051906. [Google Scholar] [CrossRef]
- Liu, L.G.; Du, G.; Sun, M. Aerodynamic-force production mechanisms in hovering mosquitoes. J. Fluid Mech. 2020, 898, A19. [Google Scholar] [CrossRef]
- Calado, A.; Poletti, R.; Koloszar, L.K.; Mendez, M.A. A robust data-driven model for flapping aerodynamics under different hovering kinematics. Phys. Fluids 2023, 35, 047122. [Google Scholar] [CrossRef]
- Wu, Y.K.; Sun, M.; Liu, Y.P. The wing− wing interaction mechanism of bristled wing pair in fling motion. Phys. Fluids 2022, 34, 071903. [Google Scholar] [CrossRef]
- Verma, S.; Hemmati, A. Characterization of bifurcated dual vortex streets in the wake of an oscillating foil. J. Fluid Mech. 2022, 945, A7. [Google Scholar] [CrossRef]
- Cianferra, M.; Armenio, V.; Ianniello, S. Hydroacoustic noise from different geometries. Int. J. Heat Fluid Flow 2018, 70, 348–362. [Google Scholar] [CrossRef]
- Cianferra, M.; Ianniello, S.; Armenio, V. Assessment of methodologies for the solution of the Ffowcs Williams and Hawkings equation using LES of incompressible single-phase flow around a finite-size square cylinder. J. Sound Vib. 2019, 453, 1–24. [Google Scholar] [CrossRef]
- Ricciardi, T.R.; Wolf, W.R.; Spalart, P.R. On the application of incomplete Ffowcs Williams and Hawkings surfaces for aeroacoustic predictions. AIAA J. 2022, 60, 1971–1977. [Google Scholar] [CrossRef]
- Brentner, K.S.; Farassat, F. Analytical comparison of the acoustic analogy and Kirchhoff formulation for moving surfaces. AIAA J. 1998, 36, 1379–1386. [Google Scholar] [CrossRef]
- Seo, J.H.; Hedrick, T.L.; Mittal, R. Mechanism and scaling of wing tone generation in mosquitoes. Bioinspir. Biomim. 2019, 15, 016008. [Google Scholar] [CrossRef]
- Seo, J.H.; Hedrick, T.L.; Mittal, R. Mosquitoes buzz and fruit flies don’ta comparative aeroacoustic analysis of wing-tone generation. Bioinspir. Biomim. 2021, 16, 046019. [Google Scholar] [CrossRef]
- Arthur, B.J.; Emr, K.S.; Wyttenbach, R.A.; Hoy, R.R. Mosquito (Aedes aegypti) flight tones: Frequency, harmonicity, spherical spreading, and phase relationships. J. Acoust. Soc. Am. 2014, 135, 933–941. [Google Scholar] [CrossRef]
- Avitabile, D.; Homer, M.; Champneys, A.R.; Jackson, J.C.; Robert, D. Mathematical modelling of the active hearing process in mosquitoes. J. R. Soc. Interface 2010, 7, 105–122. [Google Scholar] [CrossRef][Green Version]
- Eldredge, J.D.; Jones, A.R. Leading-edge vortices: Mechanics and modeling. Annu. Rev. Fluid Mech. 2019, 51, 75–104. [Google Scholar] [CrossRef]
- Trizila, P.; Kang, C.K.; Aono, H.; Shyy, W.; Visbal, M. Low-Reynolds-number aerodynamics of a flapping rigid flat plate. AIAA J. 2011, 49, 806–823. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, Y.; Zhou, Z.; Xie, Z. Characterization of the Wing Tone around the Antennae of a Mosquito-like Model. Fluids 2024, 9, 31. https://doi.org/10.3390/fluids9020031
Wang Y, Zhou Z, Xie Z. Characterization of the Wing Tone around the Antennae of a Mosquito-like Model. Fluids. 2024; 9(2):31. https://doi.org/10.3390/fluids9020031
Chicago/Turabian StyleWang, Yongtao, Zhiteng Zhou, and Zhuoyu Xie. 2024. "Characterization of the Wing Tone around the Antennae of a Mosquito-like Model" Fluids 9, no. 2: 31. https://doi.org/10.3390/fluids9020031
APA StyleWang, Y., Zhou, Z., & Xie, Z. (2024). Characterization of the Wing Tone around the Antennae of a Mosquito-like Model. Fluids, 9(2), 31. https://doi.org/10.3390/fluids9020031