On the Numerical Investigation of Two-Phase Evaporative Spray Cooling Technology for Data Centre Applications
Abstract
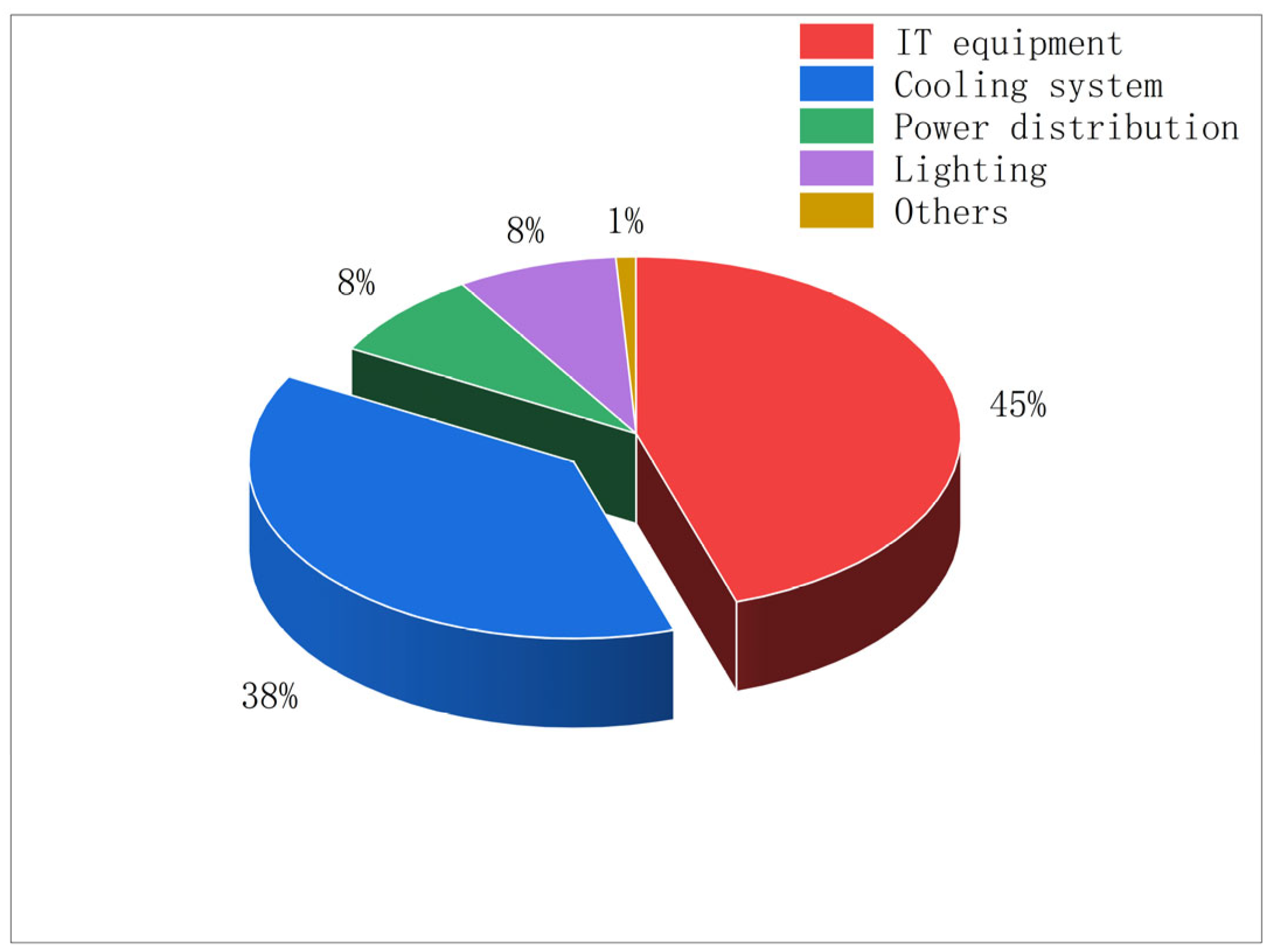
1. Introduction
2. Materials and Methods
2.1. Computational Model
2.2. Modelling Assumptions
- The Eulerian–Lagrangian approach is employed to couple conservation equations between the water droplets and air.
- The continuous phase (humid air) is modelled using the Eulerian approach, while the dispersed phase (water droplets) is tracked in a Lagrangian frame of reference.
- The volume fraction of the dispersed phase (water droplets) is less than 0.02% of the continuous phase (humid air). Hence, dilute phase assumption is valid, which means droplet collisions and droplet-to-droplet interactions are neglected. Droplet breakup and coalescence are neglected.
- Water droplets are assumed to be spherical and do not deform while moving in the continuous phase.
- Water droplets are treated as point masses and the trajectory for each discrete phase droplet is determined by integrating the force balance on the droplets.
- Two-way coupling was used to model the interaction between the continuous and dispersed phases.
- Droplet evaporation occurs only at its surface with a quasi-steady assumption. Temperature equilibrates instantaneously within the droplet (i.e., lumped heat transfer assumption).
2.3. Governing Equations
2.4. Boundary Conditions
2.5. Discrete Phase Model
2.6. Droplet Distribution
2.7. Spray Nozzle Characteristics
2.8. Solver Settings
2.9. Coefficient of Performance
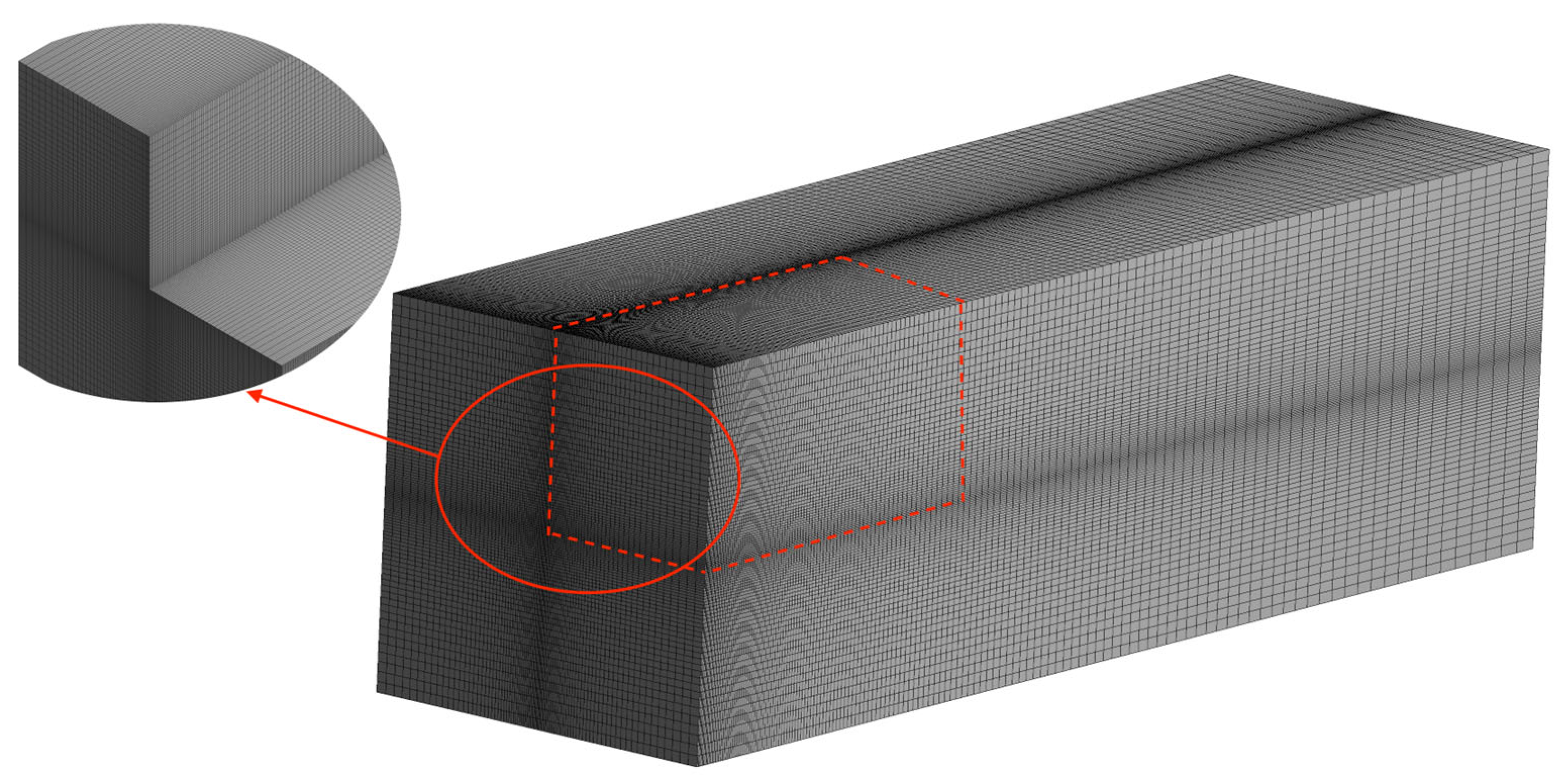
2.10. Grid Independence Study
3. Results and Discussion
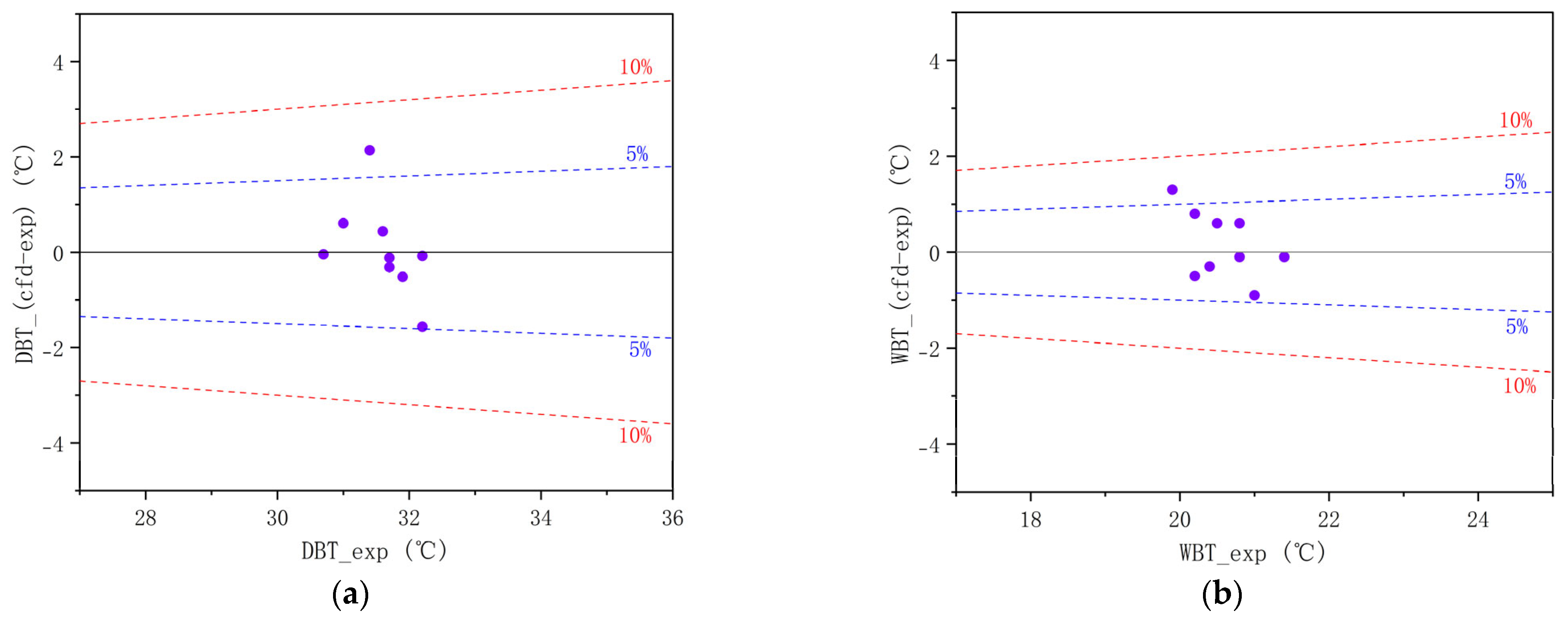
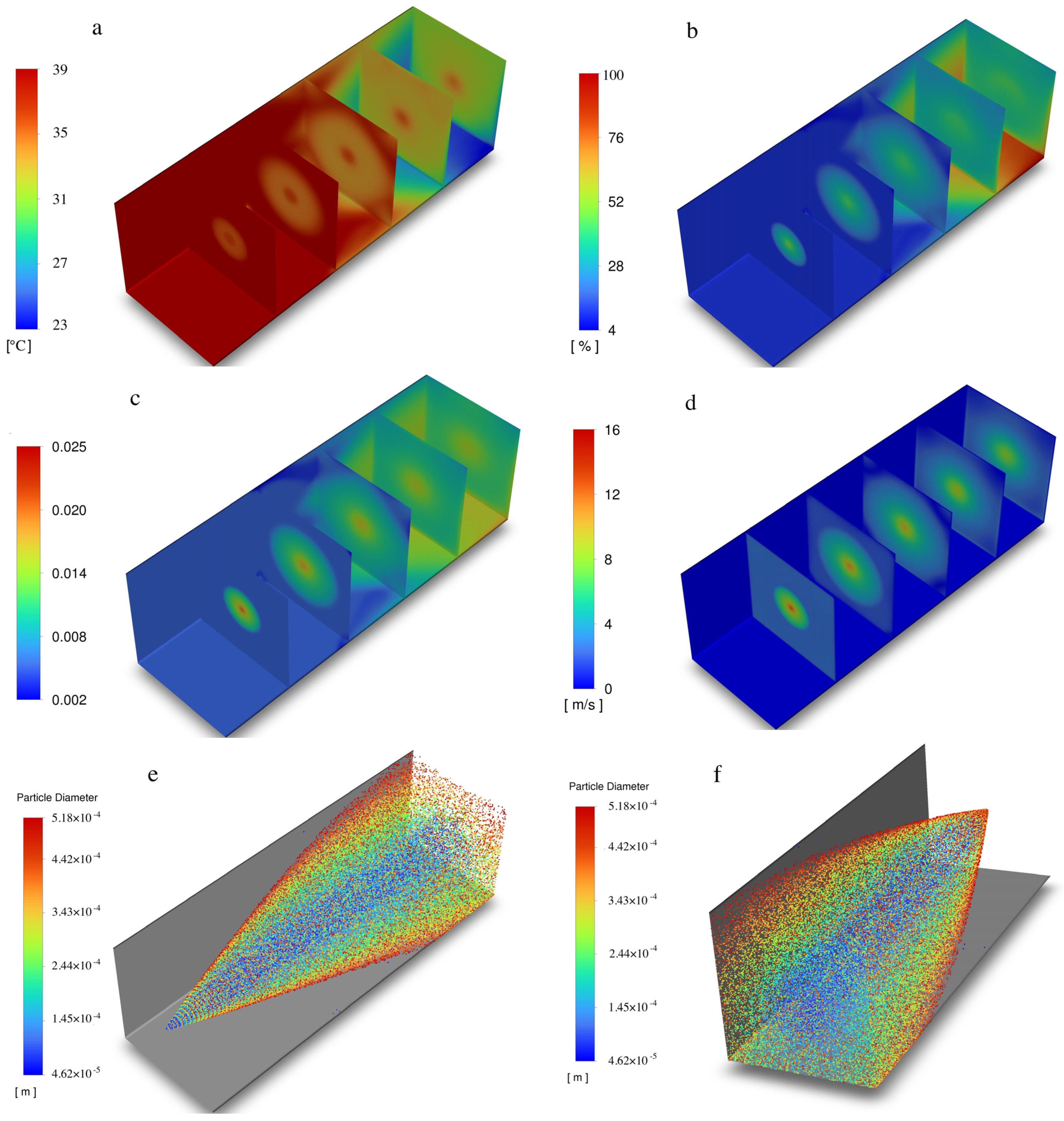
3.1. Validation and Initial Analysis
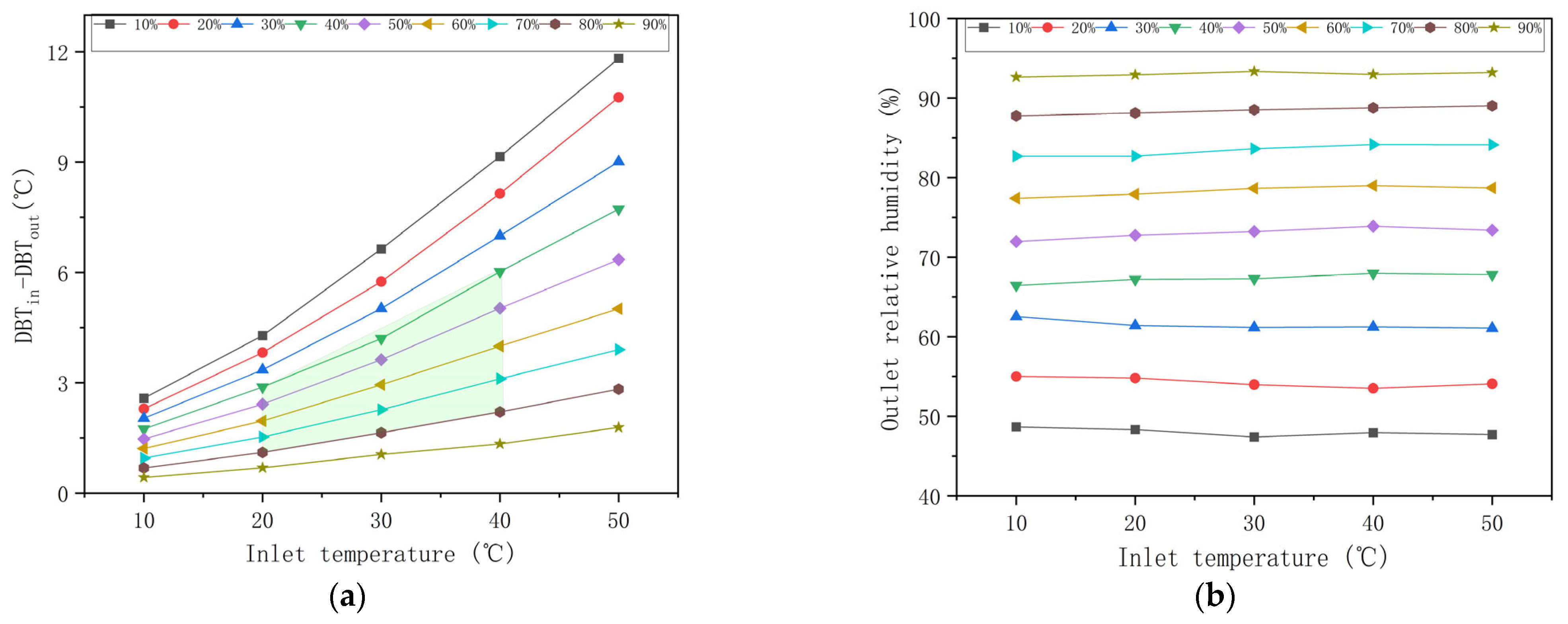
3.2. Impact of Different Geographical Conditions
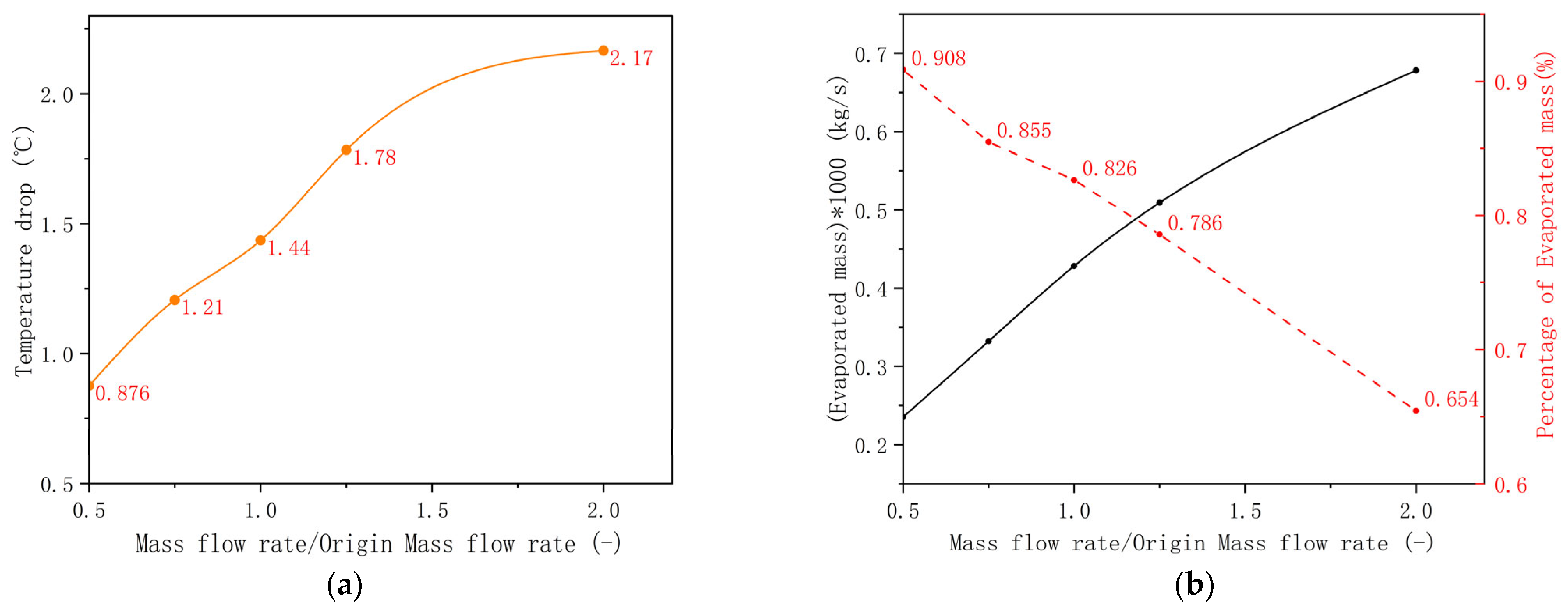
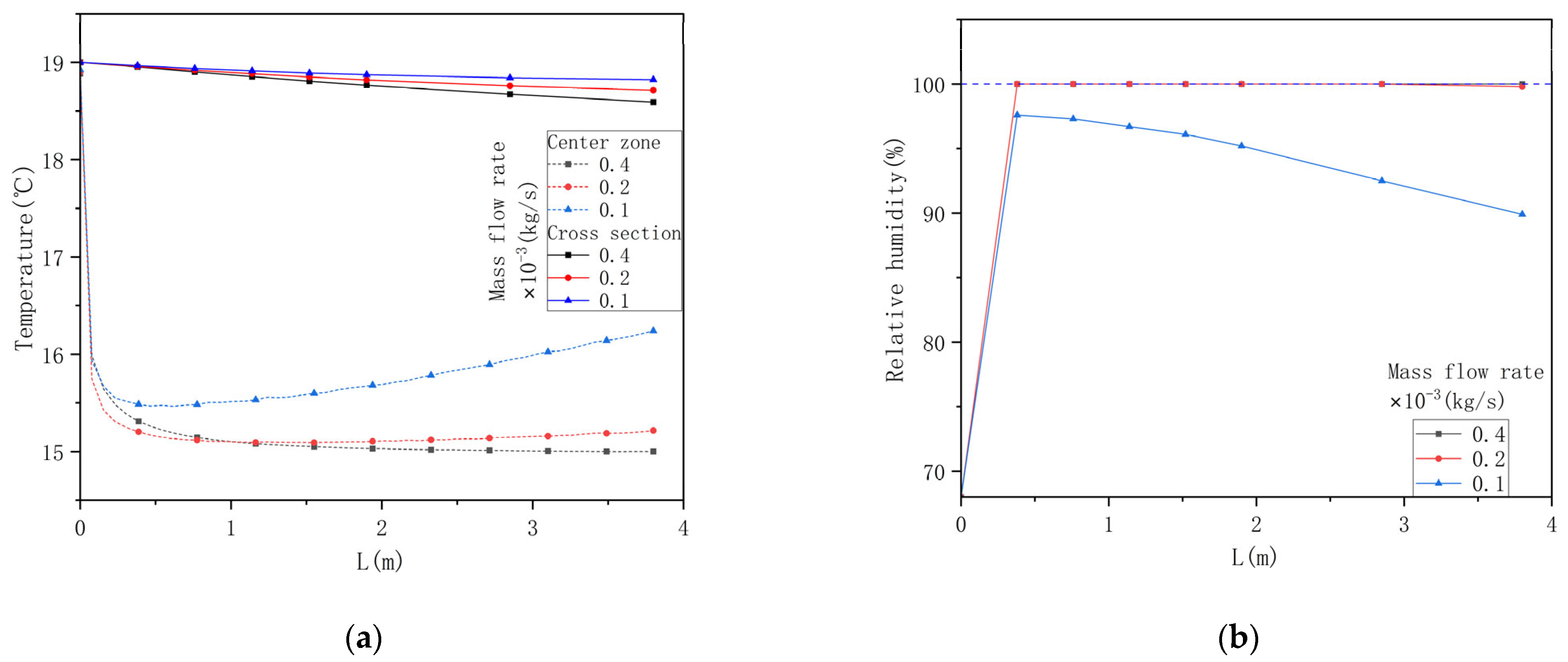
3.3. Impact of Mass Flow Rate
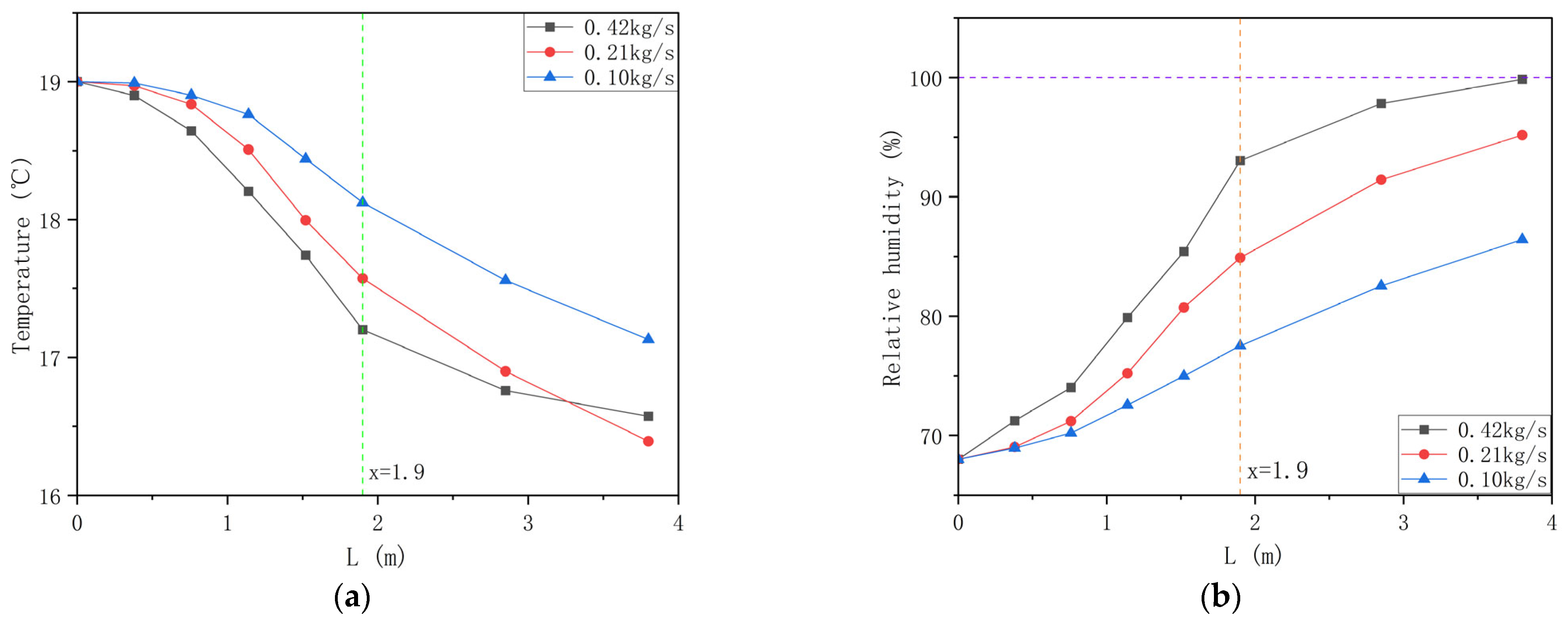
3.4. Impact of the Length of the Domain
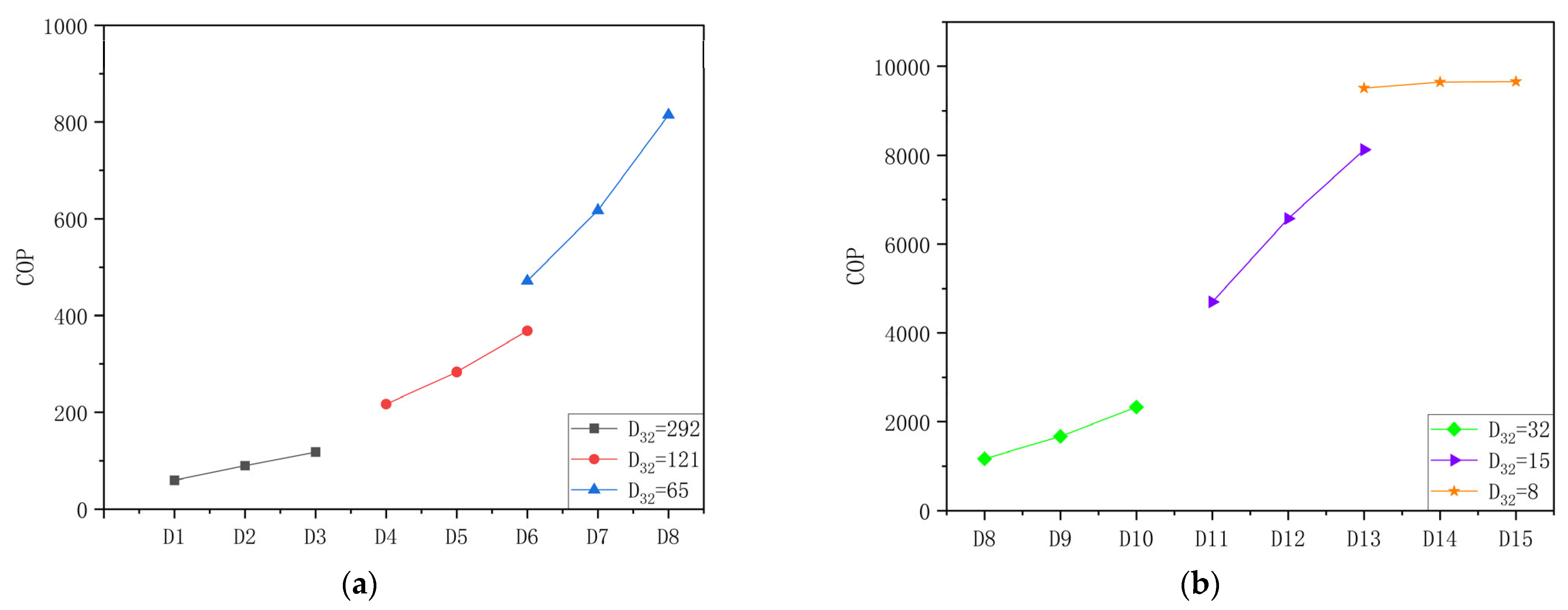
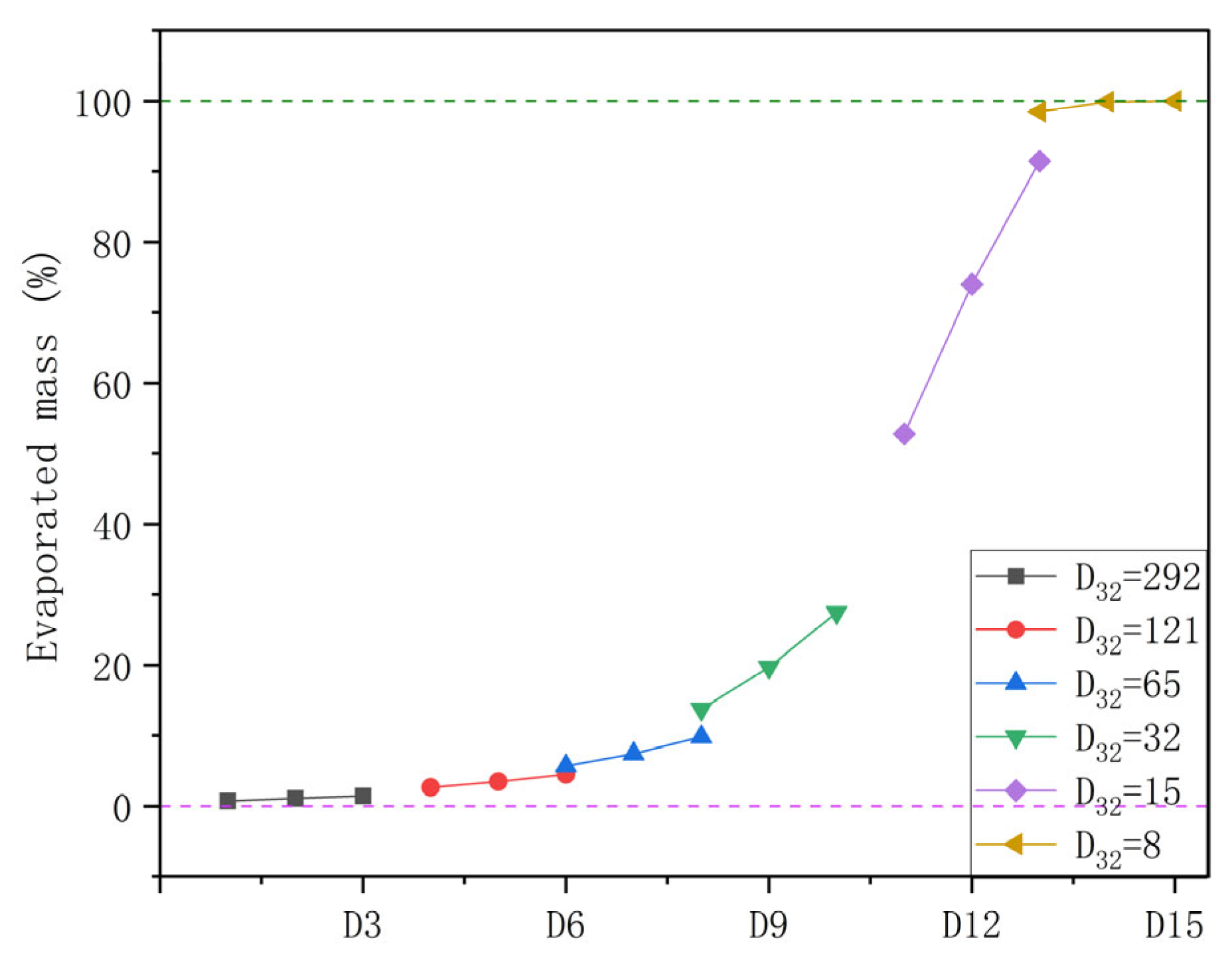
3.5. Average Droplet Size and Mass Flow Rate
3.6. Optimization Strategy for the Injector Design
- Fixing the upper limit of the droplet diameter and reducing the water mass flow rate in a geometric progression with ratio ½, and obtaining a relative humidity value close to 100%.
- Reducing the droplet diameter in a geometric progression with ratio ½, repeating Step 1 until the 100% relative humidity point is achieved.
4. Limitations
5. Conclusions
- (1)
- The temperature and humidity of the air have a big influence on the evaporation system. Regions with high temperatures and low humidity experience the greatest cooling effect, with temperature drops of up to 12 °C at 50 °C and 10% humidity. Most regions fall within moderate temperature and humidity ranges, where evaporation systems typically reduce temperatures by 1.5 to 6 °C for an average Sauter mean diameter (SMD) of 292 μm.
- (2)
- Increasing mass flow rates in evaporation systems significantly enhances the temperature drop, with a 50.8% rise in ΔT as the flow rate increases from ṁ to 2ṁ. However, this is accompanied by a decrease in the percentage of evaporated mass reducing the overall system performance (COP) in terms of water consumption.
- (3)
- Extending the domain length significantly enhances cooling efficiency, with the temperature disparity between the inlet and outlet increasing by 85.7% and relative humidity rising by 61%. An increase in the mass flow rate results in a more rapid decline in temperature. However, beyond a certain distance, the evaporation rate declines due to the air becoming saturated, thereby limiting the evaporation process.
- (4)
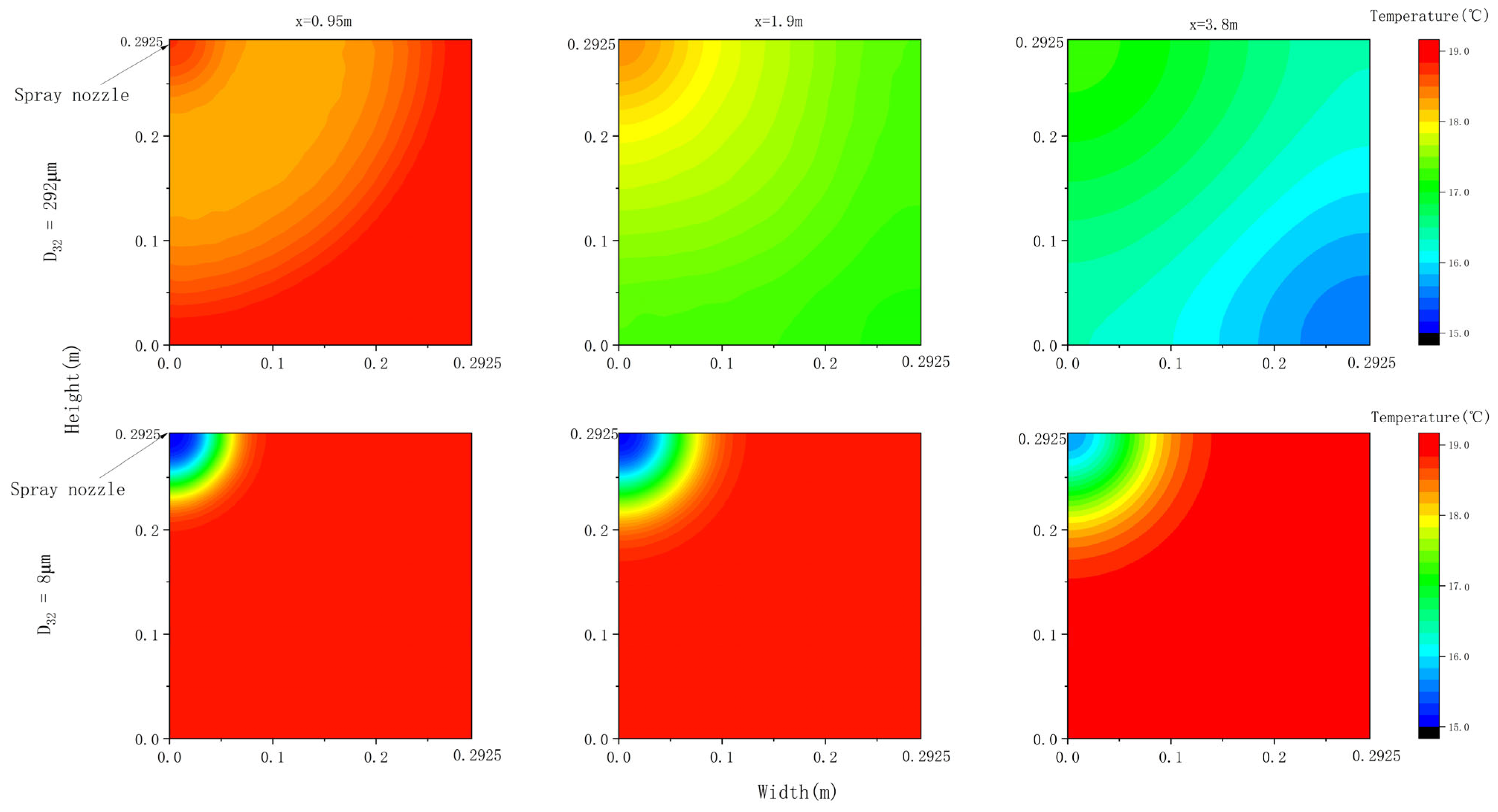
- Larger droplets (D32 = 292 μm, ṁ = 0.21 kg/s) result in a notable reduction in temperature across the domain, with higher temperatures observed in the central region. In contrast, smaller droplets (D32 = 8 μm, ṁ = 0.0001 kg/s) exert a dominant influence on the airflow, resulting in the concentration of cooling in the centre region with minimal temperature change along the domain. Therefore, to achieve the same amount of cooling, a multi-nozzle configuration is required.
- (5)
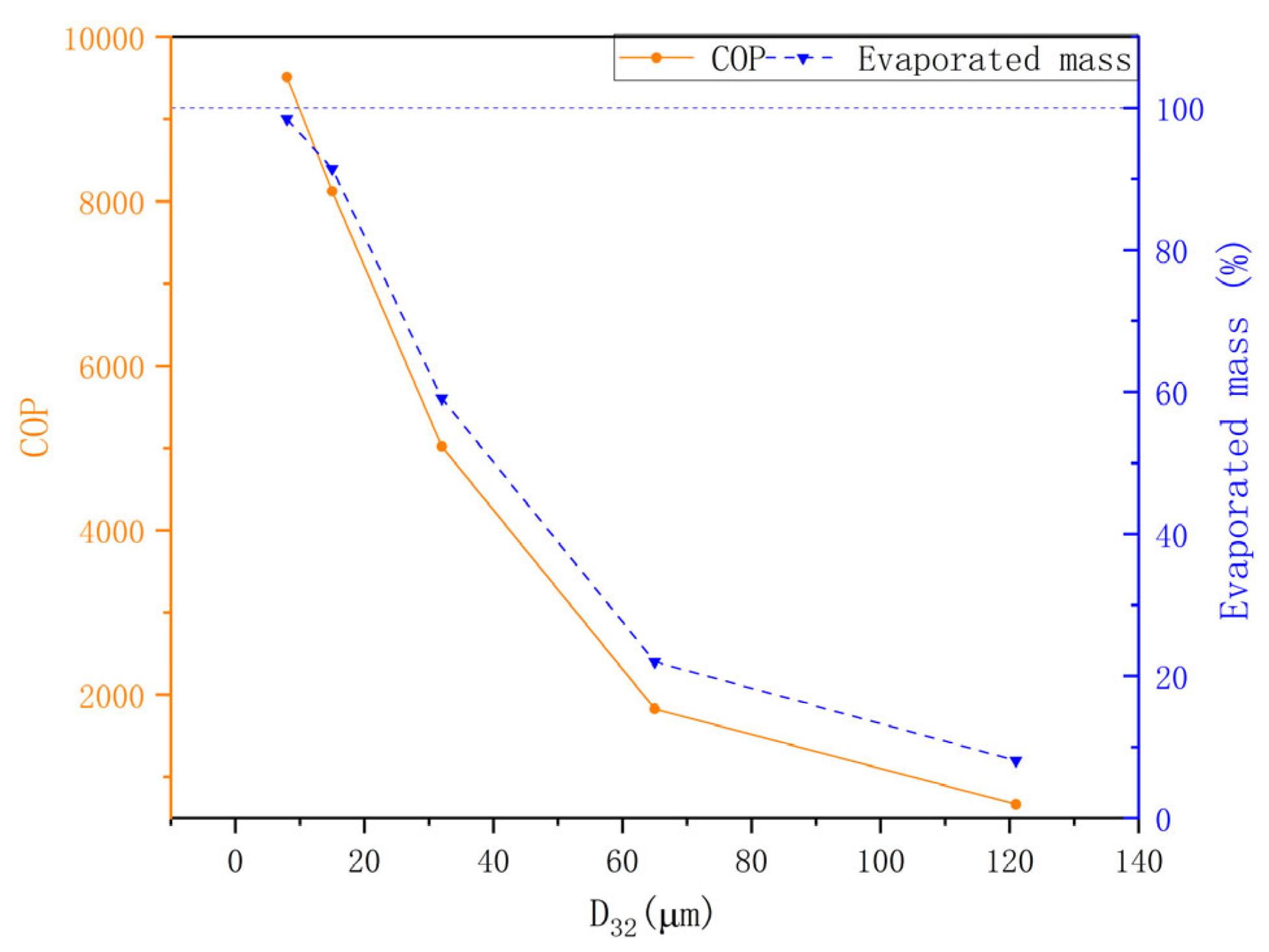
- Reducing droplet size also enhances the evaporation rate, with smaller droplets (D32 < 20 μm) exceeding 50% evaporation, and at 8 μm, achieving 100% evaporation. Additionally, smaller droplet sizes reduce water consumption, with D32 = 15 μm using just 3% of the original water volume. The analysis of the coefficient of performance (COP) reveals that smaller droplets and lower mass flow rates result in higher COP, as the efficiency of the whole system is improved.
- (6)
- It was demonstrated that both the evaporation rate and the coefficient of performance (COP) decrease as the size of the droplets increases. This reduction is attributable to the fact that larger droplet sizes reduce the overall surface area available for evaporation under a given water mass flow rate.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| A | Surface area | m2 |
| Bm | Spalding number | - |
| Turbulence viscosity coefficient | - | |
| Drag coefficient | - | |
| Cv | Nozzle velocity coefficient | - |
| Empirical coefficient | - | |
| Heat capacity | ||
| D | Diameter | |
| Mean diameter | ||
| Di,m | Diffusion coefficient | m2/s |
| G | Gravity term | N |
| g | Gravitational acceleration | m/s2 |
| h | Enthalpy | J/kg |
| I | Turbulent intensity | % |
| k | Turbulent kinetic energy | m2/s2 |
| kc | Mass transfer coefficient | - |
| LHV | Latent heat of water vaporization | kJ/kg |
| l | Turbulent length scale | M |
| m | Mass | Kg |
| Mass flow rate | kg/s | |
| Nu | Nusselt number | - |
| P | Pressure | Pa |
| Pk | Production of turbulent kinetic energy | kg/m·s3 |
| Pr | Prandtl number | - |
| Q | Enthalpy change | W |
| Re | Relative Reynolds number | - |
| Source term of energy | W/m3 | |
| Sm | Source term of mass | kg/m3·s |
| Source term of momentum | kg/(m2·s2) | |
| Source term for species transport | kg/m3·s | |
| Sc | Schmidt number | - |
| Sh | Sherwood number | - |
| T | Temperature | °C |
| Mean velocity vector | m/s | |
| U0 | Initial nozzle velocity | m/s |
| Volumetric flow rate | m3/s | |
| v | velocity | m/s |
| Mass fraction of species | - | |
| Vapour masa fraction at the surface | - | |
| Vapour masa fraction in the air | - | |
| Greek symbols | ||
| Half cone angle | ° | |
| Kronecker delta | - | |
| dissipation rate | m3/s3 | |
| Mixture thermal conductivity | ) | |
| Dynamic viscosity | ||
| Turbulent viscosity | ||
| Density | kg/m3 | |
| Turbulent dissipation rate | m2/s3 | |
| Dissipation rate | W/m3 | |
| Turbulent Prandtl number for | - | |
| Mass fraction | - | |
| Droplet surface tension | N/m | |
| Specific humidity | - | |
| Subscripts | ||
| a | Air | |
| COP | Coefficient of performance | |
| d | Droplet | |
| DBT | Dry bulb temperature | |
| i,j | Cartesian coordinate directions | |
| SEP | Surface energy per unit | |
| tur | Turbulent | |
| WBT | Wet bulb temperature |
Appendix A. Derivation of Surface Energy Power (SEP)
Appendix B. Sample Calculation for COP
References
- Cai, S.; Gou, Z. Towards Energy-Efficient Data Centers: A Comprehensive Review of Passive and Active Cooling Strategies. Energy Built Environ. 2024; in press. [Google Scholar] [CrossRef]
- Central Statistics Office. Key Findings. Data Centres Metered Electricity Consumption 2022. Available online: https://www.cso.ie/en/releasesandpublications/ep/p-dcmec/datacentresmeteredelectricityconsumption2022/keyfindings/ (accessed on 3 August 2024).
- IEA. International Energy Agency. Available online: https://www.iea.org (accessed on 3 August 2024).
- Du, S.; Zhang, Q.; Zou, S.; Meng, F.; Liu, L. Simulation Analysis on Energy Consumption and Economy of CPU Cooling System Based on Loop Heat Pipe for Data Center. Therm. Sci. Eng. Prog. 2023, 45, 102115. [Google Scholar] [CrossRef]
- Zhang, Z.; Wang, X.; Yan, Y. A Review of the State-of-the-Art in Electronic Cooling. E-Prime-Adv. Electr. Eng. Electron. Energy 2021, 1, 100009. [Google Scholar] [CrossRef]
- Kapilan, N.; Isloor, A.M.; Karinka, S. A Comprehensive Review on Evaporative Cooling Systems. Results Eng. 2023, 18, 101059. [Google Scholar] [CrossRef]
- McCay, O.; Nimmagadda, R.; Ali, S.M.; Persoons, T. A Parametric Design Study of Natural-Convection-Cooled Heat Sinks. Fluids 2023, 8, 234. [Google Scholar] [CrossRef]
- Zhang, Q.; Meng, Z.; Hong, X.; Zhan, Y.; Liu, J.; Dong, J.; Bai, T.; Niu, J.; Deen, M.J. A Survey on Data Center Cooling Systems: Technology, Power Consumption Modeling and Control Strategy Optimization. J. Syst. Archit. 2021, 119, 102253. [Google Scholar] [CrossRef]
- Zhang, H.; Shao, S.; Xu, H.; Zou, H.; Tian, C. Free Cooling of Data Centers: A Review. Renew. Sustain. Energy Rev. 2014, 35, 171–182. [Google Scholar] [CrossRef]
- Hnayno, M.; Chehade, A.; Klaba, H.; Polidori, G.; Maalouf, C. Experimental Investigation of a Data-Centre Cooling System Using a New Single-Phase Immersion/Liquid Technique. Case Stud. Therm. Eng. 2023, 45, 102925. [Google Scholar] [CrossRef]
- Bapu, B.R.R.; Kayalvizhi, S.; Murugavalli, S. Two Phase Cooling with Nano-Fluid for Highly Dense Electronic Systems-on-Chip—A Pilot Study. Microelectron. Reliab. 2020, 108, 113640. [Google Scholar] [CrossRef]
- Pearlmutter, D.; Erell, E.; Etzion, Y.; Meir, I.A.; Di, H. Refining the Use of Evaporation in an Experimental Down-Draft Cool Tower. Energy Build. 1996, 23, 191–197. [Google Scholar] [CrossRef]
- Shehabi, A.; Ganguly, S.; Gundel, L.A.; Horvath, A.; Kirchstetter, T.W.; Lunden, M.M.; Tschudi, W.; Gadgil, A.J.; Nazaroff, W.W. Can Combining Economizers with Improved Filtration Save Energy and Protect Equipment in Data Centers? Build. Environ. 2010, 45, 718–726. [Google Scholar] [CrossRef]
- Shehabi, A.; Horvath, A.; Tschudi, W.; Gadgil, A.J.; Nazaroff, W.W. Particle Concentrations in Data Centers. Atmos. Environ. 2008, 42, 5978–5990. [Google Scholar] [CrossRef]
- Bao, L.; Wang, J.; Kang, L. The Applied Effect Analysis of Heat Exchanger Installed in a Typical Communication Base Station in Beijing of China. Energy Procedia 2012, 14, 620–625. [Google Scholar] [CrossRef]
- Sureshkumar, R.; Kale, S.R.; Dhar, P.L. Heat and Mass Transfer Processes between a Water Spray and Ambient Air—I. Experimental Data. Appl. Therm. Eng. 2008, 28, 349–360. [Google Scholar] [CrossRef]
- Shao, S.; Liu, H.; Zhang, H.; Tian, C. Experimental Investigation on a Loop Thermosyphon with Evaporative Condenser for Free Cooling of Data Centers. Energy 2019, 185, 829–836. [Google Scholar] [CrossRef]
- Tissot, J.; Boulet, P.; Labergue, A.; Castanet, G.; Trinquet, F.; Fournaison, L. Experimental Study on Air Cooling by Spray in the Upstream Flow of a Heat Exchanger. Int. J. Therm. Sci. 2012, 60, 23–31. [Google Scholar] [CrossRef]
- Kachhwaha, S.S.; Dhar, P.L.; Kale, S.R. Experimental studies and numerical simulation of evaporative cooling of air with a water spray—I. Horizontal Parallel flow. Int. J. Heat Mass Transf. 1998, 41, 447–464. [Google Scholar] [CrossRef]
- Kachhwaha, S.S.; Dhar, P.L.; Kale, S.R. Experimental studies and numerical simulation of evaporative cooling of air with a water spray—II. Horizontal Counter Flow. Int. J. Heat Mass Transf. 1998, 41, 465–474. [Google Scholar] [CrossRef]
- Mao, R.; Wu, H.; Li, C.; Zhang, Z.; Liang, X.; Zhou, J.; Chen, J. Experimental Investigation on the Application of Cold-Mist Direct Evaporative Cooling in Data Centers. Int. J. Therm. Sci. 2025, 208, 109500. [Google Scholar] [CrossRef]
- Zhang, Y.; Chen, Y.; Yang, H.; Zhang, H.; Leung, C.W. Experimental Performance Investigation on a Desiccant-Assisted Two-Stage Evaporative Cooling System in Hot and Humid Areas. Appl. Energy 2025, 377, 124704. [Google Scholar] [CrossRef]
- Zhang, Y.; Han, H.; Zhang, Y.; Li, J.; Zheng, Y.; Liu, C.; Liu, Y.; Gao, D. Experimental Study on the Performance of a Novel Data Center Air Conditioner Combining Air Cooling and Evaporative Cooling. Int. J. Refrig. 2024; in press. [Google Scholar] [CrossRef]
- Montazeri, H.; Blocken, B.; Hensen, J.L.M. Evaporative Cooling by Water Spray Systems: CFD Simulation, Experimental Validation and Sensitivity Analysis. Build. Environ. 2015, 83, 129–141. [Google Scholar] [CrossRef]
- Montazeri, H.; Blocken, B.; Hensen, J.L.M. CFD Analysis of the Impact of Physical Parameters on Evaporative Cooling by a Mist Spray System. Appl. Therm. Eng. 2015, 75, 608–622. [Google Scholar] [CrossRef]
- Alkhedhair, A.; Gurgenci, H.; Jahn, I.; Guan, Z.; He, S. Numerical Simulation of Water Spray for Pre-Cooling of Inlet Air in Natural Draft Dry Cooling Towers. Appl. Therm. Eng. 2013, 61, 416–424. [Google Scholar] [CrossRef]
- Alkhedhair, A.; Jahn, I.; Gurgenci, H.; Guan, Z.; He, S.; Lu, Y. Numerical Simulation of Water Spray in Natural Draft Dry Cooling Towers with a New Nozzle Representation Approach. Appl. Therm. Eng. 2016, 98, 924–935. [Google Scholar] [CrossRef]
- Raoult, F.; Lacour, S.; Carissimo, B.; Trinquet, F.; Delahaye, A.; Fournaison, L. CFD Water Spray Model Development and Physical Parameter Study on the Evaporative Cooling. Appl. Therm. Eng. 2019, 149, 960–974. [Google Scholar] [CrossRef]
- Sureshkumar, R.; Kale, S.R.; Dhar, P.L. Heat and Mass Transfer Processes between a Water Spray and Ambient Air—II. Simulations. Appl. Therm. Eng. 2008, 28, 361–371. [Google Scholar] [CrossRef]
- Tissot, J.; Boulet, P.; Trinquet, F.; Fournaison, L.; Macchi-Tejeda, H. Air Cooling by Evaporating Droplets in the Upward Flow of a Condenser. Int. J. Therm. Sci. 2011, 50, 2122–2131. [Google Scholar] [CrossRef]
- Peng, D.; Li, Y.; Cheng, N. Study on the Performance of Tube Evaporative Cooling Dehumidifier Based on CFD. Appl. Therm. Eng. 2024, 241, 122419. [Google Scholar] [CrossRef]
- Climate & Weather Averages in Dublin, Ireland. Available online: https://www.timeanddate.com/weather/ireland/dublin/climate (accessed on 21 October 2024).
- Li, J.; Kawano, H. Simulating Water-Drop Movement from Noncircular Sprinkler Nozzles. J. Irrig. Drain. Eng. 1995, 121, 152–158. [Google Scholar] [CrossRef]
- Morsi, S.A.; Alexander, A.J. An Investigation of Particle Trajectories in Two-Phase Flow Systems. J. Fluid Mech. 1972, 55, 193–208. [Google Scholar] [CrossRef]
- Sazhin, S.S. Advanced Models of Fuel Droplet Heating and Evaporation. Prog. Energy Combust. Sci. 2006, 32, 162–214. [Google Scholar] [CrossRef]
- Miller, R.S.; Harstad, K.; Bellan, J. Evaluation of equilibrium and non-equilibrium evaporation models for many-droplet gas-liquid flow simulations. Int. J. Multiph. Flow 1998, 24, 1025–1055. [Google Scholar] [CrossRef]
- ANSYS, version 2023. ANSYS Fluent Theory Guide. Ansys Inc.: Canonsburg, PA, USA, 2023.
- Babinsky, E.; Sojka, P.E. Modeling Drop Size Distributions. Prog. Energy Combust. Sci. 2002, 28, 303–329. [Google Scholar] [CrossRef]
- Alderliesten, M. Mean Particle Diameters. Part VII. The Rosin-Rammler Size Distribution: Physical and Mathematical Properties and Relationships to Moment-Ratio Defined Mean Particle Diameters. Part. Part. Syst. Charact. 2013, 30, 244–257. [Google Scholar] [CrossRef]
- Ashgriz, N. (Ed.) Handbook of Atomization and Sprays: Theory and Applications; Springer: Boston, MA, USA, 2011. [Google Scholar] [CrossRef]
- Center for Sustainability and the Global Environment. Average Annual Relative Humidity. Available online: https://sage.nelson.wisc.edu/data-and-models/atlas-of-the-biosphere/mapping-the-biosphere/ecosystems/average-annual-relative-humidity/ (accessed on 30 July 2024).

| K1 | K2 | K3 | Re |
|---|---|---|---|
| 24 | 0 | 0 | Re < 0.1 |
| 22.73 | 0.0903 | 3.69 | 0.1 < Re < 1 |
| 29.1667 | −3.8889 | 1.222 | 1 < Re < 10 |
| 46.5 | −116.67 | 0.6167 | 10 < Re < 100 |
| 98.33 | −2778 | 0.3644 | 100 < Re < 1000 |
| 148.62 | −4.75 × 104 | 0.357 | 1000 < Re < 5000 |
| −490.546 | 5.787 × 105 | 0.46 | 5000 < Re < 10,000 |
| −1662.5 | 5.4167 × 106 | 0.5191 | 10,000 < Re < 50,000 |
| Configuration | |
|---|---|
| Convergence criteria (residuals) | 10−6 |
| Pressure-velocity coupling | PISO |
| Order of numerical schemes | Second order |
| Spatial gradient discretization | Least squares cell based |
| Time step size | 0.001 s |
| Iterations per time step | 20 |
| Relaxation coefficients (for pressure, density, body force, momentum, turbulent kinetic energy, turbulent dissipation rate, turbulent viscosity, H2O, energy, discrete phase source, respectively) | (0.3, 1, 1, 0.7, 0.8, 0.8, 1, 1, 1, 0.9) |
| Grid | Number of Elements | Average Outlet Temperature (°C) | Relative Change in (%) | Computational Time (h) |
|---|---|---|---|---|
| M1 | 128,000 | 30.6448 | - | 6 |
| M2 | 302,500 | 30.6973 | 0.55 | 12 |
| M3 | 612,500 | 30.7579 | 0.38 | 20 |
| M4 | 1,083,750 | 30.8077 | 0.18 | 26 |
| M5 | 1,800,000 | 30.8128 | 0.019 | 35 |
| M6 | 2,420,000 | 30.8137 | 0.003 | 43 |
| Air | Water | Nozzle | |||||
|---|---|---|---|---|---|---|---|
| DBT (°C) | va (m/s) | P (bar) | (kg/s) | Tw (°C) | D (mm) | (°) | |
| 39.2 | 3 | 0.0052 | 3 | 0.21 | 35.2 | 4 | 18 |
| Design Point | D32 (μm) | Mass Flow Rate × 10−3 (kg/s) | Evaporated Mass Flow Rate (%) | SEP (W) | Q (W) | (W) |
|---|---|---|---|---|---|---|
| D1 | 292 | 420 | 0.73 | 0.5926 | 7737.9 | 124.8 |
| D2 (O) | 292 | 210 | 1.10 | 0.2963 | 5572.8 | 62.4 |
| D3 | 292 | 100 | 1.44 | 0.1479 | 3656.0 | 31.1 |
| D4 | 121 | 52 | 2.63 | 0.1836 | 3351.2 | 15.6 |
| D5 | 121 | 26 | 3.44 | 0.0918 | 2187.7 | 7.8 |
| D6 | 121 | 13 | 4.47 | 0.0459 | 1422.3 | 3.9 |
| D6 | 65 | 13 | 5.67 | 0.0791 | 1804.6 | 3.9 |
| D7 | 65 | 6.4 | 7.43 | 0.0395 | 1163.1 | 1.9 |
| D8 | 65 | 3.2 | 9.80 | 0.0198 | 767.3 | 0.96 |
| D8 | 32 | 3.2 | 13.75 | 0.0397 | 1076.4 | 0.96 |
| D9 | 32 | 1.6 | 19.67 | 0.0199 | 770.0 | 0.48 |
| D10 | 32 | 0.8 | 27.46 | 0.0099 | 537.4 | 0.24 |
| D11 | 15 | 0.4 | 52.86 | 0.0101 | 517.2 | 0.12 |
| D12 | 15 | 0.2 | 74.00 | 0.0050 | 362.0 | 0.06 |
| D13 | 15 | 0.1 | 91.45 | 0.0025 | 223.7 | 0.03 |
| D13 (OP) | 8 | 0.1 | 98.44 | 0.0047 | 240.8 | 0.03 |
| D14 | 8 | 0.05 | 99.87 | 0.0024 | 122.2 | 0.015 |
| D15 | 8 | 0.025 | 100 | 0.0012 | 61.2 | 0.0075 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gao, N.; Ali, S.M.; Persoons, T. On the Numerical Investigation of Two-Phase Evaporative Spray Cooling Technology for Data Centre Applications. Fluids 2024, 9, 284. https://doi.org/10.3390/fluids9120284
Gao N, Ali SM, Persoons T. On the Numerical Investigation of Two-Phase Evaporative Spray Cooling Technology for Data Centre Applications. Fluids. 2024; 9(12):284. https://doi.org/10.3390/fluids9120284
Chicago/Turabian StyleGao, Ning, Syed Mughees Ali, and Tim Persoons. 2024. "On the Numerical Investigation of Two-Phase Evaporative Spray Cooling Technology for Data Centre Applications" Fluids 9, no. 12: 284. https://doi.org/10.3390/fluids9120284
APA StyleGao, N., Ali, S. M., & Persoons, T. (2024). On the Numerical Investigation of Two-Phase Evaporative Spray Cooling Technology for Data Centre Applications. Fluids, 9(12), 284. https://doi.org/10.3390/fluids9120284






