Multiple Steady States in Laminar Rayleigh–Bénard Convection of Air
Abstract
:1. Introduction
2. Governing Equations
3. Numerical Setup
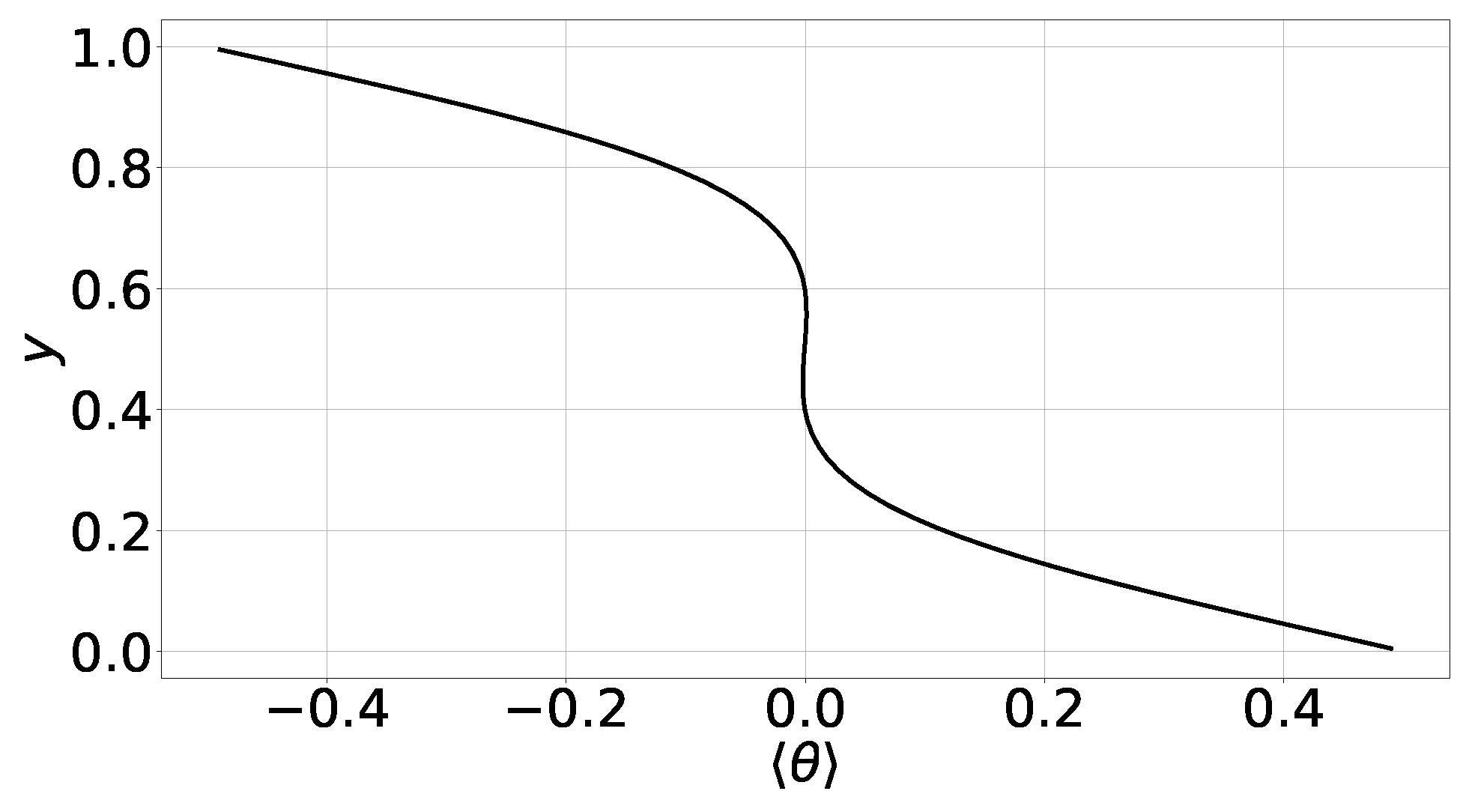
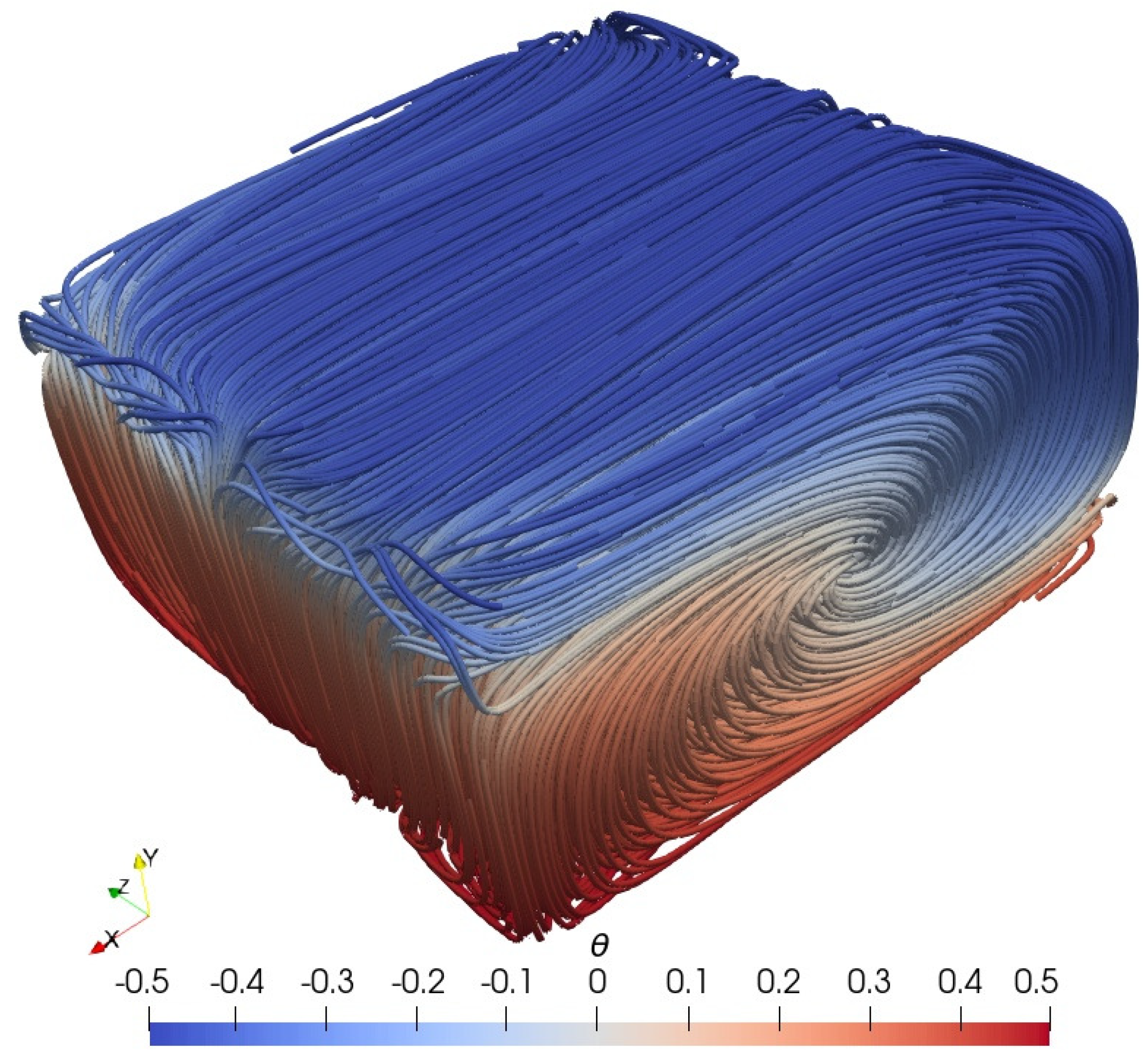
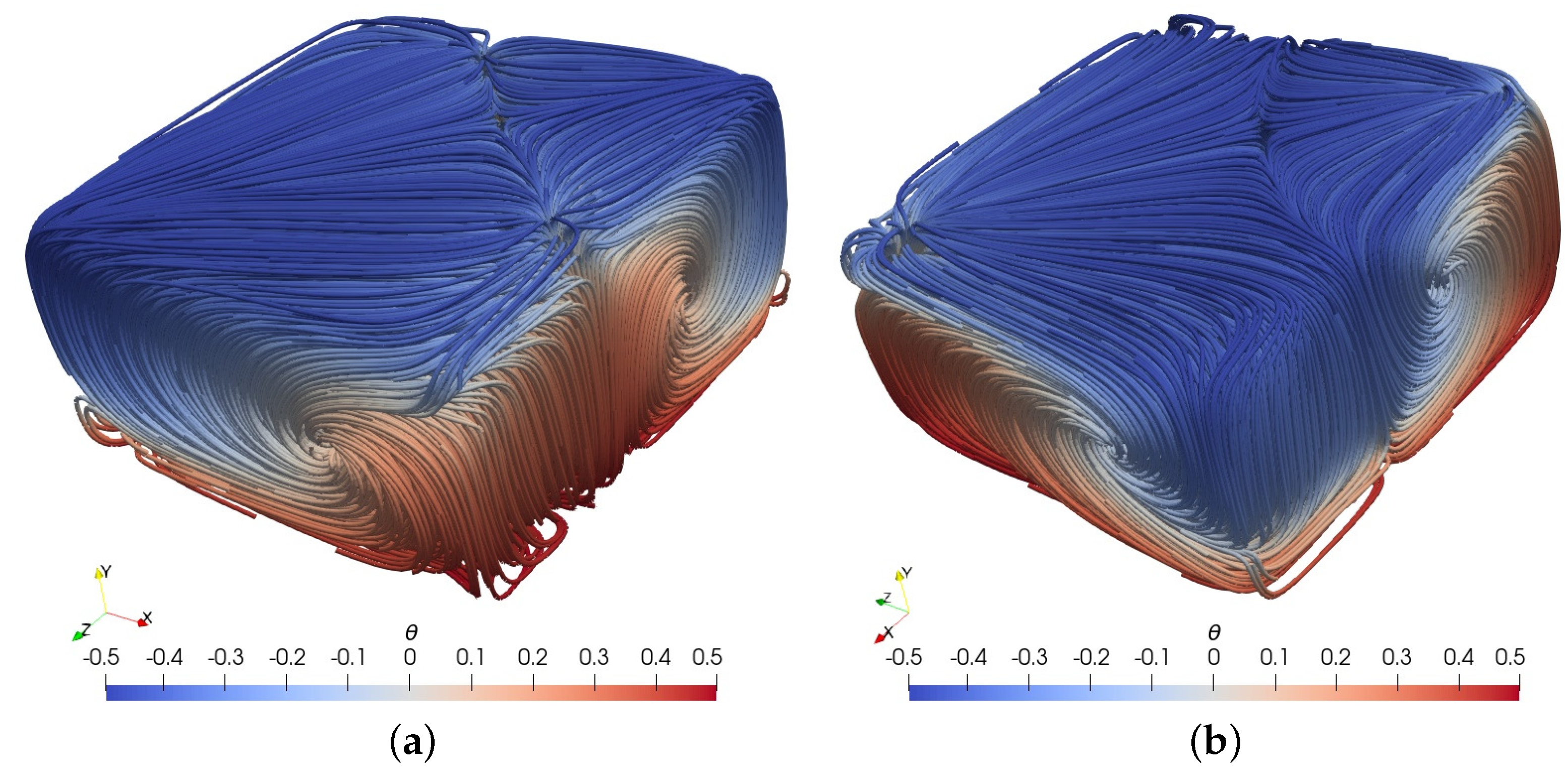
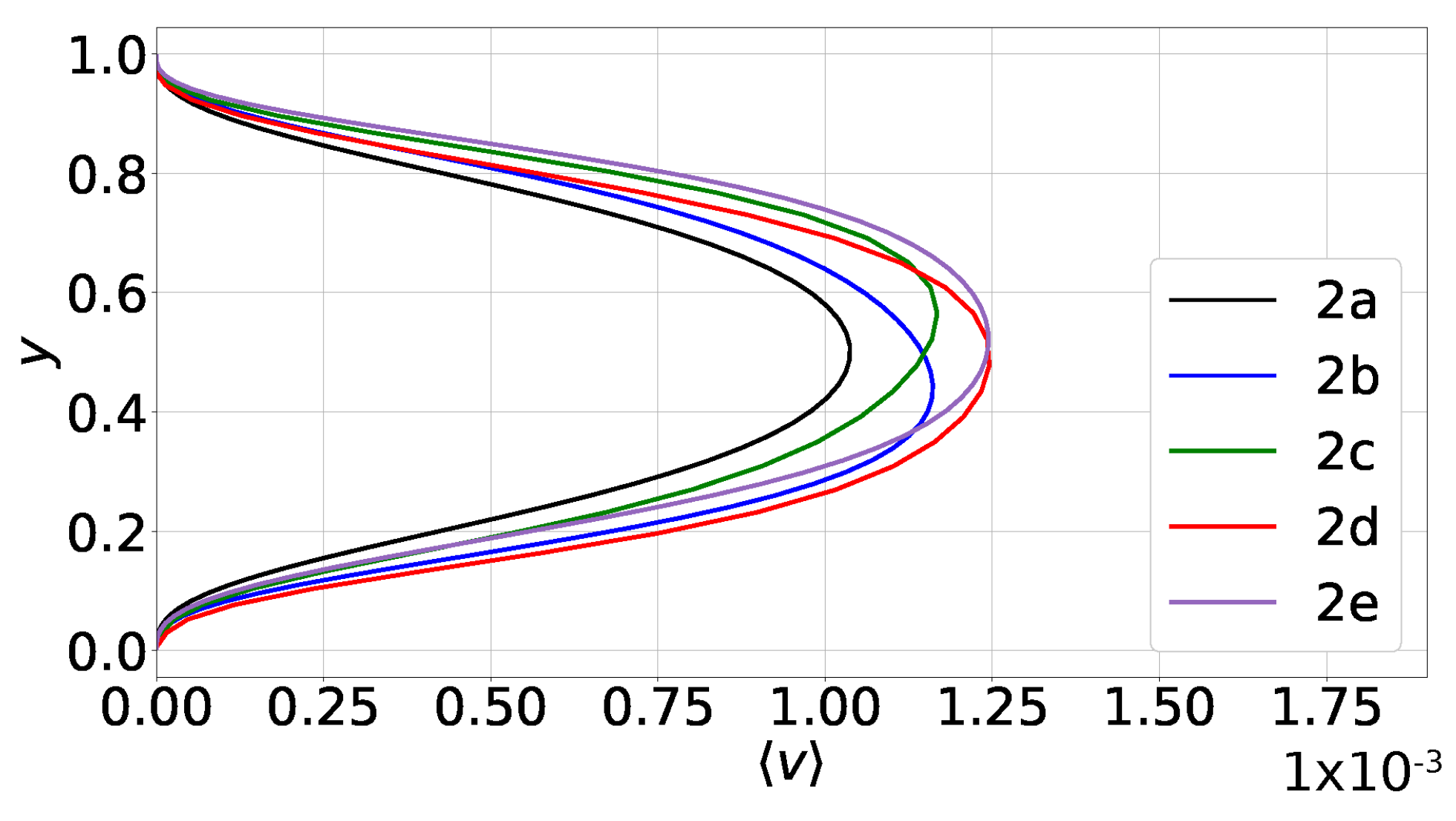
4. Results and Discussion
4.1. Case 1: Aspect Ratio
4.2. Case 2: Aspect Ratio = 2
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Chillà, F.; Schumacher, J. New perspectives in turbulent Rayleigh-Bénard convection. Eur. Phys. J. E 2012, 35, 58. [Google Scholar] [CrossRef] [PubMed]
- Wagner, S.; Shishkina, O. Aspect-ratio dependency of Rayleigh-Bénard convection in box-shaped containers. Phys. Fluids 2013, 25, 085110. [Google Scholar] [CrossRef]
- Yigit, S.; Poole, R.J.; Chakraborty, N. Effects of aspect ratio on laminar Rayleigh-Bénard convection of power-law fluids in rectangular enclosures: A numerical investigation. Int. J. Heat Mass Tran. 2015, 91, 1292–1307. [Google Scholar] [CrossRef]
- Wang, Q.; Verzicco, R.; Lohse, D.; Shishkina, O. Multiple states in turbulent large-aspect-ratio thermal convection: What determines the number of convection rolls? Phys. Rev. Lett. 2020, 125, 074501. [Google Scholar] [CrossRef] [PubMed]
- Gelfgat, A.Y. Different modes of Rayleigh-Bénard instability in two- and three-dimensional rectangular enclosures. J. Comput. Phys. 1999, 156, 300–324. [Google Scholar] [CrossRef]
- Hof, B.; Lucas, P.G.J.; Mullin, T. Flow state multiplicy in convection. Phys. Fluids 1999, 11, 2815–2817. [Google Scholar] [CrossRef]
- Ma, D.-J.; Sun, D.-J.; Yin, X.-Y. Multiplicty of steady states in cylindrical Rayleigh-Bénard convection. Phys. Rev. E 2010, 74, 037302. [Google Scholar] [CrossRef]
- Borońska, K.; Tuckerman, L.S. Extreme multiplicity in cylindrical Rayleigh-Bénard convection. II. Bifurcation diagram and symmetry classification. Phys. Rev. E 2010, 81, 036321. [Google Scholar] [CrossRef]
- Venturi, D.; Wan, X.; Karniadakis, G.E. Stochastic bifurcation analysis of Rayleigh-Bénard convetion. J. Fluid Mech. 2010, 650, 391–413. [Google Scholar] [CrossRef]
- Silano, G.; Sreenivasan, X.R.; Verzicco, R. Numerical simulations of Rayleigh-Bénard convection for Prandtl numbers between 10−1 and 104 and Rayleigh numbers between 105 and 109. J. Fluid Mech. 2010, 662, 409–446. [Google Scholar] [CrossRef]
- Erenburg, V.; Gelfgat, A.Y.; Kit, E.; Bar-Yoseph, P.Z.; Solan, A. Multiple states, stability and bifurcations of natural convection in a rectangular cavity with partially heated vertical walls. J. Fluid Mech. 2003, 492, 63–89. [Google Scholar] [CrossRef]
- Yigit, S.; Poole, R.J.; Chakraborty, N. Effects of aspect ratio on natural convection of Bingham fluids in rectangular enclosures with differentially heated horizontal walls heated from below. Int. J. Heat Mass Tran. 2015, 80, 727–736. [Google Scholar] [CrossRef]
- Shishkina, O. Rayleigh-Bénard convection: The container shape matters. Phys. Rev. Fluids 2021, 6, 090502. [Google Scholar] [CrossRef]
- Paolucci, S. Filtering of sound from the Navier–Stokes equations. Tech. Rep. SAND 1982, SAND, 82–8257. [Google Scholar]
- Lessani, B.; Papalexandris, M.V. Time-accurate calculation of variable density flows with strong temperature gradients and combustion. J. Comput. Phys. 2006, 212, 218–246. [Google Scholar] [CrossRef]
- Hong, W.; Walker, D.T. Reynolds-averaged equations for free-surface flows with application to high-Froude-number jet spreading. J. Fluid Mech. 2000, 417, 183–209. [Google Scholar] [CrossRef]
- Antoniadis, P.D.; Papalexandris, M.V. Numerical study of unsteady, thermally-stratified shear flows in superposed porous and pure-fluid domains. Int. J. Heat Mass Tran. 2016, 96, 643–659. [Google Scholar] [CrossRef]
- Papalexandris, M.V. On the applicability of Stokes’ hypothesis to low-Mach-number flows. Contin. Mech. Therm. 2020, 32, 1245–1249. [Google Scholar] [CrossRef]
- Rhie, C.M.; Chow, W.L. Numerical study of the turburlent flow past an airfoil with trailing edge separation. AIAA J. 1983, 21, 1525–1532. [Google Scholar] [CrossRef]
- Balay, S.; Abhyankar, S.; Adams, M.; Brown, J.; Brune, P.; Buschelman, K.; Dalcin, L.; Dener, A.; Eijkhout, V.; Gropp, W.; et al. PETSc Users Manual. Technical Report ANL-95/11-Revision 3.11, Argonne National Laboratory; Argonne National Lab. (ANL): Argonne, IL, USA, 2019. [Google Scholar]
- Georgiou, M.; Papalexandris, M.V. Numerical study of turbulent flow in a rectangular T-junction. Phys. Fluids 2017, 29, 065106. [Google Scholar] [CrossRef]
- Georgiou, M.; Papalexandris, M.V. Direct numerical simulation of turbulent heat transfer in a T-junction. J. Fluid Mech. 2018, 845, 581–614. [Google Scholar] [CrossRef]
- Marichal, J.; Papalexandris, M.V. On the dynamics of the large scale circulation in turbulent convection with a free-slip upper boundary. Int. J. Heat Mass Tran. 2021, 183, 122220. [Google Scholar] [CrossRef]
- Shishkina, O.; Stevens, R.; Grossmann, S.; Lohse, D. Boundary layer structure in turbulent thermal convection and its consequences for the required numerical resolution. New J. Phys. 2010, 12, 075022. [Google Scholar] [CrossRef]
- Grötzbach, G. Spatial resolution requirements for direct numerical simulation of the Rayleigh-Bénard convection. J. Comput. Phys. 1983, 49, 214–264. [Google Scholar] [CrossRef]
- Stevens, R.; Verzicco, R.; Lohse, D. Radial boundary layer structure and Nusselt number in Rayleigh-Bénard convection. J. Fluid Mech. 2010, 643, 495–507. [Google Scholar] [CrossRef]
- Horn, S.; Shishkina, O.; Wagner, C. On non-Oberbeck-Boussinesq effects in three-dimensional Rayleigh-Bénard convection in glycerol. J. Fluid Mech. 2013, 724, 175–202. [Google Scholar] [CrossRef]






Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Carlier, J.; Papalexandris, M.V. Multiple Steady States in Laminar Rayleigh–Bénard Convection of Air. Fluids 2024, 9, 7. https://doi.org/10.3390/fluids9010007
Carlier J, Papalexandris MV. Multiple Steady States in Laminar Rayleigh–Bénard Convection of Air. Fluids. 2024; 9(1):7. https://doi.org/10.3390/fluids9010007
Chicago/Turabian StyleCarlier, Julien, and Miltiadis V. Papalexandris. 2024. "Multiple Steady States in Laminar Rayleigh–Bénard Convection of Air" Fluids 9, no. 1: 7. https://doi.org/10.3390/fluids9010007
APA StyleCarlier, J., & Papalexandris, M. V. (2024). Multiple Steady States in Laminar Rayleigh–Bénard Convection of Air. Fluids, 9(1), 7. https://doi.org/10.3390/fluids9010007






