Modelling of Peristaltic Pumps with Respect to Viscoelastic Tube Material Properties and Fatigue Effects
Abstract
:1. Introduction
1.1. General Function Principle of Peristaltic Pumps
1.2. General Setup for Current Study
1.3. Problem Definition
1.4. Numerical Modelling of Peristaltic Pumps
1.5. The 1D Peristaltic Pump Solver
- Experimentally derived material properties (describing viscoelastic behavior, fatigue, stiffness, and pressure sensitivity).
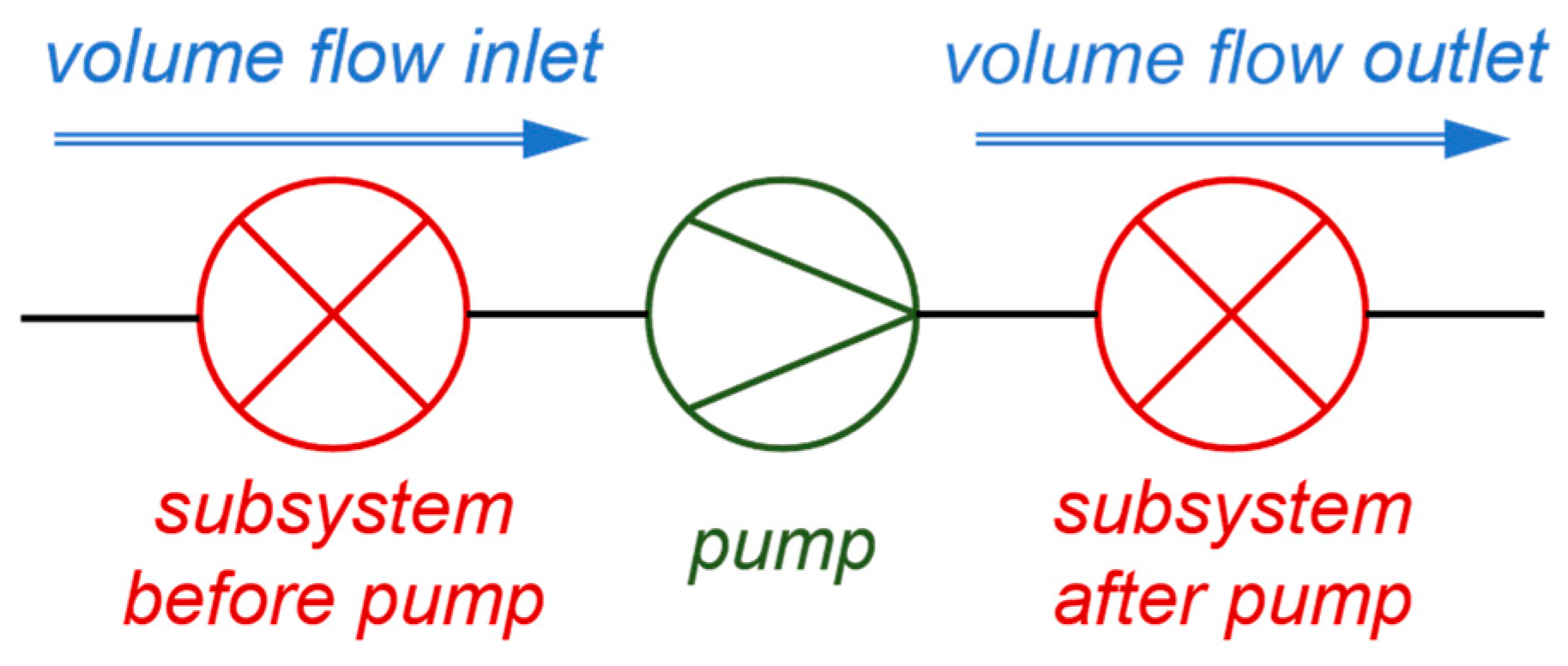
- Hydraulic characteristics of the upstream- and downstream subsystems.
- Frequency of the pump cycle.
2. Methodology
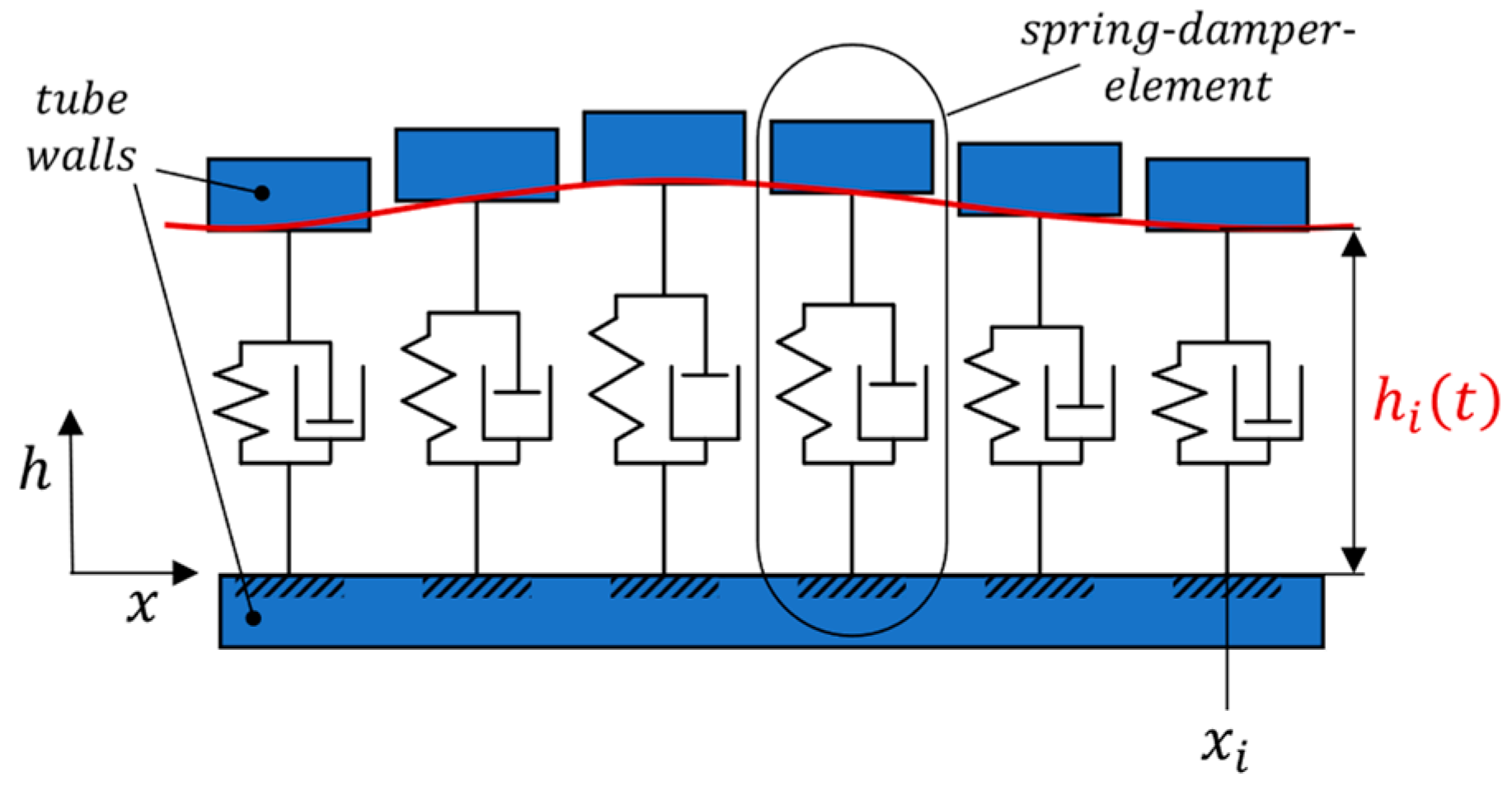
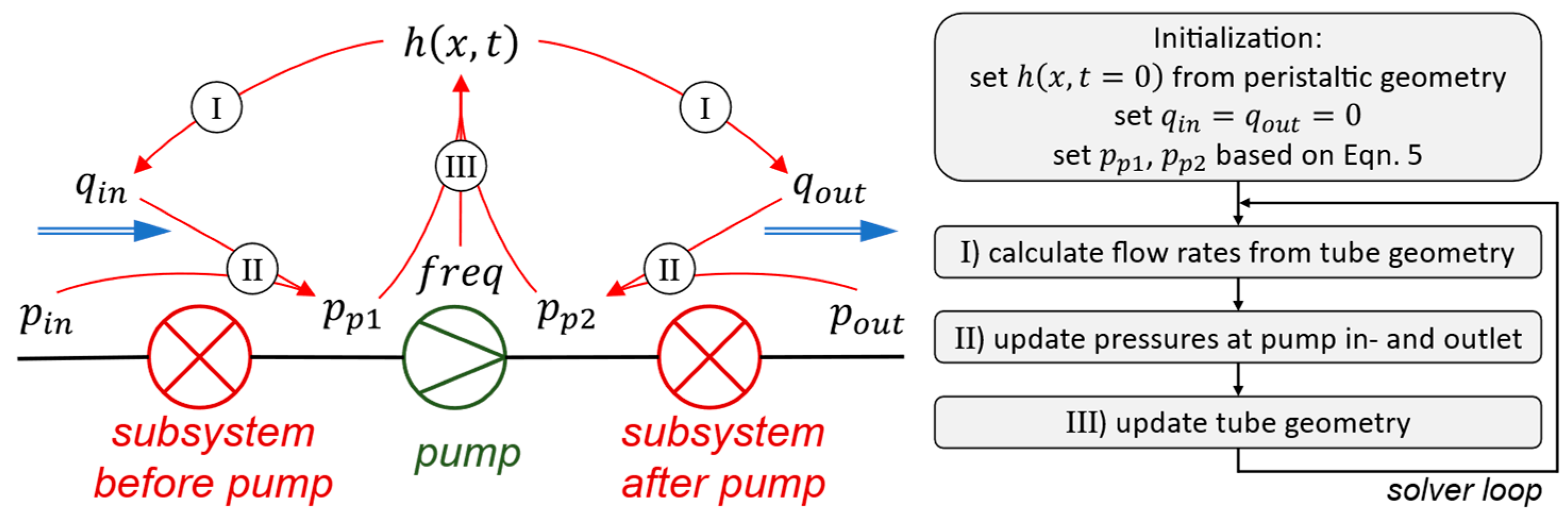
2.1. Physics and Logic behind the 1D Peristaltic Pump Solver
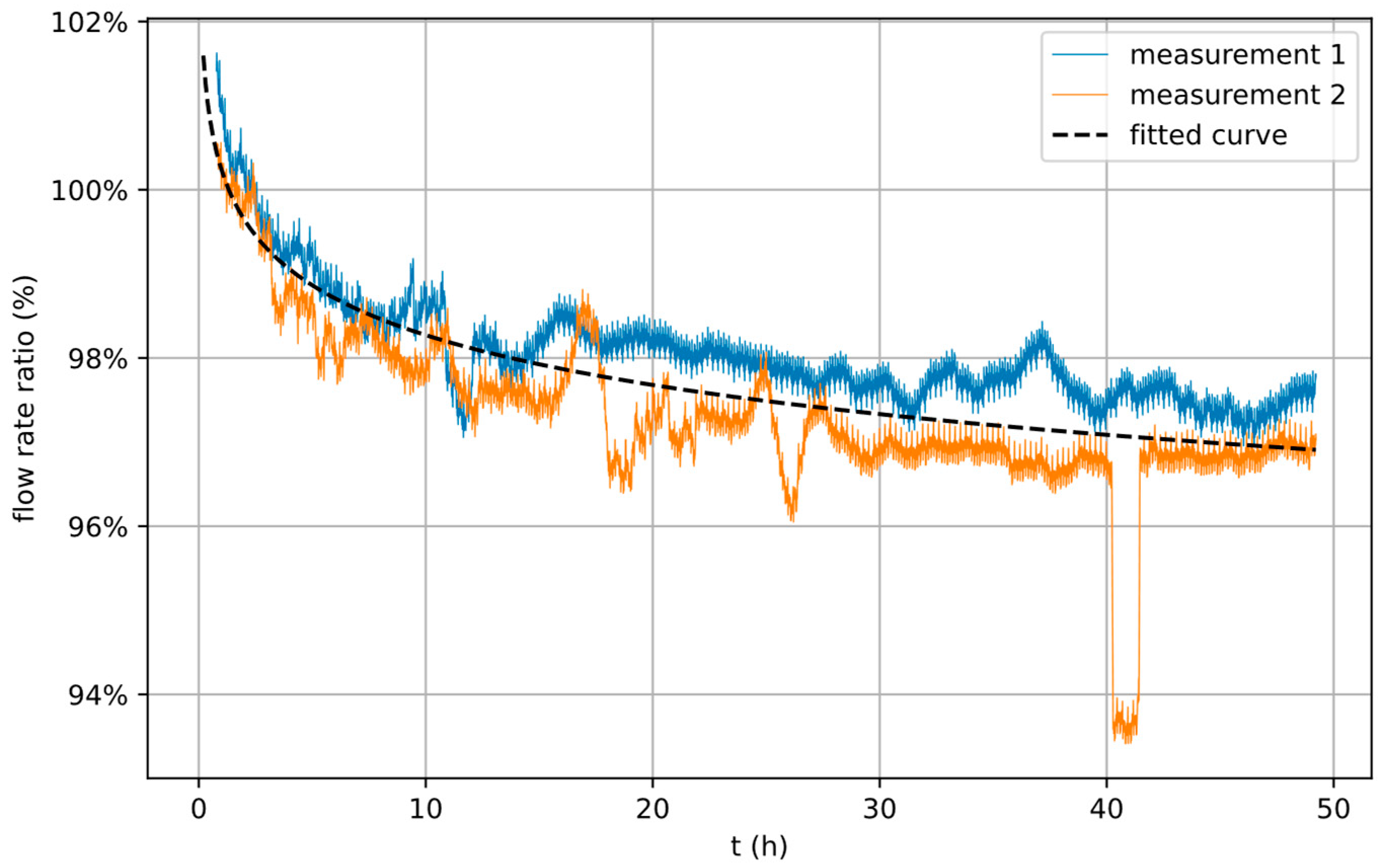
2.2. Experimental Determination of Material Properties
2.3. Implementation of the 1D Peristaltic Pump Solver
- (I)
- The inlet and outlet flow rates and as well as their rates of change follow from the geometric constraints of the tube geometry (Equations (2) and (4))
- (II)
- The pump inlet and outlet pressures and can then be calculated via Equation (5), applied to both subsystems. Therefore, the rate of change in the flow rates over the corresponding subsystem as well as appropriate system boundary conditions need to be inserted. The boundary conditions are determined by system parameters, namely:
- Inlet and outlet pressures, and (e.g., both equal to the environmental pressure).
- Difference in geodetic height within subsystems.
- Pressure loss function for the respective subsystem.
- (III)
- The tube height is then updated for the next time step, taking into account the outward motion of the actuators and the material-dependent spring-damping properties of the tube via Equation (3).
3. Results
3.1. Validation Procedure
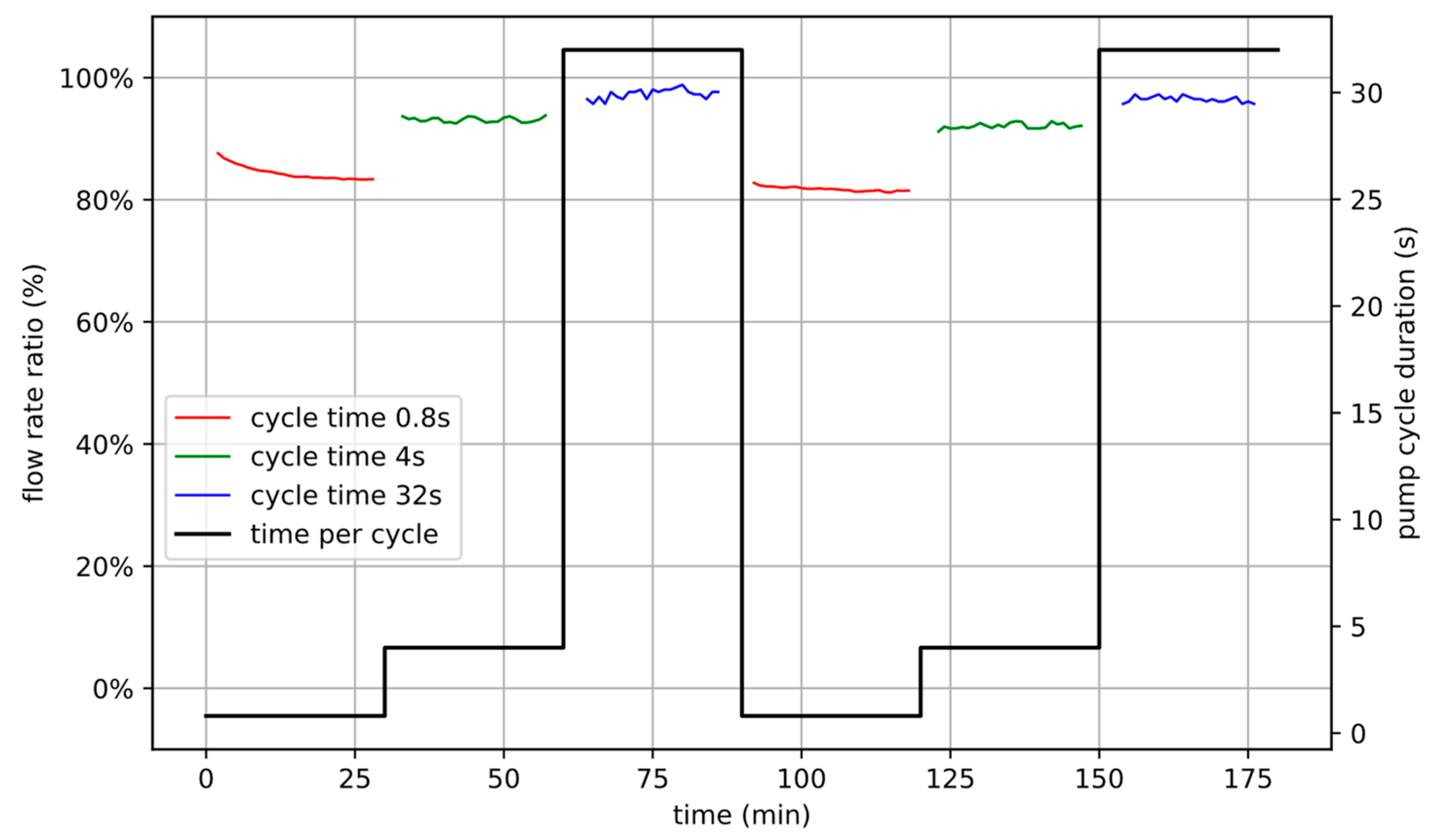
- High pump rate with of 0.8 s.
- Medium pump rate with of 4 s.
- Low pump rate with of 32 s.
3.2. Measurement Setup
3.3. Qualitative Results
3.4. Quantitative Results
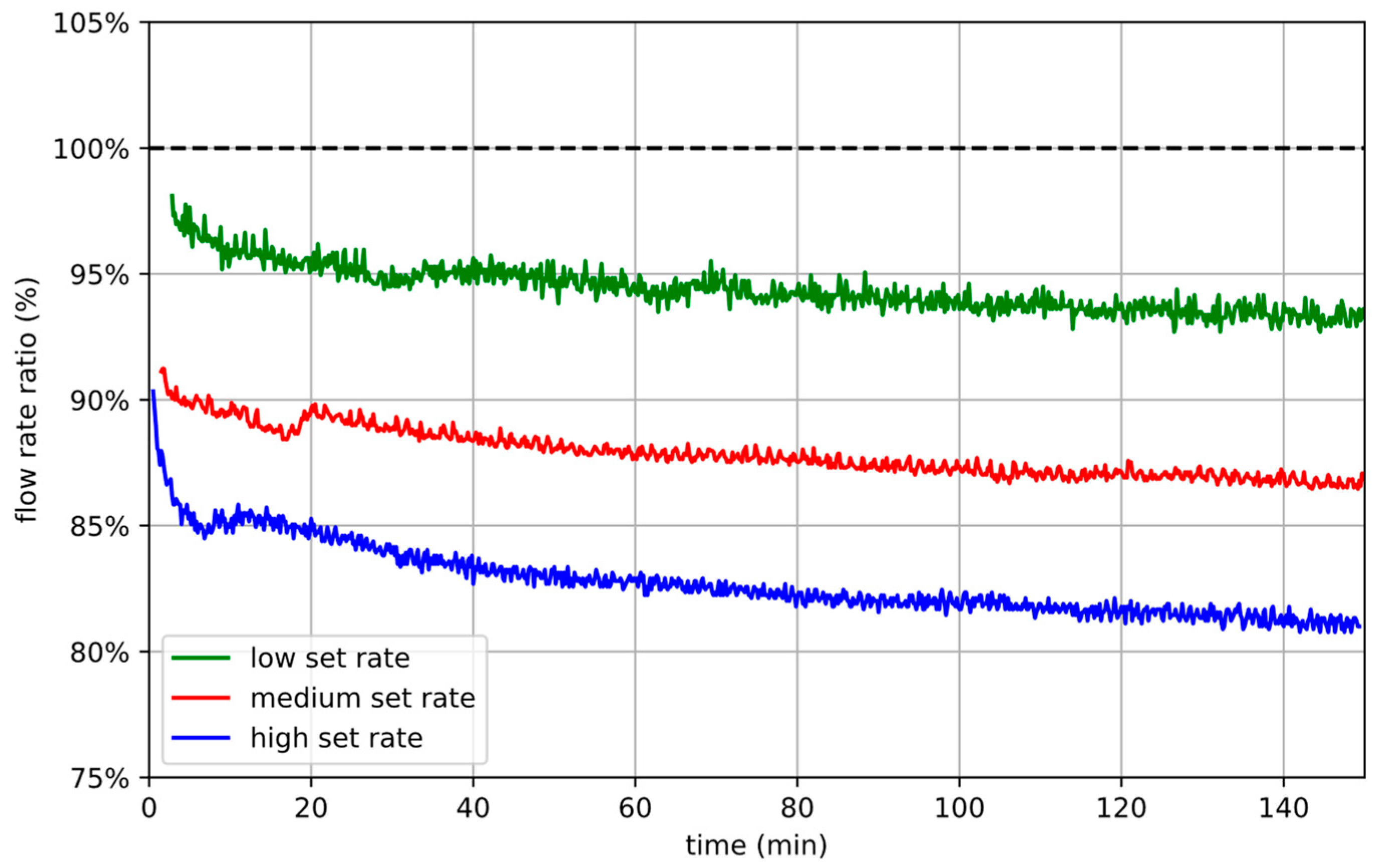
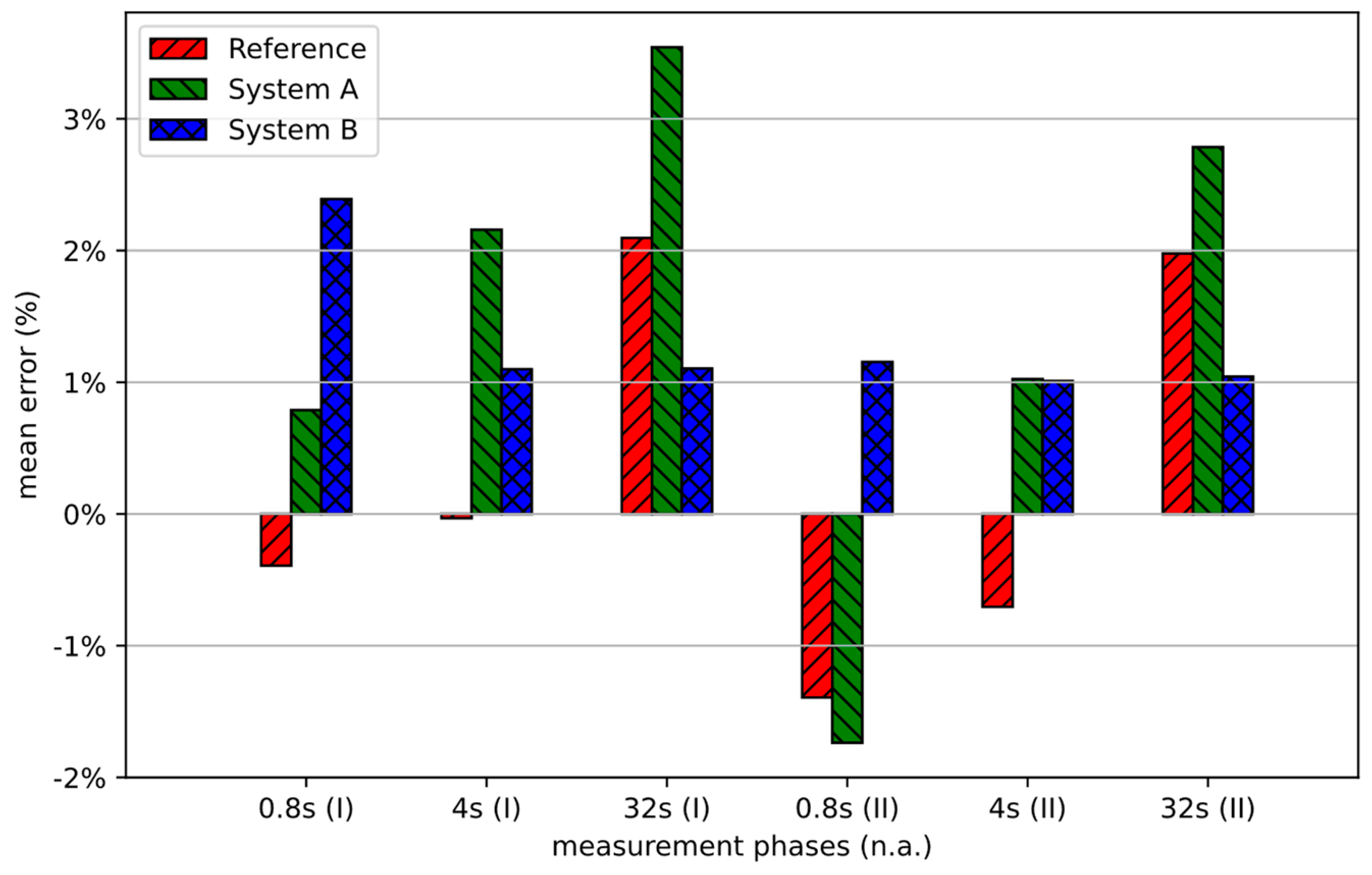
- Overall Accuracy: The largest observed relative deviation amounts to −3.5% (phase 32 s(I), System A), compared to an estimated accuracy of measurements of +/−2.3%. This speaks for the high degree of overall accuracy of the applied predictive method as well as for the general validity of the scheme. The presented investigations cover a wide parameter window, as follows:
- Three different pump rates (indicated as 0.8 s, 4 s, and 32 s cycle times).
- Three different hydraulic subsystem setups (Reference System, System A, and System B).
- Two material types (reference material: Reference System and System A vs. alternative material: System B).
- Material fatigue effects at different operating times (initial vs. latter experimental phases, indicated as (I) and (II), respectively).
- Impact of Pump Rate: As a general tendency, the solver appears to show a positive result deviation correlation to the applied pump cycle time. While no clear over- or underestimation of the flow rate ratio occurs with low cycle times (see phases with a cycle time of 0.8), a more pronounced overestimation of the flow rate ratios becomes apparent with increasing cycle times (see phases with a cycle time of 32 s).
- Impact of Fatigue Effect: The tube material fatigue effect can best be observed by comparing experimental phases 0.8 s(I) vs. 0.8 s(II), 4 s(I) vs. 4 s(II), and 32 s(I) vs. 32 s(II). As a general tendency, the solver appears to show a slight positive deviation correlation to progressing fatigue. The longer the actual tube material is exposed to application conditions, the smaller the underestimation and the higher the overestimation of the flow rate ratios becomes.
- Material Effect: As a general tendency, the described impact of both pump rate and fatigue effect on solver accuracy appears somewhat more pronounced for the model of the reference material (Reference System and System A) than for the model of the alternative tube material (System B). However, general qualitative tendencies correspond for both material model types.
4. Conclusions and Outlook
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| Expected flow rate in | |
| Duration of one pump cycle in | |
| Encapsulated liquid volume in the peristalsis in | |
| Time in | |
| Coordinate along the axis of the tube in | |
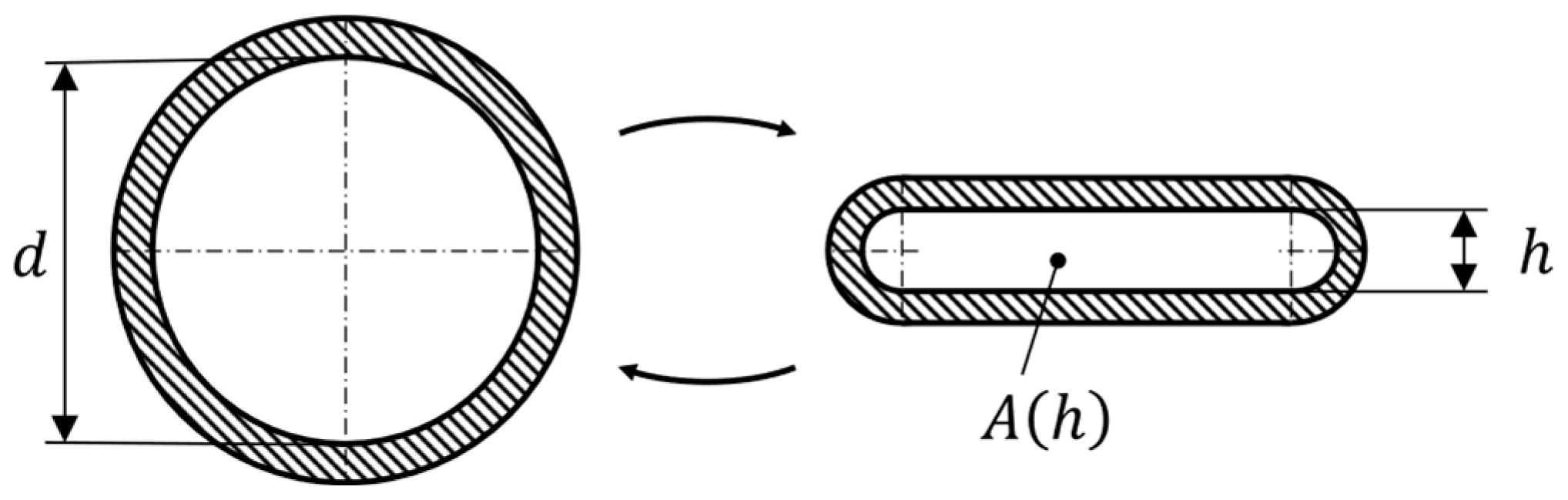
| Cross-sectional area of the tube in | |
| Diameter of the tube in | |
| Height of the (deformed) tube in | |
| Density of the liquid in | |
| Length of a tube segment | |
| Coefficient for the elastic behavior of the tube in | |
| Coefficient for the viscous behavior of the tube in | |
| Coefficient for the pressure dependency of the tube in | |
| Coefficient for the stiffness of the tube in | |
| Pressure in the tube in | |
| Environment pressure in | |
| Desired height of the tube considering the material-fatigue in | |
| Flow rate upstream of the pump in | |
| Flow rate downstream of the pump in | |
| Liquid volume upstream of the closure in the pump in | |
| Liquid volume upstream of the closure in the pump in | |
| Pressure at the inlet of a generalized tube segment in | |
| Pressure at the outlet of a generalized tube segment in | |
| Pressure loss over a generalized tube segment in | |
| Pressure at the inlet of the pump in | |
| Pressure at the outlet of the pump in | |
| Model parameters for curve fitting of the equilibrium height | |
| Variable used to transform the second order differential equation for the tube height into matrix form | |
| Gravity of Earth in |
References
- Klespitz, J.; Levente, K. Peristaltic pumps—A review on working and control possibilities. In Proceedings of the IEEE 12th International Symposium on Applied Machine Intelligence and Informatics (SAMI), Herl’any, Slovakia, 23–25 January 2014; pp. 191–194. [Google Scholar]
- Berg, J.; Dallas, T. Peristaltic Pumps. In Encyclopedia of Microfluidics and Nanofluidics; Li, D., Ed.; Springer: Boston, MA, USA, 2008. [Google Scholar] [CrossRef]
- Esser, F.; Masselter, T.; Speck, T. Silent Pumpers: A Comparative Topical Overview of the Peristaltic Pumping Principle in Living Nature, Engineering, and Biomimetics. Adv. Intell. Syst. 2019, 1, 1900009. [Google Scholar] [CrossRef]
- Panday, S.; Floryan, J.M.; Faisal, K.M. On the peristaltic pumping. Phys. Fluids 2021, 33, 033609. [Google Scholar] [CrossRef]
- Formato, G.; Romano, R.; Formato, A.; Sorvari, J.; Koiranen, T.; Pellegrino, A.; Villecco, F. Fluid–Structure Interaction Modeling Applied to Peristaltic Pump Flow Simulations. Machines 2019, 7, 50. [Google Scholar] [CrossRef]
- Yazdanpanah-Ardakani, K.; Niroomand-Oscuii, H. New approach in modeling peristaltic transport of non-Newtonian fluid. J. Mech. Med. Biol. 2013, 13, 1350052. [Google Scholar] [CrossRef]
- Elabbasi, N.; Bergström, J.; Brown, S. Fluid-Structure Interaction Analysis of a Peristaltic Pump. In Proceedings of the COMSOL Conference, Boston, MA, USA, 13–15 October 2011. [Google Scholar]
- Hung, N.B.; Ock, T.L. The study about deformation of a Peristaltic Pump using Numerical Simulation. Korean Hydrog. New Energy Soc. 2015, 26, 652–658. [Google Scholar] [CrossRef]
- Hayek, S.I. Mechanical Vibration and Damping. In Encyclopedia of Applied Physics; WILEY-VCH Verlag GmbH & Co KGaA: Hoboken, NJ, USA, 2003; ISBN 9783527600434. [Google Scholar] [CrossRef]
- Van Rossum, G.; Drake, F.L. Python 3 Reference Manual; CreateSpace: Scotts Valley, CA, USA, 2009. [Google Scholar]
- Lam, S.K.; Pitrou, A.; Seibert, S. Numba: A LLVM-based Python JIT compiler. In Proceedings of the Second Workshop on the LLVM Compiler Infrastructure in HPC (LLVM ‘15), Austin, TX, USA, 15 November 2015; Association for Computing Machinery: New York, NY, USA, 2015; p. 7. [Google Scholar] [CrossRef]
- Harris, C.R.; Millman, K.J.; van der Walt, S.J.; Gommers, R.; Virtanen, P.; Cournapeau, D.; Wieser, E.; Taylor, J.; Berg, S.; Smith, N.J.; et al. Array programming with NumPy. Nature 2020, 585, 357–362. [Google Scholar] [CrossRef] [PubMed]
- Burden, R.L.; Faires, J.D. Numerical Analysis, 7th ed.; Brooks/Cole: Totnes, UK, 2000; ISBN 978-0-534-38216-2. [Google Scholar]
- Atkinson, K.E. An Introduction to Numerical Analysis, 2nd ed.; John Wiley & Sons: New York, NY, USA, 1989; ISBN 978-0-471-50023-0. [Google Scholar]










Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hostettler, M.; Grüter, R.; Stingelin, S.; De Lorenzi, F.; Fuechslin, R.M.; Jacomet, C.; Koll, S.; Wilhelm, D.; Boiger, G.K. Modelling of Peristaltic Pumps with Respect to Viscoelastic Tube Material Properties and Fatigue Effects. Fluids 2023, 8, 254. https://doi.org/10.3390/fluids8090254
Hostettler M, Grüter R, Stingelin S, De Lorenzi F, Fuechslin RM, Jacomet C, Koll S, Wilhelm D, Boiger GK. Modelling of Peristaltic Pumps with Respect to Viscoelastic Tube Material Properties and Fatigue Effects. Fluids. 2023; 8(9):254. https://doi.org/10.3390/fluids8090254
Chicago/Turabian StyleHostettler, Marco, Raphael Grüter, Simon Stingelin, Flavio De Lorenzi, Rudolf M. Fuechslin, Cyrill Jacomet, Stephan Koll, Dirk Wilhelm, and Gernot K. Boiger. 2023. "Modelling of Peristaltic Pumps with Respect to Viscoelastic Tube Material Properties and Fatigue Effects" Fluids 8, no. 9: 254. https://doi.org/10.3390/fluids8090254
APA StyleHostettler, M., Grüter, R., Stingelin, S., De Lorenzi, F., Fuechslin, R. M., Jacomet, C., Koll, S., Wilhelm, D., & Boiger, G. K. (2023). Modelling of Peristaltic Pumps with Respect to Viscoelastic Tube Material Properties and Fatigue Effects. Fluids, 8(9), 254. https://doi.org/10.3390/fluids8090254







