Transition to Equilibrium and Coherent Structure in Ideal MHD Turbulence

Abstract

Transition of ideal, homogeneous, incompressible, magnetohydrodynamic (MHD) turbulence to near-equilibrium from non-equilibrium initial conditions is examined through new long-time numerical simulations on a ${128}^3$ periodic grid. Here, we neglect dissipation because we are primarily concerned with behavior at the largest scale which has been shown to be essentially the same for ideal and real (forced and dissipative) MHD turbulence. A Fourier spectral transform method is used to numerically integrate the dynamical equations forward in time and results from six computer runs are presented with various combinations of imposed rotation and mean magnetic field. There are five separate cases of ideal, homogeneous, incompressible, MHD turbulence: Case I, with no rotation or mean field; Case II, where only rotation is imposed; Case III, which has only a mean magnetic field; Case IV, where rotation vector and mean magnetic field direction are aligned; and Case V, which has nonaligned rotation vector and mean field directions. Dynamic coefficients are predicted by statistical mechanics to be zero-mean random variables, but largest-scale coherent magnetic structures emerge in all cases during transition; this implies dynamo action is inherent in ideal MHD turbulence. These coherent structures are expected to occur in Cases I, II and IV, but not in Cases III and V; future studies will determine whether they persist.

1. Introduction

“One cannot deal with the physics of cosmic fluids without encountering at almost every turn the problem of turbulence.”

[1]

In particular, one encounters the problem of magnetohydrodynamic (MHD) turbulence, since ‘cosmic fluids’, such as those found in planetary interiors and in stars, are electrically conducting and stirred into turbulence by buoyant forces. A universal feature of these objects is that they are rotating and often possess a quasi-stationary, mostly dipole magnetic field, i.e., a magnetic coherent structure. Just over a hundred years ago, ref. [2] hypothesized that internal magnetic fields coupled to fluid motions within the Sun, and by extension, the Earth, were responsible for the creation and maintenance of global magnetic dipole fields. Determining exactly how this was done came to be called ‘the dynamo problem’.

In regard to Earth, numerical simulations of the geodynamo [3,4,5] established that MHD processes within the Earth are capable of creating a magnetic field similar to the actual geomagnetic field, including reversals of the dominant dipole component. In addition, there have been many laboratory experiments [6], some of which have shown the growth of a self-generated magnetic field, i.e., a dynamo [7,8,9]. Although numerical simulations have been successful in proving the MHD nature of the geodynamo and experiments have shown a dynamo effect, the fundamental MHD origin of the dominant, quasi-steady geomagnetic dipole field still appear to be a theoretical mystery [10].

Our approach to resolving this mystery is to study three-dimensional (3-D) homogeneous, incompressible MHD turbulence, i.e., to examine a turbulent magnetofluid confined to a 3-D periodic box (a three-torus). This allows for the use of Fourier expansions to represent turbulent velocity and magnetic fields in order to study the nonlinear dynamics of a magnetofluid without the complicating factor of boundary conditions; in fact, introducing boundaries with no-slip conditions also requires introducing compressibility [11] and further complicates the problem. Fourier methods transform the problem from the partial differential equations of MHD (presented in Section 2.1) in $\mathbf{x}$-space (physical space) to a dynamical system of nonlinear, coupled ordinary differential equations in $\mathbf{k}$-space (Fourier space), which describe the evolution of the Fourier modes associated with velocity and magnetic field; this is discussed in Section 2.3. In $\mathbf{x}$-space, the continuum magnetofluid is represented on an $N^3$ grid of velocity $\mathbf{u}(\mathbf{x},t)$ and magnetic field $\mathbf{b}(\mathbf{x},t)$ values, while in $\mathbf{k}$-space, it is represented by the velocity and magnetic vector Fourier coefficients $\tilde{\mathbf{u}}(\mathbf{k},t)$ and $\tilde{\mathbf{b}}(\mathbf{k},t)$, where the wavevectors $\mathbf{k}$ define length-scales $\lambda =2\pi /k$, $k=\left|\mathbf{k}\right|\ge 1$, in the turbulent magnetofluid and explicitly separate the largest length-scale modes from all of the smaller length-scale modes. This is particularly useful as the largest length-scale modes are analogous to the magnetic dipole components in a planet or star.

Viscous and ohmic dissipation occur in a real magnetofluid but ‘cosmic fluids’ generally have very large Reynolds numbers so that one may remove dissipation from a model system and study ideal homogeneous MHD turbulence for the following reason. We neglect dissipation because we are primarily concerned with behavior of a turbulent magnetofluid at the largest scale; large-scale dynamics have been shown to be essentially the same for ideal and real (forced and dissipative) MHD turbulence [12,13]. The real MHD equations appear in (1) and (2) of Section 2.1 and become the ideal MHD equations when we set the viscosity $\nu$ and magnetic diffusivity $\eta$ equal to zero. There are differences between solutions of the real and ideal MHD equations as the highest derivative terms (the dissipative terms) in the real MHD equations methods are removed to produce the ideal MHD equations; the connection between real and ideal solutions is examined by singular perturbation theory, e.g., [14]. These differences are critical in real fluid and MHD flows since there may be thin regions, such as boundary layers, where the second-order dissipative terms become dominant, or in regions far from boundaries where there is a direct cascade of energy to the dissipation length-scale and where, again, dissipative terms are dominant. However, ideal results are often very useful. For example, in studying fluid turbulence, while it is usually necessary to retain the dissipative term in the Navier–Stokes equation because it determines the wavenumber spectrum in the medium-scale inertial and small-scale dissipative ranges, it is nevertheless possible to use ideal results to draw conclusions on the nature of real, helical fluid turbulence [15].

The fundamental difference between fluid and MHD turbulence is induced by the presence of a dynamic magnetic field, in that fluid turbulence only has a direct cascade to higher wavenumbers [16], while MHD turbulence has an inverse cascade as well as a direct one [17]. This difference between fluid and MHD turbulence, due to the MHD inverse cascade, can create an energetically dominant large-scale magnetic field, depending on the particular case of MHD turbulence under consideration; these cases are given in

Table 1. Cases and associated Runs with different integral invariants for ideal MHD turbulence. The ‘parallel helicity’ of Case IV is $H_P=H_C-\sigma H_M$ and occurs when ${\mathsf{\Omega }}_{\mathrm{o}}=\sigma {\mathbf{B}}_{\mathrm{o}}$, i.e., ${\mathsf{\Omega }}_{\mathrm{o}}$ is parallel to ${\mathbf{B}}_{\mathrm{o}}$.

Case	Mean Field	Rotation	Invariants	${128}^3$ Runs
I	${\mathbf{B}}_{\mathrm{o}}=0$	${\mathsf{\Omega }}_{\mathrm{o}}=0$	E, $H_C$, $H_M$	1
II	${\mathbf{B}}_{\mathrm{o}}=0$	${\mathsf{\Omega }}_{\mathrm{o}}\ne 0$	E, $H_M$	2a,b
III	${\mathbf{B}}_{\mathrm{o}}\ne 0$	${\mathsf{\Omega }}_{\mathrm{o}}=0$	E, $H_C$	3
IV	${\mathbf{B}}_{\mathrm{o}}\ne 0$	${\mathsf{\Omega }}_{\mathrm{o}}=\sigma {\mathbf{B}}_{\mathrm{o}}$	E, $H_P$	4
V	${\mathbf{B}}_{\mathrm{o}}\ne 0$	${\mathsf{\Omega }}_{\mathrm{o}}\times {\mathbf{B}}_{\mathrm{o}}\ne 0$	E	5

and the various helicities listed there are defined by equations described in the next two paragraphs and discussed in more detail in Appendix A. Ideal MHD statistical theory Appendix B predicts that in Cases I and II of Table 1, the large-scale magnetic field is quasi-stationary and has energy that is essentially equal to magnetic helicity, a result that also seems to apply to Case IV. These ideal MHD spectra are strongly peaked at the largest length scale, i.e., smallest wavenumber, where dissipation is weakest in the real case. That this survives the addition of forcing and dissipation to the magnetofluid has been shown in studies of helically forced, dissipative MHD turbulence using Fourier method simulations [12,13]. Thus, if we wish to study the large-scale dynamics and structure of MHD turbulence, either ideal or real MHD turbulence simulations can be employed. Here, we choose ideal MHD.

Ideal MHD turbulence, as represented by a finite set of Fourier modes, is a conservative system with energy and up to two different helicities being integral invariants; again, the different cases are shown in Table 1. The number and form of the helical invariants depend on whether or not rotation or a mean magnetic field or both are imposed on the system (a mean magnetic field ${\mathbf{B}}_{\mathrm{o}}$ is one that is spatially and temporally constant and frame rotation is given by a vector ${\mathsf{\Omega }}_{\mathrm{o}}$). Energy E, cross helicity $H_C$, magnetic helicity $H_M$ and parallel helicity $H_P$, as well as other quantities, are fully defined in Appendix A; here, we will, for easy reference and to serve as a ‘nomenclature section’, briefly discuss how E, $H_C$, $H_M$ and $H_P$, as well as other integrals, are determined.

First, we define the volume average of a quantity $\mathsf{\Phi }(\mathbf{x},t)$ multiplied by a quantity $\mathsf{\Psi }(\mathbf{x},t)$ as $\left\{\mathsf{\Phi }\mathsf{\Psi }\right\}$, which is an integral over the volume of interest, here, a periodic box of side length $2\pi$:

$$\begin{array}{ccc}\hfill \left\{\mathsf{\Phi }\mathsf{\Psi }\right\}& \equiv & {\left(2\pi \right)}^{-3}\int \mathsf{\Phi }(\mathbf{x},t)\mathsf{\Psi }(\mathbf{x},t)d^{3}x=\frac{1}{N^3}\sum _{\mathbf{k}}{\tilde{\mathsf{\Phi }}}^{*}(\mathbf{k},t)\tilde{\mathsf{\Psi }}(\mathbf{k},t).\hfill \end{array}$$

The $\mathbf{k}$-space functions ${\tilde{\mathsf{\Phi }}}^{*}(\mathbf{k},t)$ and $\tilde{\mathsf{\Psi }}(\mathbf{k},t)$ are Fourier transforms of the $\mathbf{x}$-functions $\mathsf{\Phi }(\mathbf{x},t)$ and $\mathsf{\Psi }(\mathbf{x},t)$ (Fourier transforms are defined in Section 2.2). Energy and the various helicities listed in Table 1 are then ($H_M$ is defined in terms of the magnetic vector potential $\mathbf{a}$, where $\nabla \times \mathbf{a}=\mathbf{b}$, $\nabla ·\mathbf{a}=0$)

$$\begin{array}{c}\hfill E=\frac{1}{2}\left\{{\left|\mathbf{u}\right|}^2+{\left|\mathbf{b}\right|}^2\right\},H_C=\frac{1}{2}\left\{\mathbf{u}·\mathbf{b}\right\},H_M=\frac{1}{2}\left\{\mathbf{a}·\mathbf{b}\right\},H_P=H_C-\sigma H_M.\end{array}$$

Again, velocity $\mathbf{u}$ and magnetic fields $\mathbf{b}$ can be represented in $\mathbf{x}$-space or $\mathbf{k}$-space; their basic equations are given in (1) and (2) for $\mathbf{x}$-space and (23) and (24) for $\mathbf{k}$-space, and either set can be used to prove invariance. In terms of the mean magnetic field and rotation vector magnitudes $B_{\mathrm{o}}=\left|{\mathbf{B}}_{\mathrm{o}}\right|$ and ${\mathsf{\Omega }}_{\mathrm{o}}=\left|{\mathsf{\Omega }}_{\mathrm{o}}\right|$, one defines the ratio $\sigma =B_{\mathrm{o}}/{\mathsf{\Omega }}_{\mathrm{o}}$ when ${\mathsf{\Omega }}_{\mathrm{o}}$ is parallel to ${\mathbf{B}}_{\mathrm{o}}$. Other quadratic forms, in addition to those given above, are also useful measures of the turbulent state:

$$\begin{array}{c}\hfill E_M=\frac{1}{2}\left\{{\left|\mathbf{b}\right|}^2\right\},E_K=\frac{1}{2}\left\{{\left|\mathbf{u}\right|}^2\right\},H_K=\frac{1}{2}\left\{\mathbf{u}·\mathbf{\omega }\right\},A=\frac{1}{2}\left\{{\left|\mathbf{a}\right|}^2\right\},\mathsf{\Omega }=\frac{1}{2}\left\{{\left|\mathbf{\omega }\right|}^2\right\},J=\frac{1}{2}\left\{{\left|\mathbf{j}\right|}^2\right\}.\end{array}$$

Above, we have $E_M$ (magnetic energy), $E_K$ (kinetic energy), $H_K$ (kinetic helicity, where $\mathbf{\omega }=\nabla \times \mathbf{u}$ is the vorticity), A (mean squared vector potential), $\mathsf{\Omega }$ (‘enstrophy’, i.e., mean squared vorticity) and J (mean squared electric current, where $\mathbf{j}=\nabla \times \mathbf{b}$); please note that enstrophy $\mathsf{\Omega }$ is different from rotation vector magnitude ${\mathsf{\Omega }}_{\mathrm{o}}$. For the computer runs discussed here, time-averages of these appear in

Table 2. Time averages and standard deviations (avg ± std) for various global quantities over the last half of each run are given below for six new ideal MHD turbulence long-time ${128}^3$ runs A, B, C, D, E and F. These global quantities are: energy E, kinetic energy $E_K$, magnetic energy $E_M$, mean squared vector potential A, kinetic helicity $H_K$, magnetic helicity $H_M$, cross helicity $H_C$, parallel helicity $H_P$, enstrophy $\mathsf{\Omega }$ and mean squared current J.

Run:	1	2a	2b	3	4	5
$t_{end}$	1095	1136	1037	714	895	461
${\mathsf{\Omega }}_{\mathrm{o}}$	0	$10\widehat{\mathbf{z}}$	$10\widehat{\mathbf{z}}$	0	$2\widehat{\mathbf{z}}$	$1\widehat{\mathbf{z}}$
${\mathbf{B}}_{\mathrm{o}}$	0	0	0	$1\widehat{\mathbf{z}}$	$1\widehat{\mathbf{z}}$	$\frac{1}{2}\widehat{\mathbf{y}}$
$E^{avg}$	1.0075e+00	1.0089e+00	1.0083e+00	1.0203e+00	1.0250e+00	1.0040e+00
$E^{std}$	1.3852e−03	1.4918e−03	1.5526e−03	3.9857e−03	4.8861e−03	7.6450e−04
$E_M^{avg}$	5.6108e−01	5.3617e−01	5.6590e−01	5.1059e−01	5.1355e−01	5.0233e−01
$E_M^{std}$	7.2553e−04	5.2272e−04	4.7956e−04	2.0012e−03	2.4628e−03	5.0589e−04
$E_K^{avg}$	4.4645e−01	4.7271e−01	4.4235e−01	5.0972e−01	5.1146e−01	5.0170e−01
$E_K^{std}$	7.6388e−04	1.6473e−03	1.2414e−03	2.0452e−03	2.4816e−03	5.8068e−04
$H_K^{avg}$	6.0800e−03	5.1370e−04	−1.1159e−03	3.7043e−04	3.2017e−02	8.1015e−04
$H_K^{std}$	2.1656e−02	2.2737e−02	2.1814e−02	2.4703e−02	2.4743e−02	2.3758e−02
$A^{avg}$	1.1570e−01	6.0391e−02	1.2008e−01	1.2509e−03	2.1313e−03	7.4948e−04
$A^{std}$	1.4644e−05	1.9213e−04	7.4346e−05	6.9214e−06	1.8649e−05	2.1832e−05
$H_C^{avg}$	5.6545e−02	−7.2107e−05	−5.7265e−06	5.2397e−02	−1.2683e−01	6.1309e−05
$H_C^{std}$	7.1983e−05	3.6793e−04	3.6892e−04	5.7005e−05	2.0936e−04	3.4230e−04
$H_M^{avg}$	1.1570e−01	6.0840e−02	1.2070e−01	1.0008e−05	2.1639e−03	−1.2837e−05
$H_M^{std}$	7.5363e−15	5.4128e−16	9.7293e−15	3.9642e−05	2.8651e−05	1.7278e−05
$H_P^{avg}$	⋯	⋯	⋯	⋯	−1.3116e−01	⋯
$H_P^{std}$	⋯	⋯	⋯	⋯	1.7467e−04	⋯
${\mathsf{\Omega }}^{avg}$	9.7244e+02	1.0323e+03	9.6604e+02	1.1132e+03	1.1167e+03	1.0957e+03
${\mathsf{\Omega }}^{std}$	1.7385e+00	3.6272e+00	2.7515e+00	4.4614e+00	5.4717e+00	1.3995e+00
$J^{avg}$	9.7300e+02	1.0329e+03	9.6663e+02	1.1132e+03	1.1172e+03	1.0957e+03
$J^{std}$	1.7036e+00	3.5585e+00	2.7671e+00	4.4315e+00	5.4706e+00	1.3820e+00

.

The ideal invariants given in Table 1 can be used to create a multi-invariant canonical probability density function and a statistical mechanics applicable to ideal MHD turbulence (Appendix B). This statistical theory was initiated by [18], applied to fluid turbulence by [16] and to MHD turbulence by [17,19]; these initial results were extended later, e.g., [20,21,22,23,24]. This extension is relevant to the planets and stars as it provides an, at least qualitative, explanation for the emergence of a dominant, quasi-stationary, dipole magnetic field through broken ergodicity, which is defined by [25] as occurring if ‘In a system that is non-ergodic on physical timescales the phase point is effectively confined in one subregion or component of phase space’. The phase space itself is defined by the real and imaginary parts of the coefficients of the independent Fourier modes in the model system.

Numerical simulations have played an essential part in MHD turbulence research, starting with [26] using a ${64}^3$ grid (which was large for that time), while advances in computer hardware have allowed more recent simulations to have much larger grid sizes, e.g., ${1024}^3$ [27]. However, these simulations were only run for short simulation times, with $t_{max}=3$ in the former and $t_{max}=10$ in the latter, as the emphasis was on large grid sizes rather than on longer simulation time. In contrast, we have focused on long-time runs with $t_{max}$∼10${}^3$ to gather statistics and this dictated smaller grid sizes of ${64}^3$ [22,23,24]; there is always this trade-off between grid size and simulation time. To illustrate the trade-off between grid size and maximum simulated time, ref. [28] presented runs from ${64}^3$ up to ${512}^3$, but as the grid size went up, the time-step size decreased and number of time-steps increased, so that simulation time (number of ‘eddy turnover times’) decreased and only for the ${64}^3$ run was quasi-equilibrium attained (see their figure 5).

Improved computational resources have allowed us to increase our grid size to ${128}^3$ while still keeping $t_{max}\sim {10}^3$. We have recently run six of these ${128}^3$ simulations, each for over a million time-steps, in a reasonable amount of calendar time (over half a year each) and they allow us to examine the transition of ideal MHD turbulence for each of the cases in Table 1, where the runs associated with each case are indicated. All of our ${128}^3$ runs have moved through or are near the end of their transition periods and appear either to achieve equilibrium or to approach it asymptotically. Here, we focus on this initial period of transition to near-equilibrium and plan to examine the full equilibrium phase and present results in the future when these become available.

From our earliest numerical simulations [20] to those presented here, we have consistently seen the lowest-wavenumber (largest length-scale) modes grow from very small initial values into energetic, quasi-stationary, coherent structures. To understand this, it is necessary to develop the statistical theory of ideal MHD turbulence beyond that introduced by [17]. In particular, we analyzed the entropy of the model dynamical system to discover the origin of this energetic structure; we have presented this analysis before [24,29] and will place it here in Appendix B. Furthermore, the statistical mechanics of an ideal, turbulent magnetofluid in a periodic box is essentially the same as that of one contained in a spherical shell [30] and thus, the smallest wavenumber Fourier modes are surrogates for geomagnetic dipole components.

Key discoveries in our past work are: (i) That the energy of a quasi-stationary magnetic dipole in Cases I and II is directly proportional to the absolute value of the magnetic helicity of the equilibrium turbulent magnetofluid [29,31]; this also appears to apply to Case IV, as will be shown here; and (ii) that the assumed ergodicity of the statistical ensemble is actually broken at the largest scale [20,24]. Again, these ideal results have been seen to apply at the largest length-scale to real (i.e., forced and dissipative) MHD turbulence [12,13]; thus, modeling and theory provide a solution to the ‘dynamo problem,’ at least for the model systems considered here. (Again, ideal MHD statistical theory is reviewed in Appendix B of this paper for ease of referral).

Next, following a brief discussion of the mathematical model and numerical procedure, new computational results drawn from ${128}^3$ simulations are presented. These are followed by a discussion of these results and their relevance to the dynamo problem. Lastly, we offer a conclusion to summarize our results and emphasize their importance.

2. Mathematical Model

2.1. Basic Equations

Magnetohydrodynamics (MHD) is the physics of electrically conducting fluids and principles and applications can be found in, e.g., [32,33], as well as in many other texts. The nondimensional form of the 3-D incompressible MHD equations in a rotating frame of reference with constant angular velocity ${\mathsf{\Omega }}_{\mathrm{o}}$ and mean magnetic field ${\mathbf{B}}_{\mathrm{o}}$ (i.e., constant in space and time) can be written as

$$\begin{array}{ccc}\hfill \frac{\partial \mathbf{\omega }}{\partial t}& =& \nabla \times \left[\mathbf{u}\times \left(\mathbf{\omega }+2{\mathsf{\Omega }}_{\mathrm{o}}\right)+\mathbf{j}\times \left(\mathbf{b}+{\mathbf{B}}_{\mathrm{o}}\right)\right]+\nu {\nabla }^2\mathbf{\omega },\hfill \\ \hfill \end{array}$$

(1)

$$\begin{array}{ccc}\hfill \frac{\partial \mathbf{b}}{\partial t}& =& \nabla \times \left[\mathbf{u}\times \left(\mathbf{b}+{\mathbf{B}}_{\mathrm{o}}\right)\right]+\eta {\nabla }^2\mathbf{b}.\hfill \end{array}$$

(2)

These are described, for example, by [22]; these equations can be augmented to include thermal effects in the Boussinesq approximation [34], but we will not consider this here. Above, $\mathbf{u}(\mathbf{x},t)$ and $\mathbf{b}(\mathbf{x},t)$ are the turbulent velocity and magnetic fields, respectively. Velocity and magnetic fields are solenoidal: $\nabla ·\mathbf{u}=\nabla ·\mathbf{b}=0$, as is appropriate for laboratory experiments and the Earth’s outer core [3]. The vorticity $\mathbf{\omega }(\mathbf{x},t)$ and electric current density $\mathbf{j}(\mathbf{x},t)$ are defined by

$$\mathbf{\omega }=\nabla \times \mathbf{u},\mathbf{j}=\nabla \times \mathbf{b}.$$

(3)

Nondimensional density does not appear in (1) because it equals unity. The symbols $\nu$ in (1) and $\eta$ in (2) are shorthand for $1/R_E$ and $1/R_M$, i.e., the inverses of the kinetic and magnetic Reynolds numbers, respectively. In the dimensional form of the equations, $\nu$ is the kinematic viscosity, while $\eta$ is the magnetic diffusivity and $\nu =\eta =0$ for ideal MHD turbulence. Again, we avoid the complication of boundary conditions by placing the magnetofluid in a periodic box and expanding the various fields in terms of Fourier series.

In studying MHD turbulence, it is also possible to use other orthogonal function expansions, such as those appropriate to a cylinder [35], sphere [36] or spherical shell [30]. However, the statistical mechanics of ideal MHD turbulence has the same form and predictions as in the Fourier case. In addition, the Fourier spectral transform methods used to simulate MHD turbulence in a periodic box are much more computationally efficacious in that they allow for larger grid sizes (more dynamical resolution) and longer runs (for time averaging). In fact, no spectral transform methods for these other geometries yet exist (the creation of which may be considered a ‘grand computational challenge’) and nontransform methods [36,37], in comparison, will always have insufficient resolution. In essence, Fourier transform methods in a periodic box are efficient and also serve as a surrogate for methods based on other orthogonal function expansions more suited to other geometries.

2.2. Fourier Representation

Discrete Fourier transforms for $\mathbf{u}$ and $\mathbf{b}$, connecting $\mathbf{x}$-space to $\mathbf{k}$-space, are

$$\left[\begin{array}{c}\mathbf{u}(\mathbf{x},t)\hfill \\ \mathbf{b}(\mathbf{x},t)\hfill \end{array}\right]=\sum _{\mathbf{k}}\left[\begin{array}{c}\tilde{\mathbf{u}}(\mathbf{k},t)\\ \tilde{\mathbf{b}}(\mathbf{k},t)\end{array}\right]\frac{exp\left(i\mathbf{x}·\mathbf{k}\right)}{N^{3/2}},$$

(4)

$$\left[\begin{array}{c}\tilde{\mathbf{u}}(\mathbf{k},t)\hfill \\ \tilde{\mathbf{b}}(\mathbf{k},t)\hfill \end{array}\right]=\sum _{\mathbf{x}}\left[\begin{array}{c}\mathbf{u}(\mathbf{x},t)\\ \mathbf{b}(\mathbf{x},t)\end{array}\right]\frac{exp\left(-i\mathbf{x}·\mathbf{k}\right)}{N^{3/2}}.$$

(5)

Here, N is the number of grid points in each $\mathbf{x}$-space dimension, so we have a grid of $N^3$ points. The set of positions $\mathbf{x}$ and wave vectors (modes) $\mathbf{k}$ appearing in (4) and (5) are

$$\begin{array}{ccc}& & \mathbf{x}=x\widehat{\mathbf{x}}+y\widehat{\mathbf{y}}+z\widehat{\mathbf{z}}=\frac{2\pi }{N}\left(n_x\widehat{\mathbf{x}}+n_y\widehat{\mathbf{y}}+n_z\widehat{\mathbf{z}}\right),\hfill \\ \hfill \end{array}$$

(6)

$$\begin{array}{ccc}& & \mathbf{k}=k_x\widehat{\mathbf{x}}+k_y\widehat{\mathbf{y}}+k_z\widehat{\mathbf{z}}.\hfill \end{array}$$

(7)

The components $n_j$ and $k_j$, $j=x,y,z$, are integers. The $n_j$ satisfy $0\le n_j<N$, while the integers $k_j$ lie in the range $-N/2+1$ and $+N/2$; thus, there are $N^3$ points in both spaces. The dot product $\mathbf{k}·\mathbf{x}$ in (4) and (5) is

$$\mathbf{k}·\mathbf{x}=\frac{2\pi }{N}\left(n_x{k}_x+n_y{k}_y+n_z{k}_z\right).$$

(8)

The Fourier coefficients $\tilde{\mathbf{u}}(\mathbf{k},t)$ and $\tilde{\mathbf{b}}(\mathbf{k},t)$ are nonzero only for $1\le k\le K<N/2$, where $k=\left|\mathbf{k}\right|$. The exact value of K is set by a de-aliasing requirement of [38]: $K^2$ is the largest integer such that $K^2\le 2N^2/9$, e.g., for $N=128$, $K^2=3640$.

Note that the reality of $\mathbf{u}(\mathbf{x},t)$ and $\mathbf{b}(\mathbf{x},t)$ imply that $\tilde{\mathbf{u}}(\mathbf{k},t)={\tilde{\mathbf{u}}}^{*}(-\mathbf{k},t)$ and $\tilde{\mathbf{b}}(\mathbf{k},t)={\tilde{\mathbf{b}}}^{*}(-\mathbf{k},t)$. In a Cartesian coordinate system, $\tilde{\mathbf{u}}(\mathbf{k},t)$ and $\tilde{\mathbf{b}}(\mathbf{k},t)$ are

$$\begin{array}{ccc}\hfill \tilde{\mathbf{u}}(\mathbf{k},t)& =& {\tilde{u}}_x(\mathbf{k},t)\widehat{\mathbf{x}}+{\tilde{u}}_y(\mathbf{k},t)\widehat{\mathbf{y}}+{\tilde{u}}_z(\mathbf{k},t)\widehat{\mathbf{z}},\hfill \\ \hfill \end{array}$$

(9)

$$\begin{array}{ccc}\hfill \tilde{\mathbf{b}}(\mathbf{k},t)& =& {\tilde{b}}_x(\mathbf{k},t)\widehat{\mathbf{x}}+{\tilde{b}}_y(\mathbf{k},t)\widehat{\mathbf{y}}+{\tilde{b}}_z(\mathbf{k},t)\widehat{\mathbf{z}}.\hfill \end{array}$$

(10)

The vector fields $\mathbf{u}(\mathbf{x},t)$ and $\mathbf{b}(\mathbf{x},t)$ can be defined in a similar manner.

The periodic box of the model system is a cube of equal length edges. Although we will not do so here, we could have allowed for the possibility that it might be elongated or compressed in the x, y or z directions, which simulates a difference in oblateness that may occur in the Earth’s inner and outer cores. We ignore this possibility because we have examined it and the dynamical effect is insignificant when compared to rotation [29].

Dynamically interacting coefficients fit within a sphere of radius K in $\mathbf{k}$-space; all coefficients outside this $\mathbf{k}$-sphere, as well as at $\mathbf{k}=0$, are initially zero and remain so during any numerical simulation. Since $\tilde{\mathbf{u}}(\mathbf{k},t)={\tilde{\mathbf{u}}}^{*}(-\mathbf{k},t)$ and $\tilde{\mathbf{b}}(\mathbf{k},t)={\tilde{\mathbf{b}}}^{*}(-\mathbf{k},t)$, only half of the $\mathbf{k}$ that satisfy $1\le k^2\le K^2$ identify independent coefficients. Therefore, the number of independent modes $\mathbf{k}$ is $\mathcal{M}\cong 2\pi K^3/3$; for example, with $N=128$ and $K^2=3640$, we have $\mathcal{M}\cong$ 459,950, while the actual numerical count is $\mathcal{M}$ = 459,916.

In $\mathbf{k}$-space, the requirements $\nabla ·\mathbf{u}=\nabla ·\mathbf{b}=0$ become $i\mathbf{k}·\tilde{\mathbf{u}}(\mathbf{k},t)=i\mathbf{k}·\tilde{\mathbf{b}}(\mathbf{k},t)=0$. Thus, $\tilde{\mathbf{u}}(\mathbf{k},t)$ and $\tilde{\mathbf{b}}(\mathbf{k},t)$ have two independent complex vector coefficients each, which can be defined as follows: First, determine a coordinate system for each $\mathbf{k}$ by starting with a unit vector ${\widehat{\mathbf{e}}}_3\left(\mathbf{k}\right)=\mathbf{k}/k=\widehat{\mathbf{k}}$; then choosing a unit vector ${\widehat{\mathbf{e}}}_1\left(\mathbf{k}\right)$ orthogonal to ${\widehat{\mathbf{e}}}_3\left(\mathbf{k}\right)$, and then the remaining unit vector ${\widehat{\mathbf{e}}}_2\left(\mathbf{k}\right)$ is a vector product of the first two, forming a right-handed orthonormal basis for each $\mathbf{k}$:

$$\begin{array}{ccc}{\widehat{\mathbf{e}}}_1\left(\mathbf{k}\right)·{\widehat{\mathbf{e}}}_3\left(\mathbf{k}\right)=0,\hfill & \hfill & {\widehat{\mathbf{e}}}_2\left(\mathbf{k}\right)={\widehat{\mathbf{e}}}_3\left(\mathbf{k}\right)\times {\widehat{\mathbf{e}}}_1\left(\mathbf{k}\right),\hfill \\ \\ {\widehat{\mathbf{e}}}_i\left(\mathbf{k}\right)·{\widehat{\mathbf{e}}}_j\left(\mathbf{k}\right)={\delta }_{ij},\hfill & \hfill & {\widehat{\mathbf{e}}}_1\left(\mathbf{k}\right)·{\widehat{\mathbf{e}}}_2\left(\mathbf{k}\right)\times {\widehat{\mathbf{e}}}_3\left(\mathbf{k}\right)=1.\hfill \end{array}$$

(11)

(This is similar to a Craya–Herring decomposition [39]). Explicit choices [23] for any of the ${\widehat{\mathbf{e}}}_j\left(\mathbf{k}\right)$ will be mentioned as needed.

In terms of the ${\widehat{\mathbf{e}}}_j\left(\mathbf{k}\right)$ defined above, the Fourier vector coefficients (9) and (10) are

$$\begin{array}{ccc}\hfill \tilde{\mathbf{u}}(\mathbf{k},t)& =& {\tilde{u}}_1(\mathbf{k},t){\widehat{\mathbf{e}}}_1\left(\mathbf{k}\right)+{\tilde{u}}_2(\mathbf{k},t){\widehat{\mathbf{e}}}_2\left(\mathbf{k}\right),\hfill \end{array}$$

(12)

$$\begin{array}{ccc}\hfill \tilde{\mathbf{b}}(\mathbf{k},t)& =& {\tilde{b}}_1(\mathbf{k},t){\widehat{\mathbf{e}}}_1\left(\mathbf{k}\right)+{\tilde{b}}_2(\mathbf{k},t){\widehat{\mathbf{e}}}_2\left(\mathbf{k}\right).\hfill \end{array}$$

(13)

However, an equivalent but very useful helical representation is:

$$\begin{array}{ccc}\hfill \tilde{\mathbf{u}}(\mathbf{k},t)& =& {\tilde{u}}_{+}(\mathbf{k},t){\widehat{\mathbf{e}}}_{+}\left(\mathbf{k}\right)+{\tilde{u}}_{-}(\mathbf{k},t){\widehat{\mathbf{e}}}_{-}\left(\mathbf{k}\right),\hfill \end{array}$$

(14)

$$\begin{array}{ccc}\hfill \tilde{\mathbf{b}}(\mathbf{k},t)& =& {\tilde{b}}_{+}(\mathbf{k},t){\widehat{\mathbf{e}}}_{+}\left(\mathbf{k}\right)+{\tilde{b}}_{-}(\mathbf{k},t){\widehat{\mathbf{e}}}_{-}\left(\mathbf{k}\right).\hfill \end{array}$$

(15)

Here, the positive and negative helicity unit vectors and components are

$$\begin{array}{ccc}\hfill {\widehat{\mathbf{e}}}_{\pm }\left(\mathbf{k}\right)& =& \frac{1}{\sqrt{2}}\left[{\widehat{\mathbf{e}}}_1\left(\mathbf{k}\right)\pm i{\widehat{\mathbf{e}}}_2\left(\mathbf{k}\right)\right],\hfill \\ \hfill \end{array}$$

(16)

$$\begin{array}{ccc}\hfill {\tilde{u}}_{\pm }(\mathbf{k},t)& =& \frac{1}{\sqrt{2}}\left[{\tilde{u}}_1(\mathbf{k},t)\mp i{\tilde{u}}_2(\mathbf{k},t)\right],\hfill \\ \hfill \end{array}$$

(17)

$$\begin{array}{ccc}\hfill {\tilde{b}}_{\pm }(\mathbf{k},t)& =& \frac{1}{\sqrt{2}}\left[{\tilde{b}}_1(\mathbf{k},t)\mp i{\tilde{b}}_2(\mathbf{k},t)\right].\hfill \end{array}$$

(18)

Note that ${\widehat{\mathbf{e}}}_{\pm }^{*}\left(\mathbf{k}\right)={\widehat{\mathbf{e}}}_{\mp }\left(\mathbf{k}\right)$. The orthonormality properties of the ${\widehat{\mathbf{e}}}_{\pm }\left(\mathbf{k}\right)$ are

$${\widehat{\mathbf{e}}}_{\pm }\left(\mathbf{k}\right)·{\widehat{\mathbf{e}}}_{\pm }^{*}\left(\mathbf{k}\right)=1,{\widehat{\mathbf{e}}}_{\pm }\left(\mathbf{k}\right)·{\widehat{\mathbf{e}}}_{\pm }\left(\mathbf{k}\right)=0={\widehat{\mathbf{e}}}_3\left(\mathbf{k}\right)·{\widehat{\mathbf{e}}}_{\pm }\left(\mathbf{k}\right).$$

(19)

An important property of the helical unit vectors concerns the curl operation:

$$i\mathbf{k}\times {\widehat{\mathbf{e}}}_{\pm }\left(\mathbf{k}\right)=\pm k{\widehat{\mathbf{e}}}_{\pm }\left(\mathbf{k}\right),$$

(20)

The vorticity and current in helical form are then

$$\begin{array}{ccc}\hfill \tilde{\mathbf{\omega }}(\mathbf{k},t)& =& k[{\tilde{u}}_{+}(\mathbf{k},t){\widehat{\mathbf{e}}}_{+}\left(\mathbf{k}\right)-{\tilde{u}}_{-}(\mathbf{k},t){\widehat{\mathbf{e}}}_{-}\left(\mathbf{k}\right)],\hfill \\ \hfill \end{array}$$

(21)

$$\begin{array}{ccc}\hfill \tilde{\mathbf{j}}(\mathbf{k},t)& =& k[{\tilde{b}}_{+}(\mathbf{k},t){\widehat{\mathbf{e}}}_{+}\left(\mathbf{k}\right)-{\tilde{b}}_{-}(\mathbf{k},t){\widehat{\mathbf{e}}}_{-}\left(\mathbf{k}\right)].\hfill \end{array}$$

(22)

Thus, the helical ± components of vorticity ${\tilde{\omega }}_{\pm }(\mathbf{k},t)=\pm k{\tilde{u}}_{\pm }(\mathbf{k},t)$ and current ${\tilde{j}}_{\pm }(\mathbf{k},t)=\pm k{\tilde{b}}_{\pm }(\mathbf{k},t)$ are directly connected to velocity and magnetic field ± helical components. The helical representation is very useful for theoretical analysis and graphical presentation of numerical data, although numerical simulation is actually performed using the Cartesian components (9) and (10).

2.3. A Dynamical System

The Fourier-transformed 3-D MHD equations are found by placing expansions for $\mathbf{\omega }(\mathbf{x},t)$ and $\mathbf{b}(\mathbf{x},t)$ of the form (4) into (1) and (2). The result is a set of coupled, nonlinear ordinary differential equations that represents a dynamical system in the sense of [40]:

$$\begin{array}{ccc}\hfill \frac{\mathrm{d}\tilde{\mathbf{\omega }}(\mathbf{k},t)}{\mathrm{d}t}& =& \tilde{\mathbf{S}}(\mathbf{u},\mathbf{\omega };\mathbf{k},t)+\tilde{\mathbf{S}}(\mathbf{j},\mathbf{b};\mathbf{k},t)+2i(\mathbf{k}·{\mathsf{\Omega }}_{\mathrm{o}})\tilde{\mathbf{u}}(\mathbf{k},t)-\nu k^2\tilde{\mathbf{\omega }}(\mathbf{k},t),\hfill \\ \hfill \end{array}$$

(23)

$$\begin{array}{ccc}\hfill \frac{\mathrm{d}\tilde{\mathbf{b}}(\mathbf{k},t)}{\mathrm{d}t}& =& \tilde{\mathbf{S}}(\mathbf{u},\mathbf{b};\mathbf{k},t)+i(\mathbf{k}·{\mathbf{B}}_{\mathrm{o}})\tilde{\mathbf{u}}(\mathbf{k},t)-\eta k^2\tilde{\mathbf{b}}(\mathbf{k},t).\hfill \end{array}$$

(24)

The nonlinear terms denoted by $\tilde{\mathbf{S}}$ are vector convolutions:

$$\begin{array}{ccc}\hfill \tilde{\mathbf{S}}(\mathbf{u},\mathbf{b};\mathbf{k},t)& =& \frac{i}{N^{3/2}}\mathbf{k}\times \sum _{\mathbf{p}+\mathbf{q}=\mathbf{k}}\left[\tilde{\mathbf{u}}(\mathbf{p},t)\times \tilde{\mathbf{b}}(\mathbf{q},t)\right].\hfill \end{array}$$

(25)

The double summation in (25) is over all wavevectors $\mathbf{p}$ and $\mathbf{q}$ inside the truncation volume in $\mathbf{k}$-space that satisfy $\mathbf{p}+\mathbf{q}=\mathbf{k}$. In 3-D numerical simulations, these equations are integrated as described in Section 3. For $N=128$, the phase space $\Gamma$ of the dynamical system (23) and (24) has a dimension of ${\mathcal{N}}_{\Gamma }$ = 3,679,328, as discussed in Appendix B. For ideal MHD, Table 1 indicates that the dynamical system conserves energy and various helicities depending on whether ${\mathbf{B}}_{\mathrm{o}}$ and ${\mathsf{\Omega }}_{\mathrm{o}}$ are zero or not; these quantities and others are defined in Appendix A. The important Case II of Table 1, where ${\mathsf{\Omega }}_{\mathrm{o}}\ne 0$ and ${\mathbf{B}}_{\mathrm{o}}=0$, applies to the Earth and most other planets (and stars), so in these cases, energy and magnetic helicity are of primary interest. The Cases III, IV and V, where ${\mathbf{B}}_{\mathrm{o}}\ne 0$, are appropriate for laboratory experiments and perhaps compact regions of solar wind turbulence where ${\mathbf{B}}_{\mathrm{o}}$ is the local interplanetary magnetic field.

2.4. Linear Modes

Using (11)–(18), the MHD Equations (23) and (24) can be linearized to yield

$$\begin{array}{ccc}\hfill \frac{\mathrm{d}{\tilde{u}}_{\pm }(\mathbf{k},t)}{\mathrm{d}t}& =& \pm 2i{\mathsf{\Omega }}_{\mathrm{o}}·\widehat{\mathbf{k}}{\tilde{u}}_{\pm }(\mathbf{k},t)+i{\mathbf{B}}_{\mathrm{o}}·\mathbf{k}{\tilde{b}}_{\pm }(\mathbf{k},t),\hfill \\ \hfill \end{array}$$

(26)

$$\begin{array}{ccc}\hfill \frac{\mathrm{d}{\tilde{b}}_{\pm }(\mathbf{k},t)}{\mathrm{d}t}& =& i{\mathbf{B}}_{\mathrm{o}}·\mathbf{k}{\tilde{u}}_{\pm }(\mathbf{k},t).\hfill \end{array}$$

(27)

These can be put into a matrix form:

$$\begin{array}{ccc}\hfill \frac{\mathrm{d}{\mathsf{U}}^{\pm }(\mathbf{k},t)}{\mathrm{d}t}& =& i{\mathsf{M}}^{\pm }{\mathsf{U}}^{\pm }(\mathbf{k},t),{\mathsf{U}}^{\pm }(\mathbf{k},t)=\left[\begin{array}{c}{\tilde{u}}_{\pm }(\mathbf{k},t)\\ {\tilde{b}}_{\pm }(\mathbf{k},t)\end{array}\right],\hfill \end{array}$$

(28)

$${\mathsf{M}}^{\pm }=\left[\begin{array}{cc}\pm 2A& B\\ B& 0\end{array}\right],A={\mathsf{\Omega }}_{\mathrm{o}}·\widehat{\mathbf{k}},B={\mathbf{B}}_{\mathrm{o}}·\mathbf{k}.$$

(29)

It is a straightforward procedure to find linear eigenmodes of (28) by diagonalizing ${\mathsf{M}}^{\pm }$; there is one transform matrix for each $\mathbf{k}$:

$$\mathsf{T}=\left[\begin{array}{cc}{T}_{11}& T_{12}\\ \\ T_{21}& T_{22}\end{array}\right]=\left[\begin{array}{cc}\left(A+\sqrt{A^2+B^2}\right)/N^{+}& B/N^{+}\\ \\ \left(A-\sqrt{A^2+B^2}\right)/N^{-}& B/N^{-}\end{array}\right],$$

(30)

$$\begin{array}{c}\hfill N^{\pm }={\left[2\left(A^2+B^2\pm A\sqrt{A^2+B^2}\right)\right]}^{1/2}.\end{array}$$

(31)

The solution to (28) are the eigenmodes

$${\mathsf{W}}^{\pm }(\mathbf{k},t)=\mathsf{T}{\mathsf{U}}^{\pm }(\mathbf{k},t)={\mathsf{E}}^{\pm }{\mathsf{V}}^{\pm }(\mathbf{k},t),$$

(32)

where the time dependence of the linear modes is in the matrix

$${\mathsf{E}}^{\pm }=\left[\begin{array}{cc}exp[\pm i{\mathsf{\Omega }}_1\left(\mathbf{k}\right)t]& 0\\ \\ 0& exp[\pm i{\mathsf{\Omega }}_2\left(\mathbf{k}\right)t]\end{array}\right],\begin{array}{c}{\mathsf{\Omega }}_1\left(\mathbf{k}\right)=A+\sqrt{A^2+B^2},\\ \\ {\mathsf{\Omega }}_2\left(\mathbf{k}\right)=A-\sqrt{A^2+B^2}\end{array}.$$

(33)

In the linear case, ${\mathsf{V}}^{\pm }(\mathbf{k},t)$ is constant, while in the nonlinear case, it becomes time-dependent. In slightly more detail, ${\mathsf{V}}^{\pm }(\mathbf{k},t)$ has the form

$$\begin{array}{ccc}\hfill {\mathsf{V}}^{\pm }(\mathbf{k},t)& =& \left[\begin{array}{c}{\tilde{V}}_1^{\pm }(\mathbf{k},t)\\ \\ {\tilde{V}}_2^{\pm }(\mathbf{k},t)\end{array}\right]={{\mathsf{E}}^{\pm }}^{†}\mathsf{T}{\mathsf{U}}^{\pm }(\mathbf{k},t)\hfill \\ \hfill \\ & =& \left[\begin{array}{c}exp[\mp i{\mathsf{\Omega }}_1\left(\mathbf{k}\right)t]T_{11}{\tilde{u}}_{\pm }(\mathbf{k},t)+exp[\mp i{\mathsf{\Omega }}_1\left(\mathbf{k}\right)t]T_{12}{\tilde{b}}_{\pm }(\mathbf{k},t)\\ \\ exp[\mp i{\mathsf{\Omega }}_2\left(\mathbf{k}\right)t]T_{21}{\tilde{u}}_{\pm }(\mathbf{k},t)+exp[\mp i{\mathsf{\Omega }}_2\left(\mathbf{k}\right)t]T_{22}{\tilde{b}}_{\pm }(\mathbf{k},t).\end{array}\right]\hfill \end{array}$$

(34)

The matrix elements $T_{nm}$, $n,m=1,2$, are given in (30).

If there is no nonlinearity, ${\tilde{V}}_1^{\pm }(\mathbf{k},t)$ and ${\tilde{V}}_2^{\pm }(\mathbf{k},t)$ of (34) will have constant, complex values determined by the initial conditions; they are the coefficients of traveling helical waves∼$exp\left[i(\mathbf{k}·\mathbf{x}\pm {\mathsf{\Omega }}_{1,2}\left(\mathbf{k}\right)t)\right]$ if the ${\mathsf{\Omega }}_{1,2}\left(\mathbf{k}\right)$ are nonzero. If there is nonlinearity, then these coefficients will generally change with time and it is these changes that contain information about MHD turbulence.

3. Numerical Procedure

A Fourier spectral transform method [41] on an $N^3$ grid with $N=128$ is used; the minimum wave number is $k=\left|\mathbf{k}\right|=1$ and the maximum wave number is $K=\sqrt{3640}\simeq 60.34$. Time-integration is performed with a third-order Adams–Bashforth-Adams–Moulton method [42] with a time-step of $\Delta t=0.0005$; initial, non-equilibrium magnetic and kinetic modal energy values (spectra) are ${\overline{\mathcal{E}}}_M\left(k^2\right)$∼${\overline{\mathcal{E}}}_K\left(k^2\right)$∼$k^{4}exp(-k^2/k_{\mathrm{o}}^2)$, where $k_{\mathrm{o}}=6$. Viscosity and magnetic diffusivity are set to zero so that the flow is ideal. A grid size of ${128}^3$ was used so that the six single core Runs listed in Table 1 could be completed in a reasonable amount of time with the resources available, the Hopper Cluster at George Mason University, with each simulation running at ≈11 $\mathrm{s}/\Delta t$; thus, a single run of $2\times {10}^6\Delta t\mathrm{s}$ requires about 36 weeks of CPU time.

As mentioned, six computer simulations covering the five cases in Table 1 have been run and are identified in that table. The ideal invariants for each case are quadratic forms (global quantities) with terms that are scalar products of the vector Fourier coefficients $\tilde{\mathbf{u}}(\mathbf{k},t)$ and $\tilde{\mathbf{b}}(\mathbf{k},t)$, with $0<k\le K$, as defined in Appendix A. The partial differential equations for MHD in $\mathbf{x}$-space are given by (1) and (2), while the transformed set of ordinary differential equations in $\mathbf{k}$-space are given by (23) and (24). The set of equations in $\mathbf{k}$-space is a finite dynamical system, as discussed in Section 2.3. The $\mathbf{k}$-space Equations (23) and (24) are numerically integrated to advance the $\tilde{\mathbf{u}}(\mathbf{k},t)$ and $\tilde{\mathbf{b}}(\mathbf{k},t)$, as described in Section 3.

As seen in Table 1, the integral invariants of ideal MHD turbulence are the volume-averaged energy E and magnetic helicity $H_M$ when ${\mathbf{B}}_{\mathrm{o}}=0$, as well as the cross helicity $H_C$ when ${\mathsf{\Omega }}_{\mathrm{o}}=0$ and $H_P$ when ${\mathsf{\Omega }}_{\mathrm{o}}=\sigma {\mathbf{B}}_{\mathrm{o}}\ne 0$. 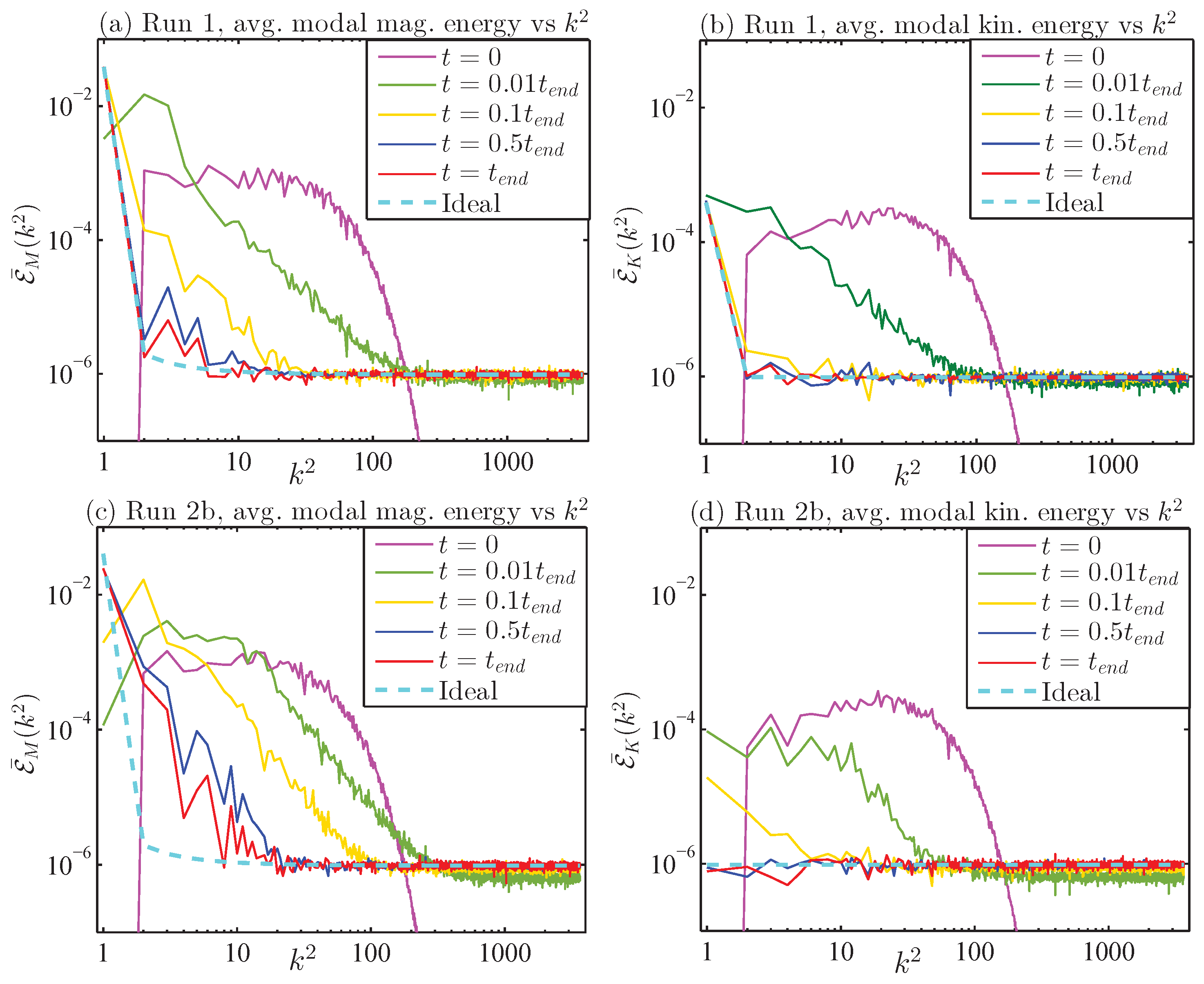In a numerical simulation, these ideal invariants typically have a standard deviation of less than 1% per million time-steps, while kinetic helicity $H_K$, though an ideal invariant for hydrodynamic turbulence, falls to zero very quickly and then has small fluctuations about that value, as Table 2 shows. We mentioned that these runs came to ‘near equilibrium’. To understand what this means, consider Figure 1 where we show, at different times for Runs 1 and 4, the average magnetic and kinetic energy spectra, ${\overline{\mathcal{E}}}_M\left(k^2\right)$ and ${\overline{\mathcal{E}}}_K\left(k^2\right)$, for modes $\mathbf{k}$ with the same value of $k^2={\left|\mathbf{k}\right|}^2$. There are 3036 different values of $k^2$, where $1\le k^2\le 3640$, for $N=128$; the number of independent $\mathbf{k}$ (i.e., $\mathbf{k}$ but not $-\mathbf{k}$) is $\mathsf{n}\left(k^2\right)$.

The definitions of the averaged spectra ${\overline{\mathcal{E}}}_M\left(k^2\right)$ and ${\overline{\mathcal{E}}}_K\left(k^2\right)$ are

$$\begin{array}{ccc}\hfill {\overline{\mathcal{E}}}_M\left(k^2\right)& =& \frac{1}{\mathsf{n}\left(k^2\right)}\sum _{\mathbf{k}}^{{\left|\mathbf{k}\right|}^2=k^2}\frac{|\tilde{b}{\left(\mathbf{k}\right)|}^2}{N^3},\hfill \\ \hfill \end{array}$$

(35)

$$\begin{array}{ccc}\hfill {\overline{\mathcal{E}}}_K\left(k^2\right)& =& \frac{1}{\mathsf{n}\left(k^2\right)}\sum _{\mathbf{k}}^{{\left|\mathbf{k}\right|}^2=k^2}\frac{|\tilde{u}{\left(\mathbf{k}\right)|}^2}{N^3}.\hfill \end{array}$$

(36)

Here, $\mathsf{n}\left(k^2\right)$ is the number of independent $\mathbf{k}$ that satisfy ${\left|\mathbf{k}\right|}^2=k^2$. The number $\mathsf{n}\left(k^2\right)$ jumps around as $k^2$ increases; for example,

$$\begin{array}{cccc}\mathsf{n}\left(1\right)=3,\hfill & \mathsf{n}\left(2\right)=6,\hfill & \mathsf{n}\left(3\right)=4,\hfill & \mathsf{n}\left(4\right)=3,\hfill \\ \mathsf{n}\left(5\right)=12,\hfill & \mathsf{n}\left(6\right)=12,\hfill & \mathsf{n}\left(7\right)=0\hfill & \cdots \hfill \\ \mathsf{n}\left(3628\right)=36,\hfill & \mathsf{n}\left(3629\right)=552,\hfill & \mathsf{n}\left(3630\right)=264,\hfill & \mathsf{n}\left(3631\right)=0,\hfill \\ \mathsf{n}\left(3632\right)=60,\hfill & \mathsf{n}\left(3633\right)=144,\hfill & \mathsf{n}\left(3634\right)=144\hfill & \cdots \hfill \end{array}$$

(37)

(We have $\mathsf{n}\left(k^2\right)=0$ whenever $k^2=4^a(8m+7),a,m=0,1,2,\cdots$; see [43]). The full energy spectra are $\mathsf{n}\left(k^2\right){\overline{\mathcal{E}}}_{M,K}\left(k^2\right)$ at each value of $k^2$ and thus jumps wildly as $k^2$ increases, which is why we prefer to look at the average energy spectra (35) and (36), as in Figure 1. (However, its running average over near neighbors $\overline{\mathsf{n}}\left(k^2\right)$ is well approximated by $\overline{\mathsf{n}}\left(k^2\right)=\pi k$.)

If we use the results in Appendix C or in [24], we find that the ideal expectation values of ${\overline{\mathcal{E}}}_M\left(k^2\right)$ and ${\overline{\mathcal{E}}}_K\left(k^2\right)$ are

$$\begin{array}{ccc}\hfill 〈{\overline{\mathcal{E}}}_M\left(k^2\right)〉& =& \frac{2}{N^3}\frac{\widehat{\alpha }{}^2}{{}^4-{\widehat{\alpha }}^2{\widehat{\gamma }}^2/k^2},\hfill \\ \hfill \end{array}$$

(38)

$$\begin{array}{ccc}\hfill 〈{\overline{\mathcal{E}}}_K\left(k^2\right)〉& =& \frac{2}{N^3}\frac{\widehat{\alpha }{(}^2-{\widehat{\gamma }}^2/k^2)}{{}^4-{\widehat{\alpha }}^2{\widehat{\gamma }}^2/k^2}.\hfill \end{array}$$

(39)

Here, ${}^2={\widehat{\alpha }}^2-{\widehat{\beta }}^2/4$ and $\widehat{\alpha }$, $\widehat{\beta }$ and $\widehat{\gamma }$ are the normalized inverse temperatures related to inverse temperatures appearing in the phase space probability density (A20); please see Appendix B for details. For each of the cases, $\widehat{\alpha }$, $\widehat{\beta }$ and $\widehat{\gamma }$ are determined by numerically finding the minimum of the entropy functional (A38) with the proviso that for Case II (Runs 2a and 2b), $\widehat{\beta }=0$; for Case III (Run 3), $\widehat{\gamma }=0$; and for Case V (Run 5), $\widehat{\beta }=\widehat{\gamma }=0$. The values of $\widehat{\alpha }$, $\widehat{\beta }$ and $\widehat{\gamma }$ for the different runs are given in

Table 3. Values of the inverse temperatures $\widehat{\alpha }$, $\widehat{\beta }$ and $\widehat{\gamma }$ for the Runs in Table 1 with the proviso that for Runs 2a and 2b, $\widehat{\beta }\equiv 0$; for Run 3, $\widehat{\gamma }\equiv 0$; and for Run 5, $\widehat{\beta }\equiv 0$ and $\widehat{\gamma }\equiv 0$. When needed, $\mathcal{E}$, ${\mathcal{H}}_C$, and ${\mathcal{H}}_M$ took their values from $E^{avg}$, $H_C^{avg}$ and $H_M^{avg}$, respectively, in Table 2. (The need for precision here is due to the possible smallness of the denominators in (38) and (39)).

Run	$\widehat{\mathit{\alpha }}$	$\widehat{\mathit{\beta }}$	$\widehat{\mathit{\gamma }}$
1	0.99078516013	$-0.19837547796$	$-0.98084304500$
2a	0.92168445878	0	$-0.92166079071$
2b	0.98388128912	0	$-0.98386939908$
3	0.83881629327	$-0.17608431628$	0
4	0.90195599886	0.42568629498	$-0.85137258997$
5	0.86630471441	0	0

.

The spectra are shown in Figure 1 for times $t=0$, $0.01t_{end}$, $0.1t_{end}$, $0.5t_{end}$ and $t_{end}$, where $t_{end}=1095$ for Run 1 and 1037 for Run2b. The spectra are approaching their expectation values and are near equilibrium; when they fully match the ideal expectation values, then they are in true equilibrium. The spectra ${\overline{\mathcal{E}}}_M\left(k^2\right)$ and ${\overline{\mathcal{E}}}_K\left(k^2\right)$ for Runs 3 and 5 will both be similar to those in Figure 1d, i.e., becoming flat, while the spectra for Run 4 will be similar to Figure 1a,b, though not as highly peaked at $k^2=1$.

4. Computational Results

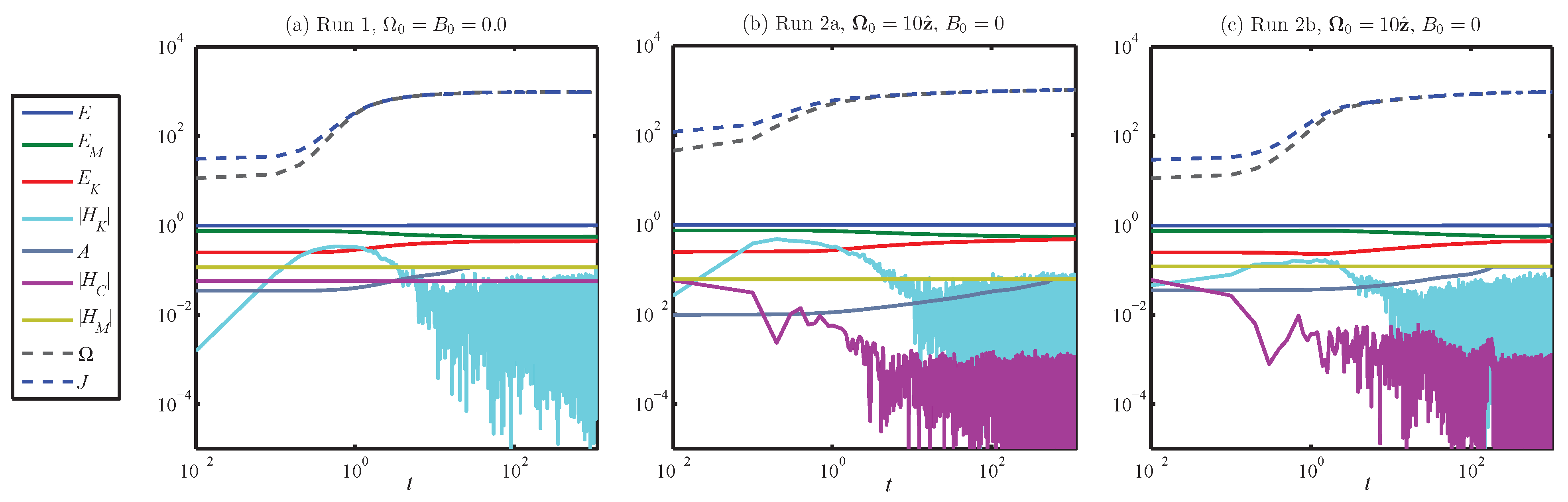
In Figure 2 and Figure 3, we show how the six runs in Table 1 change over time with respect to volume-averaged energy E, magnetic energy $E_M$, kinetic helicity $H_K$, mean squared magnetic vector potential A, cross helicity $H_C$, magnetic helicity $H_M$, enstrophy (mean squared vorticity) $\mathsf{\Omega }$ and mean squared electric current J; for Run 4, parallel helicity $H_P$ is also shown; again, please see Appendix A for precise definitions of these quantities. The time-averages and standard deviations for these quantities over the course of the runs are given in Table 2. For each run in Table 2, the quantities that are supposed to be conserved are, in fact, conserved as shown in Figure 2 and Figure 3. The most conserved quantity, by far, is the magnetic helicity $H_M$ in Runs 1, 2a and 2b. Those quantities in Table 2 that have standard deviations larger in magnitude than their average values are basically just fluctuating around zero; in particular, this seems to be true for the kinetic helicity $H_K$ in every run except for Run 4. Note also that statistics for the enstrophy $\mathsf{\Omega }$ and mean squared current J are essentially equal in each run, indicating that smaller length-scales have equipartition in magnetic and kinetic energy.

For all the runs in Table 1, the values of all the $\tilde{\mathbf{u}}(\mathbf{k},t)$ and $\tilde{\mathbf{b}}(\mathbf{k},t)$ with $k^2\le 3$ were saved every 0.1 units of simulation time t (i.e., every 200 $\Delta t\mathrm{s})$. From these, we can calculate a time history of modal energies

$$\begin{array}{ccc}\hfill E_K(\mathbf{k},t)& =& N^{-3}{\left|\tilde{\mathbf{u}}(\mathbf{k},t)\right|}^2,E_K^{\left(k\right)}\left(t\right)=\sum _{k=\left|\mathbf{k}\right|}{E}_K(\mathbf{k},t),\hfill \\ \hfill \end{array}$$

(40)

$$\begin{array}{ccc}\hfill E_M(\mathbf{k},t)& =& N^{-3}{\left|\tilde{\mathbf{b}}(\mathbf{k},t)\right|}^2,E_M^{\left(k\right)}\left(t\right)=\sum _{k=\left|\mathbf{k}\right|}{E}_M(\mathbf{k},t).\hfill \end{array}$$

(41)

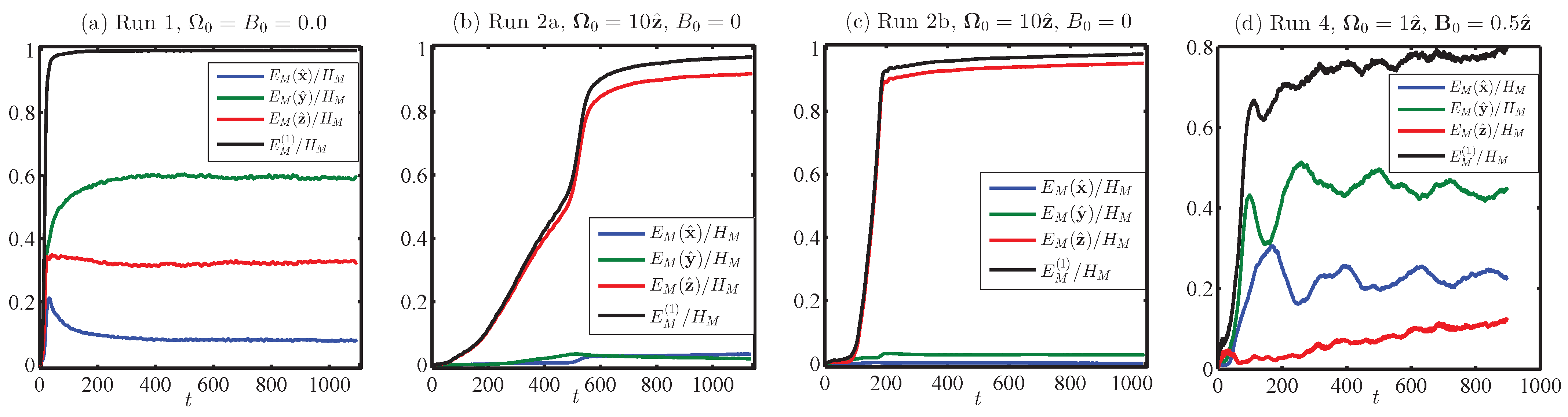
In Figure 4 and Figure 5, we see how the $E_M(\widehat{\mathbf{k}},t)$, $\widehat{\mathbf{k}}=\widehat{\mathbf{x}},\widehat{\mathbf{y}},\widehat{\mathbf{z}}$, for each run varies with time. In Figure 4, $E_M(\widehat{\mathbf{k}},t)$ and $E_M^{\left(1\right)}\left(t\right)$ are compared to $H_M\left(t\right)$ in each of Runs 1, 2a, 2b and 4; the result is compelling: $E_M^{\left(1\right)}\left(t\right)$ is tending to become equivalent to $H_M\left(t\right)$, even for Run 4 where $H_M\left(t\right)$ is not an integral invariant of the dynamical equations (instead, we use its value at $t=t_{end}$ for Run 4). This is again verification of the theoretical result $E_M^{\left(1\right)}=\left|H_M\right|$ given by [29,31], which also seems to apply to Run 4 because $H_M$ has reached a steady constant value. 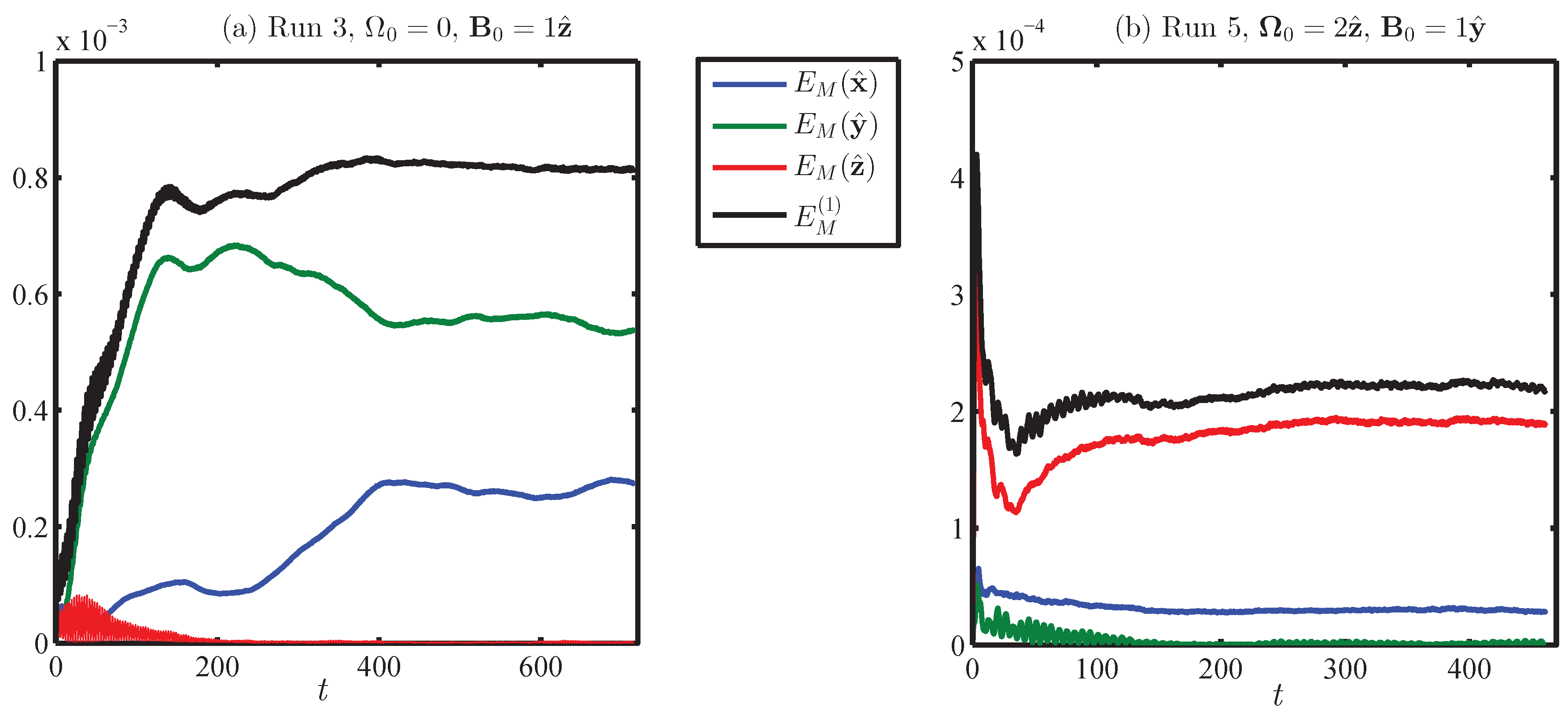Figure 5 shows the evolution of $E_M(\widehat{\mathbf{k}},t)$ for Runs 3 and 5; in these runs, $H_M\left(t\right)$∼0, as Table 2 shows. Furthermore, in Figure 5a (Case III) and Figure 5b (CaseV), we see $E_M(\widehat{\mathbf{k}},t)\approx 0$ for $\widehat{\mathbf{k}}$ aligned with ${\mathbf{B}}_{\mathrm{o}}$; additionally, in Figure 5b, $E_M(\widehat{\mathbf{k}},t)$ is largest for $\widehat{\mathbf{k}}$ aligned with ${\mathsf{\Omega }}_{\mathrm{o}}$, a ‘dipole alignment’ that has been seen before [29]. The ensemble prediction for both (a) and (b) in Figure 5 is $〈E_M^{\left(1\right)}〉=3/\left(4\mathcal{M}\right)\simeq 2\times {10}^{-7}$, which is to be equally divided among the three $E_M(\widehat{\mathbf{k}},t)$; thus, the steady values during transition here are several orders of magnitude too large, perhaps being supported by an increased magnetic energy inverse cascade during transition (and they do not correlate with $|H_M|$). Continuing these runs well into equilibrium (to be done) will determine whether these anomalous values persist.

In all six runs, a running time-average of the vector coefficients $\tilde{\mathbf{u}}(\mathbf{k},t)$ and $\tilde{\mathbf{b}}(\mathbf{k},t)$ was kept: ${\tilde{\mathbf{u}}}^{avg}(\mathbf{k},t)$ and ${\tilde{\mathbf{b}}}^{avg}(\mathbf{k},t)$. The expectation has been that these will tend to zero; however, this is not the case for $k=1$. These time-averaged vector coefficients can be used to calculate the ‘coherent total energy’ $E^C$, ‘coherent magnetic energy’ $E_M^C$ and ‘coherent kinetic energy’ $E_K^C$. The coherent kinetic and magnetic energies for the six runs are shown in Figure 6; the coherent kinetic energy $E_K^C$ levels off to a constant nonzero only for Run 1, as seen in Figure 6a, while the coherent magnetic energy $E_M^C$ levels off to a constant nonzero for Runs 1, 2a, 2b and 4, as seen in Figure 6b and these values of $E_M^C$ appear to approach their respective values of $|H_M|$, which are shown in Figure 6c. With regard to Runs 3 and 5, Figure 6a,b indicates what seems to be exponential fall-off, while Figure 6c shows that $H_M$ for Runs 3 and 5 is fluctuating about zero; thus, the anomalously high values of $E_M^{\left(1\right)}$ for Runs 3 and 5 seen in Figure 5 may only be transitory.

After numerical integration, components of the vectors $\tilde{\mathbf{u}}(\mathbf{k},t)$ and $\tilde{\mathbf{b}}(\mathbf{k},t)$ can be transformed into helical components ${\tilde{u}}_{+}(\mathbf{k},t)$, ${\tilde{u}}_{-}(\mathbf{k},t)$, ${\tilde{b}}_{+}(\mathbf{k},t)$ and ${\tilde{b}}_{-}(\mathbf{k},t)$, as discussed in Section 2.2. These are useful but can be further transformed into cyclic linear modes whose noncyclic factors are ${\tilde{V}}_1^{+}(\mathbf{k},t)$, ${\tilde{V}}_2^{+}(\mathbf{k},t)$, ${\tilde{V}}_1^{-}(\mathbf{k},t)$ and ${\tilde{V}}_2^{-}(\mathbf{k},t)$, as defined in Section 2.4. If there were no nonlinear interactions in the MHD equations, the ${\tilde{V}}_{1,2}^{\pm }(\mathbf{k},t)$ would be complex constants; however, the MHD equations are nonlinear, so the ${\tilde{V}}_{1,2}^{\pm }(\mathbf{k},t)$ may wander around with time. Since we have recorded data that can give us the time-histories of these for $k^2\le 3$, we can plot their trajectories on a complex plane and clearly see the nature of ideal MHD turbulence, at least at the larger length-scales (i.e., smaller wave numbers k). These ‘phase portraits’ are projections of the dynamical trajectory in the high-dimensional phase space onto a 2-D plane which enables us to visualize the concepts of coherent structure, broken ergodicity and broken symmetry (please see Appendix E for a review of these concepts). For the six runs discussed here, some phase portraits are shown in Figure 7, Figure 8, Figure 9, Figure 10, Figure 11, Figure 12 and Figure 13.

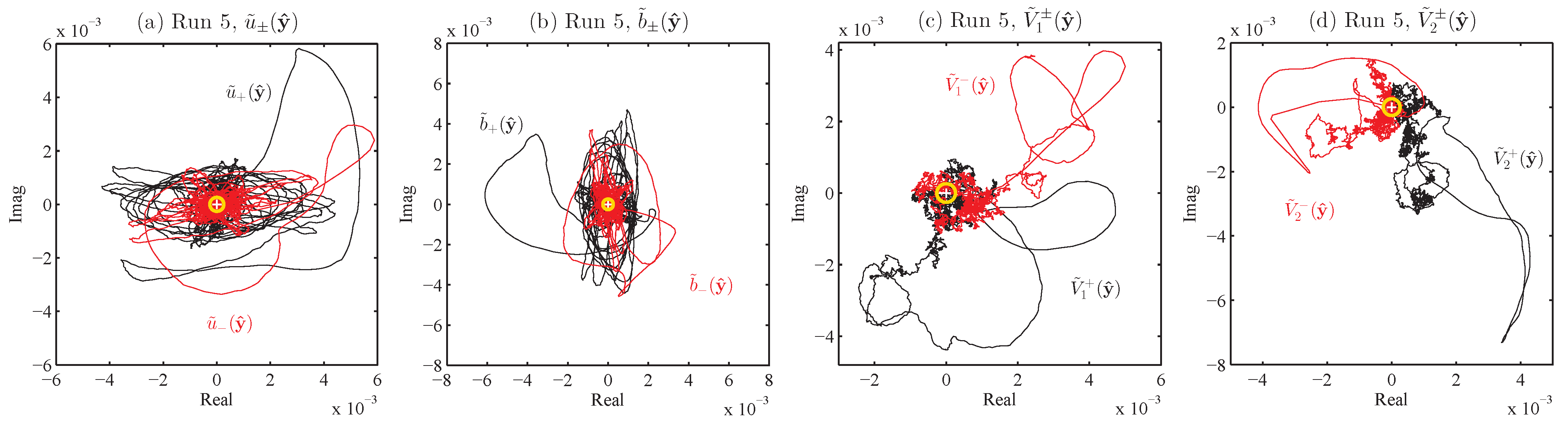
Phase portraits from Run 5 are presented in Figure 7. Referring to Section 2.4, we see that (with ${\mathsf{\Omega }}_1\left(\widehat{\mathbf{y}}\right)=0.5$ and ${\mathsf{\Omega }}_2\left(\widehat{\mathbf{y}}\right)=-0.5$)

$$\begin{array}{ccc}\hfill {\tilde{V}}_1^{\pm }(\widehat{\mathbf{y}},t)& =& \frac{1}{\sqrt{2}}exp[\mp i{\mathsf{\Omega }}_1\left(\widehat{\mathbf{y}}\right)t][{\tilde{b}}_{\pm }(\widehat{\mathbf{y}},t)+{\tilde{u}}_{\pm }(\widehat{\mathbf{y}},t)],\hfill \\ \hfill \\ \hfill {\tilde{V}}_2^{\pm }(\widehat{\mathbf{y}},t)& =& \frac{1}{\sqrt{2}}exp[\mp i{\mathsf{\Omega }}_2)\left(\widehat{\mathbf{y}}\right)t][{\tilde{b}}_{\pm }(\widehat{\mathbf{y}},t)-{\tilde{u}}_{\pm }(\widehat{\mathbf{y}},t)].\hfill \end{array}$$

(42)

The transformed variables ${\tilde{V}}_{1,2}^{\pm }(\widehat{\mathbf{y}},t)$ have no cyclic time variation and their evolution from initial values (which are all close to zero) shows the effect of the nonlinear terms in (23) and (24). In Figure 7, the ${\tilde{V}}_{1,2}^{\pm }(\widehat{\mathbf{y}},t)$ appear to be zero-mean random variables whose fluctuations match statistical expectations as to mean and standard deviation; in fact, all ${\tilde{V}}_{1,2}^{\pm }(\mathbf{k},t)$ for Case V of Table 1 are expected to be zero-mean random variables with the same standard deviation. (Please see Appendix B of a review of the statistical theory.)

However, if we take a look at Figure 8 corresponding to Run 5, we see the unexpected nonlinear behavior of (a) ${\tilde{V}}_2^{\pm }(\widehat{\mathbf{x}},t)$ and (c) ${\tilde{V}}_2^{\pm }(\widehat{\mathbf{z}},t)$; we compare this with the trajectory of ${\tilde{V}}_{1,2}^{\pm }(\widehat{\mathbf{y}},t)$ that is shown in Figure 8b (which is the same as Figure 7c). In other words, what we see in Figure 8a,c are not the expected zero-mean random variables of small fluctuation, but very large nonzero mean random variables of small fluctuation; we see ‘broken ergodicity’ (as discussed in Appendix E). The quasi-steady end stage of the trajectories of Figure 8a,c represent a quasi-stationary coherent structure in the magnetofluid. This is, at first, surprising, but nonetheless, it occurs: ergodicity is dynamically broken for Case V, at least during transition to equilibrium; however, longer run-times (which are planned) are needed to examine behavior in equilibrium. At the largest length-scales, where $k^2=1$, these coherent structures seem very robust during transition in Run 5 and in other runs where they appear, while at smaller length-scales, $k^2>1$, ‘random walk’ trajectories appear to be converging towards zero-mean, both for Run 5 and for the other Runs in Table 1; again, longer run-times are planned to study equilibrium behavior.

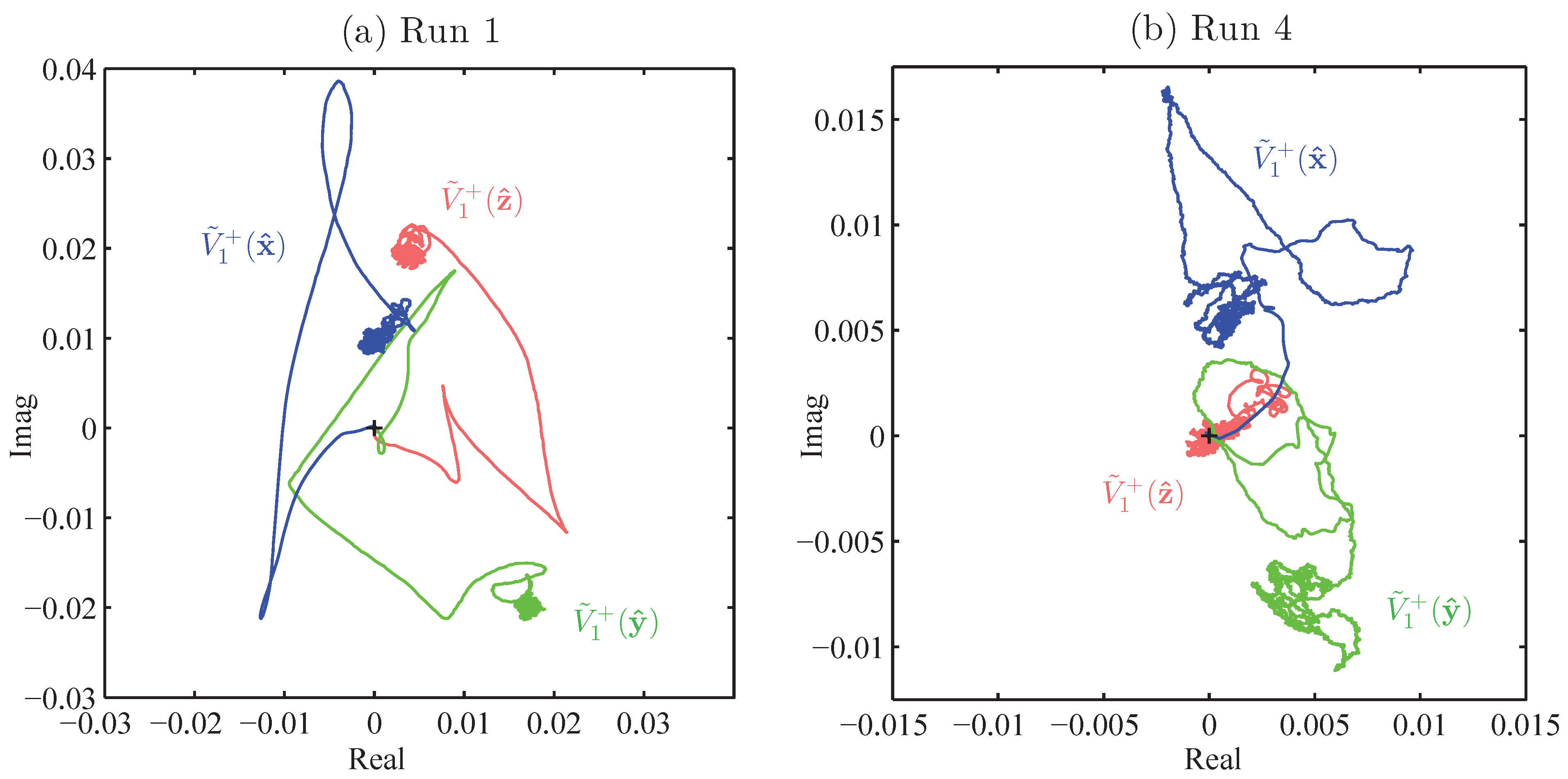
Phase portraits from Run 4 are shown in Figure 9. Parts (a) and (b) show phase trajectories of ${\tilde{u}}_{\pm }(\widehat{\mathbf{z}},t)$ and ${\tilde{b}}_{\pm }(\widehat{\mathbf{z}},t)$ before cyclic behavior is removed. In Figure 9c, an example is given of ${\tilde{V}}_1^{\pm }(\widehat{\mathbf{z}},t)$ trajectories where cyclicity is removed. In Figure 9d, quasi-stationarity indicates coherent structure. Again, statistical expectations are that all Case IV variables ${\tilde{V}}_{1,2}^{\pm }(\widehat{\mathbf{k}},t)$ have zero-mean; yet, they do not behave as expected and indicate that broken ergodicity has occurred. In fact, the behavior seen in Figure 9d is the same as that expected of Case II in Table 1, i.e., the three $k^2=1$ magnetic modes altogether have energy $|H_M|$ and thus, average magnitudes each of $\sqrt{|H_M|/3}=0.0269$. (In Figure 9d, $H_M^{avg}$ from Table 2 is used for $H_M$.) In Figure 9, these Run 4 ${\tilde{V}}_{1,2}^{\pm }(\widehat{\mathbf{k}},t)$ are, explicitly (with ${\mathsf{\Omega }}_1\left(\widehat{\mathbf{z}}\right)=2+\sqrt{5}\simeq 4.236$ and ${\mathsf{\Omega }}_2\left(\widehat{\mathbf{z}}\right)=2-\sqrt{5}\simeq -2.236$),

$$\begin{array}{ccc}\hfill {\tilde{V}}_1^{\pm }(\widehat{\mathbf{z}},t)& =& exp[\mp i{\mathsf{\Omega }}_1\left(\widehat{\mathbf{z}}\right)t][0.230{\tilde{b}}_{\pm }(\widehat{\mathbf{z}},t)+0.973{\tilde{u}}_{\pm }(\widehat{\mathbf{z}},t)],\hfill \\ \hfill \\ \hfill {\tilde{V}}_2^{\pm }(\widehat{\mathbf{x}},t)& =& {\tilde{b}}_{\pm }(\widehat{\mathbf{x}},t),{\tilde{V}}_2^{\pm }(\widehat{\mathbf{y}},t)={\tilde{b}}_{\pm }(\widehat{\mathbf{y}},t)\hfill \end{array}$$

(43)

$$\begin{array}{c}\hfill \\ \hfill {\tilde{V}}_2^{\pm }(\widehat{\mathbf{z}},t)& =& exp[\mp i{\mathsf{\Omega }}_2\left(\widehat{\mathbf{z}}\right)t][0.973{\tilde{b}}_{\pm }(\widehat{\mathbf{z}},t)-0.230{\tilde{u}}_{\pm }(\widehat{\mathbf{z}},t)].\hfill \end{array}$$

(44)

In Figure 9d, we also see ‘broken symmetry’ in that the magnitudes of the quasi-stationary values of ${\tilde{V}}_2^{+}(\widehat{\mathbf{x}},t)$, ${\tilde{V}}_2^{+}(\widehat{\mathbf{y}},t)$ and ${\tilde{V}}_2^{+}(\widehat{\mathbf{z}},t)$ are unequal.

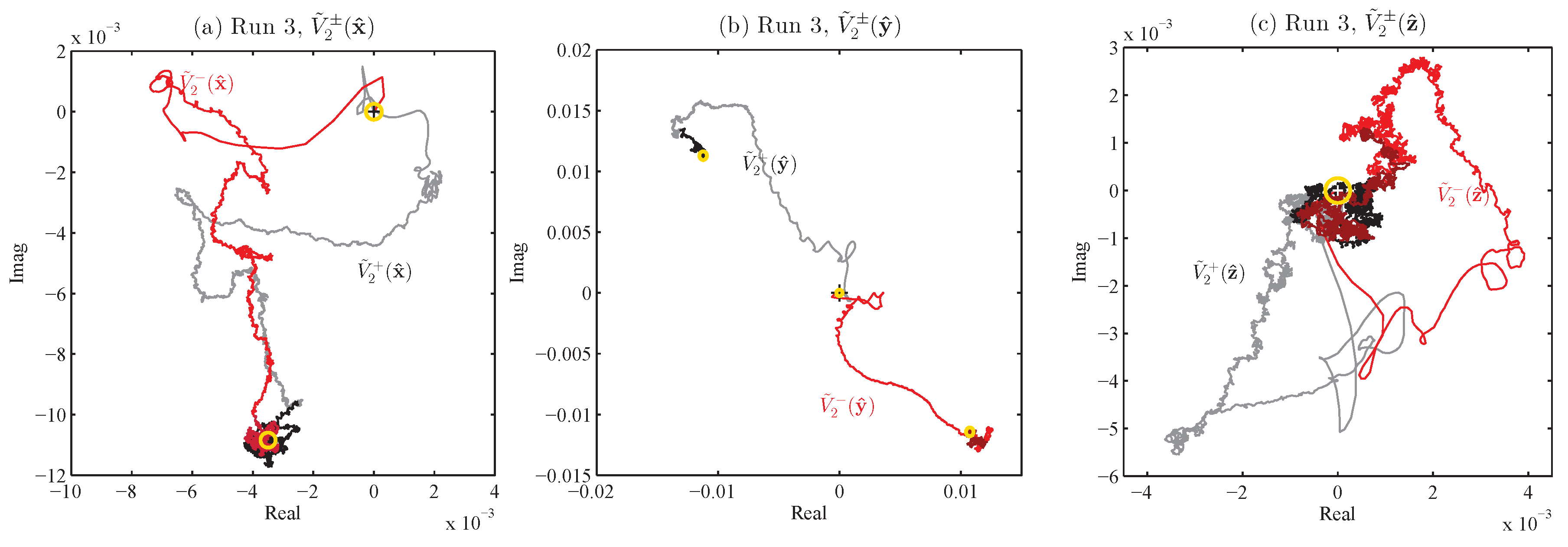
Phase portraits from Run 3 are shown in Figure 10. The yellow circles around the origin show the expected average magnitudes; in (a) and (b), these circles have also been placed around the ends of the trajectories, indicating that the fluctuation levels are as predicted, but the values are far from the predicted zero-mean, compared with the expected behavior seen in Figure 10c. In Figure 10, the ${\tilde{V}}_2^{\pm }(\widehat{\mathbf{k}},t)$ are [with ${\mathsf{\Omega }}_2\left(\widehat{\mathbf{z}}\right)=-1$], explicitly

$$\begin{array}{ccc}\hfill {\tilde{V}}_2^{\pm }(\widehat{\mathbf{x}},t)& =& {\tilde{b}}_{\pm }(\widehat{\mathbf{x}},t),{\tilde{V}}_2^{\pm }(\widehat{\mathbf{y}},t)={\tilde{b}}_{\pm }(\widehat{\mathbf{y}},t),\hfill \\ \hfill \\ \hfill {\tilde{V}}_2^{\pm }(\widehat{\mathbf{z}},t)& =& \frac{1}{\sqrt{2}}exp[\mp i{\mathsf{\Omega }}_2\left(\widehat{\mathbf{z}}\right)t][{\tilde{b}}_{\pm }(\widehat{\mathbf{z}},t)-{\tilde{u}}_{\pm }(\widehat{\mathbf{z}},t)].\hfill \end{array}$$

(45)

The statistical ensemble expectation is that the ${\tilde{V}}_{1,2}^{\pm }(\mathbf{k},t)$ for all $\mathbf{k}$, $0<k\le K$, in addition to being zero-mean, will have the same standard deviation, ${\left(4\mathcal{M}\right)}^{-1/2}=2.6\times {10}^{-4}$. In Figure 10a,b, we see that this expectation is not met and that, again, we have broken ergodicity manifested during transition as large-scale coherent structure. Additionally, the magnitudes at the ends of the trajectories in Figure 10a,b are much larger than predicted; whether or not these persist will be determined by allowing Run 3 to continue running, as is planned for the next phase of our work.

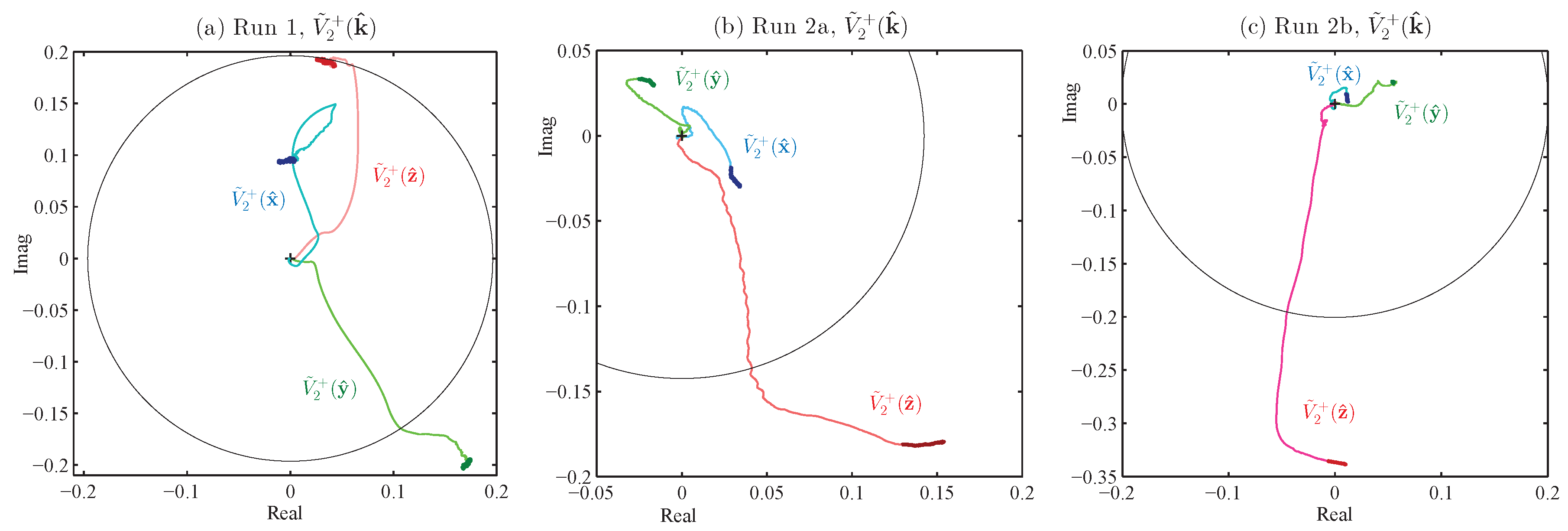
Phase portraits from Runs (a) 1, (b) 2a and (c) 2b are presented in Figure 11. Light parts are the full trajectory, while dark ends are from the last half of the trajectory, i.e., from $0.5t_{end}$ to $t_{end}$. The black circles have radius $\sqrt{H_M/3}$, where $H_M=H_M^{avg}$, is given for Runs 1, 2a and 2b in Table 2. In Figure 11, all ${\tilde{V}}_2^{+}(\widehat{\mathbf{k}},t)={\tilde{b}}_{+}(\widehat{\mathbf{k}},t)$. These phase trajectories all clearly show broken ergodicity and broken symmetry, for although their rms magnitudes are predicted, their quasi-stationarity is not and is, instead, a dynamical phenomenon. Now, we turn to Figure 12 for close-ups of ends of Run 1 trajectories in Figure 11a; these also serve as examples of the level of quasi-stationarity in Runs 2a and 2b.

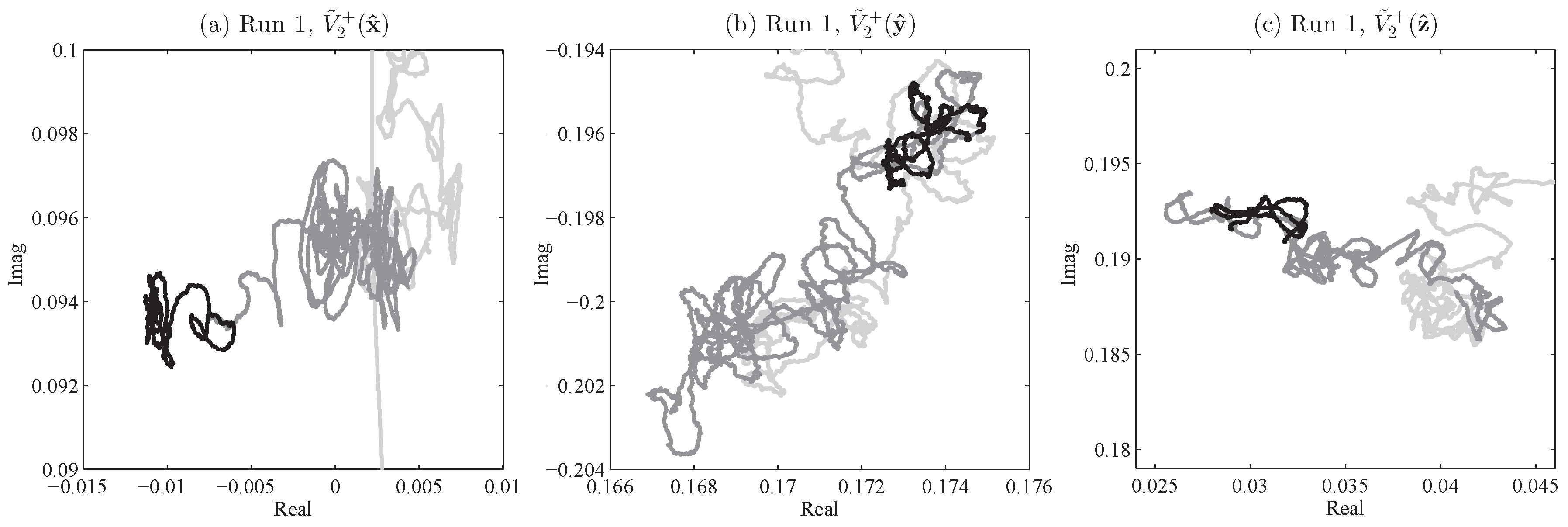
In Figure 12, we see close-up phase portraits of ${\tilde{V}}_2^{+}(\widehat{\mathbf{k}},t)$, $\widehat{\mathbf{k}}=$ (a) $\widehat{\mathbf{x}}$, (b) $\widehat{\mathbf{y}}$ and (c) $\widehat{\mathbf{z}}$, from the ends of the Run 1 phase trajectories shown in Figure 11a. Light gray is part of the full trajectory, while dark gray is the last half of the trajectory, i.e., from $0.5t_{end}$ to $t_{end}$, and the black part is from $0.9t_{end}$ to $t_{end}$. Again, in these figures, ${\tilde{V}}_2^{+}(\widehat{\mathbf{k}},t)={\tilde{b}}_{+}(\widehat{\mathbf{k}},t)$. The small extent of the parts from $0.9t_{end}$ to $t_{end}$ indicate the high level of quasi-stationarity in the coherent structure that has been created as MHD turbulence transitioned from an initially small-scale distribution of energy at $t=0$ to the states appearing in Figure 11; in this figure, Runs 2a and 2b are particularly relevant to understanding the origin of the MHD dynamo in rotating planets and stars: it is an inherent property of MHD turbulence. Again, Figure 12 presents a close-up example of the energetic, quasi-stationary, coherent structures that arise in MHD turbulence.

The energetic coherent structure generated by MHD turbulence is essentially magnetic. However, some coherent structure is exhibited by the velocity field, specifically in Runs 1 and 4. In Figure 13, you will find phase portraits of ${\tilde{V}}_1^{+}(\widehat{\mathbf{k}},t)$, where $\widehat{\mathbf{k}}=$$\widehat{\mathbf{x}}$, $\widehat{\mathbf{y}}$ and $\widehat{\mathbf{z}}$, for (a) Run 1 and (b) Run 4. These runs are the only ones with ${\tilde{V}}_1^{+}(\mathbf{k},t)$ variables (these are inertial waves) exhibiting coherent structure. These are all purely helical velocity variables ${\tilde{V}}_1^{+}(\widehat{\mathbf{k}},t)={\tilde{u}}_{+}(\widehat{\mathbf{k}},t)$, except in (b), where the one Run 4 variable exhibiting coherence is mostly kinetic but with a small part that is magnetic:

$$\begin{array}{ccc}\hfill {\tilde{V}}_1^{+}(\widehat{\mathbf{z}},t)& =& exp[-i{\mathsf{\Omega }}_1\left(\widehat{\mathbf{z}}\right)t][0.973{\tilde{u}}_{+}(\widehat{\mathbf{z}},t)+0.230{\tilde{b}}_{+}(\widehat{\mathbf{z}},t)],{\mathsf{\Omega }}_1\left(\widehat{\mathbf{z}}\right)=4.\hfill \end{array}$$

(46)

Once again, details of the variables ${\tilde{V}}_{1,2}^{\pm }(\mathbf{k},t)$ and their relation to the primary variables ${\tilde{u}}_{\pm }(\mathbf{k},t)$ and ${\tilde{b}}_{\pm }(\mathbf{k},t)$ can be found in Section 2.4.

5. Discussion

The primary discovery here is that all of the five Cases of ideal MHD turbulence in Table 1 make a transition from small-scale initial conditions into a near-equilibrium state that contains coherent structure. Since the ensemble prediction is that all variables have zero-mean, what we have is broken ergodicity (and broken symmetry; please see Appendix E). The most surprising phenomena are that Run 3 (Case III) and Run 5 (Case V) developed coherent structure during transition. This was not expected, although coherent structure was expected in Run 1 (Case I), as well as Runs 2a and 2b (both Case II), since similar coherent structure has been seen in numerical simulations of both ideal [24,44] and real [12,13] MHD turbulence.

Coherent structure is identified by quasi-stationary ${\tilde{V}}_{1,2}^{\pm }(\widehat{\mathbf{k}},t)$, as Figure 2, Figure 3, Figure 4, Figure 5, Figure 6, Figure 7, Figure 8, Figure 9, Figure 10, Figure 11, Figure 12 and Figure 13 show. This discovery was facilitated through Fourier spectral method numerical simulations on a ${128}^3$ grid. It has been shown with similar ${64}^3$ simulations that coherent structure also appears in dissipative MHD turbulence forced at an intermediate wavenumber and that these dissipative, forced results obey ideal MHD statistical theory at the largest wave lengths [12,13]. Furthermore, the statistical theory of ideal MHD turbulence developed for a periodic box has the same form as that developed for a spherical shell [30]. Therein lies the importance of ideal results to the real MHD turbulence contained in planetary liquid cores and in the convective layers of stars: it is a basic, qualitative explanation of how astrophysical dipole magnetic fields arise and thus, a solution to ‘the dynamo problem’.

MHD turbulence in astrophysical objects is covered by Cases I and II in Table 1 and as has been shown here with ${128}^3$ simulations, these are the cases with the most energetic coherent structure, i.e., quasi-stationary ‘dipole magnetic fields.’ The energy in the large-scale magnetic field is directly proportional to the total magnetic helicity in the turbulent magnetofluid; in the ideal case, this is set by initial conditions, while in the real case, the dipole field grows through inverse cascade and dynamic alignment as long as whatever forcing mechanism is present injects magnetic helicity of primarily one sign into the magnetofluid [12,13]. The exact nature of the helical forcing is hidden from view deep within an astrophysical object, but our results imply that it must be there.

Cases III, IV and V of Table 1 all contain an external magnetic field and are thus related to terrestrial experiments with turbulent magnetofluids, e.g., tokamaks, or perhaps to concentrated regions of plasma within stellar winds, for example, rather than whole planets and stars. The statistical mechanics of ideal MHD turbulence (see Appendix B) tells us that the modal energies of Cases III and V are expected to be equipartitioned, while Case IV is expected to have much more energy in the $k=1$ modes. Thus, the coherent structure seen in Runs 3 and 5 is a surprise, while in Run 4, the surprise is that it appears to be like Case I or II once $H_M$ reaches an essentially constant value.

6. Conclusions

In summary, the primary discovery here is that all of the five Cases of ideal MHD turbulence in Table 1 have developed quasi-stationary coherent structure. These coherent structures appear robust as the runs appear to have finished or are close to finishing transition from initial conditions to an equilibrium state. However, the appearance of coherent structure for Cases III and V during transition to equilibrium may be transitory and further long-term computation is needed to examine these cases once they fully reach equilibrium. The importance of the computational and theoretical results, particularly for Cases I and II which are most relevant for planets and stars, is that they show that MHD turbulence, per se, is a dynamo. We intend to continue these runs beyond the values of $t_{end}$ given in Table 2 in order to examine MHD turbulence while in full, long-term equilibrium; we will report on the results when we have them.

Another direction future investigations can take is to add dissipation and forcing to our ${128}^3$ simulations as was done on ${64}^3$ grids [12,13]. The methods employed to force a model magnetofluid at intermediate wavenumbers are speculative because physical forcing mechanisms are hidden from observation within a planet or star. Nevertheless, having used a variety of mid-wavenumber, helical forcing methods on ${64}^3$ grids, we have always seen behavior analogous to ideal MHD turbulence at the smallest wavenumber, which is the emergence of large-scale coherent magnetic structure whose energy is determined by the amount of magnetic helicity in the magnetofluid turbulence. We would expect to see this in ${128}^3$ simulations, along with perhaps some novel effects, but this remains to be done.

The methods used here, numerical simulation and statistical analysis, may also profitably be used in examining other dynamical systems that have associated integral invariants. One example is a model of quantum electrodynamic turbulence [45] in which the coupled Dirac and Maxwell equations were numerically solved in a manner similar to that used here for a magnetofluid. This work was based on classical field theory but allowed for a nonperturbative approach. Another possible application is to study geometrodynamic turbulence, i.e., the evolution of space-time from arbitrary initial conditions; a preliminary numerical simulation could be based on the Einstein equations in a synchronous reference frame [46] before moving on to more general reference frames. These seem to be ‘grand challenge problems’ but should yield interesting results in terms of mathematics with perhaps some compelling physical relevance.