CFD Analysis of Convective Heat Transfer in a Vertical Square Sub-Channel for Laminar Flow Regime
Abstract
:1. Introduction
2. Materials and Methods
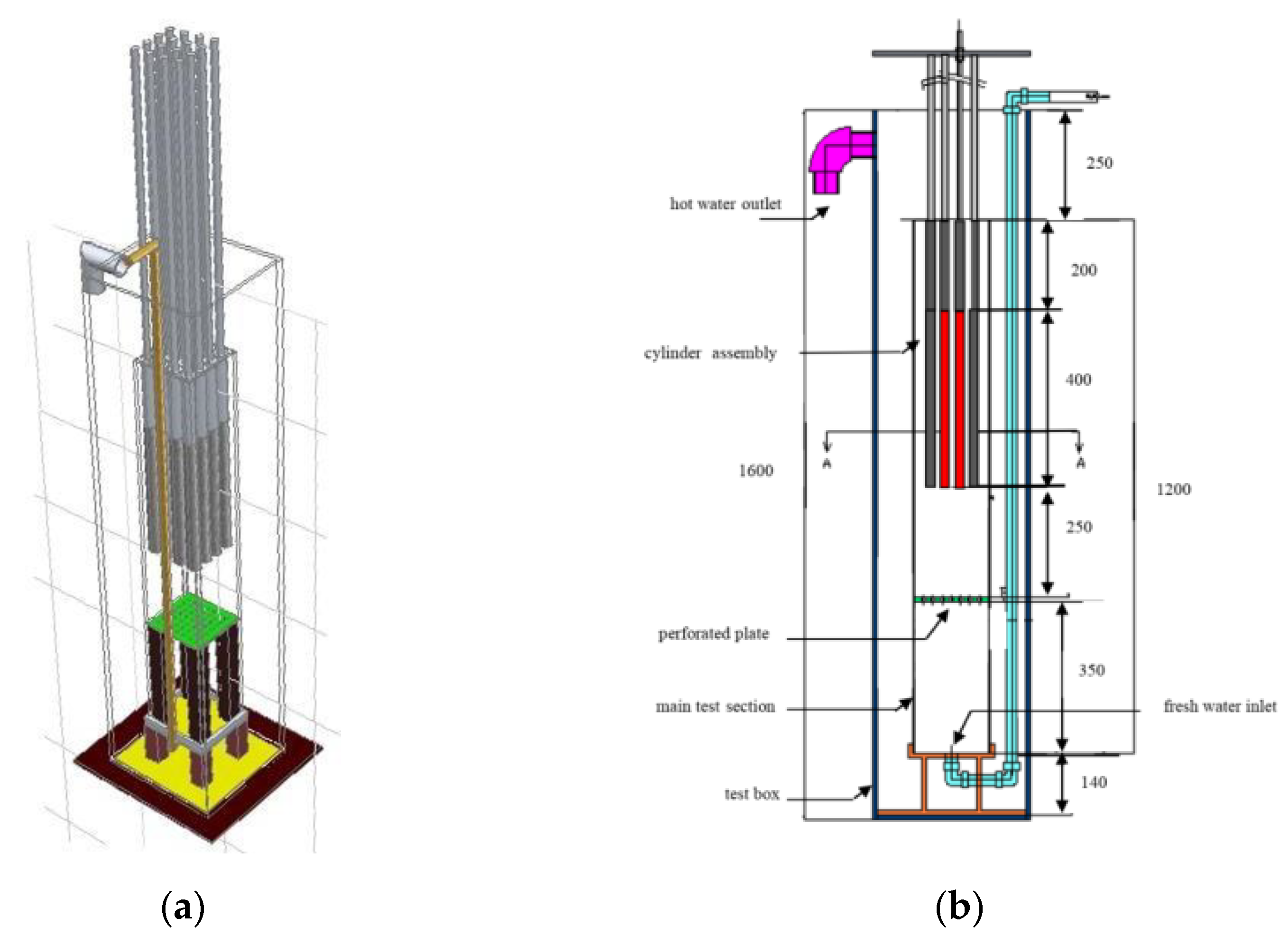
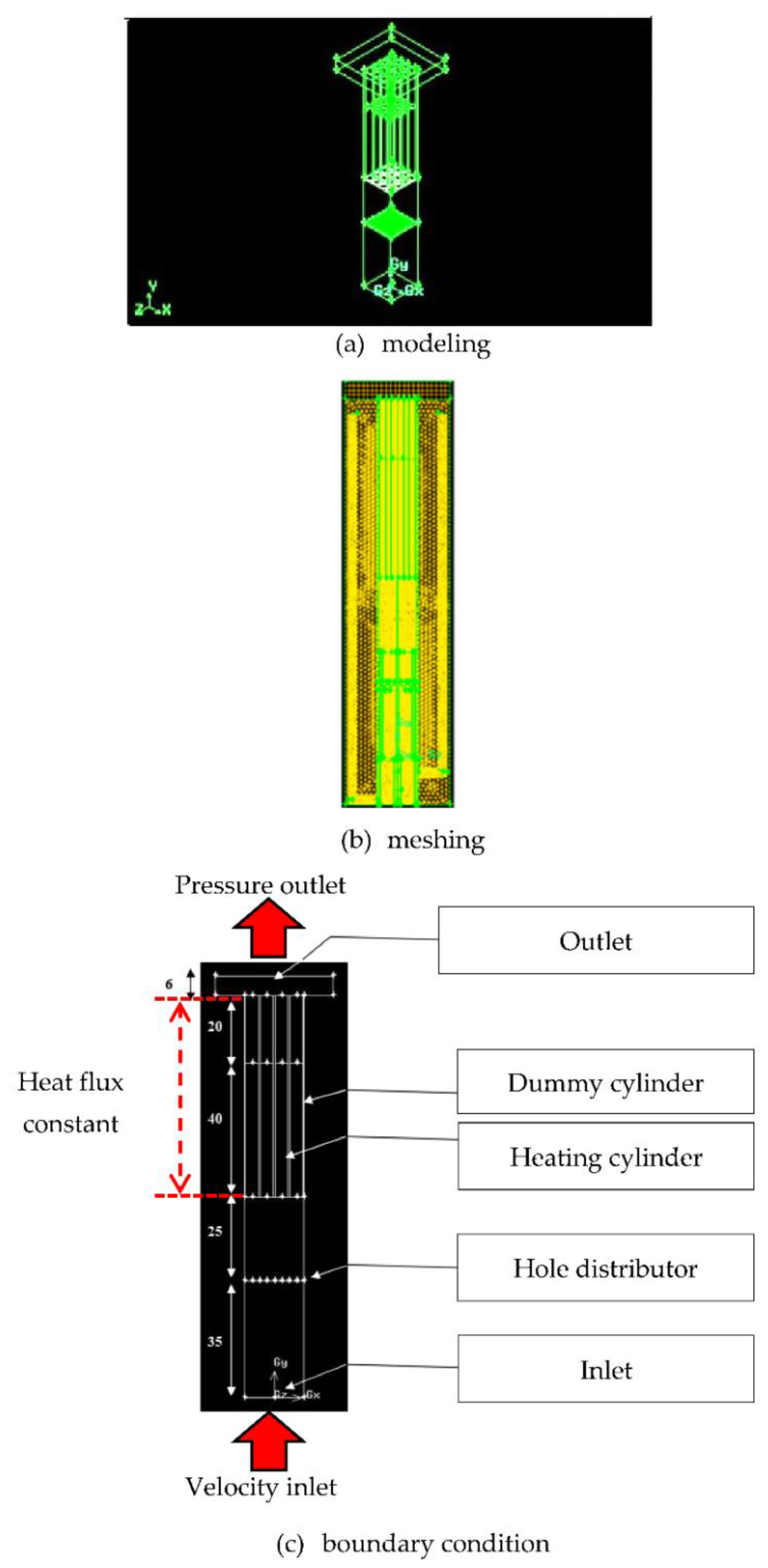
2.1. Numerical Model
2.2. Boundary Conditions
- The experiment reached its steady operating condition.
- Since the test section was opened to the atmosphere, the pressure at the water surface was constant at 1 bar, while pressures at other locations were hydrostatic pressures.
- The fresh water inlet entered the test section at a room temperature of 300 K.
- Gravity was taken as 9.8 m/s2.
- The physical properties of water followed its temperature and were obtained from the literature (Table 1).
| ρ (kg/m3) | µ (N·s/m2) | Cp (kJ/kg·K) | k (W/m·K) |
|---|---|---|---|
| 996.59 | 0.000852 | 4.179 | 0.6 |
| Boundary Type | Parameter | Value |
|---|---|---|
| Pressure outlet | Gauge pressure | 0 Pa |
| Backflow temperature | 298 K | |
| Wall (heater) | Heat flux | 100, 500, and 1000 W/m2 |
| Wall (stainless steel) | Heat flux | 0 W/m2 |
| Inlet | Velocity inlet Reynolds number (Re) | 0.7–1.0 m/s (400 ≤ Re ≤ 1700) |
2.3. Governing Equations
2.4. Parameters of Forced Convective Heat Transfer
3. Results and Discussion
3.1. Grid-Independent Test
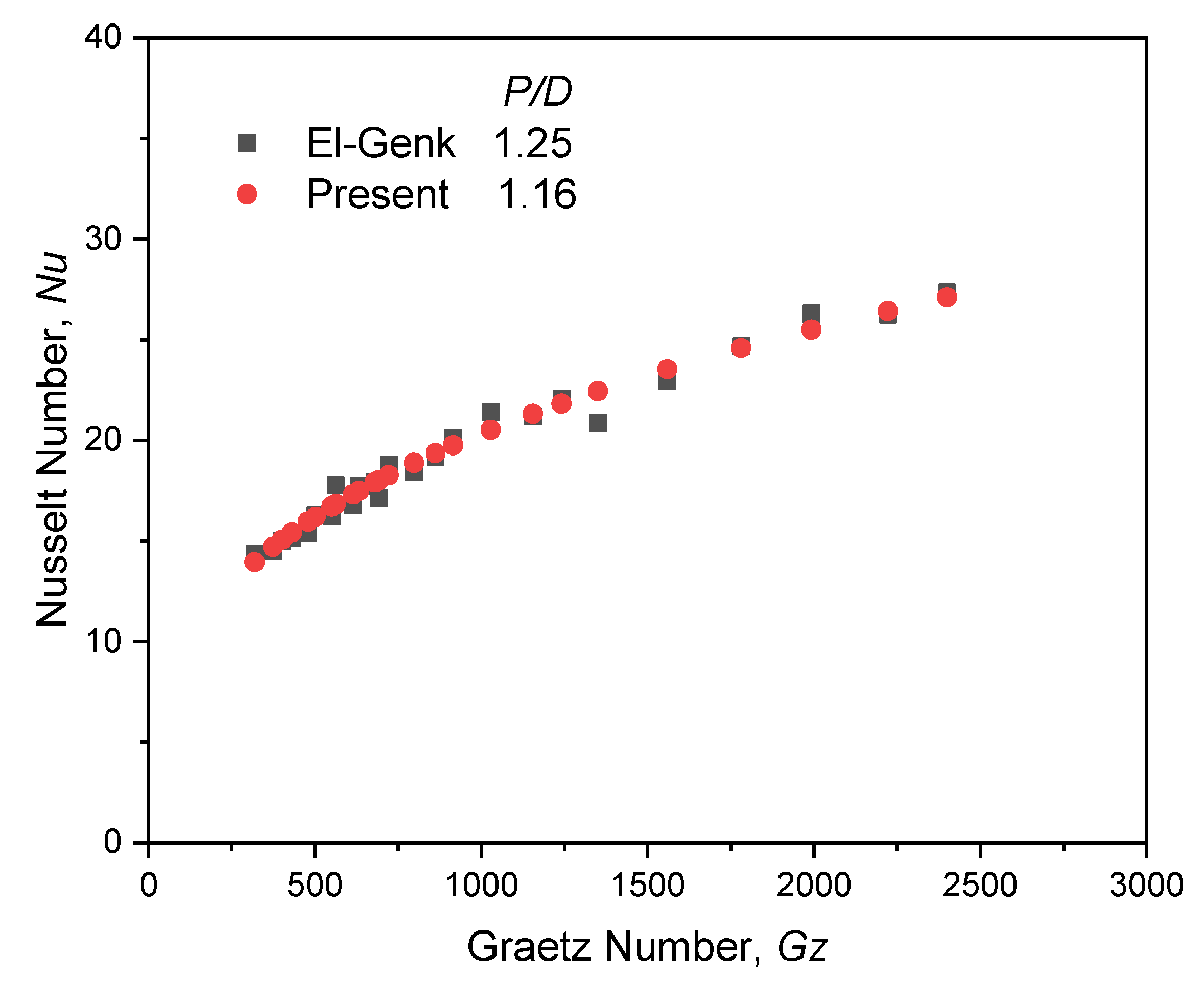
3.2. Validation of Results
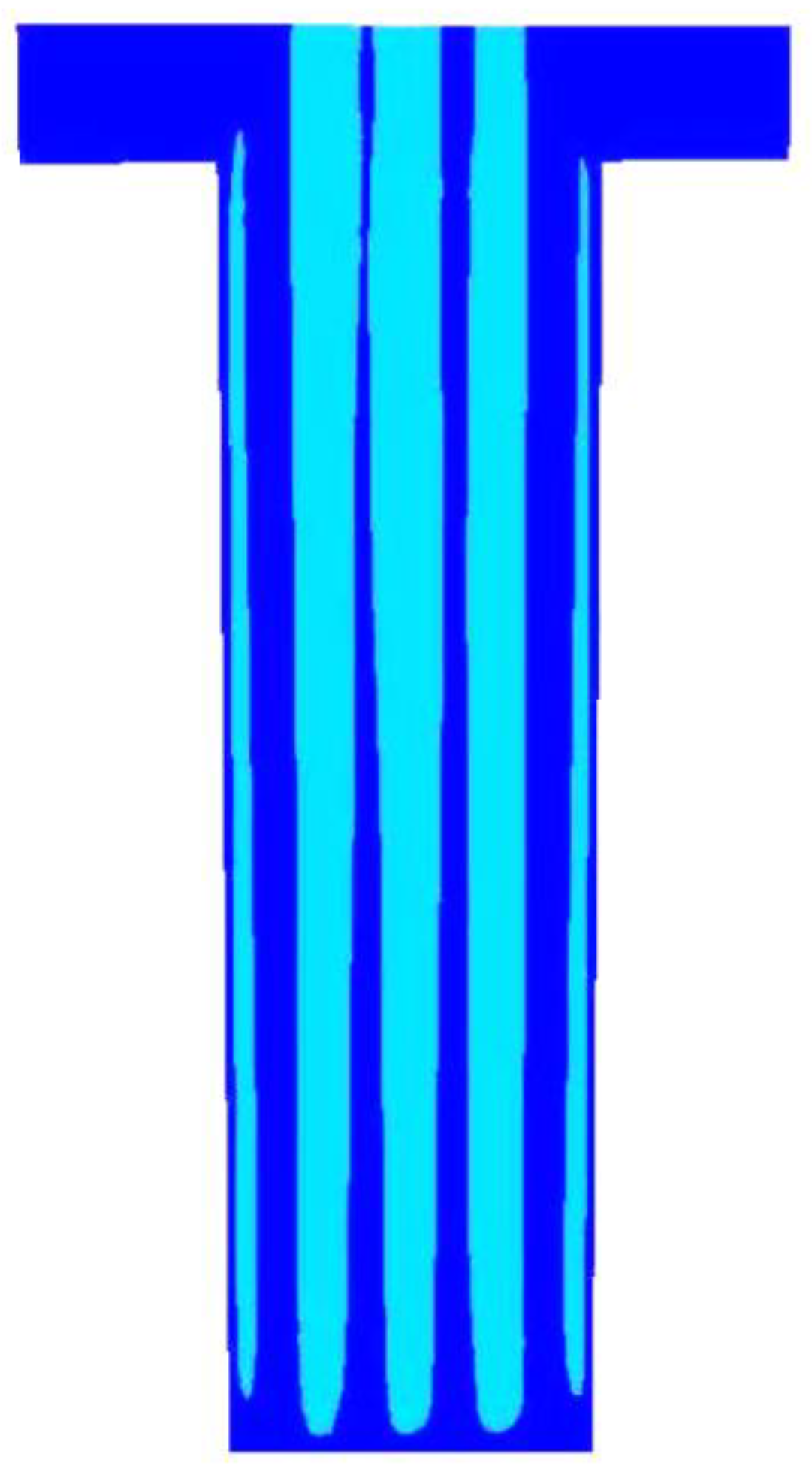
3.3. Fluid Flow Contour and Distribution of Velocity
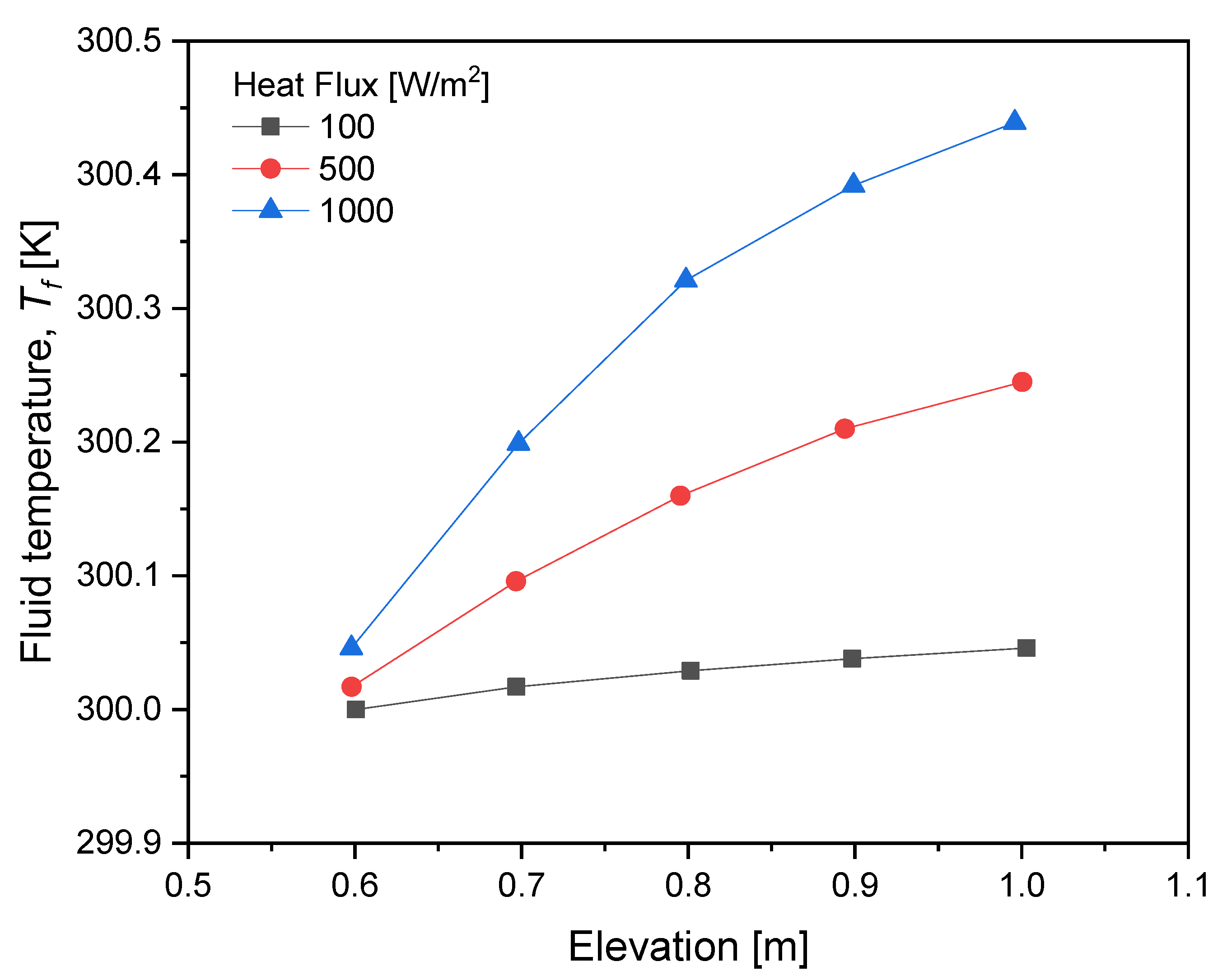
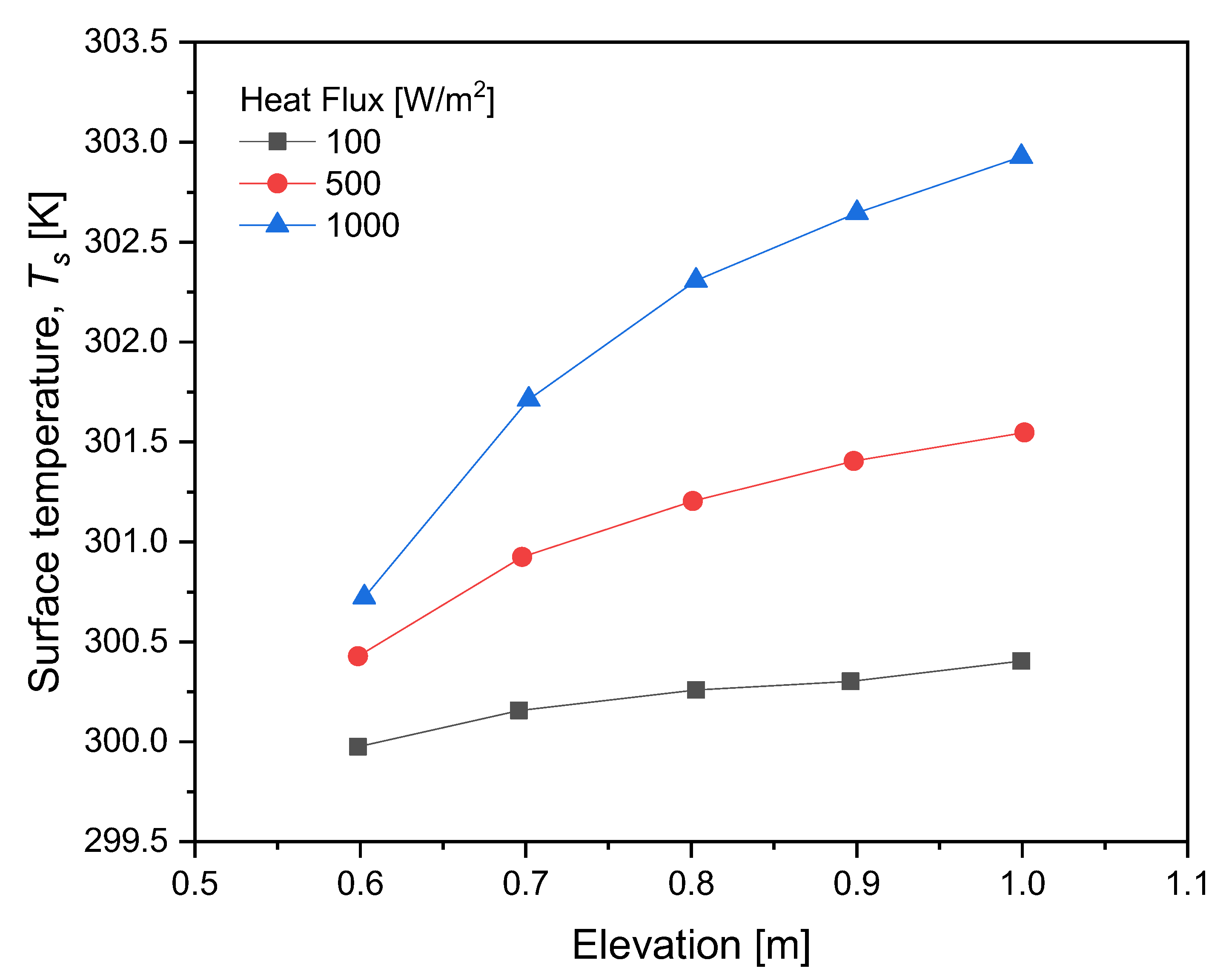
3.4. Temperature Distribution
3.5. Influences of Heat Flux
3.6. Heat Transfer Coefficient
3.7. Development of Forced Convective Heat Transfer Correlation
3.7.1. Physical Properties and Nusselt Number (Nu)
3.7.2. Graetz Number (Gz)
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Todreas, N.E.; Kazimi, M.S. Nuclear System I; Taylor and Francis Publishers: New York, NY, USA, 1993. [Google Scholar]
- Kreith, F.; Manglik, R.M.; Bohn, M.S. Principles of Heat Transfer, 7th ed.; CENGAGE Learning, Inc.: Stamford, CT, USA, 2011. [Google Scholar]
- Holman, J.P. Heat Transfer, 10th ed.; McGraw-Hill Education: New York, NY, USA, 2009. [Google Scholar]
- Oosthuizen, P.H.; David, N. Introduction to Convective Heat Transfer Analysis; McGraw Hill: New York, NY, USA, 1999. [Google Scholar]
- Gkounta, A.A.; Benos, L.T.; Sofiadis, G.N.; Sarris, I.E. A printed-circuit heat exchanger consideration by exploiting an Al2O3-water nanofluid: Effect of the nanoparticles interfacial layer on heat transfer. Therm. Sci. Eng. Progress 2021, 22, 100818. [Google Scholar] [CrossRef]
- Chauhan, A.K.; Prasad, B.V.S.S.S.; Patnaik, B.S.V. Thermal hydraulics of rod bundles: The effect of eccentricity. Nucl. Eng. Des. 2013, 263, 218–240. [Google Scholar]
- Jensen, R.T.; Newel, D.L. Thermal Hydraulic Calculations to Support to Support Increase in Operating power in Mc. Clellen Nuclear Radiation Center (MNRC) TRIGA Reactor; The 1998 RELAP5 International User’s Seminar: College Station, TX, USA, 17–22 May 1998. [Google Scholar]
- Marcum, W.R.; Woods, B.G.; Hartman, M.R.; Reese, S.R.; Palmer, T.S.; Keller, S.T. Steady state thermal-hydraulic analysis of the Oregon State University TRIGA reactor using RELAP5-3D. Nucl. Sci. Eng. 2009, 162, 261–274. [Google Scholar] [CrossRef]
- Reis, P.A.; Costa, A.L.; Pereira, C.; Veloso, M.F.; Mesquita, A.Z.; Soares, H.V.; Barros, G.P. Assessment of a RELAP5 model for the IPR-R1 TRIGA research reactor. Ann. Nucl. Energy 2010, 37, 1341–1350. [Google Scholar] [CrossRef]
- Huda, M.Q.; Bhuiyan, S.I. Investigation of thermo-hydraulic parameters during natural convection cooling of TRIGA reactor. Annu. Nucl. Energy 2006, 33, 1079–1086. [Google Scholar] [CrossRef]
- Waluyo, A.; Tandian, N.P.; Umar, E. Studi perpindahan panas konveksi pada susunan silinder vertikal dalam reaktor nuklir atau penukar panas menggunakan program CFD. In Proceedings of the Prosiding Seminar Nasional Sains dan TeknologiNuklir PTNBR, Bandung, Indonesia, 3 June 2009. [Google Scholar]
- Ramadhan, A.I.; Umar, E.; Tandian, N.P.; Suwono, A. Comparative analysis of preliminary design core of TRIGA Bandung using fuel element plate MTR in Indonesia. AIP Conf. Proc. 2017, 1799, 030007. [Google Scholar]
- Umar, E.; Kamajaya, K.; Tandian, N.P.; Hardianto, T.; Suwono, A. An experimental study of natural convection in the hot-test channel of TRIGA 2000 kW. In Proceedings of the Pacific Basin Nuclear Conference, Australian Nuclear Association, Sydney, Australia, 15–20 October 2006. [Google Scholar]
- Umar, E. Prediction of mass flow rate and pressure drop in the coolant channel of the TRIGA 2000 reactor core. At. Indones. 2001, 27, 67–84. [Google Scholar]
- Umar, E.; Kamajaya, K.; Ramadhan, A.I. Evaluation of the pump capability of the primary cooling of TRIGA 2000 research reactor. J. Phys. Conf. Ser. 2020, 1493, 012018. [Google Scholar] [CrossRef]
- Petersen, J.F. Manual of STAT-A Fortran Program for calculating the natural convection heat transfer—fluid flow in an array of heated cylinders, General Atomic. 1989. [Google Scholar]
- Basuki, P.; Umar, E. Thermal hydraulic analysis of the Bandung TRIGA 2000 research reactor. In Proceedings of the 12th International Conference on Thermo-Fluids, Yogyakarta, Indonesia, 18 September 2021. [Google Scholar]
- Sohag, F.A.; Mohanta, L.; Cheung, F. CFD analyses of mixed and forced convection in a heated vertical rod bundle. Appl. Therm. Eng. 2017, 117, 85–93. [Google Scholar] [CrossRef]
- Chen, G.; Zhang, Z.; Tian, Z.; Appah, T. Research on the Sub-channel analysis method via CFD analysis for PWR. Nucl. Sci. Eng. 2017, 188, 270–281. [Google Scholar] [CrossRef]
- Saha, P.; Aksan, N.; Andersen, J.; Yan, J.; Simoneau, J.P.; Leung, L.; Bertrand, F.; Aoto, K.; Kamide, H. Issues and future direction of thermal-hydraulics research and development in nuclear power reactors. Nucl. Eng. Des. 2013, 264, 3–23. [Google Scholar] [CrossRef]
- Ferziger, J.H.; Perić, M. Computational Methods for Fluid Dynamics; Springer: Berlin, Germany, 2002. [Google Scholar]
- Henry, R.; Tiselj, I.; Matkovic, M. Natural and mixed convection in the cylindrical pool of TRIGA reactor. Heat Mass Transf. 2017, 53, 537–551. [Google Scholar] [CrossRef]
- Fiantini, R.; Umar, E. Fluid flow characteristics simulation on the original TRIGA 2000 reactor core design using computational fluid dynamics code. AIP Conf. Proc. 2010, 1244, 215–223. [Google Scholar]
- Umar, E.; Fiantini, R. Modification of the core cooling system of TRIGA 2000 reactor. AIP Conf. Proc. 2010, 1244, 224–231. [Google Scholar]
- Sarris, I.E.; Tsiakaras, P.; Song, S.; Vlachos, N. A three-dimensional CFD model of direct ethanol fuel cells: Anode flow bed analysis. Solis State Ion. 2006, 177, 2133–2213. [Google Scholar] [CrossRef]
- El-Genk, M.; Su, B.; Zhanxiong, G. Experimental studies of forced, combined and natural convection of water in vertical nine-rod bundles with a square lattice. Int. J. Heat Mass Transf. 1993, 36, 2359–2374. [Google Scholar] [CrossRef]
- Tandian, N.P.; Umar, E.; Hardianto, T.; Febriyanto, C. Experimental study of natural convective heat transfer in a vertical hexagonal sub channel. AIP Conf. Proc. 2012, 1448, 252–260. [Google Scholar]
- Mohanta, L.; Cheung, F.; Hoxie, C.L. Experimental study of laminar mixed convection in a rod bundle with mixing vane spacer grids. Nucl. Eng. Des. 2017, 312, 99–105. [Google Scholar] [CrossRef]
- Ramadhan, A.I.; Suwono, A.; Umar, E.; Tandian, N.P. Preliminary study for design core of nuclear research reactor of TRIGA Bandung using fuel element plate MTR. Eng. J. 2017, 21, 173–181. [Google Scholar] [CrossRef] [Green Version]
- Li, J.; Xiao, Y.; Zhang, Q. Development of a correlation for mixed convection heat transfer in rod bundles. Ann. Nucl. Energy 2021, 155, 108151. [Google Scholar] [CrossRef]
- Liu, D.; Gu, H. Mixed convection heat transfer in a 5 × 5 rod bundles. Int. J. Heat Mass Transf. 2017, 113, 914–921. [Google Scholar] [CrossRef]
- Liu, B.; He, S.; Moulinec, C.; Uribe, J. Sub-Channel CFD for nuclear fuel bundles. Nucl. Eng. Des. 2019, 355, 110318. [Google Scholar] [CrossRef]
- Ramadhan, A.I.; Lasman, A.N.; Septilarso, A. Correlation equations of heat transfer in nanofluid Al2O3-water as cooling fluid in a rectangular sub channel based CFD code. Int. J. Sci. Eng. 2015, 8, 120–124. [Google Scholar]
- Shamim, J.A.; Bhowmik, P.K.; Xiangyi, C.; Suh, K.Y. A new correlation for convective heat transfer coefficient of water–alumina nanofluid in a square array sub channel under PWR condition. Nucl. Eng. Des. 2016, 308, 194–204. [Google Scholar] [CrossRef]
- Debrah, S.K.; Shitsi, E.; Chabi, S.; Sahebi, N. Assessment of heat transfer correlations in the sub-channels of proposed rod bundle geometry for supercritical water reactor. Heliyon 2019, 5, e02927. [Google Scholar] [CrossRef] [PubMed]
- Chen, S.; Liu, D.; Liu, M.; Xiao, Y.; Gu, H. Numerical simulation and analysis of flow and heat transfer in a 5 × 5 vertical rod bundle with buoyancy effects. Appl. Therm. Eng. 2019, 163, 114221. [Google Scholar] [CrossRef]
- Qiu, Q.; Du, X.; Zhao, S.; Zhu, X.; Shen, S. Numerical study on heat transfer of SCW near the pseudo-critical temperature in a hexagon sub-channel. Nucl. Eng. Des. 2018, 331, 263–273. [Google Scholar] [CrossRef]
- Gu, H.Y.; Hu, Z.X.; Liu, D.; Li, H.B.; Zhao, M.; Cheng, X. Experimental study on heat transfer to supercritical water in 2 × 2 rod bundle with wire wraps. Exp. Therm. Fluid Sci. 2016, 70, 17–28. [Google Scholar] [CrossRef]
- Chen, J.; Gu, H.; Xiong, Z. A circumferentially non-uniform heat transfer model for sub channel analysis of tight rod bundles. Ann. Nucl. Energy 2018, 121, 50–61. [Google Scholar] [CrossRef]
- Dittus, W.; Boelter, L.M.K. Heat Transfer in Automobile Radiators of the Turbular Type. Int. Commun. Heat Mass Transf. 1985, 12, 3–22. [Google Scholar] [CrossRef]
- Kamajaya, K.; Hasanah, A.; Pane, J.S. Forced convection heat transfer studies on Al2O3-water nano-fluids in a vertical sub-channels. AIP Conf. Proceeding 2018, 1488, 261–269. [Google Scholar]
- El-Genk, M.; Bedrose, S.D.; Rao, D. Forced and combined convection of water in rod bundles. Heat Transf. Eng. 1990, 11, 32–43. [Google Scholar] [CrossRef]

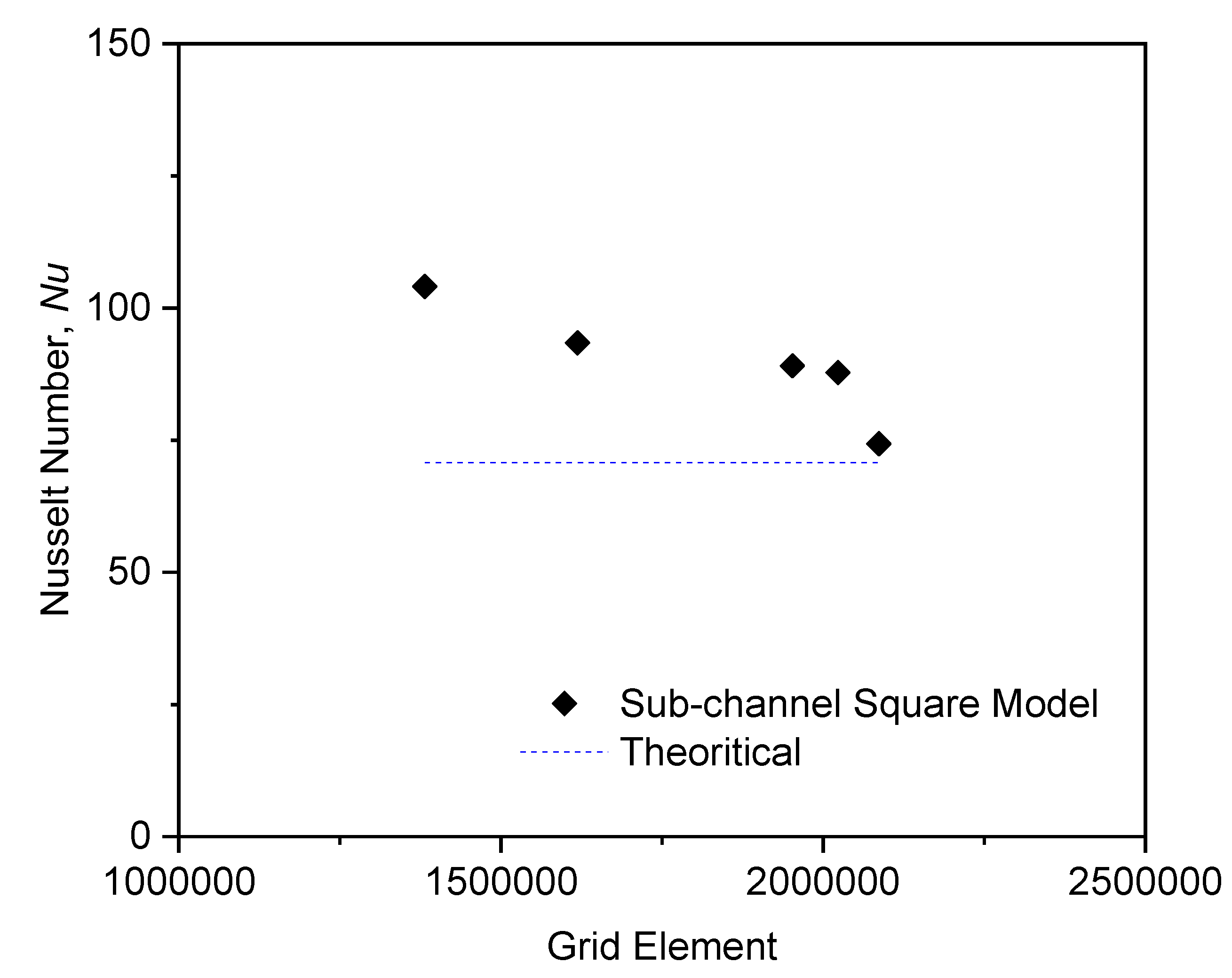

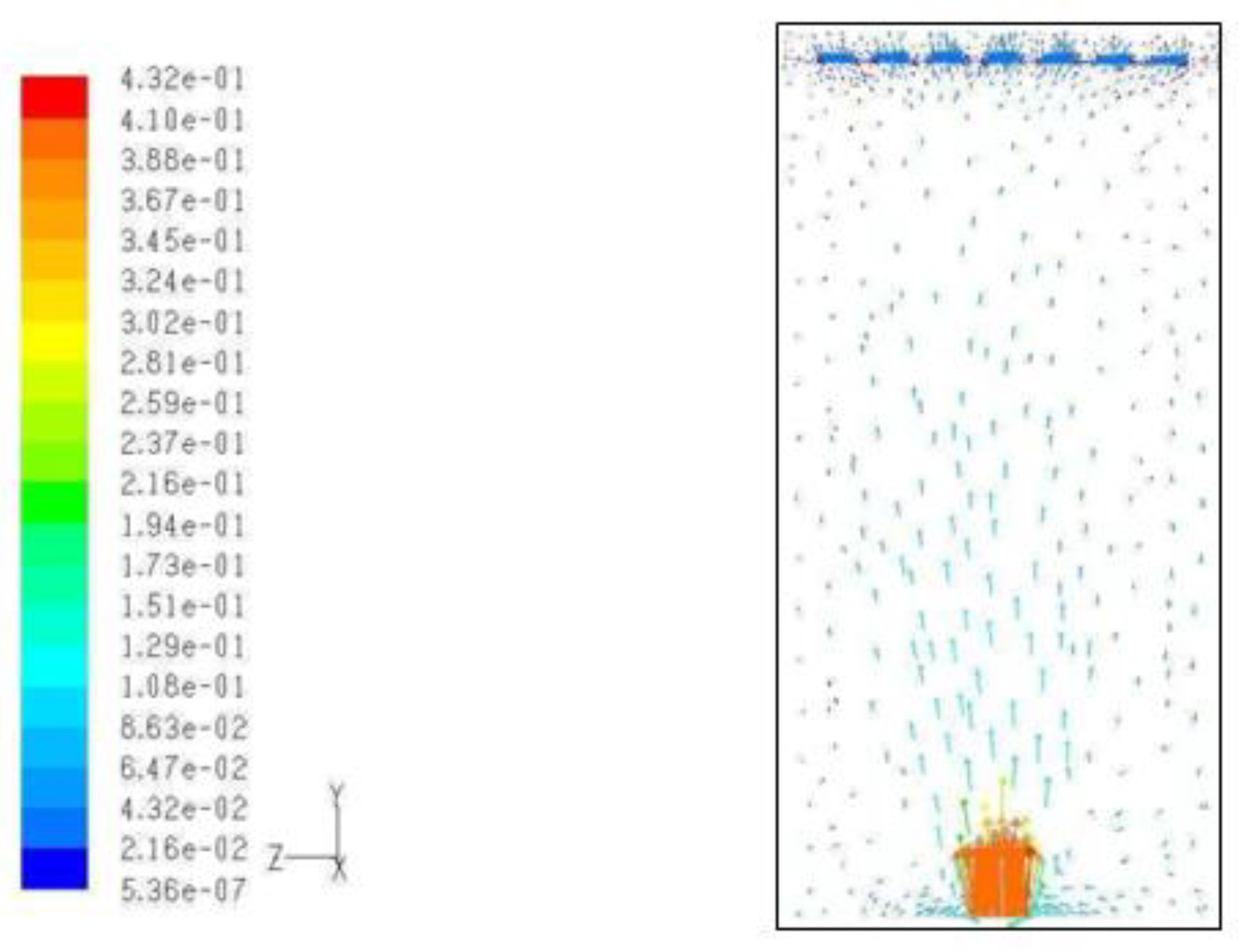
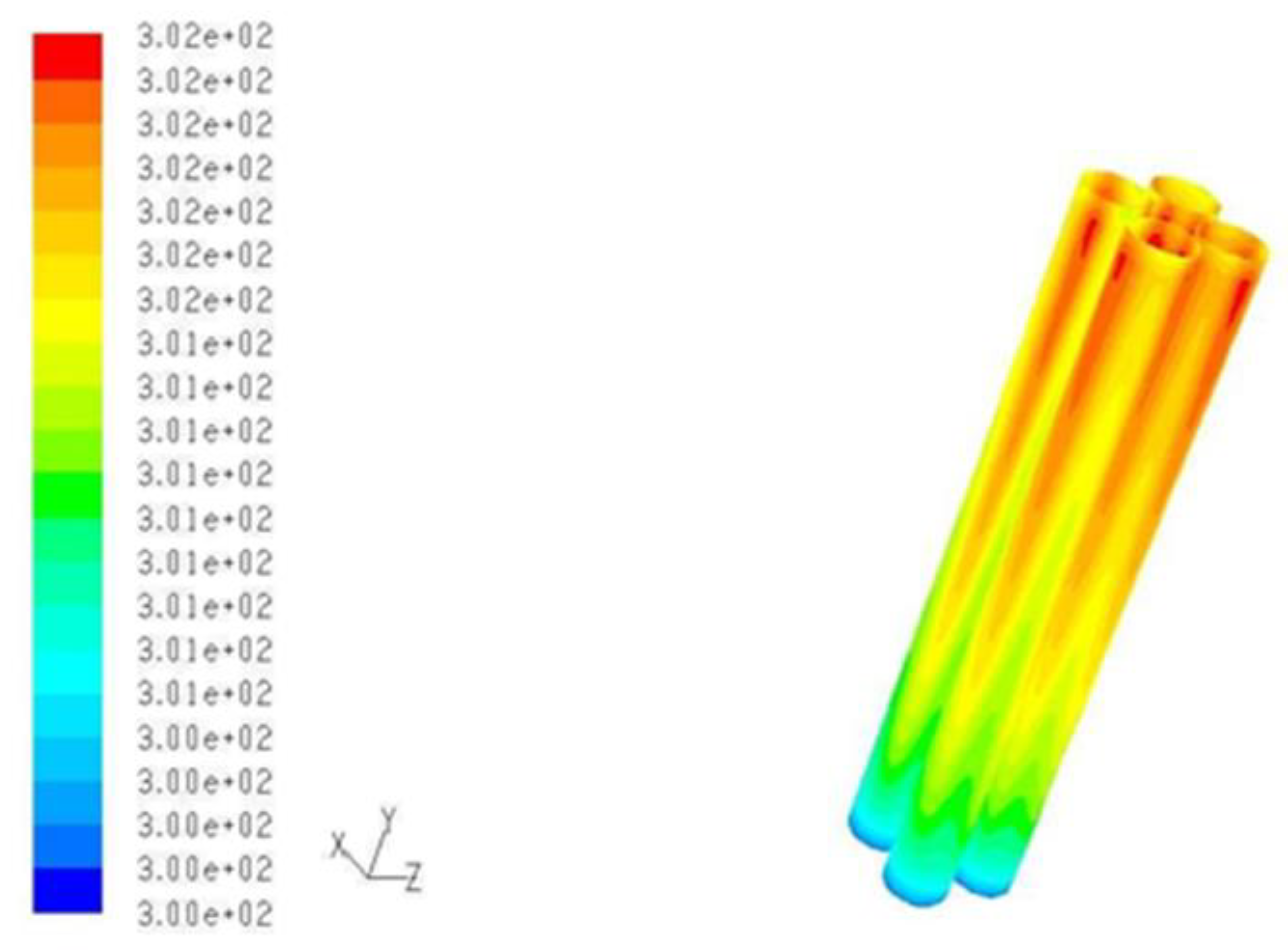
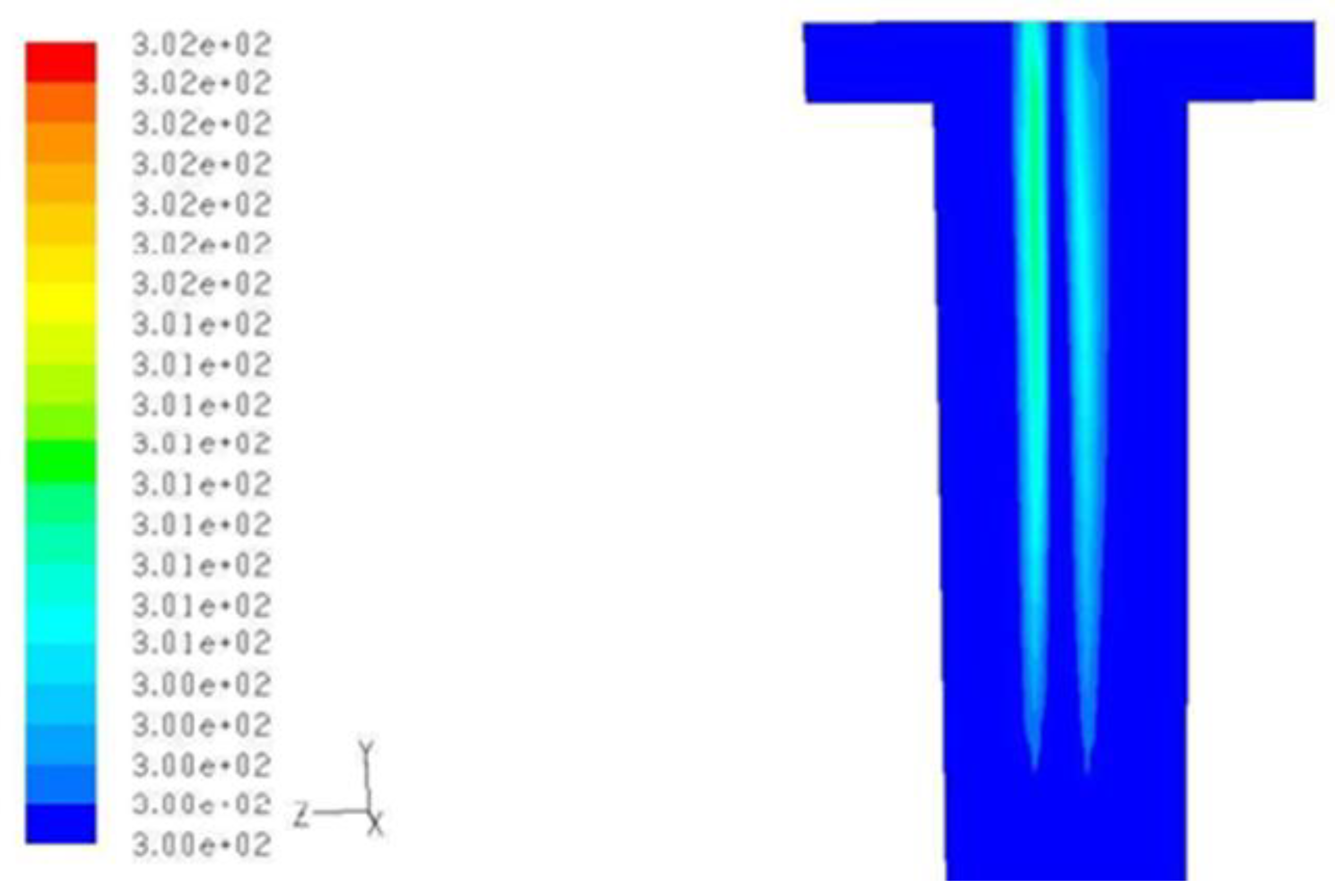
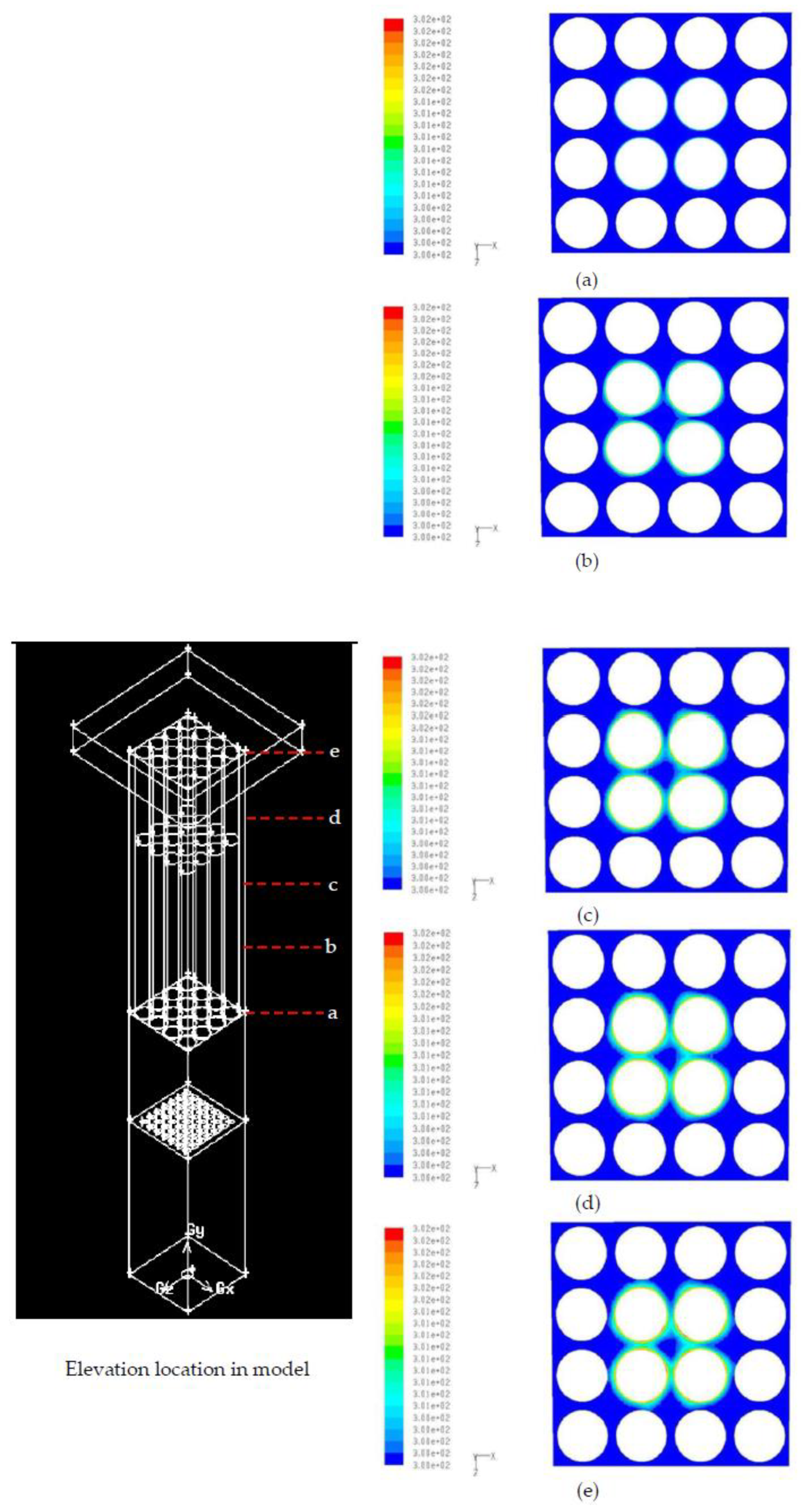



| Mesh Size (mm) | Grid Element | Nu | Error (%) |
|---|---|---|---|
| 0.1 | 1,381,804 | 104.0639 | 47.048 |
| 0.2 | 1,618,680 | 93.41835 | 32.006 |
| 0.3 | 1,952,379 | 89.07538 | 25.869 |
| 0.4 | 2,023,097 | 87.80597 | 24.075 |
| 0.5 | 2,086,436 | 74.35284 | 5.0652 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Umar, E.; Tandian, N.P.; Syuryavin, A.C.; Ramadhan, A.I.; Prayitno, J.H. CFD Analysis of Convective Heat Transfer in a Vertical Square Sub-Channel for Laminar Flow Regime. Fluids 2022, 7, 207. https://doi.org/10.3390/fluids7060207
Umar E, Tandian NP, Syuryavin AC, Ramadhan AI, Prayitno JH. CFD Analysis of Convective Heat Transfer in a Vertical Square Sub-Channel for Laminar Flow Regime. Fluids. 2022; 7(6):207. https://doi.org/10.3390/fluids7060207
Chicago/Turabian StyleUmar, Efrizon, Nathanael Panagung Tandian, Ahmad Ciptadi Syuryavin, Anwar Ilmar Ramadhan, and Joko Hadi Prayitno. 2022. "CFD Analysis of Convective Heat Transfer in a Vertical Square Sub-Channel for Laminar Flow Regime" Fluids 7, no. 6: 207. https://doi.org/10.3390/fluids7060207
APA StyleUmar, E., Tandian, N. P., Syuryavin, A. C., Ramadhan, A. I., & Prayitno, J. H. (2022). CFD Analysis of Convective Heat Transfer in a Vertical Square Sub-Channel for Laminar Flow Regime. Fluids, 7(6), 207. https://doi.org/10.3390/fluids7060207






