Puncture of a Viscous Liquid Film Due to Droplet Falling
Abstract
:1. Introduction
2. Materials and Methods
3. Results
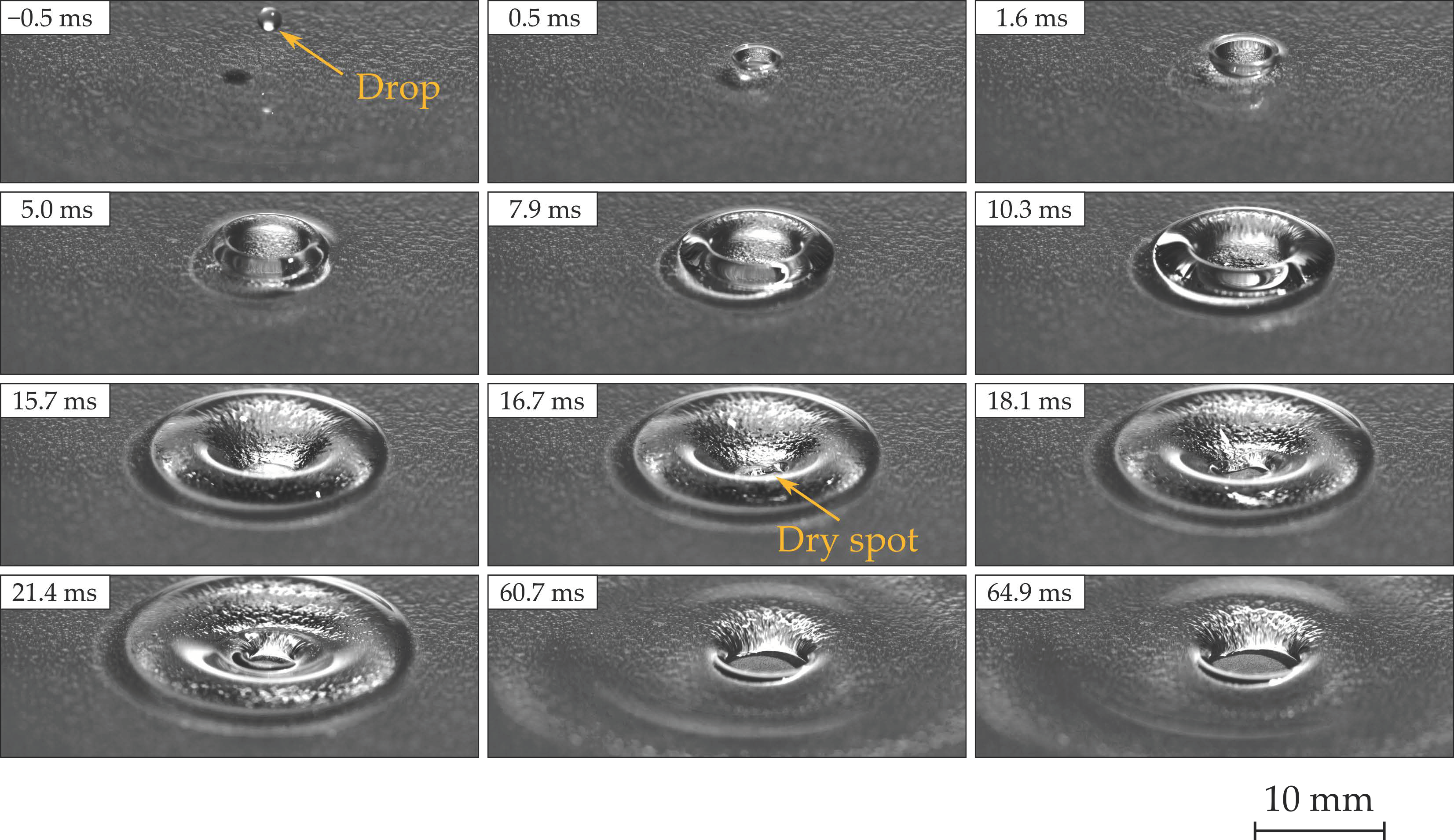
3.1. Experimental Observations
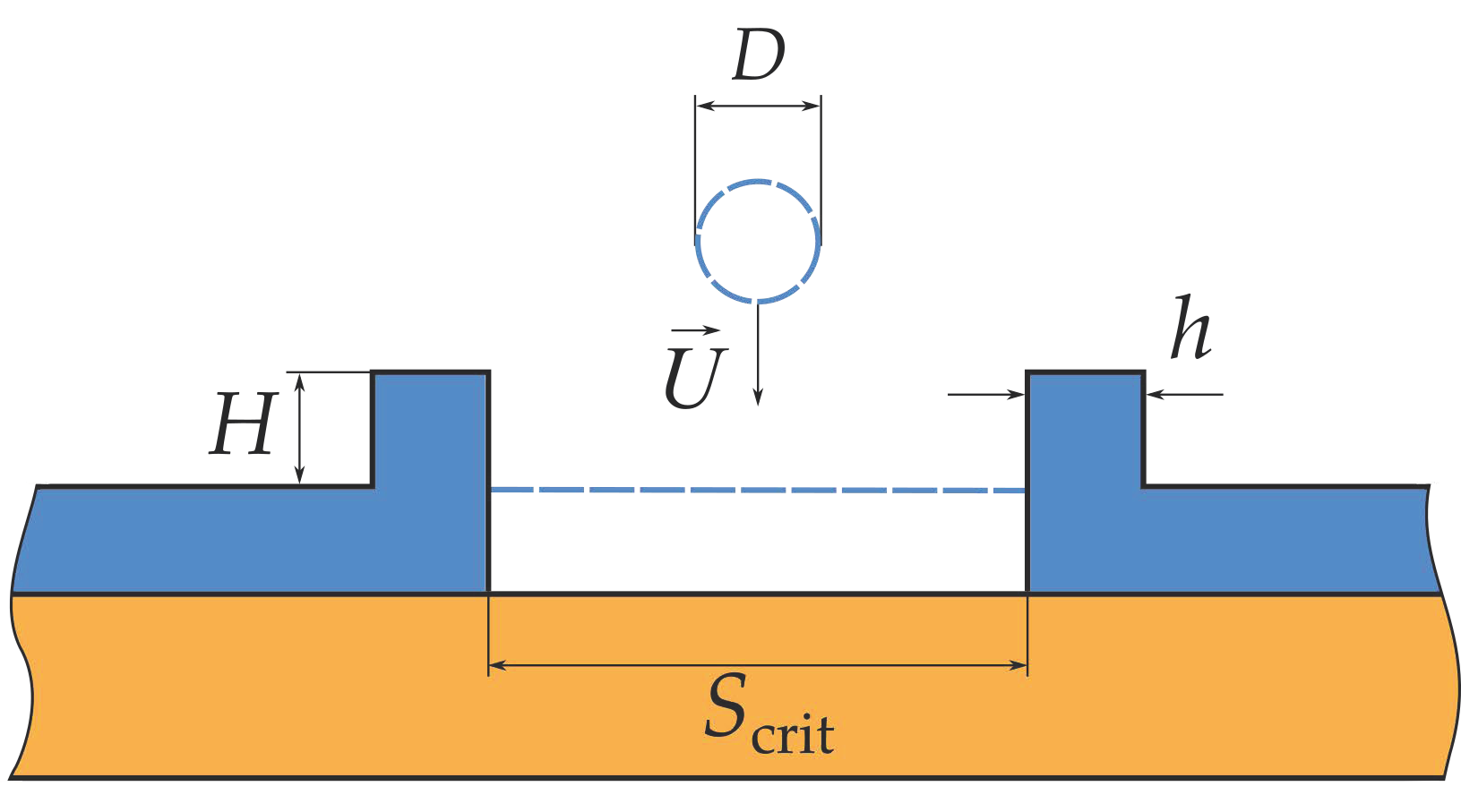
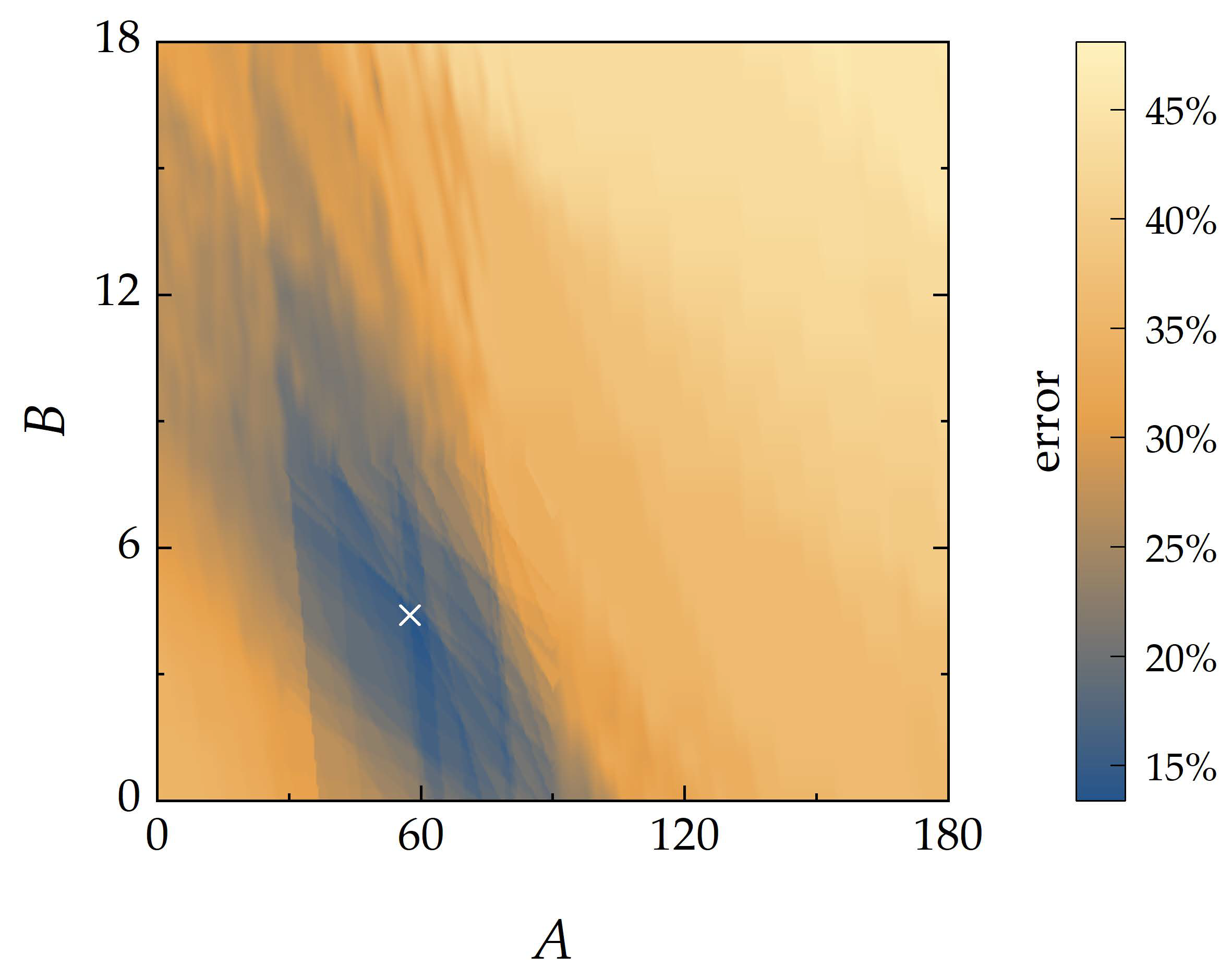
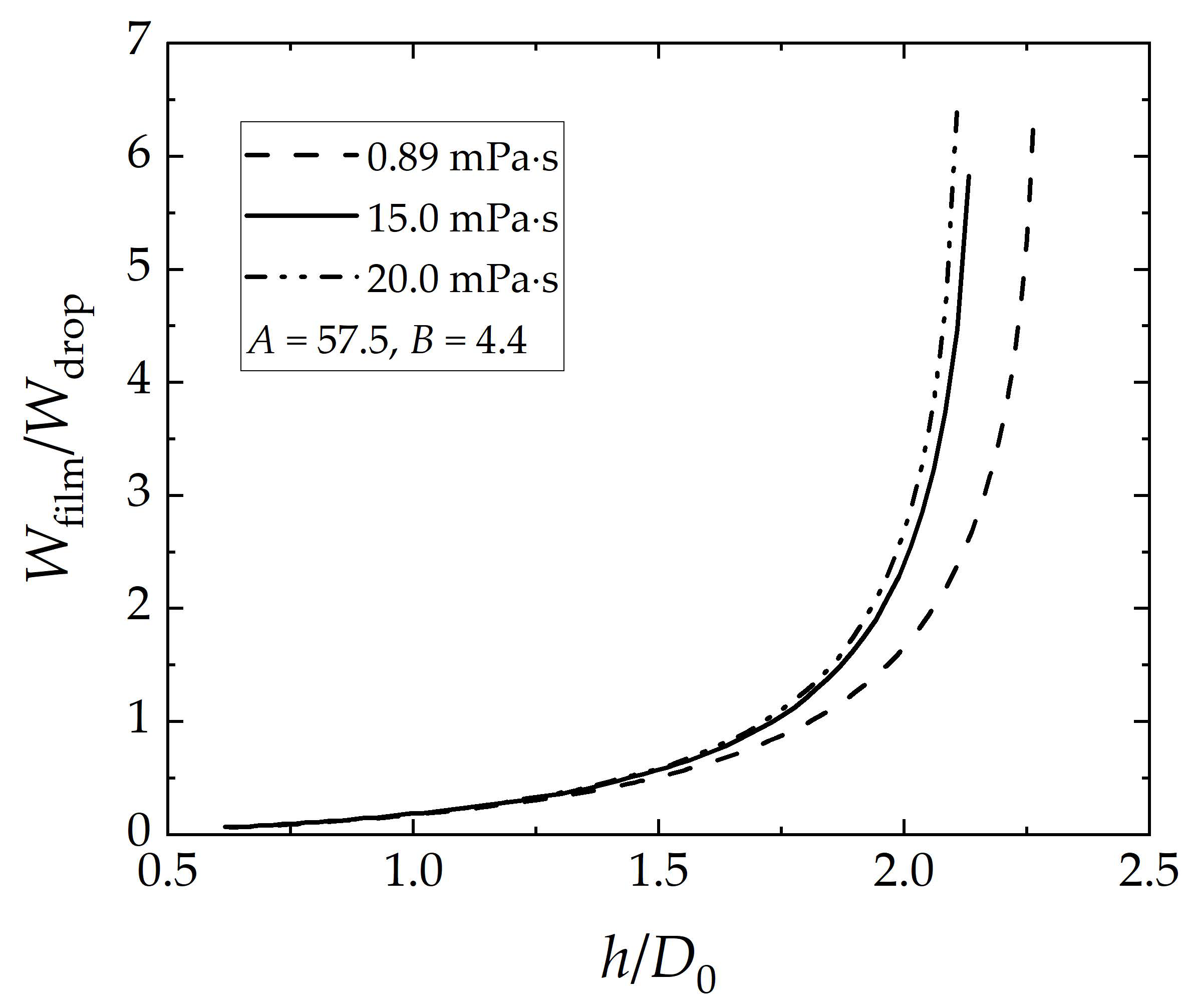
3.2. Evaluation of Required Droplet Energy
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Tropea, C.; Marengo, M. The Impact of Drops on Walls and Films. Multiph. Sci. Technol. 1999, 11, 19–36. [Google Scholar] [CrossRef]
- Cossali, G.E.; Brunello, G.; Coghe, A.; Marengo, M. Impact of a Single Drop on a Liquid Film: Experimental Analysis and Comparison With Empirical Models Experimental Set-Up. In Proceedings of the Italian Congress of Thermofluid Dynamics UIT, Ferrera, Italy, 30 June–2 July 1999. [Google Scholar]
- Huang, Q.; Zhang, H. A Study of Different Fluid Droplets Impacting on a Liquid Film. Pet. Sci. 2008, 5, 62–66. [Google Scholar] [CrossRef] [Green Version]
- van Hinsberg, N.P.; Budakli, M.; Göhler, S.; Berberović, E.; Roisman, I.V.; Gambaryan-Roisman, T.; Tropea, C.; Stephan, P. Dynamics of the Cavity and the Surface Film for Impingements of Single Drops on Liquid Films of Various Thicknesses. J. Colloid Interface Sci. 2010, 350, 336–343. [Google Scholar] [CrossRef] [PubMed]
- Josserand, C.; Ray, P.; Zaleski, S. Droplet Impact on a Thin Liquid Film: Anatomy of the Splash. J. Fluid Mech. 2016, 802, 775–805. [Google Scholar] [CrossRef] [Green Version]
- Kuhlman, J.M.; Hillen, N.L. Droplet Impact Cavity Film Thickness Measurements versus Time after Drop Impact and Cavity Radius for Thin Static Residual Liquid Layer Thicknesses. Exp. Therm. Fluid Sci. 2016, 77, 246–256. [Google Scholar] [CrossRef]
- Chen, B.; Wang, B.; Mao, F.; Wen, J.; Tian, R.; Lu, C. Experimental Study of Droplet Impacting on Inclined Wetted Wall in Corrugated Plate Separator. Ann. Nucl. Energy 2020, 137, 107155. [Google Scholar] [CrossRef]
- Ribeiro, D.F.S.; Silva, A.R.R.; Panão, M.R.O. Insights into Single Droplet Impact Models upon Liquid Films Using Alternative Fuels for Aero-Engines. Appl. Sci. 2020, 10, 6698. [Google Scholar] [CrossRef]
- Lakshman, S.; Tewes, W.; Harth, K.; Snoeijer, J.H.; Lohse, D. Deformation and Relaxation of Viscous Thin Films under Bouncing Drops. J. Fluid Mech. 2021, 920, A3. [Google Scholar] [CrossRef]
- Seemann, R.; Herminghaus, S.; Jacobs, K. Dewetting Patterns and Molecular Forces: A Reconciliation. Phys. Rev. Lett. 2001, 86, 5534–5537. [Google Scholar] [CrossRef]
- Bankoff, S.G.; Johnson, M.F.G.; Miksis, M.J.; Schluter, R.A.; Lopez, P.G. Dynamics of a Dry Spot. J. Fluid Mech. 2003, 486, 239–259. [Google Scholar] [CrossRef]
- Dhiman, R.; Chandra, S. Rupture of Thin Films Formed during Droplet Impact. Proc. R. Soc. A Math. Phys. Eng. Sci. 2010, 466, 1229–1245. [Google Scholar] [CrossRef]
- Biance, A.L.; Pirat, C.; Ybert, C. Drop Fragmentation Due to Hole Formation during Leidenfrost Impact. Phys. Fluids 2011, 23, 022104. [Google Scholar] [CrossRef]
- Rashidian, H.; Sellier, M.; Mandin, P. Dynamic Wetting of an Occlusion after Droplet Impact. Int. J. Multiph. Flow 2019, 111, 264–271. [Google Scholar] [CrossRef]
- Lv, C.; Eigenbrod, M.; Hardt, S. Stability and Collapse of Holes in Liquid Layers. J. Fluid Mech. 2018, 855, 1130–1155. [Google Scholar] [CrossRef]
- Neél, B.; Villermaux, E. The Spontaneous Puncture of Thick Liquid Films. J. Fluid Mech. 2018, 838, 192–221. [Google Scholar] [CrossRef]
- Grishaev, V.; Bakulin, I.; Amirfazli, A.; Borodulin, I.; Akhatov, I. Energy of a Drop Required to Break a Liquid Film. Langmuir 2021, 37, 10433–10438. [Google Scholar] [CrossRef]
- Ni, Z.; Chu, F.; Li, S.; Luo, J.; Wen, D. Impact-Induced Hole Growth and Liquid Film Dewetting on Superhydrophobic Surfaces. Phys. Fluids 2021, 33, 112113. [Google Scholar] [CrossRef]
- Grishaev, V. Impact of Particle-Laden Drops on Substrates with Various Wettability. Ph.D. Thesis, Université Libre de Bruxelles, Bruxelle, Belgium, 2015. [Google Scholar]
- Calculate Density and Viscosity of Glycerol/Water Mixtures. Available online: http://www.met.reading.ac.uk/~sws04cdw/viscosity_calc.html (accessed on 15 April 2022).
- Cheng, N.-S. Formula for the Viscosity of a Glycerol−Water Mixture. Ind. Eng. Chem. Res. 2008, 47, 3285–3288. [Google Scholar] [CrossRef]
- Volk, A.; Kähler, C.J. Density Model for Aqueous Glycerol Solutions. Exp. Fluids 2018, 59, 75. [Google Scholar] [CrossRef] [Green Version]
- Acheson, D.J. Elementary Fluid Dynamics. In Oxford Applied Mathematics and Computing Science Series; Oxford University Press: Oxford, UK, 2005; ISBN 0198596790. [Google Scholar]
- Chandra, S.; Avedisian, C.T. On the Collision of a Droplet with a Solid Surface. Proc. R. Soc. A Math. Phys. Eng. Sci. 1991, 432, 13–41. [Google Scholar] [CrossRef]
- Huang, H.M.; Chen, X.P. Energetic Analysis of Drop’s Maximum Spreading on Solid Surface with Low Impact Speed. Phys. Fluids 2018, 30, 022106. [Google Scholar] [CrossRef]


| Glycerol, % Wt. | Viscosity 1, mPa·s | Density 1, kg·m−3 | Surface Tension, mN·m−1 | Static Contact Angle on Test Substrates, ° |
|---|---|---|---|---|
| 0 | 0.89 ± 0.02 | 1 ± 0.0002 | 72.0 ± 0.8 | 169 ± 2 |
| 68 ± 0.5 | 15 ± 0.9 | 1.17 ± 0.002 | 66.4 ± 0.9 | 167 ± 2 |
| 71 ± 0.5 | 20 ± 1.4 | 1.18 ± 0.002 | 65.1 ± 0.8 | 166 ± 2 |
| Glycerol, % Wt. | Droplet Diameter, mm | Droplet Velocity, m·s−1 | Film Thickness, mm | We | Re |
|---|---|---|---|---|---|
| 0 | 2.0 ± 0.1 | 0.7–3.3 | 0.7–4.3 | 5–657 | 566–16,093 |
| 68 ± 0.5 | 1.9 ± 0.1 | 1.1–3.8 | 0.2–3.4 | 4–870 | 14–1013 |
| 71 ± 0.5 | 1.9 ± 0.1 | 1.2–5.1 | 0.7–3.8 | 25–1787 | 72–1137 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Grishaev, V.G.; Bakulin, I.K.; Amirfazli, A.; Akhatov, I.S. Puncture of a Viscous Liquid Film Due to Droplet Falling. Fluids 2022, 7, 196. https://doi.org/10.3390/fluids7060196
Grishaev VG, Bakulin IK, Amirfazli A, Akhatov IS. Puncture of a Viscous Liquid Film Due to Droplet Falling. Fluids. 2022; 7(6):196. https://doi.org/10.3390/fluids7060196
Chicago/Turabian StyleGrishaev, Viktor G., Ivan K. Bakulin, Alidad Amirfazli, and Iskander S. Akhatov. 2022. "Puncture of a Viscous Liquid Film Due to Droplet Falling" Fluids 7, no. 6: 196. https://doi.org/10.3390/fluids7060196
APA StyleGrishaev, V. G., Bakulin, I. K., Amirfazli, A., & Akhatov, I. S. (2022). Puncture of a Viscous Liquid Film Due to Droplet Falling. Fluids, 7(6), 196. https://doi.org/10.3390/fluids7060196







