Experimental Characterization of the Wettability of Coated and Uncoated Plates for Indirect Evaporative Cooling Systems
Abstract
1. Introduction and State of the Art
2. Materials, Methods, and Motivation
2.1. Evaluation of the Static Contact Angle
- Pre-processing of the images (e.g., cropping if needed).
- Image segmentation.
- Extraction and smoothing of drop profile.
- Fitting of the Laplace-Young equation to the experimental boundary.
- Determination of the contact angle.
2.2. Evaluation of the Dynamic Contact Angles and Analysis of the Transient Behavior of the Drops
3. Results and Discussion
3.1. Static Contact Angle
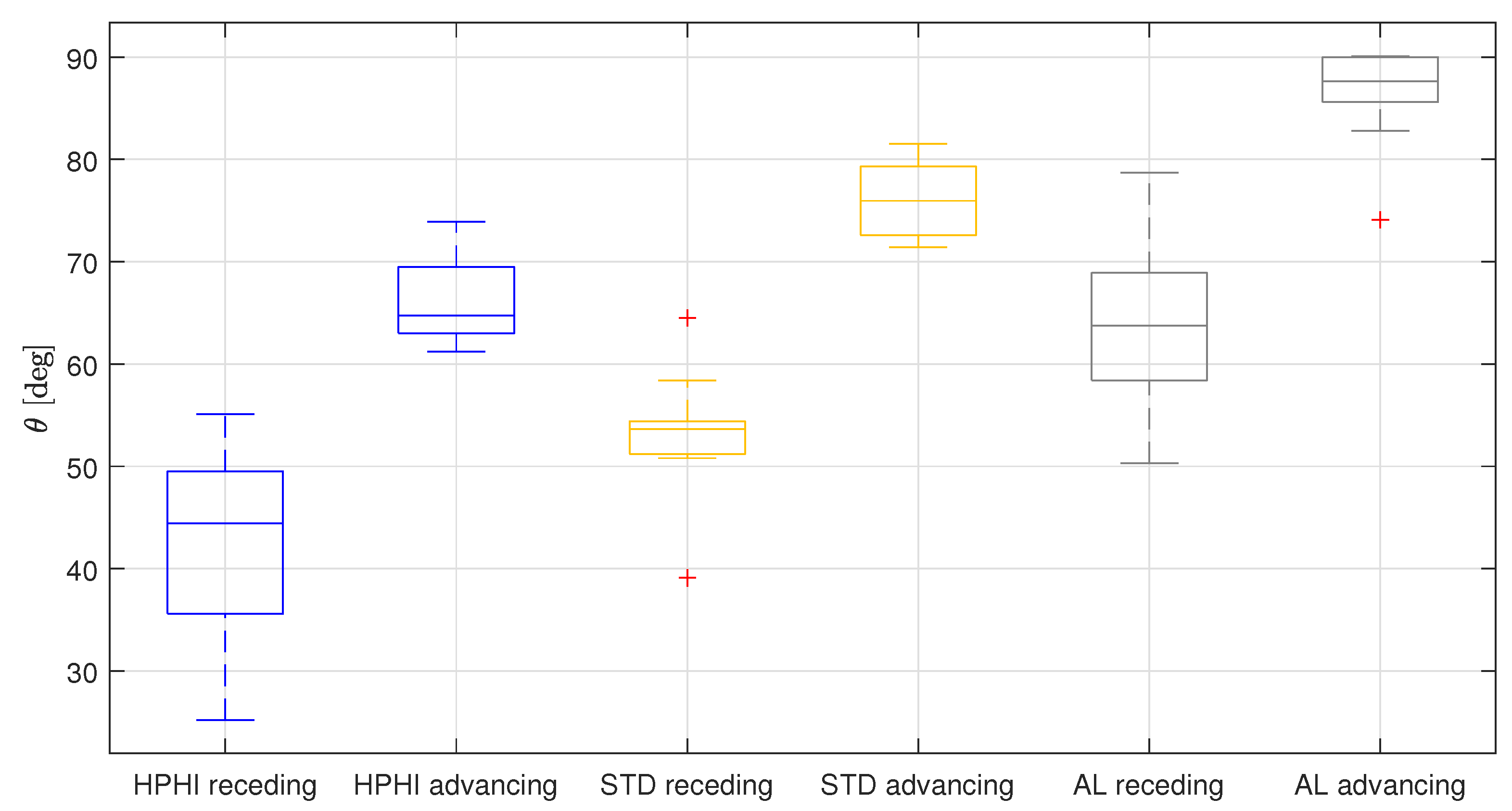
3.2. Dynamic Contact Angles and Transient Analysis
3.3. Correlation of the Wettability Results with IEC System Performance
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| ADSA | Axisymmetric Drop Shape Analysis |
| AL | Aluminum uncoated surface |
| HPHI | Aluminum surface coated with a hydrophilic lacquer |
| IEC | Indirect Evaporative Cooling |
| STD | Aluminum surface covered with a standard epoxy coating |
| V | Volume |
| deg | Degrees |
| Frames per second | |
| Seconds | |
| t | Time |
| Static contact angle | |
| Advancing contact angle | |
| Receding contact angle | |
| Advancing coefficient used in Tadmor model | |
| Receding coefficient used in Tadmor model |
References
- Sajjad, U.; Abbas, N.; Hamid, K.; Abbas, S.; Hussain, I.; Ammar, S.M.; Sultan, M.; Ali, H.M.; Hussain, M.; Wang, C.C.; et al. A review of recent advances in indirect evaporative cooling technology. Int. Commun. Heat Mass Transf. 2021, 122, 105140. [Google Scholar] [CrossRef]
- Yang, H.; Shi, W.; Chen, Y.; Min, Y. Research development of indirect evaporative cooling technology: An updated review. Renew. Sustain. Energy Rev. 2021, 145, 111082. [Google Scholar] [CrossRef]
- Duan, Z.; Zhan, C.; Zhang, X.; Mustafa, M.; Zhao, X.; Alimohammadisagvand, B.; Hasan, A. Indirect evaporative cooling: Past, present and future potentials. Renew. Sustain. Energy Rev. 2012, 16, 6823–6850. [Google Scholar] [CrossRef]
- Alonso, J.S.J.; Martinez, F.R.; Gomez, E.V.; Plasencia, M.A.G. Simulation model of an indirect evaporative cooler. Energy Build. 1998, 29, 23–27. [Google Scholar] [CrossRef]
- Chen, Y.; Luo, Y.; Yang, H. A simplified analytical model for indirect evaporative cooling considering condensation from fresh air: Development and application. Energy Build. 2015, 108, 387–400. [Google Scholar] [CrossRef]
- Heidarinejad, G.; Moshari, S. Novel modeling of an indirect evaporative cooling system with cross-flow configuration. Energy Build. 2015, 92, 351–362. [Google Scholar] [CrossRef]
- De Antonellis, S.; Joppolo, C.M.; Liberati, P.; Milani, S.; Romano, F. Modeling and experimental study of an indirect evaporative cooler. Energy Build. 2017, 142, 147–157. [Google Scholar] [CrossRef]
- Adam, A.; Han, D.; He, W.; Chen, J. Numerical analysis of cross-flow plate type indirect evaporative cooler: Modeling and parametric analysis. Appl. Therm. Eng. 2021, 185, 116379. [Google Scholar] [CrossRef]
- Chua, K.; Xu, J.; Cui, X.; Ng, K.; Islam, M. Numerical heat and mass transfer analysis of a cross-flow indirect evaporative cooler with plates and flat tubes. Heat Mass Transf. 2016, 52, 1765–1777. [Google Scholar] [CrossRef]
- Guilizzoni, M.; Milani, S.; Liberati, P.; De Antonellis, S. Effect of plates coating on performance of an indirect evaporative cooling system. Int. J. Refrig. 2019, 104, 367–375. [Google Scholar] [CrossRef]
- Zhao, X.; Liu, S.; Riffat, S.B. Comparative study of heat and mass exchanging materials for indirect evaporative cooling systems. Build. Environ. 2008, 43, 1902–1911. [Google Scholar] [CrossRef]
- Del Rıo, O.; Neumann, A. Axisymmetric drop shape analysis: Computational methods for the measurement of interfacial properties from the shape and dimensions of pendant and sessile drops. J. Colloid Interface Sci. 1997, 196, 136–147. [Google Scholar]
- Guilizzoni, M.; De Antonellis, S. Wettability analysis of desiccant beads for HVAC systems. J. Phys. Conf. Ser. 2019, 1249, 012005. [Google Scholar] [CrossRef]
- Guilizzoni, M.; Sapienza, J. Axisymmetric Drop Shape Analysis using a low-cost home-made setup. J. Phys. Conf. Ser. 2021, 1977, 012003. [Google Scholar] [CrossRef]
- Santini, M.; Guilizzoni, M.; Fest-Santini, S.; Lorenzi, M. Characterization of highly hydrophobic textiles by means of X-ray microtomography, wettability analysis and drop impact. J. Phys. Conf. Ser. 2017, 923, 012013. [Google Scholar] [CrossRef]
- Wenzel, R.N. Resistance of solid surfaces to wetting by water. Ind. Eng. Chem. 1936, 28, 988–994. [Google Scholar] [CrossRef]
- Cassie, A.; Baxter, S. Wettability of porous surfaces. Trans. Faraday Soc. 1944, 40, 546–551. [Google Scholar] [CrossRef]
- Guilizzoni, M. Drop shape visualization and contact angle measurement on curved surfaces. J. Colloid Interface Sci. 2011, 364, 230–236. [Google Scholar] [CrossRef] [PubMed]
- Laplace, P. Traité de Mécanique Céleste; Supplement au Dixième; Livre, Surl’Action Capillaire, Courcier: Paris, France, 1806. [Google Scholar]
- Rotenberg, Y.; Boruvka, L.; Neumann, A. Determination of surface tension and contact angle from the shapes of axisymmetric fluid interfaces. J. Colloid Interface Sci. 1983, 93, 169–183. [Google Scholar] [CrossRef]
- Cheng, P.; Li, D.; Boruvka, L.; Rotenberg, Y.; Neumann, A. Automation of axisymmetric drop shape analysis for measurements of interfacial tensions and contact angles. Colloids Surf. 1990, 43, 151–167. [Google Scholar] [CrossRef]
- Birdi, K.; Vu, D. Wettability and the evaporation rates of fluids from solid surfaces. J. Adhes. Sci. Technol. 1993, 7, 485–493. [Google Scholar] [CrossRef]
- Erbil, H.Y.; McHale, G.; Newton, M. Drop evaporation on solid surfaces: Constant contact angle mode. Langmuir 2002, 18, 2636–2641. [Google Scholar] [CrossRef]
- Rein, M. Phenomena of liquid drop impact on solid and liquid surfaces. Fluid Dyn. Res. 1993, 12, 61–93. [Google Scholar] [CrossRef]
- Rioboo, R.; Tropea, C.; Marengo, M. Outcomes from a drop impact on solid surfaces. At. Sprays 2001, 11, 155–166. [Google Scholar] [CrossRef]
- Josserand, C.; Thoroddsen, S.T. Drop impact on a solid surface. Annu. Rev. Fluid Mech. 2016, 48, 365–391. [Google Scholar] [CrossRef]
- Tadmor, R. Line energy and the relation between advancing, receding, and young contact angles. Langmuir 2004, 20, 7659–7664. [Google Scholar] [CrossRef]
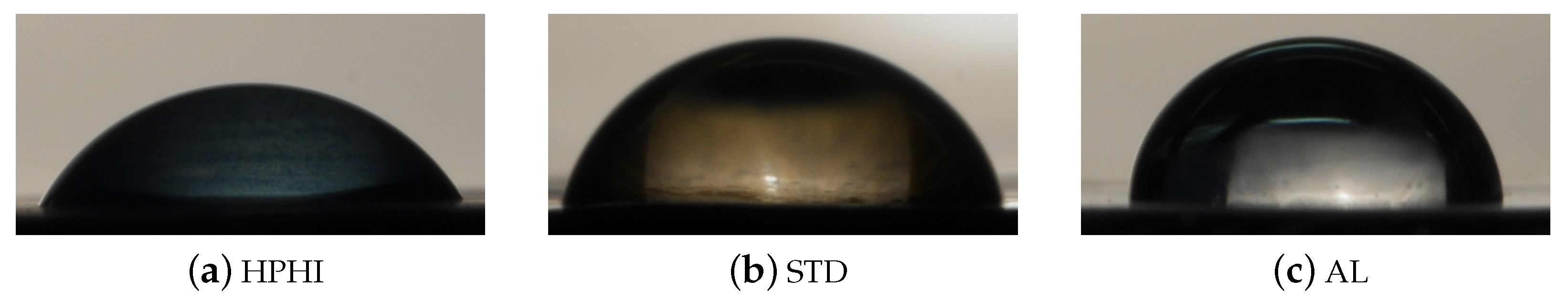
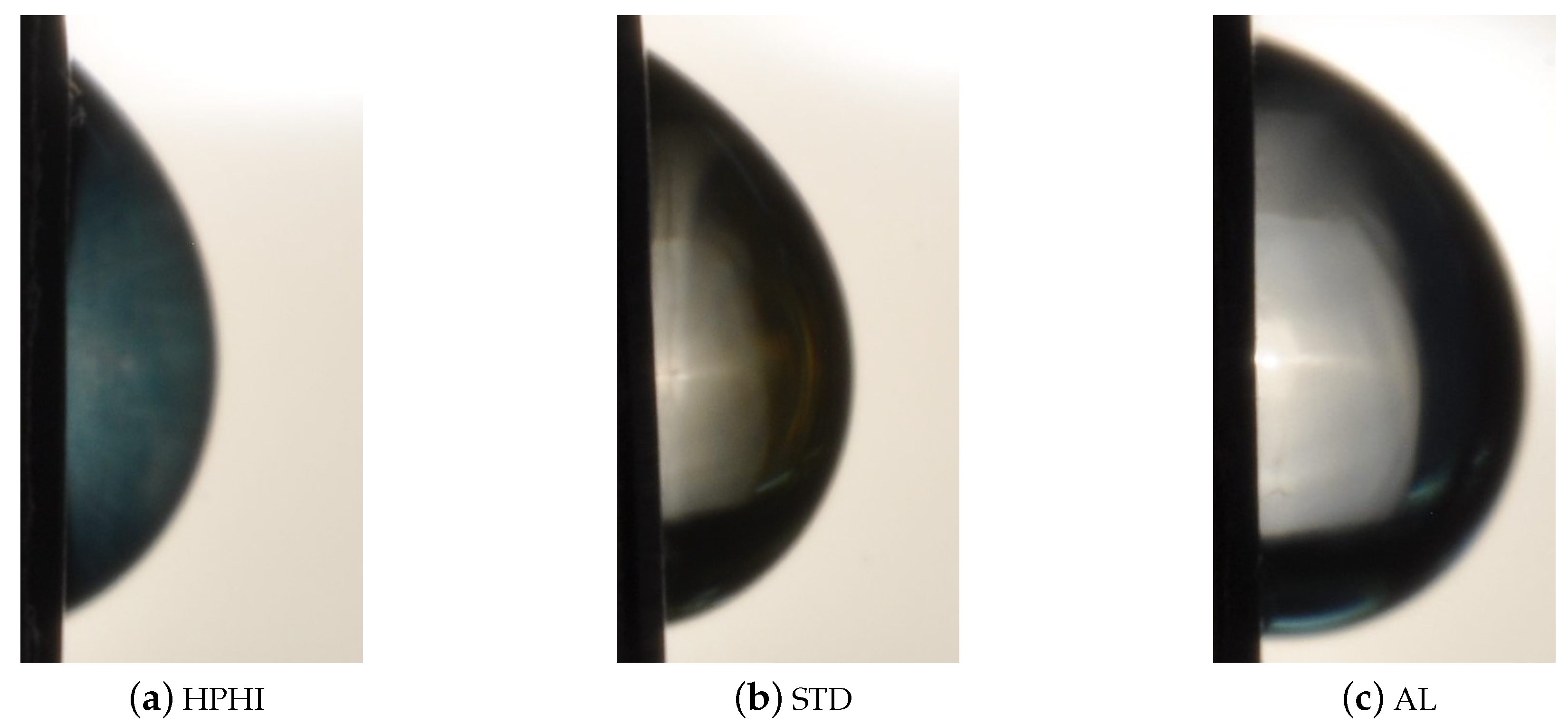
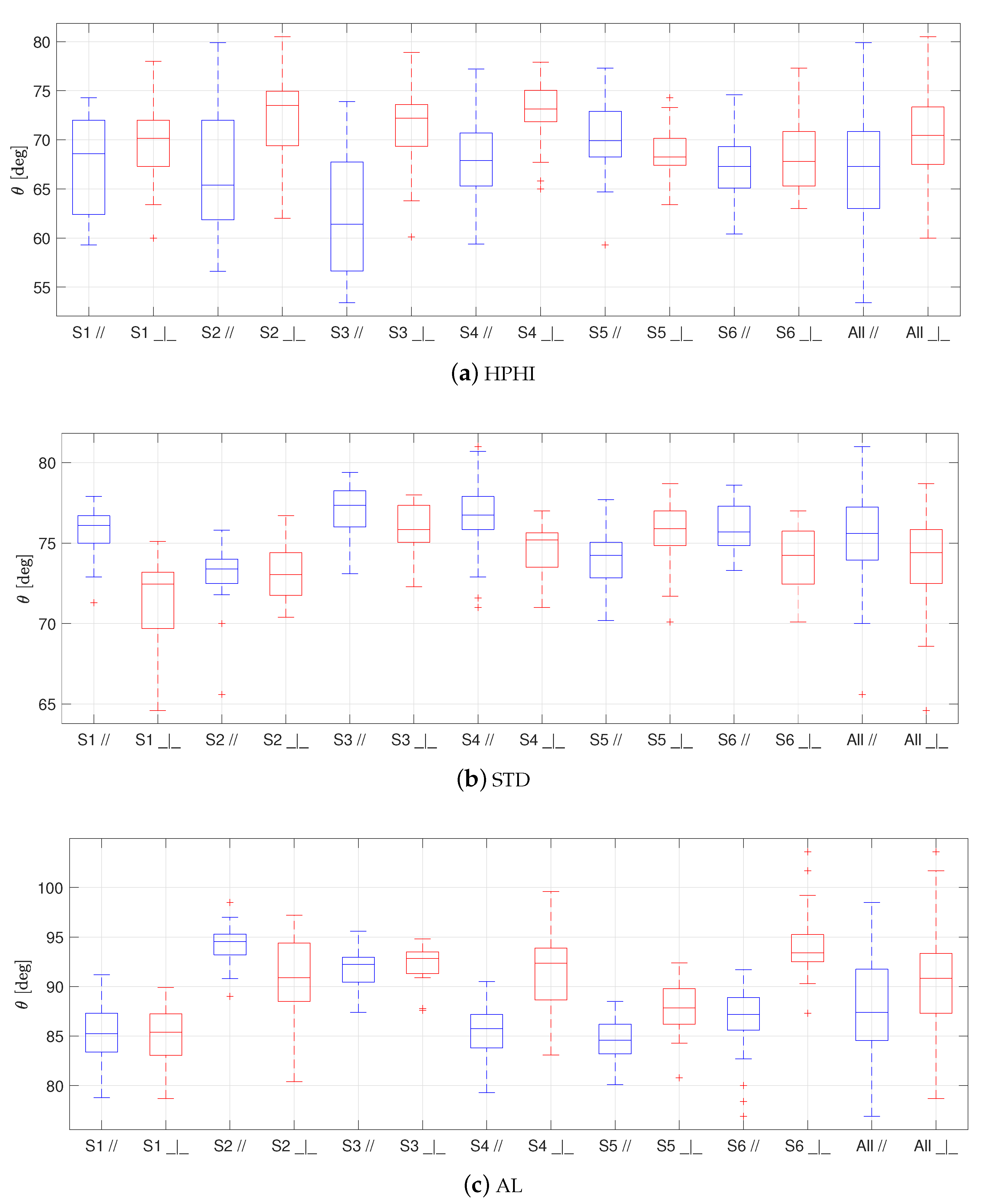
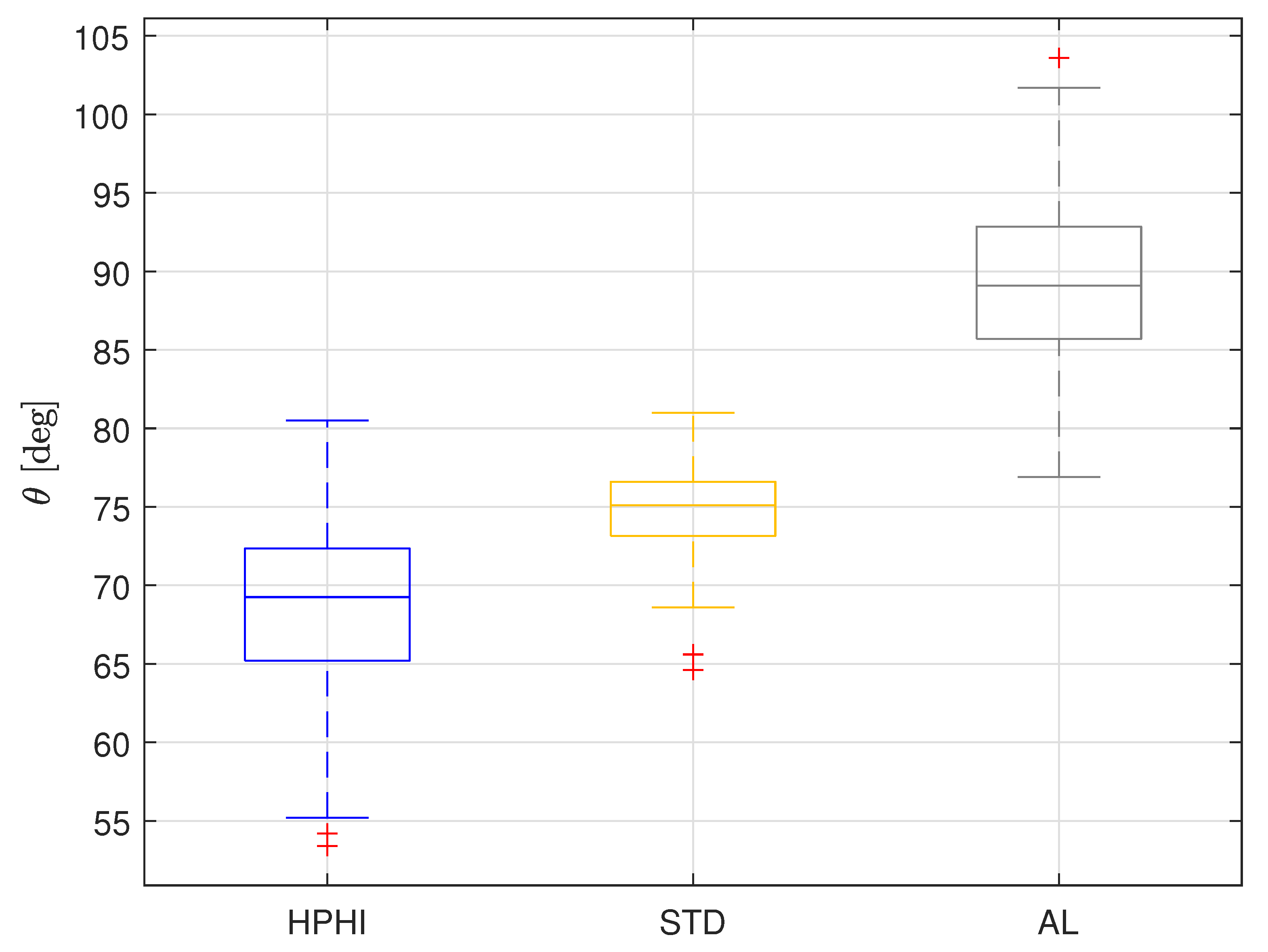
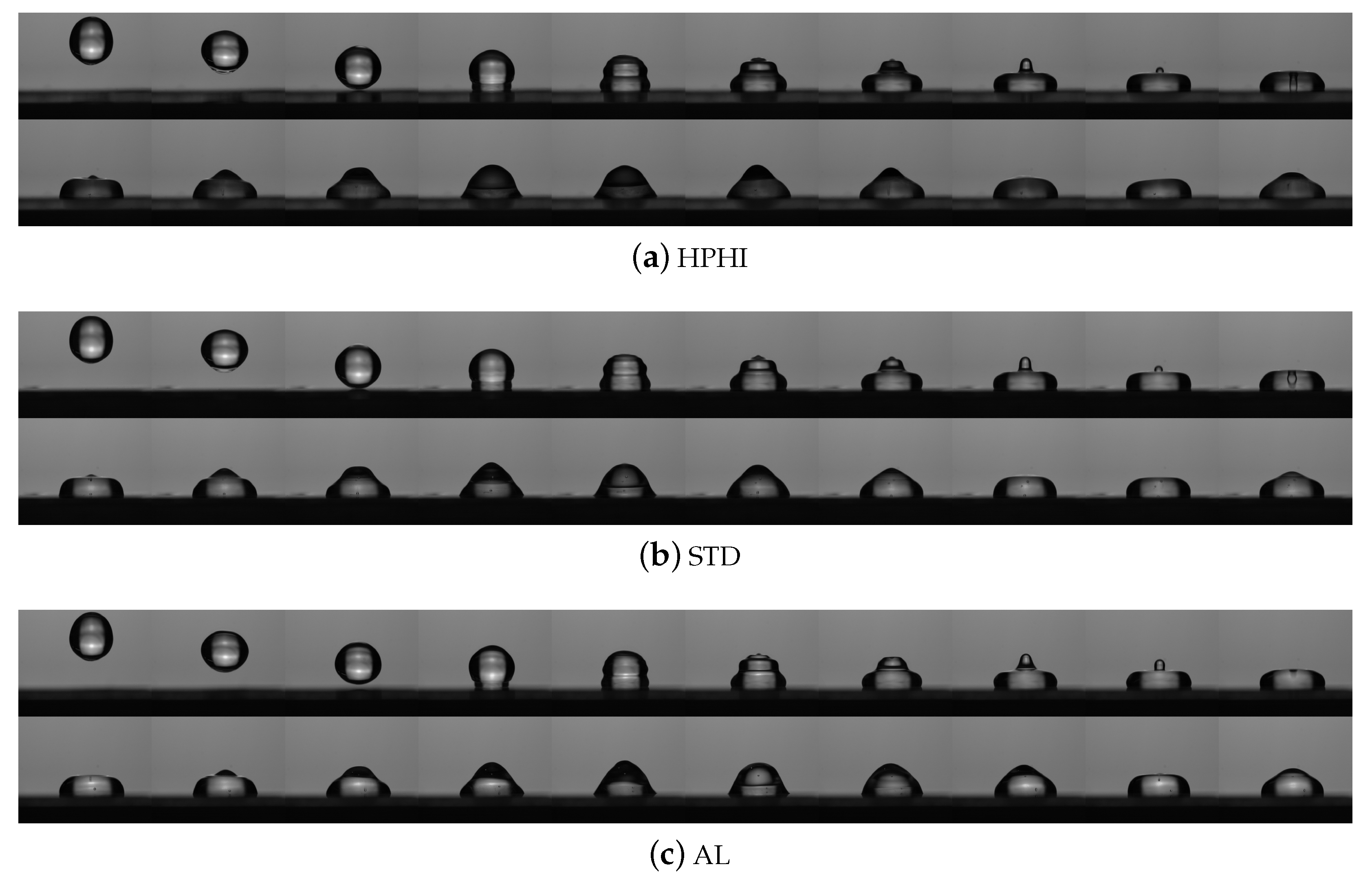
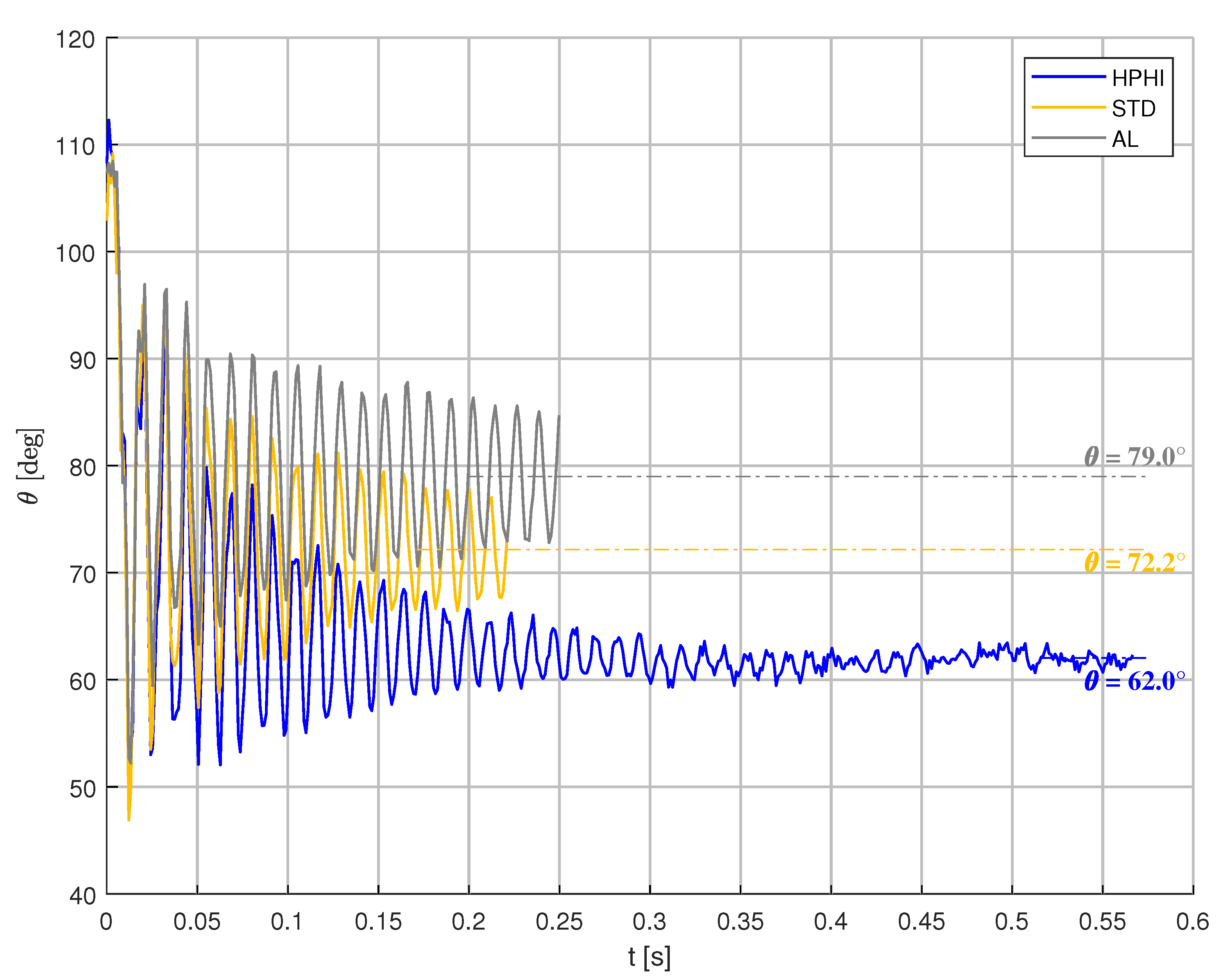
| Material | Orientation | Average | Median | Standard Deviation |
|---|---|---|---|---|
| HPHI | Parallel | 67° | 67° | 6° |
| HPHI | Perpendicular | 70° | 70° | 4° |
| STD | Parallel | 75° | 76° | 2° |
| STD | Perpendicular | 74° | 74° | 2° |
| AL | Parallel | 88° | 87° | 5° |
| AL | Perpendicular | 90° | 91° | 5° |
| Material | Dynamic Contact Angle Type | Average | Median | Standard Deviation |
|---|---|---|---|---|
| HPHI | Receding | 42° | 44° | 9° |
| HPHI | Advancing | 66° | 65° | 4° |
| STD | Receding | 53° | 54° | 6° |
| STD | Advancing | 76° | 75° | 4° |
| AL | Receding | 64° | 64° | 8° |
| AL | Advancing | 86° | 88° | 5° |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Caruana, R.; De Antonellis, S.; Marocco, L.; Liberati, P.; Guilizzoni, M. Experimental Characterization of the Wettability of Coated and Uncoated Plates for Indirect Evaporative Cooling Systems. Fluids 2023, 8, 122. https://doi.org/10.3390/fluids8040122
Caruana R, De Antonellis S, Marocco L, Liberati P, Guilizzoni M. Experimental Characterization of the Wettability of Coated and Uncoated Plates for Indirect Evaporative Cooling Systems. Fluids. 2023; 8(4):122. https://doi.org/10.3390/fluids8040122
Chicago/Turabian StyleCaruana, Roberta, Stefano De Antonellis, Luca Marocco, Paolo Liberati, and Manfredo Guilizzoni. 2023. "Experimental Characterization of the Wettability of Coated and Uncoated Plates for Indirect Evaporative Cooling Systems" Fluids 8, no. 4: 122. https://doi.org/10.3390/fluids8040122
APA StyleCaruana, R., De Antonellis, S., Marocco, L., Liberati, P., & Guilizzoni, M. (2023). Experimental Characterization of the Wettability of Coated and Uncoated Plates for Indirect Evaporative Cooling Systems. Fluids, 8(4), 122. https://doi.org/10.3390/fluids8040122









