On the Prediction of Boundary Layer Quantities at High Reynolds Numbers
Abstract
1. Introduction
2. Method
3. Results and Discussion
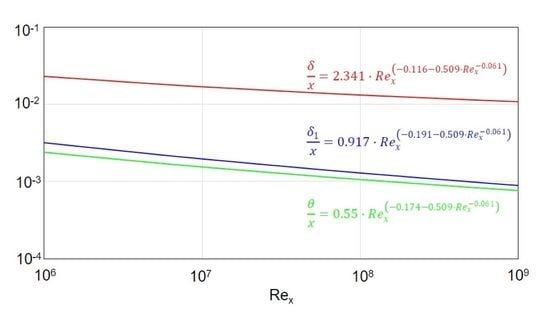
3.1. Boundary Layer Thickness
3.2. Friction Coefficients
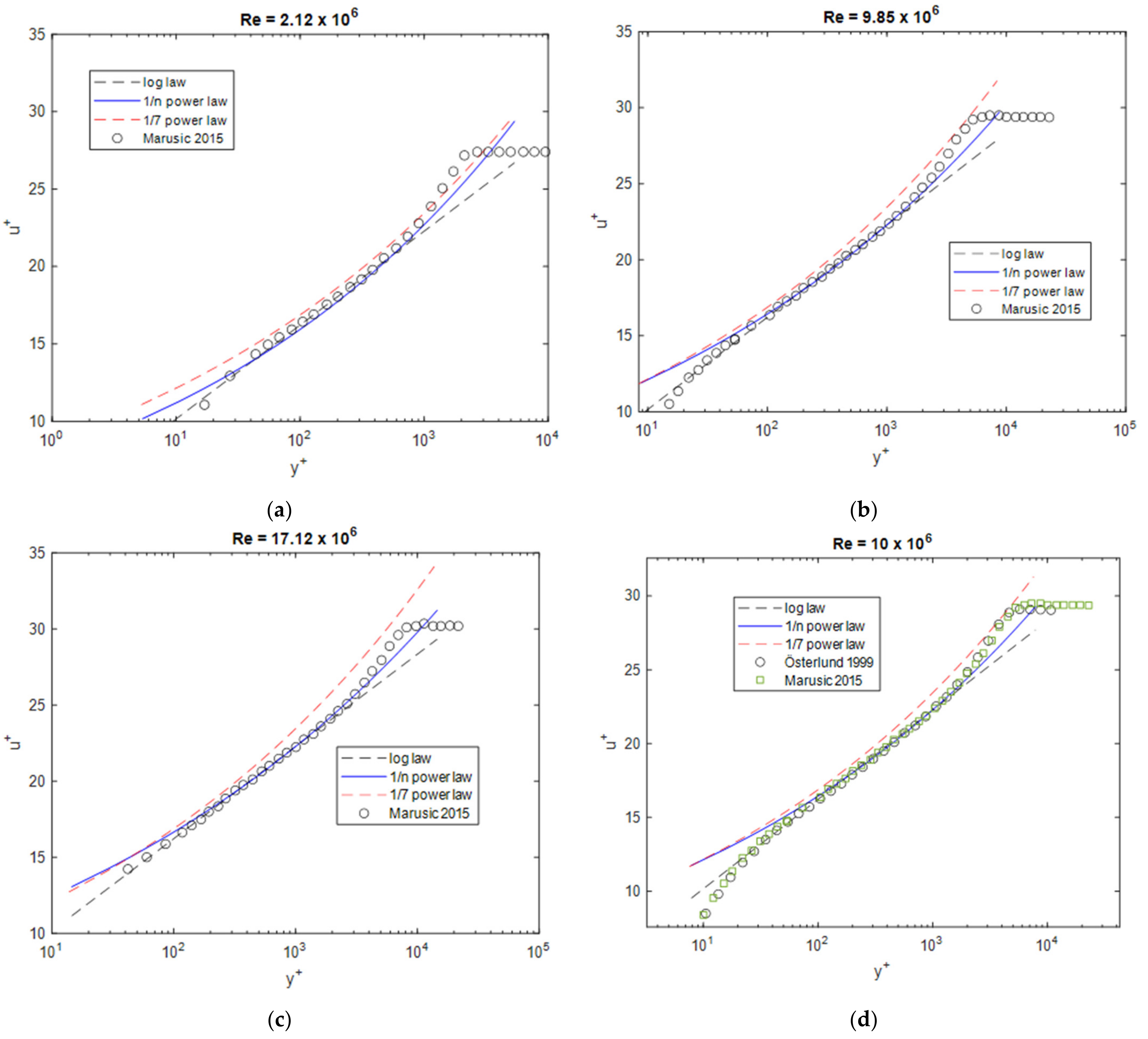
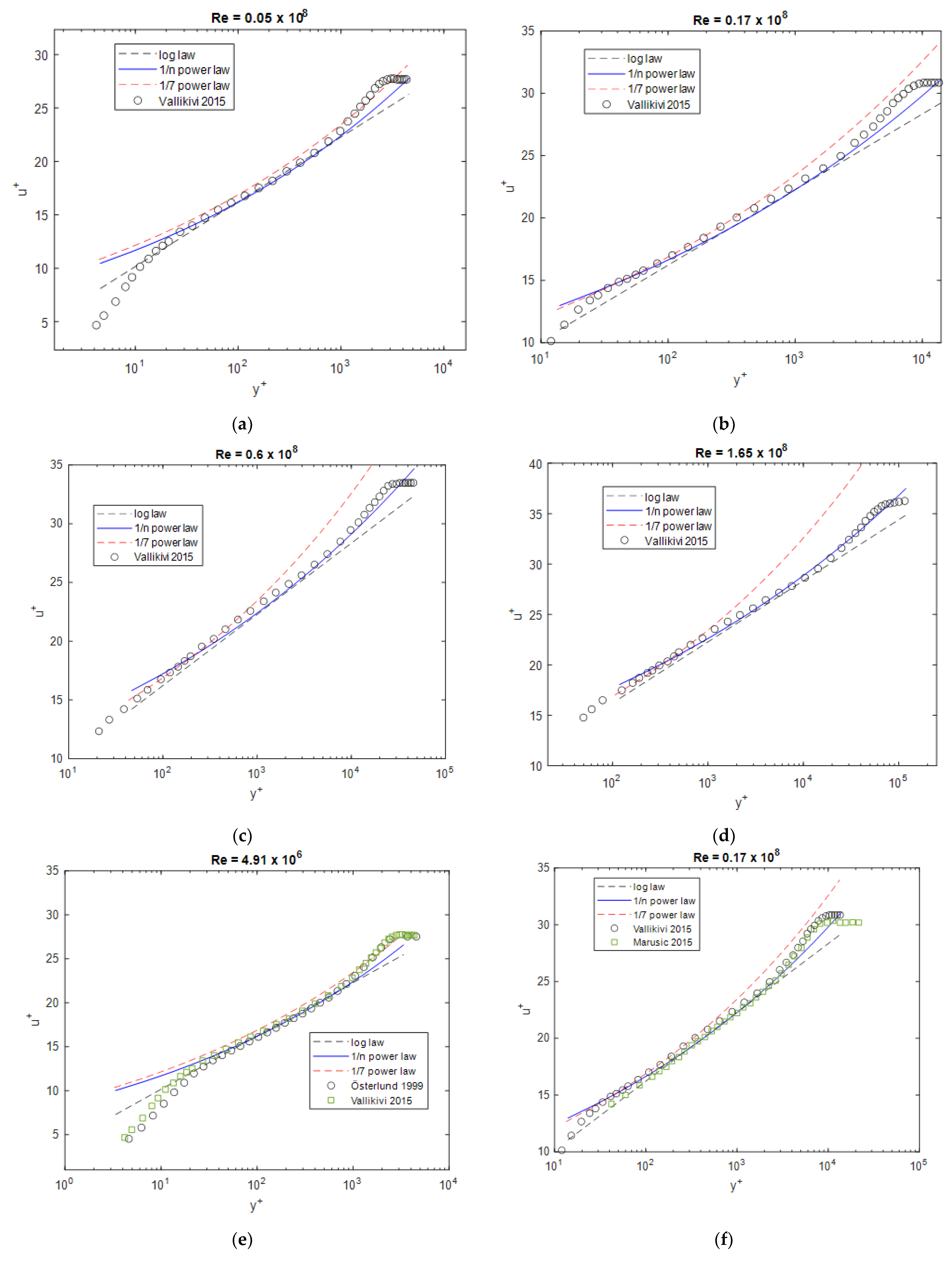
3.3. Boundary Layer Profiles
4. Conclusions
Funding
Acknowledgments
Conflicts of Interest
References
- Tschepe, J.; Maaß, J.-T.; Nayeri, C.N.; Paschereit, C.O. Experimental investigation of the aerodynamic drag of roof-mounted insulators for trains. J. Rail Rapid Transit 2019, 234, 834–846. [Google Scholar] [CrossRef]
- Bell, J.; Burton, D.; Thompson, M.; Herbst, A.; Sheridan, J. A wind-tunnel methodology for assessing the slipstream of high-speed trains. J. Wind. Eng. Ind. Aerodyn. 2017, 166, 1–19. [Google Scholar] [CrossRef]
- de Chant, L.J. The venerable 1/7th power law turbulent velocity profile: A classical nonlinear boundary value problem solution and its relationship to stochastic processes. Appl. Math. Comput. 2005, 161, 463–474. [Google Scholar] [CrossRef]
- Tschepe, J.; Nayeri, C.; Paschereit, C. On the influence of Reynolds number and ground conditions on the scaling of the aerodynamic drag of trains. J. Wind. Eng. Ind. Aerodyn. 2021, 213, 104594. [Google Scholar] [CrossRef]
- Barenblatt, G. Scaling laws for fully developed turbulent shear flows. Part 1. Basic hypotheses and analysis. J. Fluid Mech. 1993, 248, 513–520. [Google Scholar] [CrossRef]
- Buschmann, M.H.; Gad-El-Hak, M. Debate Concerning the Mean-Velocity Profile of a Turbulent Boundary Layer. AIAA J. 2003, 41, 565–572. [Google Scholar] [CrossRef]
- George, W.K. Recent Advancements Toward the Understanding of Turbulent Boundary Layers. AIAA J. 2006, 44, 2435–2449. [Google Scholar] [CrossRef]
- Buschmann, M.H.; Gad-el-Hak, M. Turbulent boundary layers: Reality and myth. Int. J. Comput. Sci. Math. 2007, 1, 159–176. [Google Scholar] [CrossRef]
- Barenblatt, G.I.; Chorin, A.J.; Prostokishin, V.M. Analysis of Experimental Investigations of Self-Similar Intermediate Structures in Zero-Pressure Boundary Layers at Large Reynolds Numbers. arXiv 2000, arXiv:math-ph/0002004. [Google Scholar]
- Österlund, J. Experimental Studies of Zero Pressure-Gradient Turbulent Boundary-Layer Flow; KTH: Stockholm, Sweden, 1999; Available online: https://www.mech.kth.se/~jens/zpg/art/zpg_screen.pdf (accessed on 5 January 2022).
- Vallikivi, M.; Hultmark, M.; Smits, A. Turbulent boundary layer statistics at very high Reynolds number. J. Fluid Mech. 2015, 779, 371–389. [Google Scholar] [CrossRef]
- Gorbushin, A.; Osipova, S.; Zametaev, V. Mean Parameters of an Incompressible Turbulent Boundary Layer on the Wind Tunnel Wall at Very High Reynolds Numbers. Flow Turbul. Combust 2021, 107, 31–50. [Google Scholar] [CrossRef]
- Marusic, I.; Chauhan, K.; Kulandaivelu, V.; Hutchins, N. Evolution of zero-pressure-gradient boundary layers from different tripping conditions. J. Fluid Mech. 2015, 783, 379–411. [Google Scholar] [CrossRef]
- Oweis, G.; Winkel, E.; Cutbrith, J.; Ceccio, S.; Perlin, M.; Dowling, D. The mean velocity profile of a smooth-flat-plate turbulent boundary layer at high Reynolds number. J. Fluid Mech. 2010, 665, 357–381. [Google Scholar] [CrossRef]
- de Graaff, D.; Eaton, J. Reynolds-number scaling of the flat-plate turbulent boundary layer. J. Fluid Mech. 2000, 422, 319–346. [Google Scholar] [CrossRef]
- Petrie, H.L.; Fontaine, A.A.; Sommer, S.T.; Brungart, T.A. Large Flat Plate Turbulent Boundary Layer Evaluation; Penn State Applied Research Laboratory Technical Memo File No. 89-207; Penn State Applied Research Laboratory: Reston, VA, USA, 1990; Available online: https://apps.dtic.mil/sti/pdfs/ADA225316.pdf (accessed on 5 January 2022).
- Schlichting, H.; Gersten, K. Boundary-Layer Theory; Springer: Berlin/Heidelberg, Germany, 2017. [Google Scholar] [CrossRef]
- Hoerner, S. Fluid-Dynamic Drag; Hoerner Fluid Dynamics: Bakersfiled, CA, USA, 1965; Available online: http://ftp.demec.ufpr.br/disciplinas/TM240/Marchi/Bibliografia/Hoerner.pdf (accessed on 3 October 2021).
- Schultz-Grunow, F. New Fricitional Resistance Law for Smoothe Plates; NASA Tech Memo No. 986; NASA: Washington, DC, USA, 1941. Available online: https://ntrs.nasa.gov/citations/19930094430 (accessed on 5 January 2022).
- ITTC. Skin Friction and Turbulence Stimulation; Committee Report; ITTC: Ledegem, Belgium, 1957; Available online: https://ittc.info/media/3139/skin-friction-and-turbulence-stimulation.pdf (accessed on 5 January 2022).
- Rona, A.; Monti, M.; Airiau, C. On the generation of the mean velocity profile for turbulent boundary layers with pressure gradient under equilibrium conditions. Aeronaut. J. 2012, 116, 569–598. [Google Scholar] [CrossRef][Green Version]
- Afzal, N.; Seena, A.; Bushra, A. Power Law Velocity Profile in Fully Developed Turbulent Pipe and Channel Flows. J. Hydraul. Eng. 2007, 133, 1080–1086. [Google Scholar] [CrossRef]



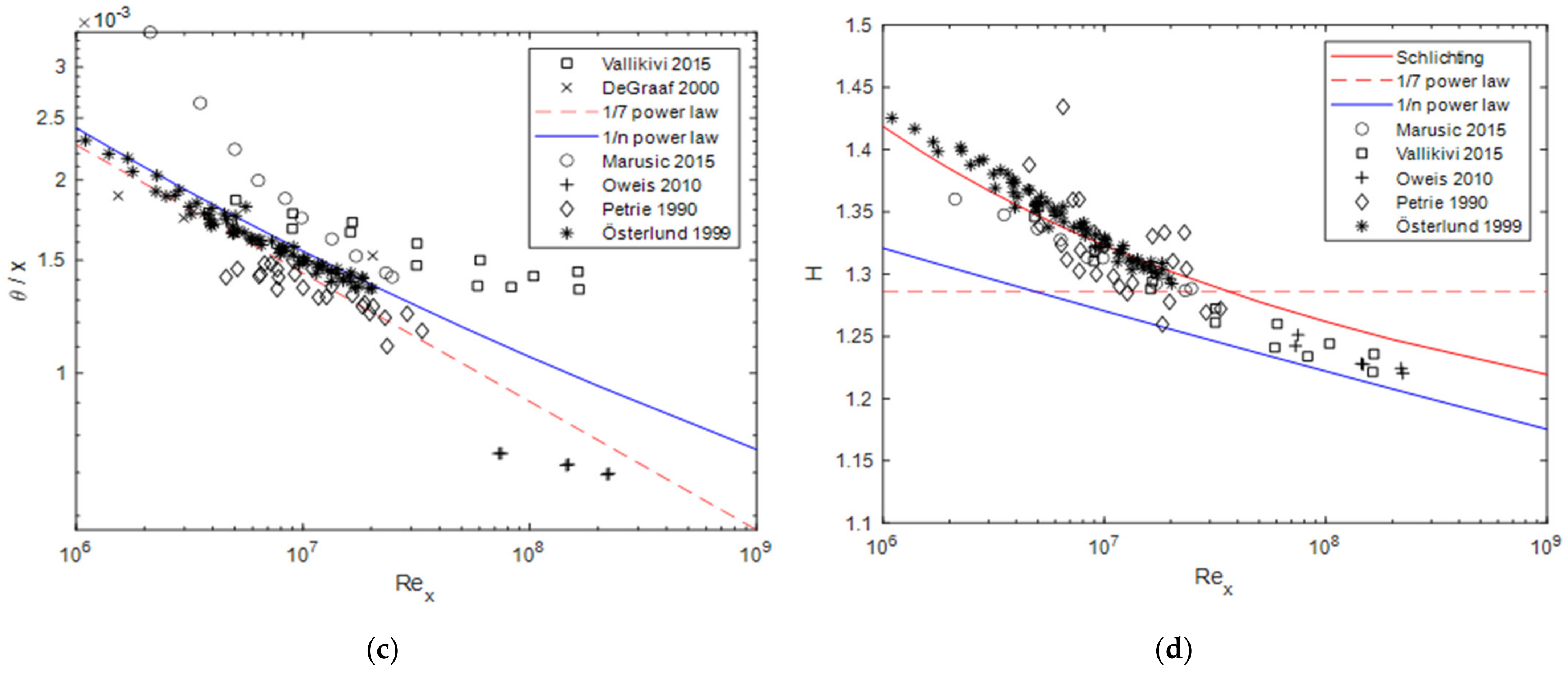

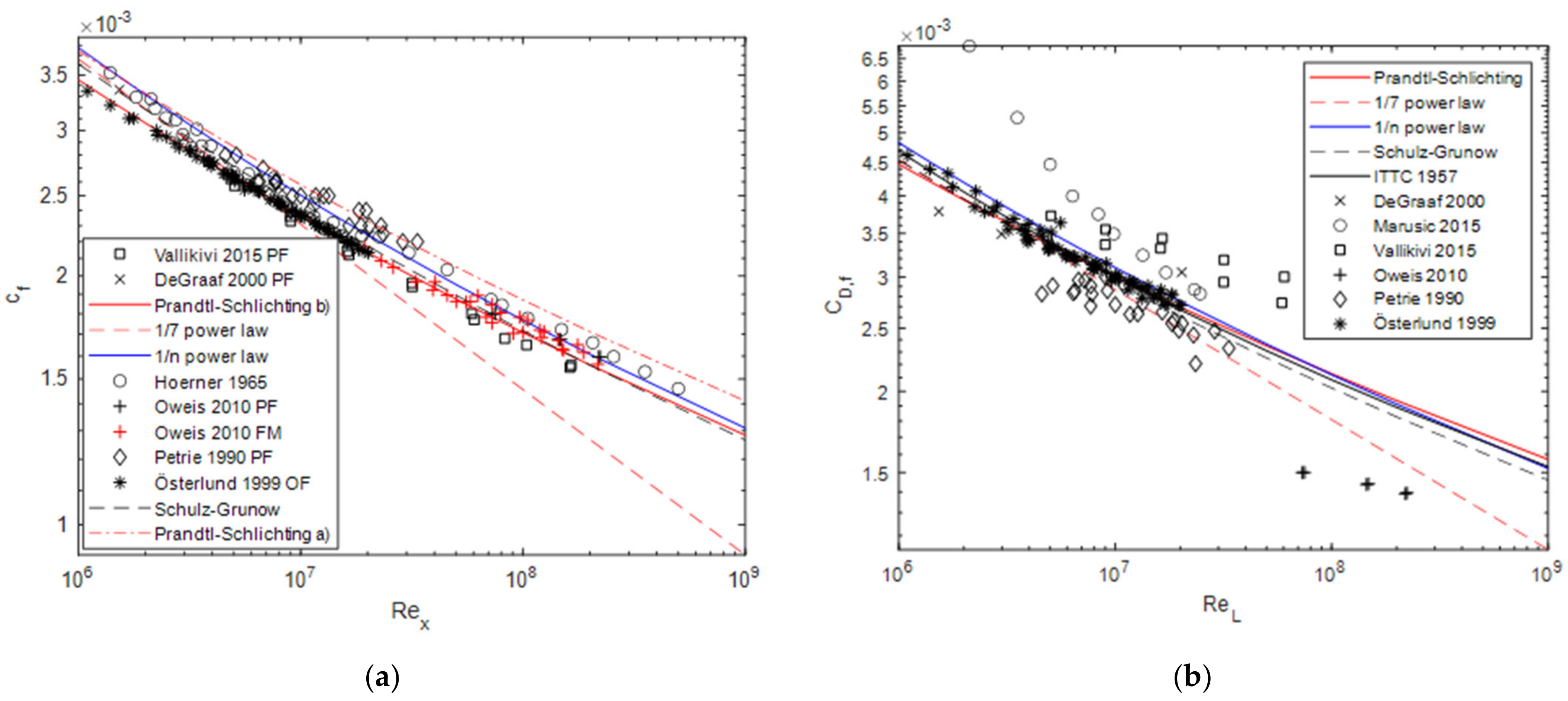
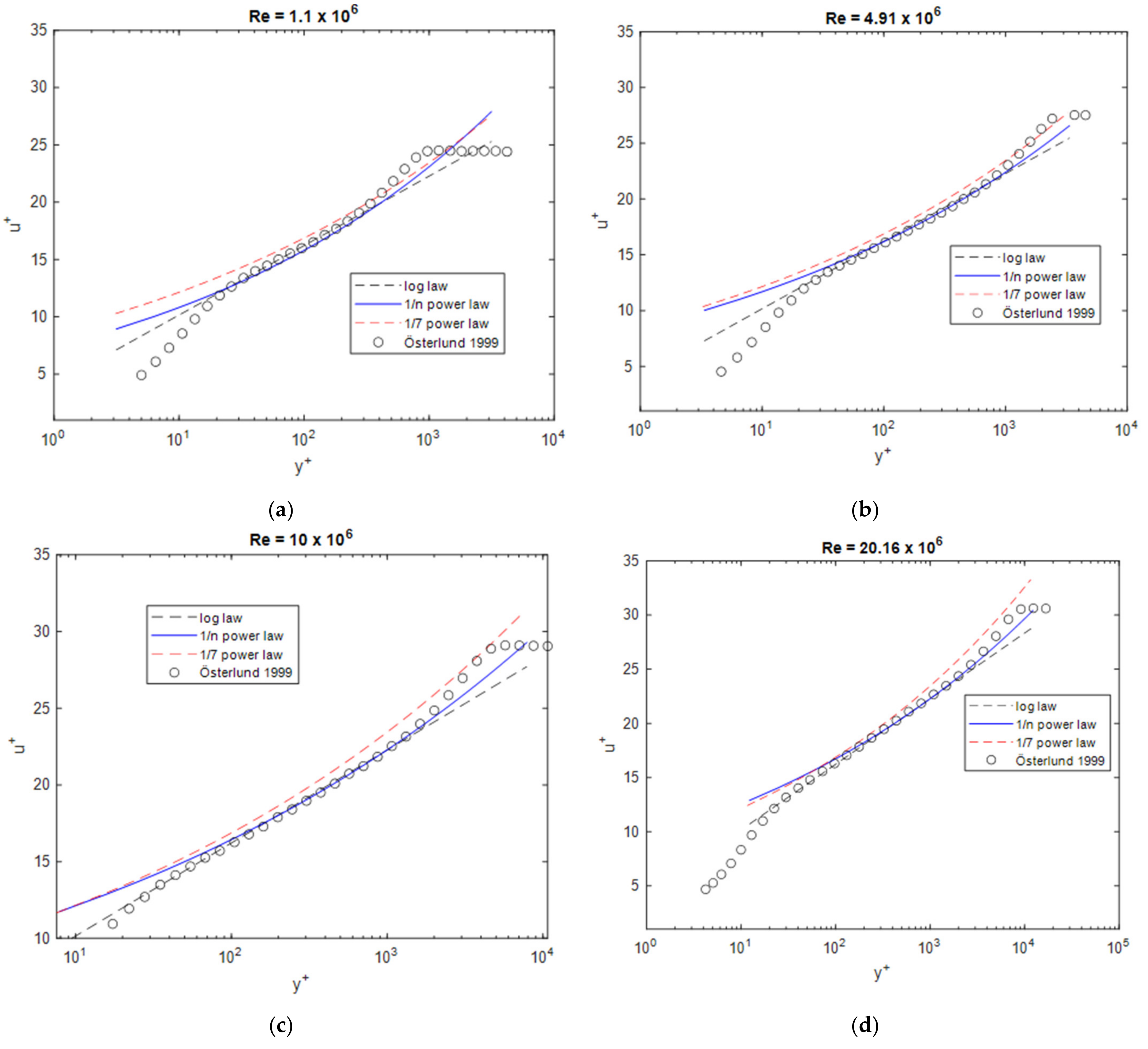

| Quantity | Formula |
|---|---|
| boundary layer thickness | |
| displacement thickness | |
| momentum thickness | |
| local friction coefficient | |
| friction drag coefficient | |
| velocity profile | |
| form parameter |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tschepe, J. On the Prediction of Boundary Layer Quantities at High Reynolds Numbers. Fluids 2022, 7, 114. https://doi.org/10.3390/fluids7030114
Tschepe J. On the Prediction of Boundary Layer Quantities at High Reynolds Numbers. Fluids. 2022; 7(3):114. https://doi.org/10.3390/fluids7030114
Chicago/Turabian StyleTschepe, Jonathan. 2022. "On the Prediction of Boundary Layer Quantities at High Reynolds Numbers" Fluids 7, no. 3: 114. https://doi.org/10.3390/fluids7030114
APA StyleTschepe, J. (2022). On the Prediction of Boundary Layer Quantities at High Reynolds Numbers. Fluids, 7(3), 114. https://doi.org/10.3390/fluids7030114