Interannual Bottom-Intensified Current Thickening Observed on the Continental Slope Off the Southeastern Coast of Hokkaido, Japan
Abstract
:1. Introduction
2. Observation and Data
2.1. ADCP Observation
2.2. OGCM for the Earth Simulator (OFES) Data
2.3. North Pacific Index
3. Results and Discussion
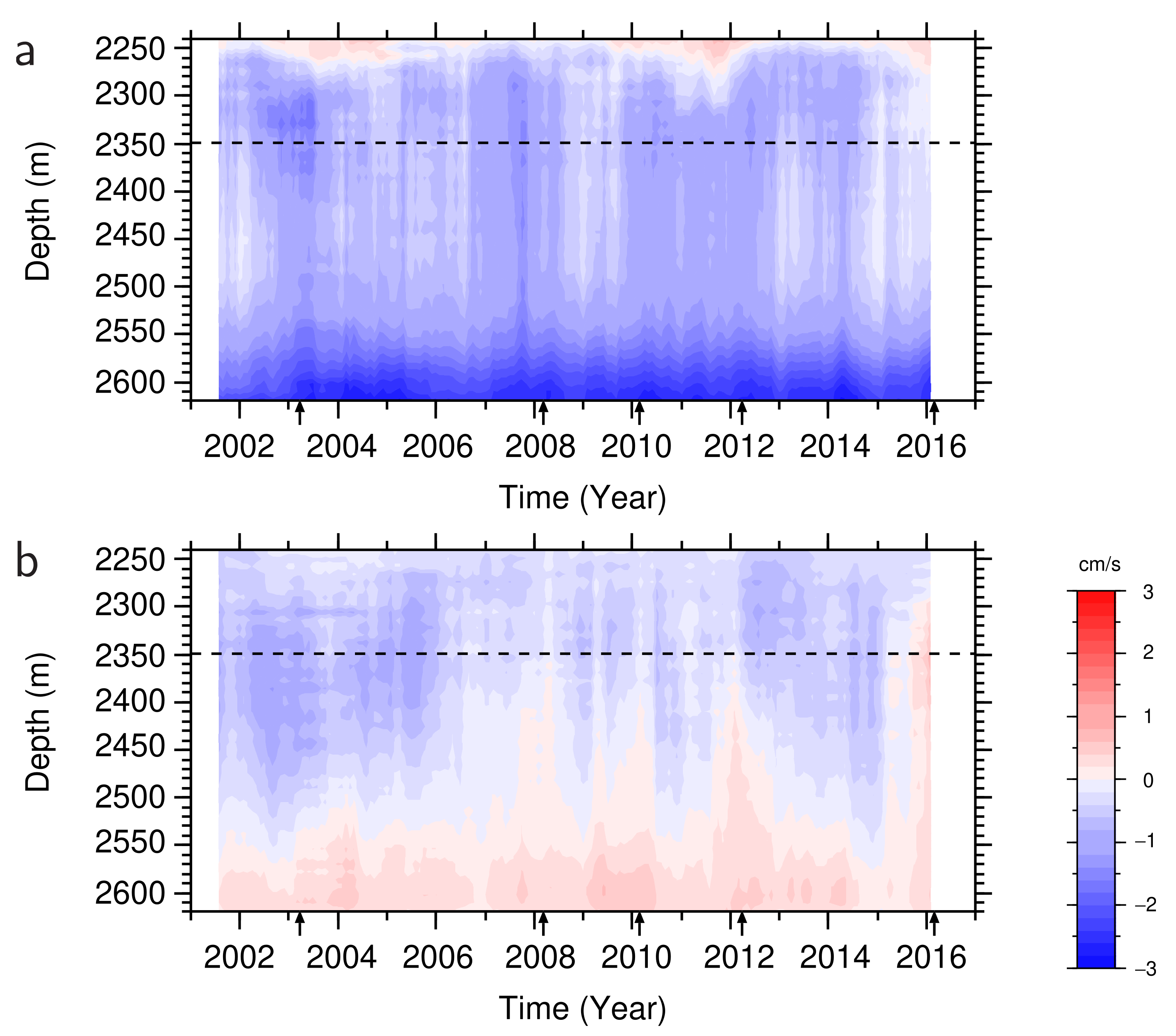
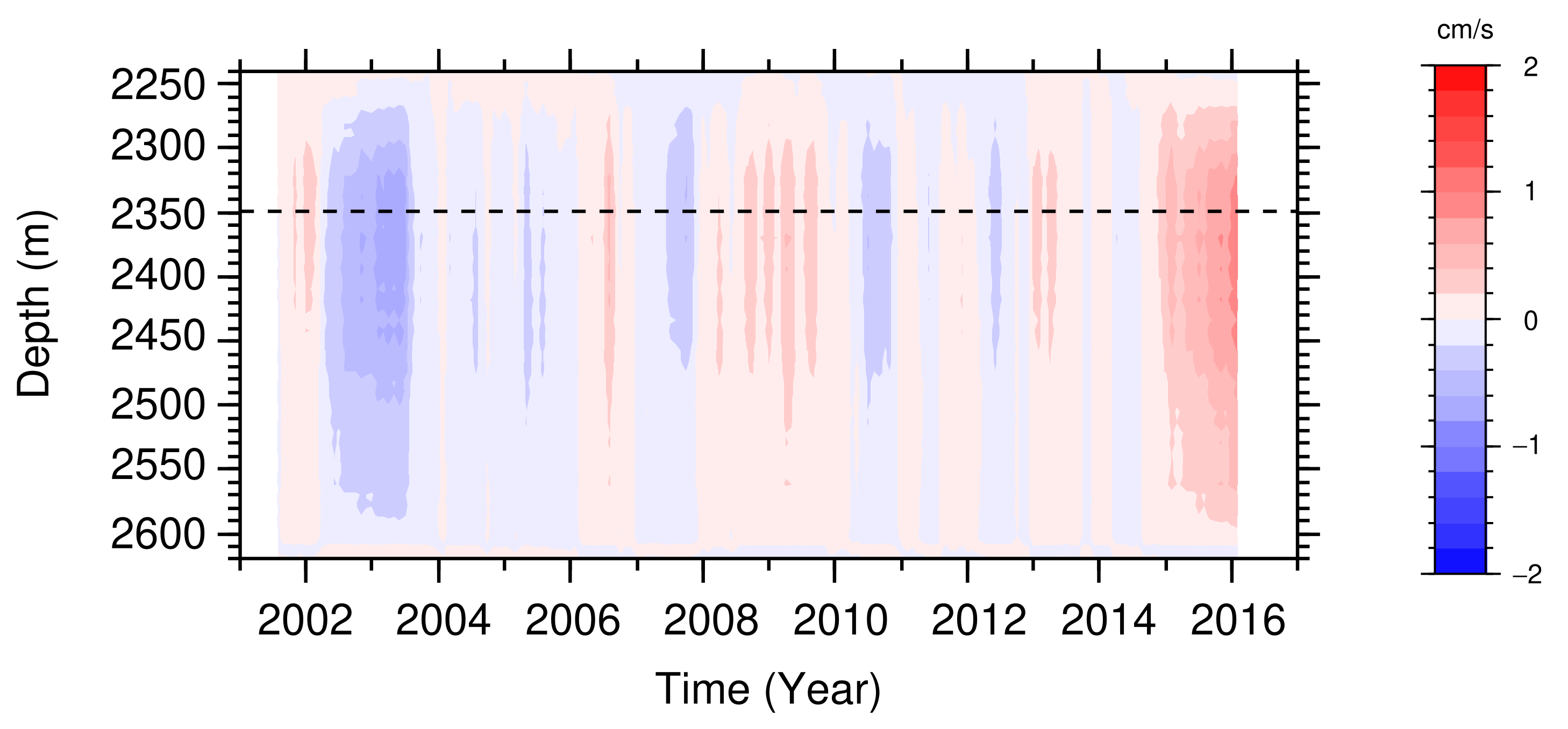
3.1. Vertical Structure of the Bottom Current
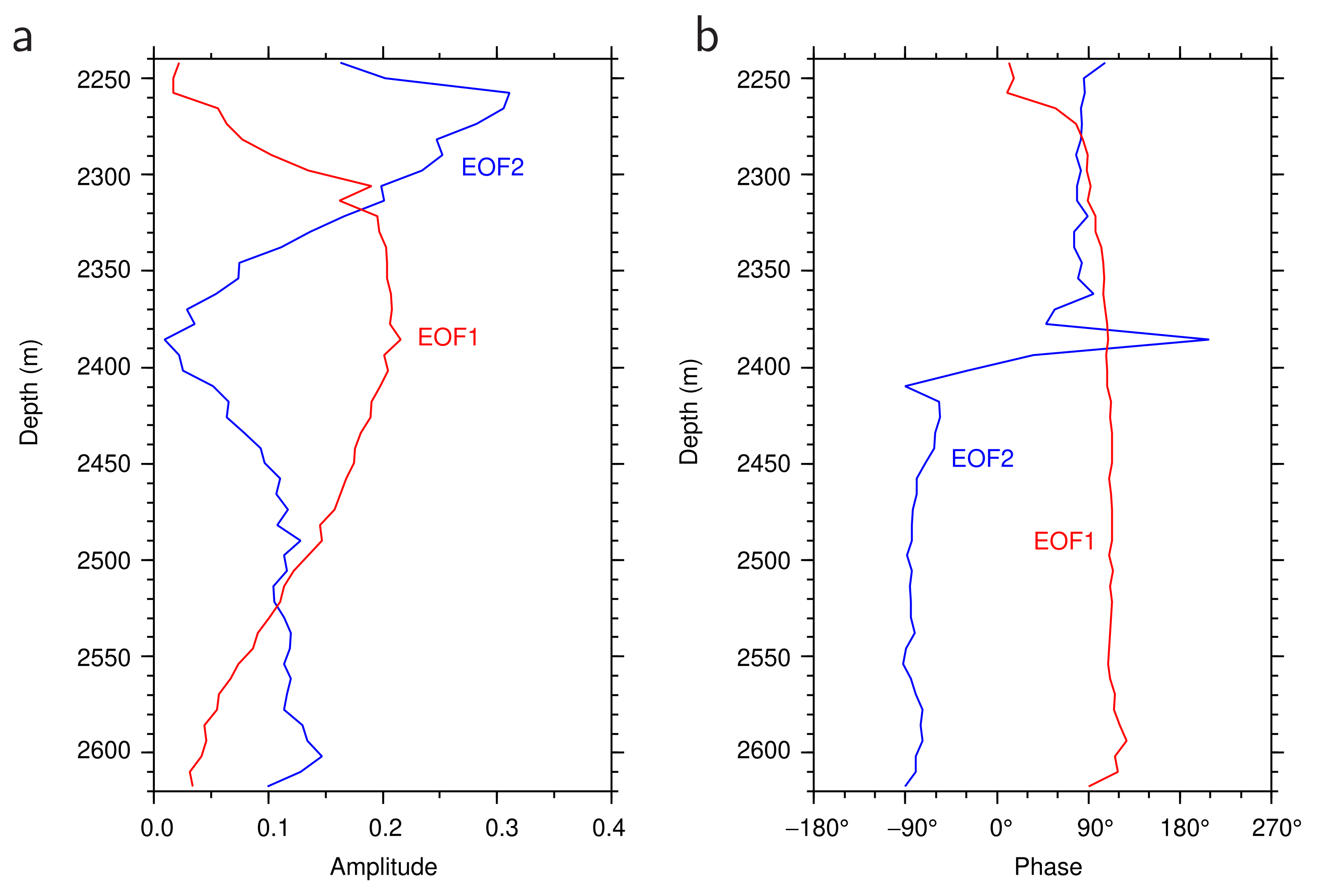
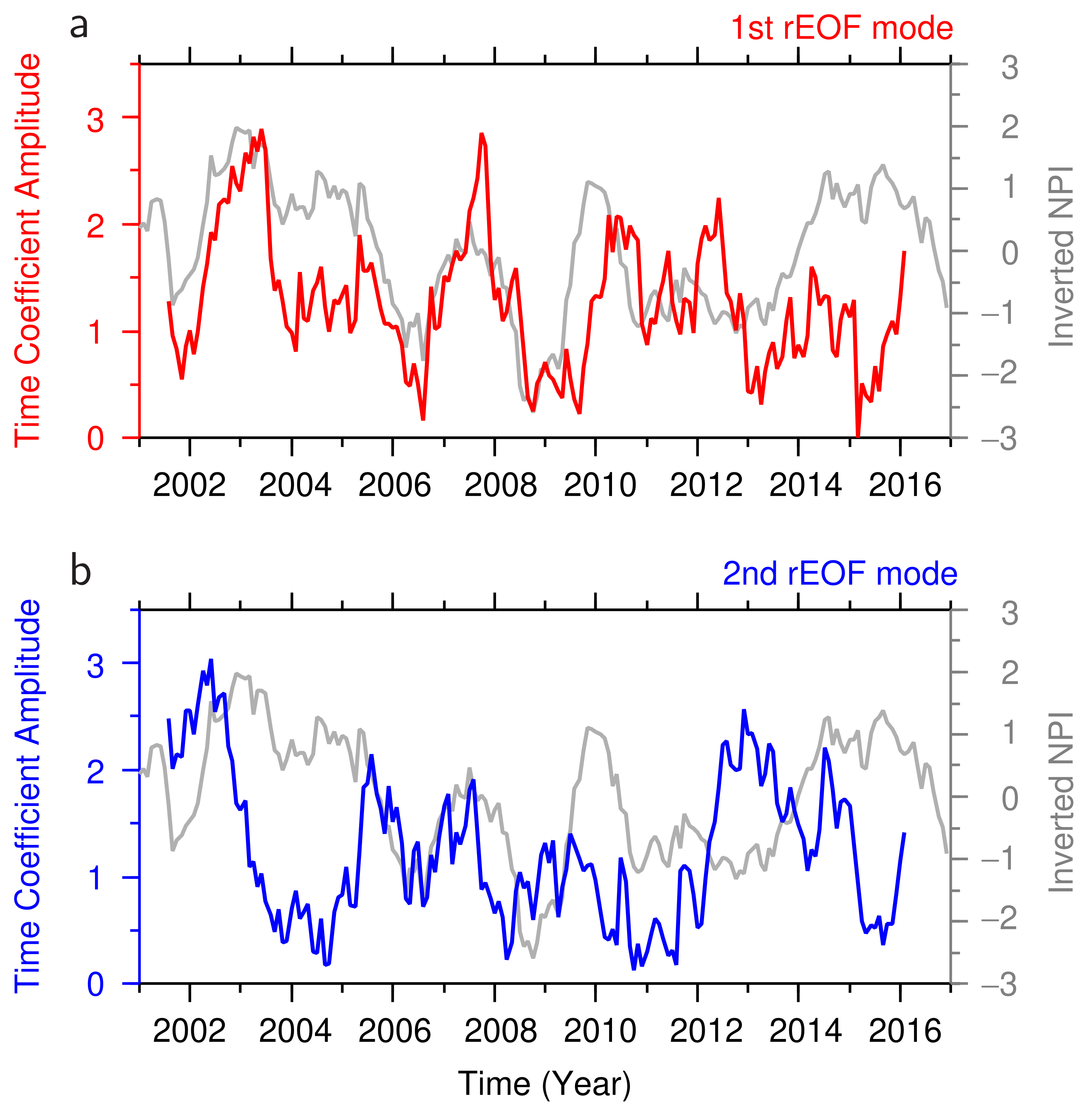
3.2. The Rotary EOF Modes of the Bottom Current Variation
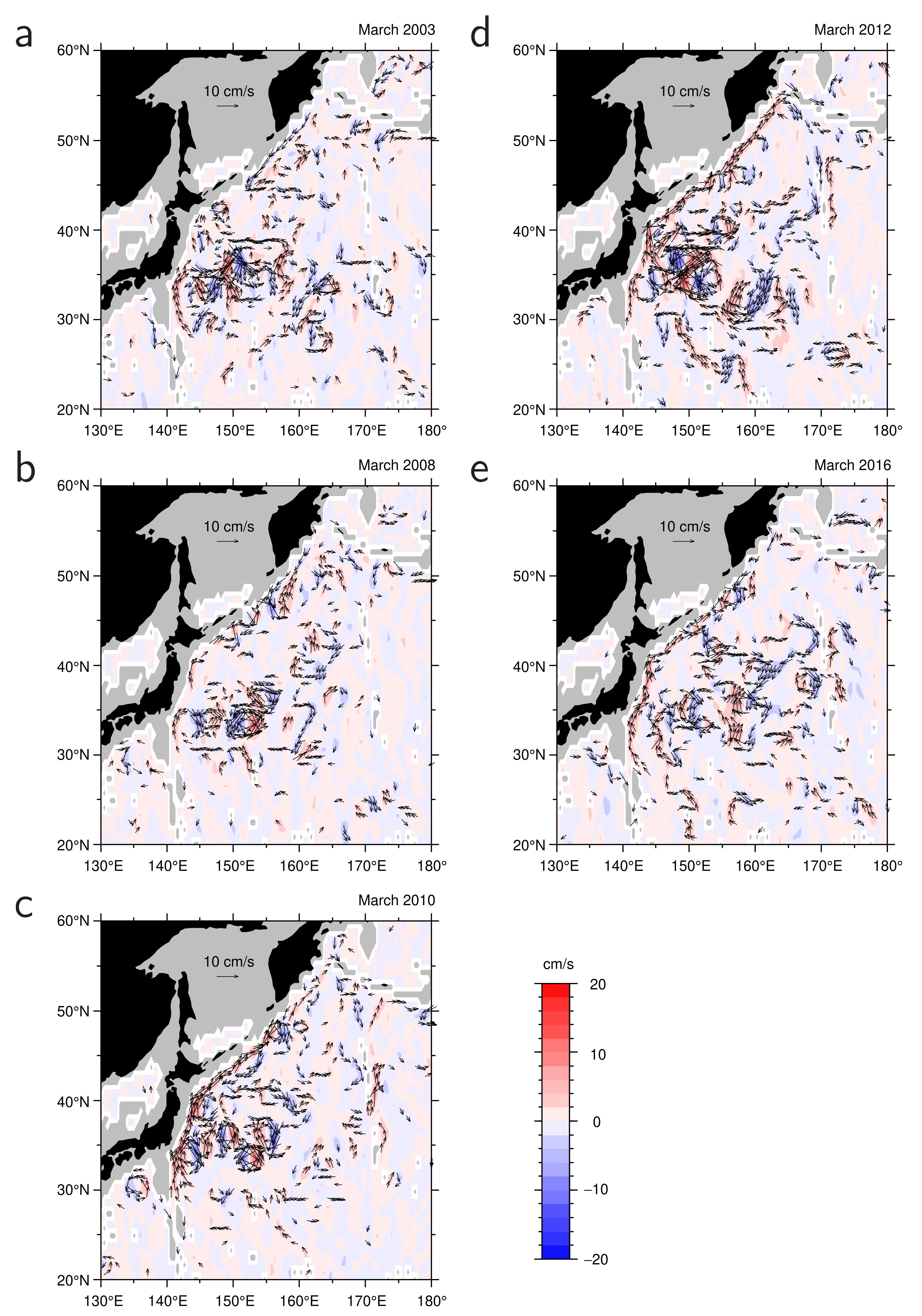
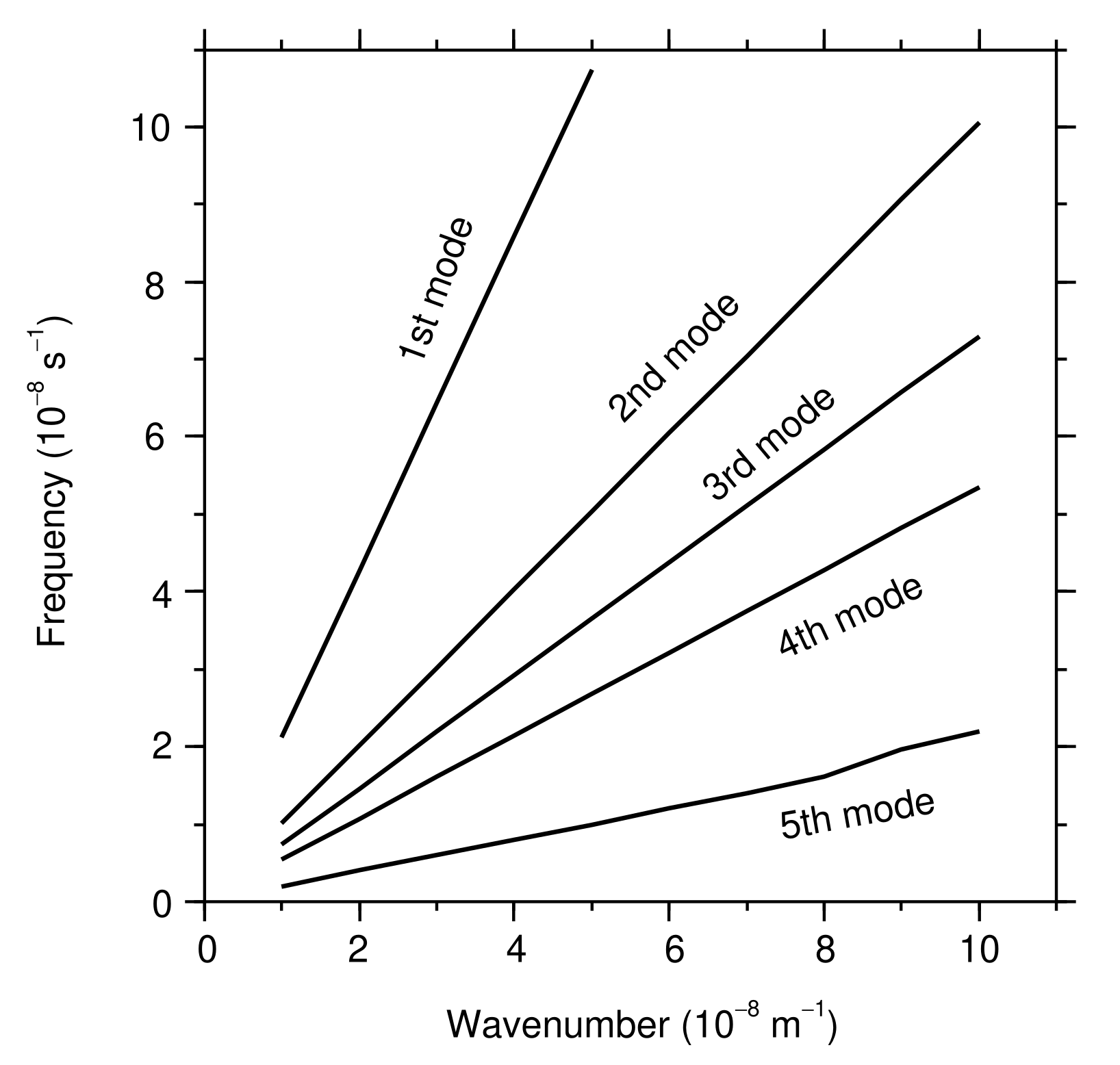
3.3. Current Structures of Coastal-Trapped Wave Modes
4. Summary
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| ADCP | Acoustic Doppler current profiler |
| CTW | Coastal-trapped wave |
| ENSO | El Niño–Southern Oscillation |
| EOF | Empirical orthogonal function |
| JAMSTEC | Japan Agency for Marine-Earth and Technology |
| NPI | North Pacific Index |
| OFES | Ocean general circulation model for the Earth Simulator |
| OGCM | Ocean general circulation model |
References
- McCane, I.N. Local and Global Aspects of the Bottom Nepheloid Layers in the World Ocean. Neth. J. Sea Res. 1986, 20, 167–181. [Google Scholar] [CrossRef]
- Bomberg, J.; Ariyoshi, K.; Hautala, S.; Johnson, H.P. The Finicky Nature of Earthquake Shaking-triggered Submarine Sediment Slope Failures and Sediment Gravity Flows. J. Geophys. Res. 2021, 126, e2021JB022588. [Google Scholar] [CrossRef]
- Komaki, K.; Nagano, A. Monitoring the deep western boundary current in the western North Pacific by echo intensity measured with lowered acoustic Doppler current profiler. Mar. Geophys. Res. 2019, 40, 515–523. [Google Scholar] [CrossRef]
- Mao, L.; Zeng, S.; Liu, Q.; Wang, G.; He, Y. Dynamical mechanics behavior and safety analysis of deep water riser considering the normal drilling condition and hang-off condition. Ocean Eng. 2020, 199, 106996. [Google Scholar] [CrossRef]
- Williamson, C.H.K.; Govardhan, R. Vortex-induced Vibrations. Annu. Rev. Fluid Mech. 2004, 36, 413–455. [Google Scholar] [CrossRef] [Green Version]
- Davidson, P.A. Turbulence: An Introduction for Scienctist and Engineers, 2nd ed.; Oxford University Press: Oxford, UK, 2015. [Google Scholar]
- Kawabe, M.; Fujio, S.; Yanagimoto, D.; Tanaka, K. Water masses and currents of deep circulation southwest of the Shatsky Rise in the western North Pacific. Deep-Sea Res. I 2009, 56, 1675–1687. [Google Scholar] [CrossRef]
- Komaki, K.; Kawabe, M. Deep-circulation current through the Main Gap of the Emperor Seamounts Chain in the North Pacific. Deep-Sea Res. I 2009, 56, 305–313. [Google Scholar] [CrossRef]
- Nagano, A.; Ichikawa, H.; Ichikawa, K.; Konda, M. Bottom Currents on the continental slope off Shikoku. In Proceedings of the OCEANS 2008—MTS/IEEE Kobe Techno-Ocean, Kobe, Japan, 8–11 April 2008; pp. 1–4. [Google Scholar] [CrossRef]
- Nagano, A.; Ichikawa, K.; Ichikawa, H.; Yoshikawa, Y.; Murakami, K. Large ageostrophic currents in the abyssal layer southeast of Kyushu, Japan, by direct measurement of LADCP. J. Oceanogr. 2013, 69, 259–268. [Google Scholar] [CrossRef]
- Kawabe, M.; Yanagimoto, D.; Kitagawa, S. Variations of deep western boundary currents in the Melanesian Basin in the western North Pacific. Deep-Sea Res. I 2006, 53, 942–959. [Google Scholar] [CrossRef]
- Taira, K.; Teramoto, T. Bottom Currents in Nankai Trough and Sagami Trough. J. Oceanogr. Soc. Jpn. 1985, 41, 388–398. [Google Scholar] [CrossRef]
- Weatherly, G.L.; Kelly, E.A., Jr. Storms and flow reversals at the HEBBLE site. Mar. Geol. 1985, 66, 205–218. [Google Scholar] [CrossRef]
- Hasegawa, T.; Nagano, A.; Matsumoto, H.; Ariyoshi, K.; Wakita, M. El Niño-related sea surface elevation and ocean bottom pressure enhancement associated with the retreat of the Oyashio southeast of Hokkaido, Japan. Mar. Geophys. Res. 2019, 40, 505–512. [Google Scholar] [CrossRef]
- Hasegawa, T.; Nagano, A.; Ariyoshi, K.; Miyama, T.; Matsumoto, H.; Iwase, R.; Wakita, M. Effect of Ocean Fluid Changes on Pressure on the Seafloor: Ocean Assimilation Data Analysis on Warm-core Rings off the Southeastern Coast of Hokkaido, Japan on an Interannual Timescale. Front. Earth Sci. 2021, 9, 600930. [Google Scholar] [CrossRef]
- Hirata, K.; Aoyagi, M.; Mikada, H.; Kawaguchi, K.; Kaiho, Y.; Iwase, R.; Morita, S.; Fujisawa, I.; Sugioka, H.; Mitsuzawa, K.; et al. Real-time geophysical measurements on the deep seafloor using submarine cable in the southern Kurile subduction zone. IEEE J. Ocean Eng. 2002, 27, 170–181. [Google Scholar] [CrossRef]
- Nagano, A.; Wakita, M. Wind-driven decadal sea surface height and main pycnocline depth changes in the western subarctic North Pacific. Prog. Earth Planet. Sci. 2019, 6, 59. [Google Scholar] [CrossRef] [Green Version]
- Gill, A.E.; Clarke, A.J. Wind-induced upwelling, coastal currents and sea-level changes. Deep-Sea Res. 1974, 21, 325–345. [Google Scholar] [CrossRef]
- Huthnance, J.M. On Coastal Trapped Waves: Analysis and Numerical Calculation by Inverse Iteration. J. Phys. Oceanogr. 1978, 8, 74–92. [Google Scholar] [CrossRef] [Green Version]
- Gill, A.E. Atmosphere-Ocean Dynamics; Academic Press: London, UK, 1982. [Google Scholar]
- Denbo, D.W.; Allen, J.S. Rotary Empirical Orthogonal Function Analysis of Currents near the Oregon Coast. J. Phys. Oceanogr. 1984, 14, 35–46. [Google Scholar] [CrossRef] [Green Version]
- Thomson, R.E.; Emery, W.J. Data Analysis Methods in Physical Oceanography, 3rd ed.; Elsevier: Amsterdam, The Netherlands, 2014. [Google Scholar] [CrossRef]
- Nagano, A.; Wakita, M.; Fujiki, T.; Uchida, H. El Niño-related Vertical Mixing Enhancement under the Winter Mixed Layer at Western Subarctic North Pacific Station K2. J. Geophys. Res. 2021, 126, e2020JC016913. [Google Scholar] [CrossRef]
- Brink, K.H.; Chapman, D.C. Programs for Computing Properties of Coastal-trapped Waves and Wind-driven Motions Over the Continental Shelf and Slope; Technical Report WHOI-87-24; Woods Hole Oceanographic Institution: Woods Hole, MA, USA, 1987; p. 02543. [Google Scholar]
- Kawaguchi, K.; Hirata, K.; Mikada, H.; Kaiho, Y.; Iwase, R. An expendable deep seafloor monitoring system for earthquake and tsunami observation network. In Proceedings of the 2000 MTS/IEEE Conference, Providence, RI, USA, 11–14 September 2000; pp. 1719–1722. [Google Scholar]
- Thèbault, E.; Finlay, C.C.; Beggan, C.D.; Alken, P.; Aubert, J.; Barrois, O.; Bertrand, F.; Bondar, T.; Boness, A.; Brocco, L.; et al. International Geomagnetic Reference Field: The 12th generation. Earth Planets Space 2015, 67, 79. [Google Scholar] [CrossRef]
- Pacanowski, R.C.; Griffies, S.M. MOM 3.0 Manual; Geophysical Fluid Dynamics Laboratory/National Oceanic and Atmospheric Administration: Princeton, NJ, USA, 2000.
- Masumoto, Y.; Sasaki, H.; Kagimoto, T.; Komori, N.; Ishida, A.; Sasai, Y.; Miyama, T.; Motoi, T.; Mitsudera, H.; Takahashi, K.; et al. A five-year-eddy-resolving simulation of the world ocean—Preliminary outcomes of OFES (OGCM for the Earth Simulator). J. Earth Simulator 2004, 1, 35–56. [Google Scholar]
- Sasai, Y.; Ishida, A.; Yamanaka, Y.; Sasaki, H. Chlorofluorocarbons in a global ocean eddy-resolving OGCM: Pathway and formation of Antarctic Bottom Water. Geophys. Res. Lett. 2004, 31, L12305. [Google Scholar] [CrossRef]
- Sasaki, H.; Sasai, Y.; Kawahara, S.; Furuichi, M.; Araki, F.; Ishida, A.; Yamanaka, Y.; Masumoto, Y.; Sakuma, H. A series of eddy-resolving ocean simulations in the world ocean: OFES (OGCM for the Earth Simulator) project. In Proceedings of the Oceans ‘04 MTS/IEEE Techno-Ocean ‘04 (IEEE Cat. No.04CH37600), Kobe, Japan, 9–12 November 2004; Volume 3, pp. 1535–1541. [Google Scholar] [CrossRef]
- Sasaki, H.; Nonaka, M.; Masumoto, Y.; Sasai, Y.; Uehara, H.; Sakuma, H. An eddy-resolving hindcast simulation of the quasi-global ocean from 1950 to 2003 on the Earth Simulator. In High Resolution Numerical Modelling of the Atmosphere and Ocean; Ohfuchi, W., Hamilton, K., Eds.; Springer: New York, NY, USA, 2008; pp. 157–185. [Google Scholar] [CrossRef]
- Kalnay, E.; Kanamitsu, M.; Kistler, R.; Collins, W.; Deaven, D.; Gandin, L.; Iredell, M.; Saha, S.; White, G.; Woollen, J.; et al. The NCEP/NCAR 40-year reanalysis project. Bull. Am. Meteorol. Soc. 1996, 77, 437–471. [Google Scholar] [CrossRef] [Green Version]
- Trenberth, K.E.; Hurrel, J.W. Decadal atmosphere-ocean variations in the Pacific. Clim. Dynam. 1994, 9, 303–319. [Google Scholar] [CrossRef]
- Mezzina, B.; Palmeiro, F.M.; Garcia-Serrano, J.; Bladé, I.; Batté, L. Multi-model assessment of the late-winter stratospheric response to El Niño and La Niña. Clim. Dyn. 2021. [Google Scholar] [CrossRef]
- Hallock, Z.R.; Teague, W.J. Evidence for a North Pacific Deep Western Boundary Current. J. Geophys. Res. 1996, 101, 6617–6624. [Google Scholar] [CrossRef]
- Mitsuzawa, K.; Holloway, G. Characteristics of deep currents along trenches in the northwest Pacific. J. Geophys. Res. 1998, 103, 13085–13092. [Google Scholar] [CrossRef] [Green Version]
- Owens, W.B.; Warren, B.A. Deep circulation in the northwest corner of the Pacific Ocean. Deep-Sea Res. I 2001, 48, 959–993. [Google Scholar] [CrossRef]
- Fujio, S.; Yanagimoto, D. Deep current measurements at 38° N east of Japan. J. Geophys. Res. 2005, 110. [Google Scholar] [CrossRef]
- Wimbush, M.; Munk, W. The benthic boundary layer. In The Sea; Maxwell, A.E., Ed.; John Wiley: New York, NY, USA, 1970; Volume 4, pp. 731–758. [Google Scholar]
- Brink, K.H. A Comparison of Long Coastal Trapped Wave Theory with Observations off Peru. J. Phys. Oceanogr. 1982, 12, 897–913. [Google Scholar] [CrossRef] [Green Version]
- Garcia, H.E.; Boyer, T.P.; Baranova, O.K.; Locarnini, R.A.; Mishonov, A.V.; Grodsky, A.; Paver, C.R.; Weathers, K.W.; Smolyar, I.V.; Reagan, J.R.; et al. World Ocean Atlas 2018: Product Documentation; Ocean Climate Laboratory NCEI/NESDIS/NOAA: Princeton, NJ, USA, 2019.
- Amante, C.; Eakins, B.W. ETOPO1 1 Arc-Minute Global Relief Model: Procedures, Data Sources and Analysis; Technical Report, NESDIS NGDC-24; NOAA: Boulder, CO, USA, 2009.




Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nagano, A.; Hasegawa, T.; Ariyoshi, K.; Matsumoto, H. Interannual Bottom-Intensified Current Thickening Observed on the Continental Slope Off the Southeastern Coast of Hokkaido, Japan. Fluids 2022, 7, 84. https://doi.org/10.3390/fluids7020084
Nagano A, Hasegawa T, Ariyoshi K, Matsumoto H. Interannual Bottom-Intensified Current Thickening Observed on the Continental Slope Off the Southeastern Coast of Hokkaido, Japan. Fluids. 2022; 7(2):84. https://doi.org/10.3390/fluids7020084
Chicago/Turabian StyleNagano, Akira, Takuya Hasegawa, Keisuke Ariyoshi, and Hiroyuki Matsumoto. 2022. "Interannual Bottom-Intensified Current Thickening Observed on the Continental Slope Off the Southeastern Coast of Hokkaido, Japan" Fluids 7, no. 2: 84. https://doi.org/10.3390/fluids7020084
APA StyleNagano, A., Hasegawa, T., Ariyoshi, K., & Matsumoto, H. (2022). Interannual Bottom-Intensified Current Thickening Observed on the Continental Slope Off the Southeastern Coast of Hokkaido, Japan. Fluids, 7(2), 84. https://doi.org/10.3390/fluids7020084





