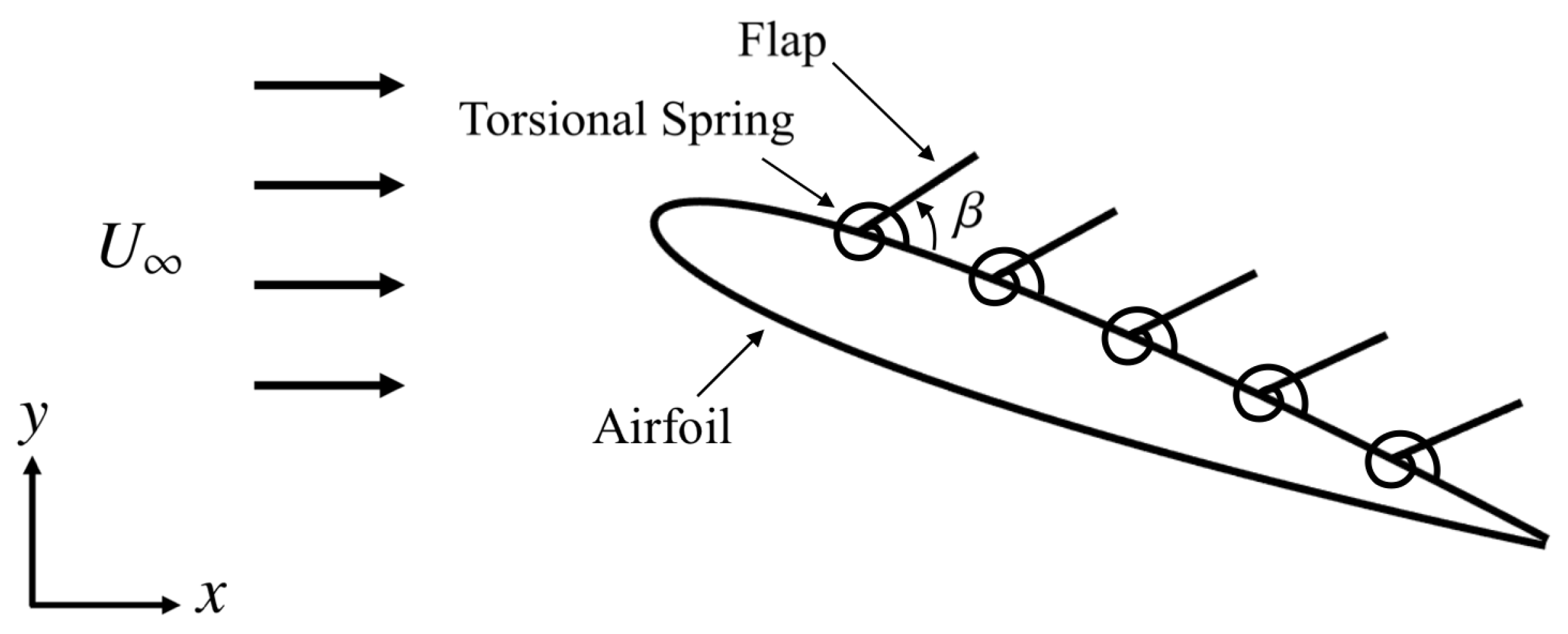
4.1. Qualitative Flow Features
We begin with an overview of the flow features for a representative case of flaps with a moment of inertia of
and hinge stiffness of
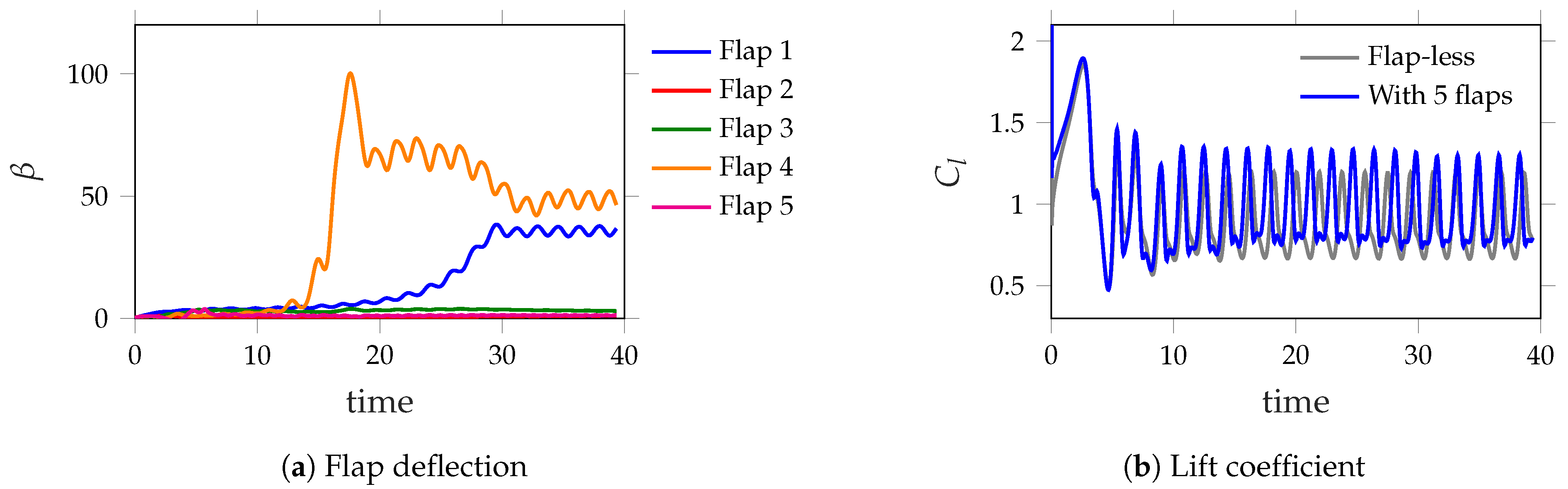
. The flap dynamics and airfoil lift are plotted in
Figure 3. From the flap deflection plots in
Figure 3a, we can see that, initially at
, all the flaps have near-zero deflection, i.e., they are rested near the airfoil surface. As flow separation and vortex shedding occur at the post-stall angle of attack of
, the flaps passively deploy into the flow. For the particular combination of
, the first and fourth flaps are significantly deployed, while the other flaps remain largely unaffected. The initial transients in the flap and lift dynamics progress onto limit cycle oscillations (LCO) after approximately
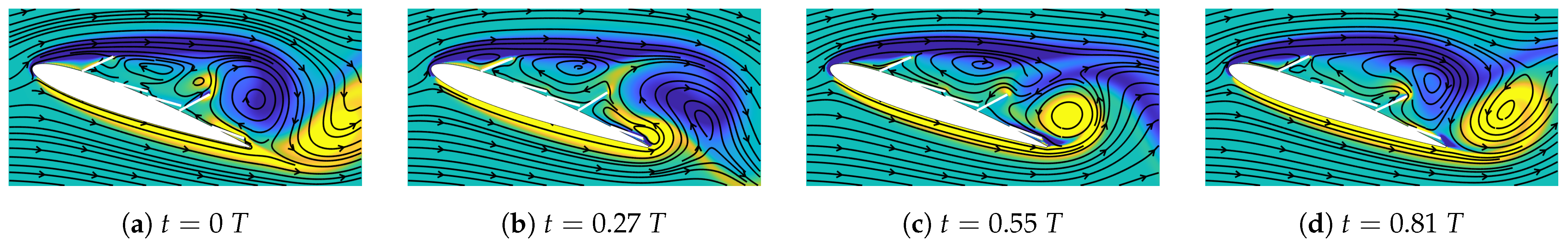
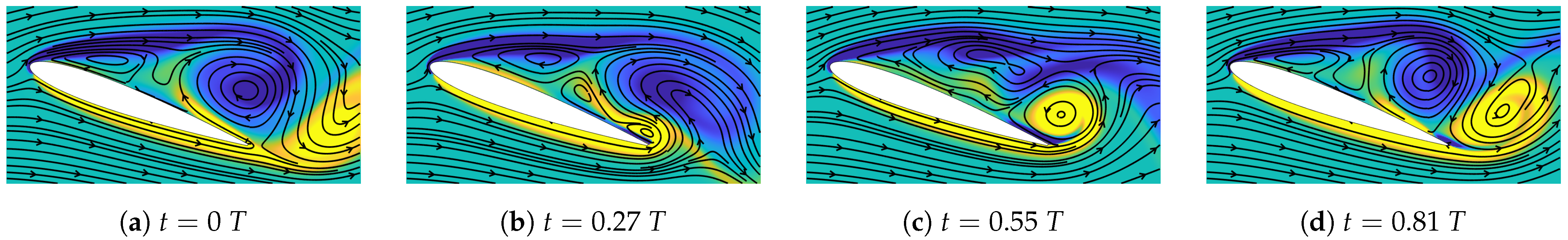
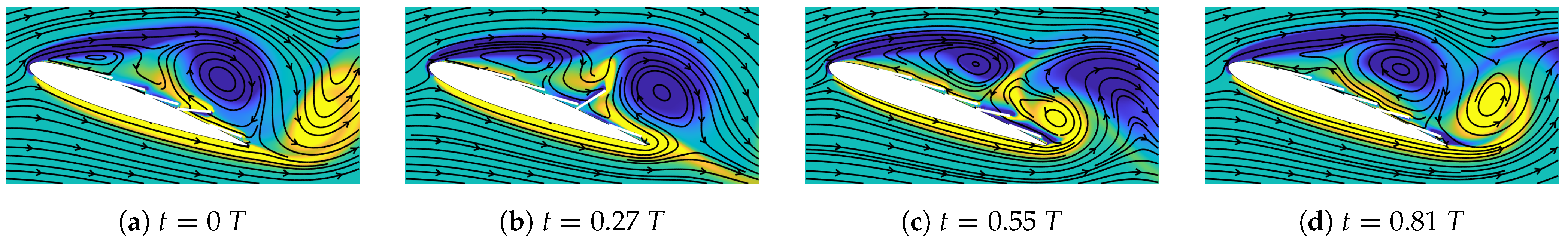
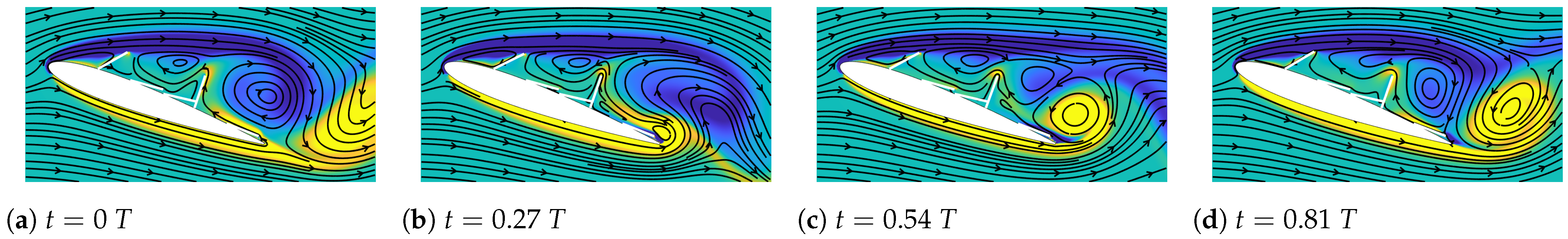
. These oscillations are the result of periodic vortex shedding at the leading and trailing edges of the airfoil. This periodic vortex shedding can be visualized via the vorticity contours plotted in one time period of the lift cycle in the LCO regime; see
Figure 4. Here, a lift cycle is defined between two consecutive peaks of
with time period
. The periodic formation, shedding and interaction of the leading- and trailing-edge vortices (LEV and TEV, respectively) can be clearly observed from these plots.
Similar vortex shedding is observed for the flap-less airfoil case, whose lift dynamics and vorticity contours are plotted in
Figure 3b and
Figure 5, respectively. From
Figure 3b, we observe that the mean lift improvement of the airfoil with torsionally mounted flaps is approximately
as compared to the baseline flap-less airfoil. In the following sections, we report the results of a parametric study of the airfoil–flap system and identify key physical mechanisms that yield significant lift improvements.
4.2. Performance Maps and Connections to Flap Dynamics
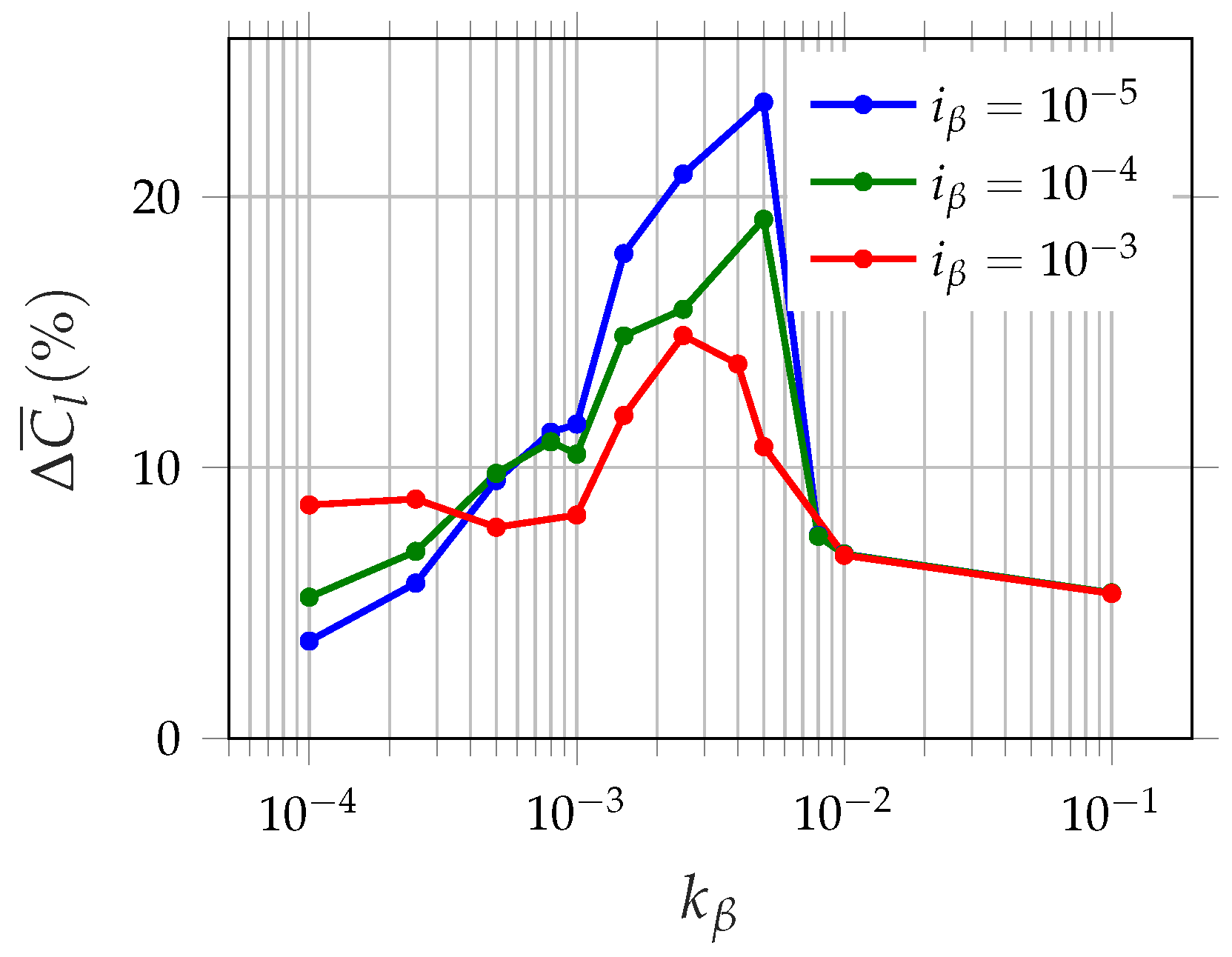
A parametric study is performed to characterize the effects of flap inertia and hinge stiffness on the lift improvements of the airfoil. In
Figure 6, we show
(lift improvement relative to the flap-less case, in percentage) against
for various
. It can be observed that significant lift improvements, up to
, can be achieved owing to the passively deployable flaps. The optimal performance is obtained for the lowest inertia case of
and stiffness of
. For a higher inertia of
, the peak performance is achieved at a similar stiffness of
. For all inertia values considered, as the stiffness is increased beyond
, all cases have identical performance. On the other hand, for very low stiffness tending towards
, the lift of the airfoil mounted with the lowest-inertia flaps deteriorates, while that with larger-inertia flaps hovers around
.
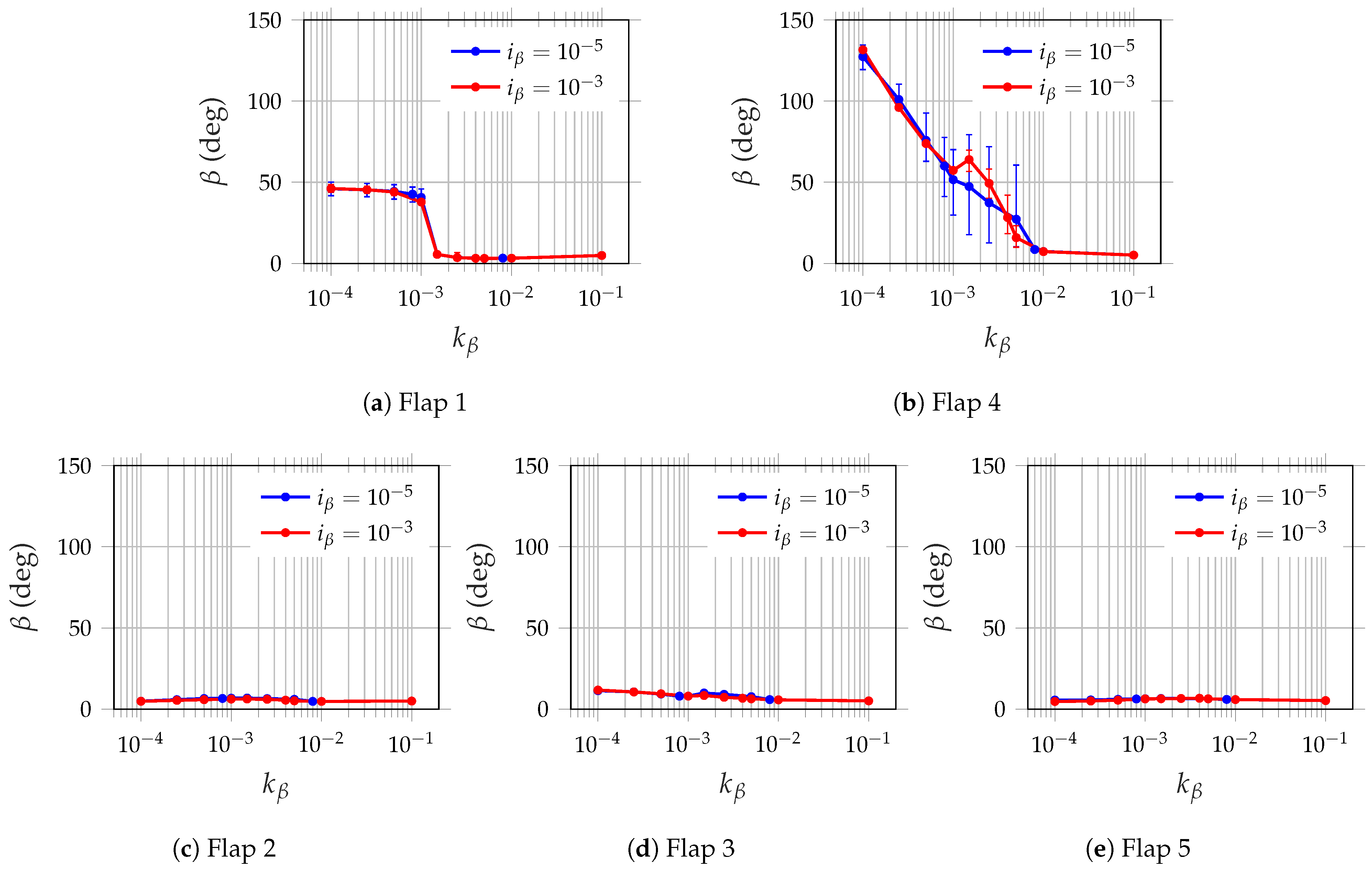
To analyze in detail the effect of flap dynamics on the aerodynamic performance, the mean flap deflections of the five flaps for the various cases of stiffness and inertia are plotted in
Figure 7. In this plot, the bars superimposed over the mean deflection markers denote the amplitude of flap oscillations about the mean. It can be observed that for the wide range of stiffness and inertia considered, only flaps 1 and 4 are significantly deployed. Since only flaps 1 and 4 are significantly deployed, hereafter, we only discuss the nature of the flap dynamics and associated effects on the flow physics of these two flaps.
We investigate the role of stiffness and inertia in governing the flap dynamics. From
Figure 7a,b, it can be observed that the mean flap deflections of flaps 1 and 4 decrease monotonically with increasing stiffness due to the increasing restoring forces of the torsional spring for all inertia. Furthermore, the mean deflection for a given stiffness is approximately the same across inertia. These similarities in mean deflection indicate that the stiffness determines the mean flap configuration, largely independent of inertia. On the other hand, inertia alters the flap amplitude about the mean, where the lower-inertia flaps undergo large amplitude oscillations, while the larger-inertia flaps have reduced amplitude. These variations in amplitude are more discernible around
in the mean deflection plot of flap 4 in
Figure 7b. The distinction of the roles of stiffness and inertia in setting the mean flap deflection and amplitude is crucial in analyzing the interaction of the flaps with the flow structures and the associated benefits in aerodynamic performance.
These flap deployment figures provide a means to identify certain flap dynamics (governed by stiffness and inertia) that are beneficial for aerodynamic lift. While the decrease in mean deflection with increasing stiffness is smooth for flap 4, as seen from
Figure 7b, a sharp drop in the mean deflection of flap 1 is observed at
in
Figure 7a. To the left of the drop for
, the deflection of flap 1 is approximately constant around
. On the other hand, flap 1 is largely undeployed for
. Now, we note from
Figure 6 that a huge boost in lift and peak performance around
= 0.0025–0.005 is achieved in this stiffness range of
, where only flap 4 is deployed for all inertia. This shows that a single flap located at
with an appropriate choice of stiffness is more beneficial than multiple flaps.
For the larger-inertia flap of
in the stiffness range of
, where both flaps 1 and 4 are deployed, modest but non-negligible lift improvements of up to
are achieved, as noted from
Figure 6. Here,
remains approximately constant, with a small peak in performance at
. On the other hand, the lower-inertia cases have monotonically decreasing lift with decreasing stiffness in the range of
, where both flaps 1 and 4 are deployed. This demonstrates that for very low stiffness, considerable lift improvements due to the deployment of multiple flaps can be still achieved provided that the flap inertia is relatively large. This result is significant despite the sub-optimal
, since many studies involving covert feathers consider a zero-stiffness hinge. Finally, beyond
, since the flap deflections of all flaps are minimal, lift improvements are not substantial.
To summarize the above discussion, we have identified two performance-conducive flap behavioral regimes: (i) only flap 4 deployed at and (ii) flaps 1 and 4 deployed at . In the following sections, we will analyze the fluid–structure mechanisms that yield performance benefits in both these regimes.
4.3. Flow Physics of the Airfoil–Flap System with Dynamics Dominated by Flap 4
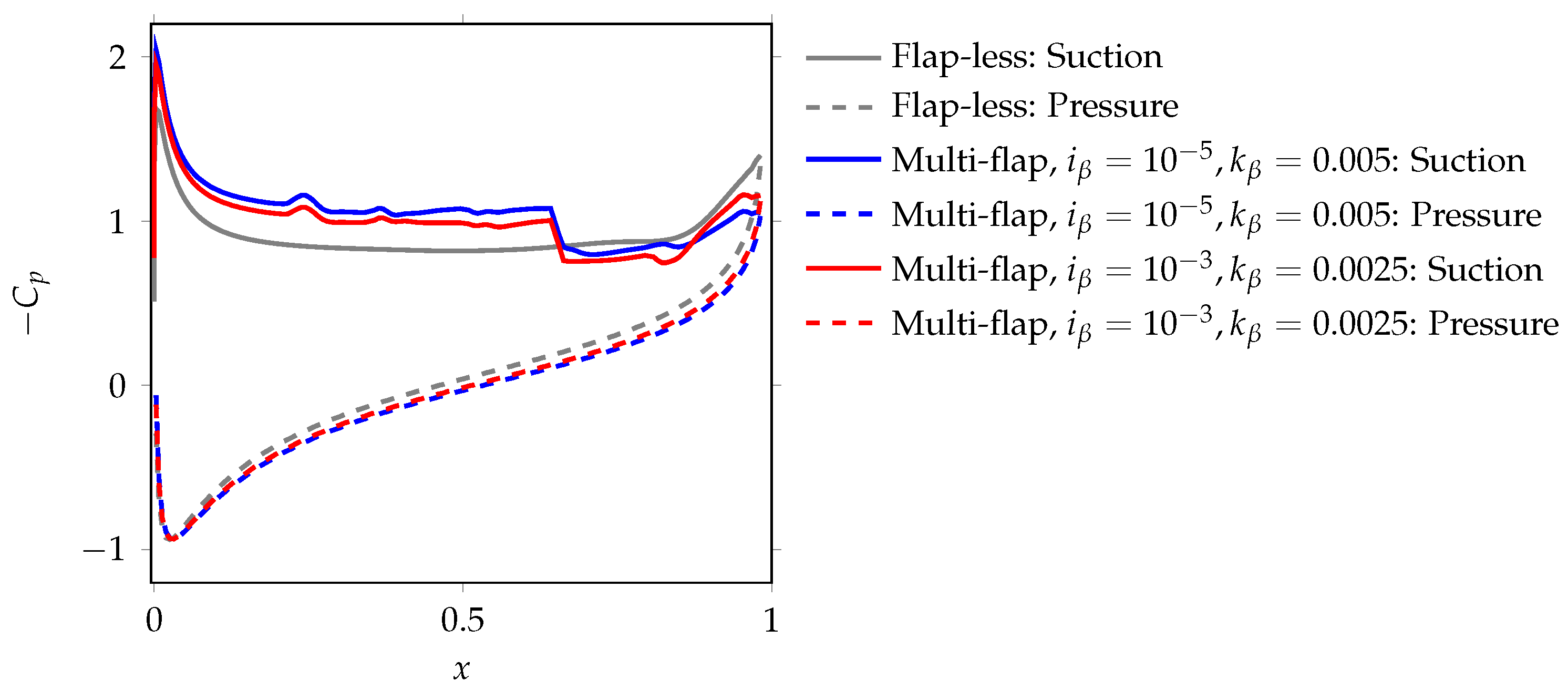
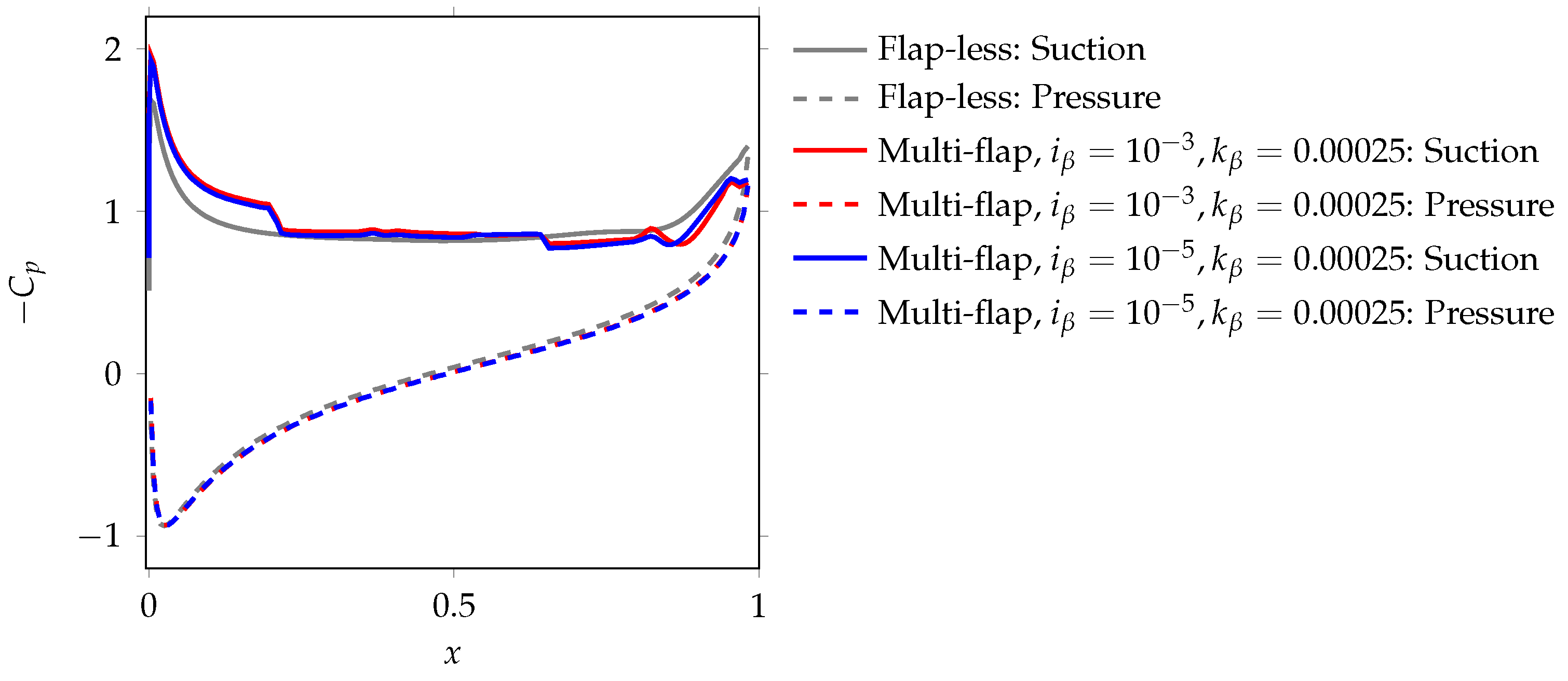
To identify the physical flow mechanisms that enhance lift when the flap 4 dynamics are the most prominent, we plotted the mean
distribution on the airfoil surface for the highest mean lift case of
and
, as shown in
Figure 8. For reference, we also plotted the mean
distribution for the baseline airfoil case, shown in the same figure. A step discontinuity in the suction surface
at the location of the hinge of the fourth flap at
is observed. (Small
perturbations are also observed at other flap hinge locations). Upstream of this discontinuity, a lower-pressure region as compared to the baseline case is formed. This phenomenon is referred to as the pressure dam effect since the flap acts as a barrier in maintaining a large suction region upstream of the flap.
To visualize the barrier constructed by the flap, vorticity contours with streamlines at four time instants in one lift cycle are plotted in
Figure 9 and compared with the vorticity contours of the flap-less airfoil case from
Figure 5. It can be observed that the number of streamlines propagating upstream from the trailing to the leading edge at
= 0.27 and 0.55 in
Figure 9b,c is significantly lower than that for the baseline case in
Figure 5b,c, respectively. These upstream-propagating streamlines represent the reverse flow induced by the TEV. At
, when the TEV is growing in strength, the flap blocks the TEV-induced reverse flow. On the other hand, when the TEV is of approximately maximum strength at
, the flap deflection is such that it is unable to fully block the TEV-induced reverse flow. However, at this instant, the reduced flap deflection facilitates the efficient downstream advection of the LEV. This advecting LEV, in addition to the slightly deployed flap, blocks the upstream propagation of TEV-induced reverse flow. Thus, in this behavioral regime, lift benefits are largely driven by the pressure dam effect seen in
Figure 8, and, in particular, the rejection of adverse high pressure from the TEV.
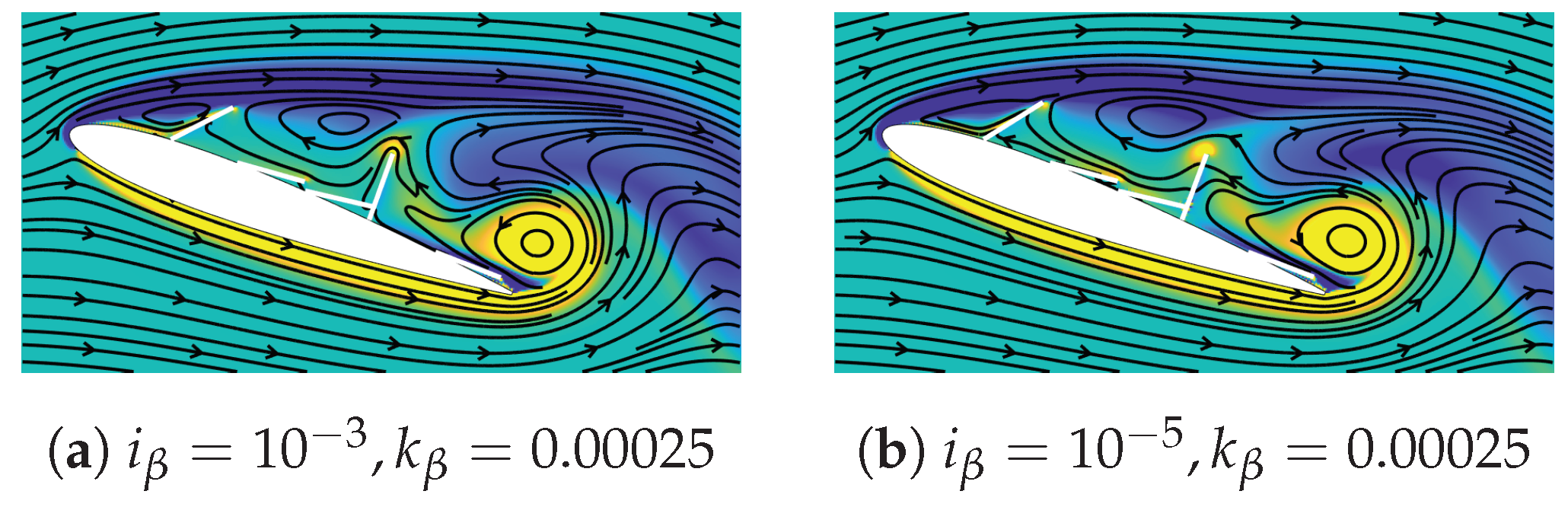
A similar pressure dam effect and associated peak performance are observed for the larger-inertia case of
at
, as observed in
Figure 8. However, the low-pressure region upstream of flap 4 for this larger-inertia case is not as lift-enhancing as that of the lower-inertia case of
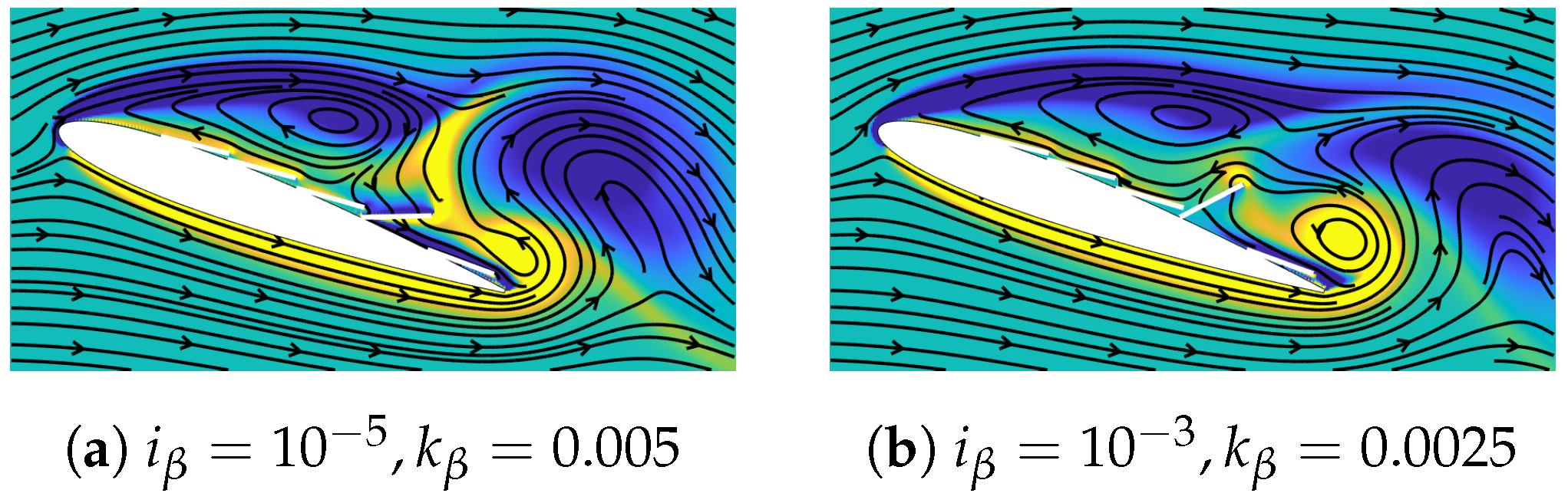
. To identify the distinguishing mechanisms between these inertia cases, the deflection of flap 4 in one time period of the lift cycle for these two cases is plotted in
Figure 10. In both cases, the flap oscillates downwards (i.e., decreasing deflection) starting at
. This time instant approximately marks the moment when the TEV is beginning to grow in strength and induce the lift-deteriorating reverse flow. While the flap itself blocks the reverse flow to some extent, as discussed above, the motion of the downward-moving flap augments this blocking by countering the upstream-propagating reverse flow. This phenomenon can be visualized from the vorticity contour at a representative time instant of
for
in
Figure 11a. Here, the downward-oscillating flap induces multiple downstream-moving streamlines through the flap, thereby restricting the TEV-induced reverse flow. We emphasize that the streamlines pass through the flap due to the no-slip boundary constraint enforced by the moving flap and that these streamlines do not imply a permeable flap.
Now, the lower-inertia flap oscillates with a larger angular velocity as compared to the larger-inertia flap, implied by the large-amplitude oscillations for
observed from
Figure 10. The larger-velocity flap counters more productively against the TEV-induced reverse flow. For comparison, the vorticity contour at the same time instant of
for
is plotted in
Figure 11b. Clearly, more upstream-propagating streamlines are discernible for the low-velocity, high-inertia flap in
Figure 11b as compared to the high-velocity, low-inertia flap in
Figure 11a. Additionally, the augmented blocking of reverse flow for the lower-inertia case mitigates the growth of the TEV, as implied by the smaller TEV size in
Figure 11a compared to that in
Figure 11b. The combined effect of increased flap velocity countering the reverse flow as well as reduced TEV strength enhances the performance of the airfoil–flap system equipped with lower-inertia flaps. This comparison highlights the need to understand both the mean dynamics (which facilitate a pressure dam phenomenon in the first place), as well as the detailed time-dependent dynamics enabled by distinct spring stiffness and flap inertia values (which allow for complex dynamics and additional lift benefits compared with a stationary flap).
4.4. Flow Physics of the Airfoil–Flap System Dominated by Dynamics of Flaps 1 and 4
In this section, we analyze the regime where both flaps are deployed. To describe the driving physics in this regime, we plot the mean
distribution on the airfoil surface in
Figure 12 for a representative case of
and
. This case corresponds to a local peak in performance observed in
Figure 6. Similar to the previous regime, a sharp pressure discontinuity on the suction surface is observed at the location of the hinge of the first flap. Additionally, a smaller discontinuity is observed at the hinge of the fourth flap. Unlike the previous regime, where the pressure dam effect was noticed upstream of flap 4, here, the performance-conducive low-pressure region is dominantly maintained upstream of flap 1. Between hinges 1 and 4, this pressure dam effect is not considerable.
To analyze the flow behavior in detail, vorticity contours at four time instants in one lift cycle are plotted in
Figure 13. It can be observed that both flaps 1 and 4 are deployed, with flap 1 extended towards the shear layer. Similar to the previous analysis, the vorticity contours and streamlines at
= 0.27 and 0.55 in
Figure 13b,c are compared with
Figure 5b,c of the flap-less airfoil case, respectively. Flap 1 is observed to restrict the upstream propagation of the TEV-induced reverse flow, resulting in a strong pressure dam effect on the suction surface till
. Flap 4 only moderately blocks the reverse flow since few streamlines are observed to propagate above the flap. Furthermore, unlike the previous regime, the advection of LEV is hindered due to the deployment of flaps 1 and 4, which detrimentally contributes to lift. Cumulatively, an already strong pressure dam effect generated by flap 1, and a sub-optimal reverse flow blocking by flap 4 and LEV distortion, result in negligible pressure benefits on the suction surface between hinges 1 and 4.
Finally, we note that the lower-inertia case of
also exhibits this sub-optimal blocking when flaps 1 and 4 are deployed. However, as deduced from
Figure 6, the lowest-inertia cases have inferior performance as compared to the higher-inertia counterparts in this flow regime where both flaps are deployed. The mean
distribution on the airfoil for
in
Figure 12 also supports this argument since the lower-inertia case has a slightly weaker suction surface
as compared to the higher-inertia case of
. To identify this deteriorating effect in the presence of lower-inertia flaps, we plotted the deflection of flap 4 in one time period of the lift cycle for these cases, as shown in
Figure 14. It can be observed that the lower-inertia case undergoes significantly larger amplitude oscillations as compared to the larger inertia case. Furthermore, during the critical time instances of the growth and shedding of TEV between
and
, the flap oscillates upwards (i.e., increasing deflection) in response to the TEV-induced reverse flow. To visualize this effect,
Figure 15 shows the vorticity contours at a representative time instant of
for the low- and high-inertia cases. More upstream-propagating streamlines passing through the flap for the lowest-inertia case in
Figure 15b can be observed as compared to the higher-inertia case in
Figure 15a. This upward movement of the flap facilitates the upstream propagation of TEV-induced reverse flow. Therefore, the lower-inertia flaps experience additional performance detriments due to their high-velocity upward flap motion as compared to the quasi-static higher-inertia case. Moreover, by comparison with the flap-4-dominated regime from the prior subsection, these results demonstrate the importance of not only flap deflection amplitudes but the phase relationship between these flap dynamics with key flow processes.