Numerical Study on an Interface Compression Method for the Volume of Fluid Approach
Abstract
1. Introduction
2. Linear Theory
3. CFD Analysis
3.1. Governing Equations
3.2. IC Method
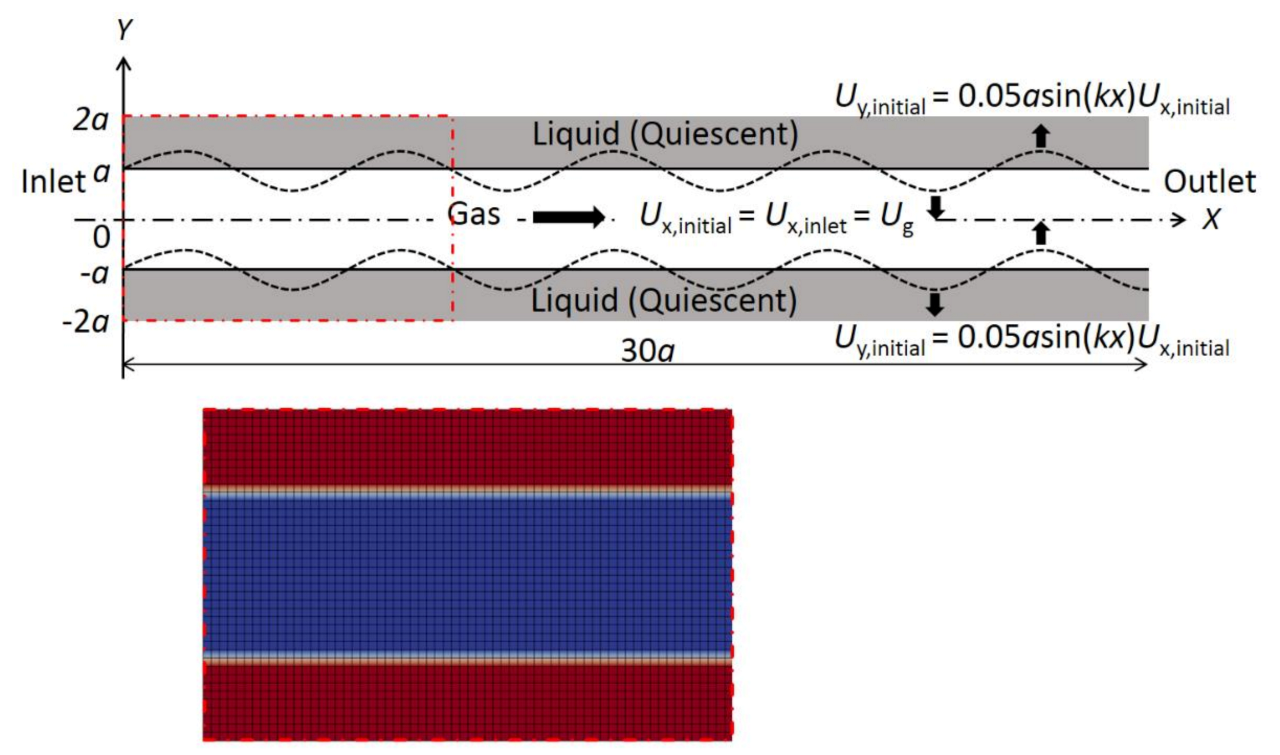
3.3. Initial and Boundary Conditions
4. Results and Discussion
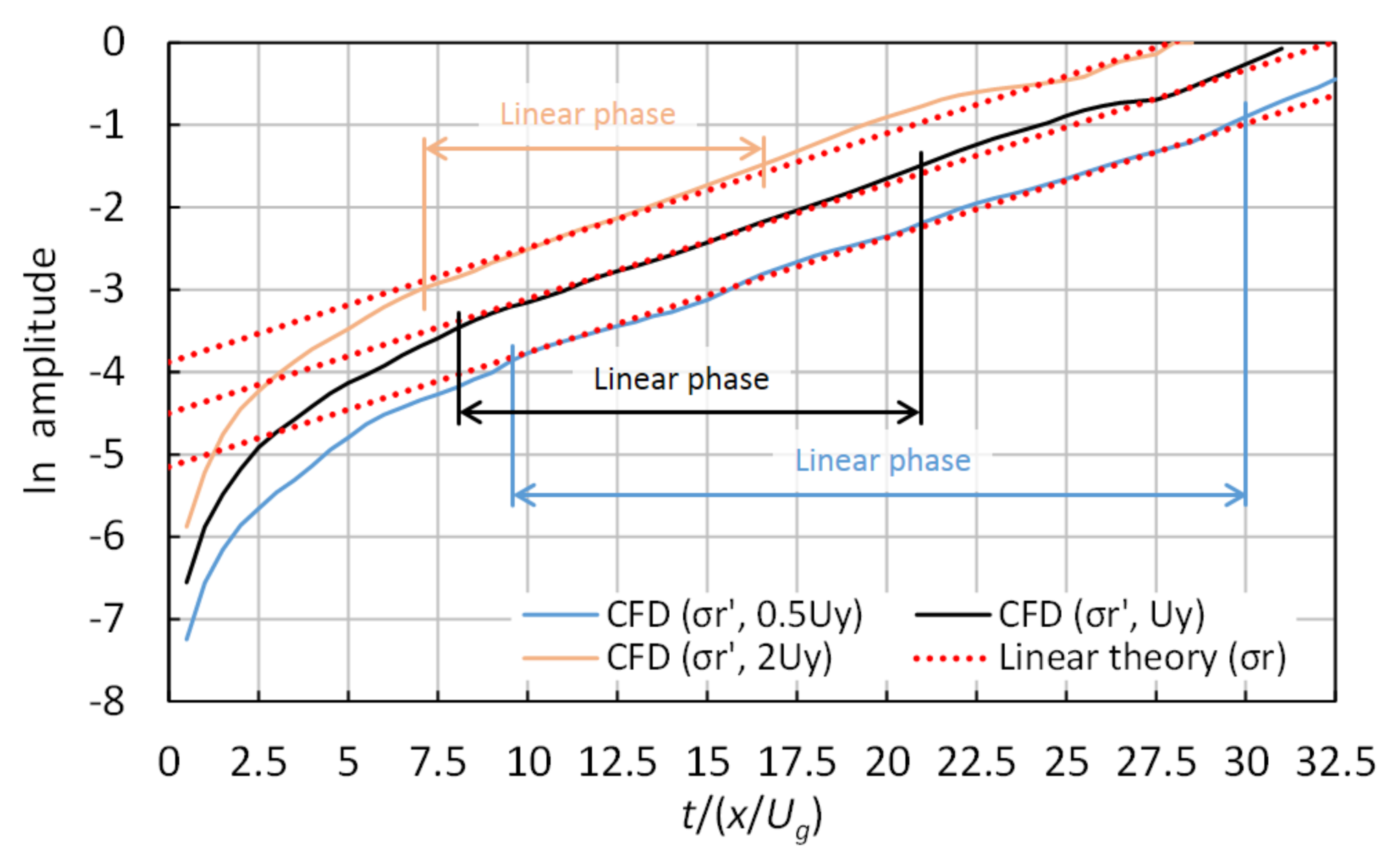
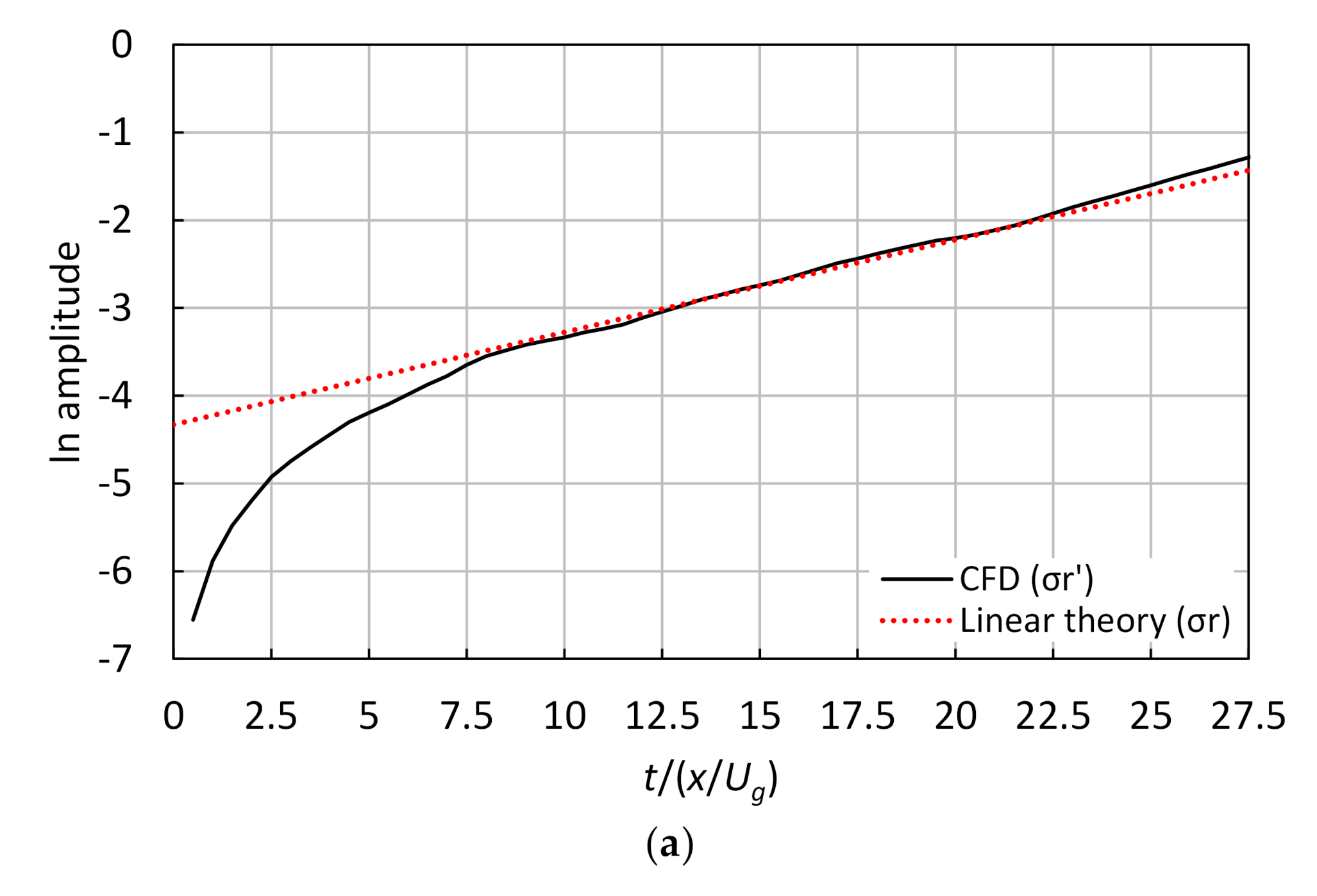
4.1. Consideration of the Validation Process
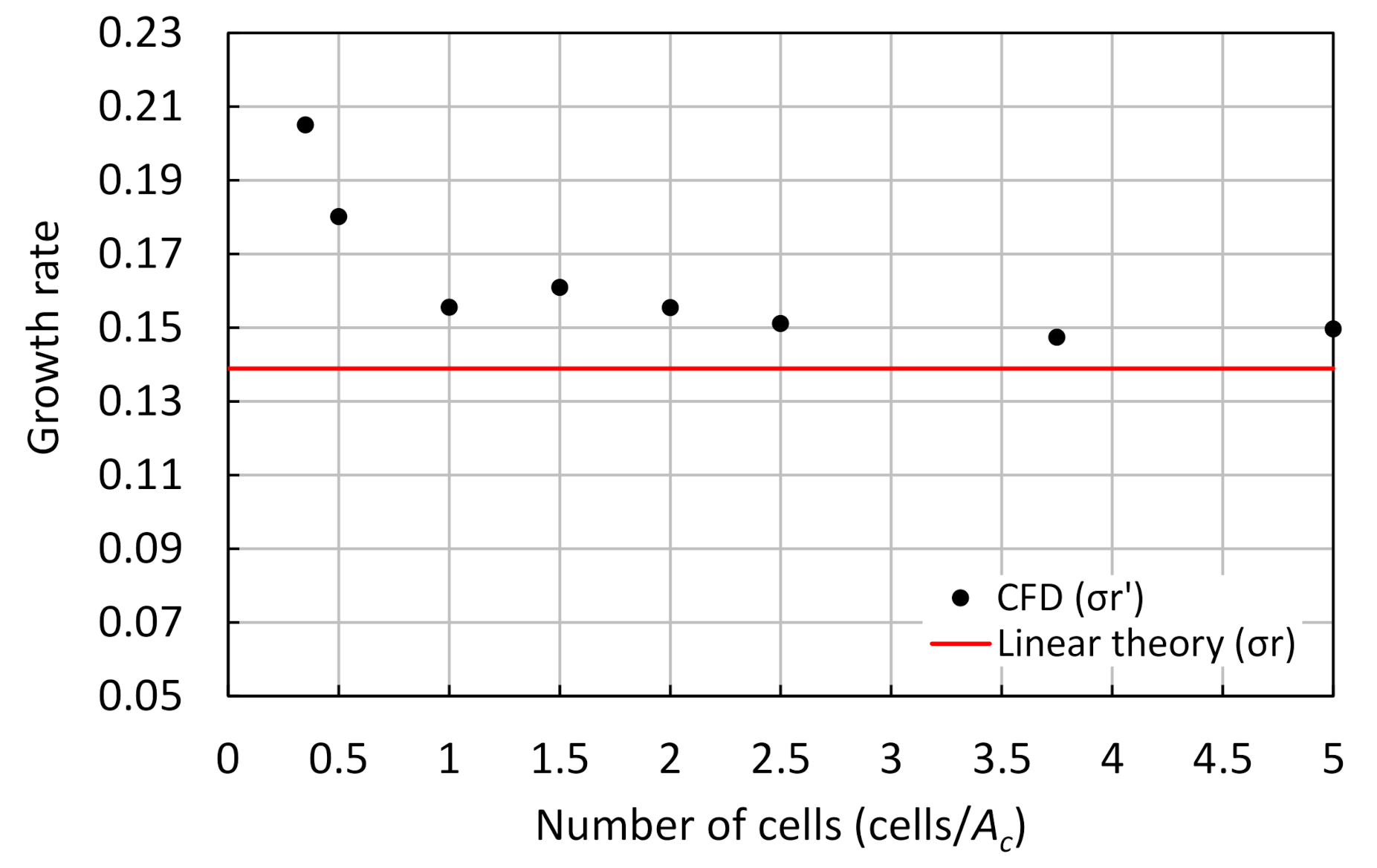
4.2. Effect of the Mesh Resolution
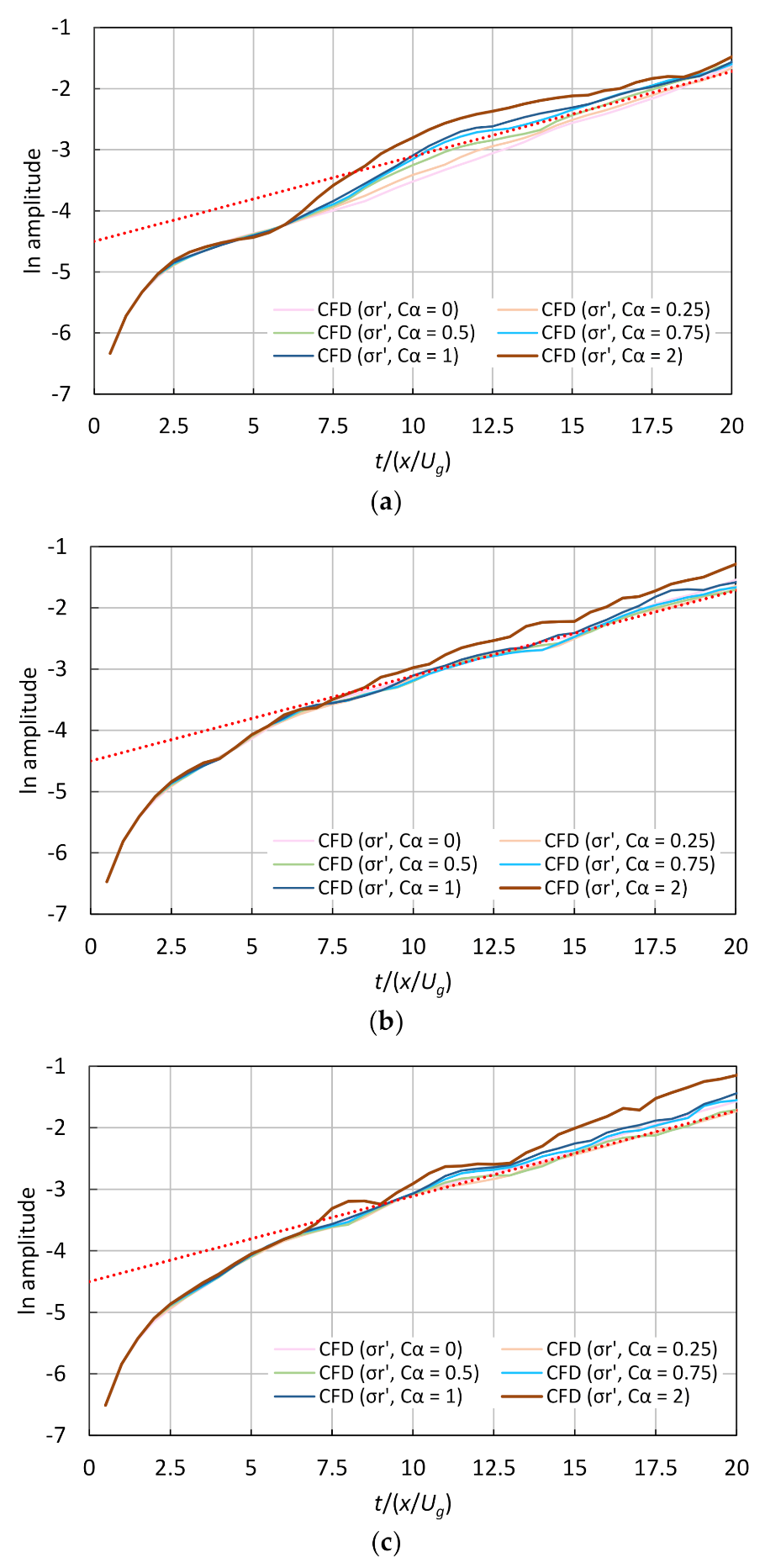
4.3. Effect of the IC Method
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Höhne, T.; Krepper, E.; Rohde, U. Application of CFD codes in nuclear reactor safety analysis. Sci. Technol. Nucl. Install. 2010, 2010, 198758. [Google Scholar] [CrossRef]
- Use of Computational Fluid Dynamics Codes for Safety Analysis of Nuclear Reactor Systems. Available online: https://inis.iaea.org/collection/NCLCollectionStore/_Public/36/103/36103090.pdf?r=1 (accessed on 8 February 2021).
- Mahaffy, J.; Chung, B.; Dubois, F.; Ducros, F.; Graffard, E.; Heitsch, M.; Henriksson, M.; Komen, E.; Moretti, F.; Morii, T.; et al. Best Practice Guidelines for the Use of CFD in Nuclear Reactor Safety Application—Revision; NEA/CSNI/R(2014)11; Organisation for Economic Co-operation and Development (OECD): Paris, France, 2015. [Google Scholar]
- Bestion, D. The difficult challenge of a two-phase CFD modelling for all flow regimes. Nucl. Eng. Des. 2014, 279, 116–125. [Google Scholar] [CrossRef]
- Hirt, C.W.; Nichols, B.D. Volume of fluid (VOF) method for the Dynamics of Free Boundaries. J. Comput. Phys. 1981, 39, 201–225. [Google Scholar] [CrossRef]
- Sussman, M.; Smereka, P.; Osher, S. A Level Set Approach for Computing Solutions to Incompressible Two-Phase Flow. J. Comput. Phys. 1994, 114, 146–159. [Google Scholar] [CrossRef]
- Tryggvason, G.; Bunner, B.; Esmaeeli, A.; Juric, D.; Al-Rawahi, N.; Tauber, W.; Han, J.; Nas, S.; Jan, Y.-J. A Front-Tracking Method for the Computations of Multiphase Flow. J. Comput. Phys. 2001, 169, 708–759. [Google Scholar] [CrossRef]
- Ohta, M.; Sakai, M.; Shimada, N.; Homma, S.; Matsukuma, Y. Numerical Simulation of Multi-Phase Flows; Maruzen Publishing Co., Ltd.: Tokyo, Japan, 2015; pp. 31–63. (In Japanese) [Google Scholar]
- Noh, W.F.; Woodward, P. SLIC (simple line interface calculation). In Proceedings of the 5th International Conference on Numerical Methods in Fluid Dynamics, Enschede, The Netherlands, 28 June–2 July 1976; Springer: Berlin/Heidelberg, Germany, 1976; pp. 330–340. Available online: https://link.springer.com/chapter/10.1007/3-540-08004-X_336 (accessed on 28 January 2021).
- Youngs, D.L. Time-dependent multi-material flow with large fluid distortion. In Numerical Methods for Fluid Dynamics; Morton, K.W., Baines, M.J., Eds.; Academic Press: London, UK, 1982; pp. 273–285. [Google Scholar]
- Sussman, M.; Puckett, E.G. A coupled level set and volume-of-fluid method for computing 3D and axisymmetric incompressible two-phase flows. J. Comput. Phys. 2000, 162, 301–337. [Google Scholar] [CrossRef]
- Yokoi, K. Efficient implementation of THINC scheme: A simple and practical smoothed VOF algorithm. J. Comput. Phys. 2007, 226, 1985–2002. [Google Scholar] [CrossRef]
- Ubbink, O.; Issa, R.I. A method for capturing sharp fluid interfaces on arbitrary meshes. J. Comput. Phys. 1999, 153, 26–50. [Google Scholar] [CrossRef]
- Wacławczyk, T.; Koronowicz, T. Comparison of CICSAM and HRIC high-resolution schemes for interface capturing. J. Theor. Appl. Mech. 2008, 46, 325–345. [Google Scholar]
- Weller, H.G. A New Approach to VOF-Based Interface Capturing Methods for Incompressible, Compressible and Cavitating Flow; OpenCFD Technical Report TR/HGW/07; OpenCFD: Salfords, UK, 2006. [Google Scholar]
- Wardle, K.E.; Weller, H.G. Hybrid multiphase CFD solver for coupled dispersed/segregated flows in liquid-liquid extraction. Int. J. Chem. Eng. 2013, 2013, 128936. [Google Scholar] [CrossRef]
- Deshpande, S.S.; Anumolu, L.; Trujillo, M.F. Evaluating the performance of the two-phase flow solver interFoam. Comput. Sci. Discov. 2012, 5, 014016. [Google Scholar] [CrossRef]
- Deshpande, S.S.; Trujillo, M.F.; Wu, X.; Chahine, G. Computational and experimental characterization of a liquid jet plunging into a quiescent pool at shallow inclination. Int. J. Heat Fluid Flow 2012, 34, 1–14. [Google Scholar] [CrossRef]
- Kawasaki, K.; Matsuura, S.; Sakatani, T. Validation of free surface analysis method in three-dimensional computational fluid dynamics tool “OpenFOAM”. J. Jpn. Soc. Civ. Eng. B3 2013, 69, I_748–I_753. (In Japanese) [Google Scholar]
- Piro, D.J.; Maki, K.J. An Adaptive Interface Compression Method for Water Entry and Exit; Technical Report 2013-350; Department of Naval Architecture and Marine Engineering, University of Michigan: Ann Arbor, MI, USA, 2013; Available online: http://deepblue.lib.umich.edu/handle/2027.42/97021 (accessed on 5 November 2020).
- Lafaurie, B.; Nardone, C.; Scardovelli, R.; Zaleski, S.; Zanetti, G. Modelling merging and fragmentation in multiphase flows with SURFER. J. Comput. Phys. 1994, 113, 134–147. [Google Scholar] [CrossRef]
- Gerlach, D.; Tomar, G.; Biswas, G.; Durst, F. Comparison of volume-of-fluid methods for surface tension-dominant two-phase flows. Int. J. Heat Mass Transf. 2006, 49, 740–754. [Google Scholar] [CrossRef]
- Hoang, D.A.; van Steijn, V.; Portela, L.M.; Kreutzer, M.T.; Kleijn, C.R. Benchmark numerical simulations of segmented two-phase flows in microchannels using the Volume of Fluid method. Comput. Fluids 2013, 86, 28–36. [Google Scholar] [CrossRef]
- Shonibare, O.Y.; Wardle, K.E. Numerical investigation of vertical plunging jet using a hybrid multifluid-VOF multiphase CFD solver. Int. J. Chem. Eng. 2015, 2015, 925639. [Google Scholar] [CrossRef]
- Anez, J.; Demoulin, F.; Hecht, N.; Reveillon, J. A general purpose LES model for atomization. In Proceedings of the 27th European Conference on Liquid Atomization and Spray Systems (ILASS-Europe 2016), Brighton, UK, 4–7 September 2016; pp. 1–7. Available online: https://hal.archives-ouvertes.fr/hal-02128542 (accessed on 5 November 2020).
- Lee, H.; Rhee, S.H. A dynamic interface compression method for VOF simulations of high-speed planing watercraft. J. Mech. Sci. Technol. 2015, 29, 1849–1857. [Google Scholar] [CrossRef]
- Matsumoto, K. Optimization of the Fluid Operating Conditions on the Filling Production Process of Liquid Foods. Ph.D. Thesis, Tokushima University, Tokushima, Japan, 2017. (In Japanese). [Google Scholar]
- Fleau, S.; Mimouni, S.; Mérigoux, N.; Vincent, S. Simulations of two-phase flows with a multifield approach. In Proceedings of the 6th International Symposium on Advances in Computational Heat Transfer (CHT-15), Piscataway, NJ, USA, 25–29 May 2015; pp. 1–17. Available online: https://hal-upec-upem.archives-ouvertes.fr/hal-01172289 (accessed on 5 November 2020).
- Bartosiewicz, Y.; Laviéille, J.; Seynhaeve, J.-M. A first assessment of the NEPTUNE_CFD code: Instabilities in a stratified flow comparison between the VOF method and a two-field approach. Int. J. Heat Fluid Flow 2008, 29, 460–478. [Google Scholar] [CrossRef]
- Štrubelj, L.; Tiselj, I. CFD simulation of Kelvin-Helmholtz instability. In Proceedings of the International Conference Nuclear Energy for New Europe 2005, Bled, Slovenia, 5–8 September 2005; pp. 002.1–002.10. Available online: https://inis.iaea.org/search/search.aspx?orig_q=RN:37104700 (accessed on 5 November 2020).
- Štrubelj, L.; Tiselj, I. Numerical simulations of basic interfacial instabilities with incompressible two-fluid model. Nucl. Eng. Des. 2011, 241, 1018–1023. [Google Scholar] [CrossRef]
- Li, X.; Bhunia, A. Temporal instability of plane gas sheets in a viscous liquid medium. Phys. Fluids 1996, 8, 103–111. [Google Scholar] [CrossRef]
- The OpenFOAM Foundation. OpenFOAM User Guide Version 2.3.0. Available online: https://openfoam.org/version/2-3-0/ (accessed on 8 February 2021).
- Issa, R.I. Solution of the implicitly discretised fluid flow equations by operator-splitting. J. Comput. Phys. 1986, 62, 40–65. [Google Scholar] [CrossRef]
- Caretto, L.S.; Gosman, A.D.; Patankar, S.V.; Spalding, D.B. Two calculation procedures for steady, three-dimensional flows with recirculation. In Proceedings of the Third International Conference on Numerical Methods in Fluid Mechanics, Paris, France, 3–7 July 1972; Springer: Berlin/Heidelberg, Germany, 1972; pp. 60–68. [Google Scholar]
- Rusche, H. Computational Fluid Dynamics of Dispersed Two-Phase Flows at High Phase Fractions. Ph.D. Thesis, Imperial College of Science, Technology and Medicine, London, UK, 2002. [Google Scholar]
- Weller, H.G. Derivation, Modelling and Solution of the Conditionally Averaged Two-Phase Flow Equations; OpenCFD Technical Report TR/HGW/02; OpenCFD: Salfords, UK, 2005. [Google Scholar]
- Giussani, F.; Montorfano, A.; Piscaglia, F.; Onorati, A.; Helie, J.; Aithal, S.M. Dynamic VOF modelling of the internal flow in GDI fuel injectors. Energy Procedia 2016, 101, 574–581. [Google Scholar] [CrossRef]
- Li, X.; Tankin, R.S. On the temporal instability of a two-dimensional viscous liquid sheet. J. Fluid Mech. 1991, 226, 425–443. [Google Scholar] [CrossRef]
- Jun, B.-I.; Norman, M.L.; Stone, J.M. A numerical study of Rayleigh-Taylor instability in magnetic fluids. Astrophys. J. 1995, 453, 332–349. [Google Scholar] [CrossRef]








| The Number of Cells | Cell Spacing | |||
|---|---|---|---|---|
| Total | Direction | Direction | ||
| 5 | 1,200,000 | 3000 | 400 | 0.01 |
| 3.75 | 675,000 | 2250 | 300 | 0.013 |
| 2.5 | 300,000 | 1500 | 200 | 0.02 |
| 2 | 192,000 | 1200 | 160 | 0.025 |
| 1.5 | 108,000 | 900 | 120 | 0.03 |
| 1 | 48,000 | 600 | 80 | 0.05 |
| 0.5 | 12,000 | 300 | 40 | 0.1 |
| 0.35 | 5880 | 210 | 28 | 0.14 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Okagaki, Y.; Yonomoto, T.; Ishigaki, M.; Hirose, Y. Numerical Study on an Interface Compression Method for the Volume of Fluid Approach. Fluids 2021, 6, 80. https://doi.org/10.3390/fluids6020080
Okagaki Y, Yonomoto T, Ishigaki M, Hirose Y. Numerical Study on an Interface Compression Method for the Volume of Fluid Approach. Fluids. 2021; 6(2):80. https://doi.org/10.3390/fluids6020080
Chicago/Turabian StyleOkagaki, Yuria, Taisuke Yonomoto, Masahiro Ishigaki, and Yoshiyasu Hirose. 2021. "Numerical Study on an Interface Compression Method for the Volume of Fluid Approach" Fluids 6, no. 2: 80. https://doi.org/10.3390/fluids6020080
APA StyleOkagaki, Y., Yonomoto, T., Ishigaki, M., & Hirose, Y. (2021). Numerical Study on an Interface Compression Method for the Volume of Fluid Approach. Fluids, 6(2), 80. https://doi.org/10.3390/fluids6020080






