The Transport and Evolution of MHD Turbulence throughout the Heliosphere: Models and Observations
Abstract
1. Introduction
2. Incompressible MHD Turbulence Models
3. 3D Solar Wind–Incompressible MHD Turbulence Model
4. Incompressible MHD Turbulence Model: Proton and Electron Heating
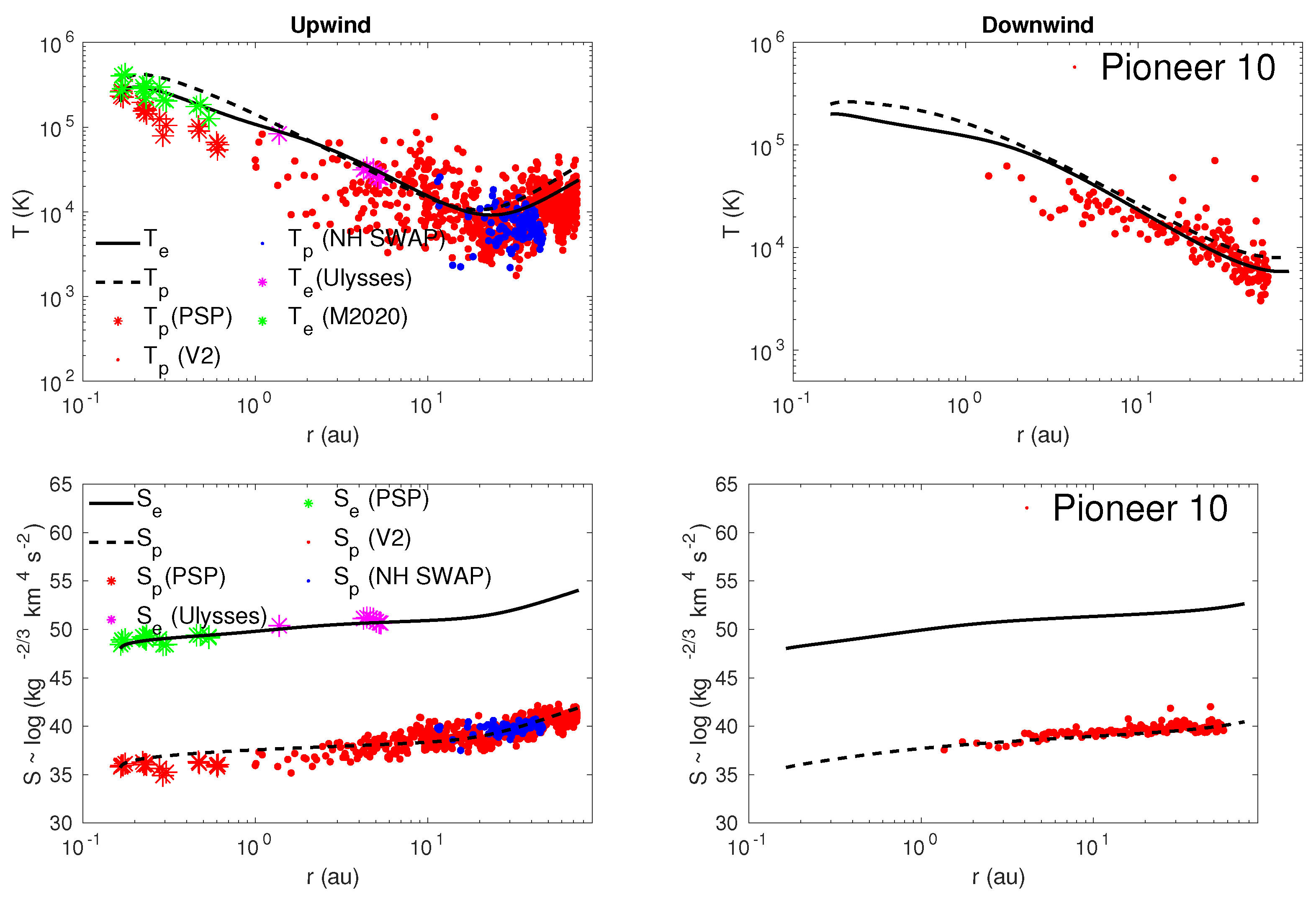
Results: Proton–Electron Heating in the Upwind and Downwind Directions
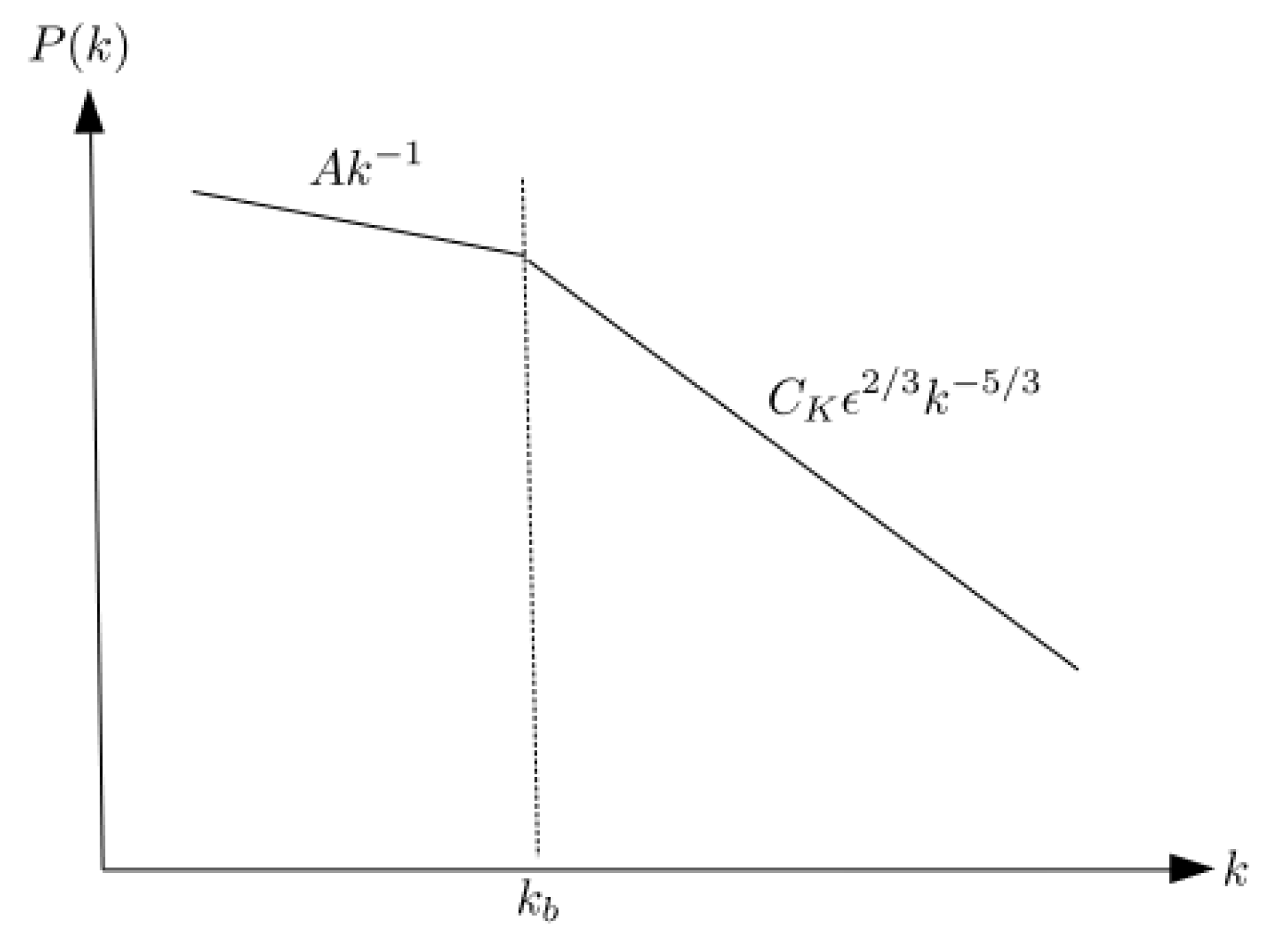
5. Turbulence Cascade in the Inertial Range throughout the Heliosphere
Results: Turbulence Cascade in the Upwind and Downwind Directions
6. Cosmic Ray Diffusion Tensor throughout the Heliosphere
6.1. Cosmic Ray Diffusion Tensor
6.2. CR Parallel Mean Free Path
6.3. CR Perpendicular Mean Free Path
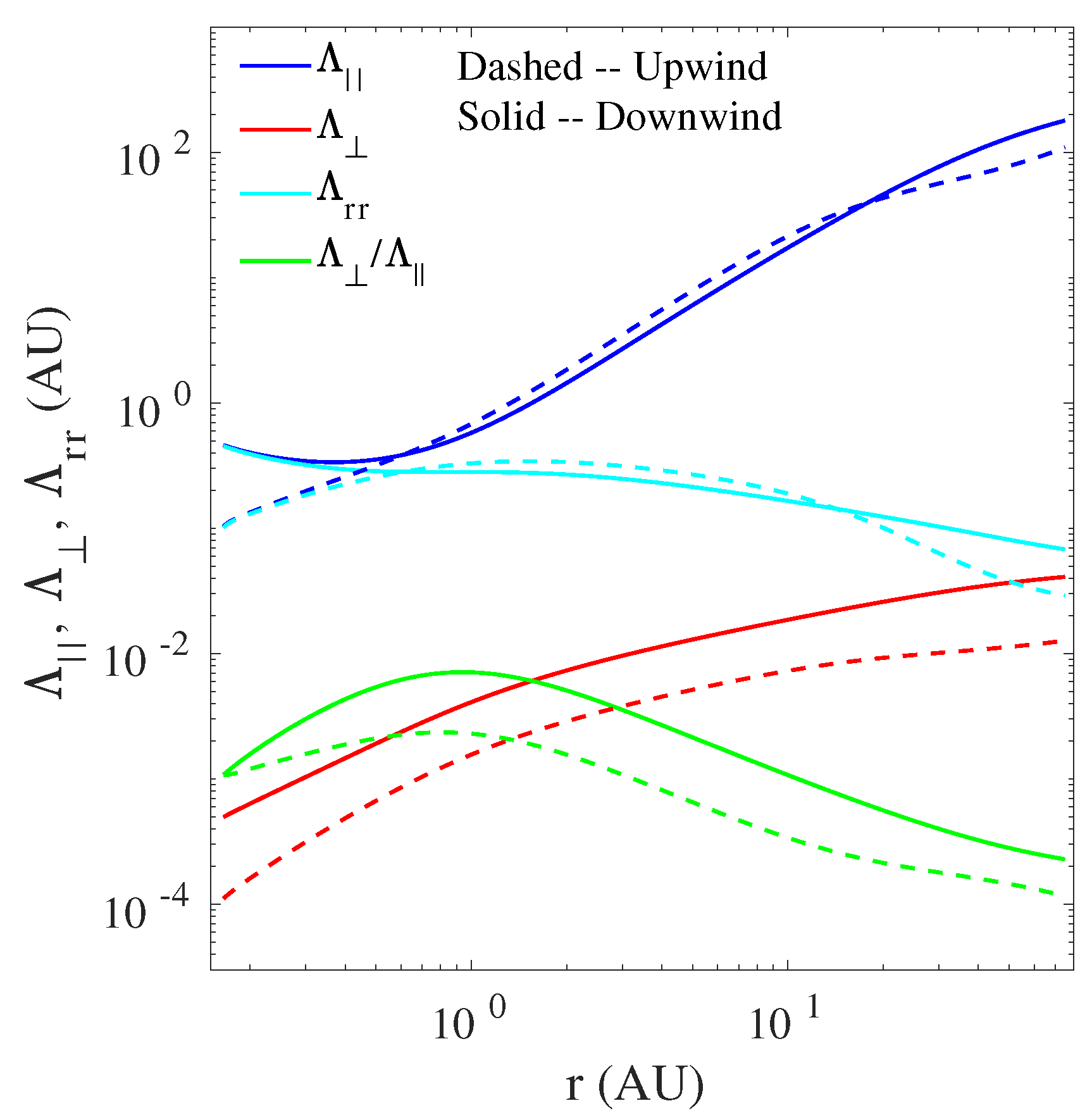
6.4. Results: CR mfp in the Upwind and Downwind Directions
7. Discussion and Conclusions
- Turbulence heats solar wind electrons and protons differently in the upwind and downwind directions. The proton and electron temperatures increase beyond ∼20 au in the upwind direction, but not in the downwind direction. The Coulomb collisions between protons and electrons, and the electron heat flux affect the radial profile of electron and proton temperatures but the effect of Coulomb collision is negligible compared to the turbulent heating term.
- The theoretical and observed electron and proton entropy increase as a function of heliocentric distance. In the upwind direction, the electron and proton entropy increases by about 12.48% and 17.24% from 0.17 au to 75 au, respectively. In the downwind direction from 0.17 to 75 au, the electron entropy increases by about 9.11% and the proton entropy by about 12.5%. The entropy in the upwind direction is higher than that in the downwind direction.
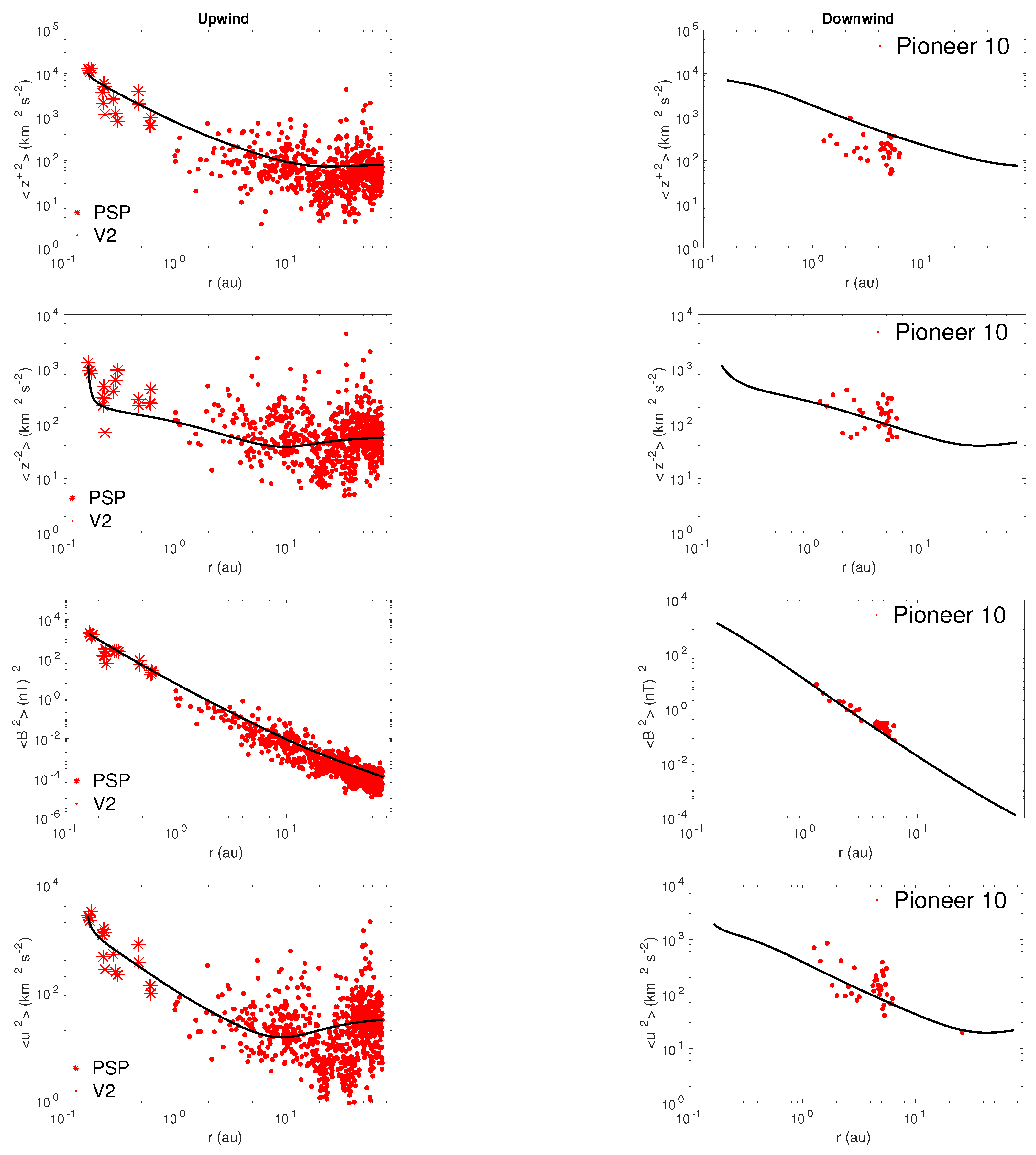
- In the upwind direction, the theoretical and observed energy in forward and backward propagating modes decreases gradually until ∼10–20 au, and then slightly increases as distance increases. In the downwind direction, the theoretical energy in forward and backward propagating modes decreases monotonically until ∼40–50 au, and then slightly increases until 75 au.
- The fluctuating magnetic energy density in the upwind direction decreases as , while that in the downwind direction decreases as . Similarly, in the upwind direction, the fluctuating kinetic energy decreases gradually until ∼10 au, and then increases as distance increases. However, in the downwind direction, the fluctuating kinetic energy decreases monotonically until ∼40 au, and then increases as a function of heliocentric distance.
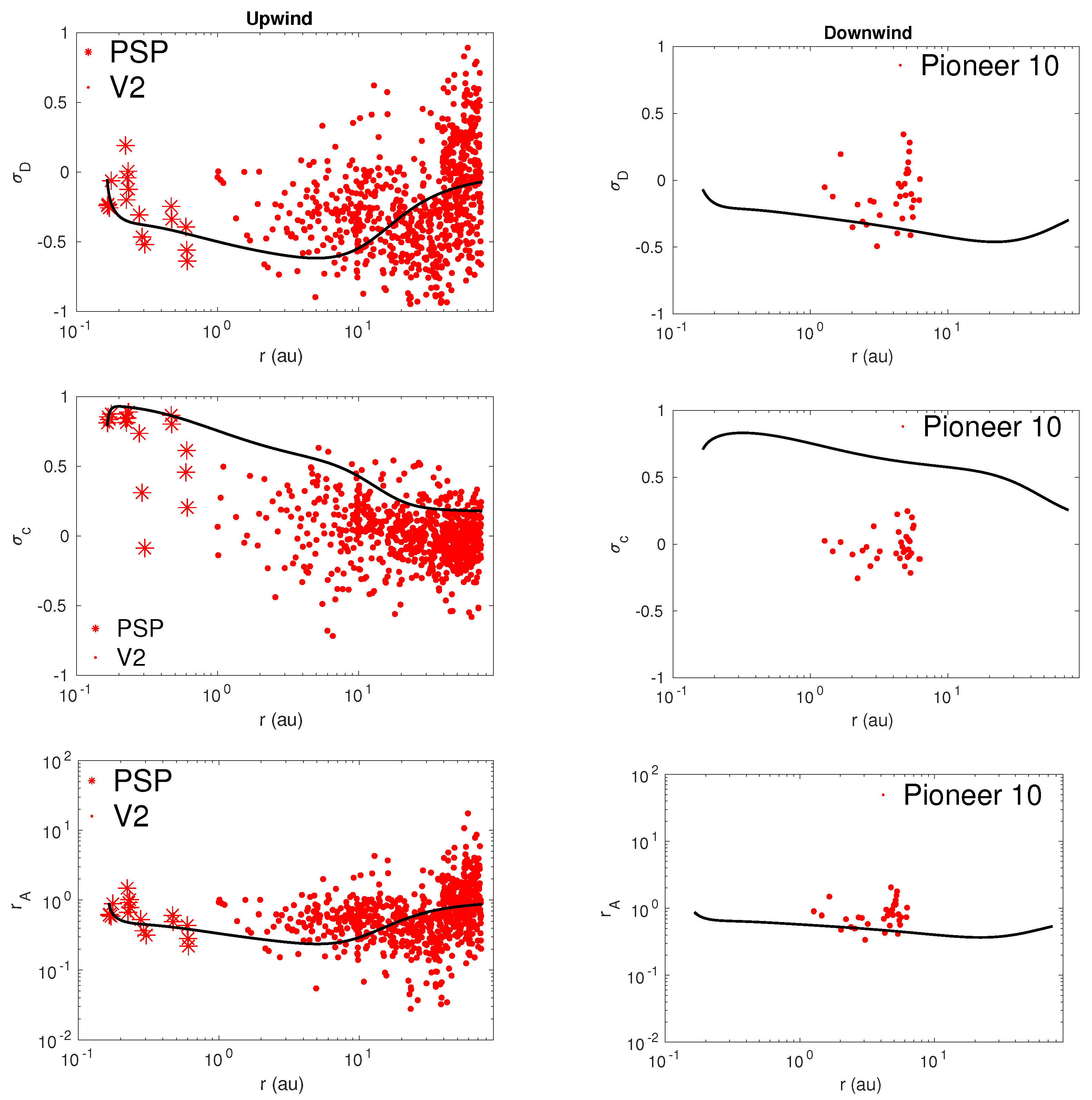
- In the upwind direction, the fluctuating kinetic and magnetic energies tend towards equipartition beyond ∼10 au. In the downwind direction, the normalized residual energy increases after ∼30 au, but the fluctuating magnetic and kinetic energy do not balance. The normalized cross-helicity in the upwind direction is approximately zero beyond ∼20 au, but not in the downwind direction.
- The turbulence cascade rate of energy in forward and backward propagating modes and the fluctuating magnetic energy decrease gradually until ∼10 au and ∼40 au in the upwind and downwind directions, respectively, and then increases slightly with increasing heliocentric distance.
- Over the heliocentric distance ∼0.2–10 au, the downwind turbulence cascade rate is larger than the upwind turbulence cascade rate. However, the turbulence cascade rate in the upwind direction is larger than that in the downwind direction beyond 10 au when pickup ions begin to influence the solar wind.
- The turbulence cascade rate of the normalized residual energy obtained from model 1 is negative throughout the heliosphere in the upwind and downwind directions.
- The turbulence cascade rate of the normalized cross-helicity in the downwind direction is larger than that in the upwind direction.
- The cosmic ray parallel mfp dominates the perpendicular mfp throughout the heliosphere in the upwind and downwind directions. The CR parallel mfp in the upwind direction is larger than that in the downwind direction between ∼0.2–10 au. The CR parallel mfp in the downwind direction is larger than that in the upwind direction when pickup ions begin to influence the solar wind.
- The CR perpendicular mfp increases monotonically as a function of heliocentric distance in the upwind and downwind directions. In the downwind direction, the CR perpendicular mfp is larger than that in the upwind direction from 0.17 au to 75 au.
- In the upwind direction, the CR radial mfp increases initially until ∼1–2 au, and then decreases gradually until ∼10 au. When pickup ions begin to affect the solar wind beyond ∼10 au, the decreases more rapidly. In the downwind direction, remains approximately constant between ∼0.1–10 au, and then decreases with increasing heliocentric distance.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Gazis, P.R.; Barnes, A.; Mihalov, J.D.; Lazarus, A.J. Solar wind velocity and temperature in the outer heliosphere. J. Geophys. Res. 1994, 99, 6561–6573. [Google Scholar] [CrossRef]
- Freeman, J.W. Estimates of solar wind heating inside 0.3 AU. Geophys. Res. Lett. 1988, 15, 88–91. [Google Scholar] [CrossRef]
- Williams, L.L.; Zank, G.P.; Matthaeus, W.H. Dissipation of pickup-induced waves: A solar wind temperature increase in the outer heliosphere? J. Geophys. Res. 1995, 100, 17059–17068. [Google Scholar] [CrossRef]
- Matthaeus, W.H.; Zank, G.P.; Smith, C.W.; Oughton, S. Turbulence, Spatial Transport, and Heating of the Solar Wind. Phys. Rev. Lett. 1999, 82, 3444–3447. [Google Scholar] [CrossRef]
- Smith, C.W.; Matthaeus, W.H.; Zank, G.P.; Ness, N.F.; Oughton, S.; Richardson, J.D. Heating of the low-latitude solar wind by dissipation of turbulent magnetic fluctuations. J. Geophys. Res. 2001, 106, 8253–8272. [Google Scholar] [CrossRef]
- Smith, C.W.; Isenberg, P.A.; Matthaeus, W.H.; Richardson, J.D. Turbulent Heating of the Solar Wind by Newborn Interstellar Pickup Protons. ApJ 2006, 638, 508–517. [Google Scholar] [CrossRef]
- Smith, C.W.; Vasquez, B.J.; Hamilton, K. Interplanetary magnetic fluctuation anisotropy in the inertial range. J. Geophys. Res. Space Phys. 2006, 111, 9111. [Google Scholar] [CrossRef]
- Isenberg, P.A.; Smith, C.W.; Matthaeus, W.H. Turbulent Heating of the Distant Solar Wind by Interstellar Pickup Protons. ApJ 2003, 592, 564–573. [Google Scholar] [CrossRef]
- Isenberg, P.A. Turbulence-driven Solar Wind Heating and Energization of Pickup Protons in the Outer Heliosphere. ApJ 2005, 623, 502–510. [Google Scholar] [CrossRef]
- Breech, B.; Matthaeus, W.H.; Minnie, J.; Bieber, J.W.; Oughton, S.; Smith, C.W.; Isenberg, P.A. Turbulence transport throughout the heliosphere. J. Geophys. Res. Space Phys. 2008, 113, 8105. [Google Scholar] [CrossRef]
- Breech, B.; Matthaeus, W.H.; Cranmer, S.R.; Kasper, J.C.; Oughton, S. Electron and proton heating by solar wind turbulence. J. Geophys. Res. Space Phys. 2009, 114, A09103. [Google Scholar] [CrossRef]
- Isenberg, P.A.; Smith, C.W.; Matthaeus, W.H.; Richardson, J.D. Turbulent Heating of the Distant Solar Wind by Interstellar Pickup Protons in a Decelerating Flow. ApJ 2010, 719, 716–721. [Google Scholar] [CrossRef]
- Ng, C.S.; Bhattacharjee, A.; Munsi, D.; Isenberg, P.A.; Smith, C.W. Kolmogorov versus Iroshnikov-Kraichnan spectra: Consequences for ion heating in the solar wind. J. Geophys. Res. Space Phys. 2010, 115, 2101. [Google Scholar] [CrossRef]
- Usmanov, A.V.; Matthaeus, W.H.; Breech, B.A.; Goldstein, M.L. Solar Wind Modeling with Turbulence Transport and Heating. ApJ 2011, 727, 84. [Google Scholar] [CrossRef]
- Oughton, S.; Matthaeus, W.H.; Smith, C.W.; Breech, B.; Isenberg, P.A. Transport of solar wind fluctuations: A two-component model. J. Geophys. Res. Space Phys. 2011, 116, 8105. [Google Scholar] [CrossRef]
- Adhikari, L.; Zank, G.P.; Hu, Q.; Dosch, A. Turbulence Transport Modeling of the Temporal Outer Heliosphere. ApJ 2014, 793, 52. [Google Scholar] [CrossRef]
- Adhikari, L.; Zank, G.P.; Bruno, R.; Telloni, D.; Hunana, P.; Dosch, A.; Marino, R.; Hu, Q. The Transport of Low-frequency Turbulence in Astrophysical Flows. II. Solutions for the Super-Alfvénic Solar Wind. ApJ 2015, 805, 63. [Google Scholar] [CrossRef]
- Adhikari, L.; Zank, G.P.; Hunana, P.; Shiota, D.; Bruno, R.; Hu, Q.; Telloni, D., II. Transport of Nearly Incompressible Magnetohydrodynamic Turbulence from 1 to 75 au. ApJ 2017, 841, 85. [Google Scholar] [CrossRef]
- Adhikari, L.; Zank, G.P.; Zhao, L.L.; Kasper, J.C.; Korreck, K.E.; Stevens, M.; Case, A.W.; Whittlesey, P.; Larson, D.; Livi, R.; et al. Turbulence Transport Modeling and First Orbit Parker Solar Probe (PSP) Observations. ApJ Suppl. 2020, 246, 38. [Google Scholar] [CrossRef]
- Adhikari, L.; Zank, G.P.; Zhao, L.L.; Webb, G.M. Evolution of Entropy and Mediation of the Solar Wind by Turbulence. ApJ 2020, 891, 34. [Google Scholar] [CrossRef]
- Wiengarten, T.; Fichtner, H.; Kleimann, J.; Kissmann, R. Implementing Turbulence Transport in the CRONOS Framework and Application to the Propagation of CMEs. ApJ 2015, 805, 155. [Google Scholar] [CrossRef]
- Wiengarten, T.; Oughton, S.; Engelbrecht, N.E.; Fichtner, H.; Kleimann, J.; Scherer, K. A Generalized Two-component Model of Solar Wind Turbulence and ab initio Diffusion Mean-Free Paths and Drift Lengthscales of Cosmic Rays. ApJ 2016, 833, 17. [Google Scholar] [CrossRef]
- Zank, G.P.; Adhikari, L.; Zhao, L.L.; Mostafavi, P.; Zirnstein, E.J.; McComas, D.J. The Pickup Ion-mediated Solar Wind. ApJ 2018, 869, 23. [Google Scholar] [CrossRef]
- Li, G.; Zank, G.P.; Rice, W.K.M. Energetic particle acceleration and transport at coronal mass ejection-driven shocks. J. Geophys. Res. Space Phys. 2003, 108, 1082. [Google Scholar] [CrossRef]
- Zank, G.P.; Li, G.; Verkhoglyadova, O. Particle Acceleration at Interplanetary Shocks. Space Sci. Rev. 2007, 130, 255–272. [Google Scholar] [CrossRef]
- Leer, E.; Holzer, T.E.; Fla, T. Acceleration of the solar wind. Space Sci. Rev. 1982, 33, 161–200. [Google Scholar] [CrossRef]
- Matthaeus, W.H.; Zank, G.P.; Oughton, S.; Mullan, D.J.; Dmitruk, P. Coronal Heating by Magnetohydrodynamic Turbulence Driven by Reflected Low-Frequency Waves. ApJ Lett. 1999, 523, L93–L96. [Google Scholar] [CrossRef]
- Oughton, S.; Matthaeus, W.H.; Dmitruk, P.; Milano, L.J.; Zank, G.P.; Mullan, D.J. A Reduced Magnetohydrodynamic Model of Coronal Heating in Open Magnetic Regions Driven by Reflected Low-Frequency Alfvén Waves. ApJ 2001, 551, 565–575. [Google Scholar] [CrossRef]
- Dmitruk, P.; Milano, L.J.; Matthaeus, W.H. Wave-driven Turbulent Coronal Heating in Open Field Line Regions: Nonlinear Phenomenological Model. ApJ 2001, 548, 482–491. [Google Scholar] [CrossRef]
- Dmitruk, P.; Matthaeus, W.H.; Milano, L.J.; Oughton, S.; Zank, G.P.; Mullan, D.J. Coronal Heating Distribution Due to Low-Frequency, Wave-driven Turbulence. ApJ 2002, 575, 571–577. [Google Scholar] [CrossRef]
- Suzuki, T.K.; Inutsuka, S.I. Making the Corona and the Fast Solar Wind: A Self-consistent Simulation for the Low-Frequency Alfvén Waves from the Photosphere to 0.3 AU. ApJ Lett. 2005, 632, L49–L52. [Google Scholar] [CrossRef]
- Suzuki, T.K.; Inutsuka, S.I. Solar winds driven by nonlinear low-frequency Alfvén waves from the photosphere: Parametric study for fast/slow winds and disappearance of solar winds. J. Geophys. Res. Space Phys. 2006, 111, 6101. [Google Scholar] [CrossRef]
- Cranmer, S.R.; van Ballegooijen, A.A.; Edgar, R.J. Self-consistent Coronal Heating and Solar Wind Acceleration from Anisotropic Magnetohydrodynamic Turbulence. ApJ Suppl. 2007, 171, 520–551. [Google Scholar] [CrossRef]
- Verdini, A.; Velli, M.; Matthaeus, W.H.; Oughton, S.; Dmitruk, P. A Turbulence-Driven Model for Heating and Acceleration of the Fast Wind in Coronal Holes. ApJ Lett. 2010, 708, L116–L120. [Google Scholar] [CrossRef]
- van Ballegooijen, A.A.; Asgari-Targhi, M.; Cranmer, S.R.; DeLuca, E.E. Heating of the Solar Chromosphere and Corona by Alfvén Wave Turbulence. ApJ 2011, 736, 3. [Google Scholar] [CrossRef]
- van Ballegooijen, A.A.; Asgari-Targhi, M. Heating and Acceleration of the Fast Solar Wind by Alfvén Wave Turbulence. ApJ 2016, 821, 106. [Google Scholar] [CrossRef]
- Lionello, R.; Velli, M.; Downs, C.; Linker, J.A.; Mikić, Z.; Verdini, A. Validating a Time-dependent Turbulence-driven Model of the Solar Wind. ApJ 2014, 784, 120. [Google Scholar] [CrossRef]
- Asgari-Targhi, M.; van Ballegooijen, A.A.; Cranmer, S.R.; DeLuca, E.E. The Spatial and Temporal Dependence of Coronal Heating by Alfvén Wave Turbulence. ApJ 2013, 773, 111. [Google Scholar] [CrossRef]
- Matsumoto, T.; Suzuki, T.K. Connecting the Sun and the solar wind: The self-consistent transition of heating mechanisms. MNRAS 2014, 440, 971–986. [Google Scholar] [CrossRef]
- Zank, G.P.; Adhikari, L.; Hunana, P.; Tiwari, S.K.; Moore, R.; Shiota, D.; Bruno, R.; Telloni, D. Theory and Transport of Nearly Incompressible Magnetohydrodynamic Turbulence. IV. Solar Coronal Turbulence. ApJ 2018, 854, 32. [Google Scholar] [CrossRef]
- Zank, G.P.; Matthaeus, W.H.; Bieber, J.W.; Moraal, H. The radial and latitudinal dependence of the cosmic ray diffusion tensor in the heliosphere. J. Geophys. Res. 1998, 103, 2085. [Google Scholar] [CrossRef]
- Florinski, V.; Zank, G.P.; Pogorelov, N.V. Galactic cosmic ray transport in the global heliosphere. J. Geophys. Res. Space Phys. 2003, 108, 1228. [Google Scholar] [CrossRef]
- Zhao, L.L.; Adhikari, L.; Zank, G.P.; Hu, Q.; Feng, X.S. Cosmic Ray Diffusion Tensor throughout the Heliosphere Derived from a Nearly Incompressible Magnetohydrodynamic Turbulence Model. ApJ 2017, 849, 88. [Google Scholar] [CrossRef]
- Zhao, L.L.; Adhikari, L.; Zank, G.P.; Hu, Q.; Feng, X.S. Influence of the Solar Cycle on Turbulence Properties and Cosmic-Ray Diffusion. ApJ 2018, 856, 94. [Google Scholar] [CrossRef]
- Chhiber, R.; Subedi, P.; Usmanov, A.V.; Matthaeus, W.H.; Ruffolo, D.; Goldstein, M.L.; Parashar, T.N. Cosmic-Ray Diffusion Coefficients throughout the Inner Heliosphere from a Global Solar Wind Simulation. ApJ Suppl. 2017, 230, 21. [Google Scholar] [CrossRef]
- Adhikari, L.; Zank, G.P.; Zhao, L.L.; Webb, G.M. Evolution of entropy in the outer heliosphere. J. Phys. Conf. Ser. 2020, 1620, 012001. [Google Scholar] [CrossRef]
- Adhikari, L.; Zank, G.P.; Zhao, L.L.; Nakanotani, M.; Tasnim, S. Modeling proton and electron heating in the fast solar wind. Astron. Astrophys. 2021, 650, A16. [Google Scholar] [CrossRef]
- Coleman, P., Jr. J. Turbulence, Viscosity, and Dissipation in the Solar-Wind Plasma. ApJ 1968, 153, 371. [Google Scholar] [CrossRef]
- Belcher, J.W.; Davis, L., Jr.; Smith, E.J. Large-amplitude Alfvén waves in the interplanetary medium: Mariner 5. J. Geophys. Res. 1969, 74, 2302. [Google Scholar] [CrossRef]
- Belcher, J.W.; Davis, L., Jr. Large-amplitude Alfvén waves in the interplanetary medium, 2. J. Geophys. Res. 1971, 76, 3534. [Google Scholar] [CrossRef]
- Bavassano, B.; Dobrowolny, M.; Mariani, F.; Ness, N.F. Radial evolution of power spectra of interplanetary Alfvenic turbulence. J. Geophys. Res. 1982, 87, 3617–3622. [Google Scholar] [CrossRef]
- Bavassano, B.; Pietropaolo, E.; Bruno, R. Cross-helicity and residual energy in solar wind turbulence—Radial evolution and latitudinal dependence in the region from 1 to 5 AU. J. Geophys. Res. 1998, 103, 6521. [Google Scholar] [CrossRef]
- Bavassano, B.; Pietropaolo, E.; Bruno, R. Radial evolution of outward and inward Alfvénic fluctuations in the solar wind: A comparison between equatorial and polar observations by Ulysses. J. Geophys. Res. 2001, 106, 10659–10668. [Google Scholar] [CrossRef]
- Roberts, D.A.; Goldstein, M.L.; Klein, L.W.; Matthaeus, W.H. Origin and evolution of fluctuations in the solar wind—HELIOS observations and Helios-Voyager comparisons. J. Geophys. Res. 1987, 92, 12023–12035. [Google Scholar] [CrossRef]
- Roberts, D.A.; Klein, L.W.; Goldstein, M.L.; Matthaeus, W.H. The nature and evolution of magnetohydrodynamic fluctuations in the solar wind—Voyager observations. J. Geophys. Res. 1987, 92, 11021–11040. [Google Scholar] [CrossRef]
- Goldstein, B.E.; Smith, E.J.; Balogh, A.; Horbury, T.S.; Goldstein, M.L.; Roberts, D.A. Properties of magnetohydrodynamic turbulence in the solar wind as observed by Ulysses at high heliographic latitudes. Geophys. Res. Lett. 1995, 22, 3393–3396. [Google Scholar] [CrossRef]
- Tu, C.Y.; Marsch, E. MHD structures, waves and turbulence in the solar wind: Observations and theories. Space Sci. Rev. 1995, 73, 1–210. [Google Scholar] [CrossRef]
- Kolmogorov, A. The Local Structure of Turbulence in Incompressible Viscous Fluid for Very Large Reynolds’ Numbers. Akad. Nauk SSSR Dokl. 1941, 30, 301–305. [Google Scholar]
- Kraichnan, R.H. Inertial-Range Spectrum of Hydromagnetic Turbulence. Phys. Fluids 1965, 8, 1385–1387. [Google Scholar] [CrossRef]
- Telloni, D.; Carbone, F.; Bruno, R.; Sorriso-Valvo, L.; Zank, G.P.; Adhikari, L.; Hunana, P. No Evidence for Critical Balance in Field-aligned Alfvénic Solar Wind Turbulence. ApJ 2019, 887, 160. [Google Scholar] [CrossRef]
- Zhao, L.L.; Zank, G.P.; Adhikari, L.; Nakanotani, M.; Telloni, D.; Carbone, F. Spectral Features in Field-aligned Solar Wind Turbulence from Parker Solar Probe Observations. ApJ 2020, 898, 113. [Google Scholar] [CrossRef]
- Zank, G.P.; Nakanotani, M.; Zhao, L.L.; Adhikari, L.; Telloni, D. Spectral Anisotropy in 2D plus Slab Magnetohydrodynamic Turbulence in the Solar Wind and Upper Corona. ApJ 2020, 900, 115. [Google Scholar] [CrossRef]
- Tu, C.Y.; Pu, Z.Y.; Wei, F.S. The power spectrum of interplanetary Alfvénic fluctuations: Derivation of the governing equation and its solution. J. Geophys. Res. 1984, 89, 9695–9702. [Google Scholar] [CrossRef]
- Adhikari, L.; Zank, G.P.; Bruno, R.; Telloni, D.; Hunana, P.; Dosch, A.; Marino, R.; Hu, Q. The transport of low-frequency turbulence in the super-Alfvénic solar wind. J. Phys. Conf. Ser. 2015, 642, 012001. [Google Scholar] [CrossRef]
- Parker, E.N. Dynamical Theory of the Solar Wind. Space Sci. Rev. 1965, 4, 666–708. [Google Scholar] [CrossRef]
- Whang, Y.C. Alfvén waves in spiral interplanetary field. J. Geophys. Res. 1973, 78, 7221. [Google Scholar] [CrossRef]
- Hollweg, J.V. Alfvén Waves in a Two-Fluid Model of the Solar Wind. ApJ 1973, 181, 547–566. [Google Scholar] [CrossRef]
- Hollweg, J.V. Alfvén waves in the solar wind: Wave pressure, poynting flux, and angular momentum. J. Geophys. Res. 1973, 78, 3643. [Google Scholar] [CrossRef]
- Barnes, A.; Hollweg, J.V. Large-amplitude hydromagnetic waves. J. Geophys. Res. 1974, 79, 2302. [Google Scholar] [CrossRef]
- Iroshnikov, P.S. Turbulence of a Conducting Fluid in a Strong Magnetic Field. Soviet Ast. 1964, 7, 566. [Google Scholar]
- Goldreich, P.; Sridhar, S. Toward a theory of interstellar turbulence. 2: Strong alfvenic turbulence. ApJ 1995, 438, 763–775. [Google Scholar] [CrossRef]
- Elsässer, W.M. The Hydromagnetic Equations. Phys. Rev. 1950, 79, 183. [Google Scholar] [CrossRef]
- Zhou, Y.; Matthaeus, W.H. Models of inertial range spectra of interplanetary magnetohydrodynamic turbulence. J. Geophys. Res. 1990, 95, 14881–14892. [Google Scholar] [CrossRef]
- Zhou, Y.; Matthaeus, W.H. Remarks on transport theories of interplanetary fluctuations. J. Geophys. Res. 1990, 95, 14863–14871. [Google Scholar] [CrossRef]
- Marsch, E.; Tu, C.Y. Dynamics of correlation functions with Elsasser variables for inhomogeneous MHD turbulence. J. Plasma Phys. 1989, 41, 479–491. [Google Scholar] [CrossRef]
- Zank, G.P.; Matthaeus, W.H.; Smith, C.W. Evolution of turbulent magnetic fluctuation power with heliospheric distance. J. Geophys. Res. 1996, 101, 17093–17108. [Google Scholar] [CrossRef]
- Zank, G.P.; Dosch, A.; Hunana, P.; Florinski, V.; Matthaeus, W.H.; Webb, G.M. The Transport of Low-frequency Turbulence in Astrophysical Flows. I. Governing Equations. ApJ 2012, 745, 35. [Google Scholar] [CrossRef]
- Zank, G.P.; Adhikari, L.; Hunana, P.; Shiota, D.; Bruno, R.; Telloni, D. Theory and Transport of Nearly Incompressible Magnetohydrodynamic Turbulence. ApJ 2017, 835, 147. [Google Scholar] [CrossRef]
- Matthaeus, W.H.; Minnie, J.; Breech, B.; Parhi, S.; Bieber, J.W.; Oughton, S. Transport of cross helicity and radial evolution of Alfvénicity in the solar wind. Geophys. Res. Lett. 2004, 31, 12803. [Google Scholar] [CrossRef]
- Breech, B.; Matthaeus, W.H.; Minnie, J.; Oughton, S.; Parhi, S.; Bieber, J.W.; Bavassano, B. Radial evolution of cross helicity in high-latitude solar wind. Geophys. Res. Lett. 2005, 32, 6103. [Google Scholar] [CrossRef]
- von Karman, T.; Howarth, L. On the Statistical Theory of Isotropic Turbulence. R. Soc. Lond. Proc. Ser. A 1938, 164, 192–215. [Google Scholar] [CrossRef]
- Batchelor, G.K. The Theory of Homogeneous Turbulence; Cambridge University Press: Cambridge, MA, USA, 1953. [Google Scholar]
- Matthaeus, W.H.; Oughton, S.; Pontius, D.H., Jr.; Zhou, Y. Evolution of energy-containing turbulent eddies in the solar wind. J. Geophys. Res. 1994, 99, 19267. [Google Scholar] [CrossRef]
- Matthaeus, W.H.; Zank, G.P.; Oughton, S. Phenomenology of hydromagnetic turbulence in a uniformly expanding medium. J. Plasma Phys. 1996, 56, 659–675. [Google Scholar] [CrossRef]
- Dosch, A.; Adhikari, L.; Zank, G.P. The Transport of Low-Frequency Turbulence in Astrophysical Flows: Correlation Lengths; American Institute of Physics Conference Series; Zank, G.P., Borovsky, J., Bruno, R., Cirtain, J., Cranmer, S., Elliott, H., Giacalone, J., Gonzalez, W., Li, G., Marsch, E., et al., Eds.; American Institute of Physics: College Park, MD, USA, 2013; Volume 1539, pp. 155–158. [Google Scholar] [CrossRef]
- Hossain, M.; Gray, P.C.; Pontius, D.H., Jr.; Matthaeus, W.H.; Oughton, S. Phenomenology for the decay of energy-containing eddies in homogeneous MHD turbulence. Phys. Fluids 1995, 7, 2886–2904. [Google Scholar] [CrossRef]
- Usmanov, A.V.; Goldstein, M.L.; Matthaeus, W.H. Three-dimensional Magnetohydrodynamic Modeling of the Solar Wind Including Pickup Protons and Turbulence Transport. ApJ 2012, 754, 40. [Google Scholar] [CrossRef]
- Usmanov, A.V.; Goldstein, M.L.; Matthaeus, W.H. Three-fluid, Three-dimensional Magnetohydrodynamic Solar Wind Model with Eddy Viscosity and Turbulent Resistivity. ApJ 2014, 788, 43. [Google Scholar] [CrossRef]
- Usmanov, A.V.; Matthaeus, W.H.; Goldstein, M.L.; Chhiber, R. The Steady Global Corona and Solar Wind: A Three-dimensional MHD Simulation with Turbulence Transport and Heating. ApJ 2018, 865, 25. [Google Scholar] [CrossRef]
- Kryukov, I.A.; Pogorelov, N.V.; Zank, G.P.; Borovikov, S.N. Numerical modeling of the solar wind turbulence; American Institute of Physics Conference Series; Heerikhuisen, J., Li, G., Pogorelov, N., Zank, G., Eds.; American Institute of Physics: College Park, MD, USA, 2012; Volume 1436, pp. 48–54. [Google Scholar] [CrossRef]
- Shiota, D.; Zank, G.P.; Adhikari, L.; Hunana, P.; Telloni, D.; Bruno, R. Turbulent Transport in a Three-dimensional Solar Wind. ApJ 2017, 837, 75. [Google Scholar] [CrossRef]
- Chhiber, R.; Usmanov, A.V.; DeForest, C.E.; Matthaeus, W.H.; Parashar, T.N.; Goldstein, M.L. Weakened Magnetization and Onset of Large-scale Turbulence in the Young Solar Wind—Comparisons of Remote Sensing Observations with Simulation. ApJ Lett. 2018, 856, L39. [Google Scholar] [CrossRef]
- Chhiber, R.; Usmanov, A.V.; Matthaeus, W.H.; Parashar, T.N.; Goldstein, M.L. Contextual Predictions for Parker Solar Probe. II. Turbulence Properties and Taylor Hypothesis. ApJ Suppl. 2019, 242, 12. [Google Scholar] [CrossRef]
- Zank, G.P. Interaction of the solar wind with the local interstellar medium: A theoretical perspective. Space Sci. Rev. 1999, 89, 413–688. [Google Scholar] [CrossRef]
- Zank, G.P.; Pauls, H.L. Modelling the Heliosphere. Space Sci. Rev. 1996, 78, 95–106. [Google Scholar] [CrossRef]
- Rice, W.K.M.; Zank, G.P. Shock propagation in the outer heliosphere 3. Pickup ions, MHD, cosmic rays, and energetic particles. J. Geophys. Res. 2000, 105, 5157–5166. [Google Scholar] [CrossRef]
- Shiota, D.; Kataoka, R.; Miyoshi, Y.; Hara, T.; Tao, C.; Masunaga, K.; Futaana, Y.; Terada, N. Inner heliosphere MHD modeling system applicable to space weather forecasting for the other planets. Space Weather 2014, 12, 187–204. [Google Scholar] [CrossRef]
- Wang, Y.M.; Sheeley, N.R.J. Solar Wind Speed and Coronal Flux-Tube Expansion. ApJ 1990, 355, 726. [Google Scholar] [CrossRef]
- Arge, C.N.; Pizzo, V.J. Improvement in the prediction of solar wind conditions using near-real time solar magnetic field updates. J. Geophys. Res. 2000, 105, 10465–10480. [Google Scholar] [CrossRef]
- Leamon, R.J.; Matthaeus, W.H.; Smith, C.W.; Wong, H.K. Contribution of Cyclotron-resonant Damping to Kinetic Dissipation of Interplanetary Turbulence. ApJ Lett. 1998, 507, L181–L184. [Google Scholar] [CrossRef]
- Cranmer, S.R.; Matthaeus, W.H.; Breech, B.A.; Kasper, J.C. Empirical Constraints on Proton and Electron Heating in the Fast Solar Wind. ApJ 2009, 702, 1604–1614. [Google Scholar] [CrossRef][Green Version]
- Engelbrecht, N.E.; Strauss, R.D.T. A Tractable Estimate for the Dissipation Range Onset Wavenumber Throughout the Heliosphere. ApJ 2018, 856, 159. [Google Scholar] [CrossRef]
- Zank, G.P.; Zhao, L.L.; Adhikari, L.; Telloni, D.; Kasper, J.C.; Bale, S.D. Turbulence transport in the solar corona: Theory, modeling, and Parker Solar Probe. Phys. Plasmas 2021, 28, 080501. [Google Scholar] [CrossRef]
- Boldyrev, S.; Forest, C.; Egedal, J. On the temperature of the solar wind. arXiv 2020, arXiv:2001.05125. [Google Scholar]
- Tang, B.; Zank, G.P.; Kolobov, V.I. Numerical Modeling of Suprathermal Electron Transport in the Solar Wind: Effects of Whistler Turbulence. ApJ 2020, 892, 95. [Google Scholar] [CrossRef]
- Weber, E.J.; Davis, L., Jr. The Angular Momentum of the Solar Wind. ApJ 1967, 148, 217–227. [Google Scholar] [CrossRef]
- Spitzer, L. Physics of Fully Ionized Gases. J. Am. Chem. Soc. 1962, 78, 19–5133. [Google Scholar] [CrossRef]
- Isenberg, P.A. Resonant acceleration and heating of solar wind ions: Anisotropy and dispersion. J. Geophys. Res. 1984, 89, 6613–6622. [Google Scholar] [CrossRef]
- Cranmer, S.R.; Field, G.B.; Kohl, J.L. Spectroscopic Constraints on Models of Ion Cyclotron Resonance Heating in the Polar Solar Corona and High-Speed Solar Wind. ApJ 1999, 518, 937–947. [Google Scholar] [CrossRef]
- Salem, C.; Hubert, D.; Lacombe, C.; Bale, S.D.; Mangeney, A.; Larson, D.E.; Lin, R.P. Electron Properties and Coulomb Collisions in the Solar Wind at 1 AU: Wind Observations. ApJ 2003, 585, 1147–1157. [Google Scholar] [CrossRef]
- Braginskii, S.I. Transport Processes in a Plasma. Rev. Plasma Phys. 1965, 1, 205. [Google Scholar]
- Spitzer, L.; Härm, R. Transport Phenomena in a Completely Ionized Gas. Phys. Rev. 1953, 89, 977–981. [Google Scholar] [CrossRef]
- Hollweg, J.V. Collisionless electron heat conduction in the solar wind. J. Geophys. Res. 1976, 81, 1649. [Google Scholar] [CrossRef]
- Scudder, J.D.; Olbert, S. A theory of local and global processes which affect solar wind electrons 1. The origin of typical 1 AU velocity distribution functions-steady state theory. J. Geophys. Res. 1979, 84, 2755–2772. [Google Scholar] [CrossRef]
- Pilipp, W.G.; Muehlhaeuser, K.H.; Miggenrieder, H.; Rosenbauer, H.; Schwenn, R. Large-scale variations of thermal electron parameters in the solar wind between 0.3 and 1 AU. J. Geophys. Res. 1990, 95, 6305–6329. [Google Scholar] [CrossRef]
- Howes, G.G. A prescription for the turbulent heating of astrophysical plasmas. MNRAS 2010, 409, L104–L108. [Google Scholar] [CrossRef]
- Howes, G.G. Prediction of the Proton-to-total Turbulent Heating in the Solar Wind. ApJ 2011, 738, 40. [Google Scholar] [CrossRef][Green Version]
- Nakanotani, M.; Zank, G.P.; Adhikari, L.; Zhao, L.L.; Giacalone, J.; Opher, M.; Richardson, J.D. The Downwind Solar Wind: Model Comparison with Pioneer 10 Observations. ApJ Lett. 2020, 901, L23. [Google Scholar] [CrossRef]
- Moncuquet, M.; Meyer-Vernet, N.; Issautier, K.; Pulupa, M.; Bonnell, J.W.; Bale, S.D.; Dudok de Wit, T.; Goetz, K.; Griton, L.; Harvey, P.R.; et al. First In Situ Measurements of Electron Density and Temperature from Quasi-thermal Noise Spectroscopy with Parker Solar Probe/FIELDS. ApJ Supp. 2020, 246, 44. [Google Scholar] [CrossRef]
- McComas, D.J.; Bame, S.J.; Barraclough, B.L.; Feldman, W.C.; Funsten, H.O.; Gosling, J.T.; Riley, P.; Skoug, R.; Balogh, A.; Forsyth, R.; et al. Ulysses’ return to the slow solar wind. Geophys. Res. Lett. 1998, 25, 1–4. [Google Scholar] [CrossRef]
- Vasquez, B.J.; Smith, C.W.; Hamilton, K.; MacBride, B.T.; Leamon, R.J. Evaluation of the turbulent energy cascade rates from the upper inertial range in the solar wind at 1 AU. J. Geophys. Res. 2007, 112, A07101. [Google Scholar] [CrossRef]
- MacBride, B.T.; Smith, C.W.; Forman, M.A. The Turbulent Cascade at 1 AU: Energy Transfer and the Third-Order Scaling for MHD. ApJ 2008, 679, 1644–1660. [Google Scholar] [CrossRef]
- Smith, C.W.; Stawarz, J.E.; Vasquez, B.J.; Forman, M.A.; MacBride, B.T. Turbulent Cascade at 1 AU in High Cross-Helicity Flows. Phys. Rev. Lett. 2009, 103, 201101. [Google Scholar] [CrossRef] [PubMed]
- Podesta, J.J. On the energy cascade rate of solar wind turbulence in high cross helicity flows. J. Geophys. Res. Space Phys. 2011, 116, A05101. [Google Scholar] [CrossRef]
- Bandyopadhyay, R.; Chasapis, A.; Chhiber, R.; Parashar, T.N.; Maruca, B.A.; Matthaeus, W.H.; Schwartz, S.J.; Eriksson, S.; Le Contel, O.; Breuillard, H.; et al. Solar Wind Turbulence Studies Using MMS Fast Plasma Investigation Data. ApJ 2018, 866, 81. [Google Scholar] [CrossRef]
- Bandyopadhyay, R.; Goldstein, M.L.; Maruca, B.A.; Matthaeus, W.H.; Parashar, T.N.; Ruffolo, D.; Chhiber, R.; Usmanov, A.; Chasapis, A.; Qudsi, R.; et al. Enhanced Energy Transfer Rate in Solar Wind Turbulence Observed near the Sun from Parker Solar Probe. ApJ Suppl. 2020, 246, 48. [Google Scholar] [CrossRef]
- Pine, Z.B.; Smith, C.W.; Hollick, S.J.; Argall, M.R.; Vasquez, B.J.; Isenberg, P.A.; Schwadron, N.A.; Joyce, C.J.; Sokół, J.M.; Bzowski, M.; et al. Turbulent Transport and Heating of the Solar Wind Using Voyager Observations. ApJ 2020, 900, 94. [Google Scholar] [CrossRef]
- Adhikari, L.; Zank, G.P.; Zhao, L.L. A Solar Coronal Hole and Fast Solar Wind Turbulence Model and First-orbit Parker Solar Probe (PSP) Observations. ApJ 2020, 901, 102. [Google Scholar] [CrossRef]
- Chandran, B.D.G.; Quataert, E.; Howes, G.G.; Xia, Q.; Pongkitiwanichakul, P. Constraining Low-Frequency Alfvénic Turbulence in the Solar Wind Using Density-Fluctuation Measurements. ApJ 2009, 707, 1668–1675. [Google Scholar] [CrossRef]
- Cranmer, S.R. Heating Rates for Protons and Electrons in Polar Coronal Holes: Empirical Constraints from the Ultraviolet Coronagraph Spectrometer. ApJ 2020, 900, 105. [Google Scholar] [CrossRef]
- Sasikumar Raja, K.; Subramanian, P.; Ingale, M.; Ramesh, R.; Maksimovic, M. Turbulent Proton Heating Rate in the Solar Wind from 5 to 45 R⊙. ApJ 2021, 914, 137. [Google Scholar] [CrossRef]
- Politano, H.; Pouquet, A. Dynamical length scales for turbulent magnetized flows. Geophys. Res. Lett. 1998, 25, 273–276. [Google Scholar] [CrossRef]
- Politano, H.; Pouquet, A. von Kármán-Howarth equation for magnetohydrodynamics and its consequences on third-order longitudinal structure and correlation functions. PhRvE 1998, 57, R21–R24. [Google Scholar] [CrossRef]
- Hollweg, J.V. Kinetic Alfvén wave revisited. J. Geophys. Res. 1999, 104, 14811–14820. [Google Scholar] [CrossRef]
- Ingale, M. Turbulent density fluctuations in the solar wind. arXiv 2015, arXiv:1509.07652. [Google Scholar]
- Adhikari, L.; Zank, G.P.; Telloni, D.; Hunana, P.; Bruno, R.; Shiota, D. Theory and Transport of Nearly Incompressible Magnetohydrodynamics Turbulence. III. Evolution of Power Anistropy in Magnetic Field Fluctuations throughout the Heliosphere. ApJ 2017, 851, 117. [Google Scholar] [CrossRef]
- Horbury, T.S.; Forman, M.; Oughton, S. Anisotropic Scaling of Magnetohydrodynamic Turbulence. Phys. Rev. Lett. 2008, 101, 175005. [Google Scholar] [CrossRef] [PubMed]
- Podesta, J.J.; Roberts, D.A.; Goldstein, M.L. Spectral Exponents of Kinetic and Magnetic Energy Spectra in Solar Wind Turbulence. ApJ 2007, 664, 543–548. [Google Scholar] [CrossRef]
- Wicks, R.T.; Horbury, T.S.; Chen, C.H.K.; Schekochihin, A.A. Power and spectral index anisotropy of the entire inertial range of turbulence in the fast solar wind. MNRAS 2010, 407, L31–L35. [Google Scholar] [CrossRef]
- Bruno, R.; Telloni, D. Spectral Analysis of Magnetic Fluctuations at Proton Scales from Fast to Slow Solar Wind. ApJ Lett. 2015, 811, L17. [Google Scholar] [CrossRef]
- Chen, C.H.K.; Bale, S.D.; Bonnell, J.W.; Borovikov, D.; Bowen, T.A.; Burgess, D.; Case, A.W.; Chandran, B.D.G.; de Wit, T.D.; Goetz, K.; et al. The Evolution and Role of Solar Wind Turbulence in the Inner Heliosphere. ApJ Supp. 2020, 246, 53. [Google Scholar] [CrossRef]
- Pine, Z.B.; Smith, C.W.; Hollick, S.J.; Argall, M.R.; Vasquez, B.J.; Isenberg, P.A.; Schwadron, N.A.; Joyce, C.J.; Sokół, J.M.; Bzowski, M.; et al. Evidence for Dissipation of Magnetic Fluctuations Using Voyager and ACE Observations. ApJ 2020, 900, 91. [Google Scholar] [CrossRef]
- Pei, C.; Bieber, J.W.; Breech, B.; Burger, R.A.; Clem, J.; Matthaeus, W.H. Cosmic ray diffusion tensor throughout the heliosphere. J. Geophys. Res. Space Phys. 2010, 115, A03103. [Google Scholar] [CrossRef]
- Engelbrecht, N.E.; Burger, R.A. An Ab Initio Model for the Modulation of Galactic Cosmic-ray Electrons. ApJ 2013, 779, 158. [Google Scholar] [CrossRef]
- Engelbrecht, N.E.; Burger, R.A. An Ab Initio Model for Cosmic-ray Modulation. ApJ 2013, 772, 46. [Google Scholar] [CrossRef]
- Engelbrecht, N.E.; Burger, R.A. Sensitivity of Cosmic-Ray Proton Spectra to the Low-wavenumber Behavior of the 2D Turbulence Power Spectrum. ApJ 2015, 814, 152. [Google Scholar] [CrossRef]
- Jokipii, J.R. Cosmic-Ray Propagation. I. Charged Particles in a Random Magnetic Field. ApJ 1966, 146, 480. [Google Scholar] [CrossRef]
- Jokipii, J.R.; Parker, E.N. Random Walk of Magnetic Lines of Force in Astrophysics. Phys. Rev. Lett. 1968, 21, 44–47. [Google Scholar] [CrossRef]
- Forman, M.A.; Jokipii, J.R.; Owens, A.J. Cosmic-Ray Streaming Perpendicular to the Mean Magnetic Field. ApJ 1974, 192, 535–540. [Google Scholar] [CrossRef]
- Shalchi, A.; Bieber, J.W.; Matthaeus, W.H. Analytic Forms of the Perpendicular Diffusion Coefficient in Magnetostatic Turbulence. ApJ 2004, 604, 675–686. [Google Scholar] [CrossRef]
- Shalchi, A.; Schlickeiser, R. Cosmic ray transport in anisotropic magnetohydrodynamic turbulence. III. Mixed magnetosonic and Alfvénic turbulence. Astron. Astrophys. 2004, 420, 799–808. [Google Scholar] [CrossRef]
- Matthaeus, W.H.; Gray, P.C.; Pontius, D.H.J.; Bieber, J.W. Spatial Structure and Field-Line Diffusion in Transverse Magnetic Turbulence. Phys. Rev. Lett. 1995, 75, 2136–2139. [Google Scholar] [CrossRef] [PubMed]
- Gray, P.C.; Pontius, D.H.J.; Matthaeus, W.H. Scaling of field-line random walk in model solar wind fluctuations. Geophys. Res. Lett. 1996, 23, 965–968. [Google Scholar] [CrossRef]
- Shalchi, A.; Bieber, J.W.; Matthaeus, W.H.; Schlickeiser, R. Parallel and Perpendicular Transport of Heliospheric Cosmic Rays in an Improved Dynamical Turbulence Model. ApJ 2006, 642, 230–243. [Google Scholar] [CrossRef]
- Shalchi, A. Analytic forms of the cosmic ray perpendicular diffusion coefficient with implicit contribution of slab modes. Adv. Space Res. 2018, 62, 2817–2827. [Google Scholar] [CrossRef]
- Shalchi, A. Perpendicular Transport of Energetic Particles in Magnetic Turbulence. Space Sci. Rev. 2020, 216, 23. [Google Scholar] [CrossRef]
- Zank, G.P. Transport Processes in Space Physics and Astrophysics; Lecture Notes in Physics; Springer: Berlin, Germany, 2014; Volume 877. [Google Scholar] [CrossRef]
- Matthaeus, W.H.; Qin, G.; Bieber, J.W.; Zank, G.P. Nonlinear Collisionless Perpendicular Diffusion of Charged Particles. ApJ Lett. 2003, 590, L53–L56. [Google Scholar] [CrossRef]
- Shalchi, A.; Bieber, J.W.; Matthaeus, W.H.; Qin, G. Nonlinear Parallel and Perpendicular Diffusion of Charged Cosmic Rays in Weak Turbulence. ApJ 2004, 616, 617–629. [Google Scholar] [CrossRef]
- Shalchi, A. Second-order quasilinear theory of cosmic ray transport. Phys. Plasmas 2005, 12, 052905. [Google Scholar] [CrossRef]
- Shalchi, A. Nonlinear Cosmic Ray Diffusion Theories; Springer: Berlin/Heidelberg, Germany, 2009; Volume 362. [Google Scholar] [CrossRef]
- Jones, F.C.; Jokipii, J.R.; Baring, M.G. Charged-Particle Motion in Electromagnetic Fields Having at Least One Ignorable Spatial Coordinate. ApJ 1998, 509, 238–243. [Google Scholar] [CrossRef]
- Tautz, R.C.; Shalchi, A. Drift Coefficients of Charged Particles in Turbulent Magnetic Fields. ApJ 2012, 744, 125. [Google Scholar] [CrossRef]
- Kubo, R. Statistical-Mechanical Theory of Irreversible Processes. I. J. Phys. Soc. Jpn. 1957, 12, 570–586. [Google Scholar] [CrossRef]
- Shalchi, A. Extended nonlinear guiding center theory of perpendicular diffusion. Astron. Astrophys. 2006, 453, L43–L46. [Google Scholar] [CrossRef]
- Shalchi, A. A Unified Particle Diffusion Theory for Cross-field Scattering: Subdiffusion, Recovery of Diffusion, and Diffusion in Three-dimensional Turbulence. ApJ Lett. 2010, 720, L127–L130. [Google Scholar] [CrossRef]
- Shalchi, A. Simple Analytical Forms of the Perpendicular Diffusion Coefficient for Two-component Turbulence. I. Magnetostatic Turbulence. ApJ 2013, 774, 7. [Google Scholar] [CrossRef][Green Version]
- Shalchi, A. Simple Analytical Forms of the Perpendicular Diffusion Coefficient for Two-component Turbulence. II. Dynamical Turbulence with Constant Correlation Time. ApJ 2014, 780, 138. [Google Scholar] [CrossRef][Green Version]
- Zank, G.P.; Matthaeus, W.H. Waves and turbulence in the solar wind. J. Geophys. Res. 1992, 97, 17189. [Google Scholar] [CrossRef]
- Bieber, J.W.; Wanner, W.; Matthaeus, W.H. Dominant two-dimensional solar wind turbulence with implications for cosmic ray transport. J. Geophys. Res. 1996, 101, 2511–2522. [Google Scholar] [CrossRef]
- Völk, H.J.; Morfill, G.; Alpers, W.; Lee, M.A. Spatial Dependence of the Pitch-Angle and Associated Spatial Diffusion Coefficients for Cosmic Rays in Interplanetary Space. Ap. & SS. 1974, 26, 403–430. [Google Scholar] [CrossRef]
- Morfill, G.E.; Völk, H.J. Latitude variation of interplanetary cosmic ray diffusion. J. Geophys. Res. 1979, 84, 4446–4450. [Google Scholar] [CrossRef]
- Zhang, M. A Markov Stochastic Process Theory of Cosmic-Ray Modulation. ApJ 1999, 513, 409–420. [Google Scholar] [CrossRef]
- Potgieter, M.S. Solar Modulation of Cosmic Rays. Living Rev. Sol. Phys. 2013, 10, 3. [Google Scholar] [CrossRef]
- Engelbrecht, N.E.; Moloto, K.D. An Ab Initio Approach to Antiproton Modulation in the Inner Heliosphere. ApJ 2021, 908, 167. [Google Scholar] [CrossRef]
- Candia, J.; Roulet, E. Diffusion and drift of cosmic rays in highly turbulent magnetic fields. J. Cosmol. Astropart. Phys. 2004, 2004, 007. [Google Scholar] [CrossRef]
- Burger, R.A.; Krüger, T.P.J.; Hitge, M.; Engelbrecht, N.E. A Fisk-Parker Hybrid Heliospheric Magnetic Field with a Solar-Cycle Dependence. ApJ 2008, 674, 511–519. [Google Scholar] [CrossRef]
- Jokipii, J.R.; Parker, E.N. on the Convection, Diffusion, and Adiabatic Deceleration of Cosmic Rays in the Solar Wind. ApJ 1970, 160, 735. [Google Scholar] [CrossRef]
- Engelbrecht, N.E.; Strauss, R.D.; le Roux, J.A.; Burger, R.A. Toward a Greater Understanding of the Reduction of Drift Coefficients in the Presence of Turbulence. ApJ 2017, 841, 107. [Google Scholar] [CrossRef]
- Zhao, L.L.; Qin, G.; Zhang, M.; Heber, B. Modulation of galactic cosmic rays during the unusual solar minimum between cycles 23 and 24. J. Geophys. Res. (Space Physics) 2014, 119, 1493–1506. [Google Scholar] [CrossRef]
- Moloto, K.D.; Engelbrecht, N.E. A Fully Time-dependent Ab Initio Cosmic-Ray Modulation Model Applied to Historical Cosmic-Ray Modulation. ApJ 2020, 894, 121. [Google Scholar] [CrossRef]
- Moloto, K.D.; Engelbrecht, N.E.; Burger, R.A. A Simplified Ab Initio Cosmic-ray Modulation Model with Simulated Time Dependence and Predictive Capability. ApJ 2018, 859, 107. [Google Scholar] [CrossRef]
- Moloto, K.D.; Engelbrecht, N.E.; Strauss, R.D.; Moeketsi, D.M.; van den Berg, J.P. Numerical integration of stochastic differential equations: A parallel cosmic ray modulation implementation on Africa’s fastest computer. Adv. Space Res. 2019, 63, 626–639. [Google Scholar] [CrossRef]
- Bieber, J.W.; Burger, R.A.; Matthaeus, W.H. The Diffusion Tensor throughout the Heliosphere. Int. Cosmic Ray Confer. 1995, 4, 694. [Google Scholar]
- Bieber, J.W.; Matthaeus, W.H.; Smith, C.W.; Wanner, W.; Kallenrode, M.B.; Wibberenz, G. Proton and Electron Mean Free Paths: The Palmer Consensus Revisited. ApJ 1994, 420, 294. [Google Scholar] [CrossRef]
- Palmer, I.D. Transport coefficients of low-energy cosmic rays in interplanetary space. Rev. Geophys. Space Phys. 1982, 20, 335–351. [Google Scholar] [CrossRef]
- Zank, G.P.; Matthaeus, W.H. Nearly incompressible fluids. II—Magnetohydrodynamics, turbulence, and waves. Phys. Fluids 1993, 5, 257–273. [Google Scholar] [CrossRef]
- Axford, W.I. The modulation of galactic cosmic rays in the interplanetary medium. Planet. Space Sci. 1965, 13, 115–130. [Google Scholar] [CrossRef]
- Gleeson, L.J.; Axford, W.I. Cosmic Rays in the Interplanetary Medium. ApJ Lett. 1967, 149, L115. [Google Scholar] [CrossRef]
- Jones, F.C. The Generalized Diffusion-Convection Equation. ApJ 1990, 361, 162. [Google Scholar] [CrossRef]
- Bieber, J.W.; Matthaeus, W.H. Perpendicular Diffusion and Drift at Intermediate Cosmic-Ray Energies. ApJ 1997, 485, 655–659. [Google Scholar] [CrossRef]
- Zank, G.P.; Li, G.; Florinski, V.; Matthaeus, W.H.; Webb, G.M.; Le Roux, J.A. Perpendicular diffusion coefficient for charged particles of arbitrary energy. J. Geophys. Res. Space Phys. 2004, 109, A04107. [Google Scholar] [CrossRef]
- Engelbrecht, N.E. The Implications of Simple Estimates of the 2D Outerscale Based on Measurements of Magnetic Islands for the Modulation of Galactic Cosmic-Ray Electrons. ApJ 2019, 872, 124. [Google Scholar] [CrossRef]
- Engelbrecht, N.E. On the Pitch-angle-dependent Perpendicular Diffusion Coefficients of Solar Energetic Protons in the Inner Heliosphere. ApJ 2019, 880, 60. [Google Scholar] [CrossRef]
- Khabarova, O.; Zank, G.P.; Li, G.; le Roux, J.A.; Webb, G.M.; Dosch, A.; Malandraki, O.E. Small-scale Magnetic Islands in the Solar Wind and Their Role in Particle Acceleration. I. Dynamics of Magnetic Islands Near the Heliospheric Current Sheet. ApJ 2015, 808, 181. [Google Scholar] [CrossRef]
- Cartwright, M.L.; Moldwin, M.B. Heliospheric evolution of solar wind small-scale magnetic flux ropes. J. Geophys. Res. Space Phys. 2010, 115, A08102. [Google Scholar] [CrossRef]
- Ruffolo, D.; Pianpanit, T.; Matthaeus, W.H.; Chuychai, P. Random Ballistic Interpretation of Nonlinear Guiding Center Theory. ApJ Lett. 2012, 747, L34. [Google Scholar] [CrossRef]
- Zank, G.P.; Hunana, P.; Mostafavi, P.; Le Roux, J.A.; Li, G.; Webb, G.M.; Khabarova, O.; Cummings, A.; Stone, E.; Decker, R. Diffusive Shock Acceleration and Reconnection Acceleration Processes. ApJ 2015, 814, 137. [Google Scholar] [CrossRef]


| Parameters | Upwind | Downwind |
|---|---|---|
| kms | kms | |
| 1190 kms | 1190 kms | |
| kms | −281.2 kms | |
| kms | kms | |
| kms | kms | |
| kms | − kms | |
| kgms | kgms | |
| kgms | kgms |
| Parameters | Upwind | Downwind |
|---|---|---|
| 0.1 | 0.3 | |
| 0.1 | 0.3 | |
| 0.01 | 0.9 | |
| U | 400 km s | 400 kms |
| 200 km s | 200 kms | |
| 90 km s | 90 kms | |
| 300 cm | 300 cm | |
| 0.1 | 0.1 | |
| 0.1 | 0.02 | |
| 0.1 cm | 0.1 cm | |
| 5 cm | 5 cm | |
| s | s | |
| L | 8 au | 2 au |
| 0.17 au | 0.17 au |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Adhikari, L.; Zank, G.P.; Zhao, L. The Transport and Evolution of MHD Turbulence throughout the Heliosphere: Models and Observations. Fluids 2021, 6, 368. https://doi.org/10.3390/fluids6100368
Adhikari L, Zank GP, Zhao L. The Transport and Evolution of MHD Turbulence throughout the Heliosphere: Models and Observations. Fluids. 2021; 6(10):368. https://doi.org/10.3390/fluids6100368
Chicago/Turabian StyleAdhikari, Laxman, Gary P. Zank, and Lingling Zhao. 2021. "The Transport and Evolution of MHD Turbulence throughout the Heliosphere: Models and Observations" Fluids 6, no. 10: 368. https://doi.org/10.3390/fluids6100368
APA StyleAdhikari, L., Zank, G. P., & Zhao, L. (2021). The Transport and Evolution of MHD Turbulence throughout the Heliosphere: Models and Observations. Fluids, 6(10), 368. https://doi.org/10.3390/fluids6100368







