The Influence of Different Unsteady Incident Flow Environments on Drag Measurements in an Open Jet Wind Tunnel
Abstract
1. Introduction
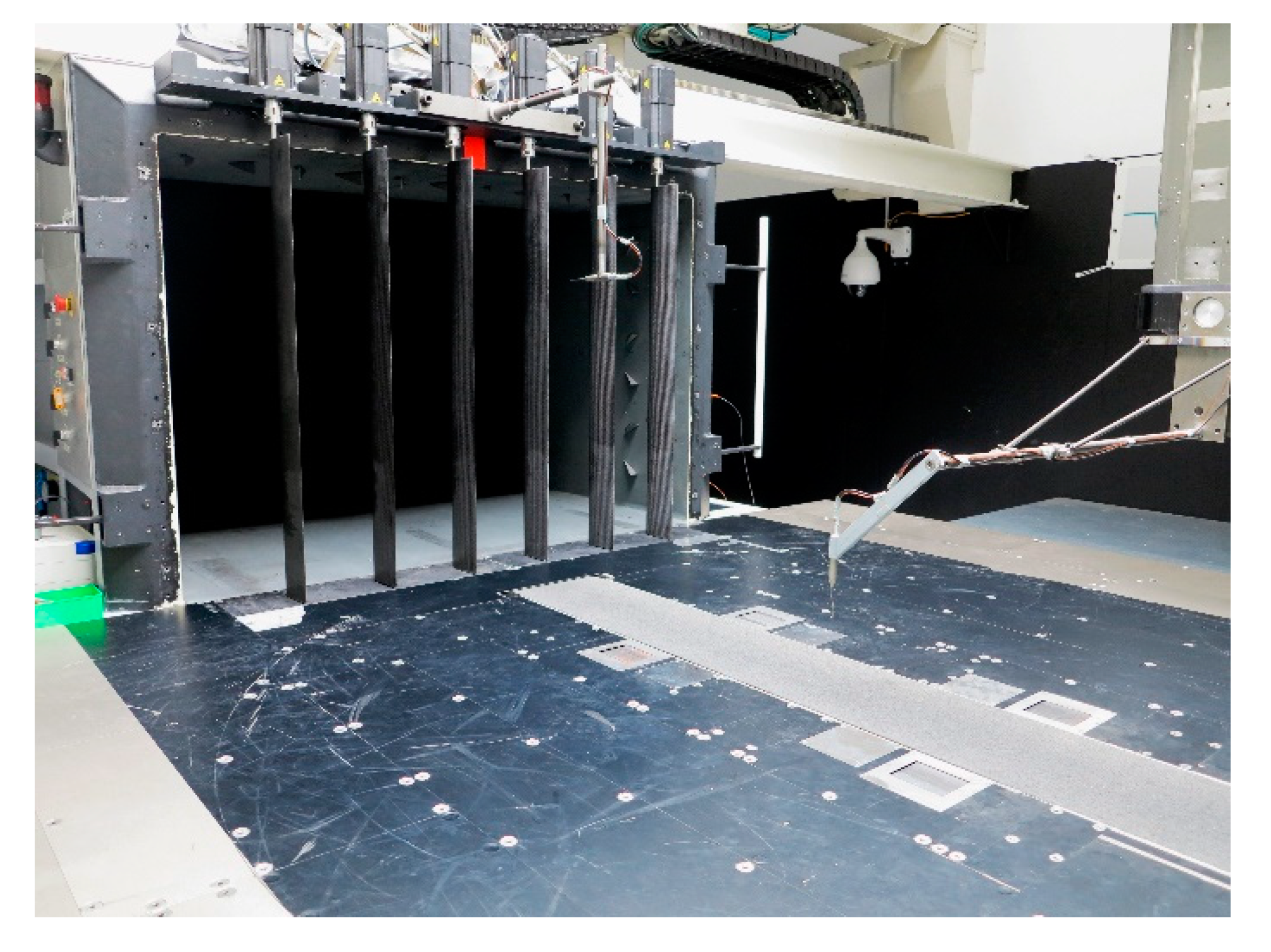
2. Experimental Setup
2.1. Unsteady Incident Flow with the FKFS swing® System
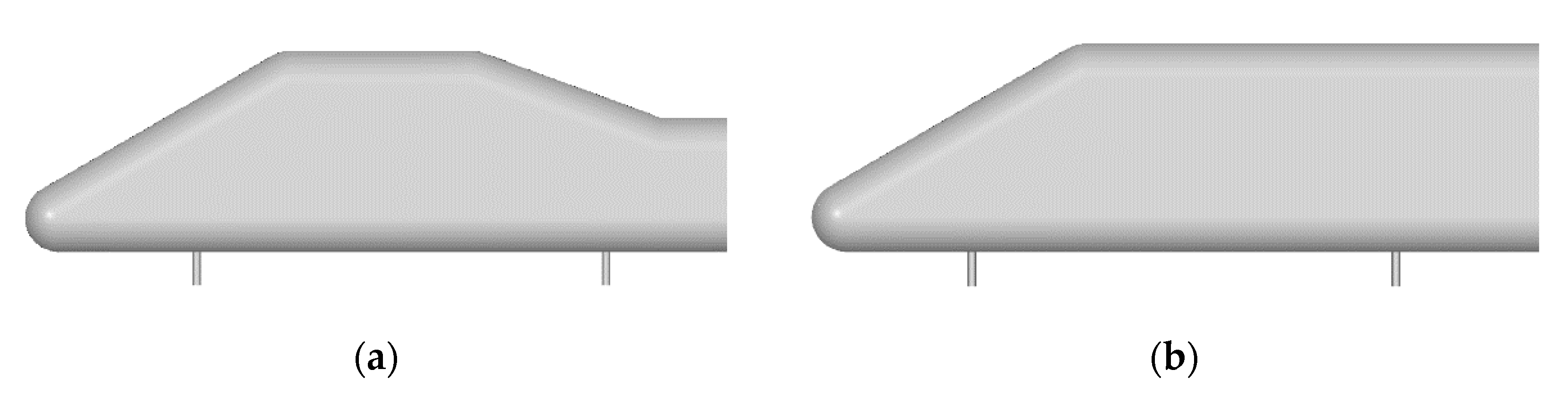
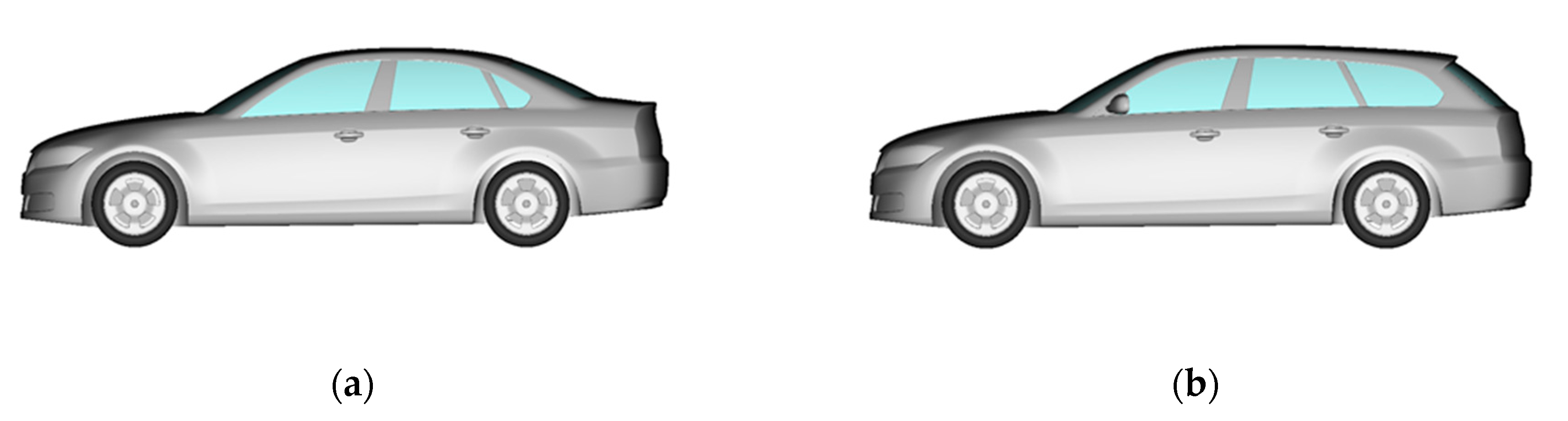
2.2. Test Vehicles
3. Evaluation Methods for Pressure and Drag Measurements
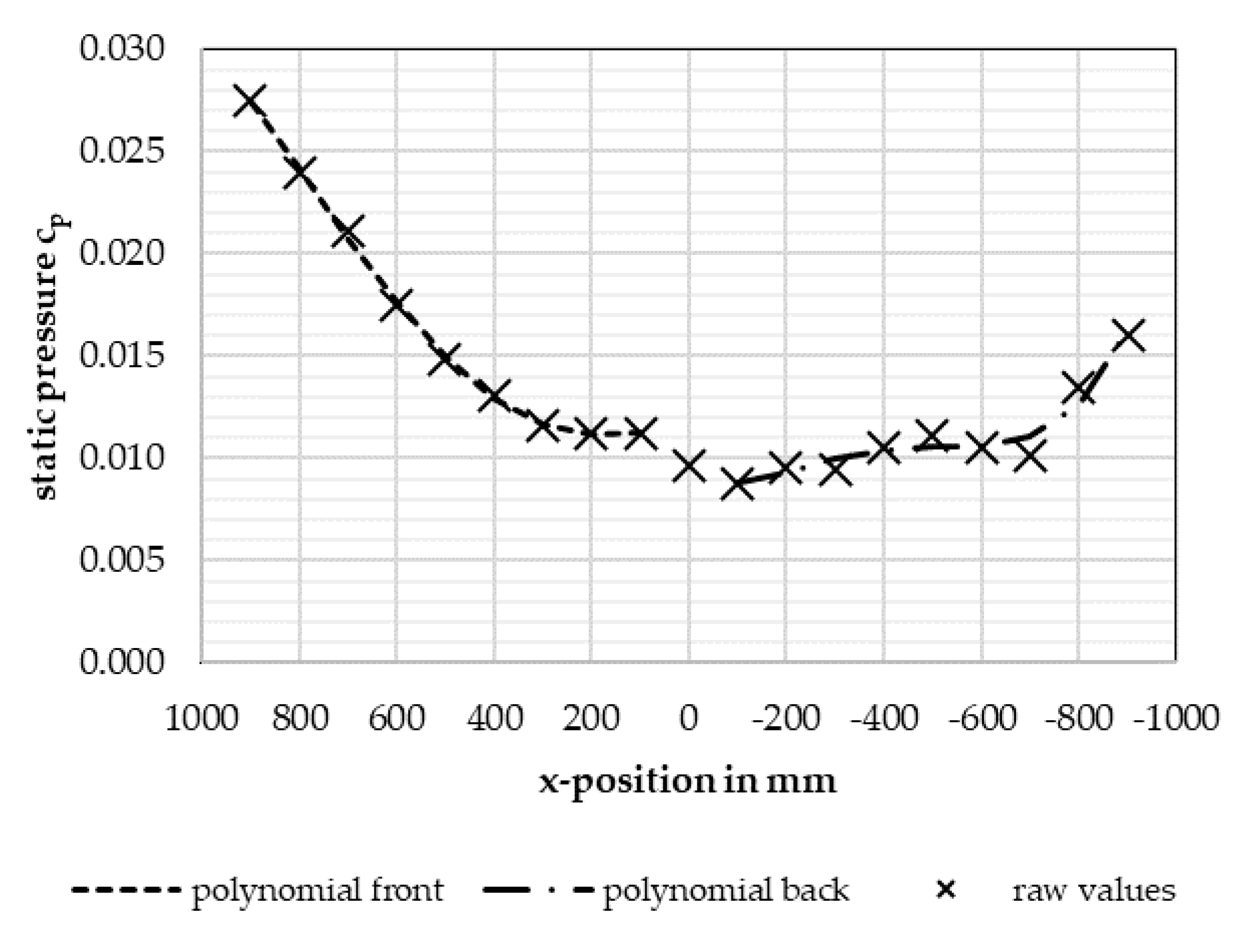
3.1. The Static Pressure Gradient
3.2. Unsteady and Quasi-Steady Aerodynamic Drag
3.3. Correction
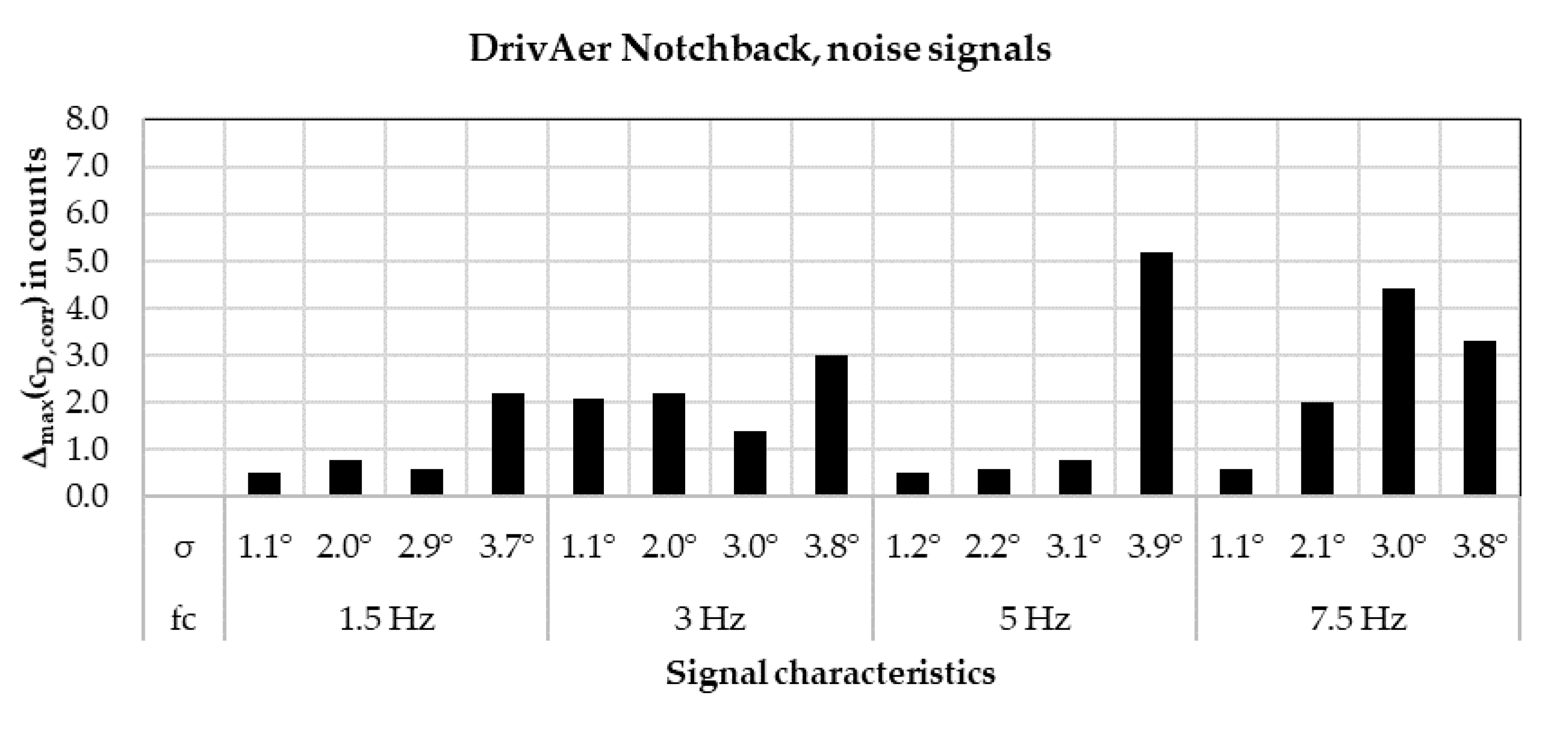
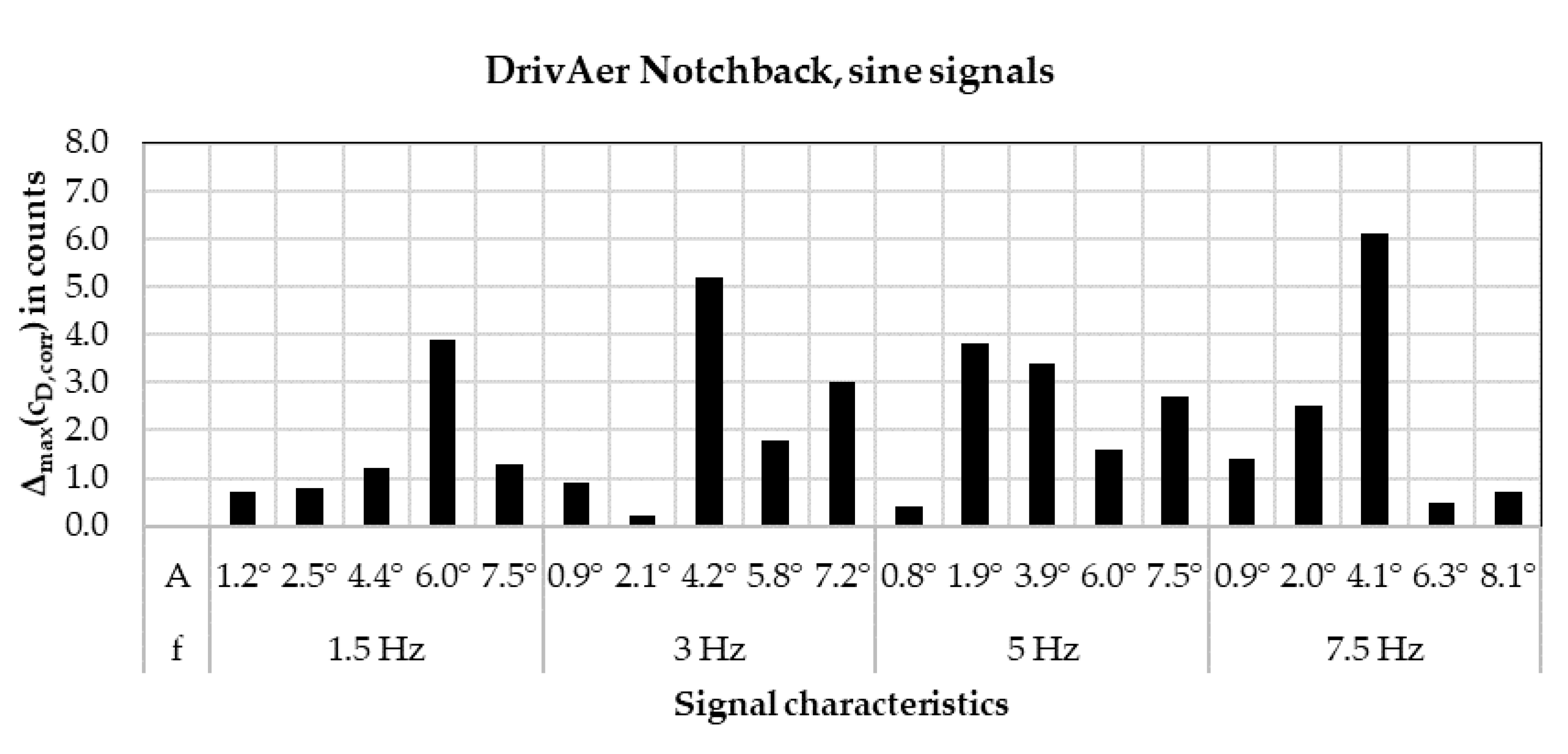
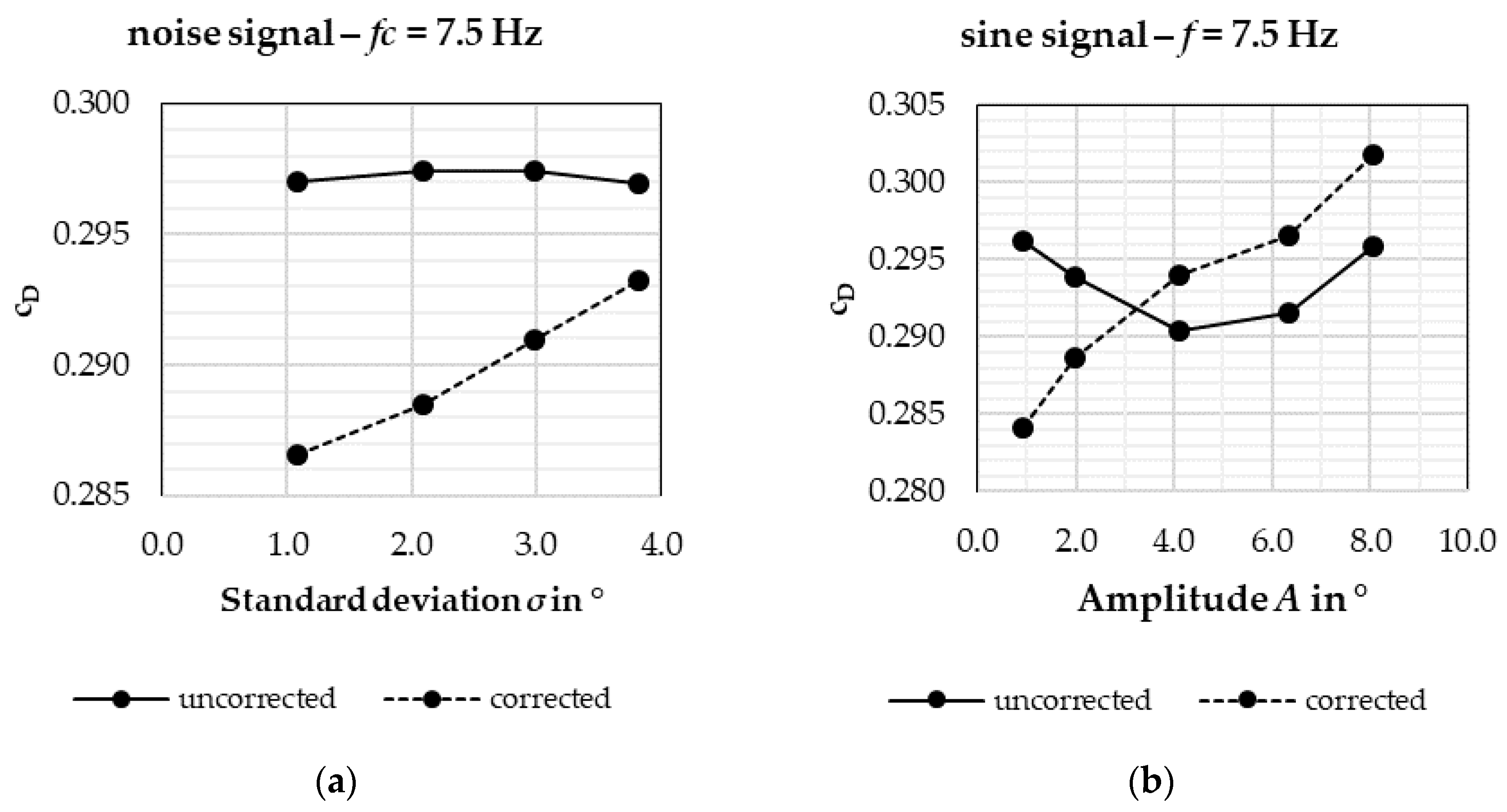
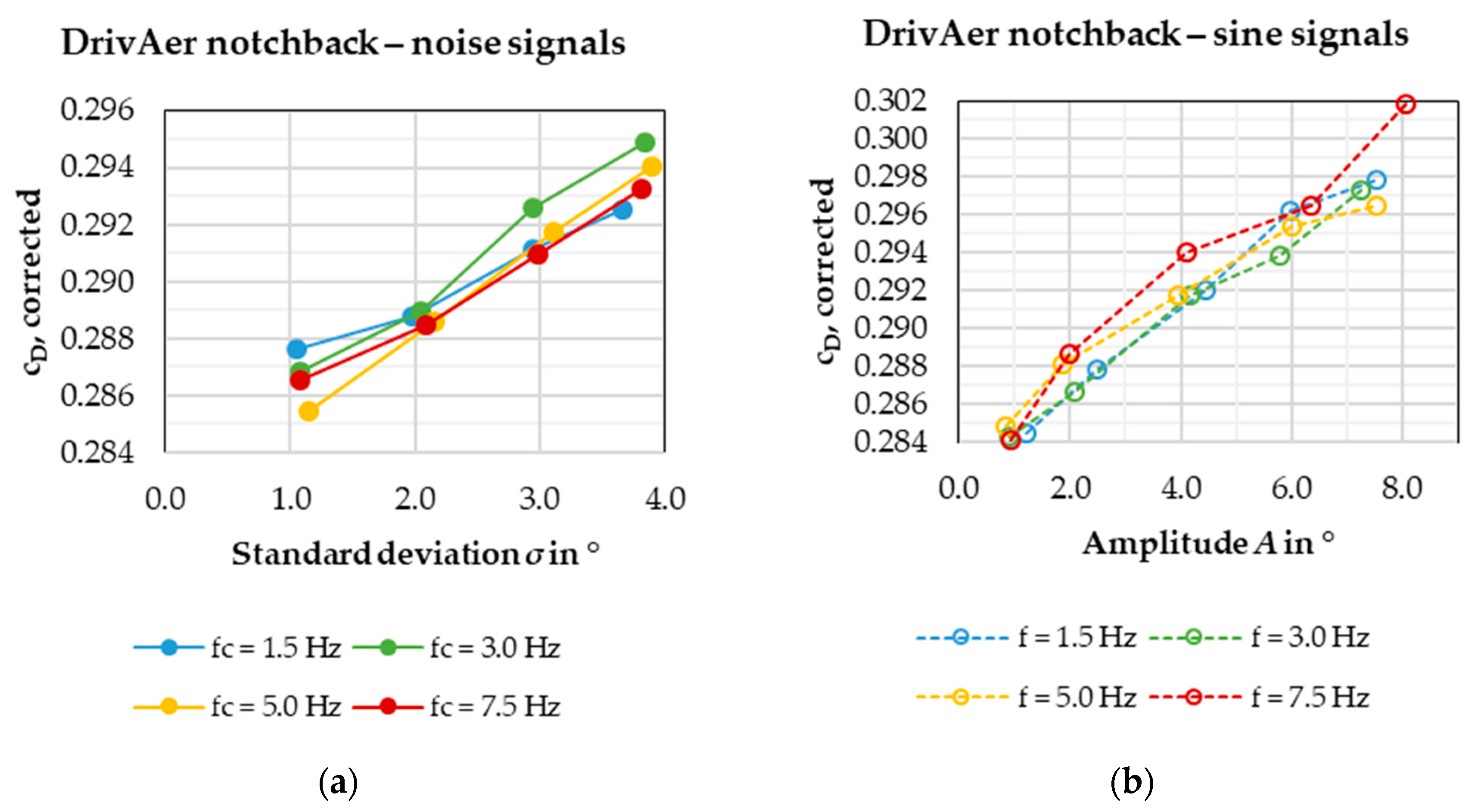
4. Experimental Results
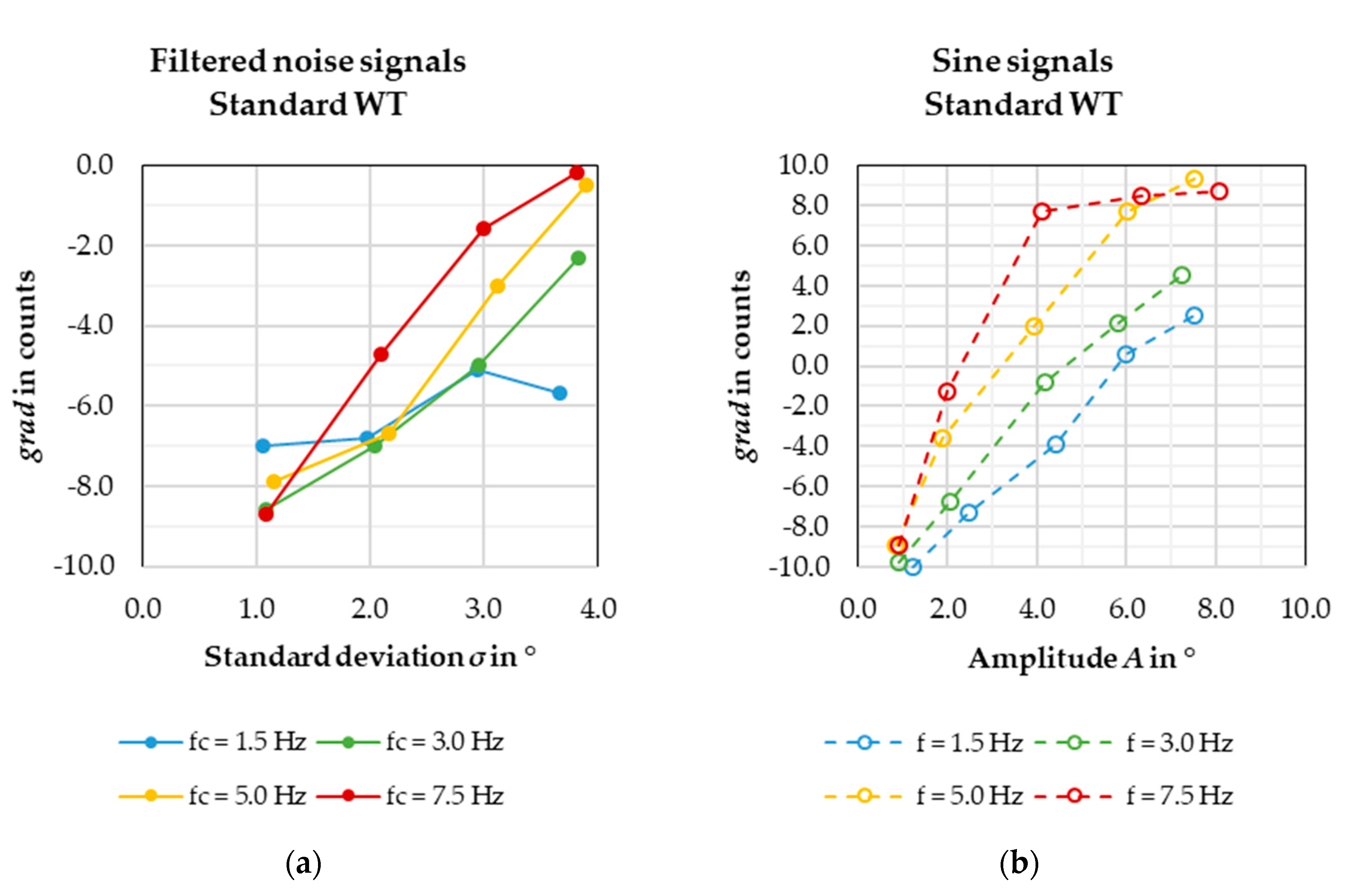
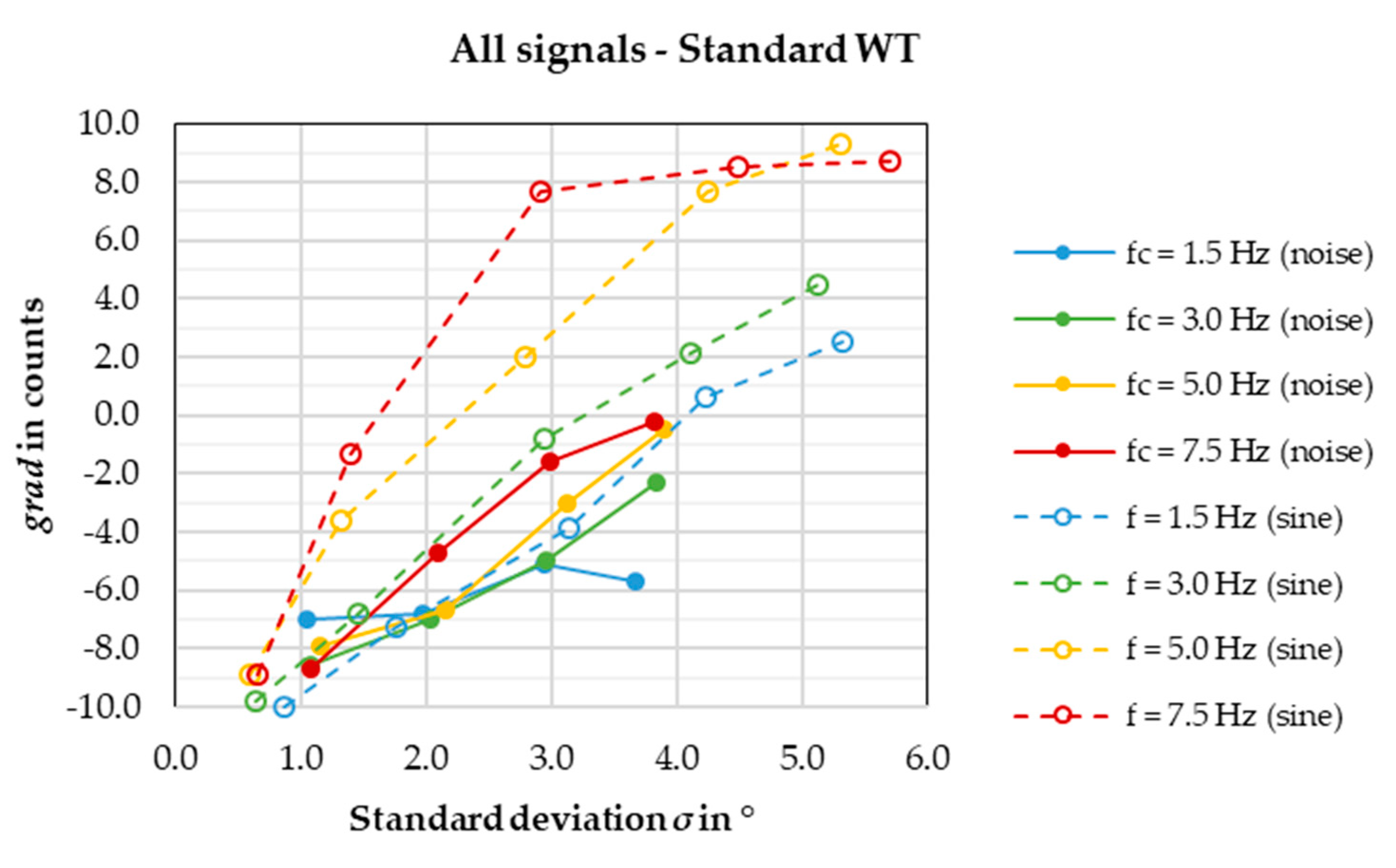
4.1. Empty Test Section Results
4.2. Correction Results
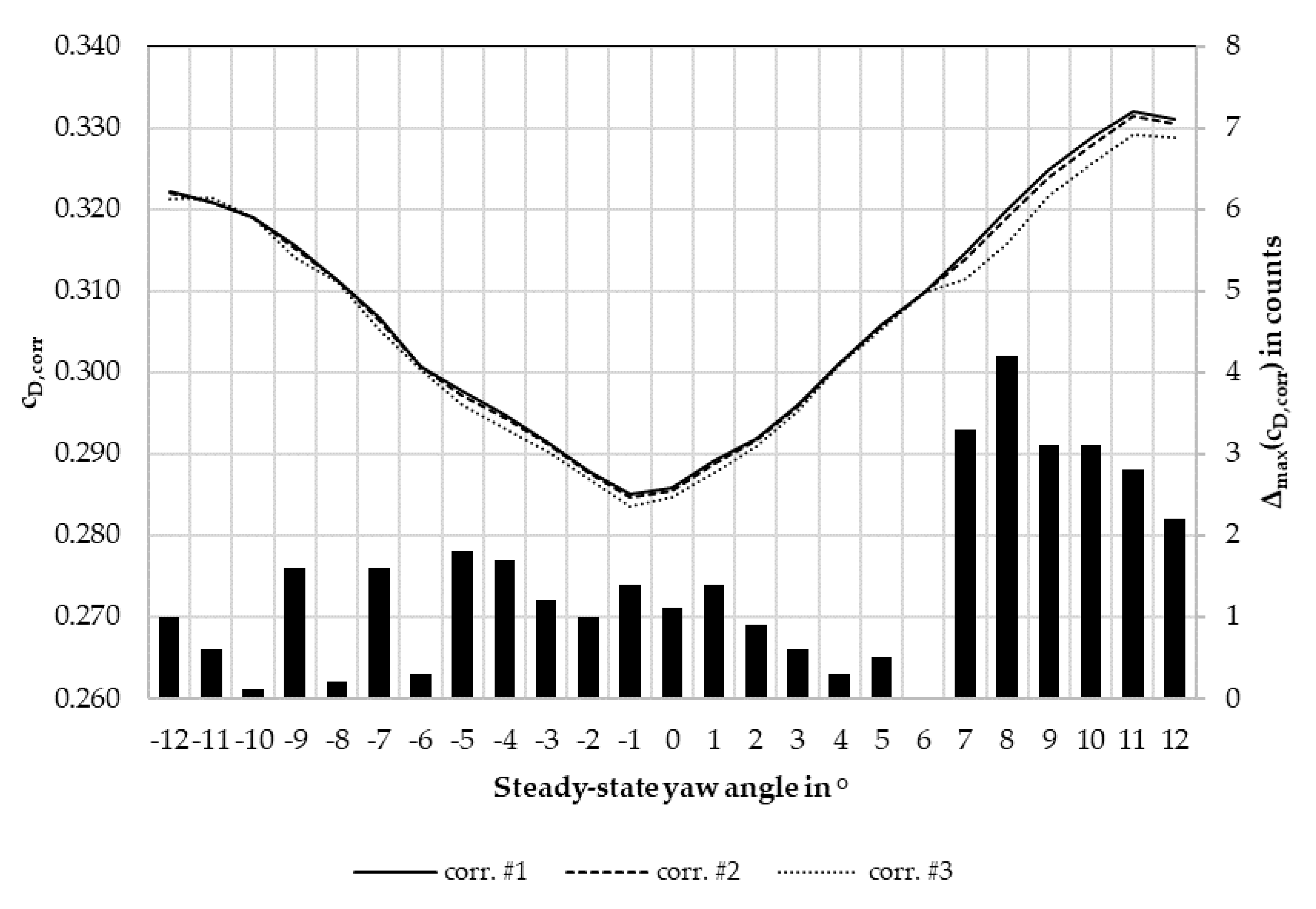
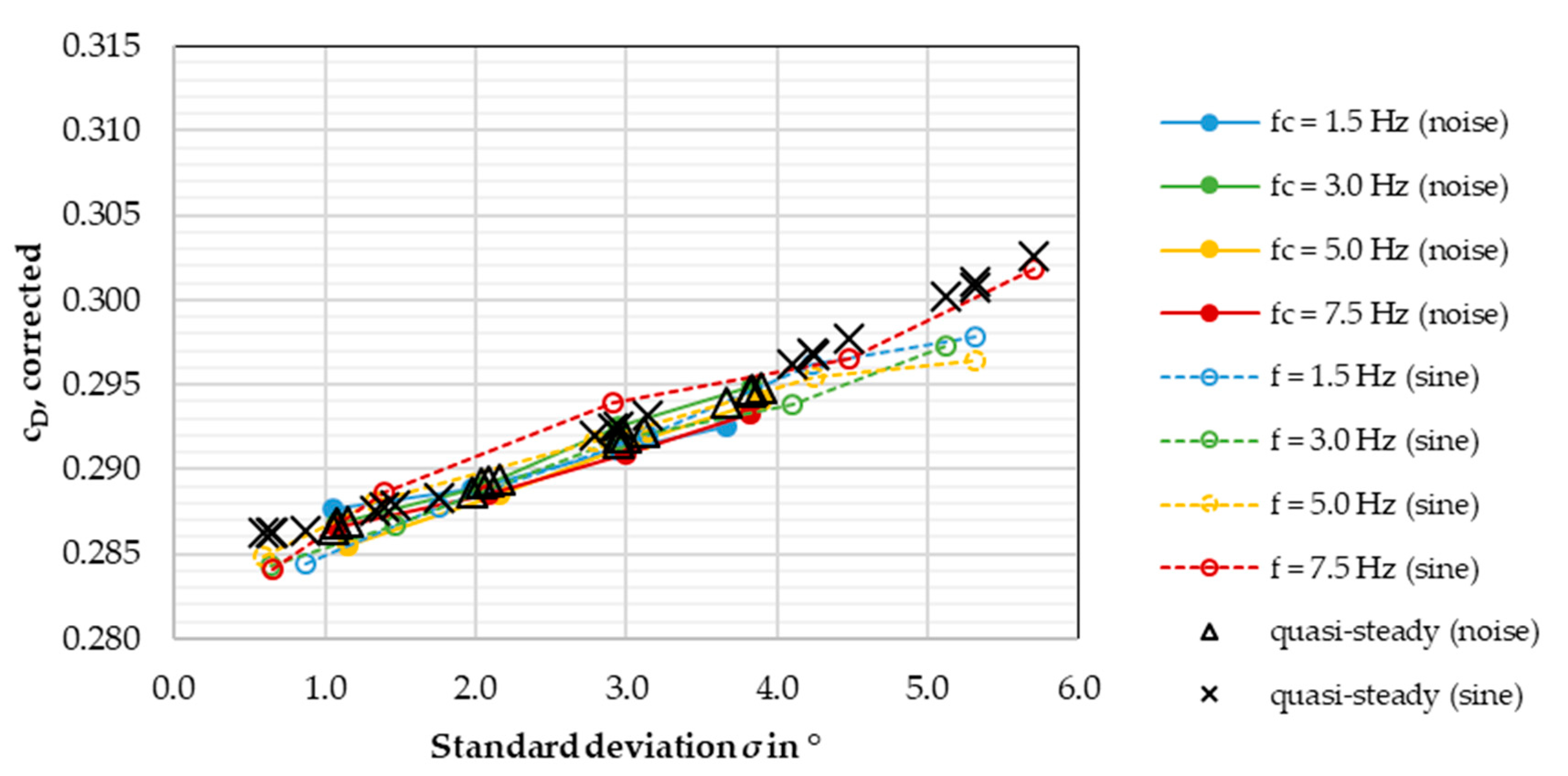
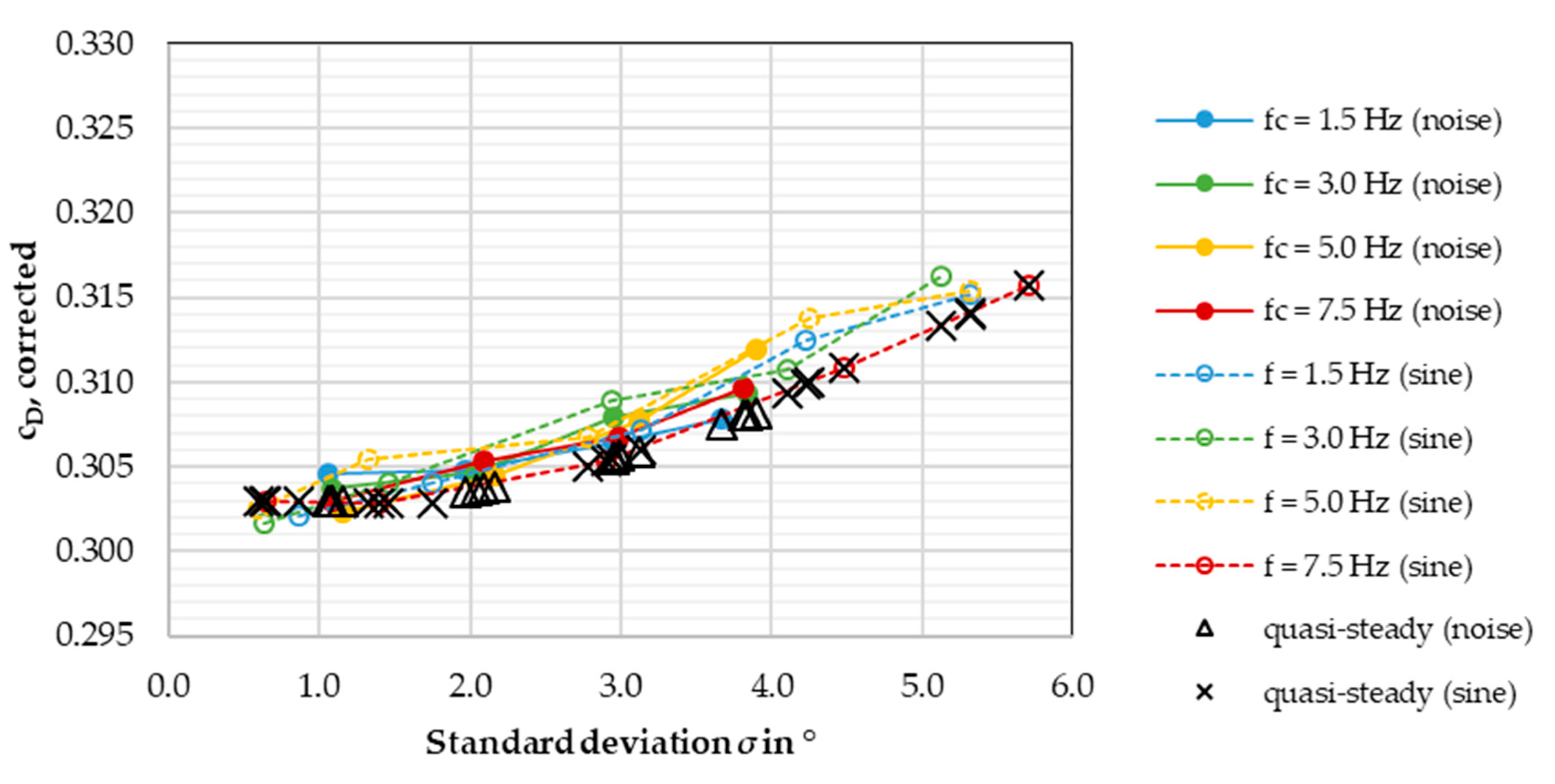
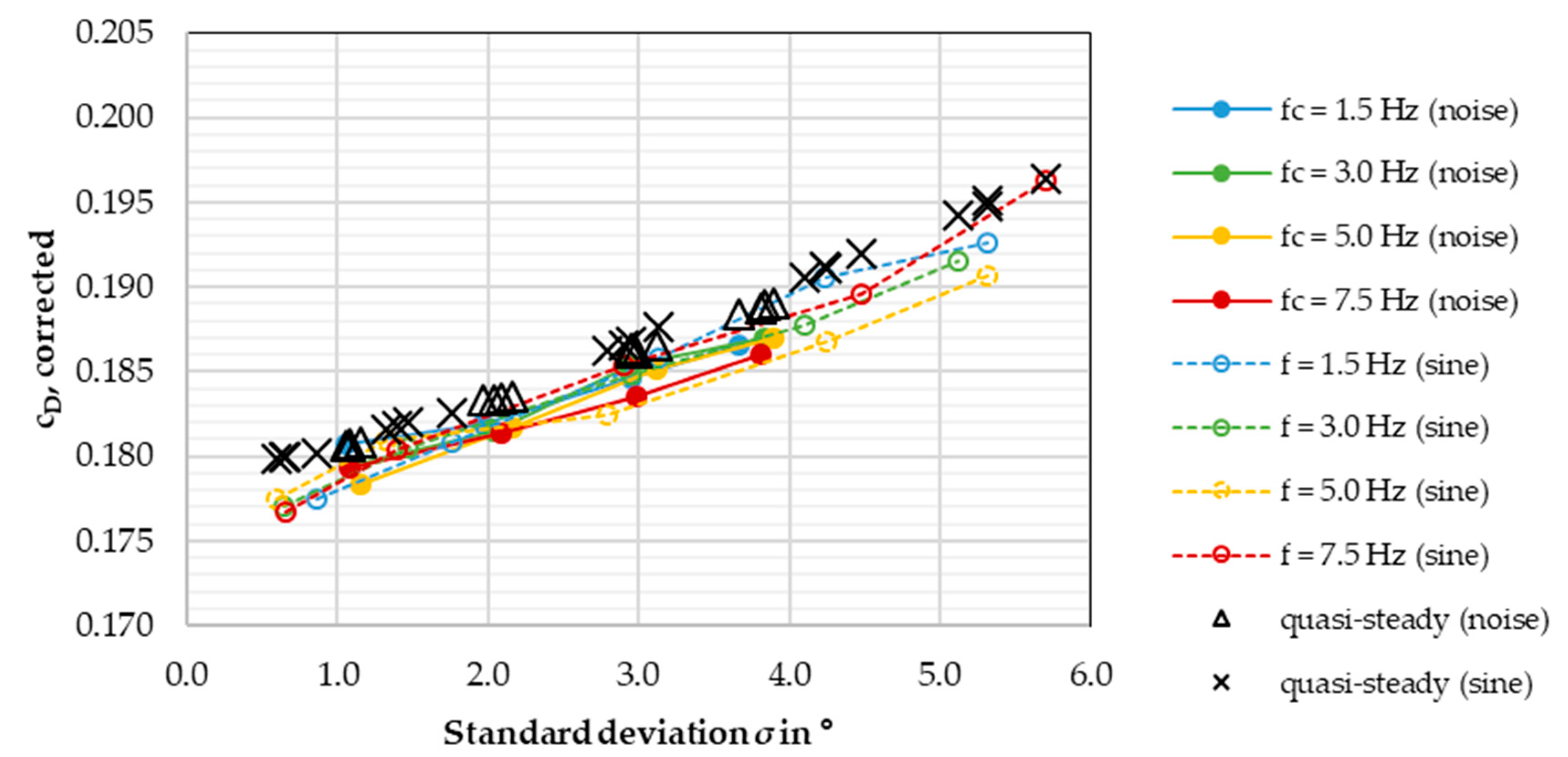
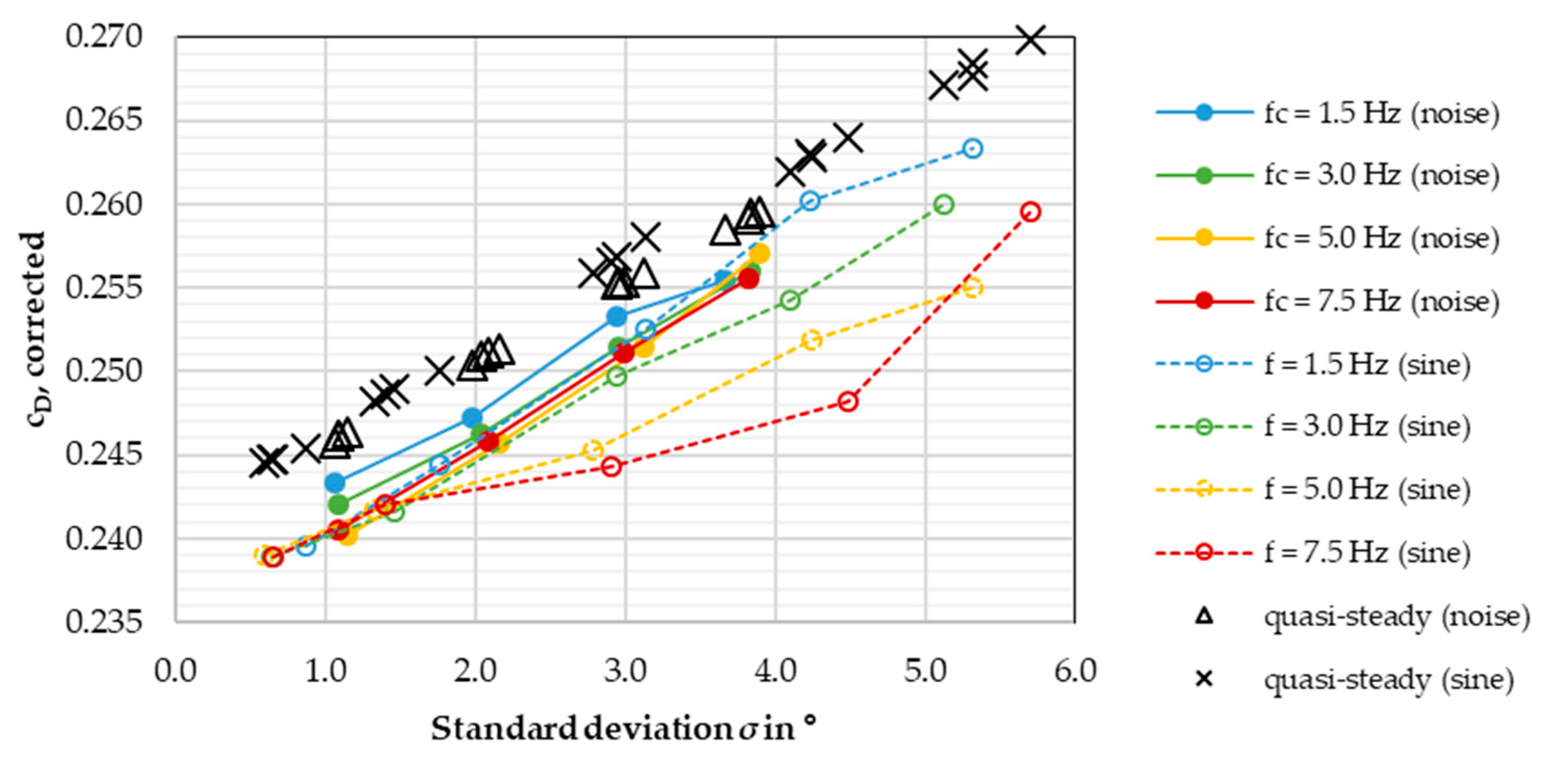
4.3. Vehicle Drag Results
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
Abbreviations
| CFD | Computational Fluid Dynamics |
| FKFS | Forschungsinstitut für Kraftfahrwesen und Fahrzeugmotoren Stuttgart |
| IFS | Institut für Fahrzeugtechnik, University of Stuttgart |
| SAE | Society of Automotive Engineers |
| swing® | side wind generator |
| Signal amplitude (for sine signals) | |
| Drag coefficient | |
| Pressure coefficient | |
| Signal frequency (for sine signals) | |
| Signal cutoff frequency (for low-pass filtered noise signals) | |
| Pressure gradient in the empty wind tunnel test section | |
| Incident flow yaw angle | |
| Signal standard deviation (for both noise and sine signals) |
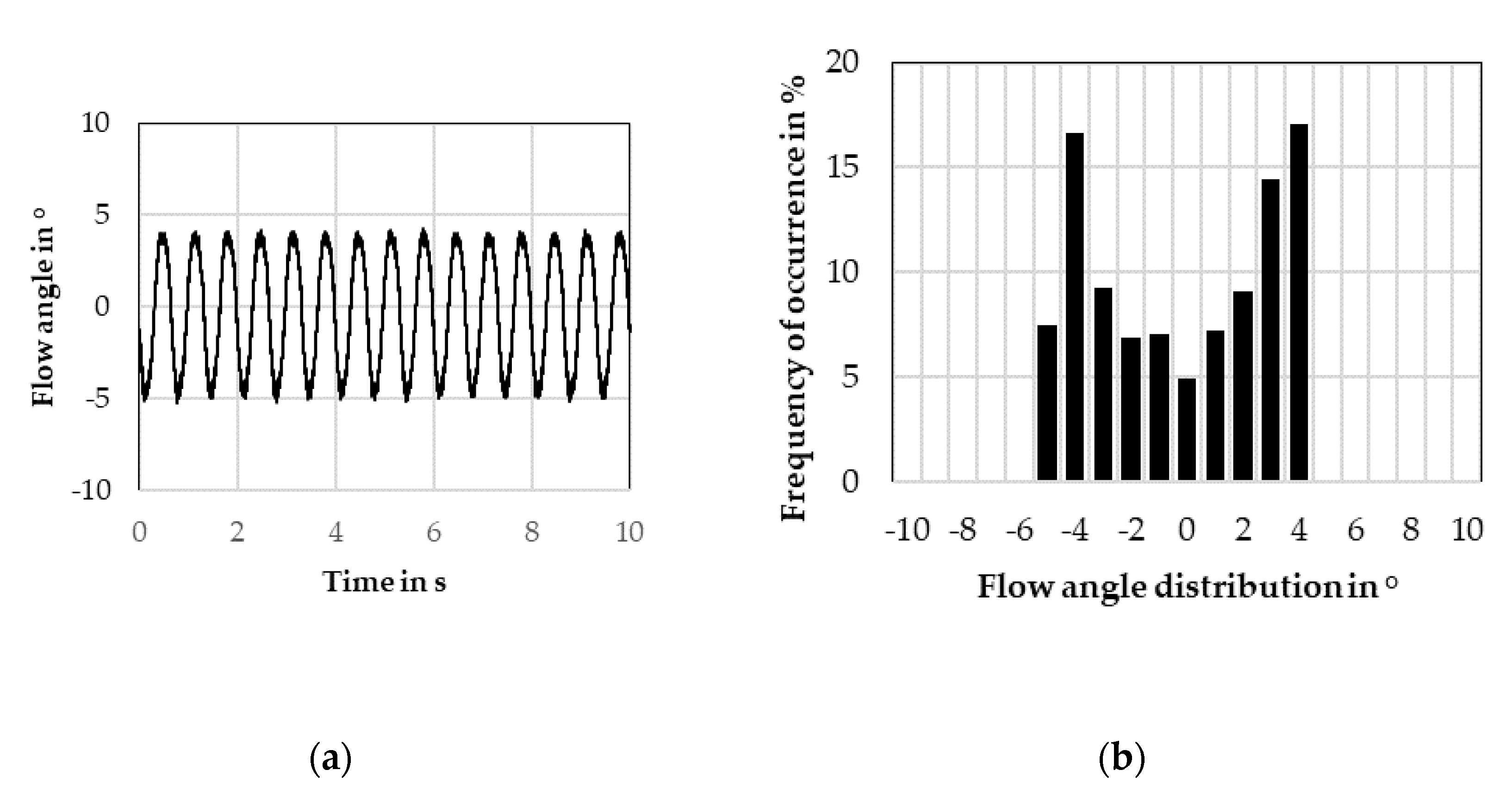
Appendix A Transient Signals Used
| Cutoff Frequency in Hz | Flap angle Standard Deviation in ° | Measured Flow Angle Standard Deviation in ° |
|---|---|---|
| 1.5 | 0.75 | 1.06 |
| 1.5 | 1.50 | 1.97 |
| 1.5 | 2.25 | 2.95 |
| 1.5 | 3.00 | 3.84 |
| 3.0 | 0.75 | 1.08 |
| 3.0 | 1.50 | 2.04 |
| 3.0 | 2.25 | 2.95 |
| 3.0 | 3.00 | 3.84 |
| 5.0 | 0.75 | 1.15 |
| 5.0 | 1.50 | 2.16 |
| 5.0 | 2.25 | 3.12 |
| 5.0 | 3.00 | 3.90 |
| 7.5 | 0.75 | 1.08 |
| 7.5 | 1.50 | 2.09 |
| 7.5 | 2.25 | 2.99 |
| 7.5 | 3.00 | 3.82 |
| Cutoff Frequency in Hz | Flap Angle Amplitude in ° | Measured Flow Angle Amplitude in ° |
|---|---|---|
| 1.5 | 1.20 | 1.23 |
| 1.5 | 2.50 | 2.48 |
| 1.5 | 5.00 | 4.44 |
| 1.5 | 7.50 | 5.98 |
| 1.5 | 10.00 | 7.53 |
| 3.0 | 1.20 | 0.90 |
| 3.0 | 2.50 | 2.07 |
| 3.0 | 5.00 | 4.17 |
| 3.0 | 7.50 | 5.80 |
| 3.0 | 10.00 | 7.25 |
| 5.0 | 1.20 | 0.84 |
| 5.0 | 2.50 | 1.88 |
| 5.0 | 5.00 | 3.94 |
| 5.0 | 7.50 | 6.01 |
| 5.0 | 10.00 | 7.52 |
| 7.5 | 1.20 | 0.92 |
| 7.5 | 2.50 | 1.98 |
| 7.5 | 5.00 | 4.11 |
| 7.5 | 7.50 | 6.34 |
| 7.5 | 10.00 | 8.07 |
References
- Mercker, E.; Cooper, K. A Two-Measurement Correction for the Effects of a Pressure Gradient on Automotive, Open-Jet, Wind Tunnel Measurements. SAE Tech. Pap. 2006. [Google Scholar] [CrossRef]
- Hennig, A. Eine Erweiterte Methode zur Korrektur von Interferenzeffekten in Freistrahlwindkanälen für Automobile. Ph.D. Thesis, University of Stuttgart, Stuttgart, Germany, 2016. [Google Scholar]
- Mercker, E.; Wiedemann, J. On the Correction of Interference Effects in Open-Jet Wind Tunnels. SAE Tech. Pap. 1996. [Google Scholar] [CrossRef]
- Cooper, K.; Watkins, S. The Unsteady Wind Environment of Road Vehicles, Part One: A Review of the On-Road Turbulent Wind Environment. SAE Tech. Pap. 2007. [Google Scholar] [CrossRef]
- Lawson, A.; Sims-Williams, D.; Dominy, R. Effects of On-Road Turbulence on Vehicle Surface Pressures in the A-Pillar Region. SAE Int. J. Passeng. Cars Mech. Syst. 2008, 1, 333–340. [Google Scholar] [CrossRef]
- Jessing, C.; Wittmeier, F.; Wiedemann, J.; Wilhelmi, H.; Dillmann, A. Characterization of the Transient Airflow around a Vehicle on Public Highways. In Proceedings of the 12th FKFS Conference, Progress in Vehicle Aerodynamics and Thermal Management, Stuttgart, Germany, 1–2 October 2019. [Google Scholar]
- Cogotti, A. Generation of a Controlled Level of Turbulence in the Pininfarina Wind Tunnel for the Measurement of Unsteady Aerodynamics and Aeroacoustics. SAE Tech. Pap. 2003. [Google Scholar] [CrossRef]
- Cogotti, A. Update on the Pininfarina “Turbulence Generation System” and its effects on the Car Aerodynamics and Aeroacoustics. SAE Tech. Pap. 2004. [Google Scholar] [CrossRef]
- Mankowski, O.; Sims-Williams, D.; Dominy, R. A Wind Tunnel Simulation Facility for On-Road Transients. SAE Int. J. Passeng. Cars Mech. Syst. 2014, 7, 1087–1095. [Google Scholar] [CrossRef]
- Yamashita, T.; Makihara, T.; Maeda, K.; Tadakuma, K. Unsteady Aerodynamic Response of a Vehicle by Natural Wind Generator of a Full-Scale Wind Tunnel. SAE Int. J. Passeng. Cars Mech. Syst. 2017, 10, 358–368. [Google Scholar] [CrossRef]
- Schröck, D.; Widdecke, N.; Wiedemann, J. Aerodynamic Response of a Vehicle Model to Turbulent Wind. In Proceedings of the 7th FKFS Conference, Progress in Vehicle Aerodynamics and Thermal Management, Stuttgart, Germany, 6–7 October 2009. [Google Scholar]
- Stoll, D.; Kuthada, T.; Wiedemann, J. Unsteady Aerodynamic Vehicle Properties of the DrivAer Model in the IVK Model Scale Wind Tunnel. In Proceedings of the 10th FKFS Conference, Progress in Vehicle Aerodynamics and Thermal Management, Stuttgart, Germany, 29–30 September 2015. [Google Scholar]
- Stoll, D.; Kuthada, T.; Wiedemann, J. Experimental and numerical investigation of aerodynamic drag in turbulent flow conditions. In Proceedings of the iMechE International Conference on Vehicle Aerodynamics, Coventry, UK, 21–22 September 2016. [Google Scholar]
- Wittmeier, F. The Recent Upgrade of the Model Scale Wind Tunnel of University of Stuttgart. SAE Int. J. Passeng. Cars Mech. Syst. 2017, 10, 203–213. [Google Scholar] [CrossRef]
- Wiedemann, J.; Fischer, O.; Jiabin, P. Further Investigations on Gradient Effects. SAE Tech. Pap. 2004. [Google Scholar] [CrossRef]
- Zhang, C.; Tanneberger, M.; Kuthada, T.; Wittmeier, F.; Wiedemann, J.; Nies, J. Introduction of the AeroSUV-A New Generic SUV Model for Aerodynamic Research. SAE Tech. Pap. 2019. [Google Scholar] [CrossRef]
- Kuthada, T.; Pfannkuchen, E.; Wiedemann, J. Evaluation of Cooling Air Drag on a Reference Body. In Proceedings of the 5th MIRA International Vehicle Aerodynamics Conference, Warwick, UK, 13–14 October 2004. [Google Scholar]
- Barros, D.; Borée, J.; Noack, B.; Spohn, A.; Ruiz, T. Bluff body drag manipulation using pulsed jets and Coanda effect. J. Fluid Mech. 2016, 805, 422–459. [Google Scholar] [CrossRef]


© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fei, X.; Jessing, C.; Kuthada, T.; Wiedemann, J.; Wagner, A. The Influence of Different Unsteady Incident Flow Environments on Drag Measurements in an Open Jet Wind Tunnel. Fluids 2020, 5, 178. https://doi.org/10.3390/fluids5040178
Fei X, Jessing C, Kuthada T, Wiedemann J, Wagner A. The Influence of Different Unsteady Incident Flow Environments on Drag Measurements in an Open Jet Wind Tunnel. Fluids. 2020; 5(4):178. https://doi.org/10.3390/fluids5040178
Chicago/Turabian StyleFei, Xiao, Christoph Jessing, Timo Kuthada, Jochen Wiedemann, and Andreas Wagner. 2020. "The Influence of Different Unsteady Incident Flow Environments on Drag Measurements in an Open Jet Wind Tunnel" Fluids 5, no. 4: 178. https://doi.org/10.3390/fluids5040178
APA StyleFei, X., Jessing, C., Kuthada, T., Wiedemann, J., & Wagner, A. (2020). The Influence of Different Unsteady Incident Flow Environments on Drag Measurements in an Open Jet Wind Tunnel. Fluids, 5(4), 178. https://doi.org/10.3390/fluids5040178





