Hydrodynamic Analysis of Surge-Type Wave Energy Devices in Variable Bathymetry by Means of BEM
Abstract
1. Introduction
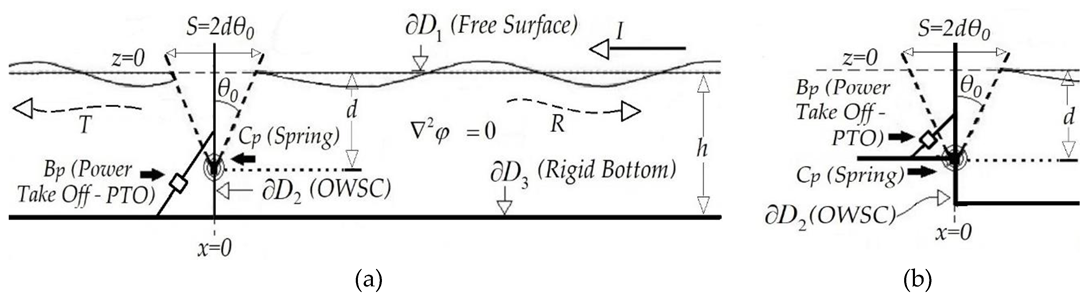
2. Hydrodynamic Analysis of A Two-dimensional Flap-Type Model in Variable Bathymetry
2.1. Mesh Generation
2.2. Discrete BEM Model
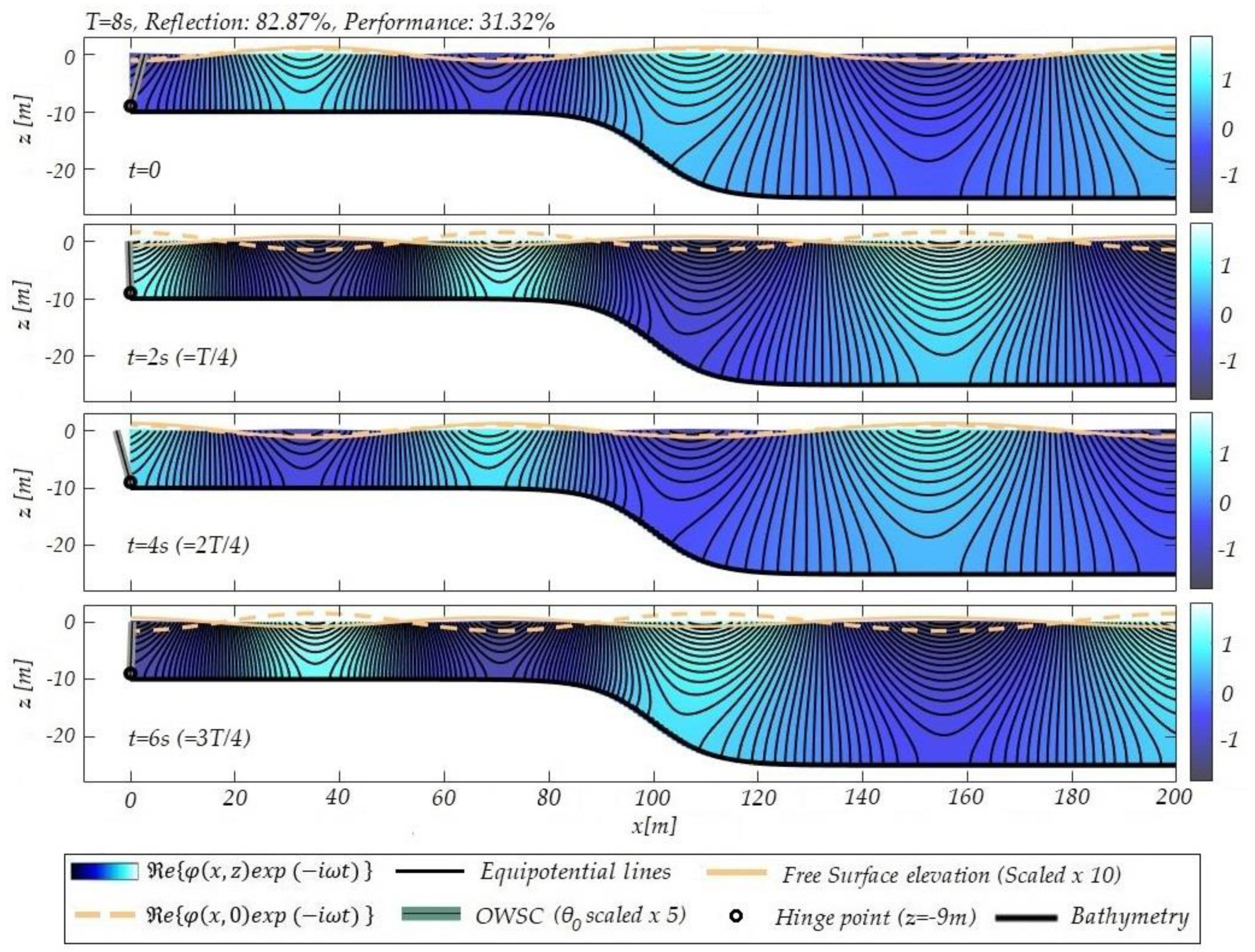
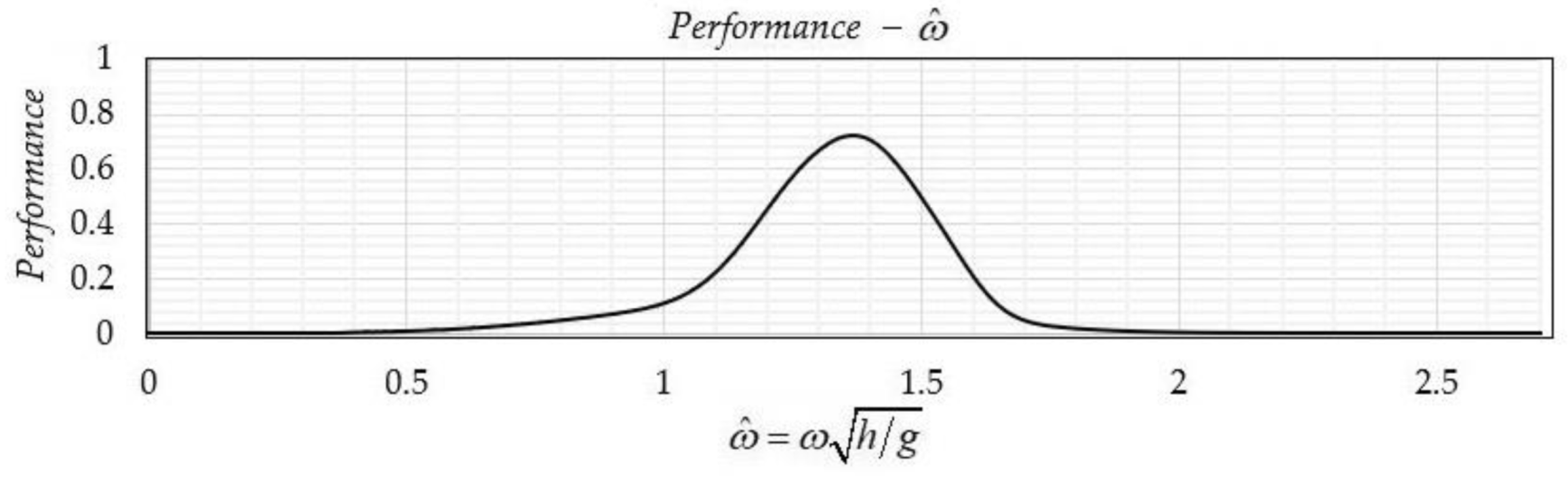
2.3. Validation of the BEM Model
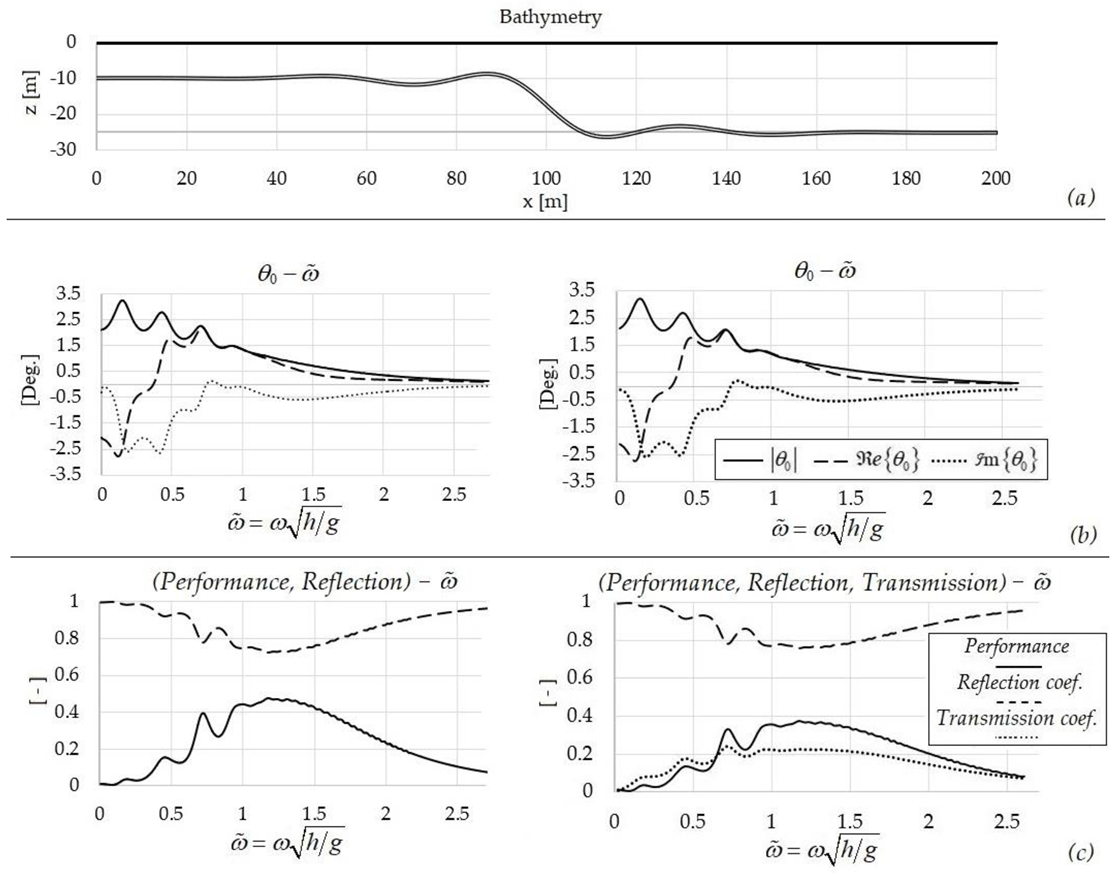
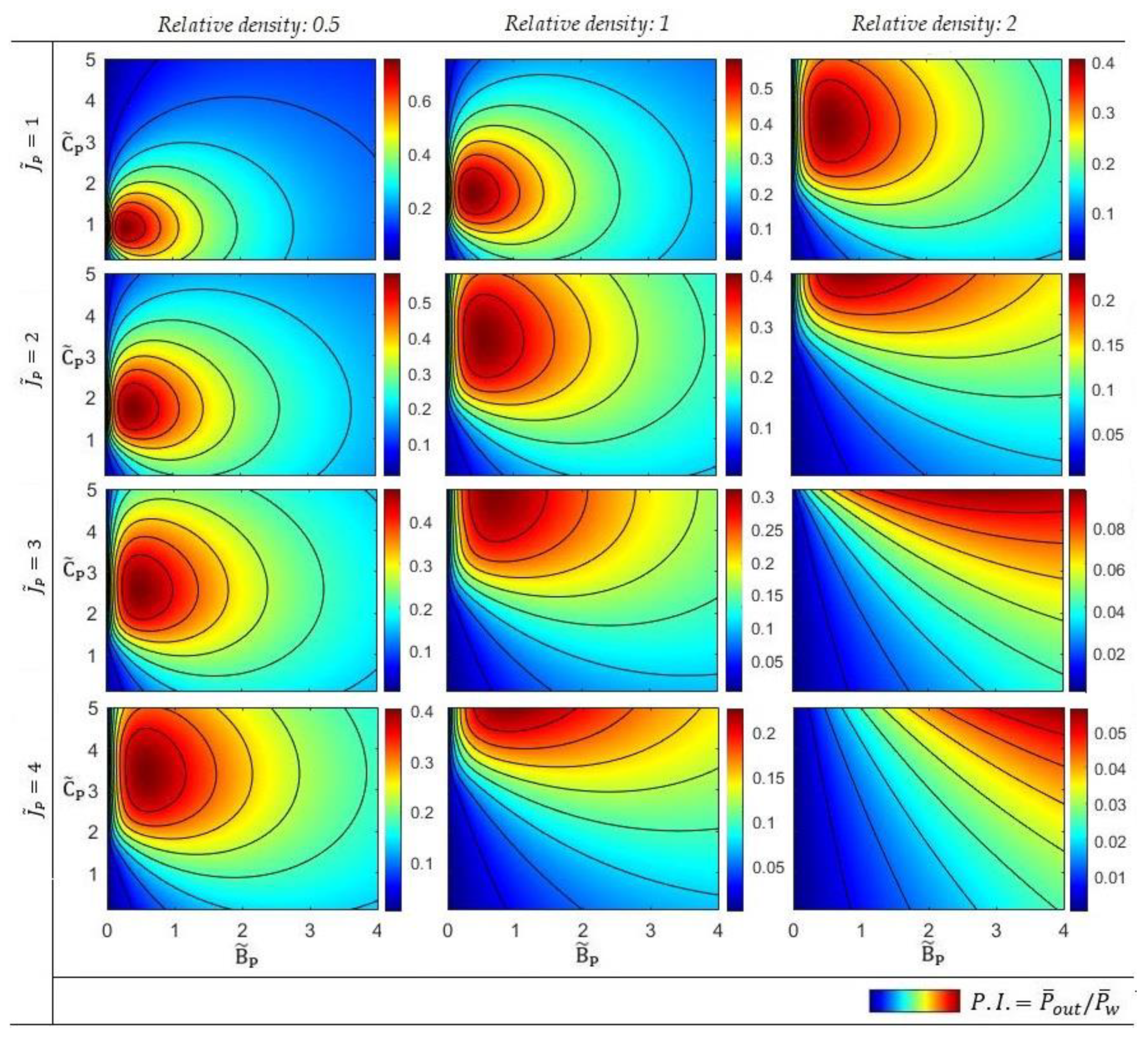
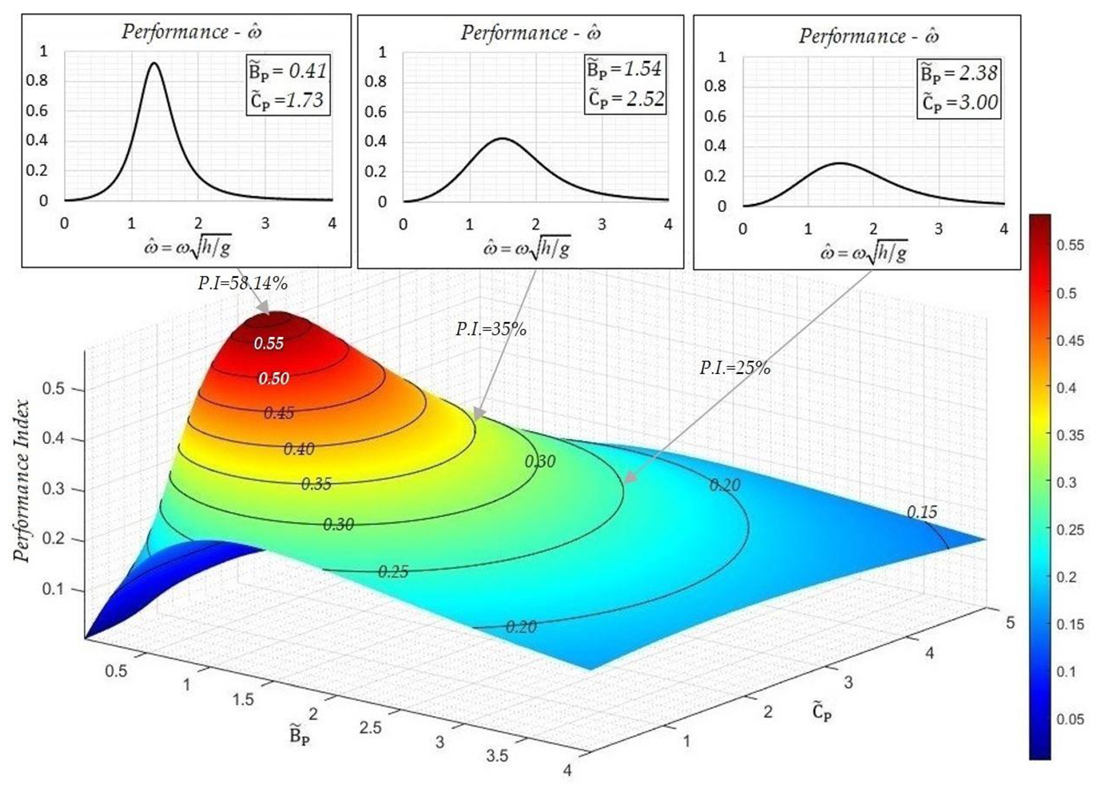
2.4. Performance of OWSC in Variable Bathymetry
3. WEC Model
Evaluation of Wave Field
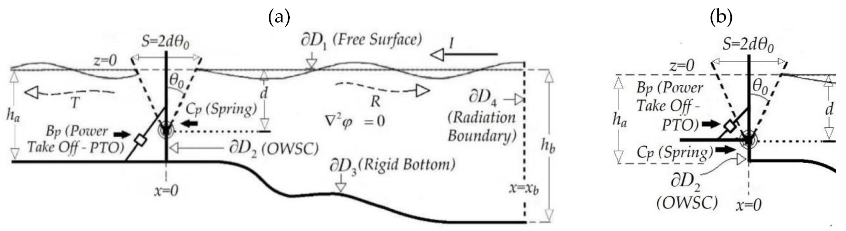
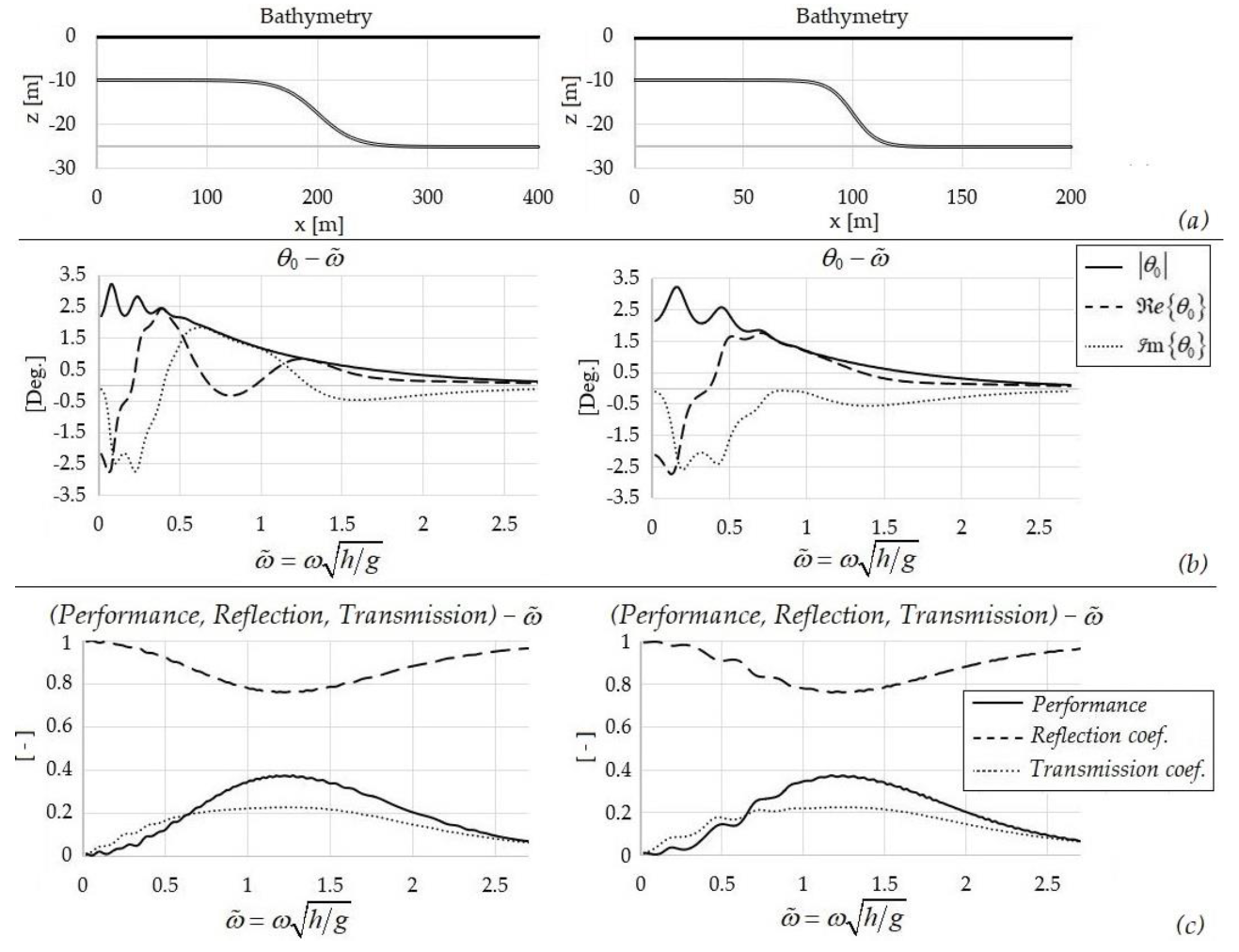
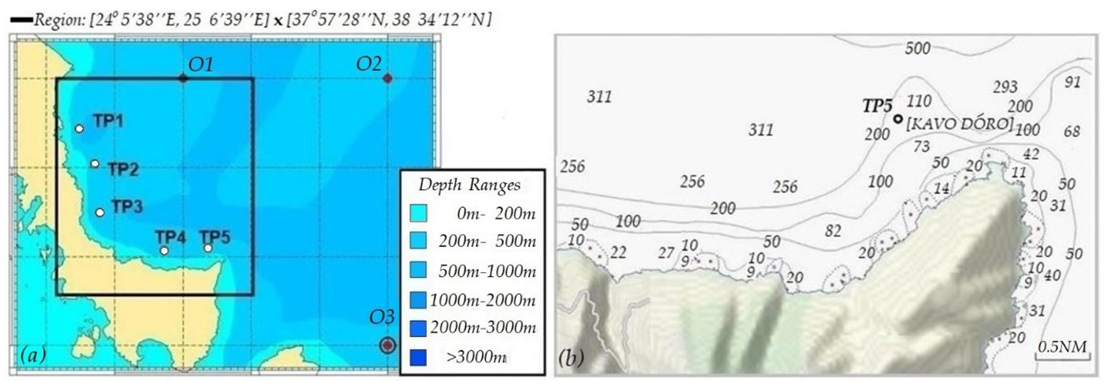
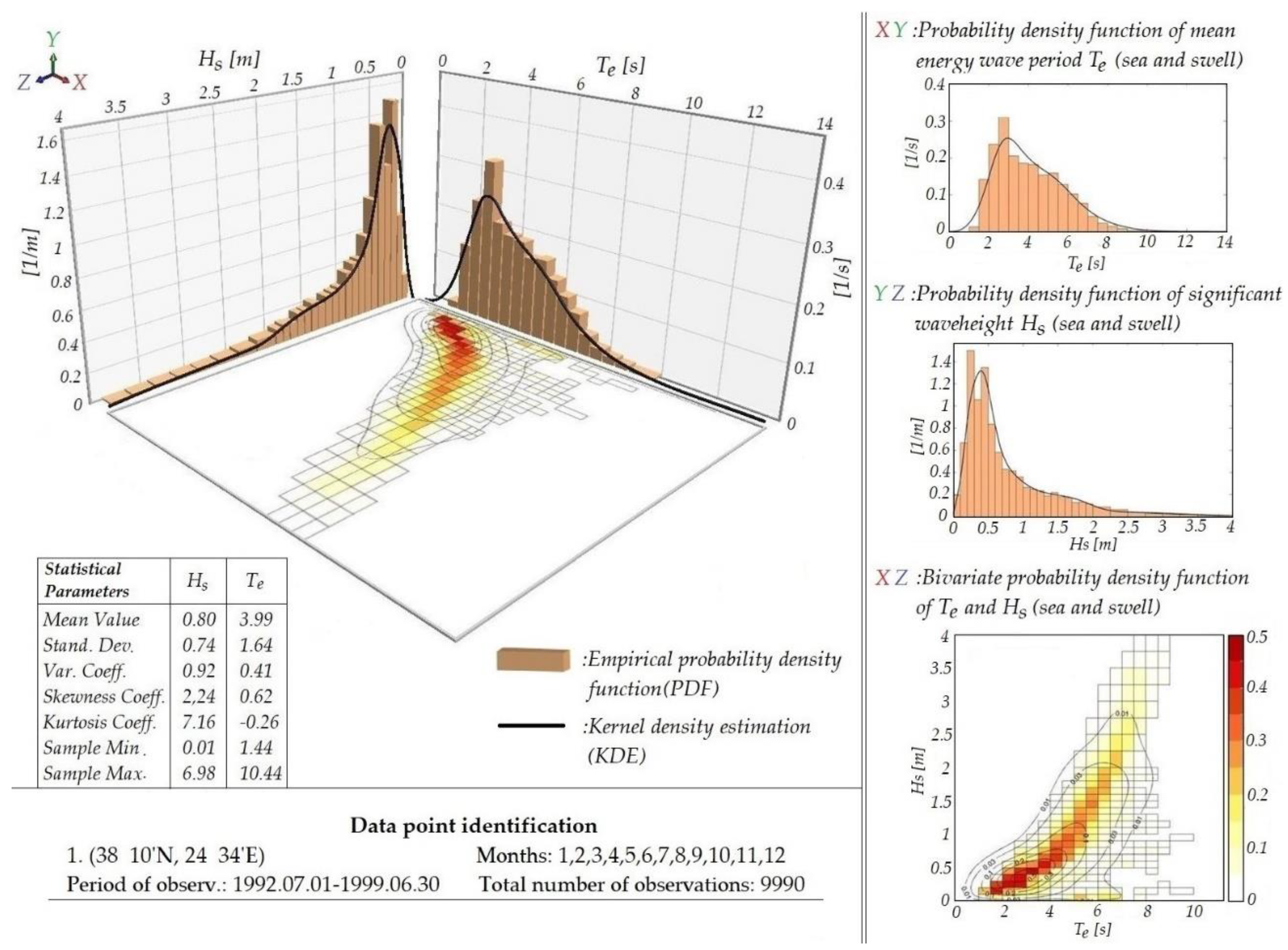
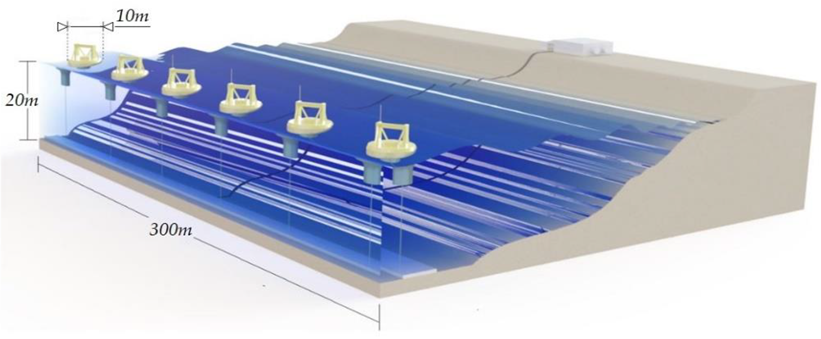
4. Application to A Specific Coastal Site in Greece
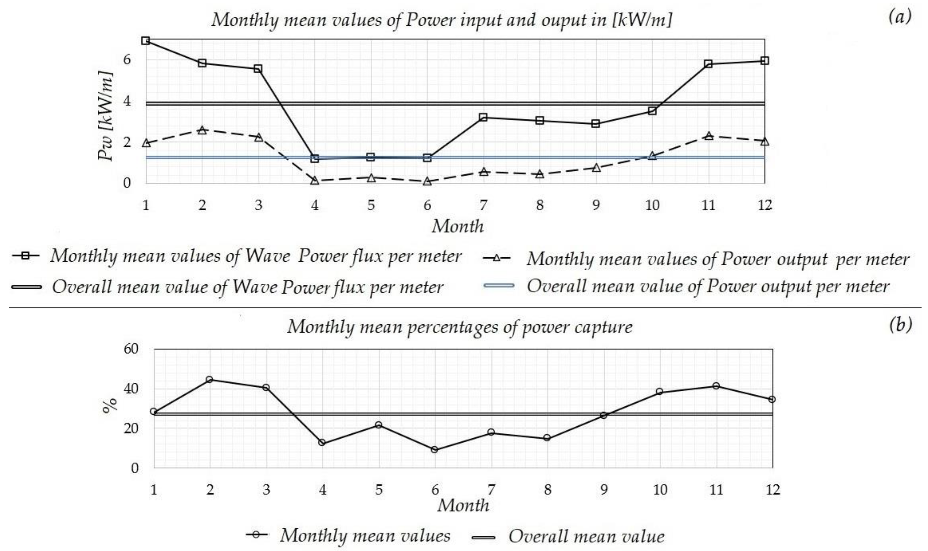
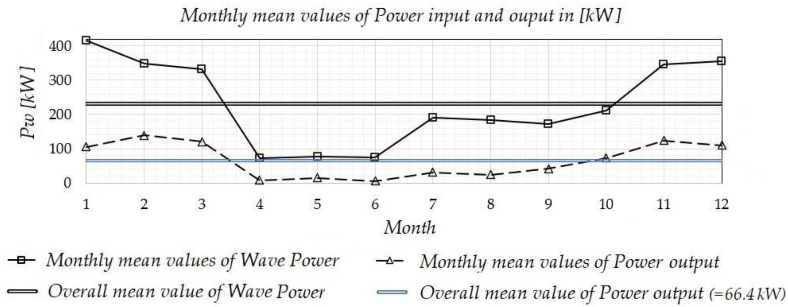
4.1. Estimation of Power Output by Application of OWSC
4.2. Bottom Topography Effects
4.3. Estimation of Power Output by Point Absorbers and Comparative Evaluation
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
Abbreviations
| BEM | Boundary Element Method |
| CMS | Coupled Mode System |
| OWSC | Oscillating Wave Surge Converter |
| PTO | Power Take Off |
| TP | Target Point |
| WEC | Wave Energy Converter |
Appendix A. Linearized 2dOWSC Model in Constant Water Depth

References
- Falnes, J. Ocean Waves and Oscillating Systems, 1st ed.; Cambridge University Press: Cambridge, UK, 2002. [Google Scholar]
- Babarit, A. Ocean Wave Energy Conversion, 1st ed.; ISTE Press-Elsevier: Amsterdam, The Netherlands, 2017. [Google Scholar]
- Whittaker, T.; Folley, M. Nearshore oscillating wave surge converters and the development of Oyster. Philos. Trans. R. Soc. 2012, 370, 345–364. [Google Scholar] [CrossRef]
- Renzi, E.; Doherty, K.; Henry, A.; Dias, F. How does Oyster work? The simple interpretation of Oyster mathematics. Eur. J. Mech.-B/Fluids 2014, 47, 124–131. [Google Scholar] [CrossRef]
- Whittaker, T.; Folley, M.; Osterried, M. The oscillating wave surge converter. In Proceedings of the International Society of Offshore and Polar Engineers (ISOPE), Toulon, France, 23–28 May 2004. [Google Scholar]
- Dias, F.; Renzi, E.; Gallagher, S.; Sarkar, D.; Wei, Y.; Abadie, T.; Cummins, C.; Rafiee, A. Analytical and computational modelling for wave energy systems: The example of oscillating wave surge converters. Acta Mech. Sin. 2017, 33, 647–662. [Google Scholar] [CrossRef] [PubMed]
- Folley, M.; Whittaker, T.; Henry, A. The effect of water depth on the performance of a small surging wave energy converter. Ocean Eng. 2007, 34, 1265–1274. [Google Scholar] [CrossRef]
- Belibassakis, K.A.; Bonovas, M.; Rusu, E. A novel method for estimating wave energy converter performance in variable bathymetry regions and applications. Energies 2018, 11, 2092. [Google Scholar] [CrossRef]
- Bonovas, M.; Belibassakis, K.A.; Rusu, E. Multi-DOF WEC performance in variable bathymetry regions using a hybrid 3D BEM and optimization. Energies 2019, 12, 2108. [Google Scholar] [CrossRef]
- Babarit, A.; Hals, J.; Muliawan, M.; Kurniawan, A.; Moan, T.; Krokstad, J. Numerical benchmarking study of a selection of wave energy converters. Renew. Energy 2012, 41, 44–63. [Google Scholar] [CrossRef]
- Katz, J.; Plotkin, A. Low-Speed Aerodynamics, 2nd ed.; Cambridge University Press: Cambridge, UK, 2001. [Google Scholar]
- Athanassoulis, G.A.; Belibassakis, K.A. A consistent coupled-mode theory for the propagation of small-amplitude water waves over variable bathymetry regions. J. Fluid Mech. 1999, 389, 275–301. [Google Scholar] [CrossRef]
- Belibassakis, K.A.; Athanassoulis, G.; Gerostathis, T. A coupled-mode model for the refraction–diffraction of linear waves over steep three-dimensional bathymetry. Appl. Ocean Res. 2001, 23, 319–336. [Google Scholar] [CrossRef]
- Belibassakis, K.A.; Gerostathis, T.; Athanassoulis, G.; Soares, C. A 3D-BEM coupled-mode method for WEC arrays in variable bathymetry. In Proceedings of the 2nd International Conference on Renewable Energies Offshore (RENEW2016), Lisbon, Portugal, 24–26 October 2016. [Google Scholar]
- Soukissian, T.; Gizari, N.; Chatzinaki, M. Wave potential of the Greek seas. WIT Trans. Ecol. Environ. 2011, 143, 203–213. [Google Scholar] [CrossRef]
- Lavidas, G. Energy and socio-economic benefits from the development of wave energy in Greece. Renew. Energy 2019, 132, 1290–1300. [Google Scholar] [CrossRef]
- Athanassoulis, G.; Belibassakis, K.A.; Gerostathis, T. The POSEIDON nearshore wave model and its application to the prediction of the wave conditions in the Nearshore/Coastal Region of the Greek Seas. J. Atmos. Ocean Sci. 2002, 8, 201–217. [Google Scholar] [CrossRef]
- Athanassoulis, G.A.; Belibassakis, K.A.; Stefanakos, C.N. Wave Power Prediction in nearshore areas based on offshore wave information. A case study. In Proceedings of the 2nd IASTED International Conference, Crete, Greece, 25–28 June 2002. [Google Scholar]
- Athanassoulis, G.; Skarsoulis, E.; Belibassakis, K.A. Bivariate distributions with given marginals with an application to wave climate description. Appl. Ocean Res. 1994, 16, 1–17. [Google Scholar] [CrossRef]
- Ochi, M.K. Ocean Waves: The Stochastic Approach; Cambridge University Press: Cambridge, UK, 1998. [Google Scholar]
- Neary, V.; Previsic, M.; Jepsen, R.; Lawson, M.; Yu, Y.; Copping, A.; Fontaine, A.; Hallett, K.; Murray, D. Methodology for Design and Economic Analysis of Marine Energy Conversion (MEC) Technologies (Report No. SAND2014-9040); Report by Sandia National Laboratories (SNL); Sandia National Laboratories: Albuquerque, NM, USA, 2014; pp. 1–261.








© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Magkouris, A.; Bonovas, M.; Belibassakis, K. Hydrodynamic Analysis of Surge-Type Wave Energy Devices in Variable Bathymetry by Means of BEM. Fluids 2020, 5, 99. https://doi.org/10.3390/fluids5020099
Magkouris A, Bonovas M, Belibassakis K. Hydrodynamic Analysis of Surge-Type Wave Energy Devices in Variable Bathymetry by Means of BEM. Fluids. 2020; 5(2):99. https://doi.org/10.3390/fluids5020099
Chicago/Turabian StyleMagkouris, Alexandros, Markos Bonovas, and Kostas Belibassakis. 2020. "Hydrodynamic Analysis of Surge-Type Wave Energy Devices in Variable Bathymetry by Means of BEM" Fluids 5, no. 2: 99. https://doi.org/10.3390/fluids5020099
APA StyleMagkouris, A., Bonovas, M., & Belibassakis, K. (2020). Hydrodynamic Analysis of Surge-Type Wave Energy Devices in Variable Bathymetry by Means of BEM. Fluids, 5(2), 99. https://doi.org/10.3390/fluids5020099





