Experimental and Numerical Study of Blood Flow in μ-vessels: Influence of the Fahraeus–Lindqvist Effect
Abstract
1. Introduction
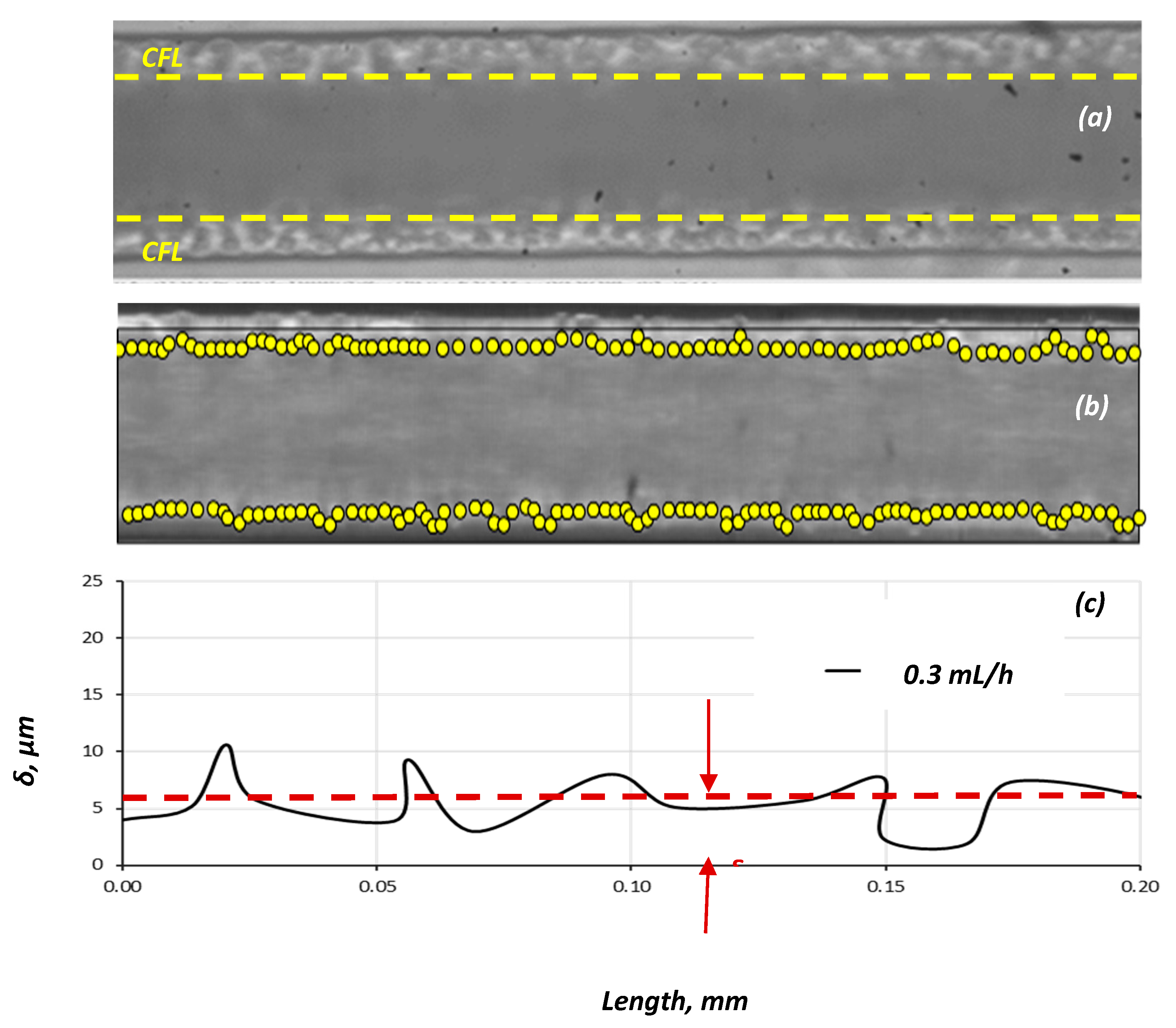
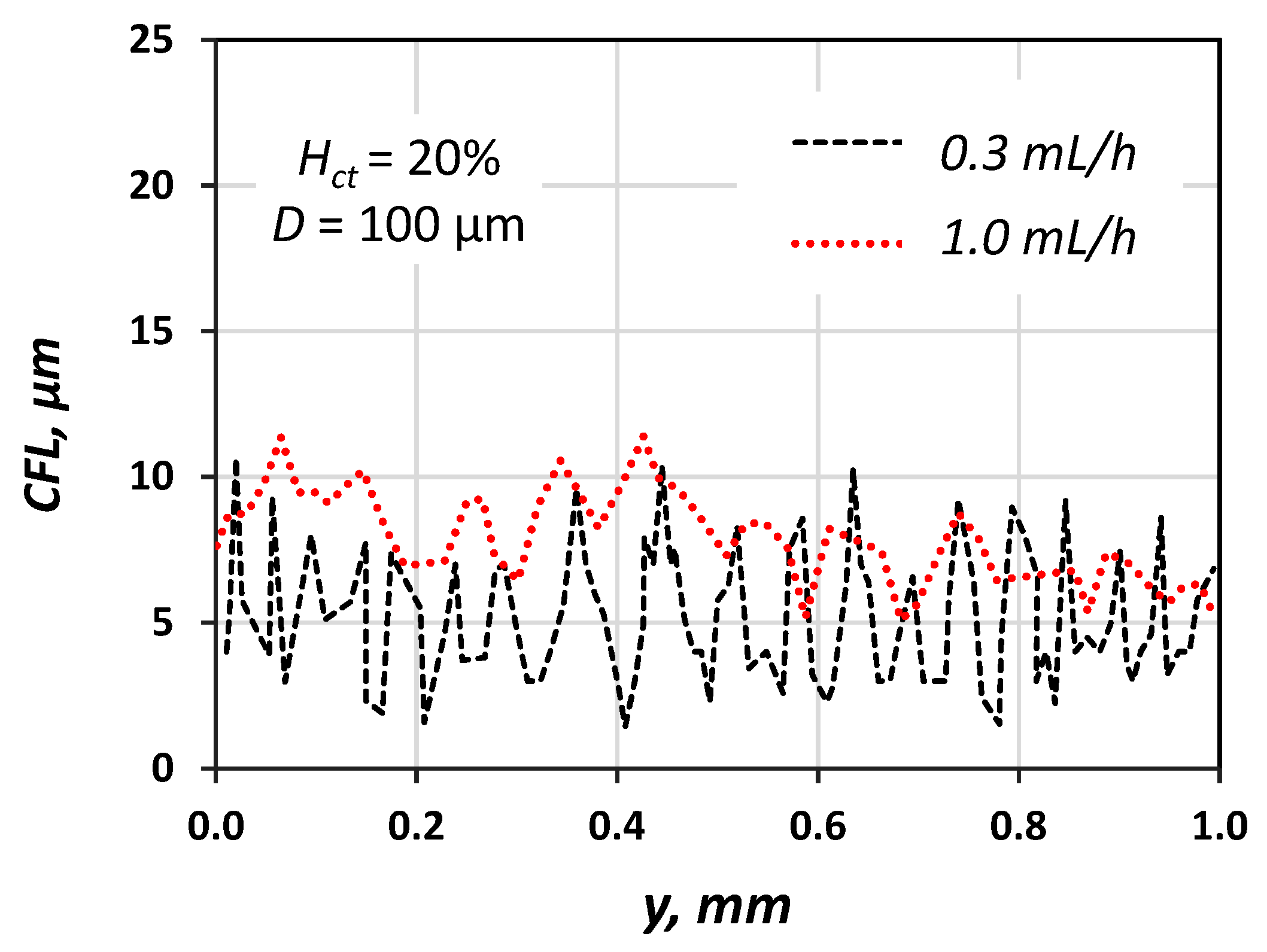
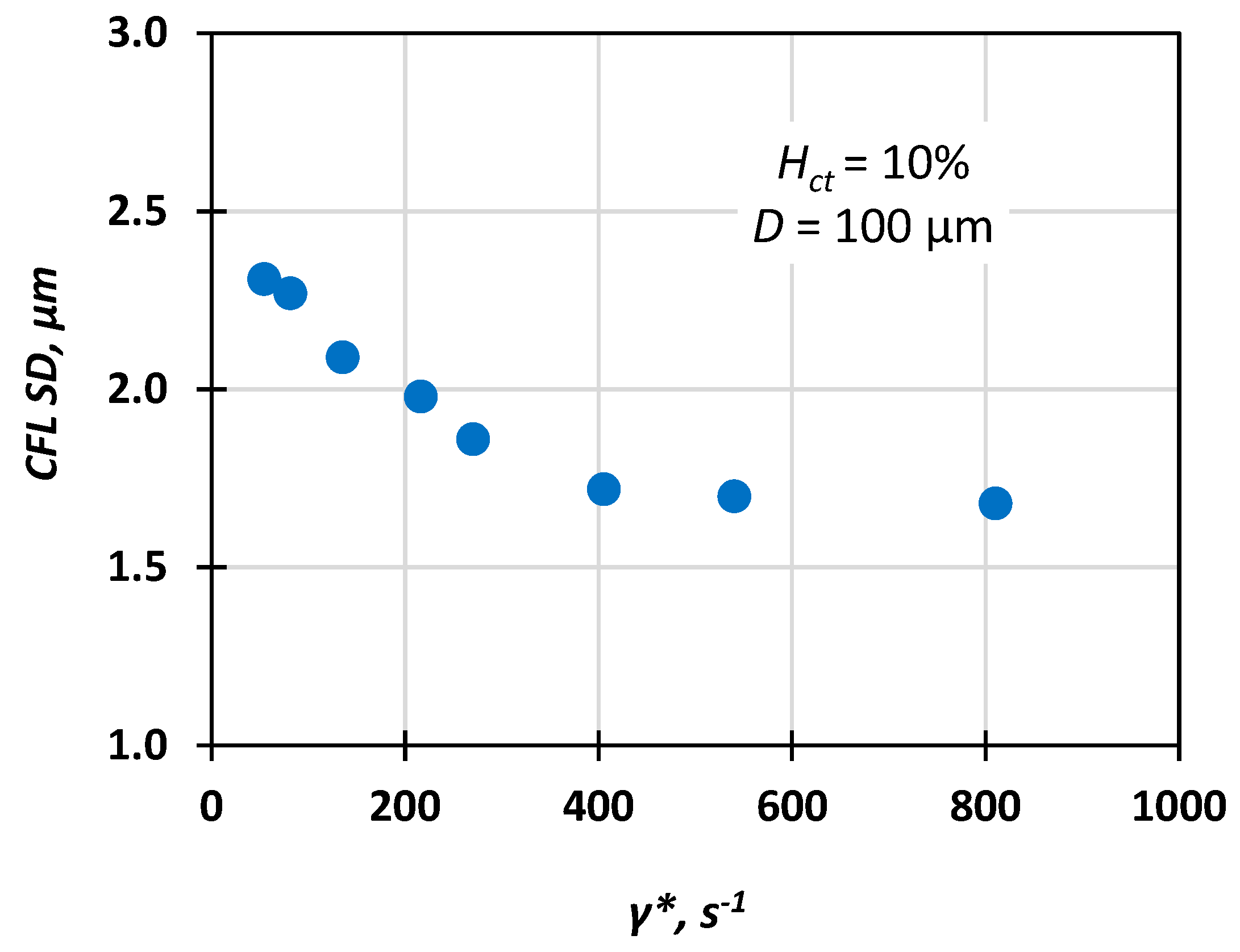
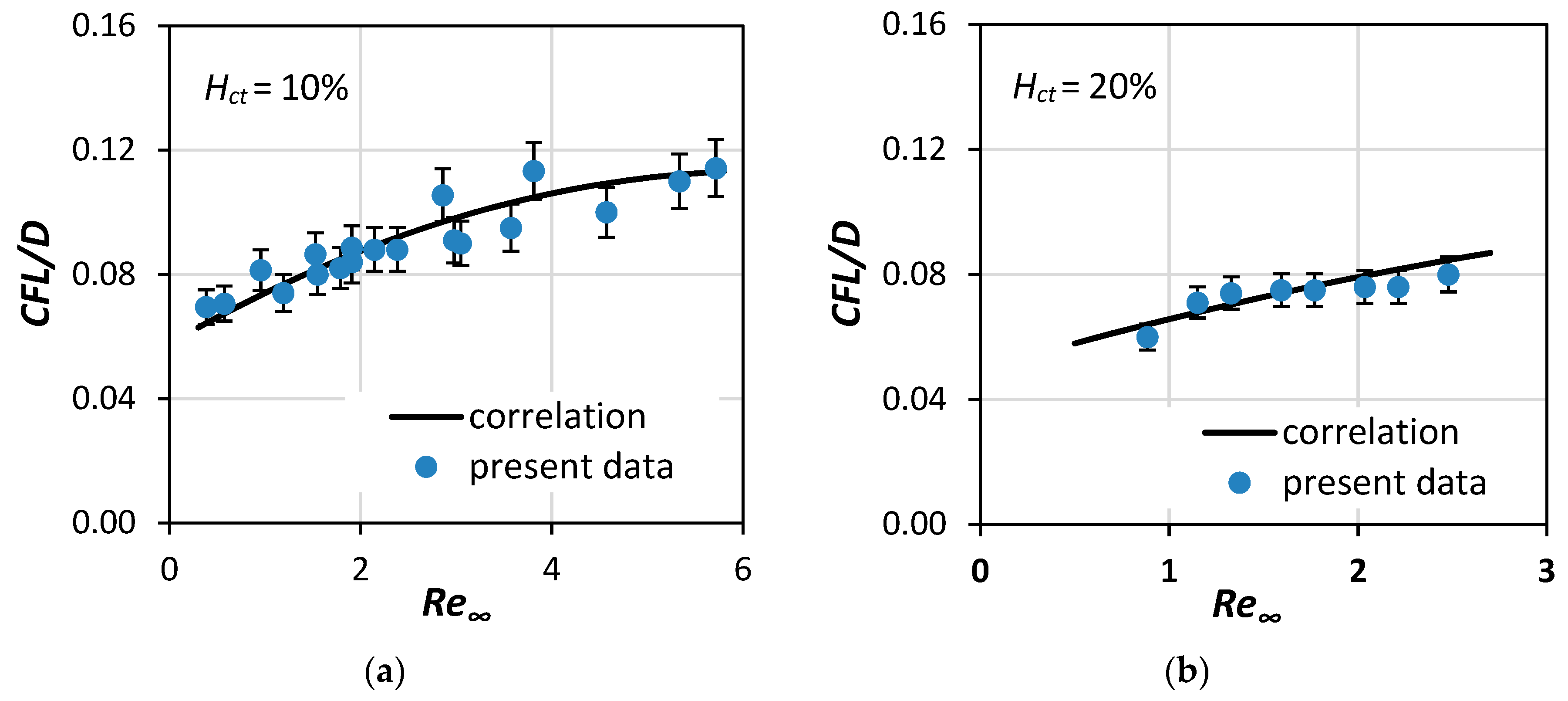
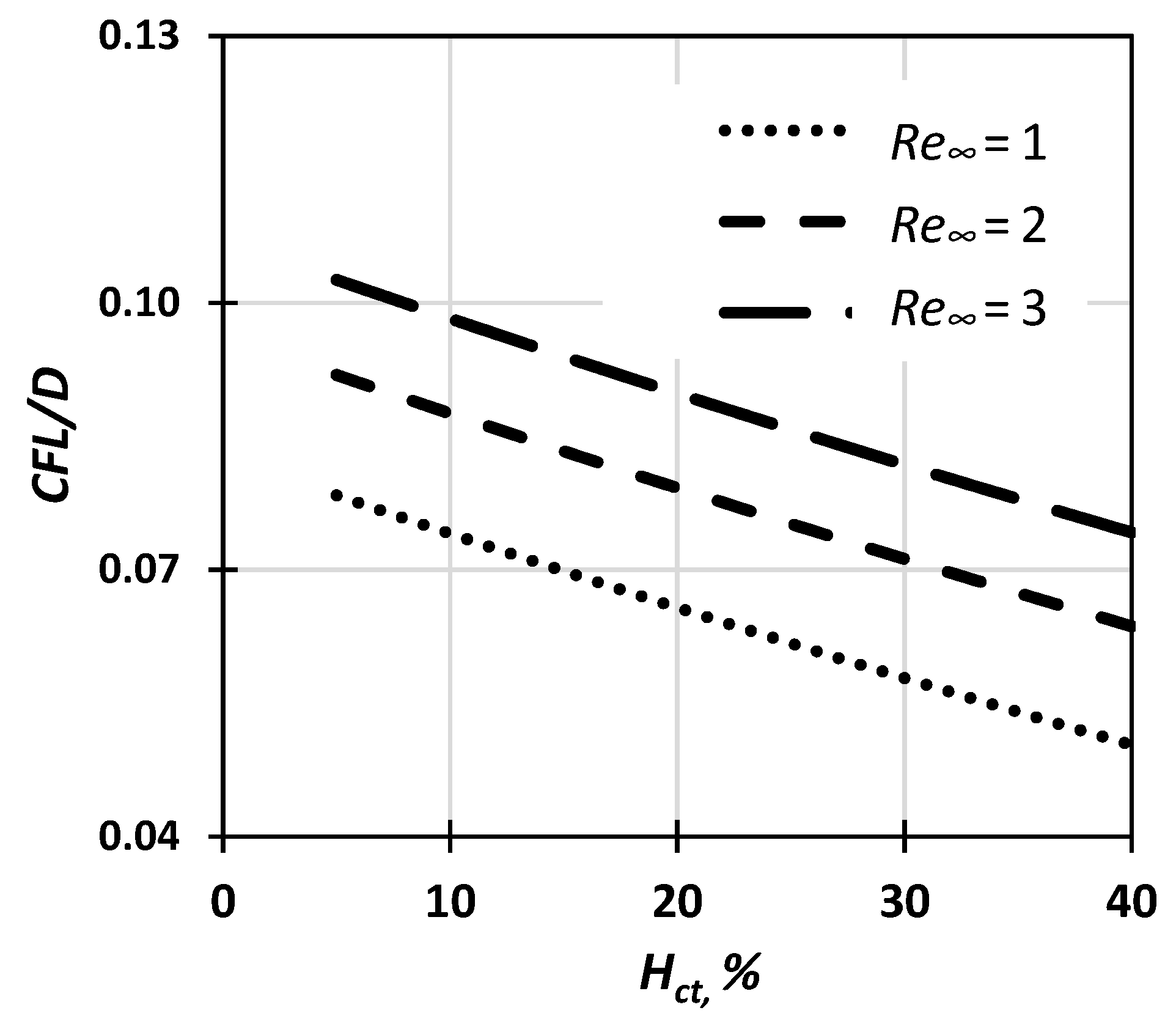
- First, estimate the CFL extent. In the relevant published research, either the Hct value [12] or the vessel diameter [13] was considered when formulating correlations for the prediction of the CFL width. In this study, we investigated the effect of both Re∞ and Hct on CFL characteristics in three microvessels with a 50, 100, and 170 μm hydraulic diameter. The experimental data were used for formulating a correlation for estimating the CFL width under the combined effect of the various flow conditions (i.e., Re∞) and hematocrit values.
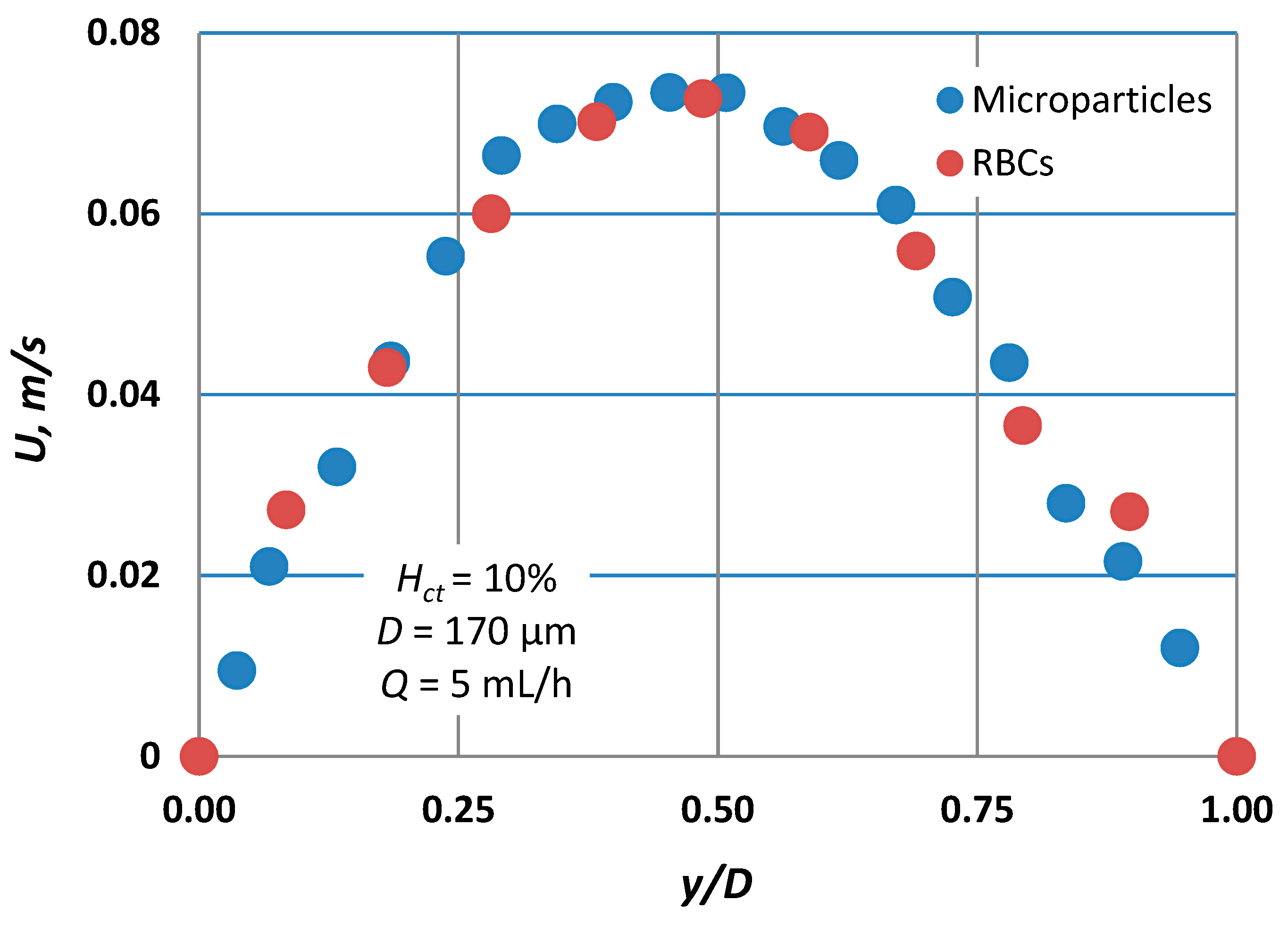
- The second one is to investigate the blood velocity profile in such microvessels using the well-established micro-particle image velocimetry (μ-PIV) technique. We employed two different tracing methods, i.e., coloured RBCs or standard PIV tracers. Previous studies suggested that the two tracing methods give significant different results [14]. This issue is clarified in this study. The acquired experimental data was used for validating the computational fluid dynamics (CFD) code.
- The final one is to develop a simplified “two-regions” model using computational fluid dynamics (CFD) by utilizing the outcome of the experimental part of this study in order to set up reliable simulations, a common practice in the literature [15]. The scope of this final step was to calculate the blood flow characteristics as well as the overall pressure drop (ΔP) across the vessels. The CFL flow domain was solved by assuming the blood properties are that of a Newtonian fluid, whereas in the vessel core, the blood rheology is formulated using a non-Newtonian model. Simulations were executed for various blood velocities and vessel diameters.
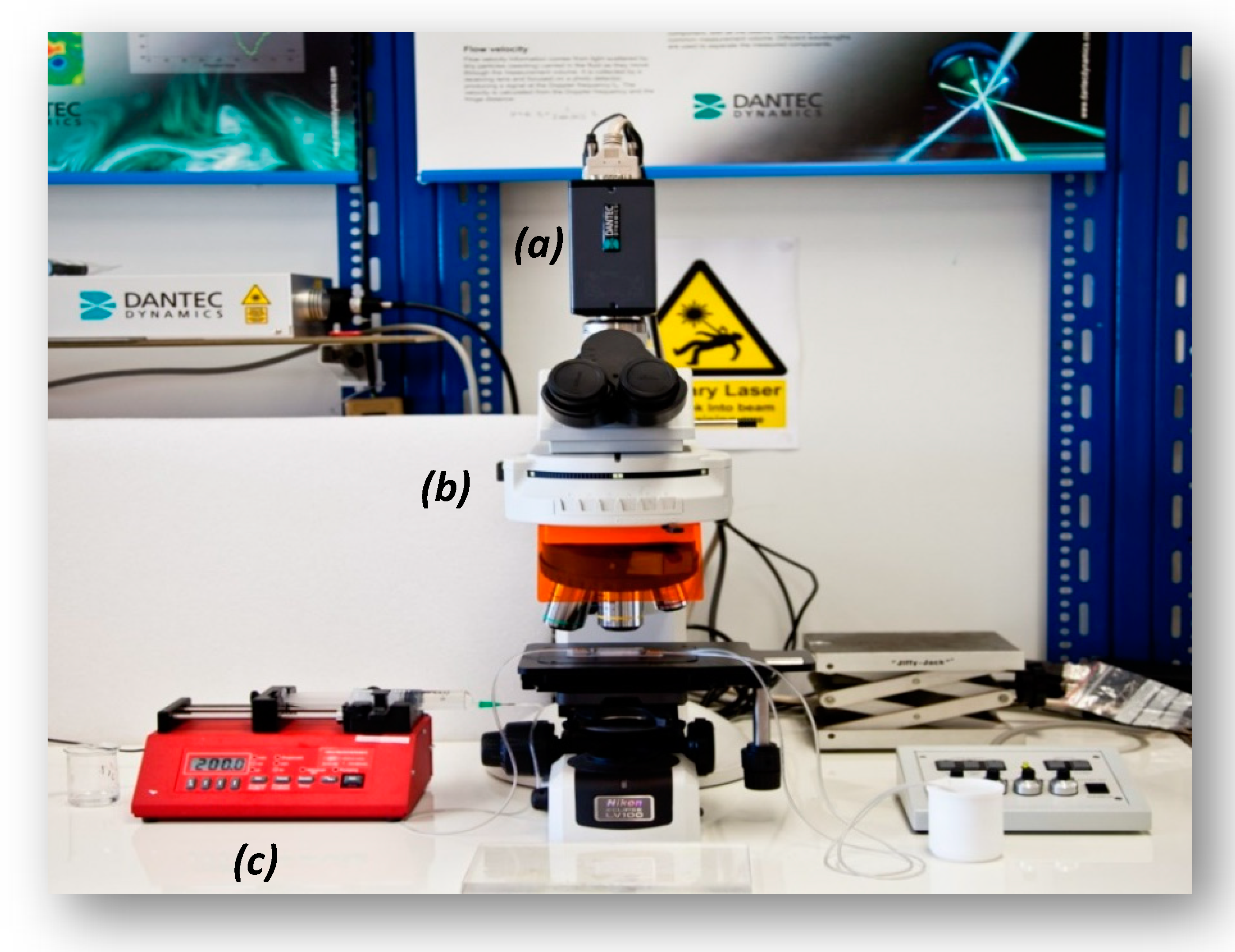
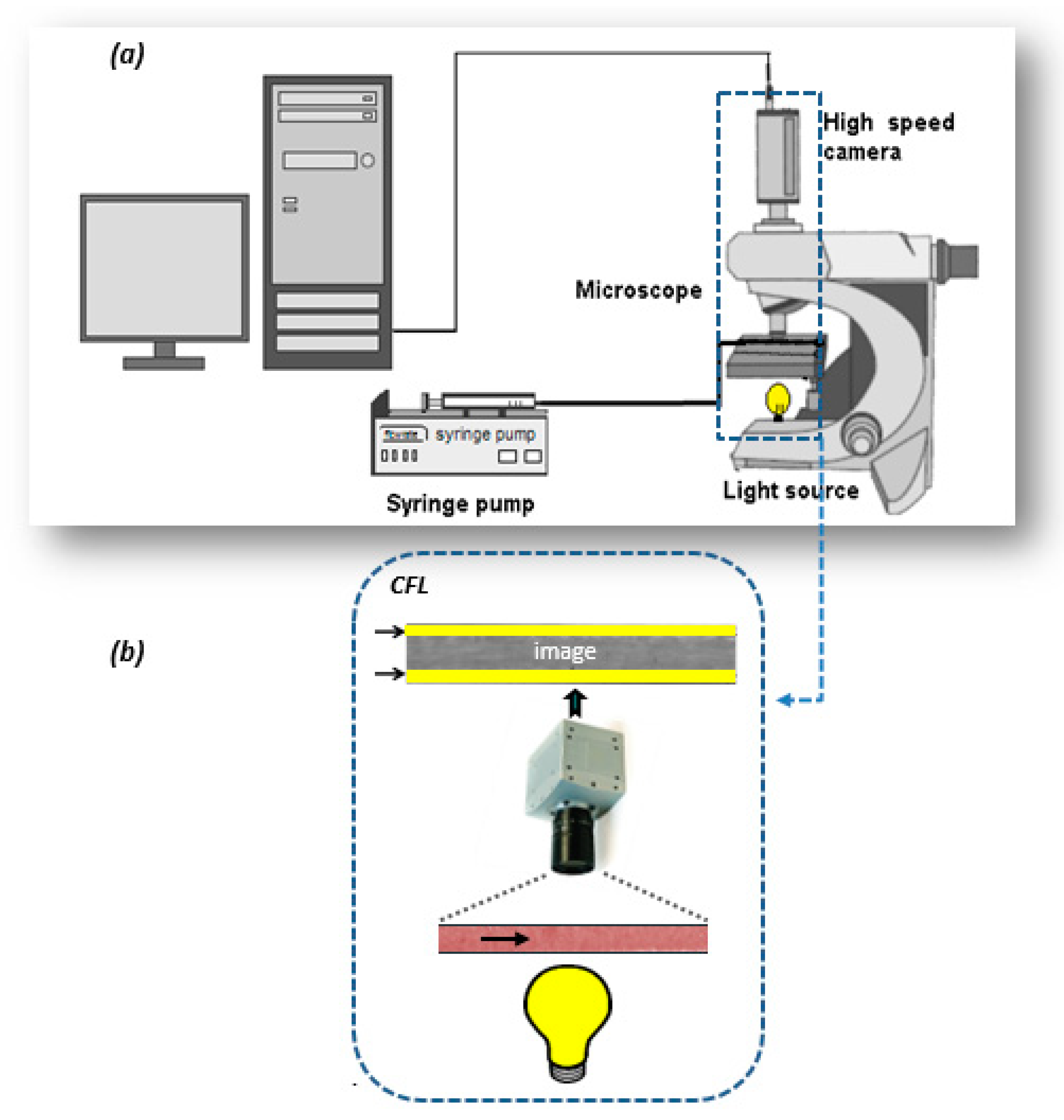
2. Experimental Procedure
- Separation of RBCs from plasma by centrifugation (at 3200 rpm) of the whole blood sample.
- Purification of the separated RBCs by washing with saline water and centrifuging twice.
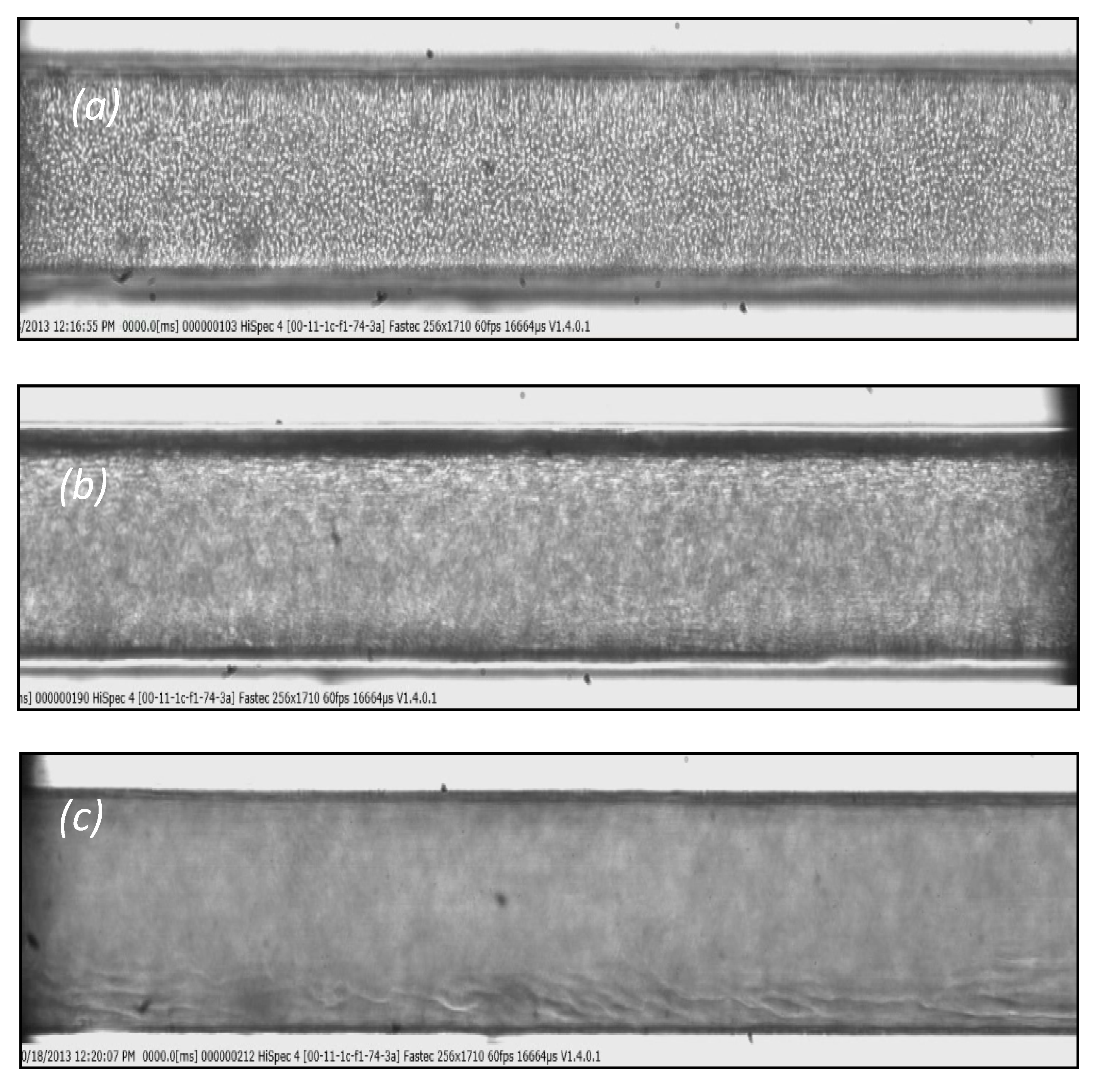
3. Cell Free Layer (CFL) and Velocity Measurements
- Spherical fluorescent particles (Invitrogen) with 1.1 μm mean diameter.
- RBCs coloured with fluorescent dye (Rhodamine B).
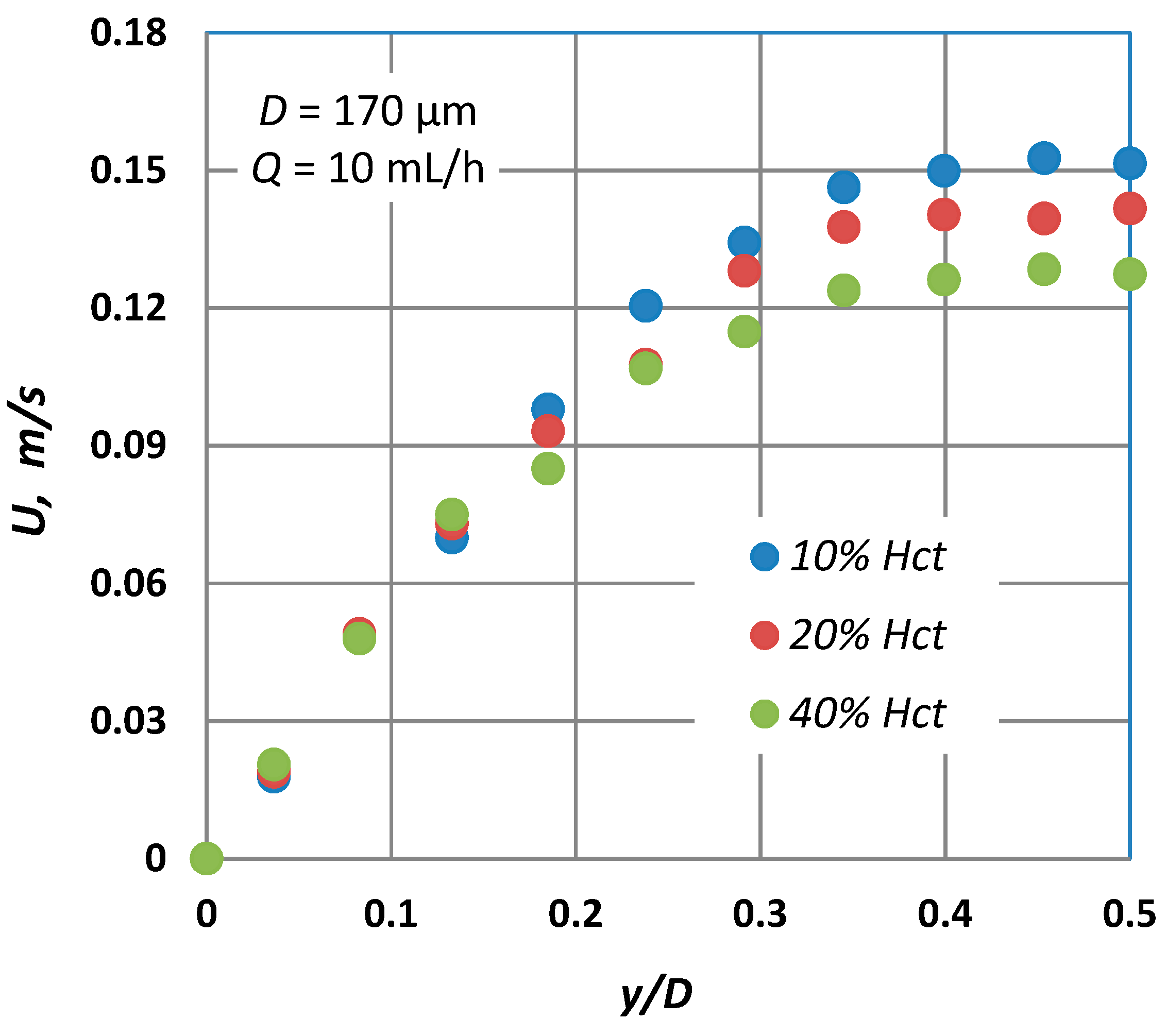
4. Experimental Results
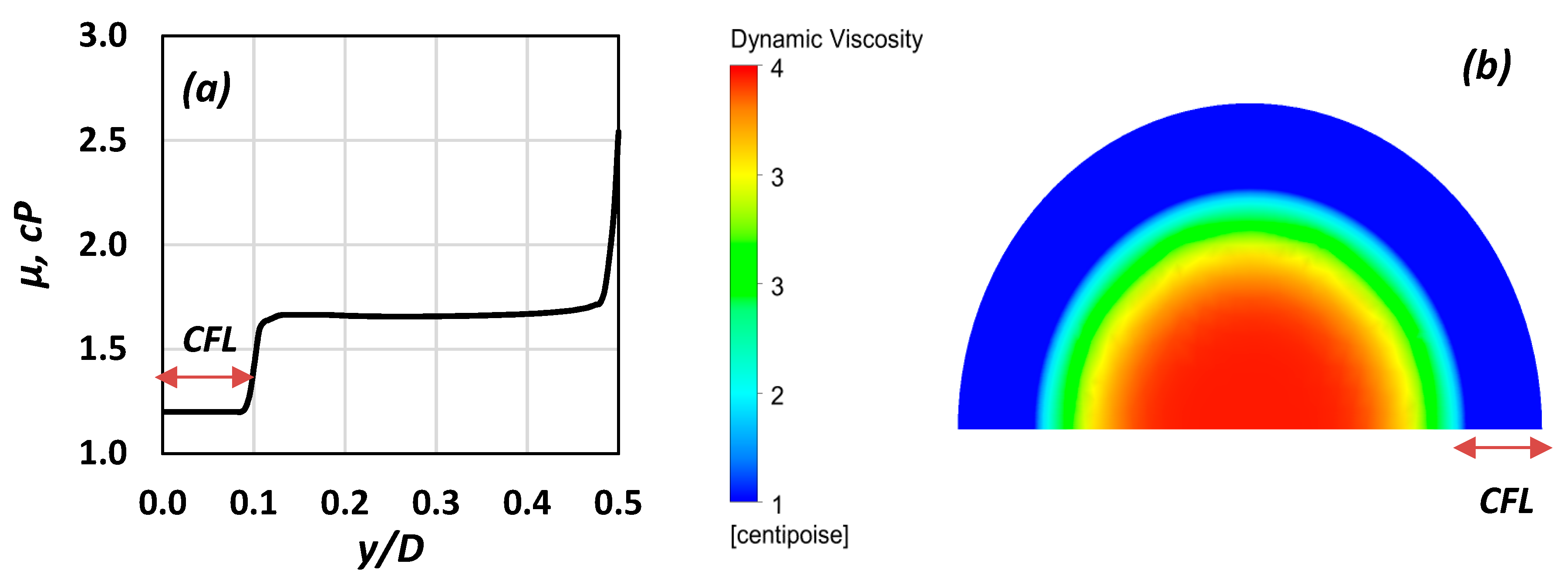
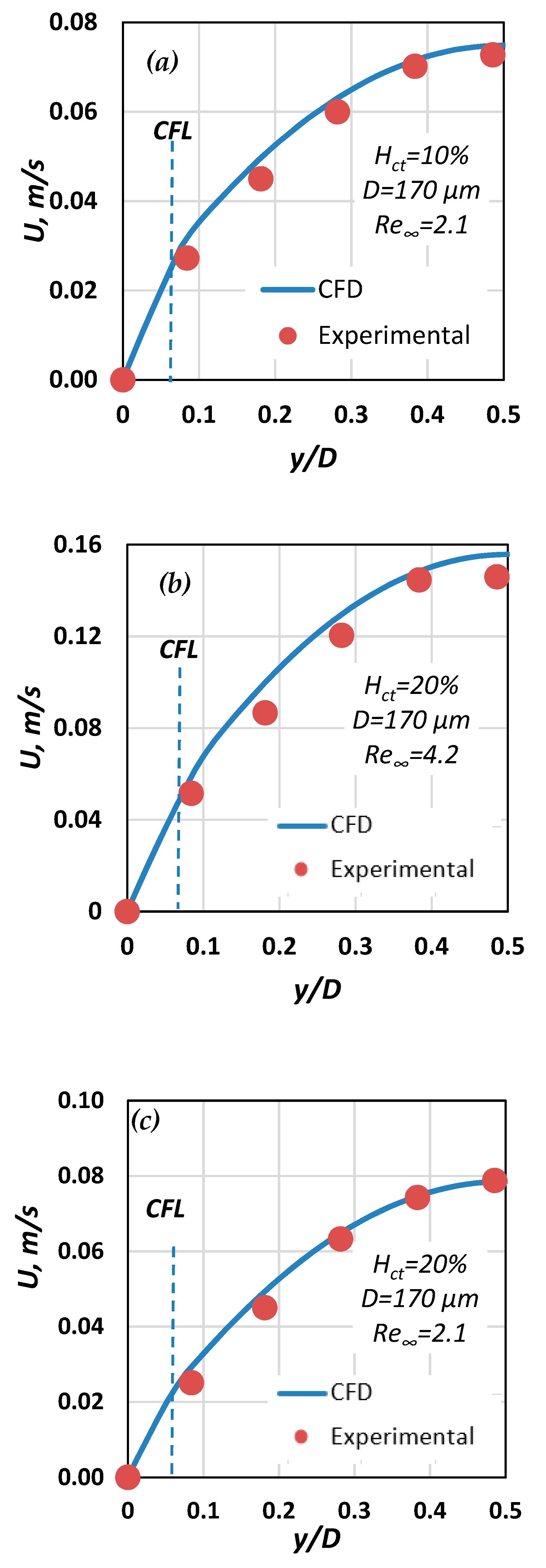
5. CFD Simulations
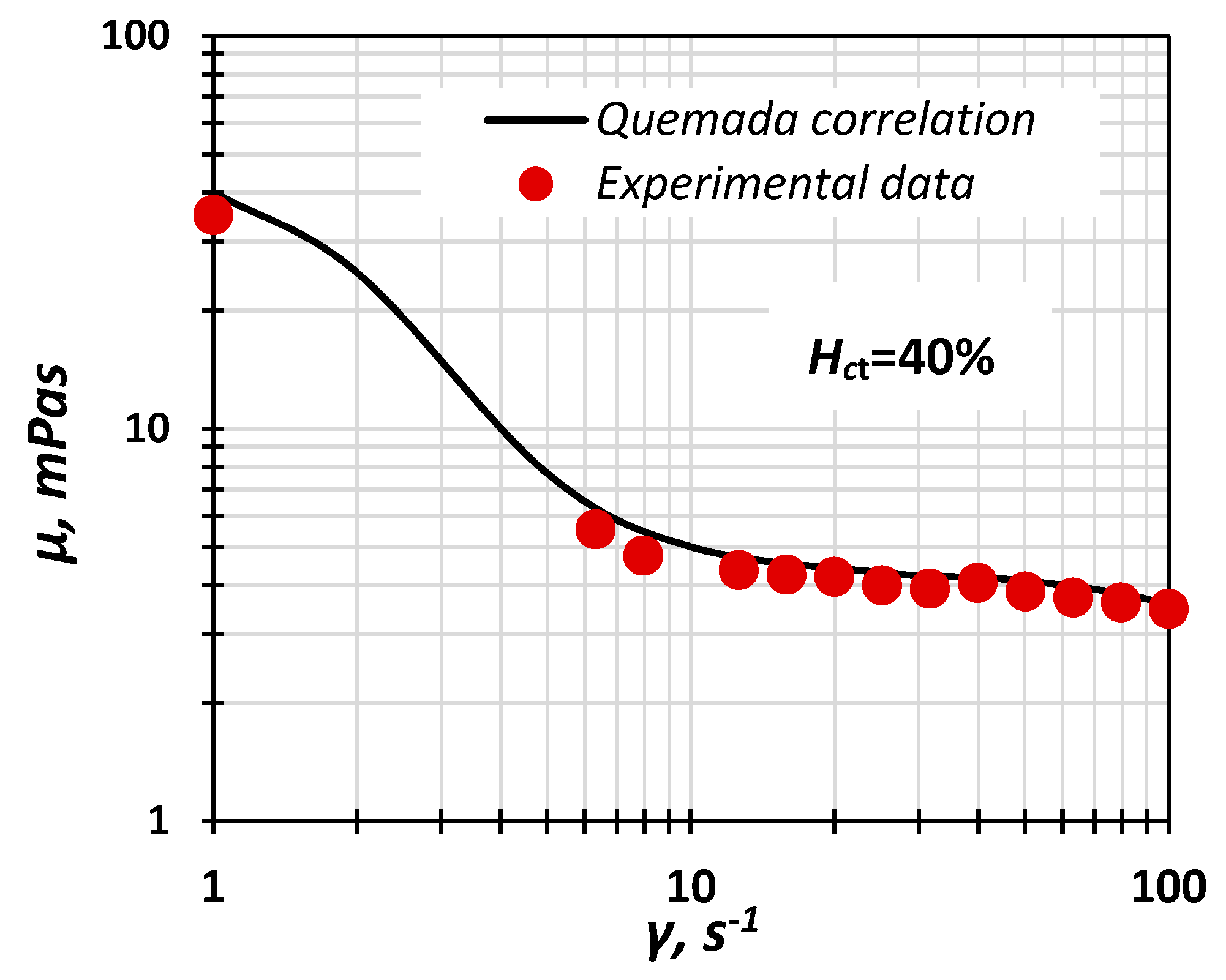
- The ΔPHP, calculated analytically using the Hagen–Poiseuille equation for straight circular tubes and considering the blood as a Newtonian fluid (e.g., μ∞ = 3.5 cP for Hct = 40%).
- The ΔPnCFL calculated numerically considering that there is no CFL and that the blood behaves as a non-Newtonian fluid (in the same way the non-Newtonian core was modelled) [29].
- The ΔP[10] calculated iteratively using the equations proposed in [10], considering blood as a Casson fluid.
6. Conclusions
Author Contributions
Funding
Conflicts of Interest
Nomenclature
| Q | volumetric flow rate, mL/h |
| U | blood axial velocity, m/s |
| Ut | settling velocity, m/s |
| g | acceleration of gravity, m/s2 |
| CFL | cell free layer width, m |
| D | hydraulic diameter, m |
| Hct | hematocrit, dimensionless |
| Re∞ | Reynolds number based on μ∞, dimensionless |
| Greek letters | |
| γ | shear rate, s−1 |
| γ* | pseudo shear rate, s−1 |
| ΔPCFL | numerically calculated pressure drop, Pa |
| ΔPnCFL | numerically calculated pressure drop without CFL modelling, Pa |
| ΔPHP | pressure drop calculated using the Hagen-Poiseuille correlation, Pa |
| ΔP[10] | pressure drop calculated using the correlation proposed in [10], Pa |
| μ | dynamic viscosity, Pa s |
| μ∞ | dynamic viscosity for high shear rates (asymptotic), Pa s |
| μp | viscosity of plasma, Pa s |
| ρp | particle density, kg/m3 |
| ρl | blood density, kg/m3 |
References
- Tuma, R.F.; Duran, W.N.; Ley, K. Microcirculation; Academic Press: Cambridge, MA, USA, 2011; ISBN 978-0-08-056993-2. [Google Scholar]
- Anastasiou, A.D.; Spyrogianni, A.S.; Koskinas, K.C.; Giannoglou, G.D.; Paras, S.V. Experimental investigation of the flow of a blood analogue fluid in a replica of a bifurcated small artery. Med. Eng. Phys. 2012, 34, 211–218. [Google Scholar] [CrossRef] [PubMed]
- Fåhræus, R.; Lindqvist, T. The viscosity of the blood in narrow capillary tubes. Am. J. Physiol.-Leg. Content 1931, 96, 562–568. [Google Scholar] [CrossRef]
- Albrecht, K.H.; Gaehtgens, P.; Pries, A.; Heuser, M. The Fahraeus effect in narrow capillaries (i.d. 3.3 to 11.0 μm). Microvasc. Res. 1979, 18, 33–47. [Google Scholar] [CrossRef]
- Ong, P.K.; Namgung, B.; Johnson, P.C.; Kim, S. Effect of erythrocyte aggregation and flow rate on cell-free layer formation in arterioles. Am. J. Physiol. Heart Circ. Physiol. 2010, 298, H1870–H1878. [Google Scholar] [CrossRef] [PubMed]
- Namgung, B.; Ju, M.; Cabrales, P.; Kim, S. Two-phase model for prediction of cell-free layer width in blood flow. Microvasc. Res. 2013, 85, 68–76. [Google Scholar] [CrossRef][Green Version]
- Pries, A.R.; Schönfeld, D.; Gaehtgens, P.; Kiani, M.F.; Cokelet, G.R. Diameter variability and microvascular flow resistance. Am. J. Physiol. Heart Circ. Physiol. 1997, 272, H2716–H2725. [Google Scholar] [CrossRef]
- Sriram, K.; Intaglietta, M.; Tartakovsky, D.M. Non-Newtonian Flow of Blood in Arterioles: Consequences for Wall Shear Stress Measurements. Microcirculation 2014, 21, 628–639. [Google Scholar] [CrossRef]
- Balogh, P.; Bagchi, P. Three-dimensional distribution of wall shear stress and its gradient in red cell-resolved computational modeling of blood flow in in vivo-like microvascular networks. Physiol. Rep. 2019, 7, e14067. [Google Scholar] [CrossRef]
- Chandran, K.B.; Rittgers, S.E.; Yoganathan, A.P.; Rittgers, S.E.; Yoganathan, A.P. Biofluid Mechanics: The Human Circulation, 2nd ed.; CRC Press: Boca Raton, MA, USA, 2012; ISBN 978-0-429-10607-1. [Google Scholar]
- Lauri, J.; Bykov, A.; Fabritius, T. Quantification of cell-free layer thickness and cell distribution of blood by optical coherence tomography. J. Biomed. Opt. 2016, 21, 040501. [Google Scholar] [CrossRef]
- Sriram, K.; Vázquez, B.Y.S.; Yalcin, O.; Johnson, P.C.; Intaglietta, M.; Tartakovsky, D.M. The effect of small changes in hematocrit on nitric oxide transport in arterioles. Antioxid. Redox Signal. 2011, 14, 175–185. [Google Scholar] [CrossRef]
- Al-Khazraji, B.K.; Jackson, D.N.; Goldman, D. A Microvascular Wall Shear Rate Function Derived From In Vivo Hemodynamic and Geometric Parameters in Continuously Branching Arterioles. Microcirculation 2016, 23, 311–319. [Google Scholar] [CrossRef] [PubMed]
- Pitts, K.L.; Fenech, M. High speed versus pulsed images for micro-particle image velocimetry: A direct comparison of red blood cells versus fluorescing tracers as tracking particles. Physiol. Meas. 2013, 34, 1363–1374. [Google Scholar] [CrossRef] [PubMed]
- Sharan, M.; Popel, A.S. A two-phase model for flow of blood in narrow tubes with increased effective viscosity near the wall. Biorheology 2001, 38, 415–428. [Google Scholar] [PubMed]
- Kim, S.; Kong, R.L.; Popel, A.S.; Intaglietta, M.; Johnson, P.C. Temporal and spatial variations of cell-free layer width in arterioles. Am. J. Physiol. Heart Circ. Physiol. 2007, 293, H1526–H1535. [Google Scholar] [CrossRef] [PubMed]
- Das, B.; Johnson, P.C.; Popel, A.S. Effect of nonaxisymmetric hematocrit distribution on non-Newtonian blood flow in small tubes. Biorheology 1998, 35, 69–87. [Google Scholar] [CrossRef]
- Batchelor, G.K. An Introduction to Fluid Dynamics; Cambridge University Press: Cambridge, UK, 2000; ISBN 978-0-521-66396-0. [Google Scholar]
- Wereley, S.T.; Meinhart, C.D. Micron-Resolution Particle Image Velocimetry. In Microscale Diagnostic Techniques; Breuer, K.S., Ed.; Springer: Berlin/Heidelberg, Germany, 2005; pp. 51–112. ISBN 978-3-540-26449-1. [Google Scholar]
- Fedosov, D.; Caswell, B.; Popel, A.S.; Karniadakis, G. Blood Flow and Cell-Free Layer in Microvessels. Microcirculation 2010, 17, 615–628. [Google Scholar] [CrossRef]
- Sriram, K.; Tsai, A.G.; Cabrales, P.; Meng, F.; Acharya, S.A.; Tartakovsky, D.M.; Intaglietta, M. PEG-albumin supraplasma expansion is due to increased vessel wall shear stress induced by blood viscosity shear thinning. Am. J. Physiol. Heart Circ. Physiol. 2012, 302, H2489–H2497. [Google Scholar] [CrossRef]
- Lima, R.; Wada, S.; Takeda, M.; Tsubota, K.; Yamaguchi, T. In vitro confocal micro-PIV measurements of blood flow in a square microchannel: The effect of the haematocrit on instantaneous velocity profiles. J. Biomech. 2007, 40, 2752–2757. [Google Scholar] [CrossRef]
- Box, G.E.P.; Wilson, K.B. On the Experimental Attainment of Optimum Conditions. J. R. Stat. Soc. Ser. B Methodol. 1951, 13, 1–45. [Google Scholar] [CrossRef]
- Reinke, W.; Gaehtgens, P.; Johnson, P.C. Blood viscosity in small tubes: Effect of shear rate, aggregation, and sedimentation. Am. J. Physiol. 1987, 253, H540–H547. [Google Scholar] [CrossRef]
- Fåhraeus, R. The suspension stability of the blood. Physiol. Rev. 1929, 9, 241–274. [Google Scholar] [CrossRef]
- Pries, A.R.; Secomb, T.W.; Gaehtgens, P.; Gross, J.F. Blood flow in microvascular networks. Experiments and simulation. Circ. Res. 1990, 67, 826–834. [Google Scholar] [CrossRef]
- Lih, M.M. A mathematical model for the axial migration of suspended particles in tube flow. Bull. Math. Biophys. 1969, 31, 143–157. [Google Scholar] [CrossRef]
- Gaehtgens, P.; Meiselman, H.J.; Wayland, H. Velocity profiles of human blood at normal and reduced hematocrit in glass tubes up to 130 μ diameter. Microvasc. Res. 1970, 2, 13–23. [Google Scholar] [CrossRef]
- Mouza, A.A.; Skordia, O.D.; Tzouganatos, I.D.; Paras, S.V. A Simplified Model for Predicting Friction Factors of Laminar Blood Flow in Small-Caliber Vessels. Fluids 2018, 3, 75. [Google Scholar] [CrossRef]



| Fluid | Red Blood Cell (RBC) | Saline | EDTA |
|---|---|---|---|
| H10 | 10 | 89.5 | 0.5 |
| H20 | 20 | 79.5 | 0.5 |
| H30 | 30 | 69.5 | 0.5 |
| H40 | 40 | 59.5 | 0.5 |
| Coefficient | Value | Coefficient | Value |
|---|---|---|---|
| a0 | 0.066384 | a11 | 0.000002 |
| a1 | −0.000887 | a22 | −0.001414 |
| a2 | 0.017796 | a12 | −0.000005 |
| Coefficient | Value | Coefficient | Value |
|---|---|---|---|
| 0.275363 | 0.100158 | ||
| 1.3435 | −2.803 | ||
| 2.711 | −0.6479 | ||
| −6.1508 | 27.923 | ||
| −25.60 | 3.697 |
| Maximum Cell Face Size (μm) | Inlet Pressure (Pa) |
|---|---|
| 5.0 | 2610 |
| 4.0 | 2578 |
| 3.0 | 2555 |
| 2.5 | 2553 |
| Re∞ | D (μm) | Hct (%) | ΔPCFL (Pa) | ΔPnCFL (Pa) | ΔPHP (Pa) | ΔP[10] (Pa) | ΔPnCFL/ΔPCFL | ΔPHP/ΔPCFL |
|---|---|---|---|---|---|---|---|---|
| 0.8 | 100 | 30 | 132 | 238 | 302 | 184 | 1.8 | 2.29 |
| 1.4 | 170 | 40 | 42 | 103 | 105 | 67 | 2.5 | 2.50 |
| 2.5 | 170 | 40 | 105 | 187 | 186 | 117 | 1.8 | 1.77 |
| 4.9 | 170 | 40 | 204 | 377 | 372 | 229 | 1.8 | 1.82 |
| 1.2 | 50 | 20 | 1463 | 2753 | 2984 | 1629 | 1.9 | 2.04 |
| 1.1 | 50 | 40 | 1466 | 2772 | 3256 | 1978 | 1.9 | 2.22 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Stergiou, Y.G.; Keramydas, A.T.; Anastasiou, A.D.; Mouza, A.A.; Paras, S.V. Experimental and Numerical Study of Blood Flow in μ-vessels: Influence of the Fahraeus–Lindqvist Effect. Fluids 2019, 4, 143. https://doi.org/10.3390/fluids4030143
Stergiou YG, Keramydas AT, Anastasiou AD, Mouza AA, Paras SV. Experimental and Numerical Study of Blood Flow in μ-vessels: Influence of the Fahraeus–Lindqvist Effect. Fluids. 2019; 4(3):143. https://doi.org/10.3390/fluids4030143
Chicago/Turabian StyleStergiou, Yorgos G., Aggelos T. Keramydas, Antonios D. Anastasiou, Aikaterini A. Mouza, and Spiros V. Paras. 2019. "Experimental and Numerical Study of Blood Flow in μ-vessels: Influence of the Fahraeus–Lindqvist Effect" Fluids 4, no. 3: 143. https://doi.org/10.3390/fluids4030143
APA StyleStergiou, Y. G., Keramydas, A. T., Anastasiou, A. D., Mouza, A. A., & Paras, S. V. (2019). Experimental and Numerical Study of Blood Flow in μ-vessels: Influence of the Fahraeus–Lindqvist Effect. Fluids, 4(3), 143. https://doi.org/10.3390/fluids4030143








