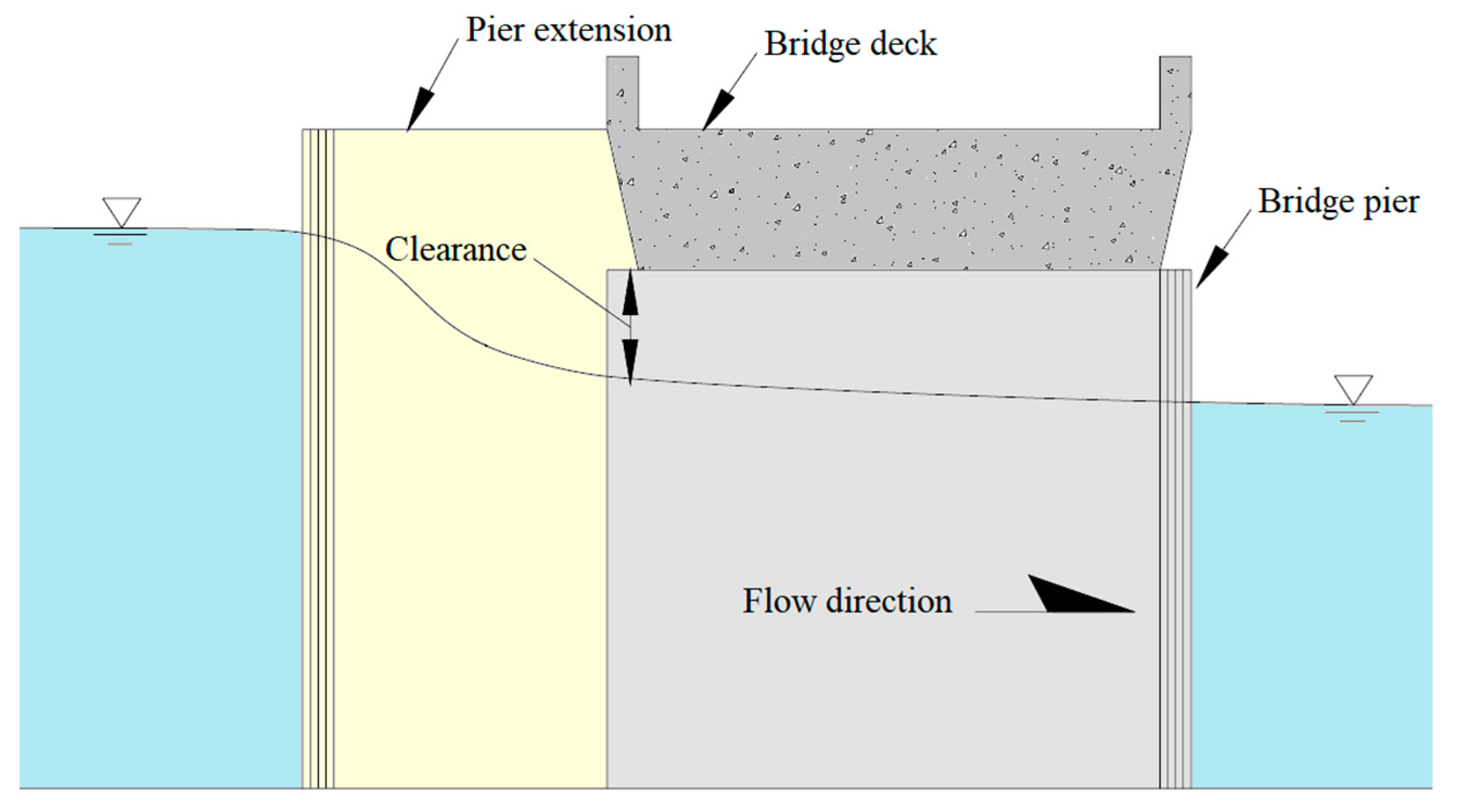
Bulbous Pier: An Alternative to Bridge Pier Extensions as a Countermeasure against Bridge Deck Splashing
Abstract
:1. Introduction
- Performing experiments in order to understand the impact of a bulbous on pier wave height;
- Developing an analytical model that predicts the effects of a bulbous pier on pier wave height based on the bulb’s geometry and location.
2. Materials and Methods
2.1. Theoretical Formulation
- The Froude number of the model (Frm) is the same as the Froude number of the flow around the pier (Frp).
- The wave-making coefficient of the bulb model (Cw) and the bulb (Cwp) are the same.
Viscous Resistance Error
2.2. Bridge Selection
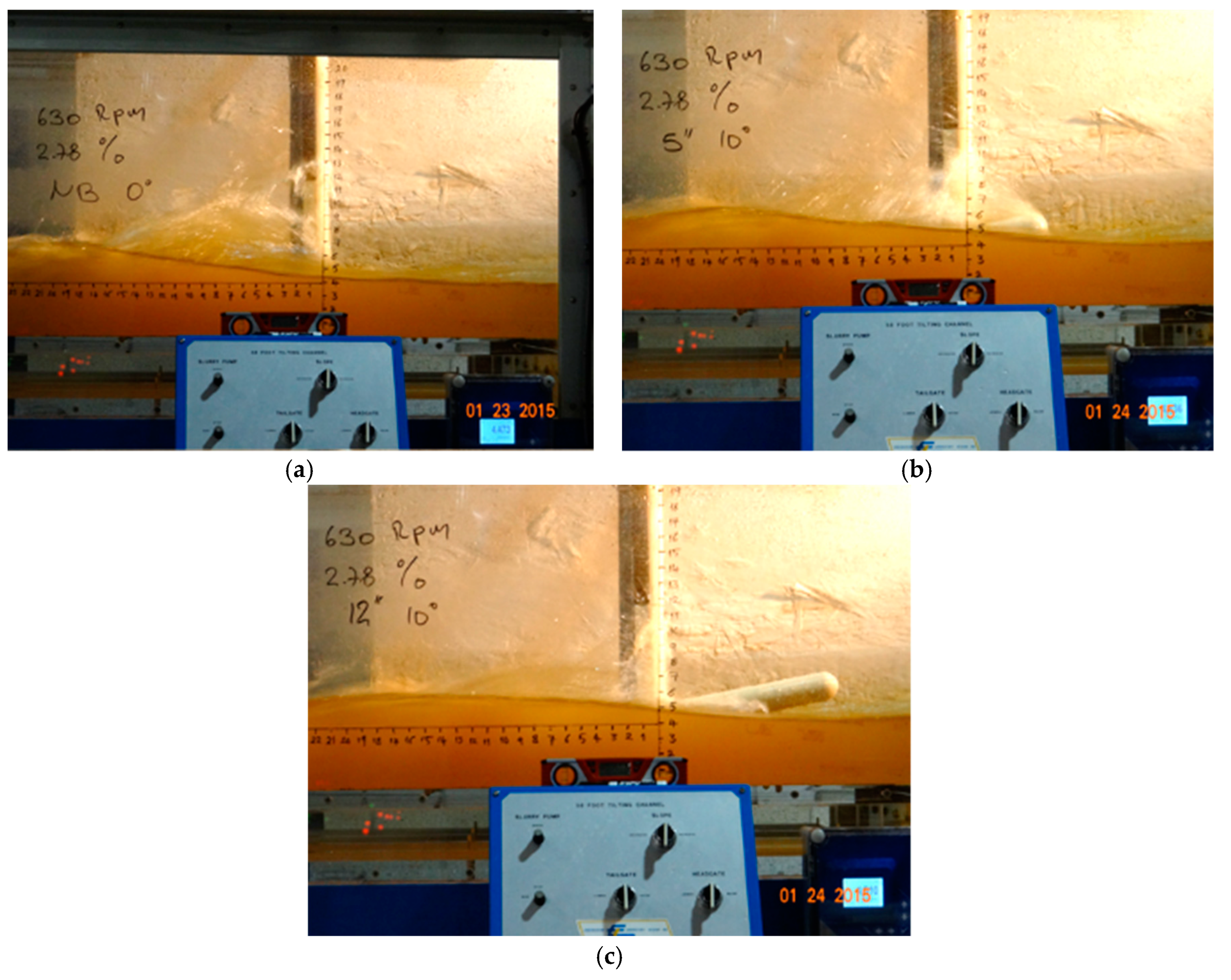
2.3. Flume Testing Plan
- Verifying the flume Manning’s roughness coefficient
- Obtaining the bulb optimal submerged depth
- Testing twelve bulb models for eight steady-state conditions in the flume (slope and the pump RPM), with the maximum hydraulic depth (Yh) of 0.375′
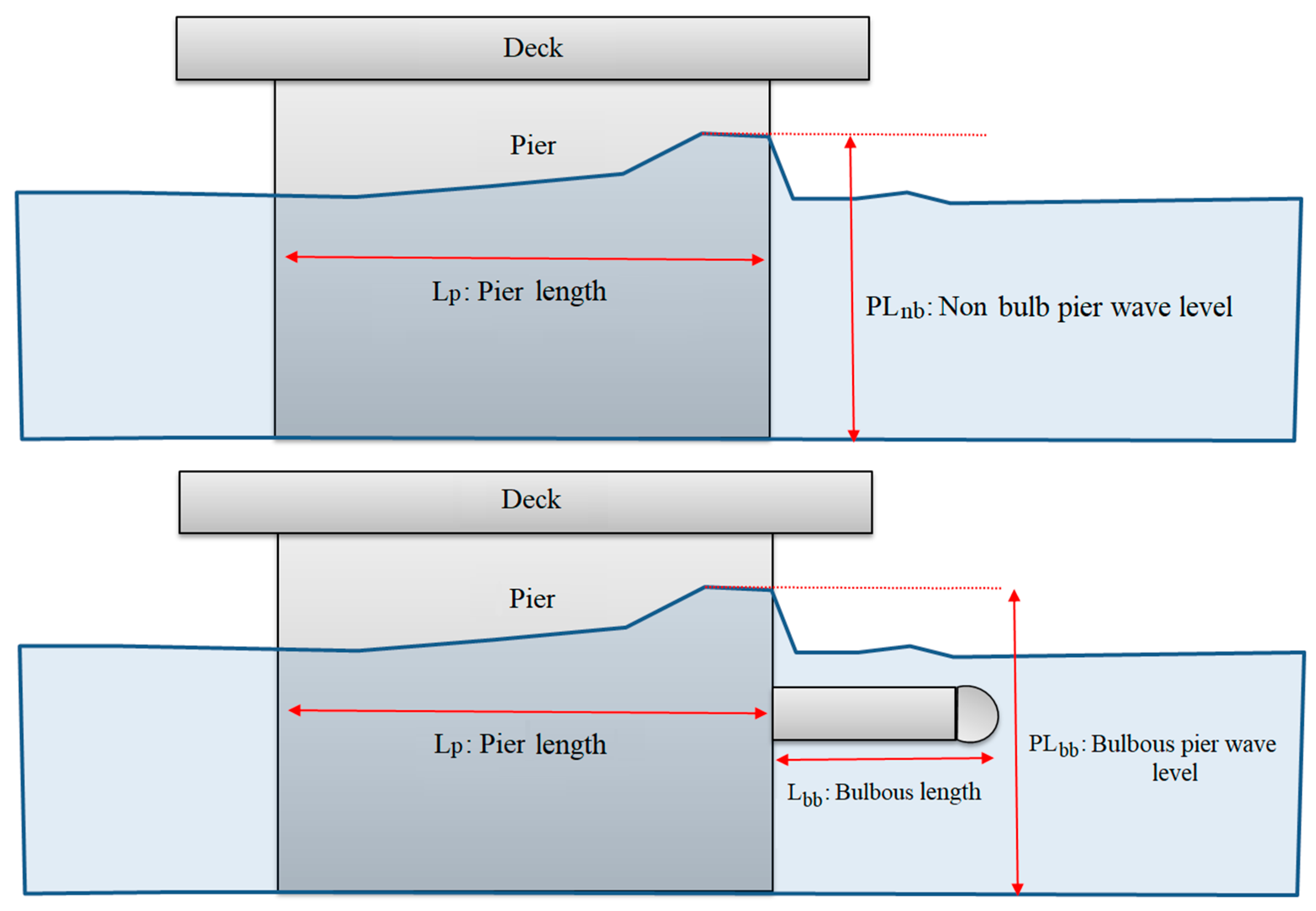
- An Endress + Hauser Liquicap capacitive level meter Liquidcap T FMI21; this equipment measured the pier level (PLnb, PLbb) (Figure 6).
- A Greyline area velocity flow meter, model AVFM 5.0; this equipment measured the flume flow rate (Q), velocity (v), and hydraulic depth (Yh). The instrument was located at the bottom of the flume in front of the pier nose, a few inches away from the bulb (Figure 7).
- A Measurement Computer USB-1608G Data Logger; this data logger collected the data coming from the pipe flow meter and level meter, as well as the three outputs from the AVFM 5.0: water velocity, water level, and flow rate.
- To scale, display, and record the data collected by the data logger, the Measurement Computer DasyLab application was used, and the data collected were stored in Excel CSV format.
2.3.1. Verifying the Manning’s Roughness Coefficient
2.3.2. Optimal Bulb Submerged Depth
2.3.3. Steady-State Test Matrix
2.4. Flume Capabilities
2.5. Dimensional Analysis
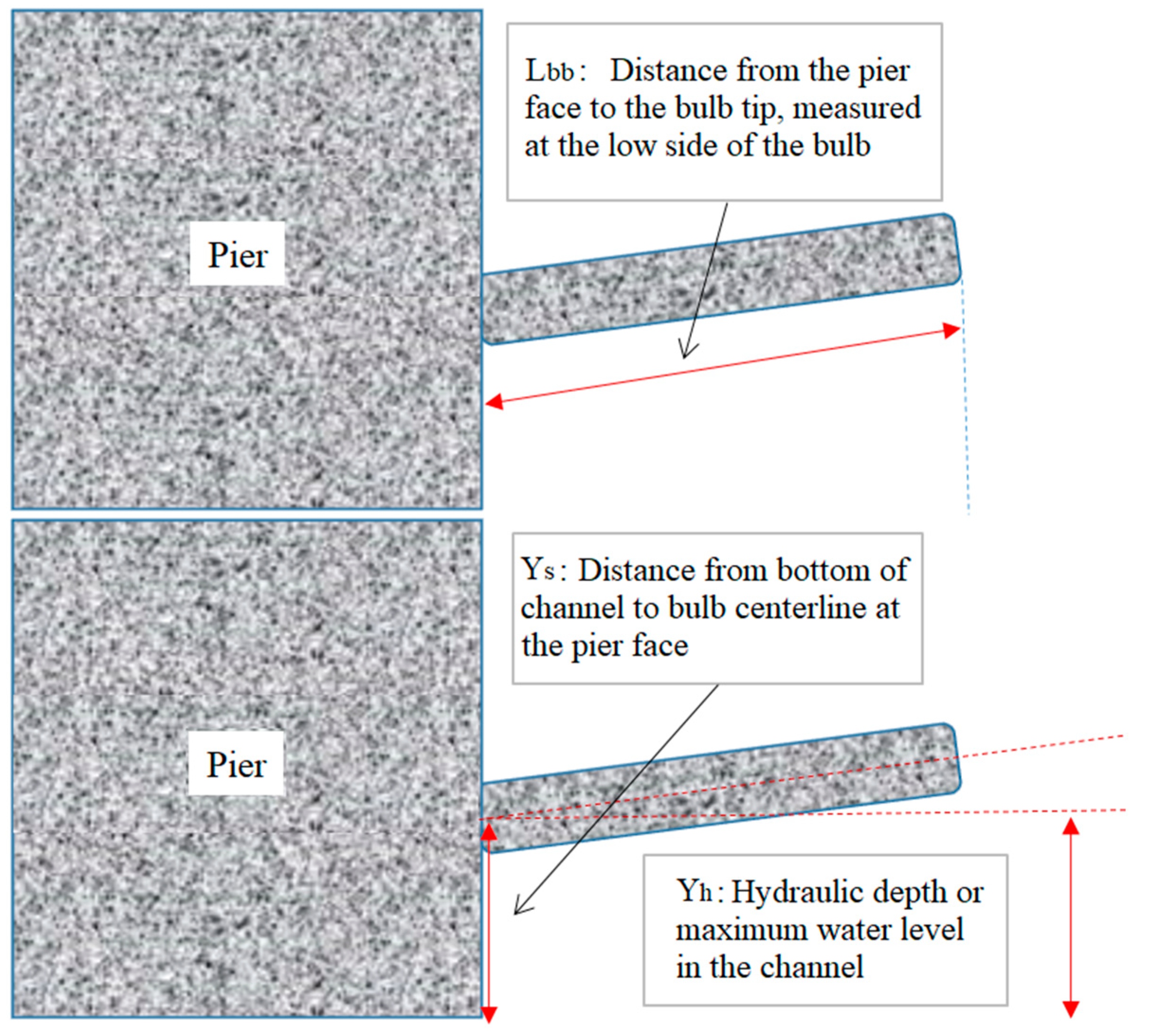
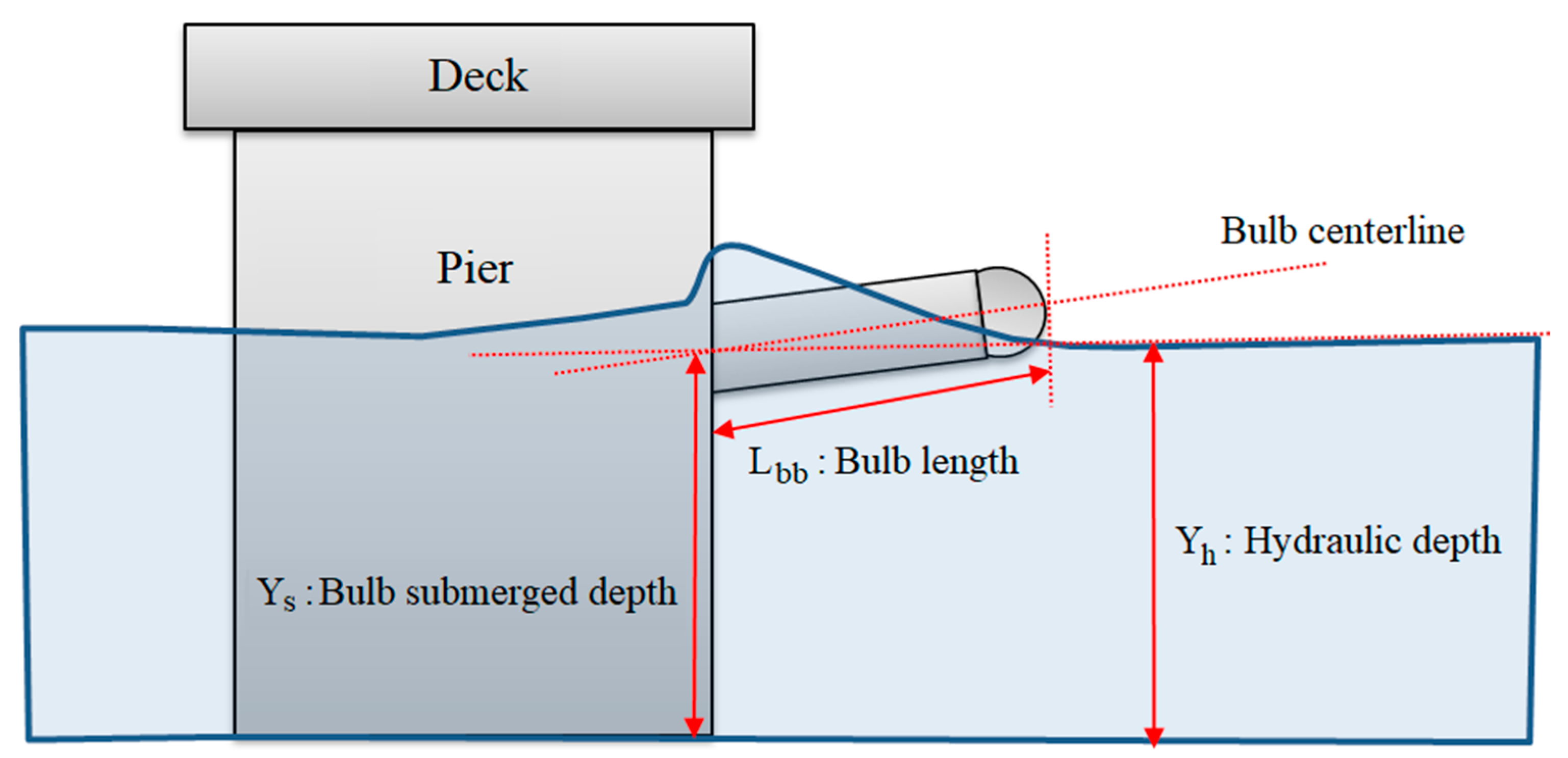
2.6. Bulbous Pier Geometry
3. Results and Discussion
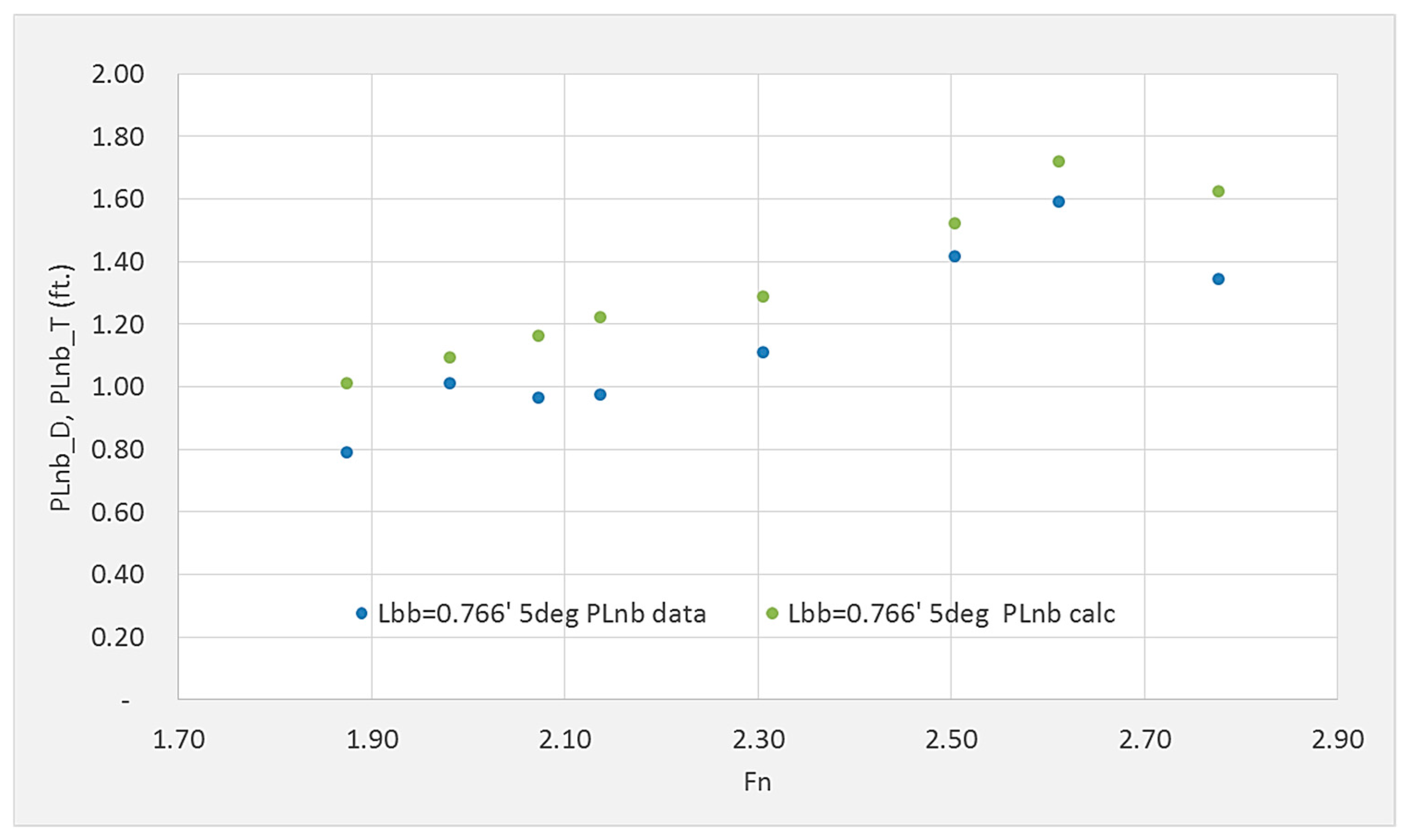
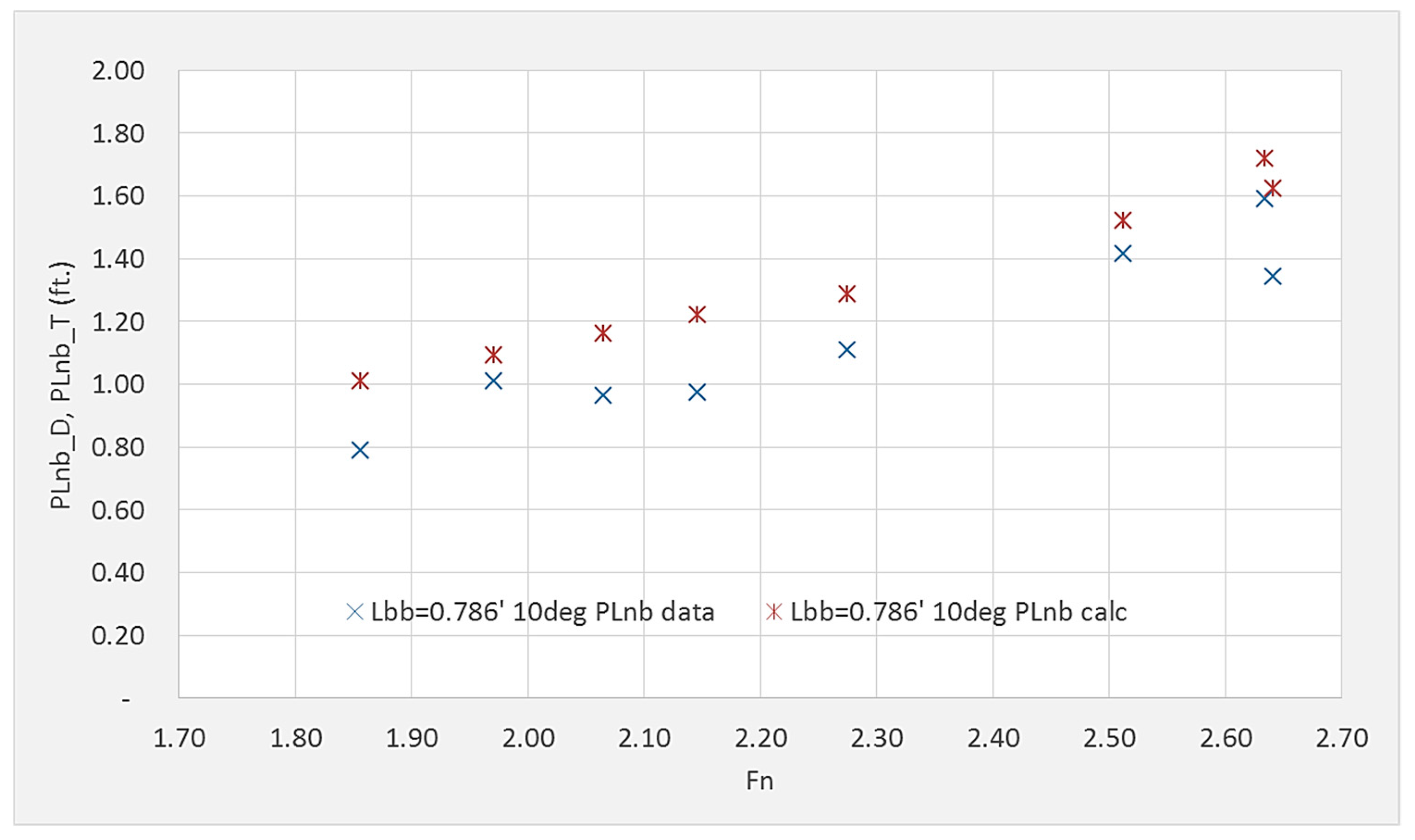
3.1. Pier Water Level and Bulbous Pier Water Level
3.2. Initial Assumptions
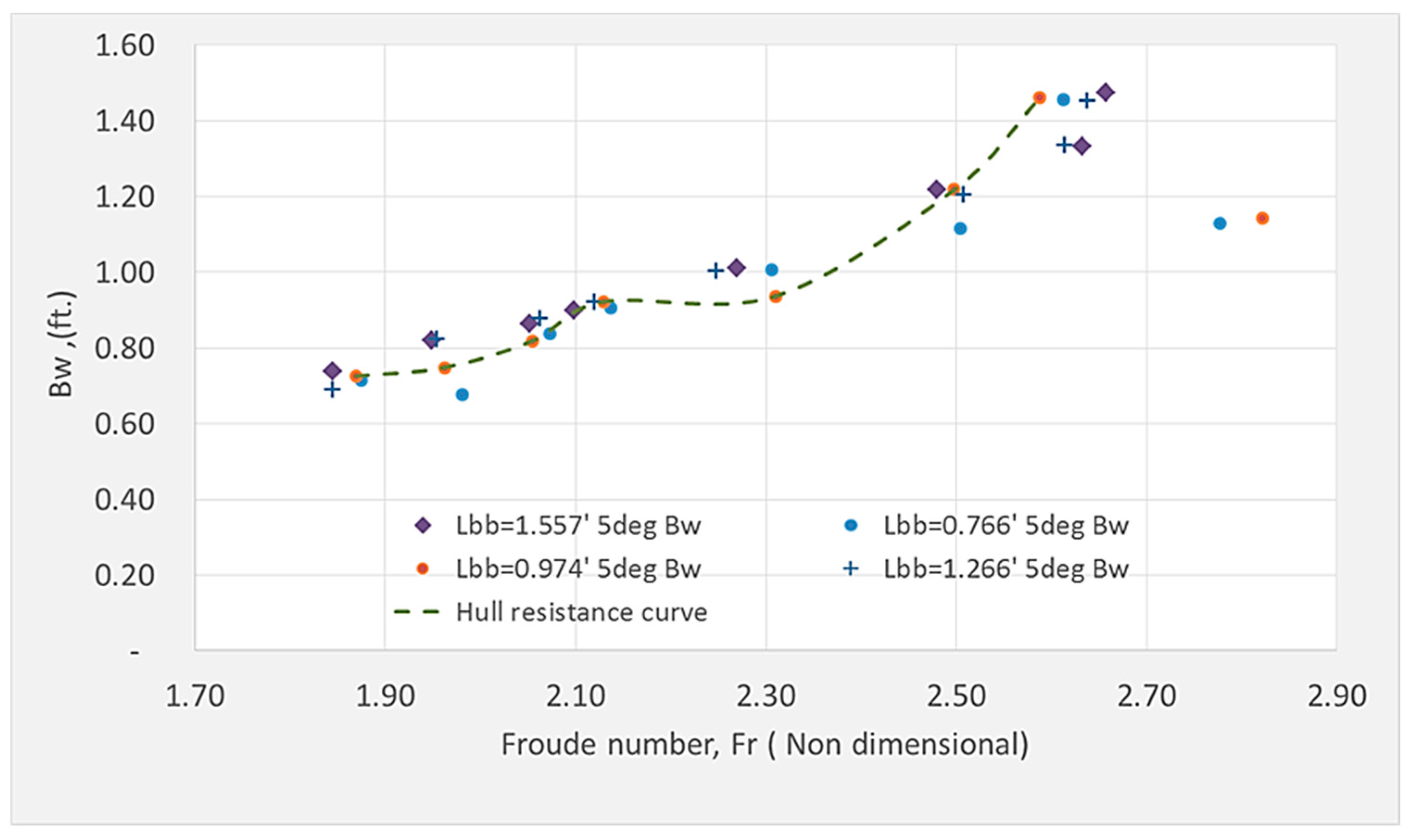
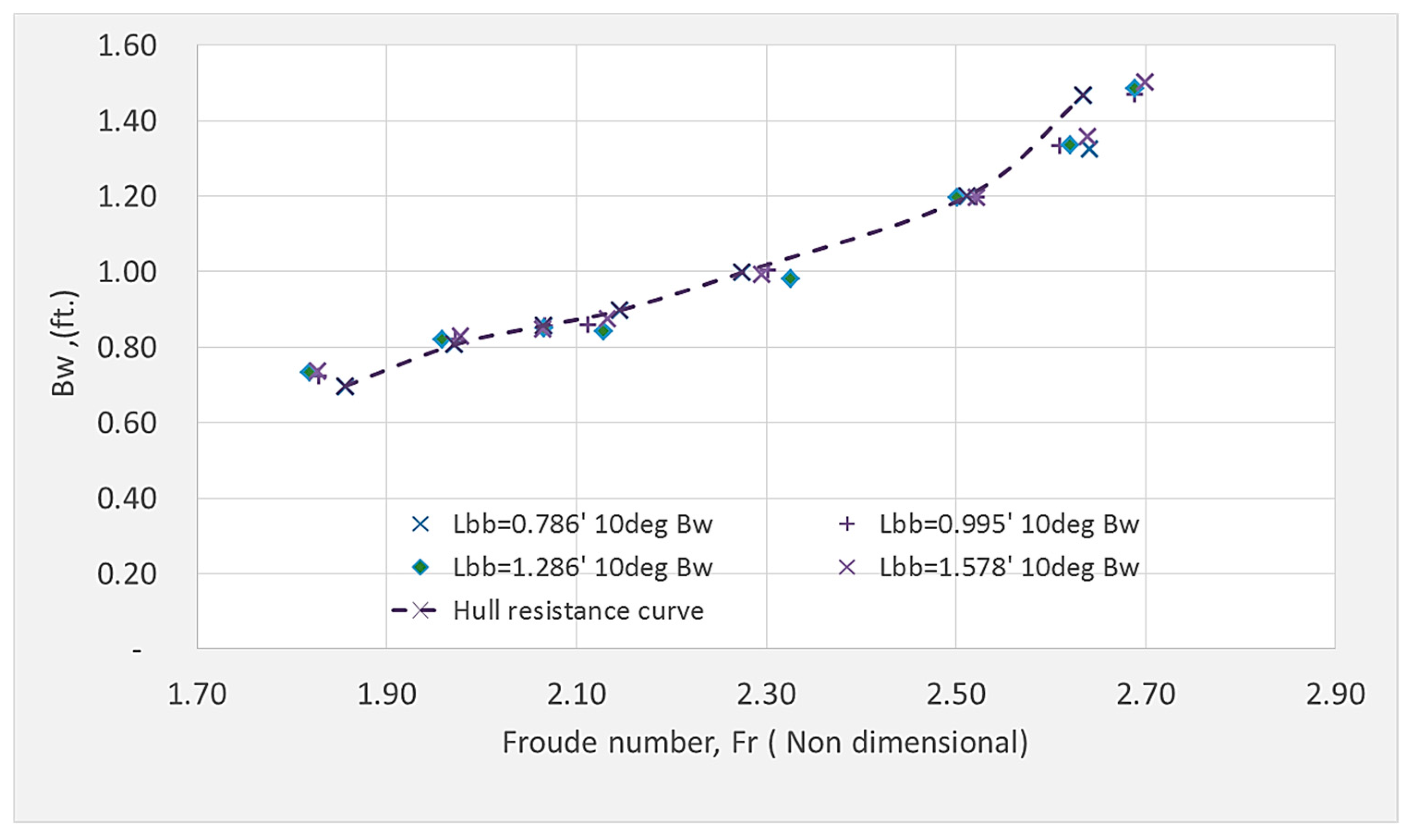
3.3. Bulbous Pier Water Level
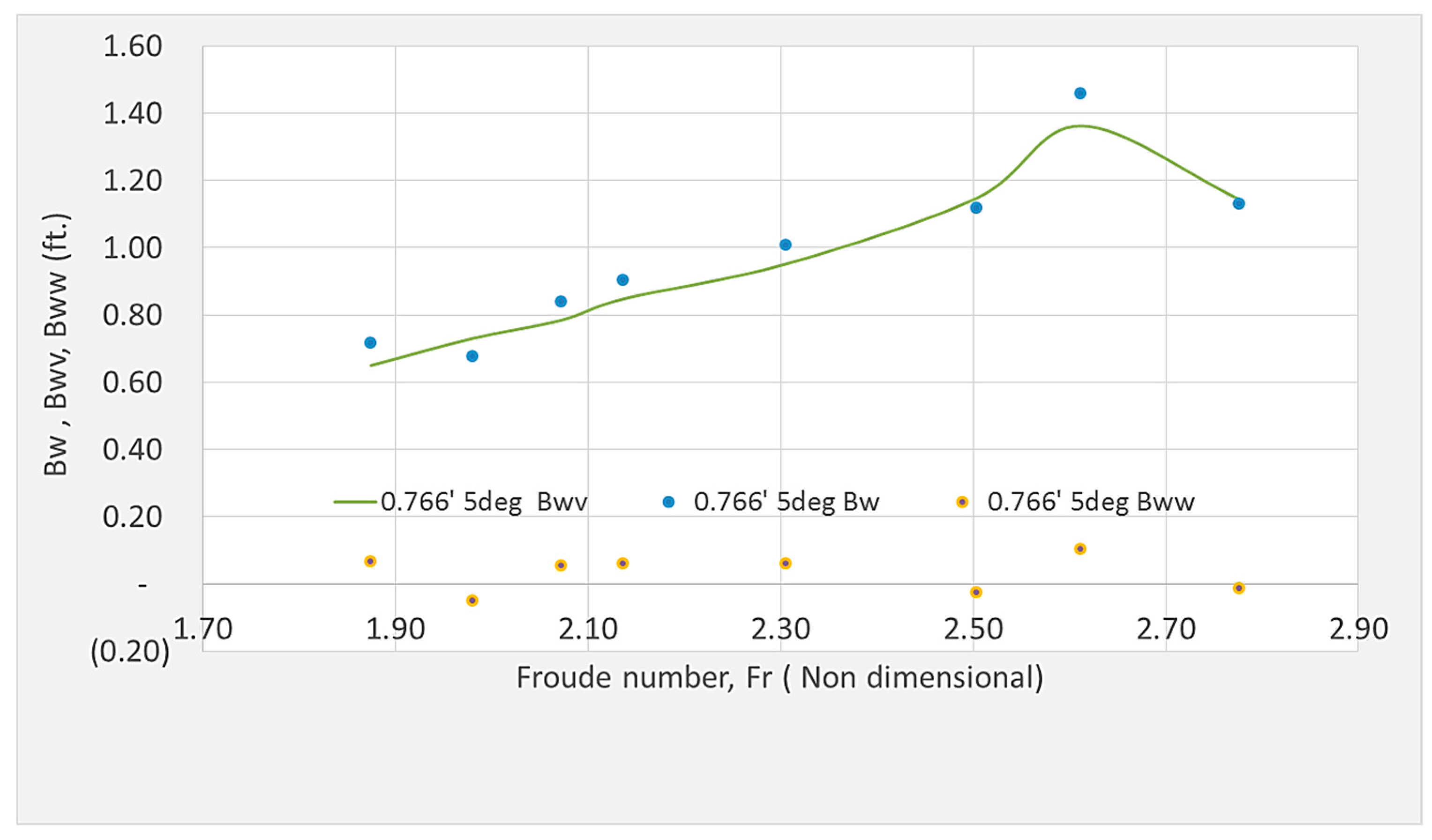
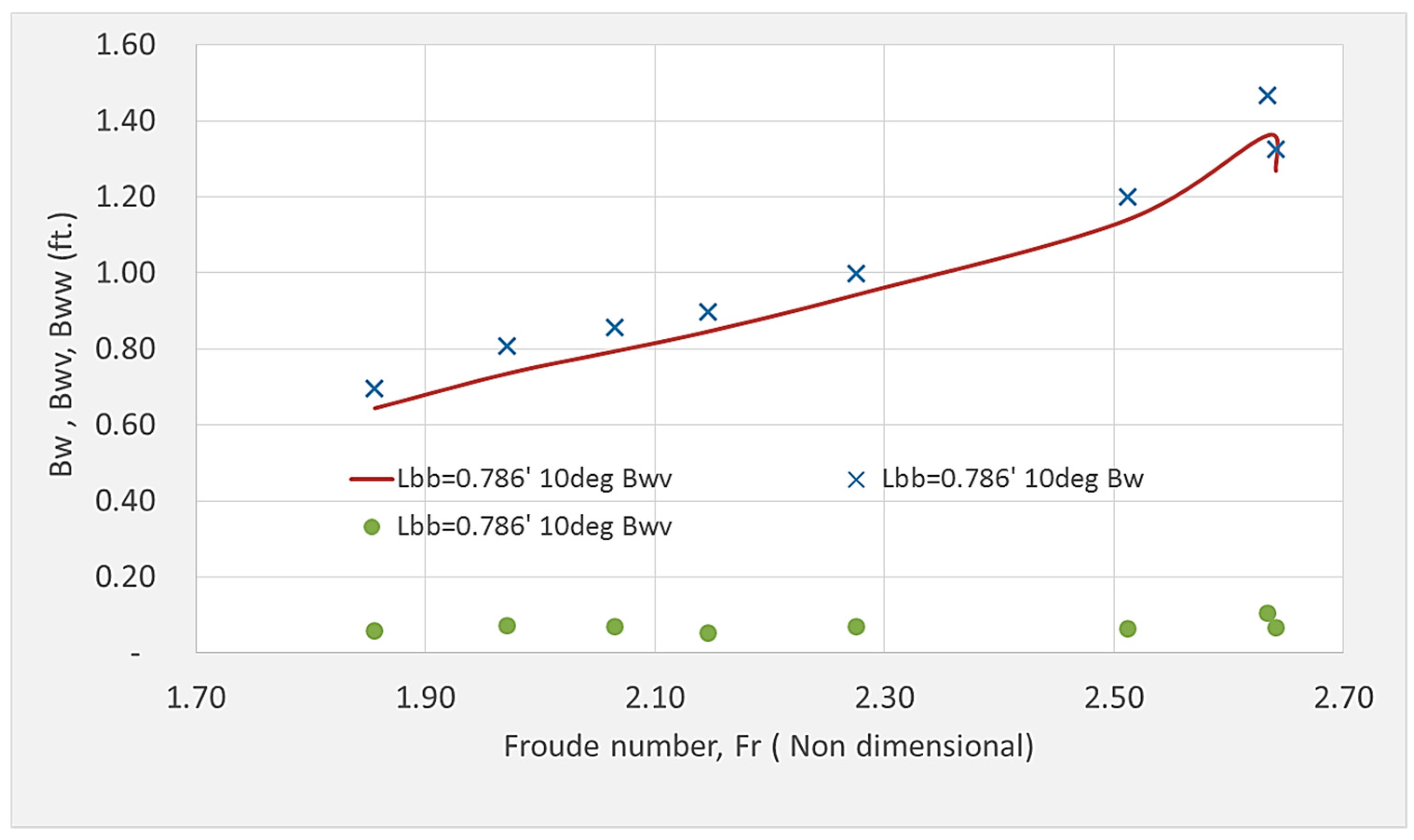
3.4. Bulb Wave
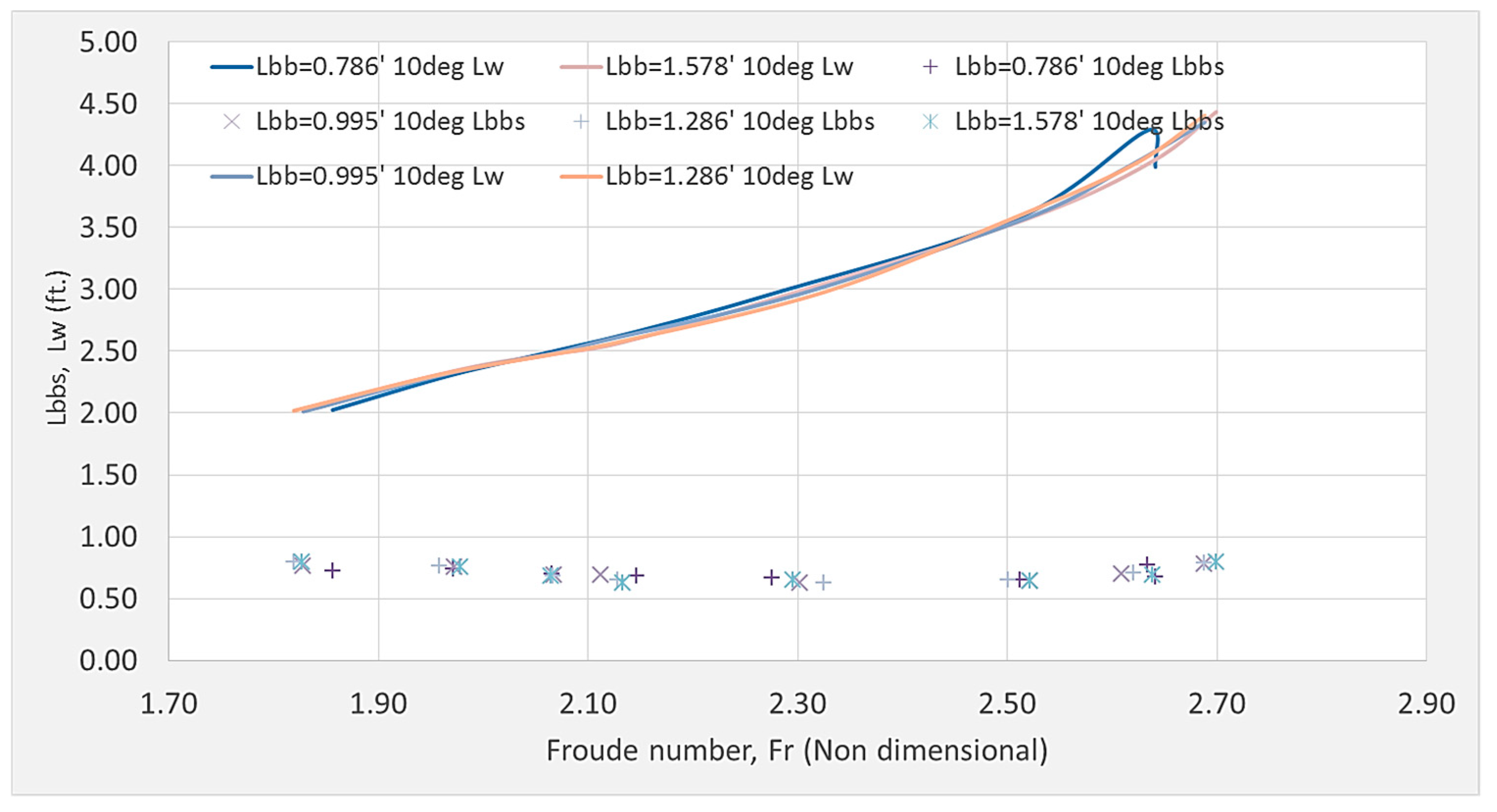
3.5. Bulbous Viscous and Wave-Making Resistance
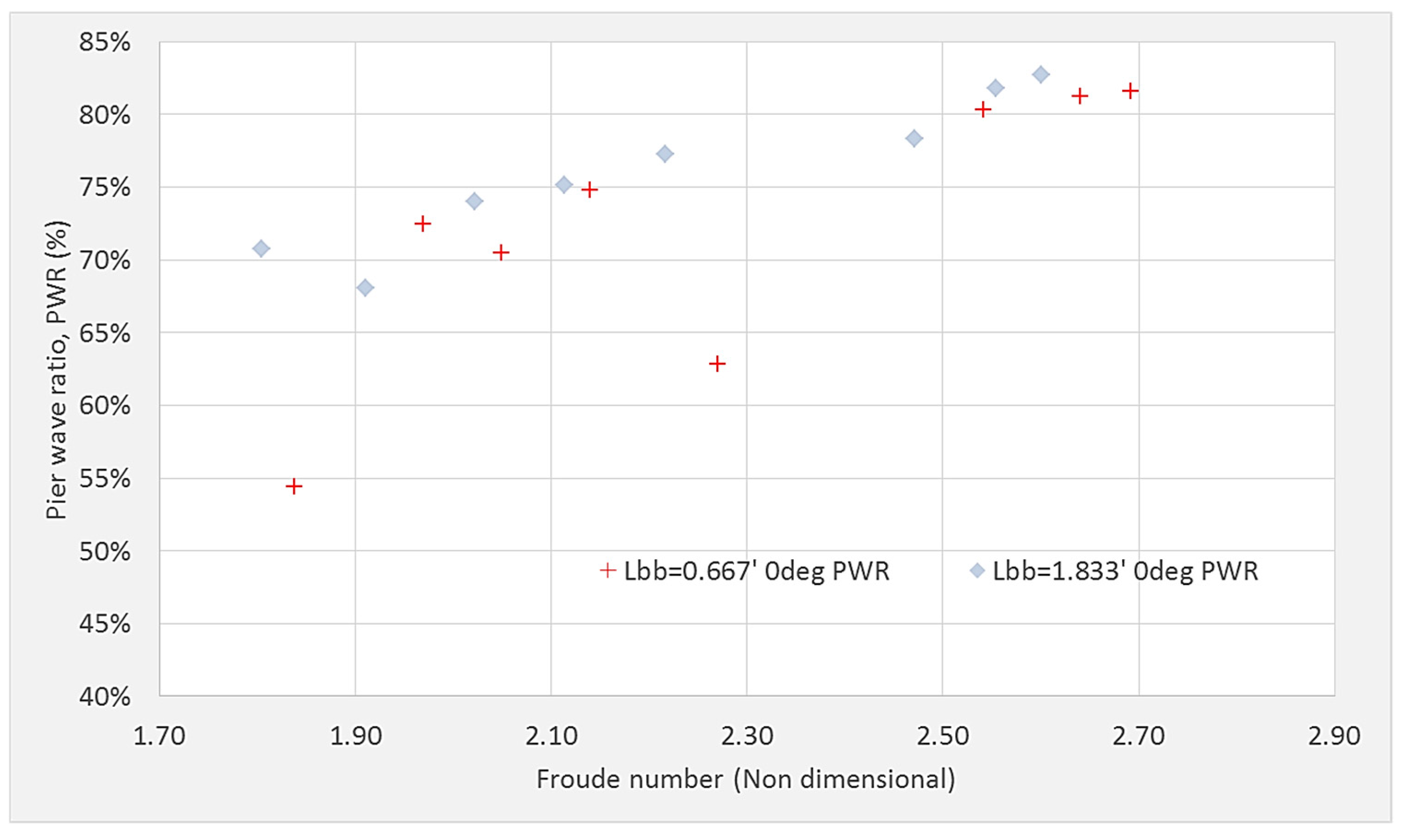
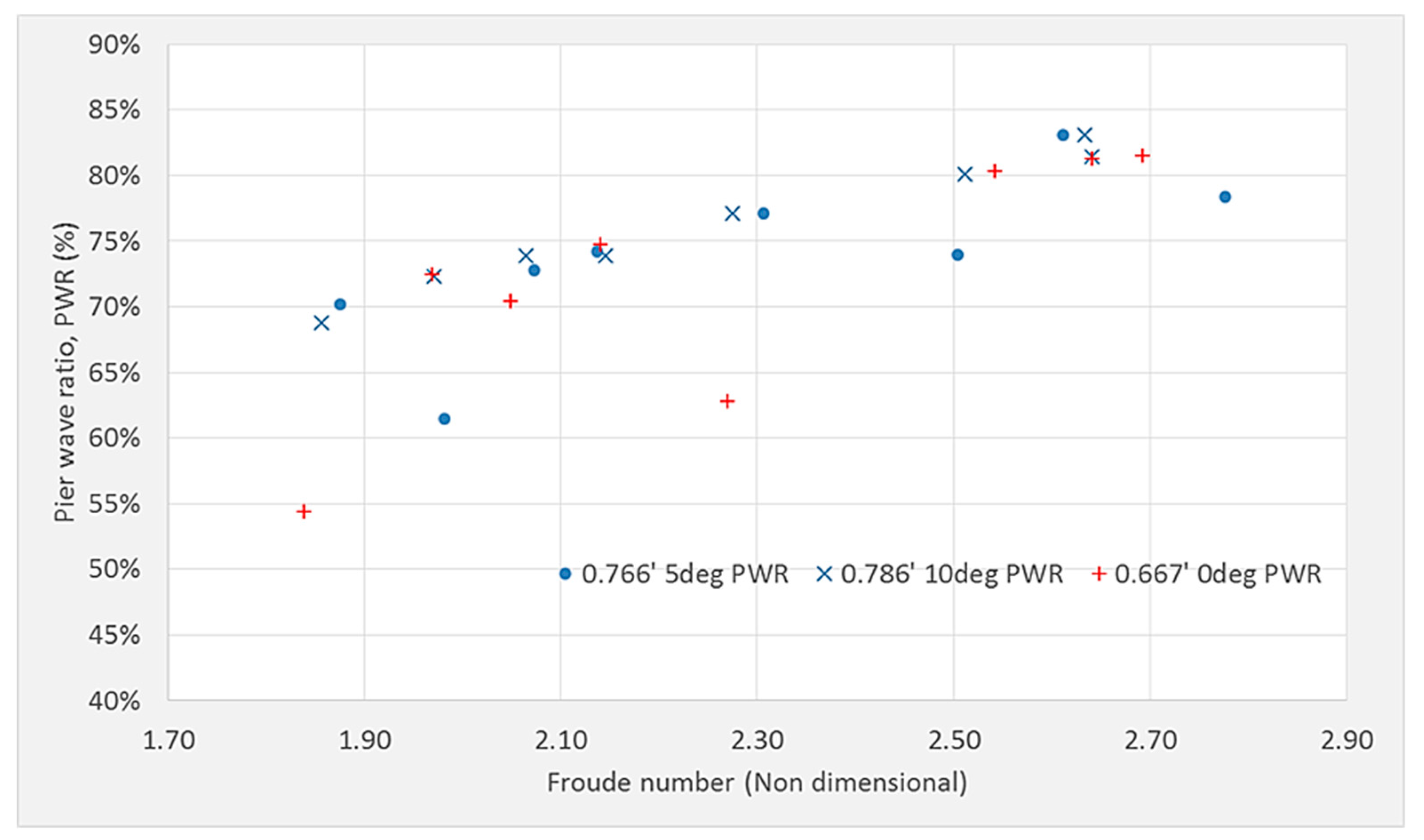
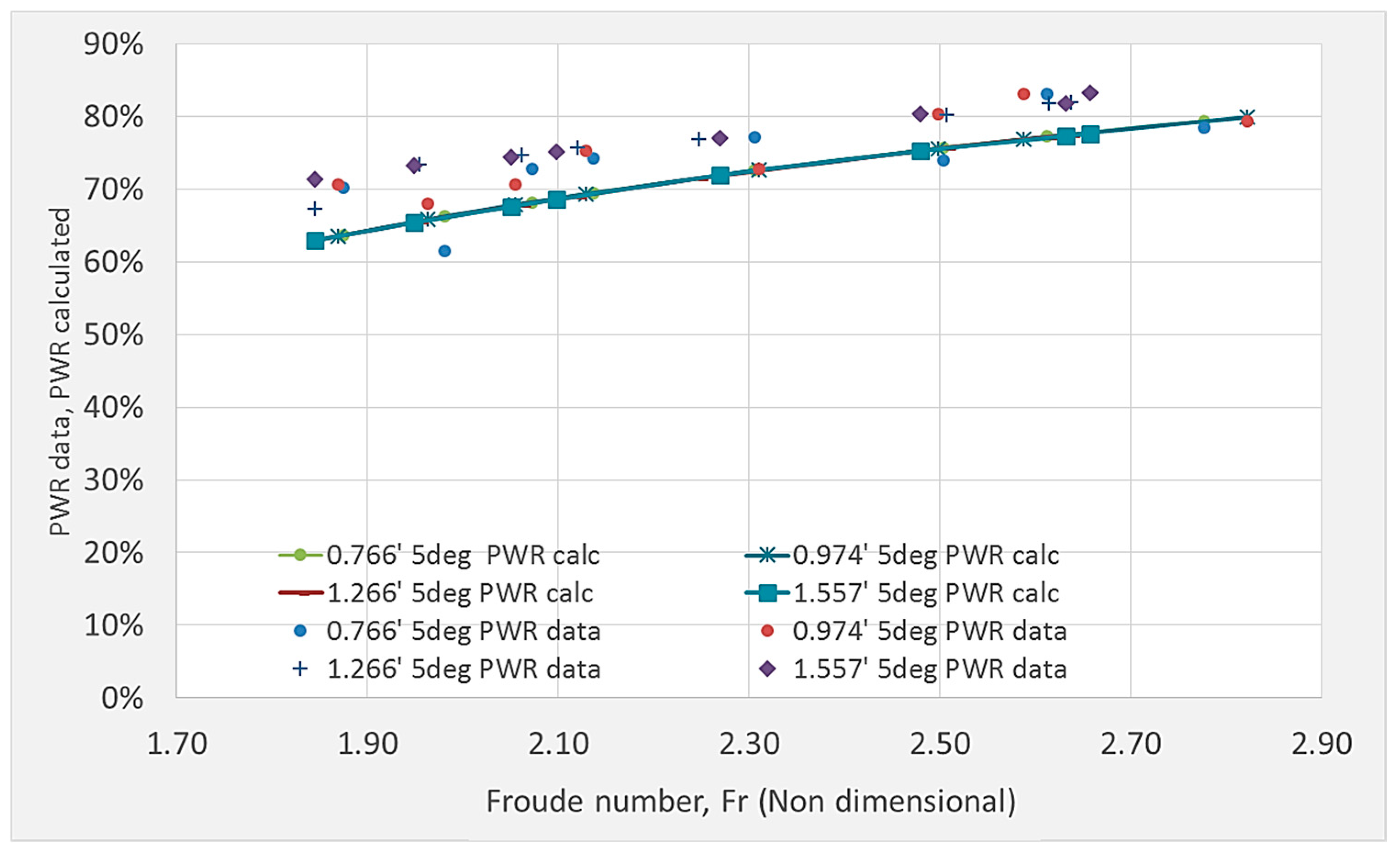
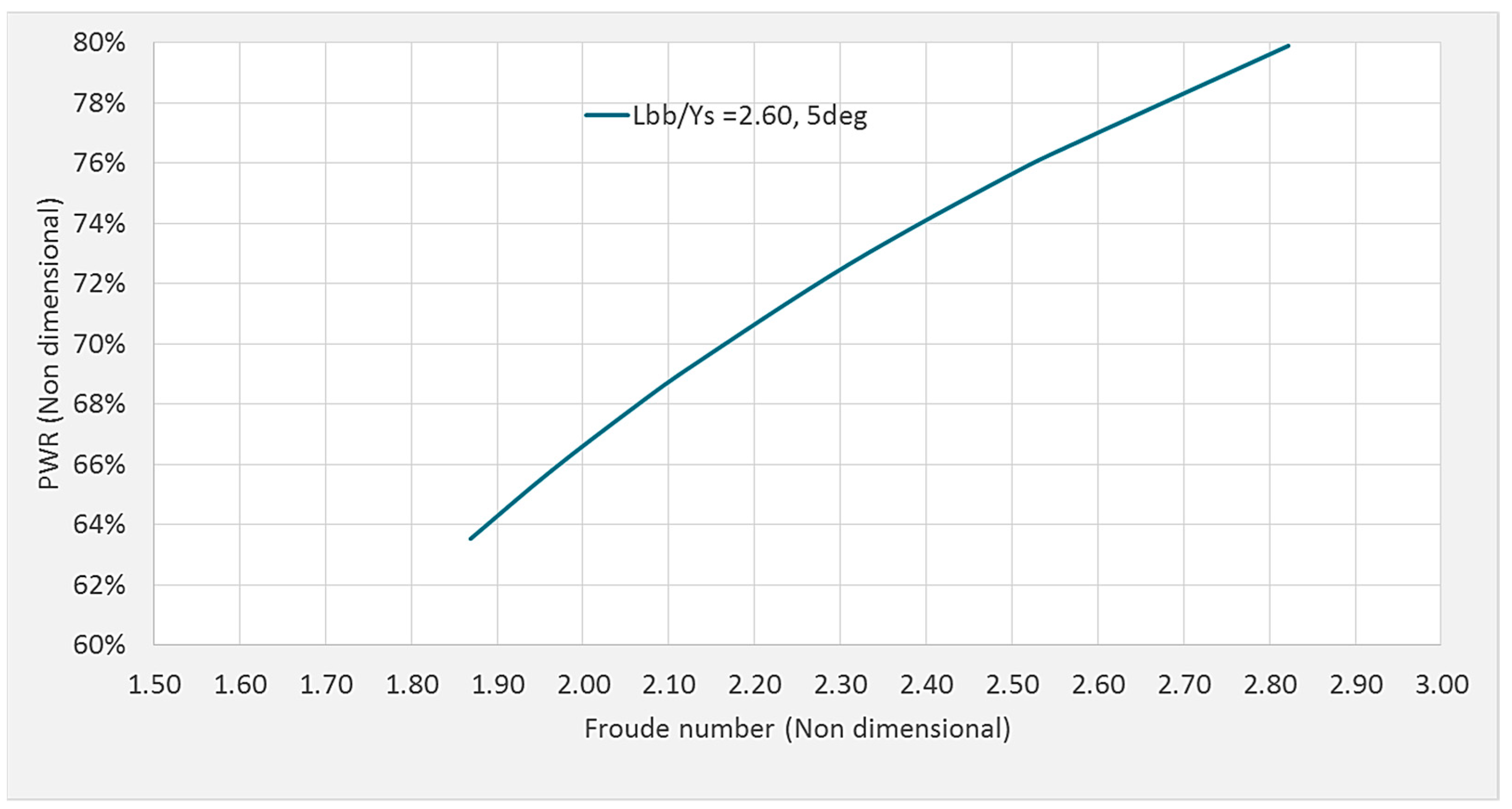
3.6. Pier Wave Level Ratio (PWR)
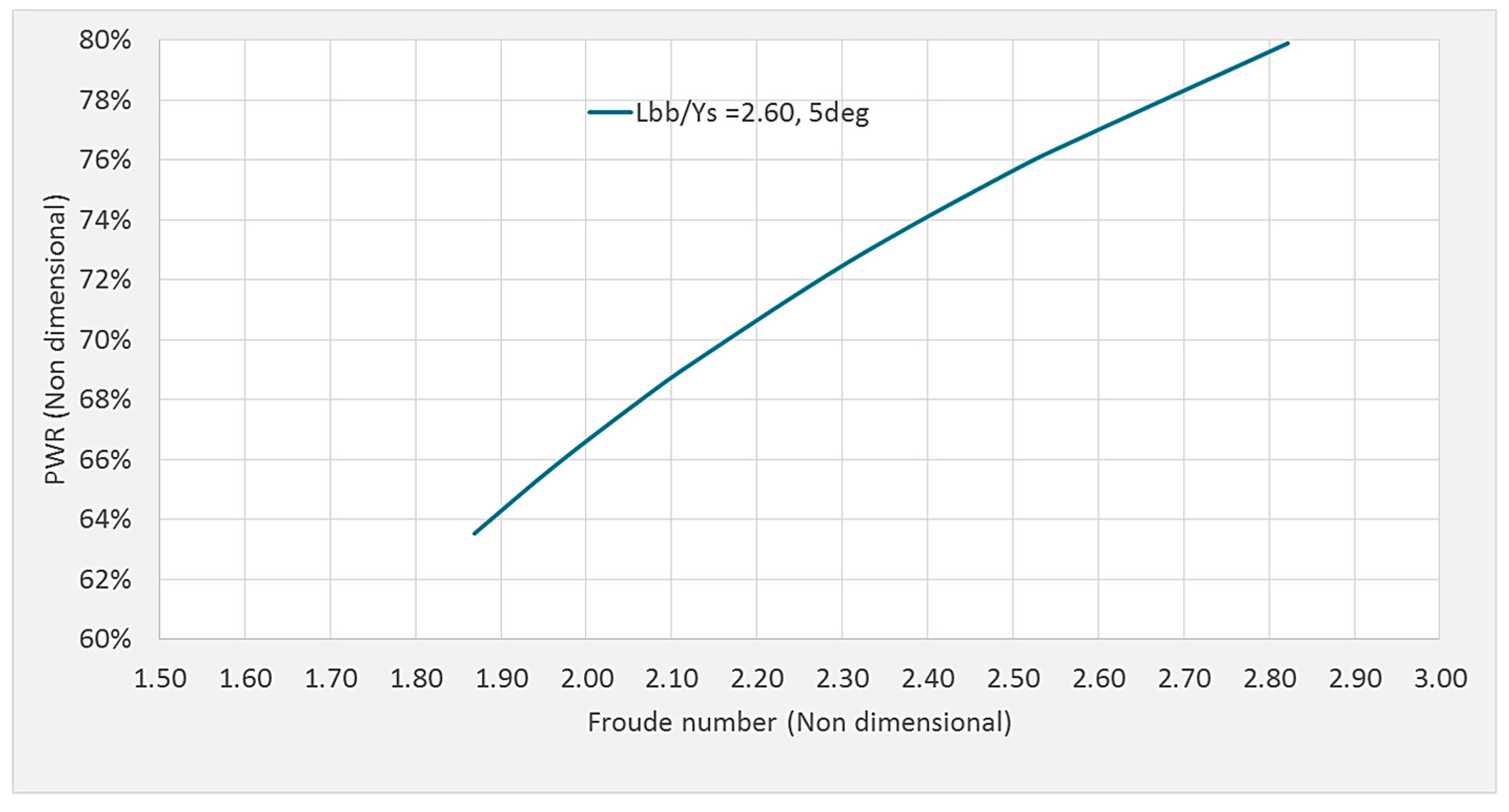
3.7. Design Curves for Pier Wave Reduction
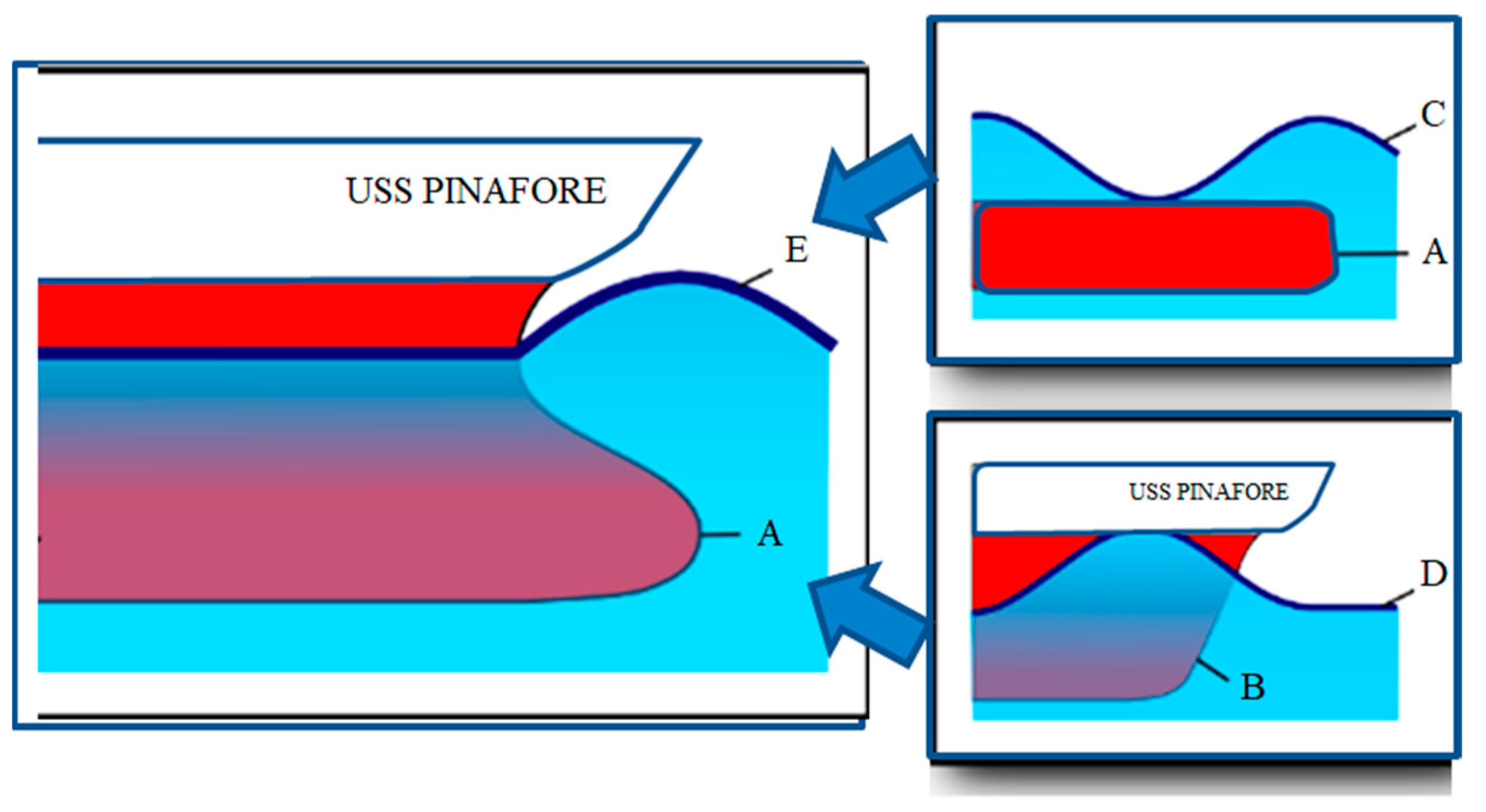
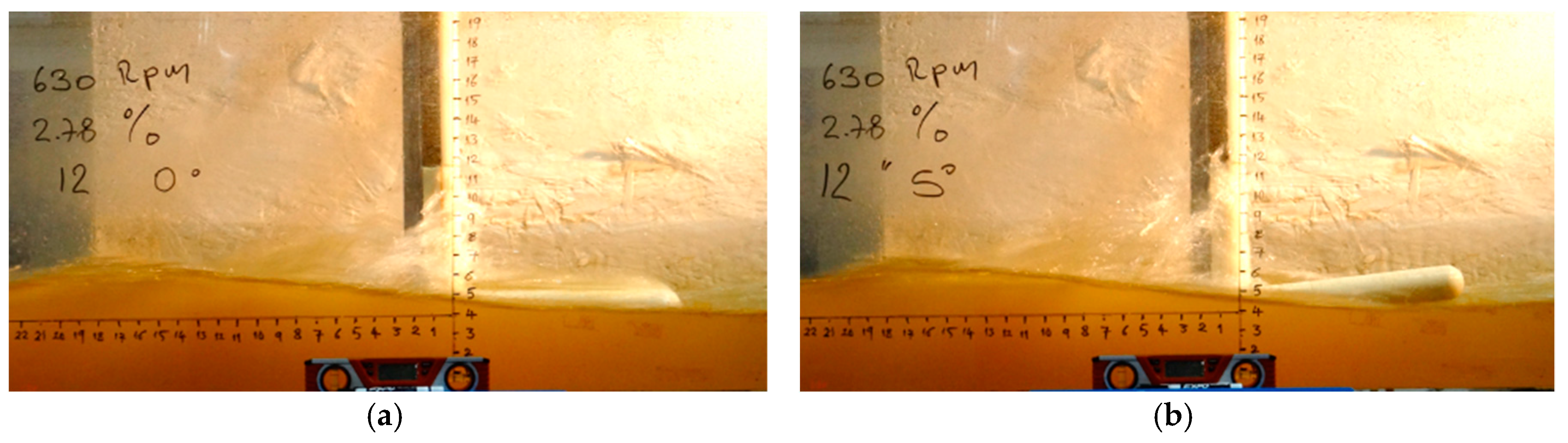
3.8. Flow Behavior After the Pier-Bulb Intersection
3.9. The Bulbous Pier vs. the Pier Extension
4. Proposed Pier Bulbous Design Method
- Determine the open channel water depth (Yh) via field data or open channel equations;
- The optimal bulb submerged depth (Ys) is equivalent to the maximum hydraulic depth (Yh) at bulb centerline, see Figure 28;
- The bulb diameter shall be equal to the pier width;
- Determine the non-bulb pier wave height using the following equation or field value:
- Calculate the open channel Froude number (Fr);
- Calculate the bulb length using Ys equal to Yh;
- Verify whether the PWR reduces the pier wave to an acceptable level; if not the cylindrical bulbous is not a solution. In order to increase the PWR, the bulb submerged area needs to be increased, which leads to a different bulb shape, which is a subject of further research.
5. Conclusions and Recommendations
- Experimental values show that a bulbous pier reduces the pier wave height by subtracting energy from the water flow.
- The viscous resistance is the main component in the PLbb; this is due to the bulb length being less than the bulb wavelength.
- A zero-degree pitch angle bulbous pier is too long for practical applications; to achieve pier reduction in the order of 0.4, the bulb length should be above 10′.
- The introduction of the pitch angle in a bulb reduces the length of the bulb at the water line by eliminating the flow over the bulb; This modification addresses the constructability problem of a long bulbous.
- The non-bulbous pier water level (PLnb_calc) overestimates the water height in comparison with the experimental data (PLnb_data).
- Making the viscous resistance the only force in the calculation of Bw underestimates it.
- For practical applications, PWRcalc provides a good approximation to the expected reduction in the pier wave level.
Recommendations
- Full-size model trials are recommended before using a pier bulbous in lieu of pier extensions.
- A cylindrical bulbous was selected based on the literature review, but it is possible that other bulb shapes could outperform a cylindrical bulb; research is needed in this area.
- The narrow channel effect, documented in the literature review, may be responsible for the distinct bulb wave found in the 0.958′ and 0.974′; research is needed in this area.
- The pitch angles used in this research are extrapolations of the parameters used for bulbous bows on boats; determining the optimum bulb pitch angle for piers requires additional research.
- Research can be conducted on how the cantilever bulb is going to affect the structural response of the pier.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A
| Two-Sample T-Test and CI: PLnb_T, PLbb_D, Lbb = 0.667′ Pitch Angle = 0° |
| Two-sample T for PLnb_T vs. PLbb_D |
| N Mean StDev SE Mean |
| PLnb_T 32000 1.323 0.239 0.0013 |
| PLbb_D 32000 0.679 0.153 0.00085 |
| Difference = μ (PLnb_T) − μ (PLbb_D) |
| Estimate for difference: 0.64407 |
| 95% lower bound for difference: 0.64146 |
| T-Test of difference = 0.7 (vs. >): T-value = −35.33 p-value = 1.000 DF = 63998 |
| Both use Pooled StDev = 0.2003 |
| p-value > 0.05 => DO NOT REJECT H0 |
| Minitab 17 report |
| Two-Sample T-Test and CI: PLnb_T, PLbb_D, Lbb = 0.958′ Pitch Angle = 0° |
| Two-sample T for PLnb_T vs. PLbb_D |
| N Mean StDev SE Mean |
| PLnb_T 32000 1.325 0.236 0.0013 |
| PLbb_D 32000 0.636 0.142 0.00079 |
| Difference = μ (PLnb_T) − μ (PLbb_D) |
| Estimate for difference: 0.68873 |
| 95% lower bound for difference: 0.68619 |
| T-Test of difference = 0.7 (vs. >): T-value = −7.31 p-value = 1.000 DF = 63998 |
| Both use Pooled StDev = 0.1949 |
| p-value > 0.05 => DO NOT REJECT H0 |
| Minitab 17 report |
| Two-Sample T-Test and CI: PLnb_T, PLbb_D, Lbb = 1.25′ Pitch Angle = 0° |
| Two-sample T for PLnb_T vs. PLbb_D |
| N Mean StDev SE Mean |
| PLnb_T 32000 1.327 0.238 0.0013 |
| PLbb_D 32000 0.617 0.132 0.00074 |
| Difference = μ (PLnb_T) − μ (PLbb_D) |
| Estimate for difference: 0.71021 |
| 95% lower bound for difference: 0.70771 |
| T-Test of difference = 0.8 (vs. >): T-value = −58.96 p-value = 1.000 DF = 63998 |
| Both use Pooled StDev = 0.1926 |
| p-value > 0.05 => DO NOT REJECT H0 |
| Minitab 17 report |
| Two-Sample T-Test and CI: PLnb_T, PLbb_D, Lbb = 1.542′ Pitch Angle = 0° |
| Two-sample T for PLnb_T vs. PLbb_D |
| N Mean StDev SE Mean |
| PLnb_T 32000 1.330 0.237 0.0013 |
| PLbb_D 32000 0.590 0.129 0.00072 |
| Difference = μ (PLnb_T) − μ (PLbb_D) |
| Estimate for difference: 0.73990 |
| 95% lower bound for difference: 0.73742 |
| T-Test of difference = 0.8 (vs. >): T-value = −39.86 p-value = 1.000 DF = 49433 |
| p-value > 0.05 => DO NOT REJECT H0 |
| Minitab 17 report |
| Two-Sample T-Test and CI: PLnb_T, PLbb_D, Lbb = 1.542′ Pitch Angle = 0° |
| Two-sample T for PLnb_T vs. PLbb_D |
| N Mean StDev SE Mean |
| PLnb_T 32000 1.330 0.236 0.0013 |
| PLbb_D 32000 0.584 0.123 0.00069 |
| Difference = μ (PLnb_T) − μ (PLbb_D) |
| Estimate for difference: 0.74570 |
| 95% lower bound for difference: 0.74326 |
| T-Test of difference = 0.8 (vs. >): T-value = −36.51 p-value = 1.000 DF = 48 |
| p-value > 0.05 => DO NOT REJECT H0 |
| Minitab 17 report |
| Two-Sample T-Test and CI: PLnb_T, PLbb_D, Lbb = 0.766′ Pitch Angle = 5° |
| Two-sample T for PLnb_T vs. PLbb_D |
| N Mean StDev SE Mean |
| PLnb_T 16000 1.311 0.228 0.0018 |
| PLbb_D 16000 0.625 0.123 0.00097 |
| Difference = μ (PLnb_T) − μ (PLbb_D) |
| Estimate for difference: 0.68658 |
| 95% lower bound for difference: 0.68321 |
| T-Test of difference = 0.8 (vs. >): T-value = −55.44 p-value = 1.000 DF = 24612 |
| p-value > 0.05 => DO NOT REJECT H0 |
| Minitab 17 report |
| Two-Sample T-Test and CI: PLnb_T, PLbb_D, Lbb = 0.974′ Pitch Angle = 5° |
| Two-sample T for PLnb_T vs. PLbb_D |
| N Mean StDev SE Mean |
| PLnb_T 16000 1.315 0.228 0.0018 |
| PLbb_D 16000 0.569 0.125 0.00099 |
| Difference = μ (PLnb_T) − μ (PLbb_D) |
| Estimate for difference: 0.74627 |
| 95% lower bound for difference: 0.74288 |
| T-Test of difference = 0.8 (vs. >): T-Value = −26.09 p-value = 1.000 DF = 24860 |
| p-value > 0.05 => DO NOT REJECT H0 |
| Minitab 17 report |
| Two-Sample T-Test and CI: PLnb_T, PLbb_D, Lbb = 1.266′ Pitch Angle = 5° |
| Two-sample T for PLnb_T vs. PLbb_D |
| N Mean StDev SE Mean |
| PLnb_T 16000 1.342 0.247 0.0020 |
| PLbb_D 16000 0.4907 0.0874 0.00069 |
| Difference = μ (PLnb_T) − μ (PLbb_D) |
| Estimate for difference: 0.85145 |
| 95% lower bound for difference: 0.84805 |
| T-Test of difference = 0.9 (vs. >): T-Value = −23.46 p-value = 1.000 DF = 19951 |
| p-value > 0.05 => DO NOT REJECT H0 |
| Minitab 17 report |
| Two-Sample T-Test and CI: PLnb_T, PLbb_D, Lbb = 1.557′ Pitch Angle = 5° |
| Two-sample T for PLnb_T vs. PLbb_D |
| N Mean StDev SE Mean |
| PLnb_T 16000 1.340 0.246 0.0019 |
| PLbb_D 16000 0.4778 0.0962 0.00076 |
| Difference = μ (PLnb_T) − μ (PLbb_D) |
| Estimate for difference: 0.86229 |
| 95% lower bound for difference: 0.85886 |
| T-Test of difference = 0.9 (vs. >): T-value = −18.08 p-value = 1.000 DF = 20790 |
| p-value > 0.05 => DO NOT REJECT H0 |
| Minitab 17 report |
| Two-Sample T-Test and CI: PLnb_T, PLbb_D, Lbb =0.787′ Pitch Angle = 10° |
| Two-sample T for PLnb_T vs. PLbb_D |
| N Mean StDev SE Mean |
| PLnb_T 16000 1.335 0.248 0.0020 |
| PLbb_D 16000 0.571 0.112 0.00088 |
| Difference = μ (PLnb_T) − μ (PLbb_D) |
| Estimate for difference: 0.76362 |
| 95% lower bound for difference: 0.76008 |
| T-Test of difference = 0.9 (vs. >): T-Value = 221263.44 p-value = 1.000 DF = 22258 |
| p-value > 0.05 => DO NOT REJECT H0 |
| Minitab 17 report |
| Two-Sample T-Test and CI: PLnb_T, PLbb_D, Lbb =0.995′ Pitch Angle = 10° |
| Two-sample T for PLnb_T vs. PLbb_D |
| N Mean StDev SE Mean |
| PLnb_T 16000 1.334 0.249 0.0020 |
| PLbb_D 16000 0.5207 0.0961 0.00076 |
| Difference = μ (PLnb_T) − μ (PLbb_D) |
| Estimate for difference: 0.81298 |
| 95% lower bound for difference: 0.80951 |
| T-Test of difference = 0.9 (vs. >): T-Value = −41.27 p-value = 1.000 DF = 20666 |
| p-value > 0.05 => DO NOT REJECT H0 |
| Minitab 17 report |
| Two-Sample T-Test and CI: PLnb_T, PLbb_D, Lbb = 1.286′ Pitch Angle = 10° |
| Two-sample T for PLnb_T vs. PLbb_D |
| N Mean StDev SE Mean |
| PLnb_T 16000 1.336 0.255 0.0020 |
| PLbb_D 16000 0.5211 0.0909 0.00072 |
| Difference = μ (PLnb_T) − μ (PLbb_D) |
| Estimate for difference: 0.81494 |
| 95% lower bound for difference: 0.81141 |
| T-Test of difference = 0.9 (vs. >): T-Value = −39.71 p-value = 1.000 DF = 19993 |
| p-value > 0.05 => DO NOT REJECT H0 |
| Minitab 17 report |
| Two-Sample T-Test and CI: PLnb_T, PLbb_D, Lbb = 1.578′ Pitch Angle = 10° |
| Two-sample T for PLnb_T vs. PLbb_D |
| N Mean StDev SE Mean |
| PLnb_T 16000 1.340 0.259 0.0020 |
| PLbb_D 16000 0.522 0.108 0.00086 |
| Difference = μ (PLnb_T) − μ (PLbb_D) |
| Estimate for difference: 0.81776 |
| 95% lower bound for difference: 0.81411 |
| T-Test of difference = 0.9 (vs. >): T-Value = −37.08 p-value = 1.000 DF = 21448 |
| p-value > 0.05 => DO NOT REJECT H0 |
| Minitab 17 report |
References
- Stonestreet, S.E.; Mulvihill, M.E.; Hite, J.E. Revised hydraulic design of the Rio Hondo Flood Control Channel. In Proceedings of the ASCE Proceeding National Conference Hydraulic, Engineering, Buffalo, NY, USA, 1–5 August 1994; pp. 401–405. [Google Scholar]
- Hite, J.E.; Stonestreet, S.E.; Mulvihill, M.E. Model study of Rio Hondo Flood Control Channel, Los Angeles, California. In Proceedings of the ASCE Proceedings National Conference on Hydraulic Engineering, San Francisco, CA, USA, 25–30 July 1993; pp. 1695–1700. [Google Scholar]
- Stockstill, R.L. Hydraulic Design of Channels Conveying Supercritical Flow. Available online: https://www.researchgate.net/publication/235103810_Hydraulic_Design_of_Channels_Conveying_Supercritical_Flow/related (accessed on 13 July 2019).
- Bulbous Bow Design and Construction. Available online: http://www.mar.ist.utl.pt/mventura/Projecto-Navios-I/EN/SD-1.5.4-Bulbous%20Bow%20Design.pdf (accessed on 6 March 2018).
- Kracht, A.M. Design of Bulbous Bows. SNAME 1978, 86, 197–217. [Google Scholar]
- Havelock, T.H. The Wave-Making Resistance of Ships: A Study of Certain Series of Model Experiments. Proc. R. Soc. Lond. Ser. A Contain. Papers Math. Phys. Character 1910, 84, 197–208. [Google Scholar]
- US Naval Academy: Resistance and Powering of Ships. Available online: http://www.usna.edu/NAOE/_files/documents/en400/TEXT%20%202011/12Chapter%207%20Text%202011.pdf (accessed on 6 March 2018).
- Yun, L.; Bliault, A. High Performance Marine Vessels; Springer: Boston, MA, USA, 2012. [Google Scholar]
- Ghani, M.A.; Wilson, P. Experimental Analysis of Catamarans Forms with Bulbous Operating in Shallow Waters. Int. Shipbuild. Prog. 2009, 2, 29–57. [Google Scholar]
- Sorensen, R.M. Basic Coastal Engineering, 3rd ed.; Springer: Boston, MA, USA, 2006. [Google Scholar]
- Karakouzian, M.; Karami, M.; Nazari-Sharabian, M.; Ahmad, S. Flow-Induced Stresses and Displacements in Jointed Concrete Pipes Installed by Pipe Jacking Method. Fluids 2019, 4, 34. [Google Scholar] [CrossRef]
- Clark County Regional Flood Control District. Available online: http://www.ccrfcd.org/ (accessed on 6 March 2018).











| (1) | 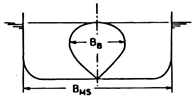 | |
| Breadth parameter (CBB): The maximum breadth (maximum width of a bulb - BB) of bulb area (ABT) at the forward perpendicular, divided by the beam of a ship at amidships (BMS). | ||
| (2) |  | |
| Length parameter (CLPR): The protruding length (LPR) divided by the length between perpendiculars (LPP) of a ship. | ||
| (3) | 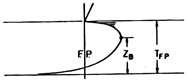 | |
| Depth parameter (CZB): The height (ZB) of the foremost point of a bulb over the baseline divided by the draft (TFP) at the forward perpendicular. | ||
| (4) |  | |
| Cross-section parameter (CABT): The cross-sectional area (ABT) of a bulbous bow at the forward perpendicular divided by a ship’s midship section area (AMS). | ||
| (5) |  | |
| Lateral parameter (CABL): The area (ABL) of a protruding bulb in the longitudinal plane divided by the midship section area of a ship (AMS). | ||
| (6) | 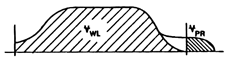 | |
| Volumetric parameter (CVPR): The volume (PR) of the protruding part of a bulb divided by the volume of displacement (WL) of the ship. NOTE: Protruding is used here to mean that part of a bulb which extends in front of the forward perpendicular. | ||
| Channel | |
|---|---|
| Parameter | Value |
| Width (ft.) | 118.00 |
| Height (ft.) | 5.70 |
| Length (ft.) | 500.00 |
| Flow Velocity (ft./sec.) | 20.14 |
| Submerged Height (ft.) | 5.00 |
| Hydraulic Depth (ft.) | 5.00 |
| Submerged Area (ft.2) | 590.00 |
| Bridge | |
| Pier Height (ft.) | 5.70 |
| Pier Hydraulic Depth (ft.) | 5.00 |
| Pier Length (ft.) | 152.43 |
| Pier Width (ft.) | 1.50 |
| Pier Nose Radius (ft.) | 1.50 |
| Pier Submerged Area (ft.2) | 7.50 |
| Ships/Bridge | Fr | v (ft./sec.) | L (ft.) |
|---|---|---|---|
| USS Sea Fighter (FSF-1) | 1.06 | 92.83 | 239.5 |
| USS Swift (HSV-2) | 0.75 | 75.95 | 321 |
| USS Independence (LCS 2) | 0.62 | 72.00 | 418 |
| Duck Creek Bridge Pier | 0.31 | 22.00 | 152 |
| Independent Variables | Value | Units | Condition |
|---|---|---|---|
| Pump Speed, Flume Slope (eight cases, Yh = 0.375) | (550, 1.24), (601, 1.59), (568, 1.99), (570, 2.38), (630, 2.78), (714, 3.16), (750, 3.59), and (765, 4.26) | RPM, % | Yh = 0.375 |
| Bulbous Horizontal Upper Length | 0.67, 0.96, 1.25, 1.54, and 1.83 | ft. | - |
| Characteristic | Values | Unit |
|---|---|---|
| Pump Nominal Speed | 1185 | RPM |
| Pump Nominal Flow | 3600 | GPM |
| Flume Pump Max. Speed | 980 | RPM |
| Manning’s Coefficient (n) | 0.010 | - |
| Flume Width | 1.5 | ft. |
| Flume Length | 58 | ft. |
| Flume Max. Slope | 4.1 | % |
| Flume Min. Slope | 0 | % |
| Parameter | Value | |
|---|---|---|
| Flume | Width (ft.) | 1.50 |
| Height (ft.) | 1.67 | |
| Length (ft.) | 25.00 | |
| Flow Velocity (ft./sec.) | 6.88 | |
| Submerged Height (ft.) | 0.58 | |
| Hydraulic Depth (ft.) | 0.58 | |
| Area (ft.2) | 2.50 | |
| Pier Model | Pier Height (ft.) | 0.67 |
| Pier Submerged Height (TFP) (ft.) | 0.58 | |
| Pier Length (LPP) (ft.) | 2.00 | |
| Pier Width (BMS) (ft.) | 0.17 | |
| Pier Submerged Area (AMS) (ft.2) | 0.10 | |
| Pier Height (VWL) (ft.) | 0.67 |
| Parameter | Min. | Max. | Unit | Abbreviation |
|---|---|---|---|---|
| Diameter | 0.17 | 0.17 | ft. | BB |
| Length | 0.03 | 0.13 | ft. | LPR |
| Area | 0.16 | 0.40 | ft.2 | - |
| Dimension | Value | Unit | Scale | Abbreviation |
|---|---|---|---|---|
| Pier Height | 0.67 | ft. | 0.12 | - |
| Pier Submerge Height | 0.58 | ft. | 0.12 | TFP |
| Pier Length | 2.00 | ft. | 0.12 | LPP |
| Pier Width | 0.17 | ft. | 0.12 | BMS |
| Pier Submerge Area | 0.10 | ft. | 0.12 | AMS |
| Pier Height | 0.67 | ft. | 0.12 | VWL |
| Pump Speed (RPM) | Flume Slope (%) | Pitch Angle (deg.) | Bulb Length (ft.) | Froude Number | Water Level (in.) |
|---|---|---|---|---|---|
| 550 | 1.24 | 0 | - | 1.85 | 7.0 |
| 550 | 1.24 | 0 | 1.25 | 1.80 | 5.5 |
| 550 | 1.24 | 5 | 1.27 | 1.84 | 6.0 |
| 550 | 1.24 | 10 | 1.29 | 1.81 | 6.0 |
| 568 | 1.99 | 0 | - | 2.05 | 5.5 |
| 568 | 1.99 | 0 | 1.25 | 2.03 | 5.0 |
| 568 | 1.99 | 5 | 1.27 | 2.06 | 5.0 |
| 568 | 1.99 | 10 | 1.29 | 2.06 | 5.0 |
| 570 | 2.38 | 0 | - | 2.13 | 5.1 |
| 570 | 2.38 | 0 | 1.25 | 2.10 | 5.2 |
| 570 | 2.38 | 5 | 1.27 | 2.11 | 5.0 |
| 570 | 2.38 | 10 | 1.29 | 2.12 | 5.0 |
| 601 | 1.59 | 0 | - | 1.97 | 8.0 |
| 601 | 1.59 | 0 | 1.25 | 1.94 | 7.5 |
| 601 | 1.59 | 5 | 1.27 | 1.95 | 7.0 |
| 601 | 1.59 | 10 | 1.29 | 1.95 | 6.8 |
| 630 | 2.78 | 0 | - | 2.25 | 8 |
| 630 | 2.78 | 0 | 1.25 | 2.26 | 6.5 |
| 630 | 2.78 | 5 | 1.27 | 2.24 | 6.5 |
| 630 | 2.78 | 10 | 1.29 | 2.31 | 6.0 |
| 714 | 3.16 | 0 | - | 2.49 | 6.0 |
| 714 | 3.16 | 0 | 1.25 | 2.52 | 6.0 |
| 714 | 3.16 | 5 | 1.27 | 2.50 | 6.0 |
| 714 | 3.16 | 10 | 1.29 | 2.49 | 6.0 |
| 750 | 3.59 | 0 | - | 2.59 | 5.5 |
| 750 | 3.59 | 0 | 1.25 | 2.58 | 5.5 |
| 750 | 3.59 | 5 | 1.27 | 2.60 | 5.5 |
| 750 | 3.59 | 10 | 1.29 | 2.60 | 5.5 |
| 765 | 4.26 | 0 | - | 2.70 | 4.5 |
| 765 | 4.26 | 0 | 1.25 | 2.63 | 5 |
| 765 | 4.26 | 5 | 1.27 | 2.63 | 4.5 |
| 765 | 4.26 | 10 | 1.29 | 2.68 | 4.5 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Karakouzian, M.; Chavez, A.; Hayes, D.; Nazari-Sharabian, M. Bulbous Pier: An Alternative to Bridge Pier Extensions as a Countermeasure against Bridge Deck Splashing. Fluids 2019, 4, 140. https://doi.org/10.3390/fluids4030140
Karakouzian M, Chavez A, Hayes D, Nazari-Sharabian M. Bulbous Pier: An Alternative to Bridge Pier Extensions as a Countermeasure against Bridge Deck Splashing. Fluids. 2019; 4(3):140. https://doi.org/10.3390/fluids4030140
Chicago/Turabian StyleKarakouzian, Moses, Amilcar Chavez, Donald Hayes, and Mohammad Nazari-Sharabian. 2019. "Bulbous Pier: An Alternative to Bridge Pier Extensions as a Countermeasure against Bridge Deck Splashing" Fluids 4, no. 3: 140. https://doi.org/10.3390/fluids4030140
APA StyleKarakouzian, M., Chavez, A., Hayes, D., & Nazari-Sharabian, M. (2019). Bulbous Pier: An Alternative to Bridge Pier Extensions as a Countermeasure against Bridge Deck Splashing. Fluids, 4(3), 140. https://doi.org/10.3390/fluids4030140








