Liquid–Liquid Flow and Mass Transfer Enhancement in Tube-in-Tube Millireactors with Structured Inserts and Advanced Inlet Designs
Abstract
1. Introduction
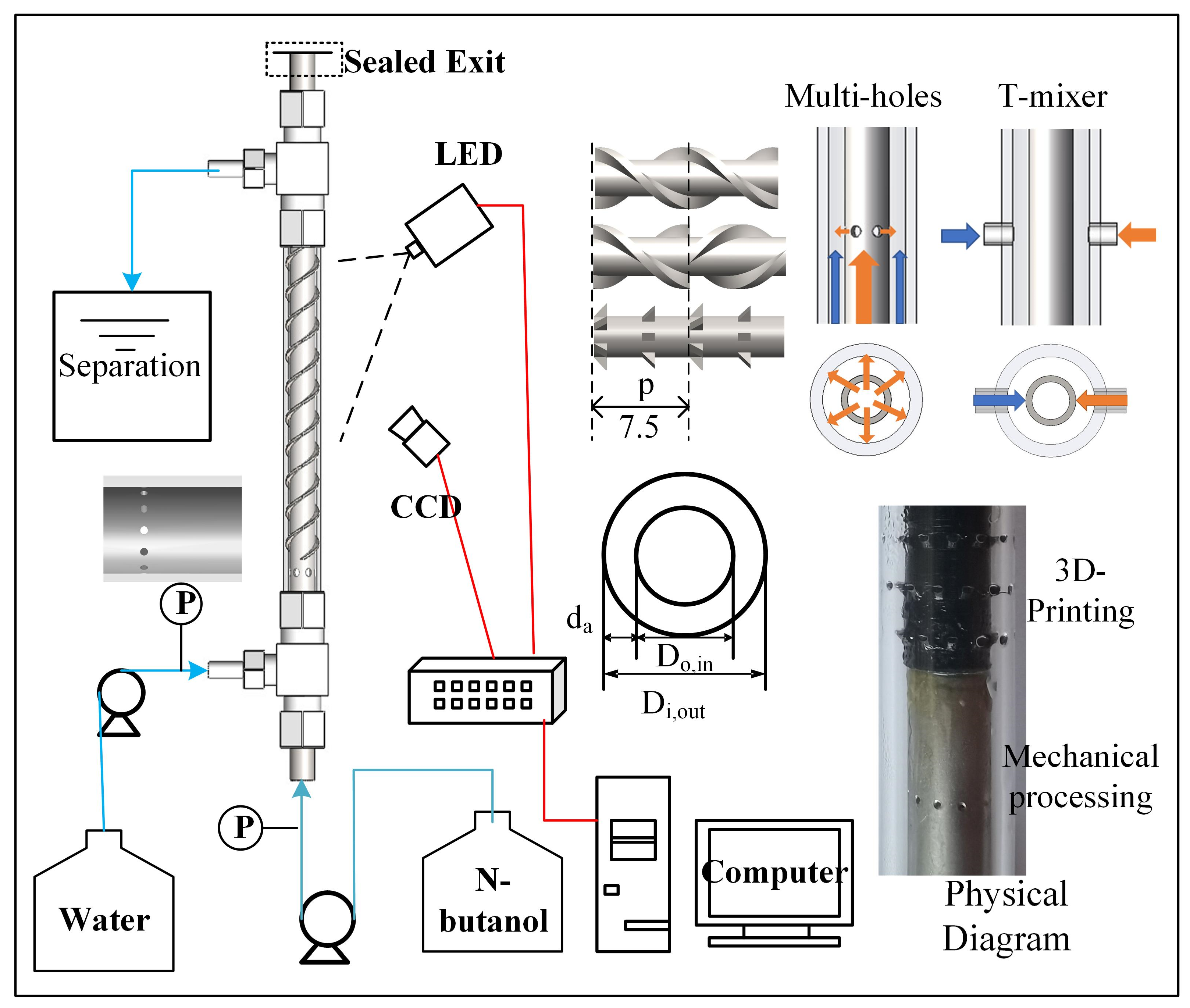
2. Materials and Methods
2.1. Visualization Experiments
2.2. Characterization of the Mass Transfer Performance
2.3. Data Simplification
3. Results and Discussion
3.1. Two-Phase Flow Patterns in Tube-in-Tube Millireactors
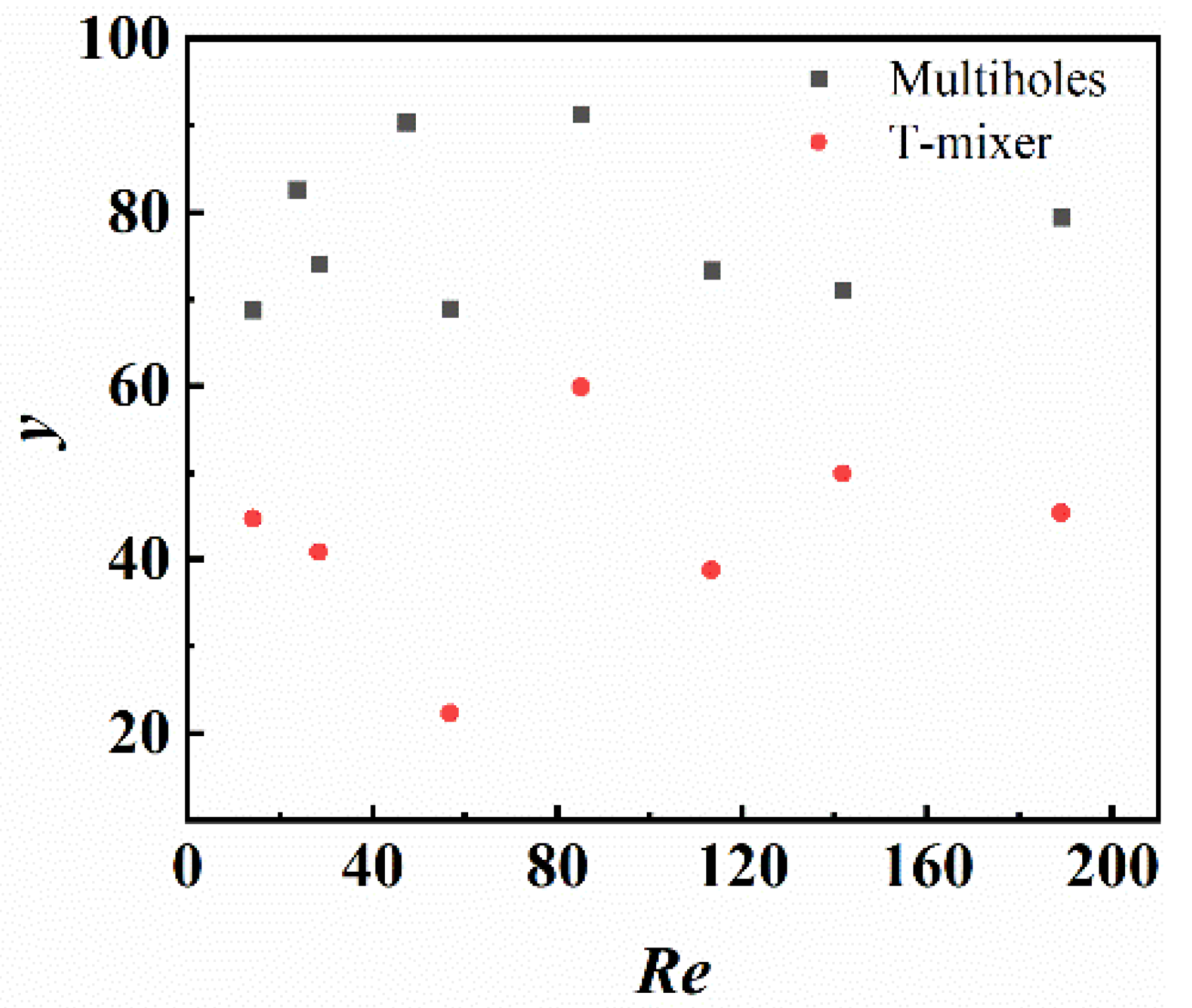
3.2. Mass Transfer Capability of the Inlet Section
3.2.1. Contribution of Mass Transfer in the Inlet Section
3.2.2. Influence of Apparent Reynolds Number on Mass Transfer in the Inlet Section
3.3. Mass Transfer in the Tube-in-Tube Millireactor System
3.3.1. Effect of Volume Flow Ratio () on Volumetric Mass Transfer Coefficient in a Plain Tube-in-Tube Millireactor
3.3.2. The Effect of Annular Gap Width on Volumetric Mass Transfer Coefficient in Plain Tube-in-Tube Millireactors
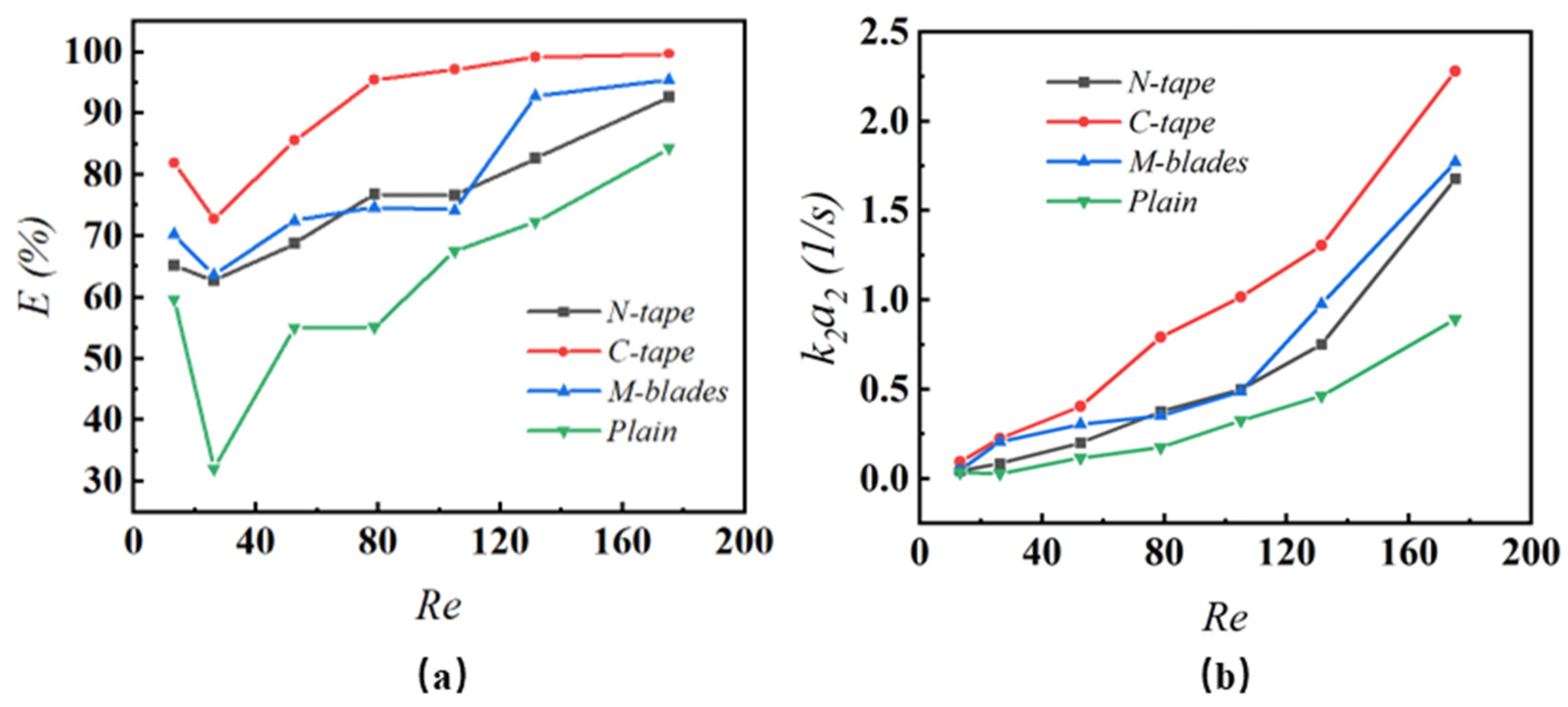
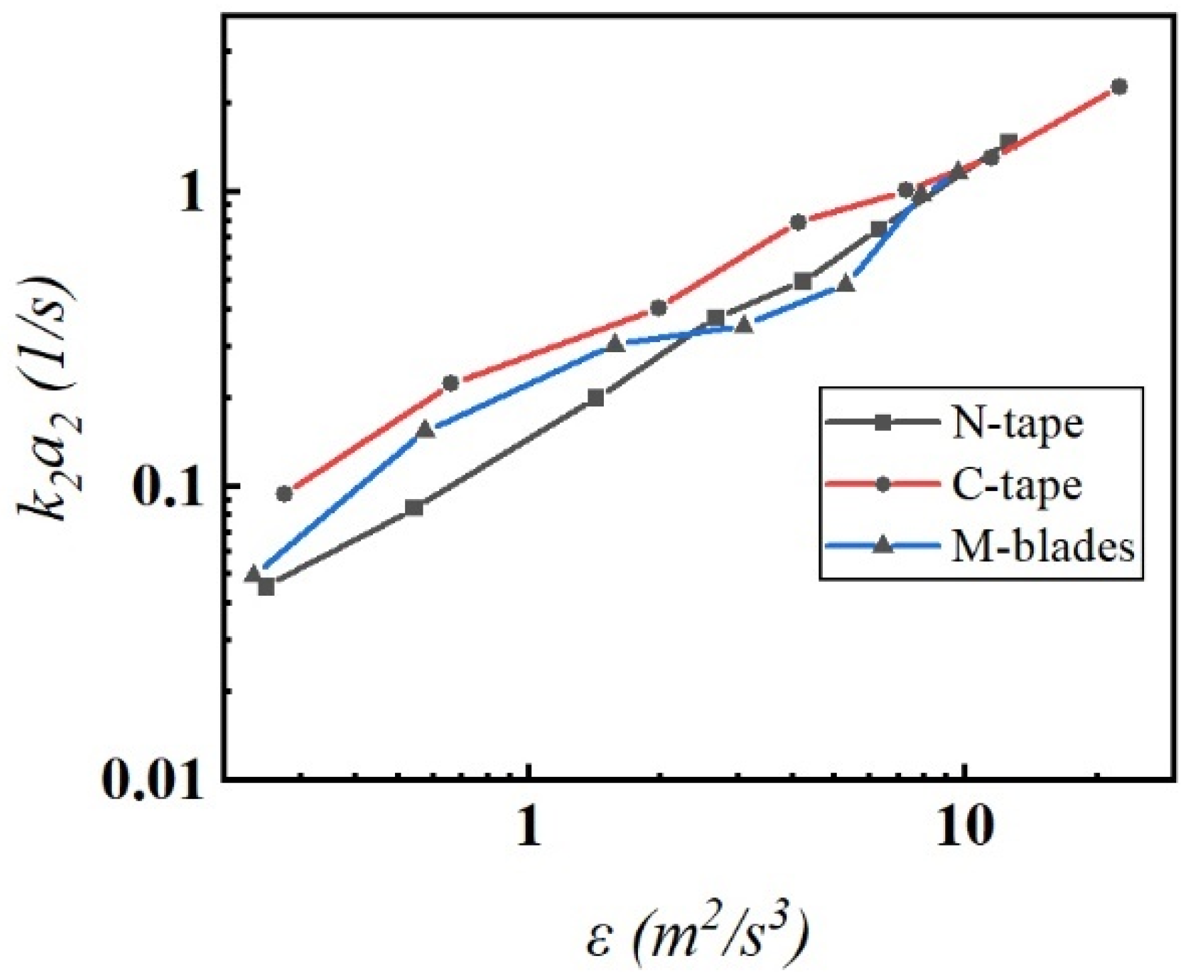
3.3.3. Two-Phase Mass Transfer in Tube-in-Tube Millireactors with Inserts
- N-tape: This configuration features helical fins that induce fluid rotation, effectively increasing the interfacial area and promoting surface renewal, which are critical for mass transfer.
- Multi-blade structure: Staggered fins in this design create flow disturbances and compression through narrow gaps. By splitting and recombining the flow, this structure extends the flow path and intensifies mass transfer processes.
- C-tape: The most effective design, the C-tape combines fluid rotation and segmentation to induce viscous shear and interfacial deformation. These mechanisms enhance droplet breakup in the dispersed phases, driving the earlier and steeper increase in observed with this design.
3.4. Comparison of Tubular Millireactors with Other Designs
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| As | cross-section area, mm2 |
| da | annular space size, mm |
| Dh | micro-hole size, mm |
| Di,out | the inner diameter of the outer tube, mm |
| dN | hydrodynamic diameter of the tube-in-tube millireactor, mm |
| Do,in | the outer diameter of the inner tube, mm |
| HPLC | high-performance liquid chromatography |
| KLa | overall volumetric mass transfer coefficient, s−1 |
| L | length of the reactor channel, mm |
| PA-TMC | pore-array tube-in-tube microreactor |
| Pr | Prandtl number |
| Re | Reynolds number for reaction channel |
| Reh | Reynolds number for micro-hole jet |
| TKE | turbulent kinetic energy, m2/s2 |
| Vin | volume flow rate in inner tube, ml/min |
| Vout | volume flow rate in outer tube, ml/min |
| y | inlet mass transfer contribution rate |
| bond number | |
| capillary number | |
| overall extraction rate | |
| Weber number | |
| gravitational acceleration | |
| flow ratio | |
| Greek letters | |
| ΔP | fluid pressure drop, Pa |
| ε | average turbulent dissipation rate, m2/s3 |
| v | kinematic viscosity of the fluid, m2/s |
| μ | dynamic viscosity, Pa s |
References
- Ramji, S.; Pushpavanam, S. Liquid-liquid extraction in laminar two-phase stratified flows in capillary microchannels. Chem. Eng. Sci. 2019, 195, 242–249. [Google Scholar] [CrossRef]
- Al-Azzawi, M.; Mjalli, F.S.; Husain, A.; Al-Dahhan, M. A Review on the Hydrodynamics of the Liquid-Liquid Two-Phase Flow in the Microchannels. Ind. Eng. Chem. Res. 2021, 60, 5049–5075. [Google Scholar] [CrossRef]
- Liu, H.; Jiang, J.; Yang, N.; Xiao, Z.; Yang, X.; Wang, R. Flow and mixing in a tube-in-tube millireactor with multiholes jet and twist tapes. J. Flow Chem. 2022, 12, 353–369. [Google Scholar] [CrossRef]
- Liu, H.; Jiang, J.; Wang, C.; Yang, N.; Yang, X.; Wang, R. Investigations of mixing and heat transfer in a structured tube-in-tube millireactor by numerical, experimental and statistical methods. Asia-Pac. J. Chem. Eng. 2022, 18, e2843. [Google Scholar] [CrossRef]
- Ke, T.; Le, Y.; Wang, J.-X.; Chu, G.-W.; Chen, J.-F.; Shao, L. Cu nanoparticle preparation in a tube-in-tube microchannel reactor and encapsulation by silica. Mater. Lett. 2010, 64, 1717–1719. [Google Scholar] [CrossRef]
- Li, T.; Zhou, Y.; Wang, J.-X.; Shao, L.; Chen, J.-F. High-throughput emulsification in a microporous tube-in-tube microchannel device: O/W emulsion formation. Chem. Eng. J. 2013, 228, 155–161. [Google Scholar] [CrossRef]
- Chen, J.-F.; Chen, G.-Z.; Wang, J.-X.; Shao, L.; Li, P.-F. High-Throughput Microporous Tube-in-Tube Microreactor as Novel Gas-Liquid Contactor: Mass Transfer Study. AIChE J. 2011, 57, 239–249. [Google Scholar] [CrossRef]
- Pan, M.-Y.; Qian, Z.; Shao, L.; Arowo, M.; Chen, J.-F.; Wang, J.-X. Absorption of carbon dioxide into N-methyldiethanolamine in a high-throughput microchannel reactor. Sep. Purif. Technol. 2014, 125, 52–58. [Google Scholar] [CrossRef]
- Sun, B.; Gao, M.; Arowo, M.; Wang, J.; Chen, J.; Meng, H.; Shao, L. Ozonation of Acid Red 14 in the Presence of Inorganic Salts in a Microporous Tube-in-Tube Microchannel Reactor. Ind. Eng. Chem. Res. 2014, 53, 19071–19076. [Google Scholar] [CrossRef]
- Sun, B.; Li, P.; Liang, Y.; Arowo, M.; Wang, J.; Meng, H.; Chen, J.; Shao, L. Oxidation of ammonium sulfite by oxygen in a microporous tube-in-tube microchannel reactor. Chem. Eng. J. 2014, 253, 258–263. [Google Scholar] [CrossRef]
- Li, W.; Xia, F.; Zhao, S.; Zhang, M.; Li, W.; Zhang, J. Characterization of liquid–liquid mass transfer performance in a novel pore-array intensified tube-in-tube microchannel. AIChE J. 2020, 66, 16839. [Google Scholar] [CrossRef]
- Yao, C.; Zhao, Y.; Ma, H.; Liu, Y.; Zhao, Q.; Chen, G. Two-phase flow and mass transfer in microchannels: A review from local mechanism to global models. Chem. Eng. Sci. 2021, 229, 116017. [Google Scholar] [CrossRef]
- Plouffe, P.; Roberge, D.M.; Sieber, J.; Bittel, M.; Macchi, A. Liquid-liquid mass transfer in a serpentine micro-reactor using various solvents. Chem. Eng. J. 2016, 285, 605–615. [Google Scholar] [CrossRef]
- Plouffe, P.; Macchi, A.; Roberge, D.M. From Batch to Continuous Chemical Synthesis-A Toolbox Approach. Org. Process Res. Dev. 2014, 18, 1286–1294. [Google Scholar] [CrossRef]
- Nieves-Remacha, M.J.; Kulkarni, A.A.; Jensen, K.F. Gas-liquid flow and mass transfer in an advanced-flow reactor. Ind. Eng. Chem. Res. 2013, 52, 8996–9010. [Google Scholar] [CrossRef]
- Zhang, F.; Cerato-Noyerie, C.; Woehl, P.; Lavric, E.D. Intensified liquid/liquid mass transfer in corning® advanced-flow ™reactors. Chem. Eng. Trans. 2011, 24, 1369–1374. [Google Scholar]
- Abrofarakh, M. Effect of incorporating Sulzer SMV static mixers on the performance of direct contact membrane distillation (DCMD): A three-dimensional computational fluid dynamics (CFD) study. Sep. Purif. Technol. 2025, 359, 130606. [Google Scholar] [CrossRef]
- Yu, Y.; Wan, H.; Meng, H.; Zhang, P.; Han, Z.; Wang, D. A study of particle motion in Kwerk-type twisted blades: Effect of configuration on particle mixing performance by CFD-DEM. Powder Technol. 2024, 448, 120248. [Google Scholar] [CrossRef]
- Meng, H.; Zhang, J.; Yu, Y.; Zhao, Y.; Li, D.; Xiang, K.; Ji, Y. Investigation into Droplet Size Distribution and Turbulent Mixing Enhancement Performance in a Lightnin Static Mixer. Ind. Eng. Chem. Res. 2024, 63, 21062–21077. [Google Scholar] [CrossRef]
- Farahani, S.; Movahedirad, S.; Sobati, M.A. Characterization of the oil water two phase flow in a novel microchannel contactor equipped with helical wire static mixer. Sci. Rep. 2024, 14, 23369. [Google Scholar] [CrossRef]
- Plouffe, P.; Roberge, D.M.; Macchi, A. Liquid-liquid flow regimes and mass transfer in various micro-reactors. Chem. Eng. J. 2016, 300, 9–19. [Google Scholar] [CrossRef]
- Yang, N.; Xiao, Z.; Liu, F.; Jiang, J.; Liu, Z.; Liu, H.; Yang, X.; Wang, R. Enhanced liquid–liquid mass transfer in a monolithic reactor with multi-jet-channel in the circumferential array. Sep. Purif. Technol. 2024, 333, 125749. [Google Scholar] [CrossRef]
- Zhang, J.; Wang, Y.; Stevens, G.W.; Fei, W. A state-of-the-art review on single drop study in liquid–liquid extraction: Experiments and simulations. Chin. J. Chem. Eng. 2019, 27, 2857–2875. [Google Scholar] [CrossRef]
- Misek, T.; Berger, R.; Schröter, J. Standard test systems for liquid extraction studies. Stand. Test Syst. Liq. Extr. 1985, 46, 10–20. [Google Scholar]
- Tang, J.; Zhang, X.; Cai, W.; Wang, F. Liquid-liquid extraction based on droplet flow in a vertical microchannel. Exp. Therm. Fluid Sci. 2013, 49, 185–192. [Google Scholar] [CrossRef]
- Li, G.; Shang, M.; Song, Y.; Su, Y. Characterization of liquid–liquid mass transfer performance in a capillary microreactor system. AIChE J. 2018, 64, 1106–1116. [Google Scholar] [CrossRef]
- Wu, B.; Li, C.; Zhang, M.; Luo, P. Liquid mixing intensification by adding swirling flow in the transverse jet mixer. AIChE J. 2021, 67, e17276. [Google Scholar] [CrossRef]
- Zizzari, A.; Carbone, L.; Cesaria, M.; Bianco, M.; Perrone, E.; Rendina, F.; Arima, V. Continuous flow scalable production of injectable size-monodisperse nanoliposomes in easy-fabrication milli-fluidic reactors. Chem. Eng. Sci. 2021, 235, 116481. [Google Scholar] [CrossRef]
- Yao, C.; Ma, H.; Zhao, Q.; Liu, Y.; Zhao, Y.; Chen, G. Mass transfer in liquid-liquid Taylor flow in a microchannel: Local concentration distribution, mass transfer regime and the effect of fluid viscosity. Chem. Eng. Sci. 2020, 223, 115734. [Google Scholar] [CrossRef]
- Sattari-Najafabadi, M.; Nasr Esfahany, M.; Wu, Z.; Sunden, B. Mass transfer between phases in microchannels: A review. Chem. Eng. Process. Process Intensif. 2018, 127, 213–237. [Google Scholar] [CrossRef]
- Yin, Y.; Guo, R.; Zhu, C.; Fu, T.; Ma, Y. Enhancement of gas-liquid mass transfer in microchannels by rectangular baffles. Sep. Purif. Technol. 2020, 236, 116306. [Google Scholar] [CrossRef]
- Lemenand, T.; Dupont, P.; Della Valle, D.; Peerhossaini, H. Comparative efficiency of shear, elongation and turbulent droplet breakup mechanisms: Review and application. Chem. Eng. Res. Des. 2013, 91, 2587–2600. [Google Scholar] [CrossRef]
- Woitalka, A.; Kuhn, S.; Jensen, K.F. Scalability of mass transfer in liquid–liquid flow. Chem. Eng. Sci. 2014, 116, 1–8. [Google Scholar] [CrossRef]






Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhu, F.; Pan, X.; Cao, X.; Chen, Y.; Wang, R.; Lin, J.; Liu, H. Liquid–Liquid Flow and Mass Transfer Enhancement in Tube-in-Tube Millireactors with Structured Inserts and Advanced Inlet Designs. Fluids 2025, 10, 26. https://doi.org/10.3390/fluids10020026
Zhu F, Pan X, Cao X, Chen Y, Wang R, Lin J, Liu H. Liquid–Liquid Flow and Mass Transfer Enhancement in Tube-in-Tube Millireactors with Structured Inserts and Advanced Inlet Designs. Fluids. 2025; 10(2):26. https://doi.org/10.3390/fluids10020026
Chicago/Turabian StyleZhu, Feng, Xingxing Pan, Xichun Cao, Yandan Chen, Rijie Wang, Jiande Lin, and Hanyang Liu. 2025. "Liquid–Liquid Flow and Mass Transfer Enhancement in Tube-in-Tube Millireactors with Structured Inserts and Advanced Inlet Designs" Fluids 10, no. 2: 26. https://doi.org/10.3390/fluids10020026
APA StyleZhu, F., Pan, X., Cao, X., Chen, Y., Wang, R., Lin, J., & Liu, H. (2025). Liquid–Liquid Flow and Mass Transfer Enhancement in Tube-in-Tube Millireactors with Structured Inserts and Advanced Inlet Designs. Fluids, 10(2), 26. https://doi.org/10.3390/fluids10020026







