Baseline Model for Bubbly Flows: Simulation of Monodisperse Flow in Pipes of Different Diameters
Abstract
:1. Introduction
2. Summary of the Experimental Data
2.1. Tests of Liu [36]
2.2. Tests of Shawkat et al. [33]
2.3. Tests of Hosokawa and Tomiyama [34]
3. Description of the Models
3.1. Two-Fluid Model Equations
3.2. Bubble Forces
3.3. Turbulence Modelling
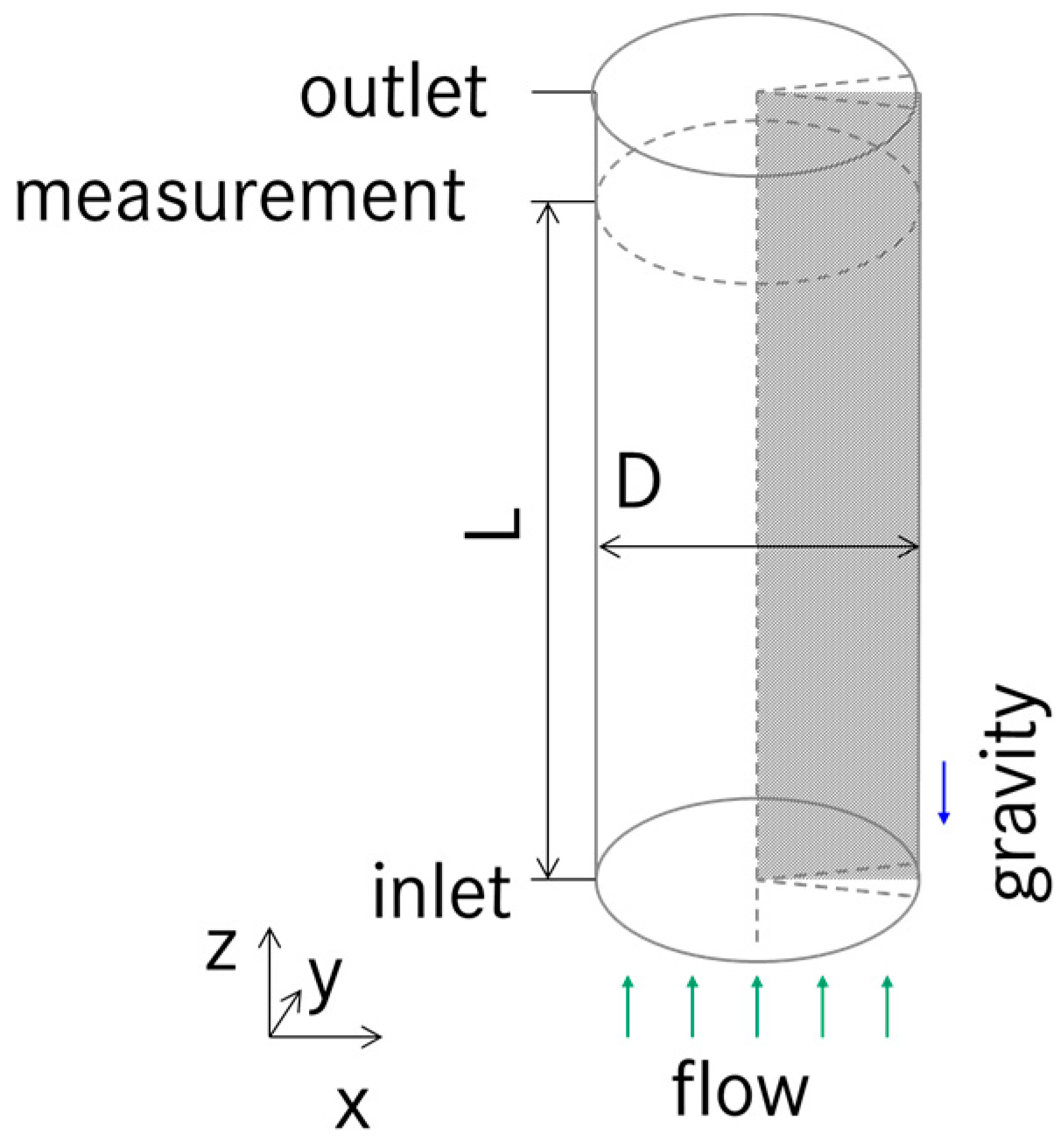
3.4. Geometry and Boundary Conditions
3.5. Other Model Aspects
4. Simulation Results
4.1. Pipe with D ≈ 5 cm: Liu [36]
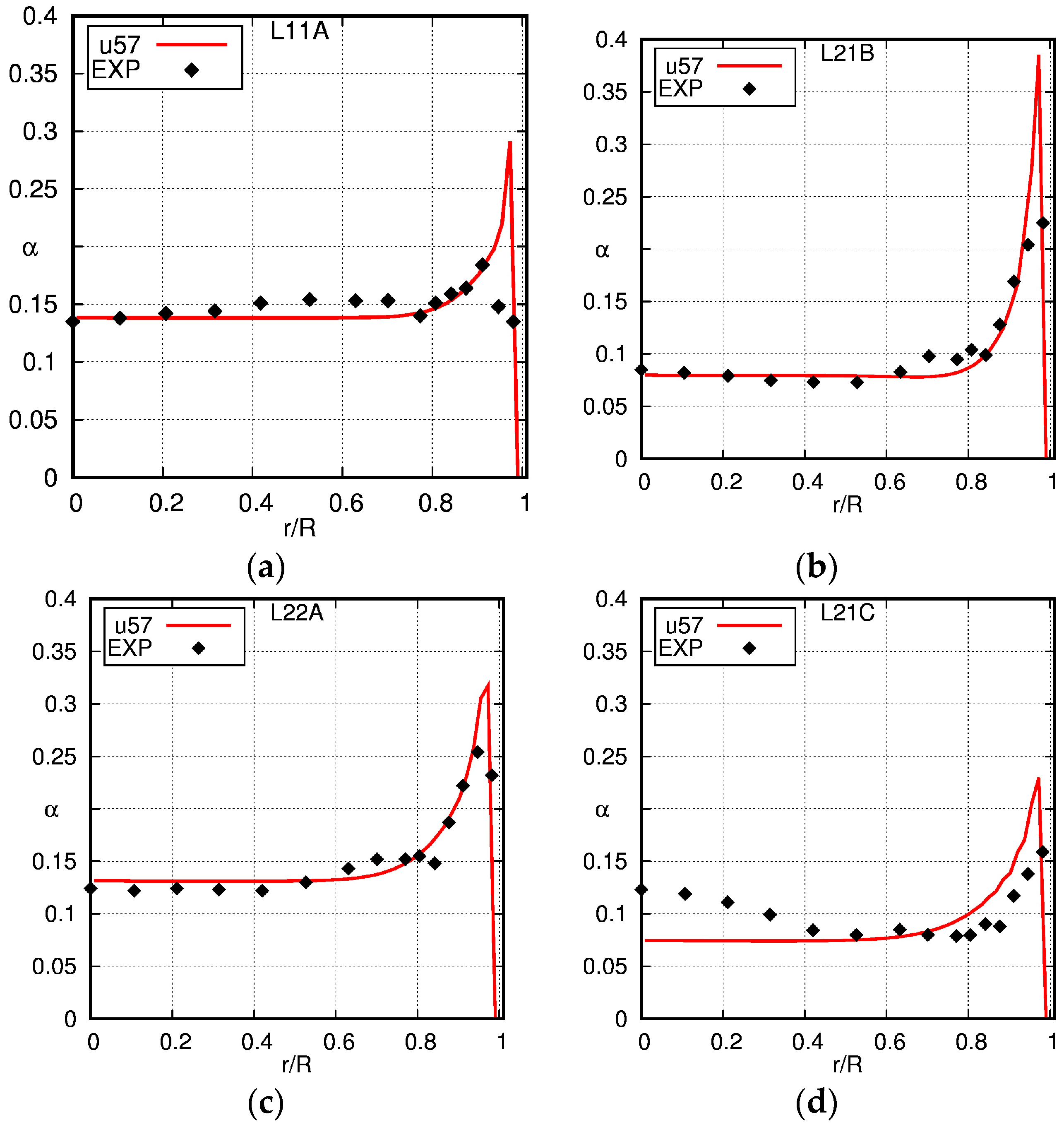
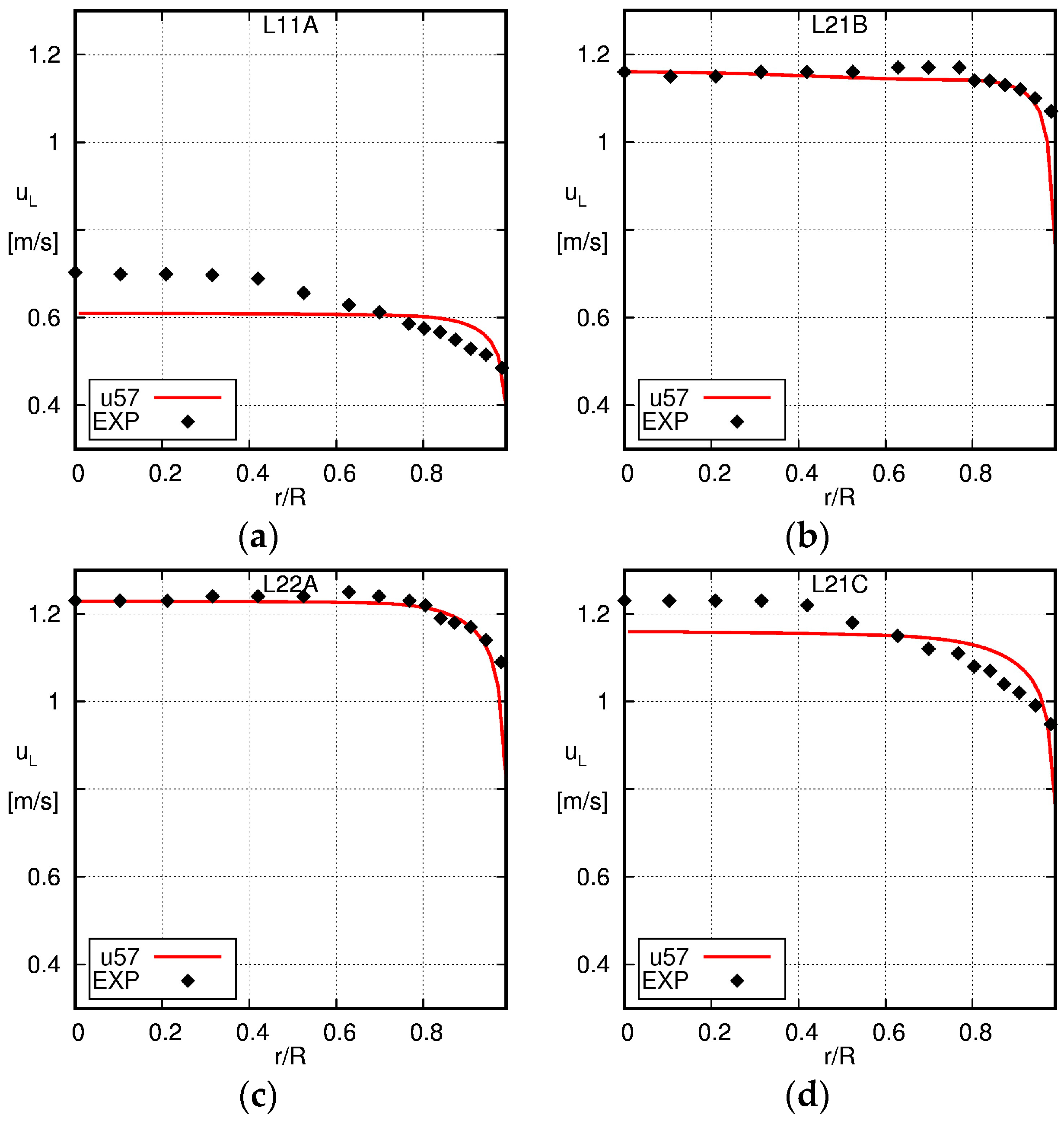
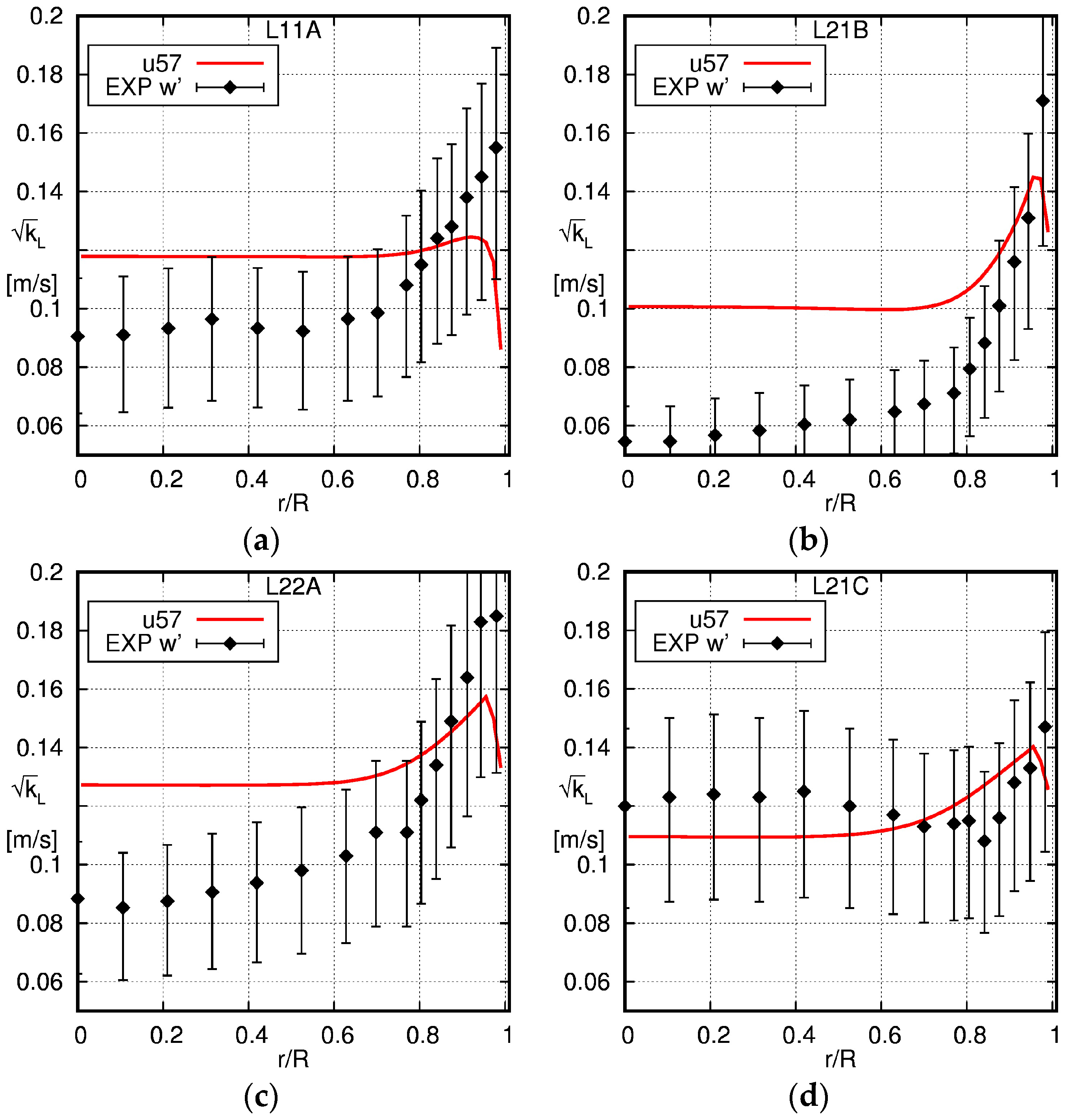
4.2. Pipe with D = 20 cm: Shawkat et al. [33]
4.2.1. Single-Phase Flow
4.2.2. Two-Phase Flow
4.3. Pipe with D = 2.5 cm: Hosokawa and Tomiyama [34]
4.3.1. Single-Phase Flow
4.3.2. Two-Phase Flow
5. Models and Data for Drag Force and Rise Velocity
5.1. Critical Assessment of the Drag Force Modelling
- the “degree of contamination” of the water in air-water flows.
- the influence of pipe walls and shear rate.
- the influence of background turbulence.
- the influence of higher gas fractions (swarm effects).
5.2. Review of Further Measurements of Bubble Rise Velocity
6. Discussion and Conclusions
- The baseline model reproduces the experimental data reasonably well independent of the pipe diameter.
- Further improvements of the baseline model are desirable, in particular for the near-wall modelling and the closure for bubble-induced turbulence.
- Knowledge of the full bubble size distribution is essential to explain the observed behavior in bubbly flows. Hence, data including measurements of bubble size distributions are necessary. In addition, such data would also allow a validation of models for bubble coalescence and breakup, as described, e.g., in [77].
- Generally, validation of CFD models for bubbly flow requires more reliable measurement techniques. In particular, reliable measurements of the bubble and liquid velocity are surprisingly scarce at present.
- It is highly desirable that experimental databases for CFD validation provide a comprehensive set of measurements, including both gas and liquid phase properties. At least bubble diameter, liquid and gas velocity profiles and profiles for the liquid turbulent kinetic energy should be given. Otherwise, important features may be missed by the validation.
- Considering the sensitivity of the air-water system to even the smallest contaminations, which are hard to avoid and even harder to quantify, measurement data of other less sensitive systems of gas and liquid should be acquired.
Acknowledgments
Author Contributions
Conflicts of Interest
Nomenclature
| Notation | Unit | Denomination |
| CD | - | drag coefficient |
| CL | - | lift coefficient |
| CTD | - | turbulent dispersion coefficient |
| CW | - | wall force coefficient |
| dB | m | bulk volume equivalent sphere bubble diameter |
| D | m | pipe diameter |
| F | N·m−3 | volumetric force density |
| g | m·s−2 | acceleration of gravity |
| It | - | turbulence intensity |
| J | m·s−1 | superficial velocity = volumetric flux |
| k | m2·s−2 | turbulent kinetic energy |
| Lt | m | turbulent length scale |
| L | m | pipe length |
| Mo | - | Morton number |
| p | Pa | pressure |
| r | m | radial coordinate |
| t | s | time |
| T | N·m−2 | stress tensor |
| u | m·s−1 | velocity |
| u’ | m·s−1 | fluctuation velocity |
| α | - | volume fraction |
| ε | m2·s−3 | turbulent dissipation rate |
| µ | kg·m−1·s−1 | dynamic viscosity |
| ρ | kg·m−3 | density |
| σ | N·m−1 | surface tension |
| ω | s−1 | characteristic eddy frequency |
References
- Marschall, H.; Mornhinweg, R.; Kossmann, A.; Oberhauser, S.; Langbein, K.; Hinrichsen, O. Numerical simulation of dispersed gas/liquid flows in bubble-columns at high phase fractions using OpenFOAM® part 1—Modeling fundamentals. Chem. Eng. Technol. 2010, 82, 2129–2140. [Google Scholar]
- Jakobsen, H.A.; Lindborg, H.; Dorao, C.A. Modeling of bubble-column reactors: Progress and limitations. Ind. Eng. Chem. Res. 2005, 44, 5107–5151. [Google Scholar] [CrossRef]
- Sokolichin, A.; Eigenberger, G.; Lapin, A. Simulation of buoyancy driven bubbly flow: Established simplifications and open questions. AIChE J. 2004, 50, 24–45. [Google Scholar] [CrossRef]
- Podowski, M. On the consistency of mechanistic multidimensional modeling of gas/liquid two-phase flows. Nucl. Eng. Des. 2009, 239, 933–940. [Google Scholar] [CrossRef]
- Serizawa, A.; Tomiyama, A. Some remarks on mechanisms of phase distribution in an adiabatic bubbly pipe flow. Multiph. Sci. Technol. 2003, 15, 79–98. [Google Scholar] [CrossRef]
- Masood, R.; Delgado, A. Numerical investigation of the interphase forces and turbulence closure in 3D square bubble columns. Chem. Eng. Sci. 2014, 108, 154–168. [Google Scholar] [CrossRef]
- Silva, M.K.; d’Avila, M.A.; Mori, M. Study of the interfacial forces and turbulence models in a bubble column. Comput. Chem. Eng. 2012, 44, 34–44. [Google Scholar] [CrossRef]
- Diaz, M.E.; Montes, F.J.; Galan, M.A. Influence of the lift force closures on the numerical simulation of bubble plumes in a rectangular bubble column. Chem. Eng. Sci. 2009, 64, 930–944. [Google Scholar] [CrossRef]
- Hibiki, T.; Ishii, M. Lift force in bubbly flow systems. Chem. Eng. Sci. 2007, 62, 6457–6474. [Google Scholar] [CrossRef]
- Selma, B.; Bannari, R.; Proulx, P. A full integration of a dispersion and interface closures in the standard k-epsilon model of turbulence. Chem. Eng. Sci. 2010, 65, 5417–5428. [Google Scholar] [CrossRef]
- Ekambara, K.; Dhotre, M. CFD simulation of bubble column. Nucl. Eng. Des. 2010, 240, 963–969. [Google Scholar] [CrossRef]
- Tabib, M.V.; Roy, A.R.; Joshi, J.B. CFD simulation of bubble column—An analysis of interphase forces and turbulence models. Chem. Eng. J. 2008, 139, 589–614. [Google Scholar] [CrossRef]
- Zhang, D.; Deen, N.G.; Kuipers, J.A.M. Numerical simulation of the dynamic flow behavior in a bubble column: A study of closures for turbulence and interface forces. Chem. Eng. Sci. 2006, 61, 7593–7608. [Google Scholar] [CrossRef]
- Politano, M.; Carrica, P.; Converti, J. A model for turbulent polydisperse two-phase flow in vertical channels. Int. J. Multiph. Flow 2003, 29, 1153–1182. [Google Scholar] [CrossRef]
- Troshko, A.A.; Hassan, Y.A. A Two-equation turbulence model of turbulent bubbly flows. Int. J. Multiph. Flow 2001, 27, 1965–2000. [Google Scholar] [CrossRef]
- Morel, C. Turbulence modeling and first numerical simulations in turbulent two-phase flows. In Proceedings of the Eleventh Symposium on Turbulent Shear Flows, Grenoble, France, 8–10 September 1997.
- Buffo, A.; Marchisio, D.L. Modeling and simulation of turbulent polydisperse gas-liquid systems via the generalized population balance equation. Rev. Chem. Eng. 2014, 30, 73–126. [Google Scholar] [CrossRef]
- Selma, B.; Bannari, R.; Proulx, P. Simulation of bubbly flows: Comparison between direct quadrature method of moments (DQMOM) and method of classes (CM). Chem. Eng. Sci. 2010, 65, 1925–1941. [Google Scholar] [CrossRef]
- Fox, R.O. Introduction and fundamentals of modeling approaches for polydisperse multiphase flows. In Multiphase Reacting Flows: Modelling and Simulation; Marchisio, D.L., Fox, R.O., Eds.; Springer-Verlag: Vienna, Austria, 2007. [Google Scholar]
- Sanyal, J.; Marchisio, D.L.; Fox, R.O.; Dhanasekharan, K. On the comparison between population balance models for CFD simulation of bubble columns. Ind. Eng. Chem. Res. 2005, 44, 5063–5072. [Google Scholar] [CrossRef]
- Sattar, M.A.; Naser, J.; Brooks, G. Numerical simulation of two-phase flow with bubble break-up and coalescence coupled with population balance modeling. Chem. Eng. Process. 2013, 70, 66–76. [Google Scholar] [CrossRef]
- Chen, P.; Sanyal, J.; Dudukovic, M. Numerical simulation of bubble columns flows: Effect of different breakup and coalescence closures. Chem. Eng. Sci. 2005, 60, 1085–1101. [Google Scholar] [CrossRef]
- Wang, T.; Wang, J.; Jin, Y. Population balance model for gas-liquid flows: Influence of bubble coalescence and breakup models. Ind. Eng. Chem. Res. 2005, 44, 7540–7549. [Google Scholar] [CrossRef]
- Sarhan, A.R.; Naser, J.; Brooks, G. CFD simulation on influence of suspended solid particles on bubbles’ coalescence rate in flotation cell. Int. J. Miner. Process. 2016, 146, 54–64. [Google Scholar] [CrossRef]
- Li, W.; Zhong, W. CFD simulation of hydrodynamics of gas-liquid-solid three-phase bubble column. Powder Technol. 2015, 286, 766–788. [Google Scholar] [CrossRef]
- Ojima, S.; Sasaki, S.; Hayashi, K.; Tomiyama, A. Effects of particle diameter on bubble coalescence in a slurry bubble column. J. Chem. Eng. Jpn. 2015, 48, 181–189. [Google Scholar] [CrossRef]
- Rzehak, R.; Krepper, E. Closure models for turbulent bubbly flows: A CFD study. Nucl. Eng. Des. 2013, 265, 701–711. [Google Scholar] [CrossRef]
- Rzehak, R.; Krepper, E. Bubbly flows with fixed polydispersity: Validation of a baseline closure model. Nucl. Eng. Des. 2015, 287, 108–118. [Google Scholar] [CrossRef]
- Rzehak, R.; Krepper, E.; Liao, Y.; Ziegenhein, T.; Kriebitzsch, S.; Lucas, D. Baseline Model for Simulation of Bubbly Flows. Chem. Eng. Technol. 2015, 38, 1972–1978. [Google Scholar] [CrossRef]
- Ziegenhein, T.; Lucas, D.; Rzehak, R.; Krepper, E. Closure relations for CFD simulation of bubble columns. In Proceedings of the 8th International Conference on Multiphase Flow (ICMF 2013), Jeju, Korea, 26–31 June 2013.
- Ziegenhein, T.; Rzehak, R.; Lucas, D. Transient simulation for large scale flow in bubble columns. Chem. Eng. Sci. 2015, 122, 1–13. [Google Scholar] [CrossRef]
- Rzehak, R.; Kriebitzsch, S.H.L. Multiphase CFD-simulation of bubbly pipe flow: A code comparison. Int. J. Multiph. Flow 2015, 68, 135–152. [Google Scholar] [CrossRef]
- Shawkat, M.; Ching, C.; Shoukri, M. Bubble and liquid turbulence characteristics of bubbly flow in a large diameter vertical pipe. Int. J. Multiph. Flow 2008, 34, 767–785. [Google Scholar] [CrossRef]
- Hosokawa, S.; Tomiyama, A. Multi-fluid simulation of turbulent bubbly pipe flows. Chem. Eng. Sci. 2009, 64, 5308–5318. [Google Scholar] [CrossRef]
- Rzehak, R.; Krepper, E. CFD modeling of bubble-induced turbulence. Int. J. Multiph. Flow 2013, 55, 138–155. [Google Scholar] [CrossRef]
- Liu, T.J. The role of bubble size on liquid phase turbulent structure in two-phase bubbly flow. In Proceedings of the 3rd International Conference on Multiphase Flow (ICMF’98), Lyon, France, 8–12 June 1998.
- Michiyoshi, I.; Serizawa, A. Turbulence in two-phase bubbly flow. Nucl. Eng. Des. 1986, 95, 253–267. [Google Scholar] [CrossRef]
- Wang, S.K.; Lee, S.J.; Jones, O.C., Jr.; Lahey, R.T., Jr. 3-D turbulence structure and phase distribution measurements in bubbly two-phase flows. Int. J. Multiph. Flow 1987, 13, 327–343. [Google Scholar] [CrossRef]
- Drew, D.A.; Passman, S.L. Theory of Multicomponent Fluids; Springer-Verlag: New York, NY, USA, 1998. [Google Scholar]
- Yeoh, G.H.; Tu, J.Y. Computational Techniques for Multiphase Flows—Basics and Applications; Butterworth-Heinemann: Oxford, UK, 2010. [Google Scholar]
- Ishii, M.; Hibiki, T. Thermo-Fluid Dynamics of Two-Phase Flow, 2nd ed.; Springer-Verlag: New York, NY, USA, 2011. [Google Scholar]
- Rzehak, R.; Ziegenhein, T.; Kriebitzsch, S.; Krepper, E.; Lucas, D. Unified modeling of bubbly flows in pipes, bubble columns, and airlift columns. Chem. Eng. Sci. 2016, in press. [Google Scholar] [CrossRef]
- Ishii, M.; Zuber, N. Drag coefficient and relative velocity in bubbly, droplet or particulate flows. AIChE J. 1979, 25, 843–855. [Google Scholar] [CrossRef]
- Tomiyama, A.; Tamai, H.; Zun, I.; Hosokawa, S. Transverse migration of single bubbles in simple shear flows. Chem. Eng. Sci. 2002, 57, 1849–1858. [Google Scholar] [CrossRef]
- Hosokawa, S.; Tomiyama, A.; Misaki, S.; Hamada, T. Lateral migration of single bubbles due to the presence of wall. In Proceedings of the ASME Joint U.S.-European Fluids Engineering Division Conference (FEDSM 2002), Montreal, QC, Canada, 14–18 July 2002.
- Rzehak, R.; Krepper, E.; Lifante, C. Comparative study of wall-force models for the simulation of bubbly flows. Nucl. Eng. Des. 2012, 252, 41–49. [Google Scholar] [CrossRef]
- Burns, A.D.; Frank, T.; Hamill, I.; Shi, J.-M. The Favre averaged drag model for turbulence dispersion in Eulerian multi-phase flows. In Proceedings of the 5th International Conference on Multiphase Flow (ICMF 2004), Yokohama, Japan, 30 May–4 June 2004.
- Sankaranarayanan, K.; Shan, X.; Kevrekidis, I.G.; Sundaresan, S. Analysis of drag and virtual mass forces in bubbly suspensions using an implicit formulation of the lattice Boltzmann method. J. Fluid Mech. 2002, 452, 61–96. [Google Scholar] [CrossRef]
- Menter, F.R. Review of the shear-stress transport turbulence model experience from an industrial perspective. Int. J. Comput. Fluid Dyn. 2009, 23, 305–316. [Google Scholar] [CrossRef]
- OpenCFD. OpenFOAM User Guide v2.2.2; OpenFOAM Foundation: London, UK, 2013. [Google Scholar]
- Kalitzin, G.; Medic, G.; Iaccarino, G.; Durbin, P. Near-wall behavior of RANS turbulence models and implications for wall functions. J. Comput. Phys. 2005, 204, 265–291. [Google Scholar] [CrossRef]
- Knopp, T. Model-consistent universal wall-functions for RANS turbulence modelling. In Proceedings of the International Conference on Boundary and Interior Layers (BAIL 2006), Göttingen, Germany, 24–28 July 2006.
- Clift, R.; Grace, J.R.; Weber, M.E. Bubbles, Drops and Particles; Acadamic Press: New York, NY, USA, 1978. [Google Scholar]
- Tomiyama, A.; Kataoka, I.; Zun, I.; Sakaguchi, T. Drag coefficients of single bubbles under normal and micro gravity conditions. JSME Int. J. Ser. B 1998, 41, 472–479. [Google Scholar] [CrossRef]
- Dijkhuizen, W.; Roghair, I.; van Sint Annaland, M.; Kuipers, J.A.M. DNS of gas bubbles behaviour using an improved 3D front tracking model—Drag force on isolated bubbles and comparison with experiments. Chem. Eng. Sci. 2010, 65, 1415–1426. [Google Scholar] [CrossRef]
- Duineveld, P.C. The rise velocity and shape of bubbles in pure water at high Reynolds number. J. Fluid Mech. 1995, 292, 325–332. [Google Scholar] [CrossRef]
- Veldhuis, C. Leonardo’s Paradox: Path and Shape Instabilities of Particles and Bubbles. Ph.D. Thesis, University of Twente, Enschede, The Netherlands, 7 February 2007. [Google Scholar]
- Haberman, W.; Morton, R. An Experimental Investigation of the Drag and Shape of Air Bubbles Rising in Various Liquids; Report 802; The David W. Taylor Model Basin: Washington, DC, USA, 1953. [Google Scholar]
- Houghton, G.; Ritchie, P.; Thomson, J. Velocity of rise of air bubbles in sea-water, and their types of motion. Chem. Eng. Sci. 1957, 7, 111–112. [Google Scholar] [CrossRef]
- Okawa, T.; Torimoto, K.; Nishiura, M.; Kataoka, I.; Mori, M. Effects of turbulence and containing wall on rise velocities of single bubbles in a vertical circular pipe. In Proceedings of the 10th International Conference on Nuclear Engineering (ICONE10), Arlington, VA, USA, 14–18 April 2002.
- Ghatage, S.V.; Sathe, M.J.; Doroodchi, E.; Joshi, J.B.; Evans, G.M. Effect of turbulence on particle and bubble slip velocity. Chem. Eng. Sci. 2013, 100, 120–136. [Google Scholar] [CrossRef]
- Simonnet, M.; Gentric, C.; Olmos, E.; Midoux, N. Experimental determination of the drag coefficient in a swarm of bubbles. Chem. Eng. Sci. 2007, 62, 858–866. [Google Scholar] [CrossRef]
- Roghair, I.; van Sint Annaland, M.; Kuipers, J.A.M. Drag force and clustering in bubble swarms. AIChE J. 2013, 59, 1791–1800. [Google Scholar] [CrossRef]
- Serizawa, A.; Kataoka, I.; Michiyoshi, I. Turbulence structure of air-water bubbly flow—I. Measuring techniques. Int. J. Multiph. Flow 1975, 2, 221–233. [Google Scholar] [CrossRef]
- Serizawa, A.; Kataoka, I.; Michiyoshi, I. Turbulence structure of air-water bubbly flow—II. Local properties. Int. J. Multiph. Flow 1975, 2, 235–246. [Google Scholar] [CrossRef]
- Serizawa, A.; Kataoka, I.; Michiyoshi, I. Turbulence structure of air-water bubbly flow—III. Transport properties. Int. J. Multiph. Flow 1975, 2, 247–259. [Google Scholar] [CrossRef]
- Serizawa, A.; Kataoka, I.; Michiyoshi, I. Experimental data set No. 24: Phase distribution in bubbly flow. Multiph. Sci. Technol. 1992, 6, 257–301. [Google Scholar] [CrossRef]
- Liu, T.; Bankoff, S. Structure of air–water bubbly flow in a vertical pipe. I: Liquid mean velocity and turbulence measurements. Int. J. Heat Mass Transf. 1993, 36, 1049–1060. [Google Scholar] [CrossRef]
- Liu, T.; Bankoff, S. Structure of air–water bubbly flow in a vertical pipe. II: Gas fraction, bubble velocity and bubble size distribution. Int. J. Heat Mass Transf. 1993, 36, 1061–1072. [Google Scholar] [CrossRef]
- Samstag, M. Experimentelle Untersuchungen von Transportphänomenen in Vertikalen Turbulenten Luft-Wasser-Blasenströmungen. Ph.D. Thesis, University Karlsruhe, Karlsruhe, Germany, 1996. [Google Scholar]
- Mudde, R.F.; Saito, T. Hydrodynamical similarities between bubble column and bubbly pipe flow. J. Fluid Mech. 2001, 437, 203–228. [Google Scholar] [CrossRef]
- Fujiwara, A.; Minato, D.; Hishida, K. Effect of bubble diameter on modification of turbulence in an upward pipe flow. Int. J. Heat Fluid Flow 2004, 25, 481–488. [Google Scholar] [CrossRef]
- Colin, C.; Fabre, J.; Kamp, A. Turbulent bubbly flow in pipe under gravity and microgravity conditions. J. Fluid Mech. 2012, 711, 469–515. [Google Scholar] [CrossRef]
- Ziegenhein, T.; Rzehak, R.; Krepper, E.; Lucas, D. Numerical simulation of polydispersed flow in bubble columns with the inhomogeneous multi-size-group model. Chem. Ing. Tech. 2013, 85, 1080–1091. [Google Scholar] [CrossRef]
- Colombo, M.; Fairweather, M. Multiphase turbulence in bubbly flows: RANS simulations. Int. J. Multiph. Flow. 2015, 77, 222–243. [Google Scholar] [CrossRef]
- Ekambara, K.; Sean Sanders, R.; Nandakumar, K.; Masliyah, J.H. CFD Modeling of gas-liquid bubbly flow in horizontal pipes: Influence of bubble-coalescence and breakup. Int. J. Chem. Eng. 2012, 2012, 620643:1–620643:20. [Google Scholar] [CrossRef]
- Liao, Y.; Rzehak, R.; Lucas, D.; Krepper, E. Baseline closure model for dispersed bubbly flow: Bubble-coalescence and breakup. Chem. Eng. Sci. 2015, 122, 336–349. [Google Scholar] [CrossRef]
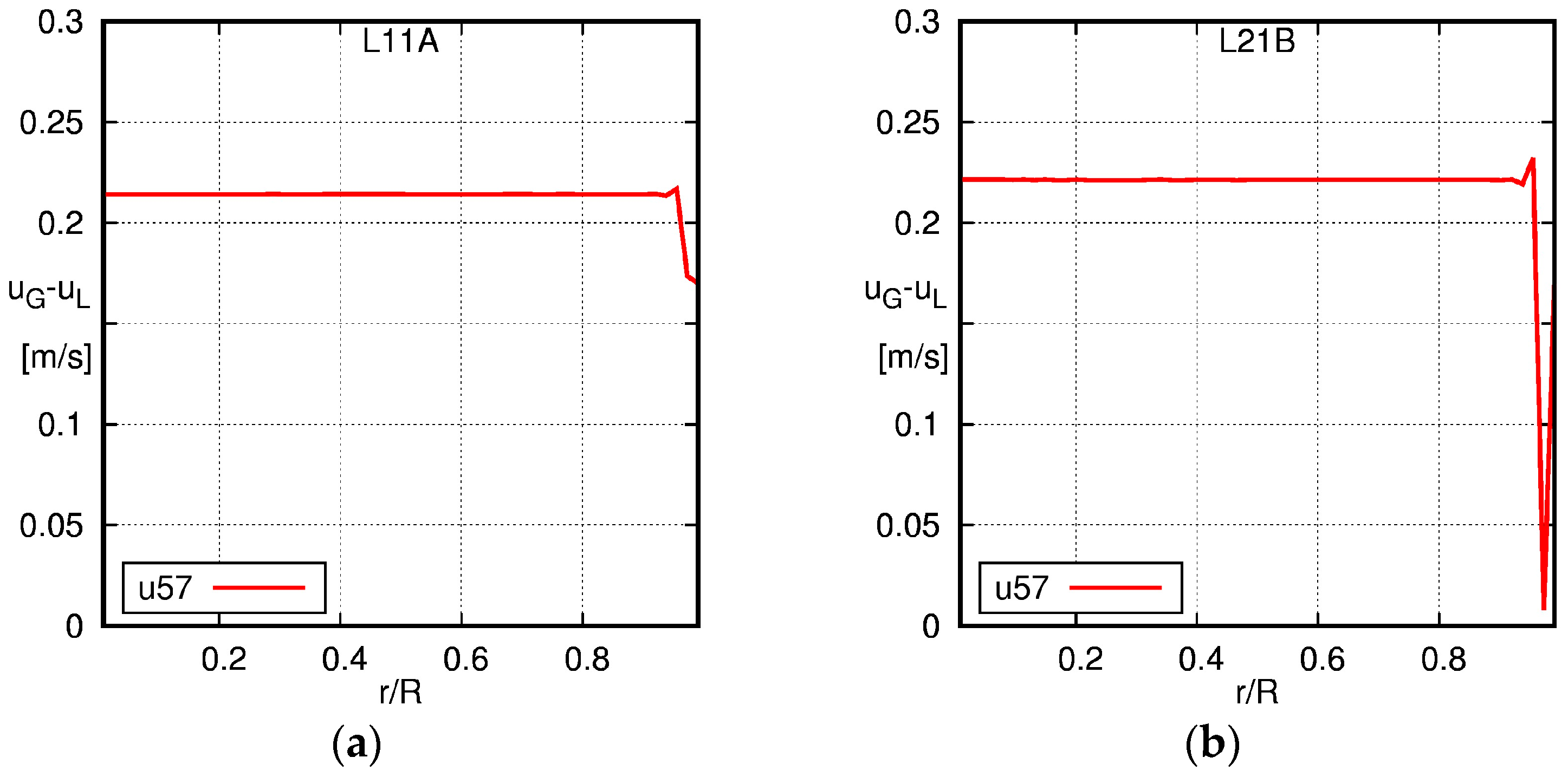
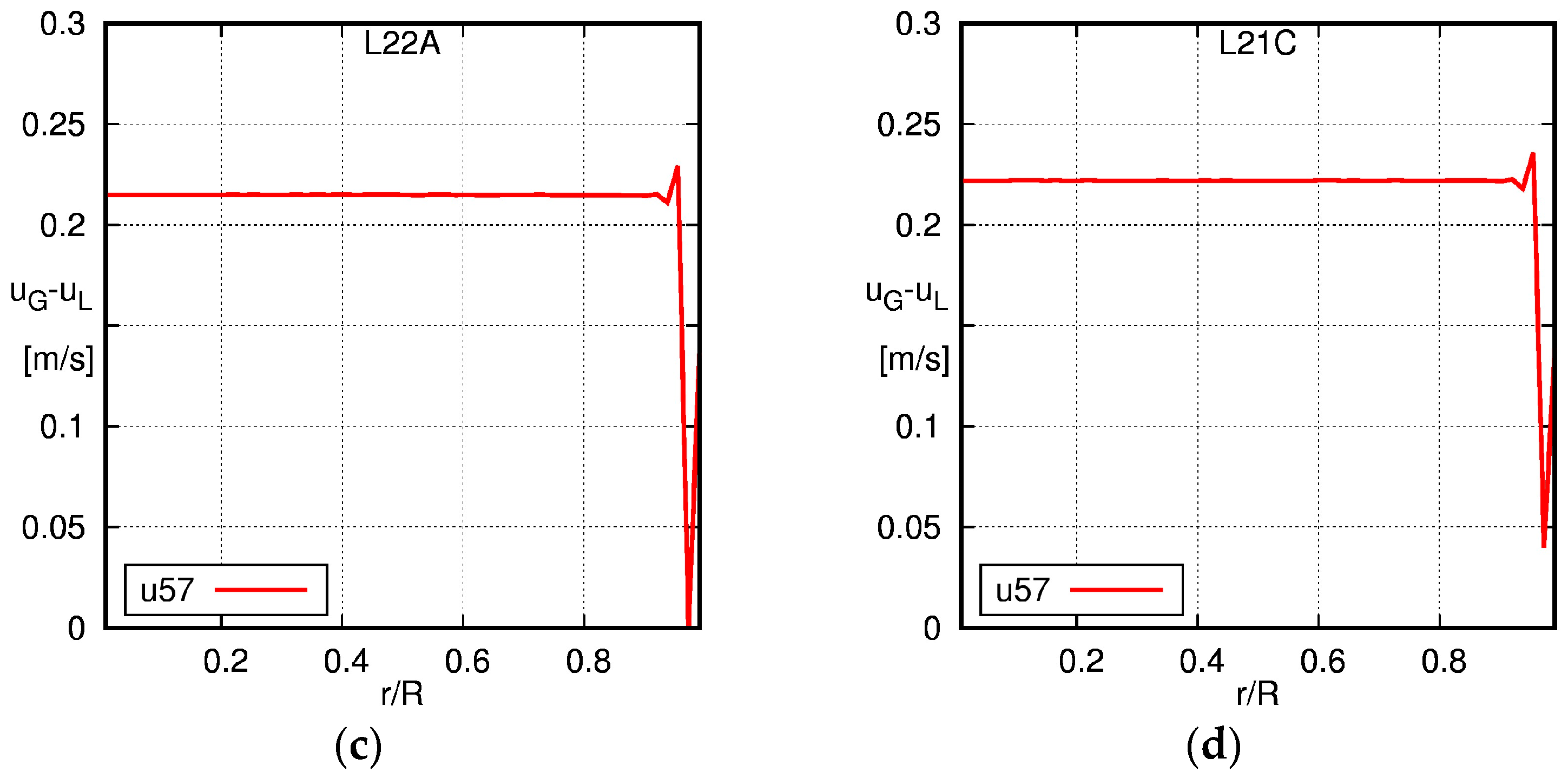
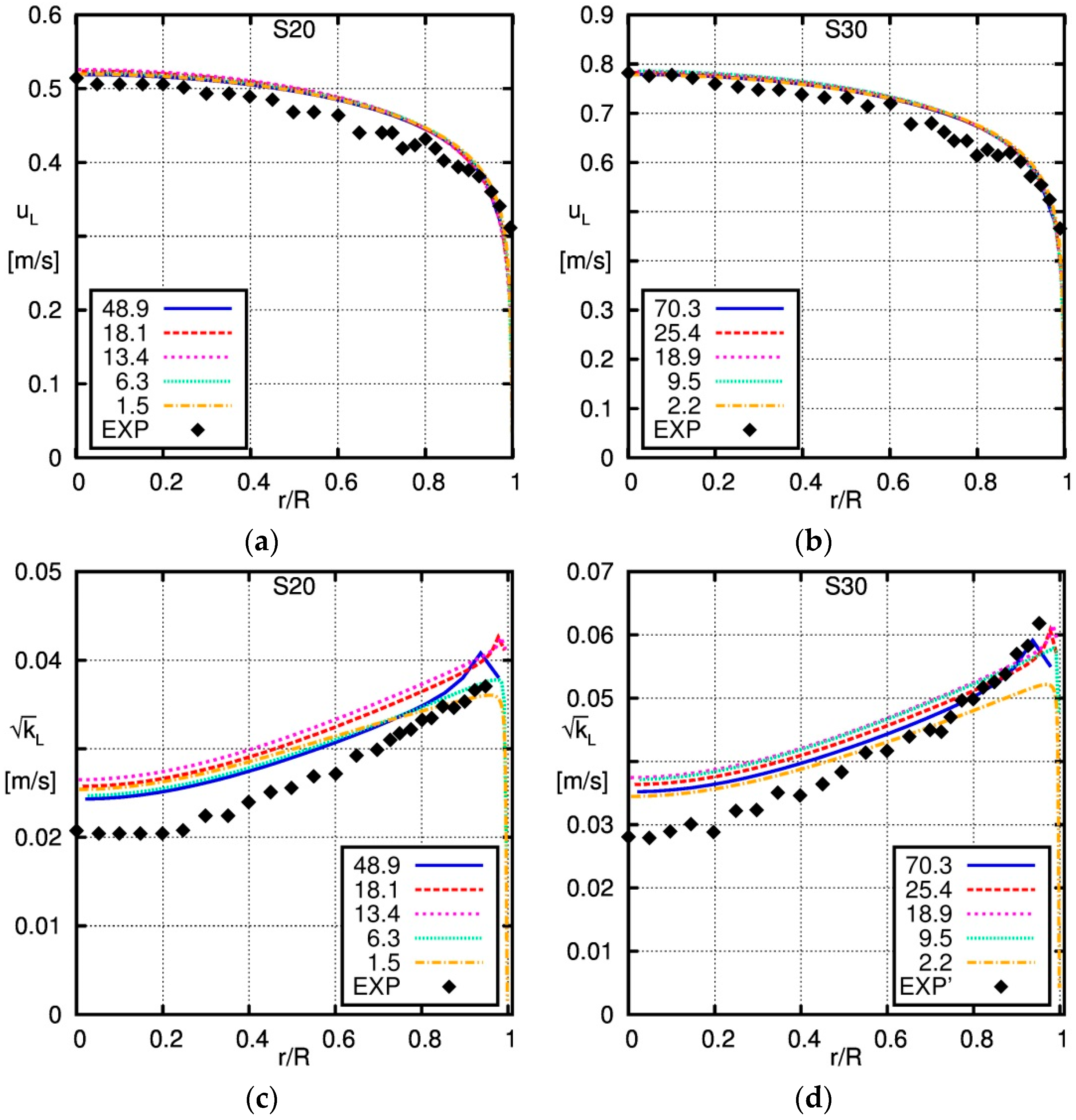
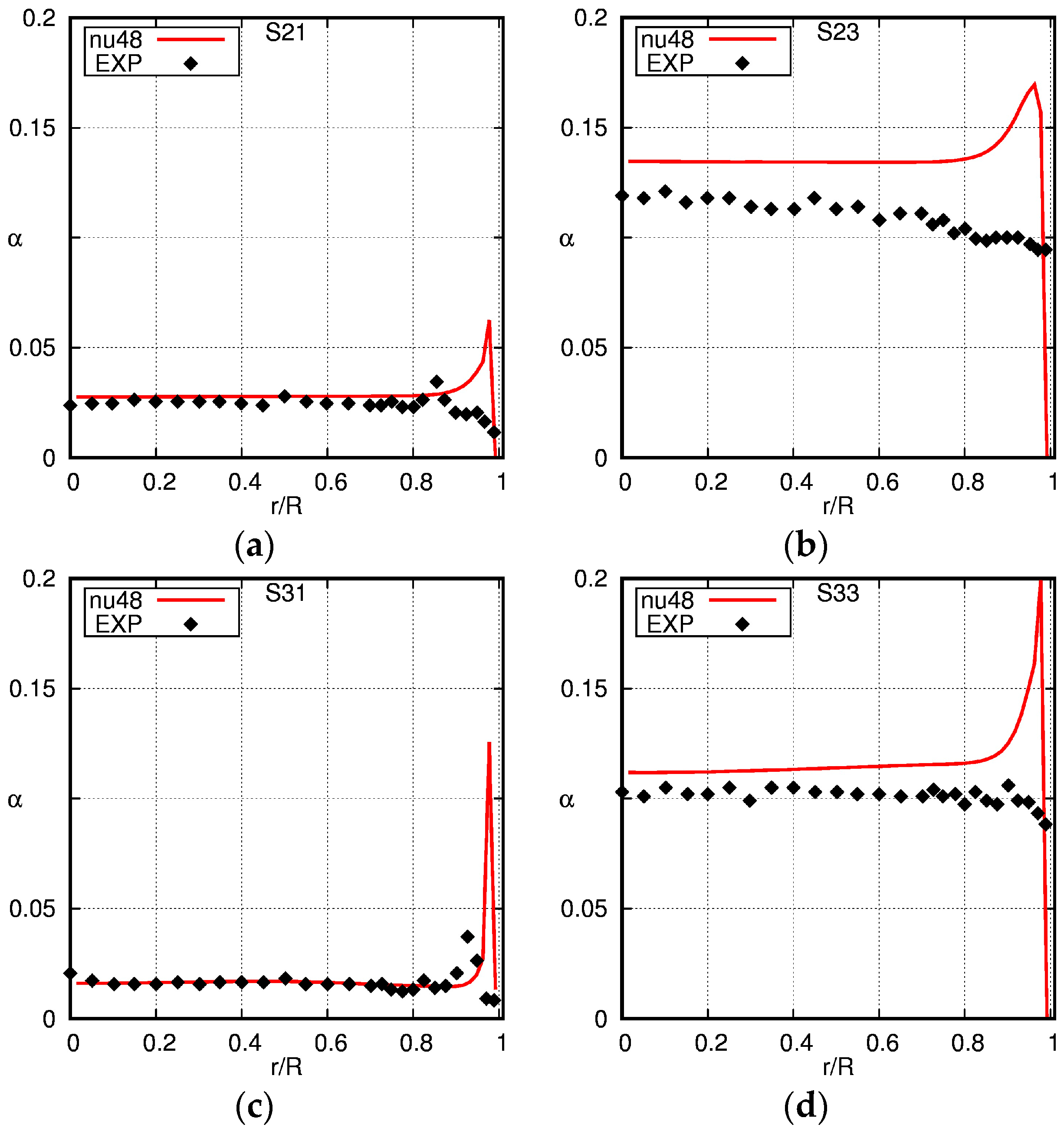
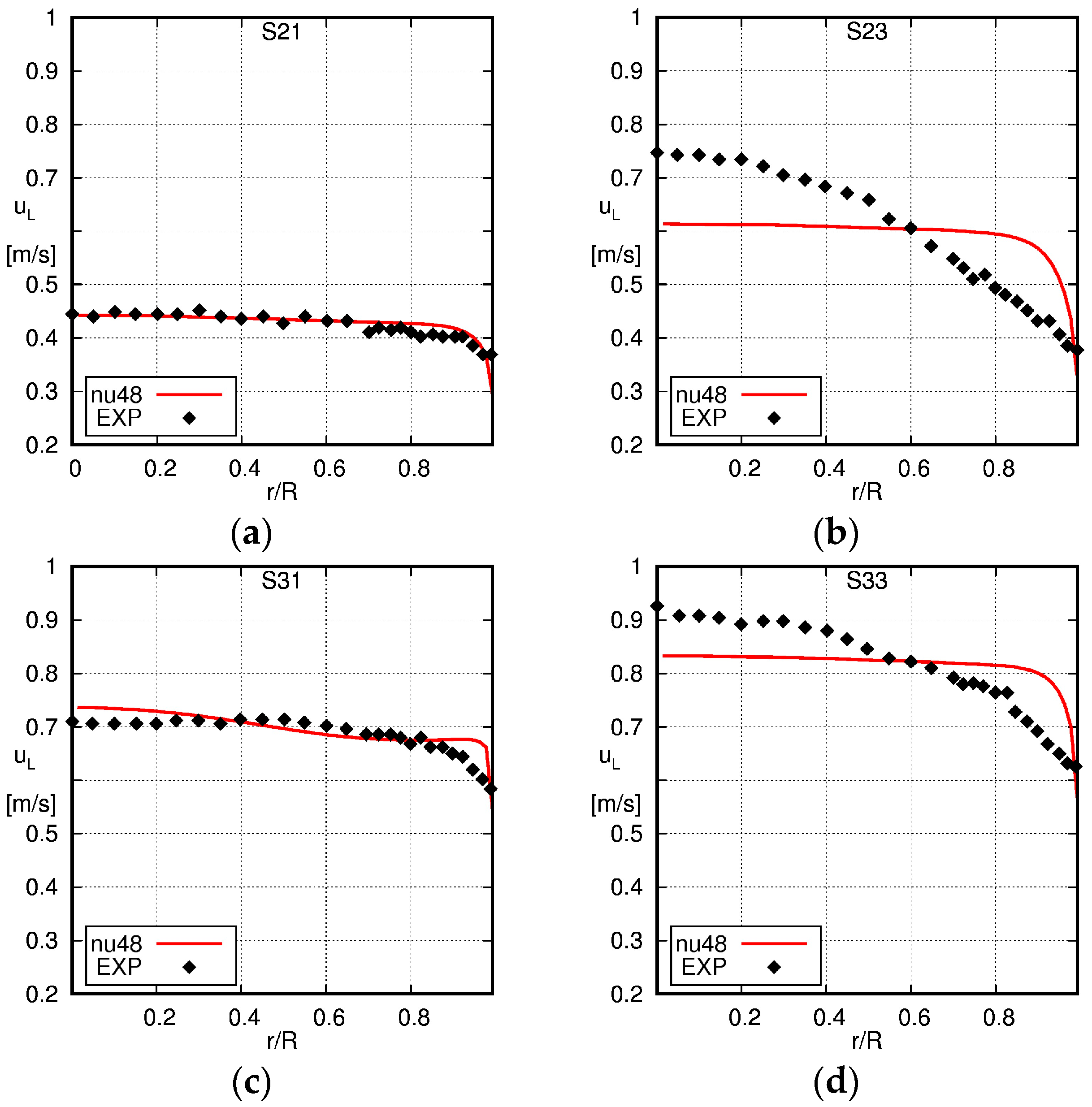
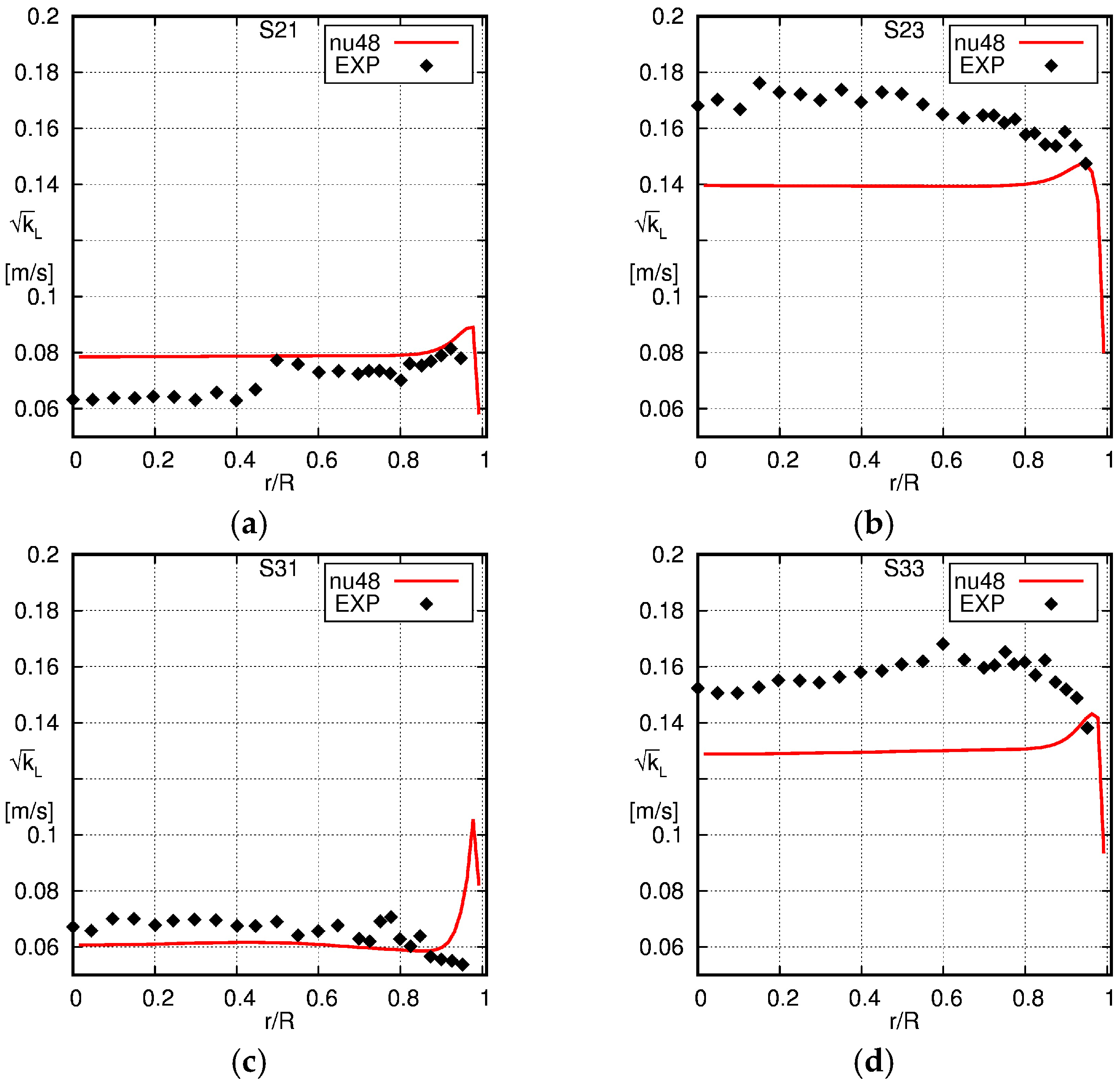
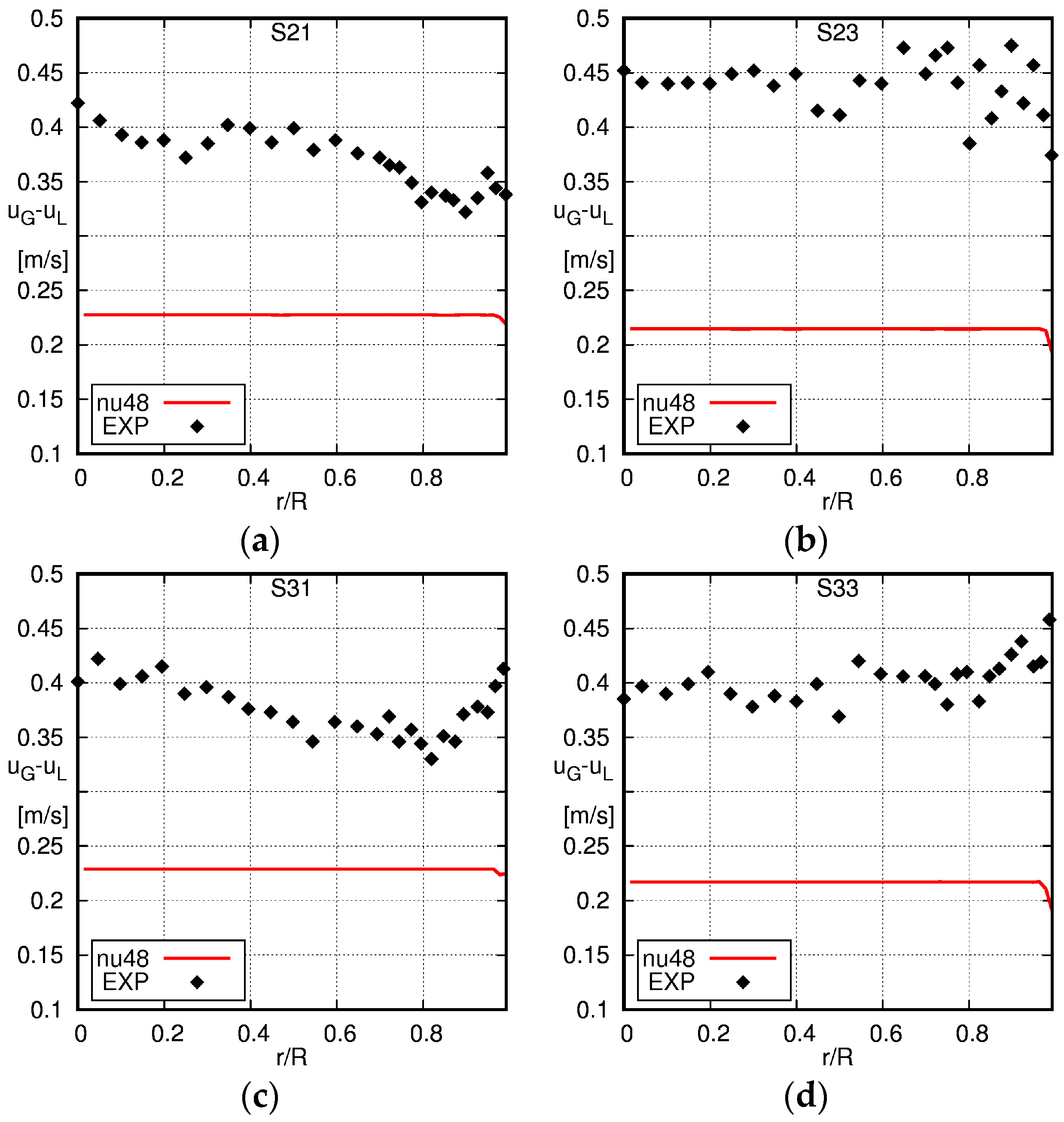
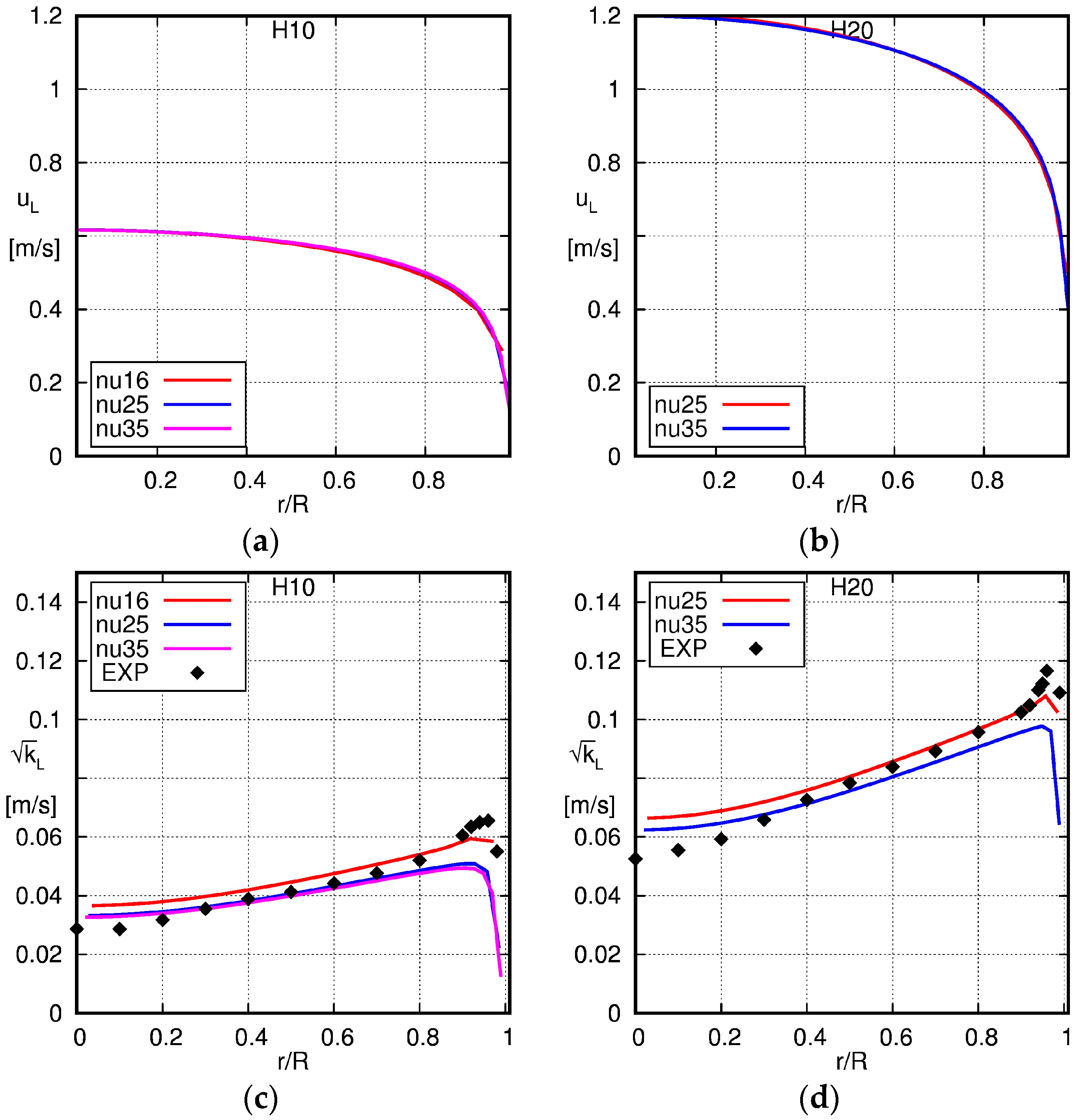
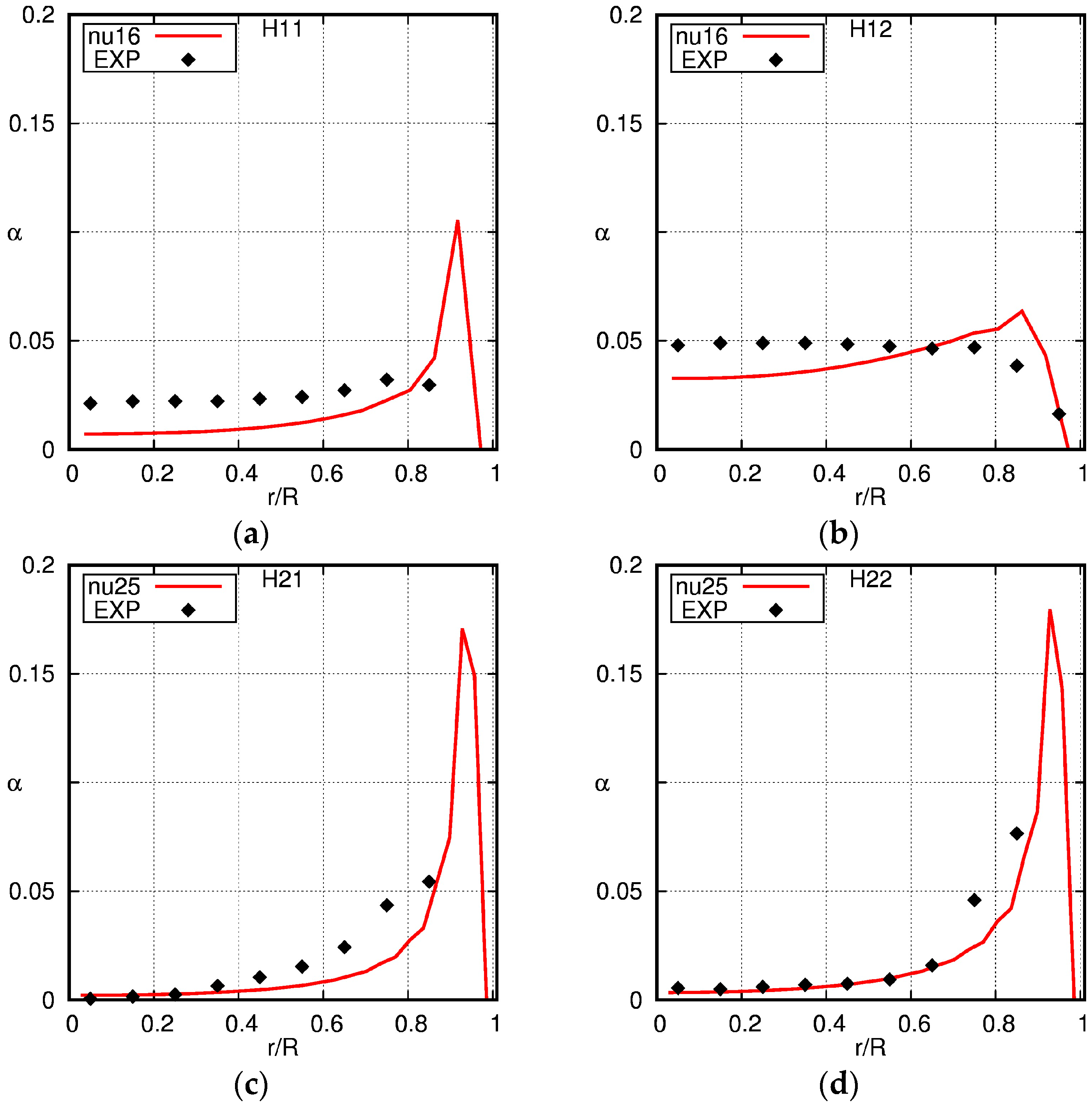


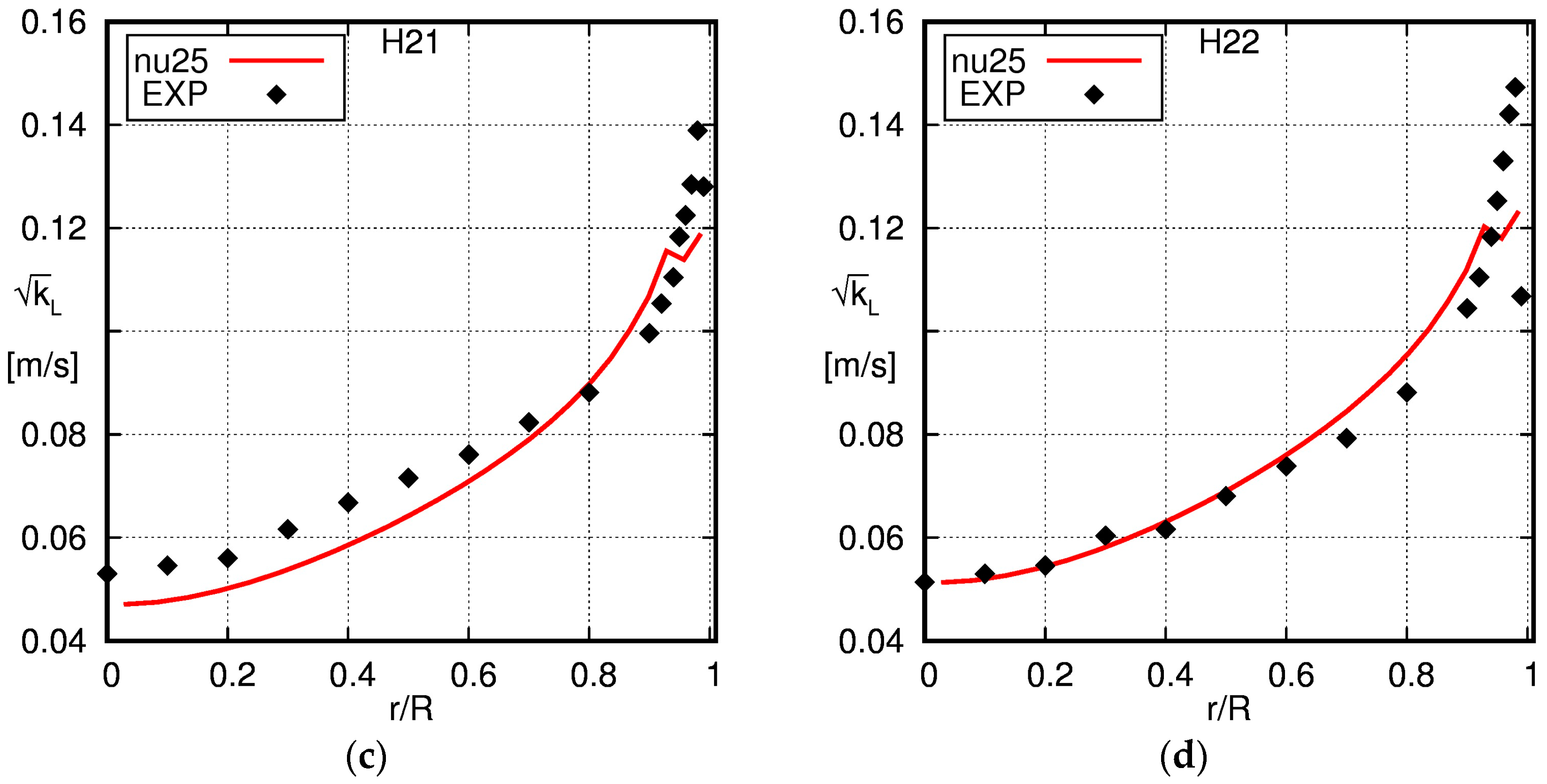

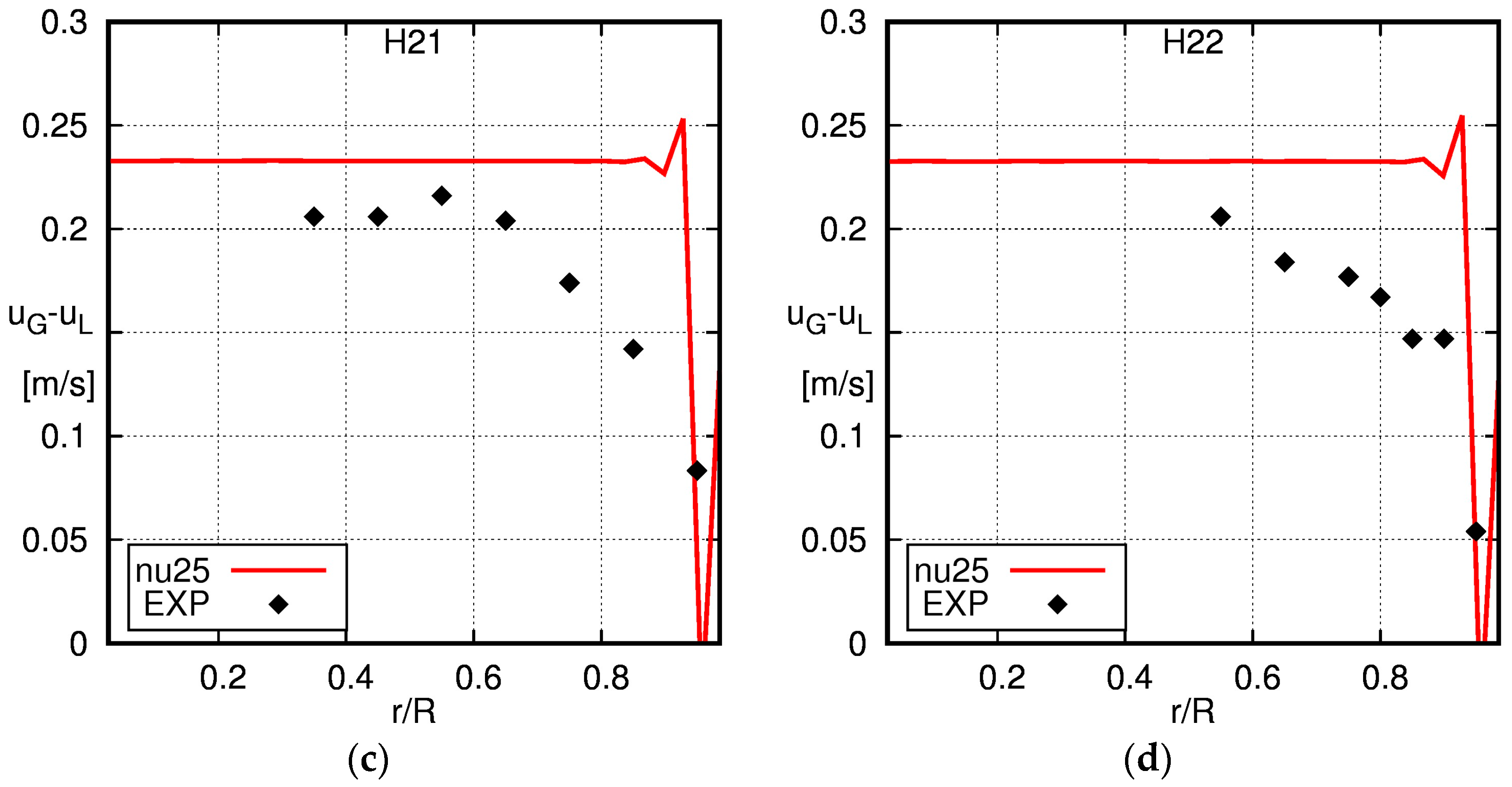

| Name | Pipe Diameter | JL (nom) | JG (nom) | JL (adj) | JG (adj) | <dB> | <αG> |
|---|---|---|---|---|---|---|---|
| mm | m/s | m/s | m/s | m/s | mm | % | |
| H11 | 25.0 | 0.5 | 0.018 | 0.5 | 0.018 | 3.2 | 2.5 |
| H12 | 25.0 | 0.5 | 0.025 | 0.5 | 0.031 | 4.3 | 4.1 |
| H21 | 25.0 | 1.0 | 0.020 | 1.0 | 0.035 | 3.5 | 2.8 |
| H22 | 25.0 | 1.0 | 0.036 | 1.0 | 0.042 | 3.7 | 3.2 |
| L11A | 57.2 | 0.5 | 0.1 | 0.5 | 0.12 | 2.9 | 15.2 |
| L21B | 57.2 | 1.0 | 0.1 | 1.0 | 0.14 | 3.0 | 10.6 |
| L21C | 57.2 | 1.0 | 0.1 | 1.0 | 0.13 | 4.2 | 9.6 |
| L22A | 57.2 | 1.0 | 0.2 | 1.0 | 0.22 | 3.9 | 15.7 |
| S21 | 200 | 0.45 | 0.015 | 0.41 | 0.019 | 4.1 | 2.4 |
| S23 | 200 | 0.45 | 0.1 | 0.5 | 0.108 | 5.0 | 10.7 |
| S31 | 200 | 0.68 | 0.015 | 0.67 | 0.018 | 3.2 | 1.7 |
| S33 | 200 | 0.68 | 0.1 | 0.71 | 0.12 | 4.7 | 10.1 |
| ρL | 997.0 | kg·m−3 |
| µL | 8.899 × 10 −4 | kg m−1·s−1 |
| ρG | 1.185 | kg·m−3 |
| µG | 1.831 × 10 −5 | kg·m−1·s−1 |
| σ | 0.072 | N·m−1 |
| Grid | nz | nr | y+ | |||
|---|---|---|---|---|---|---|
| S20 | S30 | |||||
| u24 | 224 | 24 | 1.0 | 4.167 | 48.9 | 70.3 |
| nu48 | 448 | 48 | 0.5 | 1.443 | 18.1 | 25.4 |
| u96 | 896 | 96 | 1.0 | 1.042 | 13.4 | 18.9 |
| nu48 | 448 | 48 | 0.1 | 0.528 | 6.3 | 9.5 |
| nu192 | 448 | 192 | 0.1 | 0.133 | 1.5 | 2.2 |
| Grid | nz | nr | y+ | |||
|---|---|---|---|---|---|---|
| H10 | H20 | |||||
| nu16 | 399 | 16 | 0.75 | 0.674 | 12.5 | xx |
| nu25 | 399 | 25 | 0.5 | 0.346 | 5.9 | 11.6 |
| nu35 | 399 | 35 | 0.5 | 0.247 | 4.2 | 7.8 |
© 2016 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution license ( http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kriebitzsch, S.; Rzehak, R. Baseline Model for Bubbly Flows: Simulation of Monodisperse Flow in Pipes of Different Diameters. Fluids 2016, 1, 29. https://doi.org/10.3390/fluids1030029
Kriebitzsch S, Rzehak R. Baseline Model for Bubbly Flows: Simulation of Monodisperse Flow in Pipes of Different Diameters. Fluids. 2016; 1(3):29. https://doi.org/10.3390/fluids1030029
Chicago/Turabian StyleKriebitzsch, Sebastian, and Roland Rzehak. 2016. "Baseline Model for Bubbly Flows: Simulation of Monodisperse Flow in Pipes of Different Diameters" Fluids 1, no. 3: 29. https://doi.org/10.3390/fluids1030029
APA StyleKriebitzsch, S., & Rzehak, R. (2016). Baseline Model for Bubbly Flows: Simulation of Monodisperse Flow in Pipes of Different Diameters. Fluids, 1(3), 29. https://doi.org/10.3390/fluids1030029





