Helmet Radio Frequency Phased Array Applicators Enhance Thermal Magnetic Resonance of Brain Tumors
Abstract
1. Introduction
2. Materials and Methods
2.1. RF Applicator Design
2.1.1. MRI Considerations
2.1.2. RF Heating Considerations
2.1.3. RF Antenna Building Block
2.1.4. RF Phased Array
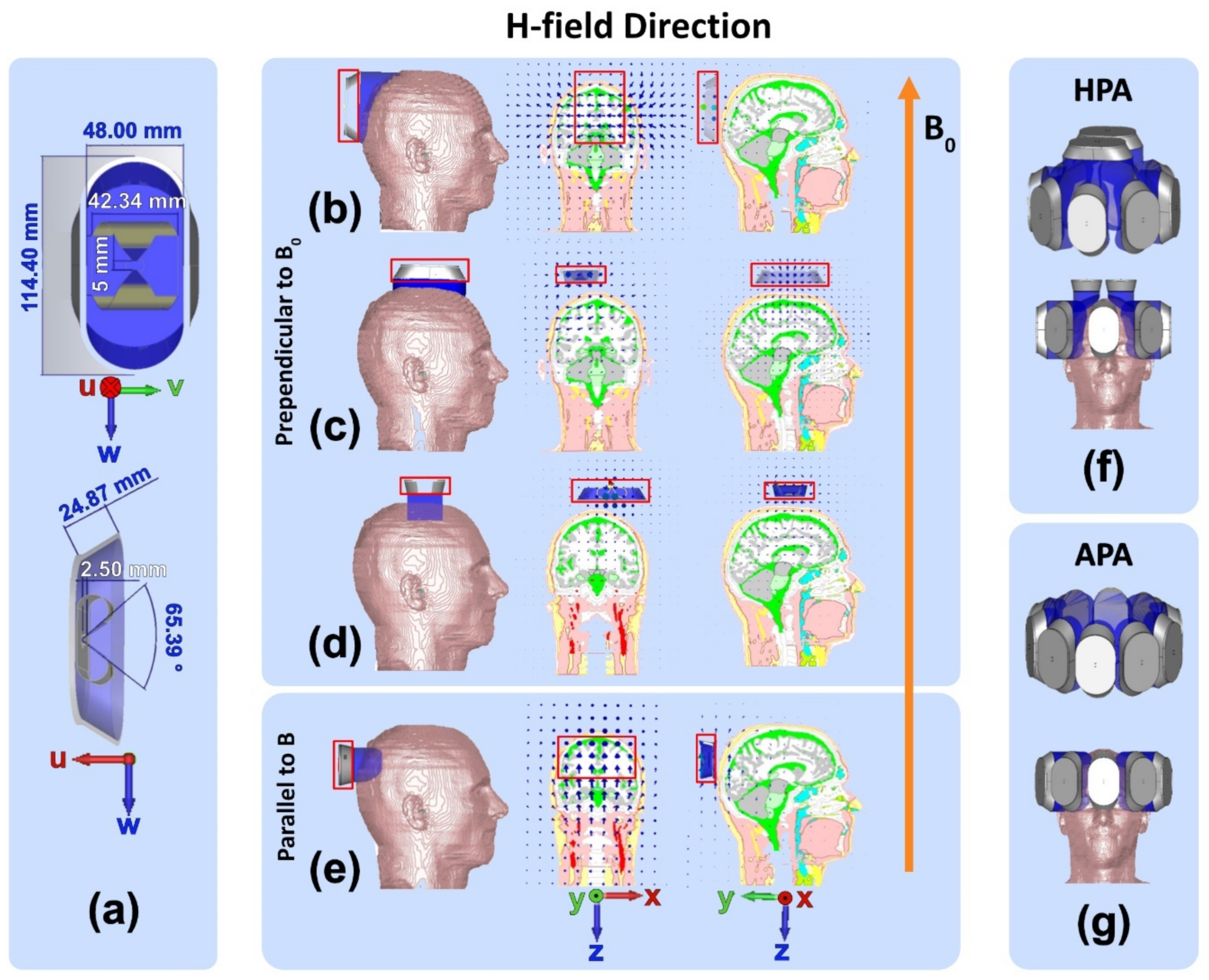
- Annular Phased RF array (APA, Figure 2g): Ten SGBT RF building blocks were placed equidistantly in a single-row annular array where the w-axis of the SGBT elements is aligned with the z-axis of the main magnetic field B0.
- Helmet Phased RF array (HPA, Figure 2f): Eight SGBT RF building blocks shown in Figure 2b were arranged equidistantly in a single-row annular array. Two SGBT RF building blocks, shown in Figure 2c, were placed on top of an eight-elements APA. This approach takes the elliptical shape of the human head into account and aligns the long axis of the SGBT with the longest axis of the head for the benefit of better head/brain coverage.
2.2. Numerical Simulations
2.2.1. EMF Simulations
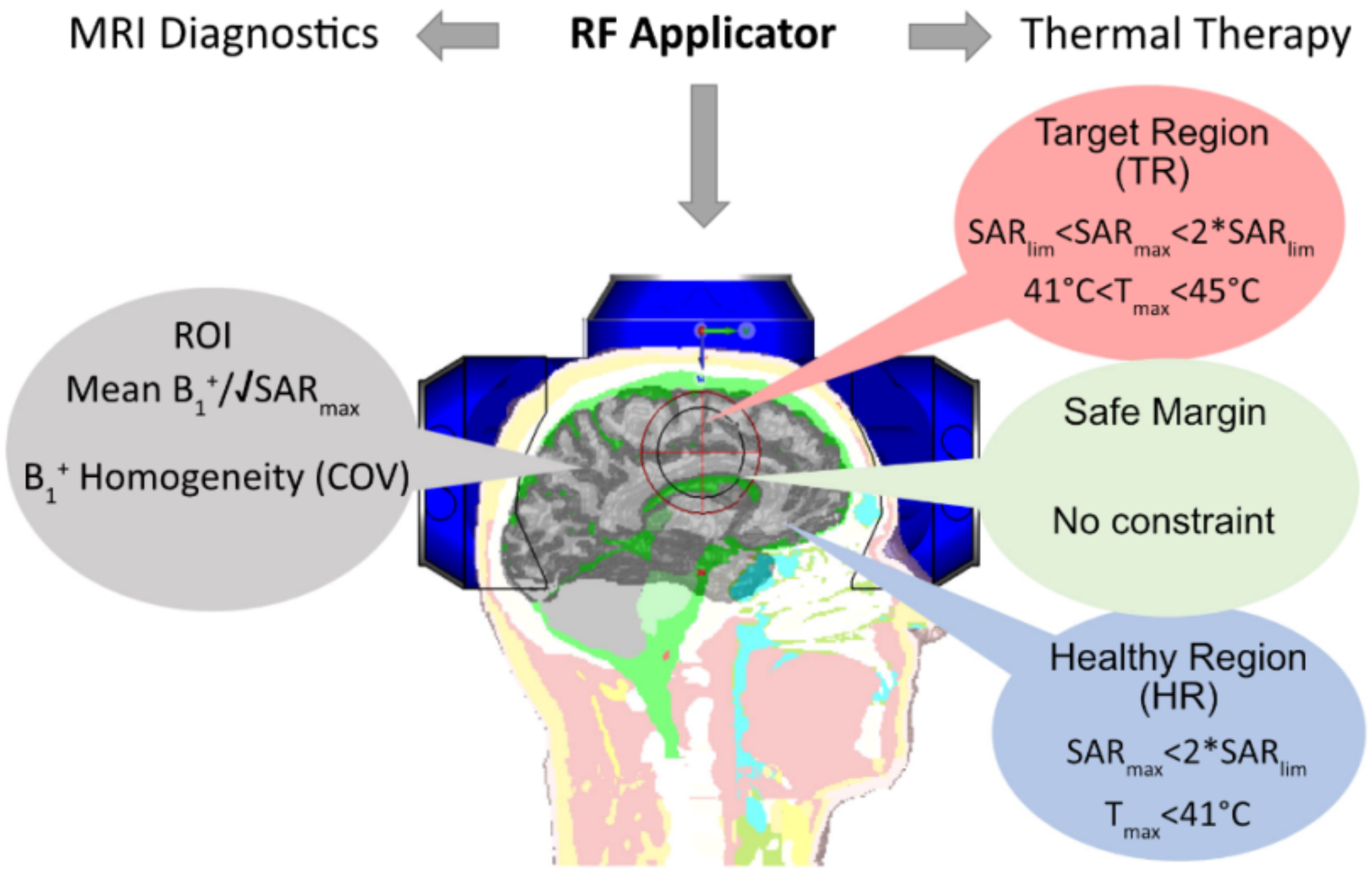
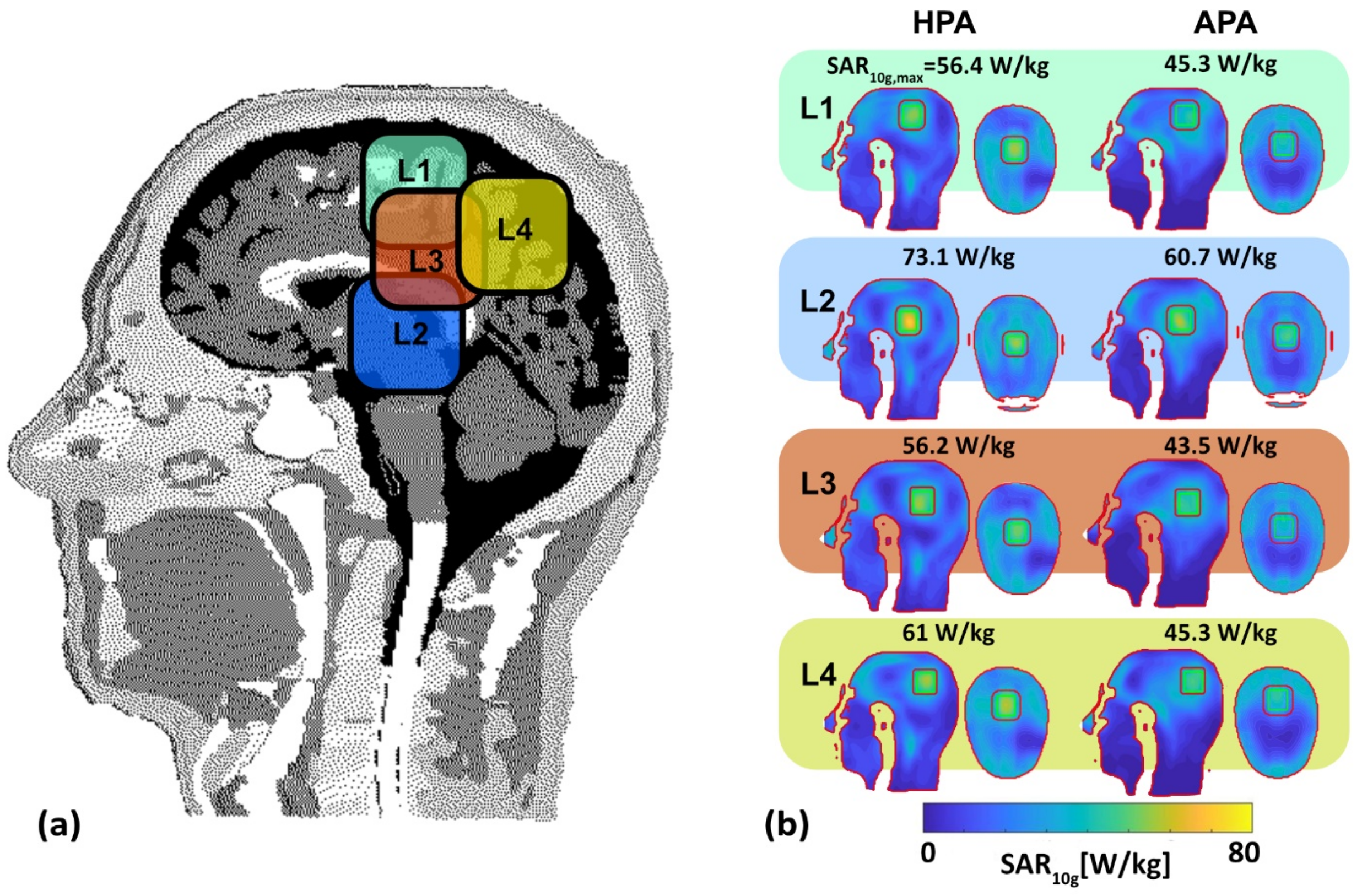
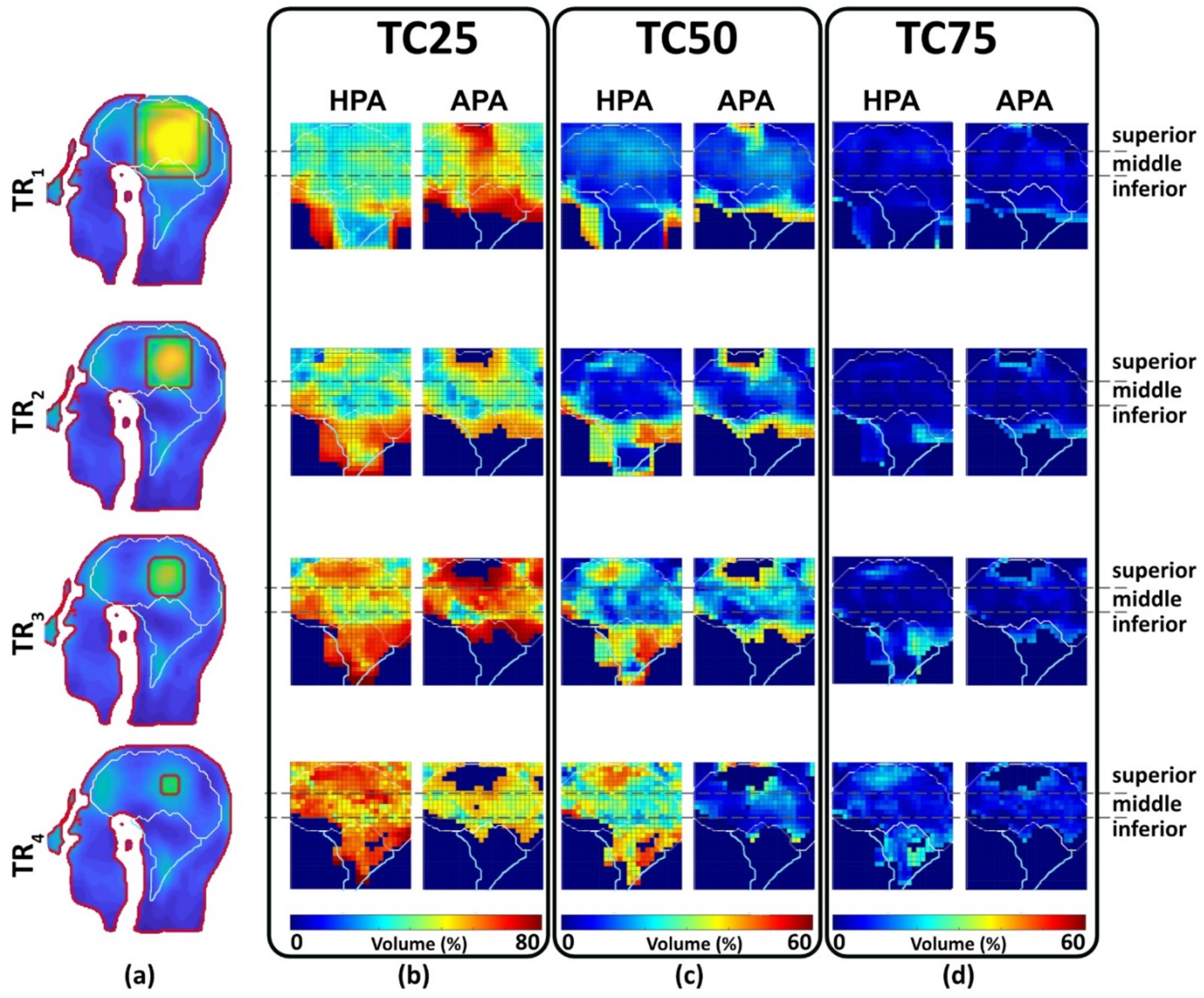
- CST Microwave Studio Suite 2020 (Dassault Systèmes, Darmstadt, Germany) [10,52]: EMF simulations were performed using broadband excitation (f = 297.2 ± 50 MHz) and the time-domain solver based on the finite integration technique (FIT) mesh size of 1.75 mm × 1.75 mm × 1.75 mm was used for the antenna. EMF simulations were applied to the human head voxel model Duke of the virtual family truncated at the level of the neck (IT’IS Foundation, Zurich, Switzerland [53]) (resolution of 1.0 × 1.0 × 1.0 mm3) and placed at the isocenter of a RF shield model of the bore of a 7.0 T MRI system. For the human voxel model, SAR10g (SAR calculations were averaged over 10 g of tissue or phantom material (SAR10g)) was evaluated for cuboid target regions (TR). For this purpose, four TR sizes with a main target region (mm3) and a gap between the target margin and safety margin (mm3) were defined: TR1 = 87.5 mm × 87.5 mm × 4 mm (22 mm), TR2 = 62.5 mm × 62.5 mm × 4 mm (10 mm); TR3 = 37.5 mm × 37.5 mm × 4 mm (10 mm); TR4 = 15 mm × 15 mm × 4 mm (10 mm). The cuboid TRs were chosen over cylindrical or spherical TRs to make the TR more tumor shaped, unpredictable, with some corners, independent from the symmetric applicator arrangement of a circular array, and more challenging than a cylindrical TR. To examine the homogeneity of SAR10g, the metric target coverage (TC) describes the target volume that covers xx% (xx = 25, 50, and 75) of the maximum SAR10g (SAR10g,max) inside the TR.
- Sim4Life Version 7.0.2 (Zurich Med Tech, Zurich, Switzerland). The Electromagnetics Full Wave Solvers finite-difference time-domain (P-EM-FDTD) was used for EM modeling (f = 297.2 ± 50 MHz). Thermodynamic Solvers (P-THERMAL) based on FDTD and a steady-state finite volume were used for advanced perfusion and thermoregulation modeling. The P-Thermal solver utilizes the Poisson differential equation to model heat transfer in living tissue, accommodating a range of adaptable boundary conditions. The transient thermal solver assumes a dynamic state where all tissue domains possess non-zero thermal conductivity or heat transfer rates. This software package supports the import of segmented real-world data obtained from computed tomography (CT) or MRI into a human voxel model. It also provides a comprehensive library of thermal and electrical tissue properties for a human model. For the simulations, clinical tumor geometry data obtained from a GBM patient were integrated into the human voxel model truncated at the level of the neck. For this purpose, a CT scan of a GBM patient was imported into Sim4Life [10]. Dielectric and thermal properties of up to 20 labeled tissue [10] used for radiotherapy planning including the GBM (volume = 172 mL, σ = 1.15 S/m, ε = 66.5 [54]) were assigned to the head geometry of the patient (headmass = 3.68 kg). Adding the cerebrospinal fluid (CSF) layer was accomplished by upscaling the brain by 5% [10]. The excitation center frequency and bandwidth were set to 297.2 ± 50 MHz. The mesh size, regarding the voxel shaping of the antenna in Sim4Life was limited to a maximum step size of 3 mm within the skull and 5 mm within the lower region of the modified human head voxel model. A much finer resolution of down to 0.1 mm was applied to the feeding points to resolve the triangular shape of the SGBT.
2.2.2. Temperature Simulations
2.3. RF Circuit Co-Simulation
2.4. Electromagnetic Field Shaping
2.4.1. Transmission Field Shimming for MRI
2.4.2. RF Excitation Vector Optimization for RF Heating and Hyperthermia Treatment Planning
2.5. Evaluation and Benchmarking
2.5.1. MRI
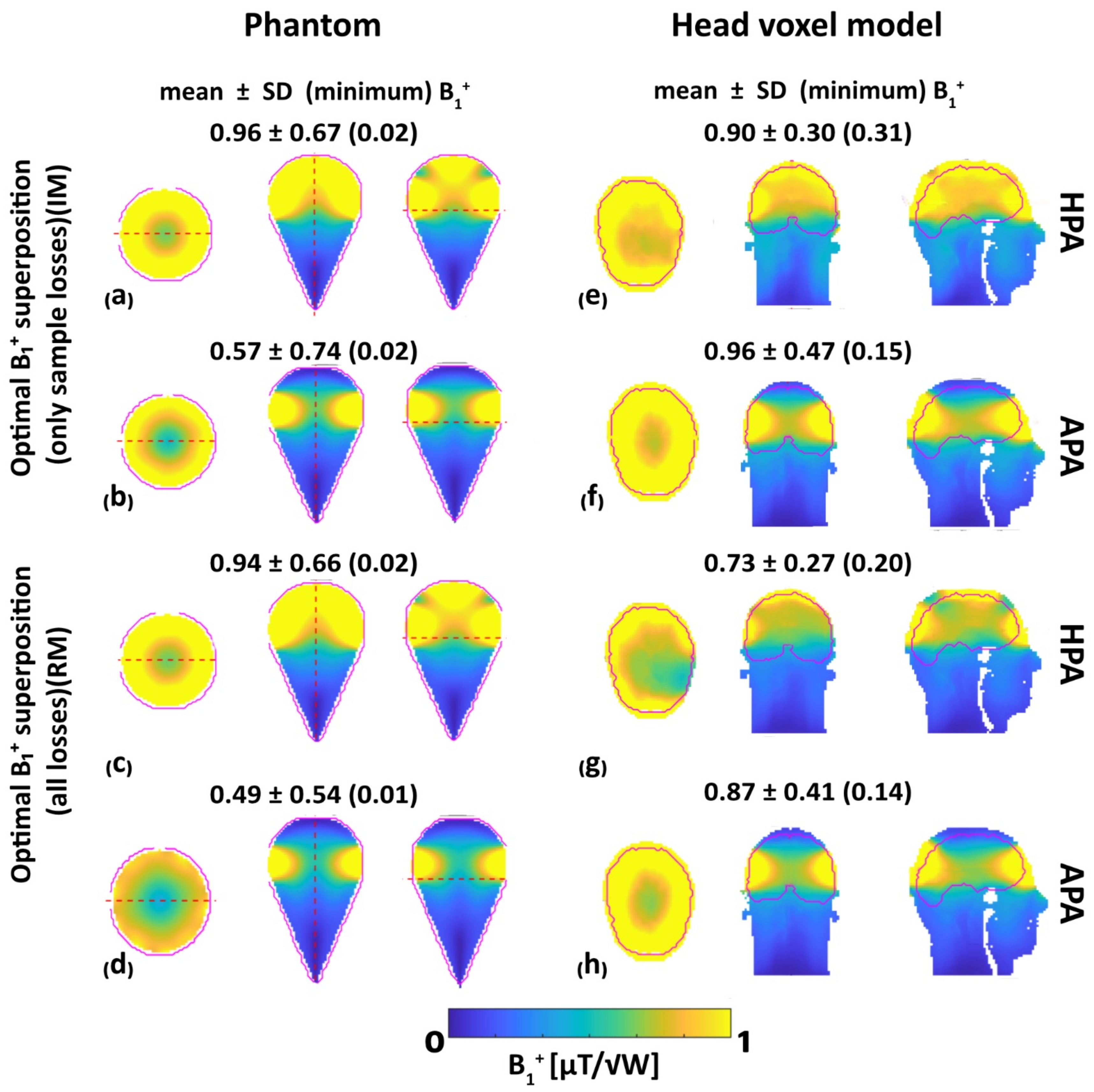
- B1+ superposition:
- Field shaping for static parallel transmission (pTX):
- Minimum B1+ optimization:
- Coefficient of variation optimization:
- Mean B1+ optimization
- SAR optimization with MRI considerations:
2.5.2. Quality of Targeted RF Heating
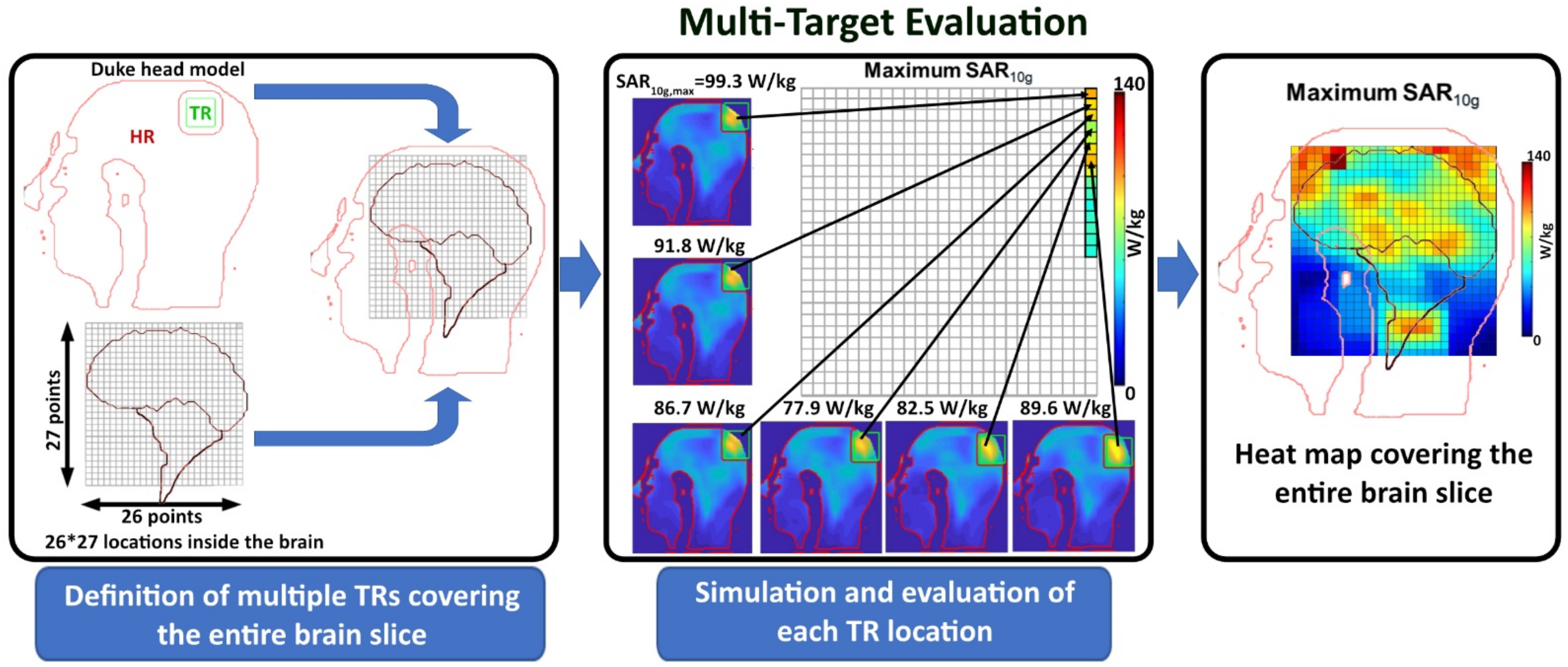
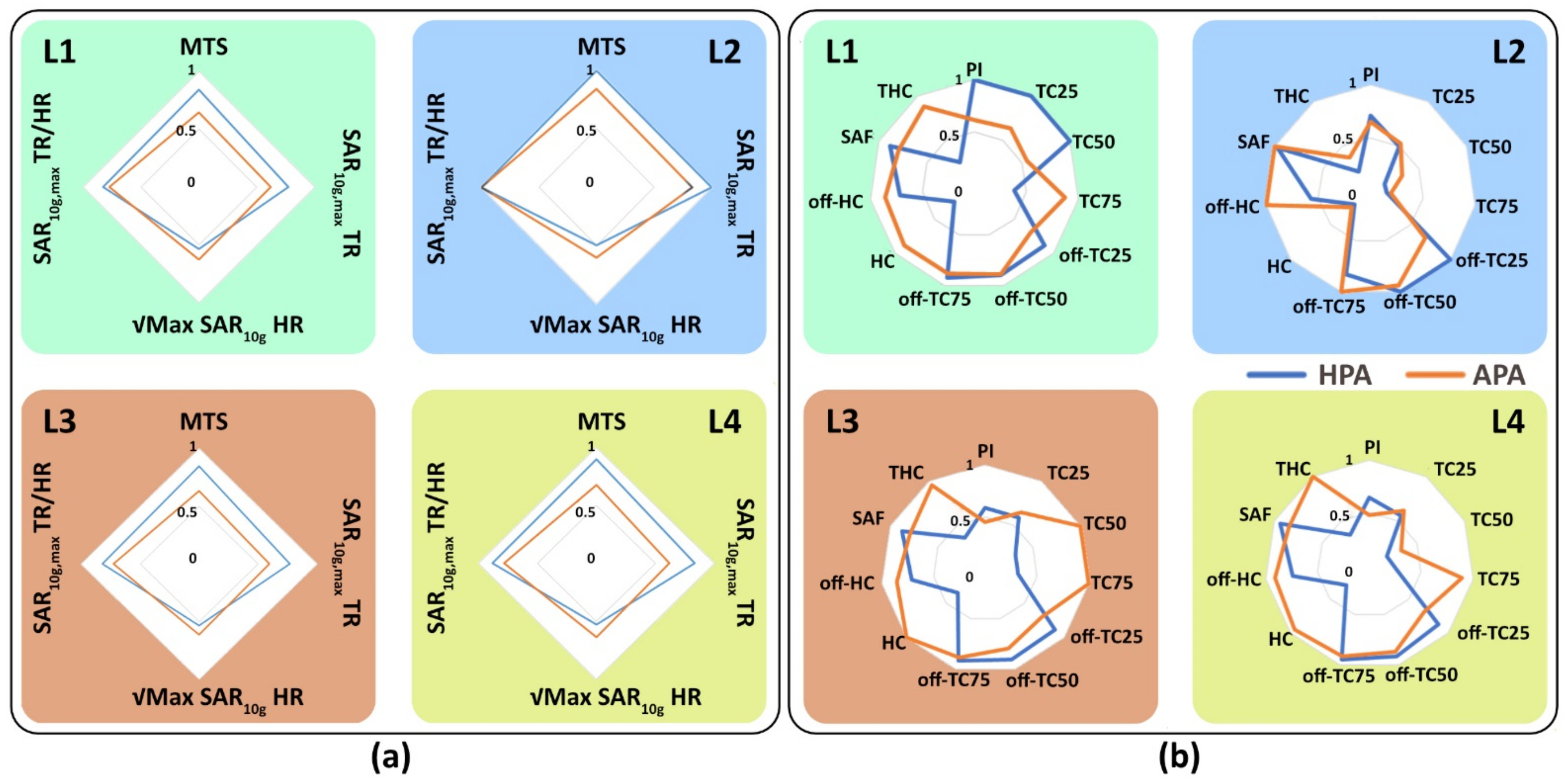
2.5.3. Multi-Target Evaluation
2.6. Data Analysis and Statistics
3. Results
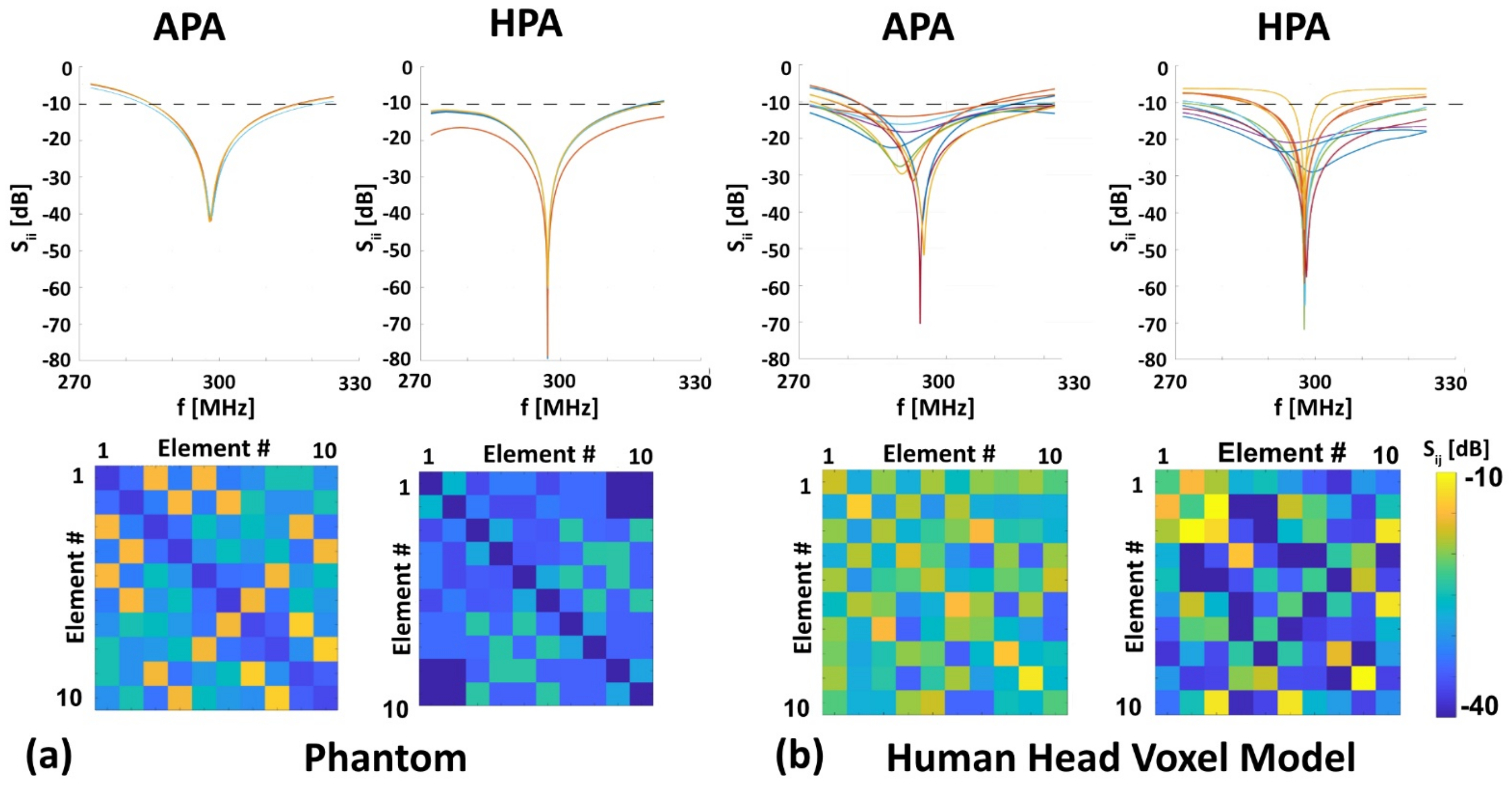
3.1. RF Characteristics of the RF Applicators
3.2. MRI: B1+ Efficiency, B1+ Uniformity and RF Power Deposition
3.3. RF Heating
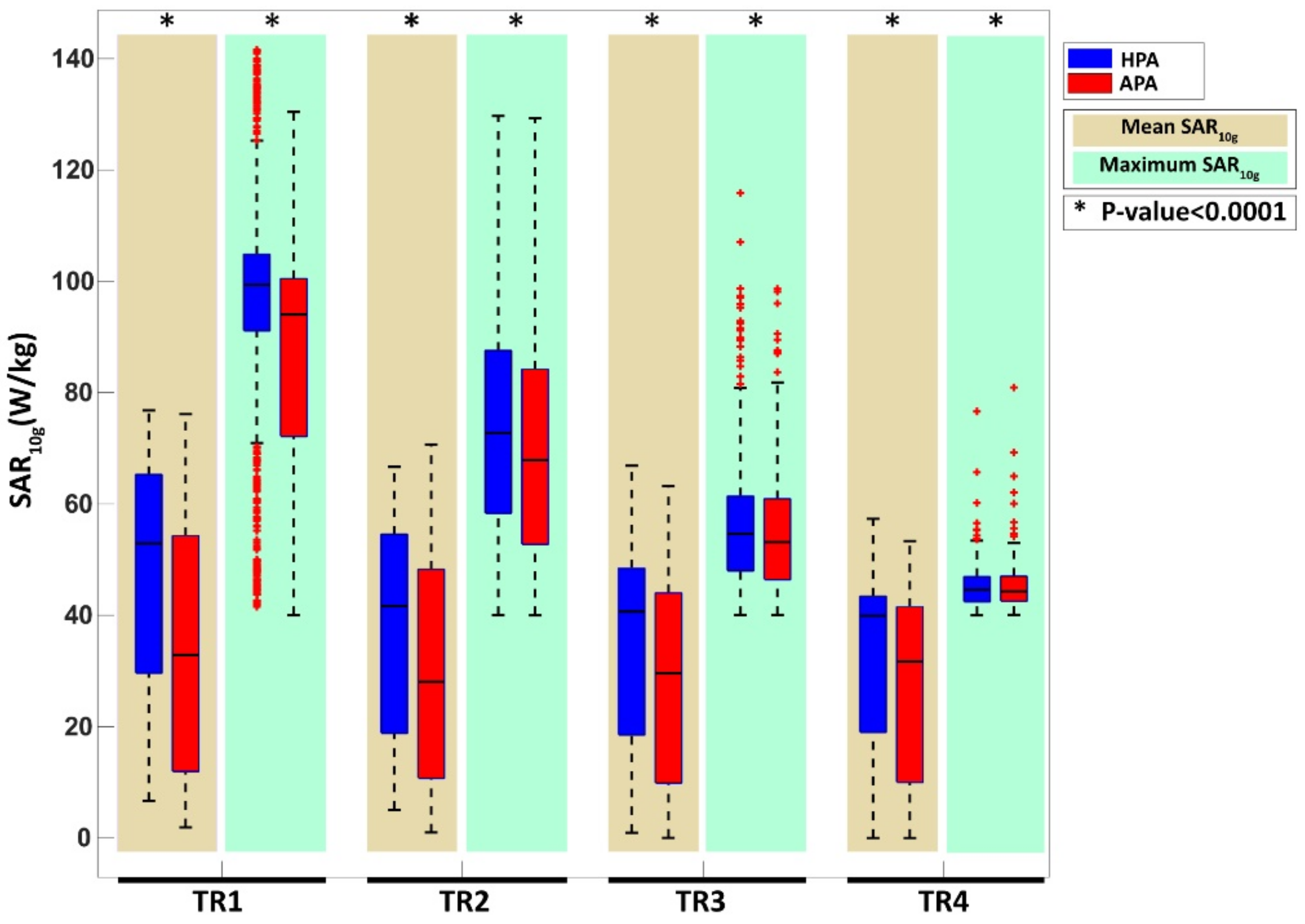
3.3.1. SAR-Based Indicators in Four Target Locations
3.3.2. Multi-Target Evaluation
3.3.3. Temperature Simulations
4. Discussion
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Lee Titsworth, W.; Murad, G.J.; Hoh, B.L.; Rahman, M. Fighting fire with fire: The revival of thermotherapy for gliomas. Anticancer Res. 2014, 34, 565–574. [Google Scholar] [PubMed]
- Wust, P.; Hildebrandt, B.; Sreenivasa, G.; Rau, B.; Gellermann, J.; Riess, H.; Felix, R.; Schlag, P.M. Hyperthermia in combined treatment of cancer. Lancet Oncol. 2002, 3, 487–497. [Google Scholar] [CrossRef] [PubMed]
- Rock, K.; McArdle, O.; Forde, P.; Dunne, M.; Fitzpatrick, D.; O’Neill, B.; Faul, C. A clinical review of treatment outcomes in glioblastoma multiforme--the validation in a non-trial population of the results of a randomised Phase III clinical trial: Has a more radical approach improved survival? Br. J. Radiol. 2012, 85, e729–e733. [Google Scholar] [CrossRef]
- Hanif, F.; Muzaffar, K.; Perveen, K.; Malhi, S.M.; Simjee Sh, U. Glioblastoma Multiforme: A Review of its Epidemiology and Pathogenesis through Clinical Presentation and Treatment. Asian Pac. J. Cancer Prev. 2017, 18, 3–9. [Google Scholar] [CrossRef] [PubMed]
- Dai, Q.; Cao, B.; Zhao, S.; Zhang, A. Synergetic Thermal Therapy for Cancer: State-of-the-Art and the Future. Bioengineering 2022, 9, 474. [Google Scholar] [CrossRef] [PubMed]
- Kok, H.P.; Cressman, E.N.K.; Ceelen, W.; Brace, C.L.; Ivkov, R.; Grull, H.; Ter Haar, G.; Wust, P.; Crezee, J. Heating technology for malignant tumors: A review. Int. J. Hyperth. 2020, 37, 711–741. [Google Scholar] [CrossRef]
- Seebass, M.; Beck, R.; Gellermann, J.; Nadobny, J.; Wust, P. Electromagnetic phased arrays for regional hyperthermia: Optimal frequency and antenna arrangement. Int. J. Hyperth. Off. J. Eur. Soc. Hyperthermic Oncol. N. Am. Hyperth. Group. 2001, 17, 321–336. [Google Scholar] [CrossRef] [PubMed]
- Wust, P.; Veltsista, P.D.; Oberacker, E.; Yavvari, P.; Walther, W.; Bengtsson, O.; Sterner-Kock, A.; Weinhart, M.; Heyd, F.; Grabowski, P.; et al. Radiofrequency Electromagnetic Fields Cause Non-Temperature-Induced Physical and Biological Effects in Cancer Cells. Cancers 2022, 14, 5349. [Google Scholar] [CrossRef] [PubMed]
- Han, H.; Eigentler, T.W.; Wang, S.; Kretov, E.; Winter, L.; Hoffmann, W.; Grass, E.; Niendorf, T. Design, Implementation, Evaluation and Application of a 32-Channel Radio Frequency Signal Generator for Thermal Magnetic Resonance Based Anti-Cancer Treatment. Cancers 2020, 12, 1720. [Google Scholar] [CrossRef]
- Oberacker, E.; Kuehne, A.; Oezerdem, C.; Nadobny, J.; Weihrauch, M.; Beck, M.; Zschaeck, S.; Diesch, C.; Eigentler, T.W.; Waiczies, H.; et al. Radiofrequency applicator concepts for thermal magnetic resonance of brain tumors at 297 MHz (7.0 Tesla). Int. J. Hyperth. 2020, 37, 549–563. [Google Scholar] [CrossRef]
- Paulides, M.M.; Curto, S.; Wu, M.; Winter, L.; Rhoon, G.C.v.; Yeo, D.T.B. Advances in magnetic resonance guided radiofrequency hyperthermia. In Proceedings of the 2017 11th European Conference on Antennas and Propagation (EUCAP), Paris, France, 19–24 March 2017; pp. 3692–3696. [Google Scholar]
- Ji, Y.; Winter, L.; Navarro, L.; Ku, M.C.; Periquito, J.S.; Pham, M.; Hoffmann, W.; Theune, L.E.; Calderon, M.; Niendorf, T. Controlled Release of Therapeutics from Thermoresponsive Nanogels: A Thermal Magnetic Resonance Feasibility Study. Cancers 2020, 12, 1380. [Google Scholar] [CrossRef] [PubMed]
- Feddersen, T.V.; Hernandez-Tamames, J.A.; Franckena, M.; van Rhoon, G.C.; Paulides, M.M. Clinical Performance and Future Potential of Magnetic Resonance Thermometry in Hyperthermia. Cancers 2021, 13, 31. [Google Scholar] [CrossRef]
- Curto, S.; Seabra, C.C.; Sumser, K.; Paulides, M.M.; Rhoon, G.C.v. MR thermometry in hyperthermia: Imaging for precise therapy monitoring with the novel Universal Arch applicator. In Proceedings of the 2022 International Workshop on Antenna Technology (iWAT), Dublin, Ireland, 16–18 May 2022; pp. 210–212. [Google Scholar]
- Paulides, M.M.; Bakker, J.F.; Neufeld, E.; van der Zee, J.; Jansen, P.P.; Levendag, P.C.; van Rhoon, G.C. Winner of the “New Investigator Award” at the European Society of Hyperthermia Oncology Meeting 2007. The HYPERcollar: A novel applicator for hyperthermia in the head and neck. Int. J. Hyperth. 2007, 23, 567–576. [Google Scholar] [CrossRef] [PubMed]
- Togni, P.; Rijnen, Z.; Numan, W.C.; Verhaart, R.F.; Bakker, J.F.; van Rhoon, G.C.; Paulides, M.M. Electromagnetic redesign of the HYPERcollar applicator: Toward improved deep local head-and-neck hyperthermia. Phys. Med. Biol. 2013, 58, 5997–6009. [Google Scholar] [CrossRef]
- Winter, L.; Ozerdem, C.; Hoffmann, W.; Santoro, D.; Muller, A.; Waiczies, H.; Seemann, R.; Graessl, A.; Wust, P.; Niendorf, T. Design and evaluation of a hybrid radiofrequency applicator for magnetic resonance imaging and RF induced hyperthermia: Electromagnetic field simulations up to 14.0 Tesla and proof-of-concept at 7.0 Tesla. PLoS ONE 2013, 8, e61661. [Google Scholar] [CrossRef]
- Curry, T.S.; Dowdey, J.E.; Murry, R.C. Christensen’s Physics of Diagnostic Radiology; Lippincott Williams & Wilkins: Philadelphia, PA, USA, 1990. [Google Scholar]
- Guerin, B.; Villena, J.F.; Polimeridis, A.G.; Adalsteinsson, E.; Daniel, L.; White, J.K.; Rosen, B.R.; Wald, L.L. Computation of ultimate SAR amplification factors for radiofrequency hyperthermia in non-uniform body models: Impact of frequency and tumour location. Int. J. Hyperth. 2018, 34, 87–100. [Google Scholar] [CrossRef] [PubMed]
- Winter, L.; Oezerdem, C.; Hoffmann, W.; van de Lindt, T.; Periquito, J.; Ji, Y.; Ghadjar, P.; Budach, V.; Wust, P.; Niendorf, T. Thermal magnetic resonance: Physics considerations and electromagnetic field simulations up to 23.5 Tesla (1GHz). Radiat. Oncol. 2015, 10, 201. [Google Scholar] [CrossRef]
- Oberacker, E.; Kuehne, A.; Nadobny, J.; Zschaeck, S.; Weihrauch, M.; Waiczies, H.; Ghadjar, P.; Wust, P.; Niendorf, T.; Winter, L. Radiofrequency applicator concepts for simultaneous MR imaging and hyperthermia treatment of glioblastoma multiforme. Curr. Dir. Biomed. Eng. 2017, 3, 473–477. [Google Scholar] [CrossRef]
- Lattanzi, R.; Wiggins, G.C.; Zhang, B.; Duan, Q.; Brown, R.; Sodickson, D.K. Approaching ultimate intrinsic signal-to-noise ratio with loop and dipole antennas. Magn. Reson. Med. 2018, 79, 1789–1803. [Google Scholar] [CrossRef]
- Raaijmakers, E.A.L.; Mestrom, R.M.C.; Sumser, K.; Salim, G.; van Rhoon, G.C.; Essers, J.; Paulides, M.M. An MR-compatible antenna and application in a murine superficial hyperthermia applicator. Int. J. Hyperth. 2018, 34, 697–703. [Google Scholar] [CrossRef]
- Eigentler, T.W.; Winter, L.; Han, H.; Oberacker, E.; Kuehne, A.; Waiczies, H.; Schmitter, S.; Boehmert, L.; Prinz, C.; Trefna, H.D.; et al. Wideband Self-Grounded Bow-Tie Antenna for Thermal MR. NMR Biomed. 2020, 33, e4274. [Google Scholar] [CrossRef] [PubMed]
- Zanoli, M.; Ek, E.; Dobsicek Trefna, H. Antenna Arrangement in UWB Helmet Brain Applicators for Deep Microwave Hyperthermia. Cancers 2023, 15, 1447. [Google Scholar] [CrossRef] [PubMed]
- Aram, M.G.; Aliakbarian, H.; Trefná, H.D. A phased array applicator based on open ridged-waveguide antenna for microwave hyperthermia. Microw. Opt. Technol. Lett. 2021, 63, 3086–3091. [Google Scholar] [CrossRef]
- Guerin, B.; Villena, J.F.; Polimeridis, A.G.; Adalsteinsson, A.; Daniel, L.; White, J.K.; Rosen, B.R.; Wald, L.L. Ultimate hyperthermia: Computation of the best achievable radio-frequency hyperthermia treatments in non-uniform body models. In Proceedings of the 24th Annual Meeting of the ISMRM, Singapore, 7–13 May 2016. [Google Scholar]
- Oberacker, E.; Diesch, C.; Nadobny, J.; Kuehne, A.; Wust, P.; Ghadjar, P.; Niendorf, T. Patient-Specific Planning for Thermal Magnetic Resonance of Glioblastoma Multiforme. Cancers 2021, 13, 1867. [Google Scholar] [CrossRef] [PubMed]
- Lakshmanan, K.; Cloos, M.; Brown, R.; Lattanzi, R.; Sodickson, D.K.; Wiggins, G.C. The “Loopole” Antenna: A Hybrid Coil Combining Loop and Electric Dipole Properties for Ultra-High-Field MRI. Concepts Magn. Reson. Part. B Magn. Reson. Eng. 2020, 2020, 8886543. [Google Scholar] [CrossRef] [PubMed]
- Guerin, B.; Angelone, L.M.; Dougherty, D.; Wald, L.L. Parallel transmission to reduce absorbed power around deep brain stimulation devices in MRI: Impact of number and arrangement of transmit channels. Magn. Reson. Med. 2020, 83, 299–311. [Google Scholar] [CrossRef] [PubMed]
- Avdievich, N.I.; Nikulin, A.V.; Ruhm, L.; Magill, A.W.; Glang, F.; Henning, A.; Scheffler, K. A 32-element loop/dipole hybrid array for human head imaging at 7 T. Magn. Reson. Med. 2022, 88, 1912–1926. [Google Scholar] [CrossRef] [PubMed]
- Erturk, M.A.; Raaijmakers, A.J.; Adriany, G.; Ugurbil, K.; Metzger, G.J. A 16-channel combined loop-dipole transceiver array for 7 Tesla body MRI. Magn. Reson. Med. 2017, 77, 884–894. [Google Scholar] [CrossRef] [PubMed]
- Woo, M.K.; DelaBarre, L.; Lee, B.Y.; Waks, M.; Lagore, R.L.; Radder, J.; Eryaman, Y.; Ugurbil, K.; Adriany, G. Evaluation of a 16-channel transceiver loop + dipole antenna array for human head imaging at 10.5 tesla. IEEE Access 2020, 8, 203555–203563. [Google Scholar] [CrossRef]
- Pfrommer, A.; Henning, A. The ultimate intrinsic signal-to-noise ratio of loop- and dipole-like current patterns in a realistic human head model. Magn. Reson. Med. 2018, 80, 2122–2138. [Google Scholar] [CrossRef]
- Dobsicek Trefna, H. EP-1271: Development of focused microwave hyperthermia of pediatric brain cance. Radiother. Oncol. 2015, 115, S685–S686. [Google Scholar] [CrossRef][Green Version]
- Lou, F.; Tang, X.; Quan, Z.; Qian, M.; Wang, J.; Qu, S.; Gao, Y.; Wang, Y.; Pan, G.; Lai, H.Y.; et al. A 16-channel loop array for in vivo macaque whole-brain imaging at 7 T. Magn. Reson. Imaging 2023, 102, 179–183. [Google Scholar] [CrossRef] [PubMed]
- Shajan, G.; Kozlov, M.; Hoffmann, J.; Turner, R.; Scheffler, K.; Pohmann, R. A 16-channel dual-row transmit array in combination with a 31-element receive array for human brain imaging at 9.4 T. Magn. Reson. Med. 2014, 71, 870–879. [Google Scholar] [CrossRef] [PubMed]
- Eigentler, T.W.; Kuehne, A.; Boehmert, L.; Dietrich, S.; Els, A.; Waiczies, H.; Niendorf, T. 32-Channel self-grounded bow-tie transceiver array for cardiac MR at 7.0T. Magn. Reson. Med. Off. J. Soc. Magn. Reson. Med./Soc. Magn. Reson. Med. 2021, 86, 2862–2879. [Google Scholar] [CrossRef] [PubMed]
- Nurzed, B.; Kuehne, A.; Aigner, C.S.; Schmitter, S.; Niendorf, T.; Eigentler, T.W. Radiofrequency Antenna Concepts for Human Cardiac MR at 14.0 T. MAGMA 2023, 36, 257–277. [Google Scholar] [CrossRef] [PubMed]
- Vaidya, M.V.; Collins, C.M.; Sodickson, D.K.; Brown, R.; Wiggins, G.C.; Lattanzi, R. Dependence of B1+ and B1- Field Patterns of Surface Coils on the Electrical Properties of the Sample and the MR Operating Frequency. Concepts Magn. Reson. Part B Magn. Reson. Eng. 2016, 46, 25–40. [Google Scholar] [CrossRef] [PubMed]
- Abragam, A. Principles of Nuclear Magnetism (International Series of Monographs on Physics); Oxford University Press: Oxford, UK, 1983. [Google Scholar]
- Lattanzi, R.; Sodickson, D.K.; Grant, A.K.; Zhu, Y. Electrodynamic constraints on homogeneity and radiofrequency power deposition in multiple coil excitations. Magn. Reson. Med. 2009, 61, 315–334. [Google Scholar] [CrossRef] [PubMed]
- Schooneveldt, G.; Bakker, A.; Balidemaj, E.; Chopra, R.; Crezee, J.; Geijsen, E.D.; Hartmann, J.; Hulshof, M.C.; Kok, H.P.; Paulides, M.M.; et al. Thermal dosimetry for bladder hyperthermia treatment. An overview. Int. J. Hyperth. 2016, 32, 417–433. [Google Scholar] [CrossRef] [PubMed]
- Kuehne, A.; Oberacker, E.; Waiczies, H.; Niendorf, T. Solving the time-and frequency-multiplexed problem of constrained radiofrequency induced hyperthermia. Cancers 2020, 12, 1072. [Google Scholar] [CrossRef]
- Takook, P.; Persson, M.; Gellermann, J.; Trefna, H.D. Compact self-grounded Bow-Tie antenna design for an UWB phased-array hyperthermia applicator. Int. J. Hyperth. 2017, 33, 387–400. [Google Scholar] [CrossRef]
- Griffiths, D.J. Introduction to Electrodynamics; Cambridge University Press: Cambridge, UK, 2023. [Google Scholar]
- Pozar, D.M. Microwave Engineering; John Wiley & Sons: Hoboken, NJ, USA, 2011. [Google Scholar]
- Kok, H.P.; Ciampa, S.; de Kroon-Oldenhof, R.; Steggerda-Carvalho, E.J.; van Stam, G.; Vörding, P.J.Z.V.S.; Stalpers, L.J.; Geijsen, E.D.; Bardati, F.; Bel, A. Toward online adaptive hyperthermia treatment planning: Correlation between measured and simulated specific absorption rate changes caused by phase steering in patients. Int. J. Radiat. Oncol. Biol. Phys. 2014, 90, 438–445. [Google Scholar] [CrossRef]
- Eryaman, Y.; Guerin, B.; Keil, B.; Mareyam, A.; Herraiz, J.L.; Kosior, R.K.; Martin, A.; Torrado-Carvajal, A.; Malpica, N.; Hernandez-Tamames, J.A.; et al. SAR reduction in 7T C-spine imaging using a “dark modes” transmit array strategy. Magn. Reson. Med. 2015, 73, 1533–1539. [Google Scholar] [CrossRef] [PubMed]
- Balanis, C. Antenna Theory: Analysis and Design; Wiley-Interscience: New York, NY, USA, 2005. [Google Scholar]
- Dorsey, W.; Stumme, A.; Coleman, J. Naval Research Lab. Tutorial: Applying Phase-Mode Theory to the Design of Cylindrical Arrays; Tech. Rep. NRL/MR/5310–19-9915; Naval Research Laboratory: Washington, DC, USA, 2019; p. 12. [Google Scholar]
- Winter, L.; Oberacker, E.; Paul, K.; Ji, Y.; Oezerdem, C.; Ghadjar, P.; Thieme, A.; Budach, V.; Wust, P.; Niendorf, T. Magnetic resonance thermometry: Methodology, pitfalls and practical solutions. Int. J. Hyperth. 2016, 32, 63–75. [Google Scholar] [CrossRef] [PubMed]
- Restivo, M.C.; van den Berg, C.A.; van Lier, A.L.; Polders, D.L.; Raaijmakers, A.J.; Luijten, P.R.; Hoogduin, H. Local specific absorption rate in brain tumors at 7 tesla. Magn. Reson. Med. 2016, 75, 381–389. [Google Scholar] [CrossRef] [PubMed]
- Paulides, M.M.; Rodrigues, D.B.; Bellizzi, G.G.; Sumser, K.; Curto, S.; Neufeld, E.; Montanaro, H.; Kok, H.P.; Dobsicek Trefna, H. ESHO benchmarks for computational modeling and optimization in hyperthermia therapy. Int. J. Hyperth. 2021, 38, 1425–1442. [Google Scholar] [CrossRef] [PubMed]
- Wust, P.; Stahl, H.; Loffel, J.; Seebass, M.; Riess, H.; Felix, R. Clinical, physiological and anatomical determinants for radiofrequency hyperthermia. Int. J. Hyperth. 1995, 11, 151–167. [Google Scholar] [CrossRef]
- Pennes, H.H. Analysis of tissue and arterial blood temperatures in the resting human forearm. J. Appl. Physiol. 1948, 1, 93–122. [Google Scholar] [CrossRef] [PubMed]
- Shih, T.C.; Yuan, P.; Lin, W.L.; Kou, H.S. Analytical analysis of the Pennes bioheat transfer equation with sinusoidal heat flux condition on skin surface. Med. Eng. Phys. 2007, 29, 946–953. [Google Scholar] [CrossRef] [PubMed]
- Tilly, W.; Wust, P.; Rau, B.; Harder, C.; Gellermann, J.; Schlag, P.; Budach, V.; Felix, R. Temperature data and specific absorption rates in pelvic tumours: Predictive factors and correlations. Int. J. Hyperth. 2001, 17, 172–188. [Google Scholar] [CrossRef]
- Neufeld, E.; Paulides, M.M.; van Rhoon, G.C.; Kuster, N. Numerical modeling for simulation and treatment planning of thermal therapy. Phys. Therm. Ther. Fundam. Clin. Appl. 2013, 8, 119–136. [Google Scholar]
- Chipperfield, A.; Fleming, P.; Fonseca, C. Genetic algorithm tools for control systems engineering. In Adaptive Computing in Engineering Design and Control; Springer: Berlin/Heidelberg, Germany, 1994; p. 133. [Google Scholar]
- Coleman, T.; Branch, M.A.; Grace, A. Optimization Toolbox. For Use with MATLAB. User’s Guide for MATLAB 5; Version 2, Relaese II 1999; The “MathWorks” Inc.: Natick, MA, USA, 1995. [Google Scholar]
- Mao, W.; Smith, M.B.; Collins, C.M. Exploring the limits of RF shimming for high-field MRI of the human head. Magn. Reson. Med. 2006, 56, 918–922. [Google Scholar] [CrossRef] [PubMed]
- Rahimi, F.; Chamaani, S. Applying a repetitive time-reversal method to reduce input power of a wearable hyperthermia applicator for breast cancer treatment. Microw. Opt. Technol. Lett. 2020, 62, 3754–3766. [Google Scholar] [CrossRef]
- Zastrow, E.; Hagness, S.C.; Van Veen, B.D.; Medow, J.E. Time-multiplexed beamforming for noninvasive microwave hyperthermia treatment. IEEE Trans. Biomed. Eng. 2011, 58, 1574–1584. [Google Scholar] [CrossRef] [PubMed]
- Georgakis, I.P.; Polimeridis, A.G.; Lattanzi, R. A formalism to investigate the optimal transmit efficiency in radiofrequency shimming. NMR Biomed. 2020, 33, e4383. [Google Scholar] [CrossRef] [PubMed]
- Lattanzi, R.; Grant, A.K.; Polimeni, J.R.; Ohliger, M.A.; Wiggins, G.C.; Wald, L.L.; Sodickson, D.K. Performance evaluation of a 32-element head array with respect to the ultimate intrinsic SNR. NMR Biomed. 2010, 23, 142–151. [Google Scholar] [CrossRef] [PubMed]
- Padormo, F.; Beqiri, A.; Hajnal, J.V.; Malik, S.J. Parallel transmission for ultrahigh-field imaging. NMR Biomed. 2016, 29, 1145–1161. [Google Scholar] [CrossRef] [PubMed]
- What’s new in MR safety: The latest on the safe use of equipment in the magnetic resonance environment. Health Devices 2005, 34, 333–349.
- Wu, X.; Chang, T.-H.; Luo, Z.-Q.; Akgun, C.E.; Vaughan, J.T.; Uğurbil, K.; Moortele, P.-F.v.d. Worst case SAR scenario as a new metric for SAR analysis in B 1 phase. In Proceedings of the Proceedings 16th Scientific Meeting, International Society for Magnetic Resonance in Medicine, Toronto, ON, Canada, 3–9 May 2008. [Google Scholar]
- Canters, R.A.; Wust, P.; Bakker, J.F.; Van Rhoon, G.C. A literature survey on indicators for characterisation and optimisation of SAR distributions in deep hyperthermia, a plea for standardisation. Int. J. Hyperth. 2009, 25, 593–608. [Google Scholar] [CrossRef] [PubMed]
- Bellizzi, G.G.; Drizdal, T.; van Rhoon, G.C.; Crocco, L.; Isernia, T.; Paulides, M.M. The potential of constrained SAR focusing for hyperthermia treatment planning: Analysis for the head & neck region. Phys. Med. Biol. 2018, 64, 015013. [Google Scholar] [CrossRef]
- Lee, H.K.; Antell, A.G.; Perez, C.A.; Straube, W.L.; Ramachandran, G.; Myerson, R.J.; Emami, B.; Molmenti, E.P.; Buckner, A.; Lockett, M.A. Superficial hyperthermia and irradiation for recurrent breast carcinoma of the chest wall: Prognostic factors in 196 tumors. Int. J. Radiat. Oncol. Biol. Phys. 1998, 40, 365–375. [Google Scholar] [CrossRef]
- Bellizzi, G.G.; Drizdal, T.; van Rhoon, G.C.; Crocco, L.; Isernia, T.; Paulides, M.M. Predictive value of SAR based quality indicators for head and neck hyperthermia treatment quality. Int. J. Hyperth. 2019, 36, 456–465. [Google Scholar] [CrossRef] [PubMed]
- Singh, M.; Singh, T.; Soni, S. Pre-operative Assessment of Ablation Margins for Variable Blood Perfusion Metrics in a Magnetic Resonance Imaging Based Complex Breast Tumour Anatomy: Simulation Paradigms in Thermal Therapies. Comput. Methods Programs Biomed. 2021, 198, 105781. [Google Scholar] [CrossRef] [PubMed]
- Brunner, D.O.; Paska, J.; Froehlich, J.; Pruessmann, K.P. Traveling-wave RF shimming and parallel MRI. Magn. Reson. Med. 2011, 66, 290–300. [Google Scholar] [CrossRef] [PubMed]
- Manpreet, S. Modified Pennes bioheat equation with heterogeneous blood perfusion: A newer perspective. Int. J. Heat. Mass. Transf. 2024, 218, 124698. [Google Scholar] [CrossRef]
- Webb, A.; Shchelokova, A.; Slobozhanyuk, A.; Zivkovic, I.; Schmidt, R. Novel materials in magnetic resonance imaging: High permittivity ceramics, metamaterials, metasurfaces and artificial dielectrics. MAGMA 2022, 35, 875–894. [Google Scholar] [CrossRef] [PubMed]
- Wang, G.; Gong, Y. Metamaterial lens applicator for microwave hyperthermia of breast cancer. Int. J. Hyperth. 2009, 25, 434–445. [Google Scholar] [CrossRef]
- Schmidt, R.; Webb, A. Metamaterial Combining Electric- and Magnetic-Dipole-Based Configurations for Unique Dual-Band Signal Enhancement in Ultrahigh-Field Magnetic Resonance Imaging. ACS Appl. Mater. Interfaces 2017, 9, 34618–34624. [Google Scholar] [CrossRef]



| Indicator | Location | Description | Unit | Valid Value | Equation |
|---|---|---|---|---|---|
| SAR10g | Whole Head | SAR10g averaging over cubes covering 10 g of tissue (SAR10g) distributions inside the human head voxel model Duke ; σ (S/m) is the electrical conductivity, ρ (kg/cm3) is the mass density, and |E| (V/m) is the magnitude of the local electric field vector | W/kg | <40 (HR) | (6) |
| <80 >40 (TR) | |||||
| TCxx (TC25/TC50/TC75) [70,71,72] | TR | Measures the percentage of volume with SAR10g over 25% (TC25), 50% (TC50) or 75% (TC75) of SAR10g,max found in the TR. This is only evaluated if SAR10g,max >40 W/kg. | % | >75 [54] | (7) |
| * Off-TCxx (off-TC25/off-TC50/off-TC75) | HR | iso-SAR contour in healthy tissue defined as off-target regions (off-TR). Measures the percentage of voxels in the HR with SAR10g over the (25%, 50%, and 70%) of the SAR10g,max found in HR (healthy constraint SAR10g (40 W/kg))). | % | (8) | |
| SAR10g Amplification Factor (SAF) [10,70,73] | Whole Head | Measures that healthy tissue preservation from mean SAR10g in HR | - | (9) | |
| Homogeneity Coefficient (HC) [70] | TR | Measures how homogenous the SAR10g,max is distributed over TR | - | ||
| - | (10) | ||||
| * Homogeneity Coefficient (off-HC) | HR | Measures how homogenous the SAR10g,max in healthy tissue is distributed over HV. | - | ||
| - | (11) | ||||
| * Total Homogeneity Coefficient (THC) | Whole Head | Measures total homogeneity of SAR10g inside the TR and HR to satisfy hyperthermia treatment goals | - | ||
| - | (12) | ||||
| Performance Indicator (PI) [72] | Whole Head | Measures the total performance of each hyperthermia treatment planning | - | ||
| W/kg | (13) | ||||
| Txx (T10/T50/T90) [54,72] | TR | In clinical practice, the assessment of tumor coverage should involve the examination of indexed temperatures, specifically T10, T50, and T90. These values correspond to the temperatures reached in at least 10%, 50%, and 90% of the target region, respectively. | °C | T50 > 40 | (14) |
| Optimization Goal | pTx Shimming Method | Min B1+ (µT/√kW) | Coefficient of Variation | Mean B1+ (µT/√kW) | |||
|---|---|---|---|---|---|---|---|
| Helmet Array | Annular Array | Helmet Array | Annular Array | Helmet Array | Annular Array | ||
| Max (min B1+) | PS | 2.12 | 2.04 | 0.98 | 1.41 | 8.82 | 9.89 |
| APS | 2.71 | 1.75 | 2.87 | 1.62 | 8.78 | 10.81 | |
| min (CoV) | PS | 0.06 | 0.48 | 0.96 | 0.97 | 8.10 | 11.01 |
| APS | 0.02 | 1.62 | 0.51 | 0.88 | 3.68 | 11.47 | |
| Max (Mean B1+) | PS | 0.047 | 0.087 | 0.95 | 1.12 | 10.6 | 13.61 |
| APS | 0.34 | 0.01 | 1.33 | 1.99 | 11.1 | 13.71 | |
| SAR10g,wc (W/kg) | |||
|---|---|---|---|
| pTx Shimming Method | RF Applicator | Phantom | Human Head Voxel Model |
| PS | HPA | 2.3 | 2.3 |
| APA | 2.4 | 2.7 | |
| APS | HPA | 11.7 | 8.4 |
| APA | 14.2 | 9.4 | |
| L1 | L2 | L3 | L4 | |||||
|---|---|---|---|---|---|---|---|---|
| Locations | Limbic Lobe and Postcentral Gyrus | Thalamus | Corpus Callosum and Limbic Lobe | Parietal Lobe of the Brain | ||||
| Metrics | ||||||||
| HPA | APA | HPA | APA | HPA | APA | HPA | APA | |
| MTS (W/kg) | 49.9 | 38.3 | 59.4 | 50.2 | 50.4 | 37.6 | 53.4 | 40.2 |
| Max SAR10g TR (W/kg) | 56.4 | 45.3 | 73.1 | 60.7 | 56.2 | 43.5 | 61.0 | 45.3 |
| Max SAR10g HR (W/kg) | 16.5 | 14.1 | 17.7 | 14.6 | 16.6 | 14.4 | 16.7 | 13.9 |
| SAF | 3.42 | 3.21 | 4.13 | 4.16 | 3.39 | 3.02 | 3.65 | 3.26 |
| Max SAR10g TR/HR (%) | 0.23 | 0.19 | 0.16 | 0.17 | 0.25 | 0.23 | 0.25 | 0.259 |
| TC25 (%) | 88.5 | 56.3 | 44.1 | 47.0 | 52.1 | 57.1 | 49.5 | 54.2 |
| TC50 (%) | 52.7 | 29.0 | 7.8 | 17.4 | 19.4 | 31.3 | 16.3 | 29.3 |
| TC75 (%) | 4.5 | 10.2 | 1.8 | 2.3 | 3.7 | 11.7 | 3.0 | 10.5 |
| HC | 0.05 | 0.18 | 0.04 | 0.05 | 0.071 | 0.21 | 0.060 | 0.19 |
| Off-TC25 (%) | 73.2 | 58.2 | 82.6 | 56.6 | 73.1 | 58.7 | 72.5 | 56.9 |
| Off-TC50 (%) | 34.9 | 34.6 | 38.9 | 36.5 | 35.2 | 35.3 | 35.7 | 33.8 |
| Off- TC75 (%) | 16.4 | 15.7 | 14.8 | 17.8 | 16.4 | 15.8 | 16.9 | 16.3 |
| Off-HC | 0.22 | 0.27 | 0.18 | 0.31 | 0.22 | 0.27 | 0.233 | 0.29 |
| THC | 0.01 | 0.05 | 0.01 | 0.02 | 0.01 | 0.06 | 0.01 | 0.06 |
| PI | 149.7 | 68.9 | 106.4 | 97 | 87.9 | 67.1 | 96.6 | 71.2 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rahimi, F.; Nurzed, B.; Eigentler, T.W.; Berangi, M.; Oberacker, E.; Kuehne, A.; Ghadjar, P.; Millward, J.M.; Schuhmann, R.; Niendorf, T. Helmet Radio Frequency Phased Array Applicators Enhance Thermal Magnetic Resonance of Brain Tumors. Bioengineering 2024, 11, 733. https://doi.org/10.3390/bioengineering11070733
Rahimi F, Nurzed B, Eigentler TW, Berangi M, Oberacker E, Kuehne A, Ghadjar P, Millward JM, Schuhmann R, Niendorf T. Helmet Radio Frequency Phased Array Applicators Enhance Thermal Magnetic Resonance of Brain Tumors. Bioengineering. 2024; 11(7):733. https://doi.org/10.3390/bioengineering11070733
Chicago/Turabian StyleRahimi, Faezeh, Bilguun Nurzed, Thomas W. Eigentler, Mostafa Berangi, Eva Oberacker, Andre Kuehne, Pirus Ghadjar, Jason M. Millward, Rolf Schuhmann, and Thoralf Niendorf. 2024. "Helmet Radio Frequency Phased Array Applicators Enhance Thermal Magnetic Resonance of Brain Tumors" Bioengineering 11, no. 7: 733. https://doi.org/10.3390/bioengineering11070733
APA StyleRahimi, F., Nurzed, B., Eigentler, T. W., Berangi, M., Oberacker, E., Kuehne, A., Ghadjar, P., Millward, J. M., Schuhmann, R., & Niendorf, T. (2024). Helmet Radio Frequency Phased Array Applicators Enhance Thermal Magnetic Resonance of Brain Tumors. Bioengineering, 11(7), 733. https://doi.org/10.3390/bioengineering11070733











