Temporal Analysis of Daily and 10 Minutes of Rainfall of Poprad Station in Eastern Slovakia
Abstract
1. Introduction
2. Materials and Methods
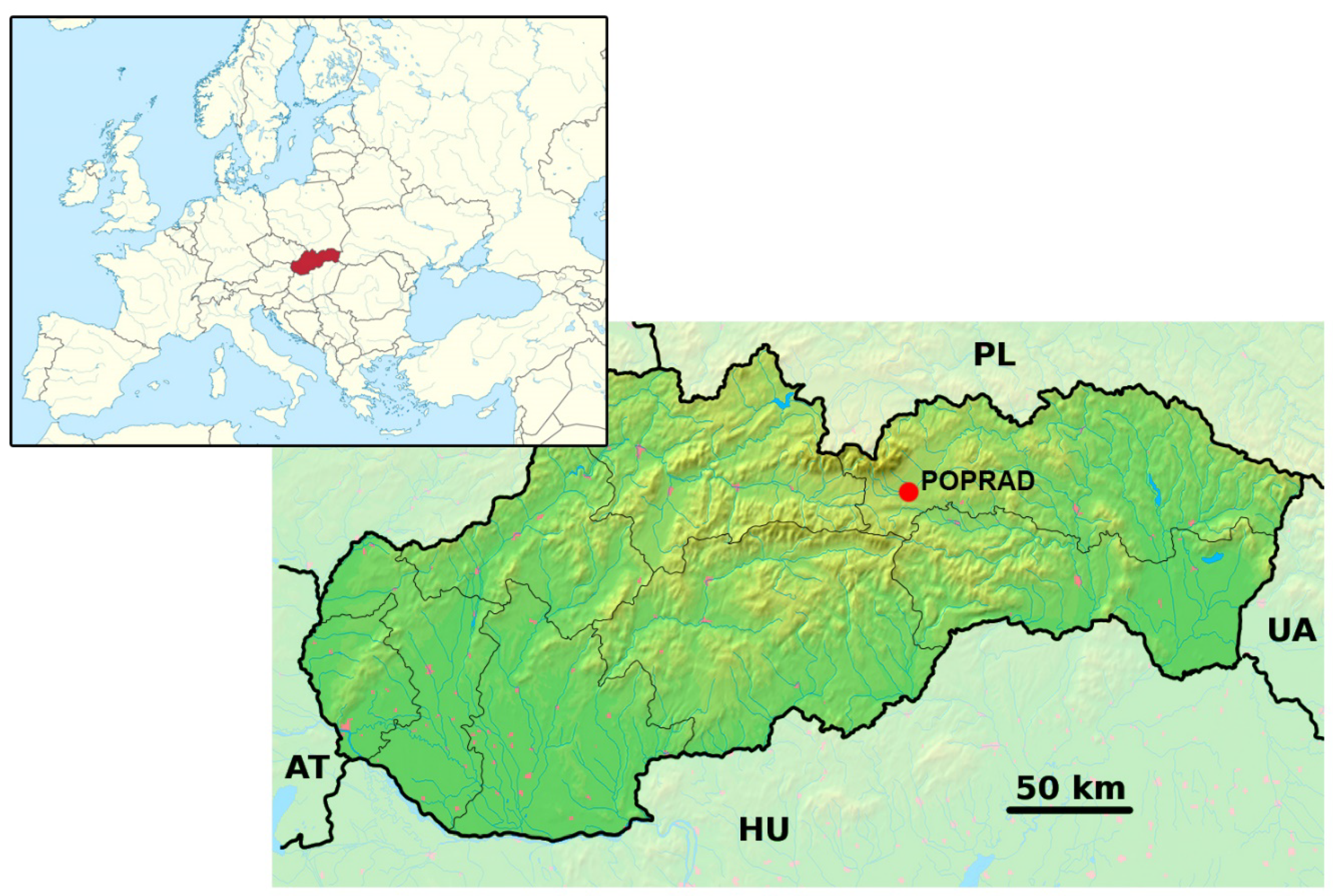
2.1. Study Area
2.2. Input Data
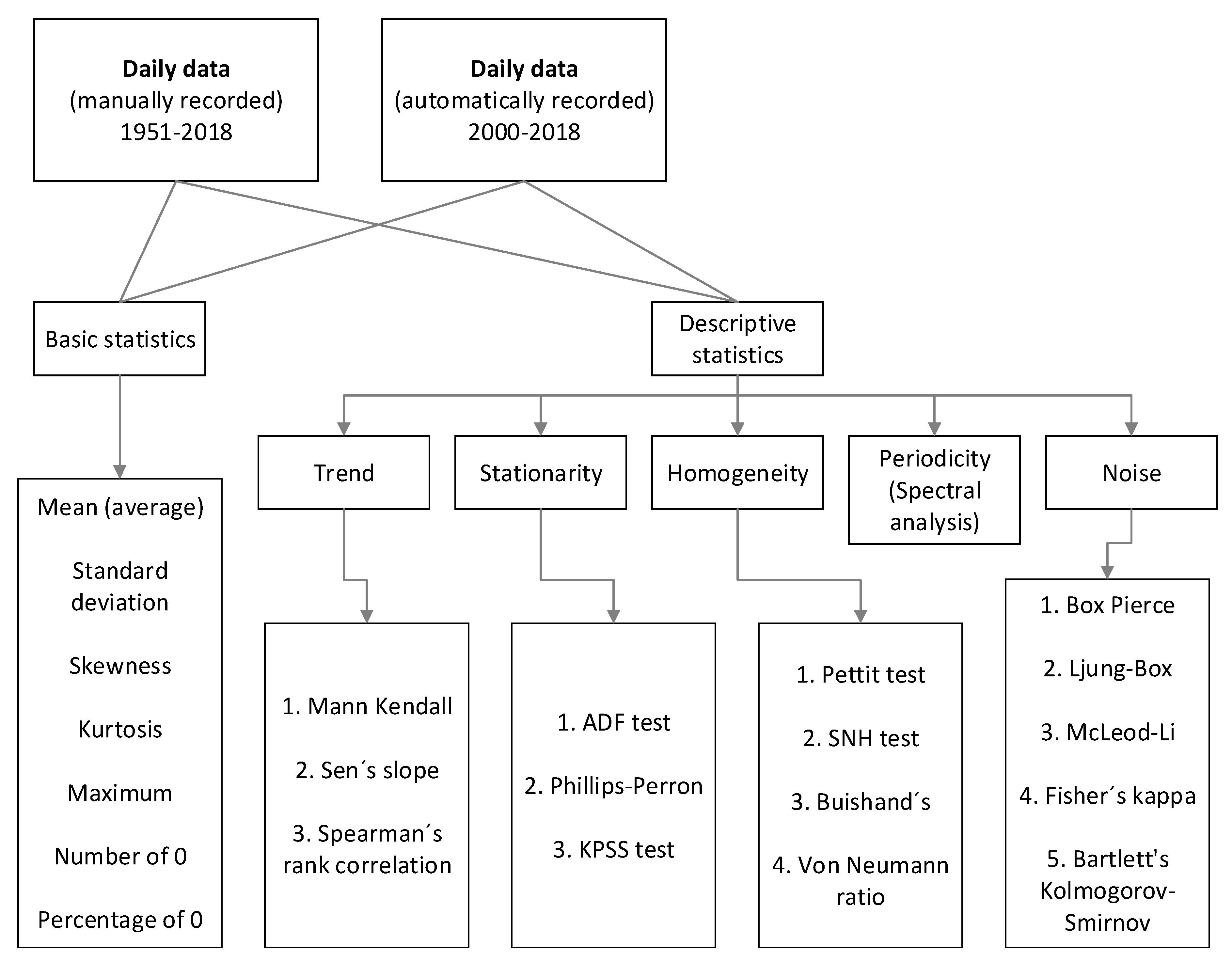
2.3. Descriptive and Statistical Analysis
3. Results and Discussion
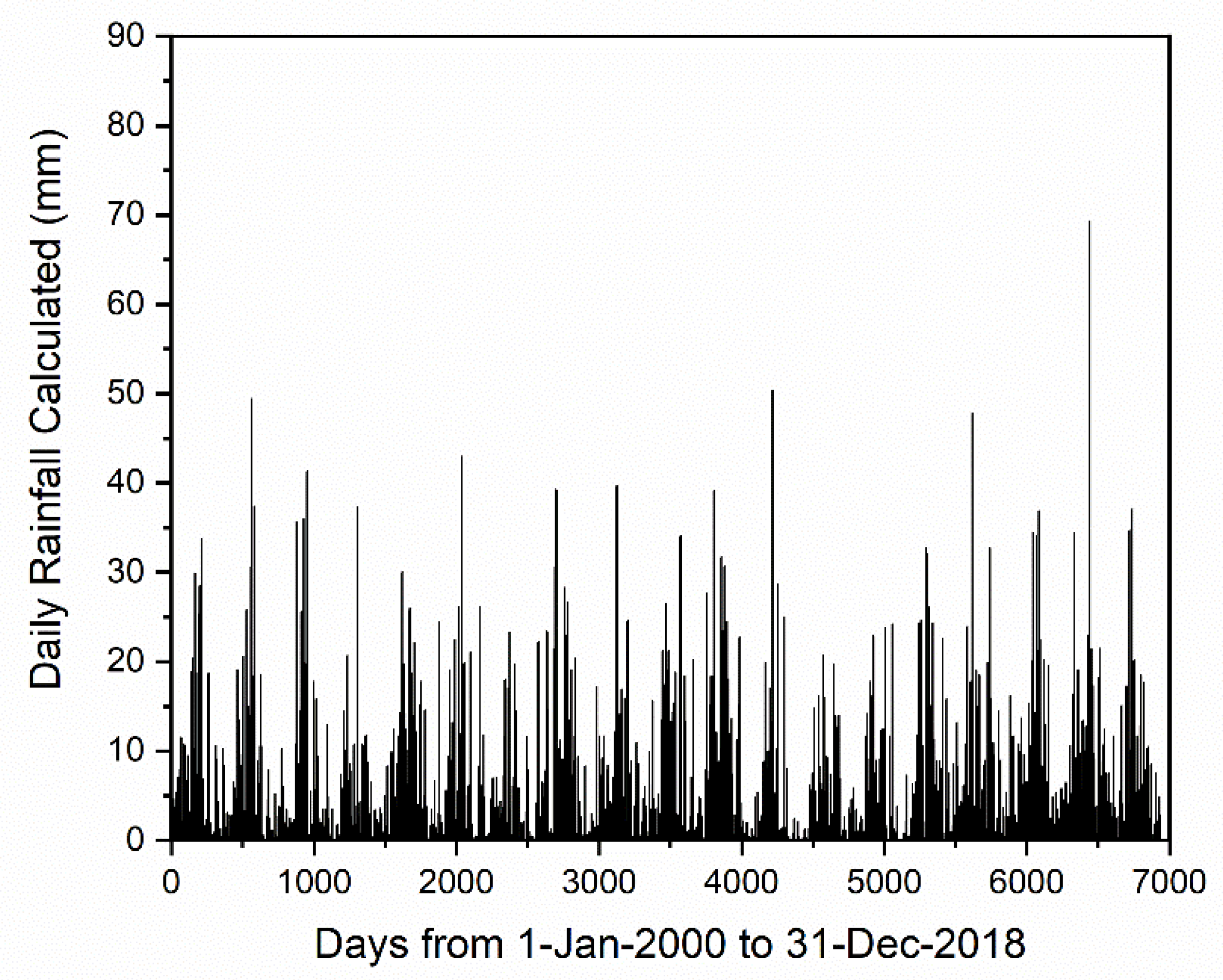
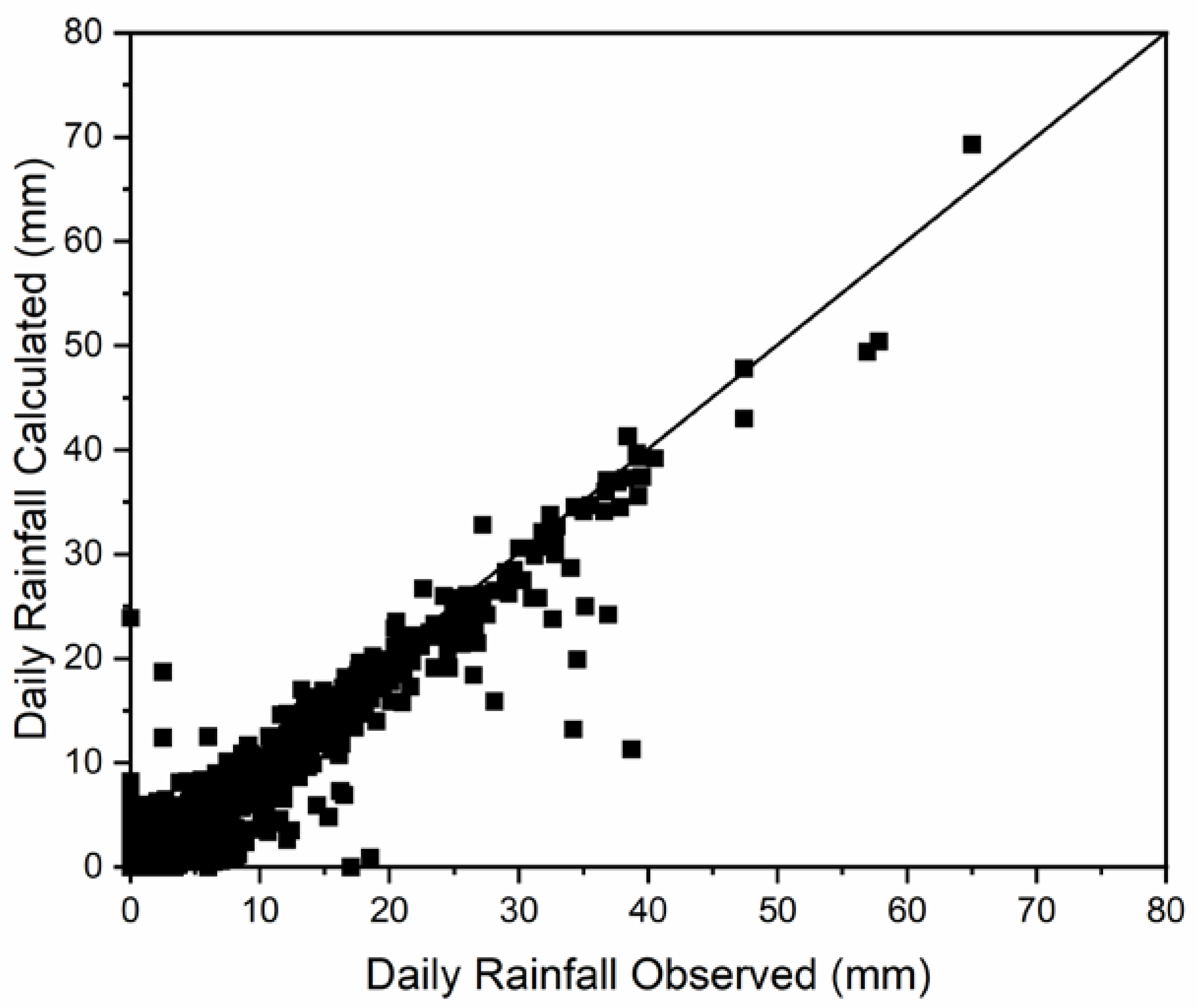
3.1. Basic Statistical Analysis
3.2. Descriptive Statistical Analysis
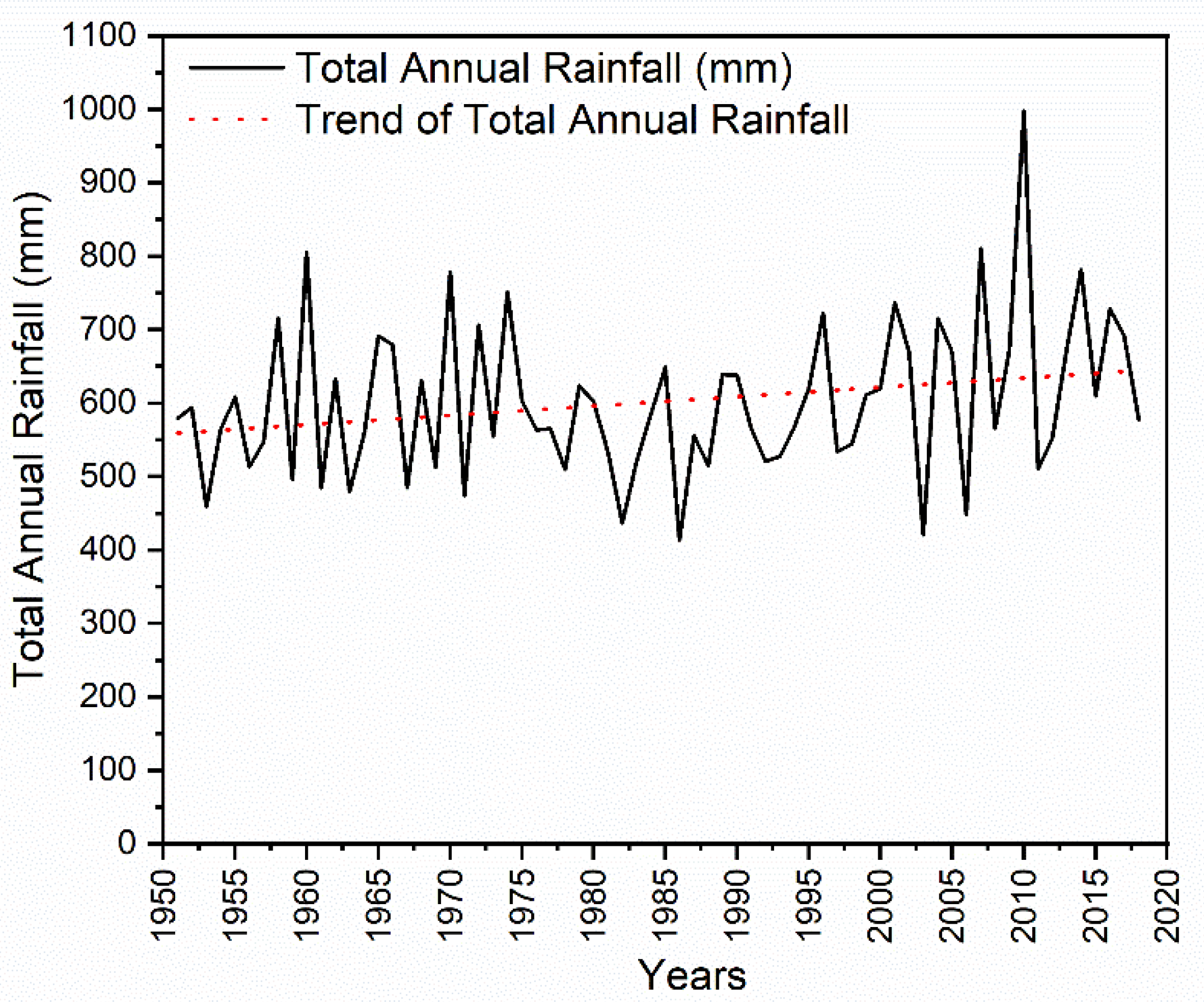
3.2.1. Trend Analysis of Daily Rainfall
3.2.2. Stationarity, Homogeneity, Noise and Periodicity of Daily Rainfall
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Chow, V.T.; Maidment, D.R.; Mays, L.W. Applied Hydrology; International edition; McGraw-Hill, Inc.: New York, NY, USA, 1988; p. 350. [Google Scholar]
- Drissia, T.K.; Jothiprakash, V.; Anitha, A.B. Statistical classification of streamflow based on flow variability in west flowing rivers of Kerala, India. Theor. Appl. Climatol. 2019, 137, 1643–1658. [Google Scholar] [CrossRef]
- Praveenkumar, C.; Jothiprakash, V. Spatio-temporal trend and homogeneity analysis of gridded and gauge precipitation in Indravati River basin, India. J. Water Clim. Chang. 2020, 11, 178–199. [Google Scholar] [CrossRef]
- Zeleňáková, M.; Alkhalaf, I.; Purcz, P.; Blišt’an, P.; Pelikán, P.; Portela, M.; Silva, A. Trends of rainfall as a support for integrated water resources management in Syria. Desalin. Water Treat. 2017, 86, 285–296. [Google Scholar] [CrossRef]
- Zeleňáková, M.; Vido, J.; Portela, M.M.; Purcz, P.; Blištán, P.; Hlavatá, H.; Hluštík, P. Precipitation Trends over Slovakia in the Period 1981–2013. Water 2017, 9, 922. [Google Scholar] [CrossRef]
- Zeleňáková, M.; Purcz, P.; Blišt’an, P.; Alkhalaf, I.; Hlavata, H.; Portela, M.M.; Silva, A.T. Precipitation trends detection as a tool for integrated water resources management in Slovakia. Desalin. Water Treat. 2017, 99, 83–90. [Google Scholar] [CrossRef]
- Zeleňáková, M.; Jothiprakash, V.; Arjun, S.; Káposztásová, D.; Hlavatá, H. Dynamic Analysis of Meteorological Parameters in Košice Climatic Station in Slovakia. Water 2018, 10, 702. [Google Scholar] [CrossRef]
- Afzal, M.; Mansell, M.G.; Gagnon, A.S. Trends and variability in daily precipitation in Scotland. Procedia Environ. Sci. 2011, 6, 15–26. [Google Scholar] [CrossRef]
- Gong, D.Y.; Shi, P.J.; Wang, J.A. Daily precipitation changes in the semi-arid region over northern China. J. Arid Environ. 2004, 59, 771–784. [Google Scholar] [CrossRef]
- Croitoru, A.E.; Piticar, A.; Burada, D.C. Changes in precipitation extremes in Romania. Quat. Int. 2016, 415, 325–335. [Google Scholar] [CrossRef]
- Keggenhoff, I.; Elizbarashvili, M.; Amiri-Farahani, A.; King, L. Trends in daily temperature and precipitation extremes over Georgia, 1971–2010. Weather Clim. Extrem. 2014, 4, 75–85. [Google Scholar] [CrossRef]
- Caloiero, T.; Coscarelli, R.; Ferrari, E.; Sirangelo, B. Trends in the daily precipitation categories of Calabria (southern Italy). Procedia Eng. 2016, 162, 32–38. [Google Scholar] [CrossRef]
- Zubieta, R.; Saavedra, M.; Silva, Y.; Giráldez, L. Spatial analysis and temporal trends of daily precipitation concentration in the Mantaro River basin: Central Andes of Peru. Stoch. Environ. Res. Risk Assess. 2017, 31, 1305–1318. [Google Scholar] [CrossRef]
- Bičárová, S.; Holko, L. Changes of characteristics of daily precipitation and runoff in the High Tatra Mountains, Slovakia over the last fifty years. Contrib. Geophys. Geod. 2013, 43, 157–177. [Google Scholar] [CrossRef]
- Gaál, L.; Kyselý, J.; Szolgay, J. Region-of-influence approach to a frequency analysis of heavy precipitation in Slovakia. Hydrol. Earth Syst. Sci. Discuss. 2008, 12, 825–839. [Google Scholar] [CrossRef]
- Bara, M.; Kohnova, S.; Gaal, L.; Szolgay, J.; Hlavčova, K. Estimation of IDF curves of extreme rainfall by simple scaling in Slovakia. Contrib. Geophys. Geod. 2009, 39, 187–206. [Google Scholar]
- Šamaj, F.; Valovič, Š. Intenzity Krátkodobých Dažďov na Slovensku; Slov. Pedagog. Nakl.: Bratislava, Slovakia, 1973; p. 93. [Google Scholar]
- Dub, O.; Nemec, J. Hydrologie; Státní Nakladatelství Technické Literatury: Praha, Czech Republic, 1969; p. 378. [Google Scholar]
- Kottegoda, N.T. Stochastic Water Resources Technology, 1st ed.; The Macmillan Press Ltd.: London, UK, 1980; p. 384. [Google Scholar]
- Chatfield, C. Time Series Forecasting; Chapman & Hall/CRC: London, UK, 2000. [Google Scholar]
- Dahmen, E.R.; Hall, M.J. Screening of Hydrological Data: Tests for Stationarity and Relative Consistency; International Institute for Land Reclamation and Improvement: Wageningen, The Netherlands, 1990. [Google Scholar]
- Akinsanola, A.A.; Ogunjobi, K.O. Recent homogeneity analysis and long-term spatio-temporal rainfall trends in Nigeria. Theor. Appl. Clim. 2015, 128, 275–289. [Google Scholar] [CrossRef]
- Anděl, J. Statistická Analýza Časových Řad; Státní Nakladatelství Technické Literatury: Praha, Czech Republic, 1976; p. 272. [Google Scholar]
- Gilbert, R.O. Statistical Methods for Environmental Pollution Monitoring; John Wiley & Sons: Hoboken, NJ, USA, 1987. [Google Scholar]
- Sen, P.K. Estimates of the regression coefficient based on Kendall’s tau. J. Am. Stat. Assoc. 1968, 63, 1379–1389. [Google Scholar] [CrossRef]
- Gautheir, T.D. Detecting trends using Spearman’s rank correlation coefficient. Environ. Forensics 2001, 2, 359–362. [Google Scholar] [CrossRef]
- Dickey, D.A.; Fuller, W.A. Distribution of the estimators for autoregressive time series with a unit root. J. Am. Stat. Assoc. 1979, 74, 427–431. [Google Scholar]
- Phillips, P.C.; Perron, P. Testing for a unit root in time series regression. Biometrika 1988, 75, 335–346. [Google Scholar] [CrossRef]
- Kwiatkowski, D.; Phillips, P.C.; Schmidt, P.; Shin, Y. Testing the null hypothesis of stationarity against the alternative of a unit root. J. Econ. 1992, 54, 159–178. [Google Scholar] [CrossRef]
- Pettitt, A.N. A non-parametric approach to the change-point problem. J. R. Stat. Soc. Ser. C (Appl. Stat.) 1979, 28, 126–135. [Google Scholar] [CrossRef]
- Alexandersson, H.; Moberg, A. Homogenization of Swedish temperature data. Part I: Homogeneity test for linear trends. Int. J. Climatol. J. R. Meteorol. Soc. 1997, 17, 25–34. [Google Scholar]
- Buishand, T.A.; De Haan, L.; Zhou, C. On spatial extremes: With application to a rainfall problem. Ann. Appl. Stat. 2008, 2, 624–642. [Google Scholar] [CrossRef]
- Von Neumann, J. Distribution of the ratio of the mean square successive difference to the variance. Ann. Math. Stat. 1941, 12, 367–395. [Google Scholar] [CrossRef]
- Box, G.E.; Pierce, D.A. Distribution of residual autocorrelations in autoregressive-integrated moving average time series models. J. Am. Stat. Assoc. 1970, 65, 1509–1526. [Google Scholar] [CrossRef]
- Ljung, G.M.; Box, G.E. On a measure of lack of fit in time series models. Biometrika 1978, 65, 297–303. [Google Scholar] [CrossRef]
- McLeod, A.I.; Li, W.K. Diagnostic checking ARMA time series models using squared-residual autocorrelations. J. Time Ser. Anal. 1983, 4, 269–273. [Google Scholar] [CrossRef]
- Fisher, R.A. Statistical methods for research workers. In Breakthroughs in Statistics; Springer: New York, NY, USA, 1992; pp. 66–70. [Google Scholar]
- Bartlett, M.S. An Introduction to Stochastic Processes: With Special Reference to Methods and Applications; CUP Archive: London, UK, 1978. [Google Scholar]
- Schuster, A. On the investigation of hidden periodicities with application to a supposed 26 day period of meteorological phenomena. Terr. Magn. 1989, 3, 13–41. [Google Scholar] [CrossRef]
- Elfeky, M.G.; Aref, W.G.; Elmagarmid, A.K. Periodicity detection in time series databases. IEEE Trans. Knowl. Data Eng. 2005, 17, 875–887. [Google Scholar] [CrossRef]


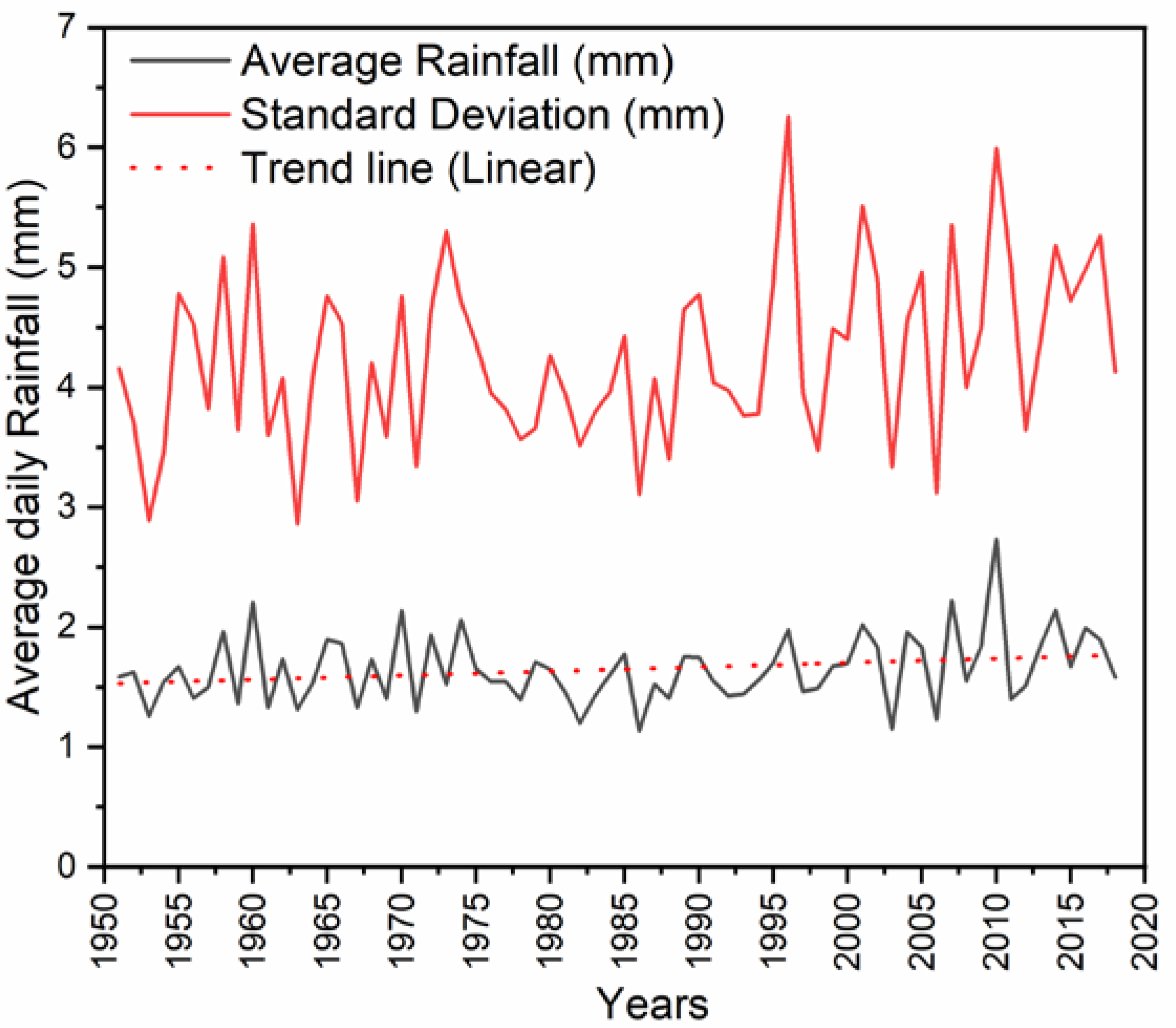
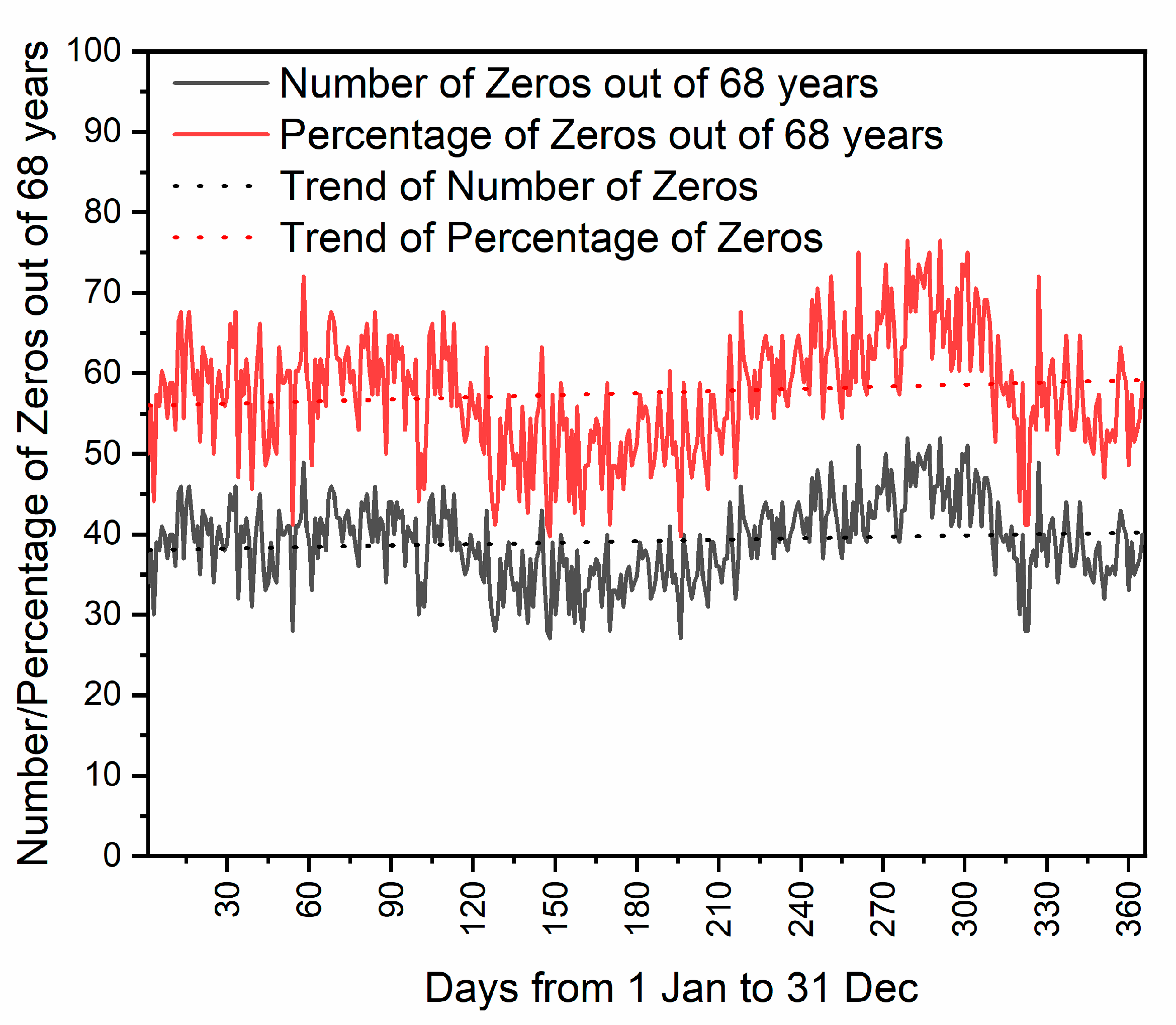
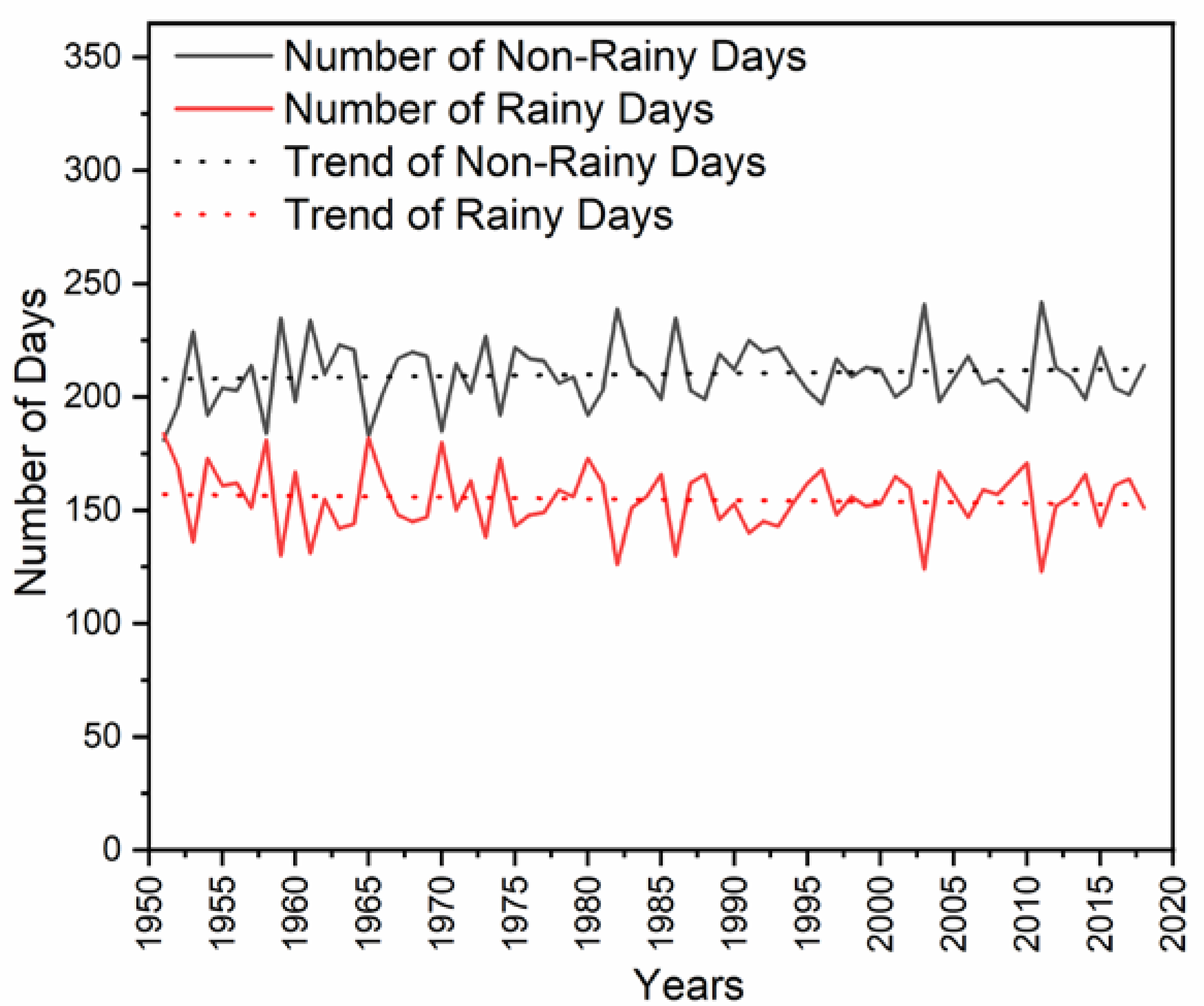
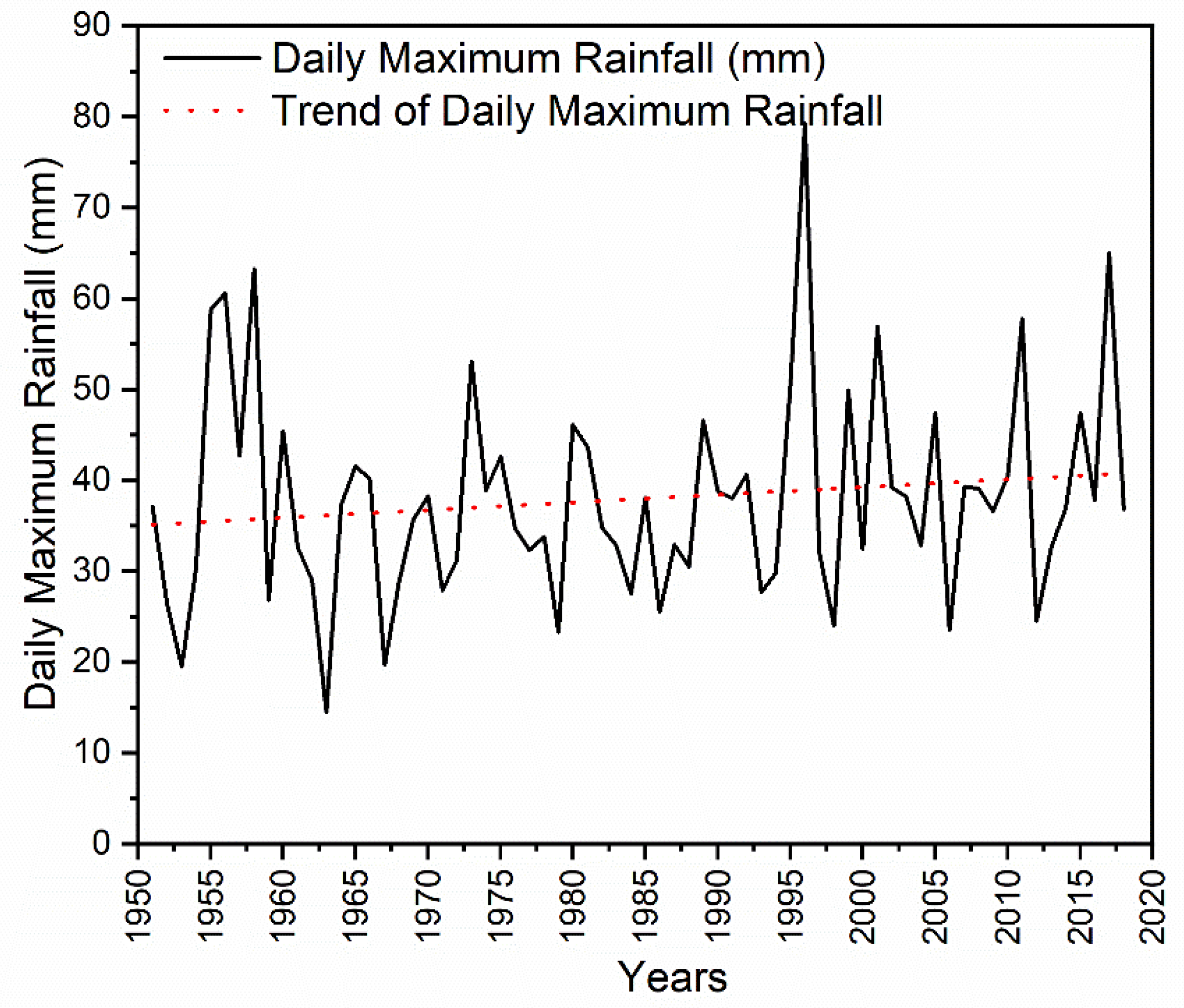
| Daily Data: Manually Recorded (2000–2018) | Daily Data Developed from Automatically Recorded 10 Min of Data (2000–2018) | Daily Data: Manually Recorded (1951–2018) | |
|---|---|---|---|
| Total of rainfall (in mm) | 12,452.8 | 11,252.6 | 40,924.3 |
| Average daily rainfall (in mm) | 1.8 | 1.6 | 1.6 |
| Average annual rainfall (in mm) | 655.4 | 592.2 | 601.8 |
| Maximum daily rainfall (in mm) | 65.0 | 69.3 | 79.3 |
| Pearson correlation coefficient | 0.9732 | - | |
| Trend | Stationarity | Homogeneity | Periodicity | Noise |
|---|---|---|---|---|
| Mann–Kendall: Kendall’s tau = −0.0025 p-value = 0.598 alpha = 0.05 NO TREND | ADF test p-value = 0.00001 alpha = 0.05 STATIONARY | Pettit test: p-value = 0.2188 alpha = 0.05 HOMOGENEOUS | 365.25 d | Box–Pierce: p-value = 0.0001 alpha = 0.05 NO NOISE |
| Sen’s slope: Slope = 0.000 NO TREND | Phillips–Perron p-value = 0.001 alpha = 0.05 STATIONARY | SNH test p-value = 0.098 alpha = 0.05 HOMOGENEOUS | Ljung–Box: p-value = 0.0001 alpha = 0.05 NO NOISE | |
| Spearman’s rank: Coeff. = 0.34 WEAK TREND | KPSS test p-value = 0.1413 alpha = 0.05 STATIONARY | Buishand’s p-value = 0.0768 alpha = 0.05 HOMOGENEOUS | McLeod–Li: p-value = 0.0001 alpha = 0.05 NO NOISE | |
| Von Neumann ratio: p-value = 0.9434 alpha = 0.05 HOMOGENEOUS | Fisher’s kappa: p-value = 0.0001 alpha = 0.05 NO NOISE | |||
| Bartlett’s Kolmogorov–Smirnov: p-value = 0.0001 alpha = 0.05 NO NOISE |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Repel, A.; Jothiprakash, V.; Zeleňáková, M.; Hlavatá, H.; Minea, I. Temporal Analysis of Daily and 10 Minutes of Rainfall of Poprad Station in Eastern Slovakia. Hydrology 2020, 7, 32. https://doi.org/10.3390/hydrology7020032
Repel A, Jothiprakash V, Zeleňáková M, Hlavatá H, Minea I. Temporal Analysis of Daily and 10 Minutes of Rainfall of Poprad Station in Eastern Slovakia. Hydrology. 2020; 7(2):32. https://doi.org/10.3390/hydrology7020032
Chicago/Turabian StyleRepel, Adam, Vinayakam Jothiprakash, Martina Zeleňáková, Helena Hlavatá, and Ionut Minea. 2020. "Temporal Analysis of Daily and 10 Minutes of Rainfall of Poprad Station in Eastern Slovakia" Hydrology 7, no. 2: 32. https://doi.org/10.3390/hydrology7020032
APA StyleRepel, A., Jothiprakash, V., Zeleňáková, M., Hlavatá, H., & Minea, I. (2020). Temporal Analysis of Daily and 10 Minutes of Rainfall of Poprad Station in Eastern Slovakia. Hydrology, 7(2), 32. https://doi.org/10.3390/hydrology7020032







