Fe Atom—Mixed Edges Fractal Graphene via DFT Calculation
Abstract
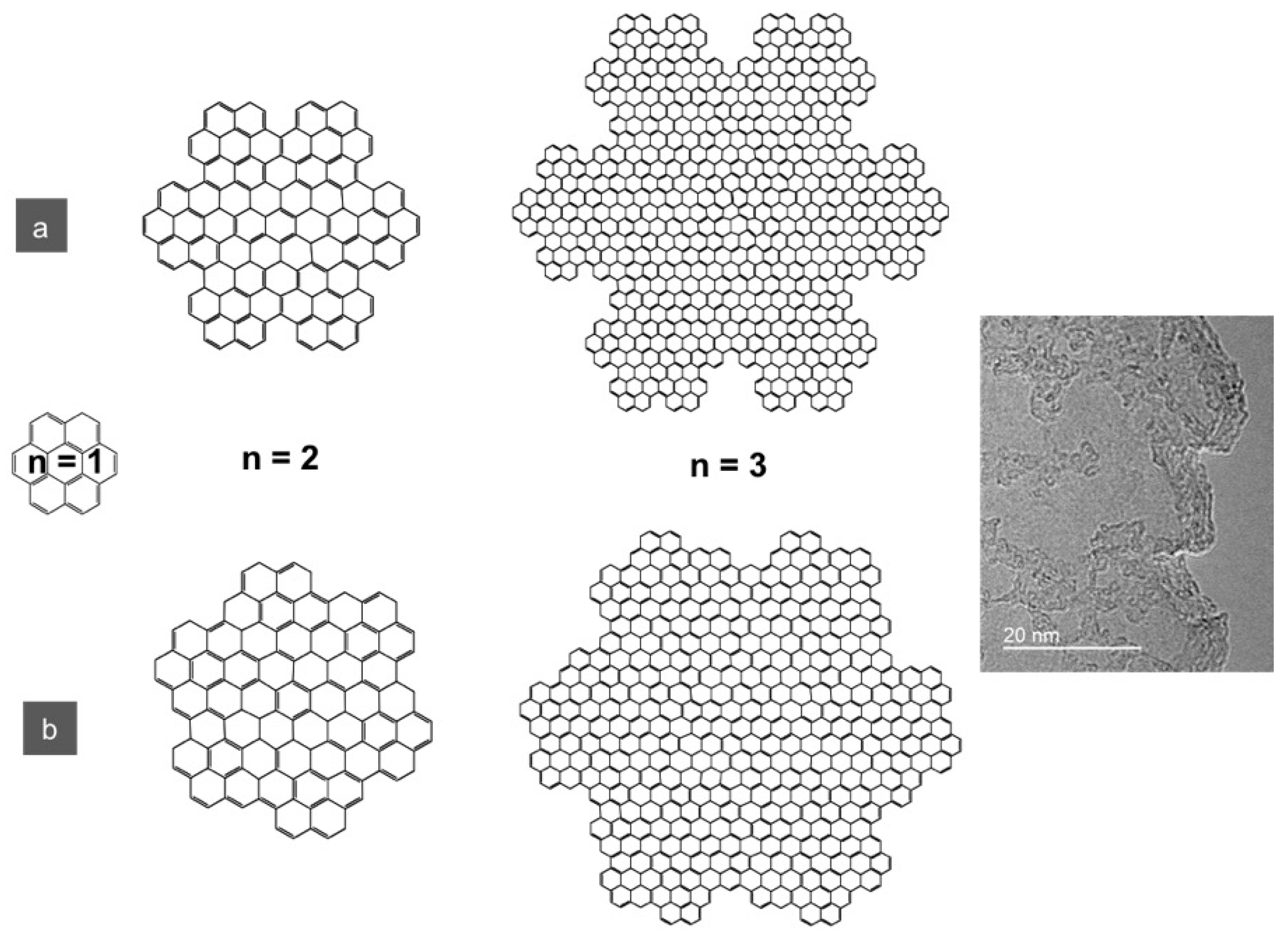
:1. Introduction
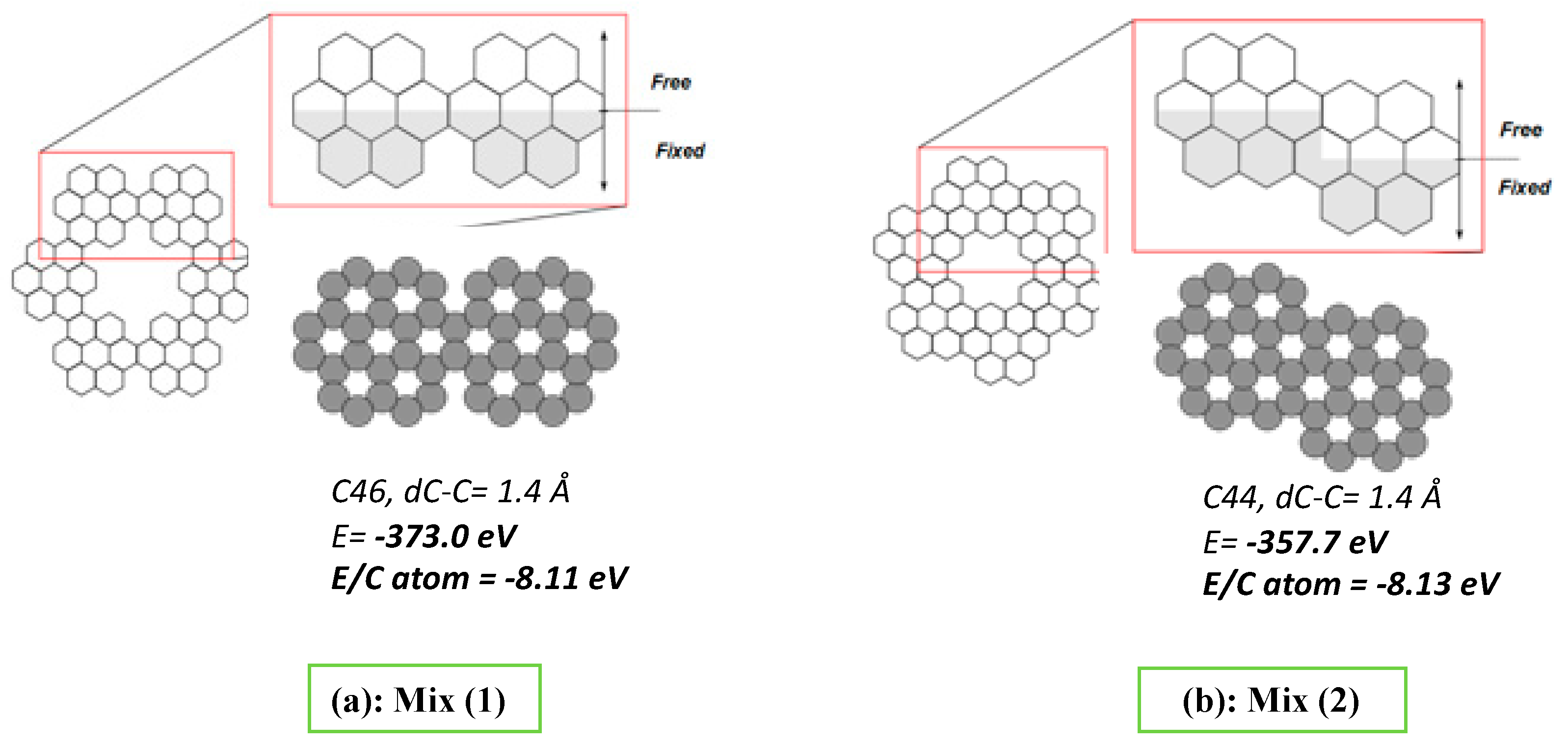
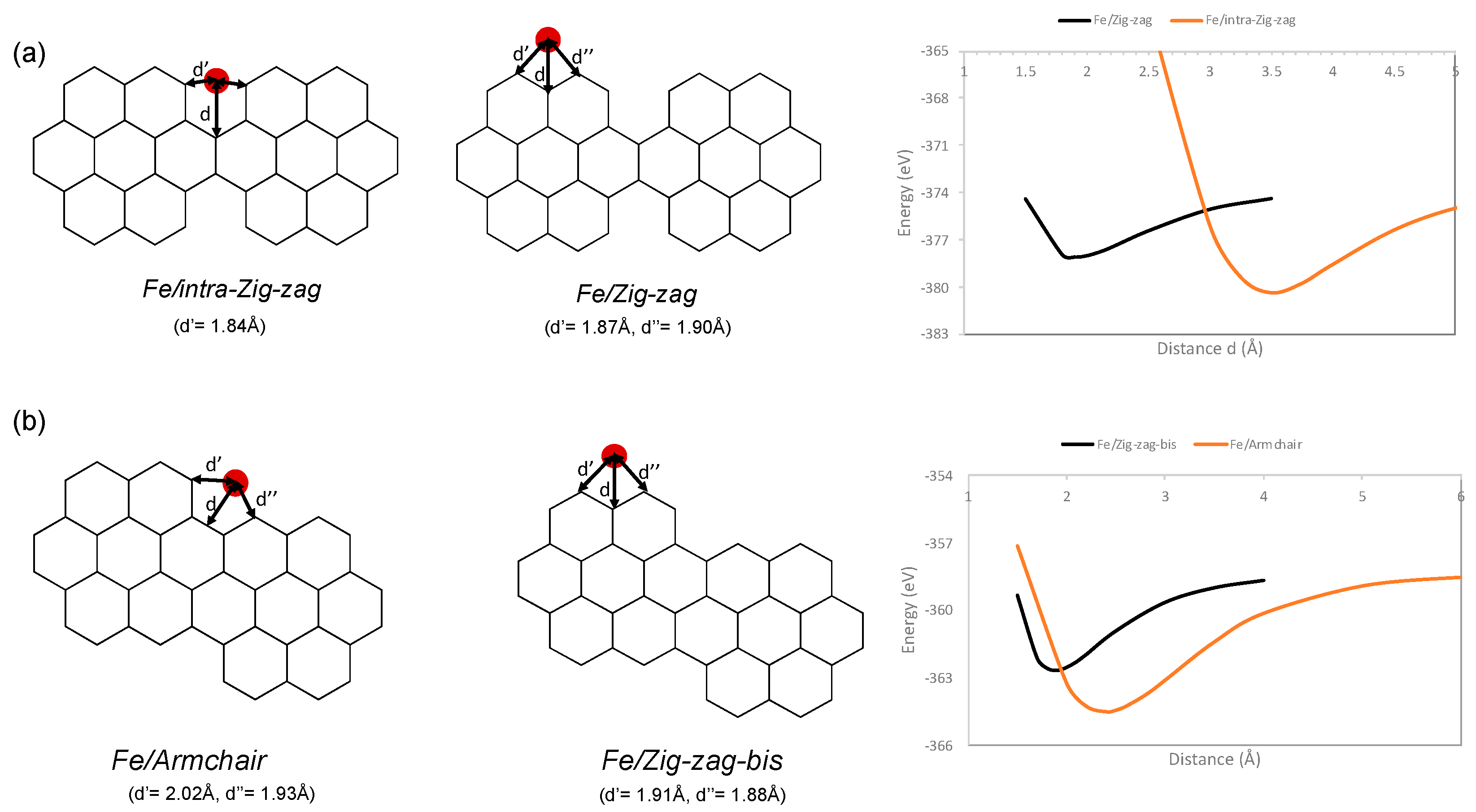
2. Materials and Methods
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Delplace, P.; Montambaux, G. WKB analysis of edge states in graphene in a strong magnetic field. Phys. Rev. B 2010, 82, 205412. [Google Scholar] [CrossRef] [Green Version]
- Park, C.; Yang, H.; Mayne, A.J.; Dujardin, G.; Seo, S.; Kuk, Y.; Ihm, J.; Kim, G. Formation of unconventional standing waves at graphene edges by valley mixing and pseudospin rotation. Proc. Natl. Acad. Sci. USA 2011, 108, 18622–18625. [Google Scholar] [CrossRef] [Green Version]
- Yamijala, S.S.R.K.C.; Bandyopadhyay, A.; Pati, S.K. Electronic properties of zigzag, armchair and their hybrid quantum dots of graphene and boron-nitride with and without substitution: A DFT study. Chem. Phys. Lett. 2014, 603, 28–32. [Google Scholar] [CrossRef] [Green Version]
- Sk, M.A.; Huang, L.; Chen, P.; Lim, K.H. Controlling armchair and zigzag edges in oxidative cutting of graphene. J. Mater. Chem. C 2016, 4, 6539–6545. [Google Scholar] [CrossRef]
- Klein, D.J. Graphitic polymer strips with edge states. Chem. Phys. Lett. 1994, 217, 261–265. [Google Scholar] [CrossRef]
- He, K.; Robertson, A.W.; Lee, S.; Yoon, E.; Lee, G.-D.; Warner, J.-H. Extended Klein Edges in Graphene. ACS Nano 2014, 8, 12272–12279. [Google Scholar] [CrossRef]
- Wagner, P.; Ivanovskaya, V.V.; Melle-Franco, M.; Humbert, B.; Adjizian, J.-J.; Briddon, P.R.; Ewels, C.P. Stable hydrogenated graphene edge types: Normal and reconstructed Klein edges. Phys. Rev. B 2013, 88, 094106. [Google Scholar] [CrossRef] [Green Version]
- Zhang, X.; Yazyev, O.V.; Feng, J.; Xie, L.; Tao, C.; Chen, Y.-C.; Jiao, L.; Pedramrazi, Z.; Zettl, A.; Louie, S.G.; et al. Experimentally Engineering the Edge Termination of Graphene Nanoribbons. ACS Nano 2013, 7, 198–202. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- He, K.; Lee, G.D.; Robertson, A.W.; Yoon, E.; Warner, J.H. Hydrogen-free graphene edges. Nat. Commun. 2014, 5, 3040. [Google Scholar] [CrossRef] [Green Version]
- Chełmecka, E.; Pasterny, K.; Kupka, T.; Stobiński, L. DFT studies of COOH tip-functionalized zigzag and armchair single wall carbon nanotubes. J. Mol. Model. 2012, 18, 2241–2246. [Google Scholar] [CrossRef] [PubMed]
- Chełmecka, E.; Pasterny, K.; Kupka, T.; Stobiński, L. OH-functionalized open-ended armchair single-wall carbon nanotubes (SWCNT) studied by density functional theory. J. Mol. Model. 2012, 18, 1463–1472. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Gusynin, V.P.; Miransky, V.A.; Sharapov, S.G.; Shovkovy, I.A. Edge states, mass and spin gaps, and quantum Hall effect in graphene. Phys. Rev. B 2008, 77, 205409. [Google Scholar] [CrossRef] [Green Version]
- Santana, A.; Popov, A.M.; Bichoutskaia, E. Stability and dynamics of vacancy in graphene flakes: Edge effects. Chem. Phys. Lett. 2013, 557, 80–87. [Google Scholar] [CrossRef] [Green Version]
- Terrones, M.; Botello-Méndez, A.R.; Campos-Delgado, J.; López-Urías, F.; Vega-Cantú, Y.I.; Rodríguez-Macías, F.J.; Elías, A.L.; Muñoz-Sandoval, E.; Cano-Márquez, A.G.; Charlier, J.-C.; et al. Graphene and graphite nanoribbons: Morphology, properties, synthesis, defects and applications. Nano Today 2010, 5, 351–372. [Google Scholar] [CrossRef]
- Yang, X.; Dou, X.; Rouhanipour, A.; Zhi, L.; Räder, H.J.; Müllen, K. Two-Dimensional Graphene Nanoribbons. J. Am. Chem. Soc. 2008, 130, 4216–4217. [Google Scholar] [CrossRef]
- Dössel, L.; Gherghel, L.; Feng, X.; Müllen, K. Graphene Nanoribbons by Chemists: Nanometer-Sized, Soluble, and Defect-Free. Angew. Chem. Int. Ed. 2011, 50, 2540–2543. [Google Scholar] [CrossRef]
- Ruffieux, P.; Wang, S.; Yang, B.; Sánchez-Sánchez, C.; Liu, J.; Dienel, T.; Talirz, L.; Shinde, P.; Pognedoli, C.A.; Passerone, D.; et al. On-surface synthesis of graphene nanoribbons with zigzag edge topology. Nature 2016, 531, 489–492. [Google Scholar] [CrossRef] [Green Version]
- Feng, J.; Li, W.; Qian, X.; Qi, J.; Qi, L.; Li, J. Patterning of graphene. Nanoscale 2012, 4, 4883–4899. [Google Scholar] [CrossRef]
- Schäffel, F.; Warner, J.H.; Bachmatiuk, A.; Rellinghaus, B.; Büchner, B.; Schultz, L.; Rümmeli, M.H. Shedding Light on the Crystallographic Etching of Multi-Layer Graphene at the Atomic Scale. Nano Res. 2009, 2, 695–705. [Google Scholar] [CrossRef] [Green Version]
- Baaziz, W.; Melinte, G.; Ersen, O.; Pham-Huu, C.; Janowska, I. Effect of nitriding/nanostructuration of few layer graphene supported iron-based particles; Catalyst in graphene etching and carbon nanofilament growth. PhysChemChemPhys 2014, 16, 15988–15993. [Google Scholar] [CrossRef]
- Geng, D.; Wu, B.; Guo, Y.; Luo, B.; Xue, Y.; Chen, J.; Yu, G.; Liu, Y. Fractal Etching of Graphene. J. Am. Chem. Soc. 2013, 135, 6431–6434. [Google Scholar] [CrossRef]
- Geng, D.; Wang, H.; Wan, Y.; Xu, Z.; Luo, B.; Xu, J.; Yu, G. Direct Top-Down Fabrication of Large-Area Graphene Arrays by an In Situ Etching Method. Adv. Mater. 2015, 27, 4195–4199. [Google Scholar] [CrossRef]
- Janowska, I.; Lafjah, M.; Papaefthymiou, V.; Pronkin, S.; Ulhaq-Bouillet, C. Edges fractal approach in graphene-Defects density gain. Carbon 2017, 123, 395–401. [Google Scholar] [CrossRef]
- Banhart, F.; Kotakoski, J.; Krasheninnikov, A.V. Structural Defects in Graphene. ACS Nano 2010, 5, 26–41. [Google Scholar] [CrossRef] [Green Version]
- Su, D.S.; Perathoner, S.; Centi, G. Nanocarbons for the Development of Advanced Catalysts. Chem. Rev. 2012, 113, 5782–5816. [Google Scholar] [CrossRef] [PubMed]
- Luo, G.; Liu, L.; Zhang, J.; Li, G.; Wang, B.; Zhao, J. Hole Defects and Nitrogen Doping in Graphene: Implication for Supercapacitor Applications. ACS Appl. Mater. Interfaces 2013, 5, 11184–11193. [Google Scholar] [CrossRef] [PubMed]
- Yuan, W.; Zhou, Y.; Li, Y.; Li, C.; Peng, H.; Zhang, J.; Liu, Z.; Dai, L.; Shi, G. The edge- and basal-plane-specific electrochemistry of a single-layer graphene sheet. Sci. Rep. 2013, 3, 2248. [Google Scholar] [CrossRef] [Green Version]
- Shen, A.; Zou, Y.; Wang, Q.; Dryfe, R.A.W.; Huang, X.; Dou, S.; Dai, L.; Wang, S. Oxygen Reduction Reaction in a Droplet on Graphite: Direct Evidence that the Edge Is More Active than the Basal Plane. Angew. Chem. Int. Ed. 2014, 53, 10804–10808. [Google Scholar] [CrossRef]
- Ricciardella, F.; Vollebregt, S.; Polichetti, T.; Miscuglio, M.; Alfano, B.; Miglietta, M.L.; Massera, E.; Di Francia, G.; Sarro, P.M. Effects of Graphene Defects on Gas Sensing Properties Towards NO2 Detection. Nanoscale 2017, 9, 6085–6093. [Google Scholar] [CrossRef] [Green Version]
- Janowska, I.; Moldovan, M.-S.; Ersen, O.; Bulou, H.; Chizari, K.; Ledoux, M.-J.; Pham-Huu, C. High-temperature stability of platinum nanoparticles on few-layer graphene investigated by in-situ HR-TEM. Nano Res. 2011, 4, 511–521. [Google Scholar] [CrossRef]
- Serp, P.; Pham, M.D. Supported Metal Single Atom Catalysis; Wiley-VCH GmbH: Weinheim, Germany, 2022. [Google Scholar]
- Zhao, J.; Deng, Q.; Avdoshenko, S.M.; Fu, L.; Eckert, J.; Rümmeli, M.H. Direct in situ observations of single Fe atom catalytic processes and anomalous diffusion at graphene edges. Proc. Natl. Acad. Sci. USA 2014, 111, 15641–15646. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Zhang, T.; Ding, K. Hierarchical fractal structure of perfect single-layer graphene. Front. Mech. Eng. 2013, 8, 371–382. [Google Scholar] [CrossRef]
- Dai, Y.-H. A perfect example for the BFGS method. Math. Program. Ser. A 2013, 138, 501–530. [Google Scholar] [CrossRef]
- Dennis, J.E.; Schnabel, R.B. Numerical Methods for Unconstrained Optimization and Nonlinear Equations; Society for Industrial and Applied Mathematics: Philadelphia, PA, USA, 1996. [Google Scholar]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Aloui, L.; Dintzer, T.; Janowska, I. Fe Atom—Mixed Edges Fractal Graphene via DFT Calculation. ChemEngineering 2022, 6, 79. https://doi.org/10.3390/chemengineering6050079
Aloui L, Dintzer T, Janowska I. Fe Atom—Mixed Edges Fractal Graphene via DFT Calculation. ChemEngineering. 2022; 6(5):79. https://doi.org/10.3390/chemengineering6050079
Chicago/Turabian StyleAloui, Lobna, Thierry Dintzer, and Izabela Janowska. 2022. "Fe Atom—Mixed Edges Fractal Graphene via DFT Calculation" ChemEngineering 6, no. 5: 79. https://doi.org/10.3390/chemengineering6050079
APA StyleAloui, L., Dintzer, T., & Janowska, I. (2022). Fe Atom—Mixed Edges Fractal Graphene via DFT Calculation. ChemEngineering, 6(5), 79. https://doi.org/10.3390/chemengineering6050079







