Modelling Particle Agglomeration on through Elastic Valves under Flow
Abstract
:1. Introduction
2. Methodology
2.1. The Theory of Discrete Multiphysics
2.1.1. Smoothed Particle Hydrodynamics (SPH)
2.1.2. Lattice Spring Model (LSM)
2.1.3. Coupling SPH and LSM (Fluid–Structure Interaction)
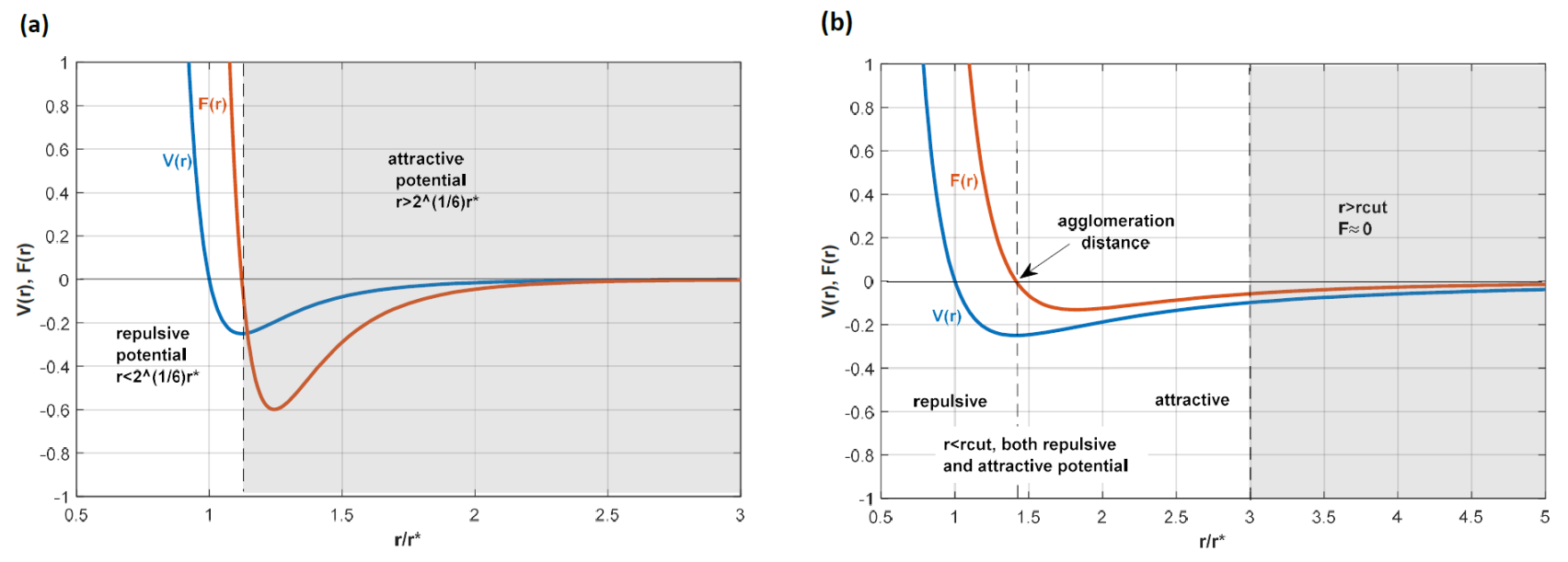
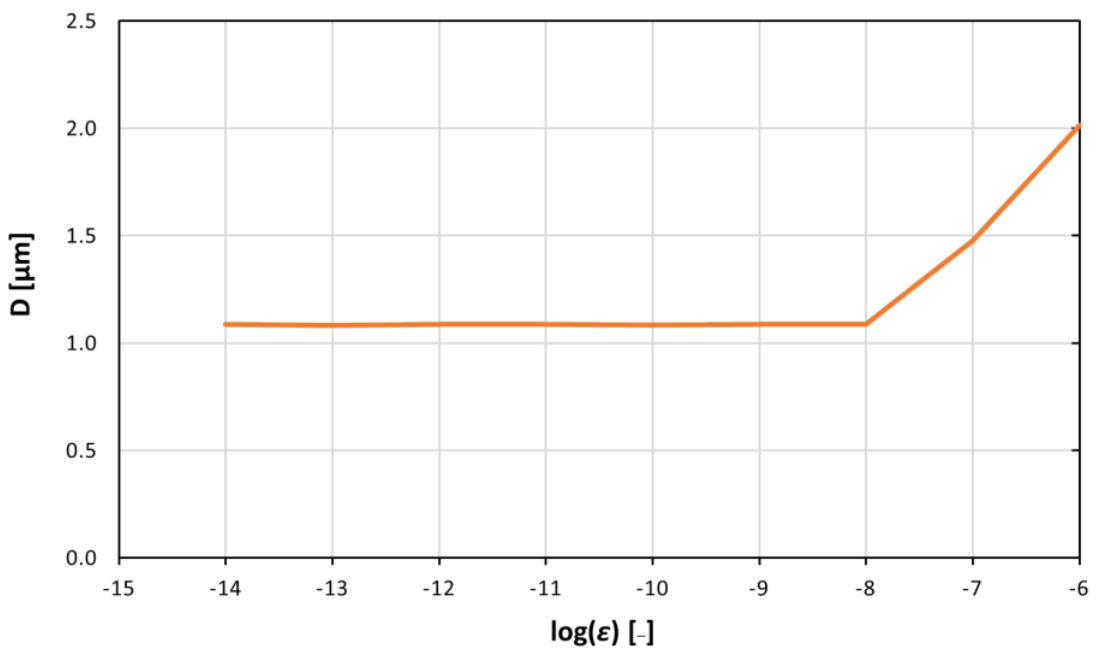
2.1.4. Solid-Solid Interaction (Agglomeration)
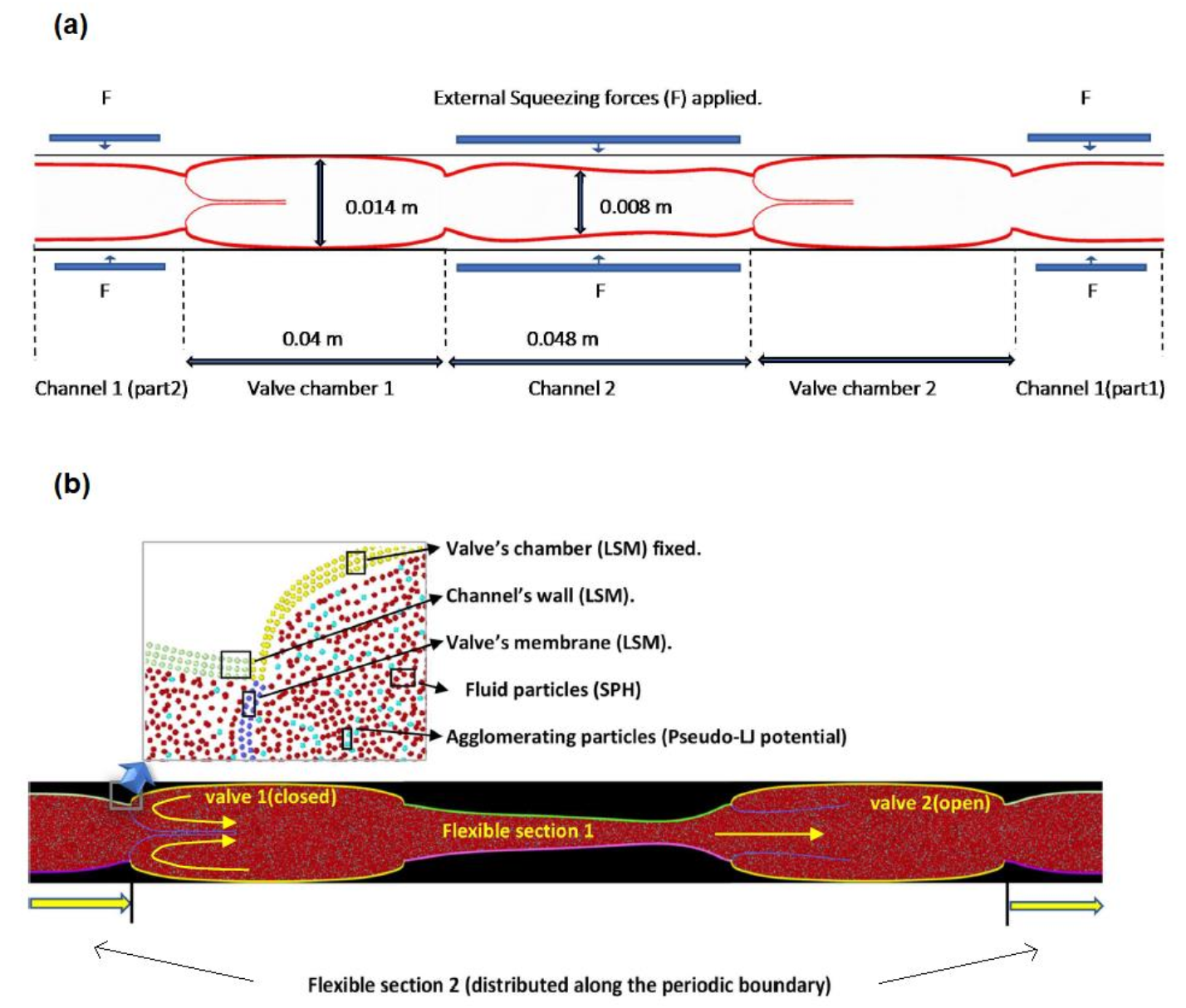
2.2. The Valve Model and Geometry
3. Results and Discussion:
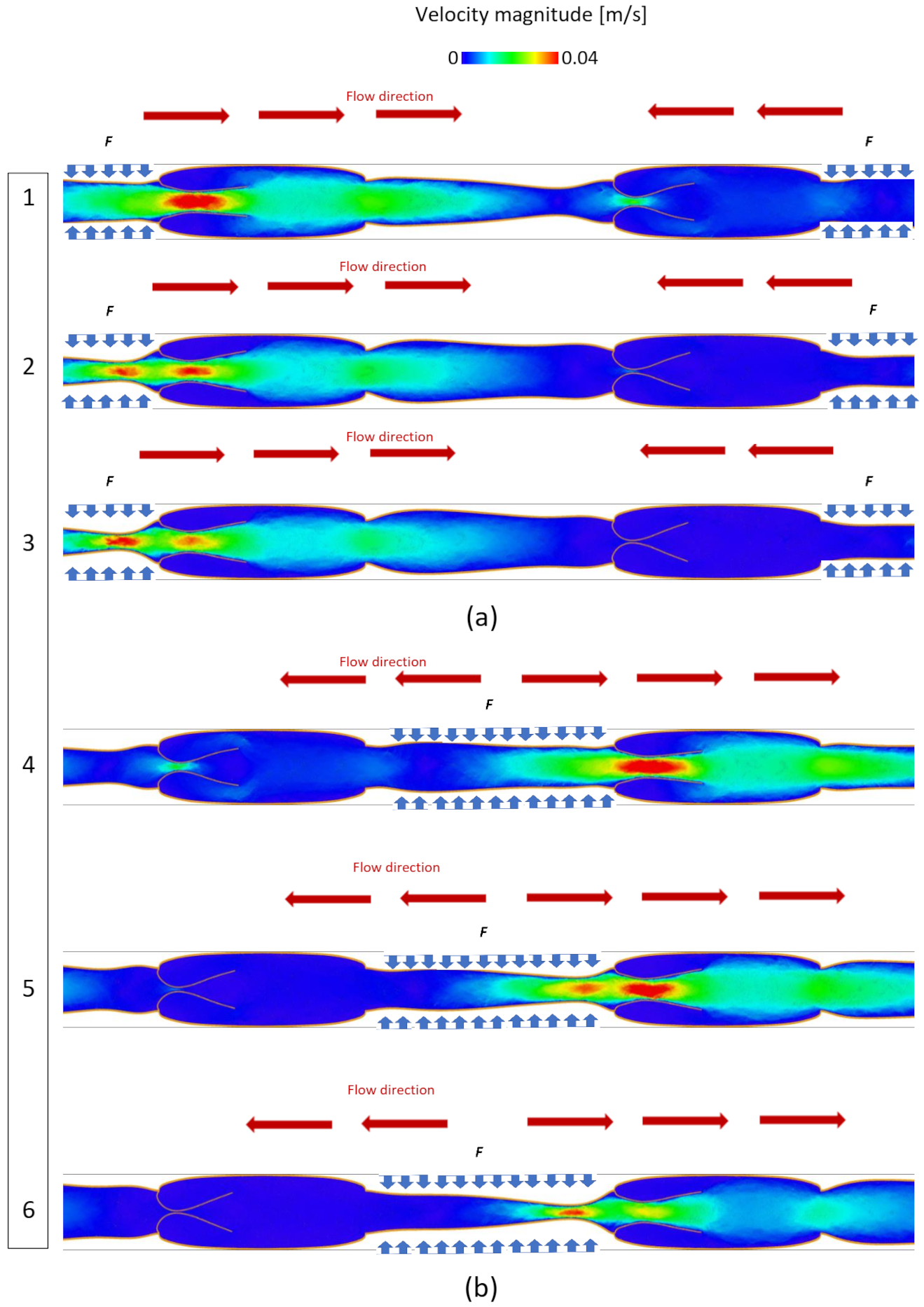
3.1. Hydrodynamics
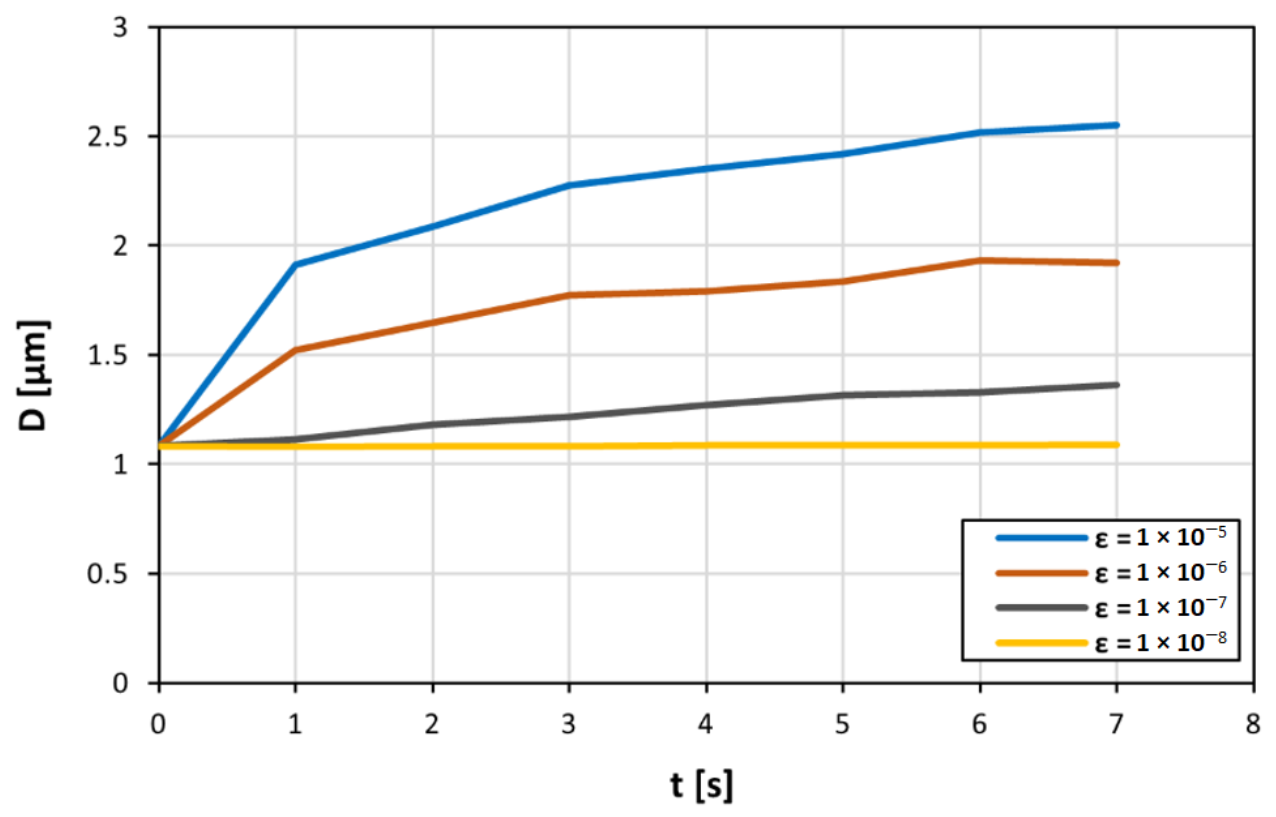
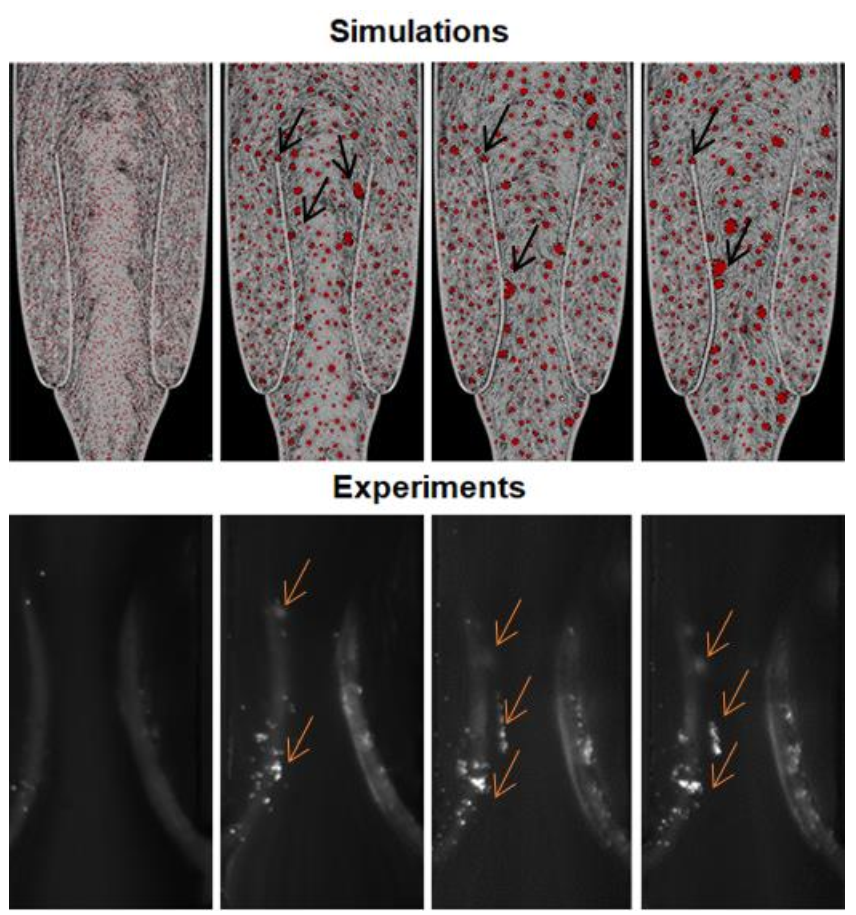
3.2. Particle Agglomeration
3.3. Larger Agglomerates
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Raskob, G.E.; Spyropoulos, A.C.; Cohen, A.T.; Weitz, J.I.; Ageno, W.; De Sanctis, Y.; Lu, W.; Xu, J.; Albanese, J.; Sugarmann, C.; et al. Association between Asymptomatic Proximal Deep Vein Thrombosis and Mortality in Acutely Ill Medical Patients. J. Am. Hear. Assoc. 2021, 10, e019459. [Google Scholar]
- Wu, W.T.; Zhussupbekov, M.; Aubry, N.; Antaki, J.F.; Massoudi, M. Simulation of thrombosis in a stenotic microchannel: The effects of vWF-enhanced shear activation of platelets. Int. J. Eng. Sci. 2020, 147, 103206. [Google Scholar] [CrossRef]
- Lurie, F.; Kistner, R.L.; Eklof, B.; Kessler, D. Mechanism of venous valve closure and role of the valve in circulation: A new concept. J. Vasc. Surg. 2003, 38, 955–961. [Google Scholar] [CrossRef]
- Shen, R.; Gao, M.; Tao, Y.; Chen, Q.; Wu, G.; Guo, X.; Xia, Z.; You, G.; Hong, Z.; Huang, K. Prognostic nomogram for 30-day mortality of deep vein thrombosis patients in intensive care unit. BMC Cardiovasc. Disord. 2021, 21, 11. [Google Scholar] [CrossRef]
- Bovill, E.G.; van der Vliet, A. Venous valvular stasis–associated hypoxia and thrombosis: What is the link? Annu. Rev. Physiol. 2011, 73, 527–545. [Google Scholar] [CrossRef] [PubMed]
- Siegal, D.M.; Eikelboom, J.W.; Lee, S.F.; Rangarajan, S.; Bosch, J.; Zhu, J.; Yusuf, S.; the Venous Thromboembolism Collaboration. Variations in incidence of venous thromboembolism in low-, middle-, and high-income countries. Cardiovasc. Res. 2021, 117, 576–584. [Google Scholar] [CrossRef]
- Das, K.; Biradar, M.S. (Eds.) Hypoxia and Anoxia; BoD–Books on Demand; IntechOpen: London, UK, 2018; ISBN 978-1-78984-828-1. [Google Scholar]
- Payne, H.; Brill, A. Stenosis of the Inferior Vena Cava: A Murine Model of Deep Vein Thrombosis. J. Vis. Exp. 2017, 2017, e56697. [Google Scholar] [CrossRef] [Green Version]
- Cook, D.J.; Crowther, M.A. Thromboprophylaxis in the intensive care unit: Focus on medical–surgical patients. Critical Care Med. 2010, 38, S76–S82. [Google Scholar] [CrossRef] [PubMed]
- Schofield, Z.; Baksamawi, H.A.; Campos, J.; Alexiadis, A.; Nash, G.B.; Brill, A.; Vigolo, D. The role of valve stiffness in the insurgence of deep vein thrombosis. Commun. Mater. 2020, 1, 1–10. [Google Scholar] [CrossRef]
- Ariane, M.; Wen, W.; Vigolo, D.; Brill, A.; Nash, F.G.B.; Barigou, M.; Alexiadis, A. Modelling and simulation of flow and agglomeration in deep veins valves using discrete multi physics. Comput. Biol. Med. 2017, 89, 96–103. [Google Scholar] [CrossRef]
- Ariane, M.; Allouche, H.; Bussone, M.; Giacosa, F.; Bernard, F.; Barigou, M.; Alexiadis, A. Discrete Multiphysics: A mesh-free approach to model biological valves including the formation of solid aggregates at the membrane surface and in the flow. PLoS ONE 2017, 12, e0174795. [Google Scholar] [CrossRef] [Green Version]
- Bain, B.J. Structure and function of red and white blood cells and platelets. Medicine 2021, 49, 183–188. [Google Scholar] [CrossRef]
- Cattaneo, M. Light Transmission Aggregometry and ATP Release for the Diagnostic Assessment of Platelet Function. Semin. Thromb. Hemost. 2009, 35, 158–167. [Google Scholar] [CrossRef]
- Alexiadis, A. The discrete multi-hybrid system for the simulation of solid-liquid flows. PLoS ONE 2015, 10, e0124678. [Google Scholar] [CrossRef] [Green Version]
- Alexiadis, A.; Stamatopoulos, K.; Wen, W.; Batchelor, H.; Bakalis, S.; Barigou, M.; Simmons, M. Using discrete multi-physics for detailed exploration of hydrodynamics in an in vitro colon system. Comput. Biol. Med. 2017, 81, 188–198. [Google Scholar] [CrossRef]
- Ariane, M.; Kassinos, S.; Velaga, S.; Alexiadis, A. Discrete multi-physics simulations of diffusive and convective mass transfer in boundary layers containing motile cilia in lungs. Comput. Biol. Med. 2018, 95, 34–42. [Google Scholar] [CrossRef] [PubMed]
- Ariane, M.; Vigolo, D.; Brill, A.; Nash, F.; Barigou, M.; Alexiadis, A. Using Discrete Multi-Physics for studying the dynamics of emboli in flexible venous valves. Comput. Fluids 2018, 166, 57–63. [Google Scholar] [CrossRef]
- Ariane, M.; Sommerfeld, M.; Alexiadis, A. Wall collision and drug-carrier detachment in dry powder inhalers: Using DEM to devise a sub-scale model for CFD calculations. Powder Technol. 2018, 334, 65–75. [Google Scholar] [CrossRef]
- Sanfilippo, D.; Ghiassi, B.; Alexiadis, A.; Hernandez, A.G. Combined peridynamics and discrete multiphysics to study the effects of air voids and freeze-thaw on the mechanical properties of asphalt. Materials 2021, 14, 1579. [Google Scholar] [CrossRef] [PubMed]
- Alexiadis, A.; Simmons, M.J.H.; Stamatopoulos, K.; Batchelor, H.K.; Moulitsas, I. The virtual physiological human gets nerves! How to account for the action of the nervous system in multiphysics simulations of human organs. J. R. Soc. Interface 2021, 18, 20201024. [Google Scholar] [CrossRef] [PubMed]
- Ruiz-Riancho, I.N.; Alexiadis, A.; Zhang, Z.; Hernandez, A.G. A discrete multi-physics model to simulate fluid structure interaction and breakage of capsules filled with liquid under coaxial load. Processes 2021, 9, 354. [Google Scholar] [CrossRef]
- Ng, K.C.; Alexiadis, A.; Chen, H.; Sheu, T.W.H. A coupled Smoothed Particle Hydrodynamics-Volume Compensated Particle Method (SPH-VCPM) for Fluid Structure Interaction (FSI) modelling. Ocean Eng. 2020, 218, 107923. [Google Scholar] [CrossRef]
- Rahmat, A.; Weston, D.; Madden, D.; Usher, S.; Barigou, M.; Alexiadis, A. Modeling the agglomeration of settling particles in a dewatering process. Phys. Fluids 2020, 32, 123314. [Google Scholar] [CrossRef]
- Mohammed, A.M.; Ariane, M.; Alexiadis, A. Using discrete multiphysics modelling to assess the effect of calcification on hemodynamic and mechanical deformation of aortic valve. ChemEngineering 2020, 4, 48. [Google Scholar] [CrossRef]
- Lucy, L.B. A numerical approach to the testing of the fission hypothesis. Astron. J. 1977, 82, 1013–1024. [Google Scholar] [CrossRef]
- Gingold, R.A.; Monaghan, J.J. Smoothed particle hydrodynamics: Theory and application to non-spherical stars. Mon. Not. R. Astron. Soc. 1977, 181, 375–389. [Google Scholar] [CrossRef]
- Liu, G.R.; Liu, M.B. Smoothed Particle Hydrodynamics: A Meshfree Particle Method; World Scientific: Singapore, 2003. [Google Scholar]
- Ganzenmüller, G.C.; Steinhauser, M.O.; Van Liedekerke, P.; Leuven, K.U. The implementation of Smooth Particle Hydrodynamics in LAMMPS. Paul Van Liedekerke Kathol. Univ. Leuven 2011, 1, 1–26. [Google Scholar]
- Morris, J.P.; Fox, P.J.; Zhu, Y. Modeling Low Reynolds Number Incompressible Flows Using SPH. J. Comput. Phys. 1997, 136, 214–226. [Google Scholar] [CrossRef]
- Monaghan, J.J. Simulating Free Surface. J. Comput. Phys. 1994, 110, 399–406. [Google Scholar] [CrossRef]
- Lee, E.S.; Moulinec, C.; Xu, R.; Violeau, D.; Laurence, D.; Stansby, P. Comparisons of weakly compressible and truly incompressible algorithms for the SPH mesh free particle method. J. Comput. Phys. 2008, 227, 8417–8436. [Google Scholar] [CrossRef]
- Kot, M.; Nagahashi, H.; Szymczak, P. Elastic moduli of simple mass spring models. Vis. Comput. 2015, 31, 1339–1350. [Google Scholar] [CrossRef]
- Pazdniakou, A.; Adler, P.M. Lattice Spring Models. Transp. Porous Media 2012, 93, 243–262. [Google Scholar] [CrossRef]
- Schütt, M.; Stamatopoulos, K.; Simmons, M.; Batchelor, H.; Alexiadis, A. Modelling and simulation of the hydrodynamics and mixing profiles in the human proximal colon using Discrete Multiphysics. Comput. Biol. Med. 2020, 121, 103819. [Google Scholar] [CrossRef] [PubMed]
- Boström, M.; Deniz, V.; Franks, G.; Ninham, B. Extended DLVO theory: Electrostatic and non-electrostatic forces in oxide suspensions. Adv. Colloid Interface Sci. 2006, 123–126, 5–15. [Google Scholar] [CrossRef] [PubMed]
- Wijeratne, N.S.; Hoo, K.A. Numerical studies on the hemodynamics in the human vein and venous valve. In Proceedings of the 2008 American Control Conference, Seattle, DC, USA, 11–13 June 2008; IEEE: New York, NY, USA, 2008; pp. 147–152. [Google Scholar]
- Aird, W.C. Vascular bed-specific thrombosis. J. Thromb. Haemost. 2007, 5, 283–291. [Google Scholar] [CrossRef]
- Plimpton, S. Fast parallel algorithms for short-range molecular dynamics. J. Comput. Phys. 1995, 117, 1–19. [Google Scholar] [CrossRef] [Green Version]
- Stukowski, A. Visualization and analysis of atomistic simulation data with OVITO-the Open Visualization Tool. Model. Simul. Mater. Sci. Eng. 2010, 18, 015012. [Google Scholar] [CrossRef]
| Parameter | Values and Units | |
|---|---|---|
| SPH | ||
| Number of all particles that created our model domain. | 168,676 | |
| Number of wall stationary particles (SPH particles), three layers. | 4972 | |
| Number of wall flexible particles (LSM particles), three layers. | 5750 | |
| Number of the valve’s particles (LSM particles), two layers. | Valve particles | 1404 |
| Each leaflet | 351 | |
| Number of SPH fluid particles | 141,030 | |
| Number of SPH agglomerating particles | 15,520 | |
| Mass of each particle (Fluid) | 1.056 × 10−5 kg | |
| Mass of each particle (Solid) | 2 × 10−5 kg | |
| Initial distance between particles | 10−4 m | |
| Density | 1056 kg m−3 | |
| Smoothing length | 2.5 × 10−4 m | |
| Dynamic viscosity | 0.0035 Pa s | |
| Virtual sound speed | 10 m s−1 | |
| Contraction Forces F | 0.008 N | |
| Max velocity in the valve | 0.04 m s−1 | |
| Time step | 10−6 s | |
| LSM | ||
| Hookian coefficient | Flexible wall | 1 × 105 J m−2 |
| Valve’s membrane | 5 × 106 J m−2 | |
| Viscous damping coefficient | Flexible wall | 1 kg s−1 |
| Valve’s membrane | 0.1 kg s−1 | |
| Equilibrium distance | 10−4 m | |
| Boundaries | ||
| Repulsive radius | 1 × 10−4 m | |
| Constant | 1 × 10−4 J | |
| Attractive forces potential | ||
| Mass of solid particles | 1.056 × 10−5 kg | |
| Solid diameter | 10−4 m | |
| Particle density | 1056 kg m−3 | |
| Pair potential | 2 × 10−5 J − 1 × 10−16 J | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Baksamawi, H.A.; Ariane, M.; Brill, A.; Vigolo, D.; Alexiadis, A. Modelling Particle Agglomeration on through Elastic Valves under Flow. ChemEngineering 2021, 5, 40. https://doi.org/10.3390/chemengineering5030040
Baksamawi HA, Ariane M, Brill A, Vigolo D, Alexiadis A. Modelling Particle Agglomeration on through Elastic Valves under Flow. ChemEngineering. 2021; 5(3):40. https://doi.org/10.3390/chemengineering5030040
Chicago/Turabian StyleBaksamawi, Hosam Alden, Mostapha Ariane, Alexander Brill, Daniele Vigolo, and Alessio Alexiadis. 2021. "Modelling Particle Agglomeration on through Elastic Valves under Flow" ChemEngineering 5, no. 3: 40. https://doi.org/10.3390/chemengineering5030040
APA StyleBaksamawi, H. A., Ariane, M., Brill, A., Vigolo, D., & Alexiadis, A. (2021). Modelling Particle Agglomeration on through Elastic Valves under Flow. ChemEngineering, 5(3), 40. https://doi.org/10.3390/chemengineering5030040








