Understanding the Frying Process of Plant-Based Foods Pretreated with Pulsed Electric Fields Using Frying Models
Abstract
1. Introduction
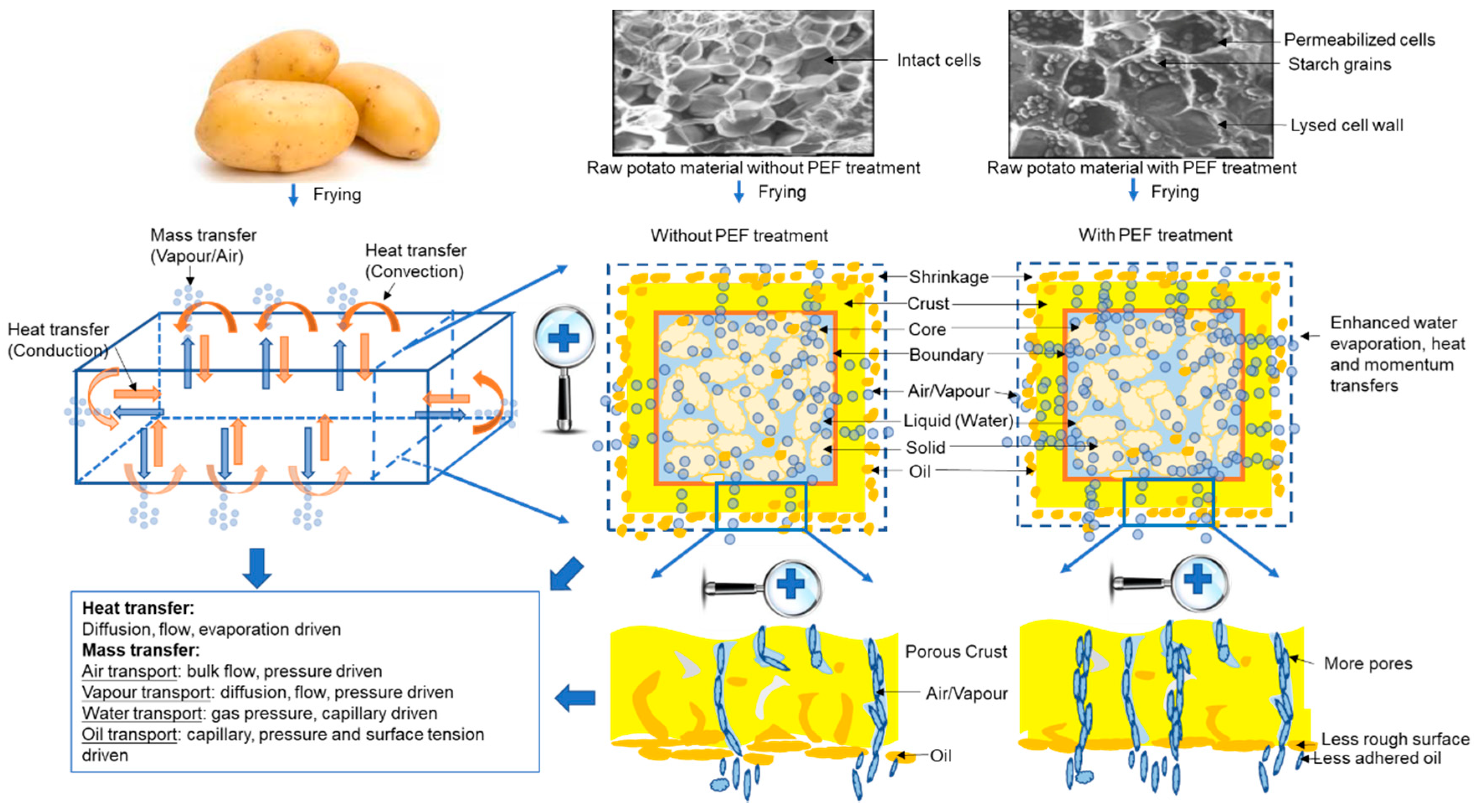
2. Frying Process of Food Materials
2.1. Heat Transfer
2.2. Mass Transfer
2.2.1. Water Transfer
2.2.2. Oil Transfer
2.3. Momentum Transfer
3. Changes in the Chemical Constituents of Plant Food Material during Frying
3.1. Maillard Reaction
3.2. Starch Swelling and Gelatinisation
4. An Overview of the Quality Parameters of Fried PEF-Treated Plant Food Materials
4.1. Colour
4.2. Moisture Content
4.3. Oil Uptake
4.4. Texture
4.4.1. Crispness
4.4.2. Porosity
4.4.3. Shrinkage
4.5. Toxic Compounds
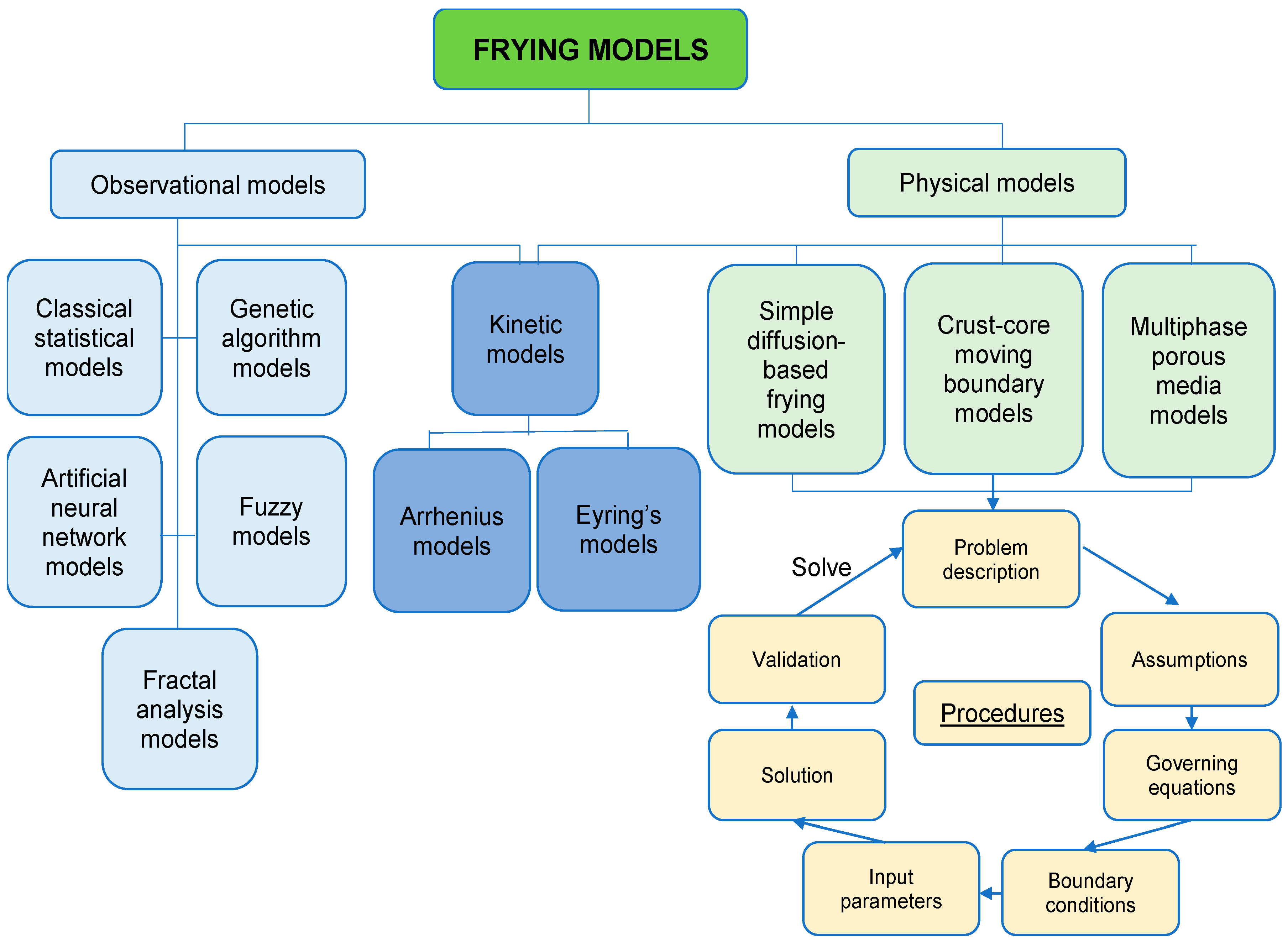
5. The Use of Frying Models to Describe the Frying Process of PEF-Treated Plant Materials
5.1. Physical Models to Describe Heat, Mass and Momentum Transfers
5.2. Observational Models for Quality Prediction of PEF-Treated Fried Foods
5.3. Kinetic Models for Predicting the Rate of Frying Reactions after PEF Treatment
6. Concluding Remarks and Future Directions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Toepfl, S.; Heinz, V.; Knorr, D. Overview of pulsed electric field processing for food. In Emerging Technologies for Food Processing; Sun, D.W., Ed.; Academic Press: London, UK, 2005; pp. 69–97. [Google Scholar]
- Leong, S.Y.; Oey, I. Pulsed electric fields processing of plant-based foods: An overview. In Reference Module in Food Science; Elsevier: Amsterdam, The Netherlands, 2018. [Google Scholar]
- Donsì, F.; Ferrari, G.; Pataro, G. Applications of pulsed electric field treatments for the enhancement of mass transfer from vegetable tissue. Food Eng. Rev. 2010, 2, 109–130. [Google Scholar] [CrossRef]
- Jalté, M.; Lanoisellé, J.L.; Lebovka, N.I.; Vorobiev, E. Freezing of potato tissue pre-treated by pulsed electric fields. Lwt Food Sci. Technol. 2009, 42, 576–580. [Google Scholar] [CrossRef]
- Parniakov, O.; Lebovka, N.I.; Bals, O.; Vorobiev, E. Effect of electric field and osmotic pre-treatments on quality of apples after freezing–thawing. Innov. Food Sci. Emerg. Technol. 2015, 29, 23–30. [Google Scholar] [CrossRef]
- Adler-Nissen, J. Continuous wok-frying of vegetables: Process parameters influencing scale up and product quality. J. Food Eng. 2007, 83, 54–60. [Google Scholar] [CrossRef]
- Da Silva, P.F.; Moreira, R.G. Vacuum frying of high-quality fruit and vegetable-based snacks. Lwt Food Sci. Technol. 2008, 41, 1758–1767. [Google Scholar] [CrossRef]
- Franklin, M.E.E.; Pushpadass, H.A.; Ravindra Menon, R.; Rao, K.J.; Nath, B.S. Modeling the heat and mass transfer during frying of gulab jamun. J. Food Process. Preserv. 2014, 38, 1939–1947. [Google Scholar] [CrossRef]
- Moreira, R.G.; Sun, X.; Chen, Y. Factors affecting oil uptake in tortilla chips in deep-fat frying. J. Food Eng. 1997, 31, 485–498. [Google Scholar] [CrossRef]
- Kita, A. The effect of frying on fat uptake and texture of fried potato products. Eur. J. Lipid Sci. Technol. 2014, 116, 735–740. [Google Scholar] [CrossRef]
- Botero-Uribe, M.; Fitzgerald, M.; Gilbert, R.G.; Midgley, J. Effect of pulsed electrical fields on the structural properties that affect french fry texture during processing. Trends Food Sci. Technol. 2017, 67, 1–11. [Google Scholar] [CrossRef]
- Fauster, T.; Schlossnikl, D.; Rath, F.; Ostermeier, R.; Teufel, F.; Toepfl, S.; Jaeger, H. Impact of pulsed electric field (pef) pretreatment on process performance of industrial french fries production. J. Food Eng. 2018, 235, 16–22. [Google Scholar] [CrossRef]
- Ignat, A.; Manzocco, L.; Brunton, N.P.; Nicoli, M.C.; Lyng, J.G. The effect of pulsed electric field pre-treatments prior to deep-fat frying on quality aspects of potato fries. Innov. Food Sci. Emerg. Technol. 2015, 29, 65–69. [Google Scholar] [CrossRef]
- Liu, C.; Grimi, N.; Lebovka, N.; Vorobiev, E. Impacts of preliminary vacuum drying and pulsed electric field treatment on characteristics of fried potatoes. J. Food Eng. 2020, 276, 109898. [Google Scholar] [CrossRef]
- Eichenlaub, S.; Koh, C. Modeling of food-frying processes. In Modeling Food Processing Operations; Bakalis, S., Knoerzer, K., Fryer, P.J., Eds.; Woodhead Publishing: Cambridge, UK, 2015; pp. 163–184. [Google Scholar]
- Krokida, M.K.; Oreopoulou, V.; Maroulis, Z.B.; Marinos-Kouris, D. Colour changes during deep fat frying. J. Food Eng. 2001, 48, 219–225. [Google Scholar] [CrossRef]
- Bouchon, P.; Aguilera, J.M. Microstructural analysis of frying potatoes. Int. J. Food Sci. Technol. 2001, 36, 669–676. [Google Scholar] [CrossRef]
- Bansal, H.S.; Takhar, P.S.; Maneerote, J. Modeling multiscale transport mechanisms, phase changes and thermomechanics during frying. Food Res. Int. 2014, 62, 709–717. [Google Scholar] [CrossRef]
- Liu, T.; Dodds, E.; Leong, S.Y.; Eyres, G.T.; Burritt, D.J.; Oey, I. Effect of pulsed electric fields on the structure and frying quality of “kumara” sweet potato tubers. Innov. Food Sci. Emerg. Technol. 2017, 39, 197–208. [Google Scholar] [CrossRef]
- Gökmen, V.; Palazoğlu, T.K. Acrylamide formation in foods during thermal processing with a focus on frying. Food Bioprocess Technol. 2008, 1, 35–42. [Google Scholar] [CrossRef]
- Alvis, A.; Vélez, C.; Rada-Mendoza, M.; Villamiel, M.; Villada, H.S. Heat transfer coefficient during deep-fat frying. Food Control 2009, 20, 321–325. [Google Scholar] [CrossRef]
- Erdogdu, F.; Palazoglu, T.K. Food frying process design. In Handbook of Food Process Design; Ahmed, J., Rahman, M.S., Eds.; Blackwell Publishing: Chichester, UK, 2012; pp. 789–810. [Google Scholar]
- Mir-Bel, J.; Oria, R.; Salvador, M.L. Influence of temperature on heat transfer coefficient during moderate vacuum deep-fat frying. J. Food Eng. 2012, 113, 167–176. [Google Scholar] [CrossRef]
- Singh, S.V.; Verma, A.K. Mathematical modeling in foods: Review. In Food Engineering: Emerging Issues, Modeling, and Applications; Meghwal, M., Goyal, M.R., Eds.; Apple Academic Press: New York, NY, USA, 2016; pp. 75–110. [Google Scholar]
- Costa, R.M.; Oliveira, F.A.R.; Delaney, O.; Gekas, V. Analysis of the heat transfer coefficient during potato frying. J. Food Eng. 1999, 39, 293–299. [Google Scholar] [CrossRef]
- Safari, A.; Salamat, R.; Baik, O.-D. A review on heat and mass transfer coefficients during deep-fat frying: Determination methods and influencing factors. J. Food Eng. 2018, 230, 114–123. [Google Scholar] [CrossRef]
- Shynkaryk, M.V.; Lebovka, N.I.; Vorobiev, E. Pulsed electric fields and temperature effects on drying and rehydration of red beetroots. Dry Technol. 2008, 26, 695–704. [Google Scholar] [CrossRef]
- Faridnia, F.; Burritt, D.J.; Bremer, P.J.; Oey, I. Innovative approach to determine the effect of pulsed electric fields on the microstructure of whole potato tubers: Use of cell viability, microscopic images and ionic leakage measurements. Food Res. Int. 2015, 77, 556–564. [Google Scholar] [CrossRef]
- Huang, P.-Y.; Fu, Y.-C. Modelling the kinetics of water loss during deep-fat frying of potato particulates. Czech J. Food Sci. 2014, 32, 585–594. [Google Scholar] [CrossRef]
- Janositz, A.; Noack, A.K.; Knorr, D. Pulsed electric fields and their impact on the diffusion characteristics of potato slices. Lwt Food Sci. Technol. 2011, 44, 1939–1945. [Google Scholar] [CrossRef]
- Farkas, B.E.; Singh, R.P.; Rumsey, T.R. Modeling heat and mass transfer in immersion frying. I, model development. J. Food Eng. 1996, 29, 211–226. [Google Scholar] [CrossRef]
- Datta, A.K. Porous media approaches to studying simultaneous heat and mass transfer in food processes. I: Problem formulations. J. Food Eng. 2007, 80, 80–95. [Google Scholar] [CrossRef]
- Vitrac, O.; Dufour, D.; Trystram, G.; Raoult-Wack, A.-L. Characterization of heat and mass transfer during deep-fat frying and its effect on cassava chip quality. J. Food Eng. 2002, 53, 161–176. [Google Scholar] [CrossRef]
- Gamble, M.H.; Rice, P.; Selman, J.D. Relationship between oil uptake and moisture loss during frying of potato slices from c. V. Record u.K. Tubers. Int. J. Food Sci. Technol. 1987, 22, 233–241. [Google Scholar] [CrossRef]
- Arslan, M.; Xiaobo, Z.; Shi, J.; Rakha, A.; Hu, X.; Zareef, M.; Zhai, X.; Basheer, S. Oil uptake by potato chips or french fries: A review. Eur. J. Lipid Sci. Technol. 2018, 120, 1800058. [Google Scholar] [CrossRef]
- Ziaiifar, A.M.; Achir, N.; Courtois, F.; Trezzani, I.; Trystram, G. Review of mechanisms, conditions, and factors involved in the oil uptake phenomenon during the deep-fat frying process. Int. J. Food Sci. Technol. 2008, 43, 1410–1423. [Google Scholar] [CrossRef]
- Bouchon, P.; Pyle, D.L. Modelling oil absorption during post-frying cooling: I: Model development. Food Bioprod. Process. 2005, 83, 253–260. [Google Scholar] [CrossRef]
- Ufheil, G.; Escher, F. Dynamics of oil uptake during deep-fat frying of potato slices. Lwt Food Sci. Technol. 1996, 29, 640–644. [Google Scholar] [CrossRef]
- Krozel, J.W.; Palazoglu, A.N.; Powell, R.L. Experimental observation of dip-coating phenomena and the prospect of using motion control to minimize fluid retention. Chem. Eng. Sci. 2000, 55, 3639–3650. [Google Scholar] [CrossRef]
- Pinthus, E.J.; Weinberg, P.; Saguy, I.S. Oil uptake in deep fat frying as affected by porosity. J. Food Sci. 1995, 60, 767–769. [Google Scholar] [CrossRef]
- Velez-Ruiz, J.F. Introductory aspects of momentum transfer phenomena. In Transport Phenomena in Food Processing; Welti-Chanes, J., Velez-Ruiz, J.F., Eds.; CRC Press: Boca Raton, FL, USA, 2016; pp. 67–90. [Google Scholar]
- Farid, M.; Kizilel, R. A new approach to the analysis of heat and mass transfer in drying and frying of food products. Chem. Eng. Process. Process Intensif. 2009, 48, 217–223. [Google Scholar] [CrossRef]
- Carrieri, G.; De Bonis, M.V.; Pacella, C.; Pucciarelli, A.; Ruocco, G. Modeling and validation of local acrylamide formation in a model food during frying. J. Food Eng. 2009, 95, 90–98. [Google Scholar] [CrossRef]
- Dellarosa, N.; Ragni, L.; Laghi, L.; Tylewicz, U.; Rocculi, P.; Dalla Rosa, M. Time domain nuclear magnetic resonance to monitor mass transfer mechanisms in apple tissue promoted by osmotic dehydration combined with pulsed electric fields. Innov. Food Sci. Emerg. Technol. 2016, 37, 345–351. [Google Scholar] [CrossRef]
- Zhang, Q.; Saleh, A.S.M.; Chen, J.; Shen, Q. Chemical alterations taken place during deep-fat frying based on certain reaction products: A review. Chem. Phys. Lipids 2012, 165, 662–681. [Google Scholar] [CrossRef]
- Lund, M.N.; Ray, C.A. Control of maillard reactions in foods: Strategies and chemical mechanisms. J. Agric. Food Chem. 2017, 65, 4537–4552. [Google Scholar] [CrossRef]
- Hofmann, T.; Bors, W.; Stettmaier, K. Studies on radical intermediates in the early stage of the nonenzymatic browning reaction of carbohydrates and amino acids. J. Agric. Food Chem. 1999, 47, 379–390. [Google Scholar] [CrossRef] [PubMed]
- Jaeger, H.; Janositz, A.; Knorr, D. The maillard reaction and its control during food processing. The potential of emerging technologies. Pathol. Biol. 2010, 58, 207–213. [Google Scholar] [CrossRef] [PubMed]
- Genovese, J.; Tappi, S.; Luo, W.; Tylewicz, U.; Marzocchi, S.; Marziali, S.; Romani, S.; Ragni, L.; Rocculi, P. Important factors to consider for acrylamide mitigation in potato crisps using pulsed electric fields. Innov. Food Sci. Emerg. Technol. 2019, 55, 18–26. [Google Scholar] [CrossRef]
- Biliaderis, C.G. Structural transitions and related physical properties of starch. In Starch, 3rd ed.; BeMiller, J., Whistler, R., Eds.; Academic Press: San Diego, CA, USA, 2009; pp. 293–372. [Google Scholar]
- Aguilera, J.M.; Cadoche, L.; López, C.; Gutierrez, G. Microstructural changes of potato cells and starch granules heated in oil. Food Res. Int. 2001, 34, 939–947. [Google Scholar] [CrossRef]
- Miranda, M.L.; Aguilera, J.M. Structure and texture properties of fried potato products. Food Rev. Int. 2006, 22, 173–201. [Google Scholar] [CrossRef]
- Ziaiifar, A.M.; Heyd, B.; Courtois, F. Investigation of effective thermal conductivity kinetics of crust and core regions of potato during deep-fat frying using a modified lees method. J. Food Eng. 2009, 95, 373–378. [Google Scholar] [CrossRef]
- Han, Z.; Yu, Q.; Zeng, X.A.; Luo, D.H.; Yu, S.J.; Zhang, B.S.; Chen, X.D. Studies on the microstructure and thermal properties of pulsed electric fields (pef)-treated maize starch. Int. J. Food Eng. 2012, 8, 15. [Google Scholar] [CrossRef]
- Han, Z.; Zeng, X.A.; Yu, S.J.; Zhang, B.S.; Chen, X.D. Effects of pulsed electric fields (pef) treatment on physicochemical properties of potato starch. Innov. Food Sci. Emerg. Technol. 2009, 10, 481–485. [Google Scholar] [CrossRef]
- Zeng, F.; Gao, Q.-Y.; Han, Z.; Zeng, X.-A.; Yu, S.-J. Structural properties and digestibility of pulsed electric field treated waxy rice starch. Food Chem. 2016, 194, 1313–1319. [Google Scholar] [CrossRef]
- Tseng, Y.-C.; Moreira, R.; Sun, X. Total frying-use time effects on soybean-oil deterioration and on tortilla chip quality. Int. J. Food Sci. Technol. 1996, 31, 287–294. [Google Scholar] [CrossRef]
- Gökmen, V.; Açar, Ö.; Serpen, A.; Morales, F. Effect of leavening agents and sugars on the formation of hydroxymethylfurfural in cookies during baking. Eur. Food Res. Technol. 2008, 226, 1031–1037. [Google Scholar] [CrossRef]
- Liu, C.; Grimi, N.; Lebovka, N.; Vorobiev, E. Effects of preliminary treatment by pulsed electric fields and convective air-drying on characteristics of fried potato. Innov. Food Sci. Emerg. Technol. 2018, 47, 454–460. [Google Scholar] [CrossRef]
- Isik, B.; Sahin, S.; Sumnu, G. Pore development, oil and moisture distribution in crust and core regions of potatoes during frying. Food Bioprocess Technol. 2016, 9, 1653–1660. [Google Scholar] [CrossRef]
- Krokida, M.K.; Oreopoulou, V.; Maroulis, Z.B.; Marinos-Kouris, D. Deep fat frying of potato strips—Quality issues. Dry Technol. 2001, 19, 879–935. [Google Scholar] [CrossRef]
- Saguy, I.S.; Dana, D. Integrated approach to deep fat frying: Engineering, nutrition, health and consumer aspects. J. Food Eng. 2003, 56, 143–152. [Google Scholar] [CrossRef]
- Mellema, M. Mechanism and reduction of fat uptake in deep-fat fried foods. Trends Food Sci. Technol. 2003, 14, 364–373. [Google Scholar] [CrossRef]
- Cahayadi, J.; Leong, S.Y.; Oey, I.; Peng, M. Textural effects on perceived satiation and ad libitum intake of potato chips in males and females. Foods 2020, 9, 85. [Google Scholar] [CrossRef]
- Bazhal, M.I.; Lebovka, N.I.; Vorobiev, E. Optimisation of pulsed electric field strength for electroplasmolysis of vegetable tissues. Biosyst. Eng. 2003, 86, 339–345. [Google Scholar] [CrossRef]
- Kawas, M.L.; Moreira, R.G. Characterization of product quality attributes of tortilla chips during the frying process. J. Food Eng. 2001, 47, 97–107. [Google Scholar] [CrossRef]
- Taiwo, K.A.; Baik, O.D. Effects of pre-treatments on the shrinkage and textural properties of fried sweet potatoes. Lwt Food Sci. Technol. 2007, 40, 661–668. [Google Scholar] [CrossRef]
- Witrowa-Rajchert, D.; Wiktor, A.; Sledz, M.; Nowacka, M. Selected emerging technologies to enhance the drying process: A review. Dry Technol. 2014, 32, 1386–1396. [Google Scholar] [CrossRef]
- Pedreschi, F. Frying of potatoes: Physical, chemical, and microstructural changes. Dry Technol. 2012, 30, 707–725. [Google Scholar] [CrossRef]
- Powers, S.J.; Mottram, D.S.; Curtis, A.; Halford, N.G. Acrylamide levels in potato crisps in europe from 2002 to 2016. Food Addit. Contam. Part A 2017, 34, 2085–2100. [Google Scholar] [CrossRef] [PubMed]
- Medeiros Vinci, R.; Mestdagh, F.; De Meulenaer, B. Acrylamide formation in fried potato products – present and future, a critical review on mitigation strategies. Food Chem. 2012, 133, 1138–1154. [Google Scholar] [CrossRef]
- Naous, G.E.-Z.; Merhi, A.; Abboud, M.I.; Mroueh, M.; Taleb, R.I. Carcinogenic and neurotoxic risks of acrylamide consumed through caffeinated beverages among the lebanese population. Chemosphere 2018, 208, 352–357. [Google Scholar] [CrossRef]
- Datta, A.K.; Sablani, S.S. Mathematical modeling techniques in food and bioprocesses: An overview. In Handbook of Food and Bioprocess Modeling Techniques; Sablani, S.S., Datta, A.K., Rahman, M.S., Mujumdar, A.S., Eds.; CRC Press: Boca Raton, FL, USA, 2007; Volume 166, p. 1. [Google Scholar]
- Wang, L.; Sun, D.-W. Recent developments in numerical modelling of heating and cooling processes in the food industry—A review. Trends Food Sci. Technol. 2003, 14, 408–423. [Google Scholar] [CrossRef]
- Rice, P.; Gamble, M.H. Technical note: Modelling moisture loss during potato slice frying. Int. J. Food Sci. Technol. 1989, 24, 183–187. [Google Scholar] [CrossRef]
- Pedreschi, F.; Hernández, P.; Figueroa, C.; Moyano, P. Modeling water loss during frying of potato slices. Int. J. Food Prop. 2005, 8, 289–299. [Google Scholar] [CrossRef]
- Halder, A.; Dhall, A.; Datta, A.K. An improved, easily implementable, porous media based model for deep-fat frying: Part i: Model development and input parameters. Food Bioprod. Process. 2007, 85, 209–219. [Google Scholar] [CrossRef]
- Farid, M.M.; Chen, X.D. The analysis of heat and mass transfer during frying of food using a moving boundary solution procedure. Heat Mass Transf. 1998, 34, 69–77. [Google Scholar] [CrossRef]
- Southern, C.R.; Farid, M.M.; Chen, X.D.; Howard, B.; Eyres, L. Thermal validation of a simple moving boundary model to determine the frying time of a thin potato crisp. Heat Mass Transf. 2000, 36, 407–412. [Google Scholar] [CrossRef]
- Van Koerten, K.N.; Somsen, D.; Boom, R.M.; Schutyser, M.A.I. Modelling water evaporation during frying with an evaporation dependent heat transfer coefficient. J. Food Eng. 2017, 197, 60–67. [Google Scholar] [CrossRef]
- Bakalis, S.; Knoerzer, K.; Fryer, P. Conclusions and future trends in modelling food processing operations. In Modeling Food Processing Operations; Bakalis, S., Knoerzer, K., Fryer, P., Eds.; Elsevier: Cambridge, UK, 2015; pp. 333–337. [Google Scholar]
- Khan, M.I.H.; Joardder, M.U.H.; Kumar, C.; Karim, M.A. Multiphase porous media modelling: A novel approach to predicting food processing performance. Crit. Rev. Food Sci. Nutr. 2018, 58, 528–546. [Google Scholar] [CrossRef]
- Kumar, C.; Joardder, M.U.H.; Farrell, T.W.; Karim, M.A. Multiphase porous media model for intermittent microwave convective drying (imcd) of food. Int. J. Therm. Sci. 2016, 104, 304–314. [Google Scholar] [CrossRef]
- Ni, H.; Datta, A.K. Moisture, oil and energy transport during deep-fat frying of food materials. Food Bioprod. Process. 1999, 77, 194–204. [Google Scholar] [CrossRef]
- Warning, A.; Dhall, A.; Mitrea, D.; Datta, A.K. Porous media based model for deep-fat vacuum frying potato chips. J. Food Eng. 2012, 110, 428–440. [Google Scholar] [CrossRef]
- Halder, A.; Datta, A.K. Surface heat and mass transfer coefficients for multiphase porous media transport models with rapid evaporation. Food Bioprod. Process. 2012, 90, 475–490. [Google Scholar] [CrossRef]
- Hsu, H.-T.; Chen, M.-J.; Tseng, T.-P.; Cheng, L.-H.; Huang, L.-J.; Yeh, T.-S. Kinetics for the distribution of acrylamide in french fries, fried oil and vapour during frying of potatoes. Food Chem. 2016, 211, 669–678. [Google Scholar] [CrossRef]
- Farid, M.; Butcher, S. A generalized correlation for heat and mass transfer in freezing, drying, frying, and freeze drying. Dry Technol. 2003, 21, 231–247. [Google Scholar] [CrossRef]
- Naghavi, E.-A.; Dehghannya, J.; Ghanbarzadeh, B. 3d computational simulation for the prediction of coupled momentum, heat and mass transfer during deep-fat frying of potato strips coated with different concentrations of alginate. J. Food Eng. 2018, 235, 64–78. [Google Scholar] [CrossRef]
- Van Koerten, K.; Schutyser, M.; Somsen, D.; Boom, R. A pore inactivation model for describing oil uptake of french fries during pre-frying. J. Food Eng. 2015, 146, 92–98. [Google Scholar] [CrossRef]
- Mohebbi, M.; Fathi, M.; Shahidi, F. Genetic algorithm–artificial neural network modeling of moisture and oil content of pretreated fried mushroom. Food Bioprocess Technol. 2011, 4, 603–609. [Google Scholar] [CrossRef]
- Sobowale, S.S.; Adebiyi, J.A.; Adebo, O.A. Optimization of blanching and frying conditions of deep-fat fried bonga fish (Ethmalosa fimbriata). J. Food Process Eng. 2017, 40, e12551. [Google Scholar] [CrossRef]
- Rywotycki, R. Food frying process control system. J. Food Eng. 2003, 59, 339–342. [Google Scholar] [CrossRef]
- Ahmed, J.; Dolan, K.; Mishra, D. Chemical reaction kinetics pertaining to foods. In Handbook of Food Process Design; Jasim Ahmed, J., Rahman, M.S., Eds.; Blackwell Publishing: Chichester, UK, 2012; pp. 113–166. [Google Scholar]
- Balagiannis, D.P.; Mottram, D.S.; Higley, J.; Smith, G.; Wedzicha, B.L.; Parker, J.K. Kinetic modelling of acrylamide formation during the finish-frying of french fries with variable maltose content. Food Chem. 2019, 284, 236–244. [Google Scholar] [CrossRef]
- Van Boekel, M.A.J.S. Kinetic modeling of food quality: A critical review. Compr. Rev. Food Sci. Food Saf. 2008, 7, 144–158. [Google Scholar] [CrossRef]
- Baik, O.; Mittal, G. Kinetics of tofu color changes during deep-fat frying. Lwt Food Sci. Technol. 2003, 36, 43–48. [Google Scholar] [CrossRef]
- Moyano, P.C.; Ríoseco, V.K.; González, P.A. Kinetics of crust color changes during deep-fat frying of impregnated french fries. J. Food Eng. 2002, 54, 249–255. [Google Scholar] [CrossRef]
- Moyano, P.C.; Zúñiga, R.N. Enthalpy–entropy compensation for browning of potato strips during deep-fat frying. J. Food Eng. 2004, 63, 57–62. [Google Scholar] [CrossRef]
| Plant | Experimental Setup | PEF Parameters | Frying Parameters | Quality Parameters | Key Findings | References |
|---|---|---|---|---|---|---|
| Potato | PEF treatment was applied on peeled potatoes in cube form with dimension of 2 × 2 × 2 cm, followed by frying | E = 0.75, 2.50 kV/cm, N = 810, 9000 pulses, W = 18.9 kJ/kg, t = N/A, f = N/A, τ = N/A | Temperature = 190 °C, Frying time = 1 min | Weight, Moisture, Drip loss, Colour, Texture, and Oil content (fried) | Before frying: Moisture content=, Texture: peak force↓, Colour: lightness↓, a* ↑ After frying: Moisture content=, Texture: peak force=, Colour: lightness↑, a* ↓, Oil content ↓ | [13] |
| Potato | Whole unpeeled potatoes were PEF-treated and then cut into 9 mm straight fries, followed by blanching, hot-air drying and finally par-frying | E = 1.0 kV/cm, N = N/A,W = 0.05, 0.2, 0.8, 1.0 kJ/kg, t = N/A, f = 1 Hz, τ = 75 μs | N/A | Cell disintegration index, Cutting force, Peeling loss, Feathering, Starch loss during cutting, and Fat uptake (par-frying) | Before frying:Cell disintegration index↑, Cutting force↓, Peeling loss= After cutting: Feathering↓, Starch loss↓ After frying: Fat content↓ | [12] |
| Potato | Whole peeled potatoes were PEF-treated and then cut into 10 × 10 × 40 mm strips, followed by drying (100 °C for 10 min) and finally frying | E = 1.5, 2.5, 5 kV/cm, N = N/A, W = N/A, t = N/A, f = 2 Hz, τ = 100, 400 μs | Temperature = 190 °C Frying time = 3 min | Microscopic visualisation, Sugar content (sucrose, D-glucose, D- fructose) (before frying), Salt uptake (before frying), Drying efficiency (before frying), and Fat uptake (fried) | Before frying: Cell wall is affected, Sugar content↓, Conductivity and uptake of sodium chloride↑ After drying: Water content↓ After frying: Fat uptake↓ | [30] |
| Potato | PEF treatment was applied on peeled potatoes in slice form with thickness of 1.5 mm, followed by frying | E = 1.5 kV/cm, N = 1000 pulses, W = N/A, t = 10 ms, f = 100 Hz, τ = 10 μs | Temperature = 175 °C Frying time = 3 min | Colour, Texture, and Acrylamide | After frying: Colour: hue angle↑, Texture: firmness↓, crispness↓, Acrylamide↓ | [49] |
| Potato | PEF treatment was applied on potatoes in disk form with 25 mm diameter and 2.5 mm thickness, followed by vacuum drying (40–70 °C, up to 7200 s) and finally frying at low temperature for long time | E = 0.6 kV/cm, N = 10, 100 pulses, W = N/A, t = 10 ms, f = N/A, τ = 100 μs | Temperature = 130 °C * Frying time = Kinetic approach, up to 1400 s | Texture, Moisture content, and Oil uptake | After frying: Texture: firmness↑, peak force↑, Oil uptake↓ | [14] |
| Potato | PEF treatment was applied on potatoes in disk form with and after cutting into disk form with 25 mm diameter and 2.5 mm thickness, followed by convective air drying (50 °C, up to 2000 s) and finally frying | E = 0.6 kV/cm, N = 10, 100 pulses, W = N/A, t = 10 ms, f = N/A, τ = 100 μs | Temperature = 130 °C * Frying time = Kinetic approach, up to 2000 s | Water content, Oil uptake, and Texture | After frying: Effective moisture diffusivity↑, Water content↓, Oil uptake↓, Texture: peak force↑ | [59] |
| Plant | Product Dimensions and Pretreatment | Frying Parameters | Model Type | Key Findings | References |
|---|---|---|---|---|---|
| Potato | Cylinder: 0.006 m diameter × 0.006 m length | T = 160, 190 and 220 °C; t = Kinetic approach, up to 240 s | A fractional conversion first-order kinetic and Arrhenius model | A two-stage rate process (the rapid and slow process) was found to describe the mechanisms of the water loss during frying. | [29] |
| Potato | Strips: 20 mm × 7 mm × 7 mm Preheating: 70 °C for 2 h | T = 135, 160, 175 and 190 °C; t = Kinetic approach, up to 12 min | An exponential decay and Arrhenius model | A high level of acrylamide was volatilised during frying, where acrylamide was detected in French fries, frying oil and air. | [87] |
| Potato | Slices: 2 mm thickness, 50 mm diameter | T = 170, 180 and 190 °C; t = 200–300 s; Location in the fryer: centre, 0.3 mm off-centre and 0.75 mm off-centre | A simple moving boundary model (Note: The core temperature was defined as a value of a region rather than a point) | The model was able to predict the temperature at different locations of the potato slice. The model considered the effect of varying convection heat transfer coefficients of oil. | [79] |
| Potato | 2,3, 25.4 mm thick chip | T = 170, 180 °C; t = 50–800 s | A one-dimensional core-crust moving boundary model | The model was able to describe deep fat frying, air-drying, freeze drying, steam drying and spray drying. | [42] |
| Potato | 2, 3, 4, 5, 6, 25.4 mm thick potato chips | T = 180 °C; t = 240 and 540 s | A one-dimensional moving boundary model | The frying process of multidimensional geometry for other food-types can be described with this model. The numerical finite difference method required the computation to start with the presence of two regions (crust and core). This was achieved by approximating the time required to form a thin layer of crust. | [78] |
| Potato | 12 mm thick potato slices with an average diameter of 50 mm | T = 158 °C; t = 3300 s | A one-dimensional core-crust moving boundary model based on quasi-steady state analysis | The model showed the existence of a moving interface that recedes towards the core of the samples as time progresses during frying, freeze-drying and air-drying. | [88] |
| Potato | Uniform cylinders of three different diameters: 8.5, 10.5, and 14 mm | T = 140, 160, and 180 °C; t = 20, 40, 60, 120, and 180 s | A cylindrical crust-core frying model | A Nusselt relationship was applied for cylindrical bodies to describe the release of vapour bubbles during the frying process. The characteristic length and velocity for the Reynolds number were taken as the average diameter of the vapour bubbles and vapour bubble release frequency multiplied with the bubble diameter, respectively. | [80] |
| Potato | Slices: thickness 1.5 mm | Frying pressures and T: 1.33 kPa (118, 125, 140 °C), 9.89 kPa (118, 132, 144 °C), 16.7 kPa (118, 132, 144 ℃), and 101 kPa (150, 165, 180 °C) | A multiphase porous media model | Core pressure of potato slices reached approximately 40 kPa higher than the surface. Acrylamide formation was modelled on chip temperature rather than oil temperature. | [85] |
| Potato | Strips: 1.2 cm × 1.2 cm × 4 cm Coating with alginate gum: 0, 1, 1.5, and 2% | T = 170 °C; t = 590, 180, 270, and 360 s; | A 3D model | Effects of 0–2% alginate and four locations of potatoes on variables were studied. Moisture content and oil uptake in the coated samples were found to be significantly reduced. | [89] |
| Potato | Cylinders: 50 mm length, 8.5, 10.5, 14 mm diameter Soaked for 10 min in tap water | T = 140, 160, and 180 °C; t = 20, 40, 60, 120, and 180 s. | A pore inactivation model | The model was able to describe the overall trend of oil uptake compared to linear model. The model assumed that the moisture vapour from the porous crust inhibits oil migration. | [90] |
| Mushroom | Disk-shaped slices of 8 mm in thickness and 30 mm in diameter Osmotic dehydration (5 and 10%) and gum coating (2%) | T = 150, 170, and 180 °C; t = 0.5, 1, 2, 3, 4 min | A genetic algorithm–artificial neural network model (GA–ANN) | GA–ANN model was able to provide an accurate prediction for moisture and oil contents of fried mushroom. | [91] |
| Model Types | Mass Transfer | Heat Transfer | Momentum Transfer |
|---|---|---|---|
| Diffusion-based frying model | Fick’s law of diffusion | Arrhenius equation | N/A |
| Crust-core moving boundary model | 1. Core region: Mass balance equation; Fick’s law of diffusion 2. Crust region: Mass balance equation; Idea gas law; Clausius–Clapeon equation | 1. Initial heating period in both core and crust regions: Fourier’s equation 2. After crust formation: Core region: Fourier’s equation Crust region: modified Fourier’s equation including heat exchange and water vapour | N/A |
| Multiphase porous media model | 1. Liquid: Mass balance equation; capillarity pressure (Kelvin’s law/ Flory–Rehner theory/Darcy’s law); gas pressure due to evaporation or gas release (Darcy’s law) 2. Gas (Vapour or air): Mass balance equation; molecular diffusion (Fick’s law); gas pressure (Darcy’s law) 3. Phase change of water: Hertz–Knudsen equation/Volume fraction continuity | Solid, liquid and gas: Energy balance equation (Fourier’s law of heat conduction) | Liquid and gas: Conservation of momentum; Darcy’s law (low permeability); Navier–Stokes equation; continuity equation |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xu, Z.; Leong, S.Y.; Farid, M.; Silcock, P.; Bremer, P.; Oey, I. Understanding the Frying Process of Plant-Based Foods Pretreated with Pulsed Electric Fields Using Frying Models. Foods 2020, 9, 949. https://doi.org/10.3390/foods9070949
Xu Z, Leong SY, Farid M, Silcock P, Bremer P, Oey I. Understanding the Frying Process of Plant-Based Foods Pretreated with Pulsed Electric Fields Using Frying Models. Foods. 2020; 9(7):949. https://doi.org/10.3390/foods9070949
Chicago/Turabian StyleXu, Zihan, Sze Ying Leong, Mohammed Farid, Patrick Silcock, Phil Bremer, and Indrawati Oey. 2020. "Understanding the Frying Process of Plant-Based Foods Pretreated with Pulsed Electric Fields Using Frying Models" Foods 9, no. 7: 949. https://doi.org/10.3390/foods9070949
APA StyleXu, Z., Leong, S. Y., Farid, M., Silcock, P., Bremer, P., & Oey, I. (2020). Understanding the Frying Process of Plant-Based Foods Pretreated with Pulsed Electric Fields Using Frying Models. Foods, 9(7), 949. https://doi.org/10.3390/foods9070949









