1. Introduction
Design of experiments (DOE) [
1,
2] is a widely used discipline applied in a variety of areas, including engineering [
3,
4,
5], social sciences [
6] and natural sciences [
7,
8,
9]. Design of experiments is a powerful statistical methodology in its own right, with a number of software applications readily available to aid researchers in both designing and globally optimizing multi-parametric experiments to achieve the best results through analysis and interpretation. In this context, combining software-based applications with a researcher’s experience and scientific intuition is a powerfully growing trend that typically results in significant savings in time and materials. The design of experiments methodology includes formal, planned experimentation with the goal of optimizing a set of reaction parameters that may disclose synergism between reaction parameters. The optimization typically comprises six main steps: (1) selection of variables and defining their range of variation; (2) selection of responses; (3) experimental design selection; (4) performing the designed experiments in random order; (5) determination of coefficients in a mathematical model; and (6) predicting the response and evaluating the model relevance. In this context, we designed a series of experiments to optimize globally the reaction conditions for maximizing the yield of covalently functionalized tungsten disulfide inorganic fullerene-like nanotubes (WS
2 INTs). This specific functionalization reaction comprises a polycarboxylation technique [
10,
11], developed recently in our laboratories, that uses a modified highly electrophilic Vilsmeier–Haack reagent [
12].
It is well known that classical Vilsmeier–Haack reactions use DMF (secondary
N-formyl amine) and POCl
3/SOCl
2 to effect the formylation of a wide range of electrophilic substrates via intermediate electrophilic iminium salts of Type A (
Scheme 1a). Such Vilsmeier–Haack reactions have been studied extensively and found to be quite versatile, leading to a number of oxygen and nitrogen heterocycles [
13,
14,
15,
16,
17,
18], as well. For example, a modified Vilsmeier–Haack reagent that uses ethyl chloroformate in place of POCl
3 was found useful when reacted with active methylene compounds [
19]. Analogously, we have discovered that by using a mixture containing DMF (a 2nd
N-CHO amine) and O-alkylating 2-bromoacetic acid with catalysis by Ag(I)OAc, WS
2 nanotubes may be polycarboxylated readily and quite effectively according to the mechanism described in
Scheme 1c. Indeed, DMF removal or replacement with other polar, non-protic materials/solvents (e.g
., 1,4-dioxane, DME,
etc.) leads to unsuccessful polycarboxylation. This strict requirement of DMF (2nd
N-CHO amine) as an essential component of the reaction mixture led us to propose and detail a corresponding Vilsmeier–Haack-like reaction mechanism, displayed in
Scheme 1c.
Interestingly, silver acetate (Ag(I)OAc) was also included as an essential reaction factor due to its well-known ability to chemically trap halogens and, thereby, assist in halogen (Br) abstraction. Depicted in
Scheme 1b is the reaction of Ag(I)OAc with the Br atom of bromoacetic acid, leading to the formation of the Vilsmeier–Haack complex of Type B1 with subsequent precipitation of Ag(I)Br.
Because several reaction factors are involved in the polycarboxylation reaction mentioned above, we selected a DOE methodology as the most economical means of optimizing the reaction factors to produce the highest yields of polycarboxylation. In this context, the level of polycarboxylation was determined indirectly by reacting the functionalized INTs with an excess of 1,3-diaminopropane, resulting in a terminal primary amine that was quantified by the Kaiser test [
20]. Due to a one-to-one reaction between the diamine and carboxylic acid, the amount of terminal amine is equal to the amount of carboxylic acid.
Scheme 1.
(a) Generalized Vilsmeier–Haack generation of electrophilic iminium salts; (b) Vilsmeier–Haack generation of DMF-based electrophilic iminium salt complex with bromophilic Ag(I) assistance in halide abstraction; (c) sulfur-mediated nucleophilic addition of the iminium salt complex to the outermost sulfur atoms of WS2 INTs, producing the corresponding polycarboxylated INTs.
Scheme 1.
(a) Generalized Vilsmeier–Haack generation of electrophilic iminium salts; (b) Vilsmeier–Haack generation of DMF-based electrophilic iminium salt complex with bromophilic Ag(I) assistance in halide abstraction; (c) sulfur-mediated nucleophilic addition of the iminium salt complex to the outermost sulfur atoms of WS2 INTs, producing the corresponding polycarboxylated INTs.
In addition to the Kaiser test, further confirmation of the successful functionalization of the WS2 INTs was obtained by a combination of FT-IR, TGA and zeta potential analyses (see the Supplementary Information).
Thus far, we have investigated this unique functionalization method only for the polycarboxylation of WS2 nanotubes. However, we strongly believe it may prove applicable for similar polycarboxylation of other layered dichalcogenide nanomaterials (MS(Se)2, M = Mo, Sn).
2. Results and Discussion
Because the level of surface functionalization of the WS2 INTs by the Vilsmeier–Haack-like reaction is of critical importance in determining the coordination capability of the corresponding optimally surface-engineered WS2 INTs, a statistically designed experiment was implemented using the design of experiment (DOE) methodology. The goal of the design was to disclose an optimal set of reaction conditions that would result in the maximized level of surface functionalization of the WS2 INTs. This DOE study enables varying more than one factor/reaction condition at a time for process optimization, even when several influential factors are involved. In addition, this multi-factor approach not only enables running fewer experiments, but enables the study of the interactions between the reaction factors and how these interactions influence the final result. These advantages are unavailable with the more commonly used one factor at a time (OFAT) optimization methods.
Based on our current process knowledge, four factors (reaction parameters) likely to affect significantly the functionalization process were identified: DMF, silver acetate (Ag(I)OAc), WS
2 INTs and 2-bromoacetic acid (BrCH
2COOH). In addition, two other factors, time and temperature, were included in the design. The experimental design and subsequent analysis of the significance of each factor and associated interactive effects were performed using a two-level factorial statistical design in conjunction with statistical software (MiniTab
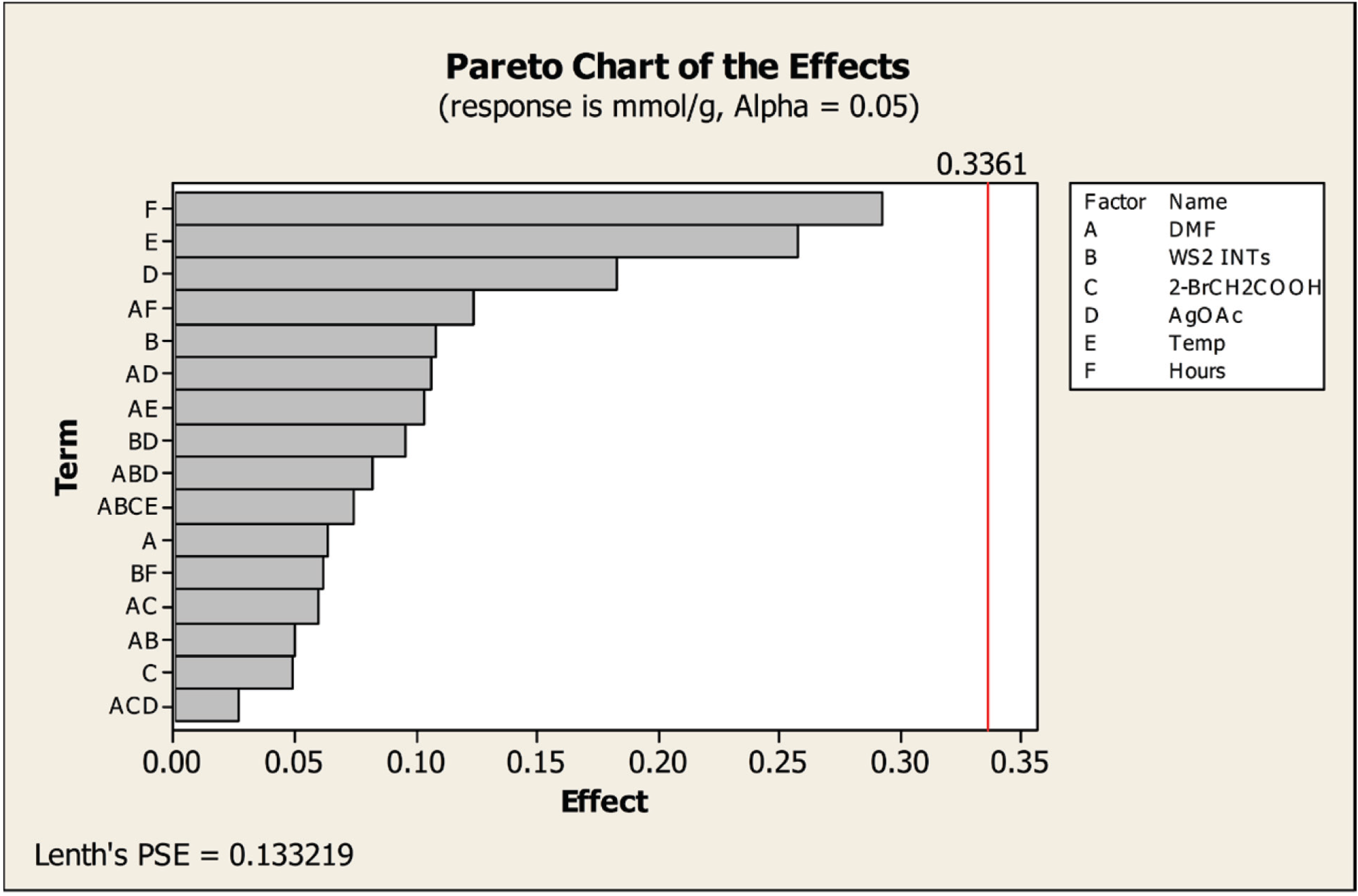
® 16) based on quadratic programming. Pareto analysis (
Figure 1) was used to disclose which reaction parameters are process active. Using the software default value for α of 0.05, all absolute magnitude effect values fall below the software error-calculated reference line for statistical significance (vertical red line, value 0.3361), indicating that none of the factors nor interactive effects are statistically significant. However, the software analysis indicates that time, temperature and the amount of silver acetate are the three most important contributors to obtaining maximum functionalization, and these warrant attention in the subsequent analyses. From the prediction model that was obtained, an optimal functionalization yield was obtained (1.21 mmol COOH groups/g) at an optimal temperature of 120 °C and a reaction time of 54 h.
Figure 1.
Pareto chart of the effects of the factors on the amount of functionalization obtained. Values to the left of the reference line (vertical red line) are not statistically significant.
Figure 1.
Pareto chart of the effects of the factors on the amount of functionalization obtained. Values to the left of the reference line (vertical red line) are not statistically significant.
Figure 2 displays the effect of the two factors, temperature and time, on the amount of functionalization obtained per gram of INTs. Clearly, a higher temperature (120 °C) for a longer time (54 h) results in the highest level of functionalization.
To assess the effect of each of the other factors together with temperature on the amount of functionalization obtained when the reaction is conducted for 54 h, a series of contour plots was made. For several of the factors, two combinations of temperature and factor (WS2 INTs, BrCH2COOH, DMF or AgOAc) concentration produce the highest amount of carboxylation. When a higher temperature is used, the factor under evaluation does not affect the yield of carboxylation. However, in a lower temperature range, the highest amount of carboxylation is achieved only when the factor concentration is within a specific range.
Figure 2.
Amount (mmol) of carboxylic acid functional groups found per gram of WS2 INTs. Results are grouped by temperature and time. A higher temperature and a longer time increase the level of functionalization.
Figure 2.
Amount (mmol) of carboxylic acid functional groups found per gram of WS2 INTs. Results are grouped by temperature and time. A higher temperature and a longer time increase the level of functionalization.
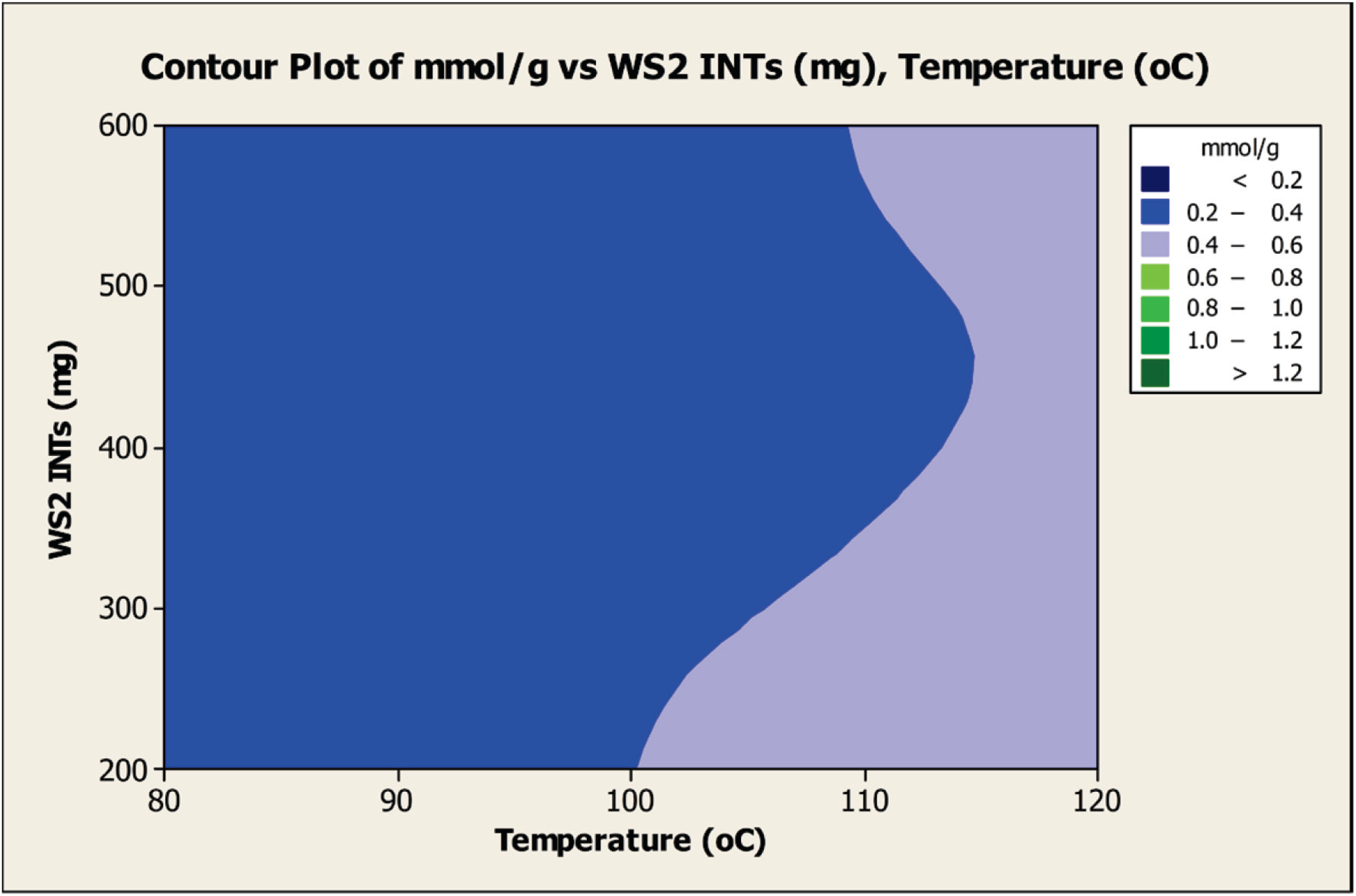
Figure 3 displays the effect of the dual factors, temperature (°C) and quantity (mg) of INTs, on the reaction mixture.
Figure 3.
Contour plot of the amount (mmol) of carboxylation per gram of INTs obtained as the dual factors, temperature and amount of INTs in the reaction mixture, are varied at a constant reaction time of 54 h.
Figure 3.
Contour plot of the amount (mmol) of carboxylation per gram of INTs obtained as the dual factors, temperature and amount of INTs in the reaction mixture, are varied at a constant reaction time of 54 h.
Two areas are revealed in which maximum carboxylation (0.4–0.6 mmol/g) can be obtained at a 54-h reaction time; by using 200–300 mg of INTs in the mixture with the temperature maintained in the range of 105–120 °C and using 200–600 mg INTs with a temperature range of 115–120 °C. Interestingly, a slightly lower amount of carboxylation (0.2–0.4 mmol/g) can be obtained using an INT level in the range of 200–600 mg with a decrease of temperature in a range of 80–100 °C. This suggests that it may be possible to customize the yield of carboxylation by tuning the amount of INTs in the reaction mixture together with the temperature.
Figure 4 displays the effect of the dual factors, temperature (°C) and quantity (mg, mmol) of 2-bromoacetic acid in the reaction mixture. Two areas are revealed in which maximum carboxylation (0.4–0.6 mmol/g) can be obtained at a 54-h reaction time; by using 800–1100 mg (5.76–7.92 mmol) of 2-bromoacetic acid in the mixture with temperature maintained in a range of 105–120 °C and using 800–2250 mg (5.76–16.2 mmol) of 2-bromoacetic acid with a temperature range of 115–120 °C. As mentioned above, with the INT concentration, by tuning the amount of 2-bromoacetic acid (800–2250 mg, 5.76–16.2 mmol) and the temperature (80–100 °C), it may be possible to produce a controlled level of carboxylation within the range of 0.2–0.4 mmol/g.
Figure 4.
Contour plot of the amount (mmol) of carboxylation per gram of INTs obtained as the dual factors, temperature and amount of 2-bromoacetic acid in the reaction mixture, are varied at a constant reaction time of 54 h.
Figure 4.
Contour plot of the amount (mmol) of carboxylation per gram of INTs obtained as the dual factors, temperature and amount of 2-bromoacetic acid in the reaction mixture, are varied at a constant reaction time of 54 h.
Figure 5 displays the effect of the dual factors, temperature (°C) and quantity (mL) of DMF in the reaction mixture. Two areas are revealed in which maximum carboxylation (0.4–0.6 mmol/g) can be obtained at a 54-h reaction time; by using 2–2.5 mL of DMF in the mixture with temperature maintained in a range of 110–120 °C, and using 2–6 mL DMF with a temperature range of 115–120 °C. As mentioned above, for both INT and the 2-bromoacetic acid concentration, by tuning the amount of DMF (2–6 mL) and temperature (80–100 °C), it may be possible to produce a controlled level of carboxylation within the range of 0.2–0.4 mmol/g.
Figure 6 displays the effect of the dual factors, temperature (°C) and quantity (mg) of AgOAc in the reaction mixture. Three zones of carboxylation yield are clearly identified, indicating that several sets of AgOAc concentration and temperature can be used to achieve the selected level of carboxylation. For example, maximum carboxylation (0.6–0.8 mmol/g) can be obtained at a 54-h reaction time by using AgOAc in a range of 55–60 mg (0.33–0.36 mmol) in the mixture with temperature maintained in a range of 115–120 °C. The following combinations of AgOAc/temperature produce a lower carboxylation level of 0.4–0.6 mmol/g: 60 mg (0.36 mmol)/100–110 °C; 50–60 mg (0.30–0.36 mmol)/105–110 °C; 40–50 mg (0.24–0.30 mmol)/115–120 °C. In addition, a carboxylation level of 0.2–0.4 can be achieved over the entire temperature range of 80–120 °C if the AgOAc level is maintained within 20–35 mg (0.12–0.21 mmol). Similarly, this lower carboxylation level is achievable over the entire range of 20–60 mg (0.12–0.36 mmol) AgOAc by maintaining a lower temperature range of 80–100 °C.
Figure 5.
Contour plot of the amount (mmol) of carboxylation per gram of INTs obtained as the dual factors, temperature and amount of DMF in the reaction mixture, are varied at a constant reaction time of 54 h.
Figure 5.
Contour plot of the amount (mmol) of carboxylation per gram of INTs obtained as the dual factors, temperature and amount of DMF in the reaction mixture, are varied at a constant reaction time of 54 h.
Figure 6.
Contour plot of the amount (mmol) of carboxylation per gram of INTs obtained as the dual factors, temperature and amount of AgOAc in the reaction mixture, are varied at a constant reaction time of 54 h.
Figure 6.
Contour plot of the amount (mmol) of carboxylation per gram of INTs obtained as the dual factors, temperature and amount of AgOAc in the reaction mixture, are varied at a constant reaction time of 54 h.
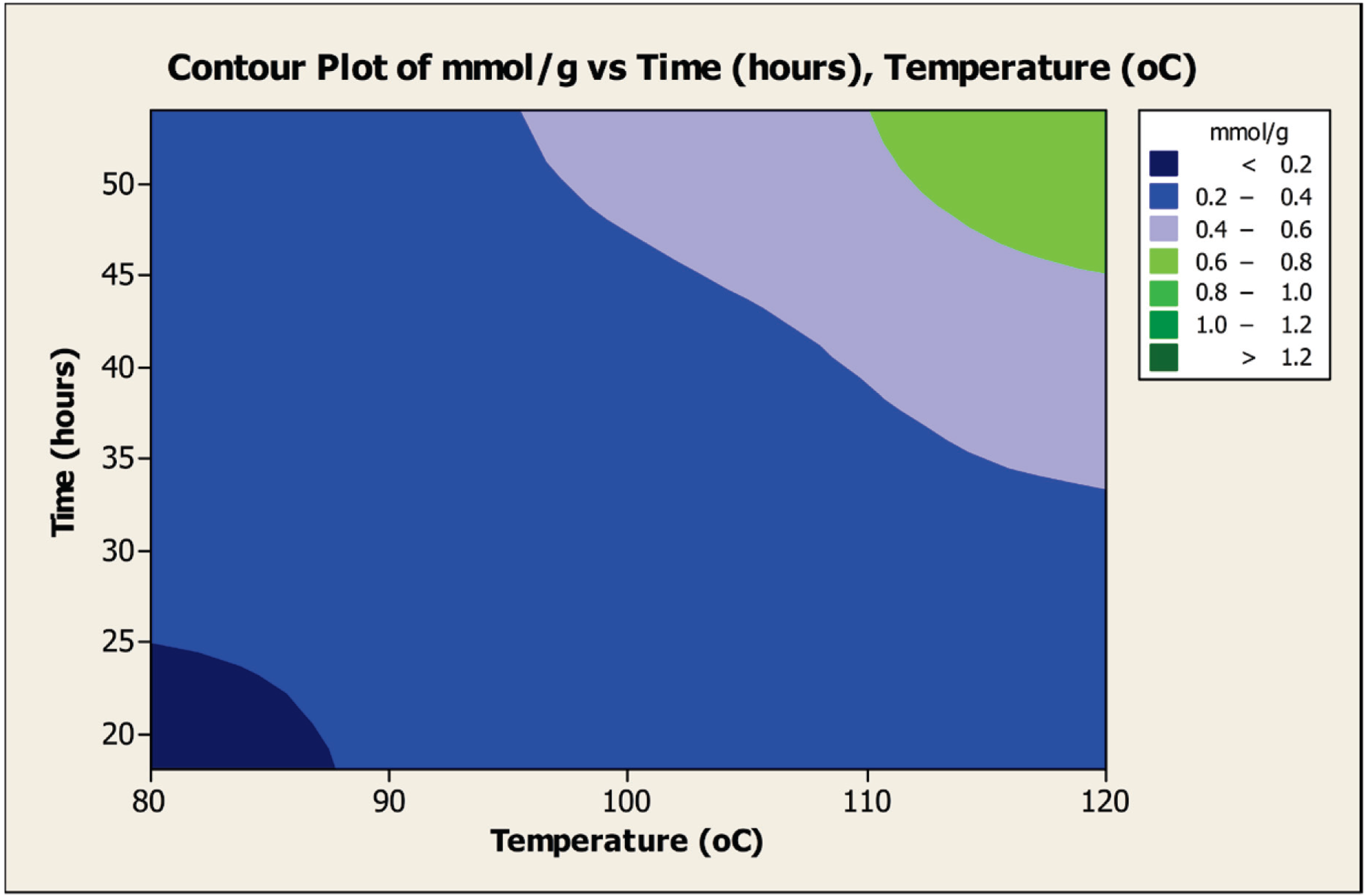
Figure 7 displays the effect on carboxylation yield with the dual factors, temperature (°C) and time (h). For this factor combination, four zones of carboxylation yield are identified. As noted with the factors temperature and AgOAc, several sets of the two factors can be used to achieve a selected level of carboxylation. As examples for achieving the highest carboxylation level (0.6–0.8 mmol/g), a time range of 45–60 h at a temperature of 120 °C or a temperature in a range of 110–120 °C for 54 h can be used. Similar analyses can be done to find ranges of temperature and time to produce the lower carboxylation levels displayed in
Figure 7.
Figure 7.
Contour plot of the amount (mmol) of carboxylation per gram of INTs obtained as the dual factors, temperature (°C) and time (h), are varied.
Figure 7.
Contour plot of the amount (mmol) of carboxylation per gram of INTs obtained as the dual factors, temperature (°C) and time (h), are varied.
Analysis of the contour plots for a 54-h reaction time (
Figure 3,
Figure 4,
Figure 5,
Figure 6 and
Figure 7) indicates that only the temperature range of 115–120 °C is common to all of the factors to produce the maximum degree of carboxylation. Using this temperature range, the levels of each factor to achieve maximum carboxylation are as follows. INTs 200–600 mg; 2-bromoacetic acid 800–2250 mg (5.76–16.2 mmol); DMF 2–6 mL and AgOAc 55–60 mg (0.33–0.36 mmol).
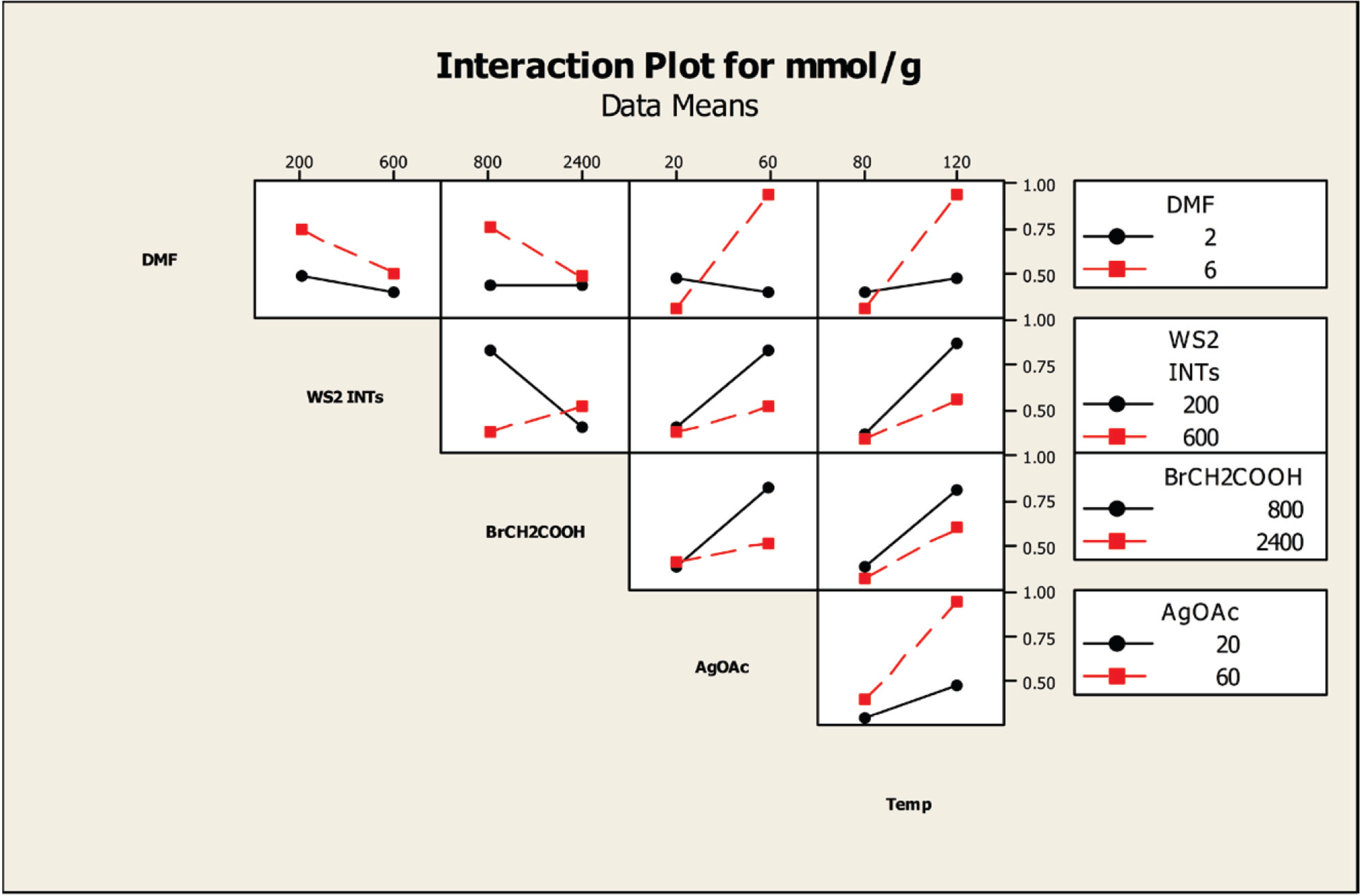
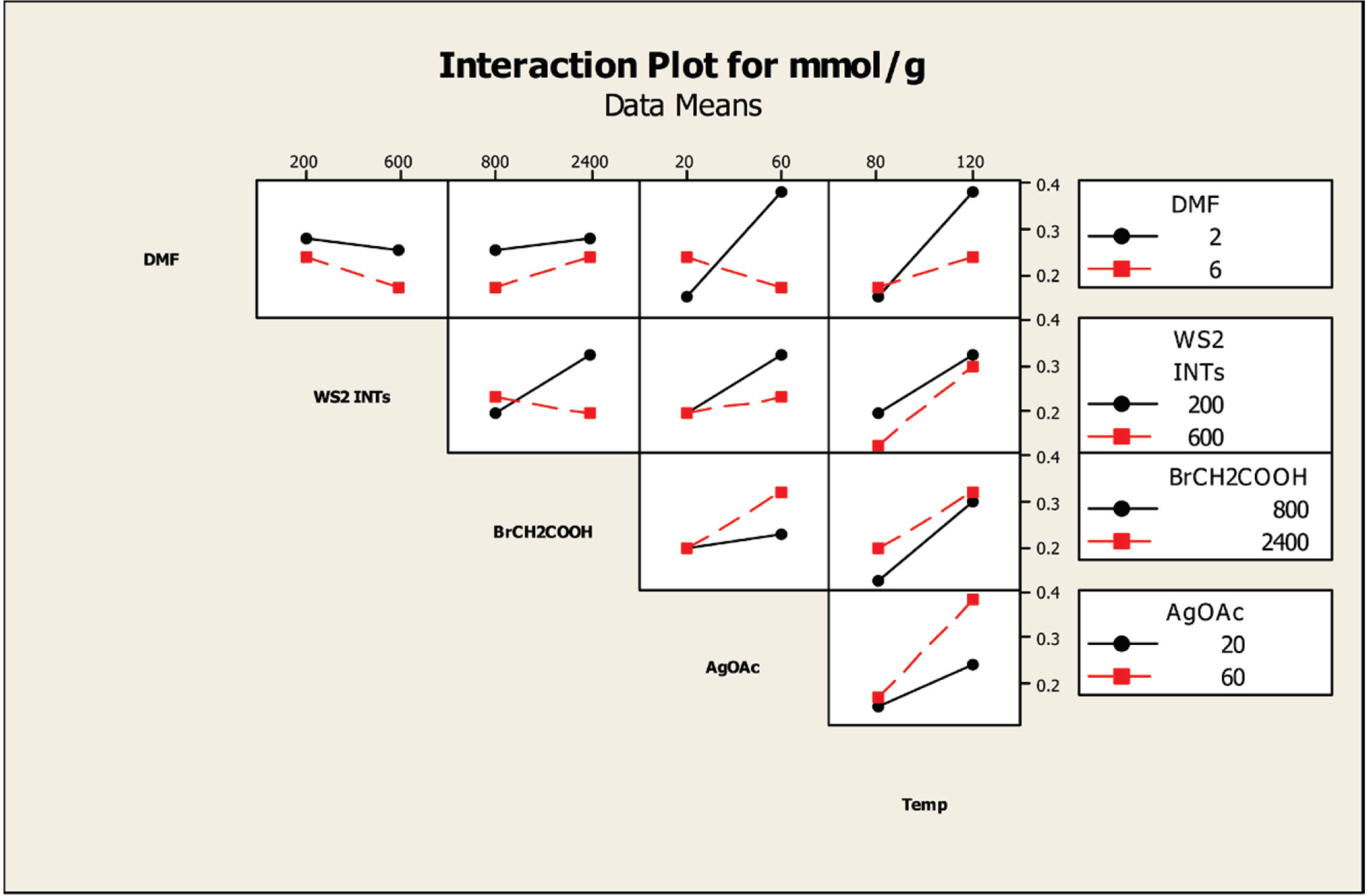
Figure 8.
Interaction plot of the effects of the factors on the degree of carboxylation at a reaction time of 54 h.
Figure 8.
Interaction plot of the effects of the factors on the degree of carboxylation at a reaction time of 54 h.
The influence of the factors on the degree of carboxylation was examined by interaction plots. Separate interaction plots were made for reaction times of 18 h and 54 h.
Figure 8 displays the interaction plot for a 54-h reaction time. The temperature column indicates that a reaction temperature of 80 °C results in not only a much lower amount of carboxylation than a reaction temperature of 120 °C, but, at the levels tested, changing the concentrations of the four factors, DMF, INT, 2-bromoacetic acid and AgOAc, has little effect on the degree of carboxylation. In contrast, at 120 °C, higher concentrations of DMF (6 mL) and AgOAc (60 mg, 0.36 mmol) with lower concentrations of INTs (200 mg) and 2-bromoacetic acid (800 mg, 5.76 mmol) produce higher degrees of carboxylation. Note that this analysis is in agreement with the findings of the contour plots (
Figure 3,
Figure 4,
Figure 5,
Figure 6 and
Figure 7).
The AgOAc column shows similar effects, with the lower concentration of AgOAc producing a lower amount of carboxylation than a higher concentration, and changing the concentration of the other factors has little effect on the degree of carboxylation. When a higher concentration of AgOAc is used, the degree of carboxylation is increased with a higher DMF concentration, but with lower concentrations of INTs and 2-bromoacetic acid. The 2-bromoacetic acid column indicates that a higher degree of carboxylation is obtained using a lower concentration of acid along with a lower concentration of INTs and a higher concentration of DMF. Similarly, the WS2 INT column indicates that using a lower concentration of INTs and a higher concentration of DMF produces a higher degree of carboxylation.
Figure 9 displays the interaction plot for an 18-h reaction time.
Figure 9.
Interaction plot of the effects of the factors on the degree of carboxylation at a reaction time of 18 h.
Figure 9.
Interaction plot of the effects of the factors on the degree of carboxylation at a reaction time of 18 h.
The temperature column indicates that a reaction time of 18 h with optimized factors of 120 °C, 2 mL DMF, 60 mg (0.36 mmol) AgOAc, 200–600 mg INTs, and 800–2400 mg (5.76–17.3 mmol) 2-bromoacetic acid gives a maximum amount of carboxylation that is less than half the amount that is obtained with optimized factors when the reaction time is 54 h.