Magnetostructural D-Correlations and Their Impact on Single-Molecule Magnetism

Abstract

Functional dependence of the axial zero-field splitting parameter D with respect to a properly chosen geometrical parameter (D_str) in metal complexes is termed the magnetostructural D-correlation. In mononuclear hexacoordinate Ni(II) complexes with the ground electronic term ³B_1g (³A_2g in the regular octahedron), it proceeds along two intercepting straight lines, allowing for predicting the sign and magnitude of the D-parameter by knowing the X-ray structure alone; D_str is constructed from the metal–ligand bond lengths. In hexacoordinate Co(II) complexes, it is applicable only in the segment of the compressed bipyramid where the ground electronic term ⁴B_1g is orbitally non-degenerate so that the spin Hamiltonian formalism holds true. The D vs. D_str correlation is strongly non-linear, and it is represented by a set of decreasing exponentials. In tetracoordinate Co(II) complexes, on the contrary, the angular distortion from the regular tetrahedron is crucial so that the appropriate structural parameter D_str is constructed of bond angles. The most complex case is represented by pentacoordinated Co(II) systems, for which it is not yet possible to define a statistically significant correlation. All of these empirical correlations originate in the electronic structure of metal complexes that can be modelled using generalized crystal-field theory. As the barrier to spin reversal in single-molecule magnets is proportional to the D-value, for rational tuning and/or prediction of the single-molecule magnetic behaviour, knowledge/prediction of the D-parameter is beneficial. In this review, we present the statistical processing of an extensive set of structural and magnetic data on Co(II) and Ni(II) complexes, which were published over the past 15 years. Magnetostructural D-correlations defined for this data set are reviewed in detail.

1. Introduction

Though a dodecanuclear plaquette-type complex, [Mn^III₈Mn^IV₄O₁₂(ac)₁₆(H₂O)₄] ·2Hac·4H₂O, abbr. Mn12ac, was synthesized in 1980, its unusual magnetic properties were reported in 1991, and it was later termed a new class of magnetic materials—single-molecule magnets [1,2,3,4,5,6,7,8,9,10,11,12]. These systems show quantum effects, of which the most important is a slow magnetic relaxation. Slow means that the extrapolated relaxation time (time extrapolated to infinity temperature) is typically of the order τ₀~10⁻⁶–10⁻⁸ s, in contrast to spin glasses, where τ₀ < 10⁻²⁰ s [13]. At a low enough temperature, the system retains its magnetization for a long enough time to find potential applications in recording techniques. Over the course of time, a plethora of 3d-metal complexes were identified as single-molecule magnets; for instance systems of different nuclearity cover Mn2, Mn3, Mn4, Mn6, Mn7, Mn8, Mn9, Mn10, Mn12, Mn16, Mn18, Mn21, Mn22, Mn25, Mn26, Mn30, Mn84, Fe4, Fe8, Fe9, Fe10, Fe11, Fe19, Co4, Co6, Ni4, Ni8, Ni12, Ni21, MnCu, V4, MnMo6, Mn2Cr, Mn2Fe, Mn2Ni2, Ni3Cr, Mn4Re4, Cu6Fe8, Co9W6, Co9Mo6, Mn8Fe4, etc. [14,15,16,17,18,19,20,21,22,23,24,25,26,27,28,29]. It can be concluded that high nuclearity is not an ultimate demand for single-molecule magnetic behaviour since many mononuclear complexes of Cr(III), Mn(III), Mn(II), Fe(III), Fe(II), Fe(I), Co(II), and Ni(II) possessing the spin S = 1 through 5/2 also show slow magnetic relaxation [30,31,32]. Moreover, some S = 1/2 spin systems, such as V(IV), low-spin Mn(IV), low-spin Co(II), low-spin Ni(III), Ni(I), and Cu(II), exhibit slow magnetic relaxation as well [33,34,35,36]. Slow magnetic relaxation and single-molecule magnetism were identified among 4f-complexes (mononuclear and polynuclear) as well as heterometallic 4f-3d complexes; for instance, Tb1, Dy1, TbCu, Dy2, Dy4, Dy6Mn6, Dy4Mn11, and many others were classified as single-molecule magnets [14].

In contrast to classical magnetic materials (like ferrites that are widely used in daily recording techniques and many useful tools), a single-molecule magnet retains its magnetization due to magnetic anisotropy. The magnetic anisotropy in single crystals is defined as the non-isotropic magnetization tensor having at least two different main axis components, or the non-isotropic susceptibility tensor. In molecules, the spin–spin interaction tensor, when rotated to its main axes, possesses the diagonal elements

$${\widehat{H}}^{\mathrm{ss}}={\hslash }^{-2}(D_{xx}{\widehat{S}}_x^2+D_{yy}{\widehat{S}}_y^2+D_{zz}{\widehat{S}}_z^2)$$

(1)

that define two eccentricity parameters:

(a)

The axial zero-field splitting (ZFS) parameter D

$$D=(-D_{xx}-D_{yy}+2D_{zz})/2\to (3/2)D_{zz}$$

(2)

(b)

The (ortho)rhombic ZFS parameter E

$$E=(D_{xx}-D_{yy})/2$$

(3)

These two parameters modify (split) the ground state energy levels in the absence of the magnetic field, and they are responsible for magnetic anisotropy. Magnetic anisotropy manifests itself in the way that, with an increasing magnetic field, the magnetization components as well as the magnetic susceptibility (Cartesian) components develop differently.

Using the ZFS parameters, the spin–spin interaction can be rewritten to the form

$${\widehat{H}}^{\mathrm{ss}}={\hslash }^{-2}[D({\widehat{S}}_z^2-{\widehat{S}}^2/3)+E({\widehat{S}}_x^2-{\widehat{S}}_y^2)]$$

(4)

and usually, a constraint $\left|D\right|\ge 3E\ge 0$ is maintained. For a uniaxial system (like D_4h or C_4v), the effective spin-Hamiltonian collapses to

$${\widehat{H}}^{\mathrm{aniso}}={\hslash }^{-2}{D}_S{\widehat{S}}_z^2$$

(5)

giving rise to energy levels depending upon the magnetic quantum number M

$$\epsilon =D_S{M}_{}^2$$

(6)

(In these formulae, it is emphasized that we are working in the strong exchange limit for polynuclear systems, so that the D-parameter depends on the (good) total molecular spin quantum number S; hence, D_S.) When D_S > 0 (easy-plane anisotropy), a model of a particle in the potential box is appropriate: a fast relaxation from the state $M=-S$ to $M=0$ (or $M=\pm 1/2$) proceeds, and the magnetic record disappears. On the contrary, when D_S < 0 (easy-axis anisotropy) a model of a particle in the double potential is applicable; the two minima are separated by a barrier to spin reversal $\mathsf{\Delta }E=\left|D_S\right|S^2$(or $\mathsf{\Delta }E=\left|D_S\right|S^2-\left|D_S\right|/4$ for the half-integral spin) and thermal activation mechanism of the relaxation, or tunnelling mechanism, apply. Based on this reasoning, a deep knowledge about the D_S-parameter is a key factor for a rational synthesis of single-molecule magnets with aimed properties. The rhombic ZFS parameter E_S is also in play through the term $E_S({\widehat{S}}_x^2-{\widehat{S}}_y^2)$, and it causes a further modification in the energy levels and consequently, the single-molecule magnetic behaviour.

2. Spin-Hamiltonian Formalism

The spin-Hamiltonian (SH) formalism is applicable when the ground electronic term is orbitally non-degenerate (e.g., ³A_2g, ⁴B_1g). In this model, the electronic wave function is substituted by spin-only kets $\left|S,M_S\right.〉$. This very restricted basis set is under the action of operators covered by three leading terms: the spin Zeeman term, the orbital Zeeman term, and the spin–orbit interaction term

$${\widehat{H}}^{\prime }={\hslash }^{-1}{\mu }_{\mathrm{B}}{g}_{\mathrm{e}}(\overrightarrow{S}\cdot \overrightarrow{B})+{\hslash }^{-1}{\mu }_{\mathrm{B}}(\overrightarrow{L}\cdot \overrightarrow{B})+{\hslash }^{-2}\lambda (\overrightarrow{L}\cdot \overrightarrow{S})$$

(7)

where g_e is the spin-only magnetogyric factor and $\lambda =\pm \xi /2S$ is the spin–orbit splitting parameter within the ground electronic term dependent upon the spin–orbit coupling constant ξ (the minus sign applies for the configurations dⁿ more than half-full). Then, the second-order perturbation theory yields the contribution [37]

$${\widehat{H}}^S={\hslash }^{-2}(\overrightarrow{S}\cdot \overline{\overline{D}}\cdot \overrightarrow{S})+{\hslash }^{-1}{\mu }_{\mathrm{B}}(\overrightarrow{B}\cdot \overline{\overline{g}}\cdot \overrightarrow{S})+(\overrightarrow{B}\cdot \overline{\overline{\kappa }}\cdot \overrightarrow{B})$$

(8)

To this end, three magnetic tensors contribute:

• The D-tensor (tensor of the spin–spin interaction)

$$D_{ab}={\lambda }^2{\Lambda }_{ab}\left[\mathrm{energy}\right]$$

(9)

• The g-tensor (tensor of the magnetogyric ratio)

$$g_{ab}=g_{\mathrm{e}}{\delta }_{ab}+2\lambda {\Lambda }_{ab}\left[\mathrm{dimensionless}\right]$$

(10)

• The κ-tensor (tensor of the temperature-independent paramagnetism)

$${\kappa }_{ab}^{}={\mu }_{\mathrm{B}}^2{\Lambda }_{ab}[\mathrm{energy}\times {\mathrm{induction}}^{–2}]$$

(11)

All of them are constituted of the Λ-tensor (tensor of the angular momentum unquenching)

$${\Lambda }_{ab}=-{\hslash }^{-2}{\displaystyle \sum _{K\ne 0}^{}\frac{〈0\left|{\widehat{L}}_a\right|K〉〈K\left|{\widehat{L}}_b\right|0〉}{E_K-E_0}}\left[{\mathrm{energy}}^{–1}\right]$$

(12)

where the summation runs over all excited states (electronic terms). The scalar product of the vectors and tensors can be developed as follows

$${\widehat{H}}^S={\displaystyle \sum _a^{x,y,z}{\displaystyle \sum _b^{x,y,z}\{{\hslash }^{-2}{\lambda }^2{\Lambda }_{ab}{\widehat{S}}_a{\widehat{S}}_b+{\hslash }^{-1}{\mu }_{\mathrm{B}}{B}_a(g_{\mathrm{e}}{\delta }_{ab}+2\lambda {\Lambda }_{ab}){\widehat{S}}_b+{\mu }_{\mathrm{B}}^2{\Lambda }_{ab}{B}_a{B}_b\}}}$$

(13)

In the zero magnetic field, we arrive at the Formulae (1)–(4), which are widely used in analysing the magnetic data (susceptibility and magnetization) as well as the spectroscopic data such as Electron–Spin–Resonance (ESR) or Far-Infra-Red (Far-IR) spectra referring to electronic transitions.

For degenerate ground electronic terms of the T-type, the above formalism is inappropriate, and one is left to pass beyond the spin-Hamiltonian formalism. In the early stages, it was covered by Griffith and Figgis’ theory, respectively, dealing with the ground T_1(g) or T_2(g) terms, eventually under symmetry lowering and configuration interaction [38,39]. A more complete approach that involves all members of the dⁿ-electron configuration was outlined by the König–Kremer theory [40,41,42].

3. Electronic Structure of 3d-Complexes

An almost complete description of the energy levels in metal d- or f-complexes can be obtained by considering five operators acting to the basis set of free-atom terms $\left|l^{n}vLS{M}_L{M}_S\right.〉$ with the seniority number ν:

(a)

The interelectron repulsion

$$\begin{array}{c}{H}_{IJ}^{\mathrm{ee}}=〈l^{n}vLS{M}_L{M}_S\left|{\widehat{V}}^{\mathrm{ee}}\right|l^n{v}^{\prime }{L}^{\prime }{S}^{\prime }{M_L}^{\prime }{M_S}^{\prime }〉={\delta }_{M_L,{M_L}^{\prime }}{\delta }_{M_S,{M_S}^{\prime }}{\delta }_{L,L^{\prime }}{\delta }_{S,S^{\prime }}\\ 〈l^{n}vLS‖{\displaystyle \sum _{i=1}^n{\displaystyle \sum _{j>i}^n{\displaystyle \sum _{k=0,2,4}^{2l}{F}_{ll}^k{\displaystyle \sum _{q=-k}^{+k}{(-1)}^q{\widehat{C}}_{-q}^k(i)}}}}{\widehat{C}}_q^k(j)‖l^n{v}^{\prime }{L}^{\prime }{S}^{\prime }〉\end{array}$$

(14)

(b)

The crystal-field potential

$$\begin{array}{l}{H}_{IJ}^{\mathrm{cf}}=〈l^{n}vLS{M}_L{M}_S\left|{\widehat{V}}^{\mathrm{cf}}\right|l^n{v}^{\prime }{L}^{\prime }{S}^{\prime }{M^{\prime }}_L{M^{\prime }}_S〉={\delta }_{S,S^{\prime }}{\delta }_{M_S,{M_S}^{\prime }}\\ 〈l^{n}vLS{M}_L{M}_S\left|{\displaystyle \sum _{i=1}^n{\displaystyle \sum _{K=1}^N{z}_K}}{\displaystyle \sum _{k=0,2,4}^{2l}{F}_k(R_K){\displaystyle \sum _{q=-k}^{+k}{(-1)}^q{\widehat{C}}_{-q}^k(K){\widehat{C}}_q^k(i)}}\right|l^n{v}^{\prime }{L}^{\prime }{S}^{\prime }{M_L}^{\prime }{M_S}^{\prime }〉\end{array}$$

(15)

(c)

The spin–orbit interaction

$$\begin{array}{ll}{H}_{IJ}^{\mathrm{so}}& =〈l^{n}vLS{M}_L{M}_S\left|{\widehat{V}}^{\mathrm{so}}\right|l^n{v}^{\prime }{L}^{\prime }{S}^{\prime }{M_L}^{\prime }{M_S}^{\prime }〉\\ & =〈l^{n}vLS{M}_L{M}_S\left|{\hslash }^{-2}{\displaystyle \sum _{i=1}^n{\xi }_i({\overrightarrow{l}}_i\cdot {\overrightarrow{s}}_i)}\right|l^n{v}^{\prime }{L}^{\prime }{S}^{\prime }{M_L}^{\prime }{M_S}^{\prime }〉\end{array}$$

(16)

(d)

The orbital Zeeman term

$$\begin{array}{cc}{H}_{IJ}^{\mathrm{lB}}& =〈l^{n}vLS{M}_L{M}_S\left|{\widehat{V}}^{\mathrm{lB}}\right|l^n{v}^{\prime }{L}^{\prime }{S}^{\prime }{M_L}^{\prime }{M_S}^{\prime }〉\\ & ={\delta }_{v,v^{\prime }}{\delta }_{L,L^{\prime }}{\delta }_{S,S^{\prime }}{\delta }_{M_S,{M_S}^{\prime }}〈l^{n}vLS{M}_L{M}_S\left|{\hslash }^{-1}{\mu }_{\mathrm{B}}\kappa (\overrightarrow{B}\cdot \overrightarrow{L})\right|l^n{v}^{\prime }{L}^{\prime }{S}^{\prime }{M_L}^{\prime }{M_S}^{\prime }〉\end{array}$$

(17)

(e)

The spin Zeeman term

$$\begin{array}{l}{H}_{IJ}^{\mathrm{sB}}=〈l^{n}vLS{M}_L{M}_S\left|{\widehat{V}}^{\mathrm{sB}}\right|l^n{v}^{\prime }{L}^{\prime }{S}^{\prime }{M_L}^{\prime }{M_S}^{\prime }〉\\ ={\delta }_{v,v^{\prime }}{\delta }_{L,L^{\prime }}{\delta }_{S,S^{\prime }}{\delta }_{M_L,{M_L}^{\prime }}〈l^{n}vLS{M}_L{M}_S\left|{\hslash }^{-1}{\mu }_{\mathrm{B}}{g}_{\mathrm{e}}(\overrightarrow{B}\cdot \overrightarrow{S})\right|l^n{v}^{\prime }{L}^{\prime }{S}^{\prime }{M_L}^{\prime }{M_S}^{\prime }〉\end{array}$$

(18)

Here, the Racah operator (rationalized spherical harmonics) ${\widehat{C}}_q^k(i)={\left(4\pi /2k+1\right)}^{1/2}{Y}_q^k(i)$ occurs, where k—rank and q—component. The parameter

$$F_k(R_K)=\left(e^2/4\pi {\epsilon }_0\right)〈r_{>}^{-(k+1)}\cdot r_{<}^k〉\approx \left(e^2/4\pi {\epsilon }_0\right)R_K^{-(k+1)}\cdot 〈r^k〉$$

(19)

refers to the crystal field strength of the K-th ligand (R_K defines the distance of the ligand from the central atom); $F_{ll}^k=\left(e^2/4\pi {\epsilon }_0\right)〈r_{>}^{-(k+1)}\cdot r_{<}^k〉$ are Slater–Condon parameters of rank k. The orbital Zeeman term includes κ—an orbital reduction factor. The advantage of this approach is that all matrix elements can be expressed using closed formulae [37]. By selecting a certain combination of operators/matrix elements, several cases can be mapped:

(i)

By diagonalizing the matrix

$$H_{IJ}=H_{IJ}^{\mathrm{ee}}+H_{IJ}^{\mathrm{cf}}$$

(20)

the resulting eigenvalues refer to the energies of crystal field terms. No constrain to the crystal field strength is needed (weak field, strong field, intermediate field) since the variation principle is applied. The resulting eigenvectors $\left|l^n(vL)S{M}_S\Gamma \gamma a\right.〉$ can be probed for symmetry operations of the respective point group of symmetry, allowing for a classification according to the irreducible representations Γγ (like A_2g, T_1g, and T_2g for the group O_h of the d⁸ system, in Mulliken notation).

(ii)

By diagonalizing the matrix

$$H_{IJ}=H_{IJ}^{\mathrm{ee}}+H_{IJ}^{\mathrm{cf}}+H_{IJ}^{\mathrm{so}}$$

(21)

one obtains the crystal field multiplets. The eigenvectors $\left|l^n(vLS)J{\Gamma }^{\prime }{\gamma }^{\prime }b\right.〉$, when probed for symmetry operations of the respective double group, yield an assignment of the Bethe symbols (Γ₁ through Γ₈ for the O’ double group) to the energy levels.

(iii)

By diagonalizing the matrix

$$H_{IJ}=H_{IJ}^{\mathrm{ee}}+H_{IJ}^{\mathrm{cf}}+H_{IJ}^{\mathrm{so}}+H_{IJ}^{\mathrm{lB}}(B)+H_{IJ}^{\mathrm{sB}}(B)$$

(22)

the Zeeman levels dependent on the magnetic field are obtained. They enter the partition function Z(B,T), from which the magnetization components M_a(B,T), the susceptibility components χ_ab(B,T), and eventually, the heat capacity c_V(B,T) can be obtained with the apparatus of statistical thermodynamics [37].

The above matrices, in general, are complex-Hermitian, so the programming requires complex*16 arithmetic. The size of the basis set for d⁵ through d⁹ systems is 256, 210, 120, 45, and 10 members, respectively. In this approach, the configuration interaction is naturally involved, and it is termed the Generalized Crystal-Field Theory (GCFT).

4. Zero-Field Splitting Parameters

The application of the spin-Hamiltonian formalism offers modelling of the energy levels, magnetization, and magnetic susceptibility depending upon the spin quantum numbers S = 1, 3/2, 2, and 5/2. The corresponding spin matrices have dimensions of 3, 4, 5, and 6 in the basis set of spin kets $\left|S,M_S\right.〉$. The members of the spin multiplets in the zero field are separated by amount D, 2D, (D, 3D), and (2D, 4D), respectively, as shown in Figure 1.

In general, the spin Zeeman term depends on the polar coordinates $(\vartheta ,\phi )$ as follows

$${\widehat{H}}_{kl,m}^Z={\mu }_{\mathrm{B}}{B}_m(g_x^{}\sin {\vartheta }_k\cos {\phi }_l{\widehat{S}}_x^{}+g_y^{}\sin {\vartheta }_k\sin {\phi }_l{\widehat{S}}_y^{}+g_z^{}\cos {\vartheta }_k{\widehat{S}}_z^{}){\hslash }^{-1}$$

(23)

where (k, l) refer to grids distributed uniformly over a sphere. In this way, the magnetization can be visualized with a 3D diagram, as shown in Figure 2. It must be noted that when |D| < 3E, a renumbering of the Cartesian axes results in the usual constraint |D| > 3E. In a very high field (50 T), the system becomes isotropic, and its 3D magnetization refers to a sphere.

At the higher level of the theory (GCFT), when all members of the dⁿ configuration are considered, the D and E values are not rigorously defined. Figure 3 brings the modelling of the lowest energy gap among crystal field multiplets beyond the SH formalism using GCFT for a wide range of crystal field strengths, which is briefly discussed below.

The octahedral d³ and d⁸ systems are fortunate cases since the ground electronic term is nondegenerate (⁴A_2g, ³A_2g), and it stays nondegenerate for the whole array of compressed and elongated tetragonal bipyramids (⁴B_1g, ³B_1g). For Ni(II) complexes (S = 1), the lowest crystal field multiplets, labelled in the D’₄ double group by Bethe (Mulliken) symbols Γ₄(B₂) and Γ₅(E₁), are separated by the energy gap $\mathsf{\Delta }=\epsilon ({\mathsf{\Gamma }}_5)-\epsilon ({\mathsf{\Gamma }}_4)$; this value adopts role of the D-parameter (the Γ₅ level is doubly degenerate in the zero field). D > 0 applies for an elongated tetragonal bipyramid, and D < 0 holds true for the compressed bipyramid. For the octahedral geometry, the energy gap collapses to zero. The range of D-values for Cr(III) is by one order of magnitude lower relative to Ni(II); this is a consequence of a much lower spin–orbit splitting parameter λ/hc = 92 vs. 315 cm⁻¹ (D~λ²).

The octahedral d⁷ system possesses the ground term ⁴T_1g, which is orbitally triply degenerate. Upon distortion to a compressed tetragonal bipyramid, it is split into {⁴A_2g, ⁴E_g} terms; upon distortion to an elongated bipyramid, the splitting is opposite {⁴E_g, ⁴A_2g}. The spin–orbit coupling causes a further splitting into six Kramer doublets: 1Γ₆, 1Γ₇, 2Γ₆, 3Γ₆, 2Γ₇, and 3Γ₇ for the compression and 1Γ₆, 2Γ₆, 1Γ₇, 2Γ₇, 3Γ₆, and 3Γ₇ for the elongation. This means that only the first case is consistent with the SH formalism. Moreover, the crystal field asymmetry should be large (much stronger axial crystal field F₄(z)); in such a case, always Δ = 2D > 0, and it is very high (typically 200 cm⁻¹).

The counter parting d² system with the ground state ³T_1g resembles tetragonal splitting into {³A_2g, ³E_g} upon compression, and the spin–orbit coupling splits the ground term into Γ₁(A₁) and Γ₅(E₁) multiplets. In this case, Δ = D > 0 holds true. For the elongated bipyramid, the six-membered multiplet manifold is Γ₃(B₁), Γ₄(B₂), Γ₅(E₁), Γ₁(A₁), and Γ₂(A₂), and it has no relationship with the SH approach.

For Mn(III) systems (d⁴, S = 2), the situation is more complex. The spin-Hamiltonian (SH) formalism predicts that for D > 0 (compressed tetragonal bipyramid), the ground state $\left|S,M_S\right.〉$ is a singlet $\left|2,0\right.〉$.The first excited Kramer doublet $\left|2,\pm 1\right.〉$ is a D-value higher, and the second excited Kramer doublet $\left|2,\pm 2\right.〉$ lies a 4D amount above the ground state. The GCFT is consistent with the SH formalism only for the first three multiplets Γ₁ and Γ₅ (doubly degenerate). The remaining two excited states, Γ₃ and Γ₄, are not exactly degenerate (they do not form a Kramer doublet), but they are split in the zero field by 0.2 cm⁻¹. (The calculated energy levels are, for instance: Γ₁(A₁) × 1 at 0; Γ₅(E₁) × 2 at 5.1 cm⁻¹ and Γ₃(B₁) × 1 at 19.8 cm⁻¹; Γ₄(B₂) × 1 at 20.0 cm⁻¹. Thus, for D = 5.1 cm⁻¹ is 4D = 20.4 cm⁻¹, but the Γ₄ level is 0.4 cm⁻¹ lower.) This demonstrates that we cannot rely on the spin-Hamiltonian formalism in all cases.

The d⁶ system, similar to high-spin Fe(II), again is a complex task. In the octahedral geometry, the ground term is orbitally triply degenerate, ⁵T_2g. Upon tetragonal elongation, it is split into {⁵B_2g, ⁵E_g} terms, and five members of the ground term ⁵B_2g are further split by the spin–orbit interaction into multiplets Γ₄, Γ₅, Γ₁, and Γ₂. Then, the SH formalism is approximately valid (the Γ₁, Γ₂ pair is quasi-degenerate and separated from the ground Γ₄ by ca. 4D); the D-value is moderate: D/hc~10 cm⁻¹. Upon tetragonal compression, the 10-membered manifold of the ⁵E_g term is split in a way having no relationship to the SH formalism, so that the D-parameter is undefined.

The electronic structure of tetrahedral dⁿ systems matches complementary octahedral systems d¹⁰⁻ⁿ. For instance, tetrahedral Co(II)—d⁷ possesses the ground state ⁴A₂, similar to octahedral Cr(III)—d³. All GCFT calculations were carried out using our own software written in Fortran [37,43].

5. Magnetostructural J-Correlations

Before proceeding to the discussion of magnetostructural D-correlations, we briefly mention the original type of Correlation Analysis of structure–magnetism relations in transition metal complexes. Relationships between selected structural parameters and the magnetic properties of dinuclear (polynuclear) metal complexes were outlined by Hatfield [44,45], his co-workers, and followers. In [Cu^II-(OH)₂-Cu^II]-type complexes, the isotropic exchange constant J correlates with the bond angle α = Cu-O-Cu along a straight line with negative slope

J[cm⁻¹] = −74.53·α[°] + 7270, r = −0.99

(24)

Involvement of more data with greater structural diversity along the superexchange path led to a differentiation according to the alkoxido- or phenoxido-class [46]. Analogous linear correlations were developed for [Cr^III-(OH)₂-Cr^III]- and [Mn^IV-(O)₂-Mn^IV]-type complexes [47,48,49].

According to Gorun and Lippard, the J-value correlates with the shortest Fe-O distance (abbr. P) along the superexchange path in [Fe^III-O-Fe^III]-type complexes [50,51]. The correlation was proposed along a decreasing exponential curve that is applicable only in the segment of negative J

−J[cm⁻¹] = (2.360 × 10¹⁰)⋅exp(−10.661 P[10⁻¹⁰ m]), r = −0.97

(25)

However, a linear correlation is also acceptable; hence

J[cm⁻¹] = 525.7⋅P[10⁻¹⁰ m] − 1055, r = 0.96

(26)

It must be critically said that any kind of magnetostructural relationship or correlation heavily depends upon the quality of structural and magnetic data. Another aspect is that almost all correlations are restricted to the linear relationships of the J-α or J-P types. Nowadays, modern statistical software packages (multivariate methods) are at our disposal. Correlation Analysis, Cluster Analysis, Principal Component Analysis, Factor Analysis, etc., can be applied to carefully filtered datasets. An approach similar to this appeared very recently [52].

6. Magnetostructural D-Correlations

It is obvious that the correlation D vs. P (a geometrical parameter) is derived from the electronic structure of complexes. The problem of electronic structure, as well as magnetic properties, is solved mainly at the level of quantum–chemical (first-principle) calculations [53,54,55]. The application of the partitioning technique and/or quasi-degenerate perturbation theory yields the matrix elements of the effective Hamiltonian spanned by the spin manifold of the orbitally non-degenerate ground electronic state $\left|0SM\right.〉$ as follows

$$\begin{array}{l}〈0SM\left|{\widehat{H}}_{\mathrm{eff}}\right|0S{M}^{\prime }〉=E_0{\delta }_{M{M}^{\prime }}+〈0SM\left|{\widehat{H}}_1\right|0S{M}^{\prime }〉\\ -{\displaystyle \sum _{b{S}^{″}{M}^{″}}{\Delta }_b^{-1}}〈0SM\left|{\widehat{H}}_1\right|b{S}^{″}{M}^{″}〉\cdot 〈b{S}^{″}{M}^{″}\left|{\widehat{H}}_1\right|0S{M}^{\prime }〉\end{array}$$

(27)

with the denominator defined by excitation energies

$${\Delta }_b^{-1}={(E_b-E_0)}^{-1},E_0=〈0SM\left|{\widehat{H}}_0\right|0SM〉$$

(28)

Then, the D-tensor components (m, n = x, y, z) arising from the spin–orbit interaction are obtained in the form

$$\begin{array}{l}{D}_{mn}^{(0)}=-\frac{1}{S^2}{\displaystyle \sum _{b(S_b=S)}{\Delta }_b^{-1}}〈0SS\left|{\displaystyle \sum _i{\widehat{h}}_m^{(i)}{\widehat{s}}_z^{(i)}}\right|bSS〉\\ \cdot 〈bSS\left|{\displaystyle \sum _i{\widehat{h}}_n^{(i)}{\widehat{s}}_z^{(i)}}\right|0SS〉\end{array}$$

(29)

$$\begin{array}{l}{D}_{mn}^{(-1)}=-\frac{1}{S(2S-1)}{\displaystyle \sum _{b(S_b=S-1)}{\Delta }_b^{-1}}〈0SS\left|{\displaystyle \sum _i{\widehat{h}}_m^{(i)}{\widehat{s}}_{+1}^{(i)}}\right|b,S-1,S-1〉\\ \cdot 〈b,S-1,S-1\left|{\displaystyle \sum _i{\widehat{h}}_n^{(i)}{\widehat{s}}_{-1}^{(i)}}\right|0SS〉\end{array}$$

(30)

$$\begin{array}{l}{D}_{mn}^{(+1)}=-\frac{1}{(S+1)(2S+1)}{\displaystyle \sum _{b(S_b=S+1)}{\Delta }_b^{-1}}〈0SS\left|{\displaystyle \sum _i{\widehat{h}}_m^{(i)}{\widehat{s}}_{-1}^{(i)}}\right|b,S+1,S+1〉\\ \cdot 〈b,S+1,S+1\left|{\displaystyle \sum _i{\widehat{h}}_n^{(i)}{\widehat{s}}_{+1}^{(i)}}\right|0SS〉\end{array}$$

(31)

where the one-electron (i) orbital operator for the spin–orbit coupling is

$${\widehat{h}}_m^{(i)}={\displaystyle \sum _A{\xi }_A^{(i)}{\widehat{l}}_{mA}^{(i)}}$$

(32)

A comparison with the simple perturbation theory applied to the crystal field terms arising from the d-electrons of the central atom [56,57,58]

$$D_{mn}^{\mathrm{CFT}}=-\frac{1}{4S^2}{\displaystyle \sum _{K\ne 0}^{}\frac{〈0\left|{\widehat{L}}_m\xi \right|K〉〈K\left|{\widehat{L}}_n\xi \right|0〉{\hslash }^{-2}}{E_K-E_0}}$$

(33)

shows that more contributions are in play. Quantum–chemical calculations show that the individual contributions to the D-parameter are different in size and sign, and a lot of terms approximately cancel. The dominating part arises from the spin–orbit coupling; however, the contributions from the spin–spin interaction also cannot be completely neglected in many cases [59].

A much more simplified approach is GCFT. By inspecting Figure 4, it is possible to transform the 3D graph D = f(F₄(xy), F₄(z)) into a 2D diagram D = f(P). The crystal field poles can be expressed with the fourth electronic moment $<r^4>$, common for the complex as follows

$$F_4(R_K)\approx \left(e^2/4\pi {\epsilon }_0\right)R_K^{-5}〈r^4〉$$

(34)

hence, the relationship

$$\left(e^2/4\pi {\epsilon }_0\right)〈r^4〉=F_4(z)R_z^5=F_4(xy)R_{xy}^5$$

(35)

holds true. This method enables relatively fast mapping of the structural dependence of the D-parameter for a wide range of distortions.

6.1. Hexacoordinate Ni(II) Complexes

Magneto-structural relationships for the nearly-octahedral Ni(II) complexes were mapped along a tetragonal distortion mode characterized by the difference between axial (R_z) and equatorial (R_xy) metal–ligand bond lengths. Thus, the structural D-parameter can be expressed by introducing the X_F function as follows

$$D_{\mathrm{str}}=R_z-R_{xy}=R_z\left[1-{\left(\frac{F_4(z)}{F_4(xy)}\right)}^{1/5}\right]=R_z\cdot X_F$$

(36)

A modelling of the magnetic D-values (exact multiplet splitting) depending on the transformed structural ordinate is shown in Figure 4. The important message is that such dependence is generally non-linear; however, within the relevant experimental range, it follows a nearly linear relationship. In fact, the dependence—the correlation line of the magnetostructural D-correlation—is represented by a pair of nearly collinear straight lines intercepting at the octahedral geometry where D and D_str are zero. The calculations using GCFT were performed for the nephelauxetic ratio β = 1, and the orbital reduction factors κ_z = κ_xy = 1; therefore, the results are tentative.

The magnetic data used hereafter were collected quite recently using the SQUID magnetometer. The magnetic susceptibility (χ) was taken at the applied field of B_DC = 0.1 T between T = 1.9 and 300 K. The magnetization data (M) were taken at T = 2.0 and 4.6 K, respectively, up to B = 5 or 7 T. Both datasets were fitted simultaneously using a numerical procedure that minimizes a joint functional $F=R(\chi )\cdot R(M)$. A collection of 25 datapoints (objects = complexes under study) is displayed in Figure 5 along with a common linear correlation D vs. D_str. This simple regression analysis was performed in SigmaPlot software [60] and clearly confirms the linear dependence in the given interval of D_str values. Based on the analysis of variance (ANOVA), one can conclude that the independent variable D_str can be used to predict the dependent variable D (large F and P < 0.05). A list of the compounds and numerical data is provided in the Supplementary Information [61,62]. The correlation predicts that with increasing structural tetragonality D_str (in the direction compressed tetragonal bipyramid–octahedron–elongated tetragonal bipyramid), the axial zero-field splitting parameter D increases.

It must be mentioned that for the complexes with a heterogeneous coordination sphere, e.g., possessing {NiN₄O₂} chromophore, the evaluation of the experimental value of D_str requires a correction taking into account the averaged bond lengths of the given donor atom. For instance

$$D_{\mathrm{str}}={\mathit{\Delta }}_z-({\mathit{\Delta }}_y+{\mathit{\Delta }}_x)/2$$

(37)

where ${\mathit{\Delta }}_a=(R_a-{\overline{R}}_a)$ for a = x, y, and z is a shift relative to the mean distance ${\overline{R}}_a$ for a given bond. For the N- and O-donor ligands, these values were taken from complexes containing [Ni(NH₃)₆]²⁺ and [Ni(H₂O)₆]²⁺ units, respectively, $\overline{R}(\mathrm{Ni}-\mathrm{N})=$ 2.145 and $\overline{R}(\mathrm{Ni}-\mathrm{O})=$ 2.055 Å. For a more ionic bond, the value of $\overline{R}(\mathrm{Ni}-\mathrm{NCS})=$ 2.070 Å was used.

Another piece of information is obtained from the multivariate data analysis, which we performed using the Statgraphics software [63]. Principal Component Analysis (PCA) transforms the set of original variables into their linear combinations (principal components), bearing the maximum variability in the data. Two components bear 94.7% of the variability in the present case. The biplot in Figure 6 shows how the individual variables are interrelated: the closest rays refer to a positive correlation, and the farthest rays refer to a negative correlation (anticorrelation). The graph proves that the g-factor asymmetry (gdif = g_z − g_xy) anticorrelates with the D-parameter, in agreement with the spin-Hamiltonian formula $D=-(\xi /2S)(g_z-g_{xy})/2$. On the other hand, it confirms the strong positive correlation between D and D_str. Classification of the individual points according to the clusters allows us to visualize the clusters’ separation.

Factor Analysis (FA) constructs a small number of new variables—common factors that represent a large percentage of the variability in the original variables. Factor loadings may be obtained from either the sample covariance or sample correlation matrix. The initial loadings may be rotated (e.g., with varimax rotation). Two factors cover 96.5% of the variability in the original data (D_str, D, g_xy, and g_z) in the present case (Figure S1). Again, the strong correlation between parameters D and D_str is confirmed.

6.2. Hexacoordinate Co(II) Complexes

The above procedure outlined for Ni(II) complexes was also applied to a set of hexacoordinate Co(II) complexes. As already mentioned, only the segment of the compressed tetragonal bipyramids matches the spin-Hamiltonian formalism, and the D-values are expected to be very high [64].

A collection of 16 datapoints is displayed in Figure 7 (left), from which it is evident that a linear correlation does not apply in this case. This is understood from the transformed 3D graph D = f(F₄(xy), F₄(z)) into a 2D diagram D = f(D_str) (Figure 7, right). For the complexes with a heterogeneous coordination sphere, the mean distances $\overline{R}(\mathrm{Co}-\mathrm{N})=$ 2.185, $\overline{R}(\mathrm{Co}-\mathrm{O})=$ 2.085, and $\overline{R}(\mathrm{Co}-\mathrm{Cl})=$ 2.475 Å were used to calculate the experimental D_str values. The data worksheet is listed in the SI and processed using multivariate statistical methods [65,66]. The Cluster Analysis based on three variables (labelled as Dstr, D2 = 2D and gxy) shows that the distance separates the objects into two principal clusters (Figure 8). Their separation is also well-seen from the Principal Component Analysis (Figure S2). The assumption on which the correlation 2D vs. D_str is based is thus statistically confirmed. It is clear that the discovered clustering classifies objects based on close D_str values, with cluster 1 including complexes with significantly more negative D_str. However, for the purposes of Correlation Analysis, we excluded outliers A and M from individual clusters. We assume that these objects may indicate new clusters in the segment D_str < 0. If we accept this assumption, then the non-linear correlation 2D vs. D_str has a satisfactory predictive character.

Co(II) complexes were also studied using ab initio methods (CASSCF + NEVPT2) to address the electronic origin of the ZFS and support the experimental results. This is a highly sophisticated quantum chemistry method based on the definition of multi-configurational self-consistent field (MCSCF) wave functions, which are used as reference states for multi-reference perturbation theories (MRPT), similar to n-electron valence state perturbation theory (NEVPT2) [57]. The combination of MCSCF and MRPT adequately describes multi-reference systems, such as, e.g., transition metal complexes, as it involves much of the electron correlation. In general, this approach represents a significant advance in the understanding of magnetostructural correlations, since many simpler methods based on electrostatic theories inappropriately solve the details of electronic structure-magnetism relations. It is therefore obvious that the combination of highly reliable experimental and computational techniques is currently a standard tool that, e.g., reveals how subtle structural modifications significantly affect the magnetic behaviour of molecules.

6.3. Tetracoordinate Co(II) Complexes

A collection of the spin-Hamiltonian parameters, in this case, is based upon High-Frequency/High-Field Electron Spin Resonance (syn. EPR, EMR) with 10 datapoints and completed using five results from a simultaneous fitting of the susceptibility/magnetization data for [CoA₂B₂]-type complexes [67,68]. The basic problem arises from the fact that in addition to radial variations (bond lengths Co-A, Co-B), the angular distortion (bond angles A-Co-A, B-Co-B) is substantial. Moreover, twisting angles A-Co-B come into play (in total, seven coordinates are independent).

The importance of the angular distortion was proven with modelling using the GCFT, as shown in Figure 9. The energy gap between the lowest crystal field multiplets refers to $\mathsf{\Delta }=2{(D^2+3E^2)}^{1/2}\doteq 2D$. On the right, the transformed ordinate was used for the 2D plot, giving rise to a general route to be followed by the magnetostructural D-correlation in tetracoordinate Co(II) complexes. It proceeds according to an increasing exponential. The correlation D vs. A₋ is shown in Figure 10, and it can be concluded that the experimental data matches the general course predicted using GCFT modelling. A fit to exponential curves $D=a+b\cdot \exp (c\cdot A_{-})$ is also shown with dashed lines.

For the multivariate methods, seven variables were considered: R(Co-A), R(Co-B), α(A-Co-A), β(B-Co-B), g_z, g_xy, D abbreviated as RA, RB, alpha, beta, gz, gxy, and D. Also, three transformed variables were added: Aplus = (alpha + beta)/2, Aminus = (alpha − beta)/2, gdif = gz − gxy. It must be mentioned that the data involve very different chromophores as follows: {CoN₄}, {CoN₂N₂}, {CoCl₄}, {CoBr₄}, {CoCl₂P₂}, {CoBr₂P₂}, and the most numerous {CoCl₂N₂} one. (Two datapoints with {CoN₂O₂} and {CoO₂S₂} chromophores were deleted from consideration due to evident dissimilarity). The Cluster Analysis points to two principal clusters (Figure 11) containing 11 and 4 members, respectively. Eventually, the compound with large D/hc = 41 cm⁻¹ could be classified as a new cluster. The PCA method (Figure 12) shows a rather weak correlation between the D-values and the individual geometric parameters; the best correlation is D vs. A_. The PCA also confirms an anticorrelation of D and gdif, as expected with the spin-Hamiltonian formalism. On the other hand, the PCA evidently separates the individual clusters. The non-linear correlation D vs. A_ in Figure 10 is predictive for cluster 1 but not for cluster 2. The expansion of the data in the segment of A_ > 0 will therefore be necessary.

6.4. Pentacoordinate Co(II) Complexes

For pentacoordinate complexes of the [CoXA₂B₂] type, a basic obstacle arises from the fact that too many (12) geometrical parameters come into play, and the coordination polyhedron is often far from the ideal tetragonal pyramid and/or trigonal bipyramid. Also, the description of the intermediate geometries with the angular distortion (trigonality) τ-parameter represents a strong idealization. Moreover, there are only a few reliable magnetic datapoints based on both the susceptibility and magnetization measurements (HF/HF EPR cannot be used since D-values are too large).

The angular dependence of the D-parameter with respect to two geometrical parameters is shown in Figure 13. The polar angles θ(A) and θ(B) were used to map the Berry-kind of transformation between the trigonal bipyramid and tetragonal pyramid using GCFT. It is worth noting the fact that the majority of the complexes under study possess trigonality parameter τ > 1 due to a rigid tridentate ligand with the {XA₂} donor set.

Eleven datapoints were collected (see the Supplementary Material) [69,70] and the variables R(Co-X), R(Co-A), R(Co-B), α(A-Co-A), β(B-Co-B), θ(X-Co-A), θ(X-Co-B), D, and g_xy are abbreviated for statistical analysis as RX, RA, RB, alpha, beta, thetaA, thetaB, D, and gxy. Moreover, three transformed variables were considered: γ = 360 − 2θ(A), τ = (α – β)/60 or τ = (γ − β)/60 when γ > 180 deg, and δ = (α − β)/2 abbreviated as gamma, tau, and dis. The cluster analysis distinguishes two main clusters with eight and three datapoints, respectively (Figure 14). For the first cluster, γ > 180 deg and τ > 1 holds true; for the second cluster: γ < 180 deg, τ < 1. The PCA revealed a significant correlation between parameter D and parameters R(Co-X), R(Co-B), and g_x; however, this does not provide more fundamental information in this case. Rather, a weak positive correlation with the γ parameter and anticorrelation with α appear to be more significant. Moreover, the PCA again visibly separates two clusters (Figure 15).

A trial set of functions to fit the observed D vs. structural parameter relationships is shown in Figure 16. Notice that the actual geometry is far from an ideal polyhedron, where a crude averaging of bond angles was applied. Moreover, some of the [Co(L^Cn)Cl₂] systems form a supramolecular dimer with exchange interaction J > 0. In such a case, the local D-tensors are not necessarily collinear, and this circumstance affects the fitted D-values. The correlation coefficient between D and g_x (r = 0.83) proves that the spin-Hamiltonian formula D = λ(g_z − g_x)/2 is fulfilled for pentacoordinate complexes. This conclusion matches the forecast that only D > 0 applies for pentacoordinate Co(II) complexes [71]. In this light, the reported values of D/hc= −40 cm⁻¹ in {CoN₂N₃}-type complexes seem be problematic: the authors fail in fitting the magnetization data and, moreover, they used closed formula for the addition of three Cartesian components, and neither their average nor a correct powder average was applied [72].

7. Selected Single-Molecule Magnets

The list of known single-molecule magnets based upon one metal centre (single-ion magnets, SIM) is rapidly increasing. They cover, for instance, Mn(III), Mn(II), Fe(III), Fe(II), Fe(I), Co(II), Ni(III), and Ni(II) complexes [73,74,75,76,77,78,79,80,81,82,83,84,85,86,87,88,89]. Representative examples for Co(II) are presented in

Table 1. List of single-molecule (single-ion) magnets containing Co(II).

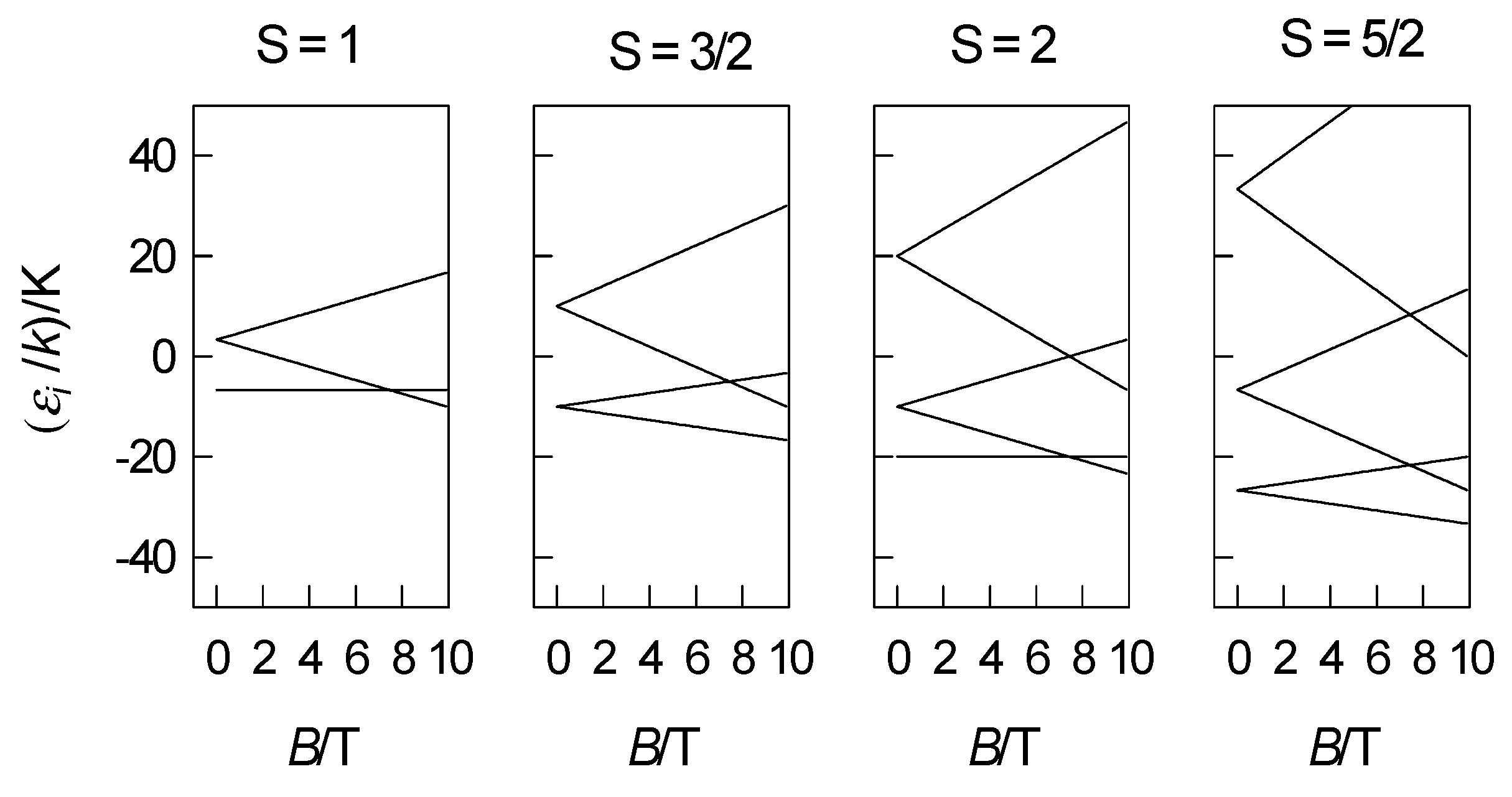
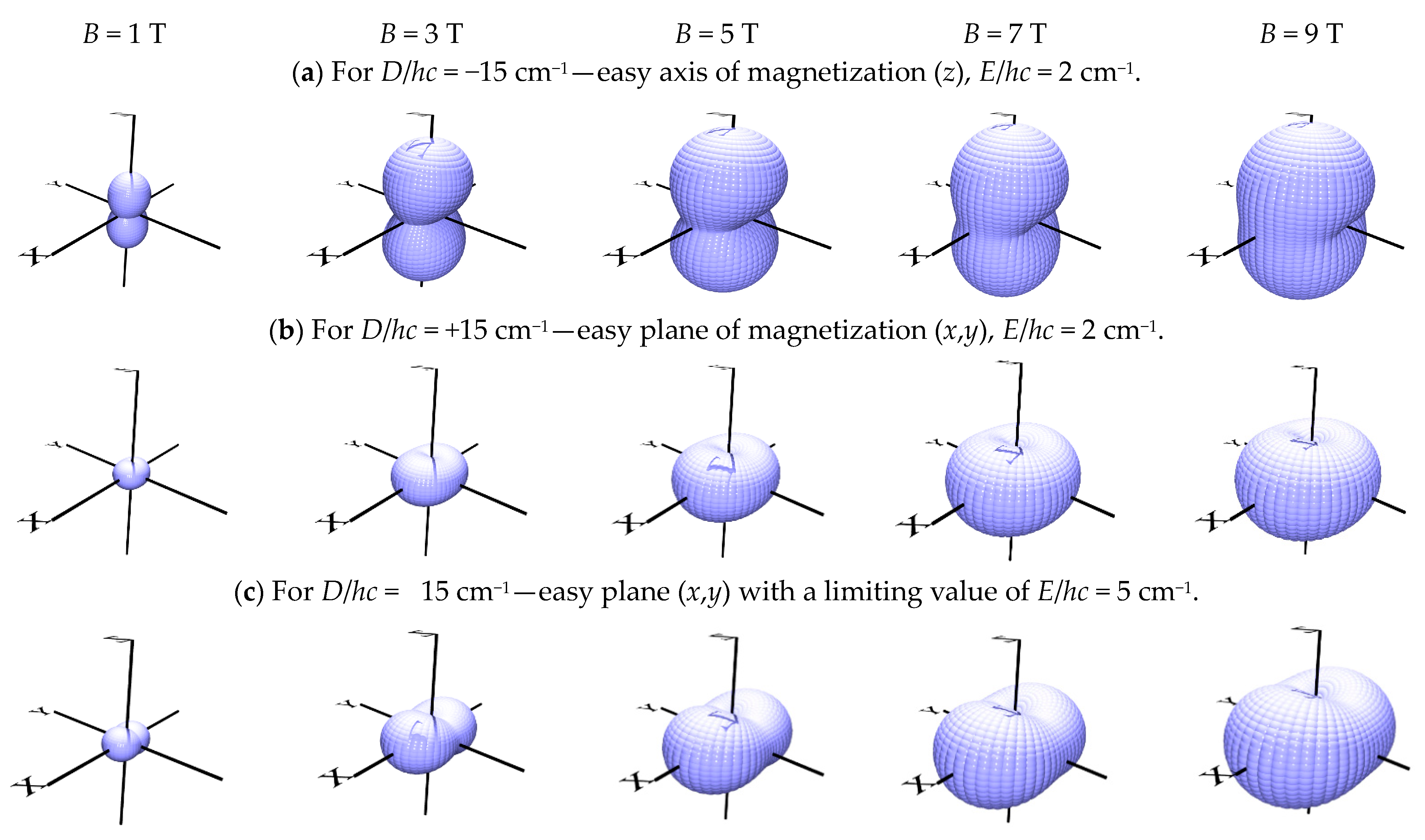
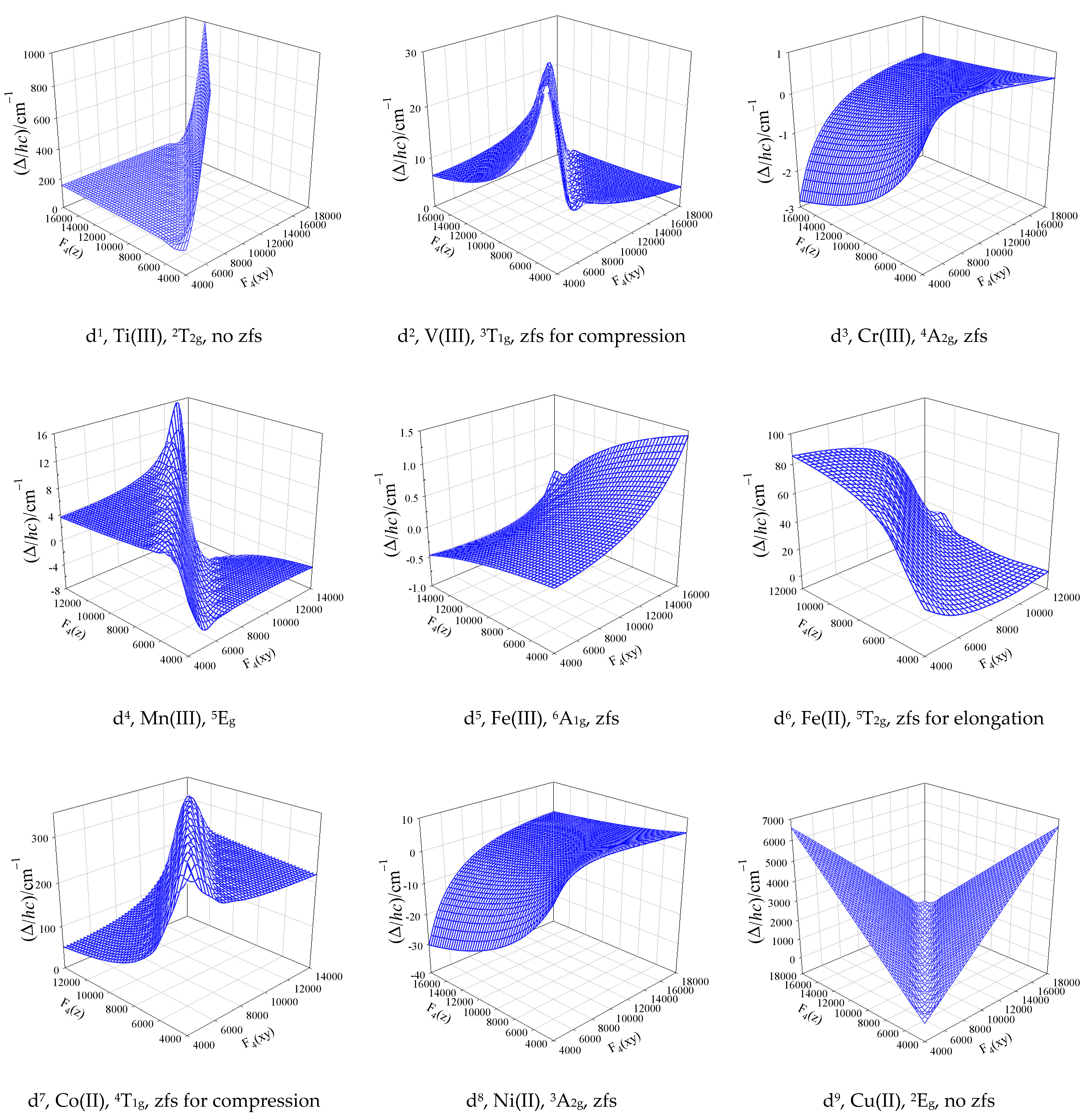
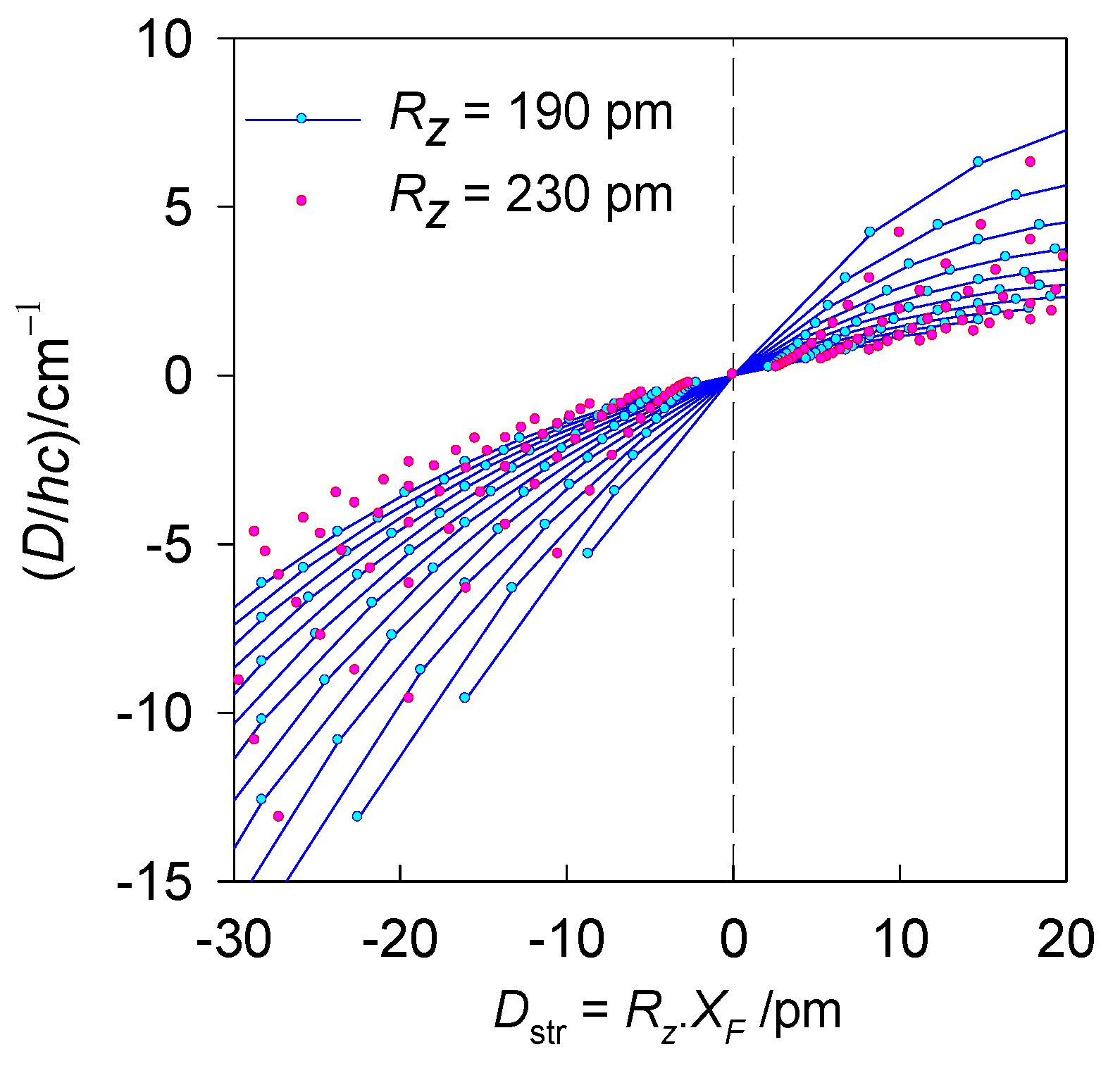
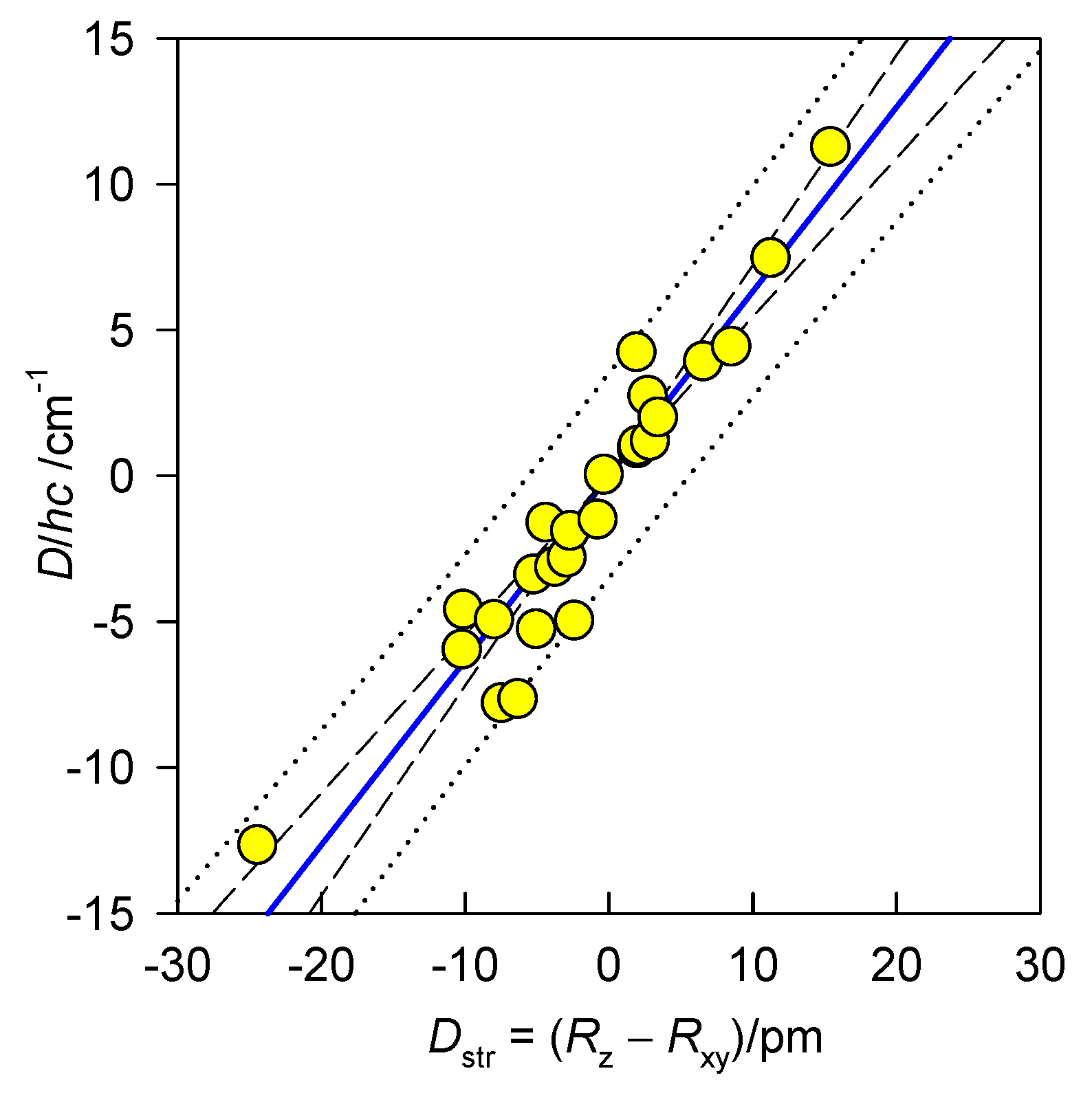
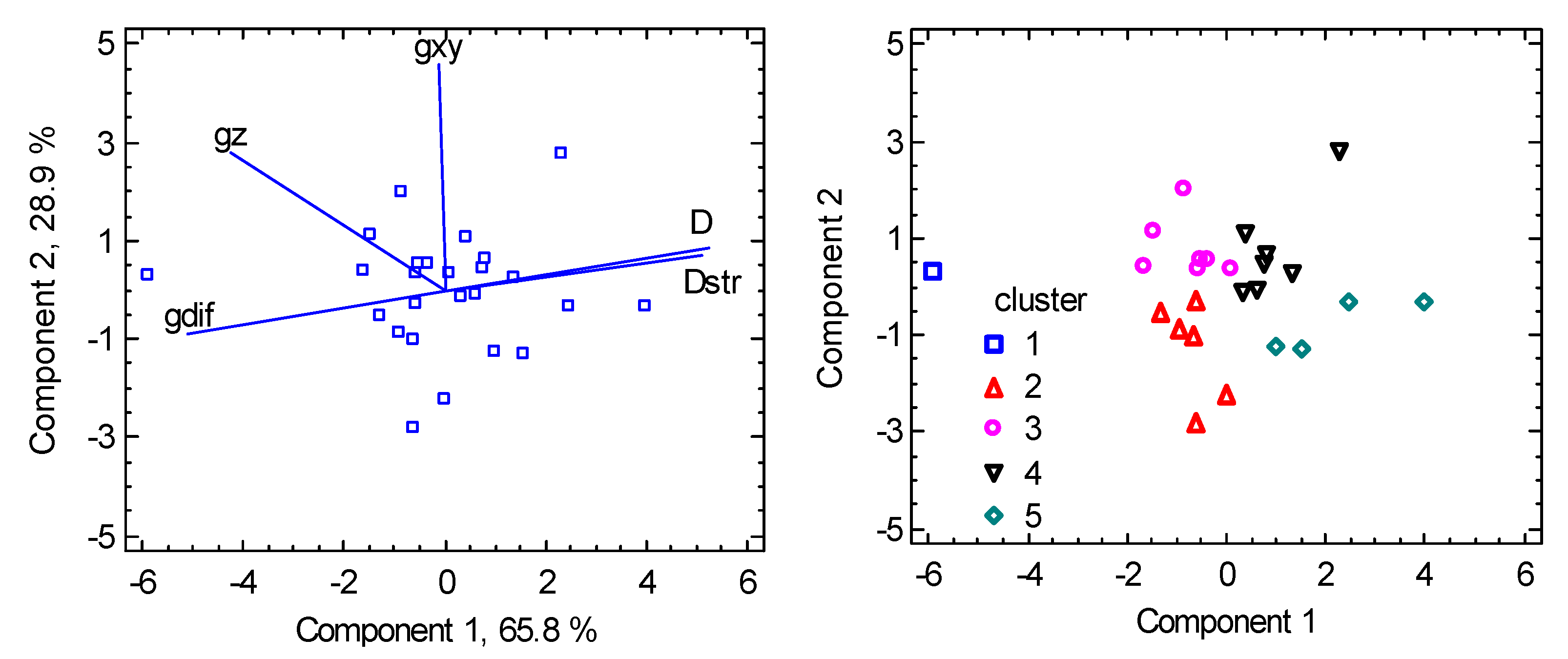
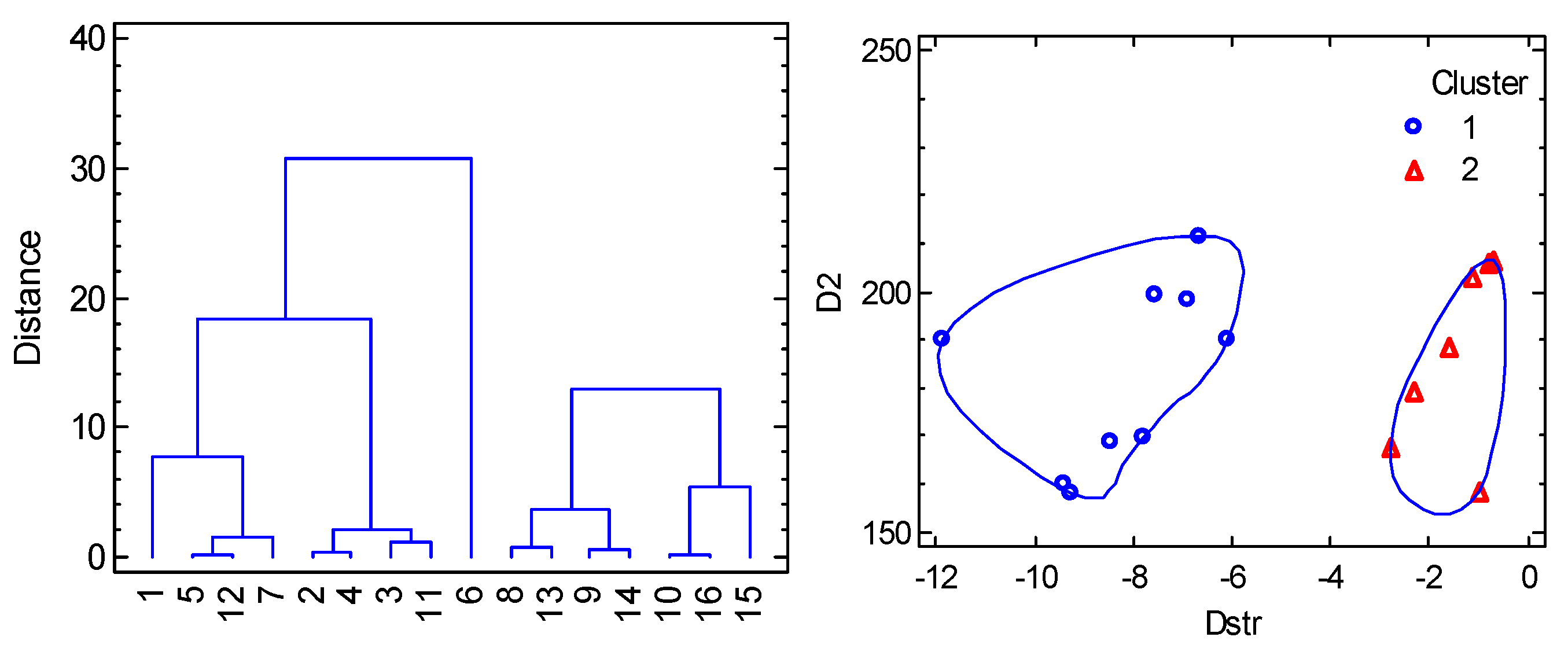
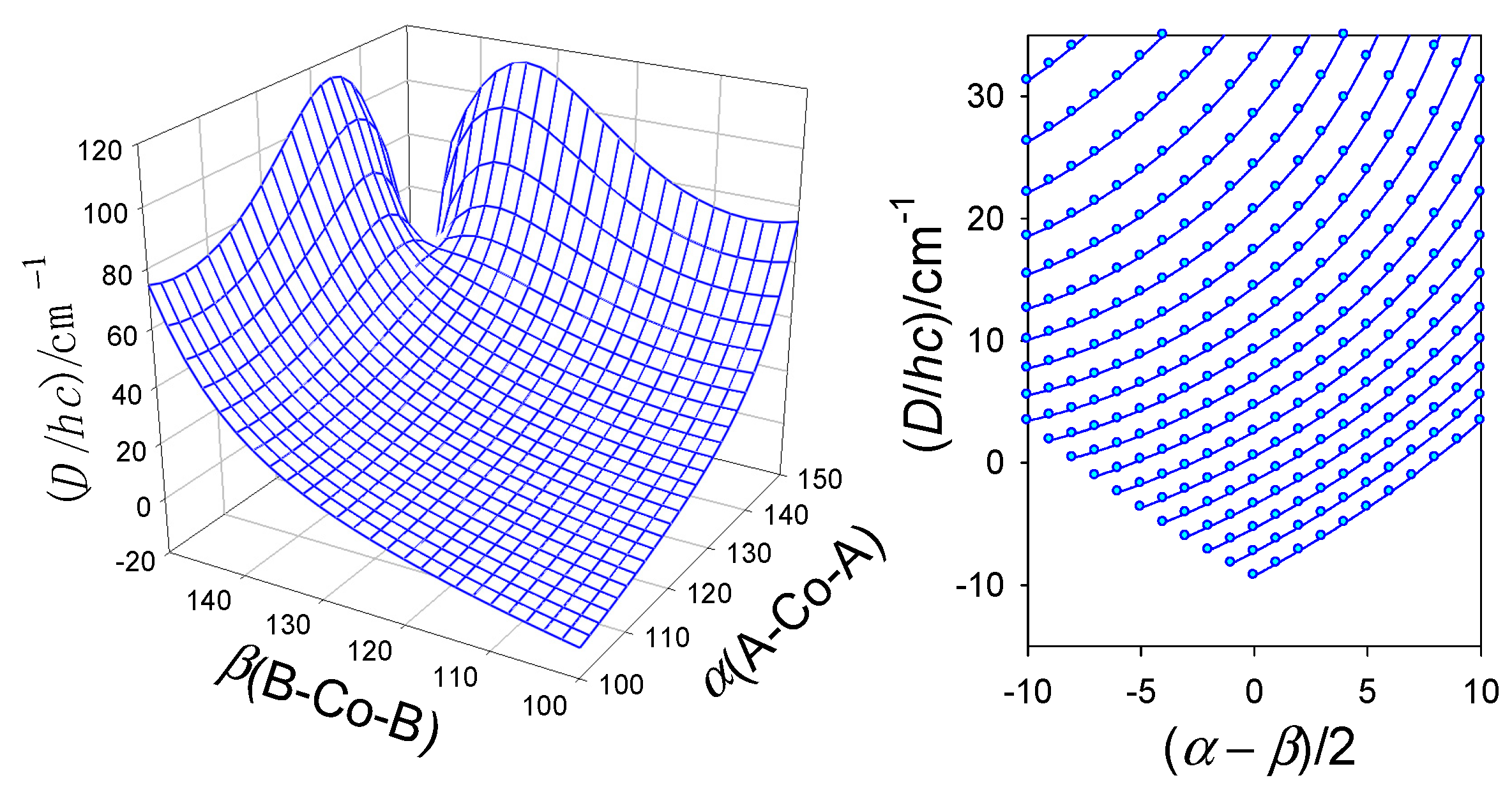
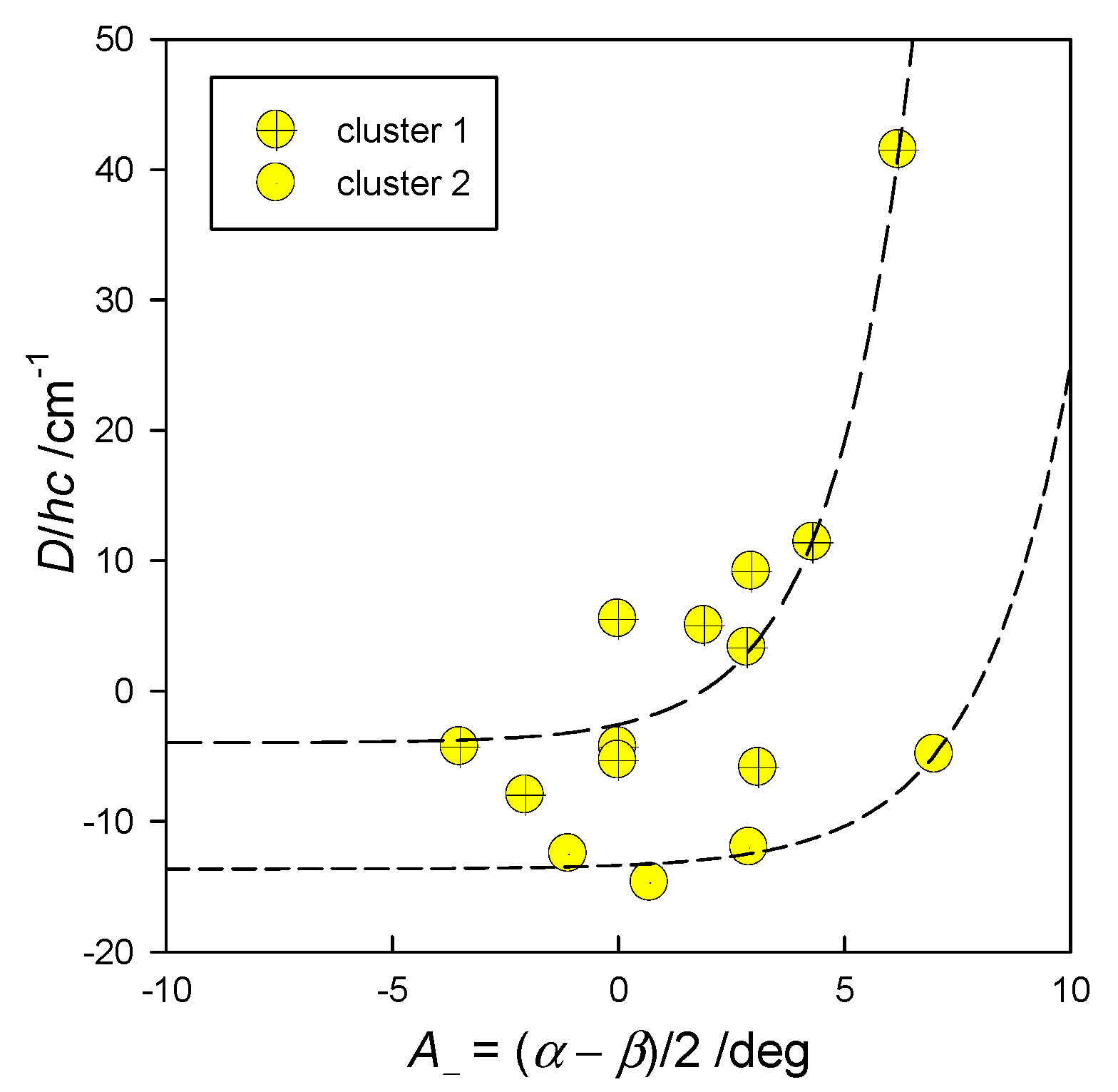
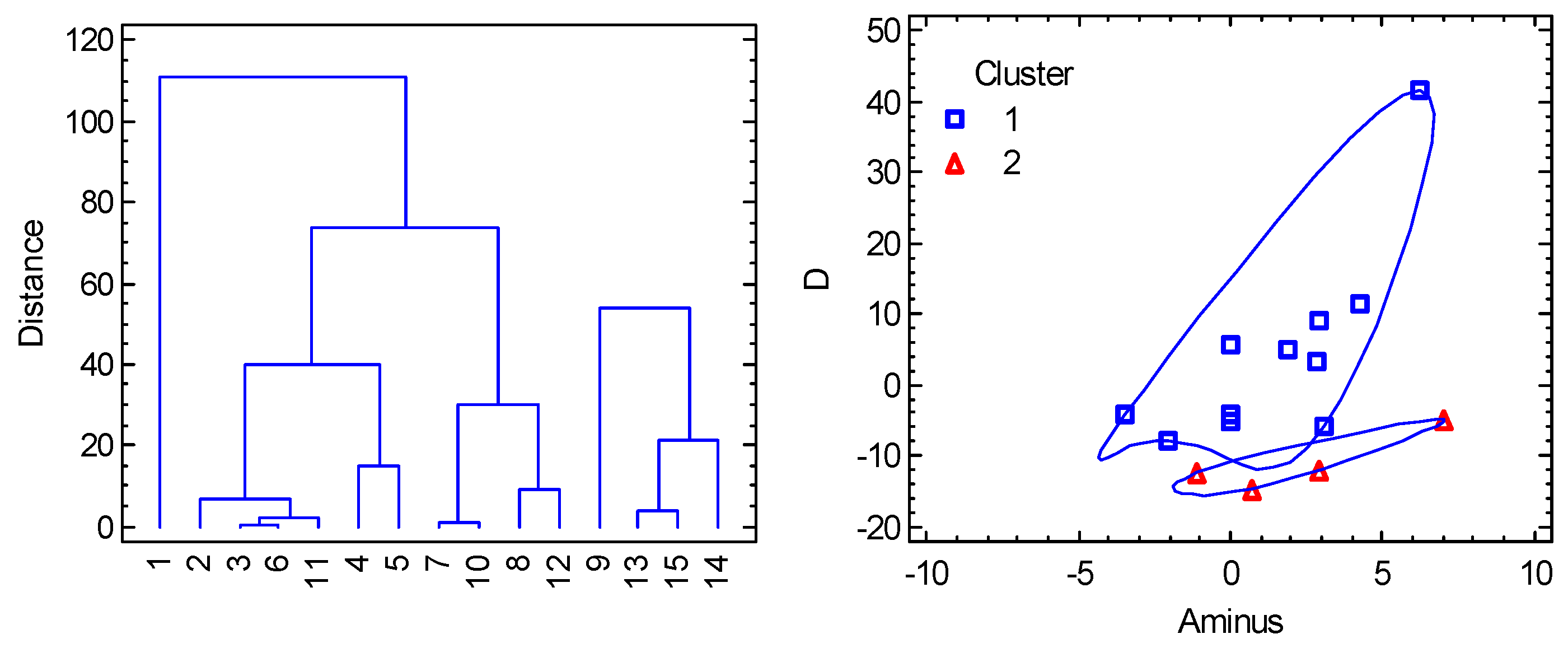
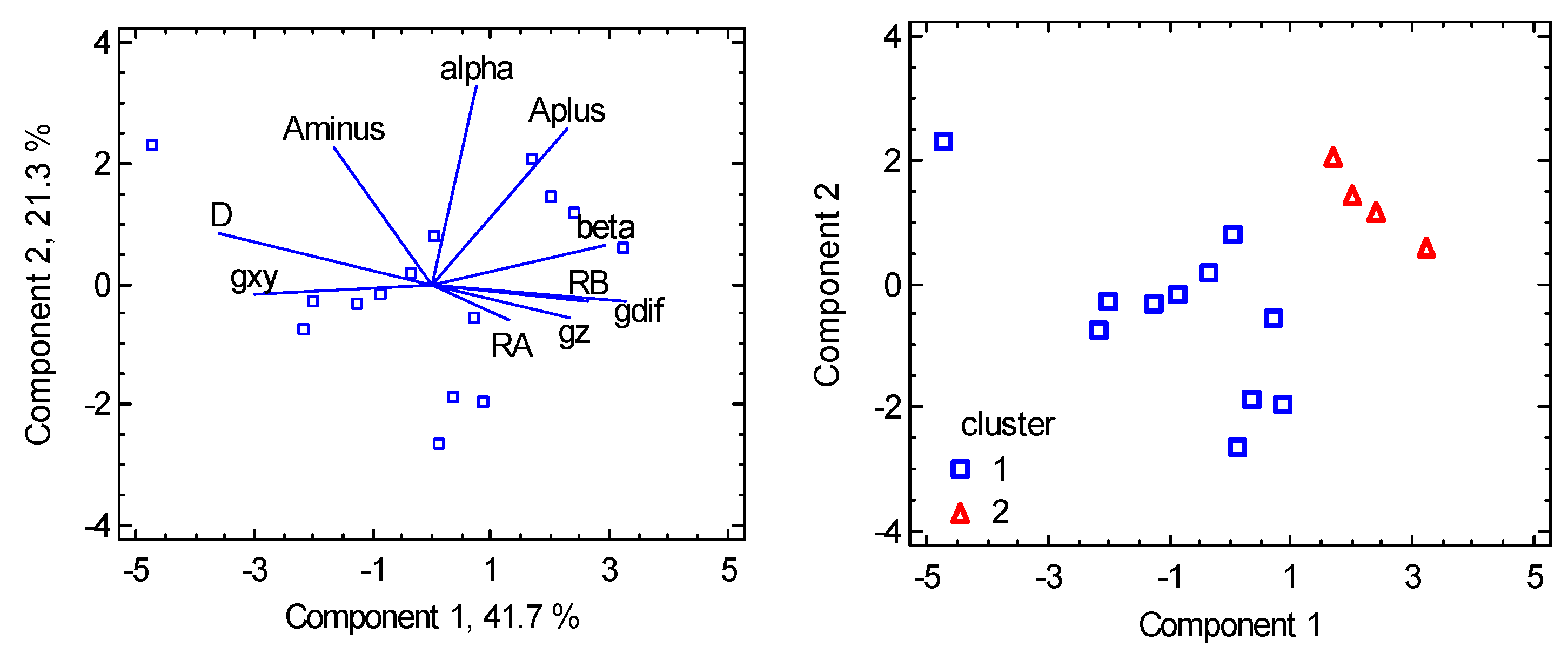
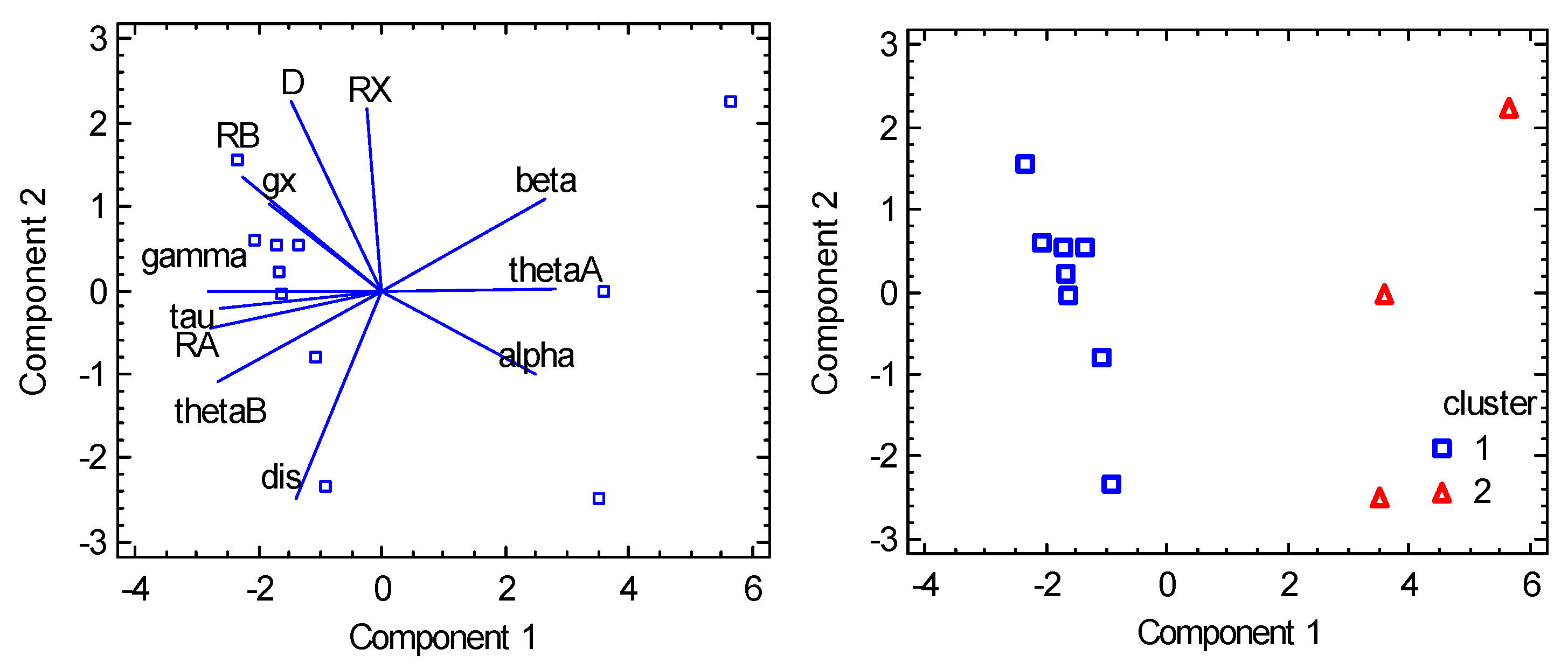
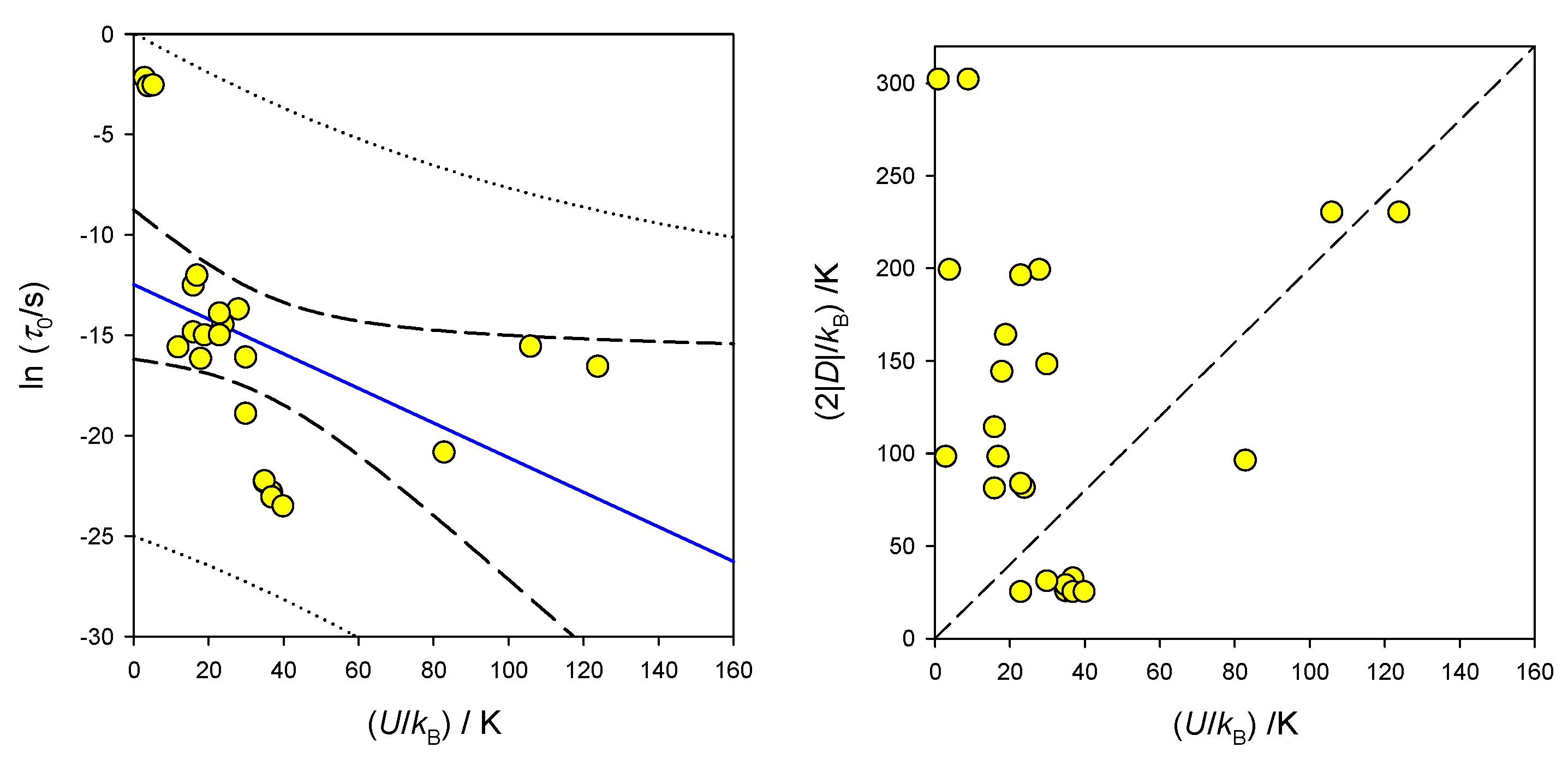
C.n.	Chromophore	Geometry a	(D/hc)/cm−1	(E/hc)/cm−1	BDC/T	(U/kB)/K	τ0/s	Ref.
3	{CoN3}		−57	12.7	0.08	16	3.5 × 10−7	[82]
	{CoN2O}		−72	13.5	0.06	18	9.3 × 10−8	[82]
	{CoN2P}		−82		0.075	19	3.0 × 10−7	[82]
4	{CoS4}		−74		0.10	30	1.0 × 10−7	[83]
	{CoN3Cl}		+12.7	1.2	0.15	35	2 × 10−10	[84]
	{CoP2Cl2}		−16.2	0.9	0.10	37	1.2 × 10−10	[85]
	{CoP2Cl2}		−14.4	1.7	0.10	35	2.1 × 10−10	[85]
	{CoP2Cl2}		−15.4	1.3	0.10	30	6.0 × 10−9	[85]
	{CoP2Br2}		−12.5		0.10	37	9.4 × 10−11	[86]
					0.20	40	6.0 × 10−11	[86]
5	{CoN3N2}	4py	−40.5	J > 0	0.20	16	3.6 × 10−6	[86]
	{CoN3N2}	4py	−40.6		0.20	24	5.1 × 10−7	[72]
	{CoN3N2}	3bpy	(+49.0), calc		0.06	17	5.85 × 10−6	[90]
					0.56	3	0.110	[90]
	{CoN3Cl2}	4py	(+99.5), calc		0.06	28	1.1 × 10−6	[90]
					0.56	4	0.074	[90]
	{CoN3Cl2}2	4py	151	11.6, J > 0	0.20	9	3.1 × 10−7	[69]
					0.20	1	0.27	[69]
6	{CoN4N2}		+48	13.0	0.30	83	8.7 × 10−10	[64]
	{CoN4N2}		+98	8.4	0.10	23	3.0 × 10−7	[72]
	{CoO3N3}		+41.7	1.6	0.10	23	8.9 × 10−7	[88]
	{CoO6}		−115	2.8	0	106	1.7 × 10−7	[89]
					0.15	124	6.3 × 10−8	[89]

^a C.n.—coordination number; 4py—tetragonal pyramid, 3bpy—trigonal bipyramid; calc—estimate from the calculated energy gap between the lowest Kramer doublets as $\Delta =2{(D^2+3E^2)}^{1/2}~2D$.

. It is worth noting that there is an inverse relationship between the extrapolated relaxation time lnτ₀ and barrier to spin reversal U, as shown in Figure 17.

The existence of the barrier to spin reversal is traditionally expected only for D < 0 (easy-axis of magnetization). However, SMM (SIM) behaviour was also registered in the case of D > 0; a presence of sufficient rhombic anisotropy (E > 0) rationalizes this observation. In this case, the easy-plane allowing unhindered relaxation no longer exists; a small barrier to spin reversal is determined by the E parameter.

The above outlined magnetostructural correlations allow us to predict whether the system under study is a potential candidate for single-molecule magnetism. For instance, the observed value of D/hc = −12.5 cm⁻¹ in a [Co(PPh₃)₂Br₂] complex from the DC magnetization studies motivated the AC susceptibility measurements and, indeed, the SMM behaviour was confirmed [86].

The expected relationship for the half-integral spin S = 3/2 is $U=2\left|D_S\right|$ (in the zero field), and this is evidently not obeyed (Figure 17). The way of extracting the SMM parameters is also questionable: usually, they arise from the Arrhenius-like linear relationship $\ln \tau =\ln {\tau }_0+(U/k_{\mathrm{B}})T^{-1}$ applied to higher-temperature points. However, a more complex equation that involves the Raman, direct, and quantum tunnelling processes is available

$${\tau }^{-1}={\tau }_0^{-1}\exp \left(-U/k_{\mathrm{B}}T\right)+C{T}^n+A{B}^{2}T+D(B)$$

(38)

This can be enriched by the phonon bottleneck effect $F{T}^2$ and the reciprocating thermal behaviour term $G{T}^{-1}$.

Some samples exhibit multiple relaxation processes that vary with the applied magnetic field, so it is problematic to relate single D-values with different U-values. The presence of various relaxation processes is visible only if a search for them is applied, e.g., for low (ν < 1 Hz) or high (ν > 1500 Hz) frequencies of the AC field. Co(II) complexes represent a rich class of single-ion magnets. These exhibit large magnetic anisotropy that necessarily is positive for a compressed tetragonal bipyramid. The Orbach relaxation mechanism, however, requires D < 0. For (pseudo)octahedral systems, we found that the ZFS parameter D, and thus magnetic anisotropy, strongly decreases with tetragonal distortion. For near-octahedral structures, the D-parameter achieves large positive values (up to 350 cm⁻¹ for ideal O_h—Jahn–Teller systems). Hexacoordinate Co(II) complexes with markedly negative D-values were also reported [91,92]. An example is also (HNEt₃)⁺(Co^IICo^III₃L₆)⁻, D/hc = −115 cm⁻¹. However, the symmetry of this structure is D₃ [89].

8. Summary

Magnetostructural D-correlations, i.e., dependence of the axial zero-field splitting parameter D vs. the structural anisotropy parameter D_str, originate in the electronic structure of the central atom. They are tuned by the ligand diversity and structural variability in the individual metal complexes. Intuitive linear relationships (as in hexacoordinate Ni(II) systems) are not always supported with deep theoretical analysis, which can rationalize various types of non-linear dependencies. This is the case of the hexa-, penta-, and tetracoordinate Co(II) complexes. Magnetostructural D-correlations represent an effective tool for the design of magnetically anisotropic molecules. Only from the knowledge of the structural characteristics of the complexes can the magnitude and sign of the axial zero-field splitting parameter D be predicted, which enables the creation of relevant prediction models using contemporary machine learning methods [93].