Development of High Refractive Index Polydimethylsiloxane Waveguides Doped with Benzophenone via Solvent-Free Fabrication for Biomedical Pressure Sensing
Abstract
1. Introduction
2. Materials and Methods
2.1. Refractive Index Measurement
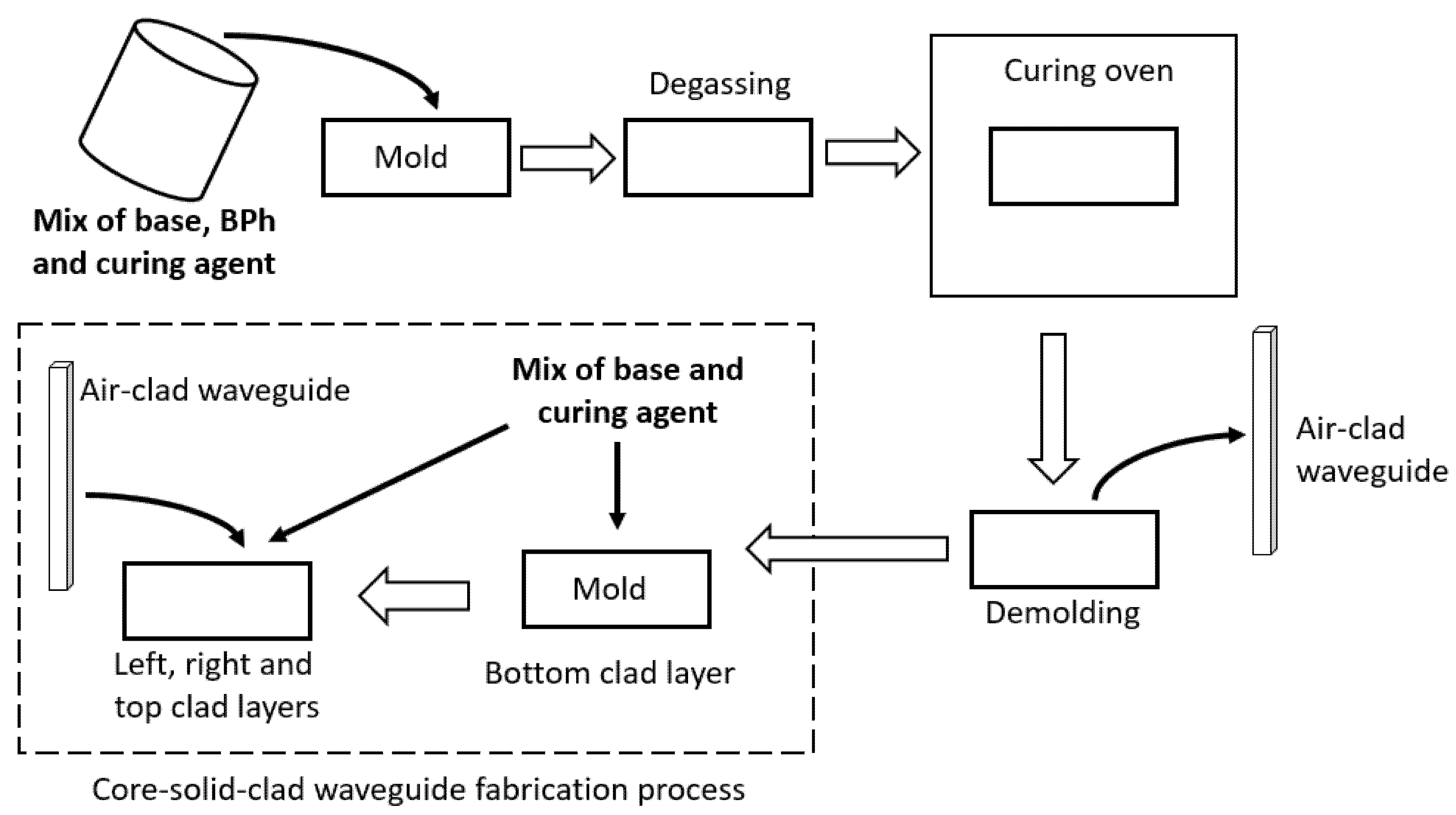
2.2. Waveguide Fabrication
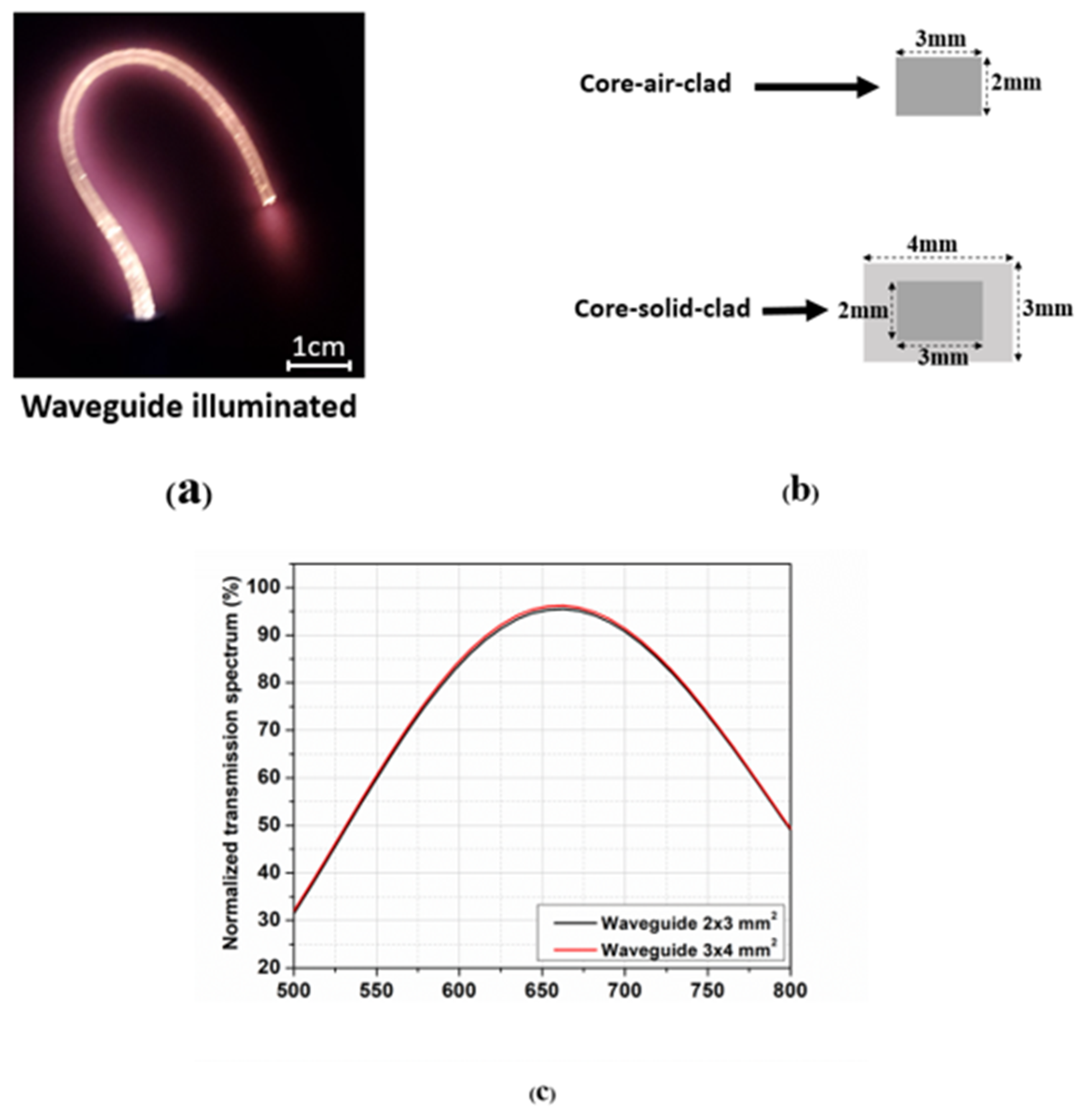
3. Characterization of Waveguides
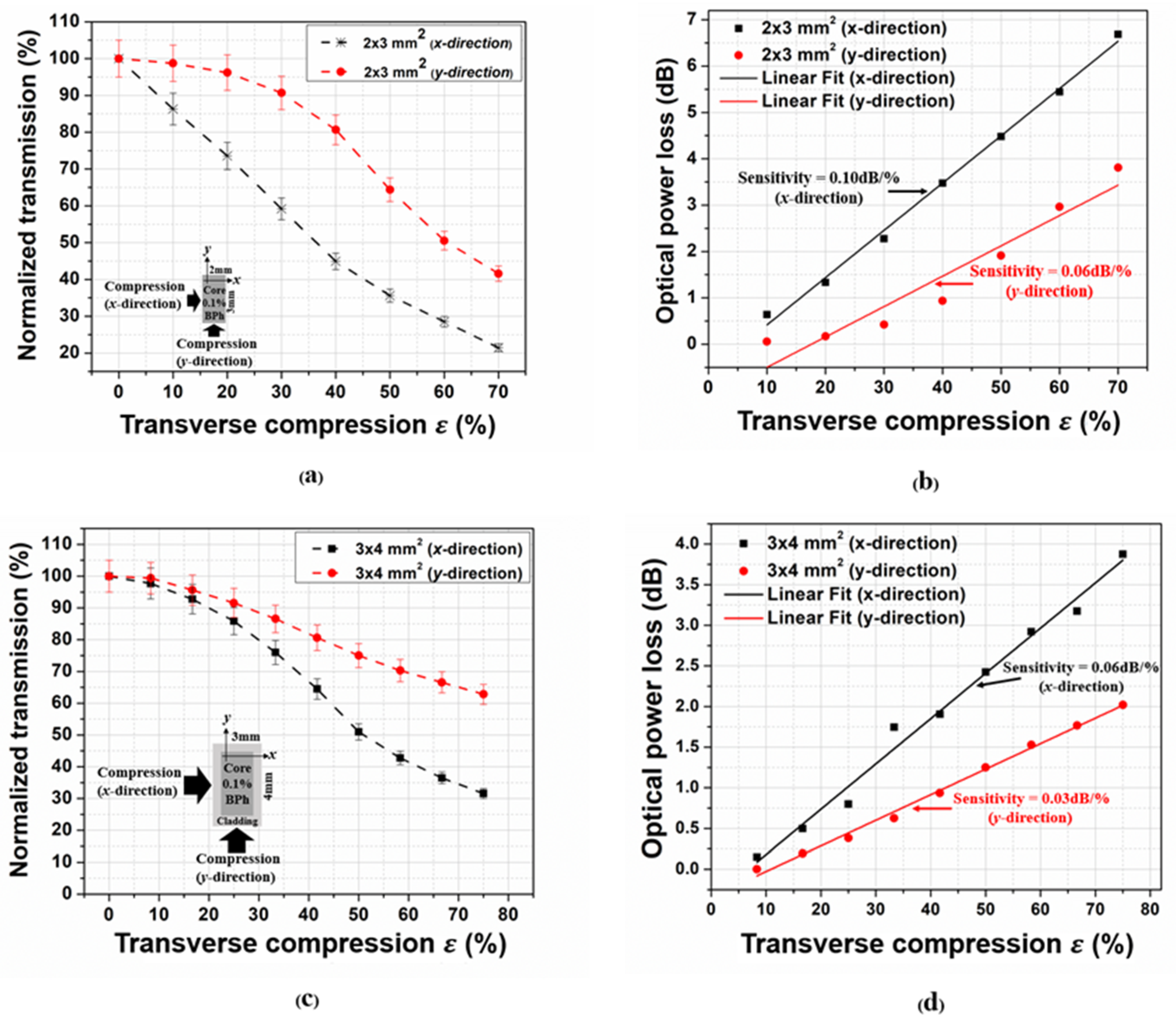
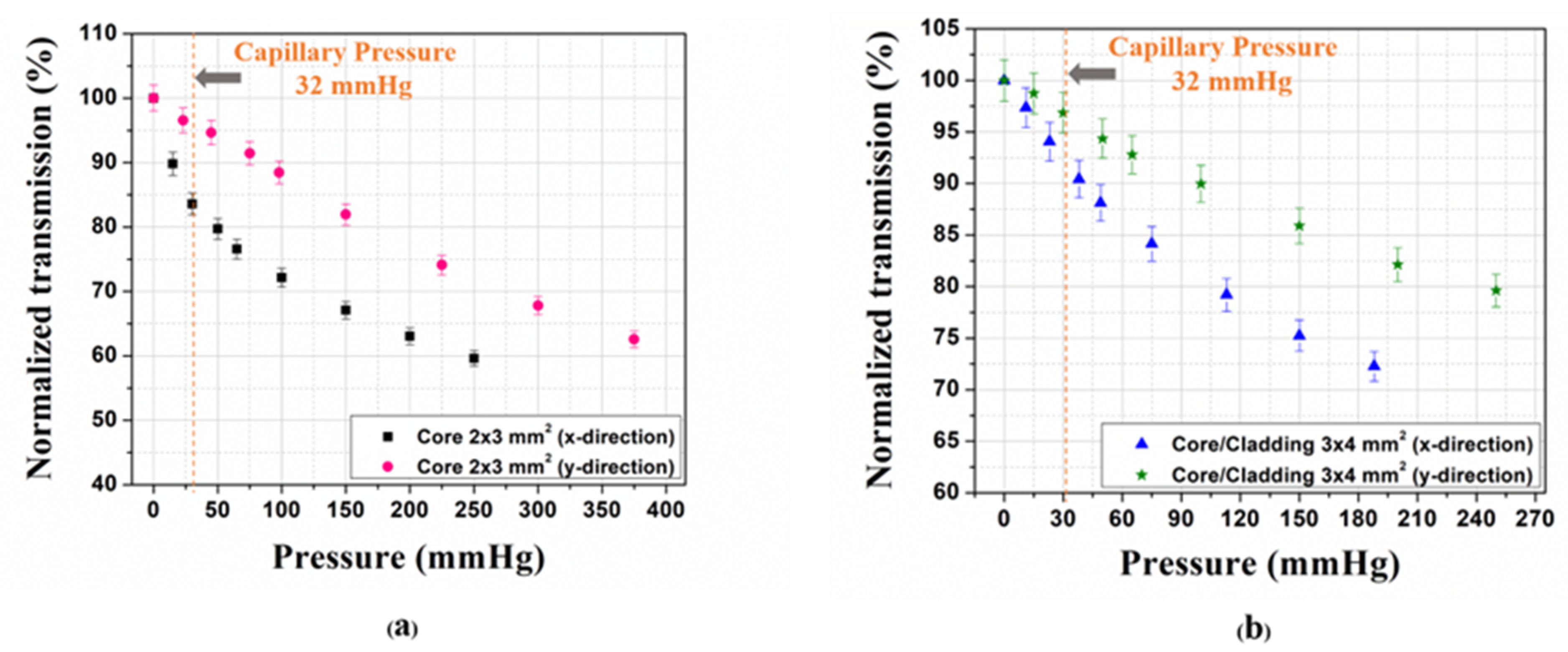
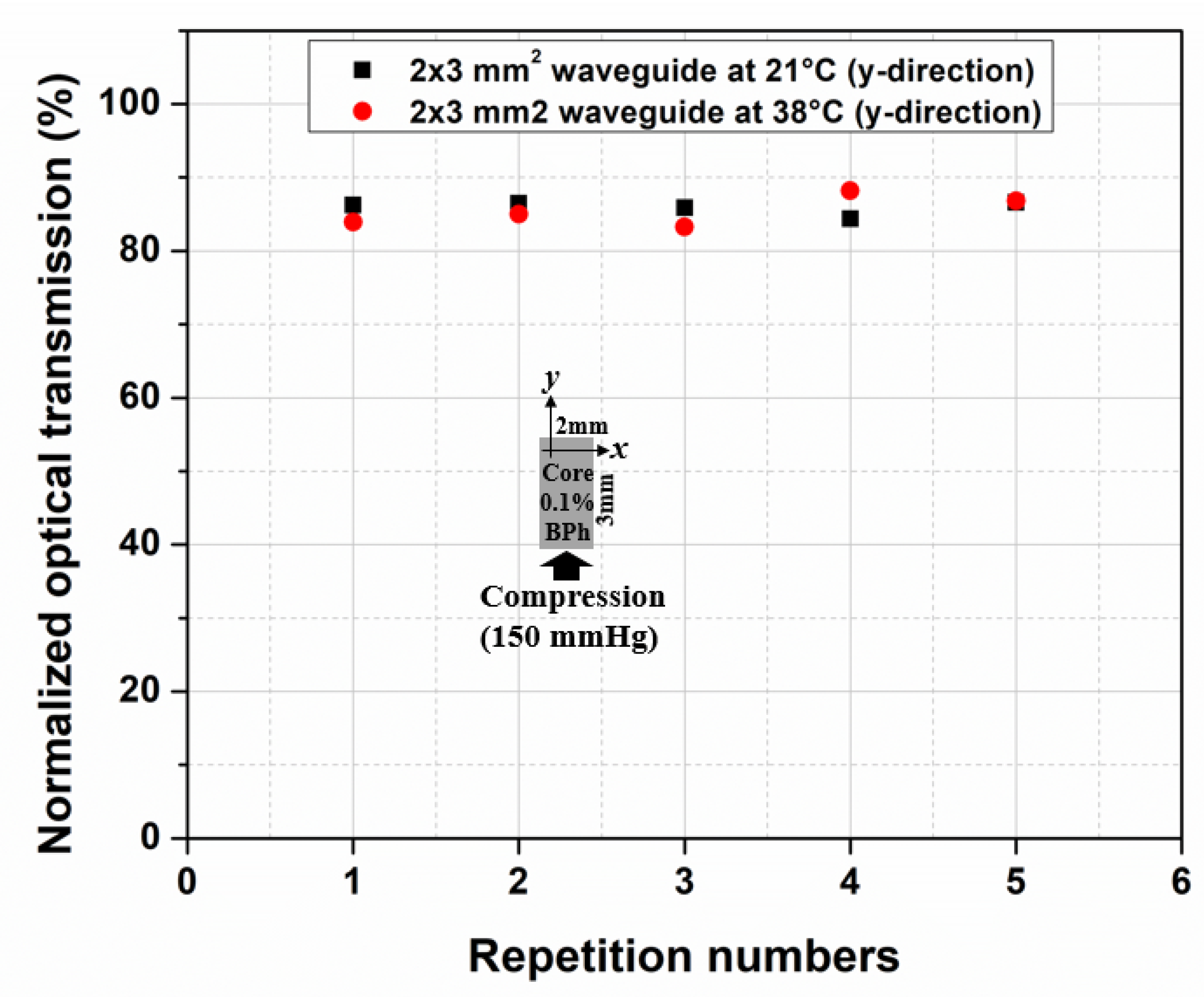
3.1. Transverse Compression
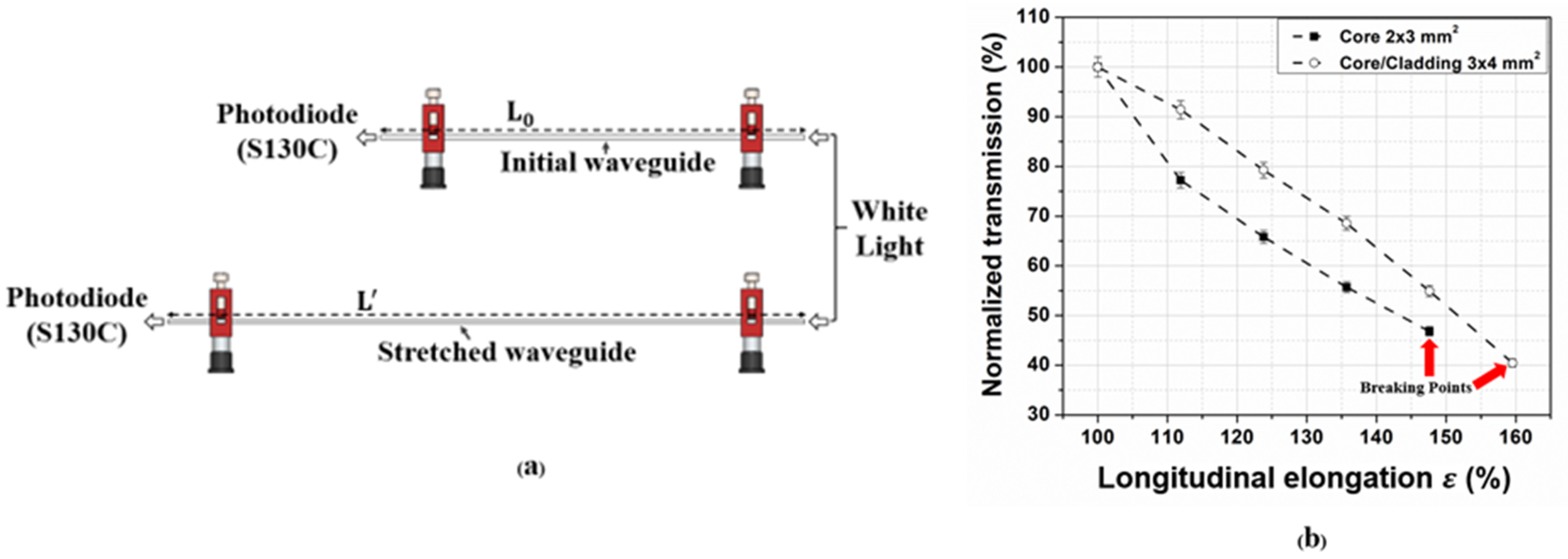
3.2. Longitudinal Elongation
3.3. Bending and Propagation Loss Measurements
3.4. Waveguide Thermal Stability
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Butt, M.A.; Kazanskiy, N.L.; Khonina, S.N. Revolution in Flexible Wearable Electronics for Temperature and Pressure Monitoring—A Review. Electronics 2022, 11, 716. [Google Scholar] [CrossRef]
- Farooq, M.; Iqbal, T.; Vazquez, P.; Farid, N.; Thampi, S.; Wijns, W.; Shahzad, A. Thin-Film Flexible Wireless Pressure Sensor for Continuous Pressure Monitoring in Medical Applications. Sensors 2020, 20, 6653. [Google Scholar] [CrossRef] [PubMed]
- Fong, D.T.-P.; Chan, Y.-Y.; Hong, Y.; Yung, P.S.-H.; Fung, K.-Y.; Chan, K.-M. A three-pressure-sensor (3PS) system for monitoring ankle supination torque during sport motions. J. Biomech. 2008, 41, 2562–2566. [Google Scholar] [CrossRef]
- Li, L.; Zheng, J.; Chen, J.; Luo, Z.; Su, Y.; Tang, W.; Gao, X.; Li, Y.; Cao, C.; Liu, Q.; et al. Flexible Pressure Sensors for Biomedical Applications: From Ex Vivo to In Vivo. Adv. Mater. Interfaces 2020, 7, 2000743. [Google Scholar] [CrossRef]
- Meng, K.; Xiao, X.; Wei, W.-X.; Chen, G.-R.; Nashalian, A.; Shen, S.; Chen, J. Wearable Pressure Sensors for Pulse Wave Monitoring. Adv. Mater. 2022, 34, 2109357. [Google Scholar] [CrossRef]
- Rum, L.; Sten, O.; Vendrame, E.; Belluscio, V.; Camomilla, V.; Vannozzi, G.; Truppa, L.; Notarantonio, M.; Sciarra, T.; Lazich, A.; et al. Wearable Sensors in Sports for Persons with Disability: A Systematic Review. Sensors 2021, 21, 1858. [Google Scholar] [CrossRef] [PubMed]
- Shen, G. Recent advances of flexible sensors for biomedical applications. Prog. Nat. Sci. Mater. Int. 2021, 31, 872–882. [Google Scholar] [CrossRef]
- Zhang, J.-W.; Zhang, Y.; Li, Y.-Y.; Wang, P. Textile-Based Flexible Pressure Sensors: A Review. Polym. Rev. 2022, 62, 65–94. [Google Scholar] [CrossRef]
- Chenu, O.; Vuillerme, N.; Bucki, M.; Diot, B.; Cannard, F.; Payan, Y. TexiCare: An innovative embedded device for pressure ulcer prevention. Preliminary results with a paraplegic volunteer. J. Tissue Viability 2013, 22, 83–90. [Google Scholar]
- Chhetry, A.; Yoon, H.; Park, J.Y. A flexible and highly sensitive capacitive pressure sensor based on conductive fibers with a microporous dielectric for wearable electronics. J. Mater. Chem. C 2017, 5, 10068–10076. [Google Scholar] [CrossRef]
- Choi, D.; Jang, S.; Kim, J.S.; Kim, H.-J.; Kim, D.H.; Kwon, J.-Y. A Highly Sensitive Tactile Sensor Using a Pyramid-Plug Structure for Detecting Pressure, Shear Force, and Torsion. Adv. Mater. Technol. 2019, 4, 1800284. [Google Scholar] [CrossRef]
- Hayn, D.; Falgenhauer, M.; Morak, J.; Wipfler, K.; Willner, V.; Liebhart, W.; Schreier, G. An eHealth System for Pressure Ulcer Risk Assessment Based on Accelerometer and Pressure Data. J. Sens. 2015, 2015, 106537. [Google Scholar] [CrossRef][Green Version]
- Homayounfar, S.Z.; Andrew, T.L. Wearable Sensors for Monitoring Human Motion: A Review on Mechanisms, Materials, and Challenges. SLAS Technol. Transl. Life Sci. Innov. 2020, 25, 9–24. [Google Scholar] [CrossRef] [PubMed]
- Owings, T.M.; Apelqvist, J.; Stenström, A.; Becker, M.; Bus, S.A.; Kalpen, A.; Ulbrecht, J.S.; Cavanagh, P.R. Plantar pressures in diabetic patients with foot ulcers which have remained healed. Diabet. Med. 2009, 26, 1141–1146. [Google Scholar] [CrossRef] [PubMed]
- Chen, W.F.; Yan, X. Progress in achieving high-performance piezoresistive and capacitive flexible pressure sensors. J. Mater. Sci. Technol. 2020, 43, 175–188. [Google Scholar] [CrossRef]
- Correia, R.; James, S.W.; Lee, S.-W.; Morgan, S.P.; Korposh, S. Biomedical application of optical fibre sensors. J. Opt. 2018, 20, 073003. [Google Scholar] [CrossRef]
- Mishra, S.; Mac-Thiong, J.-M.; Wagnac, E.; Petit, Y.; Ung, B. A Sensitive and Fast Fiber Bragg Grating-Based Investigation of the Biomechanical Dynamics of In Vitro Spinal Cord Injuries. Sensors 2021, 21, 1671. [Google Scholar] [CrossRef]
- Roriz, P.; Frazão, O.; Ribeiro, A.L.; Santos, J.L.; Simões, J.A. Review of fiber-optic pressure sensors for biomedical and biomechanical applications. J. Biomed. Opt. 2013, 18, 050903. [Google Scholar] [CrossRef]
- Wang, W.-C.; LeDoux, W.R.; Sangeorzan, B.J.; Reinhall, P.G. A shear and plantar pressure sensor based on fiber-optic bend loss. J. Rehabil. Res. Dev. 2005, 42, 315–326. [Google Scholar] [CrossRef]
- Zhang, X.Y.; Yang, L.Z.Y. A fiber Bragg grating quasi distributed sensing network with a wavelength-tunable chaotic fiber laser. Syst. Sci. Control. Eng. An Open Access J. 2014, 2, 268–274. [Google Scholar] [CrossRef]
- Arute, V.K.; Syed, A.; Khandelwal, A. Time–space–weight calibrated plastic optical fiber-based pressure sensing carpet. Opt. Eng. 2021, 60, 094106. [Google Scholar] [CrossRef]
- Shibata, Y.; Nishimura, A.; Niwa, S.; Osawa, Y.; Uemiya, T. Optical Sensors. U.S. Patent 4,750,796, 14 June 1988. [Google Scholar]
- Begej, S. Planar and finger-shaped optical tactile sensors for robotic applications. IEEE Trans. Robot. Autom 1988, 4, 472–484. [Google Scholar] [CrossRef]
- Kookootsedes, G.J.; Reese, H.H.; Gutek, B.I.; Pretzer, G.H. Touch position sensitive optical waveguides. U.S. Patent 4,701,017, 20 October 1987. [Google Scholar]
- Lagakos, N.; Schnaus, E.; Cole, J.; Jarzynski, J.; Bucaro, J. Optimizing fiber coatings for interferometric acoustic sensors. IEEE Trans. Microw. Theory Tech. 1982, 30, 529–535. [Google Scholar] [CrossRef]
- Lieberman, R.A.; Blyler, L.L.; Cohen, L.G. A distributed fiber optic sensor based on cladding fluorescence. J. Lightwave Technol. 1990, 8, 212–220. [Google Scholar] [CrossRef]
- Schueller, O.J.A.; Zhao, X.-M.; Whitesides, G.M.; Smith, S.P.; Prentiss, M. Fabrication of Liquid-Core Waveguides by Soft Lithography. Adv. Mater. 1999, 11, 37–41. [Google Scholar] [CrossRef]
- Babar Jamil, Y.C. Soft Optical Waveguide Sensors Tuned by Reflective Pigmentation for Robotic Applications. J. Korea Robot. Soc. 2021, 16, 1–11. [Google Scholar] [CrossRef]
- Bai, H.; Li, S.; Barreiros, J.; Tu, Y.; Pollock, C.R.; Shepherd, R.F. Stretchable distributed fiber-optic sensors. Science 2020, 370, 848–852. [Google Scholar] [CrossRef]
- Guo, J.; Niu, M.; Yang, C. Highly flexible and stretchable optical strain sensing for human motion detection. Optica 2017, 4, 1285–1288. [Google Scholar] [CrossRef]
- Harnett, C.K.; Zhao, H.; Shepherd, R.F. Stretchable Optical Fibers: Threads for Strain-Sensitive Textiles. Adv. Mater. Technol. 2017, 2, 1700087. [Google Scholar] [CrossRef]
- Ramuz, M.; Tee, B.C.-K.; Tok, J.B.-H.; Bao, Z. Transparent, Optical, Pressure-Sensitive Artificial Skin for Large-Area Stretchable Electronics. Adv. Mater. 2012, 24, 3223–3227. [Google Scholar] [CrossRef]
- Sandt, J.D.; Moudio, M.; Clark, J.K.; Hardin, J.; Argenti, C.; Carty, M.; Lewis, J.A.; Kolle, M. Stretchable Optomechanical Fiber Sensors for Pressure Determination in Compressive Medical Textiles. Adv. Healthc. Mater. 2018, 7, e1800293. [Google Scholar] [CrossRef] [PubMed]
- Surapaneni, R.; Park, K.; Suster, M.; Young, D.; Mastrangelo, C.H. A highly sensitive flexible pressure and shear sensor array for measurement of ground reactions in pedestrian navigation. In Proceedings of the 2011 16th International Solid-State Sensors, Actuators and Microsystems Conference, Beijing, China, 5–9 June 2011. [Google Scholar]
- To, C.; Hellebrekers, T.L.; Park, Y. Highly stretchable optical sensors for pressure, strain, and curvature measurement. Presented at 2015 IEEE/RSJ International Conference on Intelligent Robots and Systems (IROS), Hamburg, Germany, 28 September 2015. [Google Scholar]
- Toyama, S.; Tanaka, Y.; Shirogane, S.; Nakamura, T.; Umino, T.; Uehara, R.; Okamoto, T.; Igarashi, H. Development of Wearable Sheet-Type Shear Force Sensor and Measurement System that is Insusceptible to Temperature and Pressure. Sensors 2017, 17, 1752. [Google Scholar]
- Zhao, H.; O’Brien, K.; Li, S.; Shepherd, R.F. Optoelectronically innervated soft prosthetic hand via stretchable optical waveguides. Sci. Robot. 2016, 1, eaai7529. [Google Scholar] [CrossRef] [PubMed]
- Missinne, J.; Kalathimekkad, S.; Van Hoe, B.; Bosman, E.; Vanfleteren, J.; Van Steenberge, G. Stretchable optical waveguides. Opt. Express 2014, 22, 4168–4179. [Google Scholar] [CrossRef]
- Panusa, G.; Pu, Y.; Wang, J.; Moser, C.; Psaltis, D. Fabrication of Sub-Micron Polymer Waveguides through Two-Photon Polymerization in Polydimethylsiloxane. Polymers 2020, 12, 2485. [Google Scholar] [CrossRef]
- Prajzler, V.; Neruda, M.; Nekvindová, P. Flexible multimode polydimethyl-diphenylsiloxane optical planar waveguides. J. Mater. Sci. Mater. Electron. 2018, 29, 5878–5884. [Google Scholar] [CrossRef]
- Miranda, I.; Souza, A.; Sousa, P.; Ribeiro, J.; Castanheira, E.M.S.; Lima, R.; Minas, G. Properties and Applications of PDMS for Biomedical Engineering: A Review. J. Funct. Biomater. 2022, 13, 2. [Google Scholar] [CrossRef]
- Ryabchun, A.; Sakhno, O.; Wegener, M. Conventional elastomers doped with benzophenone derivatives as effective media for all-optical fabrication of tunable diffraction elements. RSC Adv. 2016, 6, 51791–51800. [Google Scholar] [CrossRef]
- Kasap, S.O. Optoelectronics and Photonics; Pearson Education: London, UK, 2013. [Google Scholar]
- Nemoto, S. Measurement of the refractive index of liquid using laser beam displacement. Appl. Opt. 1992, 31, 6690–6694. [Google Scholar] [CrossRef]
- Sengupta, D.; Ung, B. Simple optical setup for the undergraduate experimental measurement of the refractive indices and attenuation coefficient of liquid samples and characterization of laser beam profile. In Proceedings of the Fifteenth Conference on Education and Training in Optics and Photonics: ETOP 2019, Quebec City, QC, Canada, 21–24 May 2019; Optica Publishing Group: Quebec, QC, Canada, 2019. [Google Scholar]
- Bute, M.G.; Shinde, S.D.; Bodas, D.; Fouad, H.; Adhi, K.P.; Gosavi, S.W. Benzophenone doped polydimethylsiloxane: Self developable composite resist system for its use in a direct write laser lithography application. J. Phys. D Appl. Phys. 2015, 48, 175301. [Google Scholar] [CrossRef]
- Kant, M.B.; Shinde, S.D.; Bodas, D.; Patil, K.; Sathe, V.; Adhi, K.; Gosavi, S. Surface studies on benzophenone doped PDMS microstructures fabricated using KrF excimer laser direct write lithography. Appl. Surf. Sci. 2014, 314, 292–300. [Google Scholar] [CrossRef]




Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Amouzou, K.N.; Romero, A.A.; Sengupta, D.; Mishra, S.K.; Richard-Denis, A.; Mac-Thiong, J.-M.; Petit, Y.; Lina, J.-M.; Ung, B. Development of High Refractive Index Polydimethylsiloxane Waveguides Doped with Benzophenone via Solvent-Free Fabrication for Biomedical Pressure Sensing. Photonics 2022, 9, 557. https://doi.org/10.3390/photonics9080557
Amouzou KN, Romero AA, Sengupta D, Mishra SK, Richard-Denis A, Mac-Thiong J-M, Petit Y, Lina J-M, Ung B. Development of High Refractive Index Polydimethylsiloxane Waveguides Doped with Benzophenone via Solvent-Free Fabrication for Biomedical Pressure Sensing. Photonics. 2022; 9(8):557. https://doi.org/10.3390/photonics9080557
Chicago/Turabian StyleAmouzou, Koffi Novignon, Alberto Alonso Romero, Dipankar Sengupta, Satyendra Kumar Mishra, Andréane Richard-Denis, Jean-Marc Mac-Thiong, Yvan Petit, Jean-Marc Lina, and Bora Ung. 2022. "Development of High Refractive Index Polydimethylsiloxane Waveguides Doped with Benzophenone via Solvent-Free Fabrication for Biomedical Pressure Sensing" Photonics 9, no. 8: 557. https://doi.org/10.3390/photonics9080557
APA StyleAmouzou, K. N., Romero, A. A., Sengupta, D., Mishra, S. K., Richard-Denis, A., Mac-Thiong, J.-M., Petit, Y., Lina, J.-M., & Ung, B. (2022). Development of High Refractive Index Polydimethylsiloxane Waveguides Doped with Benzophenone via Solvent-Free Fabrication for Biomedical Pressure Sensing. Photonics, 9(8), 557. https://doi.org/10.3390/photonics9080557






