Improved Accuracy for Measurement of Filament Diameter Based on Image-Based Fitting Method
Abstract
:1. Introduction
2. Fraunhofer Diffraction
3. Experiments and Results
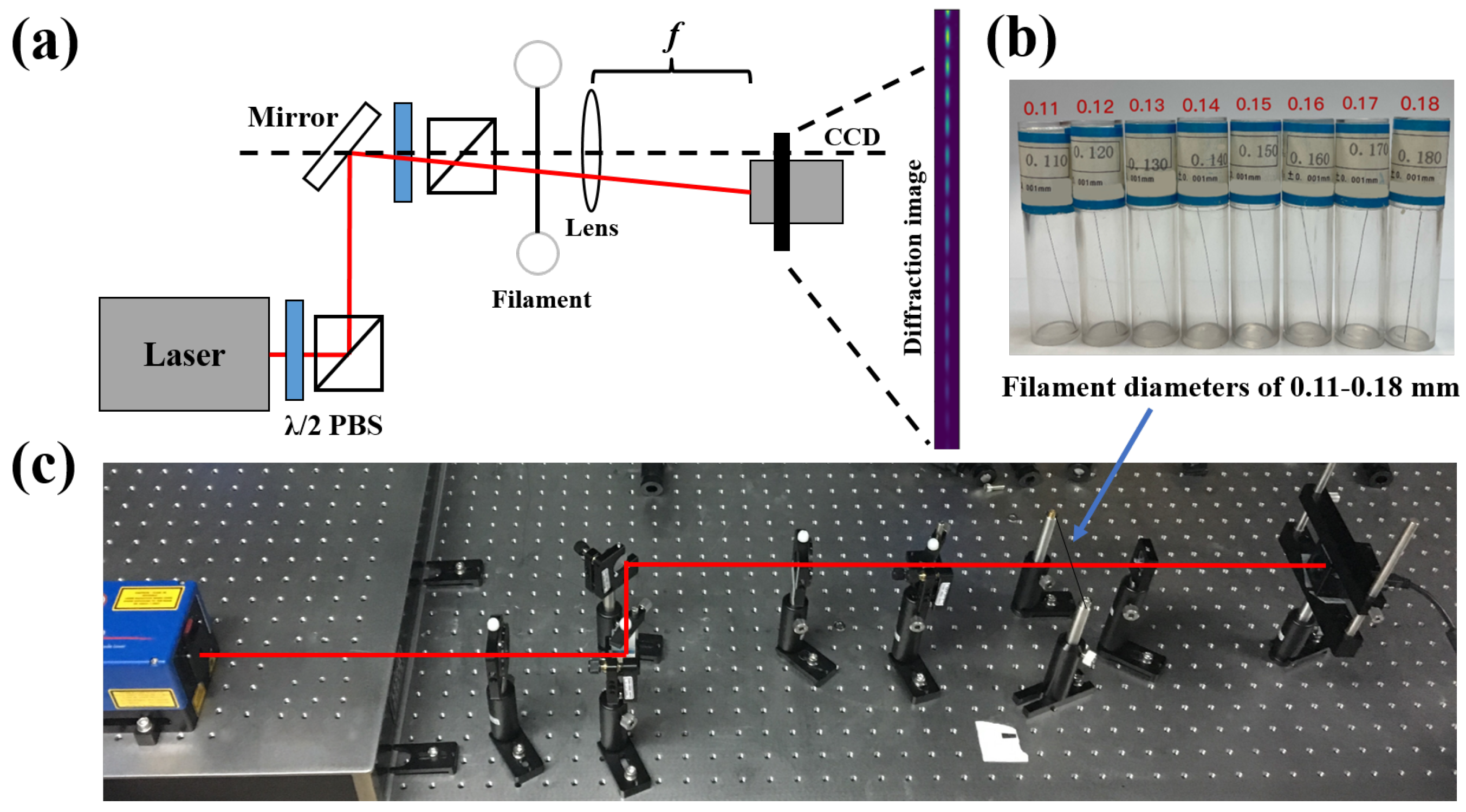
3.1. Experiments
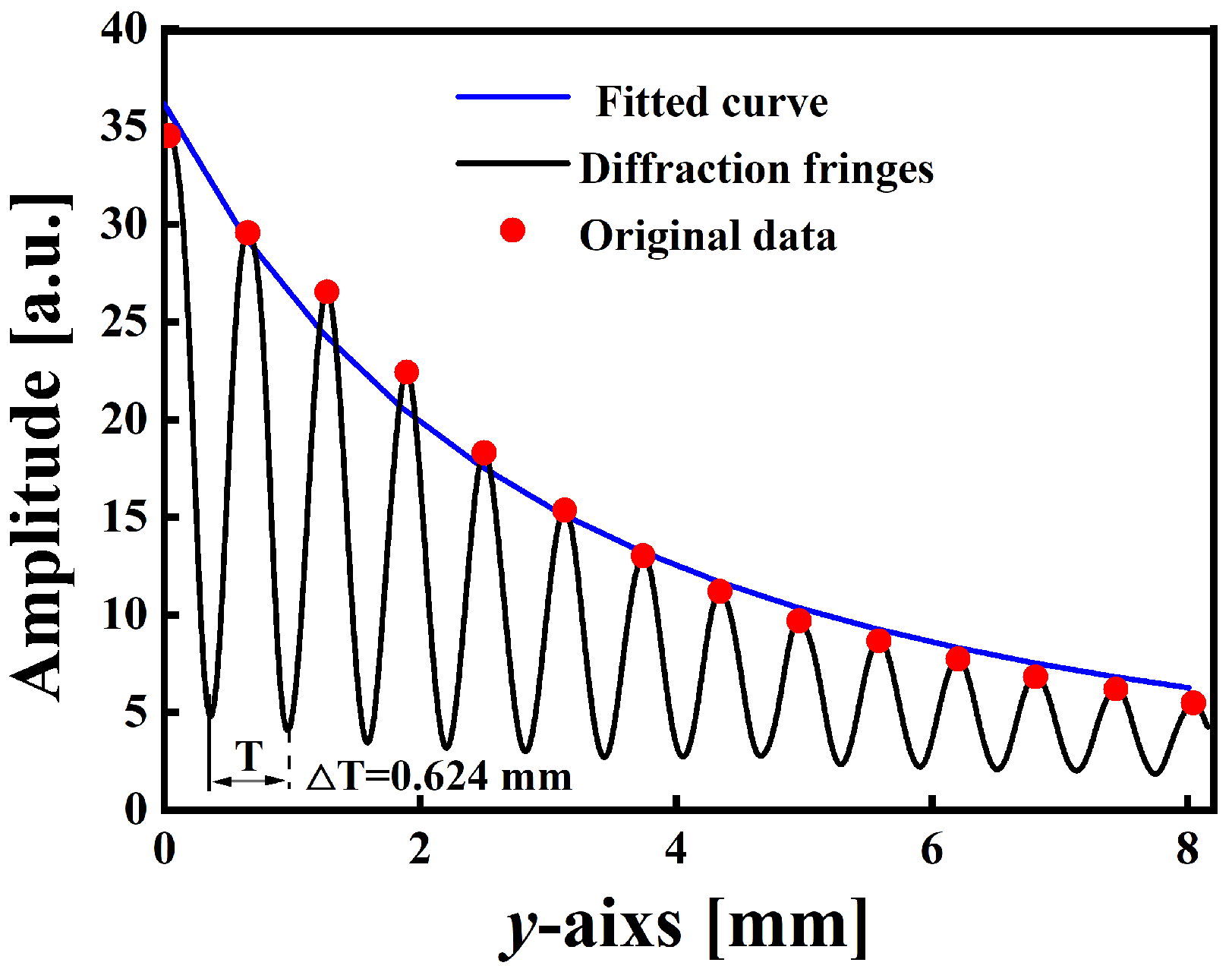
3.2. Methods of Image-Based Fitting
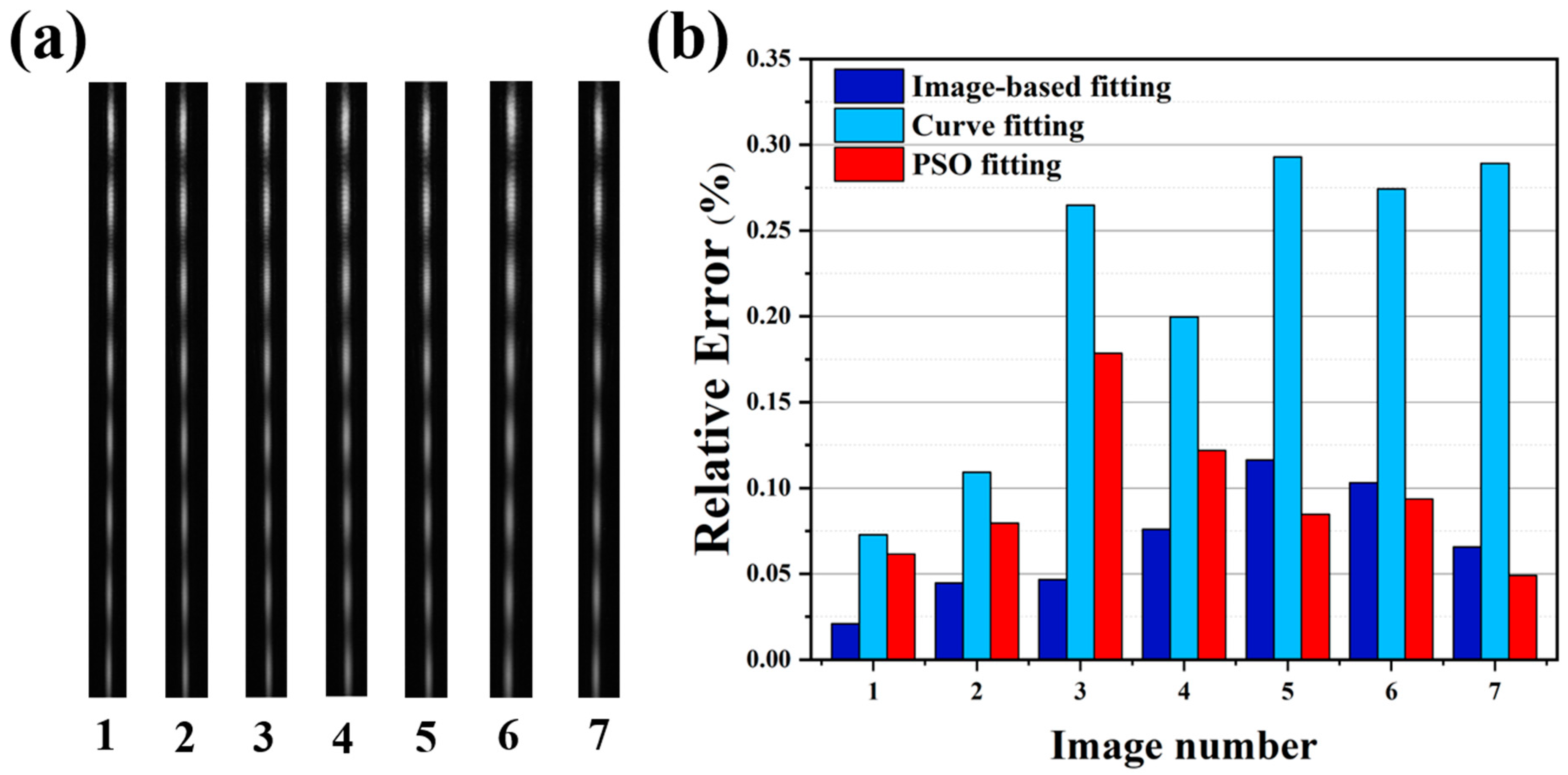
4. Results
5. Discussion
5.1. Analysis of Accuracy
5.2. Improvement of the Fitted Equation
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ma, Z.; Merkus, H.G.; de Smet, J.G.; Heffels, C.; Scarlett, B. New developments in particle characterization by laser diffraction: Size and shape. Powder Technol. 2000, 111, 66–78. [Google Scholar] [CrossRef]
- Agrawal, Y.; Whitmire, A.; Mikkelsen, O.A.; Pottsmith, H. Light scattering by random shaped particles and consequences on measuring suspended sediments by laser diffraction. J. Geophys. Res. Ocean. 2008, 113, C4. [Google Scholar] [CrossRef] [Green Version]
- Richter, C.; Zschornak, M.; Novikov, D.; Mehner, E.; Nentwich, M.; Hanzig, J.; Gorfman, S.; Meyer, D.C. Picometer polar atomic displacements in strontium titanate determined by resonant X-ray diffraction. Nat. Commun. 2018, 9, 1–9. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Khodier, S.A. Measurement of wire diameter by optical diffraction. Opt. Laser Technol. 2004, 36, 63–67. [Google Scholar] [CrossRef]
- Couto, H.J.; Nunes, D.G.; Neumann, R.; França, S.C. Micro-bubble size distribution measurements by laser diffraction technique. Miner. Eng. 2009, 22, 330–335. [Google Scholar] [CrossRef]
- Fisher, P.; Aumann, C.; Chia, K.; O’Halloran, N.; Chandra, S. Adequacy of laser diffraction for soil particle size analysis. PLoS ONE 2017, 12, e0176510. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Wu, Y.; Ma, J.; Yang, Y.; Sun, P. Improvements of measuring the width of Fraunhofer diffraction fringes using Fourier transform. Optik 2015, 126, 4142–4145. [Google Scholar] [CrossRef]
- Demeyere, M.; Eugène, C. Measurement of cylindrical objects by laser telemetry: A generalization to a randomly tilted cylinder. IEEE Trans. Instrum. Meas. 2004, 53, 566–570. [Google Scholar] [CrossRef]
- Bernabeu, E.; Serroukh, I.; Sanchez-Brea, L.M. Geometrical model for wire optical diffraction selected by experimental statistical analysis. Opt. Eng. 1999, 38, 1319–1325. [Google Scholar] [CrossRef]
- Li, C.T.; Tietz, J.V. Improved accuracy of the laser diffraction technique for diameter measurement of small fibres. J. Mater. Sci. 1990, 25, 4694–4698. [Google Scholar] [CrossRef]
- Yang, S.; Chen, B.; Lin, B.; Cao, X. Filament diameter measurement system based on dual diffraction. Chin. Opt. Lett. 2015, 13, 120501. [Google Scholar] [CrossRef]
- Khajornrungruang, P.; Kimura, K.; Suzuki, K.; Inoue, T. Micro tool diameter monitoring by means of laser diffraction for on-machine measurement. Int. J. Autom. Technol. 2017, 11, 736–741. [Google Scholar] [CrossRef]
- Gavin, H.P. The Levenberg-Marquardt Algorithm for Nonlinear Least Squares Curve-Fitting Problems; Department of Civil and Environmental Engineering, Duke University: Durham, UK, 2020. [Google Scholar]
- Liu, Q.; Wei, F. Research on the Dark Stripes Extraction Algorithm for Measuring Diameter with Diffraction. Opt. Photonics J. 2013, 3, 53–56. [Google Scholar] [CrossRef] [Green Version]
- Elfurjani, S.; Bayesteh, A.; Park, S.; Jun, M. Dimensional measurement based on rotating wire probe and acoustic emission. Measurement 2015, 59, 329–336. [Google Scholar] [CrossRef]
- Pritchett, T.M.; Trubatch, A.D. A differential formulation of diffraction theory for the undergraduate optics course. Am. J. Phys. 2004, 72, 1026–1034. [Google Scholar] [CrossRef]
- Born, M.; Wolf, E. Principles of Optics: Electromagnetic Theory of Propagation, Interference and Diffraction of Light; Elsevier: Amsterdam, The Netherlands, 2013. [Google Scholar]
- Hecht, E. Optics, 5e; Pearson Education India: Delhi, India, 2002. [Google Scholar]
- Kundu, R.; Das, S.; Mukherjee, R.; Debchoudhury, S. An improved particle swarm optimizer with difference mean based perturbation. Neurocomputing 2014, 129, 315–333. [Google Scholar] [CrossRef]

| Focal length 75 mm |  |  |  |  | ||||
| Filament diameter | 125.2 | 125.2 | 125.0 | 125.0 | ||||
| Focal length 120 mm |  |  |  |  | ||||
| Filament diameter | 125.2 | 125.2 | 125.0 | 125.0 | ||||
| Focal length 126.2 mm |  |  | 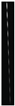 |  |  | 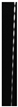 |  | 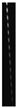 |
| Filament diameter | 110 | 120 | 130 | 140 | 150 | 160 | 170 | 180 |
| Diameter | Average of Measurements | Standard Deviation | Relative Standard Deviation (%) | Absolute Error | Relative Error (%) |
|---|---|---|---|---|---|
| 125.00 ± 0.1 | 125.06 | 0.13 | 0.10 | 0.06 | 0.05 |
| 125.20 ± 0.1 | 125.16 | 0.10 | 0.08 | 0.04 | 0.03 |
| 125.00 ± 0.1 | 124.86 | 0.03 | 0.02 | 0.14 | 0.11 |
| 125.20 ± 0.1 | 125.35 | 0.04 | 0.03 | 0.15 | 0.12 |
| Diameter | Results of Image-Based Fitting | RSD (%) | Results of Fringe Fitting | RSD (%) | Results of PSO Fitting | RSD (%) |
|---|---|---|---|---|---|---|
| 110 ± 1 | 110.51 (STD = 0.07) | 0.06 | 113.73 (STD = 1.58) | 1.38 | 110.6 (STD = 2.30) | 2.08 |
| 120 ± 1 | 119.52 (STD = 0.12) | 0.10 | 118.13 (STD = 1.57) | 1.33 | 119.80 (STD = 0.07) | 0.06 |
| 130 ± 1 | 129.24 (STD = 0.06) | 0.05 | 131.37 (STD = 0.78) | 0.59 | 129.28 (STD = 0.08) | 0.06 |
| 140 ± 1 | 139.95 (STD = 0.20) | 0.14 | 141.48 (STD = 0.43) | 0.30 | 139.62 (STD = 0.23) | 0.16 |
| 150 ± 1 | 150.27 (STD = 0.01) | 0.01 | 151.87 (STD = 0.23) | 0.15 | 149.83 (STD = 0.20) | 0.15 |
| 160 ± 1 | 159.19 (STD = 0.09) | 0.01 | 158.85 (STD = 0.77) | 0.48 | 159.45 (STD = 1.14) | 0.71 |
| 170 ± 1 | 169.68 (STD = 0.11) | 0.06 | 172.19 (STD = 0.23) | 0.13 | 169.54 (STD = 0.09) | 0.05 |
| 180 ± 1 | 180.47 (STD = 0.05) | 0.03 | 180.13 (STD = 0.99) | 0.55 | 180.16 (STD = 0.30) | 0.16 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, Y.; Lin, Y.; Li, D.; Wang, F.; Cheng, B.; Lin, Q.; Hu, Z.; Wu, B. Improved Accuracy for Measurement of Filament Diameter Based on Image-Based Fitting Method. Photonics 2022, 9, 556. https://doi.org/10.3390/photonics9080556
Zhao Y, Lin Y, Li D, Wang F, Cheng B, Lin Q, Hu Z, Wu B. Improved Accuracy for Measurement of Filament Diameter Based on Image-Based Fitting Method. Photonics. 2022; 9(8):556. https://doi.org/10.3390/photonics9080556
Chicago/Turabian StyleZhao, Yingpeng, Yutong Lin, Dianrong Li, Feichen Wang, Bing Cheng, Qiang Lin, Zhenghui Hu, and Bin Wu. 2022. "Improved Accuracy for Measurement of Filament Diameter Based on Image-Based Fitting Method" Photonics 9, no. 8: 556. https://doi.org/10.3390/photonics9080556
APA StyleZhao, Y., Lin, Y., Li, D., Wang, F., Cheng, B., Lin, Q., Hu, Z., & Wu, B. (2022). Improved Accuracy for Measurement of Filament Diameter Based on Image-Based Fitting Method. Photonics, 9(8), 556. https://doi.org/10.3390/photonics9080556





