Next Article in Journal
High-Peak-Power Sub-Nanosecond Mode-Locking Pulses Generated by a Dual-Loss-Modulated QML Laser with AOM and SnSe2
Previous Article in Journal
Photobiomodulation Using Different Infrared Light Sources Promotes Muscle Precursor Cells Migration and Proliferation
Previous Article in Special Issue
Interferometric Technique for the Spectral Characterization of High Frequency Current-Modulated Mid-Infrared Semiconductor Lasers
 
 
Search for Articles:
Title / Keyword
Author / Affiliation / Email
Journal
Article Type
 
 
Advanced Search
 
Section
Special Issue
Volume
Issue
Number
Page
 
Journals
Photonics
Volume 9
Issue 7
10.3390/photonics9070470
photonics-logo
Submit to this Journal Review for this Journal Propose a Special Issue
► Article Menu

Article Menu

share Share announcement Help format_quote Cite question_answer Discuss in SciProfiles thumb_up
...
Endorse textsms
...
Comment
first_page
settings
Order Article Reprints
Font Type:
Arial Georgia Verdana
Font Size:
Aa Aa Aa
Line Spacing:
Column Width:
Background:
Review

Advances in Mid-Infrared Single-Photon Detection

by
Stefano Dello Russo
,
Arianna Elefante
,
Daniele Dequal
,
Deborah Katia Pallotti
,
Luigi Santamaria Amato
,
Fabrizio Sgobba
and
Mario Siciliani de Cumis
*
Italian Space Agency (ASI), Centro di Geodesia Spaziale ‘Giuseppe Colombo’, Località Terlecchia, 75100 Matera, Italy
*
Author to whom correspondence should be addressed.
Photonics 2022, 9(7), 470; https://doi.org/10.3390/photonics9070470
Submission received: 28 May 2022 / Revised: 30 June 2022 / Accepted: 3 July 2022 / Published: 6 July 2022
(This article belongs to the Special Issue Near- and Mid-Infrared Photonics Technologies)
Browse Figures
Versions Notes

Abstract

:
The current state of the art of single-photon detectors operating in the mid-infrared wavelength range is reported in this review. These devices are essential for a wide range of applications, such as mid-infrared quantum communications, sensing, and metrology, which require detectors with high detection efficiency, low dark count rates, and low dead times. The technological challenge of moving from the well-performing and commercially available near-infrared single-photon detectors to mid-infrared detection is discussed. Different approaches are explored, spanning from the stoichiometric or geometric engineering of a large variety of materials for infrared applications to the exploitation of alternative novel materials and the implementation of proper detection schemes. The three most promising solutions are described in detail: superconductive nanowires, avalanche photodiodes, and photovoltaic detectors.

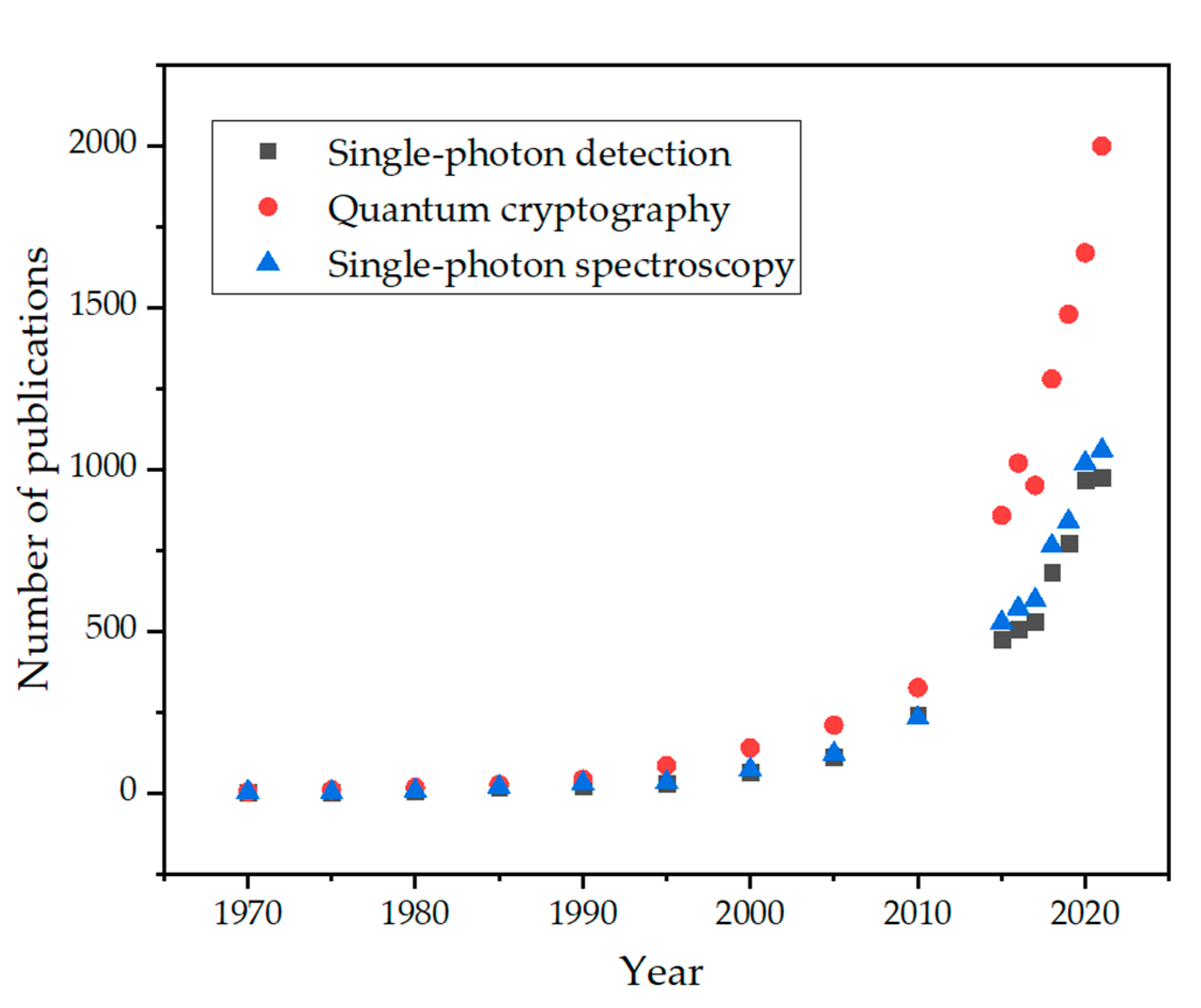
1. Introduction

Quantum technologies are pushing toward new paradigms in research and device development. In particular, on the one hand, advances in materials sciences are supporting quantum experiments. On the other hand, groundbreaking results in quantum communication, quantum computing and simulation [1,2,3], quantum sensing [4,5,6,7,8], and metrology [9,10] drive technological development useful for the public at large. Moreover, the interest in quantum experiments in the mid-infrared (MIR) range is rising [11,12,13,14], and consequently, there is a demand for high-performance photonic components in this spectral region. Indeed, a strong interest among researchers and technological communities is focused on the development of single-photon detectors (SPDs) operating in the mid-infrared spectral region. The driving force behind this trend of research relies on the existence of a broad range of applications that would find relevant improvements in the MIR region, the development of which has been prevented so far mainly due to technological limitations. Although efficient SPDs exist and are commercially available for the near-infrared (NIR)/visible region, a considerable effort is centered on how to move toward longer wavelength detection. The rapidly rising interest in MIR single-photon detectors and, consequently, in the applications that become accessible in this spectral region is highlighted in Figure 1, which shows the number of publications on these topics in the last 50 years.
Among the several applications, the current rising research focused on single-photon detectors is strongly promoted by the field of quantum communication science. SPDs are key elements for the quantum key distribution (QKD) [16] for the detection of single photons traveling along a channel, which are an essential request to guarantee the security of communication and prevent eavesdropping. QKD has widely been explored in the NIR region both for guided-wave and free-space implementations [17]. Nevertheless, several beneficial motivations drive the shift in focus to the MIR. For free-space communications with satellite-based QKD, two strength points are noticeable. First, the Rayleigh scattering cross-section is well-reduced, compared with the NIR region, offering an exploitable transparency atmosphere window between 3 and 5 µm [18]. Moreover, during daylight, the binding effect of the solar background radiation is up to 3 times lower, compared with the telecom band at 1550 nm [19,20]. At the same time, the 2 µm band is desirable for fiber telecommunications and fiber-based QKD, since it overcomes some issues of the standard telecom band. First of all, it overcomes the capacity crunch [21] shown by guided wave optics at 1.5 µm. Moreover, the 2–2.5 µm band allows the reduction in intrinsic losses mainly due to Rayleigh scattering, showing minimal losses in the hollow-core photonic band gap fiber [22,23].
Besides quantum information science, quantum sensing applications can also inherit the potential benefits of probing a strong rovibrational transition in the MIR molecular fingerprint region. MIR single-photon detectors can be used for the search of biosignatures in the atmosphere of exoplanets [5], owing to the detection of small changes in the spectrum of a star due to the absorption or emission from a transiting exoplanet’s atmosphere. For lidar, VIS–NIR single-photon avalanche photodiodes with high temporal resolutions are commonly used for the detection of very weak optical pulses. The extension of lidar to the MIR [4,24] allows gas concentration measurements in the molecular fingerprint region [25], as well as higher transmission through scattering media, such as fog and smog [26]. The emergence of MIR SPDs is encouraging the extension of the fluorescence imaging technique, realized in the NIR second region band exploiting superconductive single-photon detectors [27,28], to new MIR fluorescence spectroscopy schemes, with all its countless applications, such as monitoring volcanos, infrared chemiluminescence, thermal microscopy, and infrared telescopy [6]. In addition, interferometric schemes for mid-infrared imaging have been demonstrated [29] by using “conventional” detectors for on-field applications in harsh environments [30]. MIR single-photon development paves the way for quantum imaging schemes useful also for quantum sensing [31].
Alongside the need for MIR single-photon detectors, nonclassical sources are required to generate the MIR single photons; SPDs with photon number resolutions are necessary elements for the characterization of such quantum sources, in order to analyze the photon number distribution of the emitter [9].
The above-mentioned applications reveal the necessity of developing SPDs in the MIR region with appropriate features. The main features discussed in this review are the system detector’s efficiency (DE or SDE), the dark count rate (DCR, also referred to as background count rate), and timing. The overall device detection efficiency ηDE is the probability that a photon incident at the optical input of the detector within a detection gate will be detected and produce an output signal. The overall detector efficiency can be factorized into the product of the quantum efficiency of the detector ηqe and other contributions, depending on the specific physical processes involved in the detection scheme, as explained in the next sections. The dark count rate is the rate at which a detector registers an event within a detection gate in the absence of incident photons. Another important parameter to be optimized is the detector timing, including the dead time and the jitter. The dead time is the time interval after a detection event during which the detector is unable to provide a response to an incoming photon, while the jitter is the temporal variation in the output signal produced by the detector upon registering an event. An additional feature of single-photon detectors is the photon number resolution (PNR), which is the ability to distinguish the number of photons in an incident pulse. This last feature will not be widely discussed in this review since the reported categories of MIR single-photon detectors are not photon-number-resolving detectors. Nevertheless, different schemes based on an array of SPDs, so that each can register a photon independent of the others, can be implemented to gain the PNR capability [32].
Before discussing the MIR SPDs, in this review, we first present the state of the art in NIR SPD research and highlight their outstanding importance in commercially available technologies, as discussed in several reviews [32,33,34]. Various research lines seek to exploit or modify the well-performing NIR SPDs in order to extend their applicability in longer wavelength regions. In particular, these studies deal with three categories of detectors: superconducting nanowire, avalanche photodiode, and photovoltaic detectors. Superconductive nanowire single-photon detectors (SNSPDs) have emerged as leading technological devices in the NIR owing to their system detection efficiency of ~90%, high count rates, dark count rates as low as 10−3 cps, and low jitter time [7,35]. Different approaches to address the dramatic drop in their quantum efficiency with the increase in wavelength and extend detector sensitivity to the MIR are discussed. The second category of SPDs is the room temperature avalanche photodiodes, which are well-developed and commercially available detectors in the short IR range. Two parallel approaches are currently being developed to extend the employment of APDs to the MIR range. The first one consists of developing devices designed with appropriate material to operate at mid- and long wavelengths. The second and most widely implemented approach, instead, exploits the performances of NIR photodiodes to detect upconverted MIR photons, as is explained in Section 3. The last strategy for MIR single-photon detection investigated in this review is based on the use of two photovoltaic immersed (PVI) HgCdTe detectors in a balanced homodyne detection scheme.

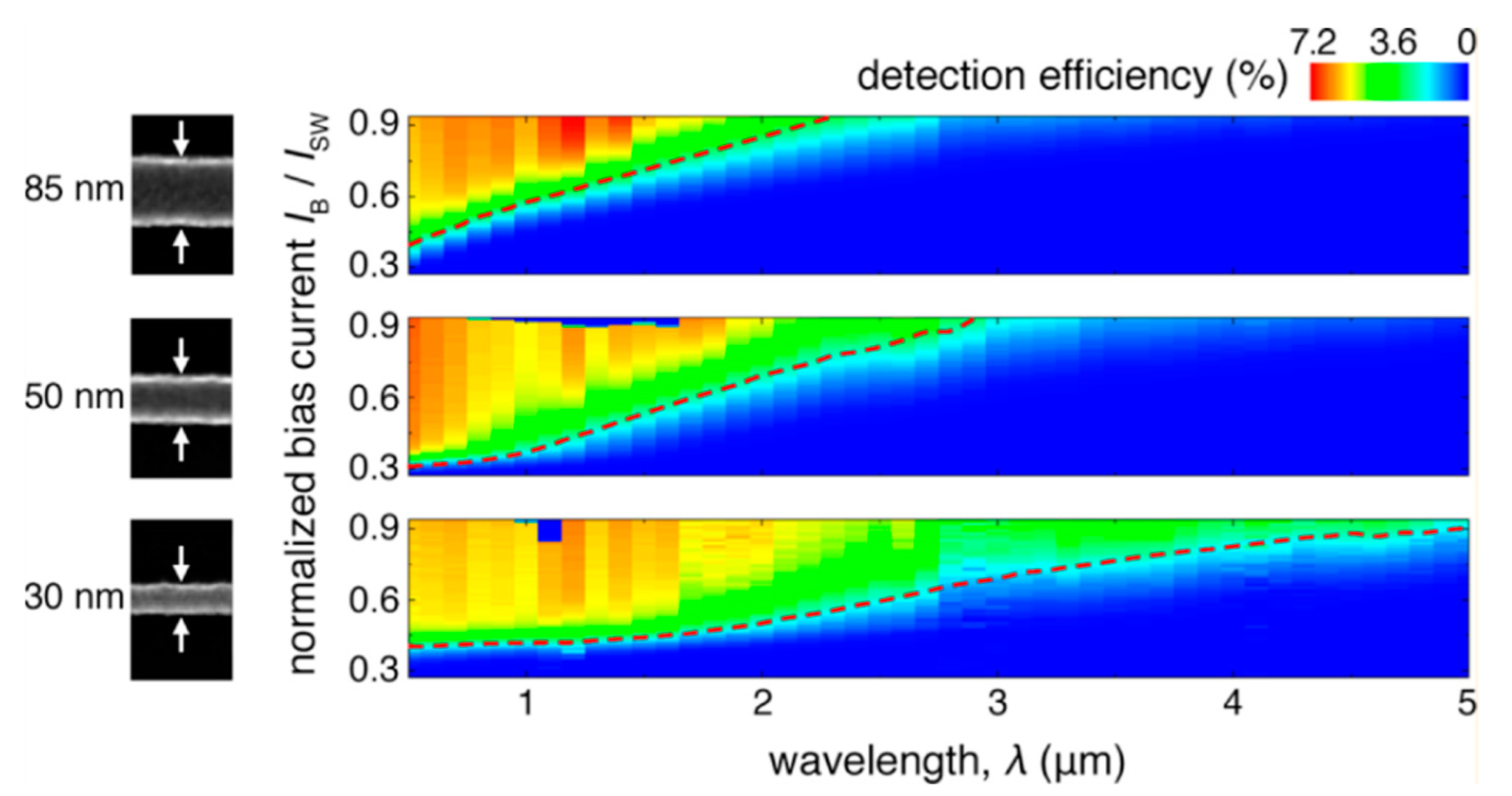
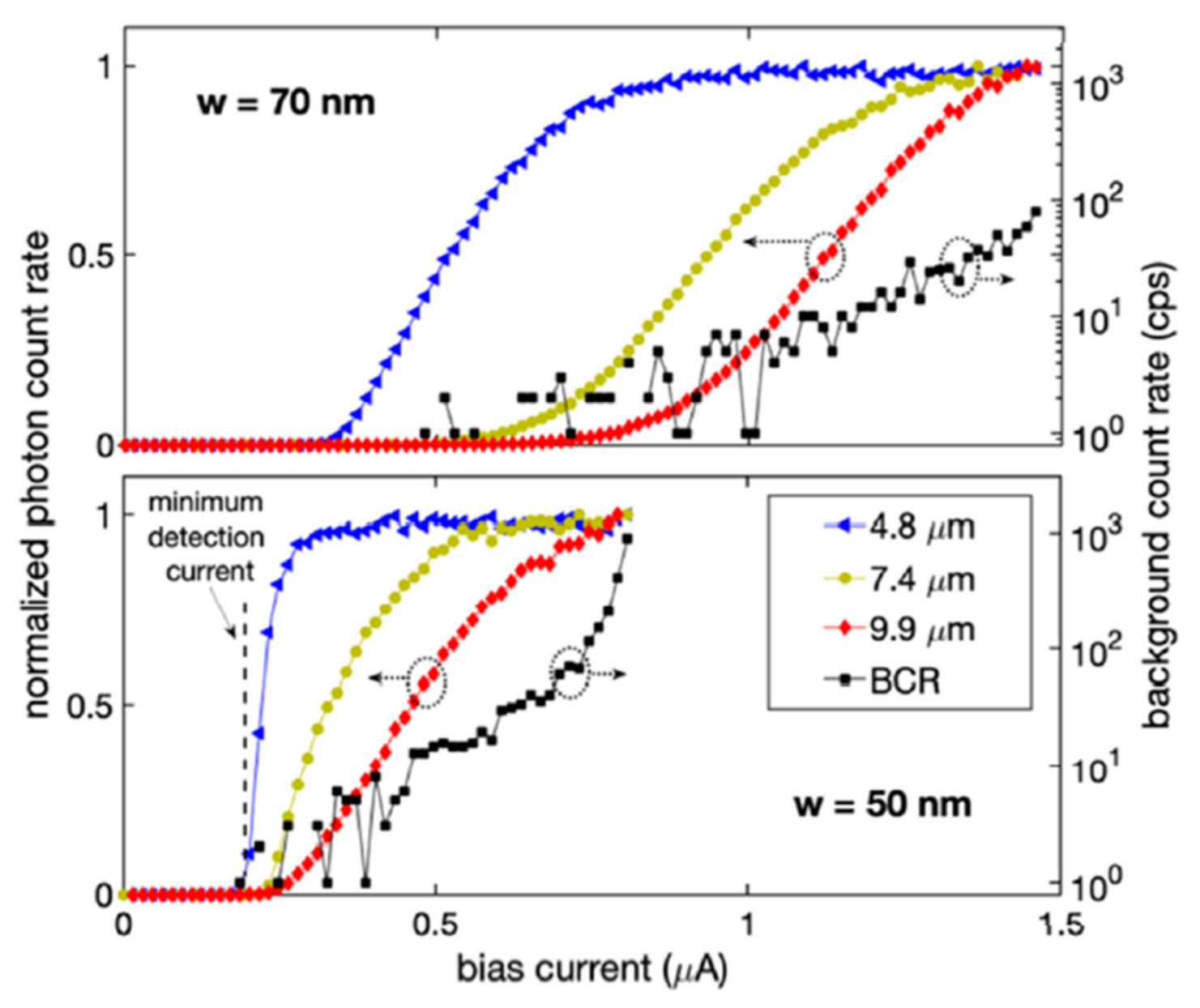
2. Superconducting Nanowire Single-Photon Detectors

Developed by Goltsman et al. in 2001 [36], superconducting nanowire single-photon detectors (SNSPDs or SNPDs), depicted in Figure 2a, consist of a thin (≈5 nm) film of superconducting material shaped into a meandering nanowire (width ≈ 100 nm, active area ≈ 100 µm2) through nanofabrication processes. The operating principle has been discussed in detail by Engel et al. [37] and is schematized in Figure 2b: the nanowire, biased with a DC current that is close to, but less than, the superconducting critical current, absorbs a photon generating quasi-particles and phonons, the diffusion of which breaks the nanowire superconductivity. This results in a drop in the current circulating in the nanowire. After the reset time, the SNSPD returns to a steady state and is again sensitive to single-photon absorption. The main limitation of SNSPDs is the operating temperature, which depends on the superconducting transition temperature of the constituent material (TC) [38].
For SNSPDs, the device efficiency ηDE is given by the product of the probability of three independent processes ηDE = ηcpl ηabs ηqe, where ηcpl represents the coupling of the device to the light source, ηabs represents the probability that a photon arriving at the region of the device will be absorbed in the nanowire, and ηqe is the intrinsic quantum efficiency, which defines the probability that a photon absorption event will produce a measurable signal [40]. The SNSPD quantum efficiency shows a sigmoidal feature [41], as the bias current varies. ηqe saturation is reached for lower bias currents as the temperature decreases. If the temperature is too high, the saturation is not reached, as the detector loses its superconducting properties, and the detection efficiency is strongly compromised.
The demonstrated advantageous efficiency and timing performance of SNSPDs comprise quasi-saturated (93%) system detection efficiency (DE) [42], lower counts per second (cps), dark count rate (DCR) [43], lower timing jitter [44], and lower reset time [45] when compared with other single-photon detectors operating at the C-band telecom wavelength (1.55 µm). Detailed analysis of the physical mechanism and performance of SNSPDs in the near-IR spectral range are reported in numerous reviews [40,41,46].
The most widely used material for nanowire realization is niobium nitride (NbN) due to its high superconducting transition temperature (TC = 1–6 K) and its fast thermal recovery time, allowing operation at high count rates [45,47]. Different approaches have been explored to extend the spectral detection efficiency up to MIR. The first attempts date back to 2012: Korneev et al. [47] demonstrated that a reduction in the strip width improves the quantum efficiency of NbN SNSPD at wavelengths longer than 1.5 μm. The reason is that, with a narrower nanowire, the energy per unit area is larger, and thermal conduction along the length of the nanowire is smaller, resulting in a higher probability to generate a hotspot [48]. A detailed experimental study of the quantum DE dependence on the variation in the NbN nanowire width was carried out by Marsili et al. [49]. As shown in Figure 3, varying the nanowire width from 85 to 30 nm, it was possible to enlarge the efficiency range up to 5 µm. In the range λ = 0.5–2.7 μm, DE showed a sigmoidal dependence on the bias current (IB) and saturated to DE~4.5−5.5% (depending on the wavelength) for IB, sufficiently higher than the cutoff current (Ico). Furthermore, 30 nm wide SNSPDs, up to longer wavelengths of 5 μm, showed two orders of magnitude higher DE (~2%) than the value previously reported for this class of detector.
As the nanowire became narrower, edge roughness and constrictions in the width of the nanowire, due to fabrication imperfections, began to have larger relative effects, degrading the device performance: the DE for small wavelengths was reduced, compared with the case of wider nanowires. Moreover, as the nanowire width decreased, an increase in the detector DCR of up to two orders of magnitude was observed, with a significant drop in its critical current density [50]. For applications in which the mean value of the DCR cannot be compensated (e.g., quantum key distribution [51]), the value of DE at a certain value of DCR is the relevant figure of merit. Considering the statistical variation of the DCR, the overall DE in the Mid-infrared region ranged from 5% around 2 µm to 1% around 5 µm wavelength. Five years later, Zolotov et al. [52] studied the quantum efficiency of NbN SNSPD devices close to saturation level. With careful optimization of the SNSPD realization mechanism, ηqe up to 40% at 2.5 µm wavelength and 10% at 3 µm wavelength were achieved, although overall SNSPD DE values were not reported. Recently, Chang et al. [53] provided an alternative approach with SNSPDs fabricated from 5–9.5 nm thick (50 nm wide) conventional NbTiN superconducting films and devices operated in conventional Gifford–McMahon cryocoolers. By optimizing the superconducting film deposition process, film thickness, and nanowire design, the fiber-coupled devices achieved >70% device detection efficiency at 2 μm and sub-15 ps timing jitter. Furthermore, detectors from the same batch demonstrated saturated quantum detection efficiency at 3 μm and 80% internal efficiency at 4 μm, paving the path for an efficient mid-infrared single-photon detection technology with unparalleled time resolution and without mK cooling requirements.
A second approach to extending the overall DE up to MIR is to choose a proper material for SNSPD fabrication. Extreme structural homogeneity and the absence of grain boundaries in metal–silicon alloys can be advantageous, compared with the granular structure in nitride superconductors. In particular, tungsten silicide (a-WxSi1−x) showed a tunable superconducting transition temperature TC up to 5 K and seemed suitable for single-photon MIR detection. Baek et al. [54] developed a nanowire device based on a-WxSi1−x alloy thin film with saturated quantum efficiency from visible to 1.85 µm and overall DE of 20% at 1.55 µm. Recently, Verma et al. [48] extended this result, increasing film resistivity by tuning the film stoichiometry during the fabrication and thus realizing an SNSPD based on W52Si48 with saturated quantum detection efficiency up to a wavelength of 10 μm with DCR between 10 and 100 cps, as shown in Figure 4.
The use of metal–silicon alloys allows the realization of larger nanowires, compared with the ones obtained with NbN, making the manufacturing process simpler. However, it requires more efficient cooling systems due to the lower operating temperature of the SNSPD. Saturated quantum efficiency in the range 1.55–5.07 µm was also obtained by Chen et al. [35], by using another metal–silicon alloy, molybdenum silicide (Mo80Si20), with DCR between 10 and 100 cps. The operating temperature (85 mK), however, was found to be an order of magnitude lower than the one obtained with the WSi SNSPD.
The single-photon detection in MIR with SNSPD has reached almost the same levels obtained in NIR in an enormously short period of time. Achievements in saturated quantum efficiency and the progress made in radiation sensor coupling represent the first great result, which has already led researchers to search for technological applications. For example, Prabhakar et al. [20] and Dada et al. [22] demonstrated the possibility of implementing device-independent quantum key distribution (DI-QKD) with SNSPD at 2.1 µm with a secure key rate of 0.254 bits/pair, by using a NbTiN SNSPDs with detection efficiency rates of roughly one order of magnitude lower than that developed by Chang et al. A lidar system with MIR SNSPDs operating at 2.3 µm has been developed [55], demonstrating the viability of these detectors for future free-space photon counting applications. Further improvements for lidar experiments can be achieved by developing lidar systems exploiting an array of detectors with an appropriate read-out circuit [56].

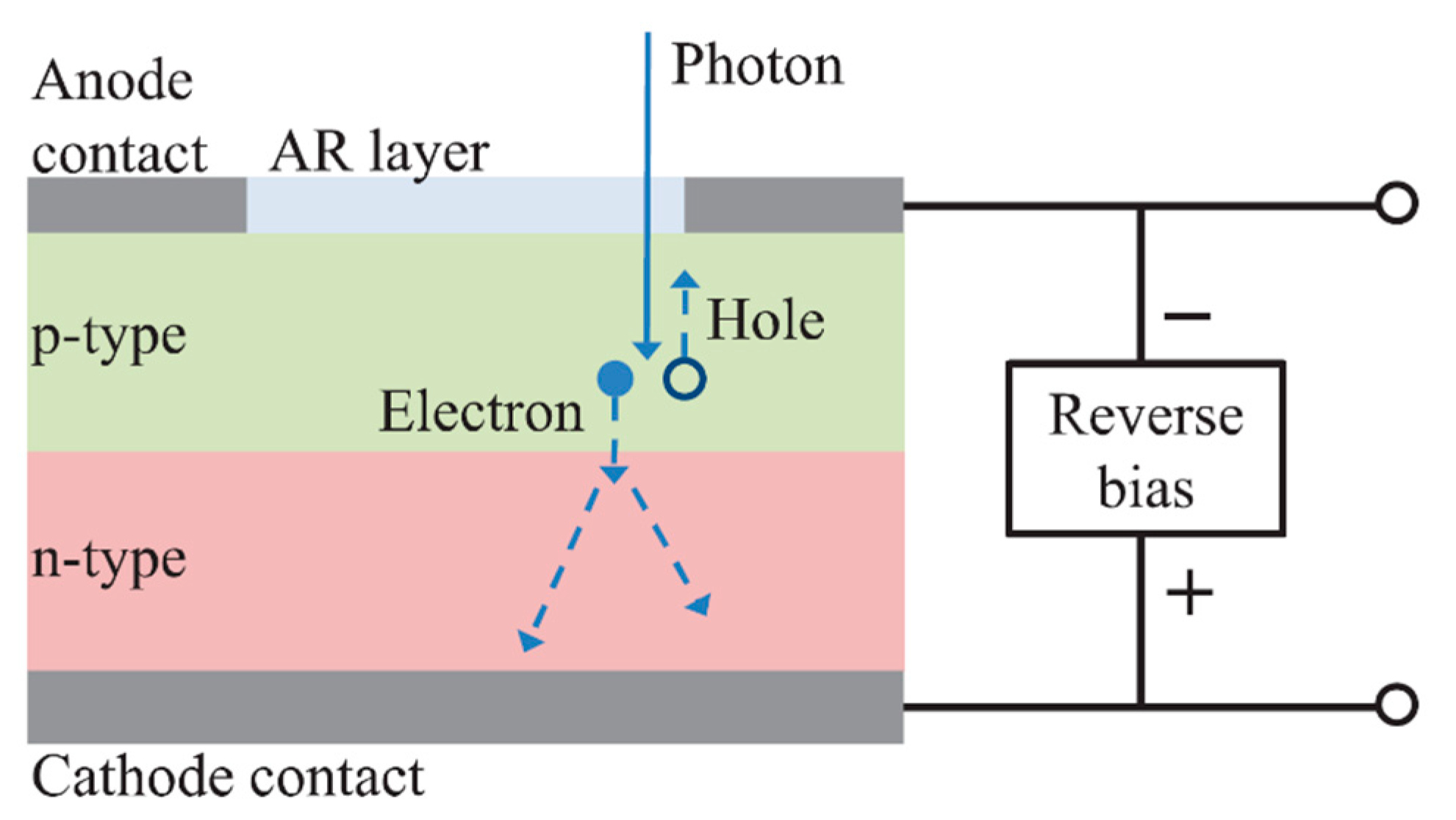
3. Single-Photon Avalanche Diodes

A single-photon avalanche diode (SPAD or SPAPD) is a p–n junction operating in “Geiger mode”: The device is biased well above its reverse-bias breakdown. Its basic principle of detection is sketched in Figure 5. First, the photon is absorbed in the p-type zone, creating an electron–hole pair. The electron then moves to the n-type zone, due to the applied voltage, generating a large avalanche of current carriers. These carriers grow exponentially and can be triggered from a single-photon-initiated carrier.
Geiger-mode SPADs can have high device efficiency DEs, up to 85%, in the visible range. To reduce dark count rates, SPADs are typically cooled with thermoelectric coolers to temperatures of 210 K to 250 K. In addition, the SPAD gain medium typically has trap sites that need time to depopulate after an avalanche has occurred and before the bias voltage can be restored. If those sites are not allowed to depopulate, carriers released from traps rather than from a new photon can initiate a second avalanche. As a result, SPAD dead times can range from tens of nanoseconds to tens microseconds. This is a particular problem for SPADs designed for IR sensitivity [32].
The most widely used materials for NIR SPAD fabrication are indium gallium arsenide (InGaAs) [57] and numerous other III–V materials [58,59,60]. Among them, InP/InGaA NIR detectors present the most developed existing and commercially available single-photon detector technology, owing to their practicality in terms of small size, low cost, and easy operation [61]. Beyond the choice of material and SPAD device design, the detection circuitry (quenching electronics) plays an important role in the overall performance of SPADs. Different schemes can be implemented, including passive quenching, active quenching, and gated quenching. In particular, gated quenching has shown the capability to suppress DCR and after-pulses [62], making it suitable for applications requiring synchronous photon detection such as QKDs. After-pulsing refers to avalanche events that originate from the emission of carriers that were trapped in deep levels during previous avalanche events, due to semiconductors’ impurities. High-frequency gating (sinusoidal gating [63,64], a self-differing technique [65,66]), can reduce the after-pulses probability since it reduces the total charge flow generated during an avalanche event, maintaining high detection rates at the same time. Recently, new studies are emerging on materials and superlattices, such as HgCdTe [67], InAs/InSb [68], AlAsSb/GaSb [69], which can extend the detection range of SPADs to MIR. However, significantly lower cooling temperatures (≈80 K) are required to suppress the dark current due to the low energy bandgap [67,70], resulting in increased costs and complexity of such devices.
To date, the best way to detect single photons in MIR with SPADs consists of creating a photon with a shorter wavelength through sum-frequency generation (SFG). With this detection scheme, the overall device efficiency is given by the product ηDE = ηqe ηopt ηSFG, where ηopt comprises the losses in all optical components, and ηSFG is the probability that the MIR photon will be upconverted inside the nonlinear crystal [11]. In 2016, Mancinelli et al. [71] paved the way toward quantum measurements in the MID-IR by demonstrating a room temperature coincidence measurement with nondegenerate twin photons at about 3.1 µm. As sketched in Figure 6a, a periodically poled lithium niobate (PPLN) crystal was used for both the entangled photon generation (spontaneous parametric downconversion (SPDC)) process and the sum-frequency generation process. By appropriately choosing the crystal poling period (Λ) and crystal temperature, it was possible to optimize the conversion efficiency, making sure that the photon produced during the SPDC would have a suitable wavelength to interact with the second crystal, as shown in Figure 6b. In the case of nondegenerate photons, losses were minimized, but the energy probability distribution of the downconverted photons was much greater (≈50 nm) than the probability distribution of photons useful for the SFG (≈7 nm). The conversion efficiency was found to be less than one unit.
Using this method, the authors demonstrated the possibility of detecting entangled photons, at room temperature for the first time, also reaching low DCR levels (1 kcps at 300 K) and an excellent coincidence-to-accidental ratio (CAR ≈ 16). Unfortunately, despite having a quantum detection efficiency of 65% at the selected wavelength (0.8 µm), considering the coupling efficiencies in SFG and SPDC processes and the losses between detector overlap and optical filters, the system DE was equal to 0.35%, about an order of magnitude lower than the results obtained with SNSPD in the same years [50]. However, improvement in the device’s detection efficiency is expected by optimizing the described system: The higher upconversion efficiency ηSFG of 20% demonstrated in [72] combined with the use of commercial silicon-based photon counters with quantum efficiency ηqe of 80% will allow reducing the coupling losses and, eventually, eliminating them, reaching device detection efficiency up to 15%. Four years later, through careful optimization of optical pulses in spectral and temporal domains, Huang et al. [73] were able to perform SFG with a conversion efficiency of 80%. In combination with a silicon avalanche photodiode, they obtained unprecedented performance, with a high device efficiency DE of 37% and a low noise equivalent power of 1.8 × 10−17 W/Hz1/2.
These results, together with the current efforts in the engineering of materials for the realization of new SPADs optimized for detection in MIR, pave the way for the detection of single photons at larger wavelengths without resorting to the use of cryogenic cooling systems, very expensive and difficult in terms of maintenance, transport, use, and availability.

4. Photovoltaic Detectors

As known in the literature, in the MIR, photovoltaic detectors are usually devoted to spectroscopy. In the last few years, some applications have been reported in the field of quantum technologies.
The most common example of photovoltaic detectors is the abrupt p–n junction prepared in a semiconductor, which is often simply referred to as a photodiode. A graphical representation of a photovoltaic (PV) detector is similar to that shown in Figure 5. However, a variety of different photodiode structures are used, according to the scopes and the wavelength, as widely discussed in [74,75,76]. Nevertheless, the basic principle is the same—namely, photons with energy greater than the energy gap, incident on the front surface of the device, create electron–hole pairs in the material on both sides of the junction. Via diffusion, the electrons and holes generated within a diffusion length from the junction reach the space-charge region. Then, electron–hole pairs are separated by the strong electric field; minority carriers are readily accelerated to become majority carriers on the other side. In this way, a photocurrent is generated, which shifts the current–voltage (I–V) characteristic in the direction of negative or reverse current.
In general, photovoltaic detectors have weaker performance than SPADs in terms of single-photon detection. However, their noise level is lower, their structure is simpler, and they have linear behavior in opportune conditions. In addition, in SPAD devices, the current gain depends on the bias applied and on thermal fluctuations. For these reasons, it is required an adequate heat sink for such detectors. These features could favor the use of PV detectors in the future. Recently, Gabbrielli et al. [77] reported a mid-infrared balanced homodyne detection (BHD) system suitable for quantum characterization by using two photovoltaic optically immersed (PVI) HgCdTe detectors, in which the active element is grown monolithically on a hyperhemispherical microlens to improve detection performances. As sketched in Figure 7, the detection scheme simply consists of a beam splitter that divides the beam; then, the photons of these two beams impinge on two almost identical detectors. A similar approach has already been used for the detection of upconverted photons, through the use of InGaAs NIR detectors in heterodyne configuration schemes, demonstrating quantum vacuum fluctuation detection [78] and highly sensitive spectroscopic applications devoted to real-world biological and medical settings [79,80].
To test the sensitivity limits of the BHD detection system, the authors estimated a shot-noise clearance, compared with differential electrical noise, up to 8, leading to an effective quantum efficiency of 36% and reaching the sub-shot noise limit. This detection scheme represents an important step toward the quantum characterization of mid-infrared light. The lower quantum efficiency of PVI detectors, compared with that of SNSPDs and SPADs, is compensated by the simpler control of thermodynamic parameters, the greater stability at high temperatures, and, without doubt, the cost and current availability of these devices. Advances in photovoltaic detectors fabrication are on the agenda [76] and could lead, in the immediate future, to sufficiently high detection performance to make them suitable for MIR single-photon counting.

5. Conclusions

Research on mid-infrared single-photon detectors shows a constantly rising interest among scholars in recent years. The considerable research effort on this topic is moved and accelerated by the benefits that several applications inherit by working in the mid-infrared spectral region. Different types of single-photon detectors are investigated in parallel, with the common aim to improve the currently well-performing SPDs in the NIR in the direction of achieving higher efficiencies, lower timing jitter, and lower dark count rates when working at longer wavelengths. Table 1 summarizes the most promising results reported in the state-of-the-art research. All these detectors can be implemented in the realization of highly integrated photon circuits, as required for future quantum applications. SNSPDs offer low dark count rates of the order of 10–100 cps. The lower device detection efficiency in the MIR, compared with the one in the NIR, has been shown to be improved with both the reduction in strip width and optimization of the superconductive film deposition process, film thickness, and nanowire design, reaching device detection efficiency of >70% at 2 µm and sub-15 ps timing jitter. Near-infrared single-photon avalanche diodes operating at room temperature with high quantum efficiency can be implemented to detect MIR photons, which are first upconverted through sum-frequency generation. It was demonstrated that, with an appropriate pulsed pumping scheme for frequency upconversion, it was possible to optimize the conversion efficiency, reaching a record device detection efficiency of 37%. PVI detectors employed in a balanced homodyne detection scheme showed the potential to achieve shot-noise limited measurements required for quantum light characterization.
To date, SNSPDs seem to be the best choice for detecting single photons in the mid-infrared region, in terms of detection efficiency and timing performance. The first application study of QKD in the mid-infrared region was carried out precisely with these devices [20,22]. SPADs and PV detectors, on the other hand, show important progress and can, in the near future, pave the way for the use of out-of-lab quantum technologies at large wavelengths, due to their easy portability, lower energy consumption, and lower costs, although their high dark count rates still remain an unsolved problem.
Thus far, the technological gap in the MIR spectral region has been a limitation in quantum research. Single-photon detectors are pillars for a large variety of schemes in quantum sensing, communication, and metrology. In the near future, emerging technologies in the MIR and innovative setups will enable developments crucial for human life, security issues, space applications, and fundamental research.

Author Contributions

S.D.R., A.E. and M.S.d.C. wrote the original draft; D.D., F.S., L.S.A., D.K.P., S.D.R., A.E. and M.S.d.C. reviewed and edited the original draft. All authors have read and agreed to the published version of the manuscript.

Funding

This research was partially supported by the QOMBS project (EC H2020 Quantum FLAGSHIP GA n.820419), the QUANCOM project (MUR PON Ricerca e Innovazione 2014–2020 ARS01_00734), and the WhiTech project (funded by Italian Space Agency).

Institutional Review Board Statement

Not applicable.

Informed Consent Statement

Not applicable.

Data Availability Statement

Not applicable.

Conflicts of Interest

The authors declare no conflict of interest.

References

  1. Spitz, O.; Didier, P.; Durupt, L.; Diaz-Thomas, D.A.; Baranov, A.N.; Cerutti, L.; Grillot, F. Free-Space Communication With Directly Modulated Mid-Infrared Quantum Cascade Devices. IEEE J. Sel. Top. Quantum Electron. 2022, 28, 1200109. [Google Scholar]
  2. Shields, T.; Prabhakar, S.; Dada, A.; Ebrahim, M.; Taylor, G.G.; Morozov, D.; Erotokritou, K.; Miki, S.; Yabuno, M.; Terai, H.; et al. Mid-infrared Quantum Interference and Polarization Entanglement. In Proceedings of the OSA High-Brightness Sources and Light-Driven Interactions Congress 2020 (EUVXRAY, HILAS, MICS), Washington, DC, USA, 16–20 November 2020. [Google Scholar]
  3. Lanning, R.N.; Harris, M.A.; Oesch, D.W.; Oliker, M.D.; Gruneisen, M.T. Quantum Communication Over Atmospheric Channels: A Framework for Optimizing Wavelength and Filtering. Phys. Rev. Appl. 2021, 16, 044027. Available online: http://arxiv.org/abs/2104.10276 (accessed on 15 March 2022).
  4. Widarsson, M.; Henriksson, M.; Mutter, P.; Canalias, C.; Pasiskevicius, V.; Laurell, F. High resolution and sensitivity up-conversion mid-infrared photon-counting LIDAR. Appl. Opt. 2020, 59, 2365. [Google Scholar]
  5. Wollman, E.E.; Verma, V.B.; Walter, A.B.; Chiles, J.; Korzh, B.; Allmaras, J.P.; Zhai, Y.; Lita, A.E.; McCaughan, A.N.; Schmidt, E.; et al. Recent advances in superconducting nanowire single-photon detector technology for exoplanet transit spectroscopy in the mid-infrared. J. Astron. Telesc. Instrum. Syst. 2021, 7, 011004. [Google Scholar]
  6. Chen, L.; Schwarzer, D.; Verma, V.B.; Stevens, M.J.; Marsili, F.; Mirin, R.P.; Nam, S.W.; Wodtke, A.M. Mid-infrared Laser-Induced Fluorescence with Nanosecond Time Resolution Using a Superconducting Nanowire Single-Photon Detector: New Technology for Molecular Science. Acc. Chem. Res. 2017, 50, 1400–1409. [Google Scholar]
  7. Verma, V.B.; Lita, A.E.; Korzh, B.A.; Wollman, E.; Shaw, M.; Mirin, R.P.; Nam, S.-W. Towards single-photon spectroscopy in the mid-infrared using superconducting nanowire single-photon detectors. In Advanced Photon Counting Techniques XIII, Proceedings of the SPIE Defense + Commercial Sensing, Baltimore, MD, USA, 14–18 April 2019; SPIE: Bellingham, WA, USA, 2019. [Google Scholar]
  8. Cheng, R.; Zou, C.-L.; Guo, X.; Wang, S.; Han, X.; Tang, H.X. Broadband on-chip single-photon spectrometer. Nat. Commun. 2019, 10, 4104. [Google Scholar]
  9. von Helversen, M.; Böhm, J.; Schmidt, M.; Gschrey, M.; Schulze, J.-H.; Strittmatter, A.; Rodt, S.; Beyer, J.; Heindel, T.; Reitzenstein, S. Quantum metrology of solid-state single-photon sources using photon-number-resolving detectors. New J. Phys. 2019, 21, 035007. [Google Scholar]
  10. Chunnilall, C.J.; Degiovanni, I.P.; Kück, S.; Müller, I.; Sinclair, A.G. Metrology of single-photon sources and detectors: A review. Opt. Eng. 2014, 53, 081910. [Google Scholar]
  11. Temporão, G.; Tanzilli, J.-S.; Zbinden, H.; Gisin, N.; Aellen, T.; Giovannini, M.E.; Faist, J. Mid-infrared single-photon counting. Opt. Lett. 2006, 31, 1094–1096. [Google Scholar]
  12. Spitz, O.; Herdt, A.; Didier, P.; Elsäßer, W.; Grillot, F. Mid-infrared free-space cryptosystem. Nonlinear Theory Its Appl. IEICE 2022, 13, 44–52. [Google Scholar]
  13. Grillot, F.; Spitz, O. Chaos-based mid-infrared communications. In Physics and Simulation of Optoelectronic Devices XXX, Proceedings of the SPIE OPTO, San Francisco, CA, USA, 22 January–28 February 2022; SPIE: Bellingham, WA, USA, 2022; Volume 11995, p. 11995. [Google Scholar]
  14. Gilaberte Basset, M.; Setzpfandt, F.; Steinlechner, F.; Beckert, E.; Pertsch, T.; Gräfe, M. Perspectives for applications of quantum imaging. Laser Photonics Rev. 2019, 13, 1900097. [Google Scholar]
  15. Available online. Available online: https://scholar.google.com/ (accessed on 15 March 2022).
  16. Scarani, V.; Bechmann-Pasquinucci, H.; Cerf, N.J.; Dušek, M.; Lütkenhaus, N.; Peev, M. The security of practical quantum key distribution. Rev. Mod. Phys. 2009, 81, 1301–1350. [Google Scholar]
  17. Pirandola, S.; Andersen, U.L.; Banchi, L.; Berta, M.; Bunandar, D.; Colbeck, R.; Englund, D.; Gehring, T.; Lupo, C.; Ottaviani, C.; et al. Advances in quantum cryptography. Adv. Opt. Photonics 2020, 12, 1012. [Google Scholar]
  18. Temporao, G.; Zibinden, H.; Tanzilli, S.; Gisin, N.; Aellen, T.; Giovannini, M.; Faist, J.; von der Weid, J. Feasibility study of free-Space quantum key distribution in the mid-infrared. Quantum Inf. Comput. 2008, 8, 1–11. [Google Scholar]
  19. E490-00a; Standard Solar Constant and Zero Air Mass Solar Spectral Irradiance Tables. ASTM International: West Conshohocken, PA, USA, 2019.
  20. Prabhakar, S.; Shields, T.; Dada, A.C.; Ebrahim, M.; Taylor, G.G.; Morozov, D.; Erotokritou, K.; Miki, S.; Yabuno, M.; Terai, H.; et al. Two-photon quantum interference and entanglement at 2.1 μm. Sci. Adv. 2020, 6, eaay5195. Available online: https://www.science.org (accessed on 22 March 2022).
  21. Ellis, A.D.; Mac Suibhne, N.; Saad, D.; Payne, D.N. Communication networks beyond the capacity crunch. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2016, 374, 20150191. [Google Scholar]
  22. Dada, A.C.; Kaniewski, J.; Gawith, C.; Lavery, M.; Hadfield, R.H.; Faccio, D.; Clerici, M. Near-Maximal Two-Photon Entanglement for Optical Quantum Communication at 2.1 μm. Phys. Rev. Appl. 2021, 16, L051005. [Google Scholar]
  23. Roberts, P.J.; Couny, F.; Sabert, H.; Mangan, B.J.; Williams, D.P.; Farr, L.; Mason, M.W.; Tomlinson, A.; Birks, T.; Knight, J.; et al. Ultimate low loss of hollow-core photonic crystal fibres. Opt. Express 2005, 13, 236. [Google Scholar]
  24. Widarsson, M.; Henriksson, M.; Barrett, L.; Pasiskevicius, V.; Laurell, F. Room temperature photon-counting lidar at 3 µm. Appl. Opt. 2022, 61, 884. [Google Scholar]
  25. Haas, J.; Mizaikoff, B. Advances in Mid-Infrared Spectroscopy for Chemical Analysis. Annu. Rev. Anal. Chem. 2016, 9, 45–68. [Google Scholar]
  26. Bukowski, R.W. Smoke measurements in large- and small-scale fire testing—Part I. Fire Technol. 1979, 15, 173–179. [Google Scholar]
  27. Chen, Y.; Xue, L.; Zhu, Q.; Feng, Y.; Wu, M. Recent advances in second near-infrared region (NIR-II) fluorophores and biomedical applications. Front. Chem. 2021, 9, 750404. [Google Scholar]
  28. Yu, J.; Zhang, R.; Gao, Y.; Sheng, Z.; Gu, M.; Sun, Q.; Liao, J.; Wu, T.; Lin, Z.; Wu, P.; et al. Intravital confocal fluorescence lifetime imaging microscopy in the second near-infrared window. Opt. Lett. 2020, 45, 3305–3308. [Google Scholar]
  29. Ravaro, M.; Locatelli, M.; Pugliese, E.; Di Leo, I.; de Cumis, M.S.; D’Amato, F.; Poggi, P.; Consolino, L.; Meucci, R.; Ferraro, P.; et al. Mid-infrared digital holography and holographic interferometry with a tunable quantum cascade laser. Opt. Lett. 2014, 39, 4843. [Google Scholar]
  30. Locatelli, M.; Pugliese, E.; Paturzo, M.; Bianco, V.; Finizio, A.; Pelagotti, A.; Poggi, P.; Miccio, L.; Meucci, R.; Ferraro, P. Imaging live humans through smoke and flames using far-infrared digital holography. Opt. Express 2013, 21, 5379. [Google Scholar]
  31. Sanna, M.; Rizzotti, D.; Signorini, S.; Pavesi, L. An integrated entangled photons source for mid-infrared ghost spectroscopy. In Quantum Sensing and Nano Electronics and Photonics XVIII, Proceedings of the SPIE OPTO, San Francisco, CA, USA, 22 January–28 February 2022; SPIE: Bellingham, WA, USA, 2022. [Google Scholar]
  32. Eisaman, M.D.; Fan, J.; Migdall, A.; Polyakov, S.V. Invited Review Article: Single-photon sources and detectors. Rev. Sci. Instrum. 2011, 82, 071101. [Google Scholar]
  33. Hadfield, R.H. Single-photon detectors for optical quantum information applications. Nat. Photonics 2009, 3, 696–705. [Google Scholar]
  34. Buller, G.S.; Collins, R.J. Single-photon generation and detection. Meas. Sci. Technol. 2010, 21, 012002. [Google Scholar]
  35. Chen, Q.; Ge, R.; Zhang, L.; Li, F.; Zhang, B.; Jin, F.; Han, H.; Dai, Y.; He, G.; Fei, Y.; et al. Mid-infrared single photon detector with superconductor Mo80Si20 nanowire. arXiv 2020, arXiv:2011.06699, preprint. [Google Scholar]
  36. Gol’Tsman, G.N.; Okunev, O.; Chulkova, G.; Lipatov, A.; Semenov, A.; Smirnov, K.; Voronov, B.; Dzardanov, A.; Williams, C.; Sobolewski, R. Picosecond superconducting single-photon optical detector. Appl. Phys. Lett. 2001, 79, 705–707. [Google Scholar]
  37. Engel, A.; Renema, J.J.; Il’In, K.; Semenov, A. Detection mechanism of superconducting nanowire single-photon detectors. Supercond. Sci. Technol. 2015, 28, 114003. [Google Scholar]
  38. Korneeva, Y.P.; Mikhailov, M.Y.; Pershin, Y.P.; Manova, N.N.; Divochiy, A.V.; Vakhtomin, Y.B.; Korneev, A.A.; Smirnov, K.V.; Sivakov, A.G.; Devizenko, A.Y.; et al. Superconducting single-photon detector made of MoSi film. Supercond. Sci. Technol. 2014, 27, 095012. [Google Scholar]
  39. Zadeh, I.E.; Chang, J.; Los, J.W.N.; Gyger, S.; Elshaari, A.W.; Steinhauer, S.; Dorenbos, S.N.; Zwiller, V. Superconducting nanowire single-photon detectors: A perspective on evolution, state-of-the-art, future developments, and applications. Appl. Phys. Lett. 2021, 118, 190502. [Google Scholar]
  40. Holzman, I.; Ivry, Y. Superconducting Nanowires for Single-Photon Detection: Progress, Challenges, and Opportunities. Adv. Quantum Technol. 2019, 2, 1800058. [Google Scholar]
  41. Wang, Y.; Li, H.; You, L.X.; Lv, C.L.; Wang, H.Q.; Zhang, X.Y.; Zhang, W.J.; Zhou, H.; Zhang, L.; Yang, X.Y.; et al. Wavelength dependence of intrinsic detection efficiency of NbN superconducting nanowire single-photon detector. Chin. Phys. B 2019, 28, 078. [Google Scholar]
  42. Marsili, F.; Verma, V.B.; Stern, J.A.; Harrington, S.; Lita, A.E.; Gerrits, T.; Vayshenker, I.; Baek, B.; Shaw, M.D.; Mirin, R.; et al. Detecting Single Infrared Photons with 93% System Efficiency. Nat. Photonics 2012, 7, 210–214. [Google Scholar]
  43. Shibata, H.; Shimizu, K.; Takesue, H.; Tokura, Y. Ultimate low system dark-count rate for superconducting nanowire single-photon detector. Opt. Lett. 2015, 40, 3428. [Google Scholar]
  44. Korzh, B.; Zhao, Q.-Y.; Allmaras, J.; Frasca, S.; Autry, T.M.; Bersin, E.A.; Beyer, A.D.; Briggs, R.M.; Bumble, B.; Colangelo, M.; et al. Demonstrating sub-3 ps temporal resolution in a superconducting nanowire single-photon detector. Nat. Photon 2020, 14, 250–255. Available online: http://arxiv.org/abs/1804.06839 (accessed on 5 March 2022).
  45. Vetter, A.; Ferrari, S.; Rath, P.; Alaee, R.; Kahl, O.; Kovalyuk, V.; Diewald, S.; Goltsman, G.N.; Korneev, A.; Rockstuhl, C.; et al. Cavity-Enhanced and Ultrafast Superconducting Single-Photon Detectors. Nano Lett. 2016, 16, 7085–7092. [Google Scholar]
  46. Yamashita, T.; Miki, S.; Terai, H. Recent Progress and Application of Superconducting Nanowire Single-Photon Detectors. IEICE Trans. Electron. 2017, E100.C, 274–282. [Google Scholar]
  47. Korneev, A.; Korneeva, Y.; Florya, I.; Voronov, B.; Goltsman, G. NbN Nanowire Superconducting Single-Photon Detector for Mid-Infrared. Phys. Procedia 2012, 36, 72–76. [Google Scholar]
  48. Verma, V.B.; Korzh, B.; Walter, A.B.; Lita, A.E.; Briggs, R.M.; Colangelo, M.; Zhai, Y.; Wollman, E.E.; Beyer, A.D.; Allmaras, J.P.; et al. Single-photon detection in the mid-infrared up to 10 μm wavelength using tungsten silicide superconducting nanowire detectors. APL Photonics 2021, 6, 056101. [Google Scholar]
  49. Marsili, F.; Bellei, F.; Najafi, F.; Dane, A.E.; Dauler, E.A.; Molnar, R.J.; Berggren, K.K. Efficient Single Photon Detection from 500 nm to 5 μm Wavelength. Nano Lett. 2012, 12, 4799–4804. [Google Scholar]
  50. Marsili, F.; Najafi, F.; Dauler, E.; Bellei, F.; Hu, X.; Csete, M.; Molnar, R.J.; Berggren, K.K. Single-photon detectors based on ultra-narrow superconducting nanowires. Nano Lett. 2011, 11, 2048–2053. [Google Scholar]
  51. Gisin, N.; Thew, R. Quantum Communication. Nat. Photonics 2007, 1, 165–171. [Google Scholar]
  52. Zolotov, P.I.; Divochiy, A.V.; Vakhtomin, Y.B.; Morozov, P.V.; Seleznev, V.A.; Smirnov, K.V. Development of high-effective superconducting single-photon detectors aimed for mid-IR spectrum range. J. Phys. Conf. Ser. 2017, 917, 062037. [Google Scholar]
  53. Chang, J.; Los, J.W.; Gourgues, R.; Steinhauer, S.; Dorenbos, S.N.; Pereira, S.F.; Urbach, H.P.; Zwiller, V.; Zadeh, I.E. Efficient mid-infrared single-photon detection using superconducting NbTiN nanowires with high time resolution in a Gifford-McMahon cryocooler. Photonics Res. 2022, 10, 1063. [Google Scholar]
  54. Baek, B.; Lita, A.E.; Verma, V.; Nam, S.W. Superconducting a-WxSi1−x nanowire single-photon detector with saturated internal quantum efficiency from visible to 1850 nm. Appl. Phys. Lett. 2011, 98, 251105. [Google Scholar]
  55. Taylor, G.G.; Morozov, D.; Gemmell, N.R.; Erotokritou, K.; Miki, S.; Terai, H.; Hadfield, R.H. Photon counting LIDAR at 2.3 µm wavelength with superconducting nanowires. Opt. Express 2019, 27, 38147–38158. [Google Scholar]
  56. Guan, Y.; Li, H.; Xue, L.; Yin, R.; Zhang, L.; Wang, H.; Zhu, G.; Kang, L.; Chen, J.; Wu, P. Lidar with superconducting nanowire single-photon detectors: Recent advances and developments. Opt. Lasers Eng. 2022, 156, 107102. [Google Scholar]
  57. Rockwell, A.K.; Ren, M.; Woodson, M.; Jones, A.; March, S.D.; Tan, Y.; Yuan, Y.; Sun, Y.; Hool, R.; Maddox, S.J.; et al. Toward deterministic construction of low noise avalanche photodetector materials. Appl. Phys. Lett. 2018, 113, 102106. [Google Scholar]
  58. Lee, S.; Kodati, S.H.; Guo, B.; Jones, A.H.; Schwartz, M.; Winslow, M.; Grein, C.H.; Ronningen, T.J.; Campbell, J.C.; Krishna, S. Low noise Al0.85Ga0.15As0.56Sb0.44 avalanche photodiodes on InP substrates. Appl. Phys. Lett. 2021, 118, 081106. [Google Scholar]
  59. Yi, X.; Xie, S.; Liang, B.; Lim, L.W.; Cheong, J.S.; Debnath, M.C.; Huffaker, D.L.; Tan, C.H.; David, J.P.R. Extremely low excess noise and high sensitivity AlAs0.56Sb0.44 avalanche photodiodes. Nat. Photonics 2019, 13, 683–686. [Google Scholar]
  60. Kang, Y.; Zadka, M.; Litski, S.; Sarid, G.; Morse, M.; Paniccia, M.J.; Kuo, Y.H.; Bowers, J.; Beling, A.; Liu, H.D.; et al. Epitaxially-grown Ge/Si avalanche photodiodes for 1.3 μm light detection. Opt. Express 2008, 16, 9365. [Google Scholar]
  61. Zhang, J.; Itzler, M.A.; Zbinden, H.; Pan, J.W. Advances in InGaAs/InP single-photon detector systems for quantum communication. Light Sci. Appl. 2015, 4, 286. [Google Scholar]
  62. Ribordy, G.; Gautier, J.-D.; Zbinden, H.; Gisin, N. Performance of InGaAs/InP avalanche photodiodes as gated-mode photon counters. Appl. Opt. 1998, 37, 2272–2277. [Google Scholar]
  63. Namekata, N.; Sasamori, S.; Inoue, S. 800 MHz single-photon detection at 1550-nm using an InGaAs/InP avalanche photodiode operated with a sine wave gating. Opt. Express 2006, 14, 10043–10049. [Google Scholar]
  64. Jiang, W.-H.; Gao, X.-J.; Fang, Y.-Q.; Liu, J.-H.; Zhou, Y.; Jiang, L.-Q.; Chen, W.; Jin, G.; Zhang, J.; Pan, J.-W. Miniaturized high-frequency sine wave gating InGaAs/InP single-photon detector. Rev. Sci. Instrum. 2018, 89, 123104. [Google Scholar]
  65. Yuan, Z.; Kardynal, B.E.; Sharpe, A.W.; Shields, A.J. High speed single photon detection in the near infrared. Appl. Phys. Lett. 2007, 91, 041114. [Google Scholar]
  66. Lu, Z.; Sun, W.; Zheng, X.; Campbell, J.; Jiang, X.; Itzler, M.A. Balanced InGaAs/InP avalanche photodiodes for single photon detection. In Biosensing and Nanomedicine V, Proceedings of the SPIE NanoScience + Engineering, San Diego, CA, USA, 12–16 August 2012; SPIE: Bellingham, WA, USA, 2012; Volume 8460. [Google Scholar]
  67. Kopytko, M.; Sobieski, J.; Gawron, W.; Martyniuk, P. Study of HgCdTe (100) and HgCdTe (111)B Heterostructures Grown by MOCVD and Their Potential Application to APDs Operating in the IR Range up to 8 µm. Sensors 2022, 22, 924. [Google Scholar]
  68. Dehzangi, A.; Li, J.; Gautam, L.; Razeghi, M. Avalanche Photodetector Based on InAs/InSb Superlattice. Quantum Rep. 2020, 2, 591–599. [Google Scholar]
  69. Li, J.; Dehzangi, A.; Brown, G.; Razeghi, M. Mid-wavelength infrared avalanche photodetector with AlAsSb/GaSb superlattice. Sci. Rep. 2021, 11, 7104. [Google Scholar]
  70. Huang, J.; Zhao, C.; Nie, B.; Xie, S.; Kwan, D.C.M.; Meng, X.; Zhang, Y.; Huffaker, D.L.; Ma, W. High-performance mid-wavelength InAs avalanche photodiode using AlAs013Sb087 as the multiplication layer. Photonics Res. 2020, 8, 755. [Google Scholar]
  71. Mancinelli, M.; Trenti, A.; Piccione, S.; Fontana, G.; Dam, J.S.; Tidemand-Lichtenberg, P.; Pedersen, C.; Pavesi, L. Mid-infrared coincidence measurements on twin photons at room temperature. Nat. Commun. 2017, 8, 15184. [Google Scholar]
  72. Dam, J.S.; Pedersen, C.; Tidemand-Lichtenberg, P. Room temperature mid-IR single photon spectral imaging. Nat. Photonics 2012, 6, 788–793. [Google Scholar]
  73. Huang, K.; Wang, Y.; Fang, J.; Kang, W.; Sun, Y.; Liang, Y.; Hao, Q.; Yan, M.; Zeng, H. Mid-infrared photon counting and resolving via efficient frequency upconversion. Photonics Res. 2021, 9, 259. [Google Scholar]
  74. Rogalski, A. HgCdTe infrared detector material: History, status and outlook. Rep. Prog. Phys. 2005, 68, 2267–2336. [Google Scholar]
  75. Rogalski, A.; Antoszewski, J.; Faraone, L. Third-generation infrared photodetector arrays. J. Appl. Phys. 2009, 105, 091101. [Google Scholar]
  76. Yadav, P.V.K.; Ajitha, B.; Kumar Reddy, Y.A.; Sreedhar, A. Recent advances in development of nanostructured photodetectors from ultraviolet to infrared region: A review. Chemosphere 2021, 279, 130473. [Google Scholar]
  77. Gabbrielli, T.; Cappelli, F.; Bruno, N.; Corrias, N.; Borri, S.; De Natale, P.; Zavatta, A. Mid-infrared homodyne balanced detector for quantum light characterization. Opt. Express 2021, 29, 14536. [Google Scholar]
  78. Riek, C.; Seletskiy, D.V.; Moskalenko, A.S.; Schmidt, J.F.; Krauspe, P.; Eckart, S.; Eggert, S.; Burkard, G.; Leitenstorfer, A. Direct sampling of electric-field vacuum fluctuations. Science 2015, 350, 420–423. [Google Scholar]
  79. Pupeza, I.; Huber, M.; Trubetskov, M.; Schweinberger, W.; Hussain, S.A.; Hofer, C.; Fritsch, K.; Poetzlberger, M.; Vamos, L.; Fill, E.; et al. Field-resolved infrared spectroscopy of biological systems. Nature 2020, 577, 52–59. [Google Scholar]
  80. Kowligy, A.S.; Timmers, H.; Lind, A.J.; Elu, U.; Cruz, F.C.; Schunemann, P.G.; Biegert, J.; Diddams, S.A. Infrared electric field sampled frequency comb spectroscopy. Sci. Adv. 2019, 5, 8794. [Google Scholar]
Photonics 09 00470 g001 550
Figure 1. Rising number of publications on mid-infrared photon detectors and their applications in the last 50 years. The number of publications per year was obtained from the Google Scholar database [15], using the keywords “mid-infrared single-photon detection” (black squares), “mid-infrared quantum cryptography” (red circles), and “mid-infrared single-photon spectroscopy” (blue triangles).
Figure 1. Rising number of publications on mid-infrared photon detectors and their applications in the last 50 years. The number of publications per year was obtained from the Google Scholar database [15], using the keywords “mid-infrared single-photon detection” (black squares), “mid-infrared quantum cryptography” (red circles), and “mid-infrared single-photon spectroscopy” (blue triangles).
Photonics 09 00470 g001
Photonics 09 00470 g002 550
Figure 2. (a) A typical SNSPD structure sketch; (b) detection mechanism of an SNSPD reproduced from [39] with the permission of AIP Publishing. Photon absorption (I) generates quasi-particles and phonons (II), with the consequent breakdown of superconductivity (III). The resistivity returns to zero spontaneously (IV), and the charge passage due to the bias current is restored within the reset time (V). Center: voltage readout signal. SS: steady state of the SNSPD. Reproduced with permission of AIP Publishing from [39], copyright 2021.
Figure 2. (a) A typical SNSPD structure sketch; (b) detection mechanism of an SNSPD reproduced from [39] with the permission of AIP Publishing. Photon absorption (I) generates quasi-particles and phonons (II), with the consequent breakdown of superconductivity (III). The resistivity returns to zero spontaneously (IV), and the charge passage due to the bias current is restored within the reset time (V). Center: voltage readout signal. SS: steady state of the SNSPD. Reproduced with permission of AIP Publishing from [39], copyright 2021.
Photonics 09 00470 g002
Photonics 09 00470 g003 550
Figure 3. Detection efficiency (in color scale) vs. λ and normalized bias current (IB/ISW) for SNSPDs based on 85 nm wide, 50 nm wide, and 30 nm wide nanowires. Each pixel of the color map corresponds to an experimental data point. The red dashed lines are the Ico vs. λ curves of the three detectors. The blue pixels for high bias current and λ < 2 μm are due to the detectors switching to the normal state. Reprinted with permission from [49], copyright 2012.
Figure 3. Detection efficiency (in color scale) vs. λ and normalized bias current (IB/ISW) for SNSPDs based on 85 nm wide, 50 nm wide, and 30 nm wide nanowires. Each pixel of the color map corresponds to an experimental data point. The red dashed lines are the Ico vs. λ curves of the three detectors. The blue pixels for high bias current and λ < 2 μm are due to the detectors switching to the normal state. Reprinted with permission from [49], copyright 2012.
Photonics 09 00470 g003
Photonics 09 00470 g004 550
Figure 4. Normalized photon count rate vs. bias current curves for SNSPDs fabricated from a WSi film with silicon content of 48 ± 10%. Two different nanowire widths (50 and 70 nm) are presented, and measurements were obtained at three wavelengths (4.8, 7.4, and 9.9 μm) at an operating temperature of 0.85 K. Black squares correspond to the measurement of the background count rates. Adapted and reproduced from [48], with the permission of AIP Publishing, copyright 2021.
Figure 4. Normalized photon count rate vs. bias current curves for SNSPDs fabricated from a WSi film with silicon content of 48 ± 10%. Two different nanowire widths (50 and 70 nm) are presented, and measurements were obtained at three wavelengths (4.8, 7.4, and 9.9 μm) at an operating temperature of 0.85 K. Black squares correspond to the measurement of the background count rates. Adapted and reproduced from [48], with the permission of AIP Publishing, copyright 2021.
Photonics 09 00470 g004
Photonics 09 00470 g005 550
Figure 5. Sketch of a single-photon avalanche diode (SPAD). The voltage is applied to accelerate the electrons toward the multiplication region. A front-illuminated geometry is illustrated, with antireflective (AR) coating to improve efficiency, but back-illuminated designs are also used. Reproduced from [32], with the permission of AIP Publishing, copyright 2011.
Figure 5. Sketch of a single-photon avalanche diode (SPAD). The voltage is applied to accelerate the electrons toward the multiplication region. A front-illuminated geometry is illustrated, with antireflective (AR) coating to improve efficiency, but back-illuminated designs are also used. Reproduced from [32], with the permission of AIP Publishing, copyright 2011.
Photonics 09 00470 g005
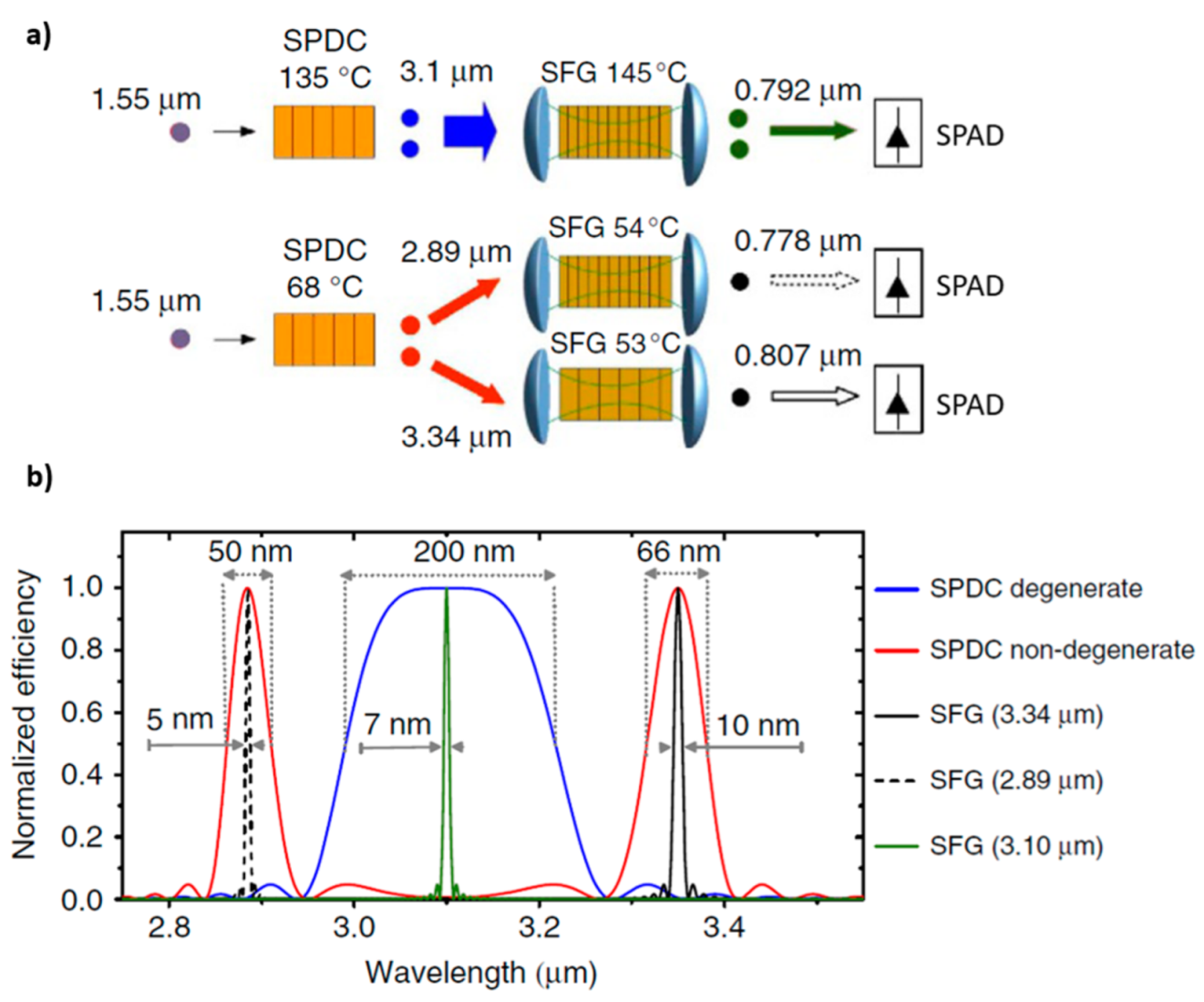
Photonics 09 00470 g006 550
Figure 6. (a) Schemes of the generation and detection stages in the case of degenerate (top) and nondegenerate (bottom) SPDC and SFG processes; (b) simulated downconversion spectra and module bandwidth as a function of the temperature. The solid blue curve refers to the degenerate downconversion process around 3.1 mm, which was achieved at 135 °C. The solid red curve refers to the nondegenerate downconversion process, which was achieved at 68 °C. Adapted with permission from [71], copyright 2017.
Figure 6. (a) Schemes of the generation and detection stages in the case of degenerate (top) and nondegenerate (bottom) SPDC and SFG processes; (b) simulated downconversion spectra and module bandwidth as a function of the temperature. The solid blue curve refers to the degenerate downconversion process around 3.1 mm, which was achieved at 135 °C. The solid red curve refers to the nondegenerate downconversion process, which was achieved at 68 °C. Adapted with permission from [71], copyright 2017.
Photonics 09 00470 g006
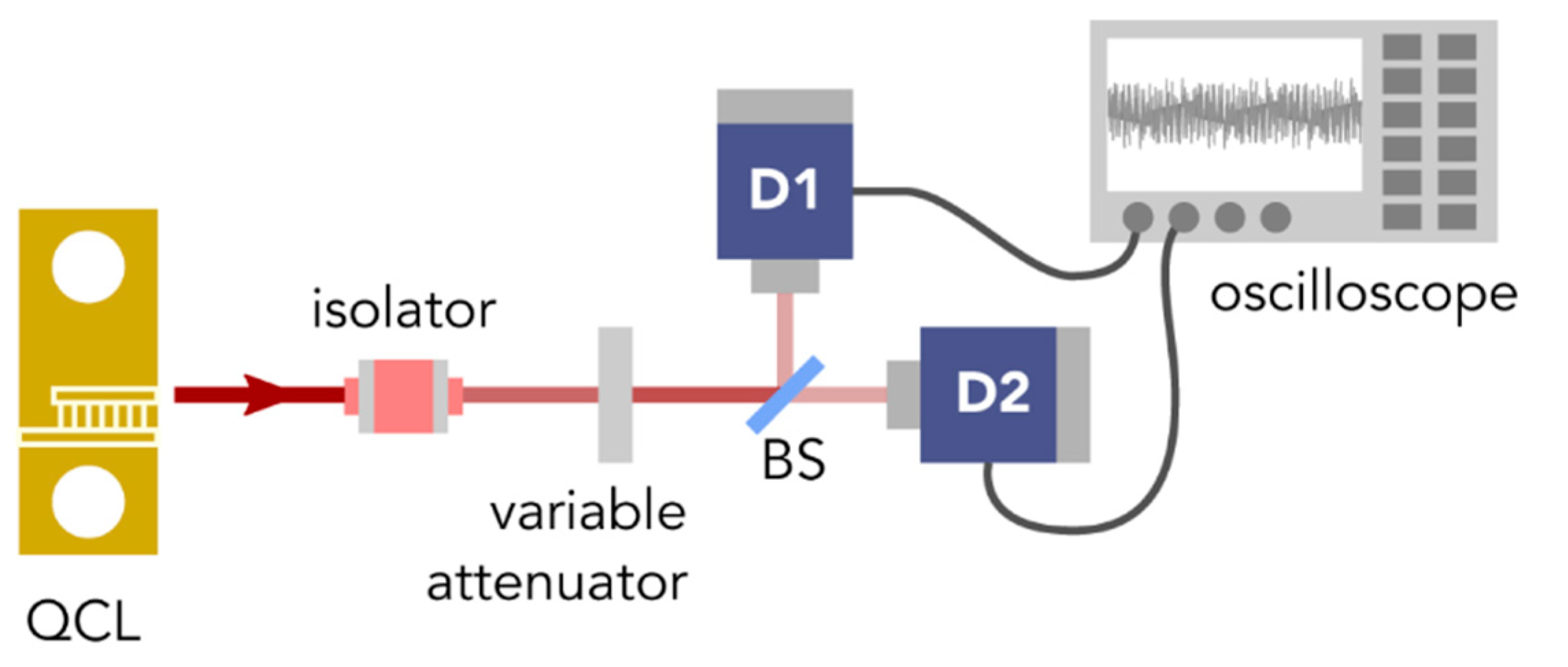
Photonics 09 00470 g007 550
Figure 7. Sketch of the BHD characterization setup. A single-mode QCL is used as a local oscillator (LO) and sent on a BHD made of a 50/50 beam splitter (BS) and two HgCdTe photovoltaic detectors. After a preamplification stage, the detector output AC signals are acquired in the time domain, using an oscilloscope. To avoid any detector power saturation, a variable attenuator is used to control the incident laser power. Reprinted with permission from [77], copyright © The Optical Society 2021.
Figure 7. Sketch of the BHD characterization setup. A single-mode QCL is used as a local oscillator (LO) and sent on a BHD made of a 50/50 beam splitter (BS) and two HgCdTe photovoltaic detectors. After a preamplification stage, the detector output AC signals are acquired in the time domain, using an oscilloscope. To avoid any detector power saturation, a variable attenuator is used to control the incident laser power. Reprinted with permission from [77], copyright © The Optical Society 2021.
Photonics 09 00470 g007
Table
Table 1. Overview and comparison of SNSPD, SPAD, and PV detectors. Upconversion detection is highlighted with *.
Table 1. Overview and comparison of SNSPD, SPAD, and PV detectors. Upconversion detection is highlighted with *.
Detector TypeMaterialWavelength
(µm)		ηQEηDEDCRT (K)Ref
SNSPDNbN0.5–5 0.5–5.5%100 cps1.5[49]
WSi4.8; 7.4; 9.9100% 100 cps0.85[48]
Mo80Si201.55–5.07100% <100 cps0.08[35]
NbTiN2–4100% up to 3 µm70% @ 2 µm 2.5[53]
SPAD *Si-based3.165%0.35%1 kcps300[71]
Si-based3 37% [73]
PVHgCdTe4.47
4.72		41%
33%		 300[77]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Share and Cite

MDPI and ACS Style

Dello Russo, S.; Elefante, A.; Dequal, D.; Pallotti, D.K.; Santamaria Amato, L.; Sgobba, F.; Siciliani de Cumis, M. Advances in Mid-Infrared Single-Photon Detection. Photonics 2022, 9, 470. https://doi.org/10.3390/photonics9070470

AMA Style

Dello Russo S, Elefante A, Dequal D, Pallotti DK, Santamaria Amato L, Sgobba F, Siciliani de Cumis M. Advances in Mid-Infrared Single-Photon Detection. Photonics. 2022; 9(7):470. https://doi.org/10.3390/photonics9070470

Chicago/Turabian Style

Dello Russo, Stefano, Arianna Elefante, Daniele Dequal, Deborah Katia Pallotti, Luigi Santamaria Amato, Fabrizio Sgobba, and Mario Siciliani de Cumis. 2022. "Advances in Mid-Infrared Single-Photon Detection" Photonics 9, no. 7: 470. https://doi.org/10.3390/photonics9070470

APA Style

Dello Russo, S., Elefante, A., Dequal, D., Pallotti, D. K., Santamaria Amato, L., Sgobba, F., & Siciliani de Cumis, M. (2022). Advances in Mid-Infrared Single-Photon Detection. Photonics, 9(7), 470. https://doi.org/10.3390/photonics9070470

Note that from the first issue of 2016, this journal uses article numbers instead of page numbers. See further details here.

Article Metrics

Back to TopTop