On Directly Modulated Reflective Semiconductor Optical Amplifier with Assistance of Birefringent Fiber Loop
Abstract
:1. Introduction
2. Setup and Modeling
2.1. Setup
2.2. Modeling
2.2.1. RSOA Input
2.2.2. RSOA Response
2.2.3. BFL Response
2.2.4. RSOA-BFL Output
2.2.5. Solution
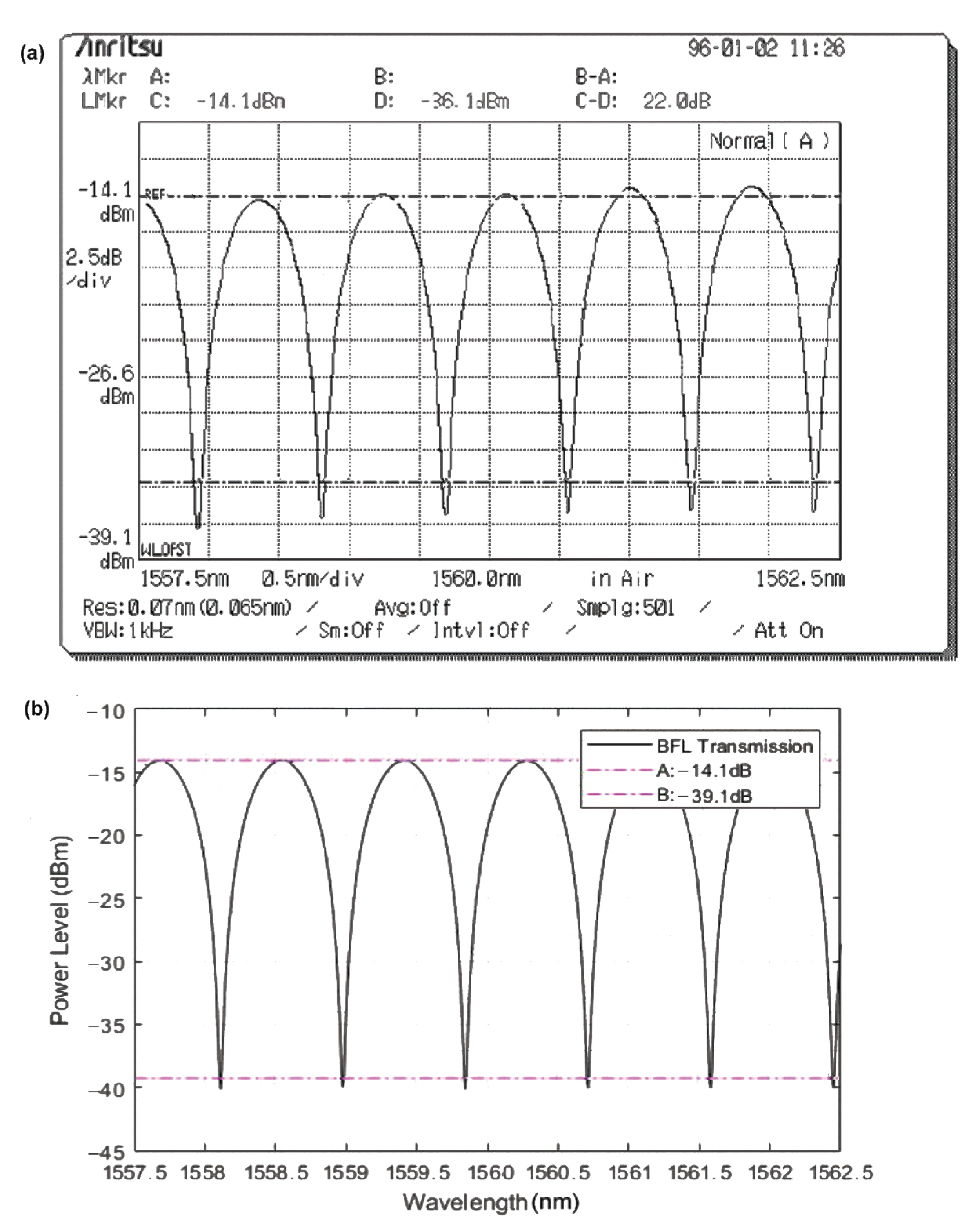
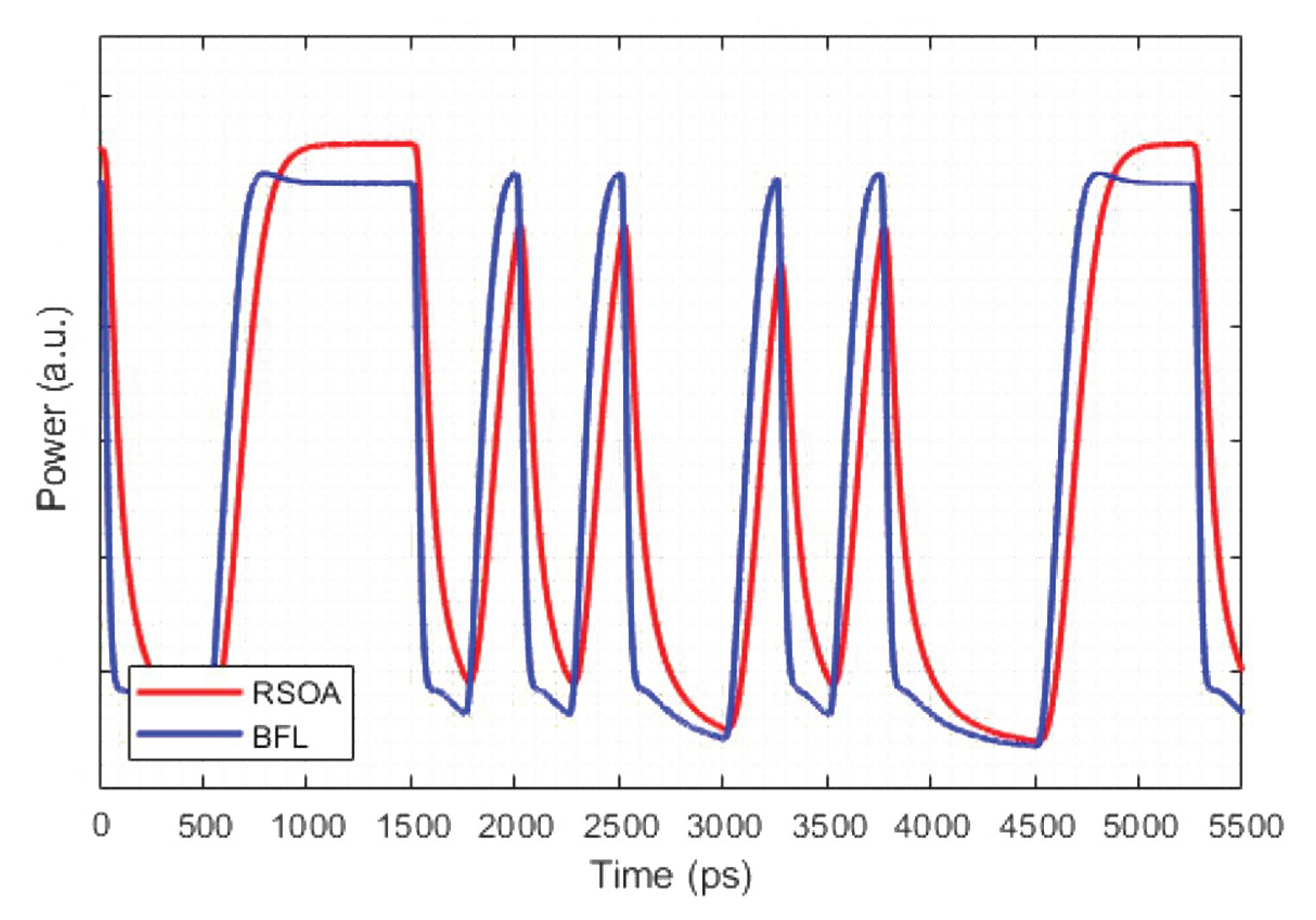
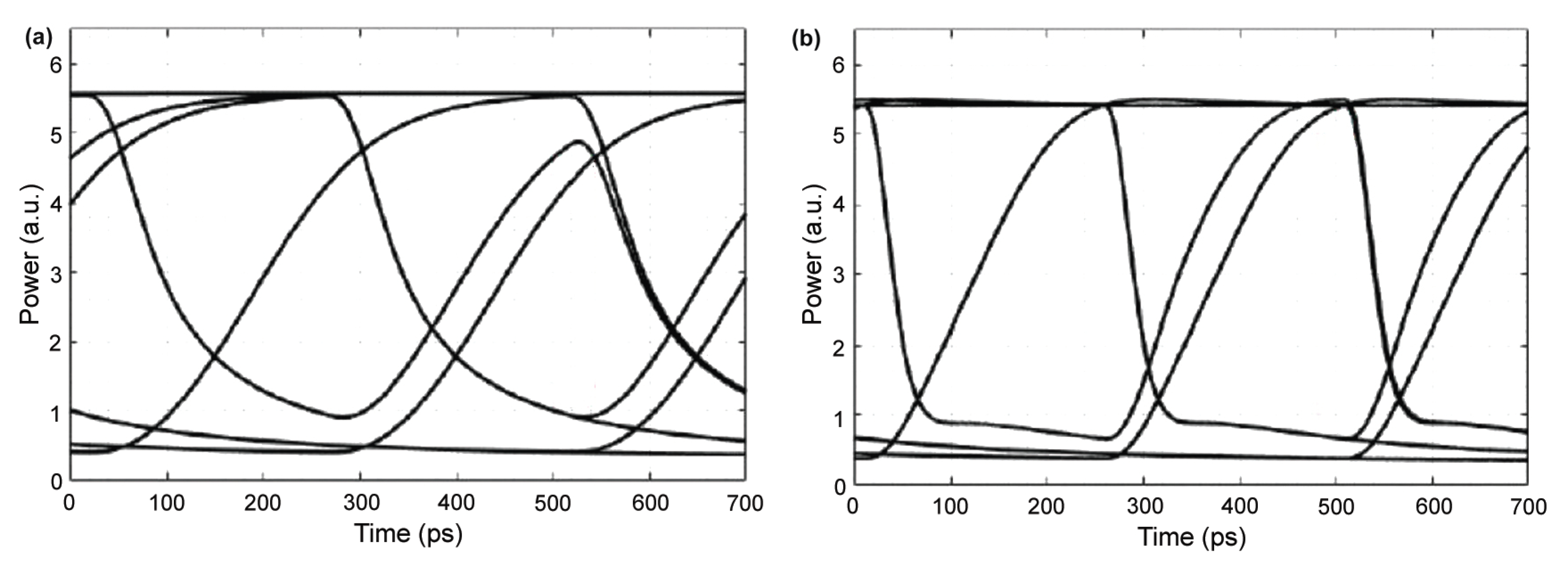
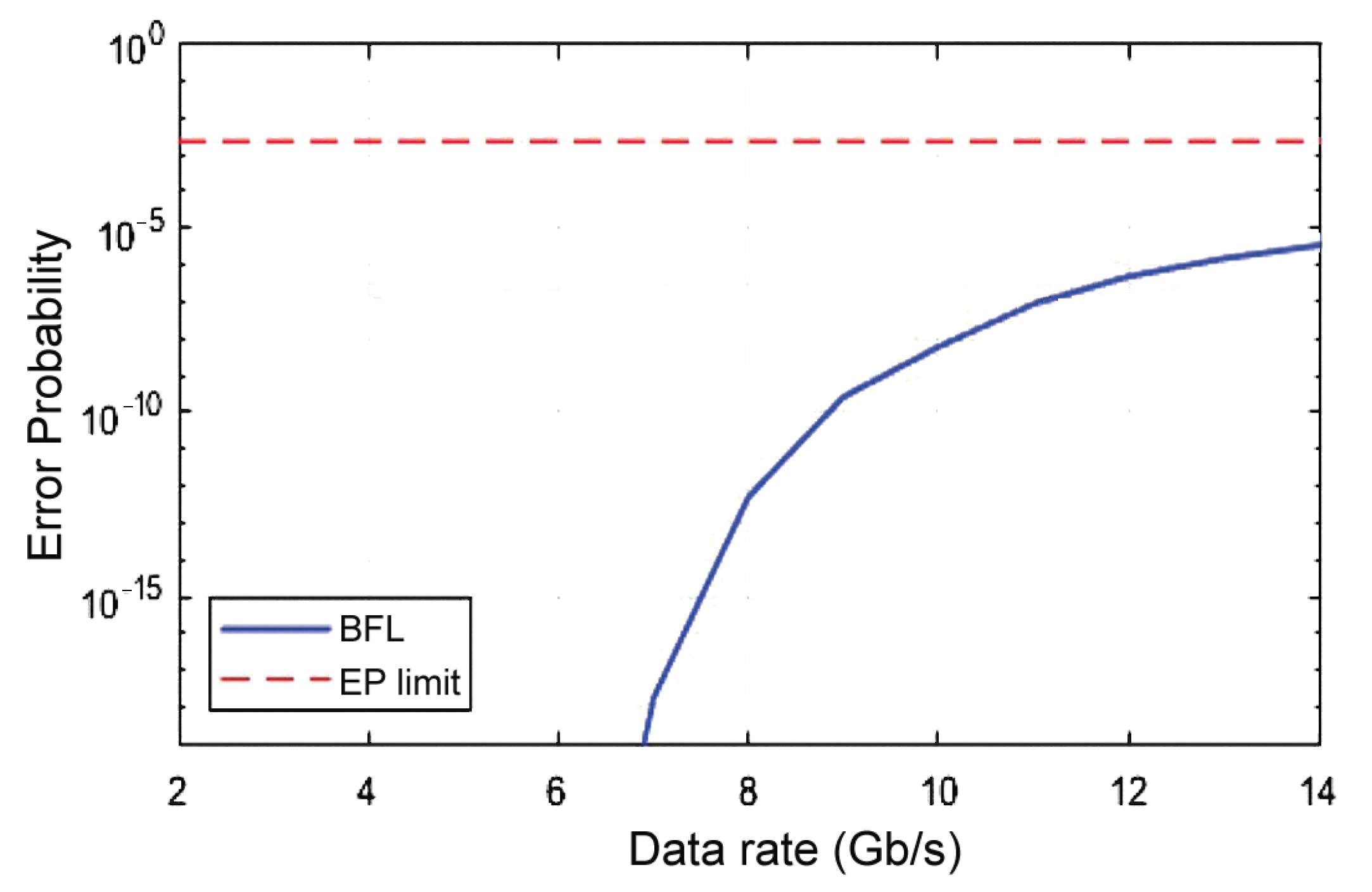
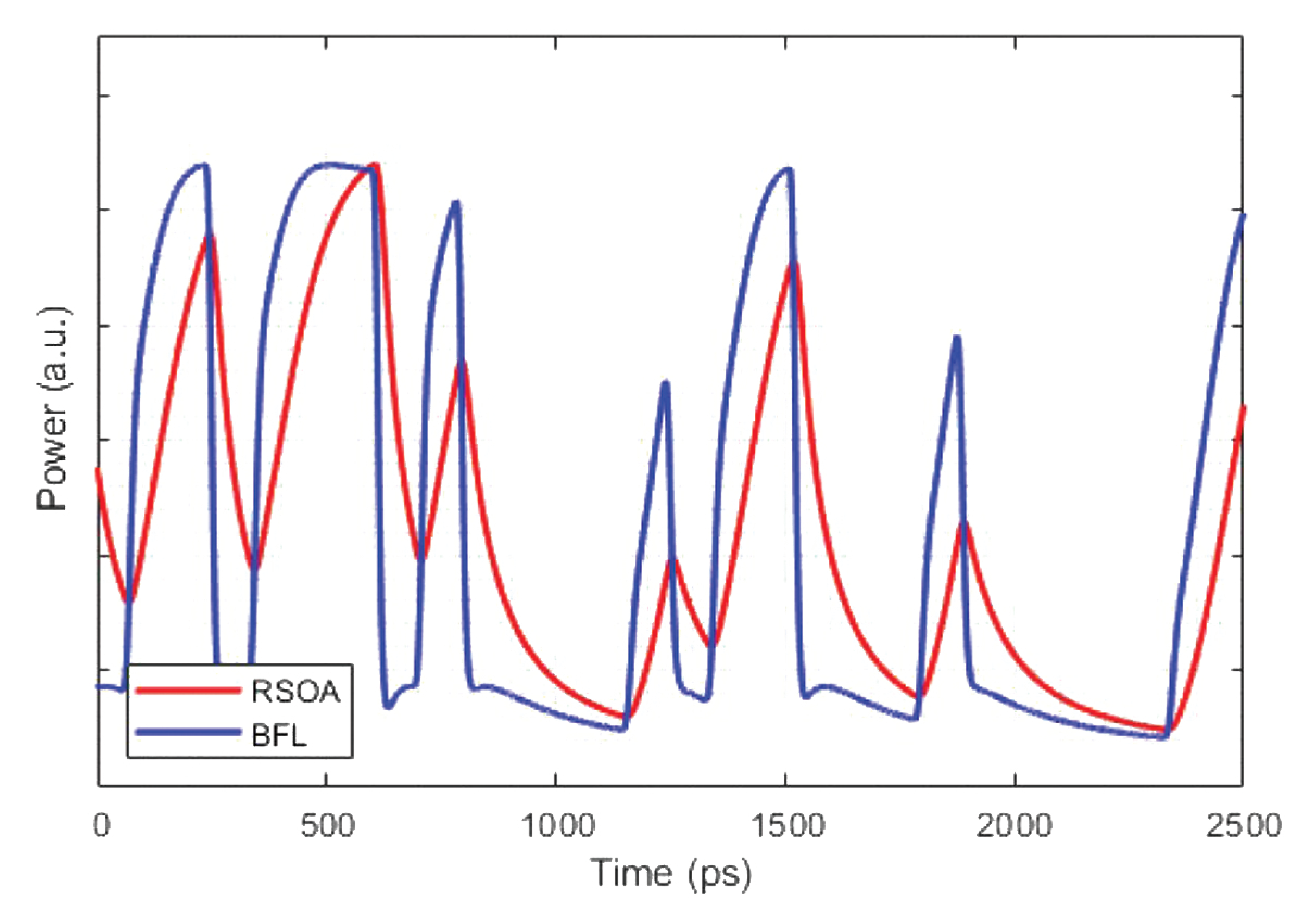
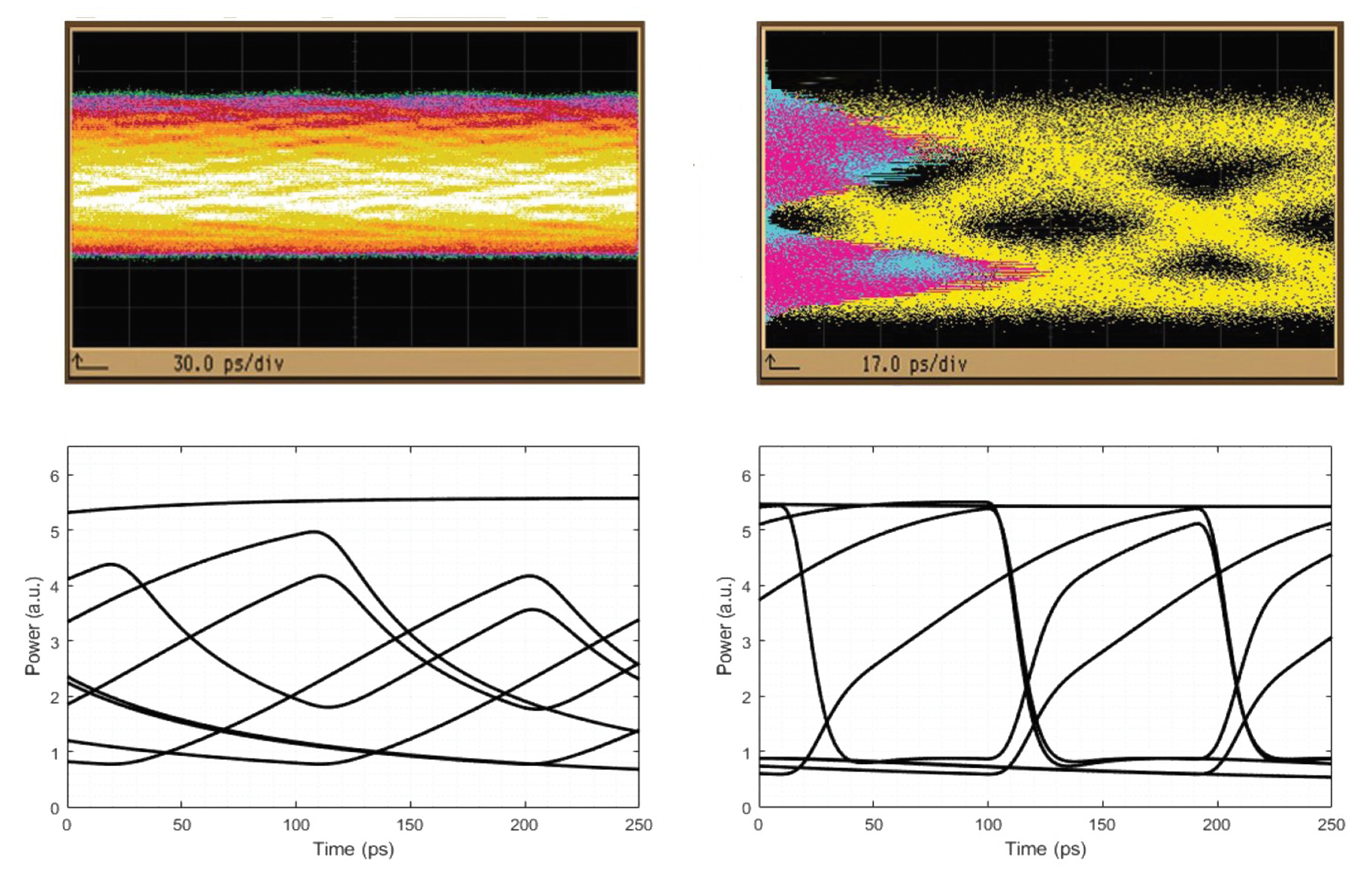
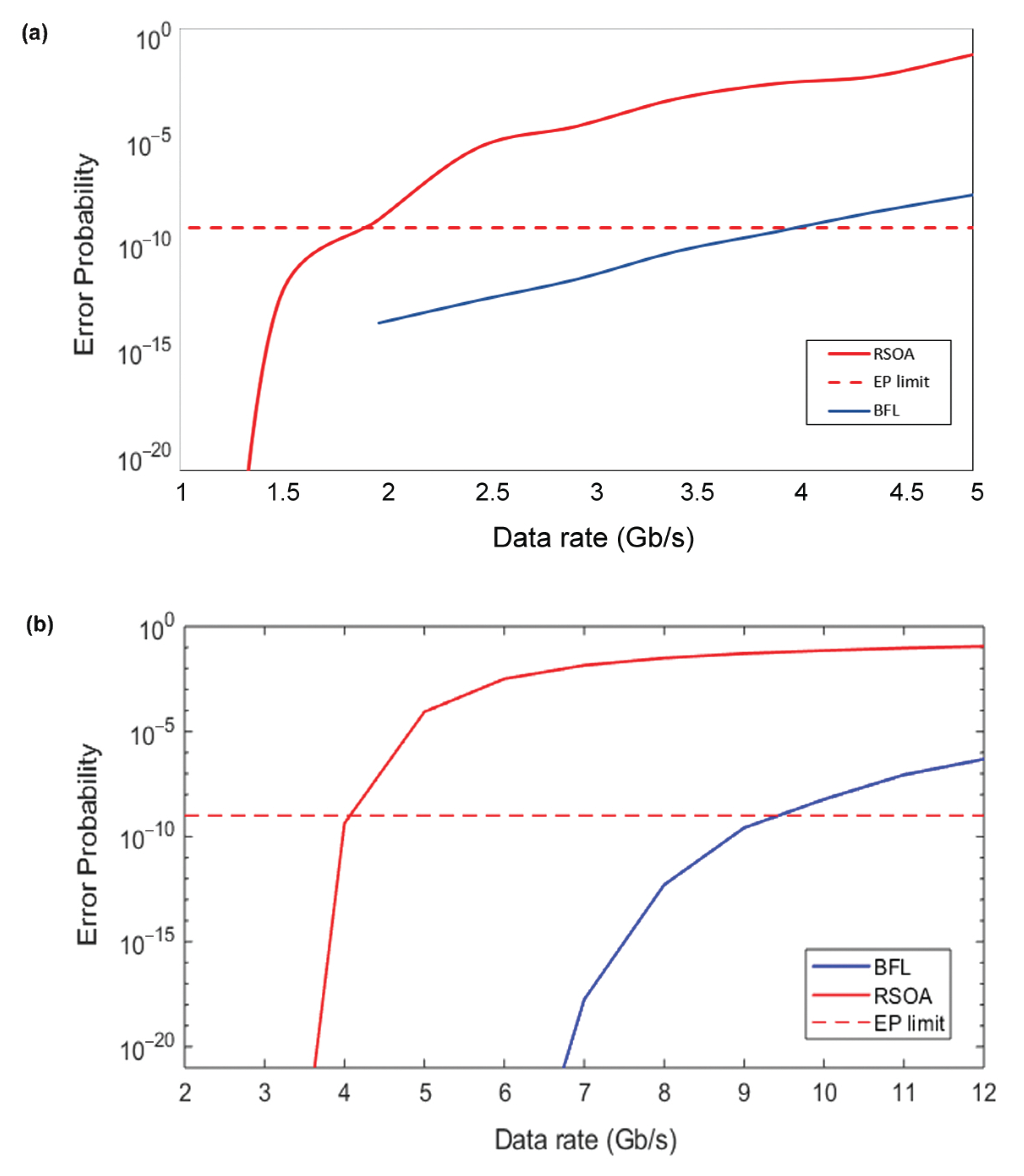
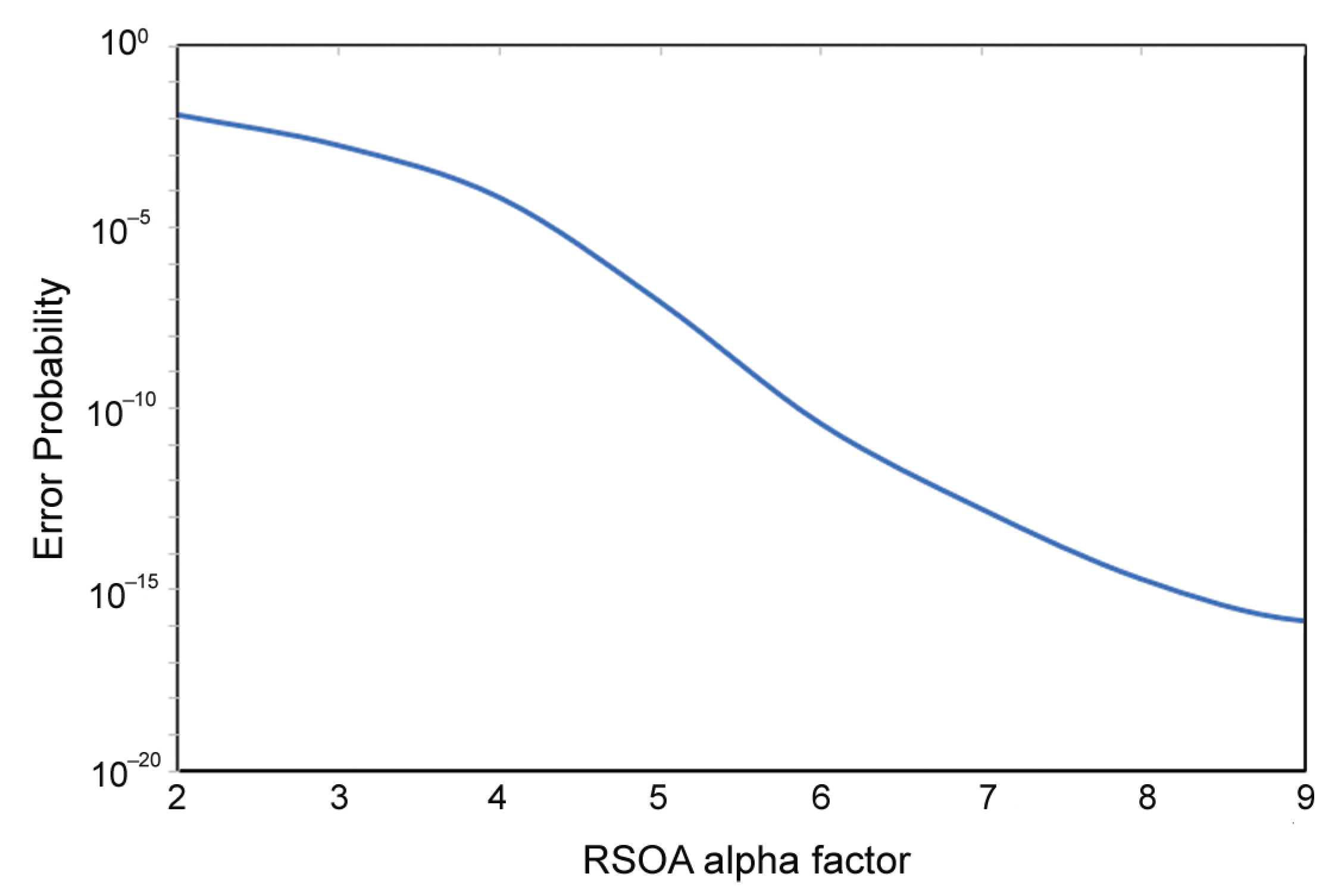
3. Model Validation and Results
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Saliou, F.; Simon, G.; Chanclou, P.; Pizzinat, A.; Lin, H.; Zhou, E.; Xu, Z. WDM PONs based on colorless technology. Opt. Fiber Technol. 2015, 26, 126–134. [Google Scholar] [CrossRef]
- Udvary, E. Performance optimization of RSOA based mm-wave radio-over-fibre access network. Opt. Quantum Electron. 2021, 53, 1–14. [Google Scholar] [CrossRef]
- Meehan, A.; Connelly, M.J. Experimental characterization and modeling of the improved low frequency response of a current modulated bulk RSOA slow light based microwave phase shifter. Opt. Commun. 2015, 341, 241–244. [Google Scholar] [CrossRef]
- Totović, A.; Gvozdić, D. Traveling-wave and reflective semiconductor optical amplifiers. In Handbook of Optoelectronic Device Modeling and Simulation; CRC Press: Boca Raton, FL, USA, 2017; pp. 631–696. [Google Scholar]
- Cho, K.Y.; Takushima, Y.; Chung, Y.C. 10-Gb/s operation of RSOA for WDM PON. IEEE Photon. Technol. Lett. 2008, 20, 1533–1535. [Google Scholar] [CrossRef]
- Totović, A.R.; Crnjanski, J.V.; Krstić, M.M.; Gvozdić, D.M. Numerical study of the small-signal modulation bandwidth of reflective and traveling-wave SOAs. J. Light. Technol. 2015, 33, 2758–2764. [Google Scholar] [CrossRef]
- Babić, J.; Totović, A.; Crnjanski, J.; Krstić, M.; Mašanović, M.; Gvozdić, D. Exploiting Inductive Peaking for Enhancing the RSOA’s Large-Signal Modulation Performance. J. Light. Technol. 2021, 39, 3502–3510. [Google Scholar] [CrossRef]
- Rizou, Z.V.; Zoiros, K.E. Semiconductor optical amplifier dynamics and pattern effects. In Handbook of Optoelectronic Device Modeling and Simulation; CRC Press: Boca Raton, FL, USA, 2017; pp. 771–796. [Google Scholar]
- de Valicourt, G.; Pommereau, F.; Poingt, F.; Lamponi, M.; Duan, G.; Chanclou, P.; Violas, M.; Brenot, R. Chirp reduction in directly modulated multi-electrode RSOA devices in passive optical networks. IEEE Photon. Technol. Lett. 2010, 22, 1425–1427. [Google Scholar] [CrossRef]
- Kim, H. 10-Gb/s operation of RSOA using a delay interferometer. IEEE Photon. Technol. Lett. 2010, 22, 1379–1381. [Google Scholar] [CrossRef]
- Presi, M.; Chiuchiarelli, A.; Corsini, R.; Choudury, P.; Bottoni, F.; Giorgi, L.; Ciaramella, E. Enhanced 10 Gb/s operations of directly modulated reflective semiconductor optical amplifiers without electronic equalization. Opt. Express 2012, 20, B507–B512. [Google Scholar] [CrossRef] [Green Version]
- Su, T.; Zhang, M.; Chen, X.; Zhang, Z.; Liu, M.; Liu, L.; Huang, S. Improved 10-Gbps uplink transmission in WDM-PON with RSOA-based colorless ONUs and MZI-based equalizers. Opt. Laser Technol. 2013, 51, 90–97. [Google Scholar] [CrossRef]
- Zhang, M.; Wang, D.; Cao, Z.; Chen, X.; Huang, S. Suppression of pattern dependence in 10 Gbps upstream transmission of WDM-PON with RSOA-based ONUs. Opt. Commun. 2013, 308, 248–252. [Google Scholar] [CrossRef]
- Shim, H.K.; Kim, H.; Chung, Y.C. 20-Gb/s polar RZ 4-PAM transmission over 20-km SSMF using RSOA and direct detection. IEEE Photon. Technol. Lett. 2015, 27, 1116–1119. [Google Scholar] [CrossRef]
- Bindhaiq, S.; Zulkifli, N.; Supa’at, A.M. The transmission of symmetric 40 Gb/s TWDM-based NG-PON2 utilizing delay interferometer (DI) for RSOA bandwidth enhancement. Opt. Fiber Technol. 2016, 30, 65–71. [Google Scholar] [CrossRef]
- Shim, H.K.; Kim, H.; Chung, Y.C. Effects of electrical and optical equalizations in 28-Gb/s RSOA-based WDM PON. IEEE Photon. Technol. Lett. 2016, 28, 2537–2540. [Google Scholar] [CrossRef]
- Rizou, Z.V.; Zoiros, K.E. Theoretical analysis of directly modulated reflective semiconductor optical amplifier performance enhancement by microring resonator-based notch filtering. Appl. Sci. 2018, 8, 223. [Google Scholar] [CrossRef] [Green Version]
- Zoiros, K.; Morel, P. Enhanced performance of semiconductor optical amplifier at high direct modulation speed with birefringent fiber loop. AIP Adv. 2014, 4, 077107. [Google Scholar] [CrossRef] [Green Version]
- Zoiros, K.E.; Morel, P.; Hamze, M. Performance improvement of directly modulated semiconductor optical amplifier with filter-assisted birefringent fiber loop. Microw. Opt. Technol. Lett. 2015, 57, 2247–2251. [Google Scholar] [CrossRef]
- Engel, T.; Rizou, Z.V.; Zoiros, K.E.; Morel, P. Semiconductor optical amplifier direct modulation with double-stage birefringent fiber loop. Appl. Phys. B Lasers Opt. 2016, 122, 158. [Google Scholar] [CrossRef]
- Rizou, Z.V.; Zoiros, K.E.; Rampone, T.; Sharaiha, A. Reflective semiconductor optical amplifier direct modulation capability enhancement using birefringent fiber loop. Appl. Sci. 2020, 10, 5328. [Google Scholar] [CrossRef]
- Duill, S.O.; Barry, L.P. Improved reduced models for single-pass and reflective semiconductor optical amplifiers. Opt. Commun. 2015, 334, 170–173. [Google Scholar] [CrossRef] [Green Version]
- Antonelli, C.; Mecozzi, A.; Hu, Z.; Santagiustina, M. Analytic study of the modulation response of reflective semiconductor optical amplifiers. J. Light. Technol. 2015, 33, 4367–4376. [Google Scholar] [CrossRef]
- Rizou, Z.V.; Zoiros, K.E.; Hatziefremidis, A.; Connelly, M.J. Performance tolerance analysis of birefringent fiber loop for semiconductor optical amplifier pattern effect suppression. Appl. Phys. B Lasers Opt. 2015, 119, 247–257. [Google Scholar] [CrossRef]
- Udvary, E.; Berceli, T. Improvements in the linearity of semiconductor optical amplifiers as external modulators. IEEE Trans. Microw. Theory Technol. 2010, 58, 3161–3166. [Google Scholar] [CrossRef]
- Gebrewold, S.A. Reflective Semiconductor Optical Amplifiers (RSOAs) as Colorless Sources in Access Networks. Ph.D. Thesis, ETH Zurich, Zurich, Switzerland, 2016. [Google Scholar]
- Shen, T.; Agrawal, G. Pulse-shape effects on frequency chirping in single-frequency semiconductor lasers under current modulation. J. Light. Technol. 1986, 4, 497–503. [Google Scholar] [CrossRef]
- Hamze, M. Study of Different SOA Structures Impact on the Transmission of IMDD OOFDM Signals. Ph.D. Thesis, Université de Bretagne Occidentale (UBO), Brest, France, 2015. [Google Scholar]
- Corless, R.M.; Gonnet, G.H.; Hare, D.E.; Jeffrey, D.J.; Knuth, D.E. On the LambertW function. Adv. Comput. Math. 1996, 5, 329–359. [Google Scholar] [CrossRef]
- Xu, J.; Zhang, X.; Mørk, J. Investigation of patterning effects in ultrafast SOA-based optical switches. IEEE J. Quantum Electron. 2009, 46, 87–94. [Google Scholar] [CrossRef]
- Gutiérrez-Castrejón, R.; Dülk, M.; Guekos, G. Novel scheme for optical time-division demultiplexing using a delayed interferometer. Opt. Commun. 2001, 192, 245–254. [Google Scholar] [CrossRef]
- Agrawal, G.P. Fiber-Optic Communication Systems; John Wiley & Sons: Hoboken, NJ, USA, 2012; Volume 222. [Google Scholar]
- Hui, R.; O’Sullivan, M. Fiber Optic Measurement Techniques; Academic Press: Cambridge, MA, USA, 2009. [Google Scholar]
- Stathi, G.; Rizou, Z.; Zoiros, K. Simulation of directly modulated RSOA. In Proceedings of the 2017 International Conference on Numerical Simulation of Optoelectronic Devices (NUSOD), Copenhagen, Denmark, 24–28 July 2017; pp. 145–146. [Google Scholar]
- Vujičić, Z.; Dionísio, R.P.; Shahpari, A.; Pavlović, N.B.; Teixeira, A. Efficient dynamic modeling of the reflective semiconductor optical amplifier. IEEE J. Sel. Top. Quantum Electron. 2013, 19, 3000310. [Google Scholar] [CrossRef]





| Symbol | Definition | Value | Unit | Reference |
|---|---|---|---|---|
| CW input power | −5 | dBm | [21] | |
| Encoded signal wavelength | 1560 | nm | [21] | |
| Electrical data pulse repetition period | 250 & 1000 | ps | [21] | |
| Electrical data pulse rise time | 17% of repetition period | ps | [21] | |
| RSOA modulation current | 20 | mA | Fitting (steady- state) | |
| RSOA dc bias current | 70 | mA | Fitting (steady- state) | |
| RSOA transparency current | 45 | mA | Fitting (steady- state) | |
| RSOA linewidth enhancement factor | 5 | - | [28] | |
| RSOA confinement factor | 0.17 | - | [28] | |
| a | RSOA differential gain | m | [28] | |
| RSOA carrier density at transparency | m | [28] | ||
| RSOA semiconductor material group refractive index | 3.6 | - | [28] | |
| RSOA active region length | 713 | um | [28] | |
| RSOA carrier lifetime | 408 | ps | Fitting (dynamic) | |
| RSOA semiconductor material saturation power | 3.2 | dBm | [28] | |
| B | BFL birefringence | - | [21] | |
| BFL PM total length | 8.5 | m | [21] | |
| BFL detuning | −0.08 | nm | [21] |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Karadimoglou, F.N.; Zoiros, K.E.; Rizou, Z.V.; Hatziefremidis, A. On Directly Modulated Reflective Semiconductor Optical Amplifier with Assistance of Birefringent Fiber Loop. Photonics 2022, 9, 147. https://doi.org/10.3390/photonics9030147
Karadimoglou FN, Zoiros KE, Rizou ZV, Hatziefremidis A. On Directly Modulated Reflective Semiconductor Optical Amplifier with Assistance of Birefringent Fiber Loop. Photonics. 2022; 9(3):147. https://doi.org/10.3390/photonics9030147
Chicago/Turabian StyleKaradimoglou, Fokion N., Kyriakos E. Zoiros, Zoe V. Rizou, and Antonios Hatziefremidis. 2022. "On Directly Modulated Reflective Semiconductor Optical Amplifier with Assistance of Birefringent Fiber Loop" Photonics 9, no. 3: 147. https://doi.org/10.3390/photonics9030147
APA StyleKaradimoglou, F. N., Zoiros, K. E., Rizou, Z. V., & Hatziefremidis, A. (2022). On Directly Modulated Reflective Semiconductor Optical Amplifier with Assistance of Birefringent Fiber Loop. Photonics, 9(3), 147. https://doi.org/10.3390/photonics9030147






