Fano Resonance Hybrid Waveguide-Coupled Plasmonic Sensor Using Transparent Conductive Oxide in the Near-Infrared Range
Abstract
:1. Introduction
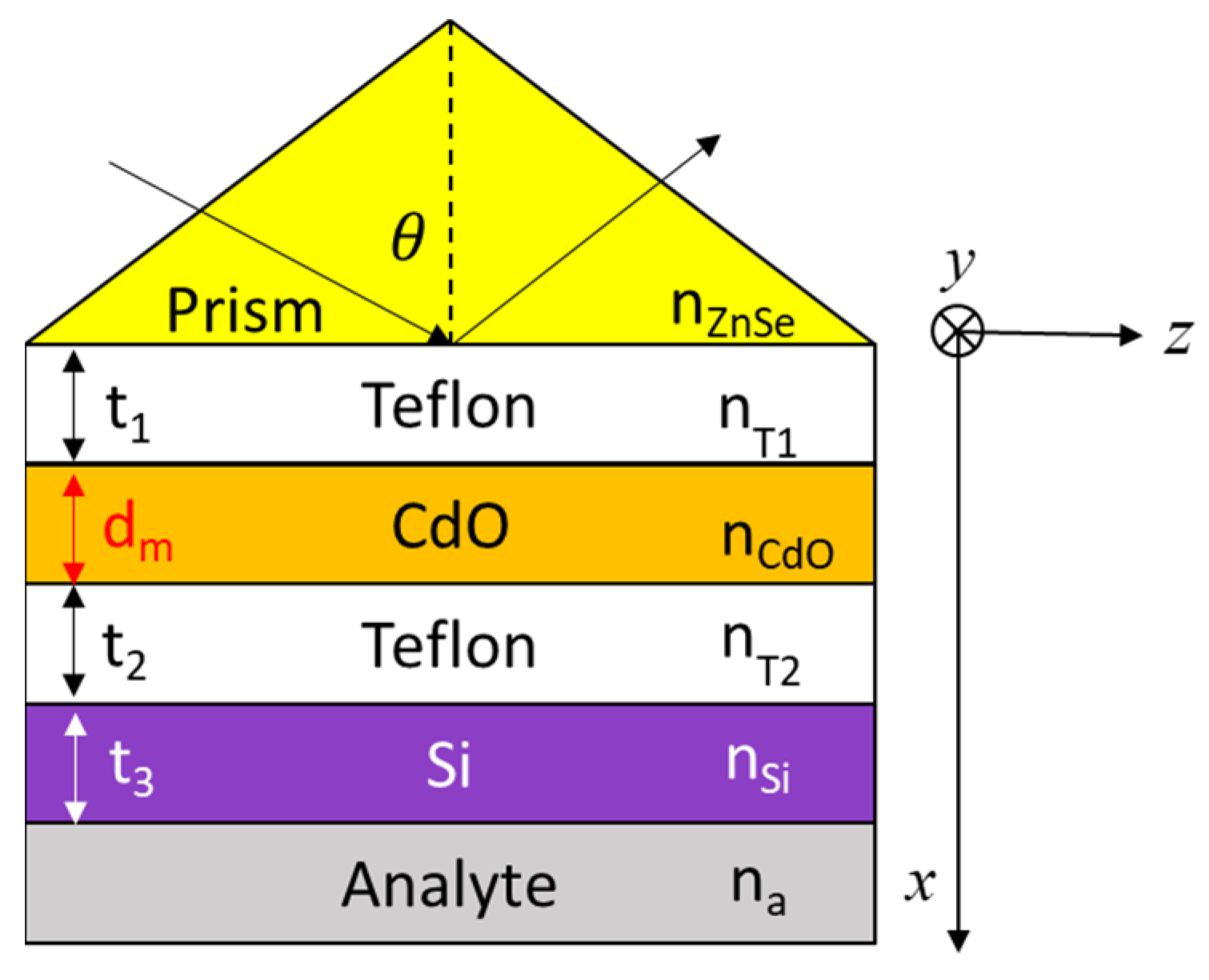
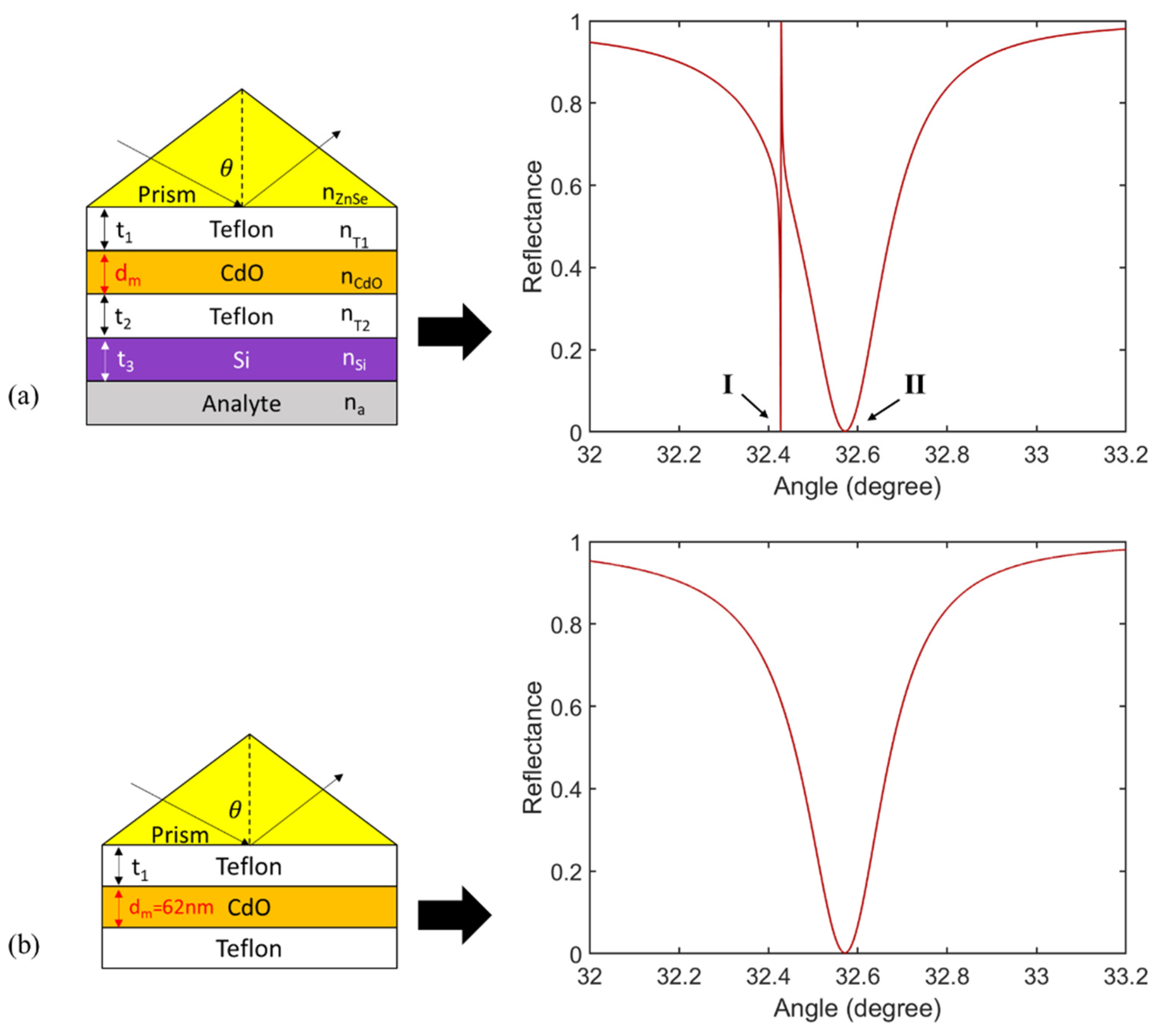
2. Theoretical Model and Analysis Methods
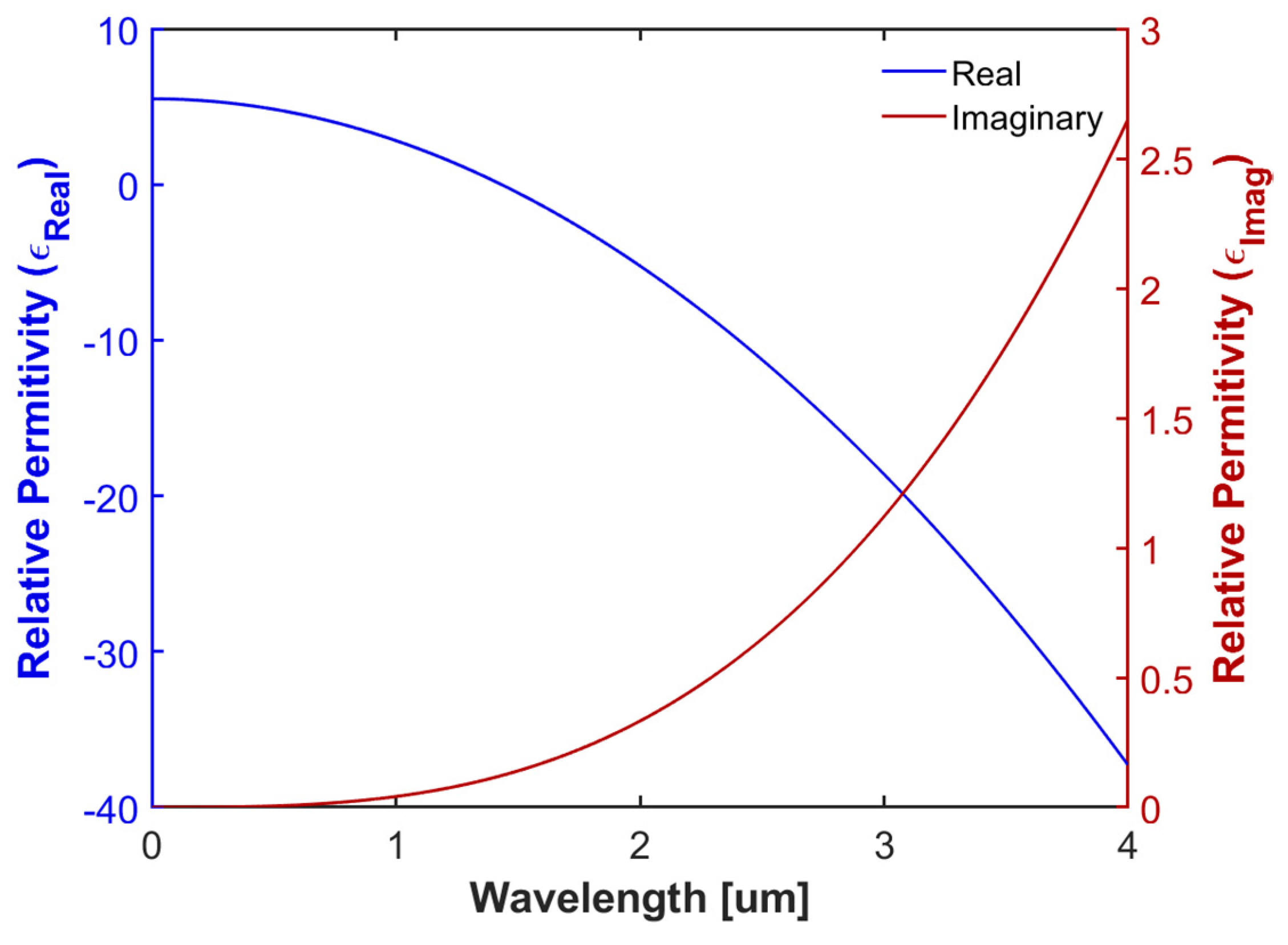
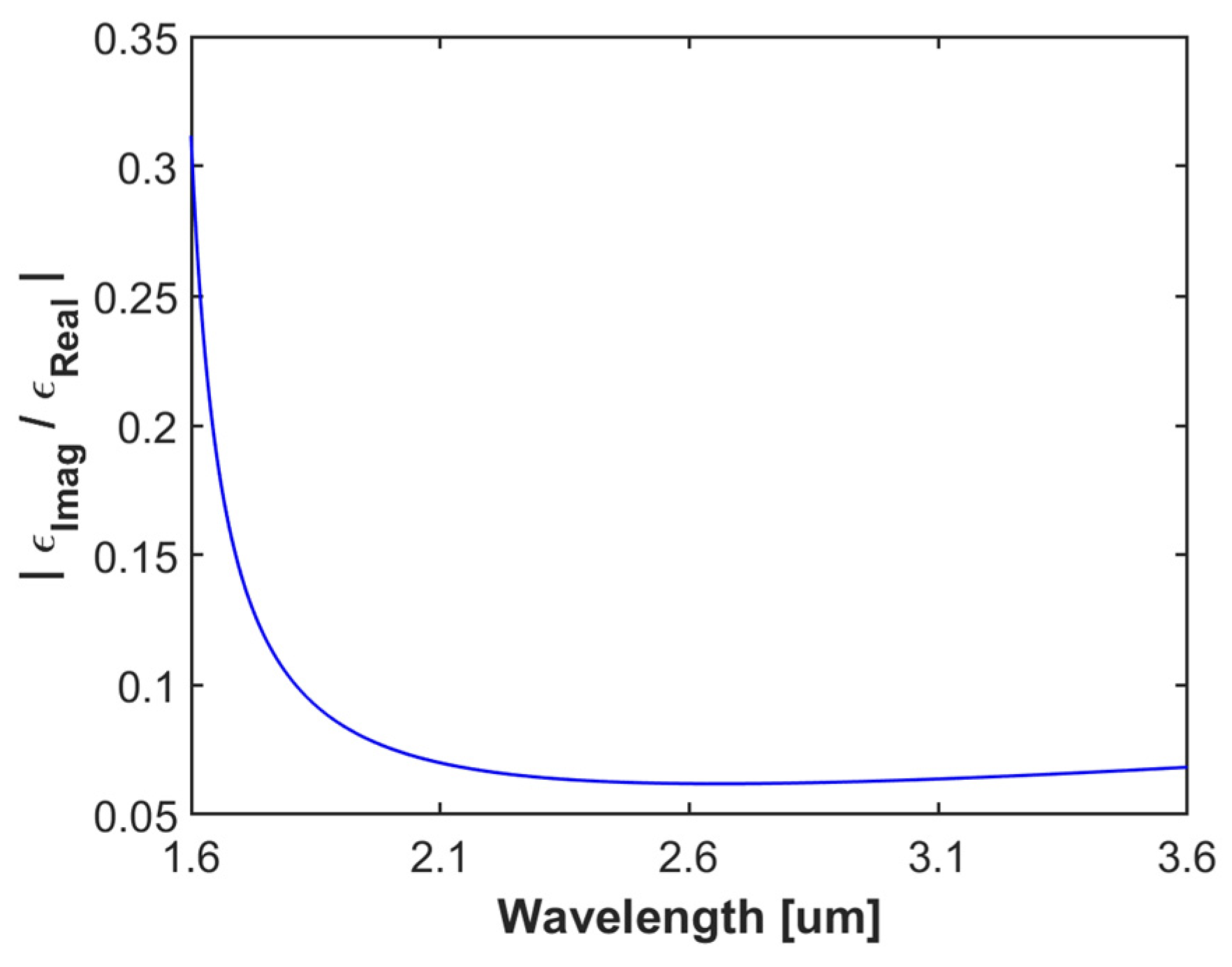
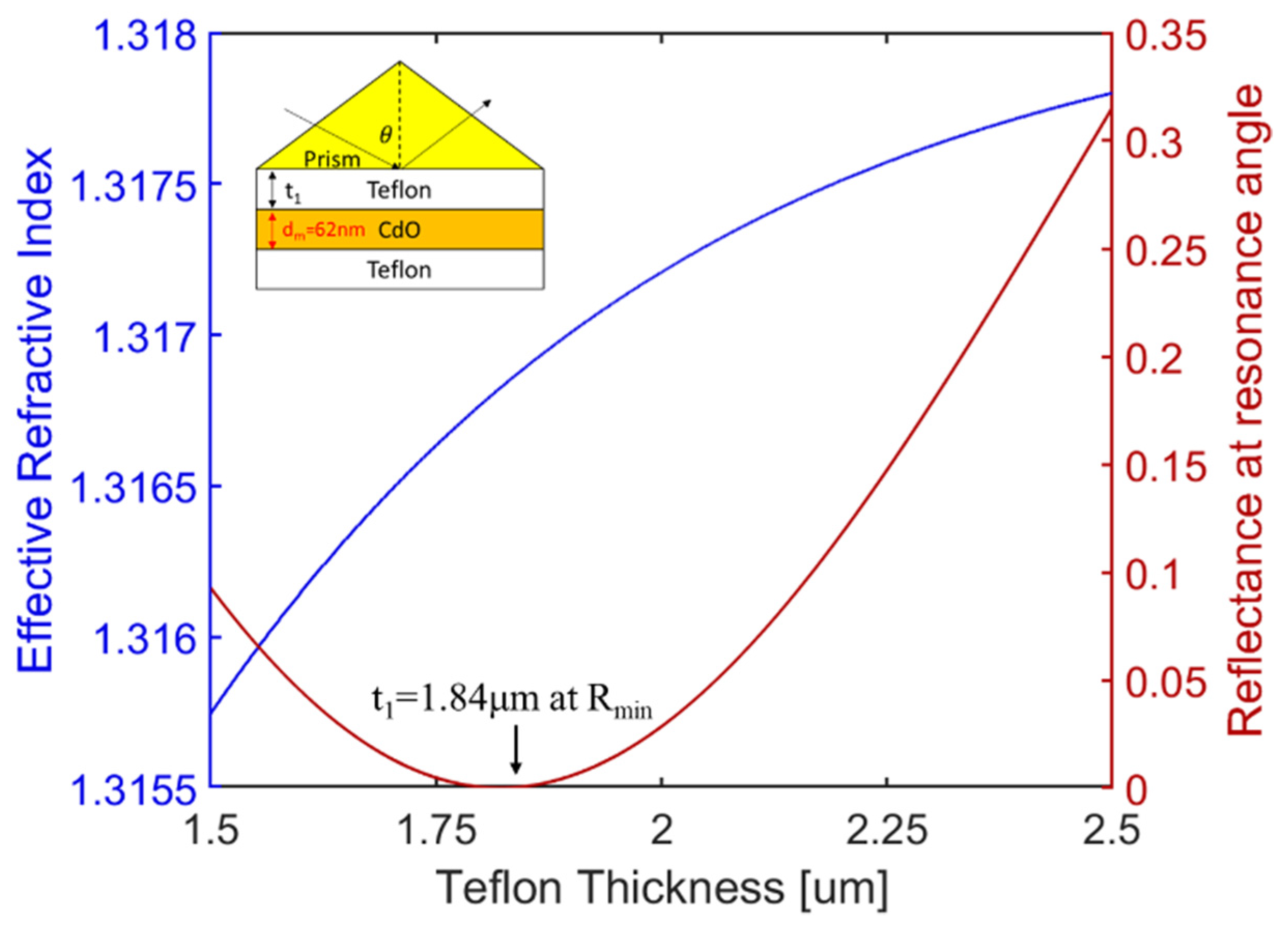
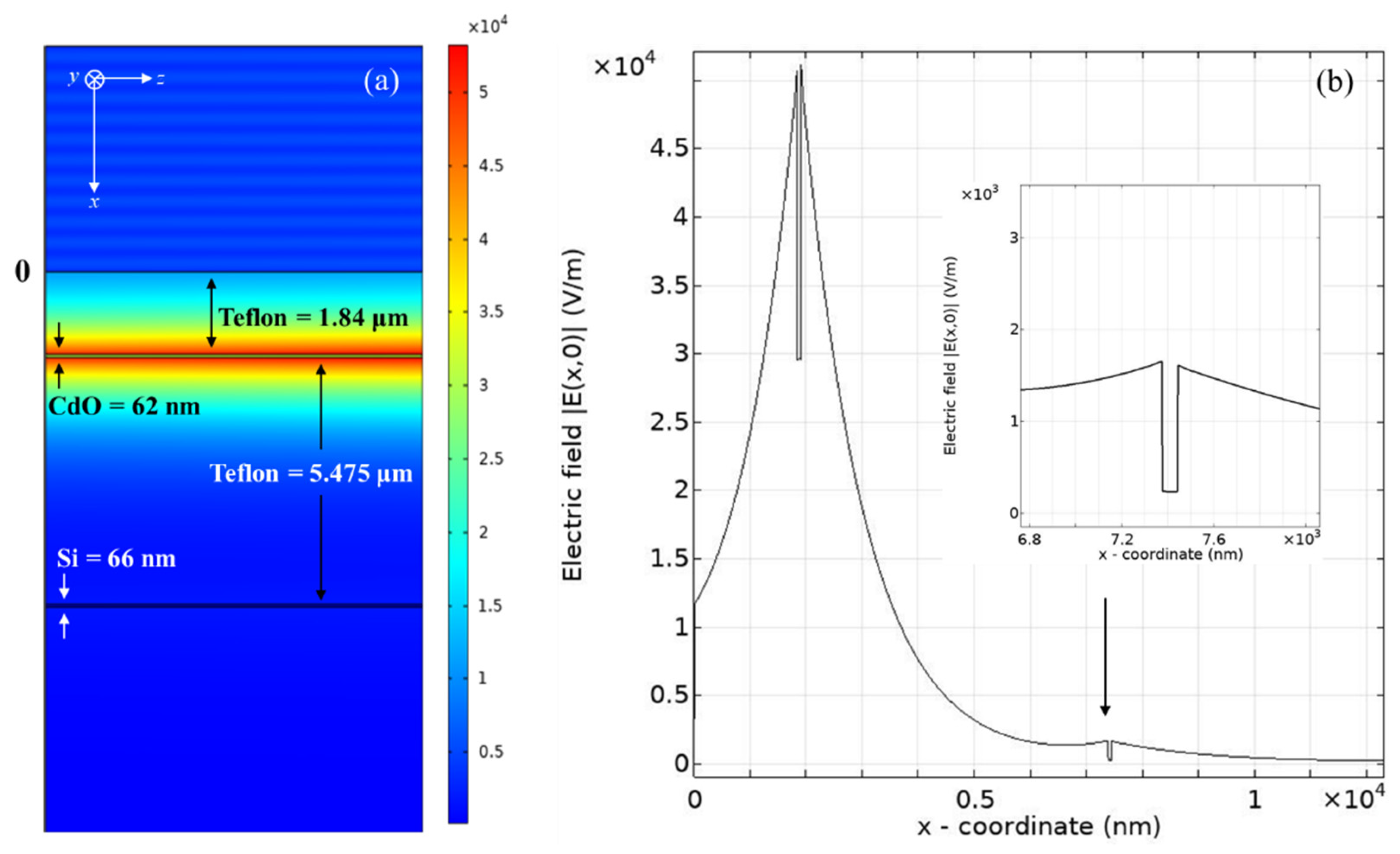
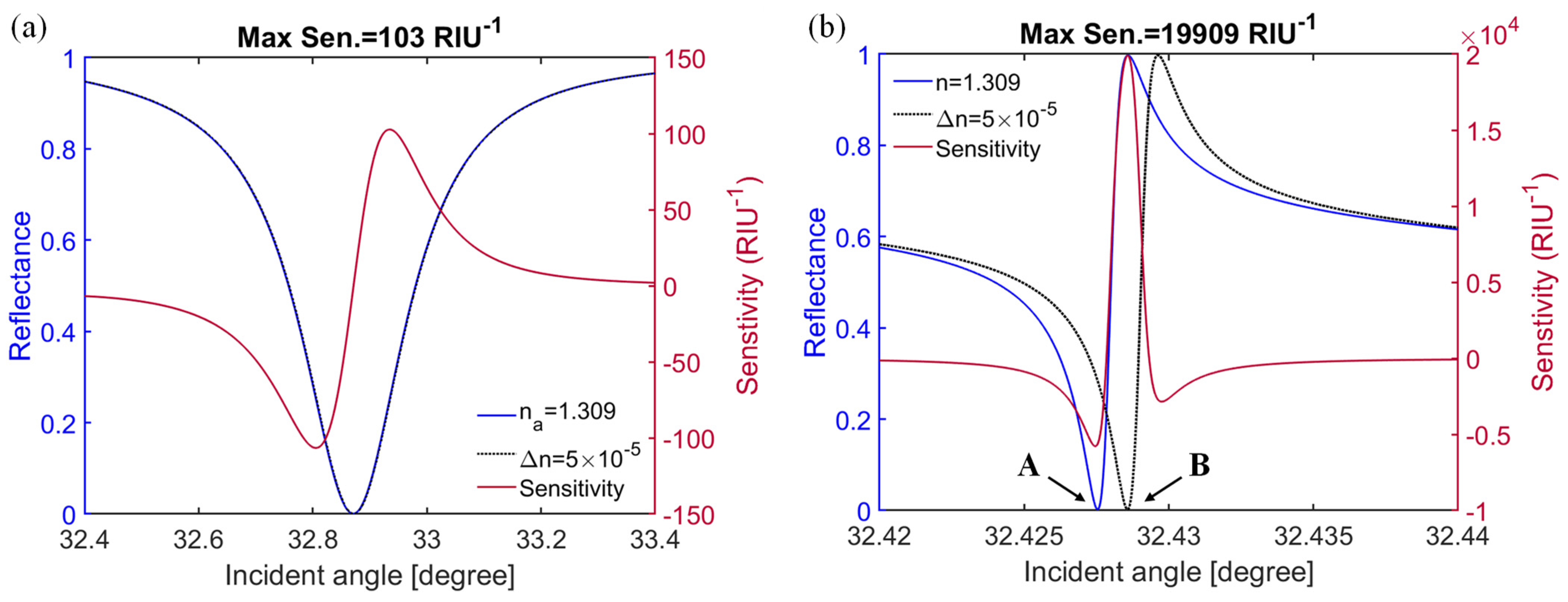
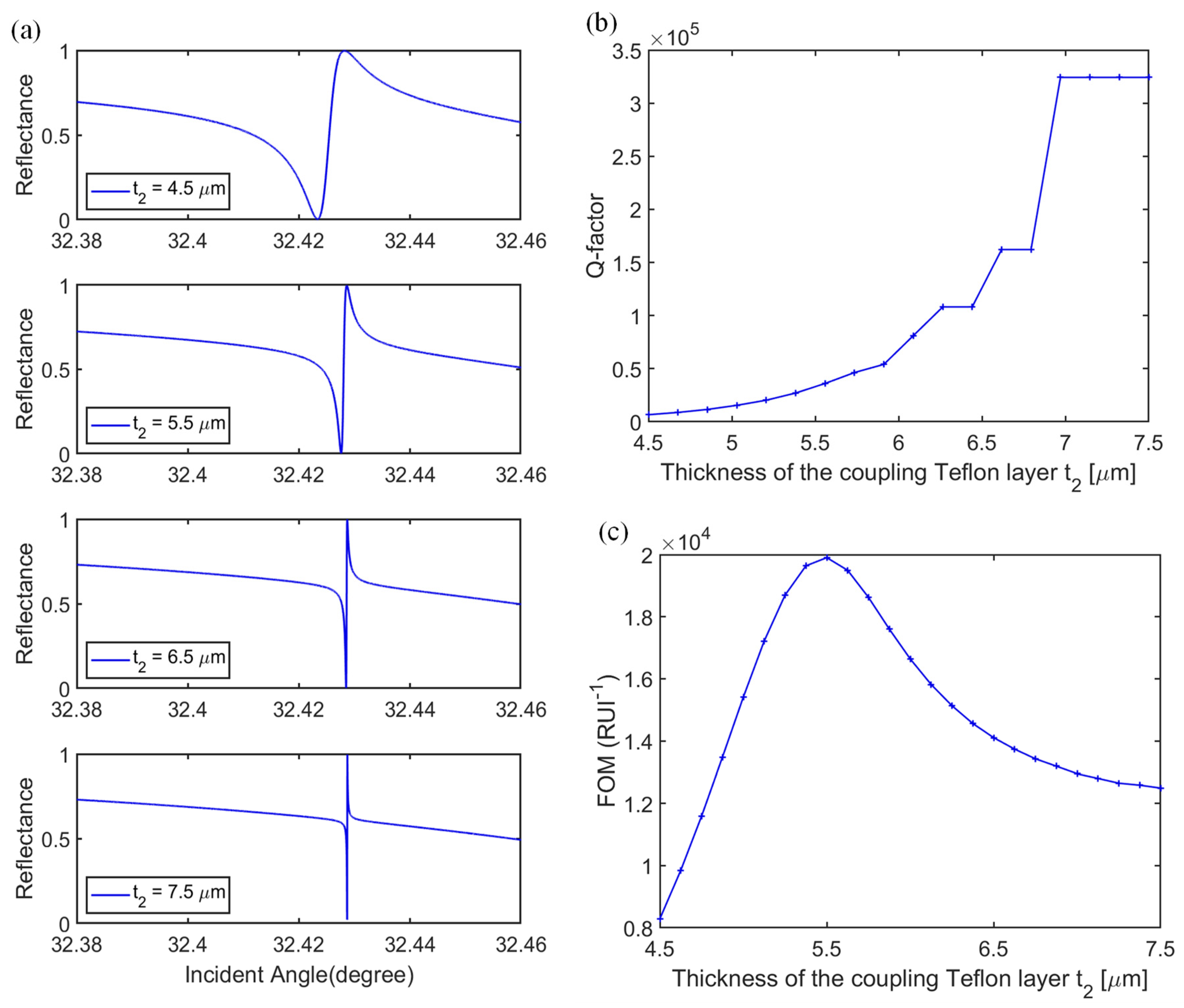
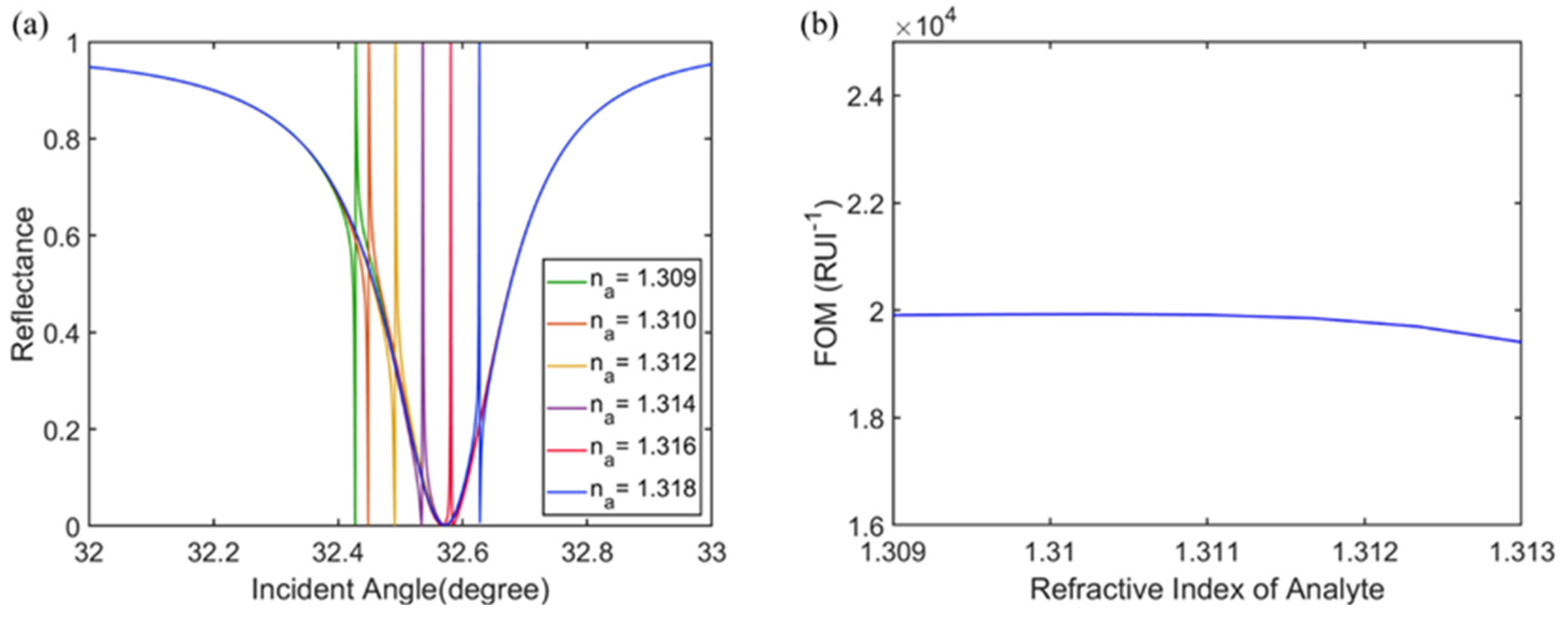
3. Numerical Results and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Otto, A. Excitation of nonradiative surface plasma waves in silver by the method of frustrated total reflection. Z. Phys. 1968, 216, 398–410. [Google Scholar] [CrossRef]
- Kretschmann, E.; Raether, H. Notizen: Radiative decay of non-radiative surface plasmons excited by light. Z. Naturforsch. A 1968, 23, 2135–2136. [Google Scholar] [CrossRef]
- Daghestani, H.N.; Day, B.W. Theory and Applications of Surface Plasmon Resonance, Resonant Mirror, Resonant Waveguide Grating, and Dual Polarization Interferometry Biosensors. Sensors 2010, 10, 9630–9646. [Google Scholar] [CrossRef] [PubMed]
- Lee, K.S.; Lee, T.S.; Kim, I.; Kim, W.M. Parametric study on the bimetallic waveguide coupled surface plasmon resonance sensors in comparison with other configurations. J. Phys. D Appl. Phys. 2013, 46, 125302. [Google Scholar] [CrossRef]
- Lahav, A.; Auslender, M.; Abdulhalim, I. Sensitivity enhancement of guided-wave surface-plasmon resonance sensors. Opt. Lett. 2008, 33, 2539–2541. [Google Scholar] [CrossRef] [PubMed]
- Shalabney, A.; Abdulhalim, I. Figure-of-merit enhancement of surface plasmon resonance sensors in the spectral interrogation. Opt. Lett. 2012, 37, 1175–1177. [Google Scholar] [CrossRef] [PubMed]
- Wu, L.; Ling, Z.; Jiang, L.; Guo, J.; Dai, X.; Xiang, Y.; Fan, D. Long-range surface plasmon with graphene for enhancing the sensitivity and detection accuracy of biosensor. IEEE Photonics J. 2016, 8, 4801409. [Google Scholar] [CrossRef]
- Xu, Y.; Hsieh, C.H.; Wu, L.; Ang, L.K. Two-dimensional transition metal dichalcogenides mediated long range surface plasmon resonance biosensors. J. Phys. D Appl. Phys. 2019, 52, 065101. [Google Scholar] [CrossRef] [Green Version]
- Sarid, D. Long-range surface-plasma waves on very thin metal films. Phys. Rev. Lett. 1981, 47, 1927. [Google Scholar] [CrossRef]
- Berini, P. Long-range surface plasmon polaritons. Adv. Opt. Photonics 2009, 1, 484–588. [Google Scholar] [CrossRef]
- Wu, L.; Guo, J.; Xu, H.; Dai, X.; Xiang, Y. Ultrasensitive biosensors based on long-range surface plasmon polariton and dielectric waveguide modes. Photonics Res. 2016, 4, 262–266. [Google Scholar] [CrossRef]
- Li, L.; Huang, T.; Zhao, X.; Wu, X.; Cheng, Z. Highly sensitive SPR sensor based on hybrid coupling between plasmon and potonic mode. IEEE Photonics Technol. Lett. 2018, 30, 1364–1367. [Google Scholar] [CrossRef]
- Hayashi, S.; Nesterenko, D.V.; Sekkat, Z. Waveguide-coupled surface plasmon resonance sensor structures: Fano lineshape engineering for ultrahigh-resolution sensing. J. Phys. D Appl. Phys. 2015, 48, 325303. [Google Scholar] [CrossRef]
- Hayashi, S.; Nesterenko, D.V.; Sekkat, Z. Fano resonance and plasmon-induced transparency in waveguide-coupled surface plasmon resonance sensors. Appl. Phys. Express 2015, 8, 022201. [Google Scholar] [CrossRef] [Green Version]
- Hayashi, S.; Nesterenko, D.V.; Rahmouni, A.; Sekkat, Z. Observation of Fano line shapes arising from coupling between surface plasmon polariton and waveguide modes. Appl. Phys. Lett. 2016, 108, 051101. [Google Scholar] [CrossRef] [Green Version]
- Nesterenko, D.V.; Hayashi, S.; Sekkat, Z. Extremely narrow resonances, giant sensitivity and field enhancement in low-loss waveguide sensors. J. Opt. 2016, 18, 065004. [Google Scholar] [CrossRef]
- Sekkat, Z.; Hayashi, S.; Nesterenko, D.V.; Rahmouni, A.; Refki, S.; Ishitobi, H.; Inouye, Y.; Kawata, S. Plasmonic coupled modes in metal-dielectric multilayer structures: Fano resonance and giant field enhancement. Opt. Express 2016, 24, 20080–20086. [Google Scholar] [CrossRef] [PubMed]
- Zheng, G.; Cong, J.; Xu, L.; Wang, J. High-resolution surface plasmon resonance sensor with Fano resonance in waveguide-coupled multilayer structures. Appl. Phys. Express 2017, 10, 042202. [Google Scholar] [CrossRef]
- Ruan, B.; You, Q.; Zhu, J.; Wu, L.; Guo, J.; Dai, X.; Xiang, Y. Fano resonance in double waveguides with graphene for ultrasensitive biosensor. Opt. Express 2018, 26, 16884. [Google Scholar] [CrossRef] [PubMed]
- Jiang, H.; Zheng, G.; Rao, W. Ultra-sensitive biosensor with hybrid coupling between molybdenum disulfide thin film and photonic waveguide mode. Results Phys. 2019, 13, 102173. [Google Scholar] [CrossRef]
- Chao, C.T.C.; Chau, Y.F.C.; Chiang, H.P. Multiple Fano resonance modes in an ultra-compact plasmonic waveguide-cavity system for sensing applications. Results Phys. 2021, 27, 104527. [Google Scholar] [CrossRef]
- Chau, Y.F.C.; Chao, C.T.C.; Jumat, S.Z.B.H.; Kooh, M.R.R.; Thotagamuge, R.; Lim, C.M.; Chiang, H.P. Improved Refractive Index-Sensing Performance of Multimode Fano-Resonance-Based Metal-Insulator-Metal Nanostructures. Nanomaterials 2021, 11, 2097. [Google Scholar] [CrossRef] [PubMed]
- Rybin, M.V.; Khanikaev, A.B.; Inoue, M.; Samusev, K.B.; Steel, M.J.; Yushin, G.; Limonov, M.F. Fano resonance between Mie and Bragg scattering in photonic crystals. Phys. Rev. Lett. 2009, 103, 023901. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Pradarutti, B.; Torosyan, G.; Theuer, M.; Beigang, R. Fano profiles in transmission spectra of terahertz radiation through one dimensional periodic metallic structures. Appl. Phys. Lett. 2010, 97, 244103. [Google Scholar] [CrossRef]
- Khan, A.D.; Miano, G. Higher order tunable Fano resonances in multilayer nanocones. Plasmonics 2013, 8, 1023–1034. [Google Scholar] [CrossRef]
- Hoang, T.T.; Ngo, Q.M.; Vu, D.L.; Nguyen, H.P.T. Controlling Fano resonances in multilayer dielectric gratings towards optical bistable devices. Sci. Rep. 2018, 8, 16404. [Google Scholar] [CrossRef] [Green Version]
- Zhao, G.; Zhao, T.; Xiao, H.; Liu, Z.; Liu, G.; Yang, J.; Ren, Z.; Bai, J.; Tian, Y. Tunable Fano resonances based on microring resonator with feedback coupled waveguide. Opt. Express 2016, 24, 20187–20195. [Google Scholar] [CrossRef]
- Limonov, M.F.; Rybin, M.V.; Poddubny, A.N.; Kivshar, Y.S. Fano resonances in photonics. Nat. Photonics 2017, 11, 543–554. [Google Scholar] [CrossRef]
- Lu, D.; Li, W.; Zhou, H.; Cao, X.; Wang, K.; Luo, H.; Li, J.; Zhang, X.; He, M.; Xu, L.; et al. Coupling between surface plasmon polariton and planar waveguide modes in the biosensor based on metal–insulator–metal/planar waveguide structure. Opt. Commun. 2020, 459, 124928. [Google Scholar] [CrossRef]
- Gu, L.; Fang, L.; Fang, H.; Li, J.; Zheng, J.; Zhao, J.; Zhao, Q.; Gan, X. Fano resonance lineshapes in a waveguide-microring structure enabled by an air-hole. APL Photonics 2020, 5, 016108. [Google Scholar] [CrossRef] [Green Version]
- Chen, J.; Gan, F.; Wang, Y.; Li, G. Plasmonic sensing and modulation based on Fano resonances. Adv. Optical Mater. 2018, 6, 1701152. [Google Scholar] [CrossRef]
- Stanley, R. Plasmonics in the mid-infrared. Nat. Photonics 2012, 6, 409–411. [Google Scholar] [CrossRef]
- Foteinopoulou, S.; Devarapu, G.C.R.; Subramania, G.S.; Krishna, S.; Wasserman, D. Phonon-polaritonics: Enabling powerful capabilities for infrared photonics. Nanophotonics 2019, 8, 2129–2175. [Google Scholar] [CrossRef] [Green Version]
- Naik, G.V.; Kim, J.; Boltasseva, A. Oxides and nitrides as alternative plasmonic materials in the optical range. Opt. Mater. Express 2011, 1, 1090–1099. [Google Scholar] [CrossRef] [Green Version]
- Khamh, H.; Sachet, E.; Kelly, K.; Maria, J.P.; Franzen, S. As good as gold and better: Conducting metal oxide materials for mid-infrared plasmonic applications. J. Mater. Chem. C. 2018, 6, 8326–8342. [Google Scholar] [CrossRef]
- Lee, H.W.; Papadakis, G.; Burgos, S.P.; Chander, K.; Kriesch, A.; Pala, R.; Peschel, U.; Atwater, H.A. Nanoscale conducting oxide PlasMOStor. Nano Lett. 2014, 14, 6463–6468. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Ruan, B.; You, Q.; Zhu, J.; Wu, L.; Guo, J.; Dai, X.; Xiang, Y. Improving the performance of an SPR biosensor using long-range surface plasmon of Ga-doped zinc oxide. Sensors 2018, 18, 2098. [Google Scholar] [CrossRef] [Green Version]
- Pal, S.; Verma, A.; Prajapati, Y.K.; Saini, J.P. Figure of merit enhancement of surface plasmon resonance biosensor using Ga-doped zinc oxide in near infrared range. Photonic Sens. 2020, 10, 340–352. [Google Scholar] [CrossRef] [Green Version]
- Pal, S.; Pal, N.; Verma, A.; Saini, J.P.; Prajapati, Y.K. Analyzing the role of Transparent conducting oxide in place of metals in SPR sensor for biomolecular detection in near infrared range. Results in Opt. 2021, 3, 100078. [Google Scholar] [CrossRef]
- Huang, T.; Zeng, S.; Zhao, X.; Cheng, Z.; Shum, P.P. Fano resonance enhanced surface plasmon resonance sensors operating in near-infrared. Photonics 2018, 5, 23. [Google Scholar] [CrossRef] [Green Version]
- Sachet, E.; Shelton, C.T.; Harris, J.S.; Gaddy, B.E.; Irving, D.L.; Curtarolo, S.; Donovan, B.F.; Hopkins, P.E.; Sharma, P.A.; Sharma, A.L.; et al. Dysprosium doped cadmium oxide: A gateway material for mid-infrared plasmonics. Nat. Mater. 2015, 14, 414–420. [Google Scholar] [CrossRef] [PubMed]
- Runnerstrom, E.L.; Kelley, K.P.; Sachet, E.; Shelton, C.T.; Maria, J.P. Epsilon-near-zero modes and surface plasmon resonance in Fluorine-doped Cadmium oxide thin films. ACS Photonics. 2017, 4, 1885–1892. [Google Scholar] [CrossRef]
- Yang, Y.; Kelley, K.; Sachet, E.; Campione, S.; Luk, T.S.; Maria, J.P.; Sinclair, M.B.; Brener, I. Femtosecond optical polarization switching using a cadmium oxide-based perfect absorber. Nat. Photonics 2017, 11, 390–395. [Google Scholar] [CrossRef]
- Salzberg, C.D.; Villa, J.J. Infrared Refractive Indexes of Silicon Germanium and Modified Selenium Glass. J. Opt. Soc. Amer. 1957, 47, 244. [Google Scholar] [CrossRef]
- Tatian, B. Fitting Refractive-index data with the Sellmeier dispersion formula. Appl. Opt. 1984, 23, 4477. [Google Scholar] [CrossRef] [PubMed]
- Yang, M.K.; French, R.H.; Tokarsky, E.W. Optical properties of Teflon (R) AF amorphous fluoropolymers. J. Micro/Nanolith. MEMS MOEMS. 2008, 7, 033010. [Google Scholar] [CrossRef]
- Equilateral Dispersive Prisms. ZnSe Equilateral Dispersive Prisms (600 nm–16 nm); Thorlabs, Inc.: Shanghai, China, 2012; Available online: www.thorlabs.com/newgrouppage9.cfm?objectgroup_id=148 (accessed on 14 March 2022).
- Huang, T.; Xu, G.; Pan, J.; Cheng, Z.; Shum, P.P.; Brambilla, G. Theoretical study of bicharacteristic waveguide for fundamental-mode phase-matched SHG from MIR to NIR. Opt. Express 2019, 27, 15236–15250. [Google Scholar] [CrossRef] [PubMed]
- Thulium-Doped Fiber Lasers: 1800 nm to 2050 nm; Thorlabs, Inc.: Shanghai, China, 2012; Available online: https://www.thorlabs.com/newgrouppage9.cfm?objectgroup_id=11137 (accessed on 14 March 2022).
- Yamamoto, M. Surface plasmon resonance (SPR) theory: Tutorial. Rev. Polarogr. (JPN) 2002, 48, 209–237. [Google Scholar] [CrossRef]
- Hansen, W.H. Electric fields produced by the propagation of plane coherent electromagnetic radiation in a stratified medium. J. Opt. Soc. Am. 1968, 58, 380–388. [Google Scholar] [CrossRef]
- Srivastava, T.; Jha, R. Black Phosphorus: A New Platform for Gaseous Sensing Based on Surface Plasmon Resonance. IEEE Photon. Technol. Lett. 2018, 30, 319–322. [Google Scholar] [CrossRef]
- Zhang, X.; Wang, Y.; Zhao, X.; Huang, T.; Zeng, S.; Ping, P.S. Fano resonance based on long range surface phonon resonance in the mid-infrared region. IEEE Photonics J. 2019, 11, 4800808. [Google Scholar] [CrossRef]
- Hale, G.M.; Querry, M.R. Optical constants of water in the 200-nm to 200-µm wavelength region. Appl. Opt. 1973, 12, 555–556. [Google Scholar] [CrossRef] [PubMed]



| Sellmeier Coefficients | Zinc Selenide | Teflon | Silicon |
|---|---|---|---|
| A | 1.00 | 1.517 | 1.00 |
| B1 | 4.298 | 0.184 | 10.6684 |
| C1 | 3.689 × 10−2 | 0.016 | 0.30152 |
| B2 | 6.278 × 10−1 | 1.00 | 3.0434 × 10−2 |
| C2 | 1.435 × 10−1 | 104.66 | 1.13482 |
| B3 | 2.896 | - | 1.5413 |
| C3 | 2.208 × 103 | - | 11042 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Khattak, A.; Wei, L. Fano Resonance Hybrid Waveguide-Coupled Plasmonic Sensor Using Transparent Conductive Oxide in the Near-Infrared Range. Photonics 2022, 9, 189. https://doi.org/10.3390/photonics9030189
Khattak A, Wei L. Fano Resonance Hybrid Waveguide-Coupled Plasmonic Sensor Using Transparent Conductive Oxide in the Near-Infrared Range. Photonics. 2022; 9(3):189. https://doi.org/10.3390/photonics9030189
Chicago/Turabian StyleKhattak, Anum, and Li Wei. 2022. "Fano Resonance Hybrid Waveguide-Coupled Plasmonic Sensor Using Transparent Conductive Oxide in the Near-Infrared Range" Photonics 9, no. 3: 189. https://doi.org/10.3390/photonics9030189
APA StyleKhattak, A., & Wei, L. (2022). Fano Resonance Hybrid Waveguide-Coupled Plasmonic Sensor Using Transparent Conductive Oxide in the Near-Infrared Range. Photonics, 9(3), 189. https://doi.org/10.3390/photonics9030189




