Modulation of Surface Plasmonic Bending Beam via Nanoslit Interactions
Abstract
1. Introduction
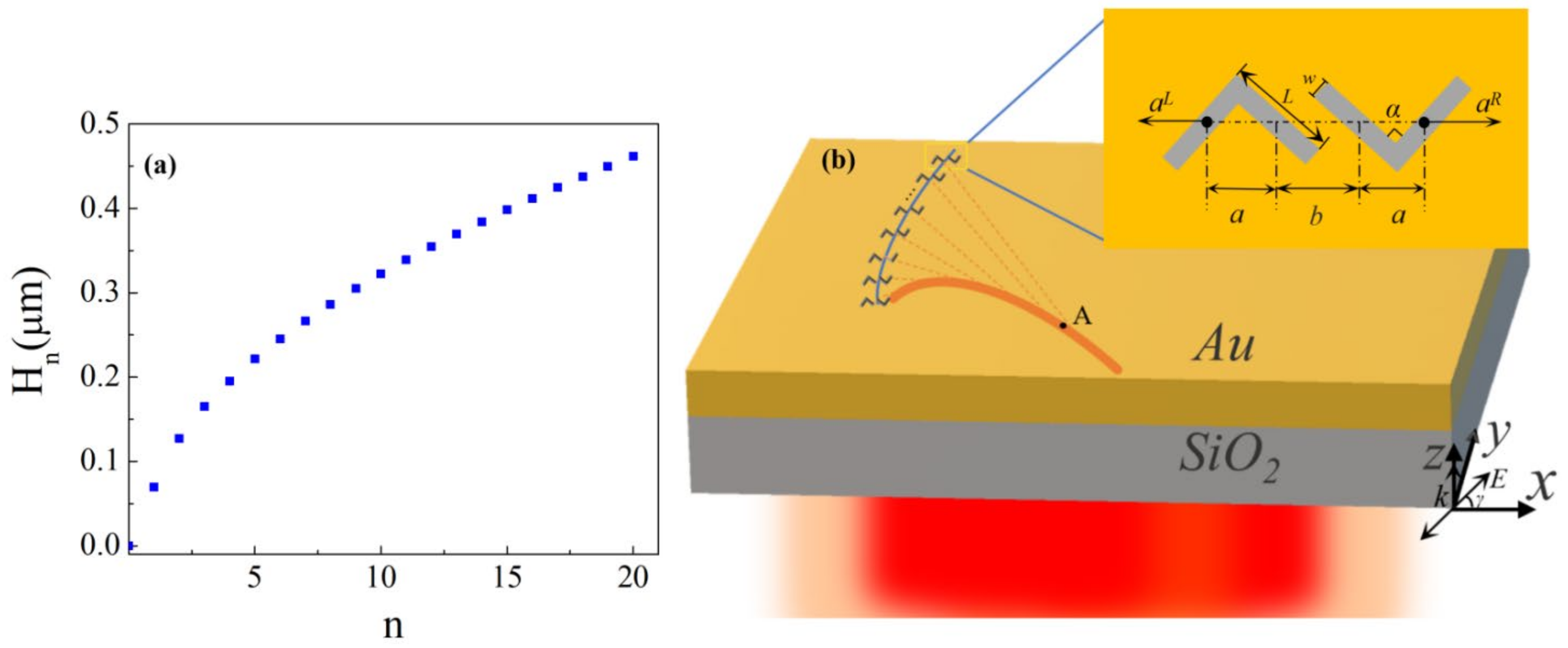
2. Theoretical Analysis and Structure
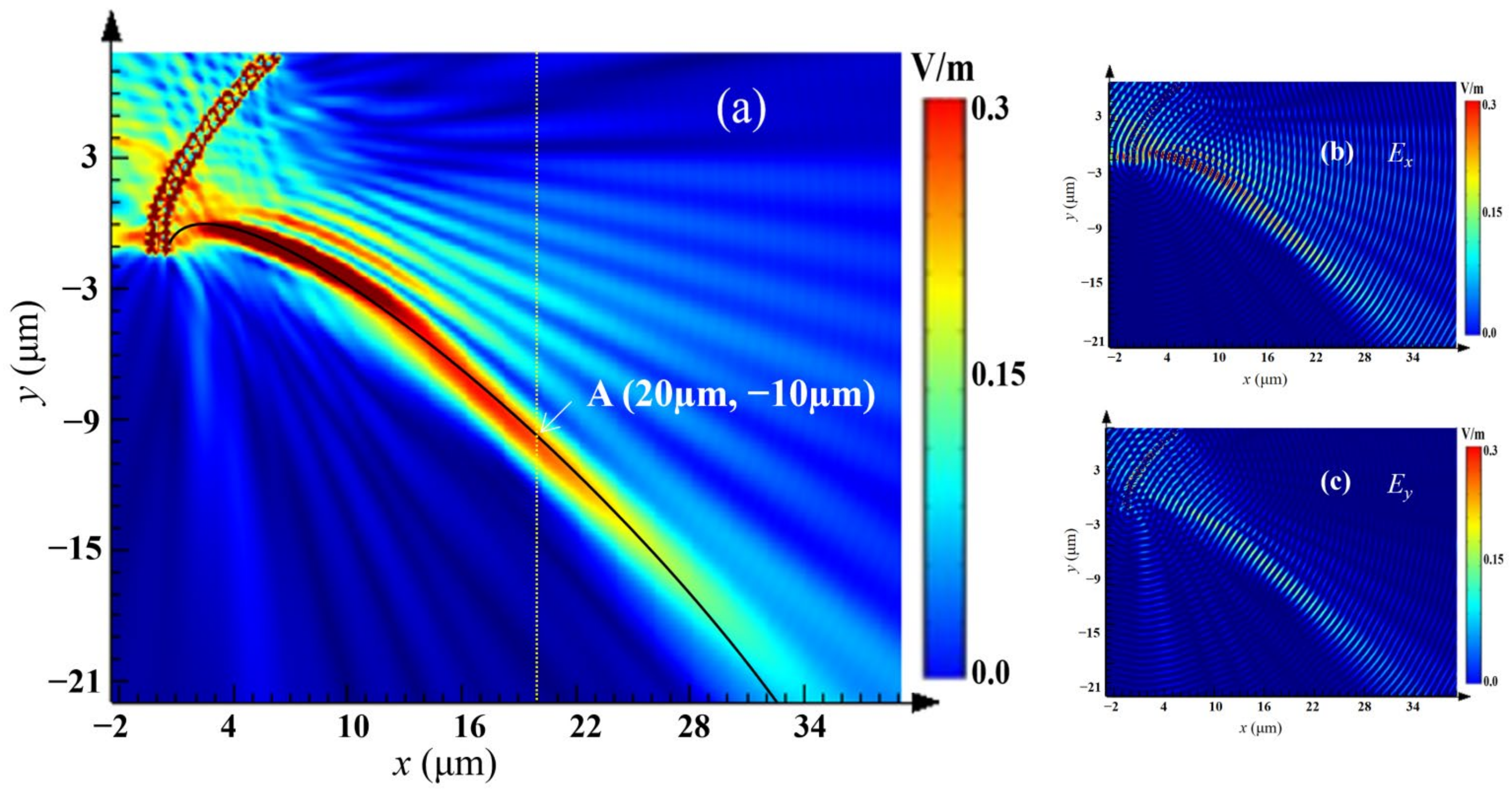
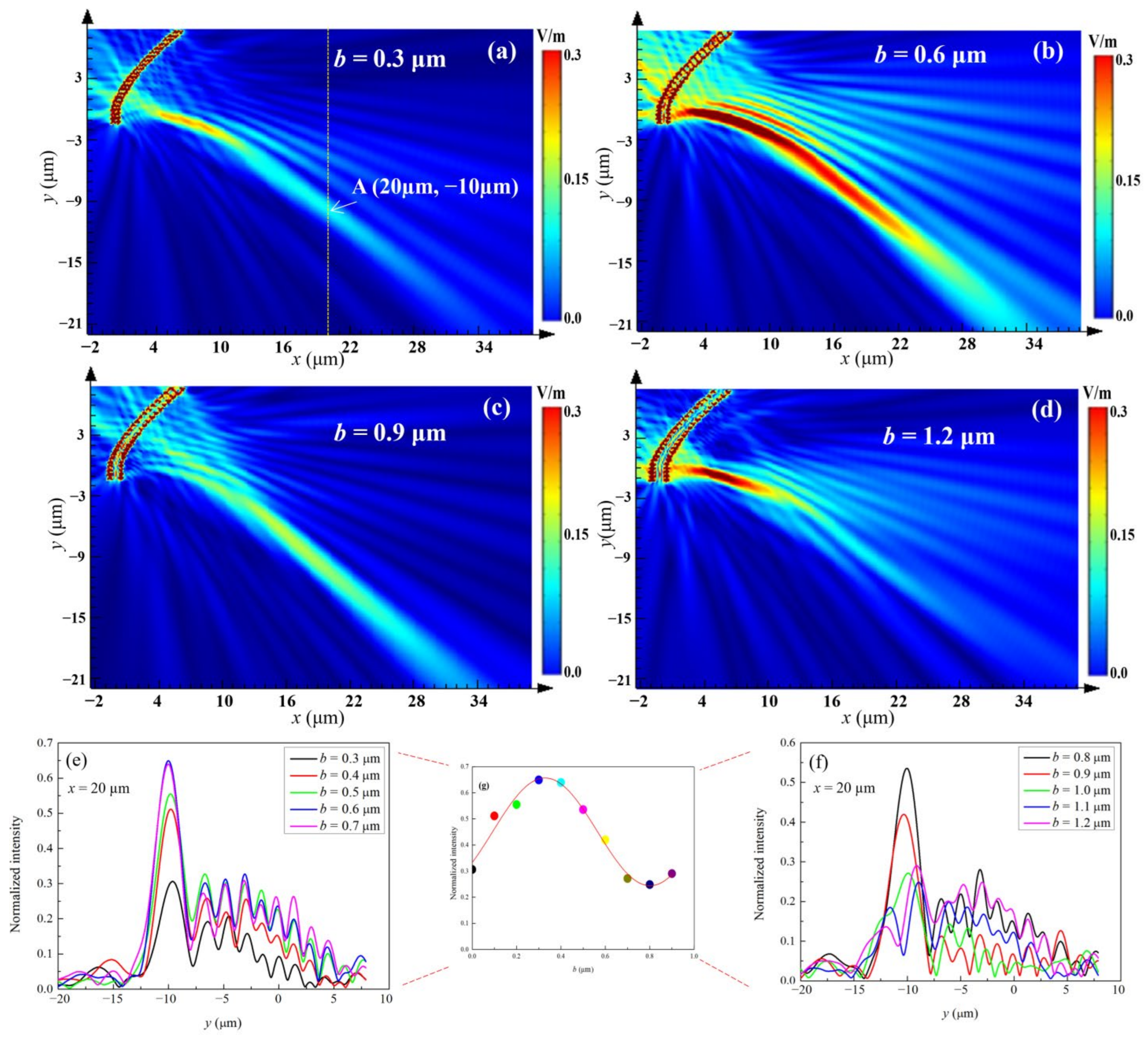
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Brongersma, M.L.; Shalaev, M.V. The case for plasmonics. Science 2010, 328, 440–441. [Google Scholar] [CrossRef] [PubMed]
- Walters, R.J.; Loon, R.V.A.V.; Brunets, I.; Schmitz, J.; Polman, A. A silicon-based electrical source of surface plasmon polaritons. Nat. Mater. 2010, 9, 21. [Google Scholar] [CrossRef] [PubMed]
- Liu, Y.; Stief, F.; Yu, M. Subwavelength optical trapping with a fiber-based surface plasmonic lens. Opt. Lett. 2013, 38, 721. [Google Scholar] [CrossRef] [PubMed]
- Sun, S.; He, Q.; Hao, J.; Xiao, S.; Zhou, L. Electromagnetic metasurfaces: Physics and applications. Adv. Opt. Photonics 2019, 11, 380. [Google Scholar] [CrossRef]
- Chen, L.; Ren, T.; Zhao, Y.; Yu, Q.; Huang, Z.; Zhang, K.; Chen, S. Polarization-Independent Wavefront Manipulation of Surface Plasmons with Plasmonic Metasurfaces. Adv. Opt. Mater. 2020, 8, 2000868. [Google Scholar] [CrossRef]
- Juan, M.L.; Righini, M.; Quidant, R. Plasmon nano-optical tweezers. Nat. Photonics 2011, 5, 349. [Google Scholar] [CrossRef]
- Min, C.; Shen, Z.; Shen, J.; Zhang, Y.; Fang, H.; Yuan, G.H.; Yuan, X.C. Focused plasmonic trapping of metallic particles. Nat. Commun. 2013, 4, 2891. [Google Scholar] [CrossRef]
- Ke, X.Z.; Qin, H.H.; Yang, S.J.; Wu, J.; Pan, X. Night background light noise model of visible light communication system in vehicle networking environment. Chin. J. Radio Sci. 2021, 36, 986–990. (In Chinese) [Google Scholar] [CrossRef]
- Ke, X.Z.; Wu, J.L.; Yang, S.J. Research progress and prospect of atmospheric turbulence for wireless optical communication. Chin. J. Radio Sci. 2021, 36, 323–339. (In Chinese) [Google Scholar] [CrossRef]
- Kaminer, I.; Bekenstein, R.; Nemirovsky, J.; Segev, M. Nondiffracting accelerating wave packets of Maxwell’s equations. Phys. Rev. Lett. 2012, 108, 163901. [Google Scholar] [CrossRef]
- Aleahmad, P.; Miri, M.A.; Mills, M.S.; Kaminer, I.; Segev, M.; Christodoulides, D.N. Fully Vectorial Accelerating Diffraction-Free Helmholtz Beams. Phys. Rev. Lett. 2012, 109, 203902. [Google Scholar] [CrossRef] [PubMed]
- Dolev, I.; Epstein, I.; Arie, A. Surface-Plasmon Holographic Beam Shaping. Phys. Rev. Lett. 2012, 109, 203903. [Google Scholar] [CrossRef] [PubMed]
- Epstein, I.; Arie, A. Arbitrary Bending Plasmonic Light Waves. Phys. Rev. Lett. 2014, 112, 023903. [Google Scholar] [CrossRef]
- Liu, W.; Cheng, H.; Tian, J.; Chen, S. Diffractive metalens: From fundamentals, practical applications to current trends. Adv. Phys. X 2020, 5, 1742584. [Google Scholar] [CrossRef]
- Baumgartl, J.; Mazilu, M.; Dholakia, K. Optically mediated particle clearing using Airy wavepackets. Nat. Photonics 2008, 2, 675. [Google Scholar] [CrossRef]
- Zhu, X.; Schülzgen, A.; Wei, H.; Kieu, K.; Peyghambarian, N. White light Bessel-like beams generated by miniature all-fiber device. Opt. Express 2011, 19, 11365–11374. [Google Scholar] [CrossRef]
- Libster-Hershko, A.; Epstein, I.; Arie, A. Rapidly Accelerating Mathieu and Weber Surface Plasmon Beams. Phys. Rev. Lett. 2014, 113, 123902. [Google Scholar] [CrossRef]
- Zhang, P.; Hu, Y.; Li, T.; Cannan, D.; Yin, X.; Morandotti, R.; Zhang, X. Nonparaxial Mathieu and Weber accelerating beams. Phys. Rev. Lett. 2012, 109, 193901. [Google Scholar] [CrossRef]
- Jiao, L.; Dellinger, J.; Genevet, P.; Cluzel, B.; Fornel, F.D.; Capasso, F. Cosine-Gauss Plasmon Beam: A Localized Long-Range Nondiffracting Surface Wave. Phys. Rev. Lett. 2012, 109, 093904. [Google Scholar] [CrossRef]
- Huidobro, P.A.; Kraft, M.; Maier, S.A.; Pendry, J.B. Graphene as a Tunable Anisotropic or Isotropic Plasmonic Metasurface. ACS Nano 2016, 10, 5499–5506. [Google Scholar] [CrossRef]
- Epstein, I.; Lilach, Y.; Arie, A. Shaping plasmonic light beams with near-field plasmonic holograms. J. Opt. Soc. Am. B 2014, 31, 1642–1647. [Google Scholar] [CrossRef]
- Li, L.; Li, T.; Wang, S.M.; Zhu, S.N. Plasmonic Airy Beam Generated by In-Plane Diffraction. Phys. Rev. Lett. 2011, 107, 126804. [Google Scholar] [CrossRef] [PubMed]
- Chen, Y.H.; Zhang, M.; Gan, L.; Wu, X.; Sun, L.; Liu, J.; Li, Z.Y. Holographic plasmonic lenses for surface plasmons with complex wavefront profile. Opt. Express 2013, 21, 17558–17566. [Google Scholar] [CrossRef] [PubMed]
- Chen, J.; Chen, X.; Li, T.; Zhu, S. On-Chip Detection of Orbital Angular Momentum Beam by Plasmonic Nanogratings. Laser Photo. Rev. 2018, 12, 1700331. [Google Scholar] [CrossRef]
- Li, Y.; Lin, J.; Guo, H.; Sun, W.; Xiao, S.; Zhou, L. A Tunable Metasurface with Switchable Functionalities: From Perfect Transparency to Perfect Absorption. Adv. Opt. Mater. 2020, 8, 1901548. [Google Scholar] [CrossRef]
- Abouelatta, M.A.; Obayya, S.S.; Hameed, M.F.O. Highly efficient transmissive metasurface for polarization control. Opt. Quantum Electron. 2021, 53, 87. [Google Scholar] [CrossRef]
- Feng, F.; Si, G.; Min, C.; Yuan, X.; Somekh, M. On-chip plasmonic spin-Hall nanograting for simultaneously detecting phase and polarization singularities. Light Sci. Appl. 2020, 9, 95. [Google Scholar] [CrossRef]
- Cheng, Q.; Wang, J.; Ma, L.; Shen, Z.; Zhang, J.; Zheng, X.; Zhou, L. A chromatic terahertz Airy beam generation with dielectric metasurfaces. Nanophotonics 2021, 10, 1123–1131. [Google Scholar] [CrossRef]
- Li, T.; Li, X.; Yan, S.; Xu, X.; Wang, S.; Yao, B.; Zhu, S. Generation and Conversion Dynamics of Dual Bessel Beams with a Photonic Spin-Dependent Dielectric Metasurface. Phys. Rev. Appl. 2021, 15, 014059. [Google Scholar] [CrossRef]
- Chen, S.; Liu, W.; Li, Z.; Cheng, H.; Tian, J. Metasurface-Empowered Optical Multiplexing and Multifunction. Adv. Mater. 2020, 32, 1805912. [Google Scholar] [CrossRef]
- Liu, Z.; Li, Z.; Cheng, H.; Liu, W.; Tang, C.; Tian, J. Single-layer plasmonic metasurface half-wave plates with wavelength-independent polarization conversion angle. ACS Photonics 2017, 4, 2061. [Google Scholar] [CrossRef]
- Cheng, H.; Liu, Z.; Chen, S.; Tian, J. Emergent Functionality and Controllability in Few-Layer Metasurfaces. Adv. Mater. 2015, 27, 5410–5421. [Google Scholar] [CrossRef]
- Zhu, W.; Zheng, H.; Zhong, Y.; Yu, J.; Chen, Z. Wave-Vector-Varying Pancharatnam-Berry Phase Photonic Spin Hall Effect. Phys. Rev. Lett. 2021, 126, 083901. [Google Scholar] [CrossRef]
- Grady, N.K.; Heyes, J.E.; Chowdhury, D.R.; Zeng, Y.; Reiten, M.T.; Azad, A.K.; Chen, H.T. Terahertz metamaterials for linear polarization conversion and anomalous refraction. Science 2013, 340, 1304. [Google Scholar] [CrossRef]
- Melentiev, P.N.; Kalmykov, A.; Kuzin, A.; Negrov, D.; Balykin, V.I. Open-Type SPP Waveguide with Ultrahigh Bandwidth up to 3.5 THz. ACS Photonics 2019, 6, 6. [Google Scholar] [CrossRef]
- Melentiev, P.N.; Kuzin, A.A.; Negrov, D.V.; Balykin, V.I. Diffraction-Limited Focusing of Plasmonic Wave by a Parabolic Mirror. Plasmonics 2018, 13, 2361–2367. [Google Scholar] [CrossRef]
- Melentiev, P.N.; Balykin, V.I. Nano-optical elements for surface plasmon waves: (50th anniversary of the Institute of Spectroscopy, Russian Academy of Sciences). Phys.-Usp. 2019, 62, 267–274. [Google Scholar] [CrossRef]
- Wang, Z.; Li, S.Q.; Zhang, X.Q.; Zhou, L.; Sun, S.L. Excite Spoof Surface Plasmons with Tailored Wavefronts Using High-Efficiency Terahertz Metasurfaces. Adv. Sci. 2020, 7, 2000982. [Google Scholar] [CrossRef]
- Gao, Y.J.; Xiong, X.; Wang, Z.H.; Chen, F.; Wang, M. Simultaneous Generation of Arbitrary Assembly of Polarization States with Geometrical-Scaling-Induced Phase Modulation. Phys. Rev. X 2020, 10, 3. [Google Scholar] [CrossRef]
- Li, H.; Hao, W.; Chen, S.; Chen, L. Broadband Generation of Airy Beams with Hyperbolic Metamaterials. Adv. Opt. Mater. 2019, 7, 20. [Google Scholar] [CrossRef]
- Li, H.; Tang, Y.; Yang, H.; Jin, G. Manipulating surface plasmon polaritons with M-shaped nanoslit array via polarized incident waves. EPL 2019, 127, 25001. [Google Scholar] [CrossRef]
- Epstein, I.; Remez, R.; Tsur, Y.; Arie, A. Generation of intensity-controlled two-dimensional shape-preserving beams in plasmonic lossy media. Optica 2016, 3, 15. [Google Scholar] [CrossRef]
- Yang, H.; Cao, G.; Ou, K.; Li, G.; Chen, X. Broadband Spin-Driven Anomalous Surface Plasmon Polariton Steering via V-Shaped Aperture Metasurfaces. Adv. Theory Simul. 2019, 2, 1800167. [Google Scholar] [CrossRef]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, X.; Wang, L.; Zhang, H.; Li, X.; Li, H. Modulation of Surface Plasmonic Bending Beam via Nanoslit Interactions. Photonics 2022, 9, 896. https://doi.org/10.3390/photonics9120896
Li X, Wang L, Zhang H, Li X, Li H. Modulation of Surface Plasmonic Bending Beam via Nanoslit Interactions. Photonics. 2022; 9(12):896. https://doi.org/10.3390/photonics9120896
Chicago/Turabian StyleLi, Xiaoming, Liang Wang, Hang Zhang, Xueli Li, and Hui Li. 2022. "Modulation of Surface Plasmonic Bending Beam via Nanoslit Interactions" Photonics 9, no. 12: 896. https://doi.org/10.3390/photonics9120896
APA StyleLi, X., Wang, L., Zhang, H., Li, X., & Li, H. (2022). Modulation of Surface Plasmonic Bending Beam via Nanoslit Interactions. Photonics, 9(12), 896. https://doi.org/10.3390/photonics9120896



