Dynamics of the Frequency Shifts in Semiconductor Lasers under the Injection of a Frequency Comb
Abstract
1. Introduction
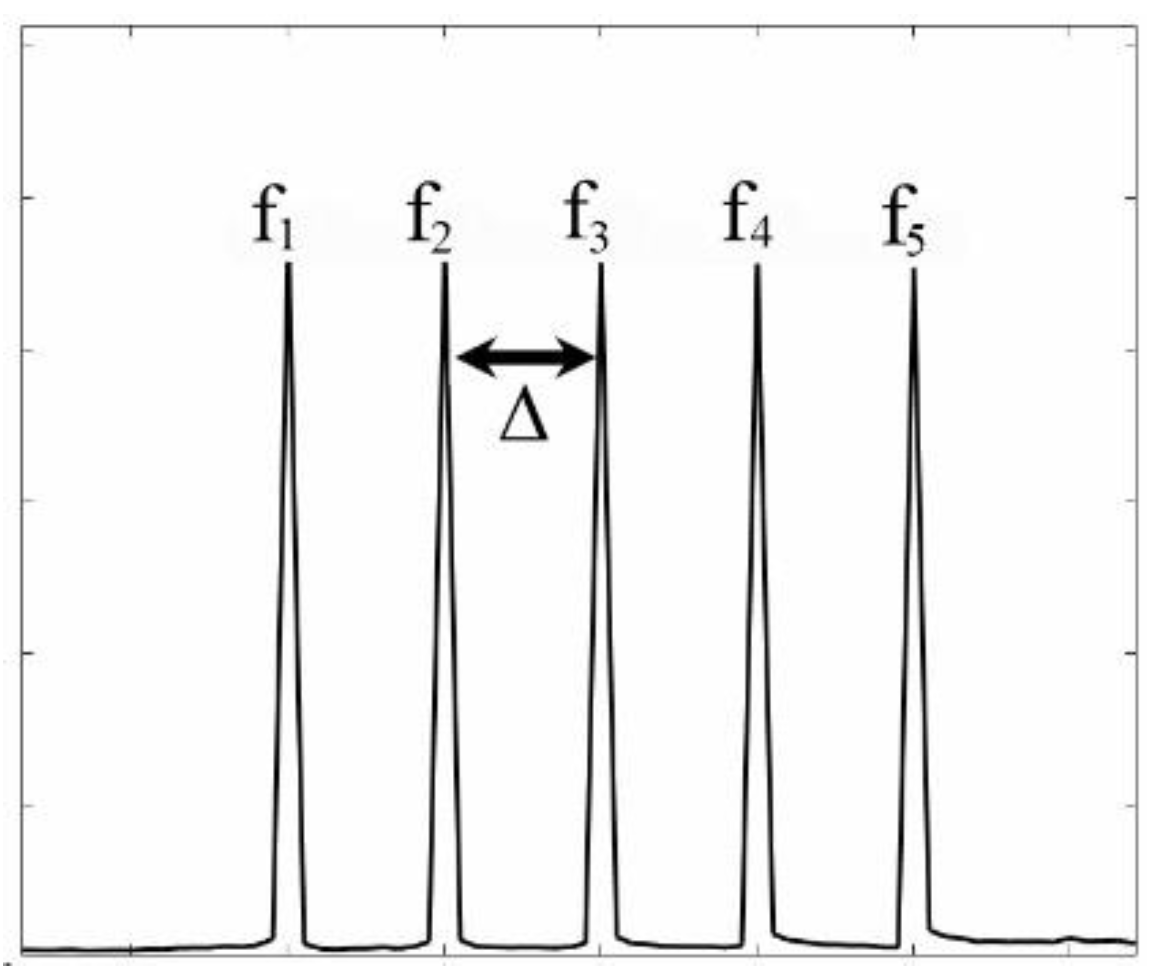
2. Materials and Methods
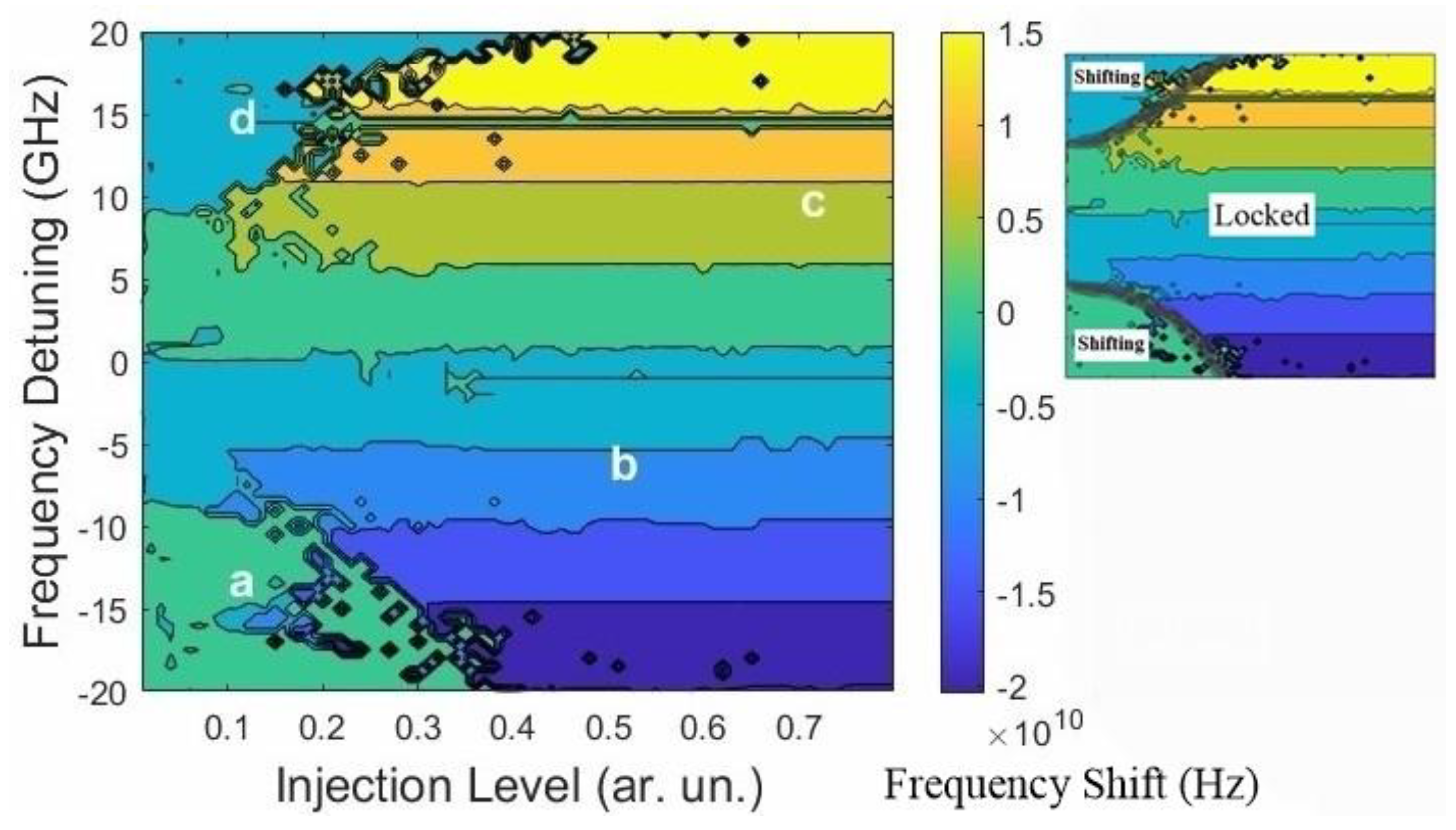
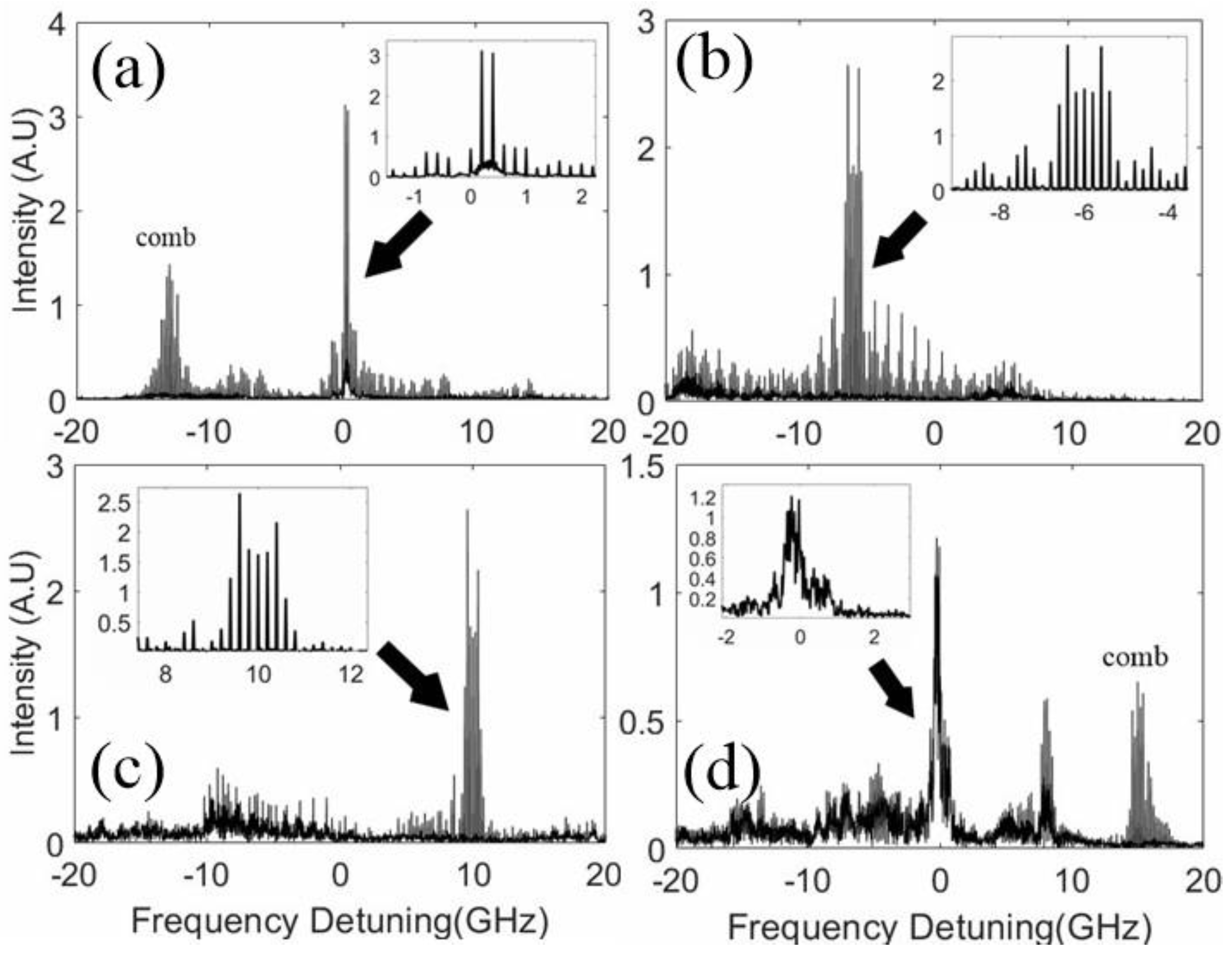
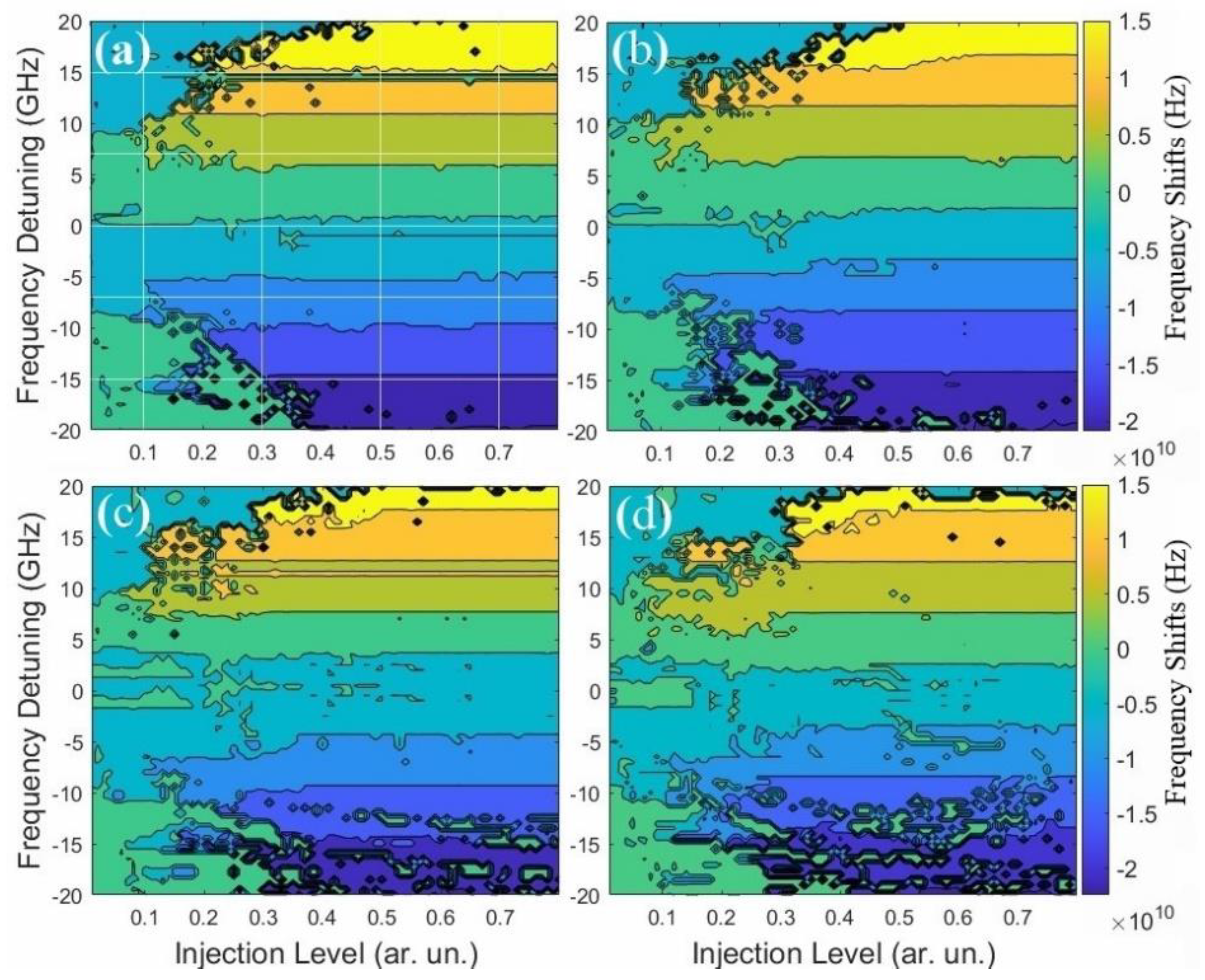
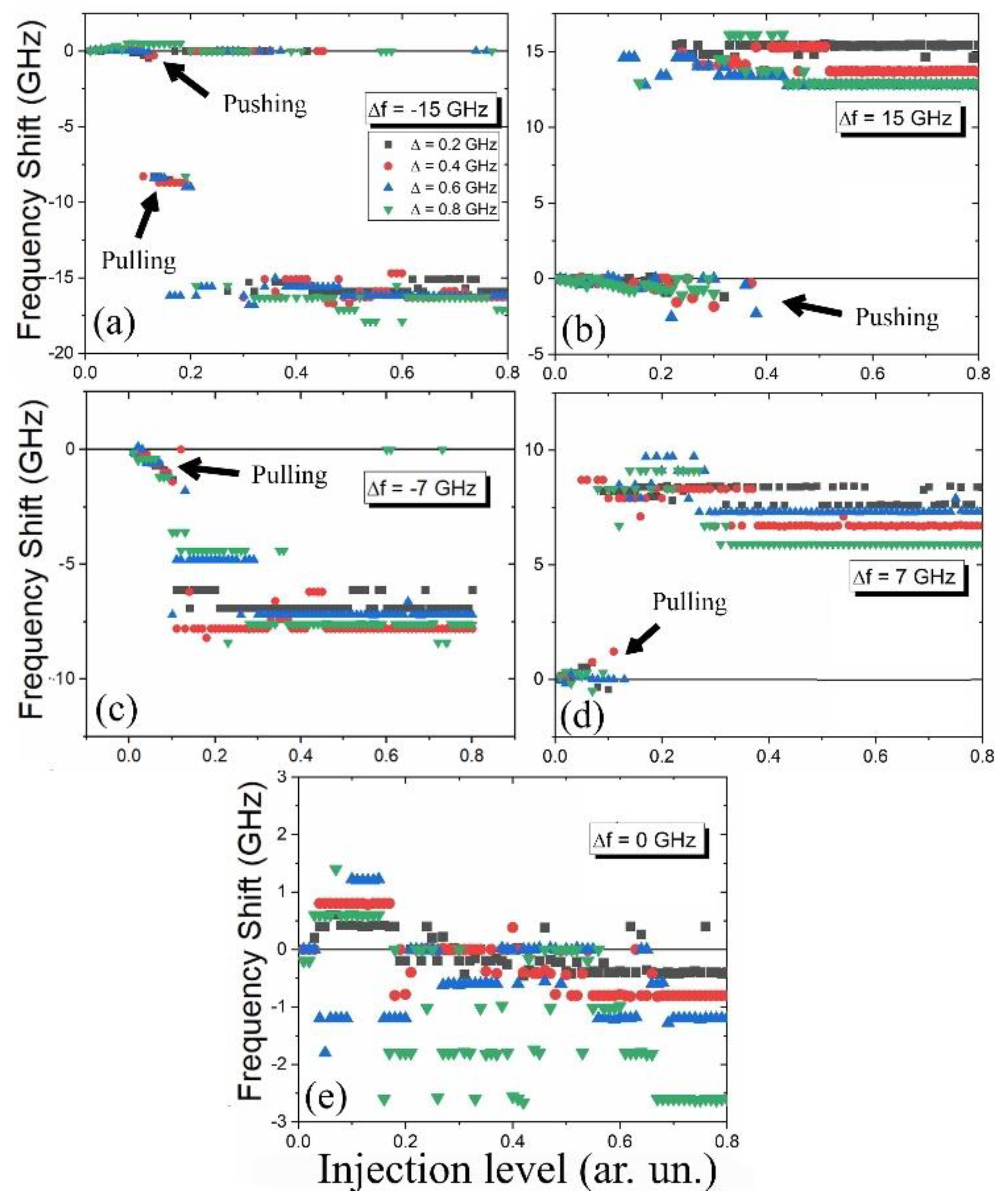
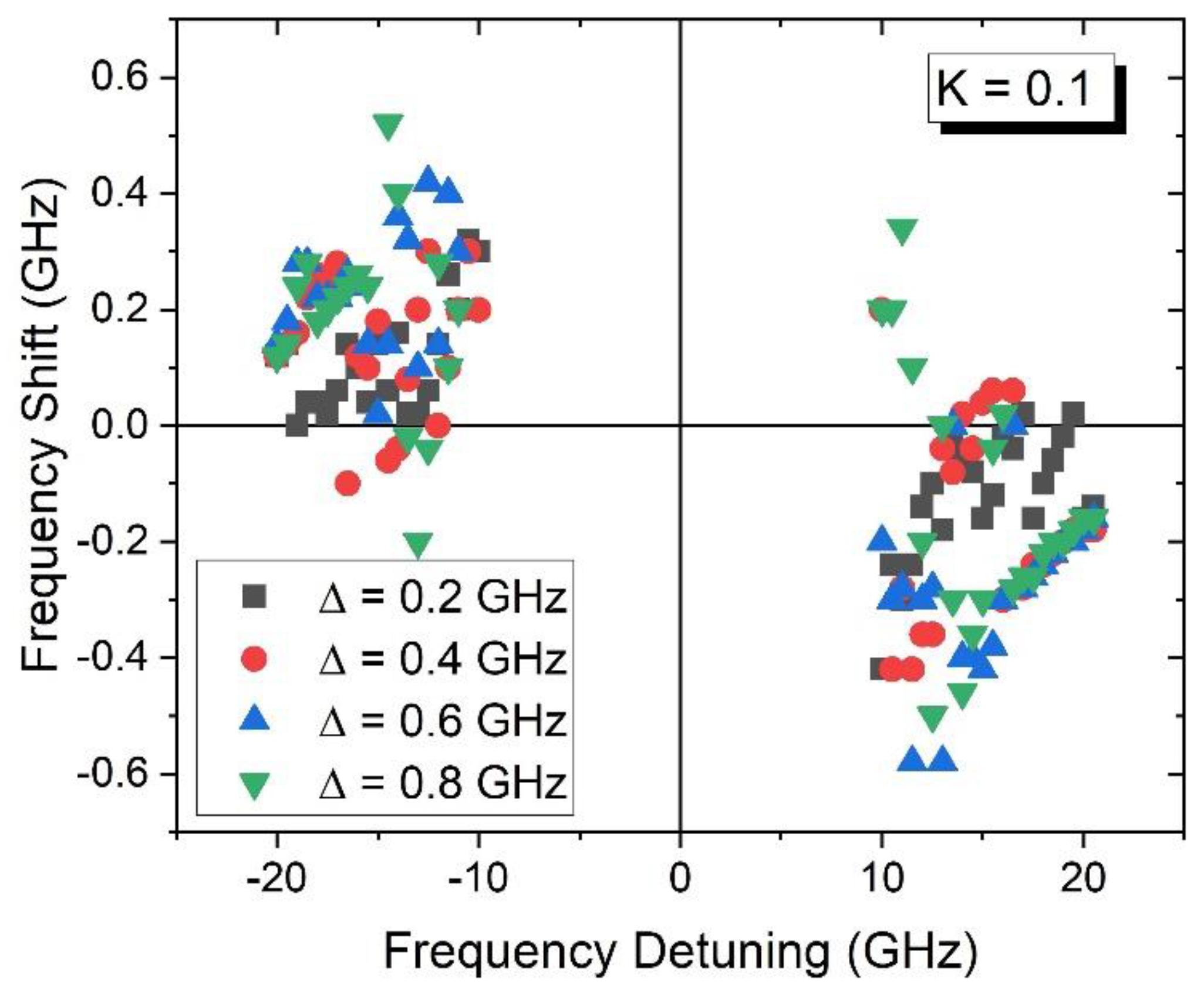
3. Results and Discussion
4. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ohtsu, M.; Nakagawa, K.; Kourogi, M.; Wang, W. Frequency control of semiconductor lasers. J. Appl. Phys. 1993, 73, R1–R17. [Google Scholar] [CrossRef]
- Sooudi, E.; Sygletos, S.; Ellis, A.D.; Huyet, G.; McInerney, J.G.; Lelarge, F.; Merghem, K.; Rosales, R.; Martinez, A.; Ramdane, A.; et al. Optical Frequency Comb Generation Using Dual-Mode Injection-Locking of Quantum-Dash Mode-Locked Lasers: Properties and Applications. IEEE J. Quantum Electron. 2012, 48, 1327–1338. [Google Scholar] [CrossRef]
- Rosado, A.; Pérez-Serrano, A.; Tijero JM, G.; Valle, Á.; Pesquera, L.; Esquivias, I. Experimental study of optical frequency comb generation in gain-switched semiconductor lasers. Opt. Laser Technol. 2018, 108, 542–550. [Google Scholar] [CrossRef]
- Rosado, A.; Perez-Serrano, A.; Tijero, J.M.G.; Gutierrez, A.V.; Pesquera, L.; Esquivias, I. Numerical and Experimental Analysis of Optical Frequency Comb Generation in Gain-Switched Semiconductor Lasers. IEEE J. Quantum Electron. 2019, 55, 2001012. [Google Scholar] [CrossRef]
- Zhao, B.-B.; Kovanis, V.; Wang, C. Tunable Frequency Comb Generation Using Quantum Cascade Lasers Subject to Optical Injection. IEEE J. Sel. Top. Quantum Electron. 2019, 25, 1900207. [Google Scholar] [CrossRef]
- Ren, H.; Fan, L.; Liu, N.; Wu, Z.; Xia, G. Generation of Broadband Optical Frequency Comb Based on a Gain-Switching 1550 nm Vertical-Cavity Surface-Emitting Laser under Optical Injection. Photonics 2020, 7, 95. [Google Scholar] [CrossRef]
- Moon, H.S.; Kim, E.B.; Park, S.E.; Park, C.Y. Selection and amplification of modes of an optical frequency comb using a femtosecond laser injection-locking technique. Appl. Phys. Lett. 2006, 89, 181110. [Google Scholar] [CrossRef]
- Ramond, T.M.; Hollberg, L.; Juodawlkis, P.W.; Calawa, S.D. Low-noise optical injection locking of a resonant tunneling diode to a stable optical frequency comb. Appl. Phys. Lett. 2007, 90, 171124. [Google Scholar] [CrossRef]
- Chan, S.-C.; Xia, G.-Q.; Liu, J.-M. Optical generation of a precise microwave frequency comb by harmonic frequency locking. Opt. Lett. 2007, 32, 1917. [Google Scholar] [CrossRef]
- Fukushima, S.; Silva, C.F.C.; Muramoto, Y.; Seeds, A.J. Optoelectronic millimeter-wave synthesis using an optical frequency comb generator, optically injection locked lasers and a unitraveling-carrier photodiode. J. Light. Technol. 2003, 21, 3043–3051. [Google Scholar] [CrossRef]
- Zhang, H.; Xu, M.; Zhang, J.; Jia, Z.; Campos, L.A.; Knittle, C. Highly Efficient Full-Duplex Coherent Optical System Enabled by Combined Use of Optical Injection Locking and Frequency Comb. J. Light. Technol. 2021, 39, 1271–1277. [Google Scholar] [CrossRef]
- Ohtsu, M. Frequency stabilization in semiconductor lasers. Opt. Quantum Electron. 1988, 20, 283–300. [Google Scholar] [CrossRef]
- Manamanni, K.; Steshchenko, T.; Wiotte, F.; Ramdane, A.C.; Sahni, M.-O.; Roncin, V.; Du-Burck, F. Frequency Stability Transfer in Passive Mode-Locked Quantum-Dash Laser Diode Using Optical Injection Locking. IEEE J. Quantum Electron. 2022, 58, 1300409. [Google Scholar] [CrossRef]
- Liu, Z.; Slavik, R. Optical Injection Locking: From Principle to Applications. J. Light. Technol. 2020, 38, 43–59. [Google Scholar] [CrossRef]
- Al-Hosiny, N.M.; Henning, I.D.; Adams, M.J. Tailoring enhanced chaos in optically injected semiconductor lasers. Opt. Commun. 2007, 269, 166–173. [Google Scholar] [CrossRef]
- Al-Hosiny, N.M. 3D Injection-locking maps of semiconductor laser under multiple optical injections. J. Opt. 2021, 50, 629–636. [Google Scholar] [CrossRef]
- Tistomo, A.S.; Gee, S. Laser frequency fixation by multimode optical injection locking. Opt. Express 2011, 19, 1081. [Google Scholar] [CrossRef]
- Gavrielides, A. Comb Injection and Sidebands Suppression. IEEE J. Quantum Electron. 2014, 50, 364–371. [Google Scholar] [CrossRef]
- Ó Duill, S.P.; Anandarajah, P.M.; Smyth, F.; Barry, L.P. Injection-locking criteria for simultaneously locking single-mode lasers to optical frequency combs from gain-switched lasers. In Physics and Simulation of Optoelectronic Devices XXV; Witzigmann, B., Osiński, M., Arakawa, Y., Eds.; SPIE: Bellingham, WA, USA, 2017; Volume 10098, pp. 51–59. [Google Scholar] [CrossRef]
- Shortiss, K.; Lingnau, B.; Dubois, F.; Kelleher, B.; Peters, F.H. Harmonic frequency locking and tuning of comb frequency spacing through optical injection. Opt. Express 2019, 27, 36976. [Google Scholar] [CrossRef]
- Doumbia, Y.; Malica, T.; Wolfersberger, D.; Panajotov, K.; Sciamanna, M. Nonlinear dynamics of a laser diode with an injection of an optical frequency comb. Optics Express 2020, 28, 30379. [Google Scholar] [CrossRef]
- Al-Hosiny, N.M.; Henning, I.D.; Adams, M.J. Correlation of Electron Density Changes with Optical Frequency Shifts in Optically Injected Semiconductor Lasers. IEEE J. Quantum Electron. 2006, 42, 570–580. [Google Scholar] [CrossRef]
- Hui, R.; D’Ottavi, A.; Mecozzi, A.; Spano, P. Injection locking in distributed feedback semiconductor lasers. IEEE J. Quantum Electron. 1991, 27, 1688–1695. [Google Scholar] [CrossRef]
- Al-Hosiny, N.; Henning, I.D.; Adams, M.J. Secondary locking regions in laser diode subject to optical injection from two lasers. Electron. Lett. 2006, 42, 759. [Google Scholar] [CrossRef]
- Simpson, T.B.; Liu, J.M.; Huang, K.F.; Tai, K. Nonlinear dynamics induced by external optical injection in semiconductor lasers. Quantum Semiclassical Opt. J. Eur. Opt. Soc. Part B 1997, 9, 765–784. [Google Scholar] [CrossRef]
- Liao, Y.-H.; Liu, J.-M.; Lin, F.-Y. Dynamical Characteristics of a Dual-Beam Optically Injected Semiconductor Laser. IEEE J. Sel. Top. Quantum Electron. 2013, 19, 1500606. [Google Scholar] [CrossRef]
- Goldberg, L.; Taylor, H.F.; Weller, J.F. Locking bandwidth asymmetry in injection-locked GaAlAs lasers. Electron. Lett. 1982, 18, 986. [Google Scholar] [CrossRef]

| Parameter | Symbol | Value |
|---|---|---|
| Wavelength | λ | 1556.6 nm |
| Differential Gain | GN | 1.4 × 10−12 m3 s−1 |
| Carrier lifetime | τs | 0.43 ns |
| Photon lifetime | τp | 1.8 ps |
| Coupling rate | η | 9 × 1010 s−1 |
| Transparency carrier density | No | 1.1 × 1024 m−3 |
| Threshold carrier density | Nth | 1.5 × 1024 m−3 |
| Normalized injection current | I/Ith | 2 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Al-Hosiny, N.M. Dynamics of the Frequency Shifts in Semiconductor Lasers under the Injection of a Frequency Comb. Photonics 2022, 9, 886. https://doi.org/10.3390/photonics9120886
Al-Hosiny NM. Dynamics of the Frequency Shifts in Semiconductor Lasers under the Injection of a Frequency Comb. Photonics. 2022; 9(12):886. https://doi.org/10.3390/photonics9120886
Chicago/Turabian StyleAl-Hosiny, Najm M. 2022. "Dynamics of the Frequency Shifts in Semiconductor Lasers under the Injection of a Frequency Comb" Photonics 9, no. 12: 886. https://doi.org/10.3390/photonics9120886
APA StyleAl-Hosiny, N. M. (2022). Dynamics of the Frequency Shifts in Semiconductor Lasers under the Injection of a Frequency Comb. Photonics, 9(12), 886. https://doi.org/10.3390/photonics9120886





