Non-Radiative Transitions of Holes on Mercury Vacancies in Narrow-Gap HgCdTe
Abstract
1. Introduction
2. Calculation Methods
3. Materials and Measurement Methods
4. Results and Discussion
4.1. The Rates of Hole Capture to the Localized Vacancy States
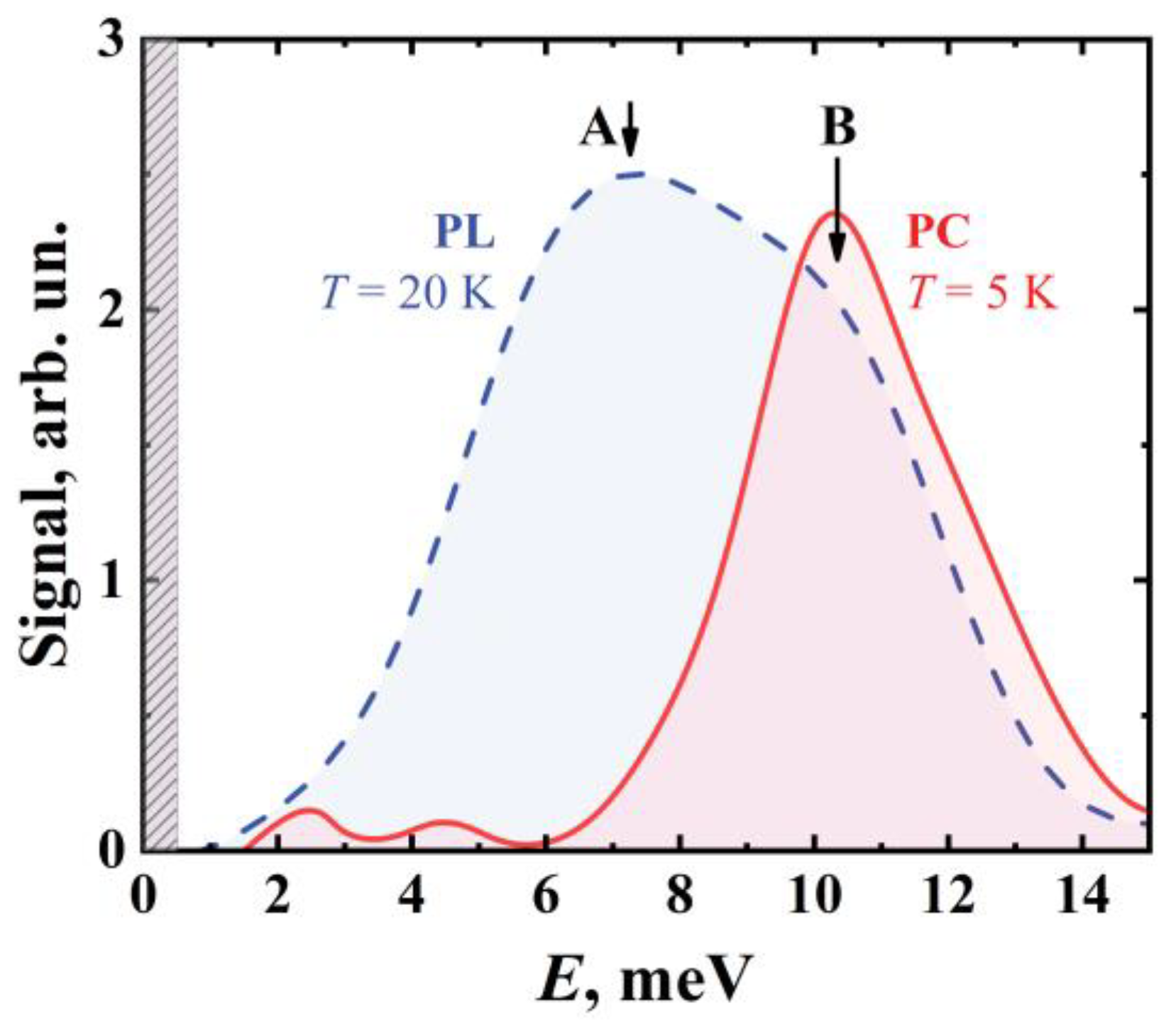
4.2. The Photoluminescence and Photoconductivity Vacancy-Related Spectra
4.3. Non-Radiative Intracenter Transitions of the Vacancy
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Kopytko, M.; Rogalski, A. New insights into the ultimate performance of HgCdTe photodiodes. Sens. Actuators A Phys. 2022, 339, 113511. [Google Scholar] [CrossRef]
- Kopytko, M.; Sobieski, J.; Gawron, W.; Martyniuk, P. Study of HgCdTe (100) and HgCdTe (111)B Heterostructures Grown by MOCVD and Their Potential Application to APDs Operating in the IR Range up to 8 µm. Sensors 2022, 22, 924. [Google Scholar] [CrossRef] [PubMed]
- Ruffenach, S.; Kadykov, A.; Rumyantsev, V.V.; Torres, J.; Coquillat, D.; But, D.; Krishtopenko, S.; Consejo, C.; Knap, W.; Winnerl, S.; et al. HgCdTe-based heterostructures for terahertz photonics. APL Mater. 2017, 5, 035503. [Google Scholar] [CrossRef]
- Gemain, F.; Robin, I.C.; De Vita, M.; Brochen, S.; Lusson, A. Identification of the double acceptor levels of the mercury vacancies in HgCdTe. Appl. Phys. Lett. 2011, 98, 131901. [Google Scholar] [CrossRef]
- Li, B.; Gui, Y.; Chen, Z.; Ye, H.; Chu, J.; Wang, S.; Ji, R.; He, L. Study of impurity states in p-type Hg1−xCdxTe using far-infrared spectroscopy. Appl. Phys. Lett. 1998, 73, 1538. [Google Scholar] [CrossRef]
- Sasaki, T.; Oda, N.; Kawano, M.; Sone, S.; Kanno, T.; Saga, M. Mercury annealing effect on the electrical properties of HgCdTe grown by molecular beam epitaxy. J. Cryst. Growth 1992, 117, 222. [Google Scholar] [CrossRef]
- Mynbaev, K.D.; Zablotsky, S.V.; Shilyaev, A.V.; Bazhenov, N.L.; Yakushev, M.V.; Marin, D.V.; Varavin, V.S.; Dvoretsky, S.A. Defects in Mercury-Cadmium Telluride Heteroepitaxial Structures Grown by Molecular-Beam Epitaxy on Silicon Substrates. Semiconductors 2016, 50, 208. [Google Scholar] [CrossRef]
- Shao, J.; Lü, X.; Guo, S.; Lu, W.; Chen, L.; Wei, Y.; Yang, J.; He, L.; Chu, J. Impurity levels and bandedge electronic structure in as-grown arsenic-doped HgCdTe by infrared photoreflectance spectroscopy. Phys. Rev. B 2009, 80, 155125. [Google Scholar] [CrossRef]
- Zhang, X.; Shao, J.; Chen, L.; Lü, X.; Guo, S.; He, L.; Chu, J. Infrared photoluminescence of arsenic-doped HgCdTe in a wide temperature range of up to 290 K. J. Appl. Phys. 2011, 110, 043503. [Google Scholar] [CrossRef]
- Andrianov, A.V.; Zakhar’in, A.O.; Ivanov, Y.L.; Kipa, M.S. Terahertz impurity luminescence under the interband photoexcitation of semiconductors. JETP Lett. 2010, 91, 96–99. [Google Scholar] [CrossRef]
- Firsov, D.A.; Vorobjev, L.E.; Panevin, V.Y.; Sofronov, A.N.; Balagula, R.M.; Makhov, I.S.; Kozlov, D.V.; Vasil’Ev, A.P. Terahertz radiation associated with impurity electron transitions in quantum wells at optical and electrical pumping. Semiconductors. 2015, 49, 28–32. [Google Scholar] [CrossRef]
- Kozlov, D.V.; Rumyantsev, V.V.; Kadykov, A.M.; Fadeev, M.A.; Kulikov, N.S.; Utochkin, V.V.; Mikhailov, N.N.; Dvoretskii, S.A.; Gavrilenko, V.I.; Hubers, H.-W.; et al. Features of Photoluminescence of Double Acceptors in HgTe/CdHgTe Heterostructures with Quantum Wells in a Terahertz Range. JETP Lett. 2019, 109, 657–662. [Google Scholar] [CrossRef]
- Kozlov, D.V.; Rumyantsev, V.V.; Morozov, S.V.; Kadykov, A.M.; Fadeev, M.A.; Zholudev, M.S.; Varavin, V.S.; Mikhailov, N.N.; Dvoretskii, S.A.; Gavrilenko, V.I.; et al. Terahertz Photoluminescence of Double Acceptors in Bulky Epitaxial HgCdTe Layers and HgTe/CdHgTe Structures with Quantum Wells. J. Exp. Theor. Phys. 2018, 127, 1125–1129. [Google Scholar] [CrossRef]
- Rumyantsev, V.V.; Kozlov, D.V.; Morozov, S.V.; Fadeev, M.A.; Kadykov, A.M.; Teppe, F.; Varavin, V.S.; Yakushev, M.V.; Mikhailov, N.N.; A Dvoretskii, S.; et al. Terahertz photoconductivity of double acceptors in narrow gap HgCdTe epitaxial films grown by molecular beam epitaxy on GaAs(013) and Si(013) substrates. Semicond. Sci. Technol. 2017, 32, 095007. [Google Scholar] [CrossRef]
- Nikolaev, I.D.; Uaman Svetikova, T.A.; Rumyantsev, V.V.; Zholudev, M.S.; Kozlov, D.V.; Morozov, S.V.; Dvoretsky, S.A.; Mikhailov, N.N.; Gavrilenko, V.I.; Ikonnikov, A.V. Probing States of a Double Acceptor in CdHgTe Heterostructures via Optical Gating. JETP Lett. 2020, 111, 575–581. [Google Scholar] [CrossRef]
- Kozlov, D.V.; Uaman Svetikova, T.A.; Ikonnikov, A.V.; Rumyantsev, V.V.; Razova, A.A.; Zholudev, M.S.; Mikhailov, N.N.; Dvoretskii, S.A.; Gavrilenko, V.I.; Morozov, S.V. Photothermal Ionization Spectroscopy of Mercury Vacancies in HgCdTe Epitaxial Films. JETP Lett. 2021, 113, 402–408. [Google Scholar] [CrossRef]
- Talwar, D.N.; Vandevyver, M. Vibrational properties of HgCdTe system. J. Appl. Phys. 1984, 56, 1601. [Google Scholar] [CrossRef]
- Gantmakher, V.F.; Levinson, Y.B. Carrier Scattering in Metals and Semiconductors; Science, Russian Federation: Moscow, Russia, 1984; 352p. [Google Scholar]
- Stillman, G.E.; Wolfe, C.M.; Dimmock, J.O. Hall coefficient factor for polar mode scattering in n-type GaAs. J. Phys. Chem. Solids 1970, 31, 1199–1204. [Google Scholar] [CrossRef]
- Novik, E.G.; Pfeuffer-Jeschke, A.; Jungwirth, T.; Latussek, V.; Becker, C.R.; Landwehr, G.; Buhmann, H.; Molenkamp, L.W. Band structure of semimagnetic Hg1−yMnyTe quantum wells. Phys. Rev. B 2005, 72, 035321. [Google Scholar] [CrossRef]
- Kushwaha, M.S.; Kushwaha, S.S. Lattice dynamics of ZnTe, CdTe, GaP, and InP. Can. J. Phys. 1980, 58, 351. [Google Scholar] [CrossRef]
- Kepa, H.; Giebultowicz, T.; Buras, B.; Lebech, B.; Claußen, K. A Neutron Scattering Study of Lattice Dynamics of HgTe and HgSe. Phys. Scr. 1982, 25, 807–809. [Google Scholar] [CrossRef]
- Rumyantsev, V.V.; Ikonnikov, A.V.; Antonov, A.V.; Morozov, S.V.; Zholudev, M.S.; Spirin, K.E.; Gavrilenko, V.I.; Dvoretskii, S.A.; Mikhailov, N.N. Specific features of the spectra and relaxation kinetics of long-wavelength photoconductivity in narrow-gap HgCdTe epitaxial films and heterostructures with quantum wells. Semiconductors 2013, 47, 1438–1441. [Google Scholar] [CrossRef]
- Morozov, S.V.; Rumyantsev, V.V.; Antonov, A.V.; Maremyanin, K.V.; Kudryavtsev, K.E.; Krasilnikova, L.V.; Mikhailov, N.N.; Dvoretskii, S.A.; Gavrilenko, V.I. Efficient long wavelength interband photoluminescence from HgCdTe epitaxial films at wavelengths up to 26 μm. Appl. Phys. Lett. 2014, 104, 072102. [Google Scholar] [CrossRef]
- Bethe, H.A.; Salpeter, E.E. Quantum Mechanics of One-and-Two-Electron Atoms; Berlin: Springer, Germany, 1957; 368p. [Google Scholar]
- Abakumov, V.N.; Perel, V.I.; Yassievich, I.N. Nonradiative Recombination in Semiconductors; Elsevier: Amsterdam, The Netherlands, 1991; pp. 191–197. [Google Scholar]
- Murzin, V.N. Submillimeter Spectroscopy of Collective and Bound Carrier States in Semiconductors; Science, Russian Federation: Moscow, Russia, 1985; 264p. [Google Scholar]
- Zholudev, M.S.; Rumyantsev, V.V.; Morozov, S.V. Calculation of Bound and Resonant Levels of Acceptors in Narrow-Gap CdHgTe Solid Solutions. JETP Lett. 2022, 116, 313–318. [Google Scholar] [CrossRef]
| State | Ei, meV | E, meV | |M|2, arb. un. | W, µs–1 | Ei*, meV | E*, meV | |M*|2, arb. un. |
|---|---|---|---|---|---|---|---|
| 1Γ8 + (ground) | 11.0 | ― | ― | 0.1 | 11.0 | ― | ― |
| 1Γ8 – | 7.01 | 3.99 | 15 | 11 | 4.65 | 6.45 | 13 |
| 2Γ8 – | 3.9 | 7.1 | 26 | 135 | 2.4 | 8.7 | 30 |
| 1Γ7 – | 3.07 | 7.93 | 134 | 620 | 1.8 | 9.3 | 152 |
| 3Γ8 – | 1.7 | 9.3 | 3 | 8000 | 1.15 | 9.95 | 3.5 |
| 4Γ8 – | 0.84 | 10.16 | 2 | 191,000 | 0.75 | 10.35 | 2.0 |
| 3Γ7 – | 0.42 | 10.58 | 43 | 422,000 | 0.33 | 10.77 | 48 |
| Transition | Ei, meV | E, meV | Frequency, µs–1 |
|---|---|---|---|
| 1Γ8 − → 1Γ8 + | 7.01 | 3.99 | 420 |
| 2Γ8 − → 1Γ8 + | 3.9 | 7.1 | 12 |
| 2Γ8 − → 1Γ8 − | 3.11 | 100 | |
| 1Γ7 − → 1Γ8 + | 3.07 | 7.93 | 3.5 |
| 1Γ7 − → 1Γ8 − | 3.94 | 30 | |
| 1Γ7 − → 2Γ8 − | 0.83 | 560 | |
| 3Γ7 − → 2Γ7 − | 0.42 | 0.13 | 8 |
| 3Γ7 − → 5Γ8 − | 0.28 | 50 | |
| 3Γ7 − → 4Γ8 − | 0.42 | 100 | |
| 3Γ7 − → 4Γ8 + | 0.43 | 95 | |
| 3Γ7 − → 3Γ8 + | 1.08 | 80 | |
| 3Γ7 − → 3Γ8 − | 1.28 | 90 | |
| 3Γ7 − → 2Γ8 + | 2.28 | 65 | |
| 3Γ7 − → 1Γ7 − | 2.65 | 60 | |
| 3Γ7 − → 2Γ8 − | 3.48 | 50 | |
| 3Γ7 − → 1Γ8 − | 6.59 | 30 | |
| 3Γ7 − → 1Γ8 + | 10.58 | 22 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kozlov, D.V.; Rumyantsev, V.V.; Ikonnikov, A.V.; Utochkin, V.V.; Razova, A.A.; Mazhukina, K.A.; Mikhailov, N.N.; Dvoretsky, S.A.; Morozov, S.V.; Gavrilenko, V.I. Non-Radiative Transitions of Holes on Mercury Vacancies in Narrow-Gap HgCdTe. Photonics 2022, 9, 887. https://doi.org/10.3390/photonics9120887
Kozlov DV, Rumyantsev VV, Ikonnikov AV, Utochkin VV, Razova AA, Mazhukina KA, Mikhailov NN, Dvoretsky SA, Morozov SV, Gavrilenko VI. Non-Radiative Transitions of Holes on Mercury Vacancies in Narrow-Gap HgCdTe. Photonics. 2022; 9(12):887. https://doi.org/10.3390/photonics9120887
Chicago/Turabian StyleKozlov, Dmitry V., Vladimir V. Rumyantsev, Anton V. Ikonnikov, Vladimir V. Utochkin, Anna A. Razova, Ksenia A. Mazhukina, Nikolay N. Mikhailov, Sergey A. Dvoretsky, Sergey V. Morozov, and Vladimir I. Gavrilenko. 2022. "Non-Radiative Transitions of Holes on Mercury Vacancies in Narrow-Gap HgCdTe" Photonics 9, no. 12: 887. https://doi.org/10.3390/photonics9120887
APA StyleKozlov, D. V., Rumyantsev, V. V., Ikonnikov, A. V., Utochkin, V. V., Razova, A. A., Mazhukina, K. A., Mikhailov, N. N., Dvoretsky, S. A., Morozov, S. V., & Gavrilenko, V. I. (2022). Non-Radiative Transitions of Holes on Mercury Vacancies in Narrow-Gap HgCdTe. Photonics, 9(12), 887. https://doi.org/10.3390/photonics9120887






