An Effective Method for Enhancing Heterodyne Efficiency by Comparing the Effect of Degree of Polarization on an Uplink Path and a Downlink Path

Abstract

By analyzing the effect of the degree of polarization (DoP) of the partially coherent Gaussian Schell-model (GSM) beam on a heterodyne system of an uplink path and a downlink path, we developed an innovative and noteworthy theory according to which σ_sy (signal beam waist radius in the y direction component) and δ_Syy (coherence length of the signal beam in the yy direction component) had a more significant impact on heterodyne efficiency and DoP than the turbulence term on uplink and downlink paths. Namely, the DoP and heterodyne efficiency of an uplink path are higher than that of a downlink path when σ_sy > 0.02 m or δ_Syy ≥ 0.03 m. This innovative rule provides an efficient way for increasing the heterodyne efficiency of a signal beam propagating along an uplink or a downlink path channel in satellite-ground communication links in free-space optical heterodyne detection communication.

1. Introduction

It is well known that optical heterodyne (coherent) detection technology is widely used in free-space optical communications (FSOCs), broadcasting, radar systems and other fields since the reception sensitivity of this technology is nearly 20 dB higher than that of intensity modulation/direct detection methods [1,2,3,4,5]. Heterodyne detection has been widely used in satellite–satellite [6], satellite–ground [7,8] and ground–ground [9] communication links in recent decades. The most significant effect on the performance of FSOC systems in the latter two applications is atmospheric turbulence [10], since the stochastic motion of the turbulent medium affects laser beam propagation. This propagation distortion causes a mismatch in the amplitude, phase, polarization and other characteristics of the signal beam and LO beam, reducing spatial coherence and the performance of the coherent optical communication system [11].

As a result, the polarization state and distorted wavefront of the signal beam should be corrected before heterodyne detection can be performed. Polarization state is a vector characteristic of electromagnetic light fields that is commonly employed in polarization control, free-space optical communications, particle capture and target detection and identification. The degree of polarization (DoP) is a physical quantity that describes the polarization state of a vector partly coherent beam.

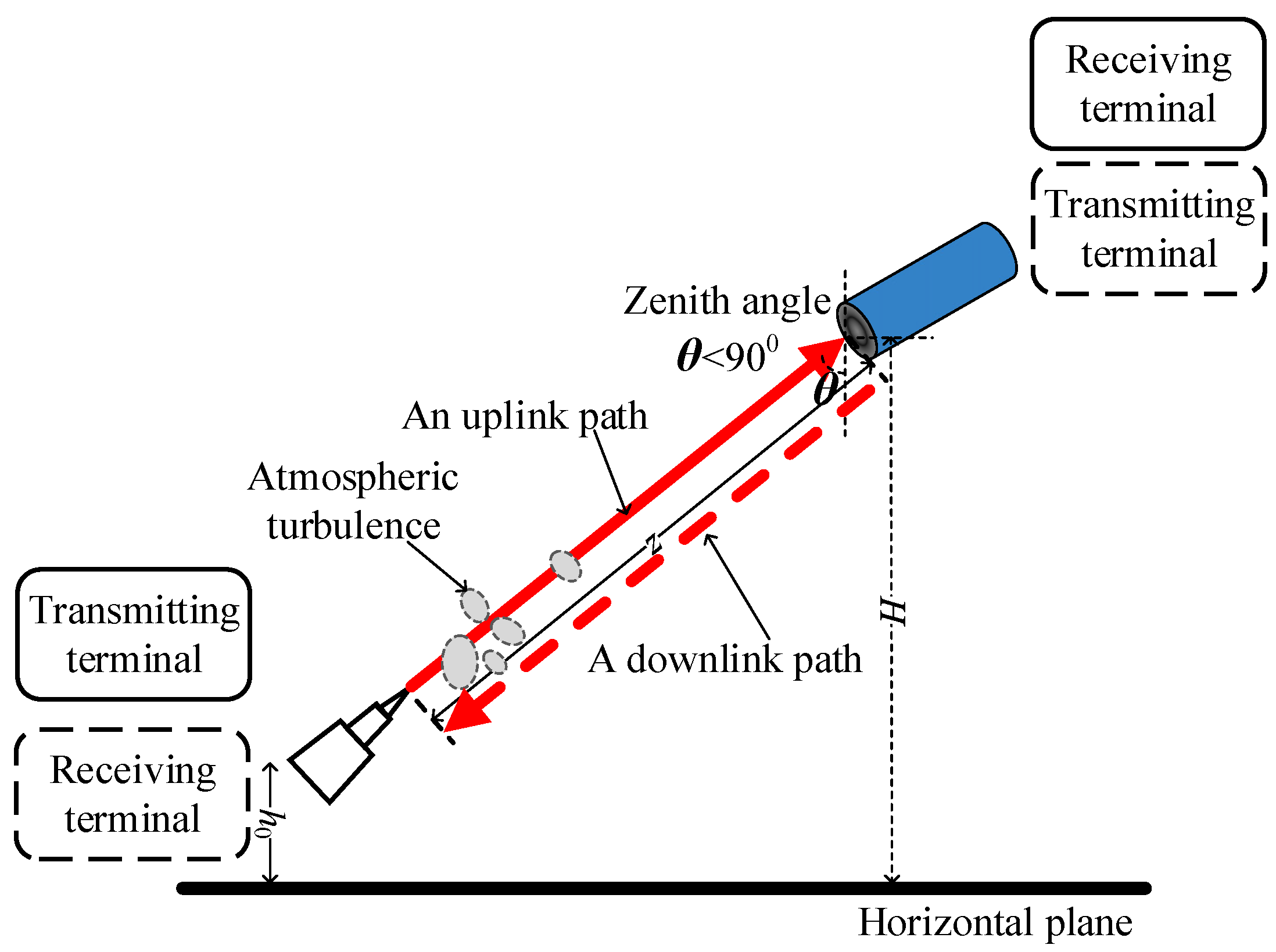
The signal beam propagating in the satellite–ground field (i.e., Figure 1) is less affected by atmospheric turbulence than the ground–ground communication links. The signal beam propagates through the atmosphere through an uplink or downlink path to reach the receiving terminal, as shown in Figure 1. The distance from the ground to the lower terminal and the vertical height of the receiving terminal, as illustrated in Figure 1, are h₀ and H, respectively. θ is the path zenith angle. The uplink and downlink transmission paths of the signal beam distorted by turbulent atmosphere are indicated by the red solid and dotted lines, respectively.

There has been an increased interest in the effect of atmospheric turbulence on the performance of heterodyne detection in recent years. Using the cross-spectral function of a GSM beam propagating through atmospheric turbulence, for example, Y.X. Ren et al. [12] derived a closed-form expression of heterodyne efficiency. C.Q. Li et al. [5] developed a mathematical model for a heterodyne detection system for partially coherent Gaussian Schell beams under the Tatarskii turbulence spectrum in 2015. Furthermore, some of the authors of this paper investigated the effect of signal beam propagation in atmospheric turbulence on heterodyne detection, e.g., angle-of-arrival fluctuation [3], beam mode of the partially coherent Gaussian Schell-model beam [1,2], irradiance and phase fluctuations [13] and wavefront correction [10]. However, these works were based on the vector theory of partially coherent light fields or Gaussian beam. In electromagnetic fields, partial polarization is a well-known phenomenon [5]. X. Z. Ke et al. investigated the polarization characteristics of the GSM beam [14] and electromagnetic Gaussian Schell-model vortex (EGSMV) beam in atmospheric turbulence [15]. In 2017, Y. F. Yang et al. [16] analyzed the effects of polarization aberrations on the output polarizations states. The signal-to-noise ratio (SNR) and the heterodyne efficiency for the partly polarized and coherent beam in free space were both expressed using the beam coherence-polarization (BCP) matrix, which was developed by Mohamed S. et al. [17,18]. Juan Pérez-Téllez et al. analyzed the polarization properties of the mixing of statistically independent optical fields in a coherent optical field mixing scheme [19]. Y. Wang et al. [20] investigated the impact of beam polarization on the effectiveness of the heterodyne detecting system.

However, the effects of the DoP of the GSM beams propagating along an uplink path and a downlink path in atmospheric turbulence on heterodyne detection have yet to be described. Therefore, the effects of the DoP of a partially coherent Gaussian Schell-model beam on a heterodyne detection system are investigated in this study as the signal beam propagating along an uplink or downlink path in atmospheric turbulence. Several analytical expressions for signal beam and LO beam as polarized and partially coherent GSM beam of the DoP and heterodyne efficiency are derived in our work based on a model of heterodyne detection for a partially coherent beam under the modified Hill spectrum of the refractive index fluctuations.

2. Mathematical Model of Heterodyne Detection with Partially Polarized GSM Beam Propagating along an Uplink Path and a Downlink Path in Turbulence

We suppose the signal beam and LO beam are partially polarized and partially coherent, although the LO beam is commonly polarized and coherent, to obtain a general heterodyne efficiency expression for a heterodyne detection system that includes the coherence and polarization characteristics of the light source. Let us consider that the signal beams and the LO beams are parallel to the optical axis and perpendicular to the detector surface. The instantaneous field of both beams on the detector surface can be expressed, respectively, as [5]:

$$U_L\left(\mathbf{\rho },t\right)=\left[A_{Lx}\left(\mathbf{\rho }\right)e_x+A_{Ly}\left(\mathbf{\rho }\right)e_y\right]e^{i{\omega }_{L}t}$$

(1)

$$U_S\left(\mathbf{\rho },t\right)=\left[A_{Sx}\left(\mathbf{\rho }\right)e_x+A_{Sy}\left(\mathbf{\rho }\right)e_y\right]e^{i{\omega }_{S}t}$$

(2)

where e_x and e_y are unit vectors, ω_L and ω_s are the central angular frequencies of the fields and A_Lx, A_Ly and A_Sx, A_Sy are the optical field distribution of the LO beam and the received signal beam in the direction of x and y, respectively. Based on the model of heterodyne detection, the detected intermediate frequency (IF) power can be evaluated according to the character of a square-law device, and the IF power is expressed as [21]:

$$P_{IF}={\Re }^2{\displaystyle \iint {\displaystyle \iint \mathrm{Re}\left\{T_r\left[{\mathit{W}}_{Lij}\left({\mathbf{\rho }}_1,{\mathbf{\rho }}_2,0\right){\mathit{W}}_{Sij}\left({\mathbf{\rho }}_1,{\mathbf{\rho }}_2,z\right)\right]\right\}{d}^2}{\rho }_1{d}^2{\rho }_2}$$

(3)

where T_r denotes the trace, W_Lij and W_Sij are the beam coherence polarization (BCP) matrix of the LO beam and signal beam, respectively [15], and ℜ is the detector responsivity, which is assumed to be uniform across the detector surface.

For coherent detection, a measurement of heterodyne performance is the dimensionless heterodyne efficiency η_het, which measures the loss in coherent power when the received signal and LO fields are not perfectly matched. The heterodyne efficiency for random fields is defined in an analogous manner in the case of deterministic fields, which can be expressed as [21]:

$${\eta }_{het}=\frac{{\displaystyle \iint {\displaystyle \iint \mathrm{Re}\left\{T_r\left[{\mathit{W}}_{Lij}\left({\mathbf{\rho }}_1,{\mathbf{\rho }}_2,0\right){\mathit{W}}_{Sij}\left({\mathbf{\rho }}_1,{\mathbf{\rho }}_2,z\right)\right]\right\}{d}^2}{\rho }_1{d}^2{\rho }_2}}{{\displaystyle \iint T_r\left[{\mathit{W}}_{Lii}\left(\mathbf{\rho },\mathbf{\rho },0\right)\right]}{d}^2\rho {\displaystyle \iint Tr\left[{\mathit{W}}_{Sii}\left(\mathbf{\rho },\mathbf{\rho },0\right)\right]}{d}^2\rho }$$

(4)

Equation (4) is the basic mathematical model for the partially coherent beam of heterodyne detection. In the following part, we will establish a mathematical model of a heterodyne detection considering the change of the DoP of the signal beam propagating along an uplink path and a downlink path in atmospheric turbulence. The signal beam and the LO beam are partially polarized and partially coherent beams at the source field, of which the BCP matrix can be expressed as [20]:

$${\mathit{W}}_{\alpha ij}^{\left(0\right)}\left({\mathit{r}}_1,{\mathit{r}}_2\right)=A_{\alpha i}{A}_{\alpha j}{B}_{\alpha ij}\exp \left[-\left(\frac{{\mathit{r}}_1^2}{4{\sigma }_{\alpha i}^2}+\frac{{\mathit{r}}_2^2}{4{\sigma }_{\alpha j}^2}\right)\right]\times \exp \left(-\frac{{\left|{\mathit{r}}_1-{\mathit{r}}_2\right|}^2}{2{\delta }_{\alpha ij}^2}\right)$$

(5)

where r₁ and r₂ denote any two-point position vector at plane z = 0 (z is the propagation distance of the signal beam). The subscript is α = L (LO beam) or S (signal beam) and (i,j) denote (x,y), respectively. The equation B_ij = 1, i = j; | B_ij | ≤ 1, I ≠ j; B_ij = $B_{ji}^{*}$ is satisfied by the parameter B_ij. A_αi and A_αj are the amplitude of the light field, and σ_αi and σ_αj are the beam waist radius in the x and y axes, respectively. The transverse coherence length of the beam is δ_αij (z = 0), which meets δ_ij = δ_ji.

Assuming that a signal beam propagates along the z axis, based on the generalized Huygens–Fresnel diffraction principle, each element of the BCP matrix of the partially polarized coherent beam propagated through the atmospheric turbulence can be easily obtained, which can be expressed as [22]:

$$\begin{array}{c}{\mathit{W}}_{\alpha ij}\left({\mathbf{\rho }}_1,{\mathbf{\rho }}_2,z\right)=\frac{1}{{\lambda }^2{z}^2}{\displaystyle \iint {\mathit{W}}_{\alpha ij}\left({\mathit{r}}_1,{\mathit{r}}_2,0\right)}\times \exp \left\{-\frac{ik}{2z}\left[{\left({\mathbf{\rho }}_1-{\mathit{r}}_1\right)}^2-{\left({\mathbf{\rho }}_2-{\mathit{r}}_2\right)}^2\right]\right\}\\ \times 〈\exp \left[{\phi }^{\ast }\left({\mathbf{\rho }}_1,{\mathit{r}}_1,z\right)+\phi \left({\mathbf{\rho }}_2,{\mathit{r}}_2,z\right)\right]〉d^2{\mathit{r}}_1{d}^2{\mathit{r}}_2\end{array}$$

(6)

where ρ₁ and ρ₂ denote the position vectors of any two points at the receiver plane, λ is the wavelength, k = 2π/λ and φ is the cross-correlation function of the complex phase of the spherical wave, which can be expressed as [23]:

$$〈\exp \left[{\phi }^{\ast }\left({\mathbf{\rho }}_1,{\mathit{r}}_1,z\right)+\phi \left({\mathbf{\rho }}_2,{\mathit{r}}_2,z\right)\right]〉\approx \exp \left[-\frac{1}{2}\left(M_1{Q}^2+M_{2}P\cdot Q+M_3{P}^2\right)\right]$$

(7)

where P = r₁− r₂, Q = ρ₁− ρ₂, M₁, M₂ and M₃ are, respectively:

$$\left\{\begin{array}{l}{M}_1=2{\pi }^2{k}^2\sec \theta {\displaystyle {\int }_{h_0}^H{\displaystyle {\int }_0^{\infty }{\Phi }_n\left(\kappa ,h\right)\times {\left(1-\xi \right)}^2{\kappa }^{3}d\kappa dh}}\\ M_2=4{\pi }^2{k}^2\sec \theta {\displaystyle {\int }_{h_0}^H{\displaystyle {\int }_0^{\infty }{\Phi }_n\left(\kappa ,h\right)\times \left(1-\xi \right)\xi {\kappa }^{3}d\kappa dh}}\\ M_3=2{\pi }^2{k}^2\sec \theta {\displaystyle {\int }_{h_0}^H{\displaystyle {\int }_0^{\infty }{\Phi }_n\left(\kappa ,h\right){\xi }^2{\kappa }^{3}d\kappa dh}}\end{array}\right.$$

where ξ denotes (h-h₀)/(H-h₀) in the downlink path and ξ denotes 1 − (h − h₀)/(H − h₀) in the uplink path. Φ_n(κ) is the spectrum of the refractive index fluctuations. Here, to overcome the application limitations of the Kolmogorov turbulence spectrum and considering both inner scale and outer scale effects at the same time, the modified Hill spectrum model Φ_n(κ) was used in this paper [22]. It is expressed as:

$${\Phi }_n\left(\kappa ,h\right)=0.033C_n^2\left(h\right)\left[1+1.802\frac{\kappa }{{\kappa }_l}-0.254{\left(\frac{\kappa }{{\kappa }_l}\right)}^{7/6}\right]\frac{\exp \left(-{\kappa }^2/{\kappa }_l^2\right)}{{\left({\kappa }^2+{\kappa }_0^2\right)}^{11/6}}$$

(8)

where κ_l = 3.3/l₀; κ₀ = 2π/L₀, l₀ is the inner scale and L₀ is the outer scale. For a signal beam propagating in a slant path, the Hufnagel–Valley model is widely used to approximate the atmospheric refractive index structure constant $C_n^2$(h) [22]:

$$C_n^2(h)=8.148\times {10}^{-56}{v}_{RMS}^2{h}^{10}{e}^{-h/1000}+2.7\times {10}^{-16}{e}^{-h/1500}+C_0{e}^{-h/100}$$

(9)

where ν_RMS = ( ${\mathit{\nu }}_g^2$ + 30.69ν_g + 348.91)^1/2 is the speed of wind in the vertical path, ν_g is the surface wind speed and h is the distance from the ground. Generally, ν_g = 21 m/s, and the refractive index structure constant C₀ is 1.7 ×10⁻¹⁴ m^−2∕3.

By substituting Equations (5), (7)–(9) by Equation (6) and a complex integral simplification [24], W_αij(ρ₁,ρ₂,z) can be simplified as:

$$\begin{array}{cc}\hfill {\mathit{W}}_{\alpha ij}\left({\mathbf{\rho }}_1,{\mathbf{\rho }}_2,z\right)=& \frac{k^2{A}_i{A}_j{B}_{ij}}{4z^2{\Delta }_{\alpha \mathrm{i}j}\sqrt{Q{\left(z\right)}_{\alpha ij}^2+T{\left(z\right)}_{\alpha ij}^2}}\hfill \\ & \exp \left[M_{\alpha ij}\left(z\right){\left({\rho }_1-{\rho }_2\right)}^2+I_{\alpha ij}\left(z\right){\left({\rho }_1+{\rho }_2\right)}^2+G_{\alpha ij}\left(z\right)\left({\mathbf{\rho }}_1^2-{\mathbf{\rho }}_2^2\right)\right]\hfill \\ & \times \exp \left[i{L}_{\alpha ij}\left(z\right){\left({\rho }_1-{\rho }_2\right)}^2+i{Y}_{\alpha ij}\left(z\right){\left({\rho }_1+{\rho }_2\right)}^2+i{N}_{\alpha ij}\left(z\right)\left({\mathbf{\rho }}_1^2-{\mathbf{\rho }}_2^2\right)\right]\hfill \end{array}$$

(10)

By substituting Equation (10) by Equation (3), and converting to the polar coordinates ρ and φ, the power associated with the IF signal photocurrent can be expressed as:

$$P_{IF}={\displaystyle {\int }_0^{2\pi }{\displaystyle {\int }_0^{2\pi }{\displaystyle {\int }_0^{D/2}{\displaystyle {\int }_0^{D/2}\left.\mathrm{Re}\left\{{\mathit{W}}_{Lij}\left({\rho }_1,{\rho }_2,z\right)\cdot {\mathit{W}}_{Sij}^{\ast }\left({\rho }_1,{\rho }_2,z\right)\right.\right\}{\rho }_1{\rho }_2\mathit{d}{\rho }_1\mathit{d}{\rho }_2\mathit{d}{\phi }_1\mathit{d}{\phi }_2}}}}$$

(11)

where D is the hard aperture diameter of the detector. To simplify the calculation, we use the Gaussian limiting aperture, or soft aperture, to replace the hard aperture. Using the relationship between the soft aperture w and the hard aperture, D is D² = 8w² [25] and ρ₁ρ₂ = ρ₁ρ₂cos(φ₁-φ₂). The complex integration is performed as shown in detail in Appendix A. Then, P_IFij can be simplified as:

$$\begin{array}{cc}\hfill P_{IFij}=& \frac{{\pi }^2{k}^4{A}_i{}^2{A}_j{}^2{B}_{ij}{}^2}{8z^4{\Delta }_{\mathrm{Li}j}{\Delta }_{\mathrm{Si}j}\left[s_{ij}-g_{ij}-i\left(e_{ij}-m_{ij}\right)\right]\sqrt{Q{\left(z\right)}_{Lij}^2+T{\left(z\right)}_{Lij}^2}\sqrt{Q{\left(z\right)}_{Sij}^2+T{\left(z\right)}_{Sij}^2}}\hfill \\ & \times \frac{1}{2\left[-\left(g_{ij}+s_{ij}+i\left(m_{ij}+e_{ij}\right)\right)-\frac{{\left(q_{ij}+i{n}_{ij}\right)}^2}{s_{ij}-g_{ij}-i\left(e_{ij}-m_{ij}\right)}\right]}\hfill \end{array}$$

(12)

Based on the results obtained in Appendix A and Equation (12), it is possible to express the heterodyne efficiency of the partly polarized GSM beam in the turbulence of heterodyne detection as:

$${\eta }_h=\frac{{\displaystyle {\sum }_{i,j=x,y}{P}_{IFij}}}{{\displaystyle {\sum }_{i=x,y}{P}_{Lii}}{\displaystyle {\sum }_{i=x,y}{P}_{Sii}}}$$

(13)

3. Mathematical Model of DoP with Partially Polarized GSM Beam Propagating along an Uplink Path and a Downlink Path in Turbulence

When the signal beam passes through the receiver in the uplink or downlink slant path, let ρ₁ = ρ₂ = ρ. Based on Equation (10) and the integral formulation, W_Sij(ρ,ρ,z) can be expressed as:

$${\mathit{W}}_{Sij}\left(\mathbf{\rho },\mathbf{\rho },z\right)=\frac{k^2{A}_i{A}_j{B}_{ij}}{4z^2{\Delta }_{\mathrm{Si}j}\sqrt{Q{\left(z\right)}_{Sij}^2+T{\left(z\right)}_{Sij}^2}}\exp \left[4I_{Sij}\left(z\right){\mathbf{\rho }}^2\right]\exp \left[i4Y_{Sij}\left(z\right){\mathbf{\rho }}^2\right]$$

(14)

The DoP of the received signal beam is described with the generalized Stokes parameter, which can be expressed as [15]:

$$P\left(\mathbf{\rho };z\right)=\frac{\sqrt{S_1^2+S_2^2+S_3^2}}{S_0}$$

(15)

where S₀, S₁, S₂ and S₃ can be expressed with the cross-spectral density matrix (CSDM), namely,

$$\left\{\begin{array}{l}{S}_0={\mathit{W}}_{Sxx}\left(\mathbf{\rho },\mathbf{\rho };z\right)+{\mathit{W}}_{Syy}\left(\mathbf{\rho },\mathbf{\rho };z\right)\\ S_1={\mathit{W}}_{Sxx}\left(\mathbf{\rho },\mathbf{\rho };z\right)-{\mathit{W}}_{Syy}\left(\mathbf{\rho },\mathbf{\rho };z\right)\\ S_2={\mathit{W}}_{Sx\mathrm{y}}\left(\mathbf{\rho },\mathbf{\rho };z\right)+{\mathit{W}}_{Syx}\left(\mathbf{\rho },\mathbf{\rho };z\right)\\ S_3=i\left[{\mathit{W}}_{Syx}\left(\mathbf{\rho },\mathbf{\rho };z\right)-{\mathit{W}}_{Sxy}\left(\mathbf{\rho },\mathbf{\rho };z\right)\right]\end{array}\right.$$

(16)

The Stokes parameter of the receiving end (z > 0), which may be expressed using Equations (15) and (16), is

$$\{\begin{array}{cc}\hfill S_0=& \frac{k^2{A}_x{A}_x{B}_{xx}}{4z^2{\Delta }_{Sxx}\sqrt{Q{\left(z\right)}_{Sxx}^2+T{\left(z\right)}_{Sxx}^2}}\exp \left[4I_{Sxx}\left(z\right){\mathbf{\rho }}^2\right]\exp \left[i4Y_{Sxx}\left(z\right){\mathbf{\rho }}^2\right]\hfill \\ & +\frac{k^2{A}_y{A}_y{B}_{yy}}{4z^2{\Delta }_{Syy}\sqrt{Q{\left(z\right)}_{Syy}^2+T{\left(z\right)}_{Syy}^2}}\exp \left[4I_{Syy}\left(z\right){\mathbf{\rho }}^2\right]\exp \left[i4Y_{Syy}\left(z\right){\mathbf{\rho }}^2\right]\hfill \\ \hfill S_1=& \frac{k^2{A}_x{A}_x{B}_{xx}}{4z^2{\Delta }_{Sxx}\sqrt{Q{\left(z\right)}_{Sxx}^2+T{\left(z\right)}_{Sxx}^2}}\exp \left[4I_{Sxx}\left(z\right){\mathbf{\rho }}^2\right]\exp \left[i4Y_{Sxx}\left(z\right){\mathbf{\rho }}^2\right]\hfill \\ & -\frac{k^2{A}_y{A}_y{B}_{yy}}{4z^2{\Delta }_{Syy}\sqrt{Q{\left(z\right)}_{Syy}^2+T{\left(z\right)}_{Syy}^2}}\exp \left[4I_{Syy}\left(z\right){\mathbf{\rho }}^2\right]\exp \left[i4Y_{Syy}\left(z\right){\mathbf{\rho }}^2\right]\hfill \\ \hfill S_2=& \frac{k^2{A}_x{A}_y{B}_{xy}}{4z^2{\Delta }_{Sxy}\sqrt{Q{\left(z\right)}_{Sxy}^2+T{\left(z\right)}_{Sxy}^2}}\exp \left[4I_{Sxy}\left(z\right){\mathbf{\rho }}^2\right]\exp \left[i4Y_{Sxy}\left(z\right){\mathbf{\rho }}^2\right]\hfill \\ & +\frac{k^2{A}_y{A}_x{B}_{yx}}{4z^2{\Delta }_{Syx}\sqrt{Q{\left(z\right)}_{Syx}^2+T{\left(z\right)}_{Syx}^2}}\exp \left[4I_{Syx}\left(z\right){\mathbf{\rho }}^2\right]\exp \left[i4Y_{Syx}\left(z\right){\mathbf{\rho }}^2\right]\hfill \\ \hfill S_3=& i\{\frac{k^2{A}_y{A}_x{B}_{yx}}{4z^2{\Delta }_{Syx}\sqrt{Q{\left(z\right)}_{Syx}^2+T{\left(z\right)}_{Syx}^2}}\exp \left[4I_{Syx}\left(z\right){\mathbf{\rho }}^2\right]\exp \left[i4Y_{Syx}\left(z\right){\mathbf{\rho }}^2\right]\hfill \\ & -\frac{k^2{A}_x{A}_y{B}_{xy}}{4z^2{\Delta }_{Sxy}\sqrt{Q{\left(z\right)}_{Sxy}^2+T{\left(z\right)}_{Sxy}^2}}\exp \left[4I_{Sxy}\left(z\right){\mathbf{\rho }}^2\right]\exp \left[i4Y_{Sxy}\left(z\right){\mathbf{\rho }}^2\right]\}\hfill \end{array}$$

(17)

The DoP of the point on the optical axis at the receiving plane can be set by choosing proper parameters according to Equations (13) and (17).

4. Numerical and Discussion

Based on Equations (13) and (17), the effects of the source parameters, turbulence parameters and propagation distance on the DoP and the performance of the partially coherent GSM beam heterodyne detection system are investigated. For convenience and with no special instructions, the simulation parameters were set as

Table 1. Simulation parameter settings.

Light Source Parameters		Transmission Medium and Other Parameters
Wavelength	λ = 1550 nm	Detector diameter		D = 2 mm
Coherence length of the signal beam (z = 0)	δsxx = 2 cm	Atmospheric turbulence parameters	Atmospheric refractive index structure constant	C0 = 10−14 m−2/3
	δsyy = 1 cm
	δsxy = 2.5 cm
	δsyx = 2.5 cm		Inner scale	l0 = 1 cm
Coherence length of LO beam (z = 0)	inf		Outer scale	L0 = 10 m
	inf
	inf		Zenith angle	θ = arccos(H/z)
	inf
Waist radius of signal beam (z = 0)	σsx = 1 cm	Propagation distance on an uplink (or downlink) path		z = 5000 m
	σsy = 1 cm
Waist radius of LO beam (z = 0)	σlx = 2 cm
	σly = 2 cm
Correlation length	Bij = 0.5exp(iπ/4)	Vertical height		H = 500 m

, the correlation length B_ij satisfies max{δ_αxx, δ_αyy } ≤ δ_αxy ≤ min{δ_αxx/|B_xy|^0.5, δ_αyy/|B_xy|^0.5}.

Figure 2a illustrates the DoP and heterodyne efficiency of on-axis points (ρ = 0) as a function of σ_sx for uplink and downlink paths. As seen in Figure 2a, the DoP of the signal beam increases and eventually stabilizes as σ_sx increases, which is similar to the heterodyne efficiency as a function of σ_sx. Overall, signal beam propagation on a downlink path has a greater Dop and heterodyne efficiency than on an uplink path, which is consistent with Ref [15]. Figure 2b illustrates the DoP of deviation from the axial position for various σ_sy on the uplink and downlink paths. The heterodyne efficiency as a function of σ_sy for the uplink and downlink paths is shown in Figure 2c. As shown in Figure 2b, the variation of DoP with the deviation from the axial position on the downlink and uplink paths was decreased with an increase in σ_sy. As seen in Figure 2c, heterodyne efficiency decreases with increasing σ_sy; however, there seems to be a key point at σ_sy= 0.02 m. When σ_sy≤ 0.02 m, the heterodyne efficiency of the signal beam propagation on a downlink path is higher than on an uplink path; however, when σ_sy> 0.02 m, the result of heterodyne efficiency on a downlink vs. an uplink path is precisely the opposite. This is attributed to the fact that σ_sy has a major effect on the DoP of the downlink path, which has a significant impact on heterodyne efficiency.

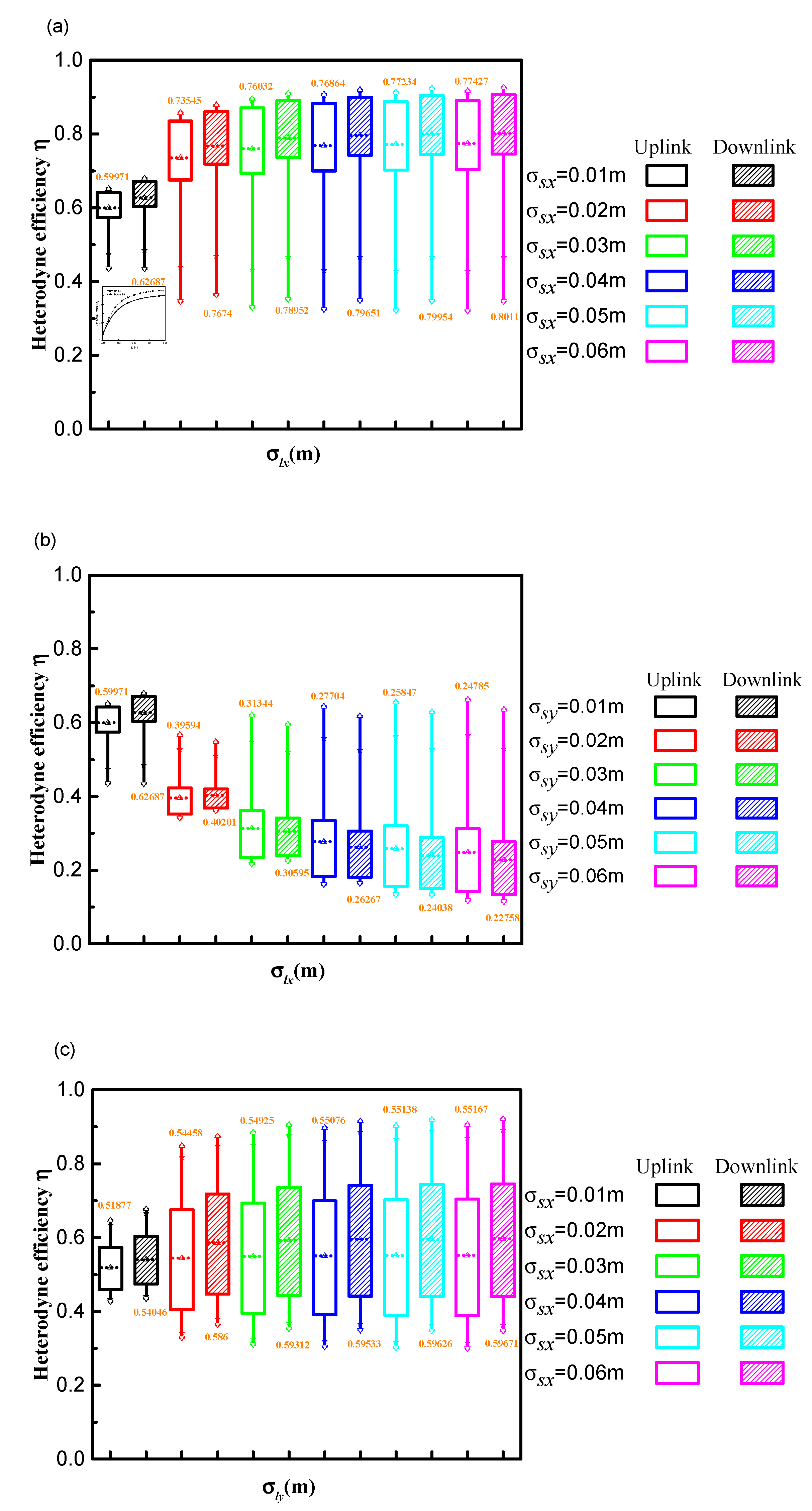
Figure 3a,b show the heterodyne efficiency as a function of σ_lx for various σ_sx and σ_sy of the uplink and downlink path, respectively. Figure 3c,d show the heterodyne efficiency as a function of σ_ly for various σ_sx and σ_sy of the uplink and downlink path, respectively. In Figure 3a–d, the top and bottom symbols in each box represent the maximum and minimum values, respectively. The mean value is indicated by the number at the top or bottom of each box. Comparing Figure 3a,c, heterodyne efficiency increases with increasing σ_lx and σ_ly, respectively. Heterodyne efficiency on the downlink path is higher than on the uplink path in Figure 3a,c. Additionally, the waist radius of the LO beam in the x direction component σ_lx has a relatively strong effect on the heterodyne efficiency. Comparing Figure 3a,b, heterodyne efficiency improves and decreases with increasing σ_Sx and σ_Sy, respectively. Finally, heterodyne efficiency eventually stabilizes. When σ_sy ≤ 0.02 m, heterodyne efficiency is higher for a downlink path than for an uplink path, as shown in Figure 3b,d. However, when σ_sy is larger than 0.02 m, the heterodyne efficiency of the downlink path is lower than that of the uplink path. That is because the degree of mismatch between the signal beam and the LO beam increases as σ_sy increases, which has a greater impact than the effect of the turbulence term on an uplink and downlink path. Comparing Figure 3c,d, heterodyne efficiency improves and decreases with increasing σ_Sx and σ_Sy, respectively, which is consistent with the results presented in Figure 3a,b.

Overall, heterodyne efficiency is significantly impacted by the signal beam waist radius in the Y direction component σ_sy. Meanwhile, σ_Sy plays a significant role in determining the trend of heterodyne efficiency with increasing σ_lx (or σ_ly) and this change of degree.

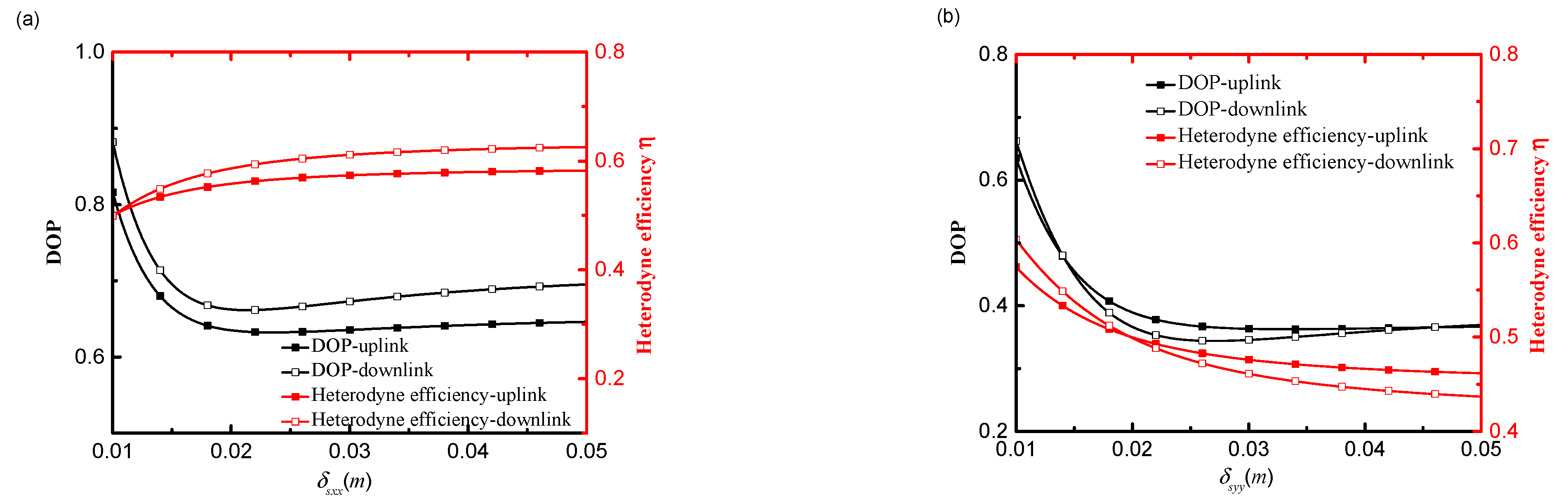
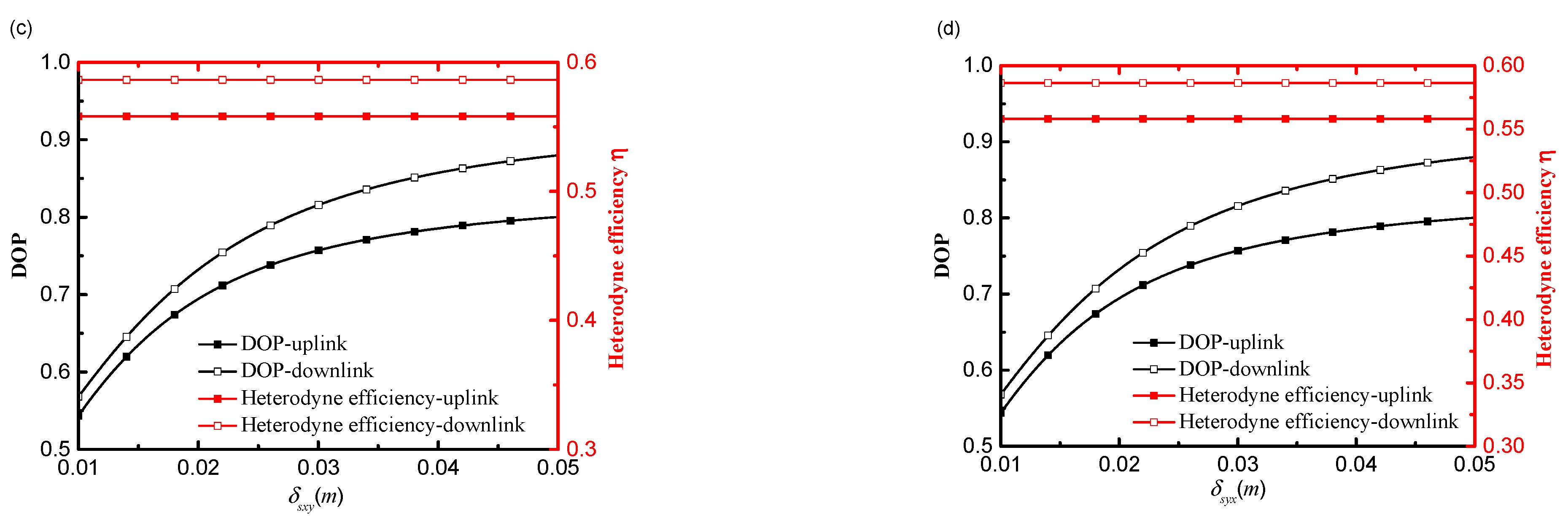
Figure 4a–d show heterodyne efficiency and DoP as a function of δ_Sxx, δ_Syy, δ_Sxy and δ_Syx of an uplink and downlink path, respectively. As seen in Figure 4a, heterodyne efficiency increases and the DoP of the signal beam on-axis points decreases with an increase in δ_Sxx. The heterodyne efficiency and DoP of the signal beam propagation on a downlink path are higher than on an uplink path. That is because the difference between uplink and downlink affected by atmospheric turbulence is expressed in the turbulence term M₃ in Equation (7), i.e., M₃ of a downlink transmission is less than that of an uplink transmission. The heterodyne efficiency and DoP on a downlink and uplink path decrease with an increase in δ_Syy, which is consistent with the results presented in Figure 2c. The heterodyne efficiency of a downlink path is lower than that of an uplink path when δ_Syy reaches a certain value. Namely, the variation of heterodyne efficiency of a downlink and uplink path is increased with an increase in δ_Syy. As seen in Figure 4c,d, heterodyne efficiency remains consistent as δ_Sxy and δ_Syx increase, but the DoP of the on-axis points increases with an increase in δ_Sxy and δ_Syx. The heterodyne efficiency and DoP of a downlink path are higher than that of an uplink path. This demonstrates that the coherence length of the signal beam in the xy (or yx) direction component has a significant effect on the DoP, but is less sensitive with regard to heterodyne efficiency. Generally, δ_Syy has the most significant effect on the heterodyne efficiency and DoP of the signal beam propagation on a downlink and uplink path.

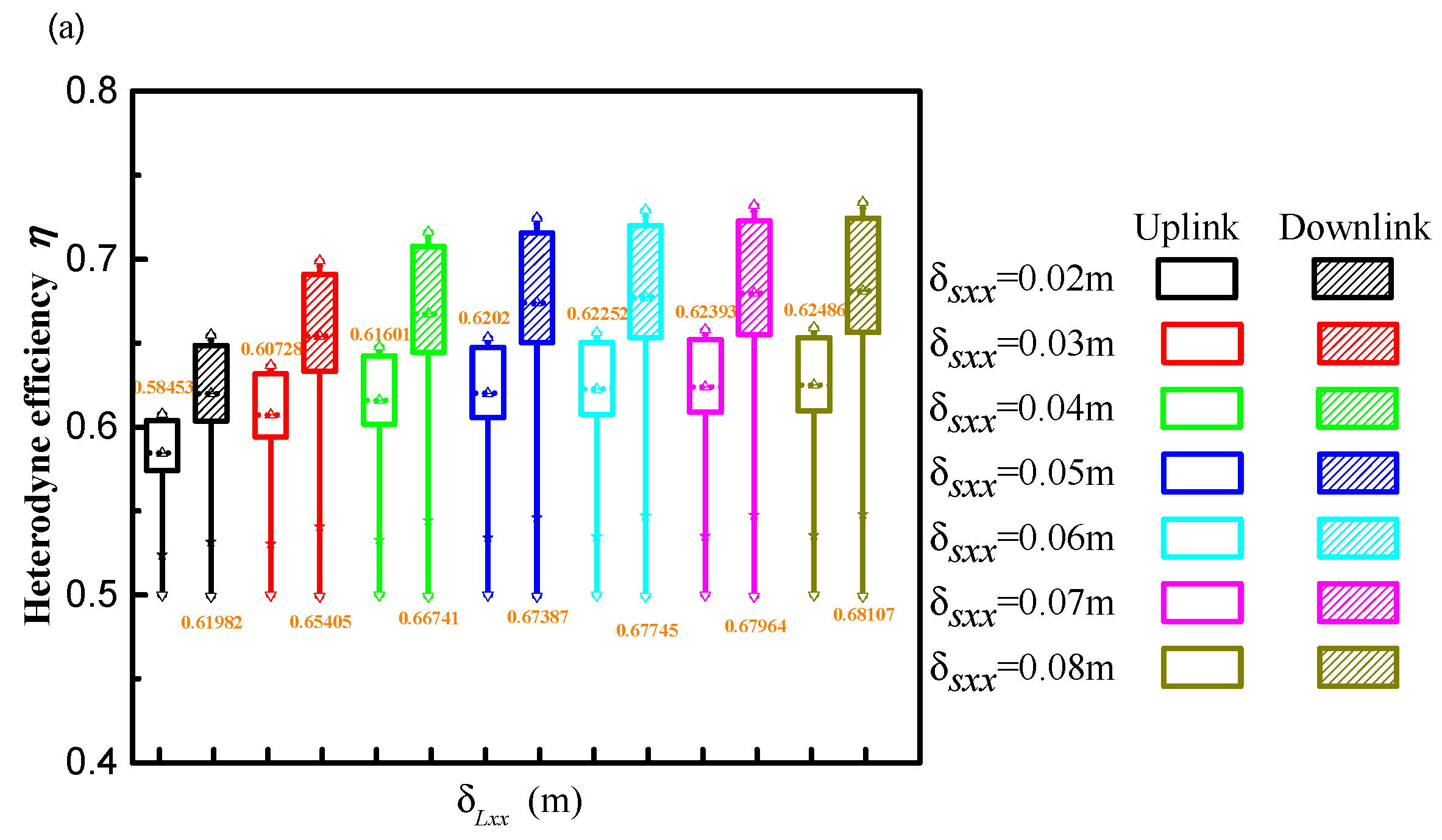
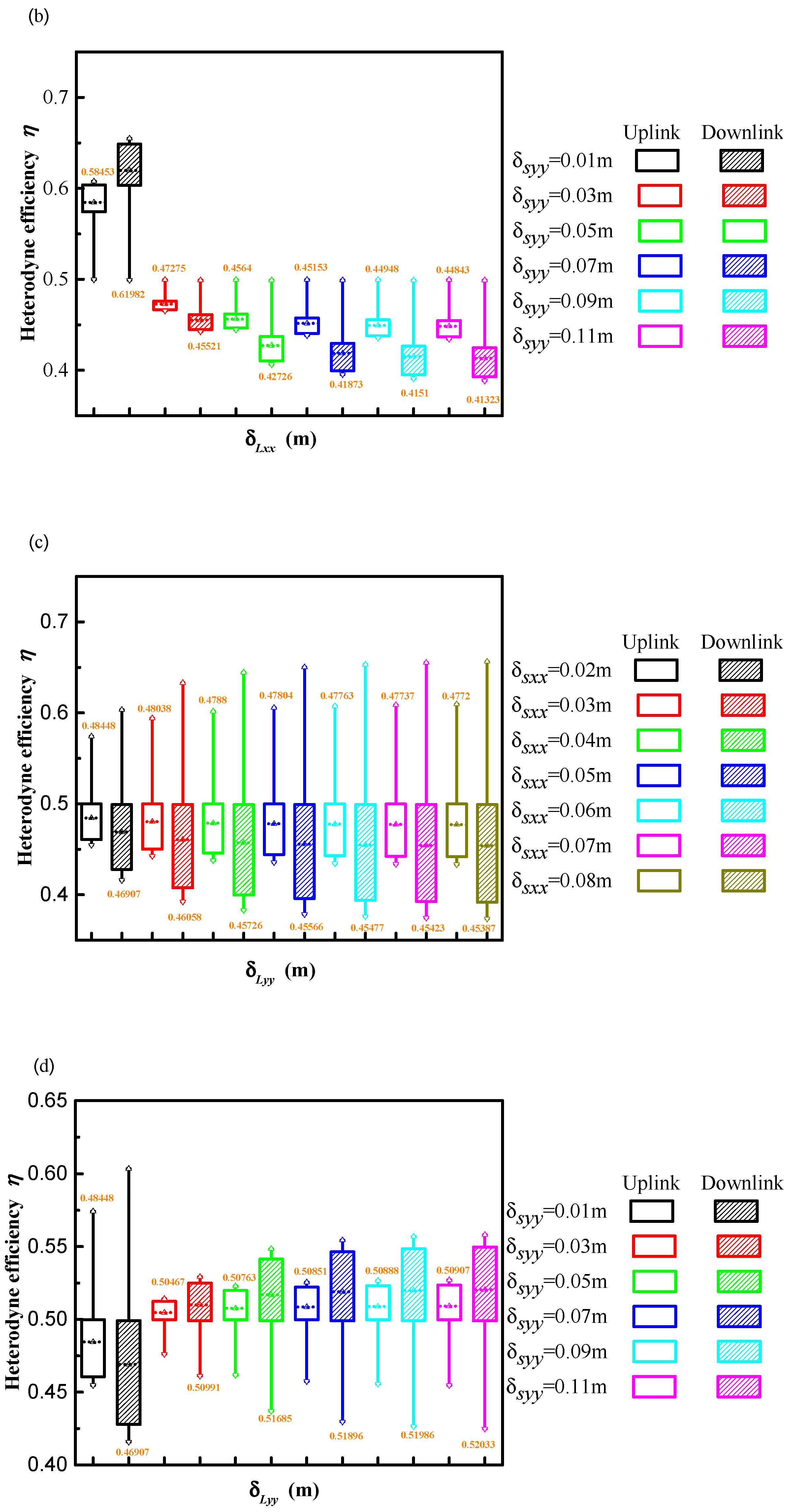
Figure 5a,b show the heterodyne efficiency as a function of δ_Lxx for various δ_Sxx and δ_Syy of the uplink and downlink path, respectively. Figure 5c,d show the heterodyne efficiency as a function of δ_Lyy for various δ_Sxx and δ_Syy of the uplink and downlink path, respectively. In Figure 5a–d, the top and bottom symbols in each box represent the maximum and minimum values, respectively. The mean value is indicated by the number at the top or bottom of each box. With increasing δ_Sxx and δ_Syy, heterodyne efficiency improves and decreases, respectively, as seen in Figure 5a,b. Finally, the heterodyne efficiency eventually stabilizes. The heterodyne efficiency on the downlink path is also higher than that on the uplink path in Figure 5a. As demonstrated in Figure 5b, the heterodyne efficiency is higher for a downlink path than for an uplink path when δ_Syy < 0.03 m, and when δ_Syy ≥ 0.03 m, the heterodyne efficiency for a downlink path is lower than that for an uplink path. Additionally, this change of heterodyne efficiency of the downlink and uplink paths is increased with an increase in δ_Syy. Comparing Figure 5a,c, the heterodyne efficiency decreases and increases with increasing δ_Lyy and δ_Lxx, respectively. The heterodyne efficiency on the downlink path is also lower than that on the uplink path in Figure 5c, which is an opposite trend to the results presented in Figure 5a. The reason has been explained by analyzing the results of Figure 3. Therefore, compared with the effect of δ_Sxx on the heterodyne efficiency, the coherence length of the signal beam in the yy direction component δ_Syy has an opposite trend to the heterodyne efficiency for a downlink path and an uplink path. The comparison of the results of Figure 5b,d can also demonstrate the correctness of this conclusion.

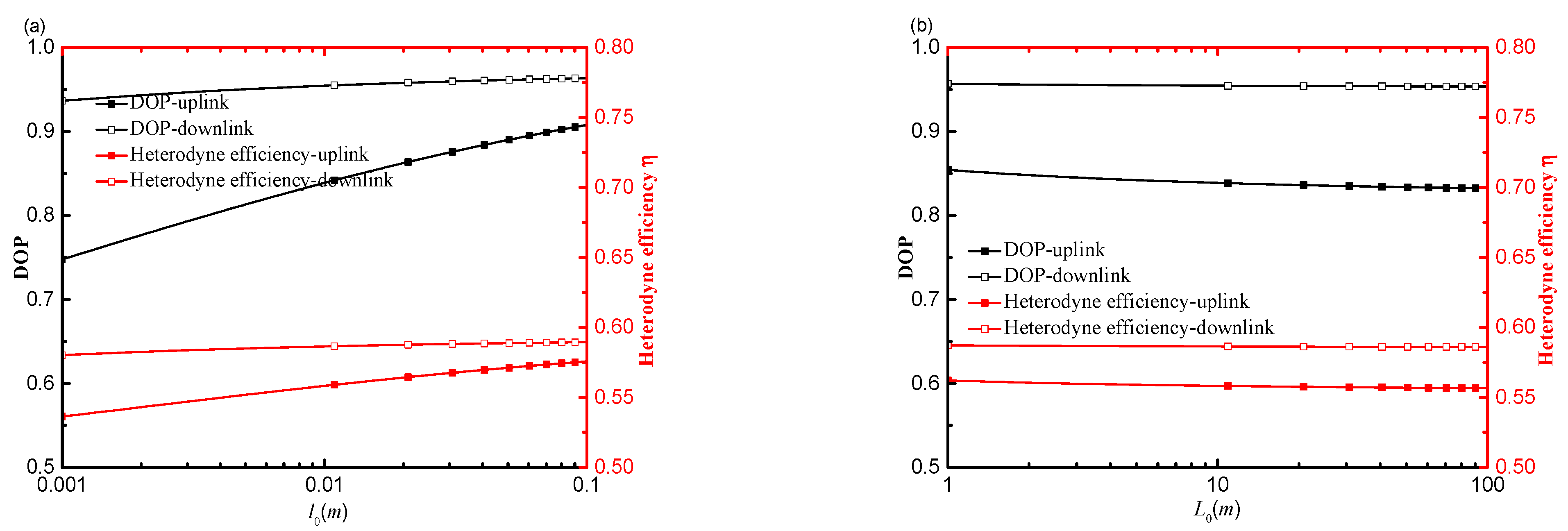
The heterodyne efficiency and DoP are displayed as functions of the inner scale and outer scale, respectively, in Figure 6a,b. The heterodyne efficiency and DoP are enhanced with the increasing inner scale l₀ and decrease with the raising outer scale L₀, as shown in Figure 6a,b. The heterodyne efficiency and DoP on the downlink path are also higher than that on the uplink path. The DoP and heterodyne efficiency are significantly affected by the inner scale carried on by atmospheric turbulence, as seen in Figure 6a,b.

Heterodyne efficiency and DoP are shown in Figure 7a–c as a function of transmission distance z for various refractive index structure parameters. As shown in Figure 7a–c, the heterodyne efficiency and DoP of an uplink path and a downlink path are extremely similar within a transmission distance of 100 m. This is because, when the transmission distance is relatively small, the random fluctuation carried upon by the refractive index may be omitted. The maximum DoP and heterodyne efficiency of a downlink propagation path correspond to a transmission distance that is greater than an uplink propagation path, and it decreases with an increasing atmospheric refractive index structure constant $C_n^2$ 10⁻¹⁶ m^−2/3 to 10⁻¹² m^−2/3. As the transmission distance increases, the DoP and heterodyne efficiency of a downlink path are significantly impacted by strong atmospheric turbulence, as compared to weak and medium-strong atmospheric turbulence intensities. This is due to the fact that when the number of small-scale gyres increases in the area of considerable atmospheric turbulence, the diffraction of the signal beam is strengthened, which is consistent with the findings in Figure 6.

For various transmission distances, Figure 8 illustrates the heterodyne efficiency as a function of detector aperture D. As shown in Figure 8, the heterodyne efficiency of a downlink path and an uplink path decreases with increasing detector size. The heterodyne efficiency of a downlink path is also higher than that of an uplink path. Additionally, the heterodyne efficiency increases with an increasing transmission distance on a downlink path and an uplink path. This is due to the fact that the zenith angle decreases as the transmission distance increases, the corresponding atmospheric turbulence intensities decrease, spatial coherence deteriorates and the impact of atmospheric turbulence on the beam is reduced.

5. Conclusions

In conclusion, the effect of the DoP of the partially coherent Gaussian Schell-model beam on a heterodyne detection system between propagations along an uplink path and a downlink path in atmospheric turbulence has been studied. We found a new result according to which the DoP and heterodyne efficiency of an uplink path are higher than that of a downlink path when σ_sy > 0.02 m or δ_Syy ≥ 0.03 m, which indicates that σ_sy and δ_Syy had a more significant impact on heterodyne efficiency and DoP than the turbulence term on the uplink and downlink paths. We propose a method to obtain a higher heterodyne efficiency based on real propagation paths based on the above description, and demonstrate that this method is valid for any waist radius, higher and lower coherence cases, any atmospheric turbulence intensity, transmission distance and detector aperture.