Design and Analysis of Femtosecond Laser-Generated Metasurface for Optical Filter Application
Abstract
1. Introduction
1.1. Background
1.2. Motivation for the Study
1.3. Contribution of the Study
2. Related Works
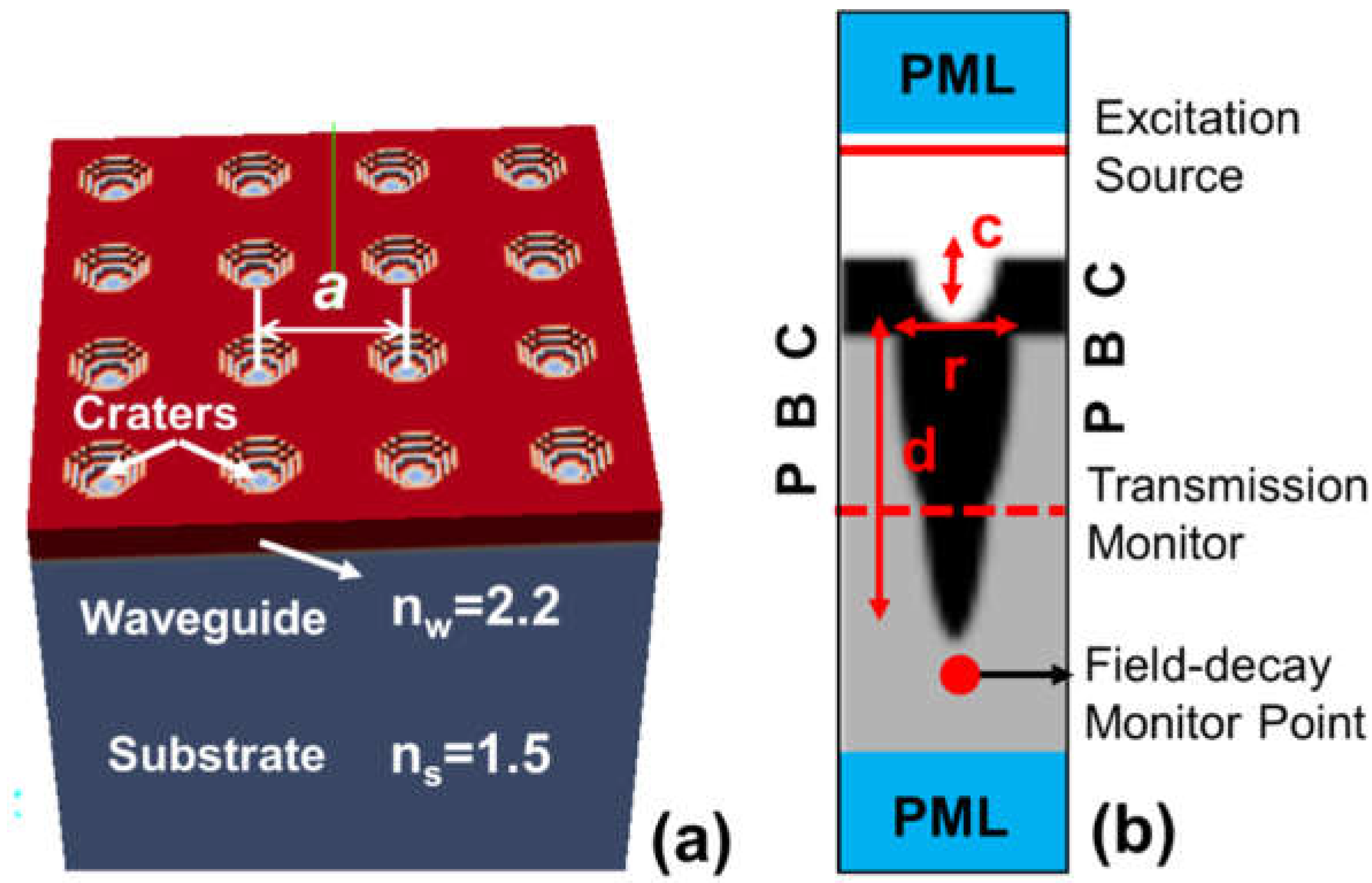
3. Materials and Methods
3.1. Experimental Work
3.2. Numerical Design
4. Results and Discussion
4.1. Surface-Radius of Nano-Holes
4.2. Depth of Nano-Holes
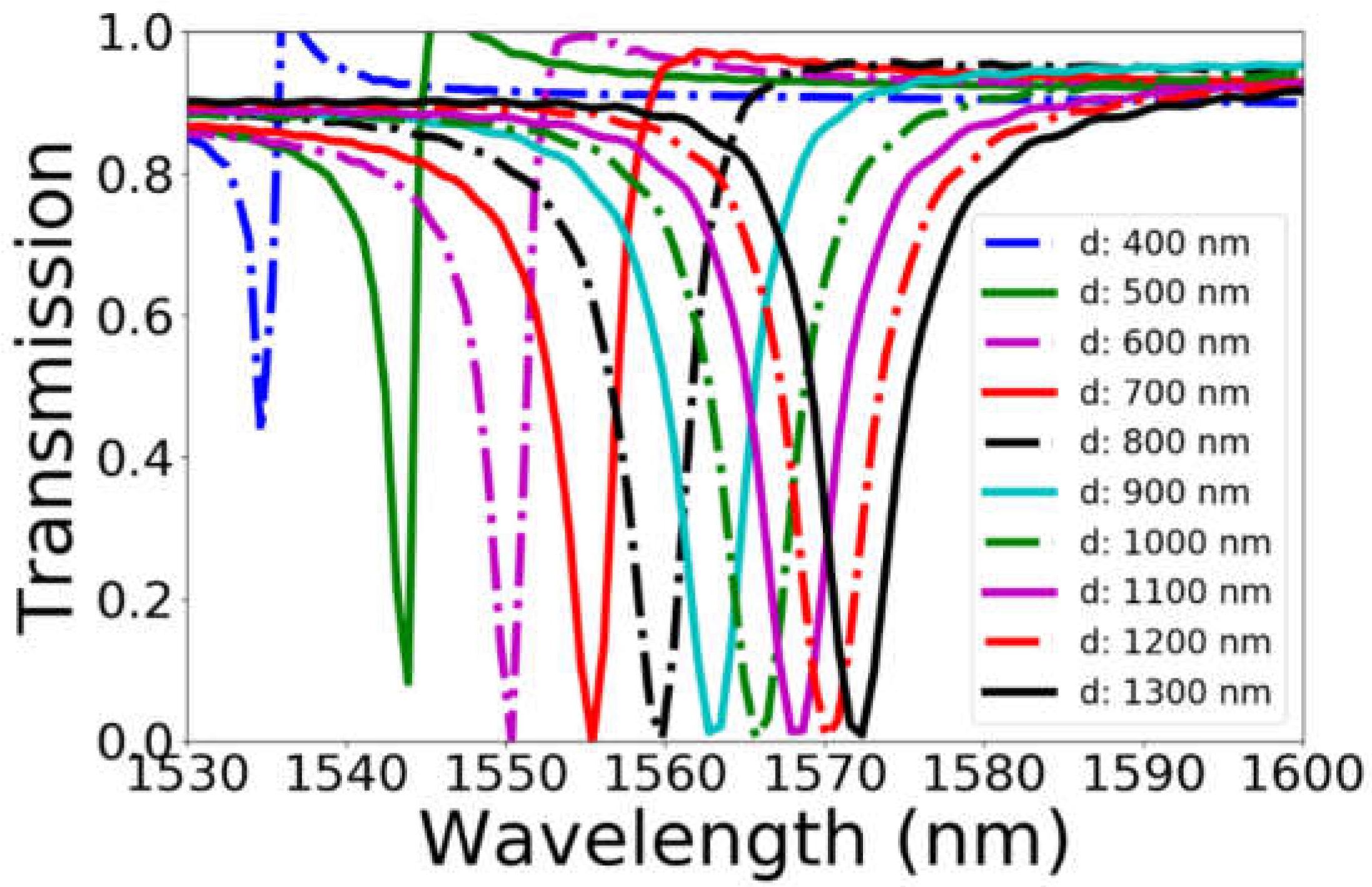
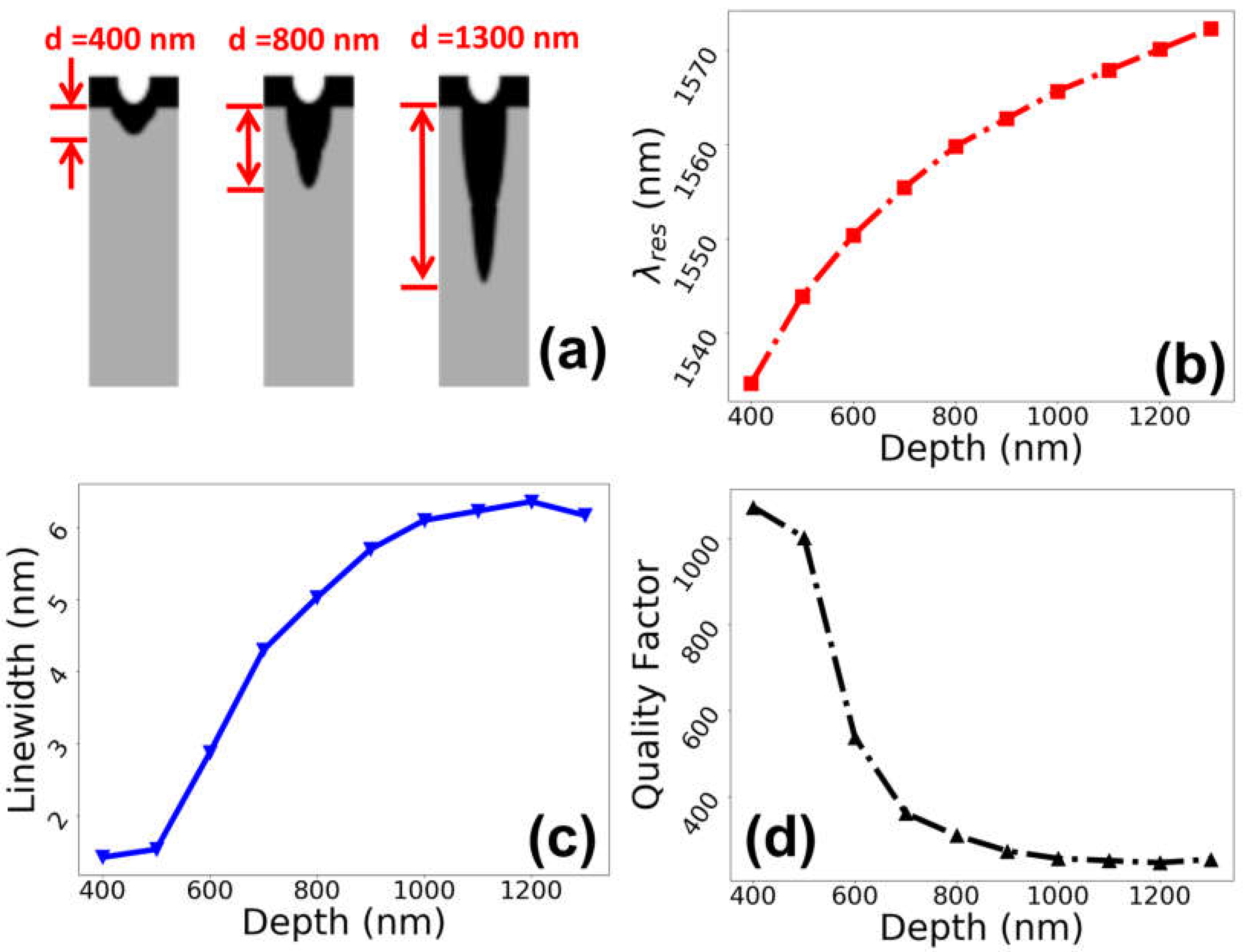
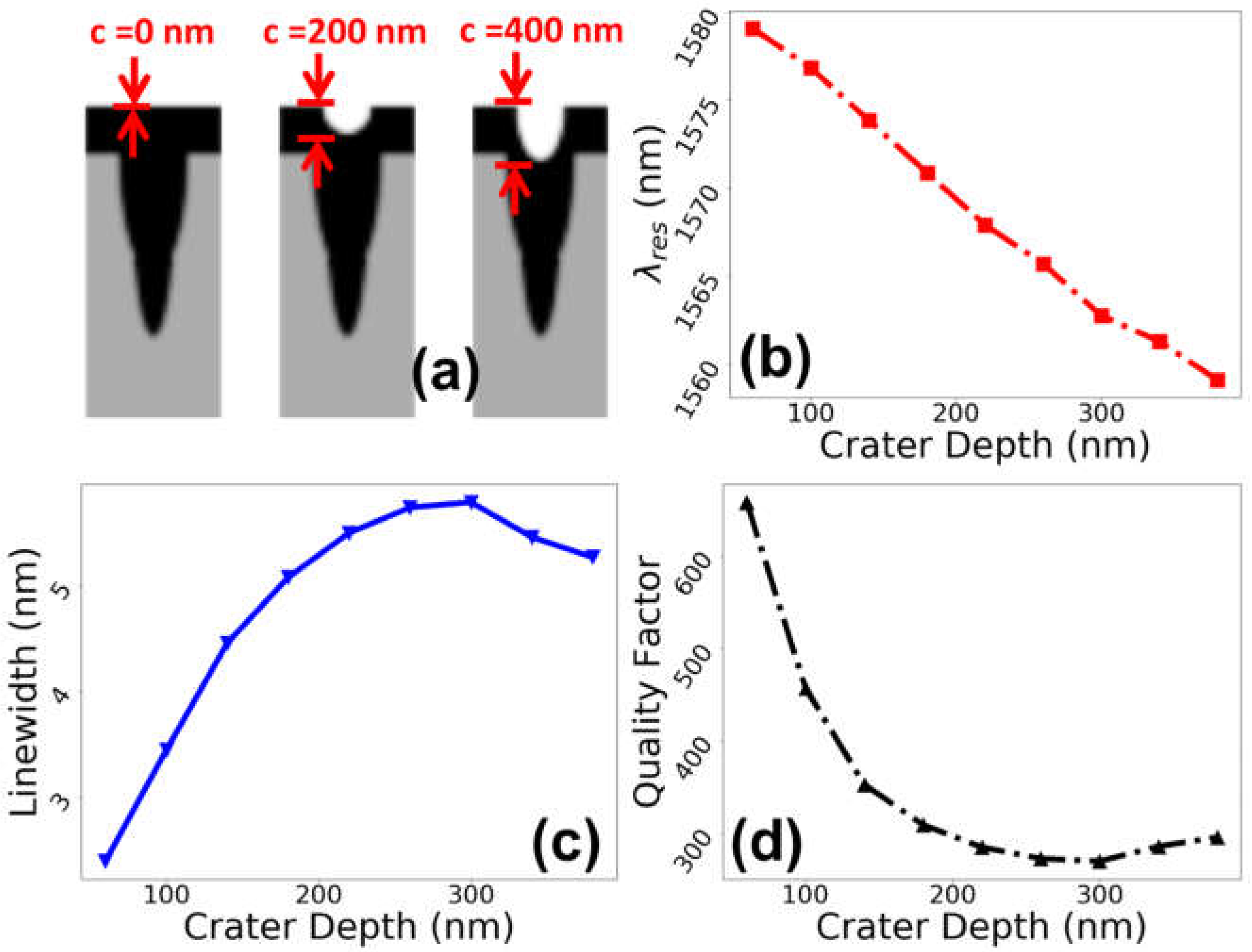
4.3. Depth of the Crater
5. Comparative Analysis
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Götte, N.; Kusserow, T.; Winkler, T.; Sarpe, C.; Englert, L.; Otto, D.; Meinl, T.; Khan, Y.; Zielinski, B.; Senftleben, A.; et al. 3. Temporally shaped femtosecond laser pulses for creation of functional sub-100 nm structures in dielectrics. Opt. Induc. Nanostruct. 2015, 23, 47–72. [Google Scholar]
- Khan, Y.; Götte, N.; Meinl, T.; Kusserow, T.; Senftleben, A.; Baumert, T.; Hillmer, H. Modelling, design and fabrication of dielectric photonic crystal structures using temporally asymmetric shaped femtosecond laser pulses. In Proceedings of the 2014 International Conference on Optical MEMS and Nanophotonics, Glasgow, UK, 17–21 August 2014; pp. 207–208. [Google Scholar]
- Paul, A.K.; Habib, S.; Hai, N.H.; Razzak, S.A. An air-core photonic crystal fiber based plasmonic sensor for high refractive index sensing. Opt. Commun. 2020, 464, 125556. [Google Scholar] [CrossRef]
- Rehman, A.U.; Khan, Y.; Irfan, M.; Butt, M.A.; Khonina, S.N.; Kazanskiy, N.L. A Novel Design of Optical Switch Based on Guided Mode Resonances in Dielectric Photonic Crystal Structures. Photonics 2022, 9, 580. [Google Scholar] [CrossRef]
- Yu, H.; Yu, J.; Sun, F.; Li, Z.; Chen, S. Systematic considerations for the patterning of photonic crystal devices by electron beam lithography. Opt. Commun. 2007, 271, 241–247. [Google Scholar] [CrossRef]
- Khan, Y.; Rehman, A.U.; Batool, B.A.; Noor, M.; Butt, M.A.; Kazanskiy, N.L.; Khonina, S.N. Fabrication and Investigation of Spectral Properties of a Dielectric Slab Waveguide Photonic Crystal Based Fano-Filter. Crystals 2022, 12, 226. [Google Scholar] [CrossRef]
- Steve, R.; Robert, P. A Review of Focused Ion Beam Applications in Microsystem Technology. J. Micromech. Microeng. 2001, 11, 287–300. [Google Scholar]
- Hadzialic, S.; Kim, S.; Sudbo, A.S.; Solgaard, O. Two-Dimensional Photonic Crystals Fabricated in Monolithic Single-Crystal Silicon. IEEE Photonics Technol. Lett. 2009, 22, 67–69. [Google Scholar] [CrossRef]
- Huang, H.W.; Lin, C.H.; Huang, J.K.; Lee, K.Y.; Lin, C.F.; Yu, C.C.; Tsai, J.Y.; Hsueh, R.; Kuo, H.C.; Wang, S.C. Investigation of GaN-based Light Emitting Diodes with Nano-Hole Patterned Sapphire Substrate (NHPSS) by Nano-Imprint Lithography. Mater. Sci. Eng. B 2009, 164, 76–79. [Google Scholar] [CrossRef]
- Chen, A.; Chua, S.-J.; Fonstad, C.G., Jr.; Wang, B.; Wilhelmi, O. Two-Dimensional Photonic Crystals Fabricated by Nanoimprint Lithography; Advanced Materials for Micro- and Nano-Systems; Massachusetts Institute of Technology: Cambridge, MA, USA, 2015. [Google Scholar]
- Amoako, G. Femtosecond laser structuring of materials: A review. Appl. Phys. Res. 2019, 11, 1–11. [Google Scholar] [CrossRef]
- Simon, P.; Ihlemann, J.; Bonse, J. Special Issue “Laser-Generated Periodic Nanostructures”. Nanomaterials 2021, 11, 2054. [Google Scholar] [CrossRef]
- Abou Khalil, A.; Lalanne, P.; Bérubé, J.P.; Petit, Y.; Vallée, R.; Canioni, L. Femtosecond laser writing of near-surface waveguides for refractive-index sensing. Opt. Express 2019, 27, 31130–31143. [Google Scholar] [CrossRef] [PubMed]
- Li, B.; Jiang, L.; Wang, S.; Tsai, H.L.; Xiao, H. Femtosecond laser fabrication of long period fiber gratings and applications in refractive index sensing. Opt. Laser Technol. 2011, 43, 1420–1423. [Google Scholar] [CrossRef]
- Wang, Y.; Wang, D.N.; Yang, M.; Hong, W.; Lu, P. Refractive index sensor based on a microhole in single-mode fiber created by the use of femtosecond laser micromachining. Opt. Lett. 2009, 34, 3328–3330. [Google Scholar] [CrossRef] [PubMed]
- Chung, S.H.; Mazur, E. Surgical applications of femtosecond lasers. J. Biophotonics 2009, 2, 557–572. [Google Scholar] [CrossRef]
- Kelemen, L.; Lepera, E.; Horváth, B.; Ormos, P.; Osellame, R.; Vázquez, R.M. Direct writing of optical microresonators in a lab-on-a-chip for label-free biosensing. Lab Chip 2019, 19, 1985–1990. [Google Scholar] [CrossRef]
- Kuchmizhak, A.; Pustovalov, E.; Syubaev, S.; Vitrik, O.; Kulchin, Y.; Porfirev, A.; Khonina, S.; Kudryashov, S.; Danilov, P.; Ionin, A. On-fly femtosecond-laser fabrication of self-organized plasmonic nanotextures for chemo-and biosensing applications. ACS Appl. Mater. Interfaces 2016, 8, 24946–24955. [Google Scholar] [CrossRef]
- Ródenas, A.; Gu, M.; Corrielli, G.; Paiè, P.; John, S.; Kar, A.K.; Osellame, R. Three-dimensional femtosecond laser nanolithography of crystals. Nat. Photonics 2019, 13, 105–109. [Google Scholar] [CrossRef]
- Ha, J.; Lee, B.J.; Hwang, D.J.; Kim, D. Femtosecond laser nanowelding of silver nanowires for transparent conductive electrodes. RSC Adv. 2016, 6, 86232–86239. [Google Scholar] [CrossRef]
- Balling, P.; Schou, J. Femtosecond-laser ablation dynamics of dielectrics: Basics and applications for thin films. Rep. Prog. Phys. 2013, 76, 036502. [Google Scholar] [CrossRef]
- Englert, L.; Wollenhaupt, M.; Sarpe, C.; Otto, D.; Baumert, T. Morphology of nanoscale structures on fused silica surfaces from interaction with temporally tailored femtosecond pulses. J. Laser Appl. 2012, 24, 042002. [Google Scholar] [CrossRef]
- Köhler, J.; Wollenhaupt, M.; Bayer, T.; Sarpe, C.; Baumert, T. Zeptosecond precision pulse shaping. Opt. Express 2011, 19, 11638–11653. [Google Scholar] [CrossRef] [PubMed]
- Englert, L.; Rethfeld, B.; Haag, L.; Wollenhaupt, M.; Sarpe-Tudoran, C.; Baumert, T. Control of ionization processes in high band gap materials via tailored femtosecond pulses. Opt. Express 2007, 15, 17855–17862. [Google Scholar] [CrossRef] [PubMed]
- Wollenhaupt, M.; Englert, L.; Horn, A.; Baumert, T. Control of ionization processes in high band gap materials. J. Laser Micro/Nanoeng. 2009, 4, 144–151. [Google Scholar] [CrossRef]
- Meinl, T.; Götte, N.; Khan, Y.; Kusserow, T.; Sarpe, C.; Köhler, J.; Wollenhaupt, M.; Senftleben, A.; Baumert, T.; Hillmer, H. Material processing of dielectrics via temporally shaped femtosecond laser pulses as direct patterning method for nanophotonic applications. In Nanoscience Advances in CBRN Agents Detection, Information and Energy Security; Springer: Dordrecht, The Netherlands, 2015; pp. 29–34. [Google Scholar]
- Khan, Y. Design and Numerical Simulation of Dielectric Photonic Crystal Devices and Investigation of an Optical Characterization Method. Ph.D. Thesis, University of Kassel, Kassel, Germany, 2017. [Google Scholar]
- Limonov, M.F.; Rybin, M.; Poddubny, A.; Kivshar, Y.S. Fano resonances in photonics. Nat. Photonics 2017, 11, 543–554. [Google Scholar] [CrossRef]
- Rybin, M.V.; Khanikaev, A.B.; Inoue, M.; Samusev, K.B.; Steel, M.J.; Yushin, G.; Limonov, M.F. Fano resonance between Mie and Bragg scattering in photonic crystals. Phys. Rev. Lett. 2009, 103, 023901. [Google Scholar] [CrossRef]
- Ciminelli, C.; Dell’Olio, F.; Brunetti, G.; Conteduca, D.; Armenise, M.N. New microwave photonic filter based on a ring resonator including a photonic crystal structure. In Proceedings of the 2017 19th International Conference on Transparent Optical Networks (ICTON), Girona, Spain, 2–6 July 2017; pp. 1–4. [Google Scholar]
- Dong, P.; Feng, N.N.; Feng, D.; Qian, W.; Liang, H.; Lee, D.C.; Luff, B.J.; Banwell, T.; Agarwal, A.; Toliver, P.; et al. GHz-bandwidth optical filters based on high-order silicon ring resonators. Opt. Express 2010, 18, 23784–23789. [Google Scholar] [CrossRef]
- Brunetti, G.; Sasanelli, N.; Armenise, M.N.; Ciminelli, C. High performance and tunable optical pump-rejection filter for quantum photonic systems. Opt. Laser Technol. 2021, 139, 106978. [Google Scholar] [CrossRef]
- Oser, D.; Mazeas, F.; Ramos, C.A.; Le Roux, X.; Vivien, L.; Tanzilli, S.; Cassan, É.; Labonté, L. On-chip photon pair source with pump rejection filter. In Proceedings of the European Quantum Electronics Conference, Munich, Germany, 23–27 June 2019; Optical Society of America: New York, NY, USA, 2019; p. eb_2_4. [Google Scholar]
- Spencer, D.T.; Bauters, J.F.; Heck, M.J.; Bowers, J.E. Integrated waveguide coupled Si3N4 resonators in the ultrahigh-Q regime. Optica 2014, 1, 153–157. [Google Scholar] [CrossRef]
- Conteduca, D.; Brunetti, G.; Pitruzzello, G.; Tragni, F.; Dholakia, K.; Krauss, T.F.; Ciminelli, C. Exploring the limit of multiplexed near-field optical trapping. ACS Photonics 2021, 8, 2060–2066. [Google Scholar] [CrossRef]
- Liu, K.; Jin, N.; Cheng, H.; Chauhan, N.; Puckett, M.W.; Nelson, K.D.; Behunin, R.O.; Rakich, P.T.; Blumenthal, D.J. Ultralow 0.034 dB/m loss wafer-scale integrated photonics realizing 720 million Q and 380 μW threshold Brillouin lasing. Opt. Lett. 2022, 47, 1855–1858. [Google Scholar] [CrossRef]
- Lambert, E.; Fiers, M.; Nizamov, S.; Tassaert, M.; Johnson, S.G.; Bienstman, P.; Bogaerts, W. Python bindings for the open source electromagnetic simulator Meep. Comput. Sci. Eng. 2010, 13, 53–65. [Google Scholar] [CrossRef]
- Oskooi, A.F.; Roundy, D.; Ibanescu, M.; Bermel, P.; Joannopoulos, J.D.; Johnson, S.G. Meep: A Flexible Free-Software Package for Electromagnetic Simulations by the FDTD Method. Comput. Phys. Commun. 2010, 181, 687–702. [Google Scholar] [CrossRef]
- Yablonovitch, E. Inhibited Spontaneous Emission in Solid-State Physics and Electronics. Phys. Rev. Lett. 1987, 58, 2059. [Google Scholar] [CrossRef] [PubMed]
- Fan, S.; Joannopoulos, J.D. Analysis of Guided Resonances in Photonic Crystal Slabs. Phys. Rev. B 2002, 65, 235112. [Google Scholar] [CrossRef]
- Li, M.; Mori, K.; Ishizuka, M.; Liu, X.; Sugimoto, Y.; Ikeda, N.; Asakawa, K. Photonic bandpass filter for 1550 nm fabricated by femtosecond direct laser ablation. Appl. Phys. Lett. 2003, 83, 216–218. [Google Scholar] [CrossRef]
- Ye, S.; Cao, Q.; Wang, Q.; Wang, T.; Peng, Q. A highly efficient, stable, durable, and recyclable filter fabricated by femtosecond laser drilling of a titanium foil for oil-water separation. Sci. Rep. 2016, 6, 37591. [Google Scholar] [CrossRef]
- Krüger, J.; Lenzner, M.; Martin, S.; Lenner, M.; Spielmann, C.; Fiedler, A.; Kautek, W. Single-and multi-pulse femtosecond laser ablation of optical filter materials. Appl. Surf. Sci. 2003, 208, 233–237. [Google Scholar] [CrossRef]
- Purlys, V.; Maigyte, L.; Gailevičius, D.; Peckus, M.; Malinauskas, M.; Staliunas, K. Spatial filtering by chirped photonic crystals. Phys. Rev. A 2013, 87, 033805. [Google Scholar] [CrossRef]
- Qiao, H.; Yang, J.; Wang, F.; Yang, Y.; Sun, J. Femtosecond laser direct writing of large-area two-dimensional metallic photonic crystal structures on tungsten surfaces. Opt. Express 2015, 23, 26617–26627. [Google Scholar] [CrossRef]

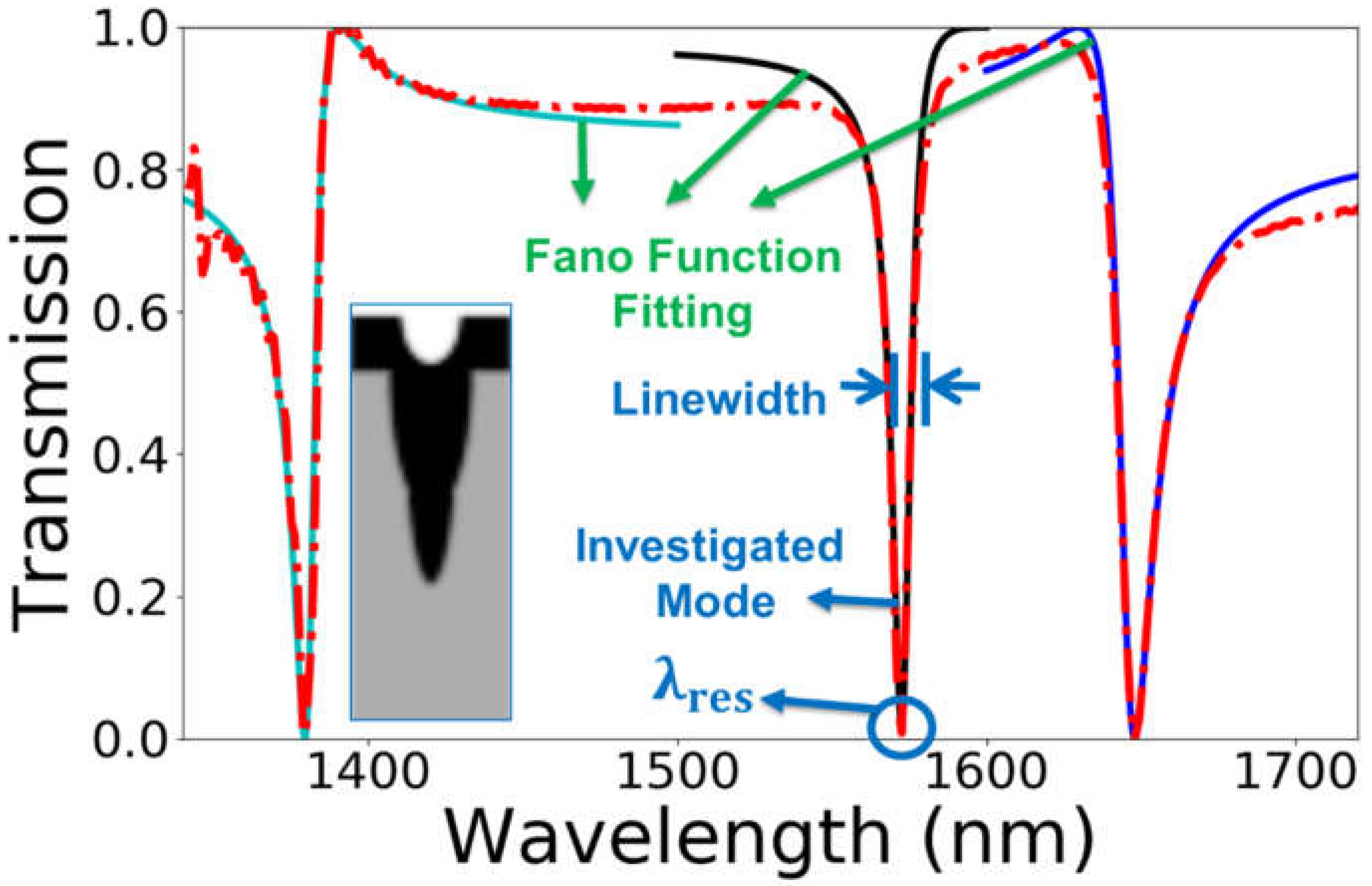



| Central Wavelength (nm) | Fano Factor (q) | Linewidth (nm) |
|---|---|---|
| 1381.398 | 0.44 | 8.191 |
| 1573.103 | 0.15 | 7.391 |
| 1645.347 | −0.41 | 12.791 |
| FS Laser Parameters | Target Material | Type of Generated Structures | Spectral Properties of the Structure | References |
|---|---|---|---|---|
| : 775 nm Width: 150 fs | Si-on-SiO2 | Nano-holes based waveguide | : 1575 nm Linewidth: 22 nm QF: 70 | [41] |
| : 800 nm Width: 50 fs | TiO2 | Periodic nano-holes | Oil–water separation with 99% efficiency | [42] |
| :800 nm Width: 30 fs | BG18 glassBG36 glass | Periodic nano-holes | : 775 nm and 835 nm Linewidth: 50 nm | [43] |
| : 1030 nm Width: 300 fs | Bulk glass | Chirped periodic nano-grating | 50% filtering efficiency for reflection peaks at 0° degree incidence | [44] |
| Two cross-polarized FS laser pulses : 800 nm Width: 50 fs | Tungsten | Periodic nano-holes | Optical filter to reduce optical reflectivity of the targeted surface below wavelength range of 2000 nm | [45] |
| Ti: Sapphire FS laser system : 790 nm Width: 35 fs | Fused silica | Periodic nano-holes | Optical filter in communication wavelength range with : 1550 nm Linewidth: 1.4 to 24.2 nm QF: 66.51 to 1090.12 | This work |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Khan, Y.; Noor, D.; Ullah, N.; Khonina, S.N.; Kazanskiy, N.L.; Butt, M.A. Design and Analysis of Femtosecond Laser-Generated Metasurface for Optical Filter Application. Photonics 2022, 9, 797. https://doi.org/10.3390/photonics9110797
Khan Y, Noor D, Ullah N, Khonina SN, Kazanskiy NL, Butt MA. Design and Analysis of Femtosecond Laser-Generated Metasurface for Optical Filter Application. Photonics. 2022; 9(11):797. https://doi.org/10.3390/photonics9110797
Chicago/Turabian StyleKhan, Yousuf, Dua Noor, Naqeeb Ullah, Svetlana N. Khonina, Nikolay L. Kazanskiy, and Muhammad A. Butt. 2022. "Design and Analysis of Femtosecond Laser-Generated Metasurface for Optical Filter Application" Photonics 9, no. 11: 797. https://doi.org/10.3390/photonics9110797
APA StyleKhan, Y., Noor, D., Ullah, N., Khonina, S. N., Kazanskiy, N. L., & Butt, M. A. (2022). Design and Analysis of Femtosecond Laser-Generated Metasurface for Optical Filter Application. Photonics, 9(11), 797. https://doi.org/10.3390/photonics9110797








